Influence of Boundary Impedance of 3D Cavity on Targeted Energy Transfer between a Damped Acoustic Mode and a Nonlinear Membrane Absorber
Abstract
:1. Introduction
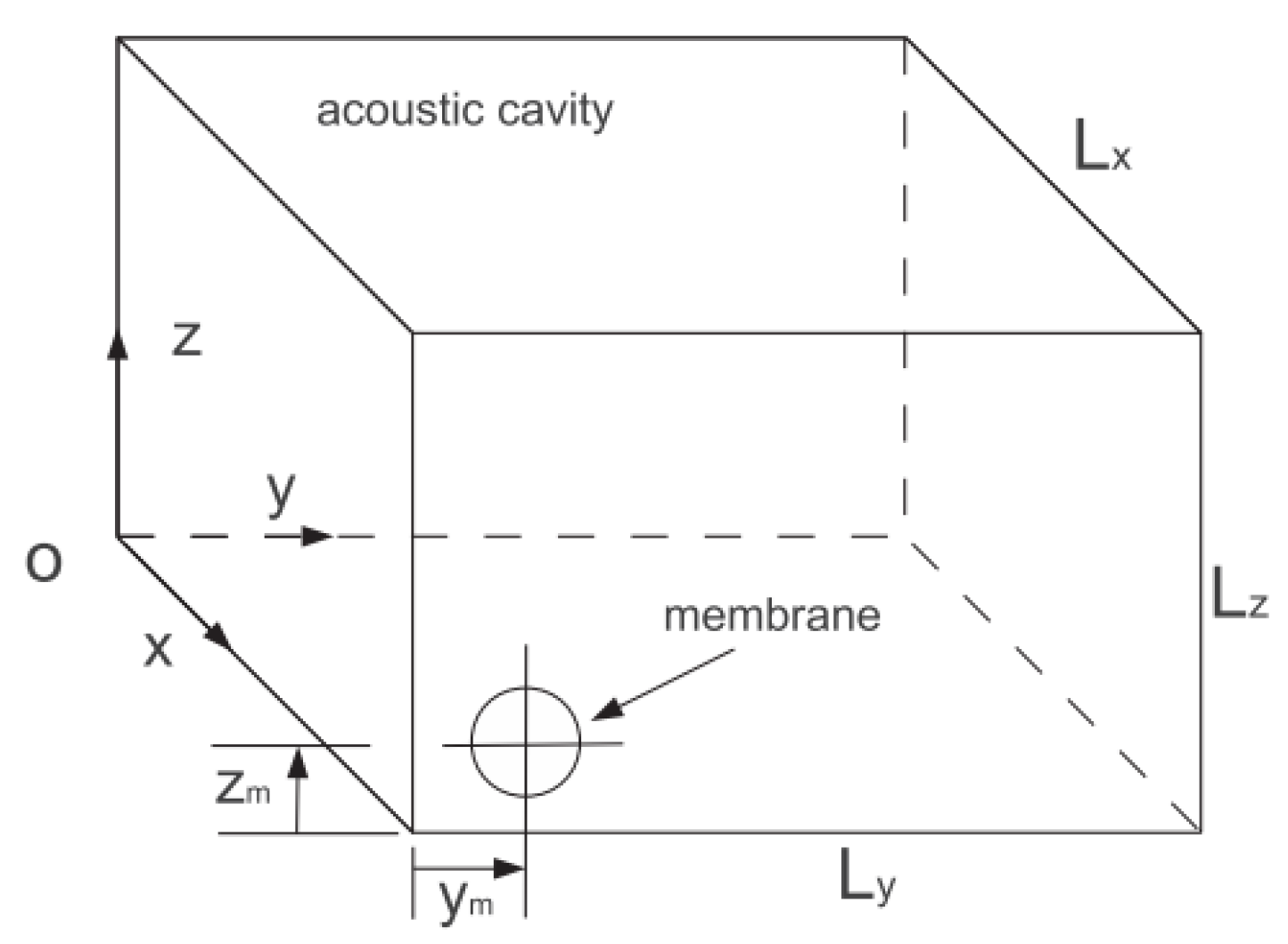
2. Description of the System
3. Acoustic Damping Coefficient
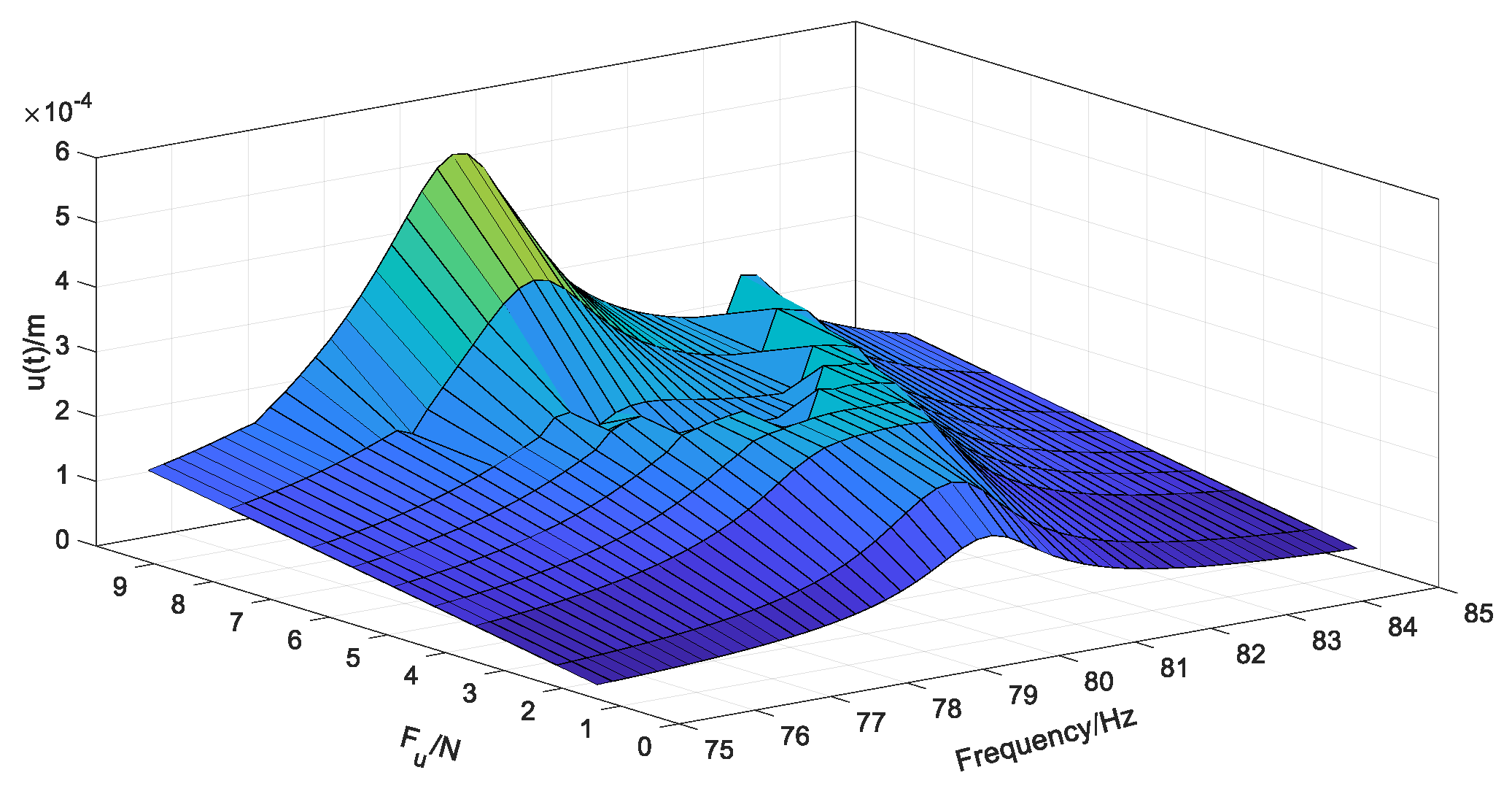
4. Experimental and Numerical Forced Responses of the System
- (1)
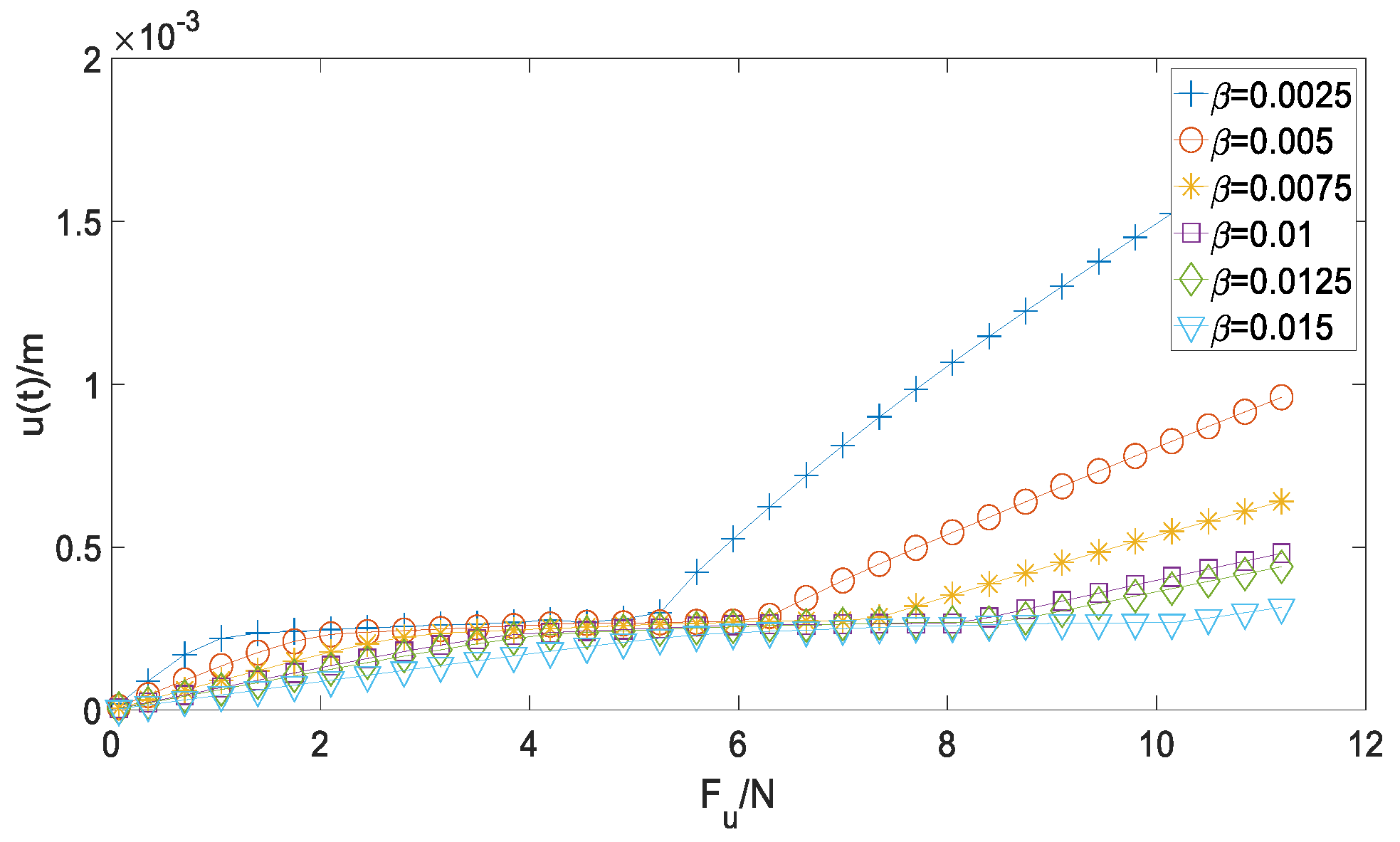
- In the low excitation amplitude range, such as , the acoustic displacement amplitude has a single resonance peak at the resonance frequency, and the response amplitude of increases with the increase in the excitation amplitude.
- (2)
- In the medium excitation amplitude range, such as , the forced response produces a plateau with an amplitude of about m within a certain frequency range, and the resonance peak is suppressed and disappears, where the regime of responses of the system is SMR. This range starts with the appearance of the plateau and ends with the appearance of another new resonance peak. As the forcing excitation amplitude increases, the energy triggers the working threshold of the membrane NES in a wider frequency band near the resonance frequency, and the frequency range of the plateau gradually increases.
- (3)
- In the high excitation amplitude range, such as , the forced response has a new resonance peak below the original resonance frequency, which is higher than the resonance peak under the low forcing excitation. In addition, the plateau of the forced response appearing near the resonance frequency under the forcing excitation with medium amplitude begins to disappear. As the forcing excitation amplitude increases, the second resonance peak increases further, and the plateau of the forced response near the resonance frequency basically disappears.
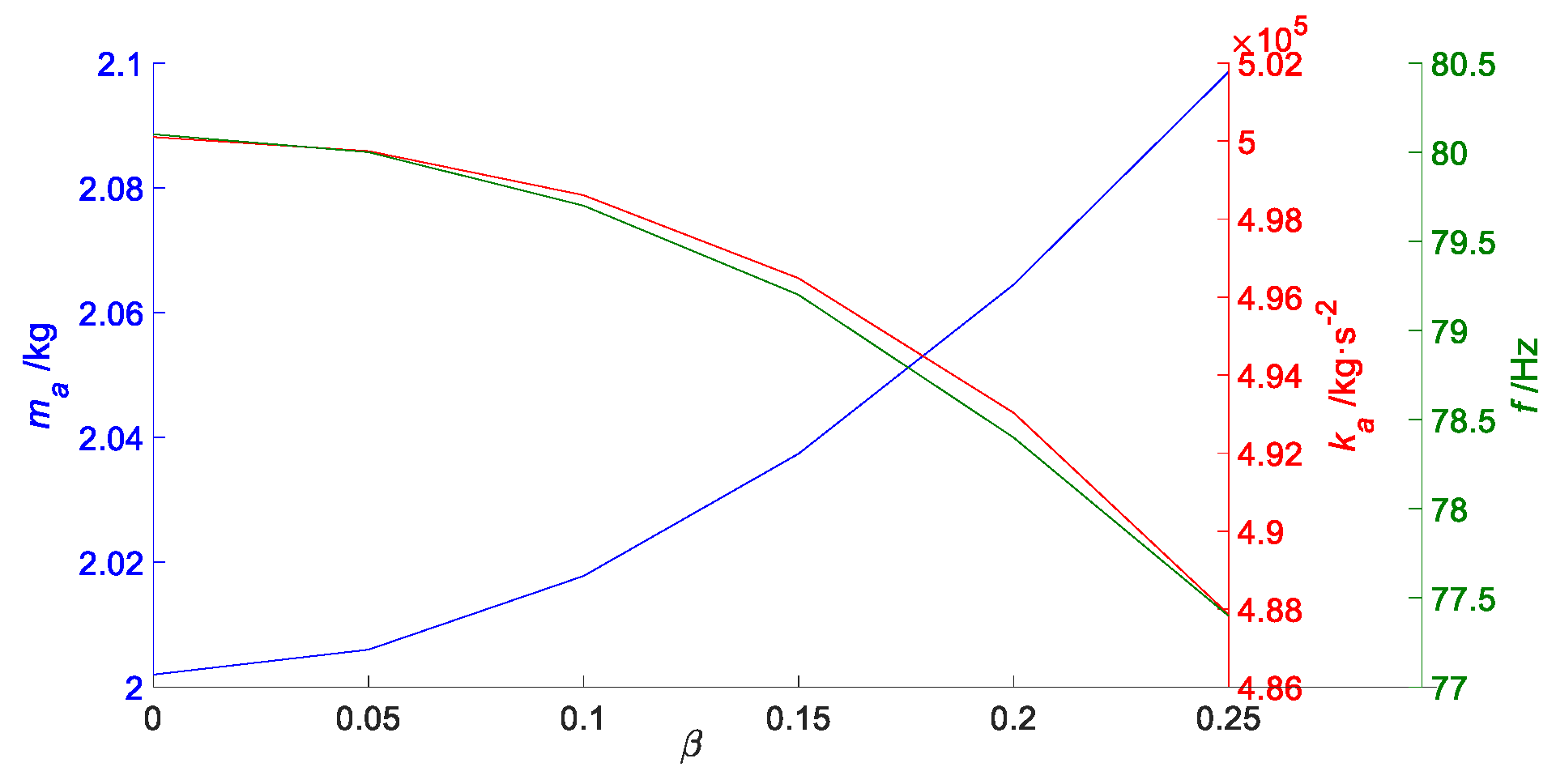
5. Influence of the Wall Impedance for the TET
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Symbol | Unit | Description |
| Corresponding normal mode in direction | ||
| Nm/s | Acoustic damping | |
| m/s | Sound wave velocity | |
| MPa | Modulus of the membrane | |
| N | External force amplitude | |
| Hz | First resonance frequency without pre-stress of the membrane | |
| Hz | First resonance frequency with pre-stress of the membrane | |
| m | Thickness of the membrane | |
| N/m | Modal stiffness of the system | |
| Wave number of the mode | ||
| N/m | Linear stiffness of the membrane | |
| Nonlinear stiffness of the membrane | ||
| m | Length of the cavity | |
| m | Width of the cavity | |
| m | Height of the cavity | |
| Normal modes | ||
| kg | Modal mass of the system | |
| kg | Mass of the membrane | |
| Boundary normal vector | ||
| Pa | Acoustic pressure | |
| Pa | Acoustic pressure of a damped mode | |
| Quality factor | ||
| m | Transversal displacement amplitude of the membrane center | |
| m | Position vector | |
| m | Radius of the membrane | |
| Surface of the cavity | ||
| Surface of the membrane | ||
| Surface of the cavity without the surface of the membrane | ||
| s | Time | |
| m | Acoustic displacement amplitude | |
| m | Position of the membrane center | |
| Acoustic impedance | ||
| Sound absorption coefficient | ||
| Sound absorption coefficient of the cavity | ||
| Sound absorption coefficient of each wall of the cavity | ||
| Specific impedance ratio | ||
| Specific impedance ratio of each wall of the cavity | ||
| Test function | ||
| Coefficients | ||
| Damping of the membrane | ||
| Poisson ratio of the membrane | ||
| Density of the membrane | ||
| Air density | ||
| Damped mode shape of the cavity | ||
| Internal volume of the cavity | ||
| rad/s | Normal angular frequency |
Appendix B
References
- Gendelman, O.; Manevitch, L.I.; Vakakis, A.F.; M’Closkey, R. Energy pumping in nonlinear mechanical oscillators: Part I—Dynamics of the underlying hamiltonian systems. J. Appl. Mech. 2001, 68, 34–41. [Google Scholar] [CrossRef]
- Vakakis, A.F.; Gendelman, O. Energy pumping in nonlinear mechanical oscillators: Part II—Resonance capture. J. Appl. Mech. 2001, 68, 42–48. [Google Scholar] [CrossRef]
- Shao, J.; Yang, J.; Wu, X.; Zeng, T. Nonlinear energy sink applied for low-frequency noise control inside acoustic cavities: A review. J. Low Freq. Noise Vib. Act. Control 2020, 40, 1453–1472. [Google Scholar] [CrossRef]
- Lee, Y.S.; Kerschen, G.; Vakakis, A.F.; Panagopoulos, P.; Bergman, L.; McFarland, D.M. Complicated dynamics of a linear oscillator with a light, essentially nonlinear attachment. Physica D 2005, 204, 41–69. [Google Scholar] [CrossRef]
- Gendelman, O.V.; Starosvetsky, Y.; Feldman, M. Attractors of harmonically forced linear oscillator with attached nonlinear energy sink (Part I & II). Nonlinear Dyn. 2008, 51, 31–46. [Google Scholar]
- Starosvetsky, Y.; Gendelman, O.V. Vibration absorption in systems with a nonlinear energy sink: Nonlinear damping. J. Sound Vib. 2009, 324, 916–939. [Google Scholar] [CrossRef]
- Vaurigaud, B.; Savadkoohi, A.T.; Lamarque, C.H. Targeted energy transfer with parallel nonlinear energy sinks. Part I: Design theory and numerical results. Nonlinear Dyn. 2011, 66, 763–780. [Google Scholar] [CrossRef]
- Mariani, R.; Bellizzi, S.; Cochelin, B.; Herzog, P.; Mattei, P.O. Toward an adjustable nonlinear low frequency acoustic absorber. J. Sound Vib. 2011, 330, 5245–5258. [Google Scholar] [CrossRef]
- Bitar, D.; Gourdon, E.; Lamarque, C.H.; Collet, M. Shunt loudspeaker using nonlinear energy sink. J. Sound Vib. 2019, 456, 254–271. [Google Scholar] [CrossRef]
- Shao, J.; Cochelin, B. Theoretical and numerical study of targeted energy transfer inside an acoustic cavity by a non-linear membrane absorber. Int. J. Non-Linear Mech. 2014, 64, 85–92. [Google Scholar] [CrossRef]
- Starosvetsky, Y.; Gendelman, O. Dynamics of a strongly nonlinear vibration absorber coupled to a harmonically excited two-degree-of-freedom system. J. Sound Vib. 2008, 312, 234–256. [Google Scholar] [CrossRef]
- Bellet, R.; Cochelin, B.; Herzog, P.; Mattei, P.O. Experimental study of targeted energy transfer from an acoustic system to a nonlinear membrane absorber. J. Sound Vib. 2010, 329, 2768–2791. [Google Scholar] [CrossRef]
- Vakakis, A.F. Passive nonlinear targeted energy transfer. Philos. Trans. R. Soc. A (Math. Phys. Eng. Sci.) 2018, 376, 20170132. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, Z.; Zhou, Y.; Lu, X. Nonlinear dissipative devices in structural vibration control: A review. J. Sound Vib. 2018, 423, 18–49. [Google Scholar] [CrossRef]
- Cochelin, B.; Herzog, P.; Mattei, P.O. Experimental evidence of energy pumping in acoustics. C. R. Mec. 2006, 334, 639–644. [Google Scholar] [CrossRef]
- Bellizzi, S.; Côte, R.; Pachebat, M. Responses of a two degree-of-freedom system coupled to a nonlinear damper under multi-forcing frequencies. J. Sound Vib. 2013, 332, 1639–1653. [Google Scholar] [CrossRef]
- Bellet, R.; Cochelin, B.; Côte, R.; Mattei, P.O. Enhancing the dynamic range of targeted energy transfer in acoustics using several nonlinear membrane absorbers. J. Sound Vib. 2012, 331, 5657–5668. [Google Scholar] [CrossRef]
- Shao, J.; Luo, Q.; Deng, G.; Zeng, T.; Yang, J.; Wu, X.; Jin, C. Experimental study on influence of wall acoustic materials of 3D cavity for targeted energy transfer of a nonlinear membrane absorber. Appl. Acoust. 2021, 184, 108342. [Google Scholar] [CrossRef]
- Shao, J.; Zeng, T.; Wu, X. Study of a nonlinear membrane absorber applied to 3D acoustic cavity for low frequency broadband noise control. Materials 2019, 12, 1138. [Google Scholar] [CrossRef]
- Wu, X.; Shao, J.; Cochelin, B. Study of targeted energy transfer inside three-dimensional acoustic cavity by two nonlinear membrane absorbers and an acoustic mode. J. Vib. Acoust. 2016, 138, 031017. [Google Scholar] [CrossRef]
- Wu, X.; Shao, J.; Cochelin, B. Parameters design of a nonlinear membrane absorber applied to 3D acoustic cavity based on targeted energy transfer (TET). Noise Control Eng. J. 2016, 64, 99–113. [Google Scholar] [CrossRef]
- Shao, J.; Zeng, T.; Wu, X.; Yang, J. Influence of the pre-stress of the nonlinear membrane absorber for targeted energy transfer applied to 3D acoustic cavity. J. Braz. Soc. Mech. Sci. Eng. 2020, 42, 557. [Google Scholar] [CrossRef]
- Bryk, P.Y.; Bellizzi, S.; Côte, R. Experimental study of a hybrid electro-acoustic nonlinear membrane absorber. J. Sound Vib. 2018, 424, 224–237. [Google Scholar] [CrossRef]
- Bryk, P.Y.; Côte, R.; Bellizzi, S. Targeted energy transfer from a resonant room to a hybrid electro-acoustic nonlinear membrane absorber: Numerical and experimental study. J. Sound Vib. 2019, 460, 114868. [Google Scholar]
- Guo, X.; Lissek, H.; Fleury, R. Improving sound absorption through nonlinear active electroacoustic resonators. Phys. Rev. Appl. 2020, 13, 014018. [Google Scholar] [CrossRef]
- Tao, J.; Jing, R.; Qiu, X. Sound absorption of a finite micro-perforated panel backed by a shunted loudspeaker. J. Acoust. Soc. Am. 2014, 135, 231–238. [Google Scholar] [CrossRef]
- Lee, Y.Y.; Li, Q.S.; Leung, A.Y.T.; Su, R.K.L. The jump phenomenon effect on the sound absorption of a nonlinear panel absorber and sound transmission loss of a nonlinear panel backed by a cavity. Nonlinear Dyn. 2012, 69, 99–116. [Google Scholar] [CrossRef]





| 0.00011 m | |||
| 0.001 s | 0.04 m | ||
| 0.47 | 1.58 MPa |
| α | 0.01 | 0.02 | 0.03 | 0.04 | 0.05 | 0.06 |
| Q | 132.68 | 66.34 | 43.98 | 33.03 | 30.27 | 21.99 |
| β | 0.0025 | 0.005 | 0.0075 | 0.01 | 0.0125 | 0.015 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, J.; Luo, Q.; Zeng, T.; Wu, X. Influence of Boundary Impedance of 3D Cavity on Targeted Energy Transfer between a Damped Acoustic Mode and a Nonlinear Membrane Absorber. Machines 2022, 10, 841. https://doi.org/10.3390/machines10100841
Shao J, Luo Q, Zeng T, Wu X. Influence of Boundary Impedance of 3D Cavity on Targeted Energy Transfer between a Damped Acoustic Mode and a Nonlinear Membrane Absorber. Machines. 2022; 10(10):841. https://doi.org/10.3390/machines10100841
Chicago/Turabian StyleShao, Jianwang, Qimeng Luo, Tao Zeng, and Xian Wu. 2022. "Influence of Boundary Impedance of 3D Cavity on Targeted Energy Transfer between a Damped Acoustic Mode and a Nonlinear Membrane Absorber" Machines 10, no. 10: 841. https://doi.org/10.3390/machines10100841
APA StyleShao, J., Luo, Q., Zeng, T., & Wu, X. (2022). Influence of Boundary Impedance of 3D Cavity on Targeted Energy Transfer between a Damped Acoustic Mode and a Nonlinear Membrane Absorber. Machines, 10(10), 841. https://doi.org/10.3390/machines10100841










