Design and Evaluation of a Low-Cost Electromechanical System to Test Dynamic Performance of Force Sensors at Low Frequencies
Abstract
1. Introduction
1.1. Applications of Force/Pressure Sensors
1.2. Machines for Testing Force/Pressure Sensors
1.3. Rationale
2. Materials and Methods
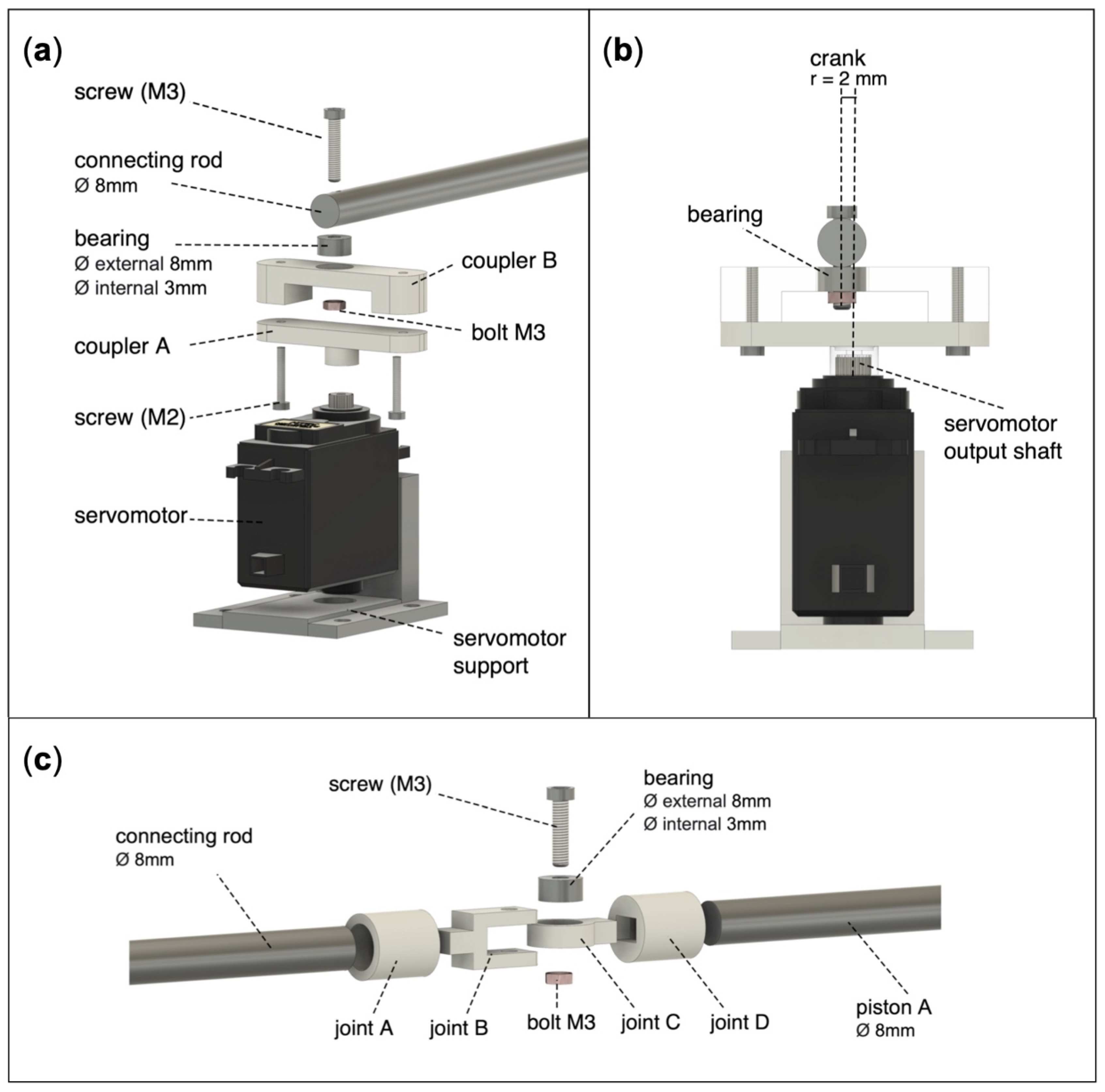
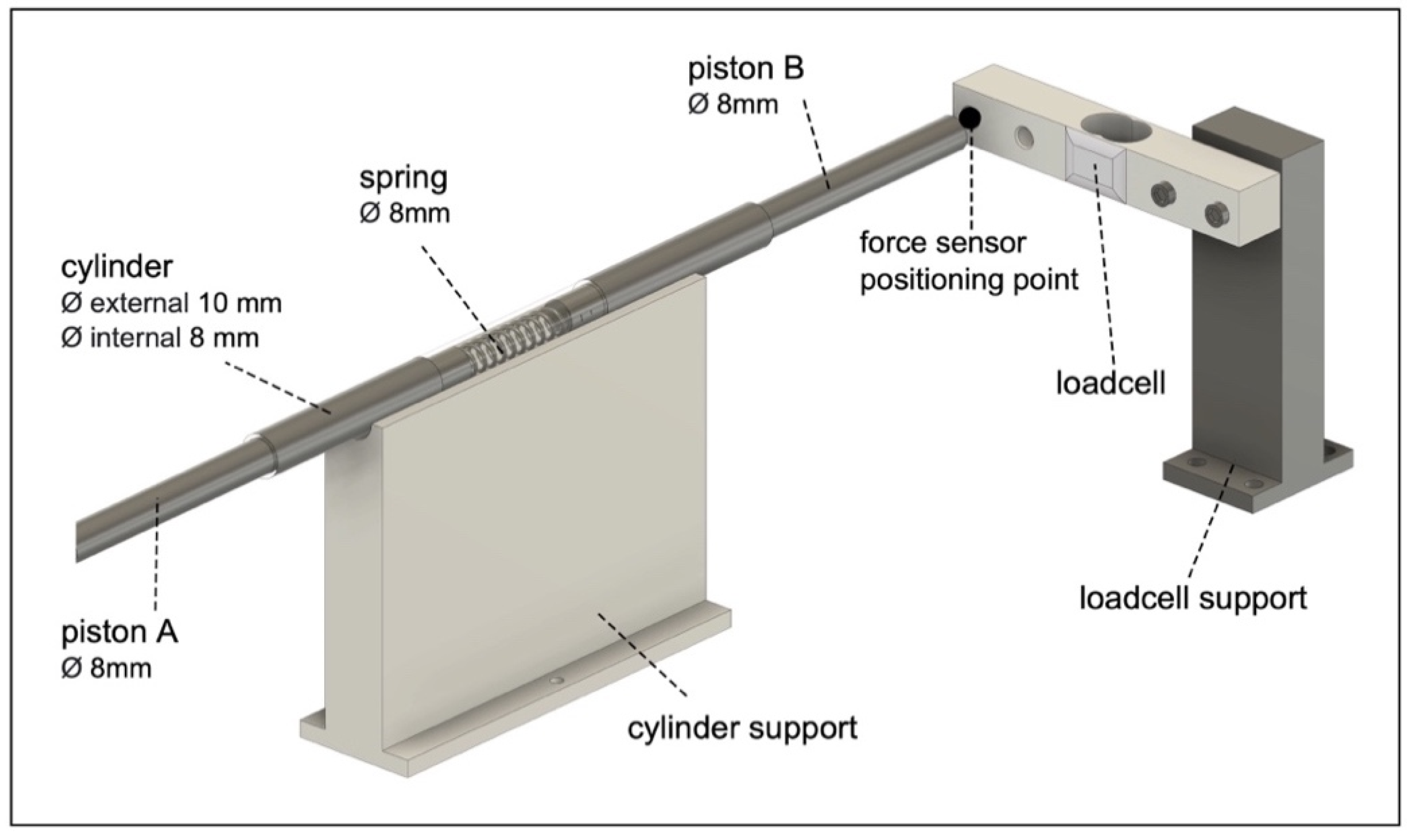
2.1. Electromechanical System Design and Operation
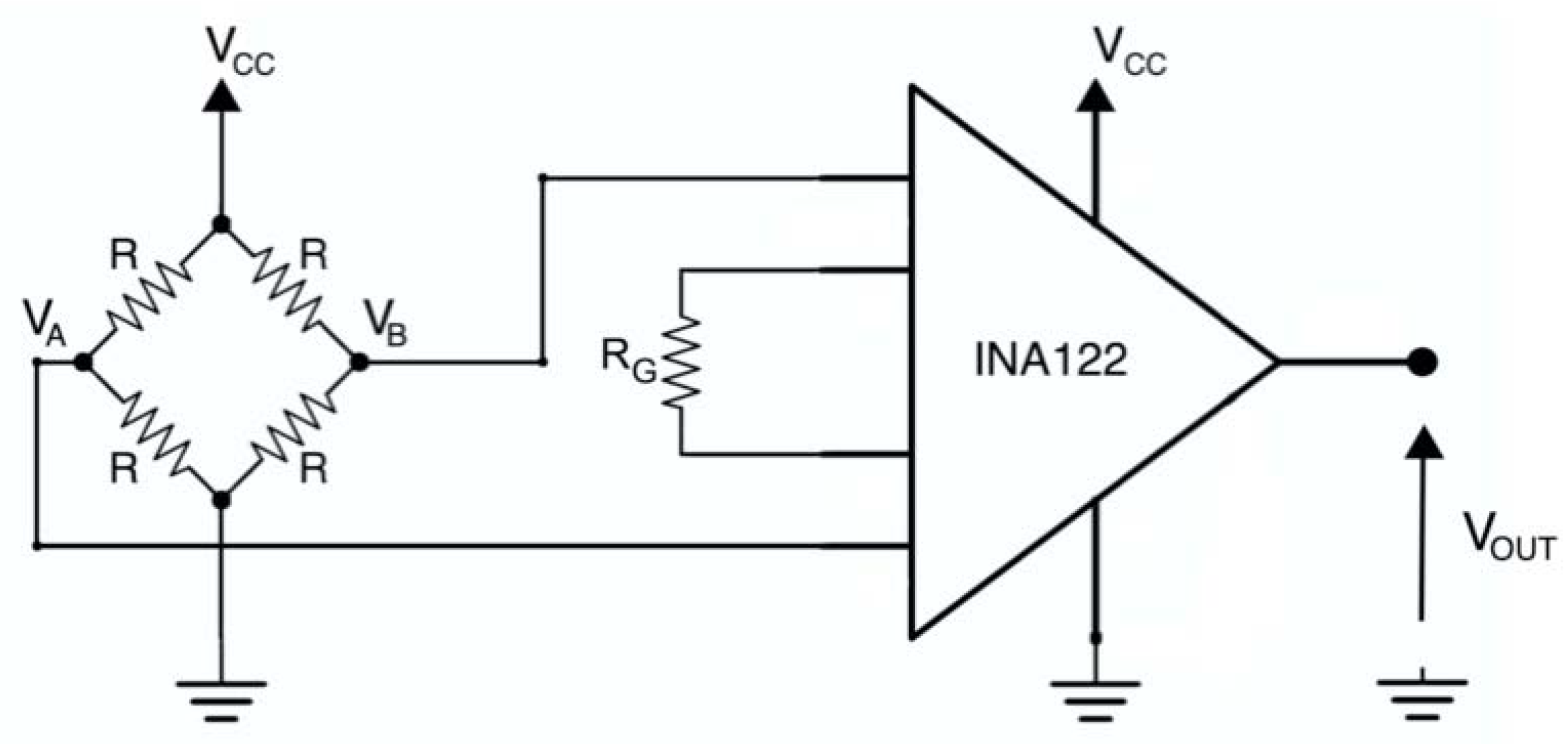
2.2. Loadcell and its Conditioning Circuit
2.3. Electromechanical System Testing
2.4. Testing Force Sensors for Biomedical Applications
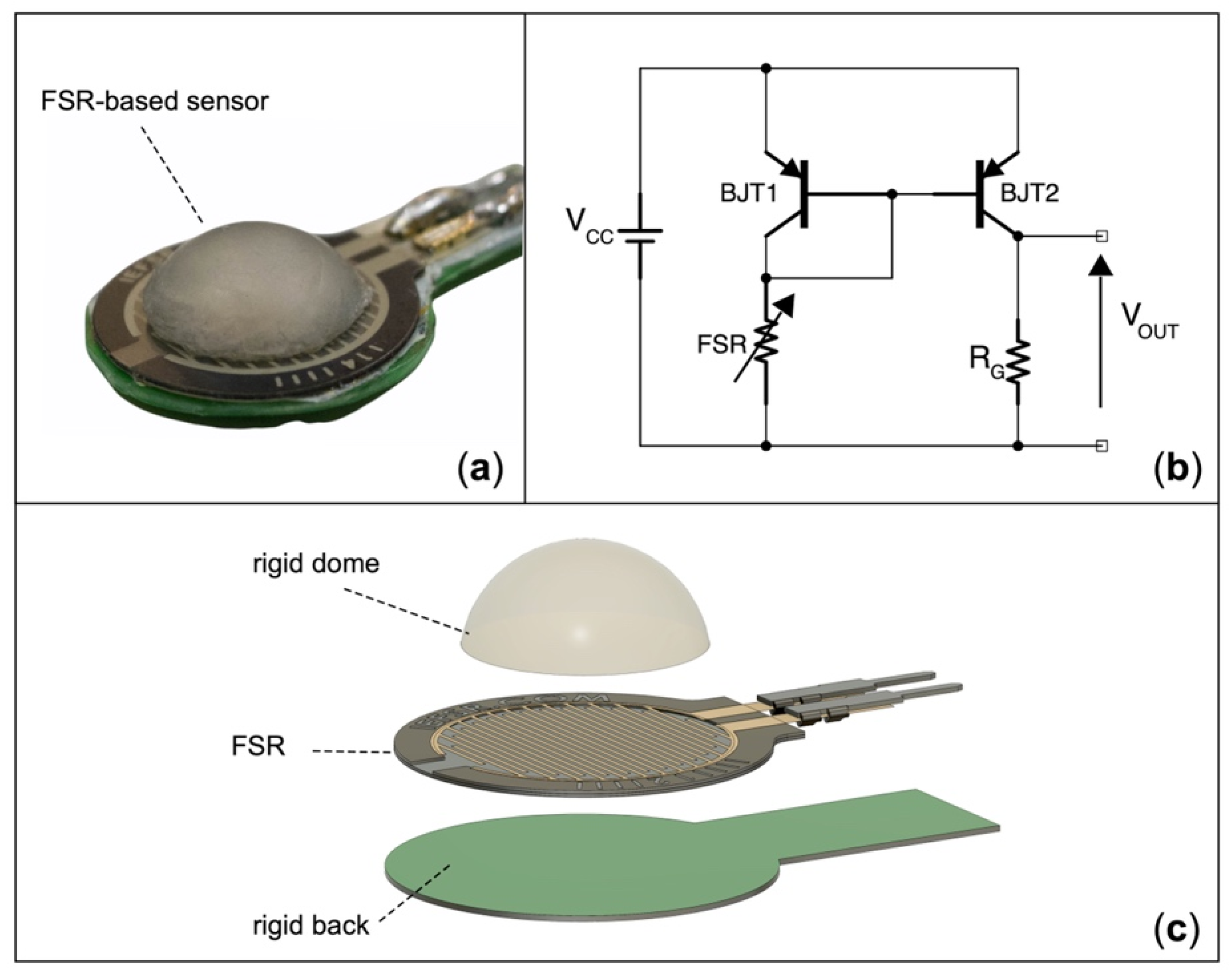
2.4.1. Low-Frequency Behavior of an FSR-Based Sensor
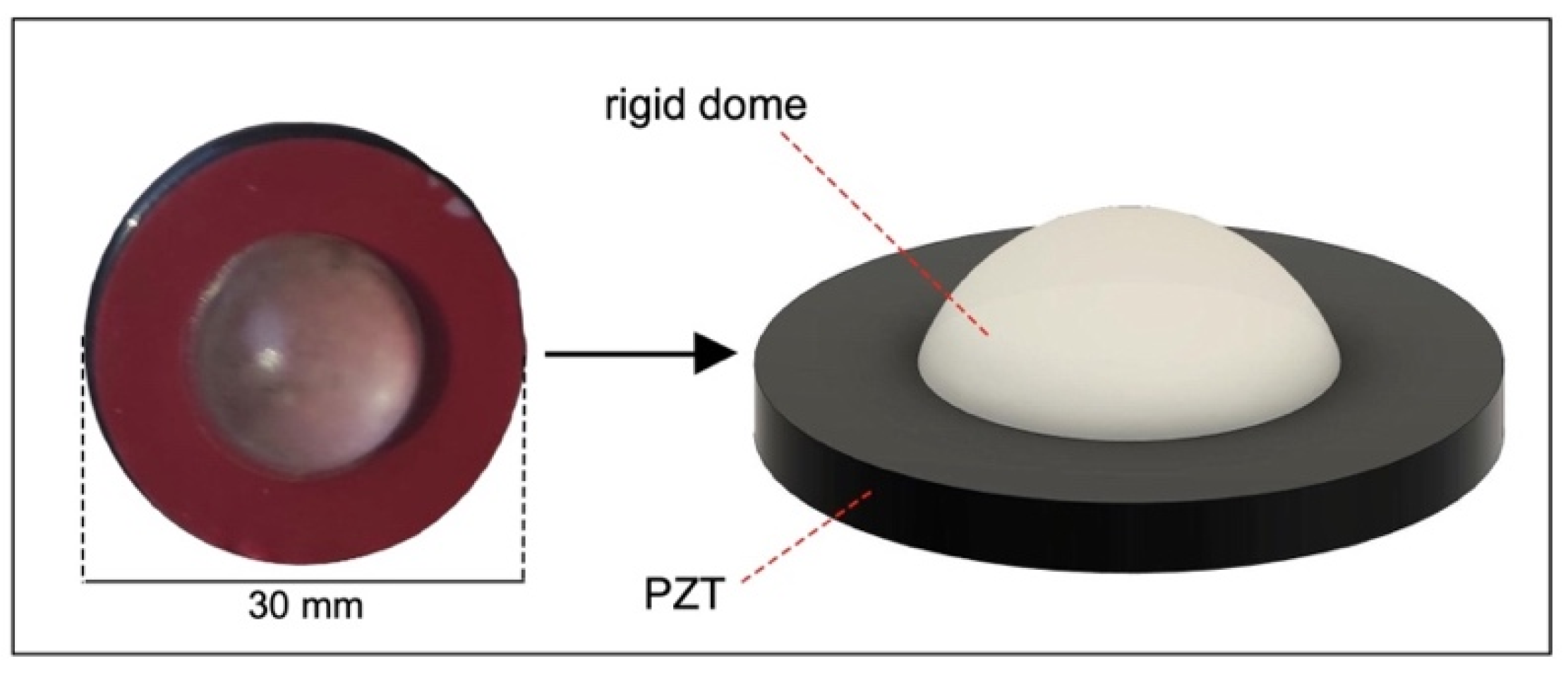
2.4.2. Low-Frequency Behavior of a PZT Sensor
3. Results
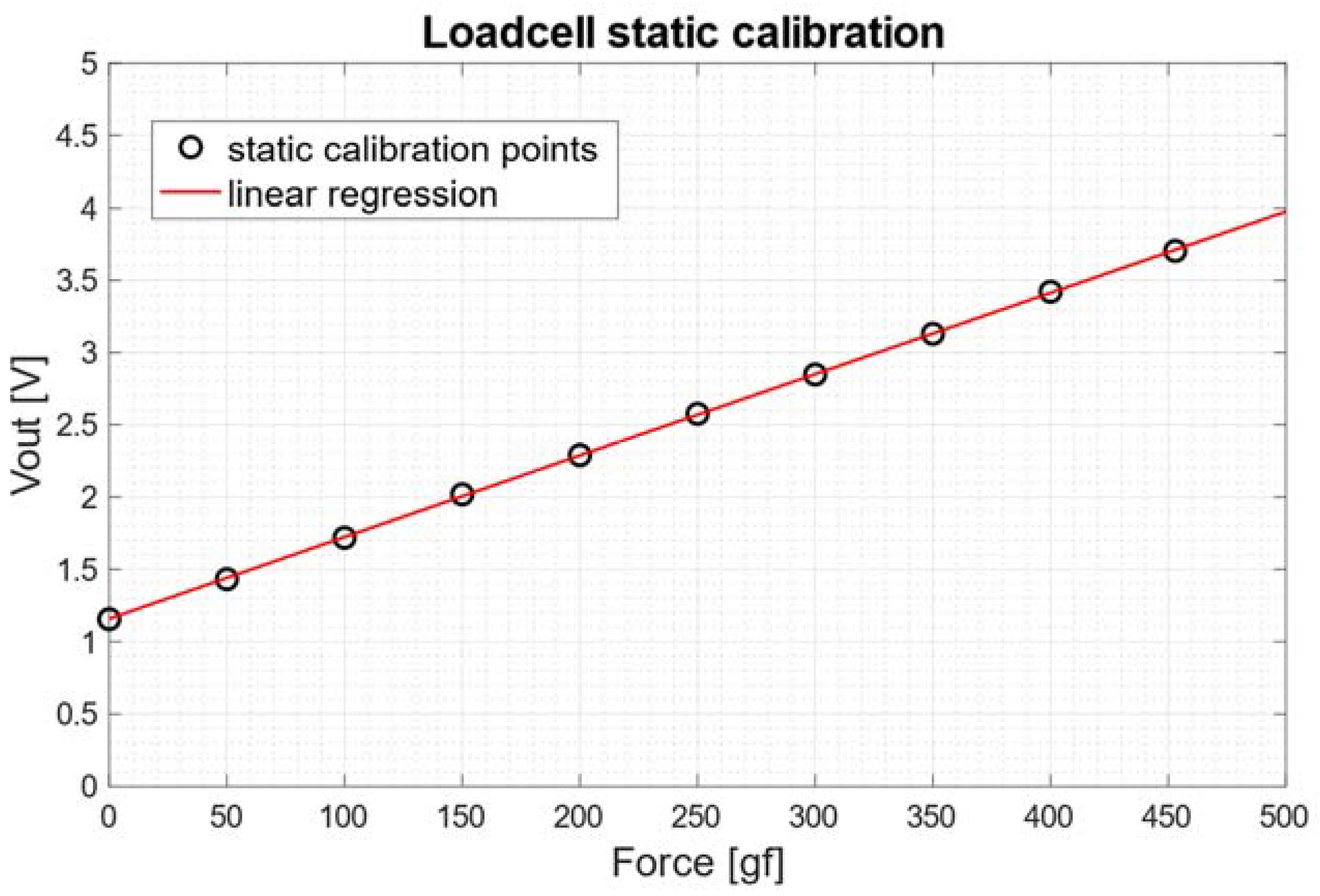
3.1. Static Calibration of the Loadcell
3.2. Static Calibration of the Slide Potentiometer
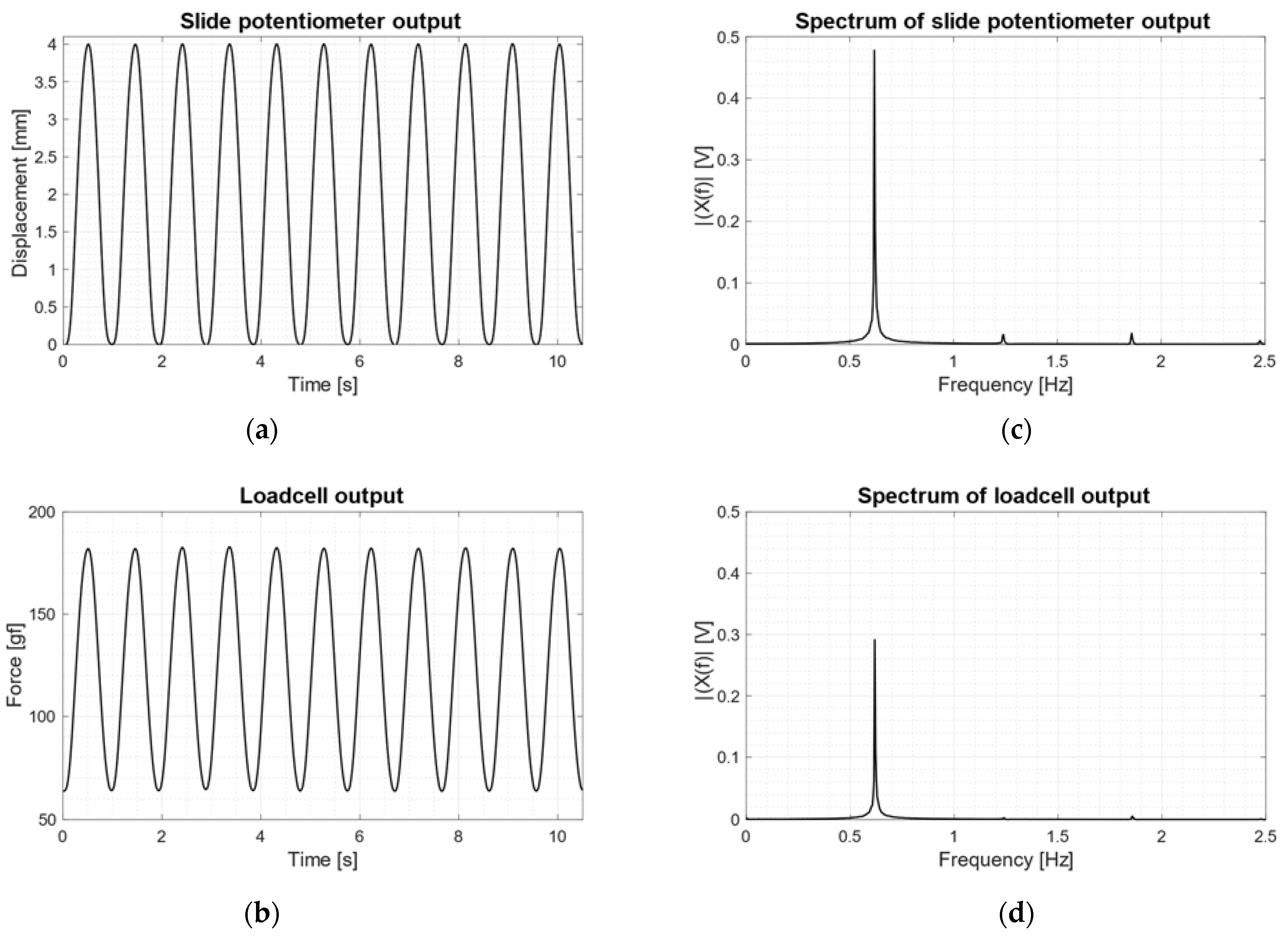
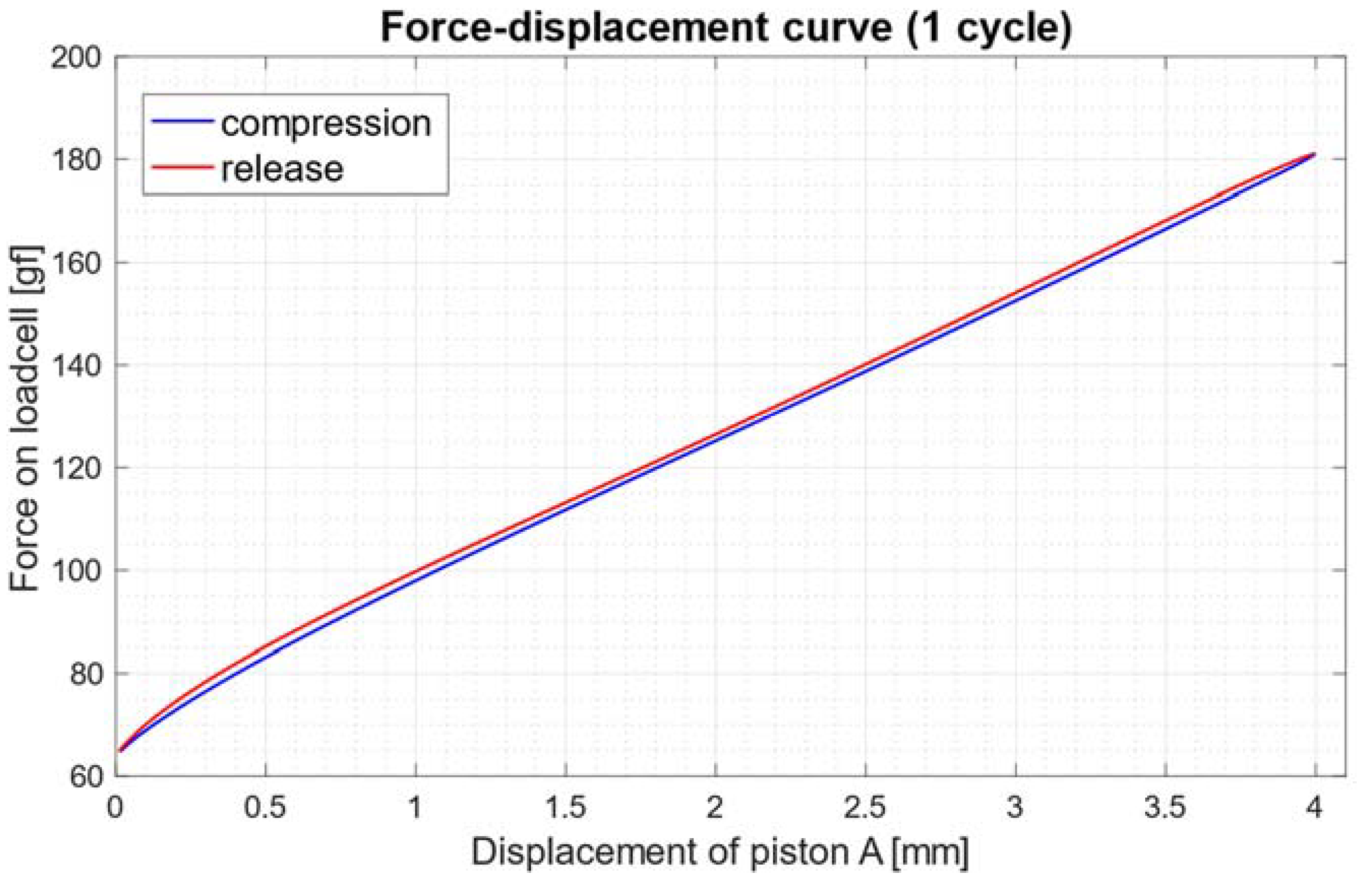
3.3. Results of the Electromechanical System Tests
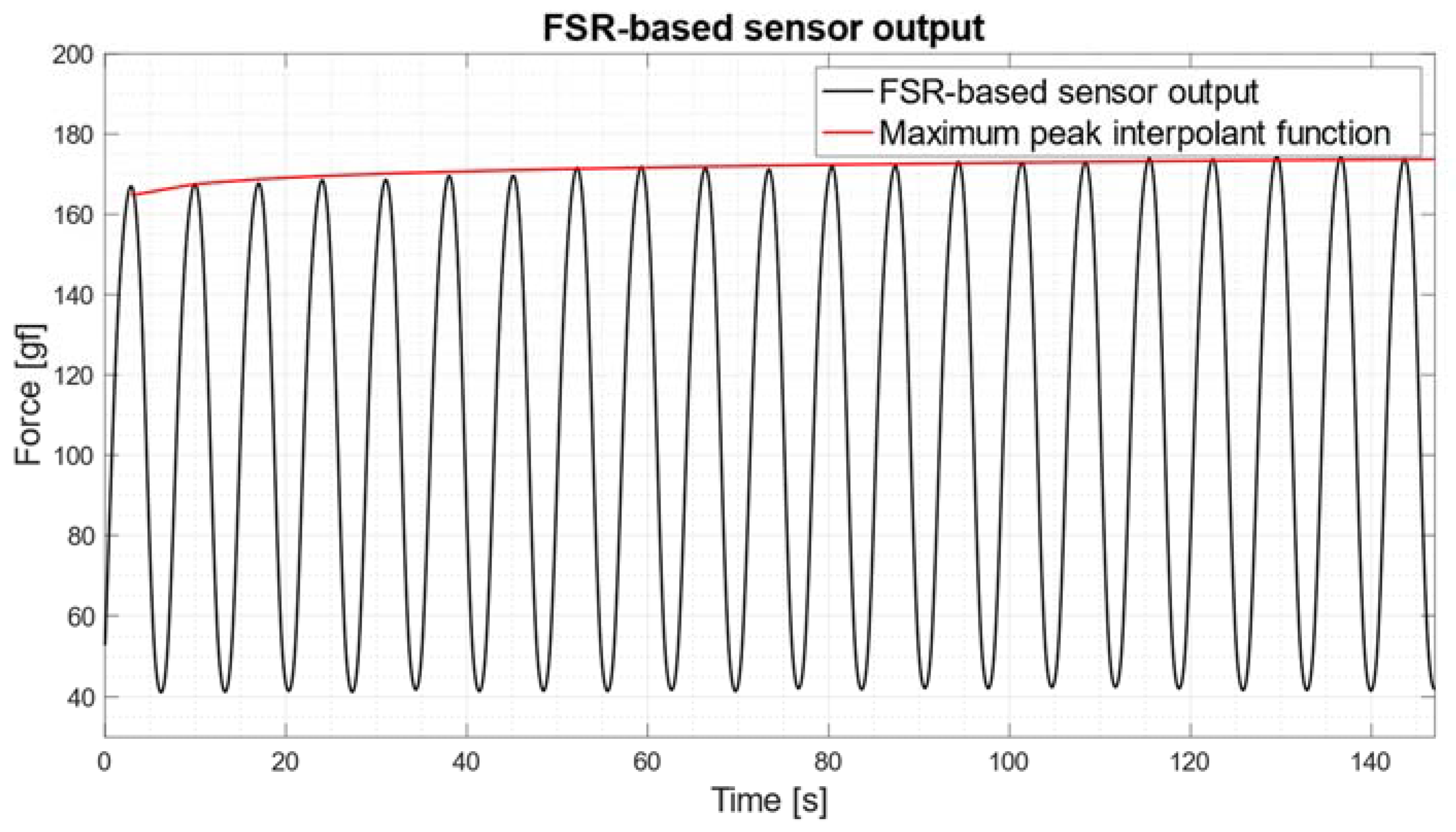
3.4. FSR-Based Sensor Test Results
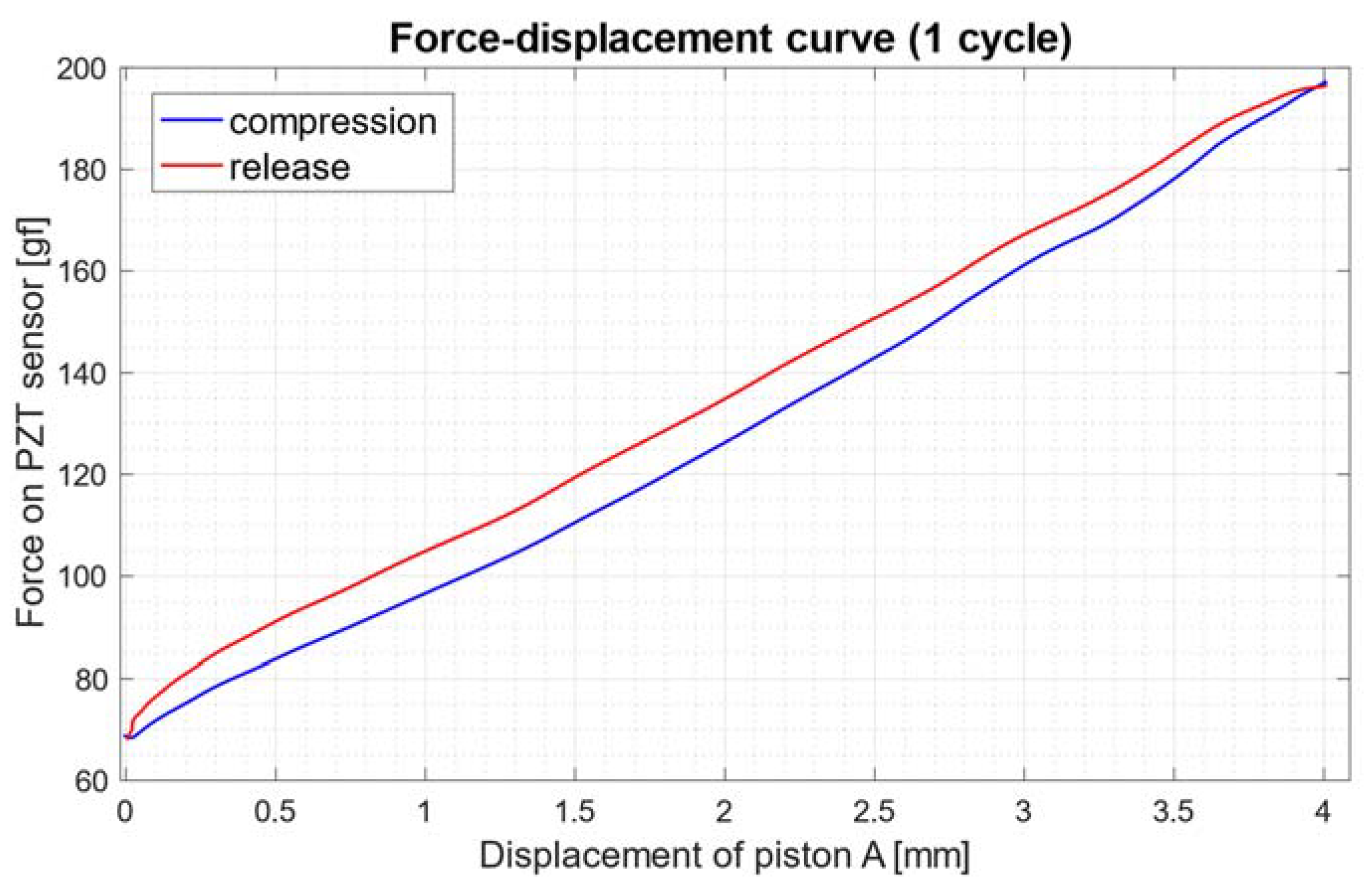
3.5. PZT Sensor Test Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Almassri, A.M.; Wan Hasan, W.Z.; Ahmad, S.A.; Ishak, A.J.; Ghazali, A.M.; Talib, D.N.; Wada, C. Pressure sensor: State of the art, design, and application for robotic hand. J. Sens. 2015, 2015, e846487. [Google Scholar] [CrossRef]
- Chuah, M.Y.; Estrada, M.; Kim, S. Composite force sensing foot utilizing volumetric displacement of a Hyperelastic polymer. In Proceedings of the 2012 IEEE/RSJ International Conference on Intelligent Robots and Systems, Vilamoura-Algarve, Portugal, 7–12 October 2012; pp. 1963–1969. [Google Scholar]
- Donati, M.; Vitiello, N.; De Rossi, S.M.M.; Lenzi, T.; Crea, S.; Persichetti, A.; Giovacchini, F.; Koopman, B.; Podobnik, J.; Munih, M.; et al. A flexible sensor technology for the distributed measurement of interaction pressure. Sensors 2013, 13, 1021–1045. [Google Scholar] [CrossRef] [PubMed]
- Hoffmann, K. Applying the Wheatstone Bridge Circuit. HBM: Darmstadt, Germany, 1974. [Google Scholar]
- Tekscan|Pressure Mapping, Force Measurement, & Tactile Sensors. Available online: https://tekscan.com/ (accessed on 26 June 2022).
- Sensitronics—Manufacturers of the Force Sensing Resistor and Other Force-Sensing Electronic Components. Available online: https://www.sensitronics.com/ (accessed on 26 June 2022).
- Andreozzi, E.; Centracchio, J.; Esposito, D.; Bifulco, P. A Comparison of Heart Pulsations Provided by Forcecardiography and Double Integration of Seismocardiogram. Bioengineering 2022, 9, 167. [Google Scholar] [CrossRef]
- Centracchio, J.; Andreozzi, E.; Esposito, D.; Gargiulo, G.D. Respiratory-induced amplitude modulation of forcecardiography signals. Bioengineering 2022, 9, 444. [Google Scholar] [CrossRef] [PubMed]
- Andreozzi, E.; Gargiulo, G.D.; Esposito, D.; Bifulco, P. A novel broadband Forcecardiography sensor for simultaneous monitoring of respiration, infrasonic cardiac vibrations and heart sounds. Front. Physiol. 2021, 12, 1988. [Google Scholar] [CrossRef]
- Esposito, D.; Centracchio, J.; Andreozzi, E.; Gargiulo, G.D.; Naik, G.R.; Bifulco, P. Biosignal-based human–machine interfaces for assistance and rehabilitation: A survey. Sensors 2021, 21, 6863. [Google Scholar] [CrossRef]
- Smith, B.T.; Coiro, D.J.; Finson, R.; Betz, R.R.; McCarthy, J. Evaluation of force-sensing resistors for gait event detection to trigger electrical stimulation to improve walking in the child with cerebral palsy. IEEE Trans. Neural. Syst. Rehabil. Eng. 2002, 10, 22–29. [Google Scholar] [CrossRef]
- Esposito, D.; Andreozzi, E.; Fratini, A.; Gargiulo, G.D.; Savino, S.; Niola, V.; Bifulco, P. A piezoresistive sensor to measure muscle contraction and mechanomyography. Sensors 2018, 18, 2553. [Google Scholar] [CrossRef]
- Esposito, D.; Andreozzi, E.; Gargiulo, G.D.; Fratini, A.; D’Addio, G.; Naik, G.R.; Bifulco, P. A piezoresistive array armband with reduced number of sensors for hand gesture recognition. Front. Neurorobot. 2020, 13, 00114. [Google Scholar] [CrossRef]
- Andreozzi, E.; Fratini, A.; Esposito, D.; Naik, G.; Polley, C.; Gargiulo, G.D.; Bifulco, P. Forcecardiography: A novel technique to measure heart mechanical vibrations onto the chest wall. Sensors 2020, 20, 3885. [Google Scholar] [CrossRef]
- Andreozzi, E.; Centracchio, J.; Punzo, V.; Esposito, D.; Polley, C.; Gargiulo, G.D.; Bifulco, P. Respiration monitoring via forcecardiography sensors. Sensors 2021, 21, 3996. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Sun, C.; Yuan, W.; Gu, W.; Cui, Z.; Chen, W. Smart mat system with pressure sensor array for unobtrusive sleep monitoring. In Proceedings of the 2017 39th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Jeju, Korea, 11–15 July 2017; pp. 177–180. [Google Scholar]
- Park, S.W.; Das, P.S.; Chhetry, A.; Park, J.Y. A flexible capacitive pressure sensor for wearable respiration monitoring system. IEEE Sens. J. 2017, 17, 6558–6564. [Google Scholar] [CrossRef]
- Munz, M.; Wolf, N. Simulation of breathing patterns and classification of sensor data for the early detection of impending sudden infant death. Curr. Dir. Biomed. Eng. 2019, 5, 401–403. [Google Scholar] [CrossRef][Green Version]
- Spilz, A.; Engleder, T.; Munz, M.; Karge, M. Development of a smart fabric force-sensing glove for physiotherapeutic applications. Curr. Dir. Biomed. Eng. 2019, 5, 513–515. [Google Scholar] [CrossRef]
- EIT-Based Fabric Pressure Sensing. Available online: https://www.hindawi.com/journals/cmmm/2013/405325/ (accessed on 26 June 2022).
- Teyeme, Y.; Malengier, B.; Tesfaye, T.; Van Langenhove, L. A fabric-based textile stretch sensor for optimized measurement of strain in clothing. Sensors 2020, 20, 7323. [Google Scholar] [CrossRef] [PubMed]
- Alias, N.M.; Razak, Z.A.; Janjori, M.; Ahmad, M.Y.; Engkasan, J.P.; Hamzaid, N.A. Epillow: A fabric-based pressure sensor array for tetraplegic patient call detection system. J. Teknol. 2022, 84, 183–192. [Google Scholar] [CrossRef]
- Martinez-Cesteros, J.; Medrano-Sanchez, C.; Plaza-Garcia, I.; Igual-Catalan, R.; Albiol-Pérez, S. A velostat-based pressure-sensitive mat for center-of-pressure measurements: A preliminary study. Int. J. Environ. Res. Public Health 2021, 18, 5958. [Google Scholar] [CrossRef] [PubMed]
- Dzedzickis, A.; Sutinys, E.; Bucinskas, V.; Samukaite-Bubniene, U.; Jakstys, B.; Ramanavicius, A.; Morkvenaite-Vilkonciene, I. Polyethylene-carbon composite (Velostat®) based tactile sensor. Polymers 2020, 12, 2905. [Google Scholar] [CrossRef] [PubMed]
- Cay, G.; Ravichandran, V.; Saikia, M.J.; Hoffman, L.; Laptook, A.; Padbury, J.; Salisbury, A.L.; Gitelson-Kahn, A.; Venkatasubramanian, K.; Shahriari, Y.; et al. An E-textile respiration sensing system for NICU monitoring: Design and validation. J. Sign. Process. Syst. 2022, 94, 543–557. [Google Scholar] [CrossRef]
- Bucinskas, V.; Dzedzickis, A.; Rozene, J.; Subaciute-Zemaitiene, J.; Satkauskas, I.; Uvarovas, V.; Bobina, R.; Morkvenaite-Vilkonciene, I. Wearable feet pressure sensor for human gait and falling diagnosis. Sensors 2021, 21, 5240. [Google Scholar] [CrossRef]
- Hudec, R.; Matúška, S.; Kamencay, P.; Benco, M. A smart IoT system for detecting the position of a lying person using a novel textile pressure sensor. Sensors 2021, 21, 206. [Google Scholar] [CrossRef] [PubMed]
- Schlegel, C.; Kiekenap, G.; Glöckner, B.; Kumme, R. Sinusoidal calibration of force transducers using electrodynamic shaker systems. Sens. Transducers 2012, 14, 95–111. [Google Scholar]
- Schlegel, C.; Kieckenap, G.; Kumme, R. Application of a scanning vibrometer for the periodic calibration of force transducers. In Proceedings of the International Conference on IMEKO Metrology for Green Growth, Busan, Korea, 9–14 September 2012; Volume 1, pp. 651–656. [Google Scholar]
- Medina, N.; De Vicente, J. Force sensor characterization under sinusoidal excitations. Sensors 2014, 14, 18454–18473. [Google Scholar] [CrossRef] [PubMed]
- Medina, N.; Robles, J.; De Vicente, J. Realization of sinusoidal forces at CEM. In Proceedings of the IMEKO Proceedings TC3 Conference 2014, Cape Town, South Africa, 3–5 February 2014; E.T.S.I. Industriales (UPM): Ciudad del Cabo, Sudáfica, 2014; pp. 58–61. [Google Scholar]
- Kobusch, M.; Link, A.; Buss, A.; Bruns, T. Comparison of shock and sine force calibration methods. In Proceedings of the IMEKO TC3 & TC16 & TC22 International Conference, Merida, Mexico, 27–30 November 2007. [Google Scholar]
- Medina, N.; de Vicente, J.; Robles, J. Magnetic fields influence on sensors with electrical output under sinusoidal excitations. ACTA IMEKO 2016, 5, 51–58. [Google Scholar] [CrossRef][Green Version]
- Andreozzi, E.; Gargiulo, G.D.; Fratini, A.; Esposito, D.; Bifulco, P. A contactless sensor for pacemaker pulse detection: Design hints and performance assessment. Sensors 2018, 18, 2715. [Google Scholar] [CrossRef]
- Swanson, E.C.; Weathersby, E.J.; Cagle, J.C.; Sanders, J.E. Evaluation of force sensing resistors for the measurement of interface pressures in lower limb prosthetics. J. Biomech. Eng. 2019, 141, 1010091–10100913. [Google Scholar] [CrossRef]
- Out of Production 5900 Series Universal Testing Systems. Available online: http://www.instron.com/en-us/products/testing-systems/universal-testing-systems/low-force-universal-testing-systems/5900-series (accessed on 18 August 2022).
- Dabling, J.G.; Filatov, A.; Wheeler, J.W. Static and cyclic performance evaluation of sensors for human interface pressure measurement. In Proceedings of the 2012 Annual International Conference of the IEEE Engineering in Medicine and Biology Society, San Diego, CA, USA, 28 August–1 September 2012; Volume 2012, pp. 162–165. [Google Scholar] [CrossRef]
- MTS. Available online: https://www.mts.com/en/applications/materials/test-type/dynamic-mechanical-analysis (accessed on 18 August 2022).
- Saadeh, M.Y.; Carambat, T.D.; Arrieta, A.M. Evaluating and modeling force sensing resistors for low force applications. In Proceedings of the ASME 2017 Conference on Smart Materials, Adaptive Structures and Intelligent Systems, Snowbird, UT, USA, 18–20 September 2017. [Google Scholar]
- Hall, R.S.; Desmoulin, G.T.; Milner, T.E. A Technique for conditioning and calibrating force-sensing resistors for repeatable and reliable measurement of compressive force. J. Biomech. 2008, 41, 3492–3495. [Google Scholar] [CrossRef]
- FlexiForce Sensor Characterization Kit. Available online: https://tekscan.com/products-solutions/electronics/flexiforce-sensor-characterization-kit (accessed on 17 August 2022).
- Hitec HITEC Robot Servo. Available online: https://hitecrcd.com/products/servos/discontinued-servos-servo-accessories/hsr-5990tg-hmi-ultra-premium-robot-servo/product (accessed on 20 August 2020).
- Crank Mechanism—An Overview|ScienceDirect Topics. Available online: https://www.sciencedirect.com/topics/engineering/crank-mechanism (accessed on 18 June 2022).
- Coefficient of Friction Reference Chart. Available online: https://www.schneider-company.com/coefficient-of-friction-reference-chart/ (accessed on 19 June 2022).
- Esposito, D.; Savino, S.; Cosenza, C.; Andreozzi, E.; Gargiulo, G.D.; Polley, C.; Cesarelli, G.; D’Addio, G.; Bifulco, P. Evaluation of Grip Force and Energy Efficiency of the “Federica” Hand. Machines 2021, 9, 25. [Google Scholar] [CrossRef]
- Total Harmonic Distortion—An Overview|ScienceDirect Topics. Available online: https://www.sciencedirect.com/topics/engineering/total-harmonic-distortion (accessed on 29 July 2022).
- Interlink Electronics FSR Sensor, Force Sensing Resistor|Interlink Electronics. Available online: https://www.interlinkelectronics.com/force-sensing-resistor (accessed on 13 November 2020).
- Esposito, D.; Gargiulo, G.D.; Parajuli, N.; Cesarelli, G.; Andreozzi, E.; Bifulco, P. Measurement of muscle contraction timing for prosthesis control: A comparison between electromyography and force-myography. In Proceedings of the 2020 IEEE International Symposium on Medical Measurements and Applications (MeMeA), Bari, Italy, 1 June–1 July 2020; pp. 1–6. [Google Scholar]
- Esposito, D.; Savino, S.; Andreozzi, E.; Cosenza, C.; Niola, V.; Bifulco, P. The “Federica” hand. Bioengineering 2021, 8, 128. [Google Scholar] [CrossRef]
- Esposito, D.; Cosenza, C.; Gargiulo, G.D.; Andreozzi, E.; Niola, V.; Fratini, A.; D’Addio, G.; Bifulco, P. Experimental study to improve “Federica” prosthetic hand and its control system. In Proceedings of the XV Mediterranean Conference on Medical and Biological Engineering and Computing—MEDICON 2019; Henriques, J., Neves, N., de Carvalho, P., Eds.; Springer International Publishing: Cham, Switzerland, 2020; pp. 586–593. [Google Scholar]
- Esposito, D.; Centracchio, J.; Andreozzi, E.; Savino, S.; Gargiulo, G.D.; Naik, G.R.; Bifulco, P. Design of a 3D-printed hand exoskeleton based on force-myography control for assistance and rehabilitation. Machines 2022, 10, 57. [Google Scholar] [CrossRef]
- Paredes-Madrid, L.; Matute, A.; Bareño, J.O.; Parra Vargas, C.A.; Gutierrez Velásquez, E.I. Underlying physics of conductive polymer composites and force sensing resistors (FSRs). A study on creep response and dynamic loading. Materials 2017, 10, 1334. [Google Scholar] [CrossRef] [PubMed]
- Centracchio, J.; Andreozzi, E.; Esposito, D.; Gargiulo, G.D.; Bifulco, P. Detection of aortic valve opening and estimation of pre-ejection period in forcecardiography recordings. Bioengineering 2022, 9, 89. [Google Scholar] [CrossRef] [PubMed]
- Cosenza, C.; Nicolella, A.; Esposito, D.; Niola, V.; Savino, S. Mechanical system control by RGB-D device. Machines 2021, 9, 3. [Google Scholar] [CrossRef]




| COMPONENT | COST (USD) |
|---|---|
| 3D printed components (PLA) | 5 |
| metal mechanical components (tubulars, bolts, screws, etc.) | 10 |
| servomotor (Hitec HSR-5990TG) | 35 |
| single axis loadcell (1 Kg) | 10 |
| electric/electronic components (battery pack, INA, potentiometer, etc.) | 10 |
| Total | 70 |
| Power Supply [V] | Stress Frequency [Hz] | Total Harmonic Distortion [%] | Electromechanical System Hysteresis [gf mm]; [µJ] |
|---|---|---|---|
| 1 | 0.09 | 3.39 | 3.09; 30.3 |
| 2 | 0.20 | 3.27 | 3.52; 34.5 |
| 3 | 0.30 | 3.13 | 3.29; 32.3 |
| 4 | 0.41 | 2.99 | 2.55; 25.0 |
| 5 | 0.49 | 3.05 | 3.67; 36.0 |
| 6 | 0.62 | 2.60 | 3.27; 32.1 |
| 7 | 0.70 | 2.46 | 3.63; 35.6 |
| 8 | 0.80 | 2.14 | 6.14; 60.2 |
| 9 | 0.91 | 1.88 | 3.43; 33.6 |
| Stress Frequency [Hz] | FSR Hysteresis [gf mm]; [µJ] | Percent Error on Minimum [%] | Percent Error on Maximum [%] | Percent Error on Mean [%] | Percent Error on SD [%] | Corr. Coeff. (r): FSR vs. Loadcell |
|---|---|---|---|---|---|---|
| 0.09 | 61.04; 598.6 | 21.33 | 4.95 | 9.33 | 2.66 | 0.99 |
| 0.20 | 59.21; 580.7 | 1.84 | 2.13 | 1.30 | 2.04 | 0.99 |
| 0.30 | 62.38; 611.8 | 8.01 | 1.07 | 3.44 | 2.28 | 0.99 |
| 0.41 | 57.09; 559.9 | 6.53 | 1.47 | 1.77 | 1.52 | 0.99 |
| 0.49 | 53.79; 527.5 | 7.39 | 2.11 | 1.66 | 1.39 | 0.99 |
| 0.62 | 47.51; 465.9 | 3.90 | 1.00 | 1.46 | 5.38 | 0.99 |
| 0.70 | 47.93; 470.0 | 7.09 | 2.25 | 0.75 | 0.52 | 0.99 |
| 0.80 | 50.33; 493.6 | 4.07 | 0.47 | 2.20 | 3.08 | 0.99 |
| 0.91 | 39.86; 390.9 | 0.90 | 2.29 | 0.10 | 1.01 | 0.99 |
| Stress Frequency [Hz] | PZT Hysteresis [gf mm]; [µJ] | Percent Error on Minimum [%] | Percent Error on Maximum [%] | Percent Error on Mean [%] | Percent Error on SD [%] | Corr. Coeff. (r): PZT vs. Loadcell |
|---|---|---|---|---|---|---|
| 0.09 | 26.55; 260.4 | 6.54 | 6.24 | 0.15 | 9.29 | 0.99 |
| 0.20 | 21.56; 211.4 | 5.11 | 0.14 | 0.84 | 1.50 | 0.99 |
| 0.30 | 19.93; 195.5 | 3.50 | 0.82 | 0.93 | 0.58 | 0.99 |
| 0.41 | 18.60; 182.4 | 3.55 | 0.22 | 1.10 | 0.51 | 0.99 |
| 0.49 | 18.25; 179.0 | 4.99 | 0.90 | 0.01 | 0.38 | 0.99 |
| 0.62 | 18.04; 177.0 | 3.51 | 0.77 | 0.16 | 1.07 | 0.99 |
| 0.70 | 17.90; 175.5 | 2.43 | 1.09 | 0.20 | 2.21 | 0.99 |
| 0.80 | 16.90; 165.7 | 7.77 | 0.84 | 0.82 | 1.67 | 0.99 |
| 0.91 | 15.92; 156.1 | 3.44 | 1.67 | 0.48 | 0.38 | 0.99 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Esposito, D.; Centracchio, J.; Andreozzi, E.; Bifulco, P.; Gargiulo, G.D. Design and Evaluation of a Low-Cost Electromechanical System to Test Dynamic Performance of Force Sensors at Low Frequencies. Machines 2022, 10, 1017. https://doi.org/10.3390/machines10111017
Esposito D, Centracchio J, Andreozzi E, Bifulco P, Gargiulo GD. Design and Evaluation of a Low-Cost Electromechanical System to Test Dynamic Performance of Force Sensors at Low Frequencies. Machines. 2022; 10(11):1017. https://doi.org/10.3390/machines10111017
Chicago/Turabian StyleEsposito, Daniele, Jessica Centracchio, Emilio Andreozzi, Paolo Bifulco, and Gaetano D. Gargiulo. 2022. "Design and Evaluation of a Low-Cost Electromechanical System to Test Dynamic Performance of Force Sensors at Low Frequencies" Machines 10, no. 11: 1017. https://doi.org/10.3390/machines10111017
APA StyleEsposito, D., Centracchio, J., Andreozzi, E., Bifulco, P., & Gargiulo, G. D. (2022). Design and Evaluation of a Low-Cost Electromechanical System to Test Dynamic Performance of Force Sensors at Low Frequencies. Machines, 10(11), 1017. https://doi.org/10.3390/machines10111017









