A Nonlinear Vibration Control of a String Using the Method Based on Its Time-Varying Length
Abstract
:1. Introduction
2. Problem Formulation
3. Dynamic Analysis of the Time-Varying Length String
3.1. Free Vibrations Equations
3.2. Forced Vibrations Equations
3.3. Discretization of the Vibration Equation
4. Results and Discussions
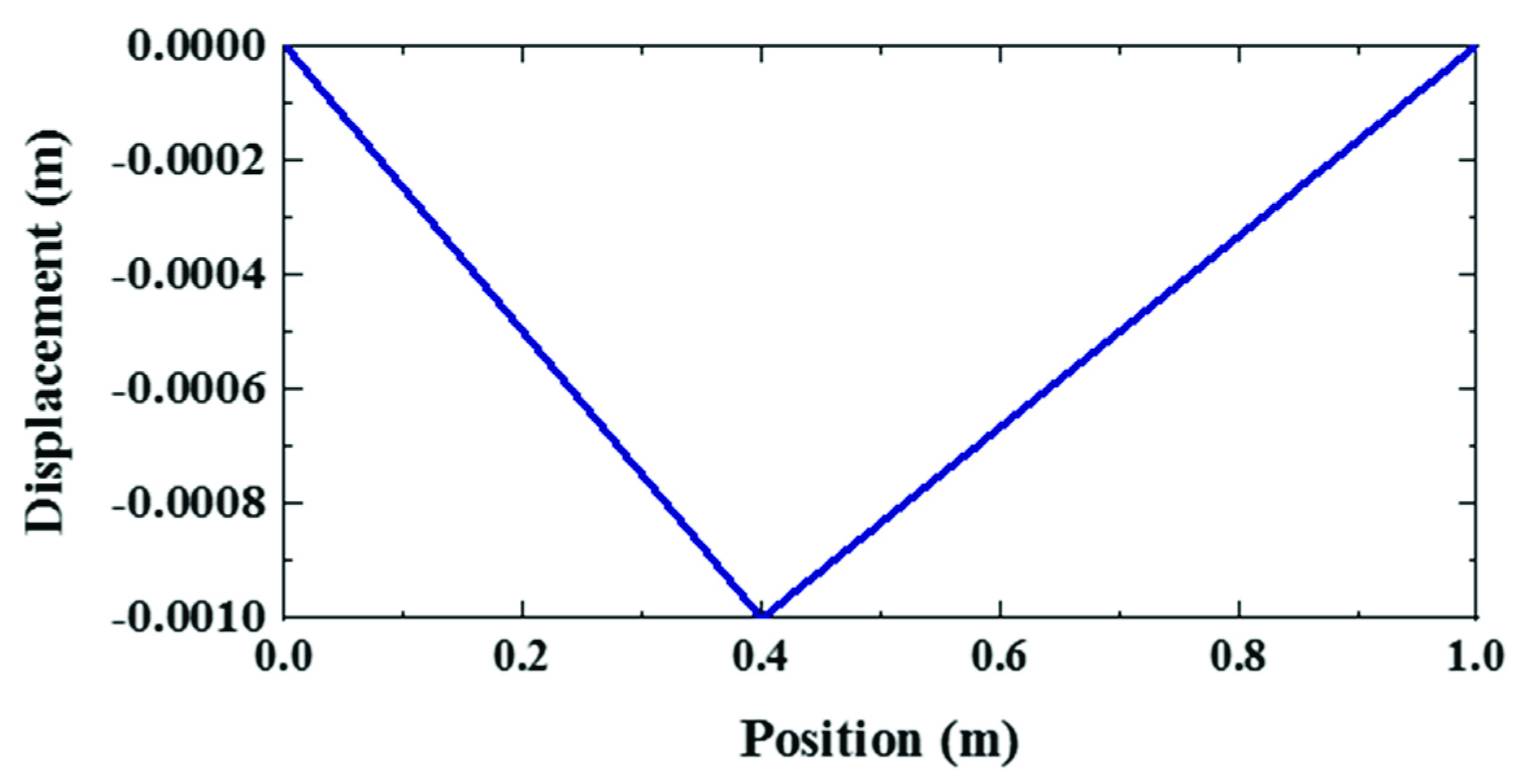
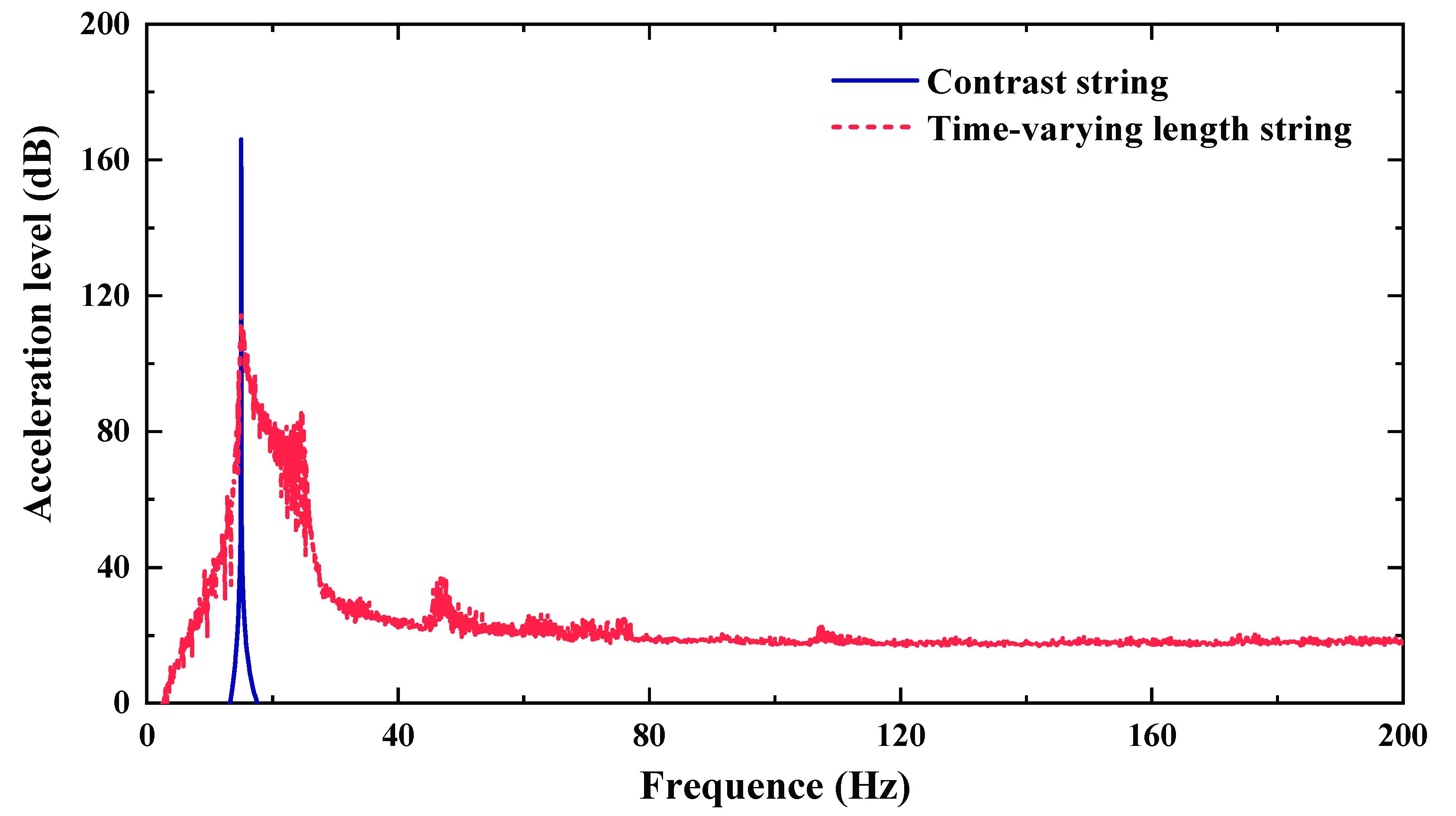
4.1. Free Vibrations of Time-Varying Length String
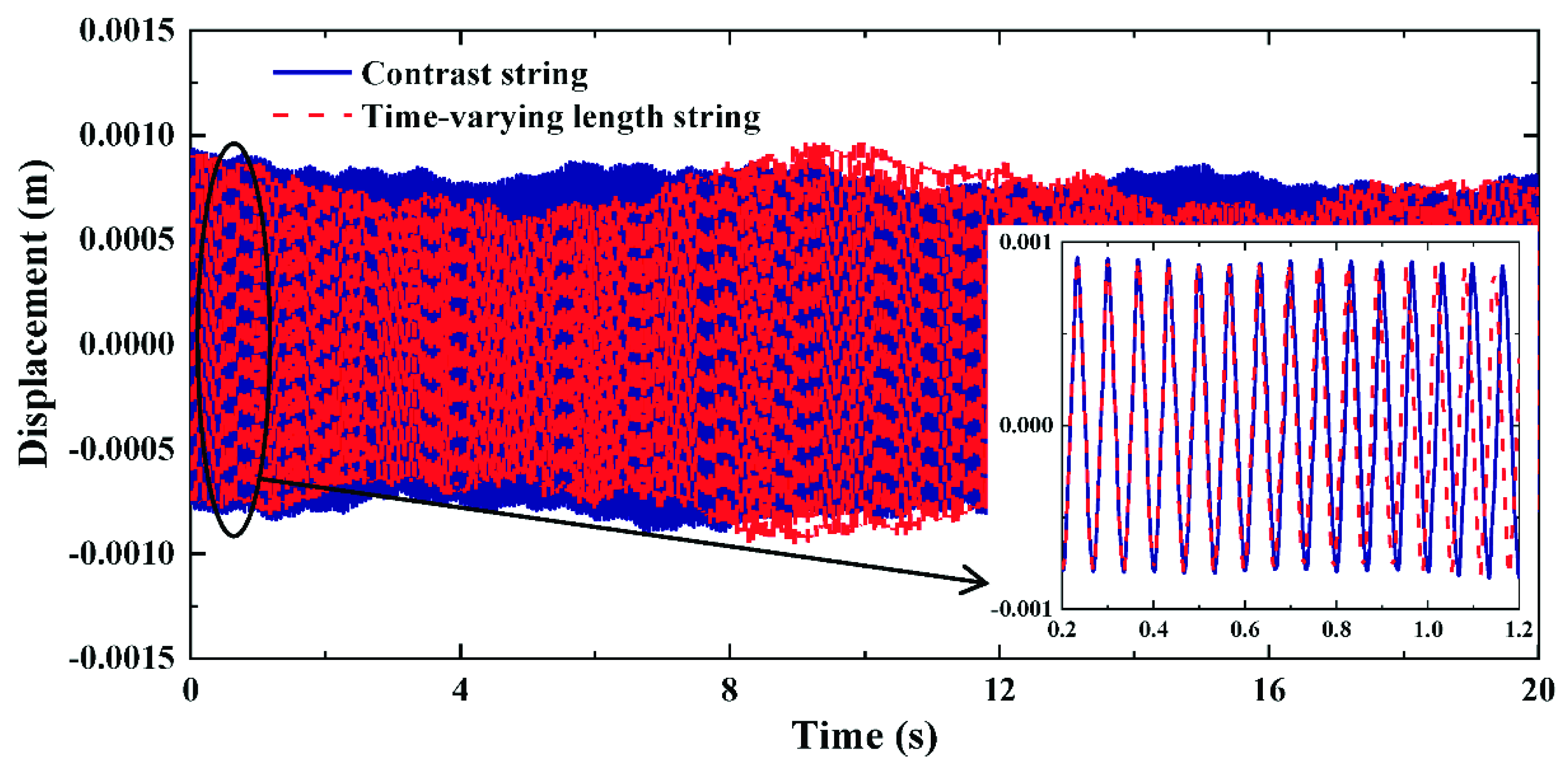
4.2. Forced Vibrations of Time-Varying Length String
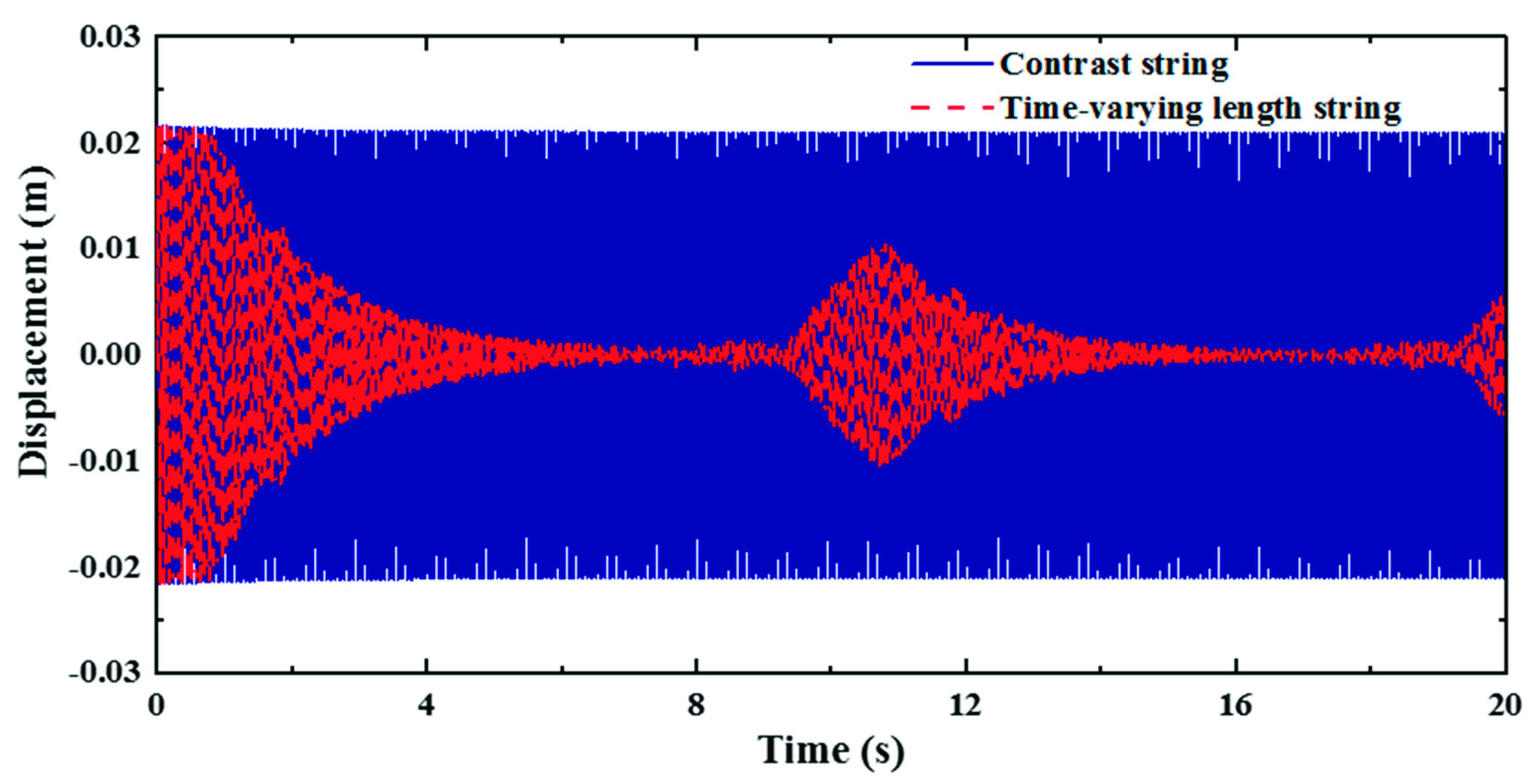
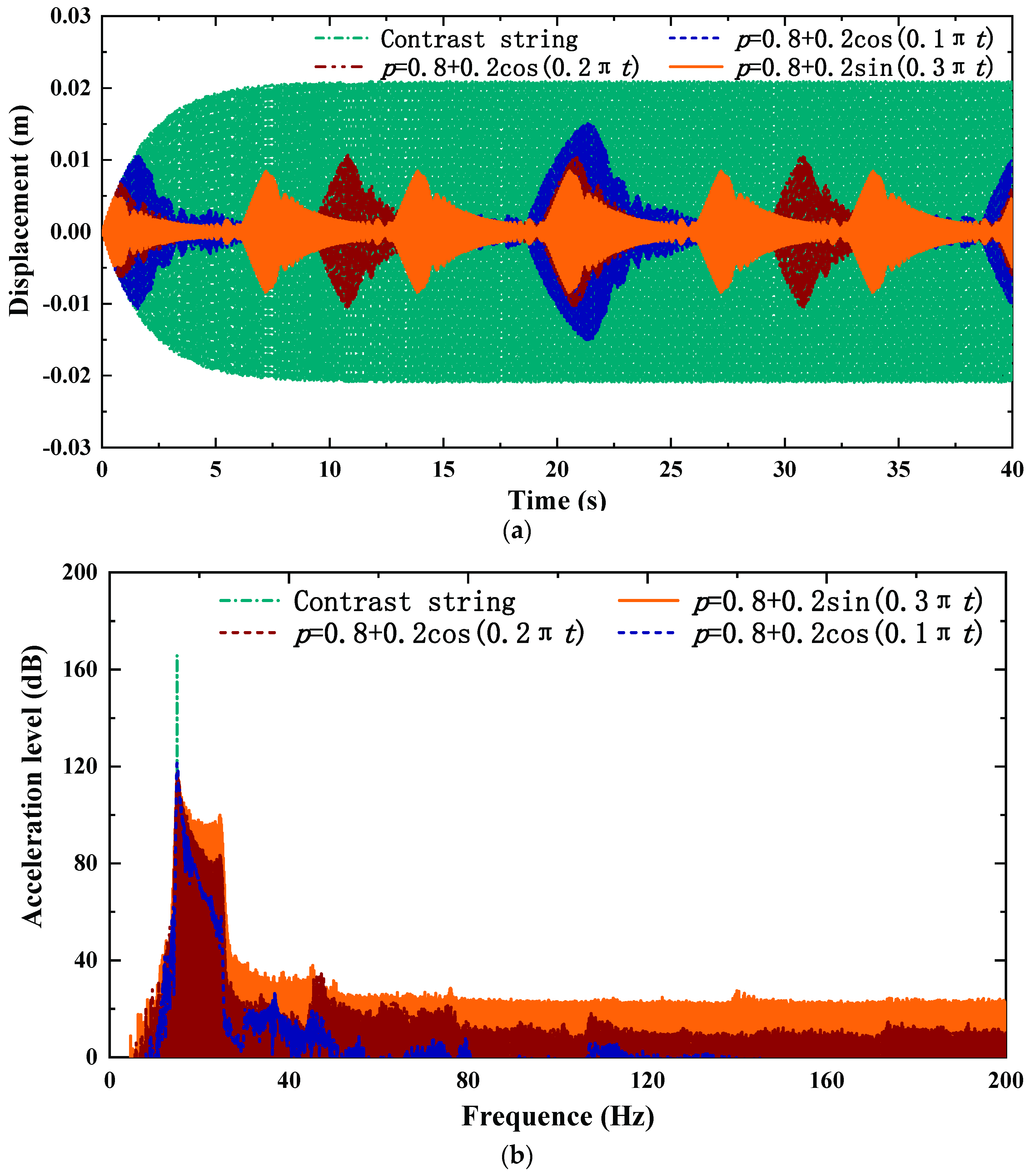
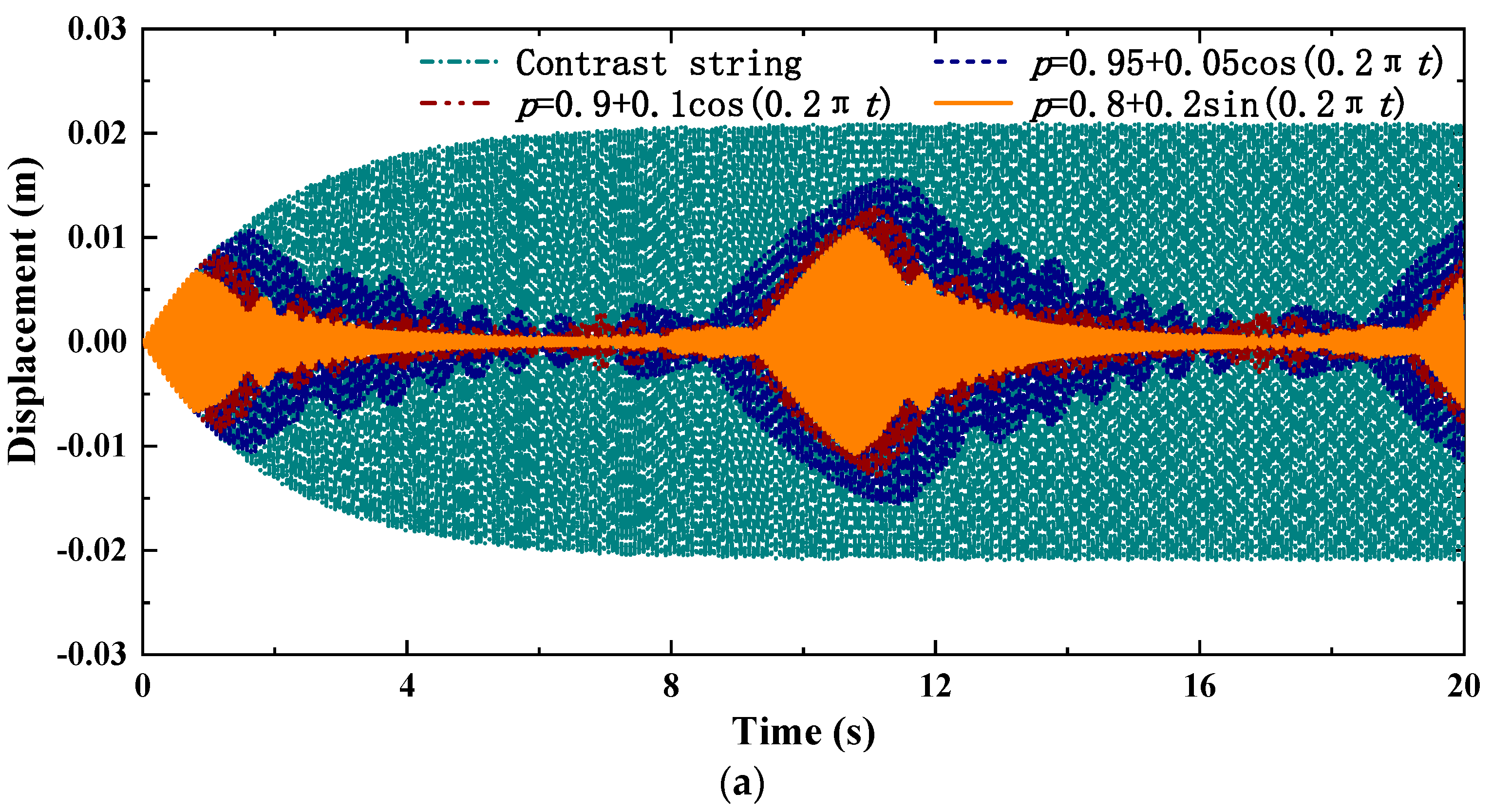
4.3. Influences of the Time-Varying Range and Time-Varying Frequency
5. Conclusions
- (1)
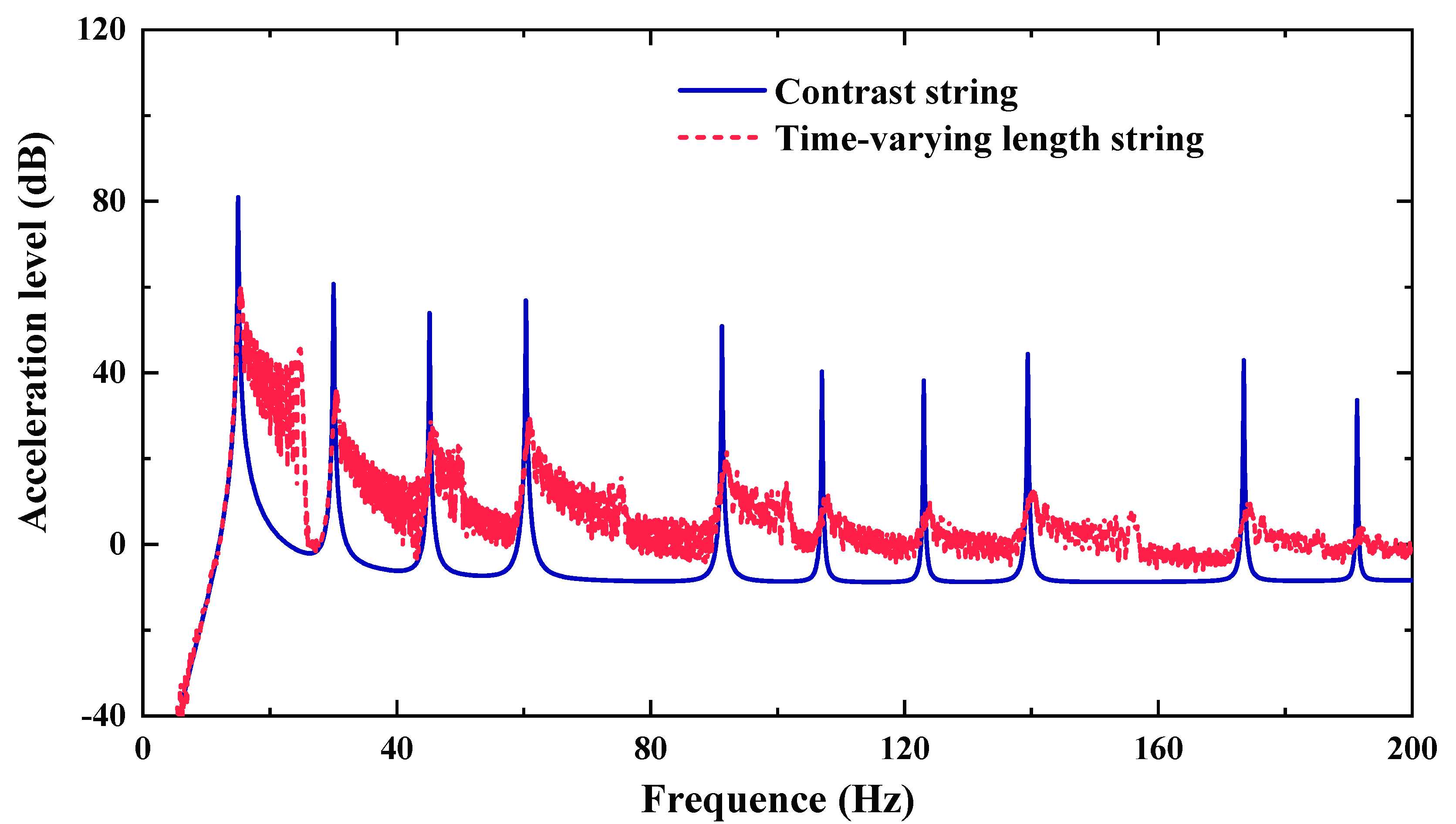
- The time-varying string length method can change the free vibration frequencies. Each modal peak decreases significantly at the same time, while the responses in other frequency bands increase. The reason is that the time-varying modal frequency disperses the peak energy to the nearby frequency band. The damping reduces the peak attenuations, but it can consume the energy dispersed to other frequency bands.
- (2)
- The time-varying string length method causes the change of resonant frequency to suppress the resonance excited by single frequency, which disperses the resonant peak energy to other frequency bands. This method can effectively suppress multi-modal resonances of string at the same time.
- (3)
- The larger the time-varying frequency and time-varying range, the greater the peak attenuations and the better the resonant peak energy dispersion performance.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- He, Y.; Chen, E.; Zhu, W.; Ferguson, N.S.; Wu, Y.; Lu, Y. An analytical wave solution for the vibrational response and energy of an axially translating string in any propagation cycle. Mech. Syst. Signal Process. 2022, 181, 109507. [Google Scholar] [CrossRef]
- Wang, N.; Cao, G.; Yan, L.; Wang, L. Modelling and passive control of flexible guiding hoisting system with time-varying length. Math. Comp. Model. Dyn. 2020, 26, 31–54. [Google Scholar] [CrossRef]
- Sorokin, V.S.; Thomsen, J.J. Vibration suppression for strings with distributed loading using spatial cross-section modulation. J. Sound Vib. 2015, 335, 66–77. [Google Scholar]
- Kakou, P.; Bukhari, M.; Wang, J.; Barry, O. On the vibration suppression of power lines using mobile damping robots. Eng. Struct. 2021, 239, 112312. [Google Scholar] [CrossRef]
- Wijnand, M.; Hélie, T.; Roze, D. Finite-Time Tracking Control of a Nonlinear String to Reference Dynamics. In Proceedings of the 10th European Nonlinear Dynamics Conference, Lyon, France, 17–22 July 2022. [Google Scholar]
- Xing, X.; Yang, H.; Liu, J.; Wang, S. Vibration control of nonlinear three-dimensional length-varying string with input quantization. J. Vib. Control 2020, 26, 1835–1847. [Google Scholar] [CrossRef]
- He, X.; Zhao, Z.; Song, Y. Active control for flexible mechanical systems with mixed deadzone-saturation input nonlinearities and output constraint. J. Franklin. Inst. 2019, 356, 4749–4772. [Google Scholar] [CrossRef]
- Zuo, S.; Liu, Y.; Zhang, K.; Hu, G. Wave boundary control method for vibration suppression of large net structures. Acta Mech. 2019, 230, 3439–3456. [Google Scholar] [CrossRef]
- Wang, J.; Pi, Y. Output feedback vibration control of a string driven by a nonlinear actuator. Appl. Math. Model. 2019, 72, 403–419. [Google Scholar] [CrossRef]
- Eshag, M.A.; Ma, L.; Sun, Y.; Zhang, K. H∞ iterative learning boundary vibration control of uncertain vibrating string with iteration-varying distributed disturbance and boundary disturbance. Appl. Sci. 2019, 9, 5122. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Z.; Xiao, Y.; Li, Z.; Liu, H. Disturbance rejection control for a vibrating string system. Asian J. Control 2019, 21, 1367–1376. [Google Scholar] [CrossRef]
- Ren, Y.; Zhu, P.; Zhao, Z.; Yang, J.; Zou, T. Adaptive fault-tolerant boundary control for a flexible string with unknown dead zone and actuator fault. IEEE Trans. Cybern. 2022, 52, 7084–7093. [Google Scholar] [CrossRef] [PubMed]
- Shi, J.; Wang, X. Boundary Control of Flexible String Systems with Guaranteed Performance. IEEE Access. 2019, 7, 122982–122987. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, F.; Mei, Y.; Wan, W. Adaptive Neural Network Vibration Control for an Output-Tension-Constrained Axially Moving Belt System with Input Nonlinearity. IEEE-ASME Trans. Mech. 2021, 27, 656–665. [Google Scholar] [CrossRef]
- Kelleche, A.; Tatar, N.E. Adaptive boundary stabilization of a nonlinear axially moving string. ZAMM-Z. Angew. Math. Me. 2021, 101, e202000227. [Google Scholar] [CrossRef]
- Xing, X.; Liu, J.; Liu, Z. Dynamic modeling and vibration control of a three-dimensional flexible string with variable length and spatiotemporally varying parameters subject to input constraints. Nonlinear Dyn. 2019, 95, 1395–1413. [Google Scholar] [CrossRef]
- Bao, J.; Zhang, P.; Zhu, C.; Sun, W. Transverse vibration of flexible hoisting rope with time-varying length. J. Mech. Sci. Technol. 2014, 28, 457–466. [Google Scholar] [CrossRef]
- Zhang, Q.; Hou, T.; Zhang, R.-J.; Liu, J. Time-Varying Characteristics of the Longitudinal Vibration of a High-Speed Traction Elevator Lifting System. Int. J. Acoust. Vib. 2020, 25, 153–161. [Google Scholar] [CrossRef]
- Li, S.; Yang, J.; Huang, Q. Independent model space vibration control of an axially moving cantilever beam, part I: Theoretical analysis of approximation. Chin. J. Appl. Mech. 2002, 19, 35–38. [Google Scholar]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Liu, J.; Pan, G. A Nonlinear Vibration Control of a String Using the Method Based on Its Time-Varying Length. Machines 2022, 10, 981. https://doi.org/10.3390/machines10110981
Wang J, Liu J, Pan G. A Nonlinear Vibration Control of a String Using the Method Based on Its Time-Varying Length. Machines. 2022; 10(11):981. https://doi.org/10.3390/machines10110981
Chicago/Turabian StyleWang, Jiahui, Jing Liu, and Guang Pan. 2022. "A Nonlinear Vibration Control of a String Using the Method Based on Its Time-Varying Length" Machines 10, no. 11: 981. https://doi.org/10.3390/machines10110981
APA StyleWang, J., Liu, J., & Pan, G. (2022). A Nonlinear Vibration Control of a String Using the Method Based on Its Time-Varying Length. Machines, 10(11), 981. https://doi.org/10.3390/machines10110981






