Fatigue Life of a Comb Plate Expansion Joint
Abstract
:1. Introduction
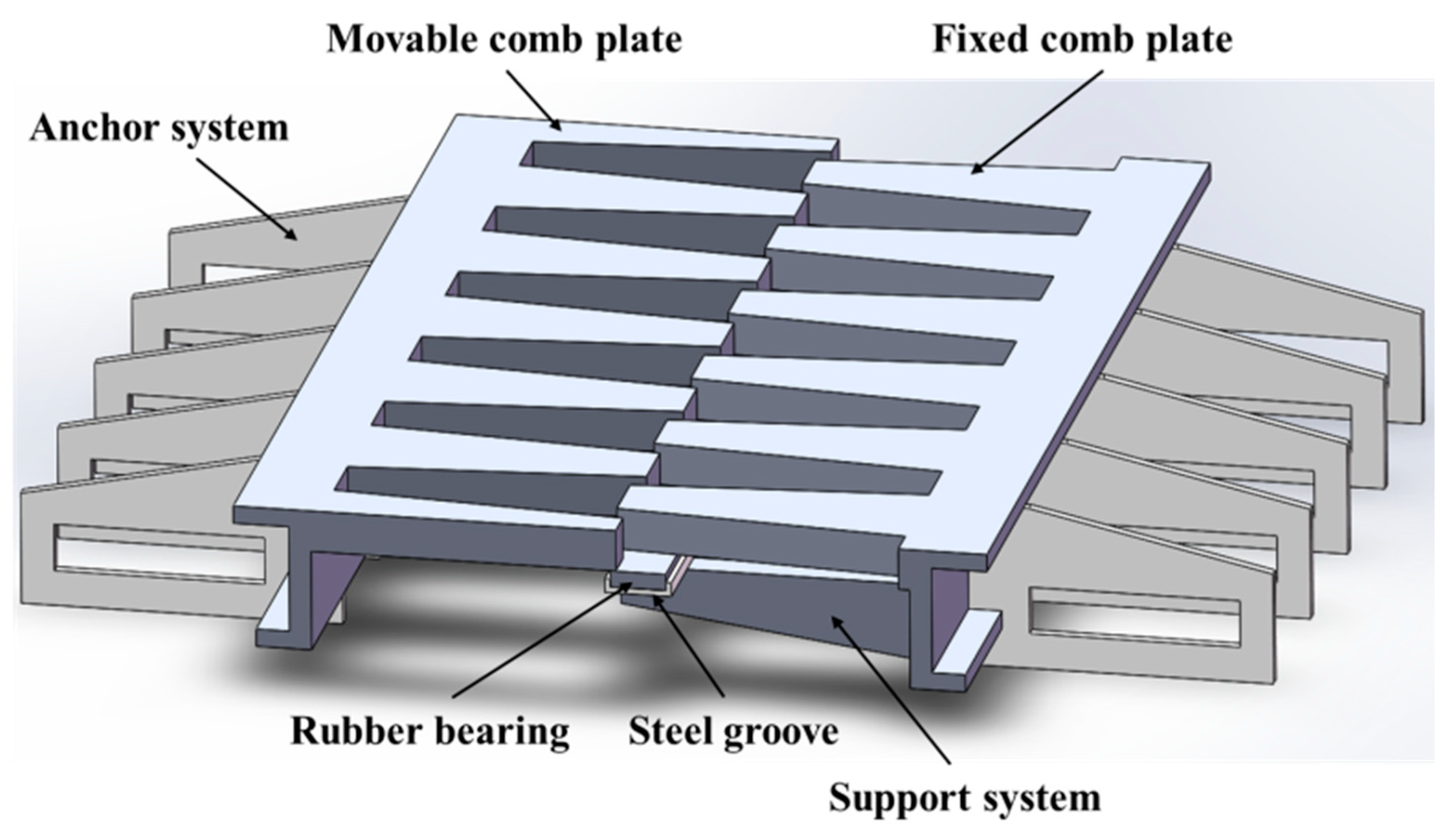
2. Finite Element Analysis of the Expansion Joint
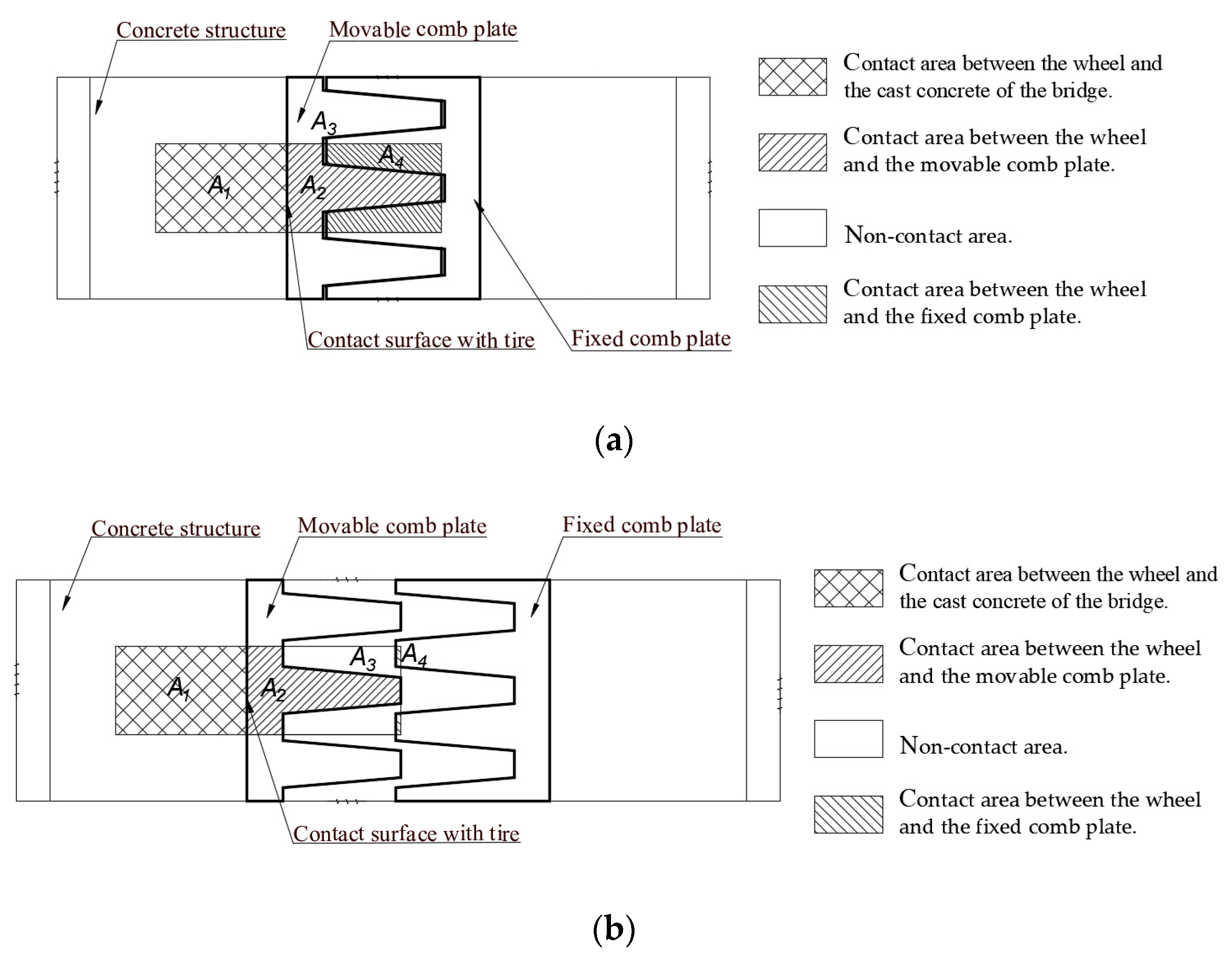
2.1. Analysis of the Working Condition of the Expansion Joint
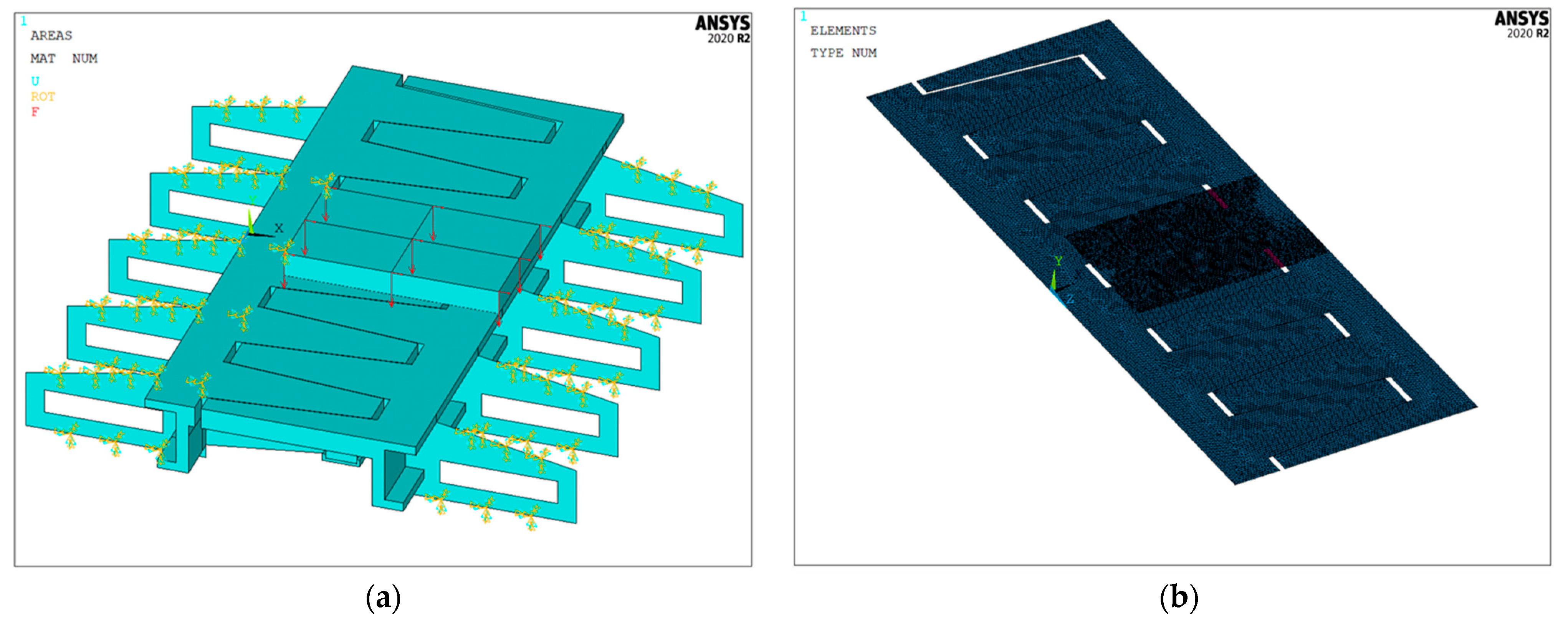
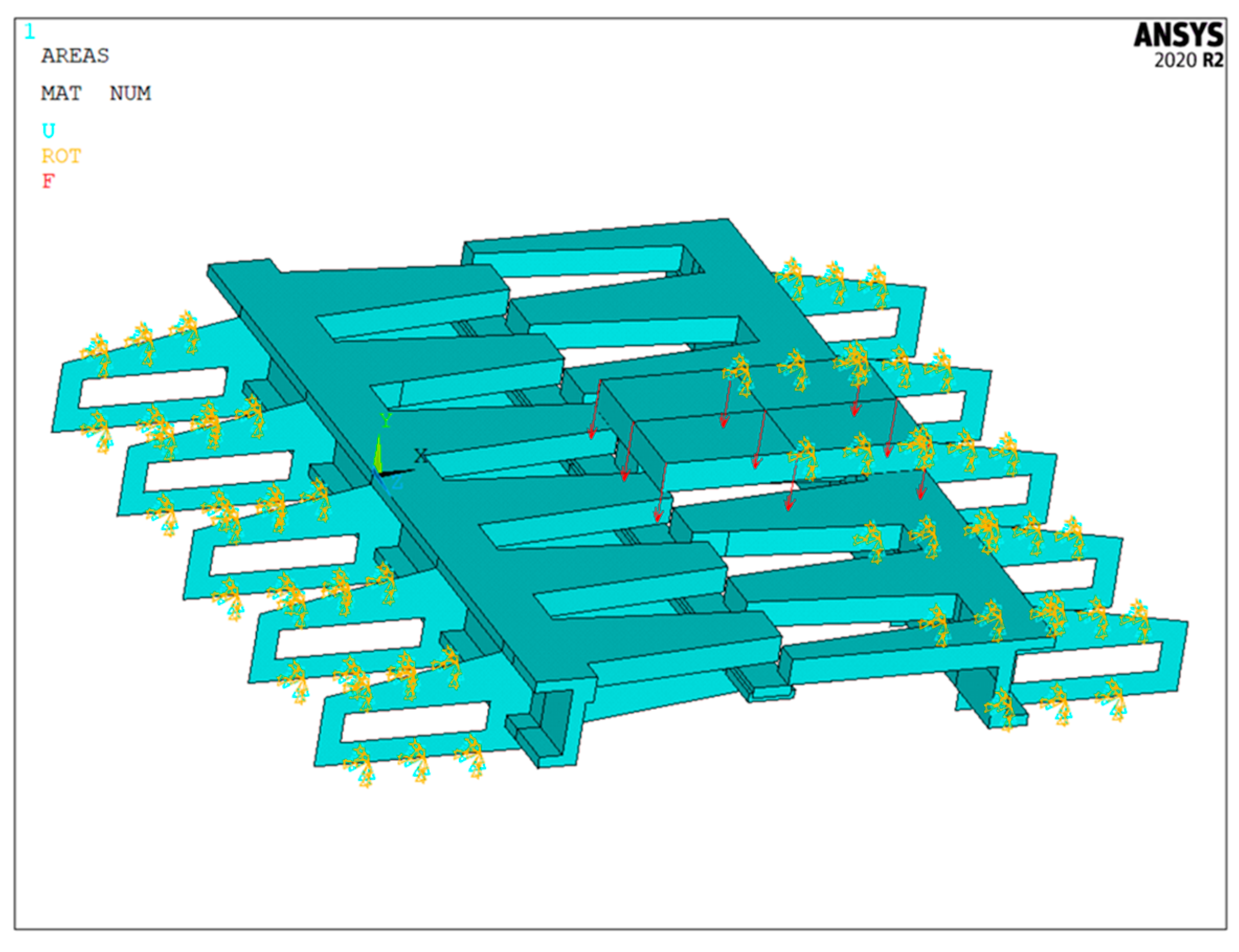
2.2. FEM of the Comb Plate Expansion Joint under Working Conditions I and II
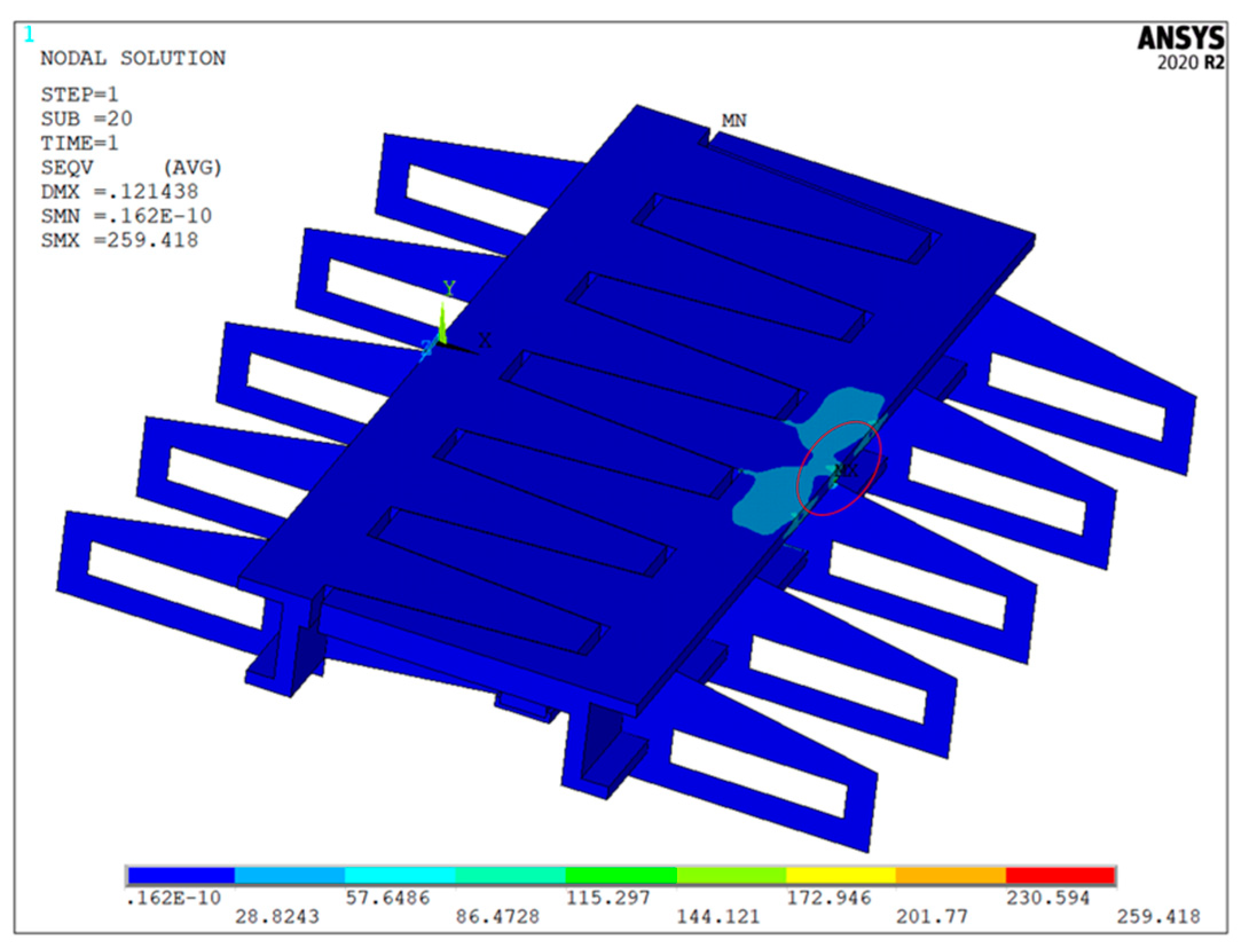
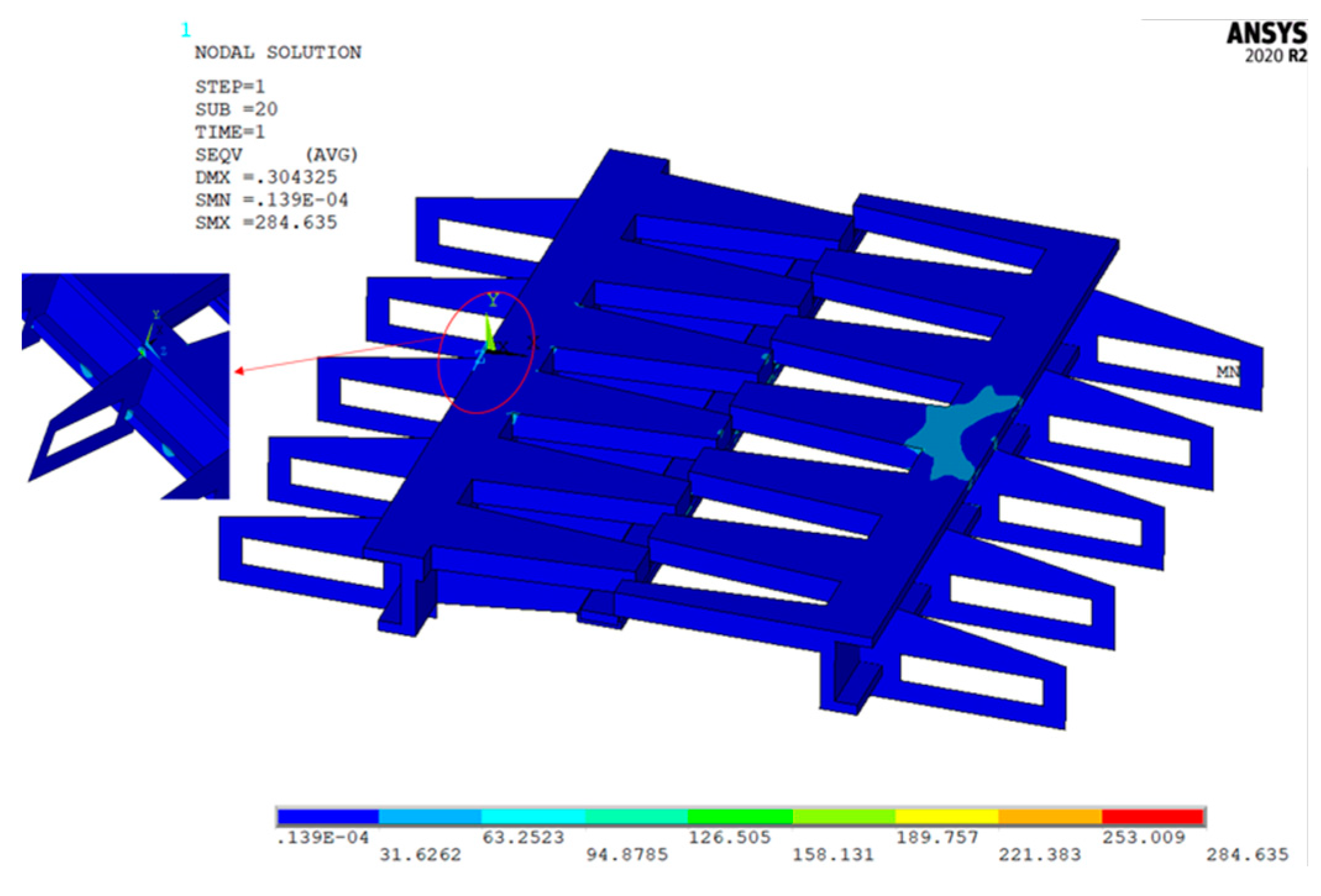
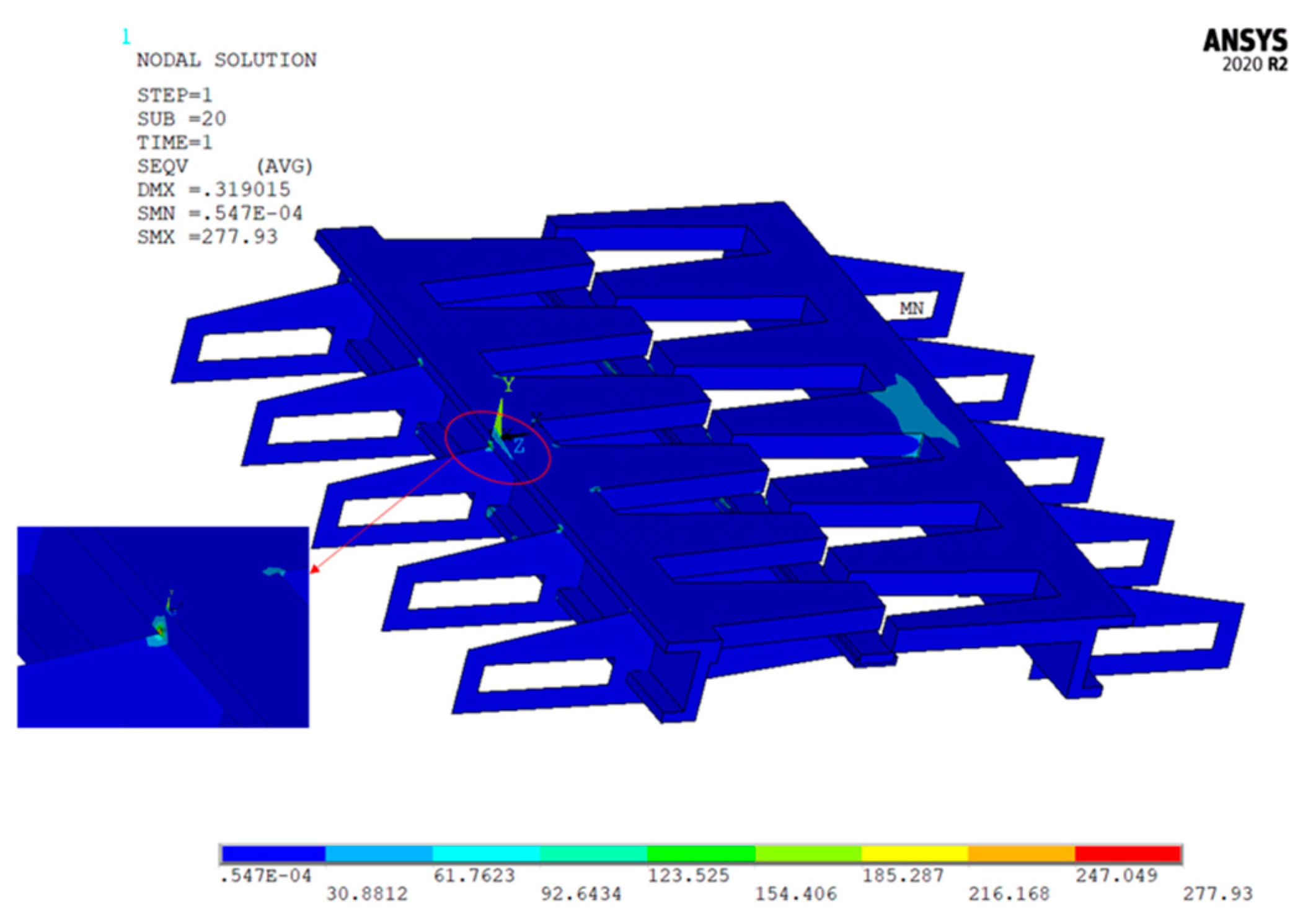
2.2.1. Stress Evaluation under Working Condition I and II by FEM
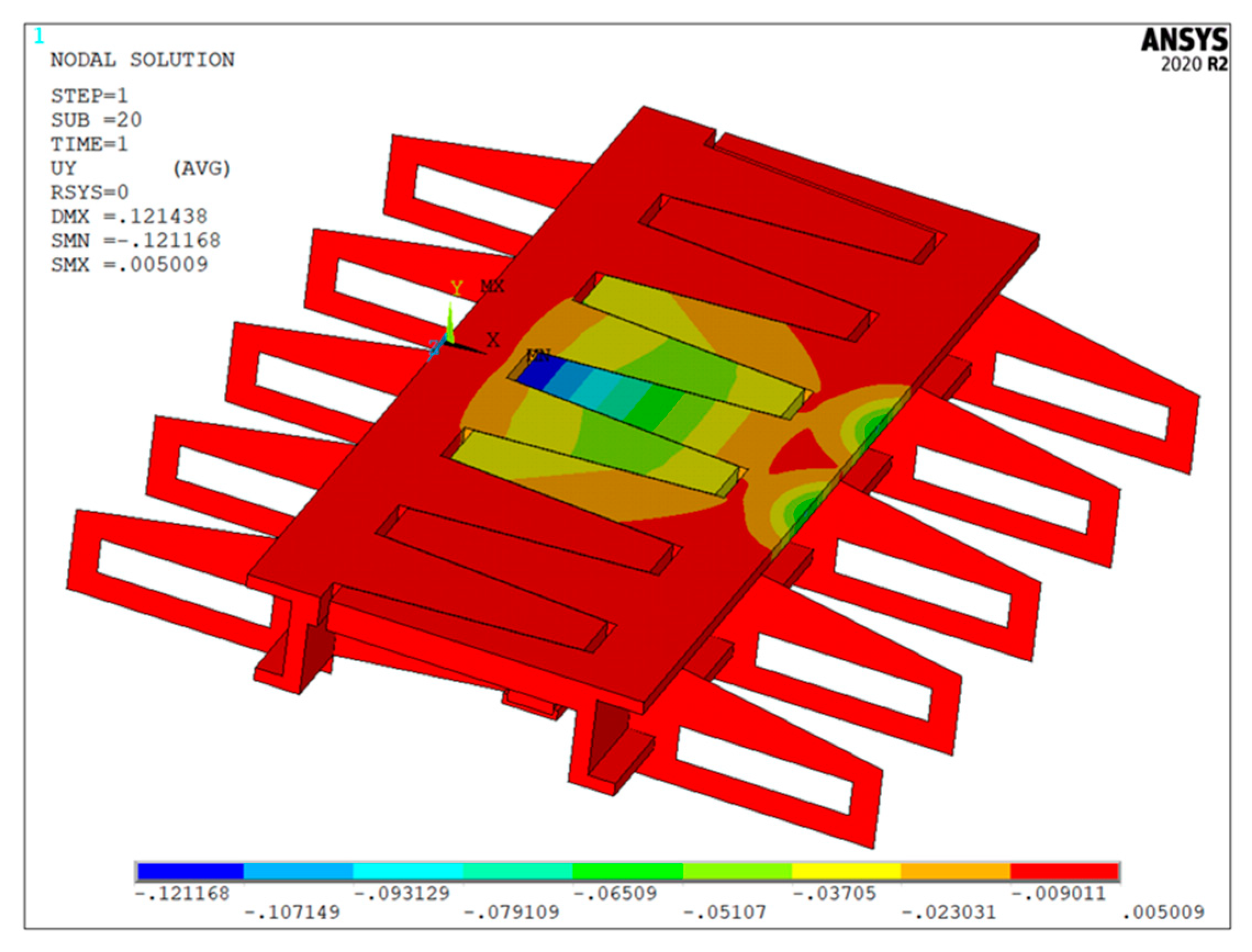
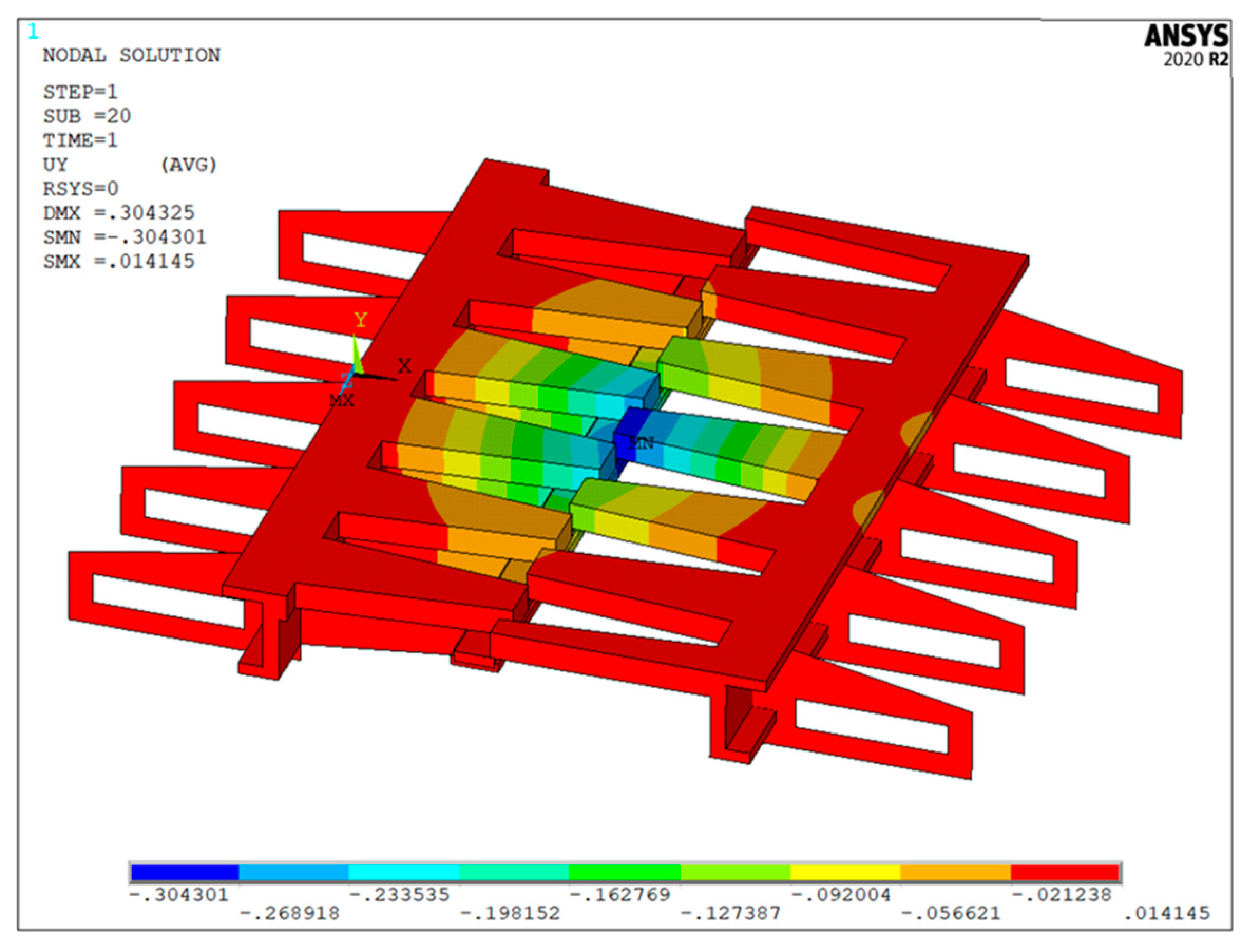
2.2.2. Deformation Evaluation under Working Conditions I and II by FEM
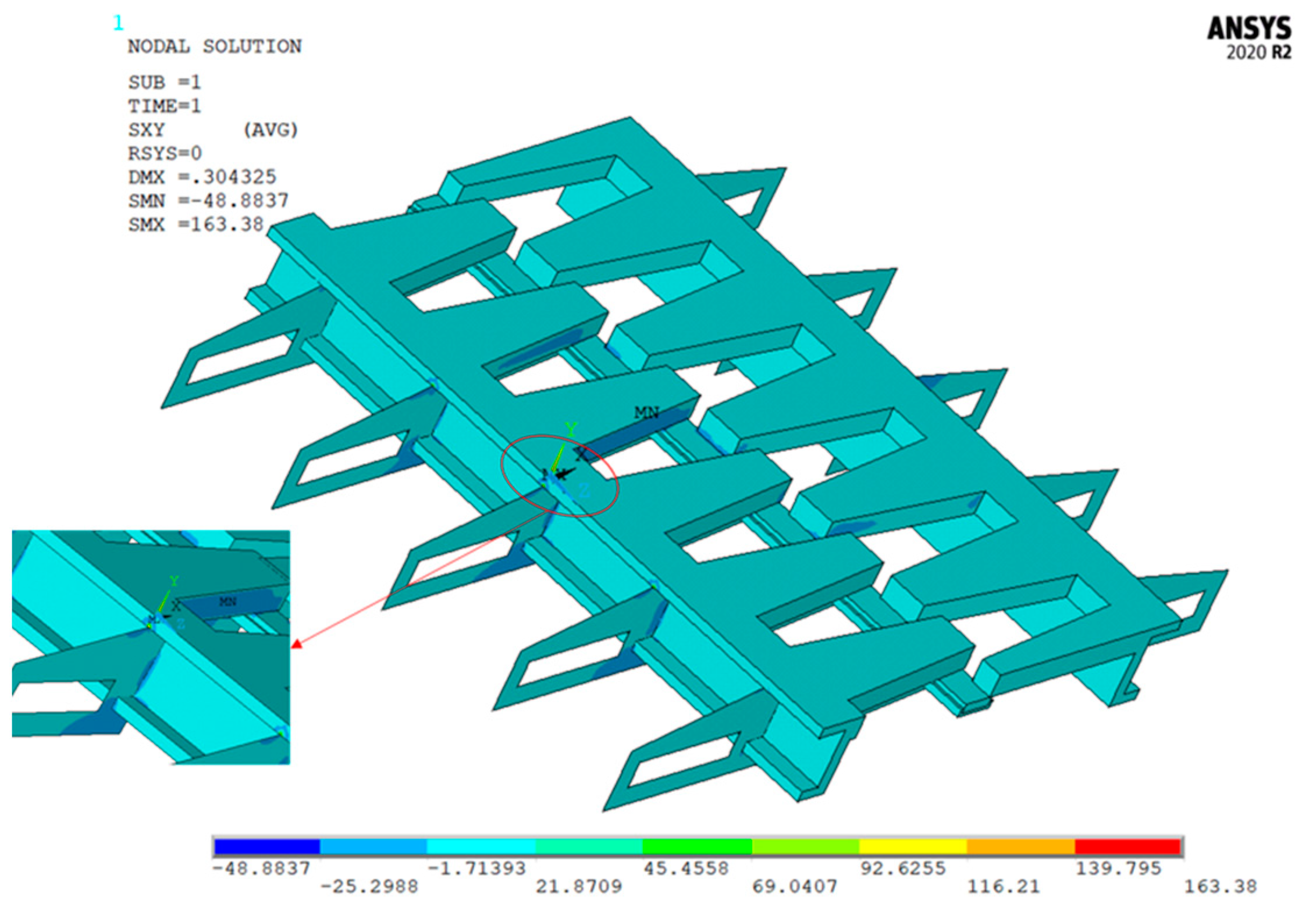
2.2.3. Shear Stress Evaluation under the Working Conditions I and II by FEM
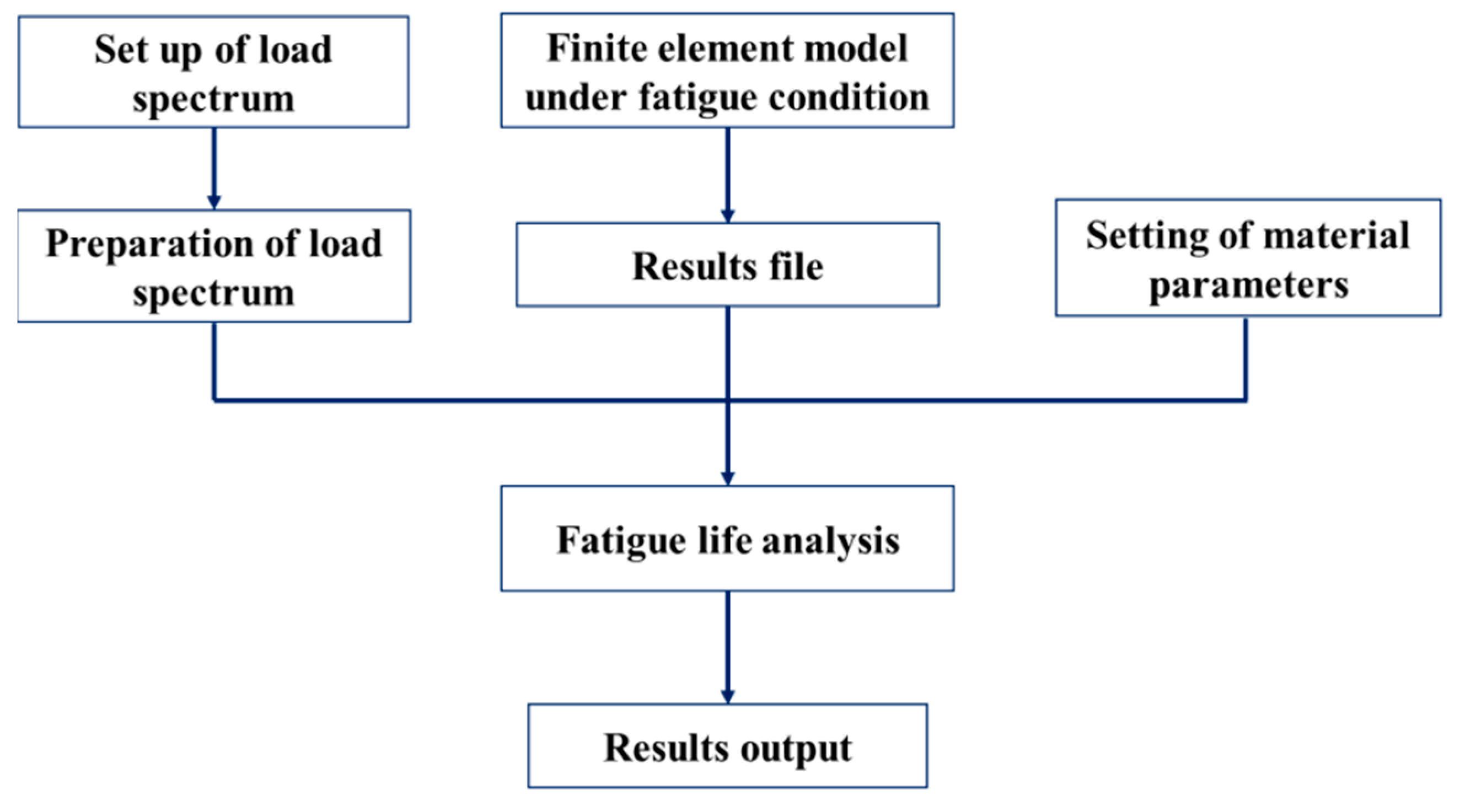
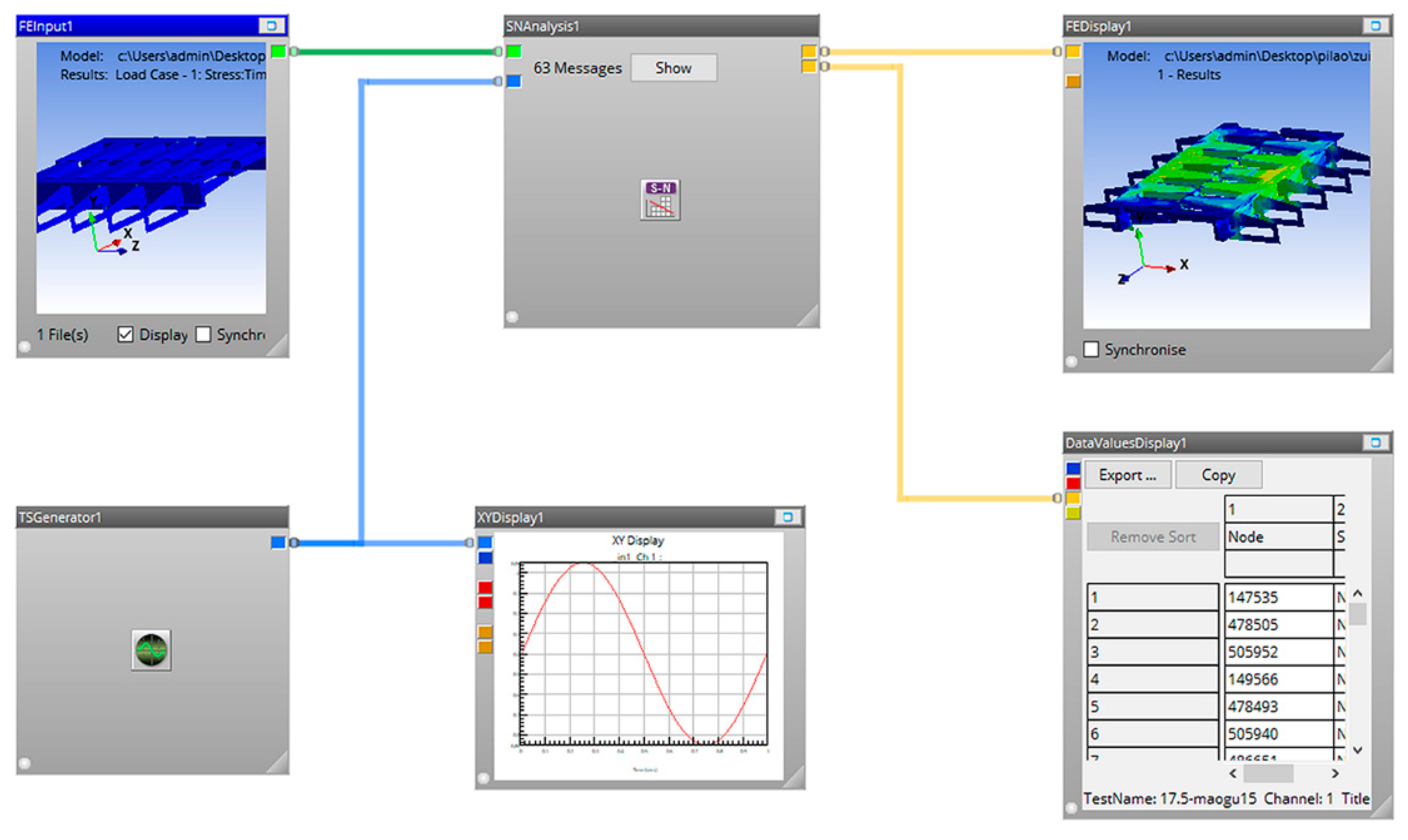
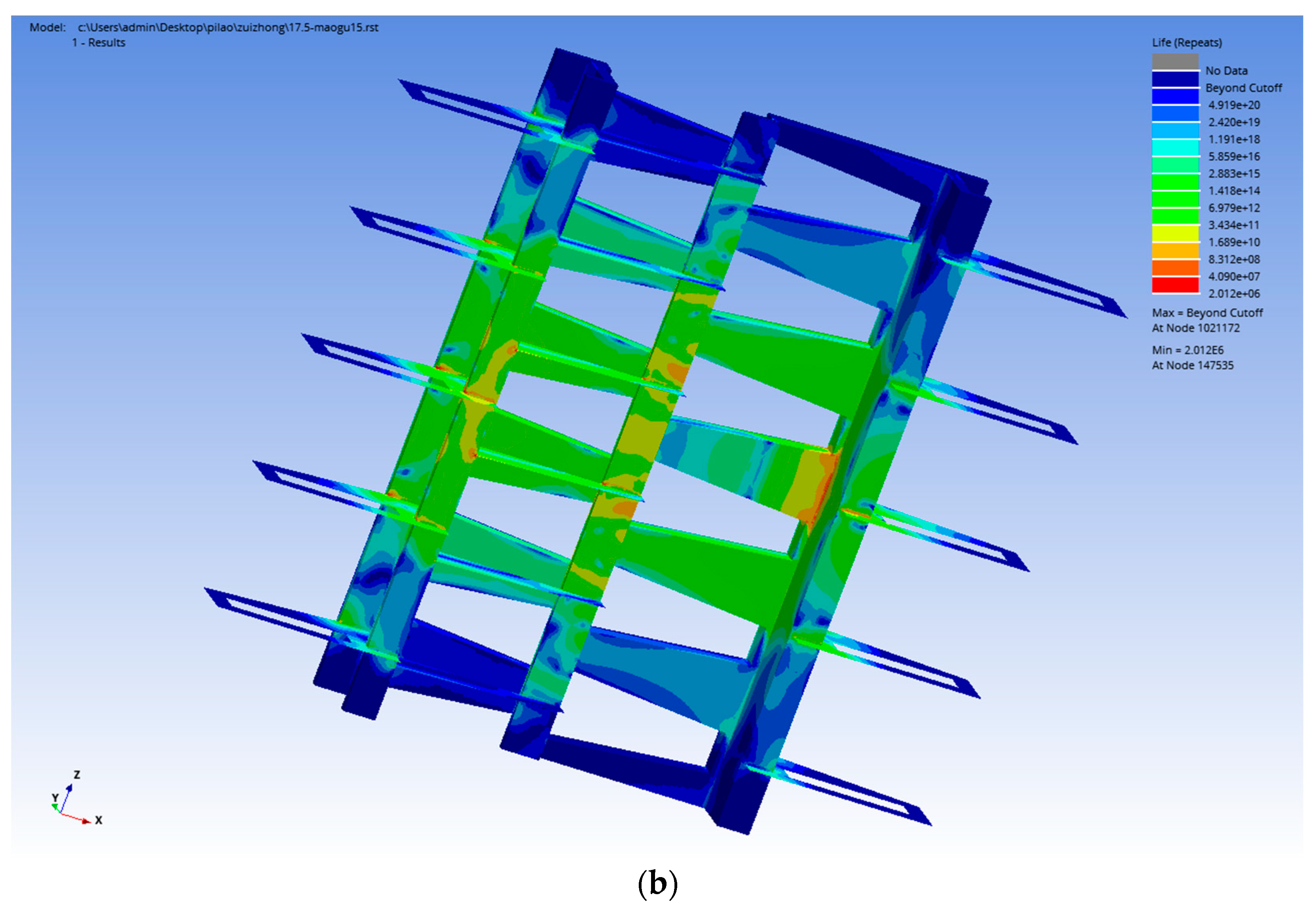
2.3. Fatigue Life Prediction of the Comb Plate Expansion Joint via nCode DesignLife
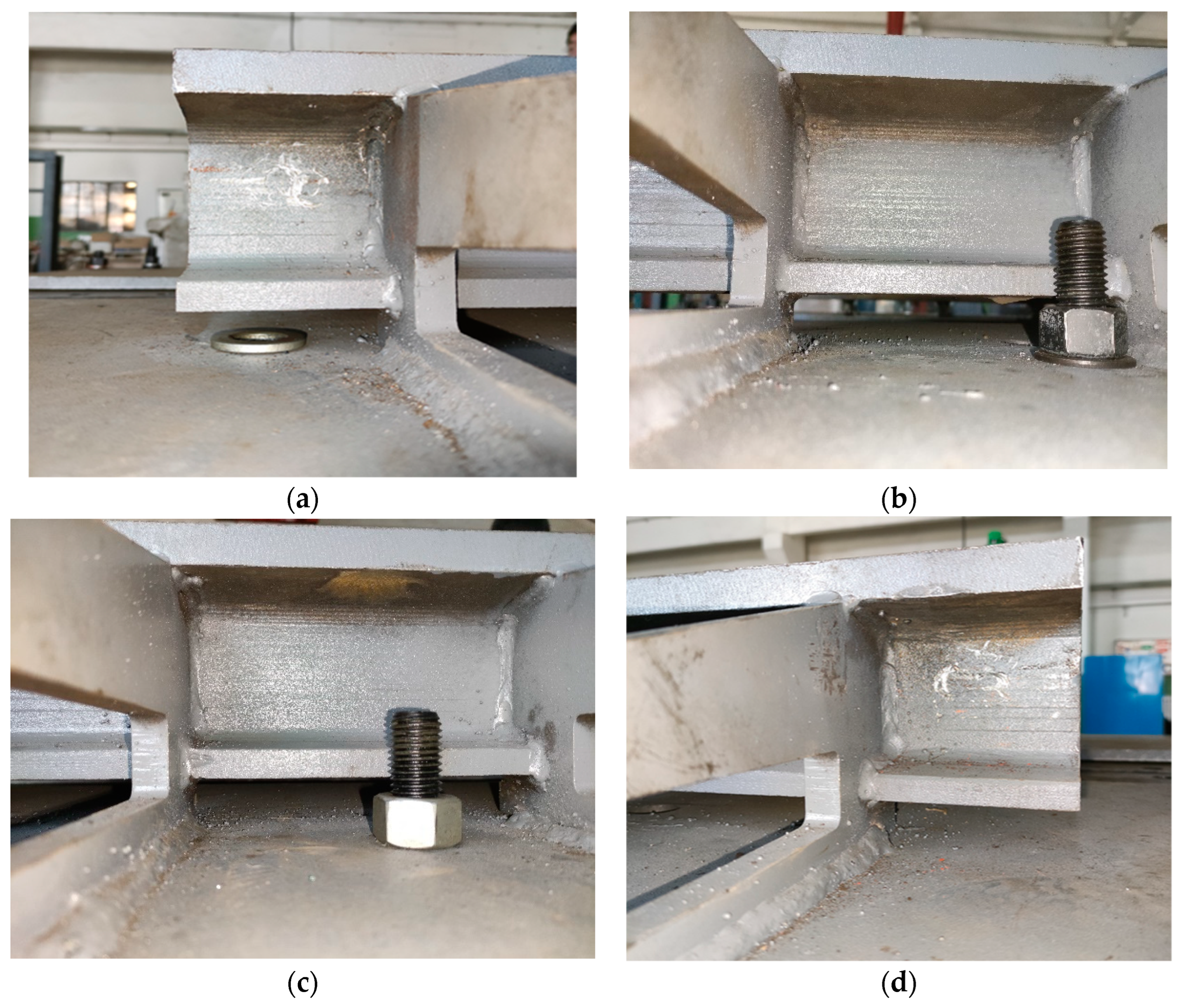
3. Tests for the Comb Plate Expansion Joint
3.1. Load-Bearing Tests
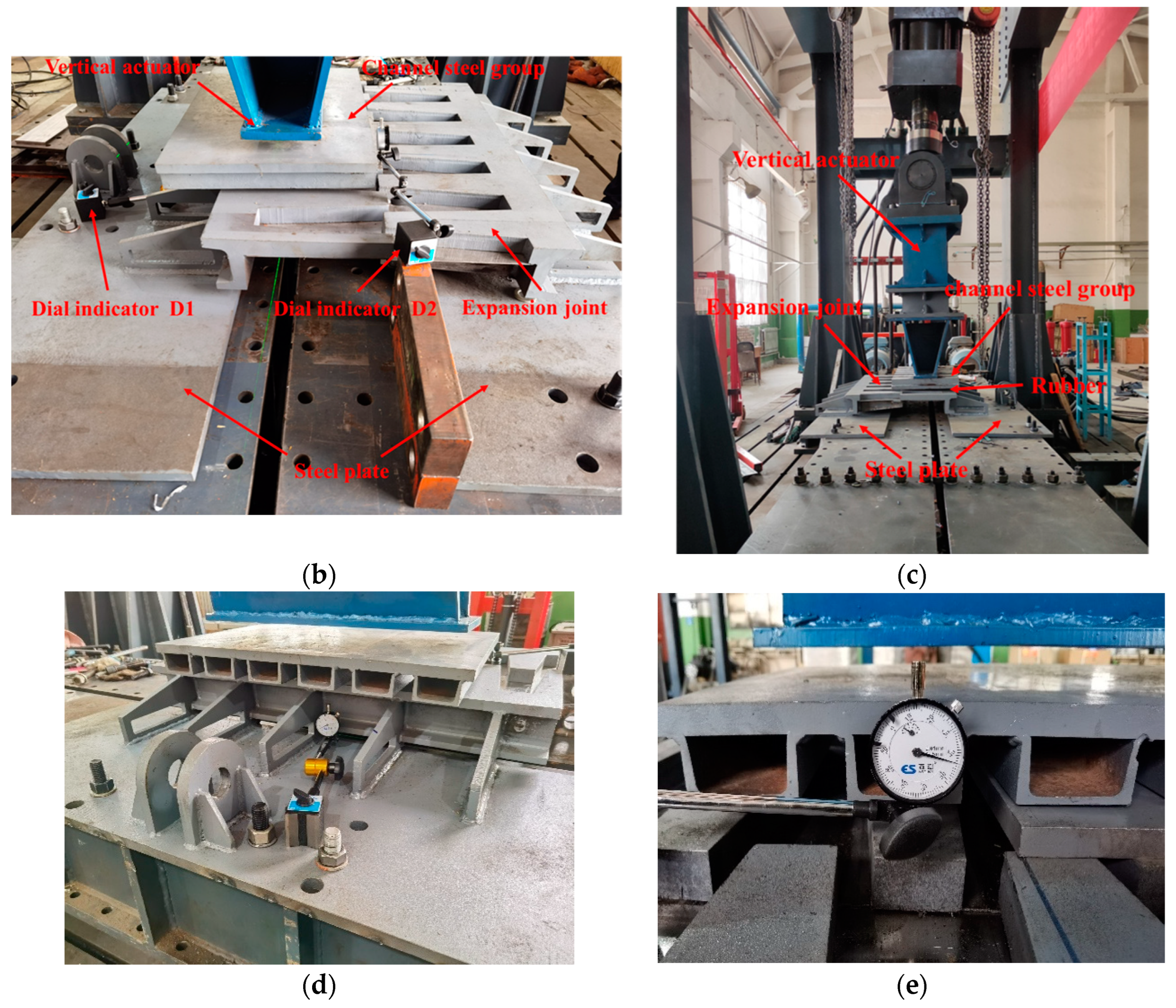
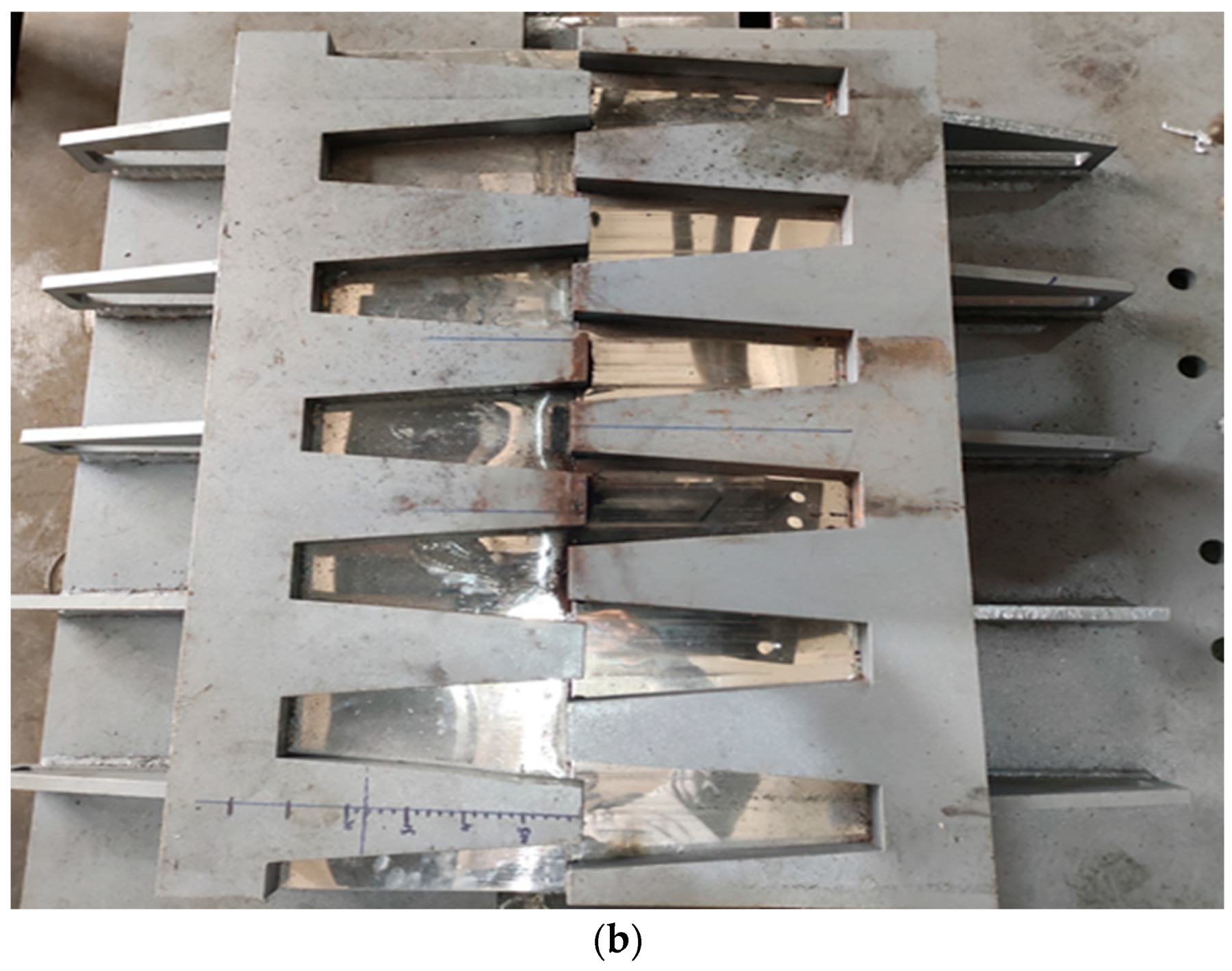
3.2. Bench Fatigue Test for the Full-Scale Specimens
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chang, L.-M.; Lee, Y.-J. Evaluation of Performance of Bridge Deck Expansion Joints. J. Perform. Constr. Facil. 2002, 16, 3–9. [Google Scholar] [CrossRef]
- Lima, J.M.; de Brito, J. Management system for expansion joints of road bridges. Struct. Infrastruct. Eng. 2008, 6, 703–714. [Google Scholar] [CrossRef]
- Okuda, M.; Yumiyama, S.; Ikeda, H. Reinforcement of expansion joint on Akashi Kaikyo Bridge. In Proceedings of the JSCE 59th Annual Meeting, Japan Society of Civil Engineers, Tokyo, Japan, 20–22 October 2004. [Google Scholar]
- Sun, Z.; Zhang, Y. Failure Mechanism of Expansion Joints in a Suspension Bridge. J. Bridge Eng. 2016, 21, 05016005. [Google Scholar] [CrossRef]
- Guo, T.; Liu, J.; Zhang, Y.; Pan, S. Displacement Monitoring and Analysis of Expansion Joints of Long-Span Steel Bridges with Viscous Dampers. J. Bridge Eng. 2015, 20, 04014099. [Google Scholar] [CrossRef]
- Coelho, B.Z.; Vervuurt, A.; Peelen, W.; Leendertz, J. Dynamics of modular expansion joints: The Martinus Nijhoff Bridge. Eng. Struct. 2013, 48, 144–154. [Google Scholar] [CrossRef]
- Lima, J.M.; de Brito, J. Inspection survey of 150 expansion joints in road bridges. Eng. Struct. 2009, 31, 1077–1084. [Google Scholar] [CrossRef]
- Crocetti, R.; Edlund, B. Fatigue Performance of Modular Bridge Expansion Joints. J. Perform. Constr. Facil. 2003, 17, 167–176. [Google Scholar] [CrossRef]
- Wang, L.; Wang, Q.; Si, B. Experimental Research on fatigue testing of modular bridge expansion joint. China Civ. Eng. J. 2004, 37, 44–49. [Google Scholar]
- Artmont, F.A.; Roy, S. Evaluation of Bolted Single Support Bar Modular Bridge Joint Systems for Infinite Fatigue Life under Simulated Vehicular Loading. In Proceedings of the Structural Engineering: Providing Solutions to Global Challenges, Geneva, Switzerland, 23–25 September 2015. [Google Scholar] [CrossRef]
- Stamatopoulos, G.N. Fatigue life of the bolted yoke connection in single support beam (SSB) modular bridge expansion joints. Int. J. Steel Struct. 2017, 17, 723–738. [Google Scholar] [CrossRef]
- Zhang, T.; Zhong, X.; Yu, C. Dynamic characteristic analysis for Maurer bridge expansion joints. Chin. J. Appl. Mech. 2019, 36, 847–854. [Google Scholar]
- Chaallal, O.; Sieprawski, G.; Guizani, L. Fatigue performance of modular expansion joints for bridges. Can. J. Civ. Eng. 2006, 33, 921–932. [Google Scholar] [CrossRef]
- Guizani, L.; Bonnell, W.; Chaallal, O. Fatigue Testing and Performance of Welded Single-Support Bar Modular Bridge Joints. J. Bridge Eng. 2015, 20, 04014079. [Google Scholar] [CrossRef]
- Ma, Y.; Peng, A.; Wang, L.; Zhang, C.; Li, J.; Zhang, J. Fatigue performance of an innovative shallow-buried modular bridge expansion joint. Eng. Struct. 2020, 221, 111107. [Google Scholar] [CrossRef]
- Moor, G.; Hoffmann, S.; O’Suilleabhain, C. AASHTO Fatigue Testing of Modular Expansion Joints—Setting New Standards. In Sustainable Bridge Structures; Mahmoud, K., Ed.; CRC Press: Boca Raton, FL, USA, 2015; pp. 155–160. ISBN 978-0-429-22579-6. [Google Scholar]
- Roeder, C.W.; Hildahl, M.; Lund, J.A.V. Fatigue Cracking in Modular Bridge Expansion Joints. Transportation Research Record 1994. Available online: https://trid.trb.org/view/425266 (accessed on 1 March 2023).
- JT/T 327-2016; General Technical Requirements of Expansion and Contraction Installation for Highway Bridge. China Communications Press: Beijing, China, 2016.
- JTG D60-2015; General Specifications for Design of Highway Bridges and Culverts. China Communications Press: Beijing, China, 2015.
- Lu, L.; Yu, Y.; Zeng, G. Comparison and Analysis of Durability Life of Roller and Sliding Bearings Based on nCode Design-Life. J. Mech. Transm. 2017, 41, 114–119. [Google Scholar]
- Zhang, G.-X.; Niu, G.-G.; Guo, S.-S. Analysis of Fatigue Life of a Torsion Beam Based on nCode Design-Life. IOP Conf. Ser. Mater. Sci. Eng. 2018, 423, 012034. [Google Scholar] [CrossRef]
- Wu, N.; Wang, Y.; Wei, M. Fatigue Life Analysis of Driving Shaft of Conveyor System Based on nCode Design-Life. Int. Core J. Eng. 2019, 5, 174–179. [Google Scholar] [CrossRef]
- Lin, C.; Li, P.; Liu, W. Analysis and calculation of bending fatigue life of curveface gear. J. Braz. Soc. Mech. Sci. Eng. 2019, 42, 3. [Google Scholar] [CrossRef]
- Luo, H.; Li, Z.; Xiong, Q.; Jiang, A. Study on the wind-induced fatigue of heliostat based on the joint distribution of wind speed and direction. Sol. Energy 2020, 207, 668–682. [Google Scholar] [CrossRef]











| Material | Grade | Young’s Modulus (MPa) | Density (kg/m3) | Poisson Ratio | Yield Stress (MPa) | Permissible Stress (MPa) | Permissible Shear Stress (MPa) |
|---|---|---|---|---|---|---|---|
| Steel | Q355 | 2.06 × 105 | 7850 | 0.3 | 355 | 236 | 204.97 |
| Rubber | Neoprene | 4 × 103 | 1300 | 0.47 | / | / | / |
| Working Condition | Comb Plate | Rubber Bearing | Steel Groove | Anchor System | Support System | |
|---|---|---|---|---|---|---|
| Maximum Stress (MPa) | I | 259.42 | 30.50 | 47.97 | 93.47 | 9.28 |
| II | 284.64 | 20.69 | 100.45 | 113.28 | 42.48 |
| Components | Comb Plate | Rubber Bearing | Steel Groove | Anchor System | Support System |
|---|---|---|---|---|---|
| Vertical deformation (mm) | 0.121 | 0.036 | 0.034 | 0.007 | 0.034 |
| Components | Comb Plate | Rubber Bearing | Steel Groove | Anchor System | Support System |
|---|---|---|---|---|---|
| Vertical deformation (mm) | 0.304 | 0.300 | 0.300 | 0.007 | 0.240 |
| Working Condition | Comb Plate | Rubber Bearing | Steel Groove | Anchor System | Support System | |
|---|---|---|---|---|---|---|
| Maximum Stress (MPa) | I | 87.74 | 7.69 | 9.28 | 42.88 | 1.61 |
| II | 163.38 | 7.00 | 48.88 | 31.17 | 14.84 |
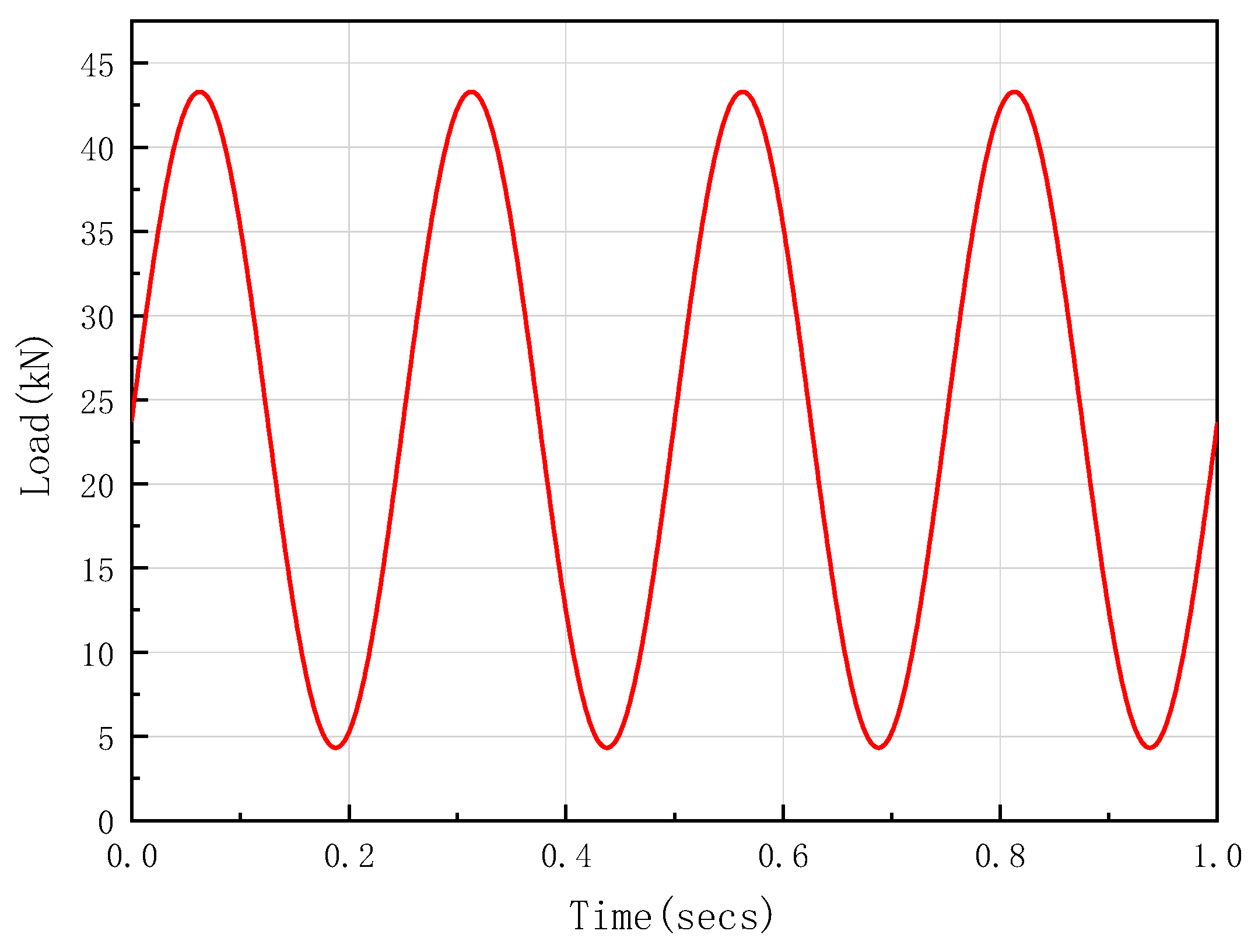
| Maximum Value (kN) | Minimum Value (kN) | Average Value (kN) | Amplitude Value (kN) | Stress Ratio | Frequency (Hz) | |
|---|---|---|---|---|---|---|
| Parameter | r | f | ||||
| Value | 43.30 | 4.33 | 23.815 | 19.485 | 0.1 | 4 |
| Components | Moveable Comb Plate | Fixed Comb Plate | Steel Groove | Anchor System | Support System |
|---|---|---|---|---|---|
| Cycles (times) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Lu, P.; Liu, C.; Ren, W.; Wen, J. Fatigue Life of a Comb Plate Expansion Joint. Machines 2023, 11, 494. https://doi.org/10.3390/machines11040494
Li Y, Lu P, Liu C, Ren W, Wen J. Fatigue Life of a Comb Plate Expansion Joint. Machines. 2023; 11(4):494. https://doi.org/10.3390/machines11040494
Chicago/Turabian StyleLi, Yao, Pengmin Lu, Chenyu Liu, Wei Ren, and Jintang Wen. 2023. "Fatigue Life of a Comb Plate Expansion Joint" Machines 11, no. 4: 494. https://doi.org/10.3390/machines11040494
APA StyleLi, Y., Lu, P., Liu, C., Ren, W., & Wen, J. (2023). Fatigue Life of a Comb Plate Expansion Joint. Machines, 11(4), 494. https://doi.org/10.3390/machines11040494





