A Study on the Running of a Joystick-Type Six-Wheeled Electric Wheelchair When Curb Climbing

Abstract

In Japan, the number of power wheelchair users is increasing as the country becomes an aging society. This trend is expected to continue in the future. Electric wheelchairs currently on the market include (1) bar-handle-type power wheelchairs for older users and (2) joystick-type power wheelchairs that change direction by operating a joystick. When such electric wheelchairs are used outdoors, the problem is curb-climbing at the boundary between the roadway and the sidewalk. It would be difficult for a wheelchair with a small front wheel diameter of 200 mm to overcome a curb height of 50 mm. Therefore, users are forced to take a detour or drive on the street to avoid the curb step. One of the most effective ways to solve this problem is to increase the wheel diameter. However, larger wheels make it more difficult for users to get in and out of the wheelchair. In addition, there are problems such as an increased footprint when turning, which makes the wheelchairs difficult to use on narrow streets. In this paper, using a joystick-type six-wheel electric wheelchair as an example, we examined the mechanism by which an electric wheelchair can overcome curb climbing and consider improvements to the chassis with a method that does not rely on increasing the wheel diameter. As a result, it became possible to overcome a curb of 96 mm in height with a front-wheel diameter of 200 mm.

1. Introduction

The number of power wheelchair users is increasing in Japan due to the declining birth rate and aging population [1,2,3]. The same is likely to be true in other countries with declining birth rates and aging populations [4,5]. Electric wheelchair users in Japan have their own unique circumstances. There is a trend for people voluntarily surrendering their driver’s licenses and giving up driving due to the decline in cognitive and operational functions required for driving as they age [6,7,8,9,10]. This is because serious accidents caused by elderly people are reported daily, and there is social criticism of elderly people driving. There are an increasing number of cases in which people who have relinquished their driver’s license use power wheelchairs as a means of traveling short distances to achieve independence. More active people load their wheelchairs into cars driven by others and use them to reach their destinations. When trying to use a power wheelchair outdoors in this way, the obstacle is the curb where the road meets the sidewalk. Problems that can occur when crossing a curb include 1. The curb itself being not negotiable. 2. The track becoming unstable when going over a curb. 3. Significant shock when encountering curb climbing. These problems cause physical and mental stress to the user. Research has been conducted to solve these problems [11,12,13]. Therefore, in this paper, we use a joystick-type six-wheeled electric wheelchair as an example to study its curb-climbing performance and improve its performance by improving the wheelchair chassis.

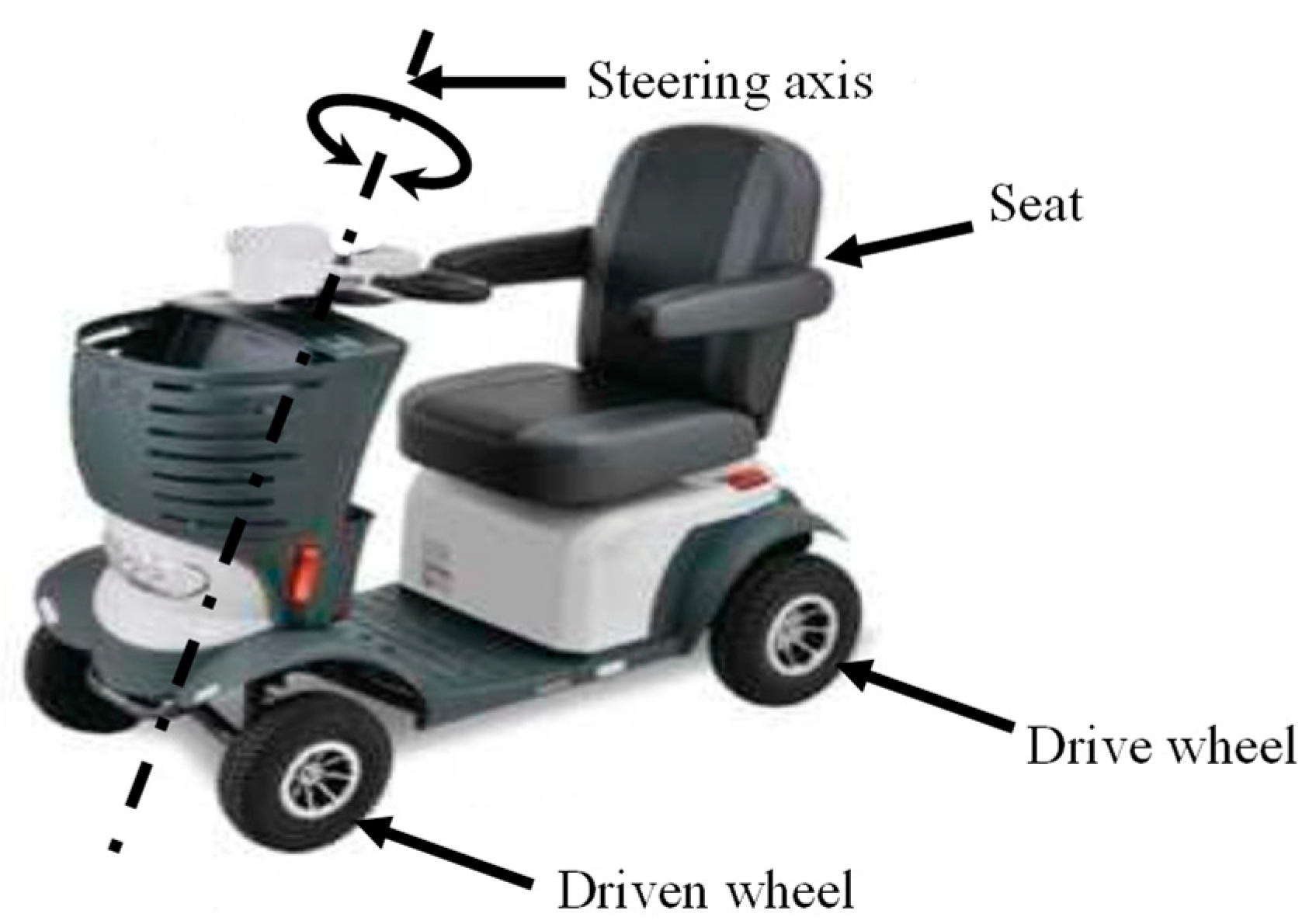
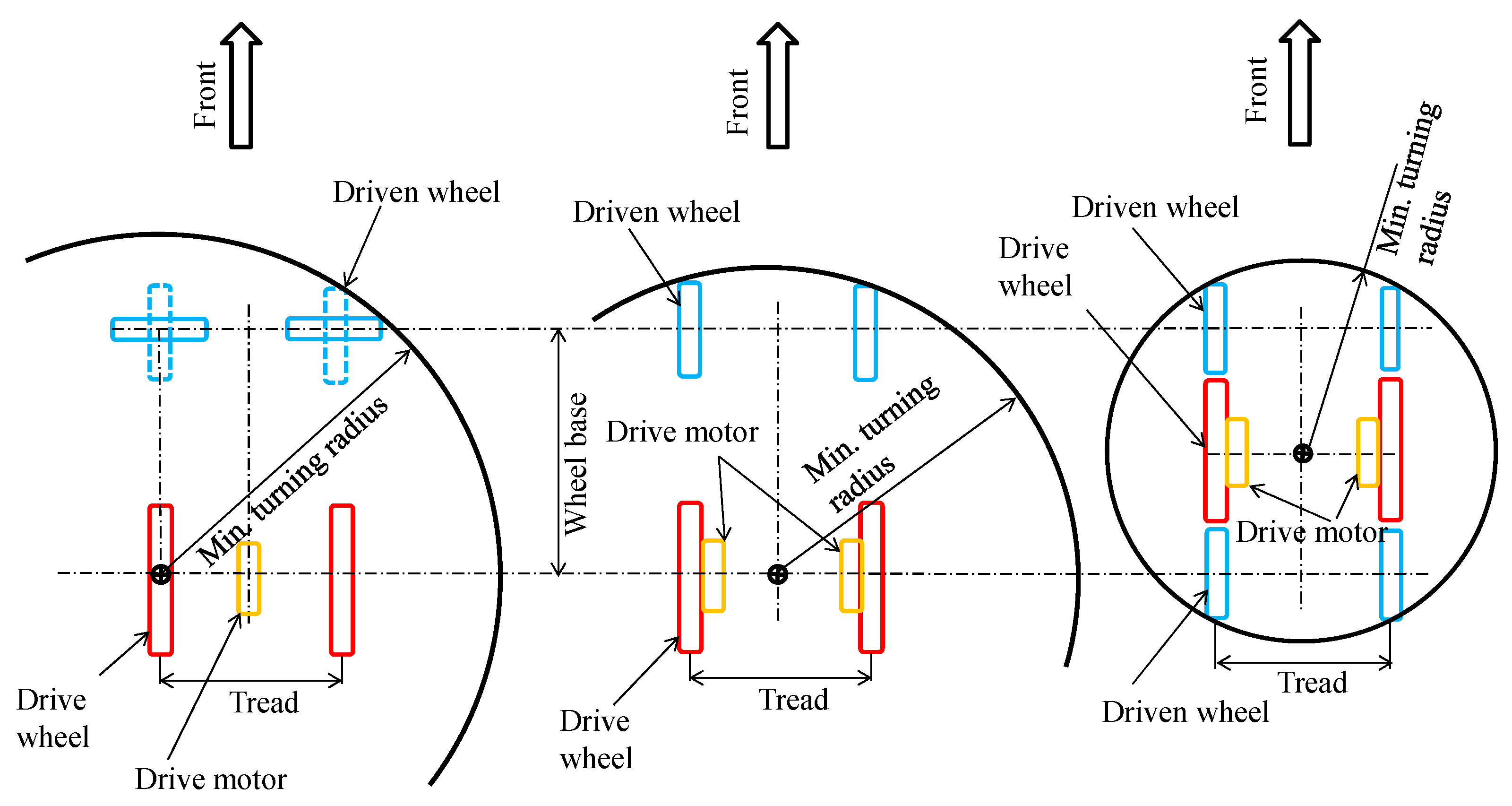
2. Explanation of Electric Wheelchairs

This chapter explains the different types of power wheelchairs available, focusing on the joystick-type six-wheel power wheelchair.

Figure 1 shows a senior car that is turned using a handlebar. The rear wheels are the drive wheels, and the power from a drive motor is sent to a differential, which distributes power to the left and right wheels. The front wheels are the driven wheels, and the toe angle of the front wheels is controlled by a handlebar, allowing the vehicle to turn with the same feeling as steering a car or motorcycle. Figure 2 and Figure 3 show a joystick-type electric wheelchair, which is equipped with two drive motors on the left and right, and turns by operating the joystick to create a difference in the rotation speed of the left and right drive motors. The driven wheels are required to roll in a direction tangential to the turning circle, determined by the difference in rotational speed between the left and right driving wheels. This is accomplished by using omniwheels [14] or casters, as shown in Figure 4 and Figure 5. Omniwheels can rotate not only perpendicular to the wheel axis, like normal wheels, but also in the direction of the wheel axis. There are many small wheels that rotate around an axis perpendicular to the wheel axis and which can roll in any direction.

Figure 3 shows a six-wheel joystick wheelchair. In a six-wheel joystick wheelchair, the left and right center wheels provide the propulsion. In this system, the left and right center wheels can rotate in opposite directions to perform pivot turns, so it can turn in a smaller area than wheelchairs with other systems. This is shown in Figure 6. Figure 6 is a top view showing the minimum turning radius of the handle-type, four-wheel joystick, and six-wheel joystick wheelchairs. All three are drawn with the same overall length and width, but it can be seen that the six-wheel type, which rotates around the center of the vehicle dimensions, can turn in a smaller area.

Many commercially available joystick-type six-wheel electric wheelchairs only have a drive motor on the central wheel in the side view, due to cost considerations. Therefore, it is necessary to apply a large load to the central wheel that provides the braking and driving force. There are various types of joystick-type six-wheel electric wheelchairs, with different suspension methods to meet such purposes. Examples are shown below. Figure 7 shows an example of a chassis with three toggle links. The front wheels and the center wheel are connected by a rocker link, and the left and right rear wheels are also connected by a rocker link. Figure 8 shows an example of a chassis with two rocker links and an H-shaped link suspended by a coil spring.

The transfer of wheel load from the center of gravity to the wheels in the two examples of power wheelchairs above is shown in Figure 9. The path from the center of gravity to the front and center wheels is completely separate from the path from the center of gravity to the rear wheels. Therefore, to increase the wheel load distribution to the center wheels, dimension c is set to be larger than d. Therefore, an electric wheelchair with dimensions c and d set so that c > d will have a wheel load distribution as shown in Figure 10 when driving downhill with a not too large forward tilt angle. In a wheelchair with a dimensional ratio of c > d, as shown in Figure 10, the line drawn vertically from the center of gravity to the ground points forward of the front pivot point P_F. This makes the load on the rear wheel pivot point P_R negative. This causes the rear wheel spring to expand, increasing the forward lean angle of the vehicle and increasing the risk of tipping forward.

This paper investigates the ability to overcome curb climbing, but in joystick-type electric wheelchairs, the contact resistance of the two front wheels with steps, etc., is input with a time lag between the left and right wheels, or there is a difference in the contact resistance of the left and right wheels, causing the vehicle to deflect to the left and right. This causes a sudden change in the yaw angle in a short period of time.

There are two possible causes of this phenomenon. One is a rotational moment about the Z-axis caused by the contact resistance generated when one of the front wheels hits an obstacle. The yaw motion moves to the side with the greater contact resistance. The other is due to the “speed x torque” characteristic of the drive motor that occurs when the front wheel hits an obstacle. The operation of the motor to restore the drive wheel speed, which has been reduced by the contact resistance of the obstacle, creates a torque difference between the left and right drive wheels. Therefore, after passing the obstacle, a yaw motion occurs in the opposite direction to the one mentioned above. These two yaw motions occur as a set in a short period of time, which is uncomfortable for the occupant.

Specific actions to address the above issues and their impact are currently under consideration and will be detailed in the next report. Here are the points under consideration. (1) One possible method is to reduce the impact force when hitting a step by lowering the front and rear support stiffness of the front wheel according to the front and rear input of the front wheel. (2) A possible method is to determine the step from the rotational speed of the engine and the yaw angle of the wheelchair, and to enable control to reduce the difference in rotational speed between the left and right drive wheels.

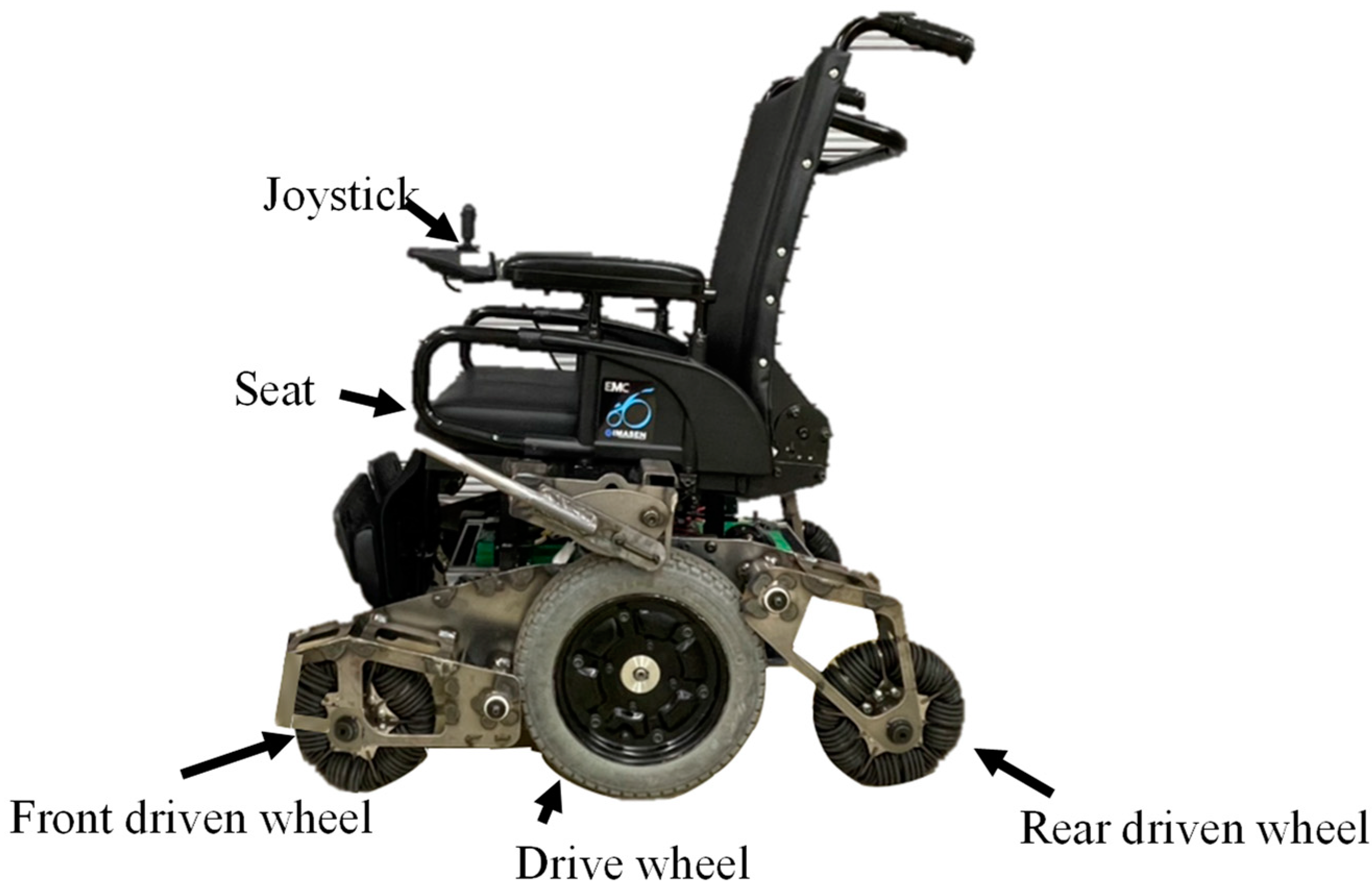
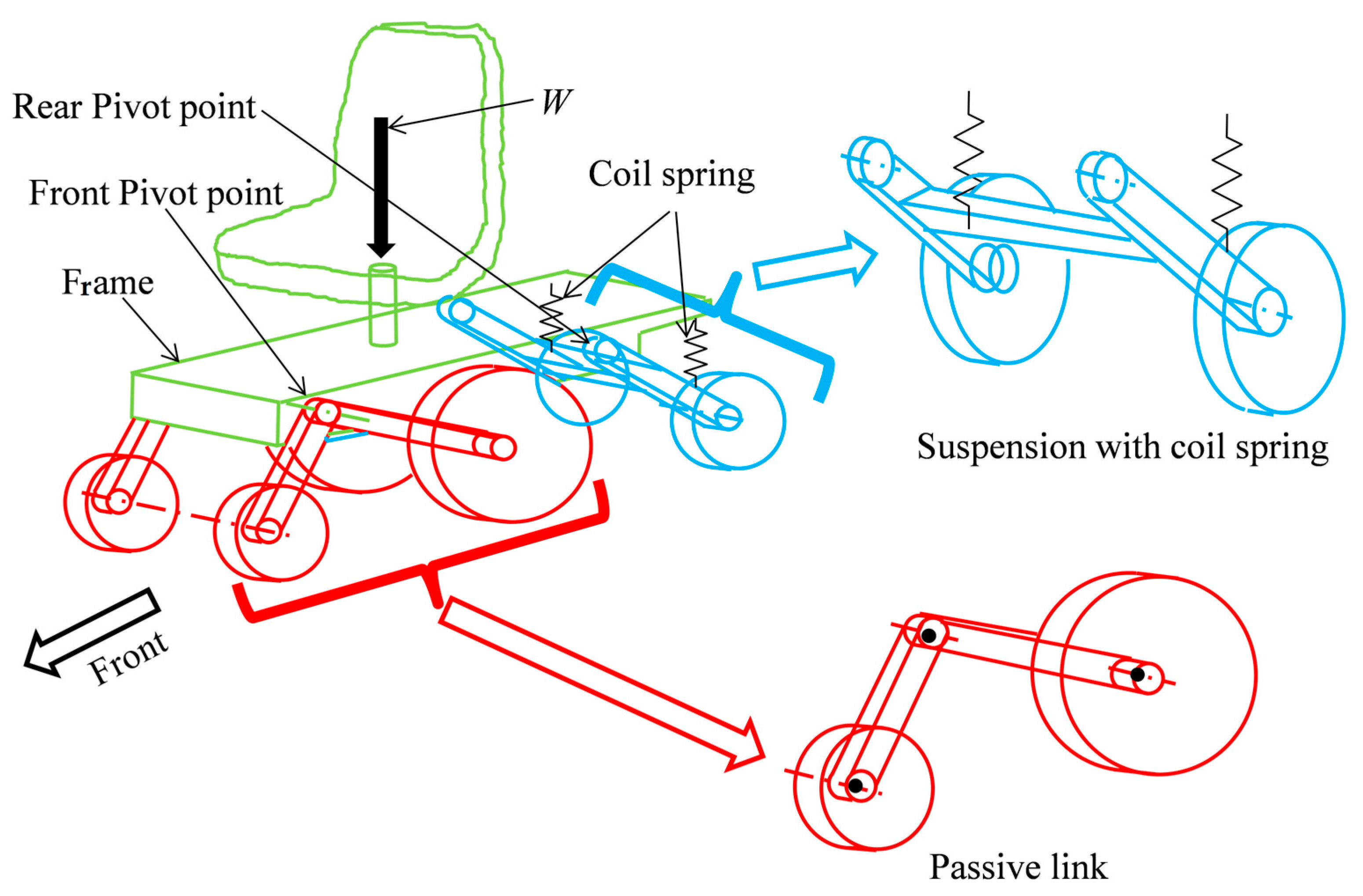
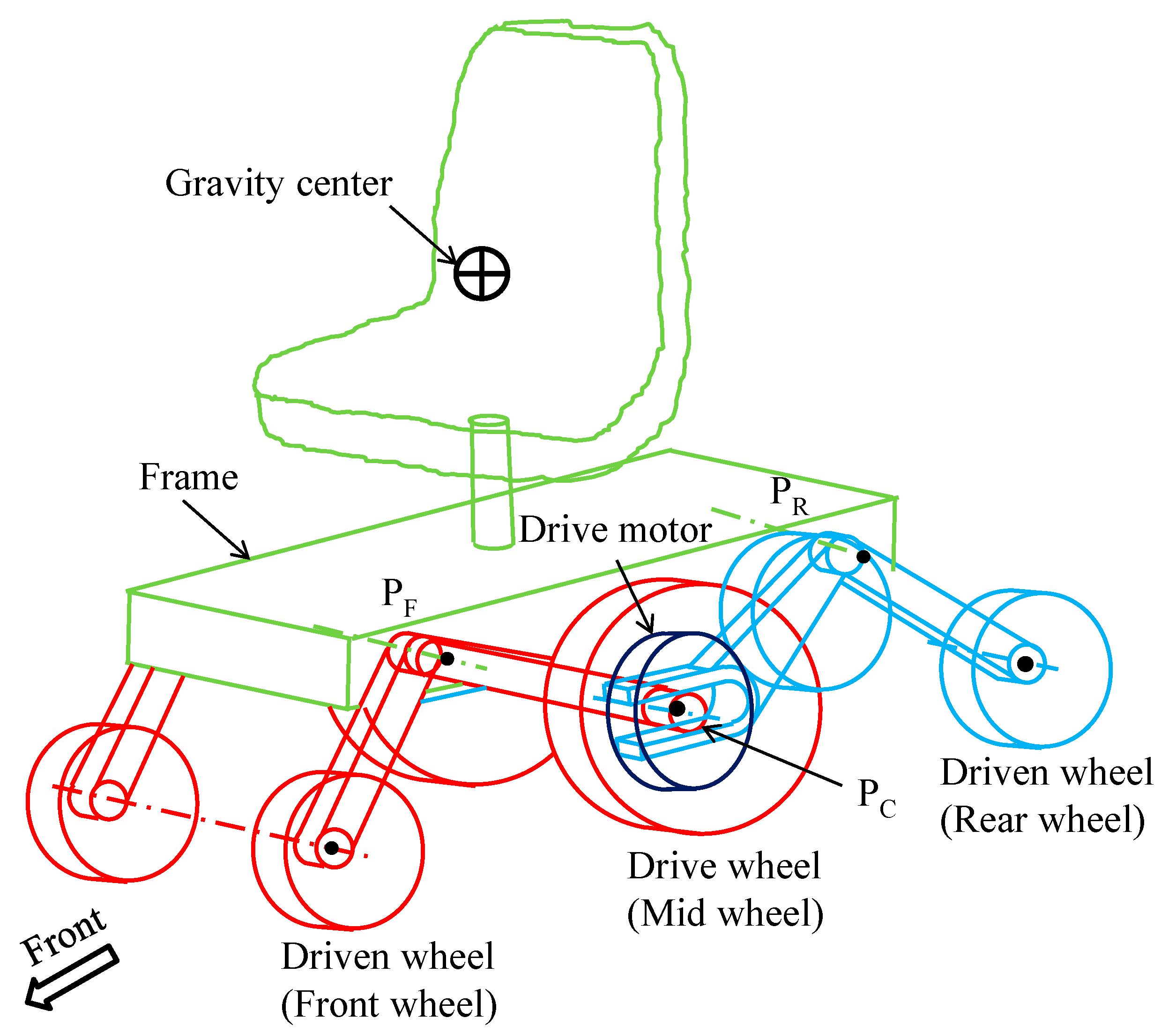
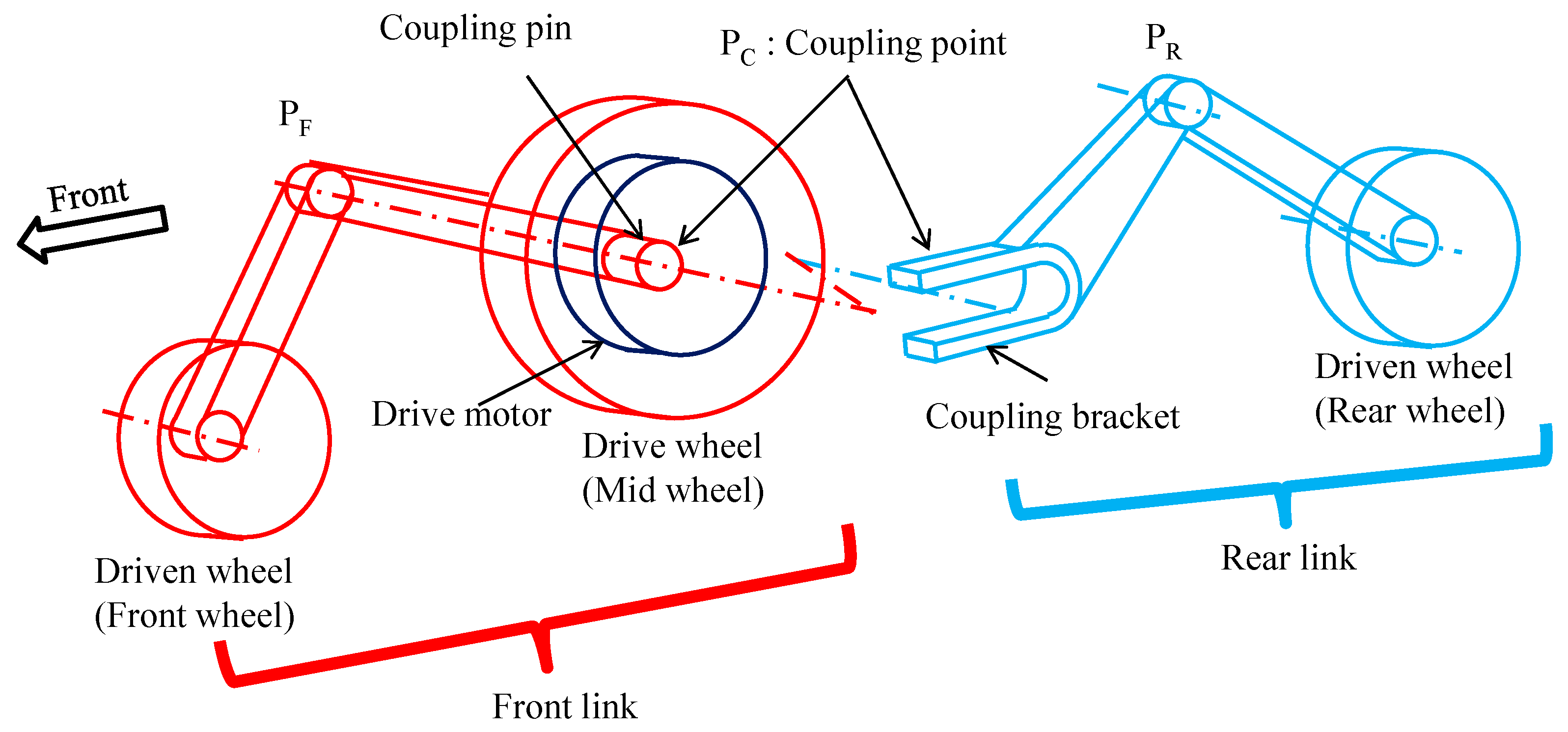
3. Description of the Chassis for the Improved Joystick-Type Six-Wheel Electric Wheelchair

This chapter describes a joystick-type six-wheel electric wheelchair with an improved chassis that is currently being developed for commercialization. It has been designed with the goal of having high resistance to tipping and a high ability to overcome obstacles. Please note that the improved chassis is currently under patent application [15,16] with the assumption of commercialization, and the text provides only a qualitative description of its structure and operation and does not provide specific numerical values. Figure 11 shows the complete wheelchair and Figure 12 shows the details of the suspension system. Figure 13 shows the weight distribution of each link from a side view. Next, the mechanism of this suspension system is explained. The front wheels, which are the driven wheels, and the middle wheel, which is the driving wheel, are attached to the front rocker, shown in red. The rear wheels, which are the driven wheels, are attached to the rear rocker, shown in blue. The front and rear rocker arms are connected to share the center wheel. The structure of the link is connected to the center wheel at the P_C connection point, and only vertical displacement can be transmitted between them. The vertical displacement of the P_C joint is transmitted by the rotating roller on the front link (red) and the U-shaped bracket on the rear link (blue). The roller is embedded in the U-shaped bracket and transmits the vertical displacement of the vehicle to each end. The relative movement of the roller and the U-bracket caused by the vertical movement of the wheel is absorbed by the roller rolling inside the U-bracket. A small gap is provided in the roller diameter and the inner width of the U-bracket to allow the roller to roll easily. The front rocker arm and the rear rocker arm are fixed to the vehicle frame so that they rotate only at the P_F and P_R pivots. By connecting the two front and rear rocker arms with a roller in the middle, it is possible to rotate the load of the aforementioned P_R pivot point to the center wheel. In this way, it is possible to make the dimensions a and b equal and to increase their values.

Next, the weight distribution to each wheel is explained using Figure 13. The load from the center of gravity is distributed to the front pivot P_F and the rear pivot P_R. The load to these two pivots is distributed to F_A and F_B, respectively, according to the ratio of dimensions a and b. The load to the front wheel and the center wheel attached to the front rocker is distributed to F_C and F_D, respectively, according to the ratio of dimensions c and d. And the load to the rear wheel and the U-shaped bracket attached to the rear rocker is distributed to F_E and F_F, respectively, according to the ratio of dimensions e and f. The roller attached to the front rocker arm is held in the U-shaped bracket attached to the rear rocker arm. Therefore, F_D and F_E are added at the inner contact point P_C and act on the center wheel.

In this section, we will explain wheel load distribution on uneven ground for the improved suspension system. We assume that the left front wheel, which is the drive wheel, drives over a curb as shown in Figure 14. We also assume that the height of the obstacle is such that the center of gravity shift caused by the overrun falls within the red lines formed by the points of the front and rear four-link suspensions in the top view of Figure 15.

Figure 16, Figure 17 and Figure 18 show the wheel loads when driving over a curb, as shown in Figure 14 and Figure 15. Figure 16 shows the wheel load distribution on the left side, where the curb is located. A downward load is generated on each wheel, as shown, and the wheels are secured in contact with the ground. Figure 17 shows the load distribution on the left and right wheels from the front of the wheelchair. As can be seen, the load is transferred to the wheel on the side without the curb. Figure 18 shows the wheel load distribution on the side without the curb, i.e., the right side. In this case, the upward displacement of the front pivot point F_P that occurs on the left side lifts the right pivot point F_P. This creates an upward force F_A on the right pivot F_P. If this force is greater than the initial front wheel load, the front wheels will lose contact with the ground. However, as shown in the figure, since the center of gravity is between the center and rear wheels, the center and rear wheels remain in contact with the ground and stable running is possible.

Finally, we will explain the wheel load distribution on each wheel when running on a slope with the improved suspension system. Figure 19 and Figure 20 show the wheel load distribution on each wheel when running at different forward tilt angles. Figure 19 shows the state in which a line drawn vertically from the center of gravity to the ground points forward of the rear pivot P_R. As can be seen from the figure, each wheel can maintain stable contact with the ground. On the other hand, Figure 19 shows the state in which the vehicle runs at a large tilt angle, where a line drawn vertically from the center of gravity to the ground points backward of the rear pivot P_R. In this state, an upward force F_A acts on the front pivot, causing the front wheels to lose contact with the ground.

From the above, the range in which stable running is possible is expanded by the size of dimensions a and b, which are derived from the ratio of dimensions c, d, e, and f of the improved suspension system shown in Figure 13.

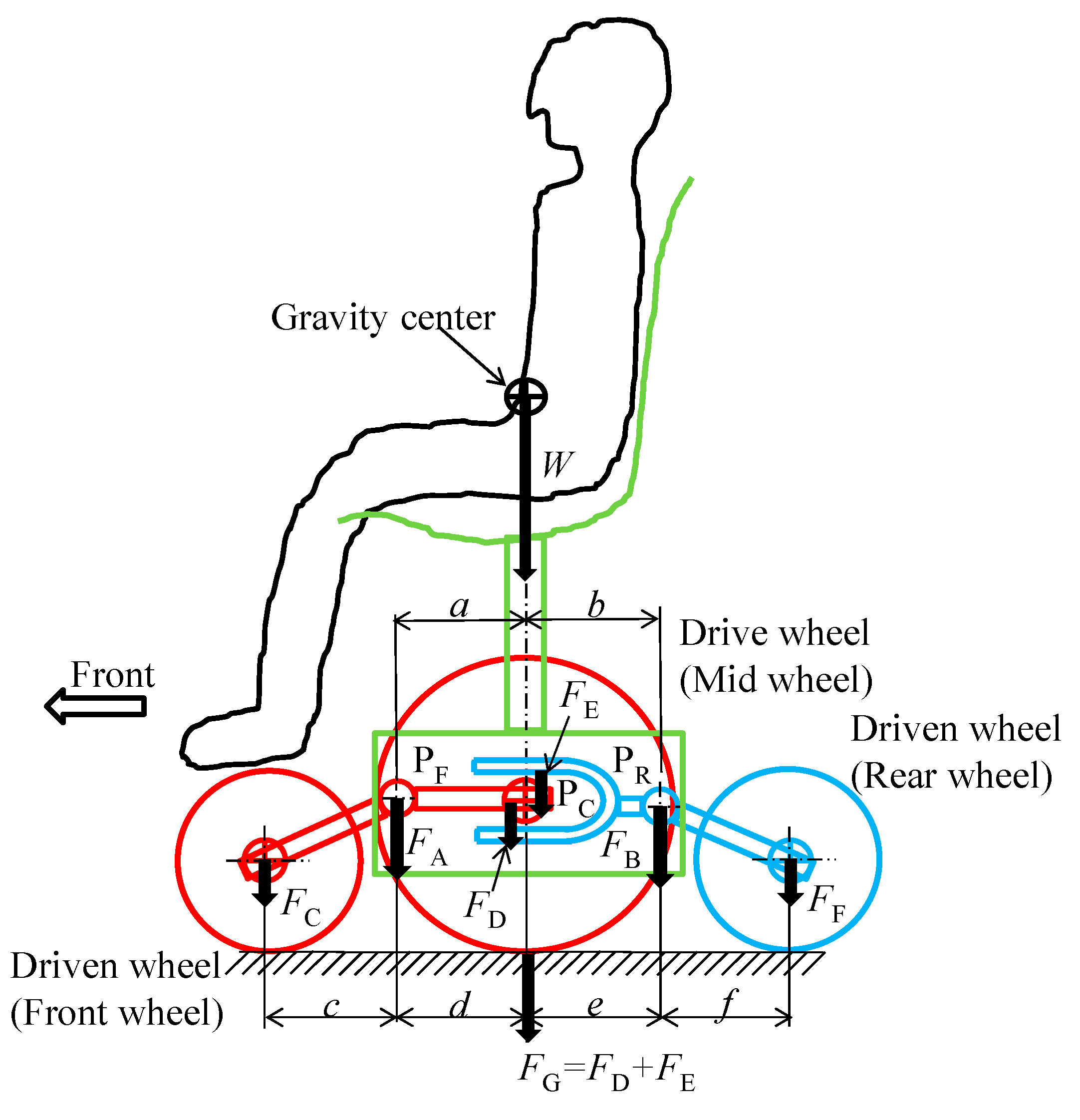
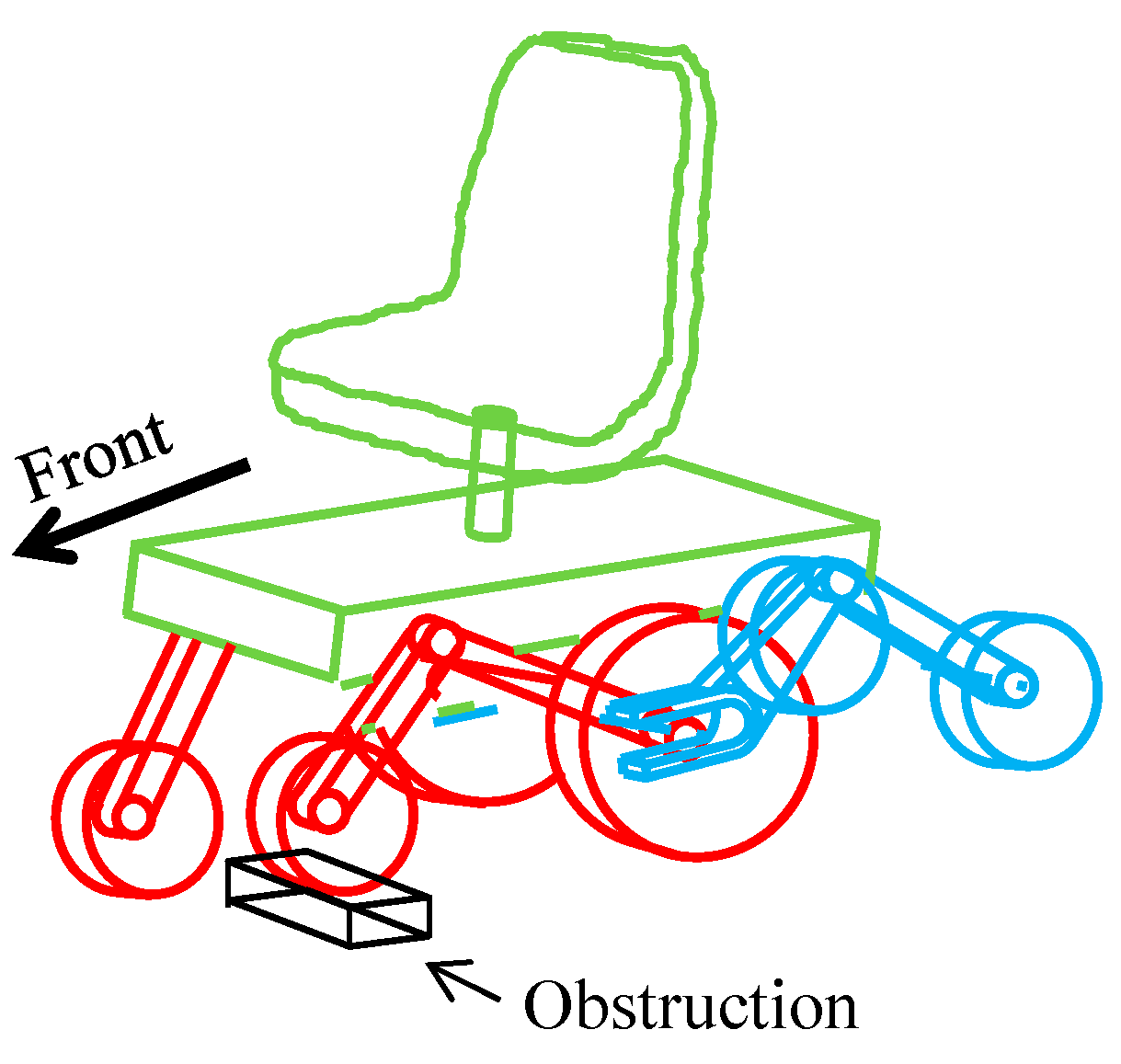
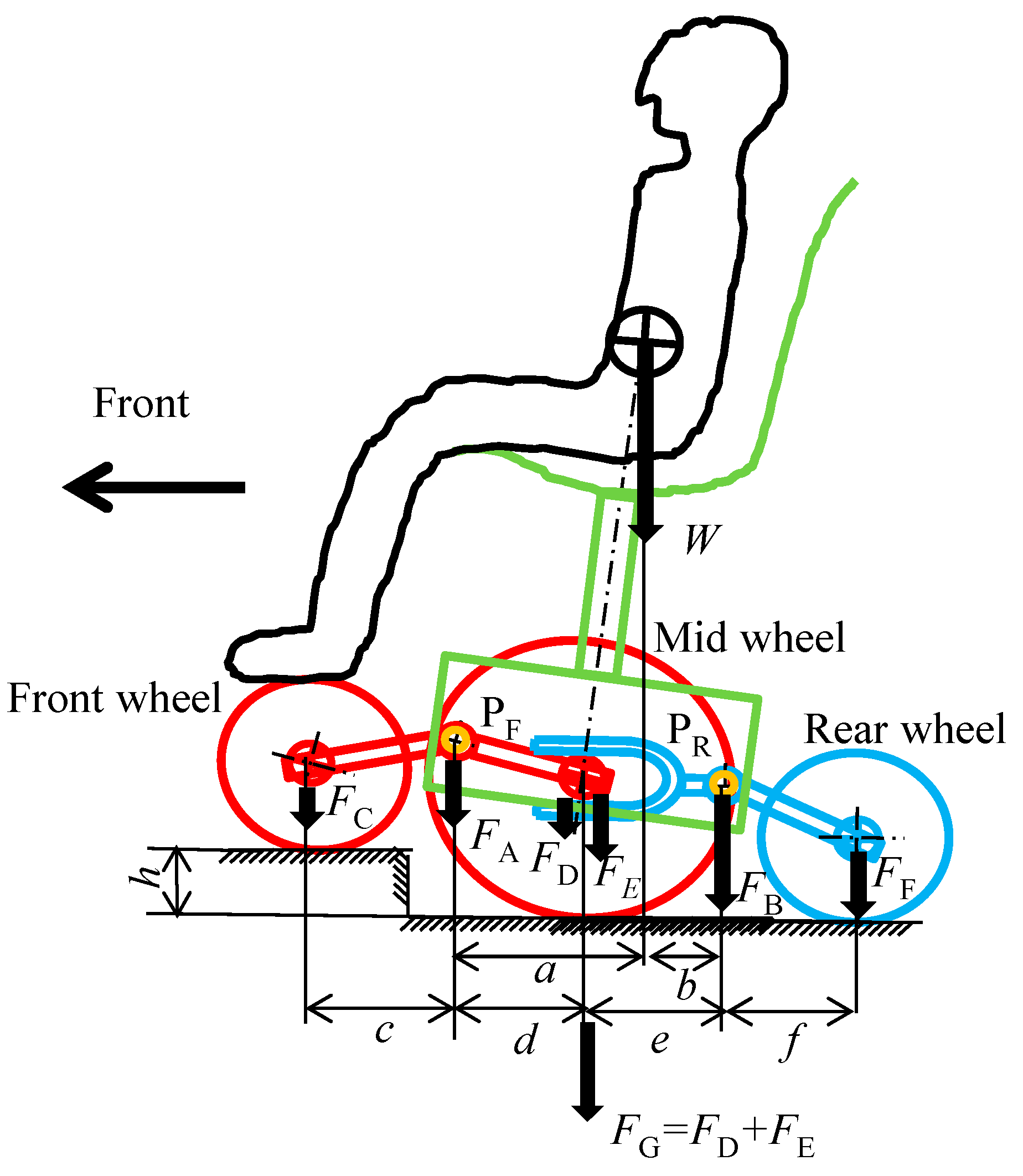
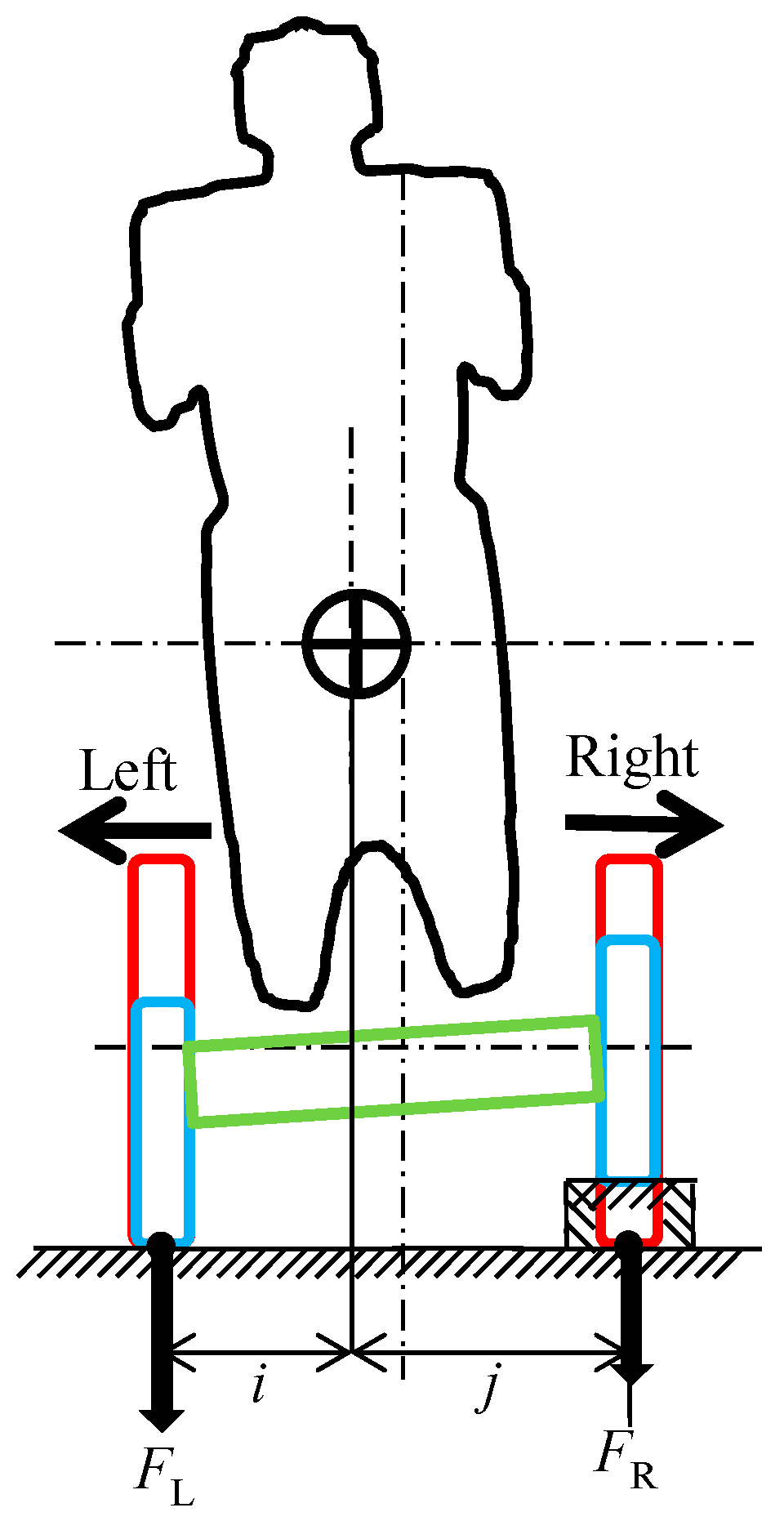
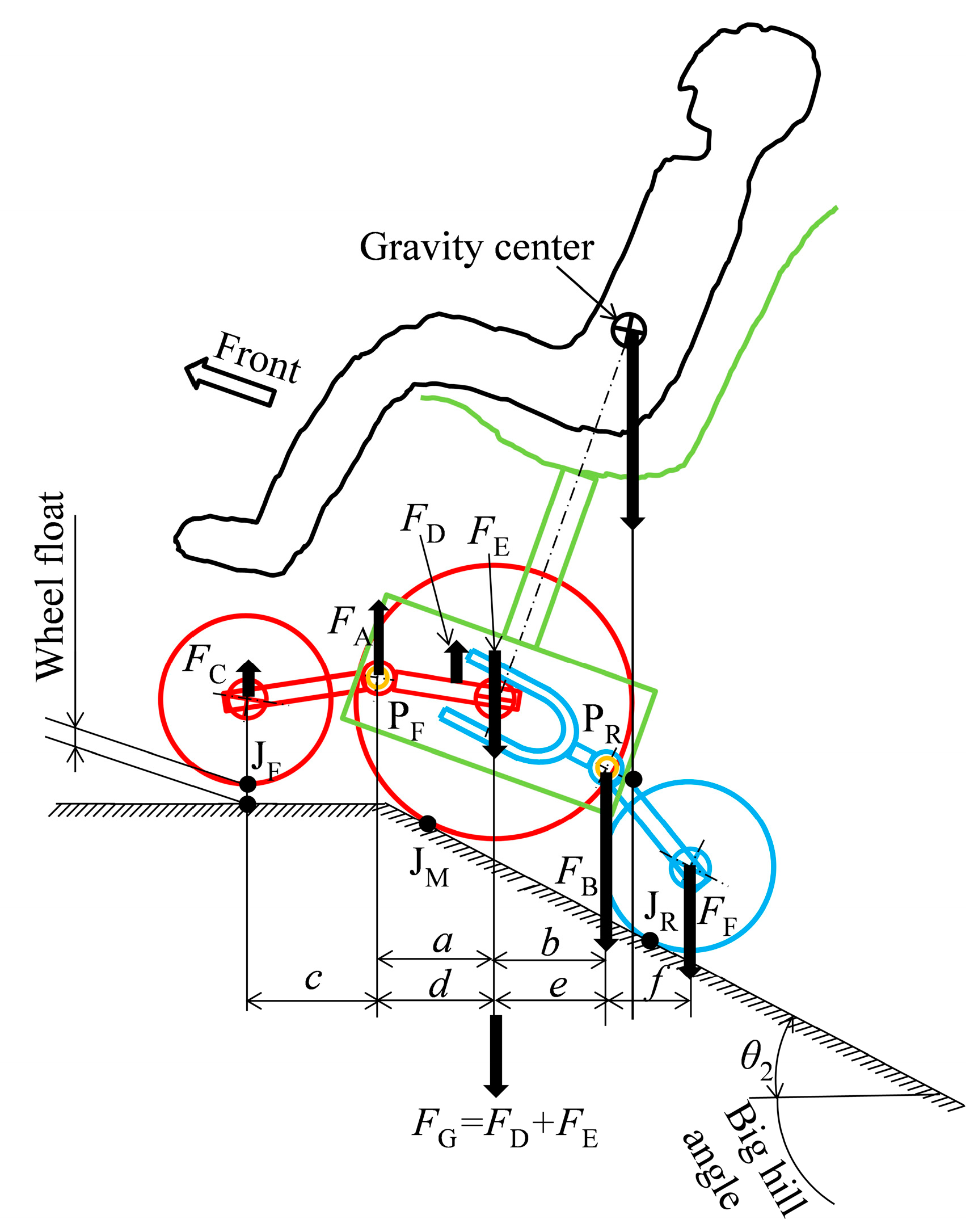
4. Joystick-Type Six-Wheeled Electric Wheelchair Wheel Mechanism for Climbing over Obstacles

For a joystick-type six-wheel electric wheelchair to get over a curb, the front wheels, which are the driven wheels, must get over the curb first. Then, the center wheels, which are the driven wheels, must get over the curb. It goes without saying that if the front and center wheels are successful in getting over the curb, the rear wheels will easily get over it.

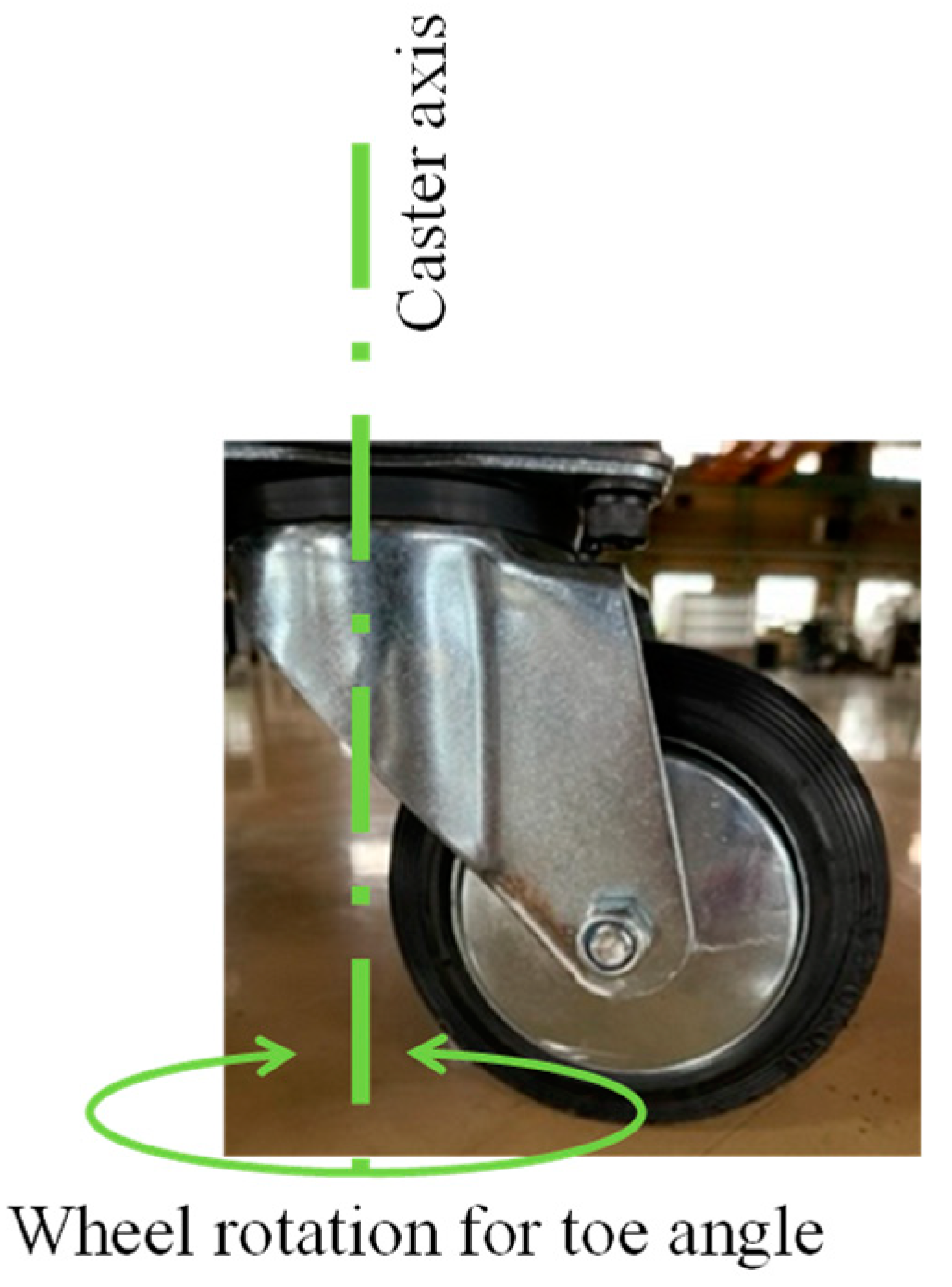
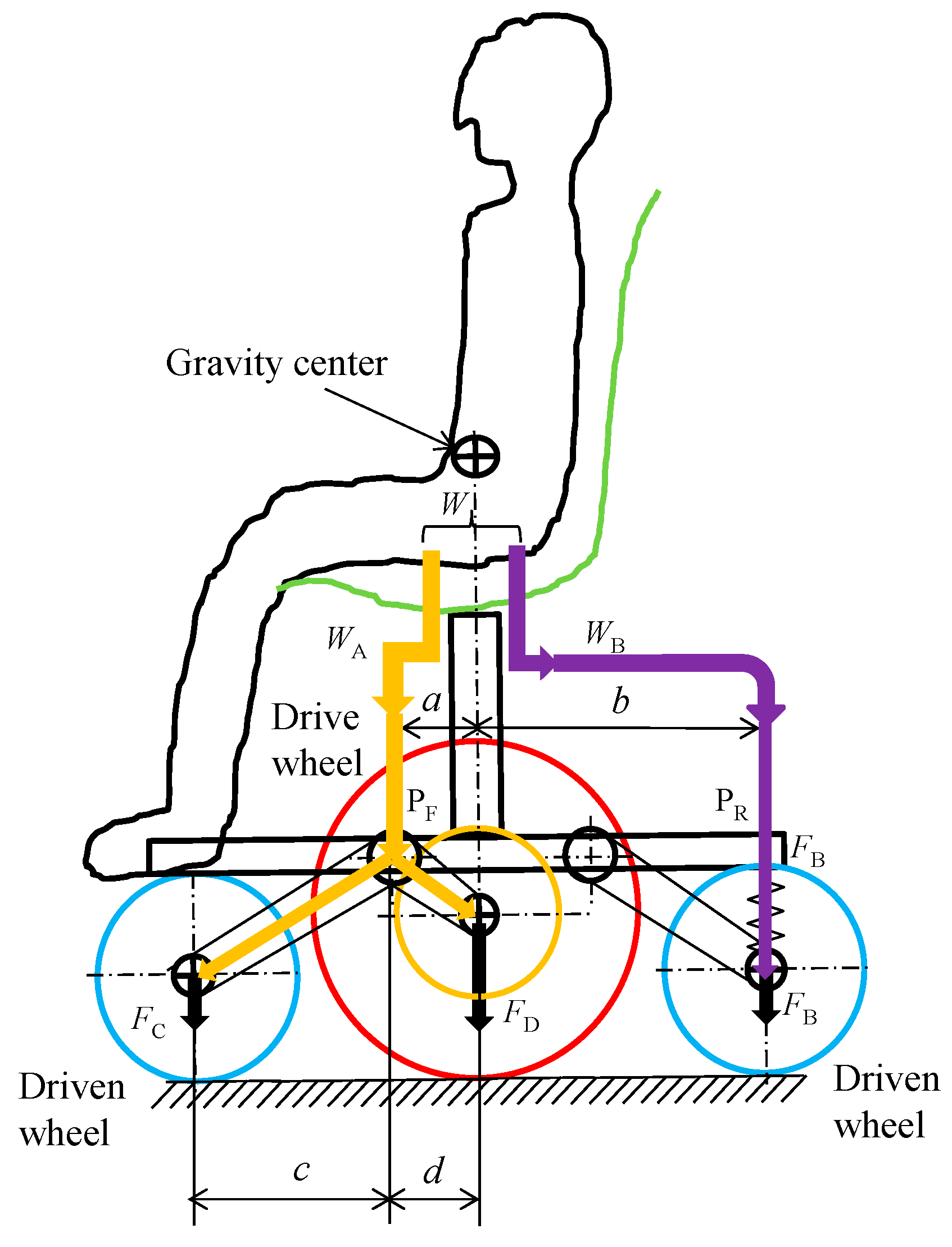
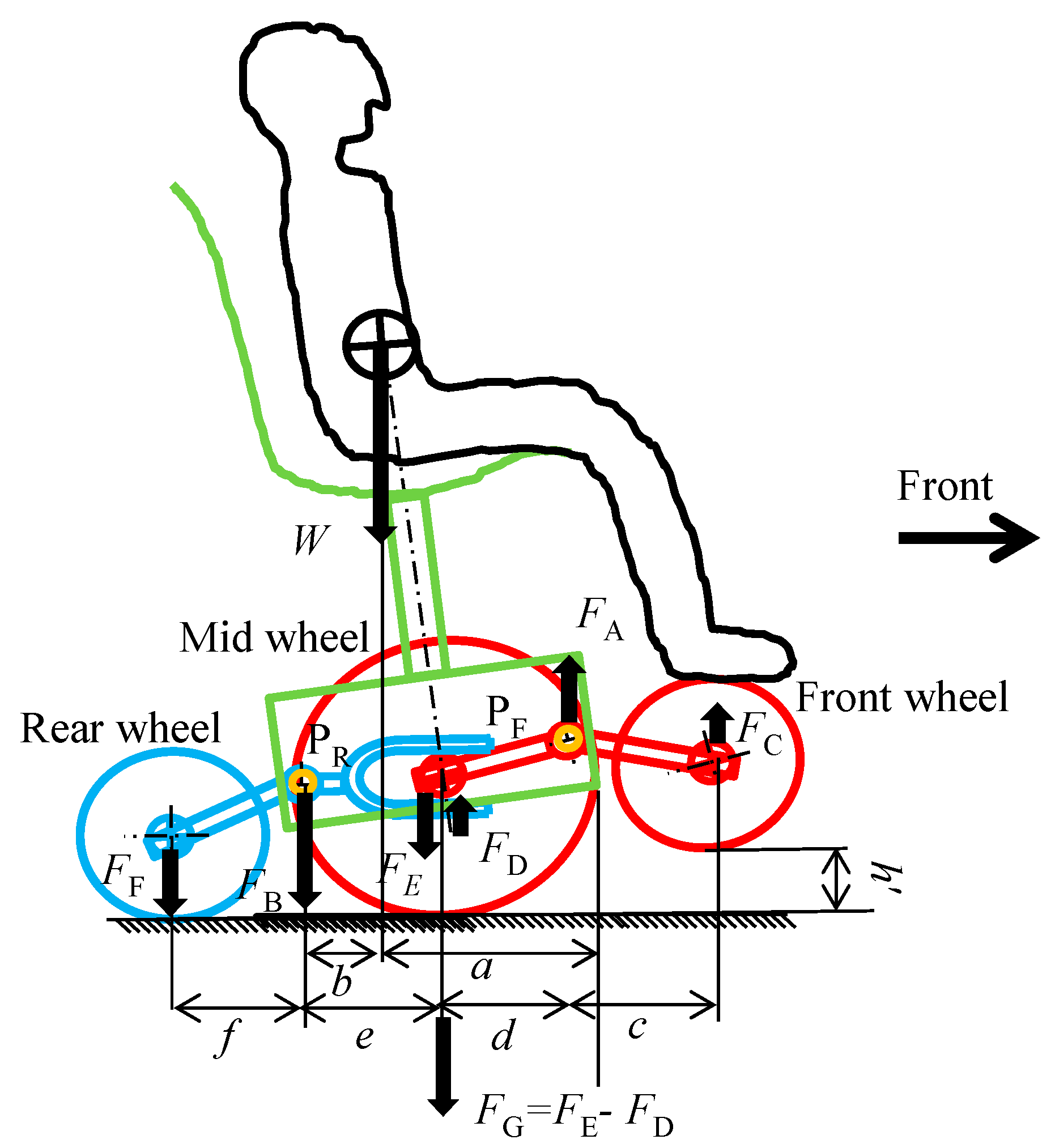
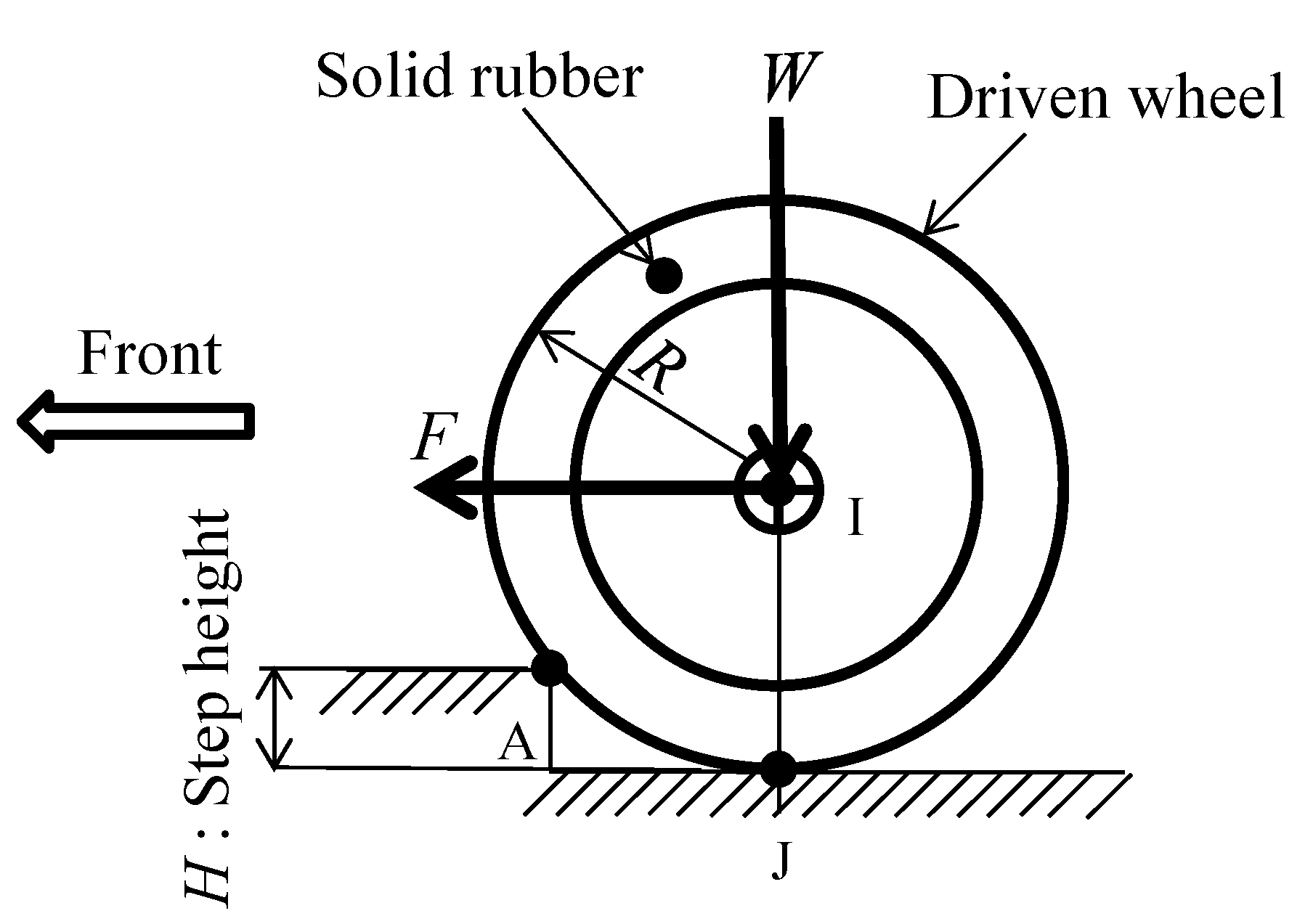
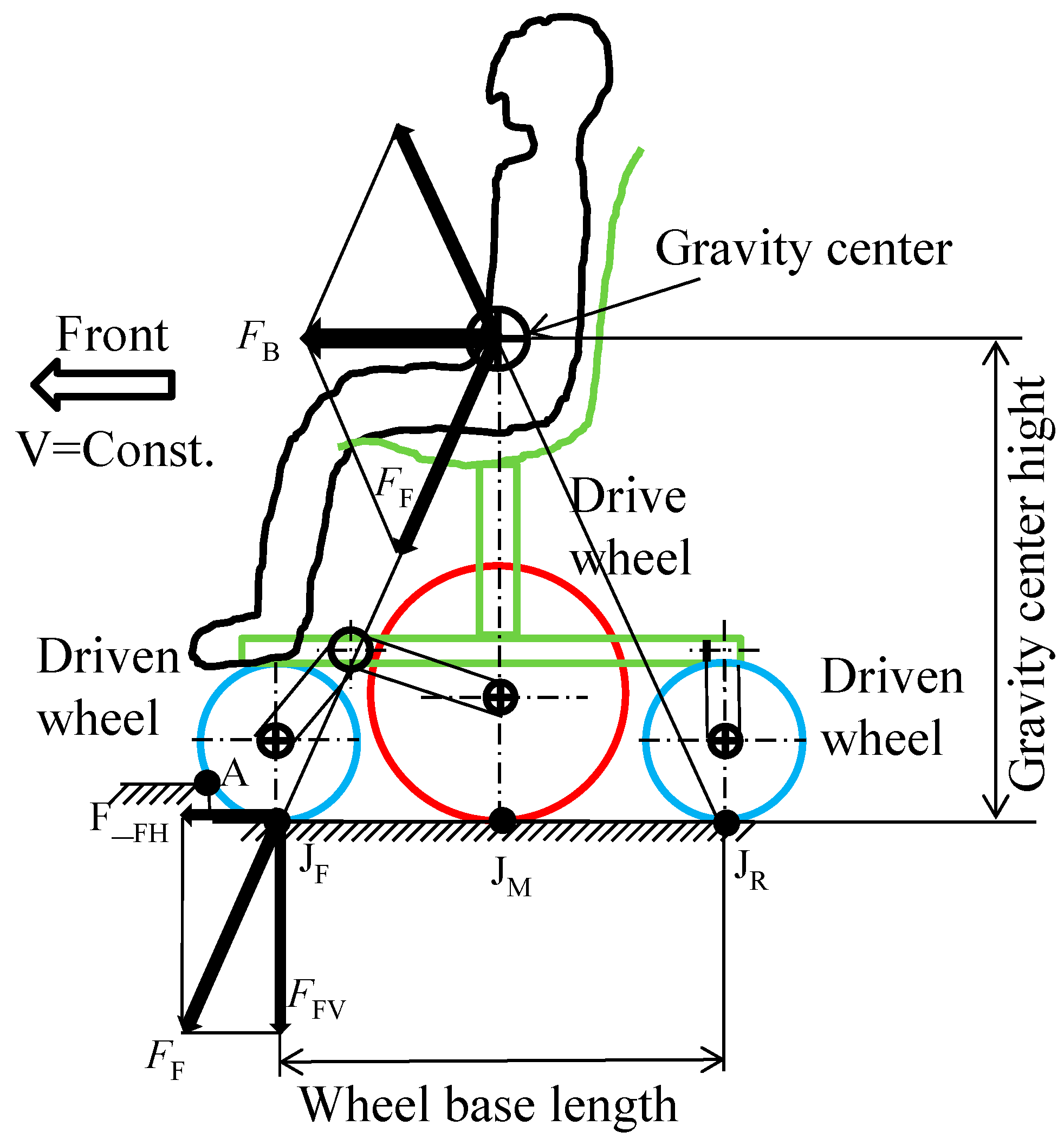
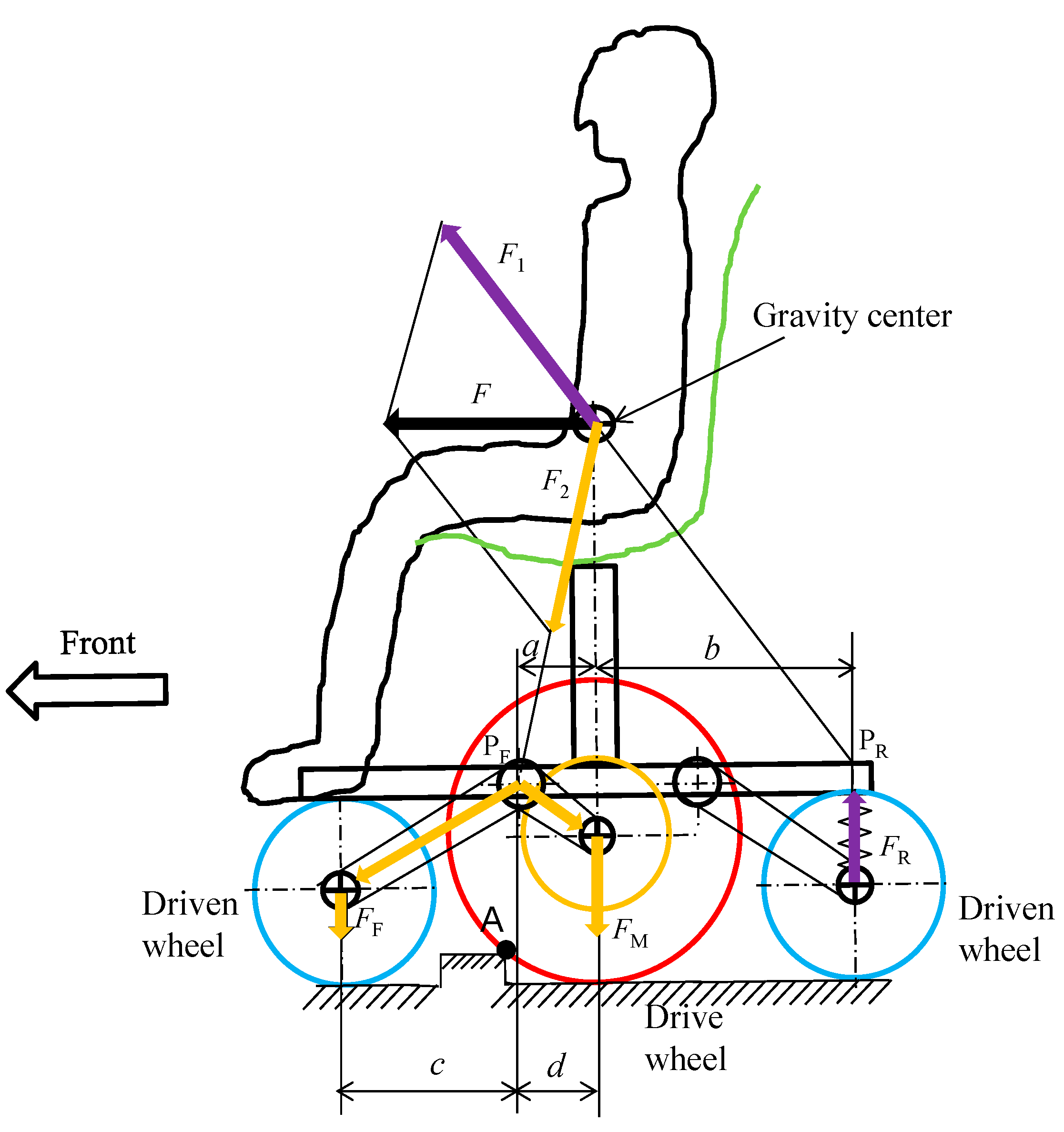
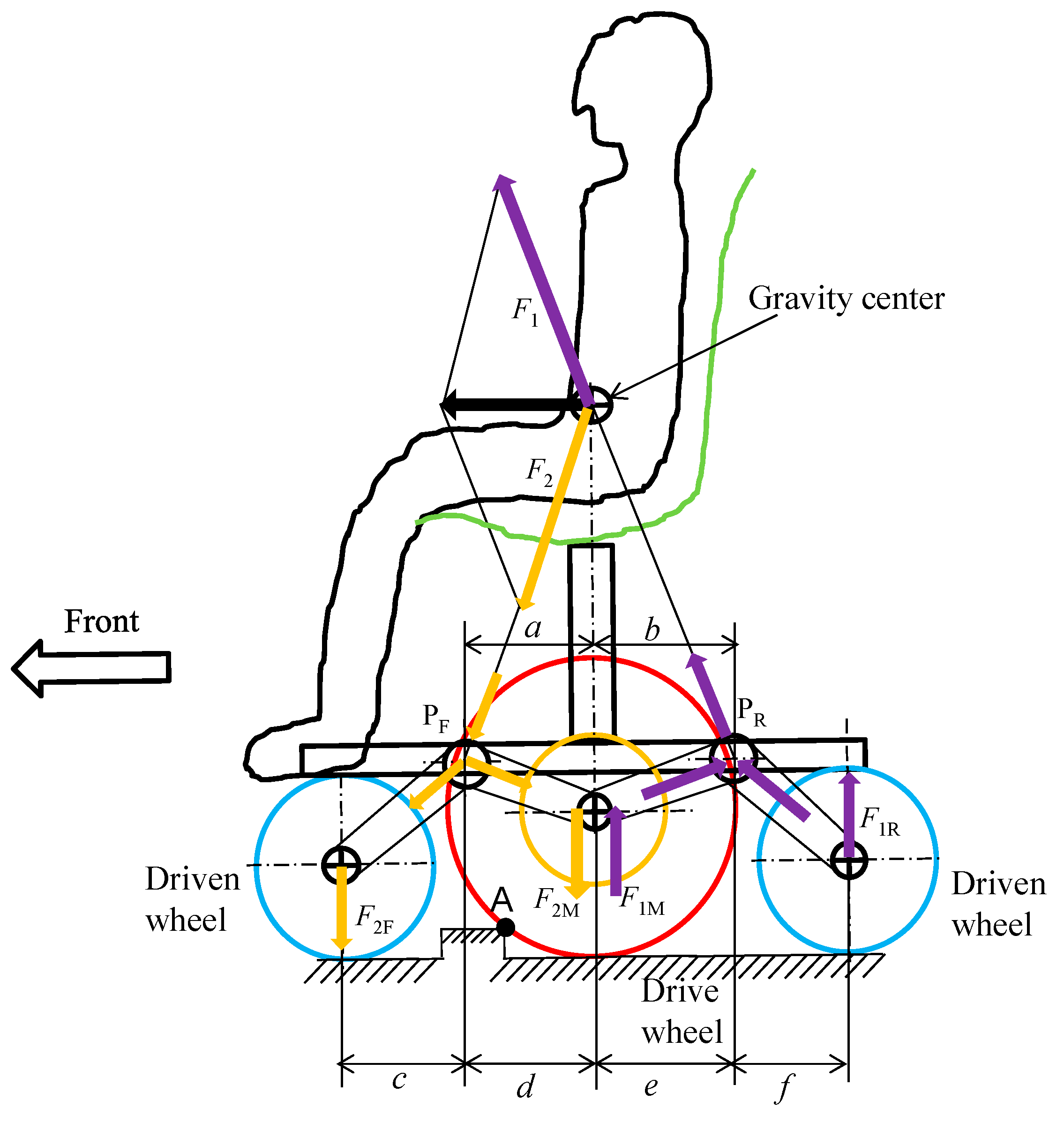
4.1. Mechanism for the Driven Wheels (Front Wheels) When Passing over a Curb

First, the mechanism by which the driven wheel passes over a curb will be explained. Figure 21 shows a diagram of the driven wheel encountering a curb. The symbols in the diagram will be explained. The radius of the driven wheel is R, and the curb-climbing height is H. The forward thrust applied to the driven wheel is F, and the wheel load of the driven wheel is W. Point I indicates the wheel center, point J indicates the contact point between the ground and the wheel, and point A indicates the contact point between the curb and the wheel. Please note that the thrust F mentioned here is not only the driving force of the motor, but also includes the inertial force generated when the wheelchair encounters the curb. Furthermore, the wheel load of the driven wheel W includes not only the static front wheel load of the electric wheelchair, but also the inertial force generated by the wheelchair. Note that the driven wheel is made of hard solid rubber, so it is assumed that it does not deform.

Figure 22 shows the balance of local forces that occurs when a wheel contacts point A. When a driven wheel contacts point A, a rotational moment M (shown in green) is generated that rotates point I counterclockwise around point A. This causes a diagonally forward upward force F₁ to act on point I. The vertical component of F₁ is F_V1. Next, when the wheel with propulsive force F contacts contact point A, a force F_A (shown in red) acts from point I towards point A, generating a reaction force F_AO. The vertical component of this force is F_V2. The sum of F_V1 and F_V2 is greater than the wheel load W of the driven wheel, allowing the wheel to overcome a curb climb. One way to make it easier to overcome a curb climb is to increase the difference between the tire radius R and the curb-climbing height H. Other methods include increasing the propulsive force F and reducing the wheel load W. The mechanisms described here have already been described in other studies [17,18]. Most of the previous studies on the mechanisms of wheels going over curbs have been for walking assistance devices, but there have been no studies on the passive or drive wheels of joystick-type six-wheel electric wheelchairs. Here, we proposed wheelchair specifications that can efficiently go over steps based on the curb-climbing mechanism.

Next, let us consider F and W when the front wheel, which is the driven wheel, encounters a curb while an actual wheelchair is moving. Figure 23 shows a state in which acceleration occurs at the center of gravity when the wheelchair encounters a curb while moving. This causes a forward inertial force F_B to act on the center of gravity. This, in turn, causes vertical and horizontal components F_FV and F_FV to act at the front wheel contact point J_F. The vertical component F_FV acts downward, increasing the driven wheel load W. The horizontal component F__FH is generated in the forward direction, increasing the propulsive force F.

From above, an increase in the driven wheel load W due to contact with a curb while moving is detrimental to getting over the curb. On the other hand, an increase in propulsive force F is advantageous for getting over the curb. In other words, it can be seen that F_FH and F_FV caused by a wheelchair encountering a curb have opposing effects on the ability to get over the curb. However, if the wheelbase length of a wheelchair is more than twice the height of the center of gravity, the absolute value of F_FH will exceed the absolute value of F_FV, and the inertial force generated when the wheelchair encounters a curb can be used to improve curb-climb-crossing performance.

Next, consider F and W when a wheelchair accelerates from a stationary state. Figure 24 shows how the force F_T acting on the center of gravity when the wheelchair accelerates acts on the front wheel, which is the driven wheel. The vertical component F_FV and horizontal component F_FV act on the front wheel contact point J_F. The vertical component F_FV acts upward and reduces the wheel load W of the driven wheel. The horizontal component F_FH is generated in the backward direction and reduces the propulsive force F.

From the above, the reduction in the wheel load W of the driven wheel due to the acceleration of the wheelchair works in favor of going over a curb. On the other hand, the reduction in the propulsive force F works against curb climbing. In other words, it can be seen that F_FH and F_FV generated by a wheelchair encountering a curb have opposite effects on the curb-climbing performance. However, if the wheelbase length of the wheelchair is less than half the height of the center of gravity, the absolute value of F_FV will exceed the absolute value of F_FH, and the inertial force generated by the wheelchair accelerating can be used to improve the curb-climbing performance. However, the relationship between the wheelbase length and the center of gravity height during acceleration in Figure 24 and during deceleration in Figure 23 is contradictory, and no dimensions exist that satisfy both.

When the dimensions c and d in Figure 13 can be set to a ratio close to 1:1, as in the improved joy-stick-type six-wheeled electric wheelchair shown in Figure 11, Figure 12 and Figure 13, the motor driving force of the center wheel affects the F and W of the driven wheels, which are the front wheels. When the dimensions c and d in Figure 13 are c > d, as in the conventional type shown in Figure 7, the effect of the driving force on F and W is small.

To explain Figure 25, the driving reaction force F₁ of the motor driving wheel acting on the contact point J_M acts on the pivot point P_F of the front rocker link, and F_M acts in the forward and upward directions. The vertical component F_U of F_M is divided into the front and center wheels by the inverse of the ratio of dimensions c and d, and acts on each of them. This reduces the W of the front and center wheels, which is advantageous for the front wheels’ ability to overcome curb climbing. For example, the torque of the motor is greatest at startup. Therefore, by bringing the front wheels into contact with a curb and moving forward from a stationary state with maximum acceleration force, it is possible to contribute to an improvement in the front wheels’ ability to overcome curb climbing.

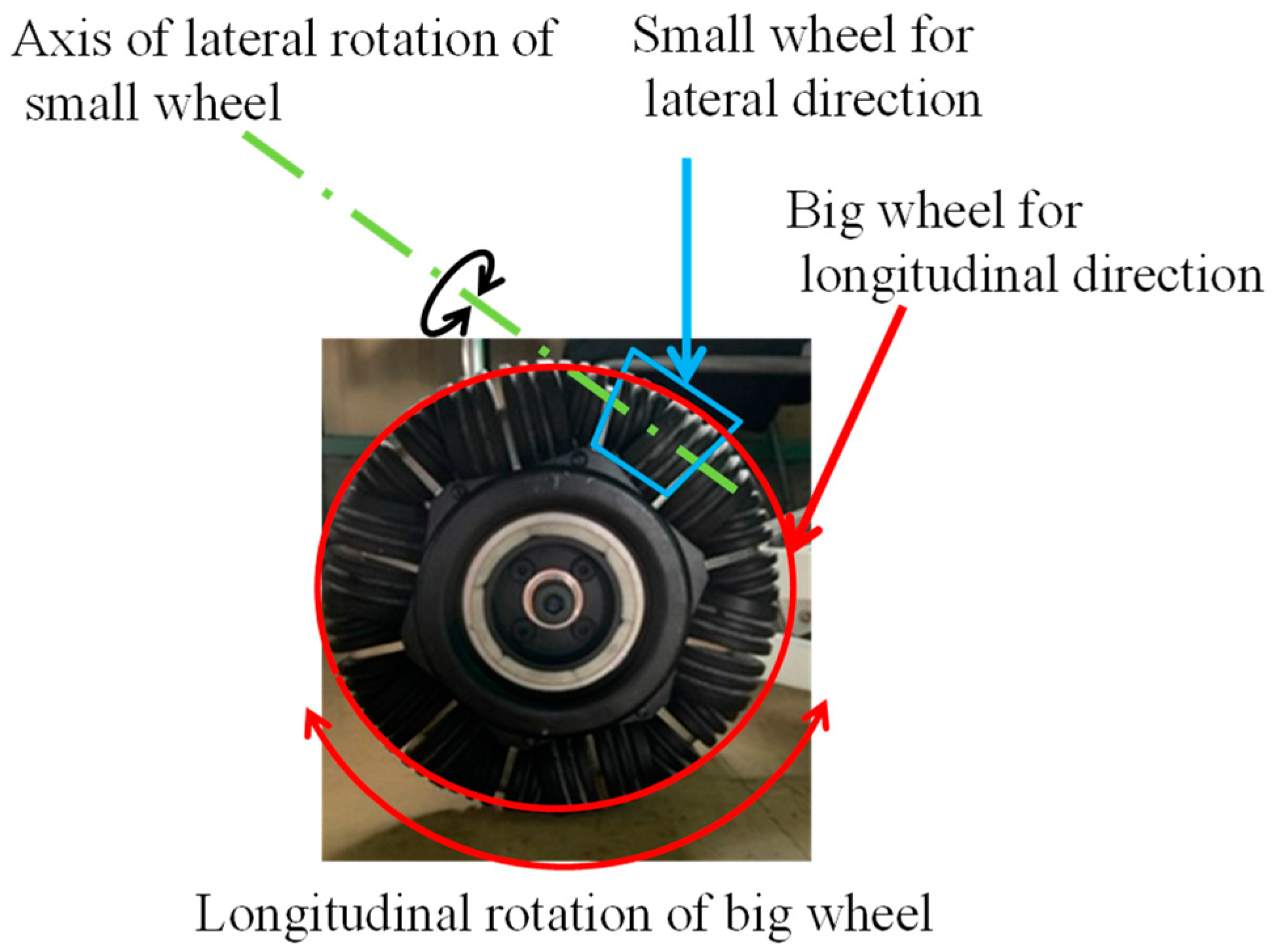
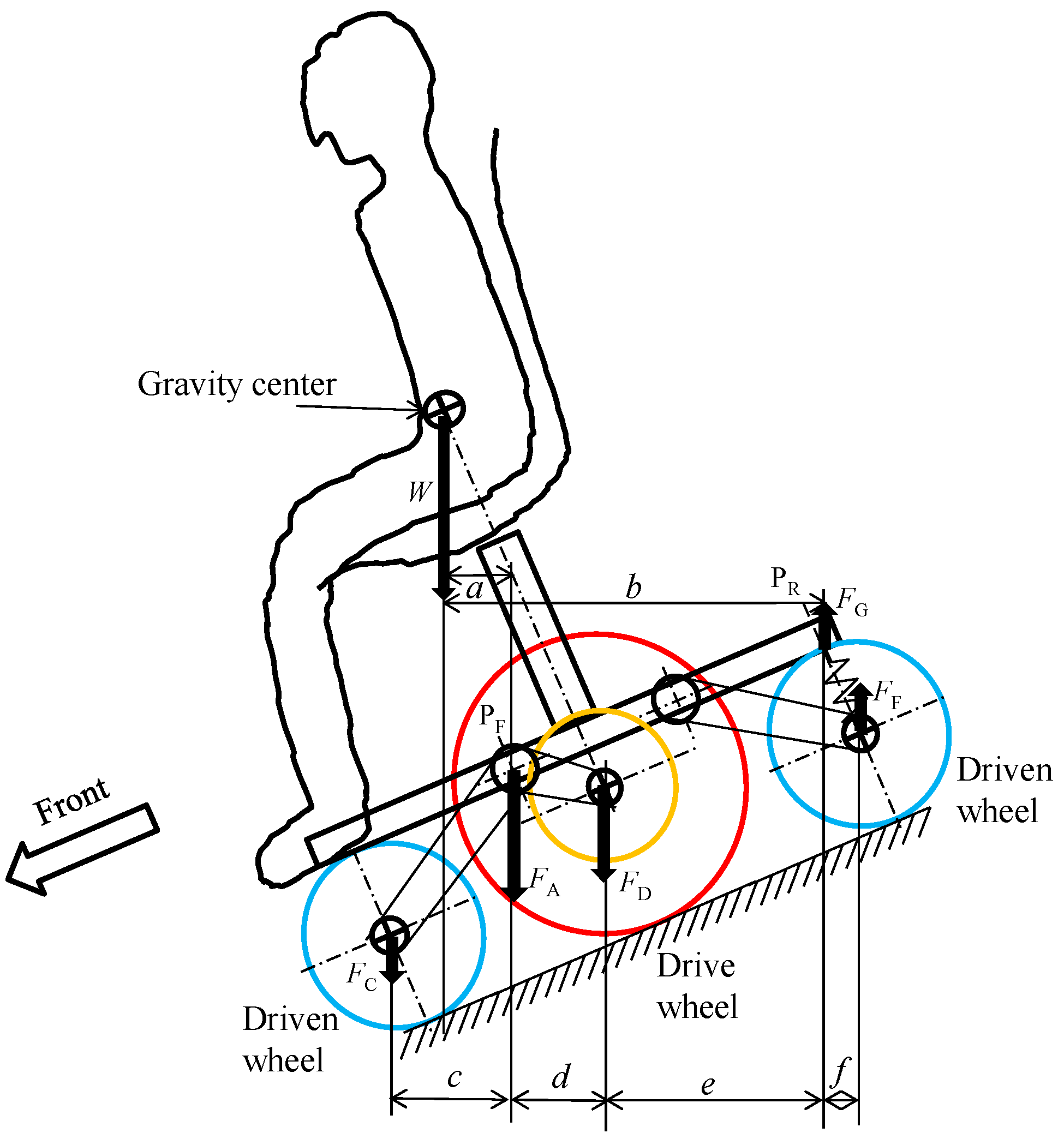
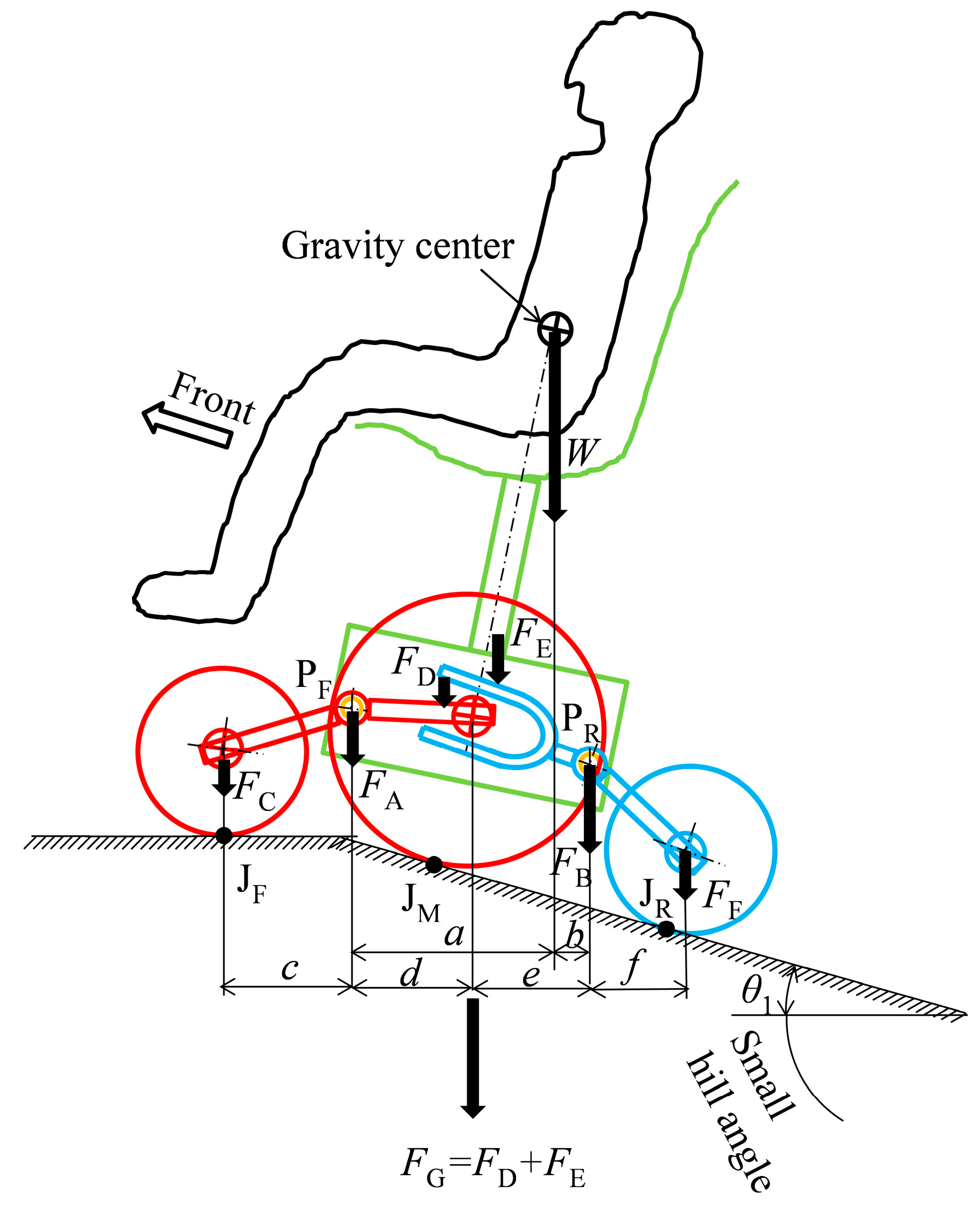
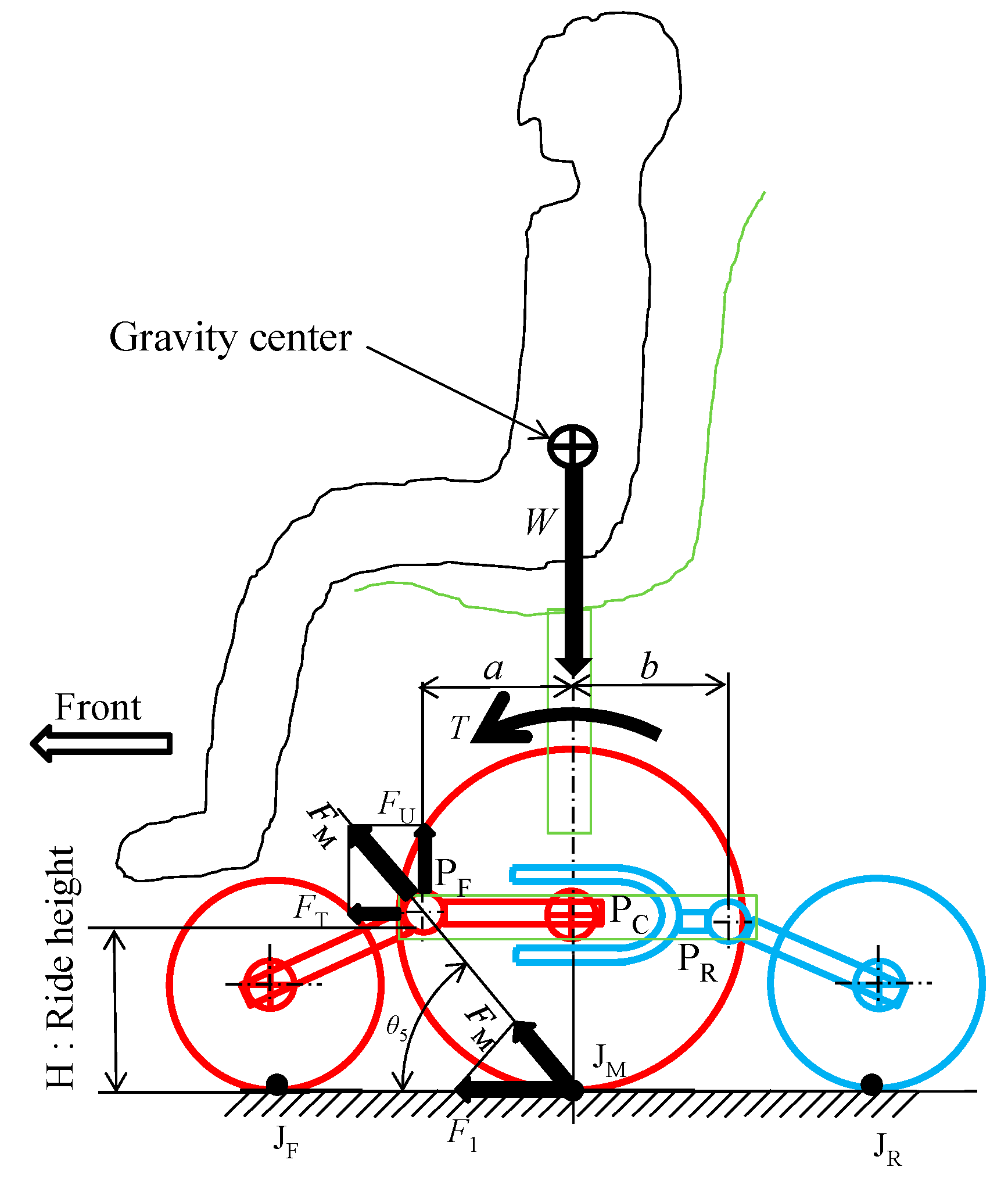
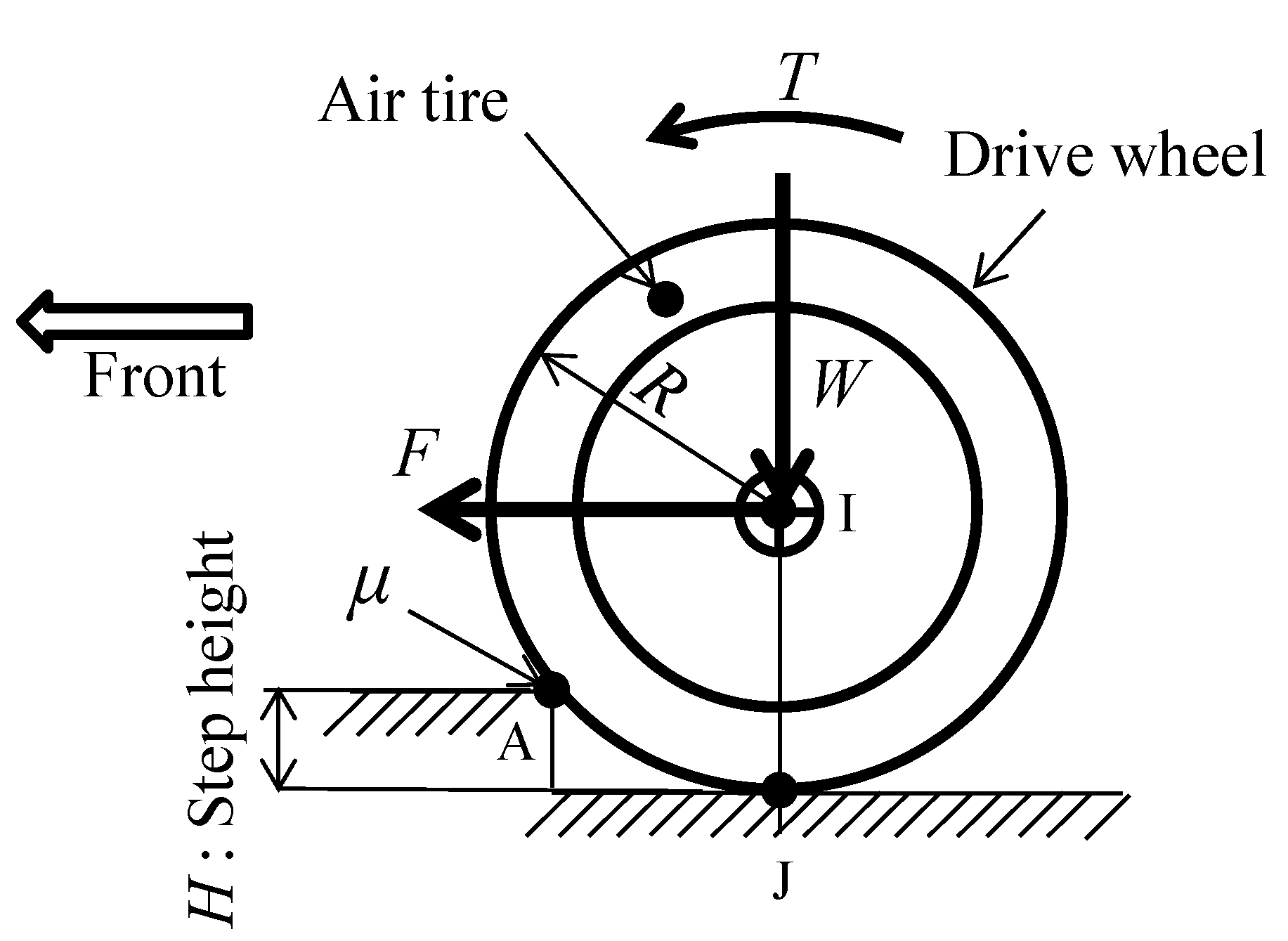
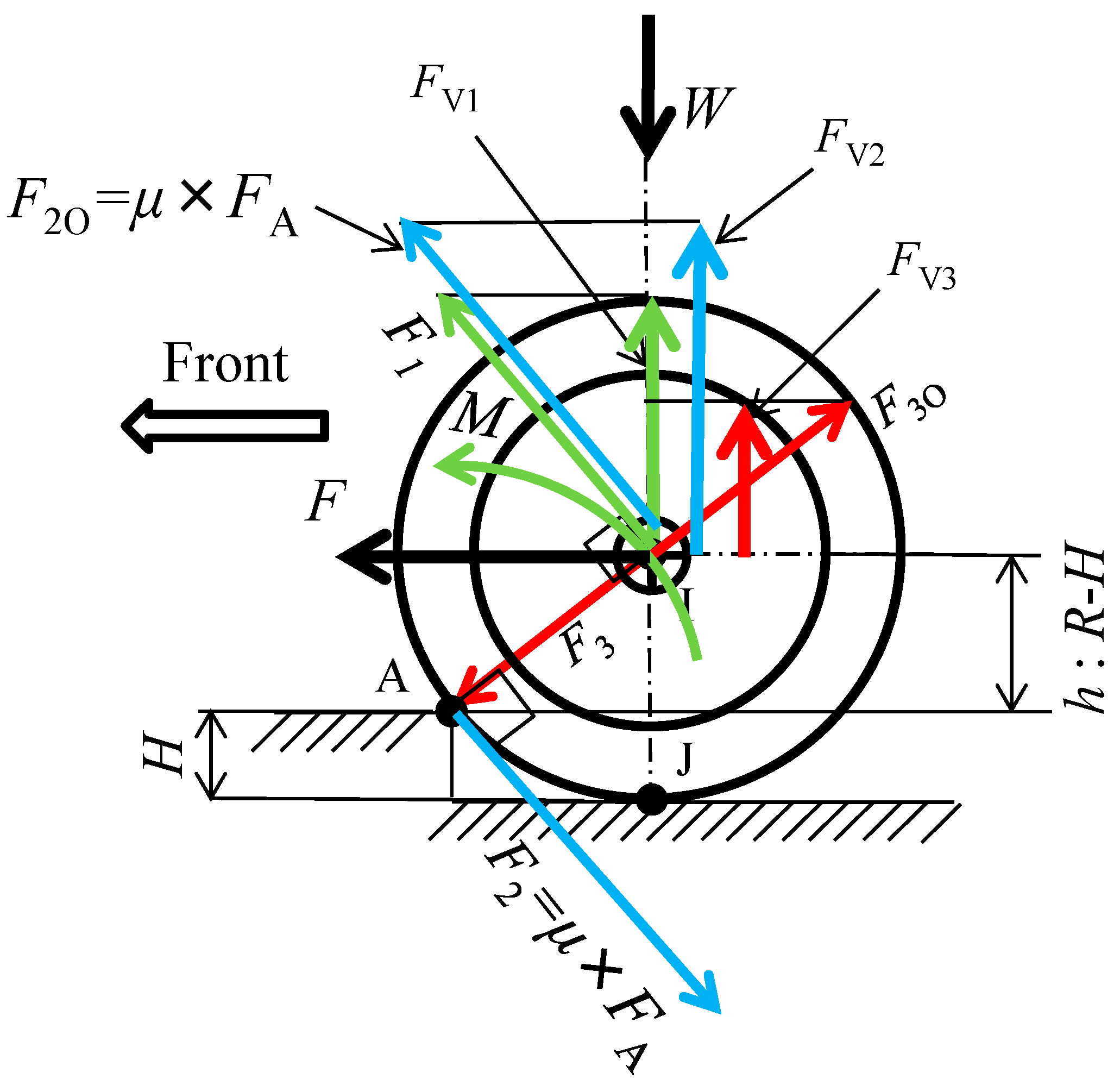
4.2. Mechanism for the Drive Wheel (Center Wheel) Passing over a Curb

Here, we will examine the mechanism by which the central wheel, which is the drive wheel, passes over a curb. Figure 26 shows a diagram of when the drive wheel encounters a curb. The symbols in the diagram are explained below. The drive wheel radius is R, and the curb-climbing height is H. The forward thrust force applied to the drive wheel is F, and the drive wheel load is W. Point I indicates the wheel center, point J indicates the contact point between the ground and the wheel, and point A indicates the contact point between the curb and the wheel. The torque of the drive motor is T, and the friction coefficient between the wheel and the contact point with the curb is μ. Note that the drive wheels are pneumatic tires and so will deform under load, but here we assumed that they would not deform.

Figure 27 shows the balance of local forces that occur when a wheel encounters point A. Just like a driven wheel, when a wheel encounters point A, a rotational moment M (shown in green) is generated that rotates point I counterclockwise around point A. This causes a diagonal upward forward force F₁ to act on point I. The vertical component of F₁ is F_V1. When the wheel encounters the curb, a frictional force F₂ (blue) acts in the tangential direction of point A on the wheel, and the reaction force F_2O acts on point I. The vertical component of this force is F_V2. When the wheel encounters the curb, a force F₃ (red) acts from point I towards point A, and the reaction force F_3O acts on the wheel point I. The vertical component of this force is F_V3. When the sum of F_V1, F_V2, and F_V3 exceeds the driving wheel load W, the wheel will be able to overcome the curb-climbing task. To make it easier to overcome a curb, increase the difference between the tire radius R and the curb-climbing height H. Increasing the propulsive force F and reducing the wheel load W are ways to do this, as well as increasing the coefficient of friction μ between the wheel and the curb.

Next, we will examine the effect of the inertial force generated when the drive wheels of an electric wheelchair encounter curb-climbing when going over a curb. Figure 28 shows the change in wheel load of the drive wheels due to the inertial force F in the conventional chassis shown in Figure 8. The inertial force F indicated by the black arrow acts on the front rocker arm pivot points P_F and P_R directly above the coil spring. The downward force F₂ indicated in green acts on the front pivot point P_F, and the upward force F₁ acts on the rear pivot point P_R. As a result, a downward force acts on the center wheel and front wheel, increasing the wheel load W of both. In the case of the conventional type with dimension c > d, the increase in wheel load of the center wheel is significantly large, which is detrimental to going over curbs.

Next, we considered the inertial force when the drive wheels in the improved chassis go over a curb. Figure 29 shows the change in the wheel load of the drive wheels due to the inertial force F in the improved chassis shown in Figure 11. The inertial force F indicated by the black arrow acts on the front rocker arm pivot point P_F and the rear rocker arm pivot point P_R. The green F₂ acting on the pivot point P_F acts downward on the front and center wheels. The blue F₁ acting on the pivot point P_R acts upward on the center and rear wheels. The center wheel receives a downward force F_2M via the front and rear pivot points P_F and P_R, and an upward force F_1M. If the dimensions in Figure 26 are a:b = 1:1, c:d:e:f = 1:1:1:1, then F_1M = F_2M and the wheel load of the center wheel does not change. Therefore, it is possible to set the drive wheels to go over a curb without being affected by the inertial force that occurs. Naturally, it is also possible to change the wheel load of the driving wheels by setting the dimensions a, b, c, d, e, and f.

5. Conclusions

In this study, we investigated how the suspension of a joystick-type six-wheel electric wheelchair can improve its curb-climbing performance. We proposed a driving method suitable for curb-climbing based on the mechanism by which the front wheels (driven wheels) and middle wheels (driven wheels) climb over curbs. As a result, we discussed the possibility of improving the climbing performance by utilizing the inertial force acting on the entire wheelchair.

However, by repeating the curb-climbing tests with a prototype vehicle, we found that the impact when the front wheels hit an obstacle was large and placed a strain on the occupant.

Table 1. Longitudinal acceleration caused by driving speed and curb height.

		Curb Height
		10 [mm]	20 [mm]	30 [mm]	40 [mm]
Wheelchair speed	2.5 [km/h]	0.256 [G]	0.429 [G]	0.781 [G]	0.692 [G]
	4.5 [km/h]	0.428 [G]	0.551 [G]	0.711 [G]	0.697 [G]
	6.0 [km/h]	0.620 [G]	0.932 [G]	1.298 [G]	2.649 [G]

shows the longitudinal acceleration generated in the wheelchair for each combination of electric wheelchair speed and curb height. Contact with a 30 mm curb at 2.5 km/h exceeds 0.7 G, which is a severe value for occupants with weak upper limbs.

Based on the above, we would like to further improve the climbing performance of the wheelchair, and at the same time, conduct research and make suggestions on road environments that enable safe and secure wheelchair driving.