Dynamic Characteristics Analysis and Optimization Design of Two-Stage Helix Planetary Reducer for Robots

Abstract

The dynamic characteristics of high-precision planetary reducers in terms of vibration response and dynamic transmission error have a significant impact on positioning accuracy and service life. However, the dynamics of high-precision two-stage helical planetary reducers have not been studied extensively enough and must be studied in depth. In this paper, the dynamic characteristics of the high-precision two-stage helical planetary reducer are investigated in combination with simulation tests, and the microscopic modification of the gears is optimized by the helix modification with drums, with the objective of reducing the vibration response and dynamic transmission error. Considering the time-varying meshing stiffness of gears and transmission errors, a translation–torsion coupled dynamics model of a two-stage helical planetary gear drive is established based on the Lagrange equations by using the centralized parameter method for analyzing the dynamic characteristics of the reducer. The differential equations of the system were derived by analyzing the relative displacement relationship between the components. On this basis, a finite element model of a certain type of high-precision reducer was established, and factors such as rotate speed and load were investigated through simulation and experimental comparison to quantify or characterize their effects on the dynamic behavior and transmission accuracy. Based on the combined modification method of helix modification with drum shape, the optimized design of this type of reducer is carried out, and the dynamic characteristics of the reducer before and after modification are compared and analyzed. The results show that the adopted modification optimization method is effective in reducing the vibration amplitude and transmission error amplitude of the reducer. The peak-to-peak value of transmission error of the reducer is reduced by 19.87%; the peak value of vibration acceleration is reduced by 14.29%; and the RMS value is reduced by 21.05% under the input speed of 500 r/min and the load of 50 N·m. The research results can provide a theoretical basis for the study of dynamic characteristics, fault diagnosis, optimization of meshing parameters, and structural optimization of planetary reducers.

1. Introduction

Gear tooth shape and other related parameters play a decisive role in the performance of the reducer, and its related parameters affect the basic parameters of the design and transmission characteristics of the basis of the study. Appropriate micro-optimization of gear teeth can effectively improve the transmission performance of the reducer. Therefore, it is essential to study the optimization of gear parameters of high-precision planetary reducers. In this paper, we investigate the dynamic nature of the reducer and the influence of the relevant parameters on its dynamic characteristics, so as to obtain the method of reducing reducer vibration and transmission error, and thus optimize the design of the reducer structure, providing a theoretical basis for optimizing the dynamic characteristics of high-precision planetary reducers and for further research on reducer fault diagnosis, identification, and monitoring.

Industrial robots are increasingly being used in the manufacturing industry for their flexibility and efficiency. Industrial robots mainly repeat the action of a certain process in production, which requires the transmission system to have high positioning and repeat positioning accuracy. The reducer is one of the key components of the transmission system, playing the basic role of reducing the input speed and increasing the torque [1,2]. With the development of industrial robots in the direction of high sophistication, the requirements for precision maintenance ability, vibration and noise, and stability of the robotic planetary reducer are becoming more and more stringent [3]. Due to its characteristics of high rigidity, high precision, high transmission efficiency (with single-stage transmission efficiency close to 98%), and high torque, the planetary reducer has been widely applied in industrial robots, aerospace, marine engineering, mining machinery, etc. [4]. During the working process of the reducer, its dynamic characteristics play an important role in the high-precision planetary reducer’s generation of dynamic excitation responses that trigger vibration and shock. If the vibration excitation is too large, it may lead to excessive noise under complex working conditions, and also cause problems such as broken gear teeth and tooth wear [5].

Planetary transmissions, as a special type of gear transmission, have certain advantages and disadvantages compared to classic gear transmissions. The main advantages are their compactness, large transmission ratios, high efficiency, even load distribution, reduced weight compared to classic transmissions, and effective utilization of the internal space of the transmission [6]. They also perform well in terms of dimensions and efficiency when a lower gear ratio is needed. However, they have no significant advantage at higher gear ratios [7]. The main disadvantages of planetary transmissions are the need for high accuracy during the manufacture and assembly of planetary transmission elements and dynamic imbalance, especially in the case of using a large number of satellites [8].

Scholars have carried out considerable research on the dynamic characteristics of the whole planetary reducer and its transmission system. Kahraman et al. [9] established a three-degrees-of-freedom dynamic model, incorporating the nonlinear radial clearances of rolling bearings and gear pair clearances, analyzing the nonlinear frequency response characteristics of the reducer gear transmission system. Ambarisha et al. [10] analyzed and compared the dynamic responses of reducers using both analytical and finite element models, validating the effectiveness of using lumped parameter models to simulate the dynamic characteristics of planetary gears. Li et al. [11] used the transfer matrix method to establish a mechanical model of torsional vibration of the speed reducer to analyze the dynamic characteristics of the system, but the model only considered the torsional vibration. Wang et al. [12] established a rigid–flexible coupling dynamic model of the reducer and analyzed the vibration characteristics of RV reducers under different operating conditions. Li et al. [13] established a healthy dynamic theoretical model of a three-stage planetary reducer which obtained the theoretical meshing force, and a fault dynamic model of the three-stage planetary reducer under different wear depths of the third-stage sun gear tooth, analyzing the influences of tooth wear depth on the meshing forces at all stages. Du et al. [14] developed a bending–torsion–axial coupled dynamic model of a two-stage transmission system, analyzing the dynamic meshing force and transmission error characteristics of the reducer. Xu et al. [15] considered multi-source error factors such as the geometric shape and positional accuracy of key transmission components, establishing a multi-body system dynamics model of the reducer contact and exploring the dynamic transmission error characteristics under torque loading. Li et al. [16] built a dynamic model of a motor two-stage helical gear transmission system in an electric vehicle at a constant speed, and analyzed the dynamic characteristics after considering the friction and the axial stiffness component. Han et al. [17] developed a translation–torsion nonlinear dynamic model of the transmission system, considering gear clearances, time-varying mesh stiffness, and composite meshing errors, and analyzed the nonlinear dynamic characteristics of the reducer through numerical solutions. Zhou et al. [18] developed linear vibration and nonlinear impact vibration models of the transmission system, calculating and analyzing the dynamic and acoustic radiation characteristics of gear reducers under various operating conditions. Li et al. [19] developed a lumped-parameter dynamic model for RV reducers, considering the tooth profile modification of cycloid gears and system errors, investigating the effects of errors on the dynamic behaviors and transmission precision. Guo et al. [20] developed a nonlinear dynamic model, considering the nonlinear oil film force, time-varying meshing stiffness, dragging torque and friction, and gear backlash with time-varying characteristics, exploring the vibration response and the nonlinear behavior of gear rattle.

However, at present, the research on the dynamic characteristics and structural optimizations of the reducer is mainly focused on the single-stage planetary reducer, RV reducer, or two-stage straight-tooth planetary reducer, and there are fewer studies on the high-precision two-stage helical planetary reducer, which is more and more widely used nowadays.

At the same time, scholars have also conducted many useful studies on the optimization of planetary reducer parameters. Zhang et al. [21] established the lumped mass model of a gear–rotor–bearing system, taking the influence of time-varying stiffness into consideration, studying the dynamic characteristics and radiated noise of helicopter main gearboxes based on finite element. Jin et al. [22] took the dynamic load amplitude of the two-stage gear system and the minimum difference between loads of bearings at both shaft sides as the main objectives, establishing a multi-objective mixed discrete optimization mode. Qin et al. [23] established a multi-objective optimization dynamics model of a multi-stage planetary gear system with system reliability, vibration level of the planetary gear, and system quality as the objective functions, and the discrete variables, such as the number of teeth and the modulus, were solved by using a hybrid discrete-variable combinatorial method. Ghosh et al. [24] proposed to use the graphical method of contour plots and the semi-analytical method for the modification of the tooth profile of a spur gear pair, and the results showed that the vibration caused by geometrical errors such as the tooth profile and pitch errors can be reduced. Xin et al. [25] used non-dominated sorting from the Genetic Algorithm II (NSGA-II), researching the multi-objective optimization problem for a lightweight and low-noise gearbox, reducing the vibrating amplitude and weight of the gearbox. Liu et al. [26] used isolation technology to effectively control the vibration of mechanical systems.

However, most of these studies have focused on optimizing the macroscopic dimensions of meshing gears, and fewer studies have been conducted on microscopic gear reshaping.

In this paper, according to the principle of high-precision planetary reducer’s transmission as well as its structural characteristics, the mutual coupling of the two-stage transmission is considered from the system point of view. Based on the centralized parameter method, the translation–torsion coupling dynamics model of the whole machine is established by simultaneously considering the time-varying meshing stiffness of gears, bearing stiffness, damping, and other factors. A constrained modal analysis of the reducer is carried out, and the inherent frequency and mode shapes of the reducer are obtained. By combining simulations and experiments, the acceleration vibration response characteristic of the high-precision planetary reducer under different loads and rotate speeds is studied, and the transmission error of the reducer under various operating conditions is measured. The paper analyzes the characteristics of the time–frequency domain plots of the reducer’s vibration acceleration signals and explores the impact of rotate speed and load on the dynamic characteristics of high-precision planetary reducers. An optimized design and simulation analysis of two-stage high-precision planetary reducers is performed to investigate the impact of combination trimming on the performance of precision planetary gearboxes, in order to obtain the best combination modification scheme.

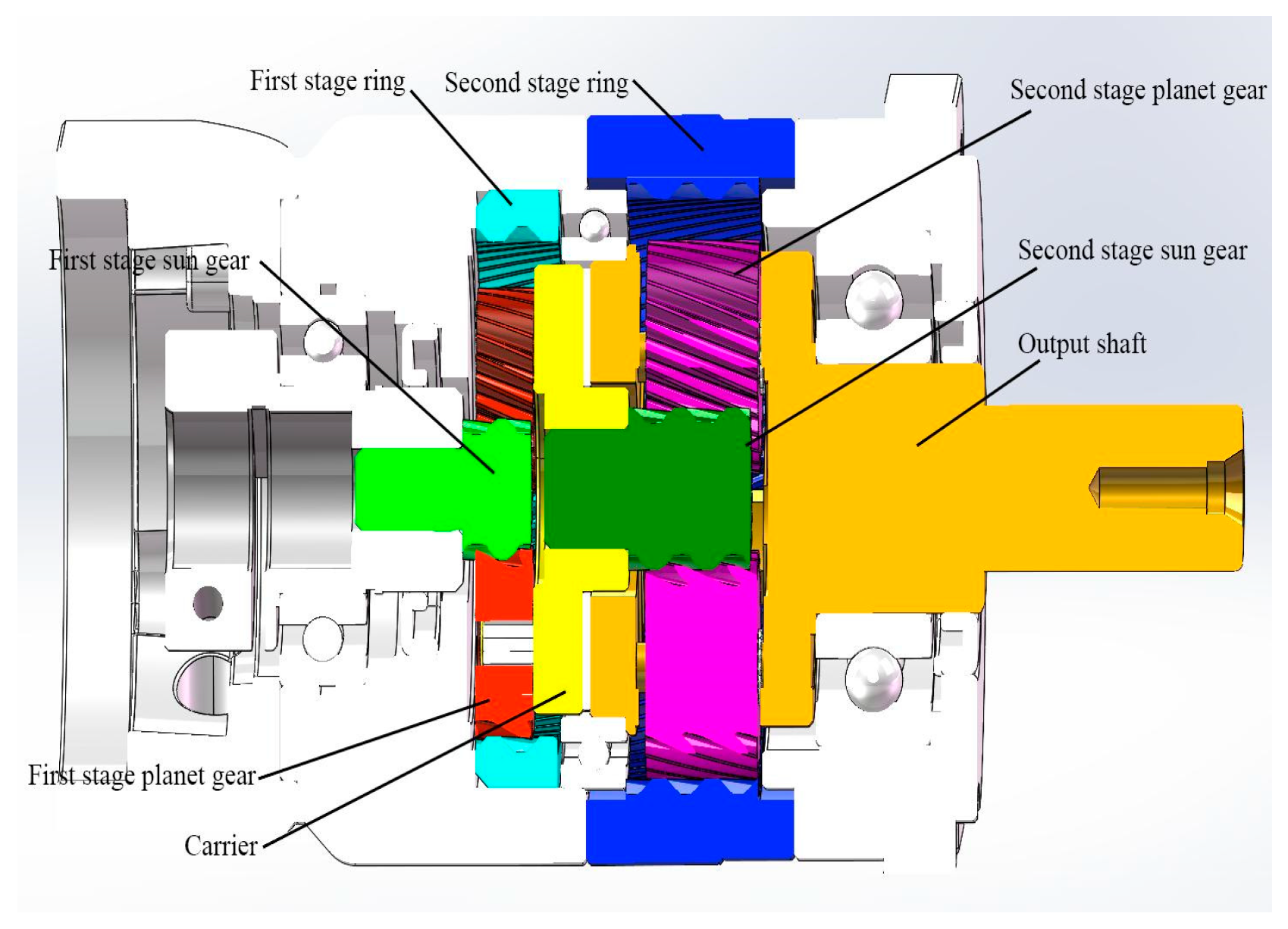
2. Transmission Principle of a Two-Stage Helix Planetary Reducer

The 90AF25-750T3WL planetary reducer of a certain company is mainly composed of four parts [27]:

• Planetary gears, consisting of three identical helical external gears symmetrically installed at 120°.
• The sun gear, the power input component, which is an external gear conjugate to the helical planetary gears.
• The ring gear, which is fixed to the housing and meshed with helical planetary external gears.
• The power output mechanism, comprising power transmission components, where the first-stage power output mechanism is the planet carrier, and the second stage is the output shaft.

The structure of the reducer is shown in Figure 1.

The transmission principle of the precision two-stage planetary reducer is that the drive motor shaft is directly connected to the first-stage sun gear shaft, transmitting power to the first-stage transmission system. Planetary gears mesh simultaneously with the sun gear and the ring gear, rotating around their own central axis while orbiting around the main axis with the first-stage planet carrier, completing the first stage of speed reduction. The planet carrier is connected to the second-stage sun gear through an interference fit. Planetary gears transmit motion via the carrier to the second-stage sun gear. The second-stage sun gear drives planetary gears, which then mesh with the ring gear. The second-stage planetary gears drive the output shaft to orbit, achieving the second stage of speed reduction and transmitting speed and torque to other components. The transmission principle is shown in Figure 2.

3. Establishment of the Dynamic Theoretical Model of a Two-Stage Helix Planetary Reducer

3.1. Basic Assumptions and Coordinate Systems

In order to study the dynamic characteristics of two-stage high-precision planetary reducers, the following assumptions need to be made in order to establish the dynamic model of two-stage planetary gears by the centralized parameter method in general [28,29]:

• Simplify planetary gear drive into a centralized parameter system.
• Only the vibration of components of the planetary gear system in the torsional direction is considered.
• The gear body and carrier are rigid bodies, and the meshing relationship between the gears is simplified as a spring–damping system.
• Ignore the influence of gear tooth side clearance, gravity, and other factors.
• The drive shaft connection between two adjacent planetary gear systems is simplified as a torsion spring.

Based on the two-dimensional planetary gear model established by Lin et al. [25], the two-stage planetary gear system can be simplified based on the above assumptions. The bending–torsion coupling model of a single pair of helical gears and the translation–torsion dynamic schematic diagram of each stage of the planetary gear system are shown in Figure 3.

In the planetary gear system, based on the concept of transformation, to analyze the relative position and motion relationship between the components, the dynamic model of the planetary gear system can be set up in a changing coordinate system, which can be transformed into a fixed-axis gear system to study. Since the system dynamics are analyzed using a dynamic coordinate system that is fixedly connected to the planetary frame, the representation of the position, velocity, and other values of the components requires a variation of the coordinates. The coordinate transformation is shown in Figure 4.

As shown in Figure 4, x_s and y_s are the components of the vector r in the corresponding directions in the fixed coordinate system, and x_c and y_c are the components of the vector r in the corresponding directions in the moving coordinate system, respectively. This can be derived from the schematic diagram [30]:

$$\left\{\begin{array}{l}{x}_c=x_s\cos {\omega }_{c}t+y_s\sin {\omega }_{c}t\\ y_c=-x_s\cos {\omega }_{c}t+y_s\sin {\omega }_{c}t\end{array}\right.$$

(1)

where ω_c is the angular velocity of the carrier/output shaft, and t is the rotation time.

The first-order derivative of Equation (1) is taken to obtain the velocity relation in generalized coordinates:

$$\left\{\begin{array}{l}{\dot{x}}_c={\dot{x}}_s\cos {\omega }_{c}t+{\dot{y}}_s\sin {\omega }_{c}t-{\omega }_c{x}_s\sin {\omega }_{c}t+{\omega }_c{y}_s\cos {\omega }_{c}t\\ {\dot{y}}_c=-{\dot{x}}_s\sin {\omega }_{c}t+{\dot{y}}_s\cos {\omega }_{c}t-{\omega }_c{x}_s\cos {\omega }_{c}t+{\omega }_c{y}_s\sin {\omega }_{c}t\end{array}\right.$$

(2)

where ẋ_s and ẏ_s are the velocity components of the vector r in the corresponding directions in the fixed coordinate system, and ẋ_c and ẏ_c are the velocity components of the vector r in the corresponding directions in the moving coordinate system.

The second-order derivative of Equation (1) is taken to obtain the acceleration relation in generalized coordinates:

$$\left\{\begin{array}{l}{\ddot{x}}_c={\ddot{x}}_s-2{\omega }_c{\dot{x}}_s+{\omega }_c^2{x}_s\\ {\ddot{y}}_c={\ddot{y}}_s+2{\omega }_c{\dot{y}}_s-{\omega }_c^2{y}_s\end{array}\right.$$

(3)

where ẍ_s and${\ddot{y}}_s$ are the acceleration components of the vector r in the corresponding directions in the fixed coordinate system, and ẍ_c and ${\ddot{y}}_{\mathrm{c}}$ are the acceleration components of the vector r in the corresponding directions in the moving coordinate system.

3.2. Mesh Stiffness Excitation and Transmission Error Excitation Analysis

The system dynamic excitation includes inner excitation and external excitation. The inner excitation of two-stage high-precision planetary reducers, including gear mesh stiffness excitation and transmission error excitation, are mainly investigated in this section.

3.2.1. Mesh Stiffness Excitation

The plots of two kinds of mesh stiffness for the gear pairs vary with the change in mesh position. Therefore, the phase angle is used to express the mesh stiffness at different meshing positions. The phase angle γ_spn is defined as the phase difference in the mesh stiffness of the nth planet gear, which is expressed as [19]:

$${\gamma }_{spn}=n_s{\phi }_n$$

(4)

where n_s is the number of sun gear teeth.

Assuming that the mesh stiffness of the gear pairs varies with the rule of the rectangle wave, it can be expanded into a Fourier series:

$$k_{\mathrm{j}n}(t)={\overline{k}}_{sp}+{\displaystyle \sum _{l=1}^{\infty }[C_{spn}^l\cos l{\omega }_m(t+{\phi }_m)+D_{spn}^l\sin l{\omega }_m(t+{\phi }_m)]}$$

(5)

where ${\overline{k}}_{sp}$ is the average mesh stiffness, ω_m is the gear meshing frequency, l is the order of harmonic waves, $C_{\mathit{spn}}^l=a_{\mathit{spn}}^l\cos l{\gamma }_{spn}+b_{\mathit{spn}}^l\sin l{\gamma }_{spn},D_{\mathit{spn}}^l=b_{\mathit{spn}}^l\cos l{\gamma }_{spn}-a_{\mathit{spn}}^l\sin l{\gamma }_{spn}$, a^l_spn and b^l_spn are the amplitude of the harmonic wave with order l, φ_m is the initial phase angle, and j = r, s, c, 1, …, n. r, s, c, and n are the ring, the sun gear, the planetary carrier (in the second stage is the output shaft), and the nth planetary gear, in that order.

3.2.2. Transmission Error Excitation

One of the sun–planet meshes of the first planetary gear stage (i = 1) is considered as the reference mesh. The transmission error of the reference mesh formed by sun–planet 1 is defined in Fourier series form as [31]:

$$e_{sp1}(t)={\displaystyle \sum _{l=1}^L{a}_{spn}^{l(i)}}\sin [l{\omega }_{m}t+{\phi }_{spl}^{(i)}]$$

(6)

where φ_spl is the phase angle of the lth harmonic term, the superscript indicates the gear stage (i = 1, 2), and L is the total number of harmonic terms considered. Defining a phase angle between this reference mesh on the gear set and the reference sun–planet 1 mesh on stage i as Γ⁽ⁱ⁾, the transmission error functions of the gear pair at the ith stage are defined as:

$$\left\{\begin{array}{l}{[e_{spn}(t)]}^{(i)}={\displaystyle \sum _{l=1}^L{a}_{spn}^{l(i)}}\sin [l{\omega }_{mn}t+{\phi }_{spl}^{(i)}+l{Z}_s^{(i)}{\psi }_n^{(\mathrm{i})}+l{\Gamma }^{(i)}]\\ {[e_{rpn}(t)]}^{(i)}={\displaystyle \sum _{l=1}^L{a}_{rpn}^{l(i)}}\sin [l{\omega }_{mn}t+{\phi }_{rpl}^{(i)}+l{Z}_r^{(i)}{\psi }_n^{(\mathrm{i})}+l{\gamma }_{sr}^{(i)}+l{\Gamma }^{(i)}]\end{array}\right.$$

(7)

where ψ_n⁽ⁱ⁾ is the position angle of planet n from the reference planet on stage i, and γ_sr⁽ⁱ⁾ is the phase angle between the sun–planet and ring–planet meshes on stage i. The terms Z_s⁽ⁱ⁾ψ_n⁽ⁱ⁾ represent the phase angles between the sun–planet n mesh and the sun–planet 1 mesh on the same stage i. Likewise, Z_r⁽ⁱ⁾ψ_n⁽ⁱ⁾ is the phase angle between the ring–planet n and ring–planet 1 meshes.

3.3. Formatting of Mathematical Components

3.3.1. Relative Displacements

To establish the motion equations, the relationships in terms of the relative displacement of all the interactional movable components are determined.

1. Relative displacement projection of the sun and planet gears along the mesh line. The relative displacement is obtained [30]:

$${\delta }_{\mathrm{sn}}=({\mathrm{y}}_s\cos {\psi }_{\mathrm{sn}}{-\mathrm{x}}_{\mathrm{s}}\sin {\psi }_{\mathrm{sn}}-{\eta }_n\cos {\alpha }_s-{\zeta }_n\sin {\alpha }_s)\cos \beta +u_s+u_n+e_{spn}$$

(8)

where δ_sn is the projection of the relative displacement between the sun gear and the nth planetary gear in the direction of the external meshing line. α_s is the external meshing angle, β is the helix angle, and e_spn is the transfer error between the sun gear and the planetary gear.

2. Relative displacement projection of the planet gears and the ring along the mesh line. The relative displacement is obtained:

$${\delta }_{\mathrm{rn}}=({\mathrm{y}}_{\mathrm{r}}\cos {\psi }_{\mathrm{rn}}{-\mathrm{x}}_{\mathrm{r}}\sin {\psi }_{\mathrm{rn}}-{\eta }_n\cos {\alpha }_r+{\zeta }_n\sin {\alpha }_r)\cos \beta +u_r-u_n+e_{rpn}$$

(9)

In the equation, δ_rn is the projection of the ring gear’s relative displacement to the nth planet gear in the direction of the internal meshing line. α_r is the internal meshing angle, and e_rpn is the transfer error between the ring and the planetary gear.

3. The displacement equation of the planetary carrier in the X and Y axes with respect to the planetary gear and the planetary carrier body in the tangential direction is obtained:

$$\left\{\begin{array}{l}{\delta }_{\mathrm{c}nx}=x_c-x_n-u_c\sin {\psi }_n\\ {\delta }_{cny}=y_c-y_n-u_c\cos {\psi }_n\\ {\delta }_{cnu}=({\mathrm{x}}_n-x_c)\sin {\psi }_n+(y_n-y_c)\cos {\psi }_n+u_c\end{array}\right.$$

(10)

3.3.2. Motion Equations

According to the Lagrange equation:

$${\displaystyle \frac{d}{dt}}({\displaystyle \frac{\partial L}{\partial \dot{q}}})-{\displaystyle \frac{\partial L}{\partial q}}+{\displaystyle \frac{\partial R}{\partial \dot{q}}}=F$$

(11)

where L= T − U, T is the kinetic energy of the system, and U is the potential energy of the system. Thus [14],

$$T={\displaystyle \frac{1}{2}}\left({\displaystyle \sum I_{\mathrm{j}}{\dot{u}}_j^2+{\displaystyle \sum m_j{\dot{x}}_j^2+{\displaystyle \sum m_j{\dot{y}}_j^2+{\displaystyle \sum m_j{\dot{z}}_j^2}}}}\right)$$

(12)

$$\begin{array}{l}U=[{\displaystyle \frac{1}{2}}({\displaystyle \sum _{\mathrm{i}=1}^3{k}_{sn}{\delta }_{sni}^2+{\displaystyle \sum _{i=1}^3{k}_{rn}{\delta }_{rn}^2}})+{\displaystyle \frac{1}{2}}({\displaystyle \sum _{i=s,r,c}{k}_{xi}{x}_i^2}+{\displaystyle \sum _{i=s,r,c}{k}_{yi}{y}_i^2}+{\displaystyle \sum _{i=s,r,c}{k}_{zi}{z}_i^2}){]}_{{\rm I}}\\ +[{\displaystyle \frac{1}{2}}({\displaystyle \sum _{\mathrm{i}=1}^3{k}_{sn}{\delta }_{sni}^2+{\displaystyle \sum _{i=1}^3{k}_{rn}{\delta }_{rn}^2}})+{\displaystyle \frac{1}{2}}({\displaystyle \sum _{i=s,r,c}{k}_{xi}{x}_i^2}+{\displaystyle \sum _{i=s,r,c}{k}_{yi}{y}_i^2}+{\displaystyle \sum _{i=s,r,c}{k}_{zi}{z}_i^2}){]}_{{\rm I}{\rm I}}\end{array}$$

(13)

where m_i and I_i (i = r, s, c, 1, …n) denote in turn the mass and moment of inertia of the member. ${\dot{u}}_j$, ${\dot{x}}_j$, ${\dot{y}}_j$, and ${\dot{z}}_j$ indicate the vibration velocity of each member. k_sn and k_rn are the meshing stiffness of the sun gear and the ring gear with the planet gear. $k_{xi}$, $k_{yi}$, and $k_{zi}$ (i = s, c) are the radial and axial support stiffness for the support bearing between the sun gear and the carrier.

The differential equations of motion for a two-stage planetary drive system are established as follows [9].

The differential equation of motion of the first-stage sun gear is:

$$\left\{\begin{array}{l}{m}_{\mathrm{s}}^{{\rm I}}({\ddot{x}}_s^{{\rm I}}-2{\Omega }_c{\dot{y}}_s^{{\rm I}}-{\Omega }_c^2{x}_s^{{\rm I}})+{\displaystyle \sum k_{sn}^{{\rm I}}}{\delta }_{sn}^{{\rm I}}\cos \beta \sin {\psi }_{sn}^{{\rm I}}+k_s^{{\rm I}}{x}_s^{{\rm I}}=0\\ m_{\mathrm{s}}^{{\rm I}}({\ddot{y}}_{\mathrm{s}}^{{\rm I}}-2{\Omega }_c{\dot{x}}_{\mathrm{s}}^{{\rm I}}-{\Omega }_c^2{y}_{\mathrm{s}}^{{\rm I}})+{\displaystyle \sum k_{sn}^{{\rm I}}}{\delta }_{sn}^{{\rm I}}\cos \beta \sin {\psi }_{sn}^{{\rm I}}+k_s^{{\rm I}}{y}_s^{{\rm I}}=0\\ (I_s^{{\rm I}}/r_s^{{\rm I}2}){\ddot{u}}_s^{{\rm I}}+{\displaystyle \sum k_{sn}^{{\rm I}}{\delta }_{sn}^{{\rm I}}=T_s^{{\rm I}}/r_s^{{\rm I}}}\end{array}\right.$$

(14)

The differential equation of motion of the first-stage planetary gear is:

$$\left\{\begin{array}{l}{m}_n^{{\rm I}}({\ddot{x}}_n^{{\rm I}}-2{\Omega }_c{\dot{y}}_n^{{\rm I}}-{\Omega }_c^2{x}_n^{{\rm I}})+k_{sn}^{{\rm I}}{\delta }_{sn}^{{\rm I}}\cos \beta \sin {\psi }_{sn}^{{\rm I}}+k_{rn}^{{\rm I}}{\delta }_{rn}^{{\rm I}}\cos \beta \sin {\psi }_{rn}^{{\rm I}}-k_n^{{\rm I}}{\delta }_{cnx}^{{\rm I}}=0\\ m_n^{{\rm I}}({\ddot{y}}_n^{{\rm I}}-2{\Omega }_c{\dot{x}}_n^{{\rm I}}-{\Omega }_c^2{y}_n^{{\rm I}})-k_{sn}^{{\rm I}}{\delta }_{sn}^{{\rm I}}\cos \beta \sin {\psi }_{sn}^{{\rm I}}-k_{rn}^{{\rm I}}{\delta }_{rn}^{{\rm I}}\cos \beta \sin {\psi }_{rn}^{{\rm I}}-k_n^{{\rm I}}{\delta }_{cny}^{{\rm I}}=0\\ (I_n^{{\rm I}}/r_n^{{\rm I}2}){\ddot{u}}_n^{{\rm I}}+{\displaystyle \sum k_{sn}^{{\rm I}}{\delta }_{sn}^{{\rm I}}}-k_{rn}^{{\rm I}}{u}_{rn}^{{\rm I}}=0\end{array}\right.$$

(15)

The differential equation of motion of the carrier is:

$$\left\{\begin{array}{l}{m}_c^{{\rm I}}({\ddot{x}}_c^{{\rm I}}-2{\Omega }_c{\dot{y}}_c^{{\rm I}}-{\Omega }_c^2{x}_c^{{\rm I}})+{\displaystyle \sum k_{cn}^{{\rm I}}}{\delta }_{cn}^{{\rm I}}\cos \beta \sin {\psi }_{cn}^{{\rm I}}+k_c^{{\rm I}}{x}_c^{{\rm I}}=0\\ m_c^{{\rm I}}({\ddot{y}}_c^{{\rm I}}-2{\Omega }_c{\dot{x}}_c^{{\rm I}}-{\Omega }_c^2{y}_c^{{\rm I}})+{\displaystyle \sum k_{cn}^{{\rm I}}}{\delta }_{cn}^{{\rm I}}\cos \beta \sin {\psi }_{cn}^{{\rm I}}+k_c^{{\rm I}}{y}_c^{{\rm I}}=0\\ (I_c^{{\rm I}}/r_c^{{\rm I}2}){\ddot{u}}_c^{{\rm I}}+{\displaystyle \sum k_{cn}^{{\rm I}}{\delta }_{cn}^{{\rm I}}=T_c^{{\rm I}}/r_c^{{\rm I}}}\end{array}\right.$$

(16)

The differential equation of motion of the first-stage ring is:

$$\left\{\begin{array}{l}{m}_r^{{\rm I}}({\ddot{x}}_r^{{\rm I}}-2{\Omega }_c{\dot{y}}_r^{{\rm I}}-{\Omega }_c^2{x}_r^{{\rm I}})+{\displaystyle \sum k_{rn}^{{\rm I}}}{\delta }_{rn}^{{\rm I}}\cos \beta \sin {\psi }_{rn}^{{\rm I}}+k_r^{{\rm I}}{x}_r^{{\rm I}}=0\\ m_r^{{\rm I}}({\ddot{y}}_r^{{\rm I}}-2{\Omega }_c{\dot{x}}_r^{{\rm I}}-{\Omega }_c^2{y}_r^{{\rm I}})+{\displaystyle \sum k_{rn}^{{\rm I}}}{\delta }_{rn}^{{\rm I}}\cos \beta \sin {\psi }_{rn}^{{\rm I}}+k_r^{{\rm I}}{y}_r^{{\rm I}}=0\\ (I_r^{{\rm I}}/r_r^{{\rm I}2}){\ddot{u}}_r^{{\rm I}}+{\displaystyle \sum k_{rn}^{{\rm I}}{\delta }_{rn}^{{\rm I}}=T_r^{{\rm I}}/r_r^{{\rm I}}}\end{array}\right.$$

(17)

The differential equation of motion of the second-stage sun gear is:

$$\left\{\begin{array}{l}{m}_{\mathrm{s}}^{{\rm I}{\rm I}}({\ddot{x}}_s^{{\rm I}{\rm I}}-2{\Omega }_c{\dot{y}}_s^{{\rm I}{\rm I}}-{\Omega }_c^2{x}_s^{{\rm I}{\rm I}})+{\displaystyle \sum k_{sn}^{{\rm I}{\rm I}}}{\delta }_{sn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{sn}^{{\rm I}{\rm I}}+k_s^{{\rm I}{\rm I}}{x}_s^{{\rm I}{\rm I}}=0\\ m_{\mathrm{s}}^{{\rm I}{\rm I}}({\ddot{y}}_{\mathrm{s}}^{{\rm I}{\rm I}}-2{\Omega }_c{\dot{x}}_{\mathrm{s}}^{{\rm I}{\rm I}}-{\Omega }_c^2{y}_{\mathrm{s}}^{{\rm I}{\rm I}})+{\displaystyle \sum k_{sn}^{{\rm I}{\rm I}}}{\delta }_{sn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{sn}^{{\rm I}{\rm I}}+k_s^{{\rm I}{\rm I}}{x}_s^{{\rm I}{\rm I}}=0\\ (I_s^{{\rm I}{\rm I}}/r_s^{{\rm I}{\rm I}2}){\ddot{u}}_s^{{\rm I}{\rm I}}+{\displaystyle \sum k_{sn}^{{\rm I}{\rm I}}{\delta }_{sn}^{{\rm I}{\rm I}}=T_s^{{\rm I}{\rm I}}/r_s^{{\rm I}{\rm I}}}\end{array}\right.$$

(18)

The differential equation of motion of the second-stage planetary gear is:

$$\left\{\begin{array}{l}{m}_n^{{\rm I}{\rm I}}({\ddot{x}}_n^{{\rm I}{\rm I}}-2{\Omega }_c{\dot{y}}_n^{{\rm I}{\rm I}}-{\Omega }_c^2{x}_n^{{\rm I}{\rm I}})+k_{sn}^{{\rm I}{\rm I}}{\delta }_{sn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{sn}^{{\rm I}{\rm I}}+k_{rn}^{{\rm I}{\rm I}}{\delta }_{rn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{rn}^{{\rm I}{\rm I}}-k_n^{{\rm I}{\rm I}}{\delta }_{cnx}^{{\rm I}{\rm I}}=0\\ m_n^{{\rm I}{\rm I}}({\ddot{y}}_n^{{\rm I}{\rm I}}-2{\Omega }_c{\dot{x}}_n^{{\rm I}{\rm I}}-{\Omega }_c^2{y}_n^{{\rm I}{\rm I}})-k_{sn}^{{\rm I}{\rm I}}{\delta }_{sn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{sn}^{{\rm I}{\rm I}}-k_{rn}^{{\rm I}{\rm I}}{\delta }_{rn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{rn}^{{\rm I}{\rm I}}-k_n^{{\rm I}{\rm I}}{\delta }_{cny}^{{\rm I}{\rm I}}=0\\ (I_n^{{\rm I}{\rm I}}/r_n^{{\rm I}{\rm I}2}){\ddot{u}}_n^{{\rm I}{\rm I}}+{\displaystyle \sum k_{sn}^{{\rm I}{\rm I}}{\delta }_{sn}^{{\rm I}{\rm I}}}-k_{rn}^{{\rm I}{\rm I}}{u}_{rn}^{{\rm I}{\rm I}}=0\end{array}\right.$$

(19)

The differential equation of motion of the output shaft is:

$$\left\{\begin{array}{l}{m}_c^{{\rm I}{\rm I}}({\ddot{x}}_c^{{\rm I}{\rm I}}-2{\Omega }_c{\dot{y}}_c^{{\rm I}{\rm I}}-{\Omega }_c^2{x}_c^{{\rm I}{\rm I}})+{\displaystyle \sum k_{cn}^{{\rm I}{\rm I}}}{\delta }_{cn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{cn}^{{\rm I}{\rm I}}+k_c^{{\rm I}{\rm I}}{x}_c^{{\rm I}{\rm I}}=0\\ m_c^{{\rm I}{\rm I}}({\ddot{y}}_c^{{\rm I}{\rm I}}-2{\Omega }_c{\dot{x}}_c^{{\rm I}{\rm I}}-{\Omega }_c^2{y}_c^{{\rm I}{\rm I}})+{\displaystyle \sum k_{cn}^{{\rm I}{\rm I}}}{\delta }_{cn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{cn}^{{\rm I}{\rm I}}+k_c^{{\rm I}{\rm I}}{y}_c^{{\rm I}{\rm I}}=0\\ (I_c^{{\rm I}{\rm I}}/r_c^{{\rm I}{\rm I}2}){\ddot{u}}_c^{{\rm I}{\rm I}}+{\displaystyle \sum k_{cn}^{{\rm I}{\rm I}}{\delta }_{cn}^{{\rm I}{\rm I}}=T_c^{{\rm I}{\rm I}}/r_c^{{\rm I}{\rm I}}}\end{array}\right.$$

(20)

The differential equation of motion of the second-stage ring is:

$$\left\{\begin{array}{l}{m}_r^{{\rm I}{\rm I}}({\ddot{x}}_r^{{\rm I}{\rm I}}-2{\Omega }_c{\dot{y}}_r^{{\rm I}{\rm I}}-{\Omega }_c^2{x}_r^{{\rm I}{\rm I}})+{\displaystyle \sum k_{rn}^{{\rm I}{\rm I}}}{\delta }_{rn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{rn}^{{\rm I}{\rm I}}+k_r^{{\rm I}{\rm I}}{x}_r^{{\rm I}{\rm I}}=0\\ m_r^{{\rm I}{\rm I}}({\ddot{y}}_r^{{\rm I}{\rm I}}-2{\Omega }_c{\dot{x}}_r^{{\rm I}{\rm I}}-{\Omega }_c^2{y}_r^{{\rm I}{\rm I}})+{\displaystyle \sum k_{rn}^{{\rm I}{\rm I}}}{\delta }_{rn}^{{\rm I}{\rm I}}\cos \beta \sin {\psi }_{rn}^{{\rm I}{\rm I}}+k_r^{{\rm I}{\rm I}}{y}_r^{{\rm I}{\rm I}}=0\\ (I_r^{{\rm I}{\rm I}}/r_r^{{\rm I}{\rm I}2}){\ddot{u}}_r^{{\rm I}{\rm I}}+{\displaystyle \sum k_{rn}^{{\rm I}{\rm I}}{\delta }_{rn}^{{\rm I}{\rm I}}=T_r^{{\rm I}{\rm I}}/r_r^{{\rm I}{\rm I}}}\end{array}\right.$$

(21)

where I and II, respectively, are the numbers of stages where the components are located. r_i (i = r, s, c, 1, …n) denotes the radius of the base circle of the member, k_i is the average support stiffness of the member, and T_s, T_c, T_r are the input torque and output torque of the system.

By assembling the matrices and dynamic equations, the motion control equations for the planetary reducer are obtained [30]:

$$M\ddot{x}+C\dot{x}+Kx=F$$

(22)

where x is the displacement, M is the mass matrix, C is the damping matrix, and K is the stiffness matrix.

3.4. Theory of Modal Analysis

Modal analysis can calculate the system’s natural frequencies and mode shapes. These inherent characteristics are crucial for analyzing dynamic characteristics of the reducer. To solve the vibration characteristics of the coupled virtual prototype model, it is necessary to use finite element methods to analyze the inherent characteristics of high-precision planetary reducers. The system’s modal motion control equation is [14]:

$$M\ddot{x}+Kx=F$$

(23)

In modal vibration characteristic analysis, the solution often involves free modes, i.e., F = 0. For free modes, the formula for resonance is:

$$(K-{\omega }_i^{2}M){\phi }_i=0$$

(24)

where ω_i is the inherent mode of the system, and φ_i is the modal shape of the system.

The condition for a non-zero solution of Equation (6) is that the coefficient determinant is equal to 0, i.e.:

$$\left|K-{\omega }_i^{2}M\right|=0$$

(25)

Normalizing the modal matrix and substituting it back into the motion differential equations, the numerical solution for the system’s natural frequencies can be obtained using the block Lanczos algorithm.

4. Establishment of Finite Element Model and Modal Analysis

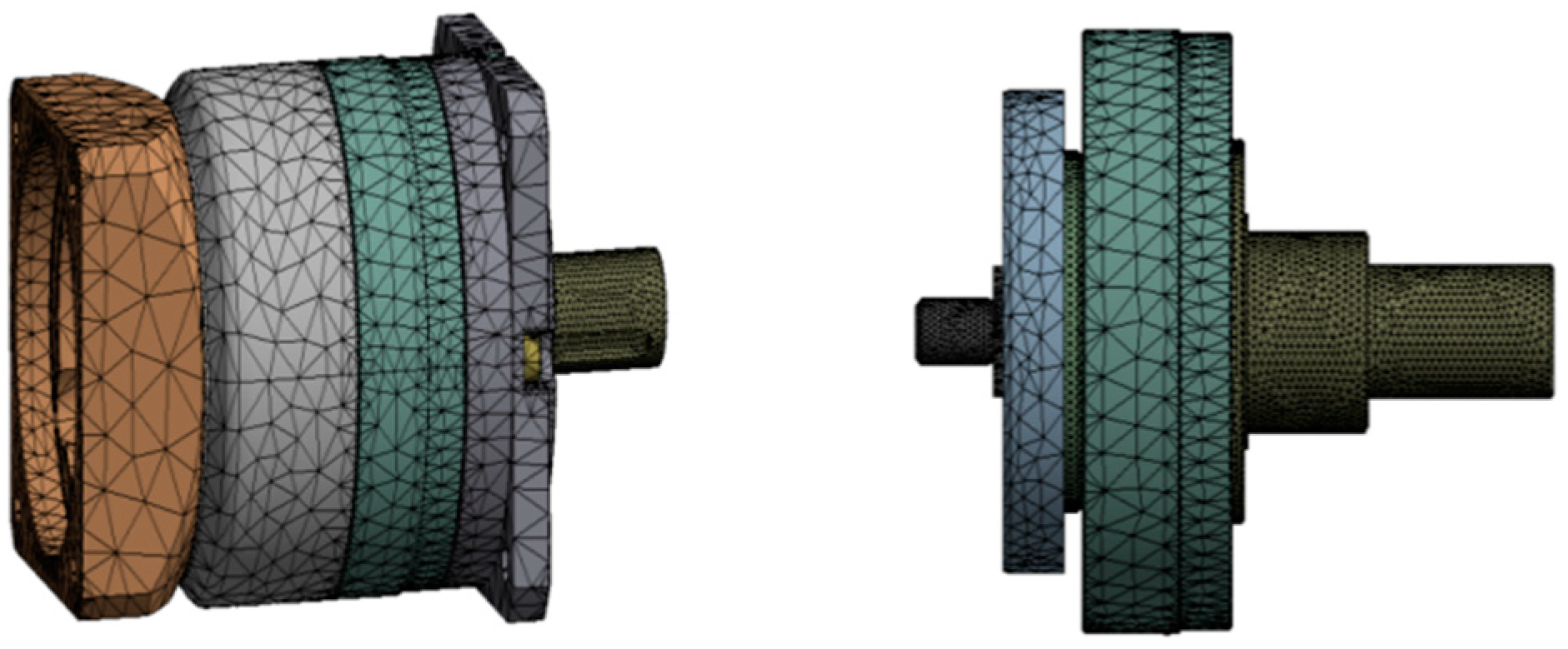
4.1. Establishing the Finite Model

As shown in Figure 5, a 3D assembly model of the precision planetary reducer is established by using Solidworks2023. The main parameters of the prototype are shown in

Table 1. Prototype parameters of the high-precision planetary reducer.

Basic Parameters	Carrier	Sun Gear	Planet Gear	Ring
The parameters of the first-stage planetary gear system
Mass (g)	135.65	22.06	18.97	99.90
Number of teeth	-	18	27	72
Moment of inertia Ii (kg·mm2)	0.4878	0.4849	1.5319	48.817
Base circle diameter (mm)	-	15.32	22.99	60.08
Pressure angle (°)	-	20	20	20
Helix angle (°)	-	20	20	20
Number of planet gears	3
Modulus	0.8
The parameters of the second-stage planetary gear system
Mass (g)	485.64	47.53	56.96	465.52
Number of teeth	-	21	31	84
Moment of inertia Ii (kg·mm2)	144.21	1.6813	5.4764	143.92
Base circle diameter (mm)	-	17.88	26.39	69.205
Pressure angle (°)	-	20	20	20
Helix angle (°)	-	20	20	20
Number of planet gears	3
Modulus	0.8

.

Considering the working conditions of the reducer during the actual working process, the model is appropriately simplified by removing features such as fillets and threads that have less impact on the research content. To shorten calculation time and improve efficiency, the connection type (bearing) is used instead of the actual bearings in the high-precision planetary reducer. The bearing stiffness and damping are calculated according to the literature [32], and the radial and axial support stiffness of the bearings between the planet carrier and the planet gears, and between the output shaft and the planet gears, are set to 5.5 × 10⁹ N/mm and 1.0 × 10⁹ N/mm, respectively. The radial and axial support stiffness of the bearings between the output shaft and the housing are set to 7.0 × 10⁹ N/mm and 4.5 × 10⁹ N/mm, respectively.

A free mesh method with tetrahedral elements is used to mesh the model. To ensure the accuracy of the analysis, dimensional constraints are applied to the components of the two-stage planetary gear transmission system. The mesh sizes for the sun gear and planetary gears are set to 1 mm, while the mesh sizes for the planet carrier and output shaft are set to 1.5 mm. The mesh refinement is applied to the contact areas between the planetary gears and the sun gear, as well as between the planetary gears and the ring gear. The mesh size for the contact surfaces is set to 1.0 mm. The final meshing of the model results in 1,184,116 nodes and 753,455 elements, with the meshing results shown in Figure 6.

4.2. Validation of the Finite Element Model

In order to ensure that the simulation matches the theoretical results, the virtual prototype model of the established high-precision planetary reducer is verified using the gray correlation analysis method. The gray correlation analysis method reflects the degree of similarity between the development and change of two systems, providing a quantitative measurement that can be effectively used for model validity testing. The formula for calculating the gray correlation coefficient between x and y is [12]:

$$\rho ={\displaystyle \frac{\min \left|x_i-y_i\right|+\xi \max \left|x_i-y_i\right|}{\left|x_i-y_i\right|+\xi \max \left|x_i-y_i\right|}}$$

(26)

where ξ is the distinguishing coefficient, which generally takes a value between [0, 1]. In this work, the distinguishing coefficient is set to 0.5. The formula for calculating the relational degree is:

$$r={\displaystyle \frac{1}{N}}{\displaystyle \sum _{i=1}^N{\rho }_i}$$

(27)

When the calculated correlation degree r is greater than 0.5, it can be considered that the two sets of data have a high correlation.

In this paper, the gray correlation analysis is conducted on the reducer model under conditions of constant input speeds of 500, 1000, 1500, and 2000 r/min. When the rotate speed is 1000 r/min and the load is 30 N·m, the output speed is as shown in Figure 7. The output speed curve has some oscillations at the beginning, but subsequently stabilizes around 40.0 r/min with slight oscillations.

The theoretical transmission ratio of the selected high-precision planetary reducer is 25; therefore, the theoretical output speeds corresponding to the set input speeds are 20, 40, 60, and 80 r/min. Five points are extracted from the output shaft speed curves in the simulation for each case, with the data shown in

Table 2. Output speed of simulation.

Input Speed /(r/min)	500	1000	1500	2000
Output speed /(r/min)	19.981	40.009	59.990	80.017
	20.027	39.986	60.013	79.994
	20.004	39.963	59.970	79.971
	19.958	40.009	60.036	80.020
	20.004	40.031	60.008	79.986

. The gray correlation degrees for operating conditions at 500, 1000, 1500, and 2000 r/min are 0.709, 0.779, 0.758, and 0.687, respectively. Since all gray correlation coefficients are greater than 0.5, it can be concluded that the established high-precision planetary reducer model can be applied to further simulations.

4.3. Modal Analysis

Constrained modal analysis of the reducer is performed according to the way the tested high-precision planetary reducer is mounted on the comprehensive performance test rig of the reducer. The material parameters of the main components are shown in

Table 3. Material properties of main parts of planetary reducer.

Part	Material	Density /(kg/m3)	Elasticity Modulus /105 MPa	Poisson’s Ratio
Sun gear	20CrMnTi	7800	2.07	0.25
Planet gear	20CrMnTi	7800	2.07	0.25
Carrier	40Cr	7850	2.0	0.3
Needle roller	GCr15	7830	2.19	0.3

.

The calculated first 10 natural frequencies and modal shapes are presented in

Table 4. First 10 natural frequencies of high-precision planetary reducer.

Order	Natural Frequency/Hz	Modal Shape
1	2193.1	Twisting around the X-axis
2	3155.7	Twisting in plane xoy
3	3529	Twisting in plane xoz
4	4313	Twisting around the X-axis
5	5909.3	Twisting around the X-axis
6	6284.7	Twisting around the X-axis
7	6456.4	Twisting in plane xoz
8	6646.3	Bending in plane xoy
9	7777.7	Bending in plane xoz
10	7988.6	Bending in plane xoy

. Based on the modal shapes, the locations within the planetary reducer that are most likely to experience resonance are the planet gears, the planet carrier, and the output shaft.

5. Analysis of Vibration Characteristics of High-Precision Reducer

5.1. Simulation of Vibration Characteristics

To further understand the vibration characteristics of the high-precision planetary reducer, a vibration simulation analysis of the prototype is conducted using a finite element model.

According to the actual operating conditions of the reducer, the simulation speeds of 500, 1000, 1500, and 2000 r/min are taken in this paper, the simulation time is 1.0 s, and the simulation step size is set to 0.001 s. Since the initial condition of the simulation is that all the parts are at rest, the sudden change of the rotate speed will form a shock, so the individual curves are more variable at the beginning stage and then gradually stabilize. The simulated signals of the stabilized part are intercepted in the paper for subsequent data analysis.

Due to space limitations, only parts of the simulation results are presented. The time-domain and frequency-domain diagrams of vibration acceleration at different speeds with the same load are shown in Figure 8. The time-domain and frequency-domain diagrams of vibration acceleration at the same speed with different loads are shown in Figure 9.

5.2. Test of Vibration Characteristics

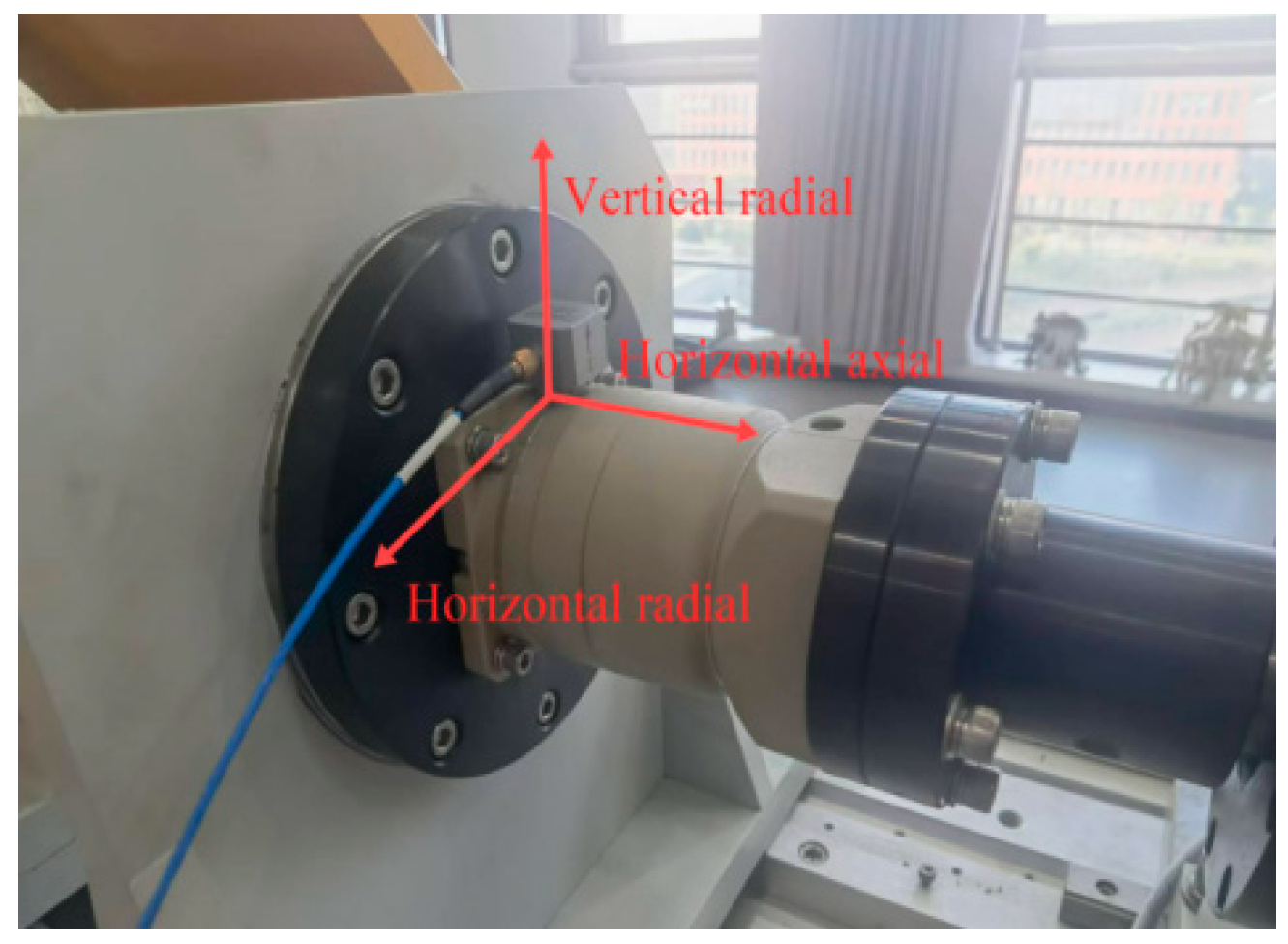
5.2.1. Vibration Testing Method and Equipment

The vibration characteristics of the planetary reducer in this work are tested according to the national standard [33]. The planetary reducer vibration test platform is shown in Figure 10. The input motor is connected to the torque sensor, which is coupled to the input shaft of the planetary reducer, driving the reducer to rotate. The output shaft is connected to the output motor through an angle encoder, torque sensor, and reducer, forming an open-loop system. The vibration response testing equipment mainly uses acceleration sensors to measure vibration acceleration signals, which are amplified and then processed by the signal acquisition and analysis instrument. The key components used in this testing platform are listed in

Table 5. Key components of test stand.

Name	Model Number
Input motor	1FL6062-1AC61-2LA1
Input torque sensor	ZJ-30A
Tested planetary reducer	90AF25-750T3WL
Angle encoder	TS5236N120
Output torque sensor	ZJ-200A
Output reducer	142ZB10-2000T5
Output motor	1FL6090-1AC61-2LA1
Vibration acceleration sensor	CT1010SLFP 211210
Control acquisition instrument	NI cDAQ-9137

.

5.2.2. Measurement Point Arrangement

As shown in Figure 11, a measurement point is set above the reducer housing, with the sensor fixed magnetically. Vibration is measured in the X, Y, and Z directions of the reducer. Considering the forces on the testing platform and the reducer, this study primarily uses acceleration vibration signals in the horizontal radial (X direction) and vertical radial (Z direction).

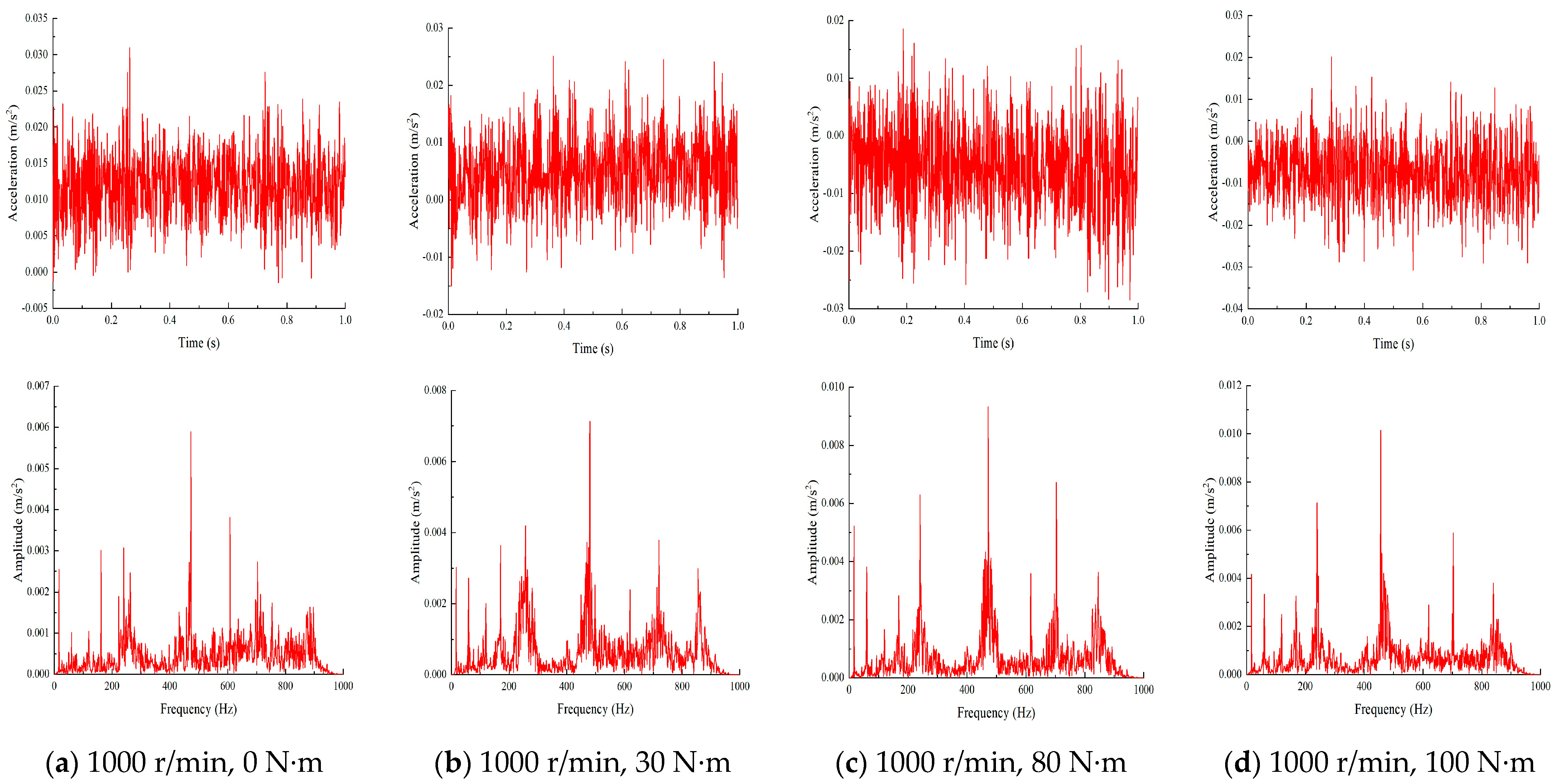
5.2.3. Reducer Vibration Test Results

The test conditions of the speed reducer are 500 r/min, 1000 r/min, 1500 r/min, and 2000 r/min for input speed and 30 N·m, 50 N·m, 80 N·m, and 100 N·m for applied load. Vibration acceleration signals are measured in three directions, with three independent signals collected for each condition, and the average value is taken as the test result. Fast Fourier-transform (FFT) is applied to the time-domain curves of the vibration acceleration response to obtain frequency-domain curves. Under the conditions of 50 N·m load, 500 r/min, 1000 r/min, 1500 r/min, and 2000 r/min, the time-domain and frequency-domain diagrams of Z-direction vibration acceleration of the reducer are shown in Figure 12, which reflect the influence of rotate speed on the vibration characteristics of the planetary reducer. Figure 13 shows the time-domain and frequency-domain graphs of Z-direction vibration acceleration under the conditions of a 1000 r/min speed and loads of 0 N·m, 30 N·m, 50 N·m, 80 N·m, and 100 N·m, reflecting the influence of load on the vibration characteristics of the planetary reducer.

5.3. Analysis of Results

5.3.1. Analysis of Peak-to-Peak Value and Eigenvalue

The peak-to-peak value is the difference between the highest and lowest values of a signal in a cycle, and describes the size of the range of variation in the signal value. The peak-to-peak value is calculated as follows:

$$V_{pp}=\max (\left|x(t)\right|)-\min (\left|x(t)\right|)$$

(28)

where max(|x(t)|) indicates the maximum value of the signal in one cycle, and min(|x(t)|) indicates the maximum value of the signal in one cycle.

RMS is the normalized second central moment of the signal. It is not sensitive to sudden, short-duration, isolated signal peaks. RMS is calculated as follows [34,35]:

$$RMS=\sqrt{{\displaystyle \frac{1}{N}}[{\displaystyle \sum _{\mathrm{k}=1}^N(y_k^2)}]}$$

(29)

where y_k = kth is the sample point of the signal, and N is total number of data points in the data.

The peak-to-peak values of the high-precision planetary reducer under different rotate speeds and load conditions in the test and simulation are shown in

Table 6. Peak-to-peak value under different working conditions.

Load /(N·m)	Speed /(r·min−1)	Simulation Peak-to-Peak Value/(m·s−2)	Test Peak-to-Peak Value/(m·s−2)
0	500	0.016	0.015
	1000	0.032	0.031
	1500	0.065	0.064
	2000	0.080	0.080
30	500	0.018	0.017
	1000	0.040	0.040
	1500	0.067	0.068
	2000	0.087	0.086
50	500	0.022	0.021
	1000	0.064	0.045
	1500	0.078	0.079
	2000	0.093	0.094
80	500	0.02	0.024
	1000	0.050	0.048
	1500	0.084	0.085
	2000	0.099	0.098
100	500	0.029	0.030
	1000	0.053	0.051
	1500	0.088	0.088
	2000	0.104	0.103

, and the RMS values are shown in

Table 7. RMS under different working conditions.

Load /(N·m)	Speed /(r·min−1)	Simulation RMS	Test RMS	Relative Error
0	500	0.0033	0.0034	2.9%
	1000	0.0058	0.0056	3.6%
	1500	0.0102	0.0108	5.5%
	2000	0.0201	0.0198	1.5%
30	500	0.0034	0.0037	8.1%
	1000	0.0060	0.0062	3.2%
	1500	0.0115	0.0114	0.9%
	2000	0.0227	0.0238	4.6%
50	500	0.0035	0.0038	7.9%
	1000	0.0068	0.0070	2.9%
	1500	0.0124	0.0128	3.1%
	2000	0.0250	0.0271	7.7%
80	500	0.0037	0.0039	5.1%
	1000	0.0070	0.0077	9.1%
	1500	0.0139	0.0145	4.1%
	2000	0.0298	0.0319	6.6%
100	500	0.0040	0.0043	7.0%
	1000	0.0073	0.0078	6.4%
	1500	0.0148	0.0159	6.9%
	2000	0.0359	0.0377	4.8%

.

From Table 6 and Table 7, it can be seen that the maximum relative error of the RMS values occurs under the condition of a rotate speed of 1000 r/min and a load of 80 N·m, with a value of 9.1%. Overall, the RMS values of the simulation signals are not significantly different from those of the test signals, indicating that the dynamic model and the finite element model of the reducer can, to a certain extent, accurately reflect the vibration characteristics of the high-precision planetary reducer, which further verifies the accuracy of the model of the reducer.

5.3.2. Spectrum Analysis

In the transmission process, because the inner gear ring is fixed, the meshing frequency in the two gear pairs of sun gear–planet gear and planet gear–inner gear ring is equal. The gear meshing frequency is as follows [36]:

$$f_s={\displaystyle \frac{n_s}{60}}$$

(30)

$$f_c={\displaystyle \frac{f_s}{i}}$$

(31)

$$f_m={\displaystyle \frac{({\mathrm{n}}_s-{\mathrm{n}}_{\mathrm{b}})\times {\mathrm{z}}_s}{60}}$$

(32)

$$f_p={\displaystyle \frac{f_m}{z_n}}$$

(33)

where n_b is the speed of the carrier, n_s is the real rotate speed of the sun gear, and z_s is the number of teeth of the sun gear. f_s is the sun’s rotate frequency, f_c is the carrier’s rotate frequency, f_m is the meshing frequency, and f_p is the planet’s rotation frequency.

The meshing frequencies of the reducer are shown in

Table 8. Frequency of reducer engagement.

Speed (r/min)	500	1000	1500	2000
The meshing frequency of the sun gear	8.33	16.67	25.00	33.33
The meshing frequency of the first transmission system	120	240	360	480
The meshing frequency of the second transmission system	28	56	84	112

.

Since the time step used in the simulation is 0.001 s, which is equivalent to a sampling frequency of 1000 Hz, in order to make it compatible with the test signal, the simulated vibration signal is down-sampled, and the spectrum is obtained by fast Fourier-transform. From Figure 12, it can be seen that when the rotate speed is 1000 r/min, the primary vibration frequencies in the Z direction for the test signals are 16.384, 59.392, 119.808, 167.936, 239.616, 480.256, 623.616, 703.488, and 839.68 Hz. In comparison, from Figure 12, the primary vibration frequencies in the Z direction for the simulation signals are 17.408, 60.416, 118.784, 168.96, 240.64, 480.256, 610.304, 702.464, and 843.776 Hz. The overall peak distribution of the simulation spectrum closely matches the test results.

By observing the main vibration frequencies of the measurement points, it can be concluded that the Z-direction vibration frequencies primarily originate from the first-stage sun gear and the two-stage planetary gear transmission system. The frequencies are 16.384 Hz from the first-stage sun gear rotation frequency excitation; 59.392, 119.808, and 167.936 Hz from the first-stage planetary gear system meshing frequency excitation; and 239.616, 480.256, 623.616, 703.488, and 839.68 Hz from the second-stage planetary gear system meshing frequency and its octave excitation.

5.3.3. Analysis of Influencing Factors

1. Influence of load on vibration of planetary reducer

Figure 12 shows that, at the same rotate speed, different load conditions have little effect on the distribution of characteristic frequencies of the planetary reducer. The primary effect of load on the reducer spectrum is that the peak values of the vibration excitation frequencies increase with the load, while other locations show no significant changes. In this paper, the amplitude of the peak near 240 Hz and 480 Hz is taken as an example to study the load variation and the effect on the frequency spectrum of the reducer. Under the speed of 1000 r/min and different load conditions, the peak values near the excitation frequencies 240 Hz and 480 Hz are shown in Figure 14a. The curves indicate that the peak values at 240 Hz and 480 Hz increase approximately linearly with the load.

The peak-to-peak values of the test signals under different conditions are shown in Figure 14b, and the RMS values are shown in Figure 14c. From these figures, it can be observed that at the same rotate speed, the effect of load on the time-domain characteristics of the high-precision planetary reducer is mainly manifested in the following way: the peak-to-peak value and the RMS value increase with the increase of the load, with the growth being approximately linear.

2. Influence of speed on vibration of planetary reducer

Comparing Figure 9 and Figure 13, it can be seen that when the rotate speed changes, the spectrum of the acceleration vibration signal of the measurement points changes significantly, which is mainly reflected in the change of the main excitation frequency. At a rotate speed of 500 r/min, the overall peak values of the acceleration vibration signals are relatively small. As the speed increases, the peak values of the excitation frequencies in the vibration signals rise rapidly, and the distribution in the frequency domain also changes significantly.

At the same rotate speed, the peak-to-peak and RMS values of the high-precision planetary reducer increase as the load increases. When the load is larger, the growth rate of increase in RMS values accelerates as the speed increases.

By combining this with Figure 14, it can be seen that changes in speed have a greater impact on the peak-to-peak values and RMS values of the vibration characteristic signals than changes in load. Therefore, it can be concluded that rotate speed has a more significant effect on the vibration responses of the high-precision planetary reducer compared to load.

6. Analysis of Transmission Error of High-Precision Reducer

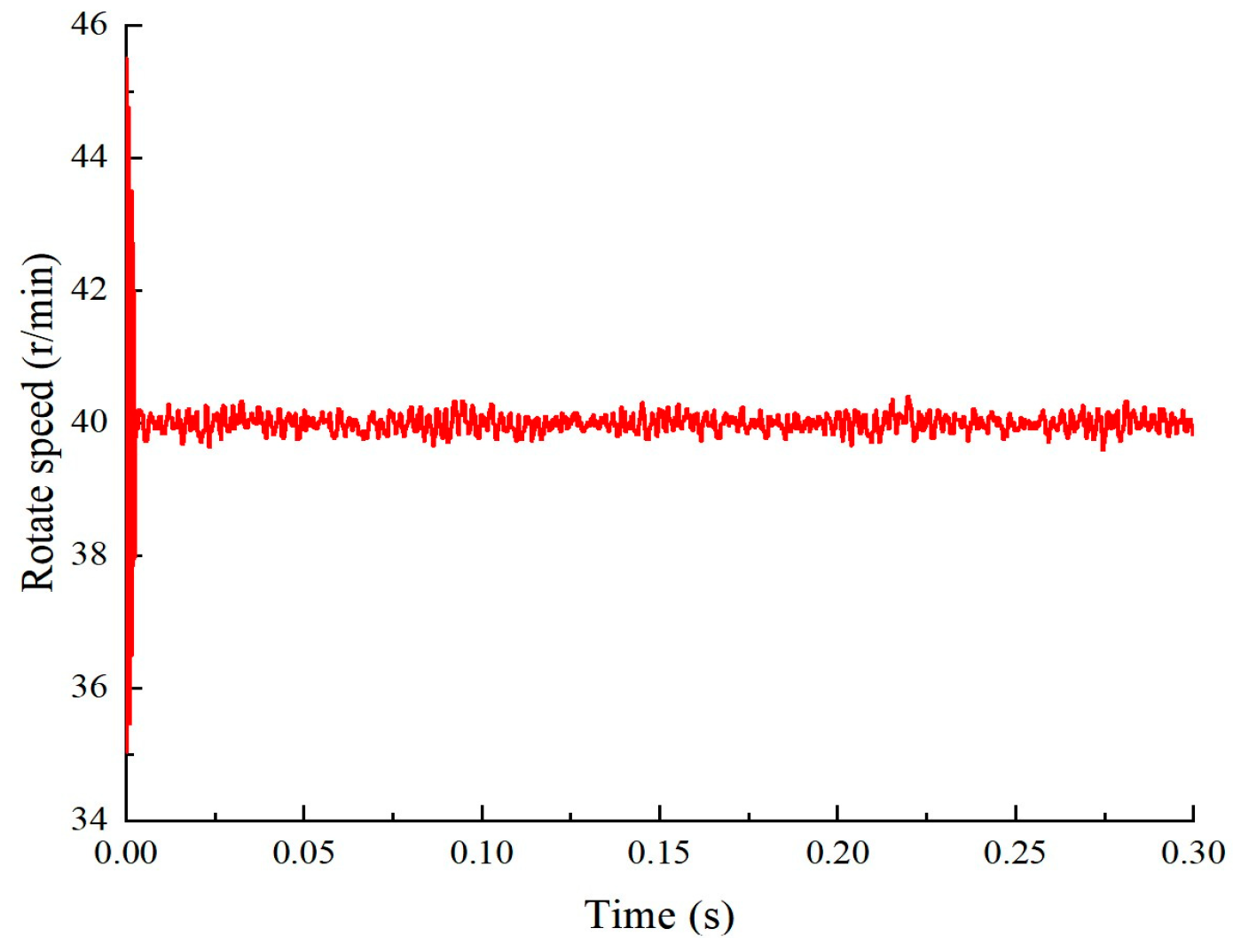
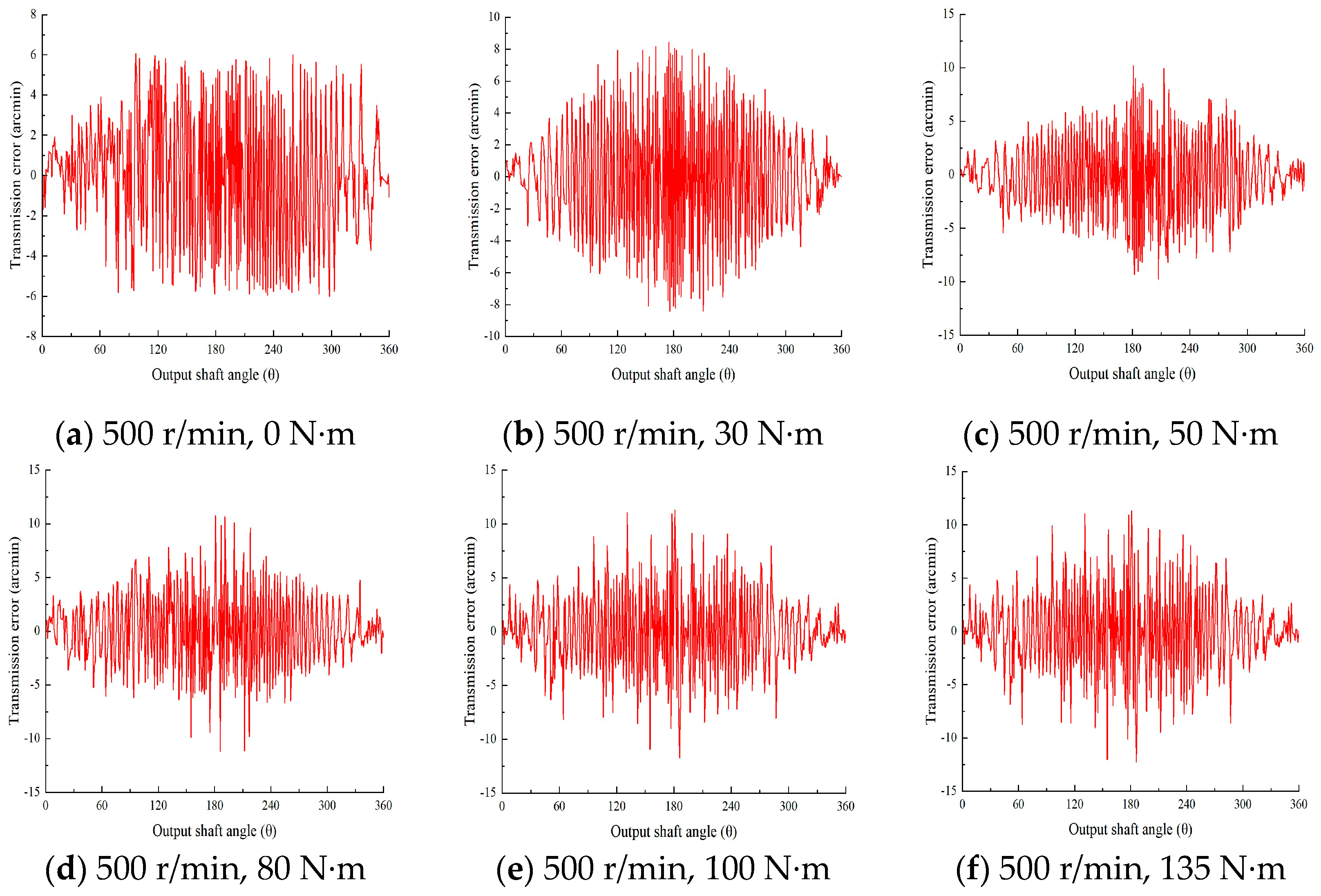
6.1. Test of Transmission Error

At a constant input speed (500 r/min), the load is varied to T = 0, 30, 50, 80, 100 N·m, and the rated torque (135 N·m). The corresponding system transmission error curves are derived from the experimental analysis. The results of the time-domain test of the transmission error of the high-precision planetary reducer are shown in Figure 15.

Under the condition of a load of T = 30 N·m, and the speed changed to 500, 1000, 1500, and 2000 r/min, the test results of transmission error of the reducer are shown in Figure 16.

6.2. Analysis of Transmission Error

Due to the presence of damping, the transmission error increases with the output shaft rotation angle at first and then gradually decreases. The transmission error amplitude under different load conditions is shown in Figure 17a. Under the load condition of T = 30 N·m, the system’s maximum transmission error at different speeds is shown in Figure 17b.

As shown in Figure 17a, the transmission error amplitudes of the high-precision planetary reducer at loads of 0, 30, 50, 80, 100 N·m and the rated torque are 12.06, 16.80, 19.92, 21.90, 22.98, and 23.16 arcmin, respectively. Compared to the no-load case, the maximum transmission error increases by 90.46%. As shown in Figure 17b, at 30 N·m, the transmission error amplitudes corresponding to different input speeds are 16.80, 18.42, 19.80, and 20.94 arcmin, respectively. The transmission error amplitude of the reducer increases with the increase in speed. Compared to the condition of 500 r/min, the maximum transmission error increase at 2000 r/min is 24.64%.

As the load torque and rotate speeds increase, the transmission error amplitude of the reducer also increases, but the rate of growth decreases. The main reason is that the elastic contact deformation between the contact interfaces of the transmission parts of the reducer increases with loads and rotate speeds, leading to a gradual increase in transmission error. However, as the load torque and the speed increase, the fit clearance between the transmission parts decreases and the number of effective contact points increases, resulting in a gradual increase in the overall torsional stiffness of the reducer. Therefore, as the load and rotate speed increase, and with the nonlinear growth of elastic contact deformation, the growth rate of the transmission error amplitude of the reducer gradually decreases.

7. Optimization Design of High-Precision Reducer

7.1. Gear Modification

Gear modification is the process of adjusting and correcting the tooth shape of gears through various machining methods and processes. This modification can be realized by removing material or re-machining the gear surface, with the aim of improving the gear’s meshing characteristics, transmission efficiency, and smoothness of operation. Manufacturing errors in gear production often lead to insufficient assembly accuracy, and gears are deformed after being subjected to force, which further affects the meshing stiffness between the tooth surfaces and causes gear impact. In addition, uneven load distribution will accelerate tooth wear and increase transmission error, which in turn causes vibration and noise of the reducer. The transmission performance of the reducer can be significantly improved by appropriate correction of the micro-parameters of the gear teeth. Gear modification mainly covers two aspects: involute direction modification (i.e., tooth profile modification) and tooth direction correction (along the direction of tooth width).

7.1.1. Modification of Tooth Contours

Tooth profile modification is the adjustment or alteration of the tooth profile of a gear to meet specific design or performance requirements during gear manufacturing. This modification can be achieved by changing the tooth top shape, tooth root shape, or tooth flank curve of the gear. The purpose is to optimize the mesh performance of the gear, reduce noise and vibration during gear transmission, or enhance the load-tcarrying capacity and service life of the gear. Tooth profile modification is usually made in the variable of involute slope.

7.1.2. Modification of Tooth Orientation

Tooth orientation modification, similar to profile modification, is the process of adjusting or changing the tooth orientation (i.e., the axial direction of the gear) of a gear to meet specific design or performance requirements. This modification can be achieved by adjusting the shape of the gear teeth, the shape of the tooth tops and roots, or by changing the tooth flank curves. Of these, drum modification and tooth orientation modification are particularly common, i.e., modification measures in the direction of tooth width. When the amount of drum modification is positive, the material in the center of the tooth width is retained, while the material on both sides of the tooth is removed; conversely, if the amount of modification is negative, the material in the center of the tooth width is reduced, while the material on both sides of the tooth remains unchanged.

The formula for drum modification is:

$$C_{cb}=b_{cal}\tan {\gamma }_d={\displaystyle \frac{b_{cal}{F}_{\beta y}}{b}}$$

(34)

When ${\displaystyle \frac{b_{cal}}{b}}<1$, $b_{\mathit{cal}}=\sqrt{{\displaystyle \frac{2F_{m}b}{F_{\beta y}{C}_y}}}$

$$C_{cb}=\sqrt{{\displaystyle \frac{2F_m{F}_{\beta y}}{B{C}_y}}}$$

(35)

When ${\displaystyle \frac{b_{cal}}{b}}>1$, $b_{\mathit{cal}}=0.5b+{\displaystyle \frac{F_m}{F_{\beta y}{C}_y}}$

$$C_{cb}=0.5F_{\beta \mathrm{y}}+{\displaystyle \frac{F_m}{b{C}_y}}$$

(36)

where $C_y$ is the combined stiffness of gear teeth, $F_{\beta y}$ is the gear mesh stiffness, $F_m$ is the transmitting circumferential force, and b is the gear width.

7.2. Shape Optimization for Gear Teeth

Drum modification is one of the modification methods to eliminate helix meshing load deviation and prevent tooth contact stress concentration due to meshing deformation and manufacturing errors. Generally, tooth contouring and helical drumming can be performed on gears [37]. Tooth contouring is the modification of tooth tops and roots to reduce the peak-to-peak value of the meshing transfer and to reduce the meshing impact due to the base joint error and the load deformation. Helix drum modification is a modification method that removes a thin layer of the tooth surface in the direction of the tooth width to make the tooth surface into a drum shape. Helix modification with drum shape refers to both tooth contouring and helix modification of gears. This combined modification has the advantages of both tooth contouring and helix modification with drum shape, which effectively reduce the effect of meshing deformation on gear meshing [38].

The principle of helix trimming with a drum is shown in Figure 18, where C_α is the helix modification volume, F_βy is the meshing skew, b_cal is the effective tooth width of meshing, C_h is the drum trim volume, and δ^’ is the contact deformation.

The specific optimization steps are: A three-dimensional model of the two-stage high-precision planetary reducer is established, and the combined modification of the helix with a drum is used in KiSSsoft2024 for optimization. Then, the overall wheel system of the two-stage high-precision planetary reducer is simulated and analyzed, and the dynamic response and transmission error of the reducer before and after modification are compared.

Load distribution and maximum Hertzian contact stress are important indicators for the study of gear transmission smoothness. The maximum Hertzian contact stress is related to tooth mesh wear and gear life, and uneven tooth load distribution K_Hβ will trigger pitting and gluing problems on the meshing tooth surface, which may lead to serious tooth root fracture and failure of the whole transmission system. Numerous studies have proved [39,40] that the vibration acceleration of the planetary drive system can be effectively reduced by reducing the maximum Hertzian contact stress and the stress concentration on the tooth surface.

In order to obtain the most suitable profile solution, it is necessary to use a reasonable combination of profiling methods, and these two parameters are used to determine the effect of the trimming method on the gear meshing performance.

Comparative analysis of tooth profile drum trimming and helix trimming with drums is carried out, and the reducer gear system is trimmed with a trimming increment of 1 μm per step; the results are shown in Figure 19 and Figure 20.

Comparison of Figure 19 and Figure 20 shows that the effect of deformation of the support device on gear meshing is reduced due to the increased helix modification with drum shape. The maximum Hertzian contact stress and tooth load distribution coefficient at the tooth meshing are smaller than the general tooth contouring with drum. The iteration steps at which the maximum Hertzian contact stress and tooth load distribution coefficient of a general tooth contour modification with drum shape are minimized are step 81 and step 104, respectively, while helix modification with drum shape is basically minimized at step 60. Therefore, compared with tooth contouring with drum shape, helix modification with drum shape can iterate faster to obtain the optimal solution of modification, which can reduce the contact stress and tooth load distribution coefficient and improve the dynamic characteristics of the high-precision reducer. In this paper, the helix modification with drum shape is adopted, and the optimal modification amount is as follows: −0.8 μm helix modification with 1.8 μm for high-speed class sun gear profile; 1.2 μm helix modification with −1.8 μm for low-speed class sun gear profile.

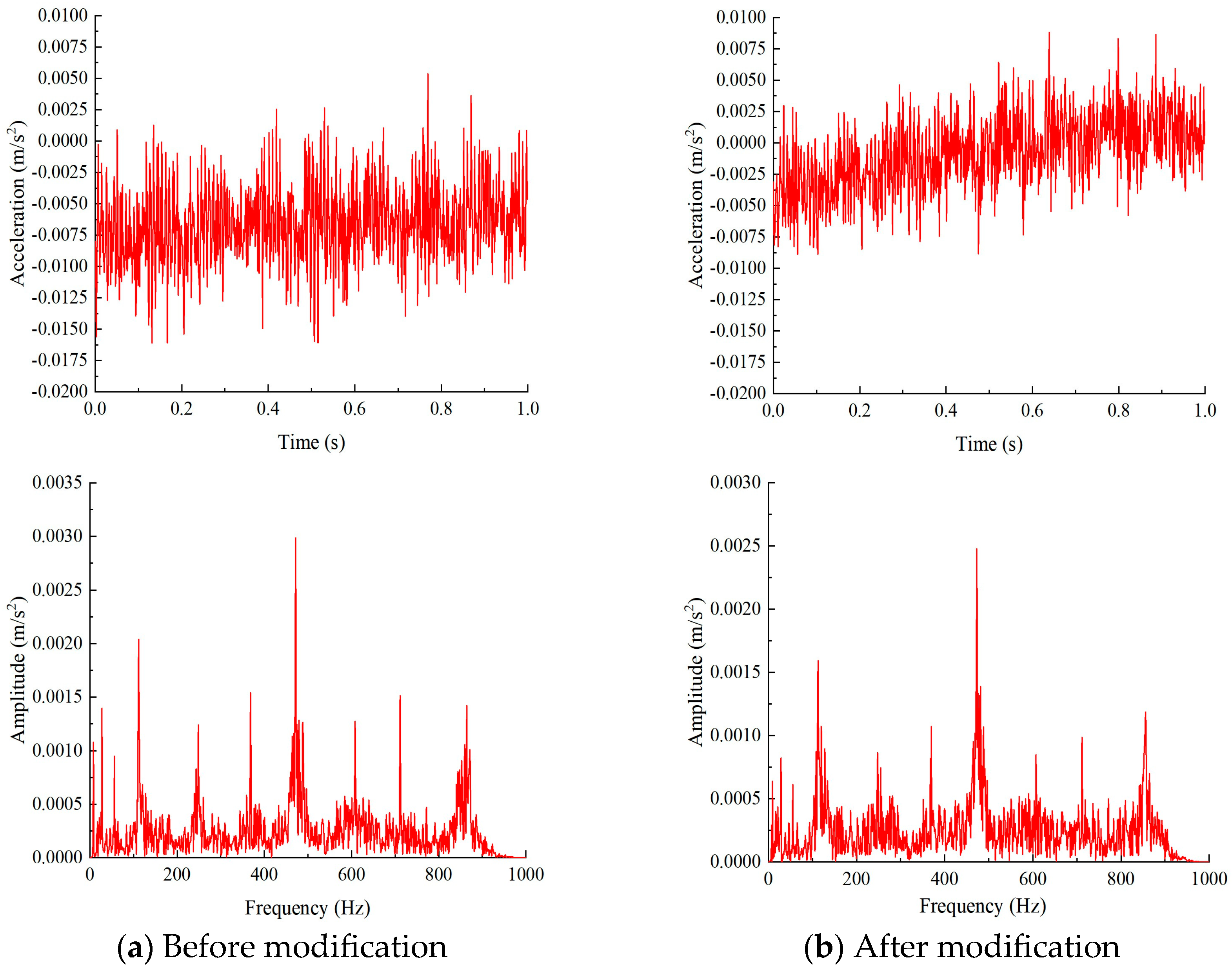
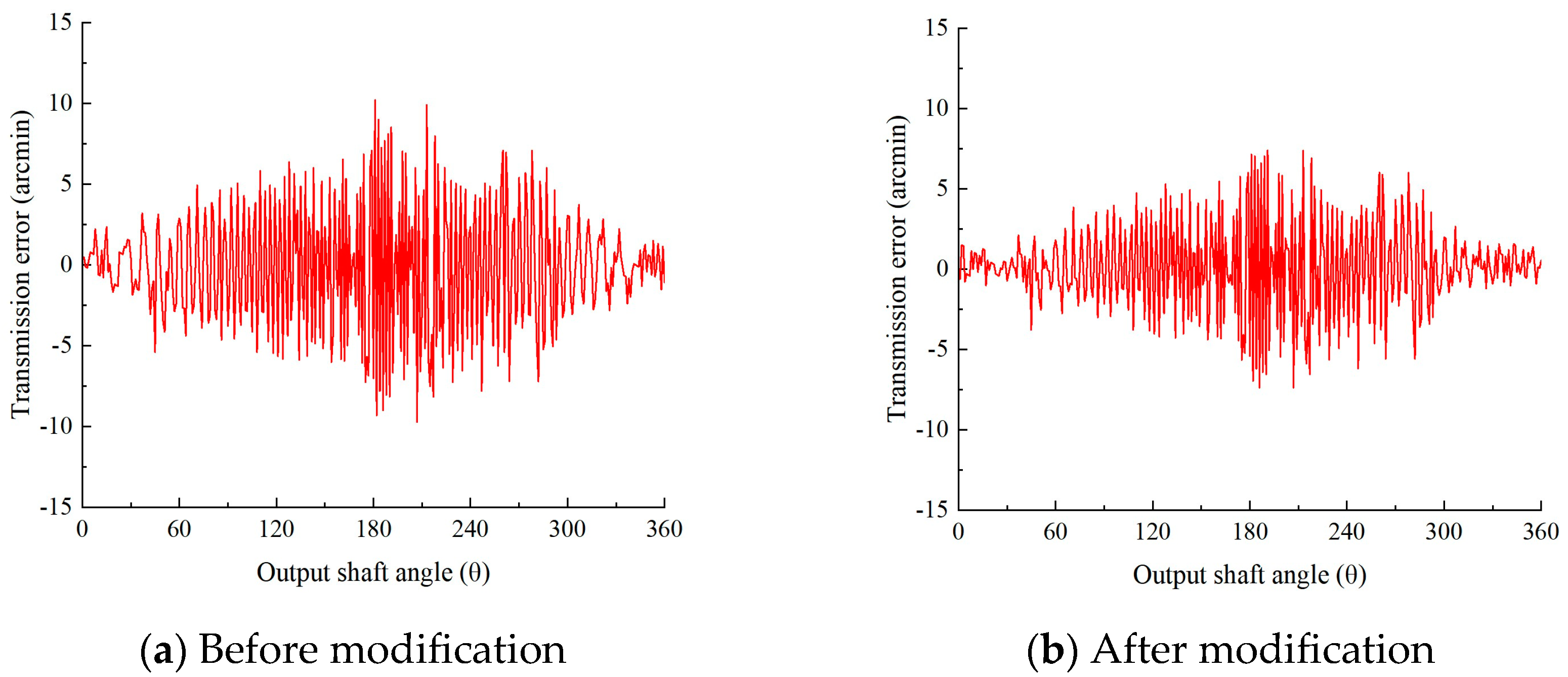
7.3. Simulation Analysis

According to the gear modification scheme determined above, the gear reducer is optimized for gear tooth modification. Through the vibration acceleration and transmission error simulation analysis, we compare the dynamic characteristics of the reducer before and after the modification, and verify the modification effect.

The dynamics of the virtual prototype model after modification are simulated and analyzed, and the input speed is set to 500 r/min and the load to 50 N·m. The time-frequency domain comparison of the vibration acceleration of the gear reducer before and after the modification is shown in Figure 21, and the comparison of the transmission error is shown in Figure 22 through the dynamics simulation.

As can be seen from Figure 21 and Figure 22, the helix modification with drum shape reduces the peak-to-peak value of transmission error from 18.42 arcmin to 14.76 arcmin, the peak-to-peak value of vibration acceleration from 0.021 m/s² to 0.018 m/s², and the RMS value from 0.0038 to 0.0030. The amplitude of vibration frequency of the reducer is also decreased. The decrease in the peak-to-peak value of the transmission error reduces the noise of the overall transmission system, and the decrease in the peak-to-peak and RMS values of the vibration acceleration curves reduces the shock of the transmission system and makes the transmission system smoother.

8. Conclusions

The dynamic characteristics of high-precision two-stage helical planetary reducers have a significant impact on the positioning accuracy and service life of industrial robots. Based on the Lagrangian equation and using the centralized parameter method, the translation–torsion coupled dynamics model of the two-stage helical planetary reducer is established by considering the time-varying meshing stiffness of gears and transmission error, and the optimization scheme of gear tooth combination modification with drum-shaped helix is proposed with the optimization objectives of reducing the vibration acceleration of the whole machine and reducing transmission error. The main conclusions are as follows:

• The first 10th-order intrinsic frequency and modal shapes of a two-stage gear train of a high-precision planetary reducer in the meshing state are simulated. The analysis results show that the most likely resonance locations in the planetary reducer are the planetary gears, the planetary carrier, and the output shaft.
• Vibration tests are conducted on the high-precision planetary reducer using a comprehensive performance testing platform. The acceleration vibration signals of the high-precision planetary reducer at different rotate speeds and torques are also obtained through dynamic simulation. Analysis of the simulation and test signals indicates that the vibration of the high-precision reducer is influenced by both load and speed. The load mainly affects the time-domain distribution of the vibration signal, with relatively minor effects on the frequency-domain distribution, whereas the speed has a significant impact on both.
• Based on the experimental test, as the load torque increases, the transmission error amplitude of the reducer increases, but the amplitude growth rate is gradually decreased. The amplitude of transmission error at no load is 12.16 arcmin, and the amplitude of transmission error at rated torque is 23.16 arcmin, which is an increase of 90.46%.The amplitude of transmission error at 500 r/min is 16.80 arcmin, and the amplitude of transmission error at 2000 r/min is 20.94 arcmin, which is an increase of 24.64%. Therefore, load has a large impact on the transmission error, while the input speed has a relatively small impact on the transmission error, so a variety of factors should be considered in the design.
• Using the helix modification with drum shape, the peak-to-peak value of the transmission error of the gearbox is reduced from 18.42 arcmin to 14.76 arcmin, a reduction of 19.87%, and the peak-to-peak value of the vibration acceleration is reduced from 0.021 m/s2 before the modification to 0.018 m/s², a reduction of 14.29%, for an input speed of 500 r/min and a load of 50 N·m. The RMS value is reduced from 0.0038 before the modification to 0.003, a reduction of 21.05%; The RMS value is reduced from 0.0038 before shape offset to 0.0030, which is 21.05% lower. The helix modification with drum shape improves the transmission accuracy of the overall gear system, reduces the noise in the transmission, and makes the gear system transmission smoother. It has greater accuracy than previous optimization design methods, can obtain the optimal solution quickly and iteratively, improves the design efficiency and design quality, has better practical value, and provides a relevant basis for exploring the combined modification.

The optimization scheme adopted in this paper belongs to microscopic modification optimization, and thus does not make large adjustments to the macroscopic size of the gear and the overall structure of the reducer. In subsequent research, the optimization design can be further improved with the objectives of lightweight structure, higher transmission efficiency, and so on.