Decoupled Multi-Loop Robust Control for a Walk-Assistance Robot Employing a Two-Wheeled Inverted Pendulum
Abstract
:1. Introduction
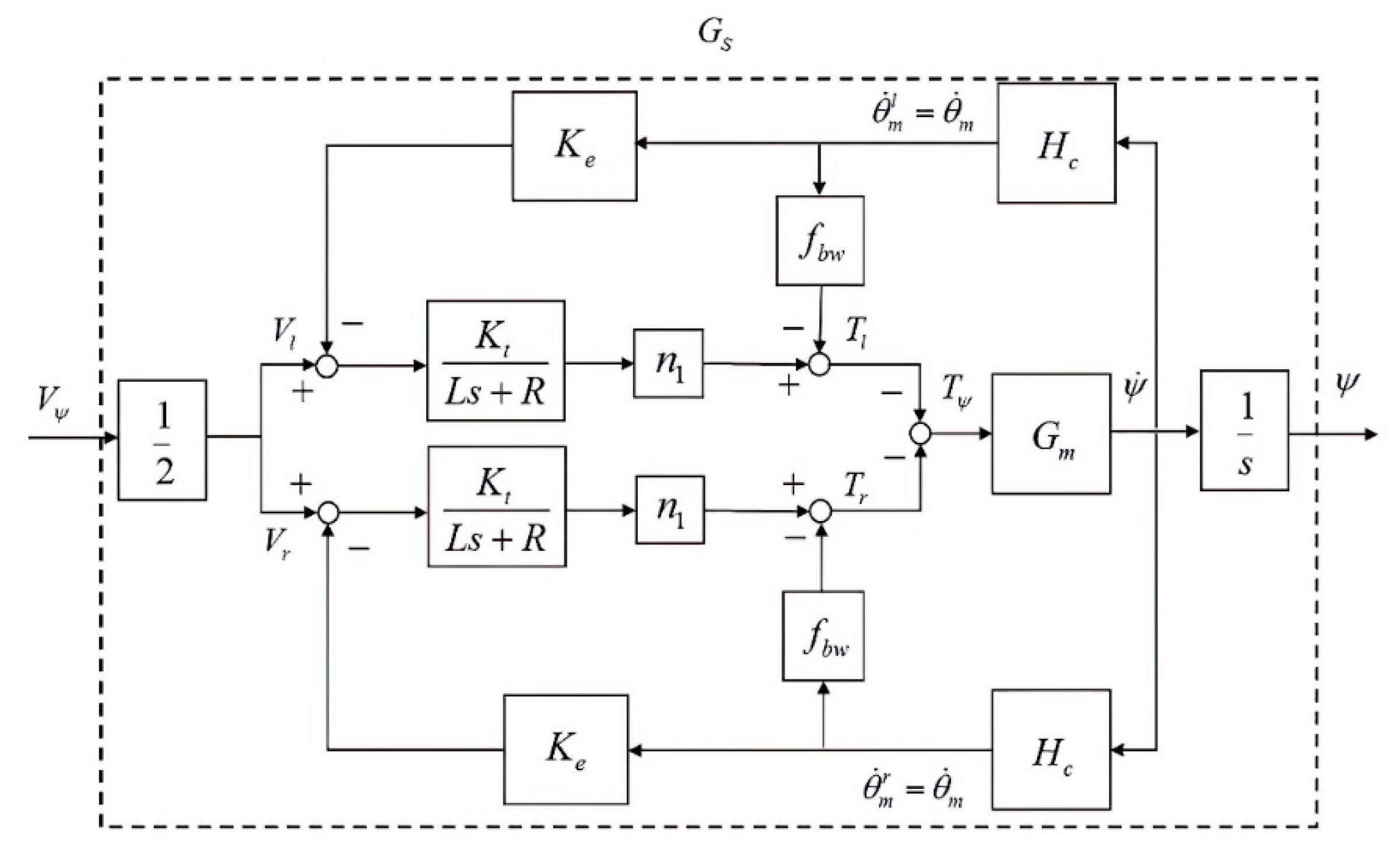
2. System Description and Modeling
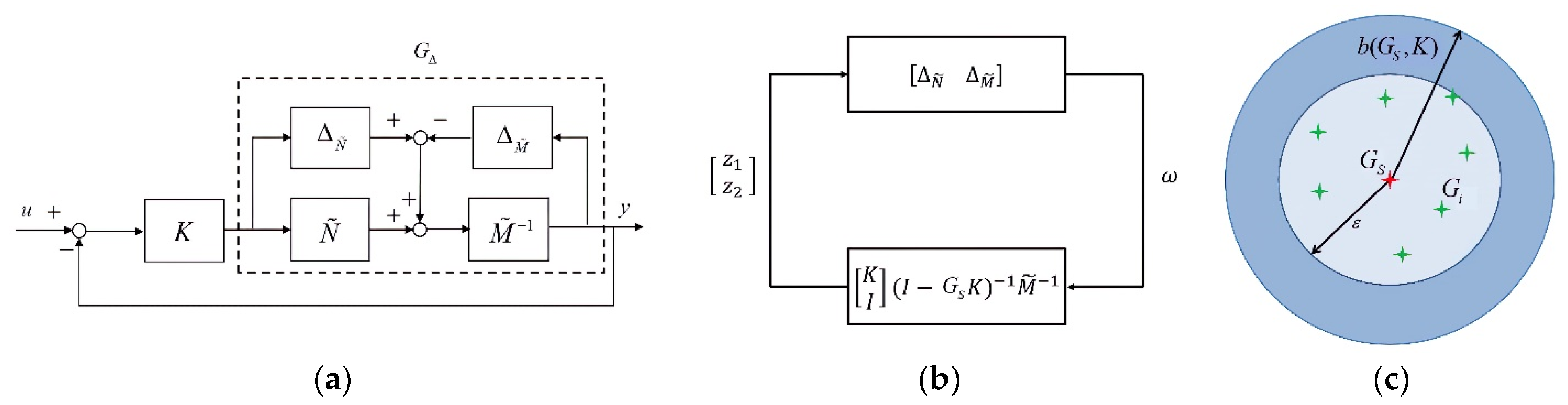
3. Robust Loop-Shaping Control Design for System Stability
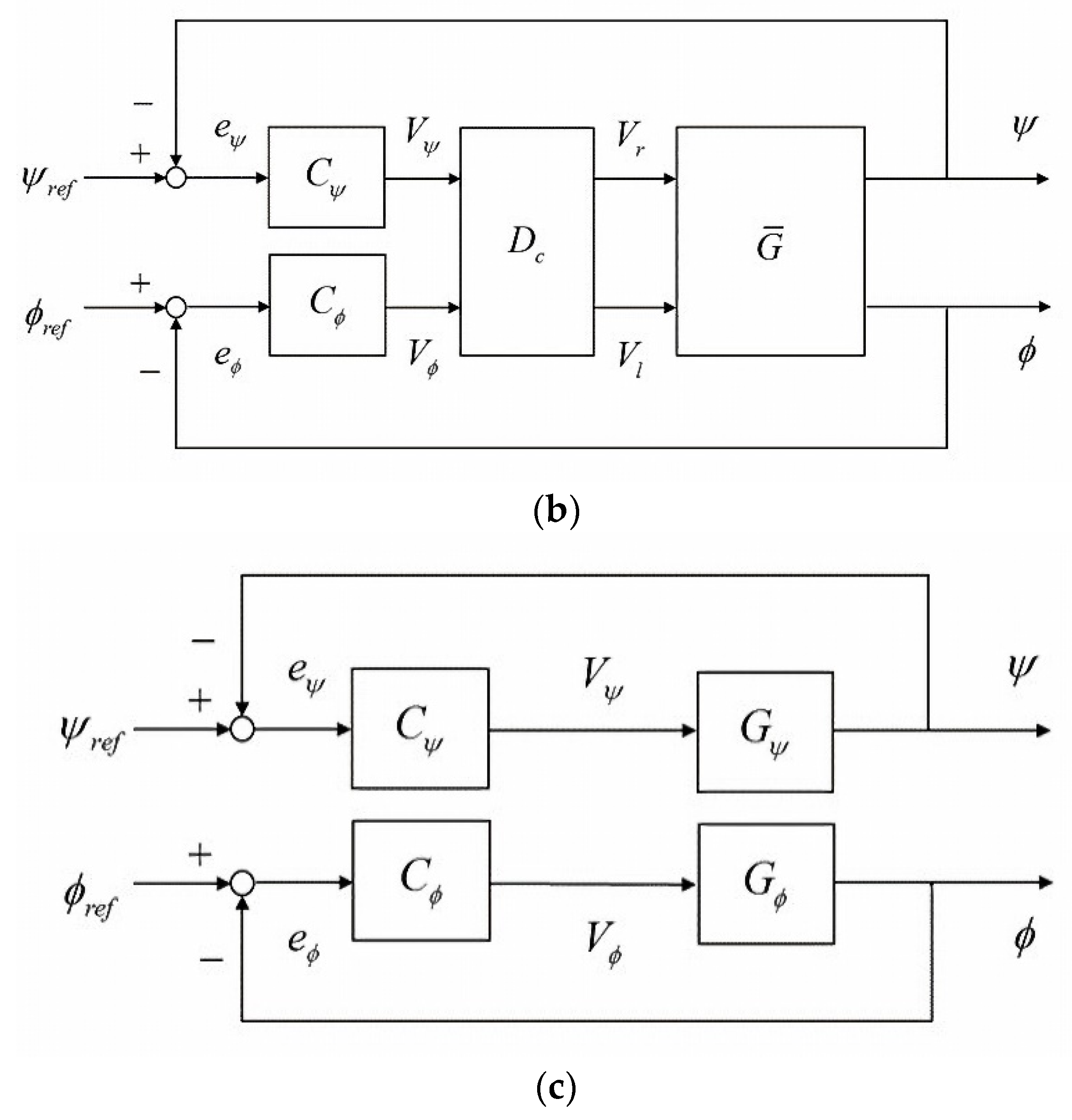
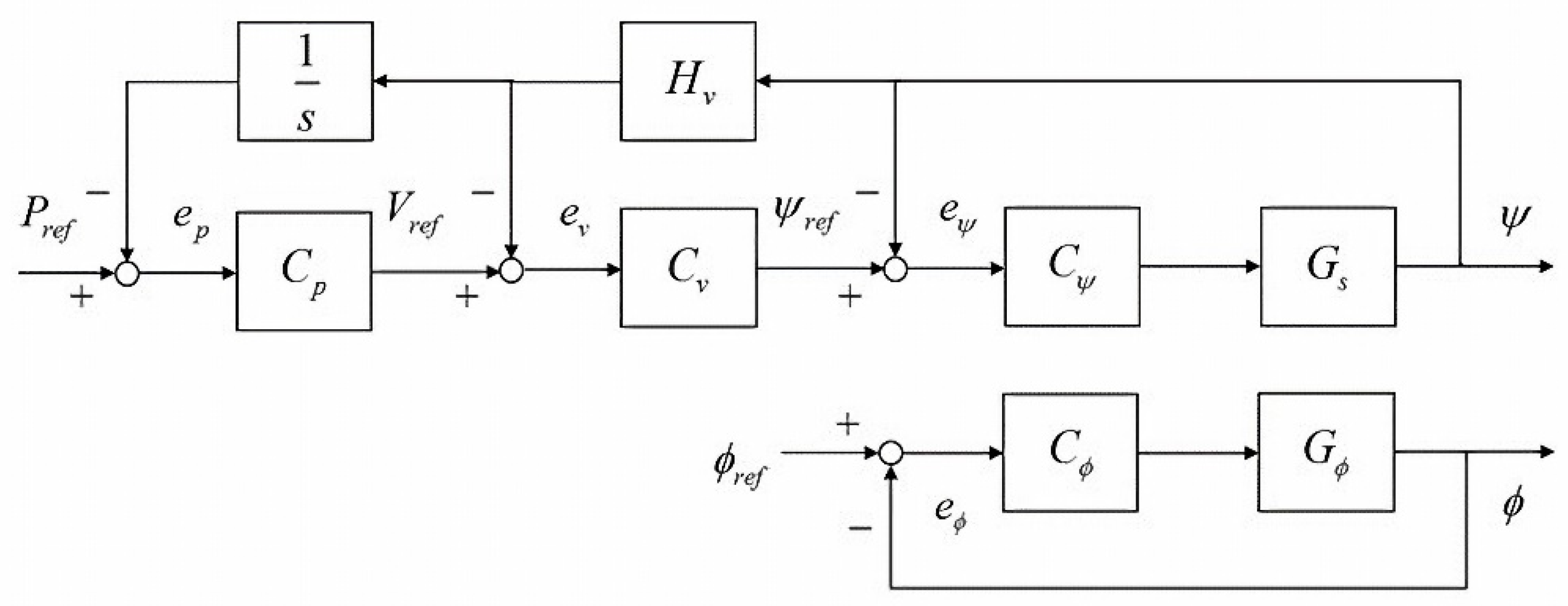
4. The Multi-Loop Control Structure
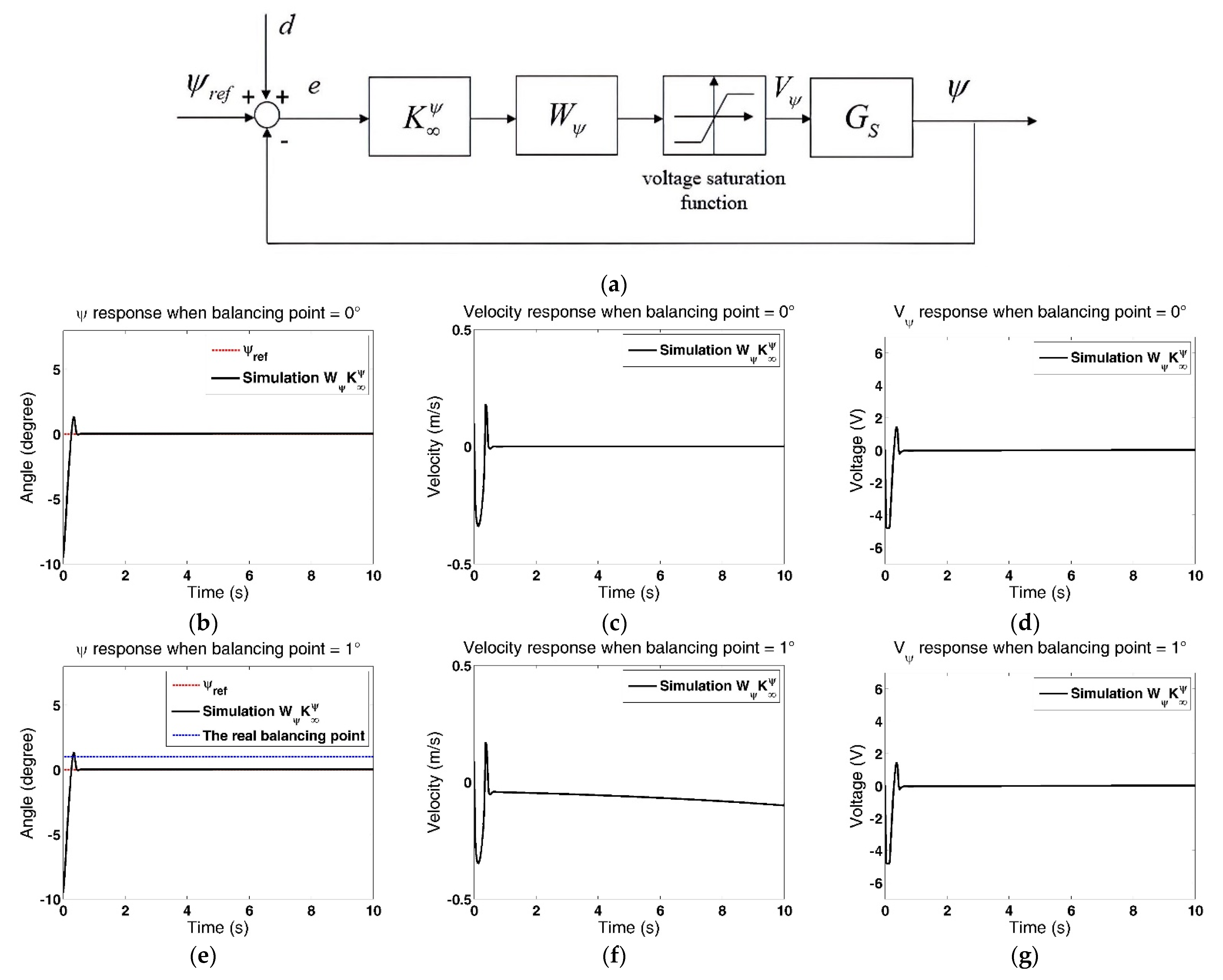
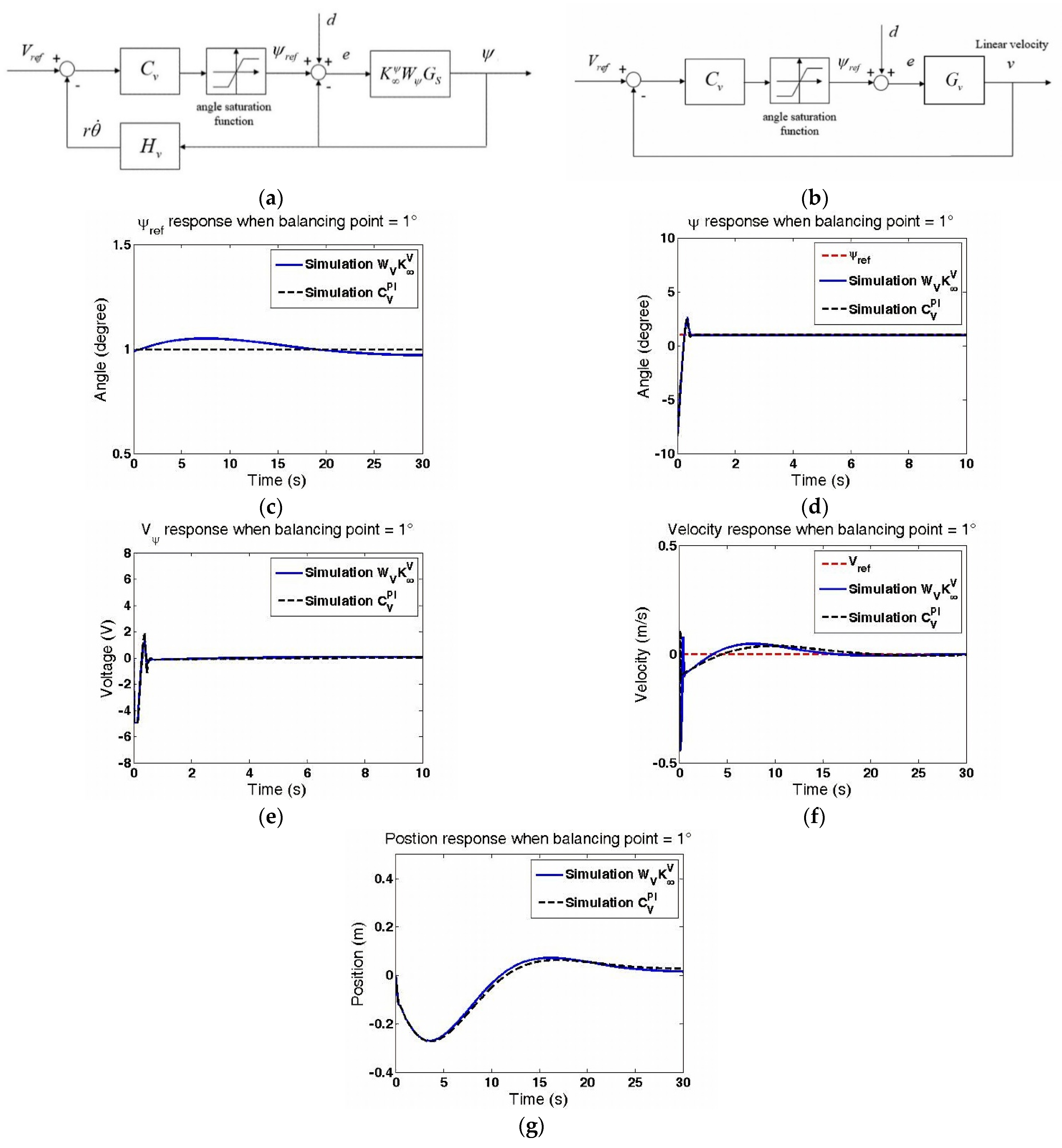
4.1. The Velocity Loop Control
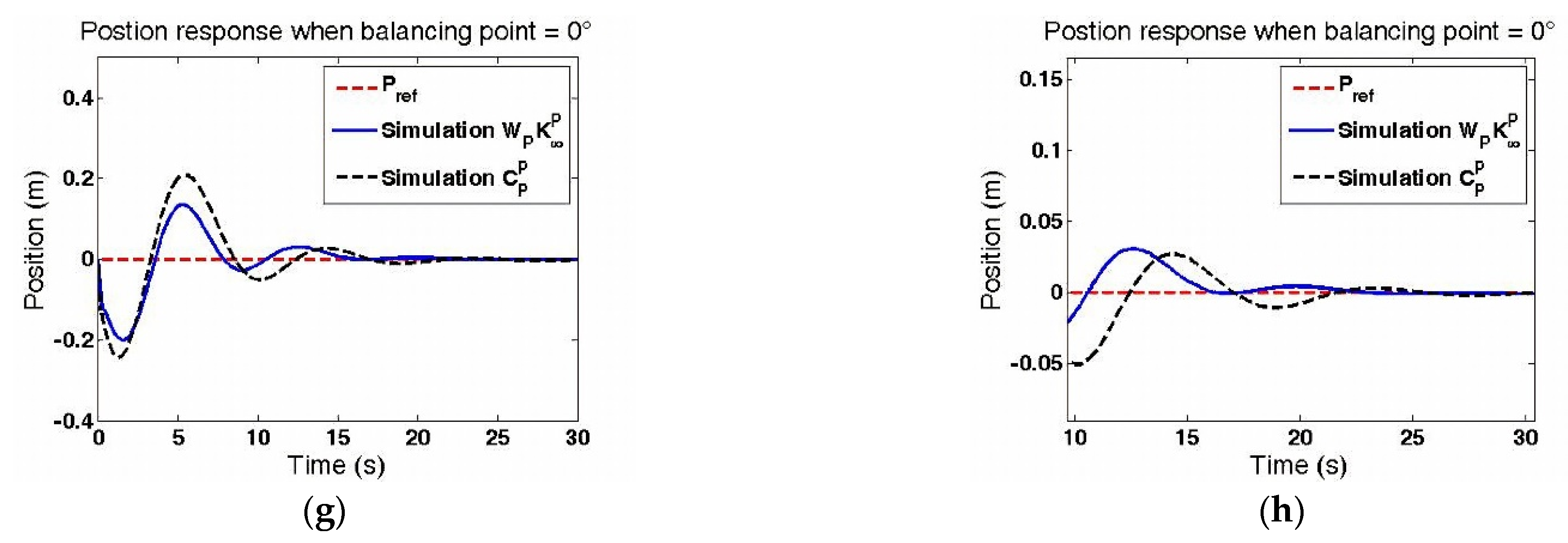
4.2. The Position Loop Control
5. Decoupled Control Loops
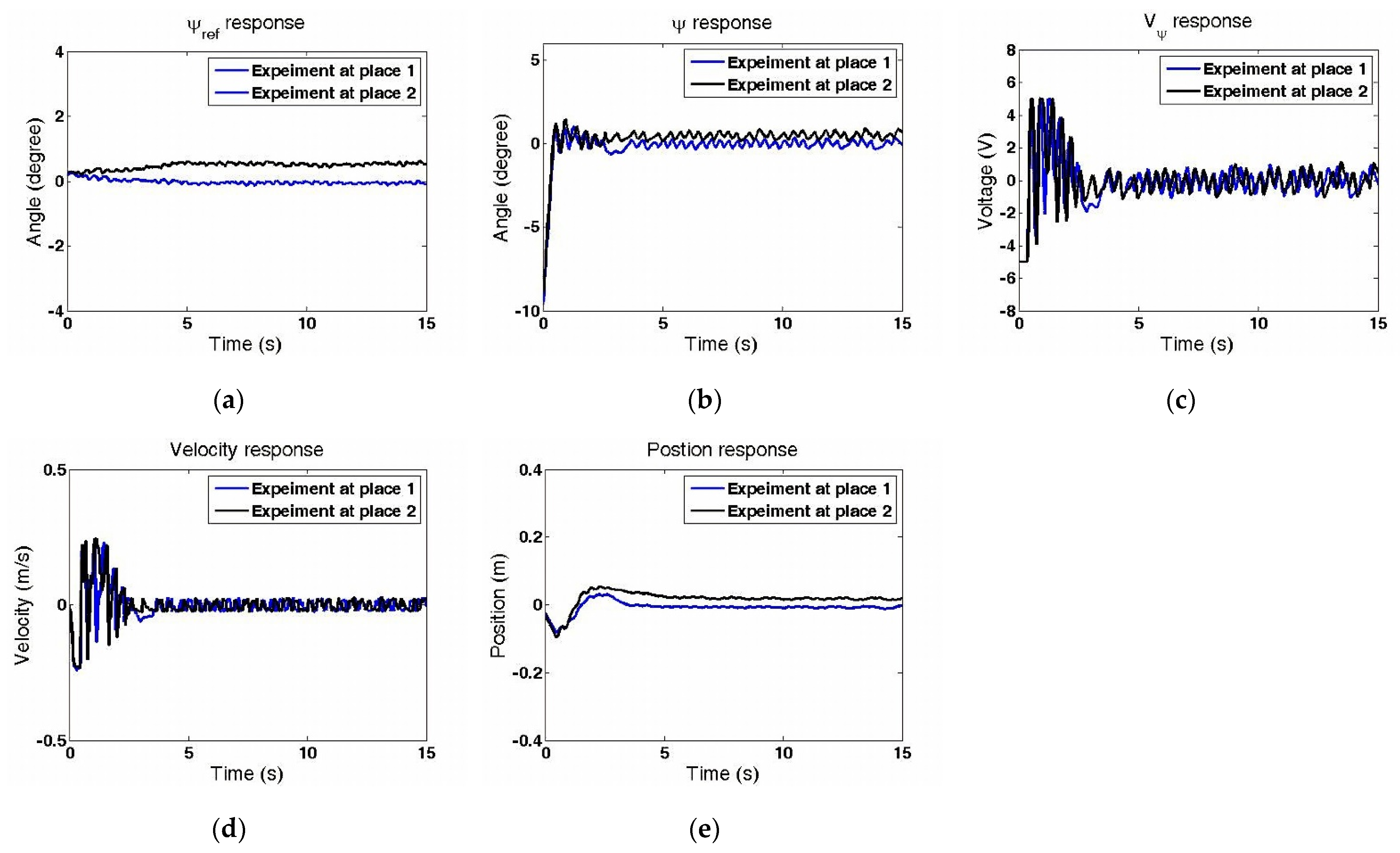
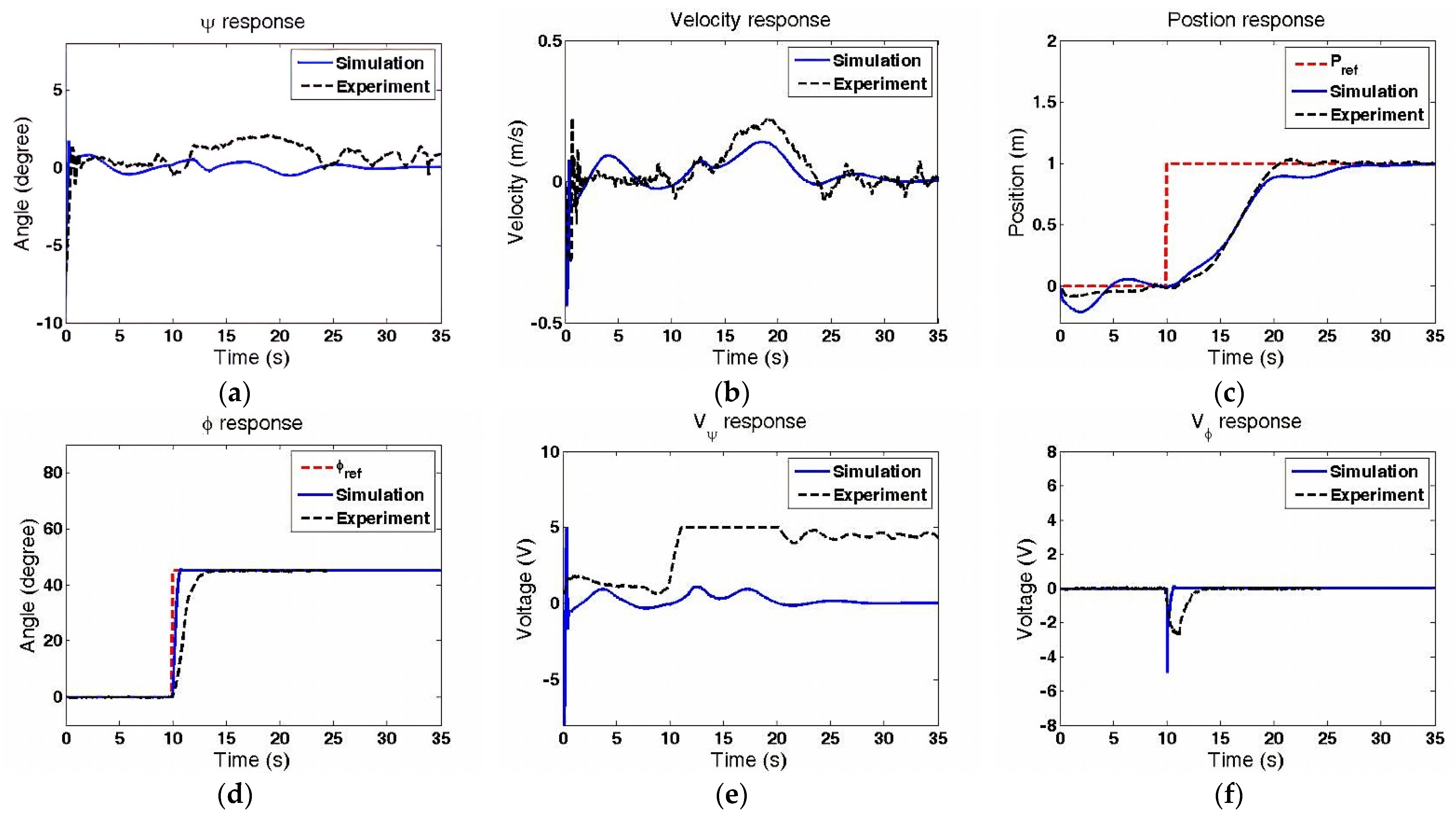
Walking Assistance by the TWIP Robot
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Appendix A. Modelling of the TWIP System
References
- Iwatani, M.; Kikuuwe, R. An identification procedure for rate-dependency of friction in robotic joints with limited motion ranges. Mechatronics 2016, 36, 36–44. [Google Scholar] [CrossRef]
- Mae, Y.; Choi, J.; Takahashi, H.; Ohara, K.; Takubo, T.; Arai, T. Interoperable vision component for object detection and 3D pose estimation for modularized robot control. Mechatronics 2011, 21, 983–992. [Google Scholar] [CrossRef]
- Michaud, F.; Boissy, P.; Labonté, D.; Brière, S.; Perreault, K.; Corriveau, H.; Grant, A.; Lauria, M.; Cloutier, R.; Roux, M.A.; et al. Exploratory design and evaluation of a homecare teleassistive mobile robotic system. Mechatronics 2010, 20, 751–766. [Google Scholar] [CrossRef]
- Wang, F.C.; Wang, Z.J. The Development of a Multi-Loop Control Structure for a Two-Wheeled Inverted Pendulum Robot. In Proceedings of the 2019 6th International Conference on Control, Decision and Information Technologies (CoDIT), Paris, France, 23–26 April 2019; pp. 551–556. [Google Scholar]
- Potkonjak, V.; Tzafestas, S.; Vukobratovic, M.; Milojevic, M.; Jovanovic, M. Human-and-Humanoid Postures Under External Disturbances: Modeling, Simulation, and Robustness. Part 1: Modeling. J. Intell. Robot. Syst. 2011, 63, 191–210. [Google Scholar]
- Janardhan, V.; Kumar, R.P. Kinematic Analysis of Biped Robot Forward Jump for Safe Locomotion. In Proceedings of the 1st International and 16th National Conference on Machines and Mechanisms (iNaCoMM 2013), Roorkee, India, 18–20 December 2013; pp. 1078–1082. [Google Scholar]
- Zhao, H.; Powell, M.; Ames, A. Human-inspired motion primitives and transitions for bipedal robotic locomotion in diverse terrain. Optim. Control Appl. Methods 2014, 35, 730–755. [Google Scholar] [CrossRef] [Green Version]
- Medrano Cerda, G.A.; Akdas, D. Stabilisation of a 12 Degree of Freedom Biped Robot. IFAC Proc. Vol. 2002, 35, 97–102. [Google Scholar] [CrossRef] [Green Version]
- Lu, J.C.; Chen, J.Y.; Lin, P.C. Turning in a bipedal robot. J. Bionic Eng. 2013, 10, 292–304. [Google Scholar] [CrossRef]
- Kim, Y.J.; Lee, J.Y.; Lee, J.J. A Balance Control Strategy for a Walking Biped Robot under Unknown Lateral External Force using a Genetic Algorithm. Int. J. Hum. Robot. 2015, 12, 1550021:1–1550021:37. [Google Scholar] [CrossRef]
- Aoustin, Y.; Hamon, A. Human like trajectory generation for a biped robot with a four-bar linkage for the knees. Robot. Auton. Syst. 2013, 61, 1717–1725. [Google Scholar] [CrossRef] [Green Version]
- Hosoda, K.; Takuma, T.; Nakamoto, A.; Hayashi, S. Biped robot design powered by antagonistic pneumatic actuators for multi-modal locomotion. Robot. Auton. Syst. 2008, 56, 46–53. [Google Scholar] [CrossRef]
- Joe, H.M.; Oh, J.H. A Robust Balance-Control Framework for the Terrain-Blind Bipedal Walking of a Humanoid Robot on Unknown and Uneven Terrain. Sensors 2019, 19, 4194. [Google Scholar] [CrossRef] [Green Version]
- Liu, C.C.; Lee, T.T.; Xiao, S.R.; Lin, Y.C.; Lin, Y.Y.; Wong, C.C. Real-Time FPGA-Based Balance Control Method for a Humanoid Robot Pushed by External Forces. Appl. Sci. 2020, 10, 2699. [Google Scholar] [CrossRef] [Green Version]
- Xi, A.; Chen, C. Stability Control of a Biped Robot on a Dynamic Platform Based on Hybrid Reinforcement Learning. Sensors 2020, 20, 4468. [Google Scholar] [CrossRef] [PubMed]
- Takei, T.; Imamura, R.; Yuta, S. Baggage Transportation and Navigation by a Wheeled Inverted Pendulum Mobile Robot. IEEE Trans. Ind. Electron. 2009, 56, 3985–3994. [Google Scholar] [CrossRef]
- Dai, F.; Gao, X.; Jiang, S.; Guo, W.; Liu, Y. A two-wheeled inverted pendulum robot with friction compensation. Mechatronics 2015, 30, 116–125. [Google Scholar] [CrossRef]
- Zhou, Y.; Wang, Z. Robust motion control of a two-wheeled inverted pendulum with an input delay based on optimal integral sliding mode manifold. Nonlinear Dyn. 2016, 85, 2065–2074. [Google Scholar] [CrossRef]
- Unluturk, A.; Aydogdu, O. Adaptive control of two-wheeled mobile balance robot capable to adapt different surfaces using a novel artificial neural network–based real-time switching dynamic controller. Int. J. Adv. Robot. Syst. 2017, 14, 172988141770089. [Google Scholar] [CrossRef]
- Kim, S.; Kwon, S. Nonlinear Optimal Control Design for Underactuated Two-Wheeled Inverted Pendulum Mobile Platform. IEEE/ASME Trans. Mechatron. 2017, 22, 2803–2808. [Google Scholar] [CrossRef]
- Jamin, N.F.; Ghani, N.M.A.; Ibrahim, Z. Movable payload on various conditions of two-wheeled double links wheelchair stability control using enhanced interval type-2 fuzzy logic. IEEE Access 2020, 8, 87676–87694. [Google Scholar] [CrossRef]
- Sánchez, B.; Cuvas, C.; Ordaz, P.; Santos-Sánchez, O.; Poznyak, A. Full-Order Observer for a Class of Nonlinear Systems with Unmatched Uncertainties: Joint Attractive Ellipsoid and Sliding Mode Concepts. IEEE Trans. Ind. Electron. 2020, 67, 5677–5686. [Google Scholar] [CrossRef]
- Grasser, F.; D’Arrigo, A.; Colombi, S.; Rufer, A.C. JOE: A mobile, inverted pendulum. IEEE Trans. Ind. Electron. 2002, 49, 107–114. [Google Scholar] [CrossRef]
- Huang, J.; Guan, Z.H.; Takayuki, M.; Fukuda, T.; Kosuke, S. Sliding-Mode Velocity Control of Mobile-Wheeled Inverted-Pendulum Systems. IEEE Trans. Robot. 2010, 26, 750–758. [Google Scholar] [CrossRef]
- Bature, A.; Buyamin, S.; Ahmad, N.M.; Muhammad, M.; Abdullahi, M.A. Intelligent Controllers for Velocity Tracking of Two Wheeled Inverted Pendulem Mobile Robot. J. Teknol. 2016, 78. [Google Scholar] [CrossRef] [Green Version]
- Oliveira, T.C.D.; Fujiwara, E.; De Paiva, E.C. Modular approach for motion control design of three-dimensional two-wheeled inverted pendulum. In Proceedings of the IEEE 15th International Workshop on Advanced Motion Control (AMC), Tokyo, Japan, 9–11 March 2018; pp. 96–101. [Google Scholar]
- Ha, Y.S.; Yuta, S.I. Trajectory tracking control for navigation of the inverse pendulum type self-contained mobile robot. Robot. Auton. Syst. 1996, 17, 65–80. [Google Scholar] [CrossRef]
- Chiu, C.H.; Lin, Y.W.; Lin, C.H. Real-time control of a wheeled inverted pendulum based on an intelligent model free controller. Mechatronics 2011, 21, 523–533. [Google Scholar] [CrossRef]
- Herrera, M.; Cuaycal, A.; Camacho, O.; Pozo, D. LQR Discrete Controller Tuning for a TWIP Robot Based on Genetic Algorithms. In Proceedings of the International Conference on Information Systems and Computer Science (INCISCOS), Quito, Ecuador, 20–22 November 2019; pp. 163–168. [Google Scholar]
- Zhou, H.T.; Li, X.; Feng, H.B.; Li, E.B.; Ding, P.C.; Zhai, Y.W.; Zhang, S.Y.; Fu, Y.L. Control of the Two-wheeled Inverted Pendulum (TWIP) Robot Moving on the Continuous Uneven Ground. In Proceedings of the IEEE International Conference on Robotics and Biomimetics (ROBIO), Dali, China, 6–8 December 2019; pp. 1588–1594. [Google Scholar]
- Jin, S.K.; Ou, Y.S. A Wheeled Inverted Pendulum Learning Stable and Accurate Control from Demonstrations. Appl. Sci. 2019, 9, 5279. [Google Scholar] [CrossRef] [Green Version]
- BLDC Motor. Available online: https://www.trumman.com.tw/2016products/EV.html (accessed on 14 August 2021).
- BNO055 Datasheet. Available online: https://cdn-shop.adafruit.com/datasheets/BST_BNO055_DS000_12.pdf (accessed on 14 August 2021).
- Arduino Due. Available online: https://store.arduino.cc/usa/due (accessed on 14 August 2021).
- Glover, K.; McFarlane, D. Robust stabilization of normalized coprime factor plant descriptions with H/sub infinity /-bounded uncertainty. IEEE Trans. Autom. Control 1989, 34, 821–830. [Google Scholar] [CrossRef]
- Zhou, K.; Doyle, J.C. Essentials of Robust Control; Prentice Hall: Upper Saddle River, NJ, USA, 1998. [Google Scholar]
- Glover, K.; McFarlane, D. A loop shaping design procedure using H∞-synthesis. IEEE Trans. Autom. Control 1992, 37, 759–769. [Google Scholar]
- Force Sensing Resister Datasheet. Available online: https://cdn-learn.adafruit.com/assets/assets/000/010/126/original/fsrguide.pdf (accessed on 14 August 2021).
- Demo Videos. Available online: http://140.112.14.7/~sic/lab/web/TWIP_Test.php (accessed on 14 August 2021).
- Wang, Y.H.; Wang, T.W.; Yen, J.Y.; Wang, F.C. Dynamic human object recognition by combining color and depth information with a clothing image histogram. Int. J. Adv. Robot. Syst. 2019, 16, 1729881419828105. [Google Scholar] [CrossRef] [Green Version]
- Li, S.A.; Chou, L.H.; Chang, T.H.; Yang, C.H.; Chang, Y.C. Obstacle Avoidance of Mobile Robot Based on HyperOmni Vision. Sens. Mater. 2019, 31, 1021–1036. [Google Scholar] [CrossRef]
- Maldonado-Bascón, S.; Iglesias-Iglesias, C.; Martín-Martín, P.; Lafuente-Arroyo, S. Fallen People Detection Capabilities Using Assistive Robot. Electronics 2019, 8, 915. [Google Scholar] [CrossRef] [Green Version]







| Symbol | Description | Value |
|---|---|---|
| M | Weight of the cart | 13 kg |
| Jψ | Pitch inertia moment of the cart | 1.6 kgm2 |
| Yaw inertia moment of the cart | 0.3985 kgm2 | |
| l | Distance of the the mass center | 0.2 m |
| W | Width of the cart | 0.569 m |
| m | Wheel weight (each wheel) | 1.56 kg |
| Jw | Wheel inertia moment (each wheel) | 0.0014 kgm2 |
| r | Wheel radius | 0.1524 m |
| R | Motor resistance | 0.065 Ω |
| L | Motor inductance | 0.138 mH |
| Kt | Motor torque constant | 0.068 Nm/A |
| Ke | Motor back EMF constant | 0.04 Vs/rad |
| Jm | Motor inertia moment | 0.0002 kgm2 |
| n1 | Gear ratio | 15 |
| fbw | Friction between the motor and the body | 0.45 Nms/rad |
| Location | Place 1 | Place 2 | ||
|---|---|---|---|---|
| Response | ||||
| Rise time | 0.40 s | ― | 0.40 s | ― |
| Settling time | 2.84 s | ― | 4.91 s | ― |
| Overshoot maximum | 0.97° | 11 mm | 1.52° | 52 mm |
| MAE | 0.178° | 8.9 mm | 0.174° | 3.3 mm |
| RMSE | 0.216° | 9.3 mm | 0.224° | 5.0 mm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, F.-C.; Chen, Y.-H.; Wang, Z.-J.; Liu, C.-H.; Lin, P.-C.; Yen, J.-Y. Decoupled Multi-Loop Robust Control for a Walk-Assistance Robot Employing a Two-Wheeled Inverted Pendulum. Machines 2021, 9, 205. https://doi.org/10.3390/machines9100205
Wang F-C, Chen Y-H, Wang Z-J, Liu C-H, Lin P-C, Yen J-Y. Decoupled Multi-Loop Robust Control for a Walk-Assistance Robot Employing a Two-Wheeled Inverted Pendulum. Machines. 2021; 9(10):205. https://doi.org/10.3390/machines9100205
Chicago/Turabian StyleWang, Fu-Cheng, Yu-Hong Chen, Zih-Jia Wang, Chi-Hao Liu, Pei-Chun Lin, and Jia-Yush Yen. 2021. "Decoupled Multi-Loop Robust Control for a Walk-Assistance Robot Employing a Two-Wheeled Inverted Pendulum" Machines 9, no. 10: 205. https://doi.org/10.3390/machines9100205
APA StyleWang, F.-C., Chen, Y.-H., Wang, Z.-J., Liu, C.-H., Lin, P.-C., & Yen, J.-Y. (2021). Decoupled Multi-Loop Robust Control for a Walk-Assistance Robot Employing a Two-Wheeled Inverted Pendulum. Machines, 9(10), 205. https://doi.org/10.3390/machines9100205







