Experimental Characterization of Large Turbomachinery Tilting Pad Journal Bearings
Abstract
:1. Introduction
2. Materials and Methods
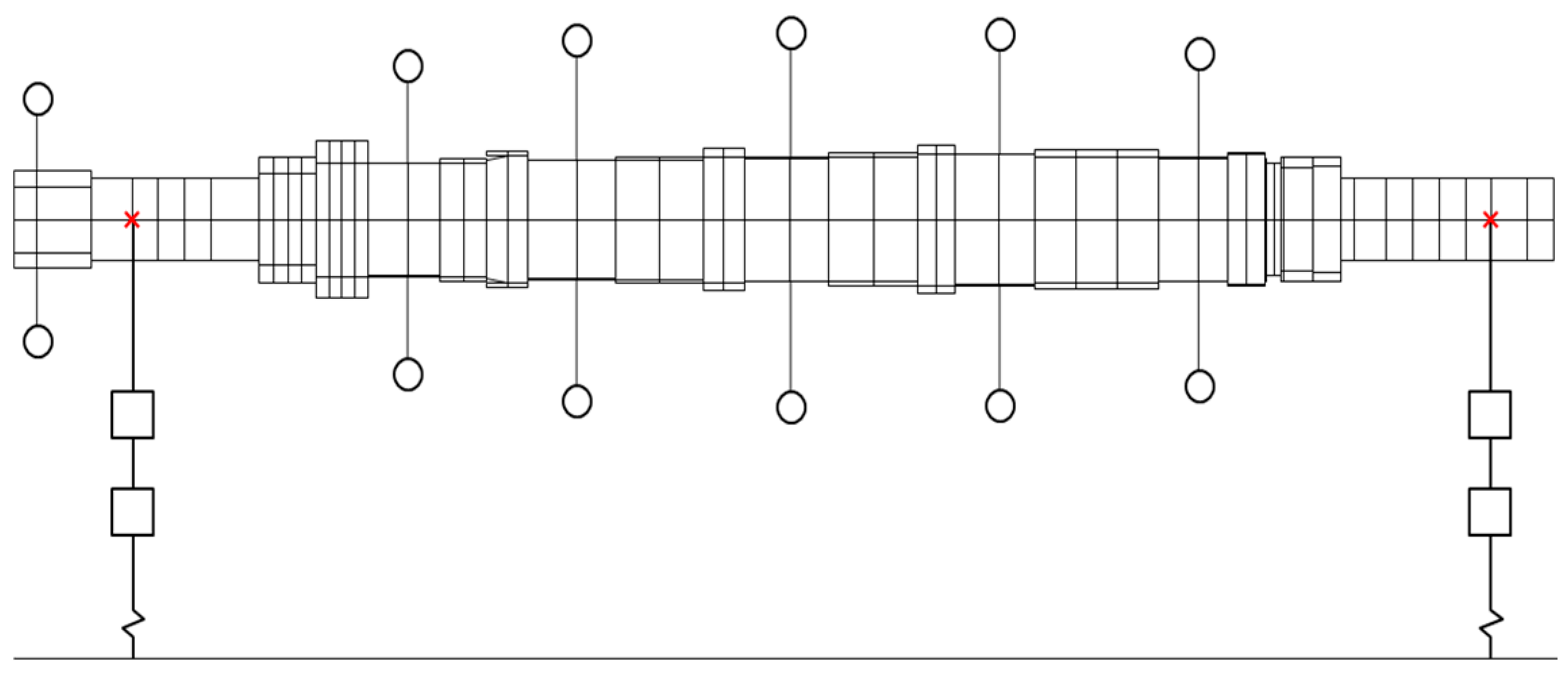
2.1. The Experimental Apparatus
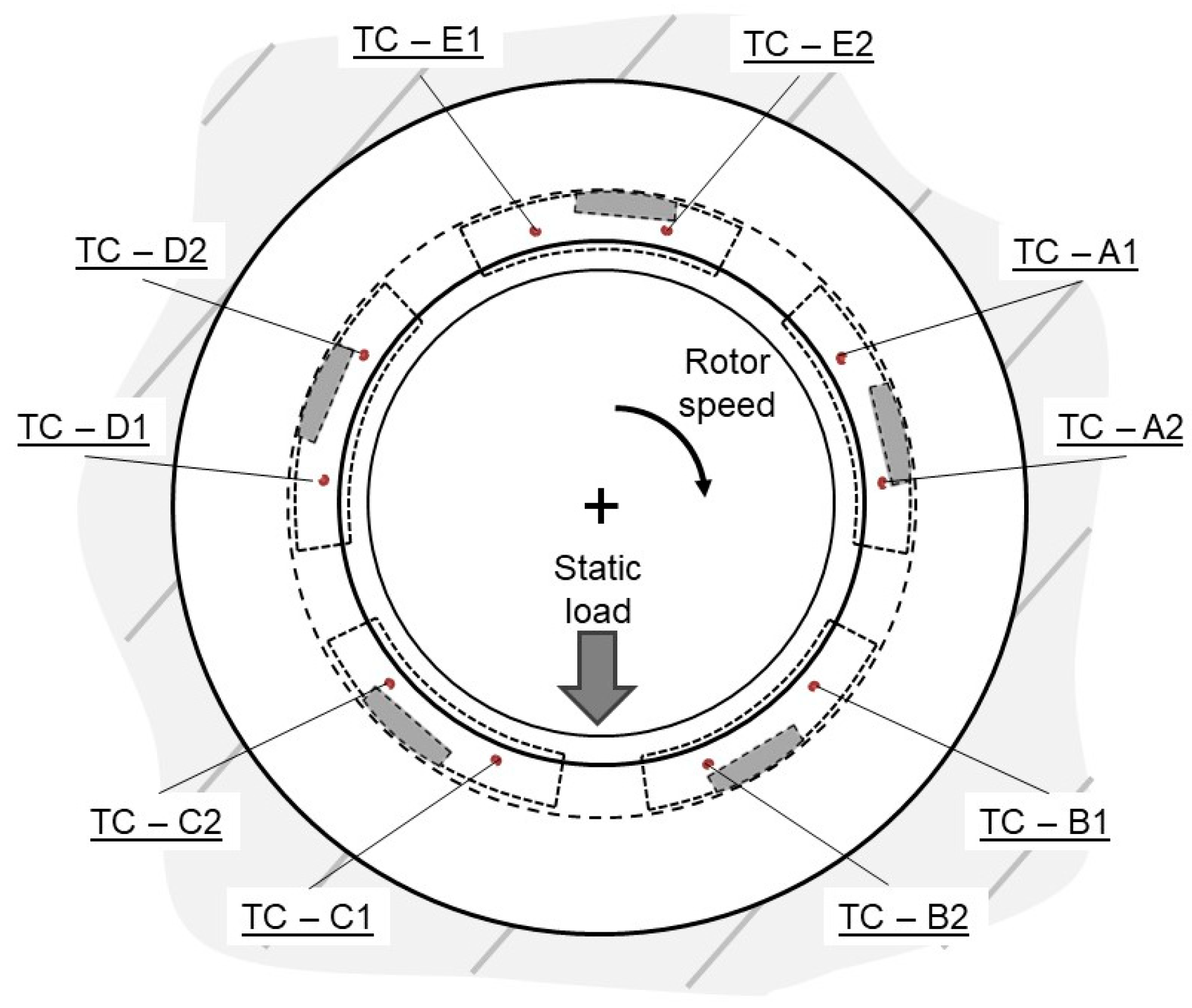
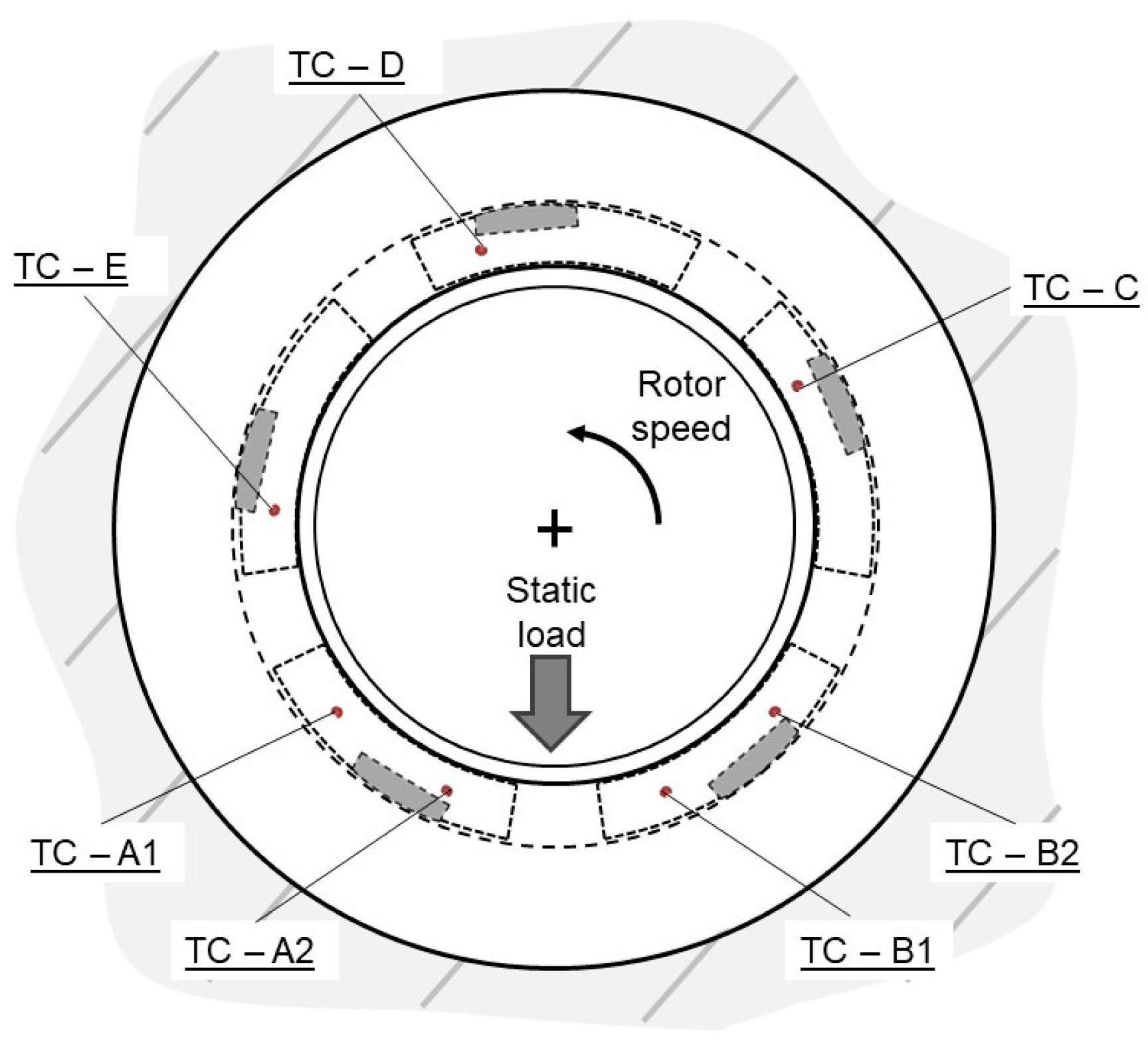
2.2. Test Section and Test Types
2.3. Dynamic Coefficients Identification Method
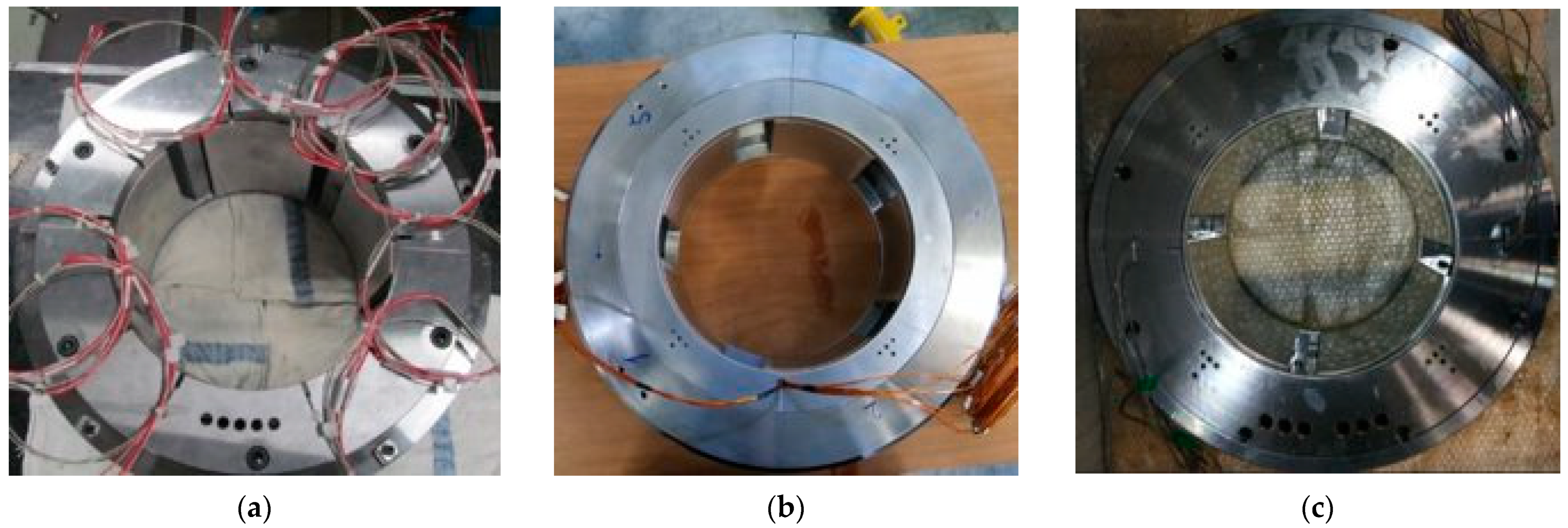
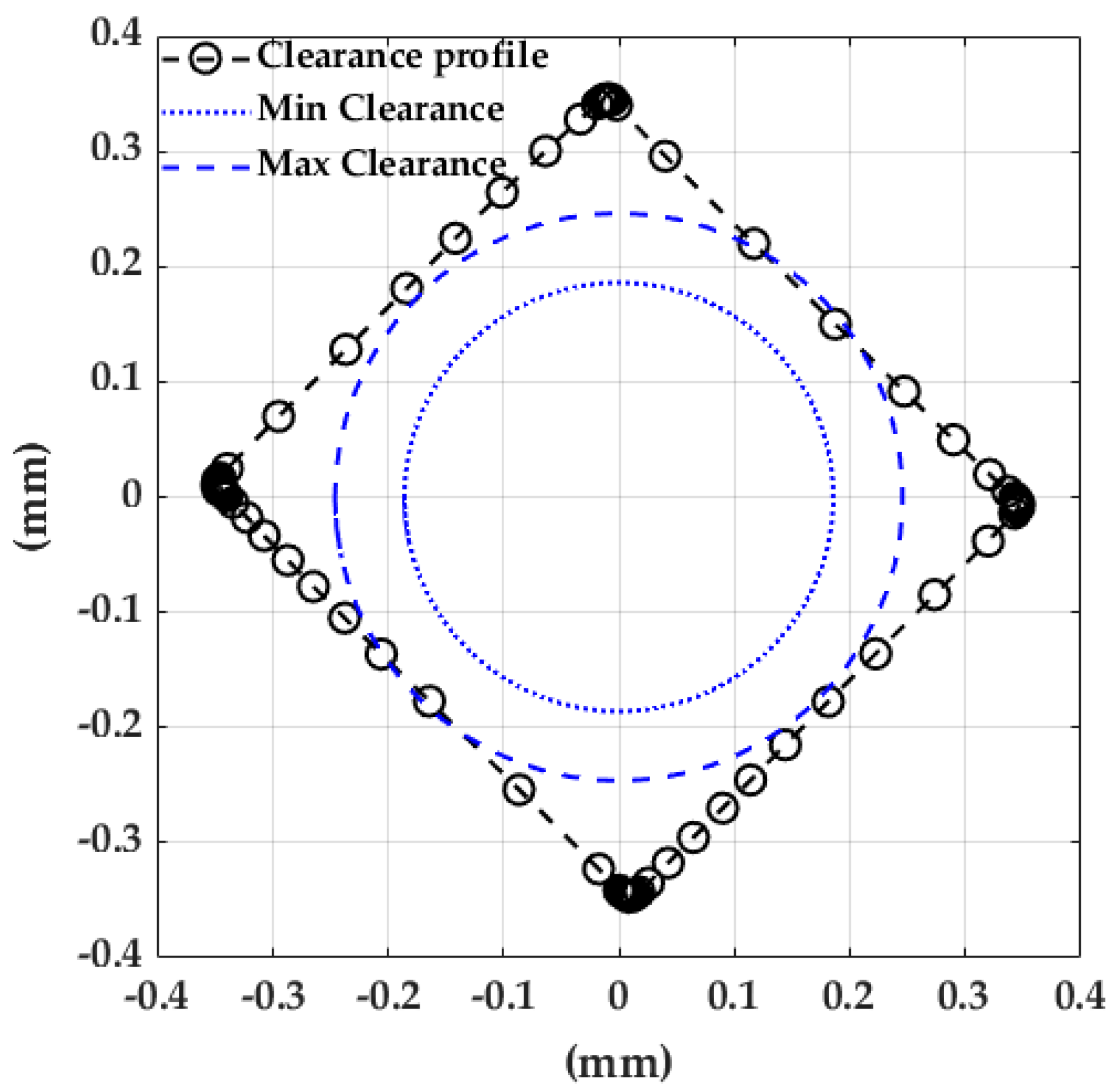
2.4. Tested Bearings
- flooded lubrication when the end seals clearance is low enough to get pressurized bearing housings;
- direct lubrication when all the required oil is supplied by oil inlet orifices/spray bars and the bearing cavity is evacuated through the adoption of larger end seals clearance with respect to flooded configurations.
3. Results
- bearing specific load (0.2–2.2 MPa).
- peripheral speed (up to 130 m/s).
- oil flow (50–100% of required flow).
- oil inlet temperature (40–60 °C).
- load direction: Load-between-Pivot (LBP).
3.1. Static Performance
3.1.1. The Bump Test Results
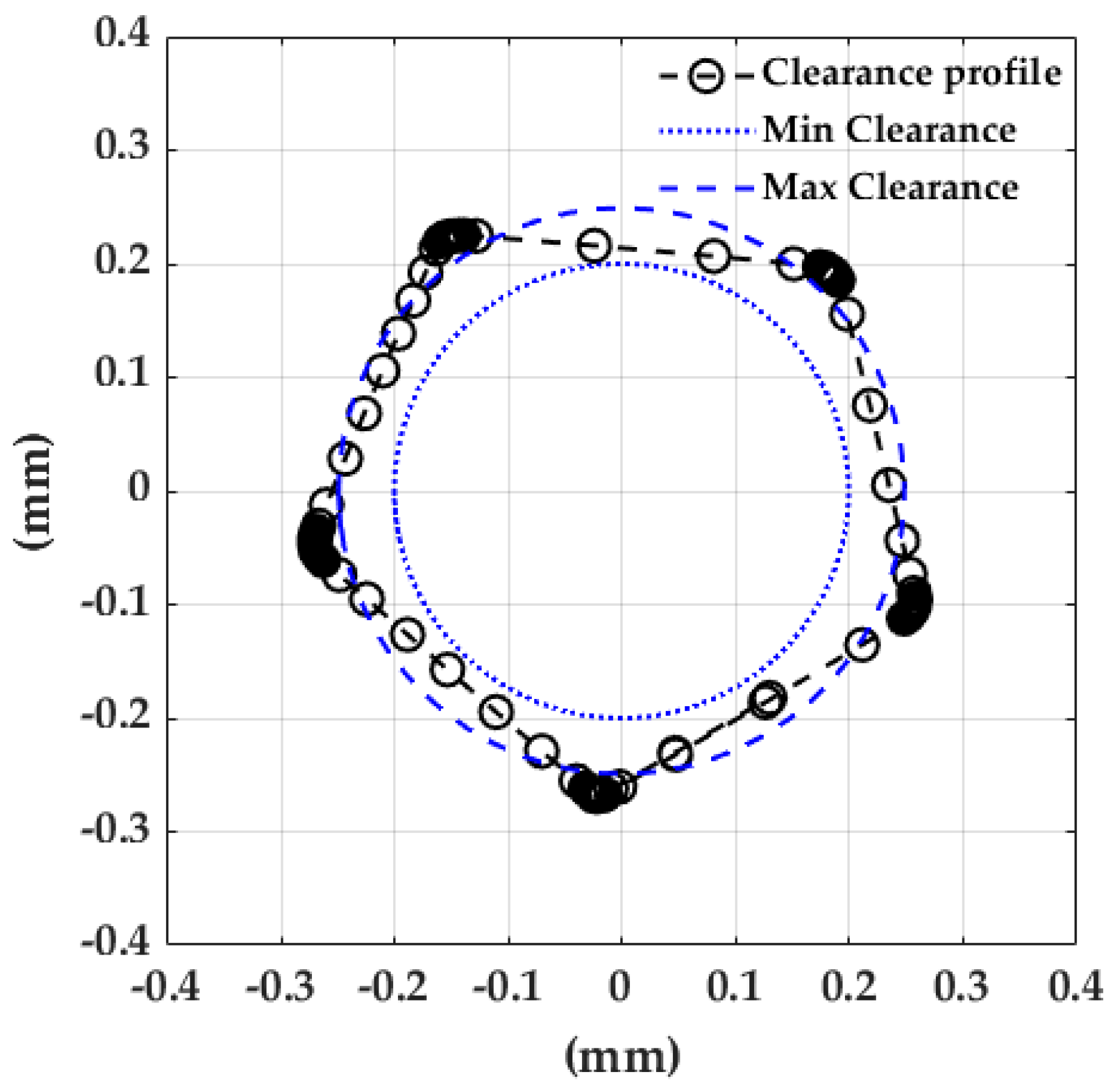
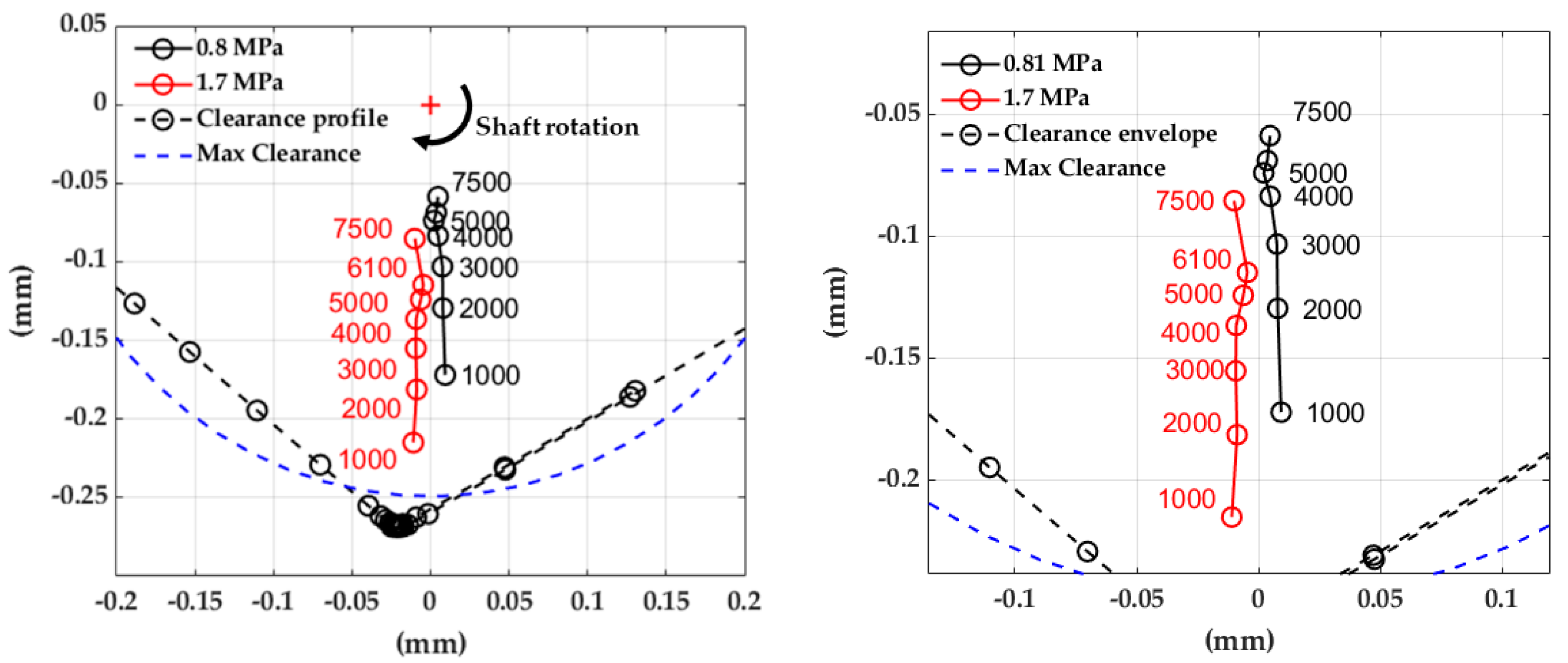
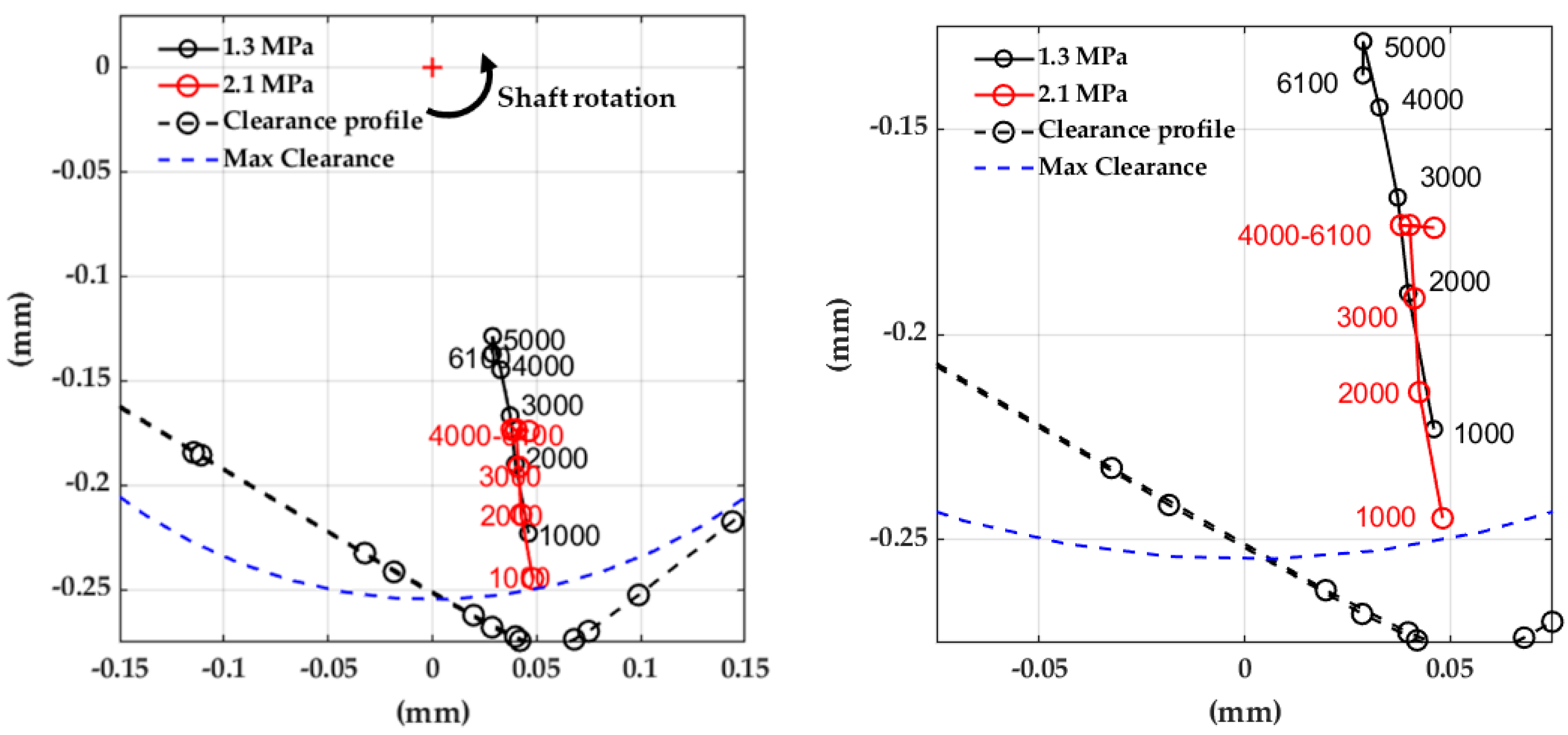
3.1.2. The Shaft Center Eccentricity
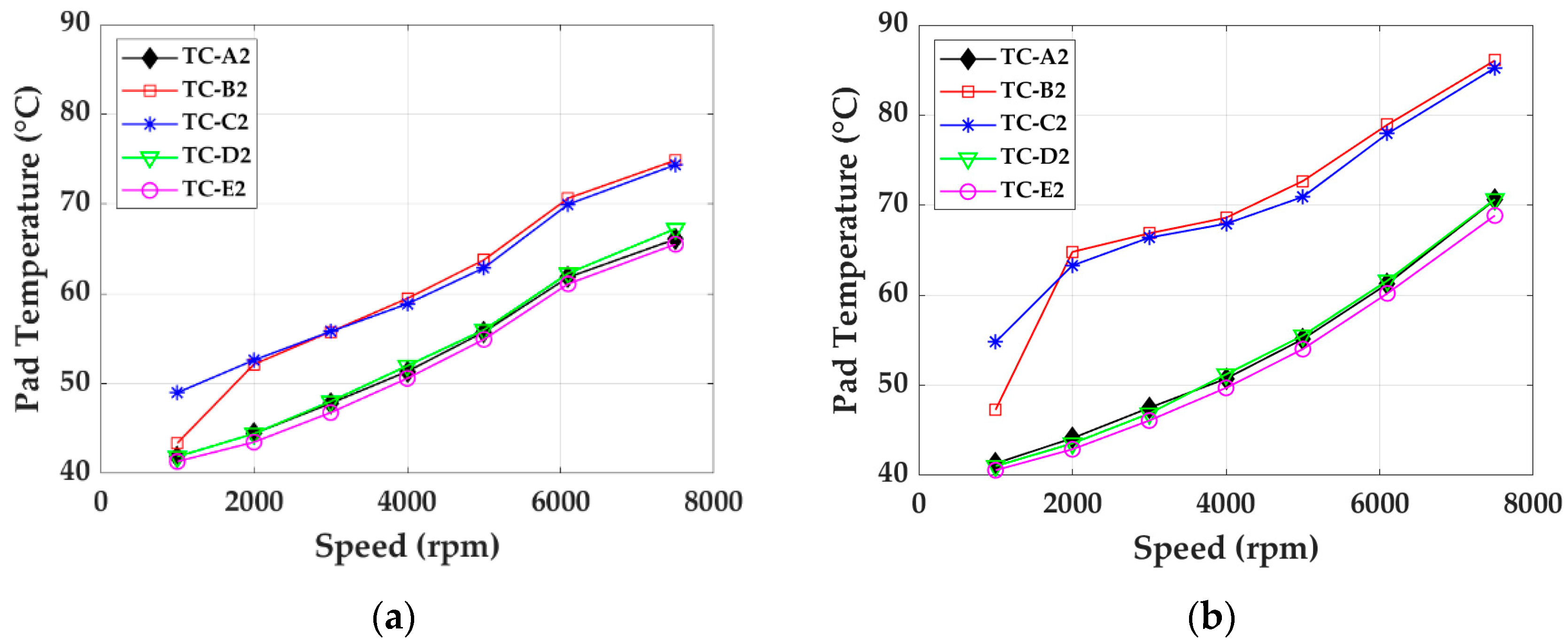
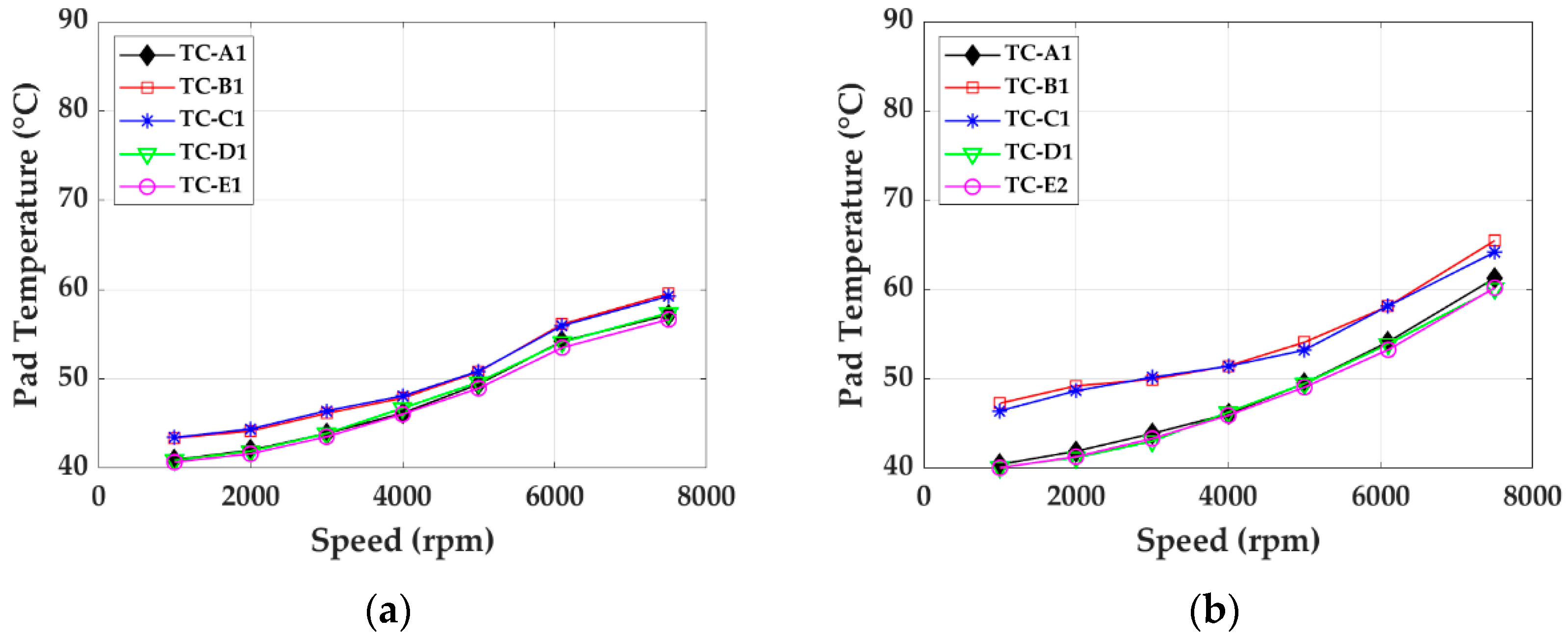
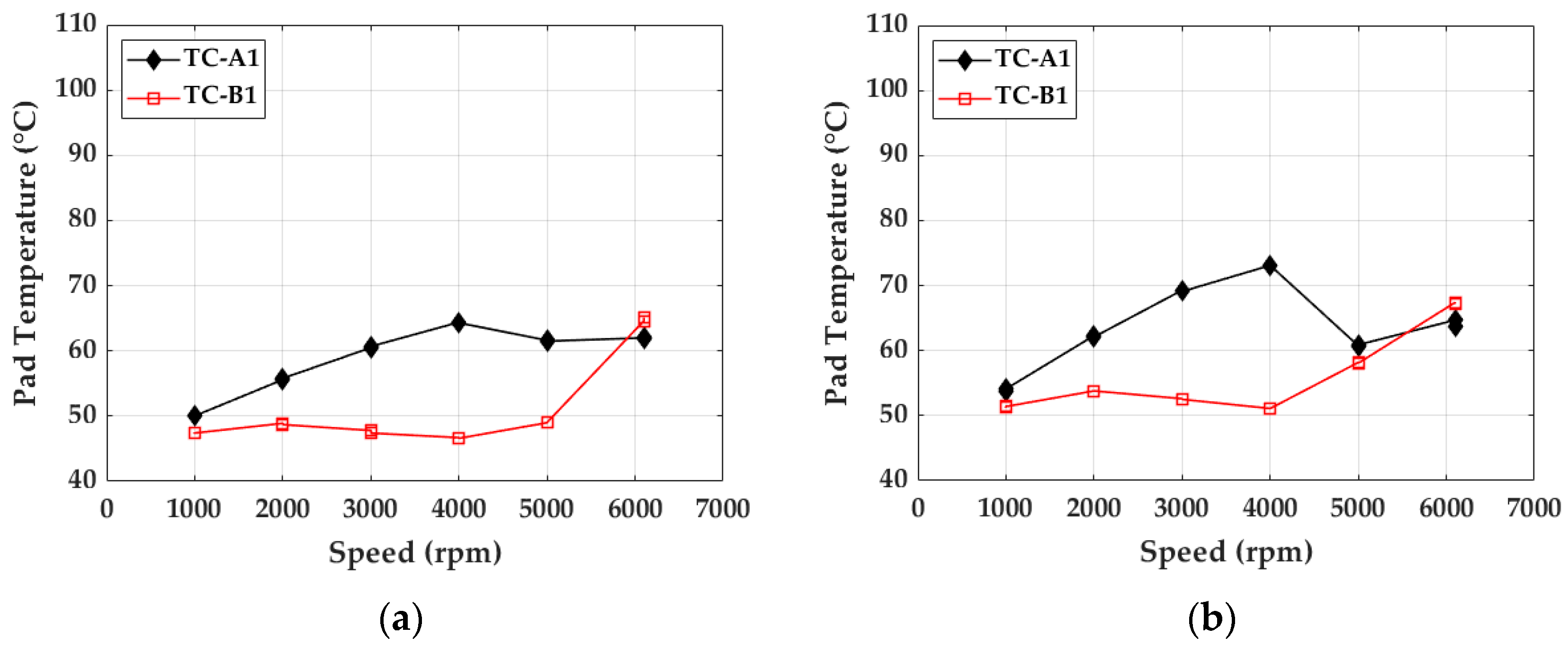
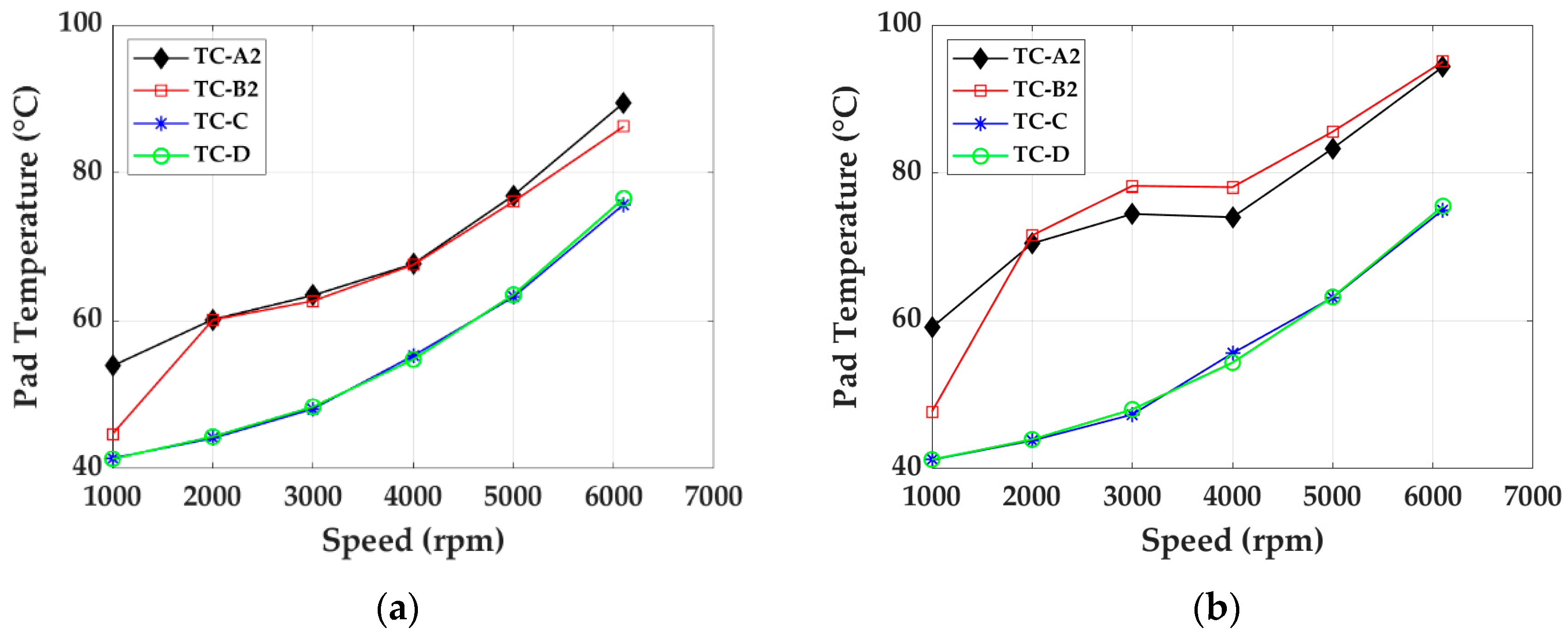
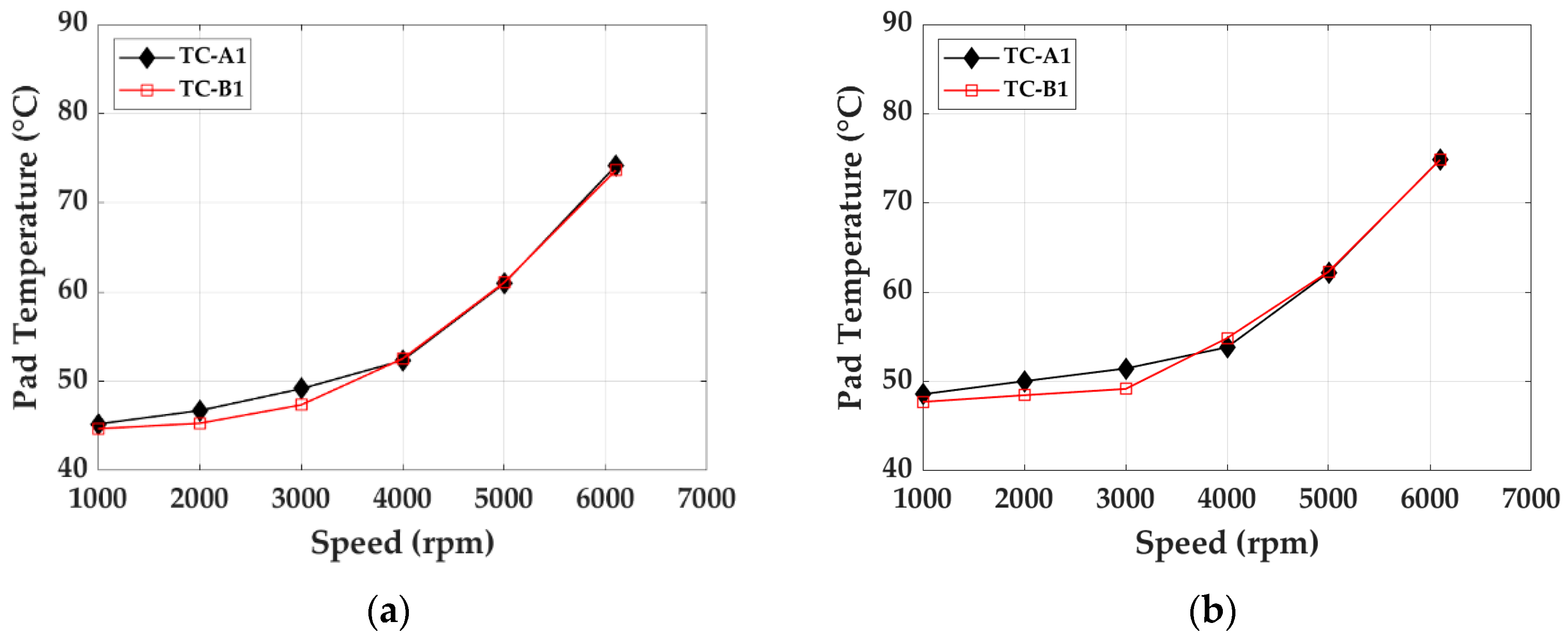
3.1.3. The Pad Temperatures
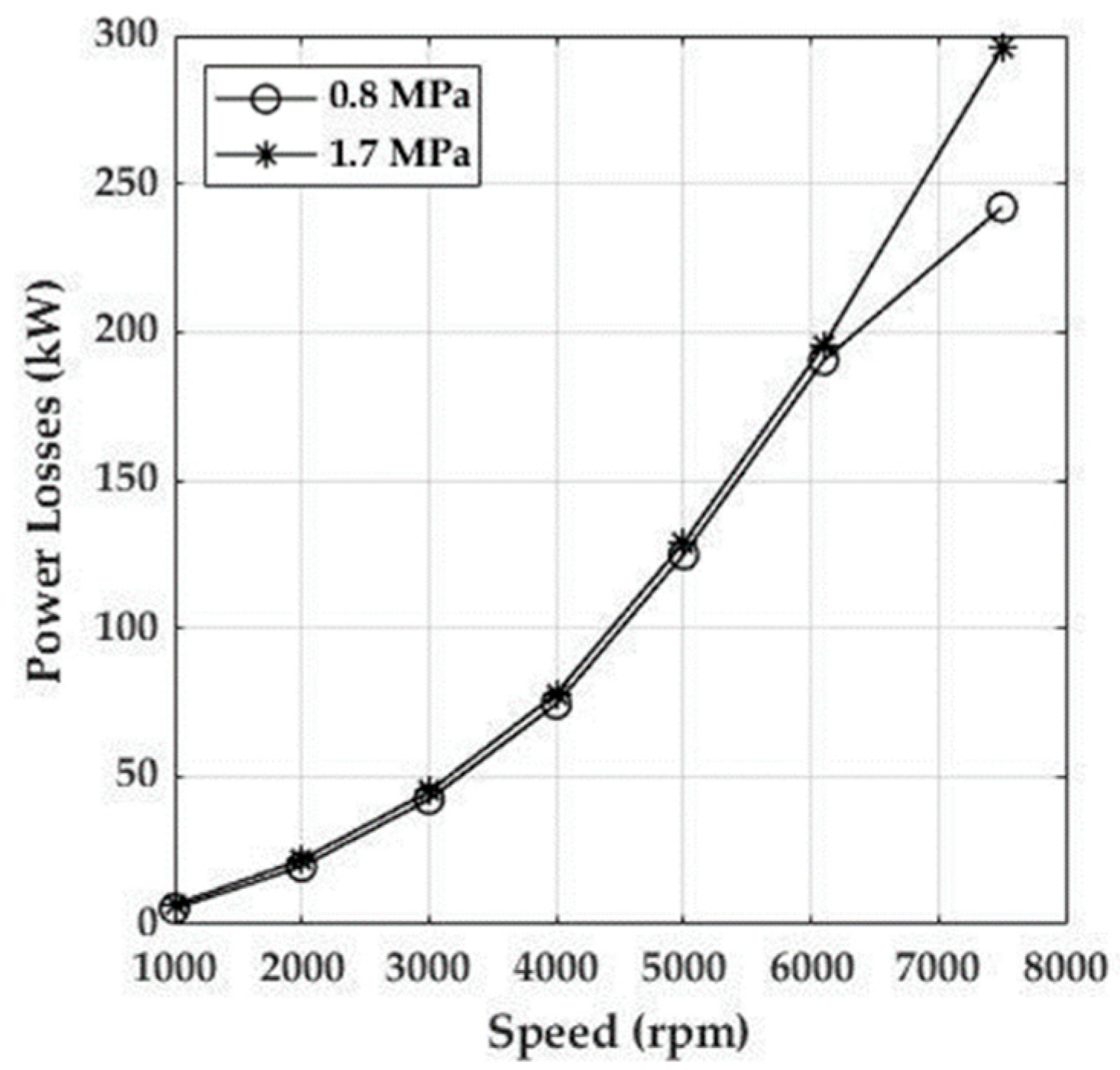
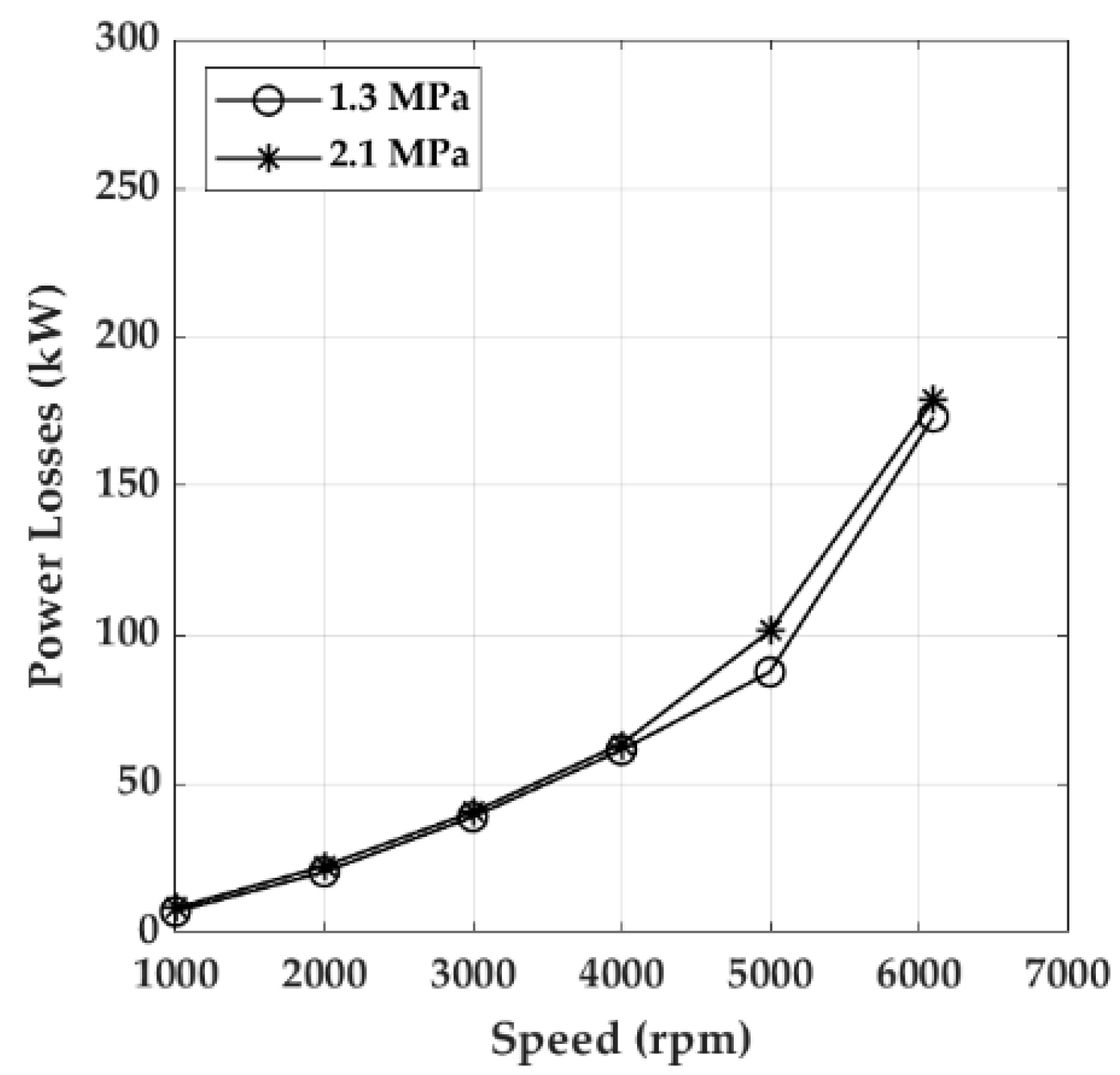
3.1.4. Power Losses
3.2. Dynamic Performance
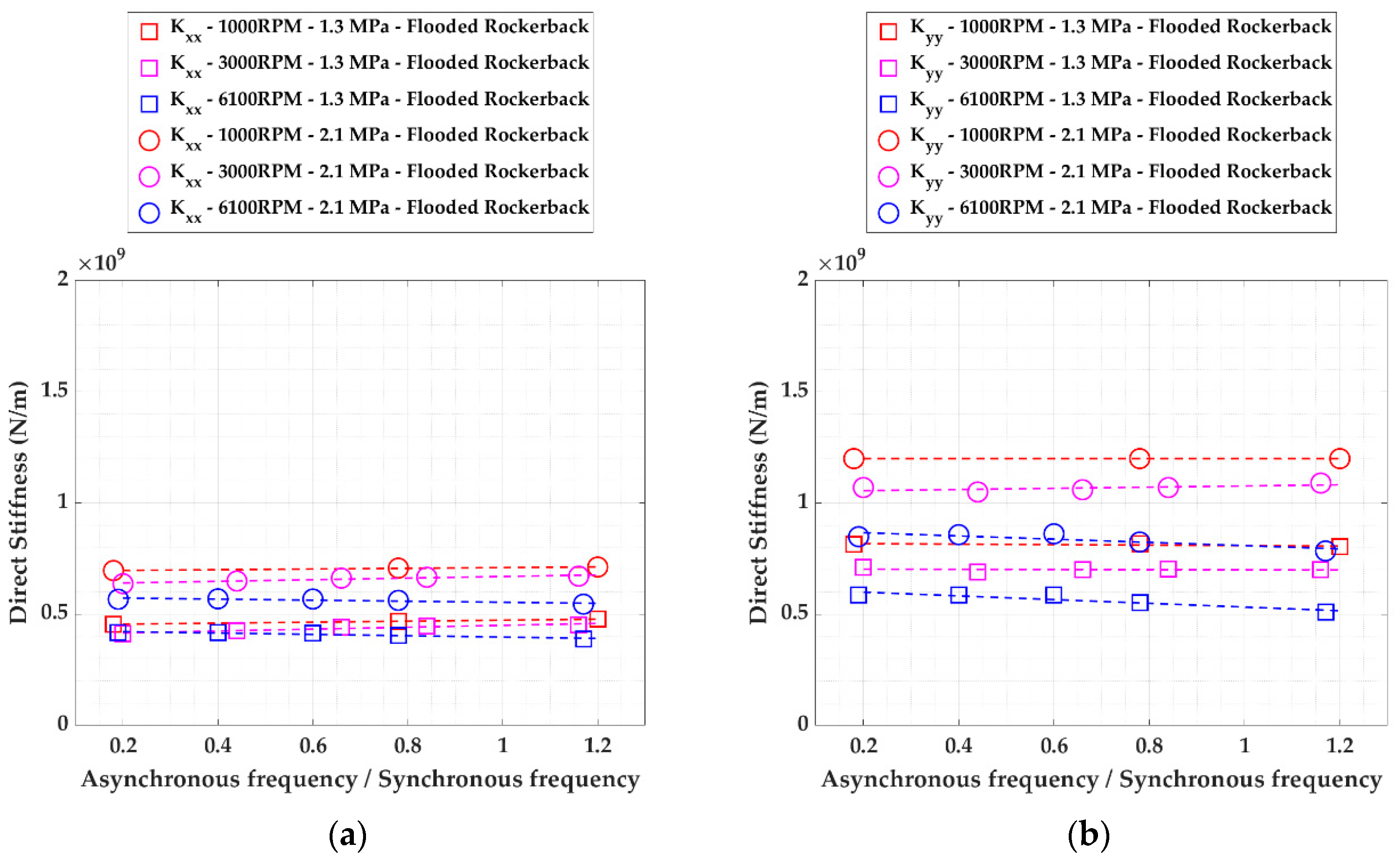
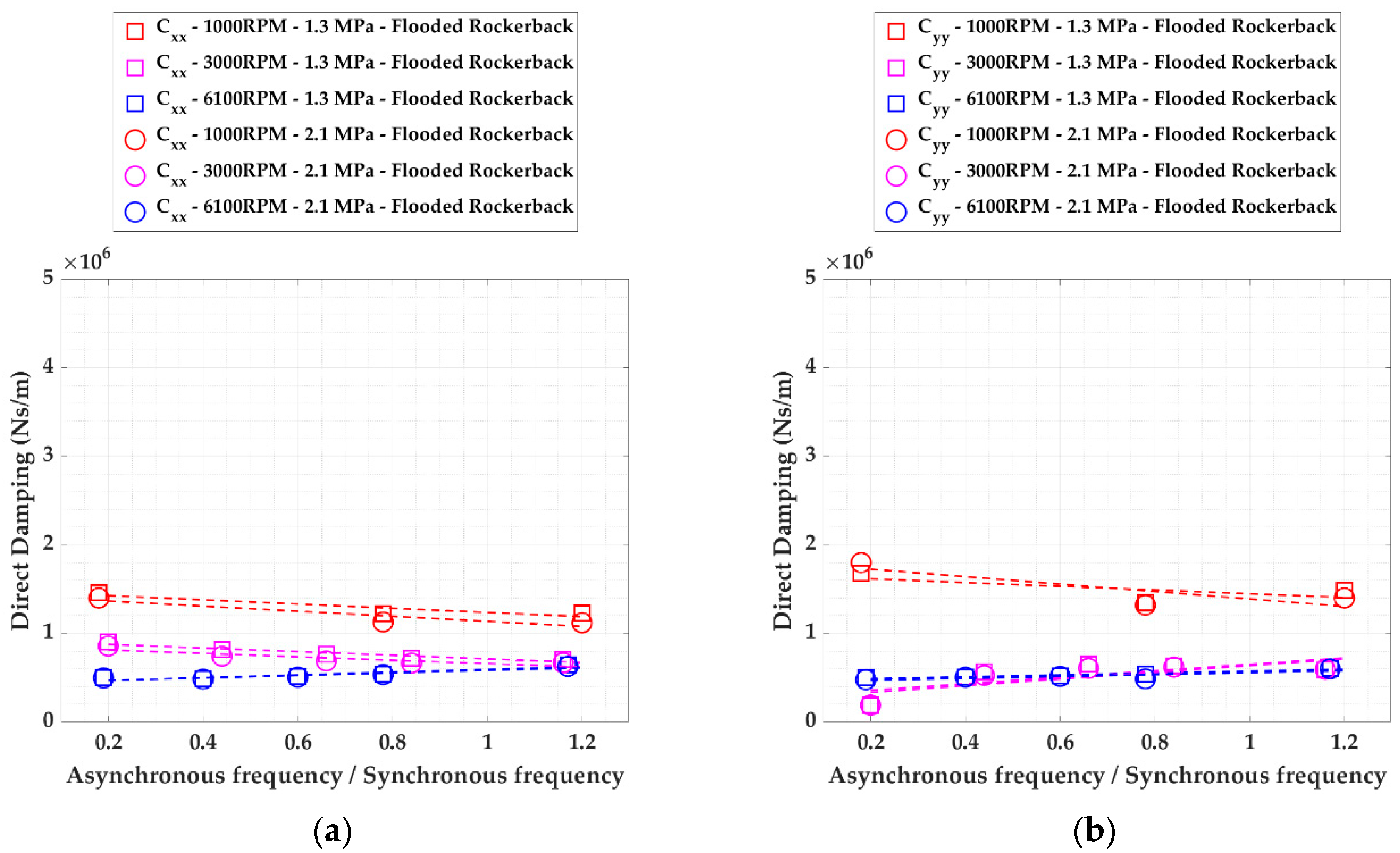
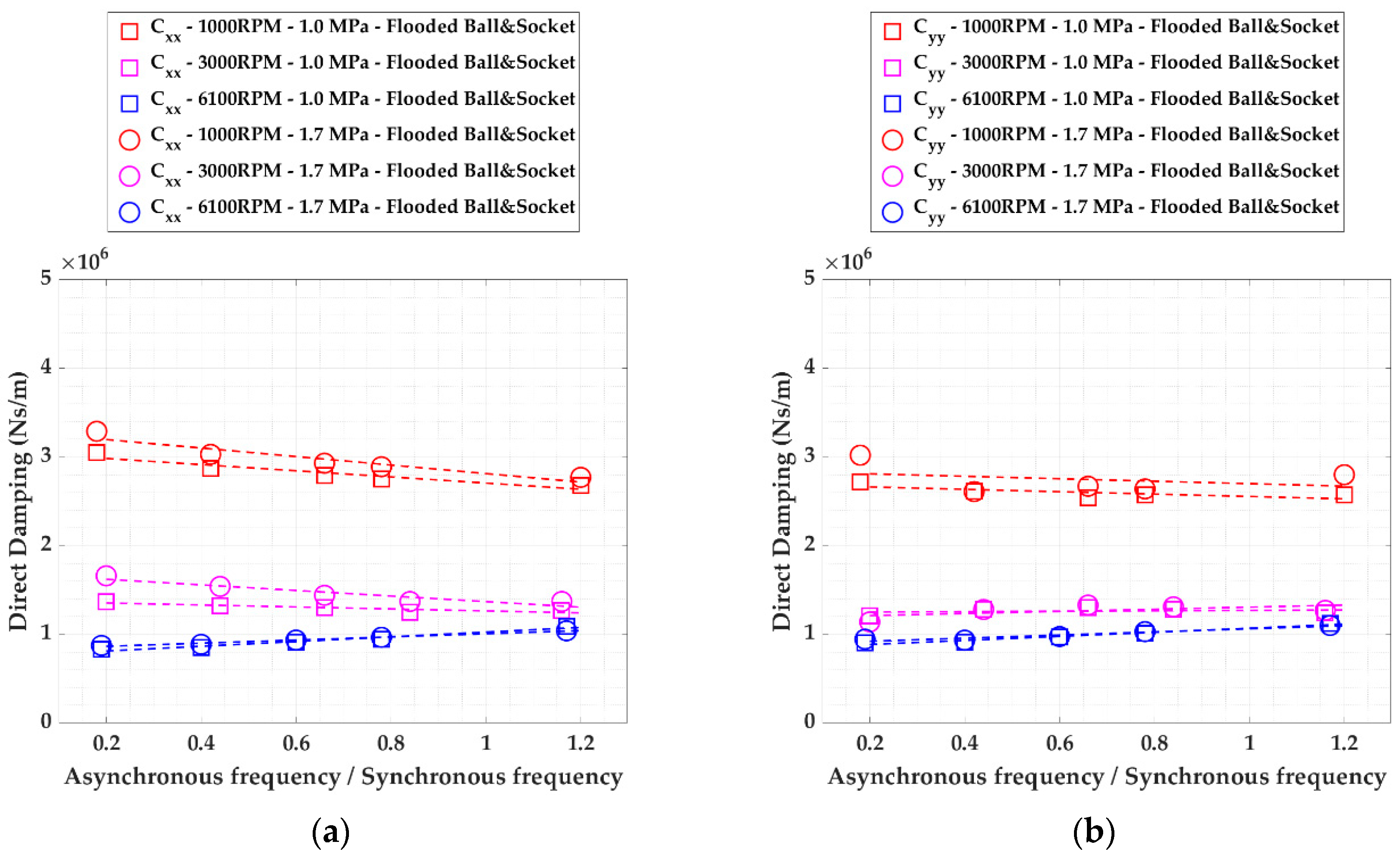
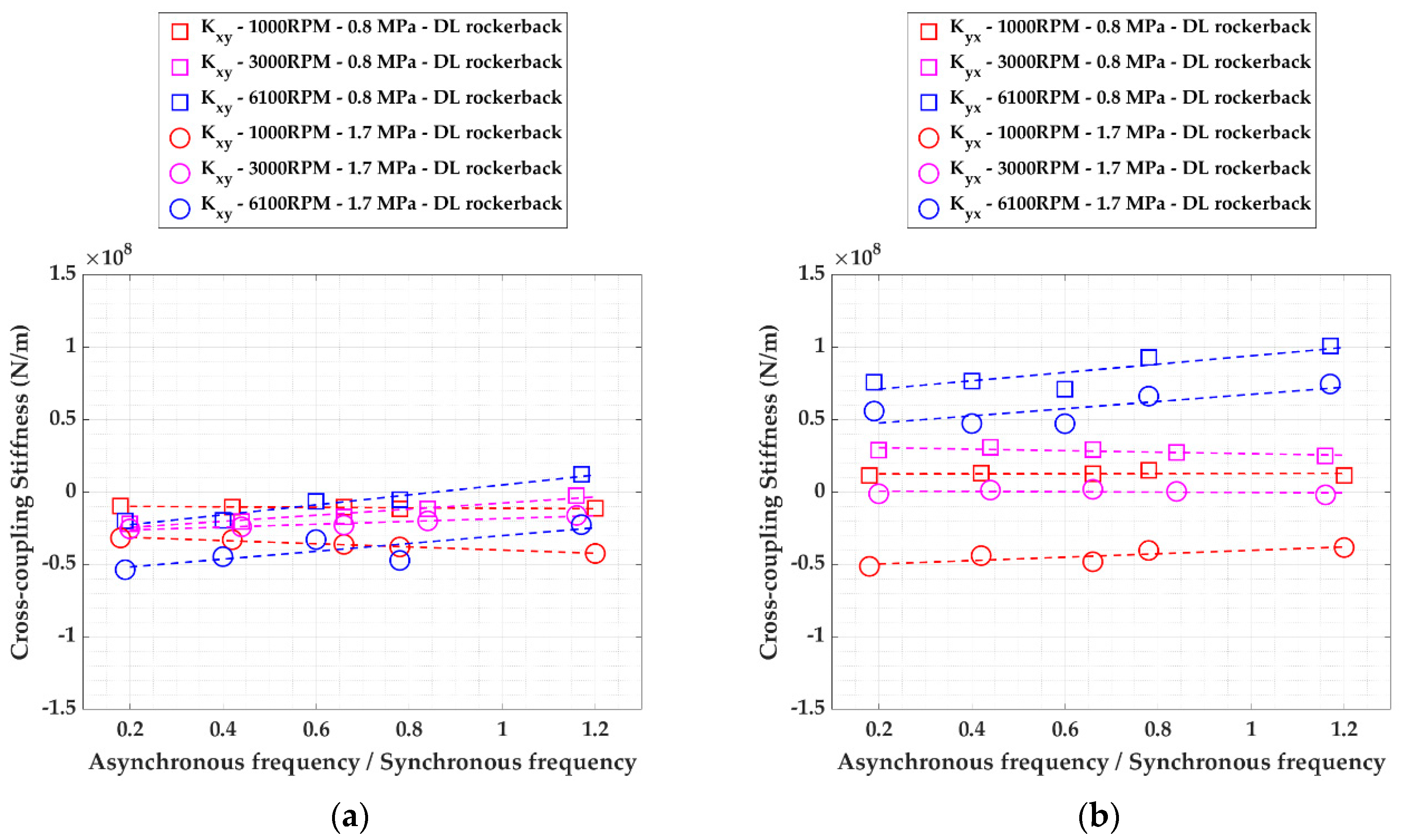
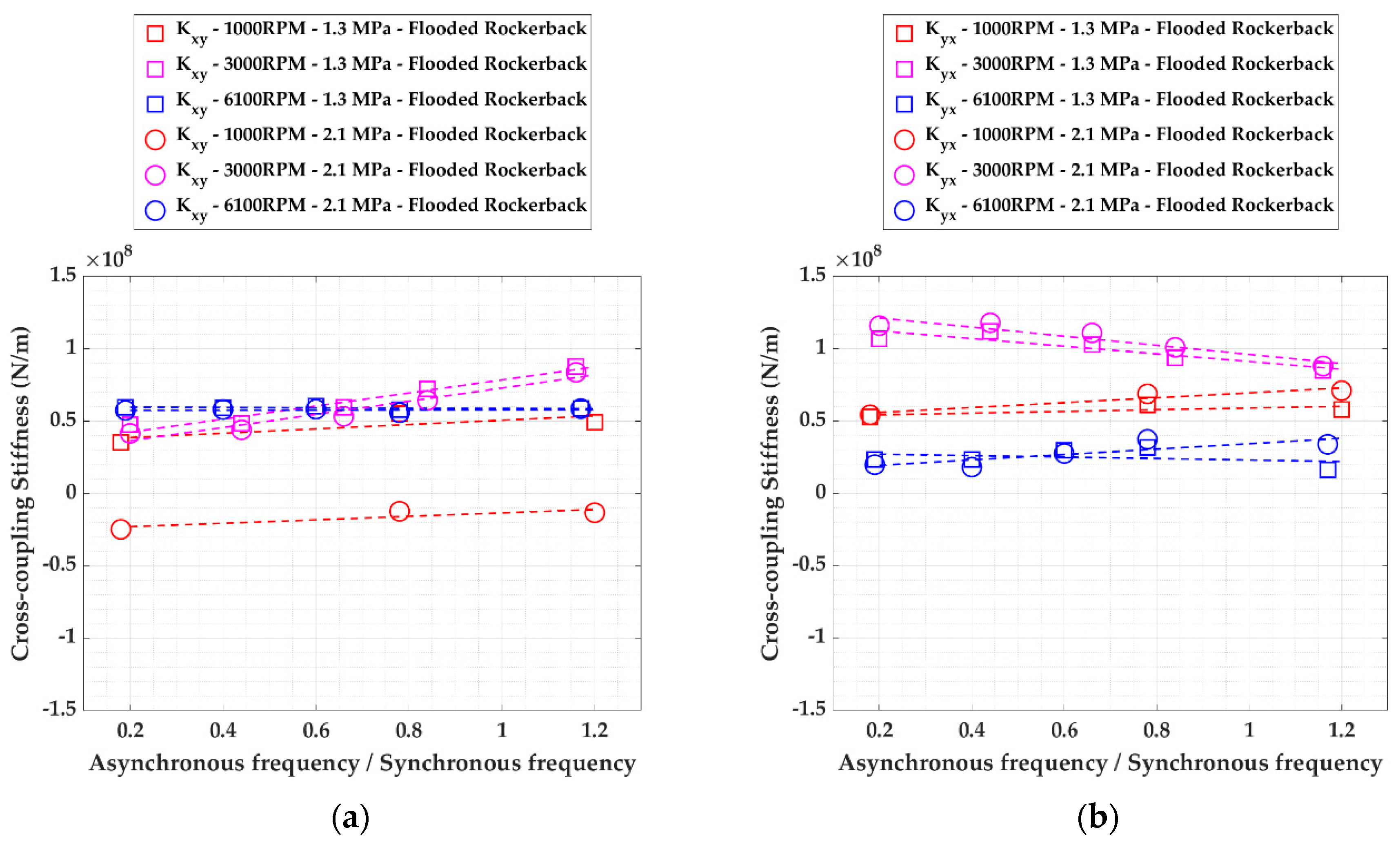
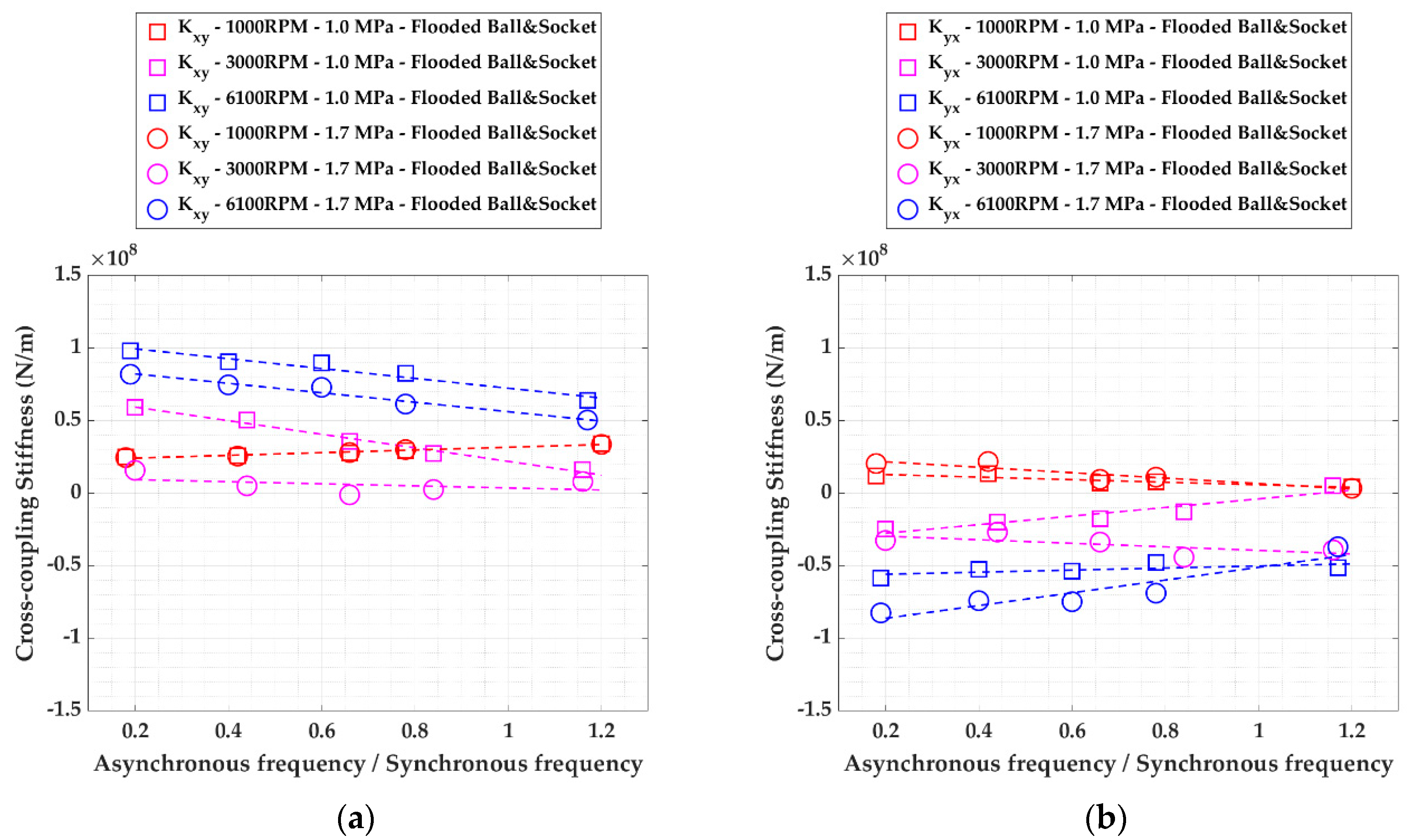
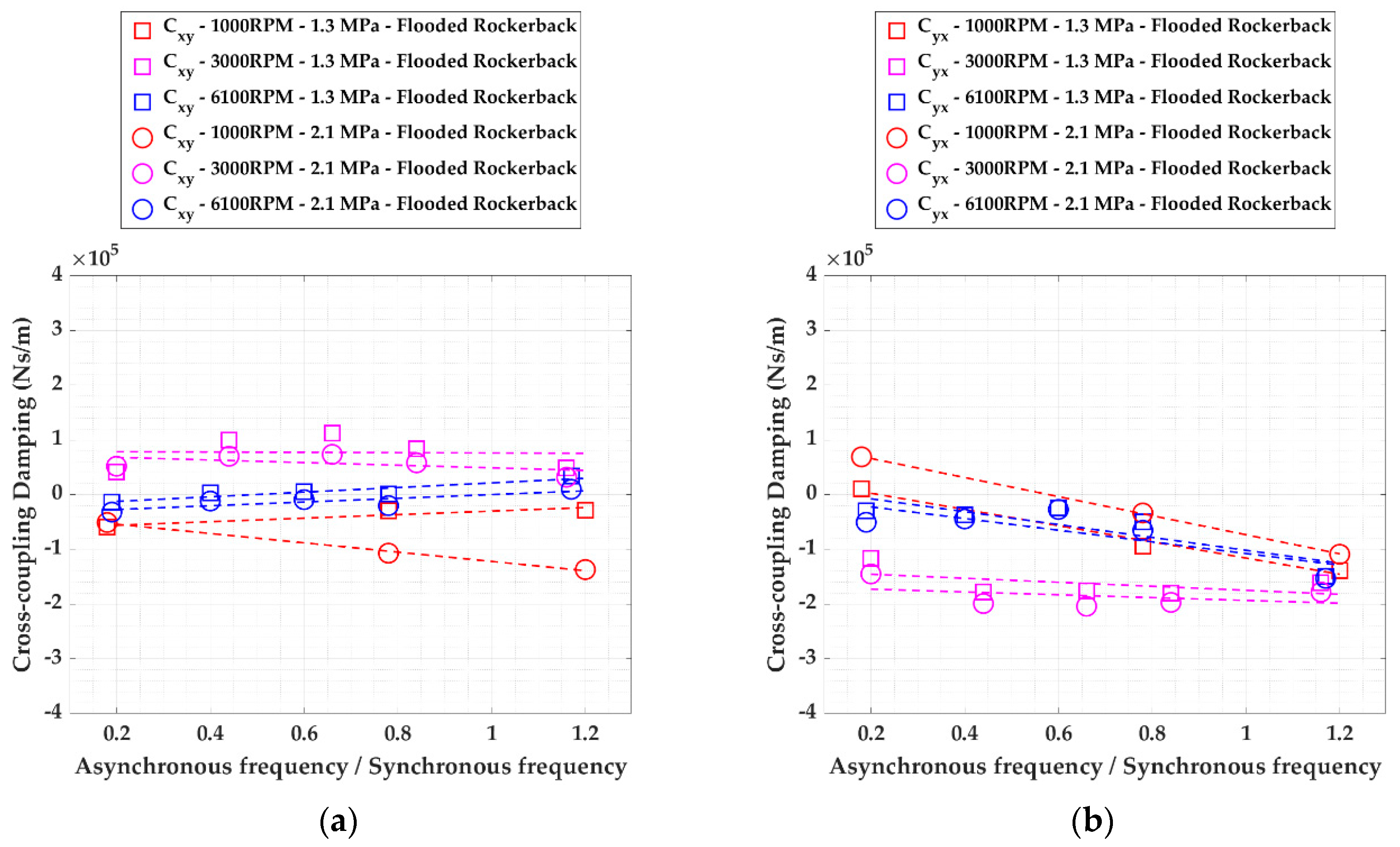
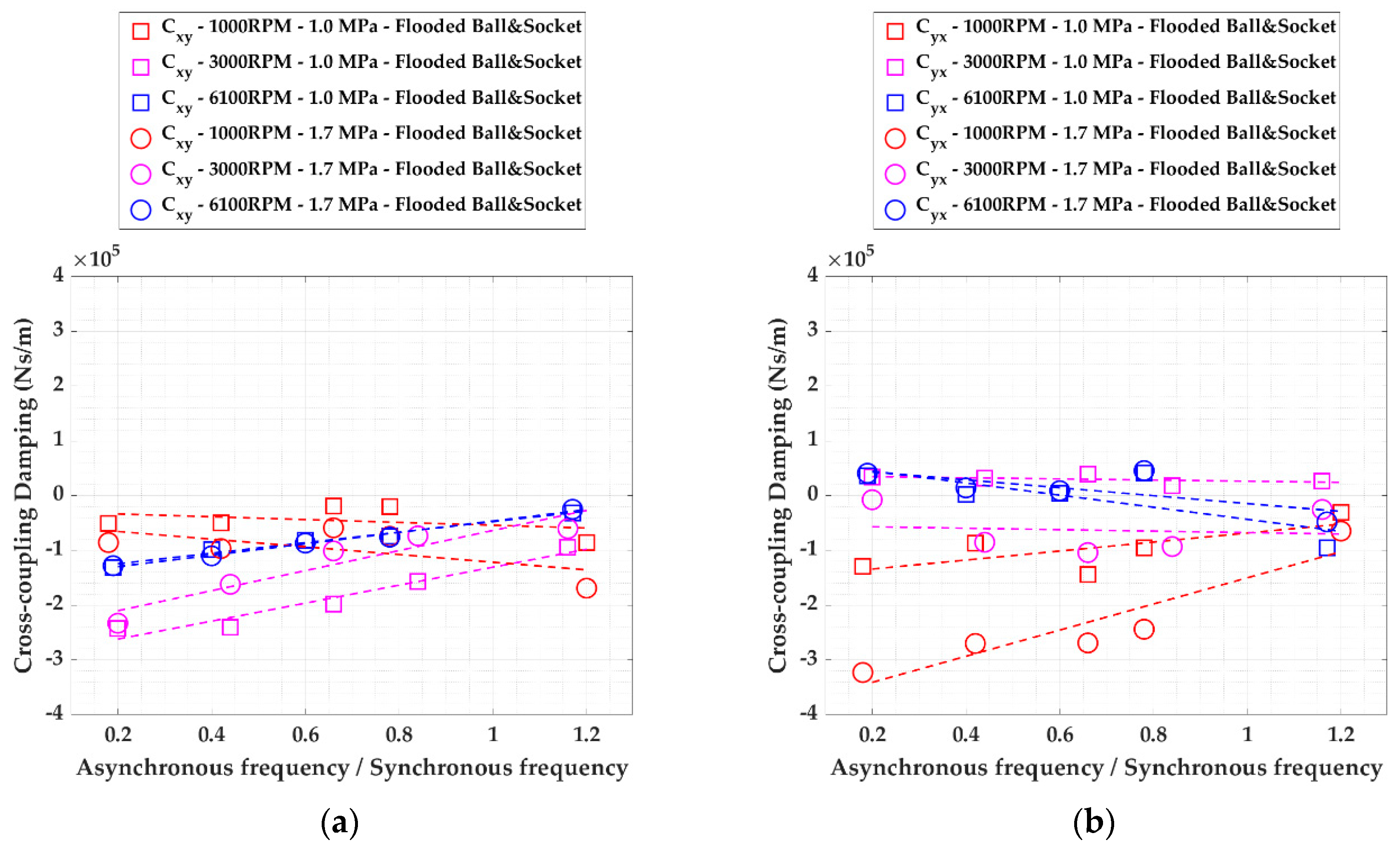
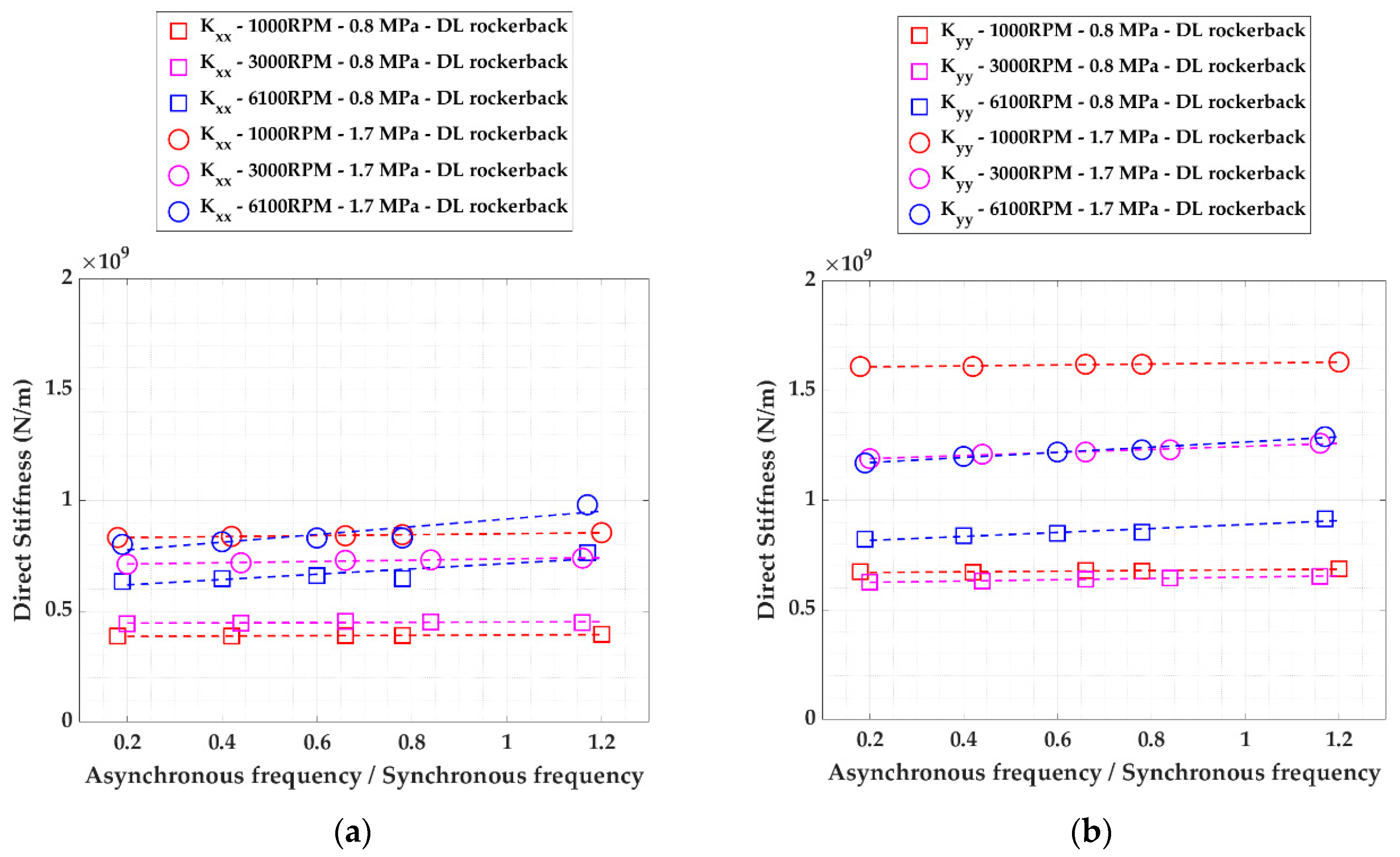
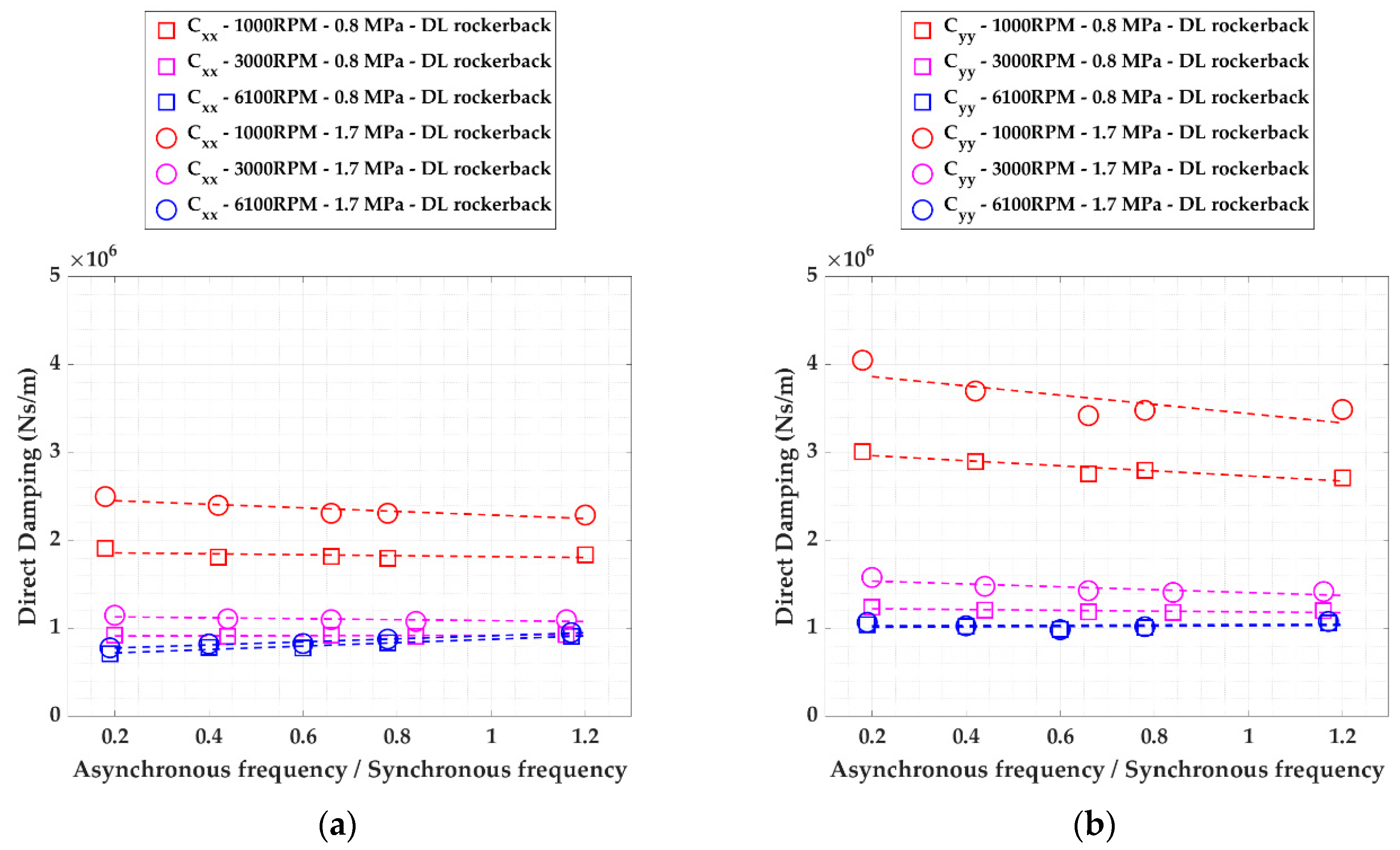
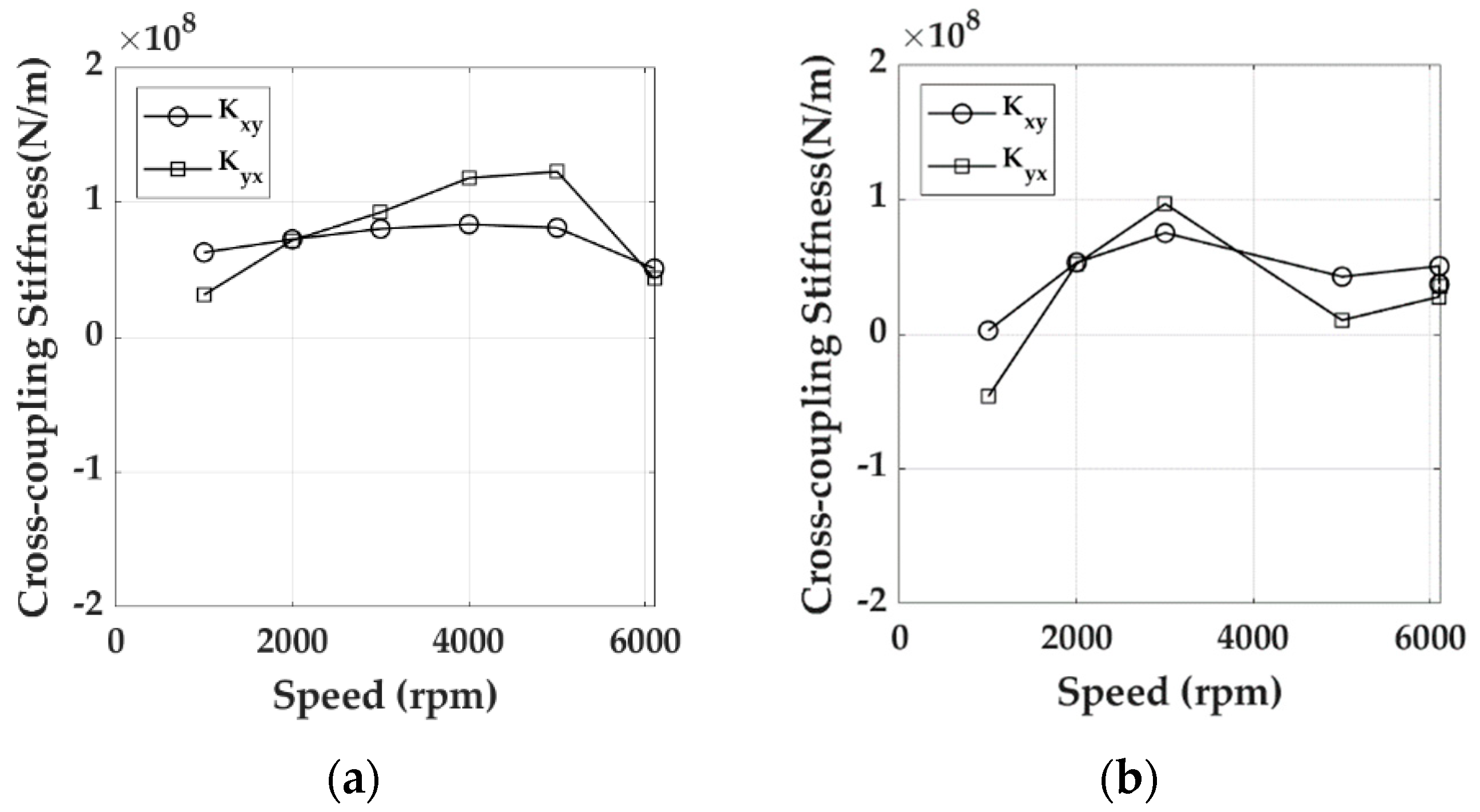
3.2.1. Asynchronous Coefficients
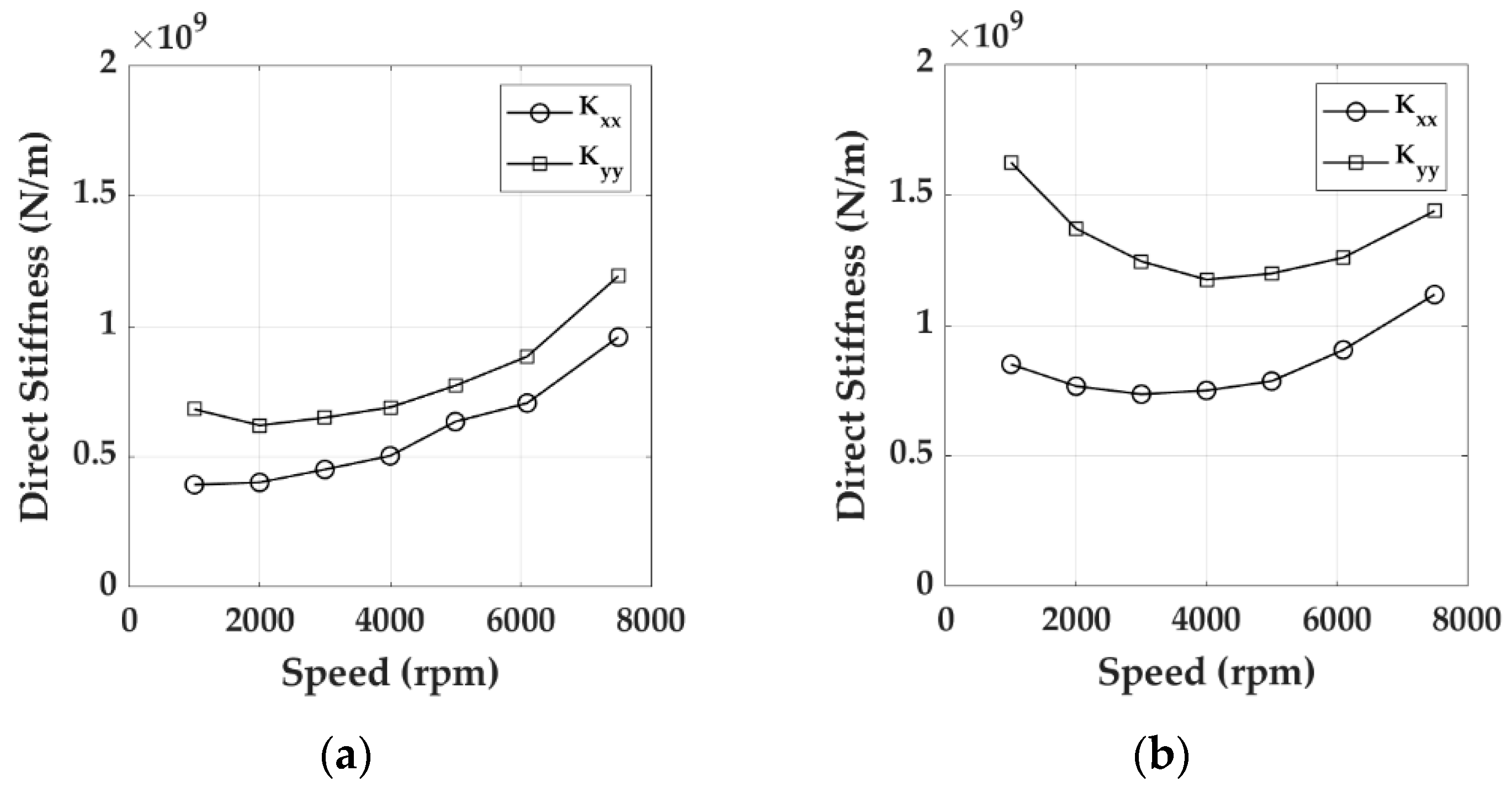
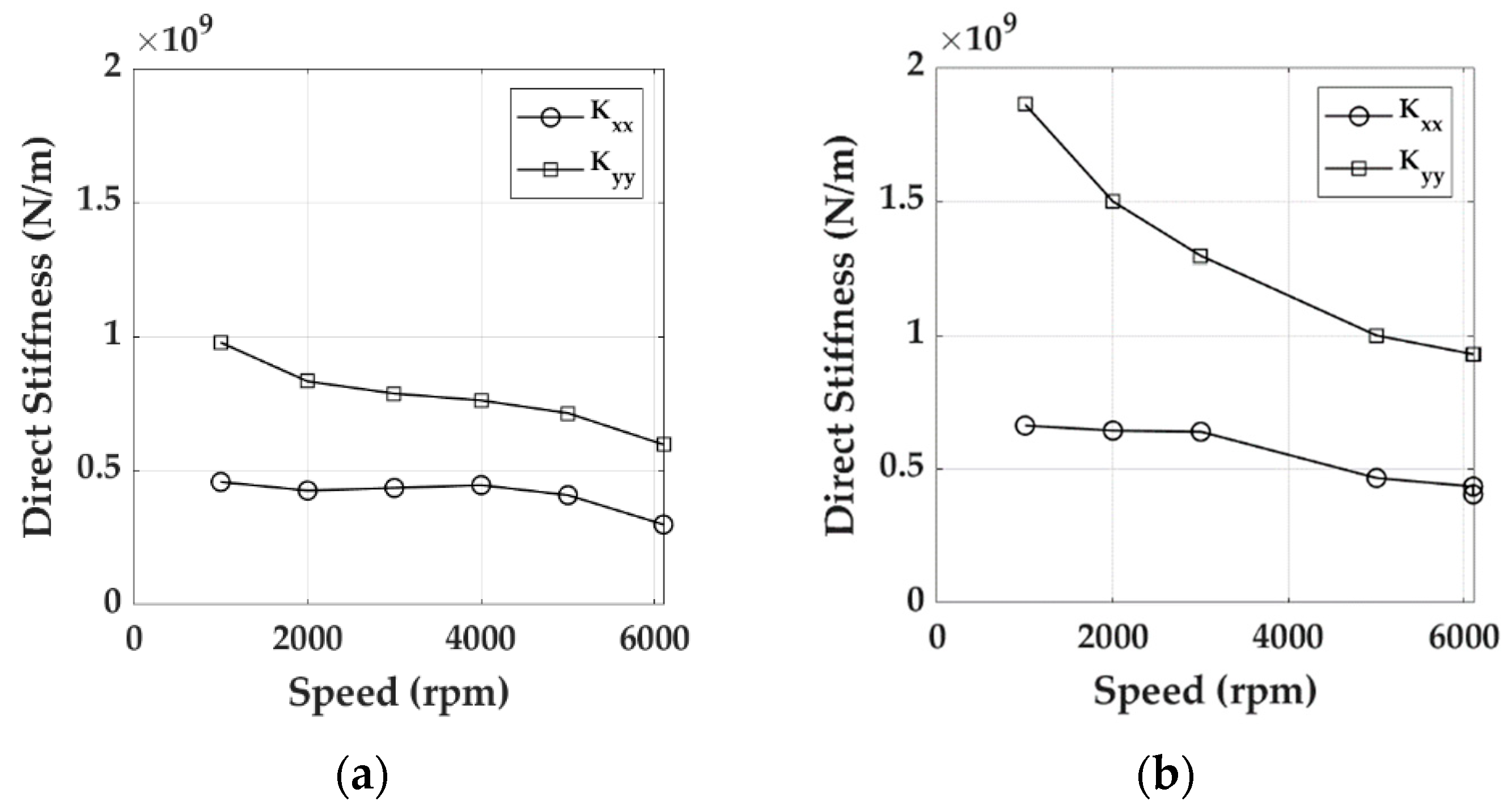
3.2.2. Synchronous Stiffness Coefficients
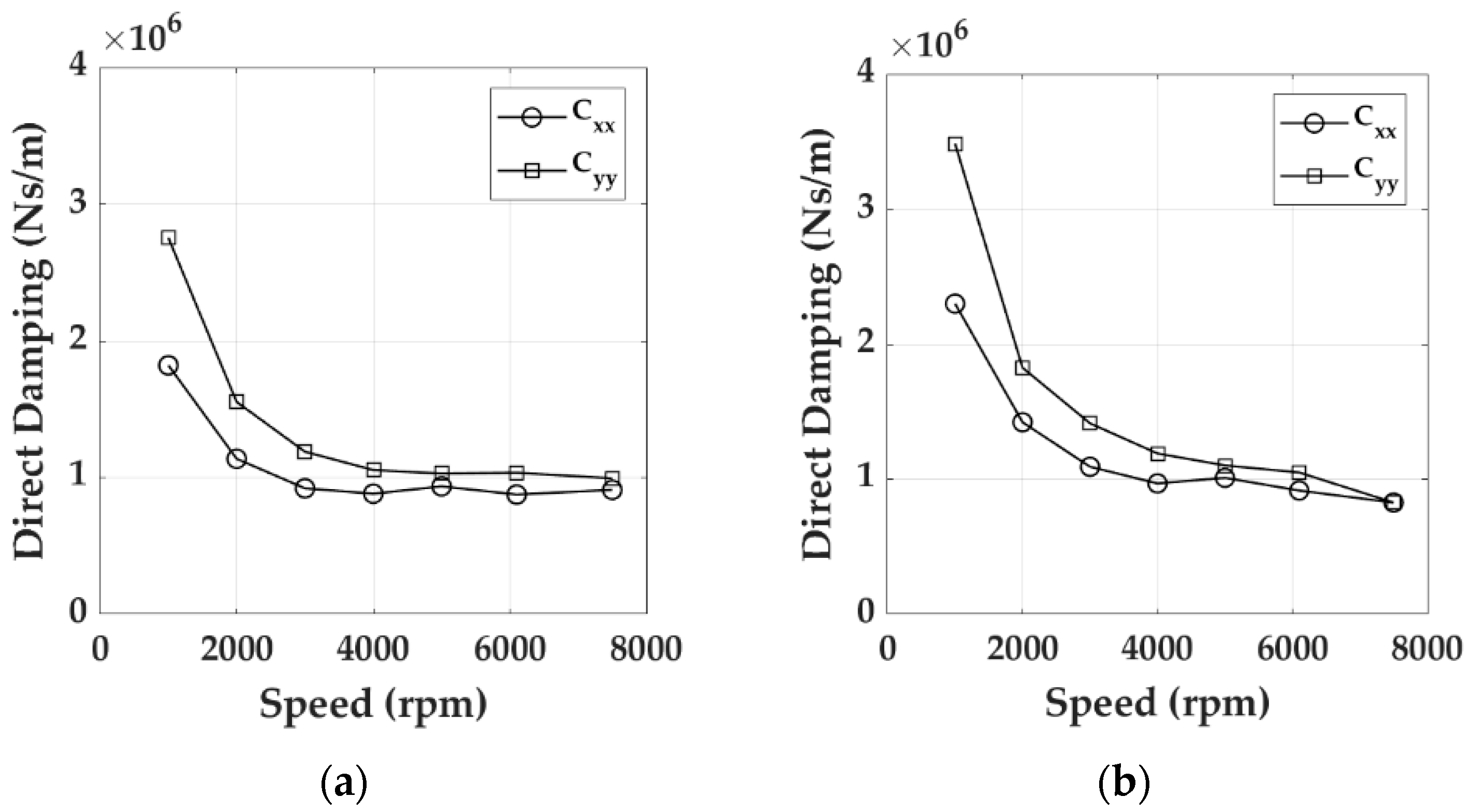
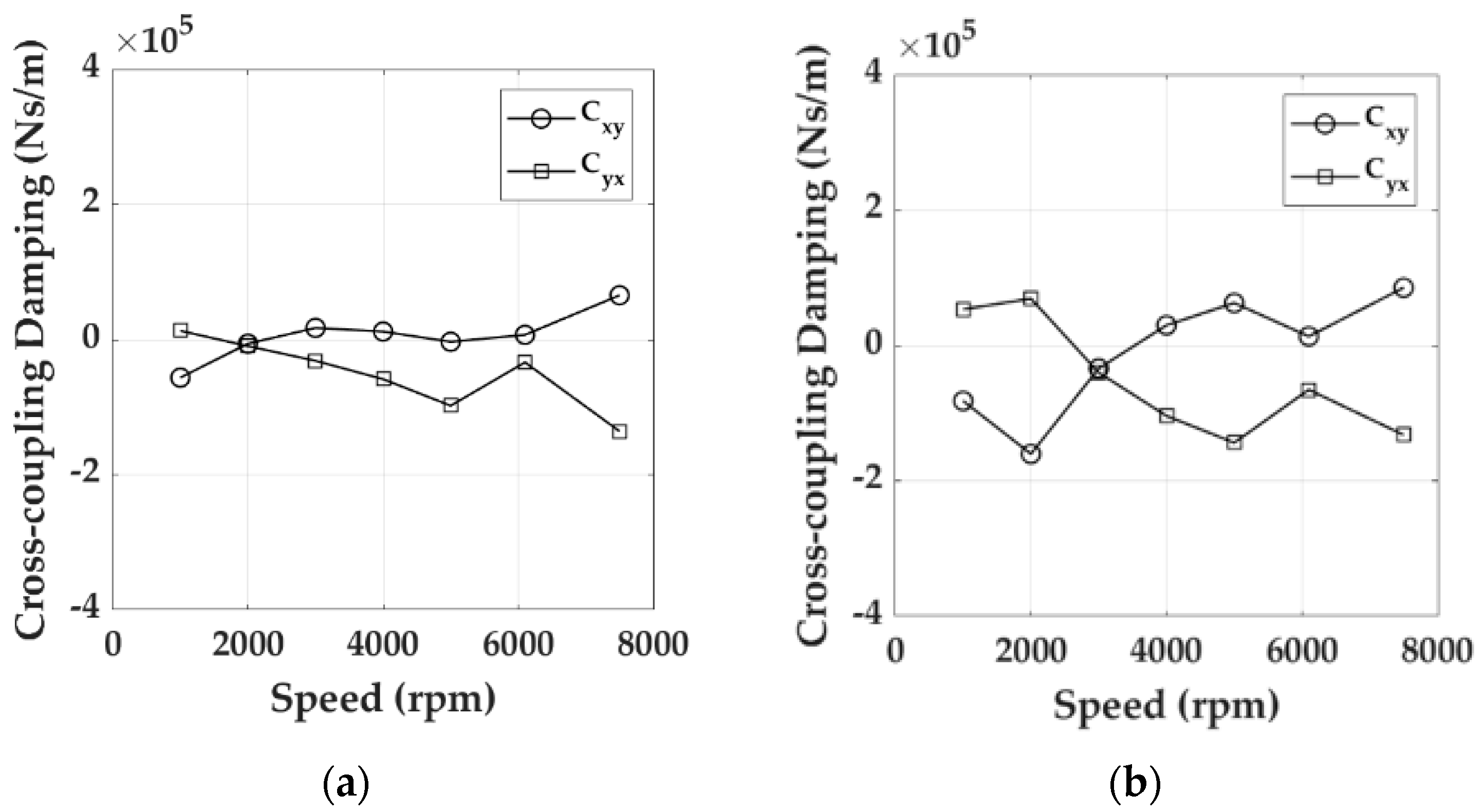
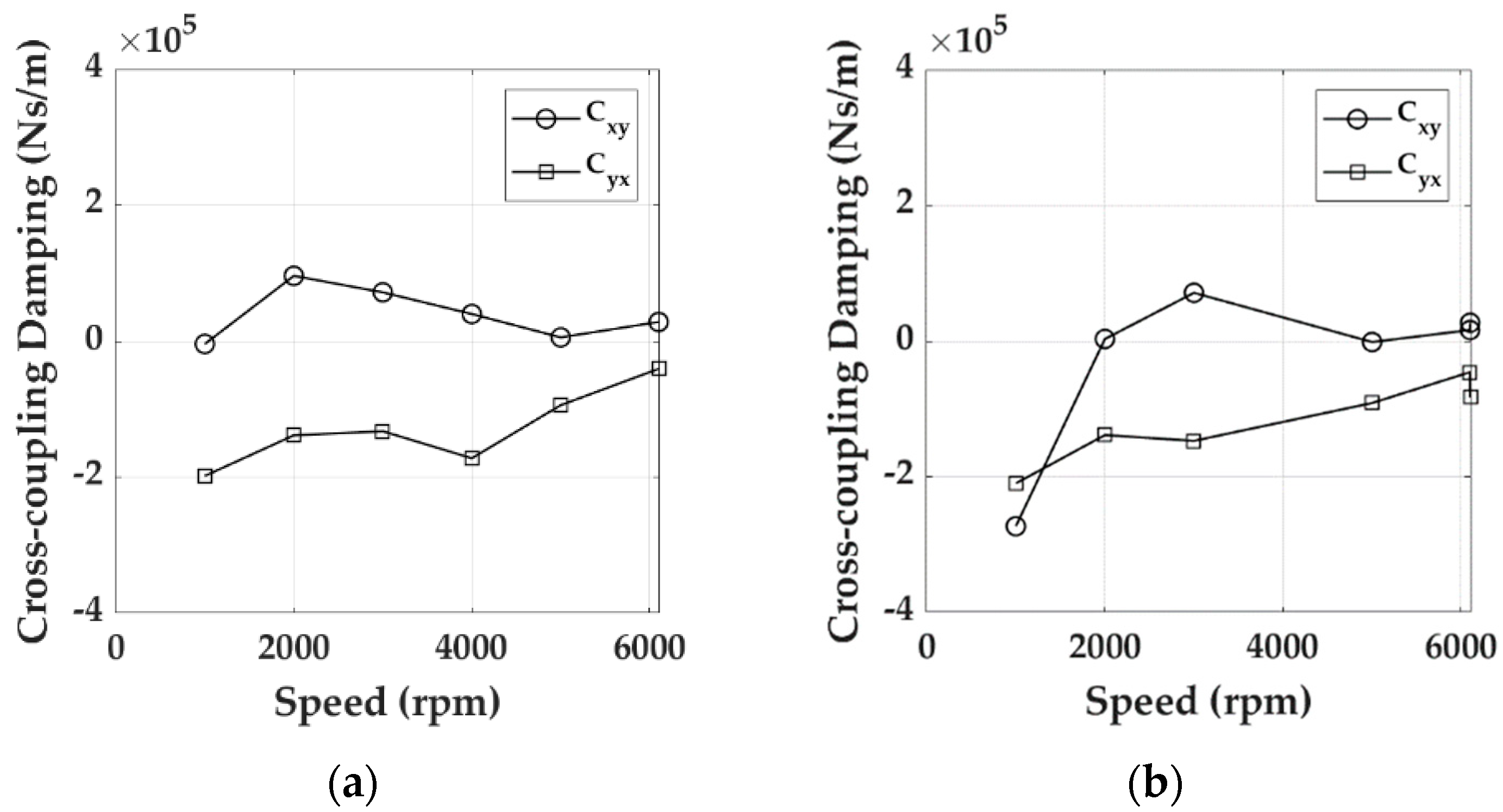
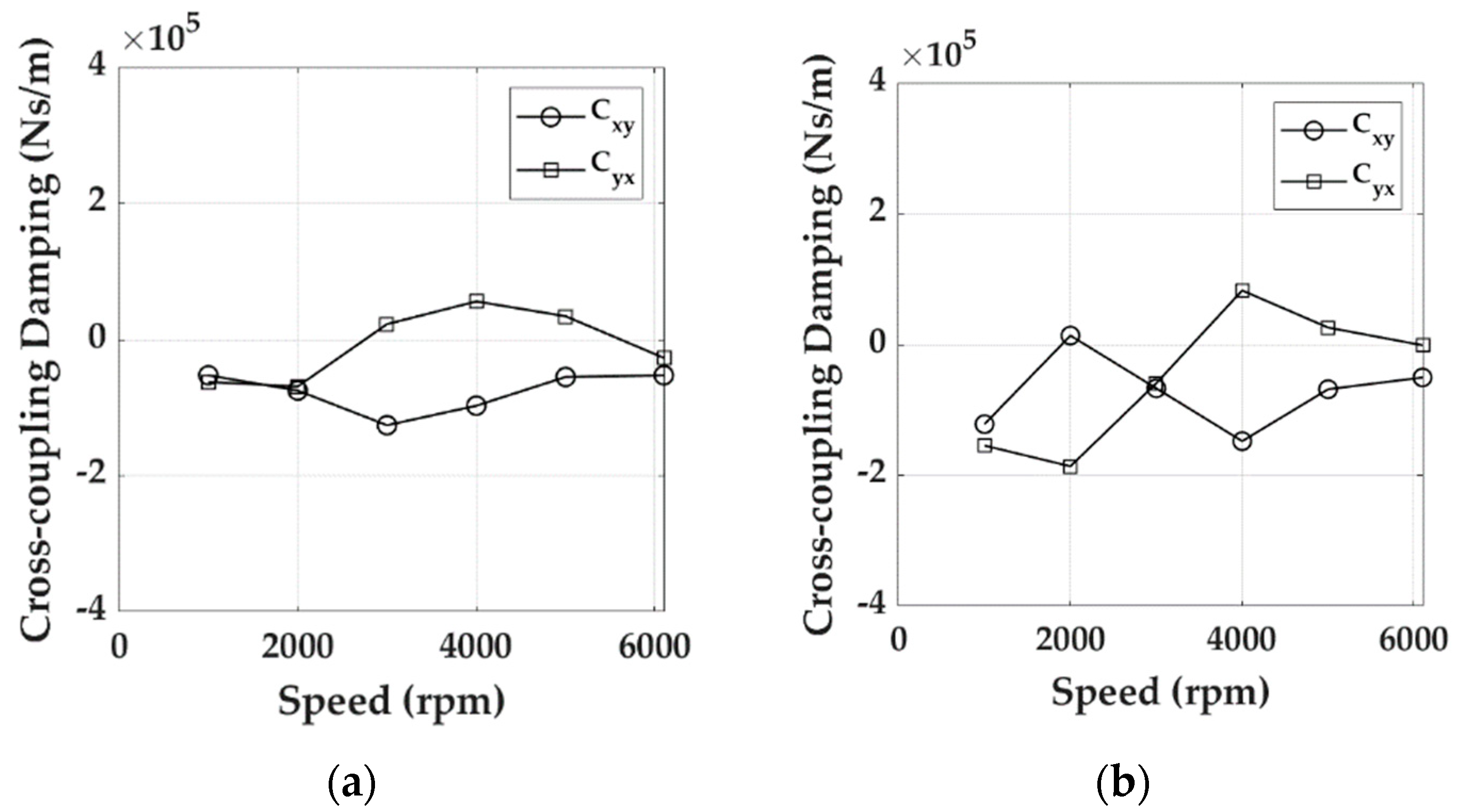
3.2.3. Synchronous Damping Coefficients
3.2.4. The Rotordynamic Behavior
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A


References
- Dimond, T.W.; Sheth, P.N.; Allaire, P.E.; He, M. Identification methods and test results for tilting pad and fixed geometry journal bearing dynamic coefficients—A review. Shock Vib. 2009, 16, 13–43. [Google Scholar] [CrossRef]
- Dimond, T.; Younan, A.; Allaire, P. A review of tilting pad bearing theory. Int. J. Rotating Mach. 2011, 2011, 908469. [Google Scholar] [CrossRef] [Green Version]
- Childs, D.W.; Hale, K.A. Test Apparatus and Facility to Identify the Rotordynamic Coefficients of High-Speed Hydrostatic Bearings. J. Tribol. 1994, 116, 337–344. [Google Scholar] [CrossRef] [Green Version]
- Ha, H.C.; Yang, S.H. Excitation Frequency Effects on the Stiffness and Damping Coefficients of a Five-Pad Tilting Pad Journal Bearing. J. Tribol. 1999, 121, 517–522. [Google Scholar] [CrossRef]
- Ikeda, K.; Hirano, T.; Yamashita, T.; Mikami, M.; Sakakida, H. An Experimental Study of Static and Dynamic Characteristics of a 580 mm (22.8 in.) Diameter Direct Lubrication Tilting Pad Journal Bearing. J. Tribol. 2006, 128, 146–154. [Google Scholar] [CrossRef]
- Wygant, K.; Flack, R.D.; Barrett, L.E. Measured Performance of Tilting Pad Journal Bearings over a Range of Preload—Part. I: Static Operating Conditions. Tribol. Trans. 2004, 47, 576–584. [Google Scholar] [CrossRef]
- Bang, K.B.; Kim, J.H.; Cho, Y.J. Comparison of power loss and pad temperature for leading edge groove tilting pad journal bearings and conventional tilting pad journal bearings. Tribol. Int. 2010, 43, 1287–1293. [Google Scholar] [CrossRef]
- Ciulli, E.; Forte, P.; Libraschi, M.; Nuti, M. Set-up of a novel test plant for high power turbomachinery tilting pad journal bearings. Tribol. Int. 2018, 127, 276–287. [Google Scholar] [CrossRef]
- Chatterton, S.; Pennacchi, P.; Dang, P.V.; Vania, A. A test rig for evaluating tilting–pad journal bearing characteristics. In Proceedings of the 9th international conference on rotor dynamics (IFToMM), Milan, Italy, 22–25 September 2014; pp. 921–930. [Google Scholar]
- Dang, P.V.; Chatterton, S.; Pennacchi, P.; Vania, A. Effect of the load direction on non-nominal five-pad tilting pad journal bearings. Tribol. Int. 2016, 98, 197–211. [Google Scholar] [CrossRef]
- Dang, P.V.; Chatterton, S.; Pennacchi, P. The Effect of the Pivot Stiffness on the Performances of Five-Pad Tilting Pad Bearings. Lubricants 2019, 7, 61. [Google Scholar] [CrossRef] [Green Version]
- Vannini, G.; Cangioli, F.; Ciulli, E.; Nuti, M.; Forte, P.; Kim, J.; Livermore-Hardy, R. Experiments on a Large Flexure Pivot Journal Bearing: Summary of Test Results and Comparison with Predictions. ASME J. Eng. Gas Turbines Power 2020, 142, 031004. [Google Scholar] [CrossRef]
- Ciulli, E.; Forte, P. Nonlinear Response of Tilting Pad Journal Bearings to Harmonic Excitation. Machines 2019, 7, 43. [Google Scholar] [CrossRef] [Green Version]
- Hagemann, T.; Kukla, S.; Schwarze, H. Measurement and Prediction of the Static Operating Conditions of a Large Turbine Tilting-Pad Bearing Under High Circumferential Speeds and Heavy Loads. In Proceedings of the ASME Turbo Expo 2013: Turbine Technical Conference and Exposition. Volume 7B: Structures and Dynamics, San Antonio, TX, USA, 3–7 June 2013; p. V07BT30A019. [Google Scholar] [CrossRef]
- Kukla, S.; Hagemann, T.; Schwarze, H. Measurement and Prediction of the Dynamic Characteristics of a Large Turbine Tilting-Pad Bearing Under High Circumferential Speeds. In Proceedings of the ASME Turbo Expo 2013: Turbine Technical Conference and Exposition. Volume 7B: Structures and Dynamics, San Antonio, TX, USA, 3–7 June 2013; p. V07BT30A020. [Google Scholar] [CrossRef]
- Hopf, G.; Schüler, D. Investigations on Large Turbine Bearings Working Under Transitional Conditions Between Laminar and Turbulent Flow. J. Tribol 1989, 111, 628–634. [Google Scholar] [CrossRef]
- Buchhorn, N.; Stottrop, M.; Bender, B. Influence of Active Cooling at the Trailing Edge on the Thermal Behavior of a Tilting-Pad Journal Bearing. Lubricants 2021, 9, 26. [Google Scholar] [CrossRef]
- Sano, T.; Magoshi, R.; Shinohara, T.; Yoshimine, C.; Nishioka, T.; Tochitani, N.; Sumi, Y. Confirmation of performance and reliability of direct lubricated tilting two pads bearing. IMechE J. Eng.Tribol. 2015, 229, 1011–1021. [Google Scholar] [CrossRef]
- Mermertas, Ü.; Hagemann, T.; Brichart, C. Optimization of a 900 mm Tilting-Pad Journal Bearing in Large Steam Turbines by Advanced Modeling and Validation. ASME J. Eng. Gas Turbines Power 2019, 141, 021033. [Google Scholar] [CrossRef]
- Ciulli, E.; Forte, P.; Libraschi, M.; Naldi, L.; Nuti, M. Characterization of High-Power Turbomachinery Tilting Pad Journal Bearings: First Results Obtained on a Novel Test Bench. Lubricants 2018, 6, 4. [Google Scholar] [CrossRef] [Green Version]
- Ciulli, E.; Forte, P.; Libraschi, M.; Nuti, M. Experimental tests on large size tilting pad journal bearings for turbomachinery. In Proceedings of the 10th International Scientific Conference BALTTRIB 2019, Kaunas, Lithuania, 14–16 November 2019; Volume 159364, pp. 79–86. [Google Scholar]
- Ferraro, R.; Innocenti, A.; Libraschi, M.; Barsanti, M.; Ciulli, E.; Forte, P.; Nuti, M. Dynamic Identification of 280 mm Diameter Tilting Pad Journal Bearings: Test Results and Measurement Uncertainties Assessment of Different Designs. In Proceedings of the ASME Turbo Expo 2020: Turbomachinery Technical Conference and Exposition, Volume 10A: Structures and Dynamics, Virtual, Online, 21–25 September 2020; p. V10AT25A013. [Google Scholar] [CrossRef]
- API Standard 670 Machinery Protection Systems, 5th ed.; API Publishing Services: Washington, DC, USA, 2014.
- Nicholas, J.C. Tilting Pad bearing Design. In Proceedings of the Twenty-Third Turbomachinery Symposium, Texas A&M University, Dallas, TX, USA, 13–15 September 1994; pp. 179–194. [Google Scholar] [CrossRef]
- Szeri, A. Tribology—Friction, Lubrication & Wear; Hemisphere Pubs.: Washington, DC, USA, 1980. [Google Scholar]
- Vannini, G.; Innocenti, A.; Cangioli, F.; Jongsoo, K. Rotordynamic Evaluation of a Large High-Speed Rotor Equipped with Flexure Pivot Journal Bearings and Integral Squeeze Film Damper. In Proceedings of the ASME Turbo Expo 2021: Turbomachinery Technical Conference and Exposition. Volume 9B: Structures and Dynamics, Virtual, Online, 7–11 June 2021; p. V09BT28A001. [Google Scholar] [CrossRef]
- API Standard 617 Axial and Centrifugal Compressors and Expander-Compressors, 8th ed.; API Publishing Services: Washington, DC, USA, 2014.
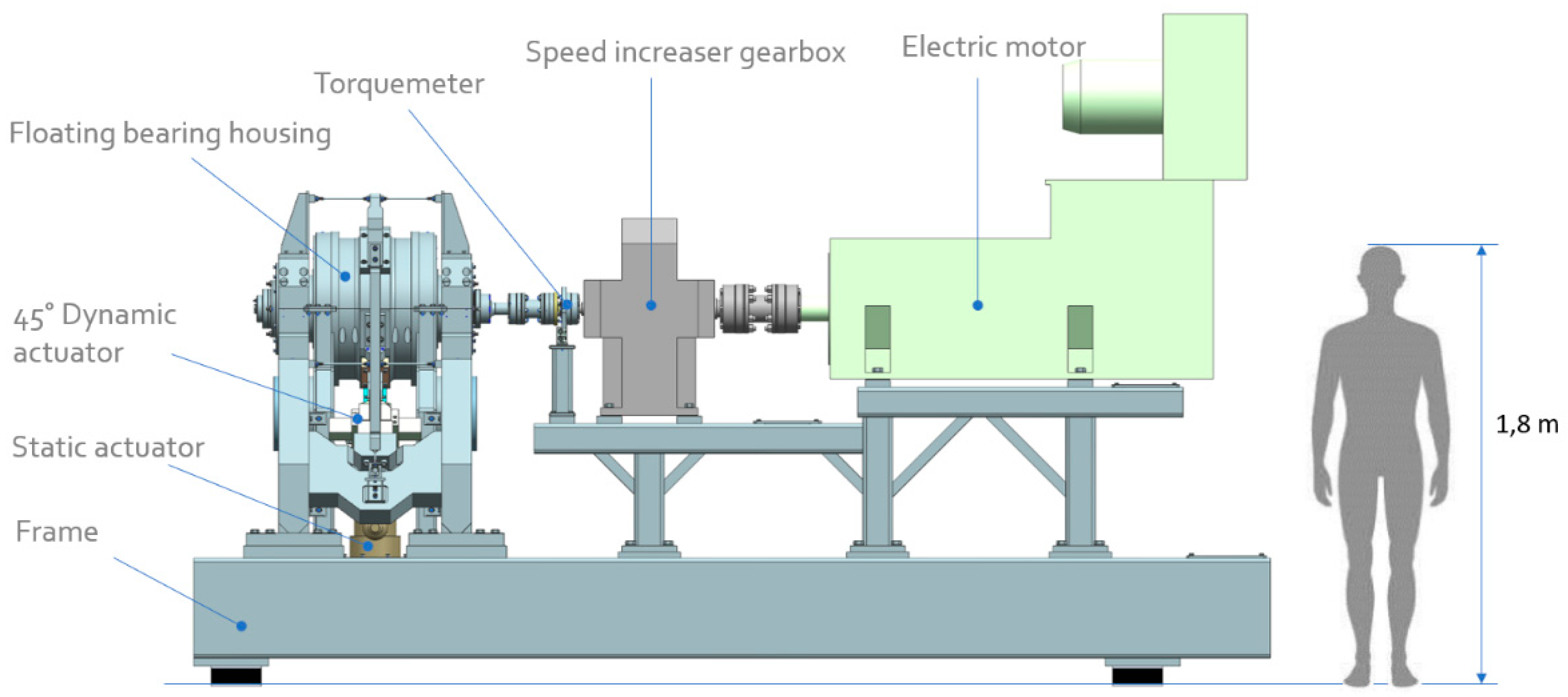
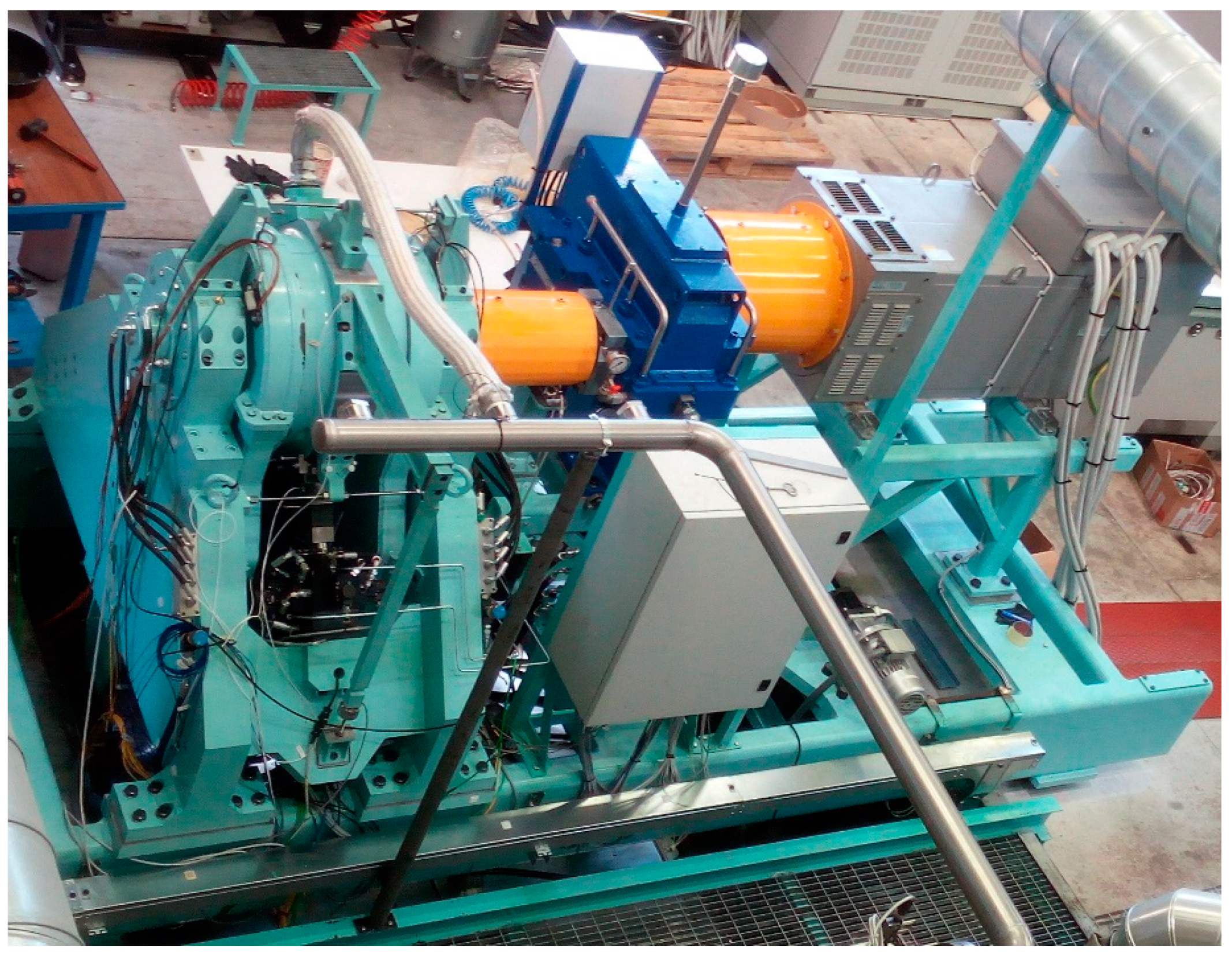
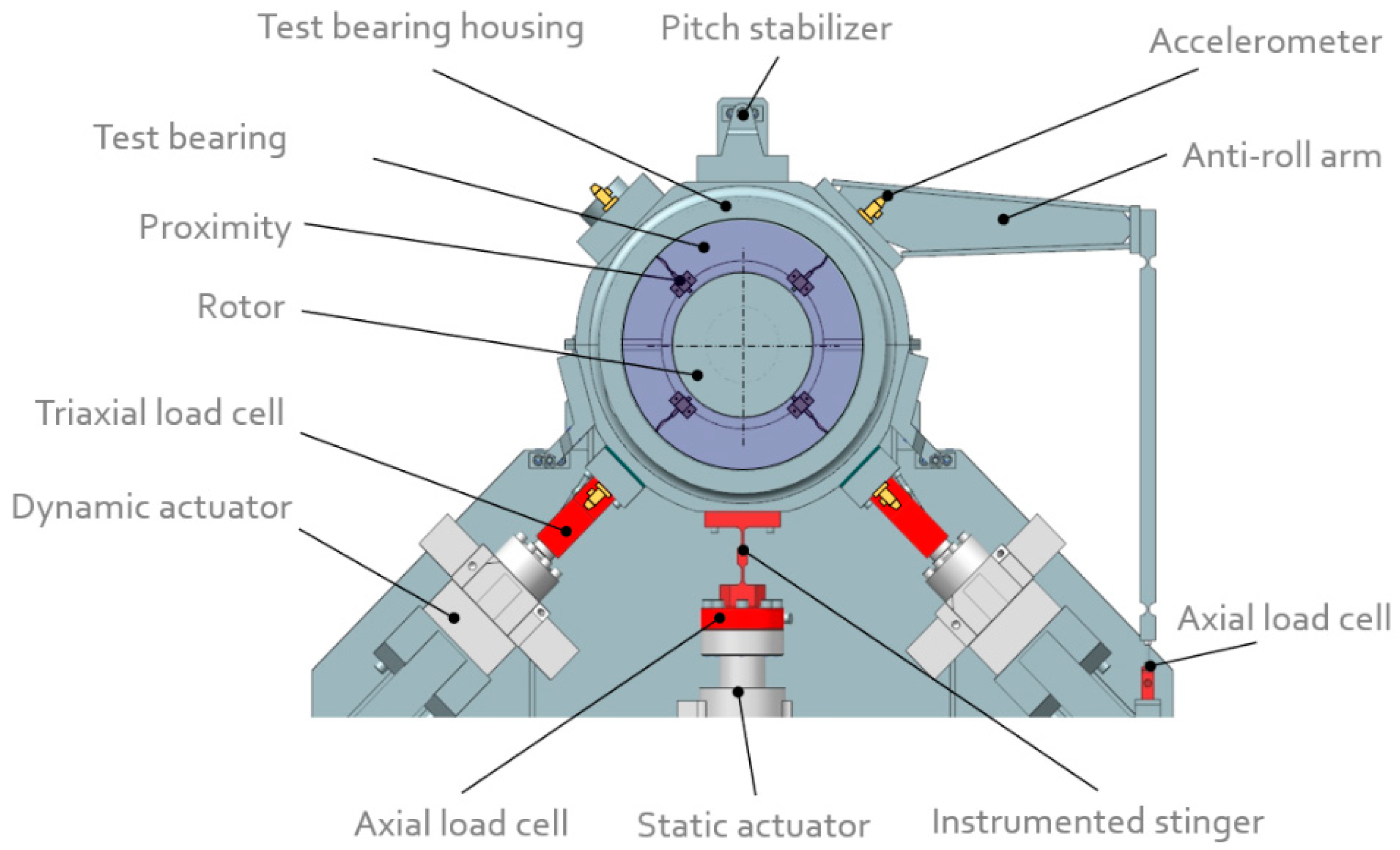
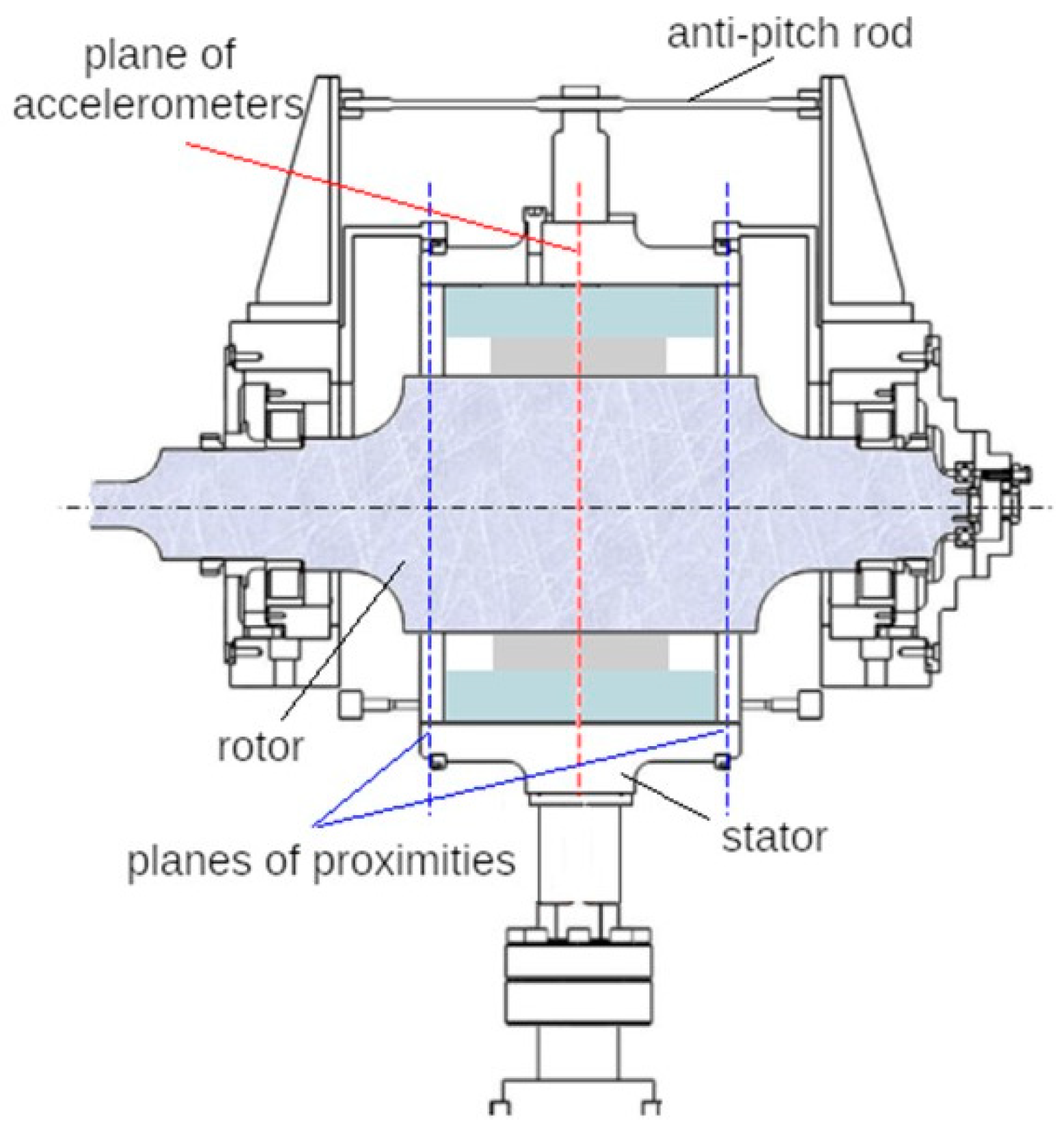











| Characteristic | Unit | Value |
|---|---|---|
| Electric motor maximum power | kW | 630 |
| Electric motor maximum rotational speed | Rpm | 4000 |
| Electric motor nominal torque | Nm | 3000 |
| Bearing rotor speed | Rpm | 0–24,000 |
| Toque meter full scale | Nm | 1000 |
| Static load actuator full scale | kN | 270 |
| Dynamic load actuator full scale | kN | 30 |
| Dynamic load frequency | Hz | 0–350 |
| Bearing oil flow rate | L/min | 125–1100 |
| Bearing oil inlet temperature | °C | 30–120 |
| Bearing storage tank capacity | L | 4000 |
| Pump for the hydraulic jacks maximum pressure | Bar | 315 |
| Plant maximum total power | kW | 1000 |
| Sensor | Type | Range | Linearity Error (%) |
|---|---|---|---|
| Proximitor | Eddy-current sensor | 0–2 mm | 0.1–1.5 |
| Accelerometer | Piezoelectric sensor | ±250 g up to 10 kHz | 0.1–2.1 |
| Triaxial load cell (axial) | Custom Strain gauges sensor | ±35 kN | 1–1.5 |
| Triaxial load cell (tangential) | Custom Strain gauges sensor | ±5 kN | 1.2–2.3 |
| Axial load cell (static load) | Commercial Strain gauges sensor | 0–300 kN | 1.4 |
| Instrumented stinger | Custom Strain gauges sensor | ±2 kN | 4.89 |
| Axial load cell (anti-roll arm) | Commercial Strain gauges sensor | ±2 kN | 0.25 |
| Characteristic | Unit | Value |
|---|---|---|
| Test bearing diameter | mm | 250–300 |
| Test bearing length to diameter ratio | - | 0.4–1 |
| Test bearing rotational speed | rpm | 300–12,000 |
| Test bearing peripheral speed | m/s | 5–150 |
| Test bearing maximum applicable torque | Nm | 500 |
| Static load | kN | 5–270 |
| Dynamic load | kN | 1–40 |
| Dynamic load frequency | Hz | 1–200 |
| Sampling frequency | kHz | 25–100 |
| Bearing oil inlet temperature | °C | 40–120 |
| Characteristic | Direct Lube Rocker Pivot | Flooded Rocker Pivot | Flooded Ball & Socket Pivot |
|---|---|---|---|
| Pad number | 5 | 5 | 4 |
| L/D | 0.7 | 0.55 | 0.7 |
| Pad arc | 54 | 52 | 75 |
| Pivot type | Cylindric | Cylindric | Ball & Socket |
| Min\Max bearing clearance (o/oo) | 1.43\1.78 | 1.57\1.82 | 1.33\1.76 |
| Min\Max bearing clearance (mm) | 0.400\0.498 | 0.440\0.51 | 0.372\0.493 |
| Design load operating conditions (MPa) | 0.8 and 1.7 | 1.3 and 2.1 | 1.0 and 1.7 |
| Shaft Sink | Direct Lube Rocker Pivot | Flooded Rocker Pivot | Flooded Ball & Socket Pivot |
|---|---|---|---|
| Below centered position, s (mm) | 0.287 | 0.294 | 0.350 |
| Below bearing clearance, s-cr (mm) | 0.046 | 0.039 | 0.103 |
| Bearing Data | 1st Synch Mode Log Dec | 1st Synch Mode Freq (rpm) | 1st Mode Log Dec @ MCS |
|---|---|---|---|
| Direct Lube Rocker Pivot | 0.211 | 1236 | 0.077 |
| Flooded Rocker Pivot | 0.041 | 1267 | 0.021 |
| Flooded Ball&Socket Pivot | 0.143 | 1278 | 0.091 |
| Bearing Data | 2nd Synch Mode Log Dec | 2nd Synch Mode Freq (rpm) | AF2 |
|---|---|---|---|
| Direct Lube Rocker Pivot | 0.385 | 4354.4 | 8.1 |
| Flooded Rocker Pivot | 0.176 | 4275.7 | 17 |
| Flooded Ball&Socket Pivot | 0.457 | 4354.8 | 6.8 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ciulli, E.; Ferraro, R.; Forte, P.; Innocenti, A.; Nuti, M. Experimental Characterization of Large Turbomachinery Tilting Pad Journal Bearings. Machines 2021, 9, 273. https://doi.org/10.3390/machines9110273
Ciulli E, Ferraro R, Forte P, Innocenti A, Nuti M. Experimental Characterization of Large Turbomachinery Tilting Pad Journal Bearings. Machines. 2021; 9(11):273. https://doi.org/10.3390/machines9110273
Chicago/Turabian StyleCiulli, Enrico, Riccardo Ferraro, Paola Forte, Alice Innocenti, and Matteo Nuti. 2021. "Experimental Characterization of Large Turbomachinery Tilting Pad Journal Bearings" Machines 9, no. 11: 273. https://doi.org/10.3390/machines9110273
APA StyleCiulli, E., Ferraro, R., Forte, P., Innocenti, A., & Nuti, M. (2021). Experimental Characterization of Large Turbomachinery Tilting Pad Journal Bearings. Machines, 9(11), 273. https://doi.org/10.3390/machines9110273







