Accretion Flow Morphology in Numerical Simulations of Black Holes from the ngEHT Model Library: The Impact of Radiation Physics
Abstract
:1. Introduction
2. Numerical Simulations
2.1. RIAF + Hotspot Solutions
2.2. GRMHD Simulations
2.3. Two-Temperature Physics
2.4. Radiative GRMHD
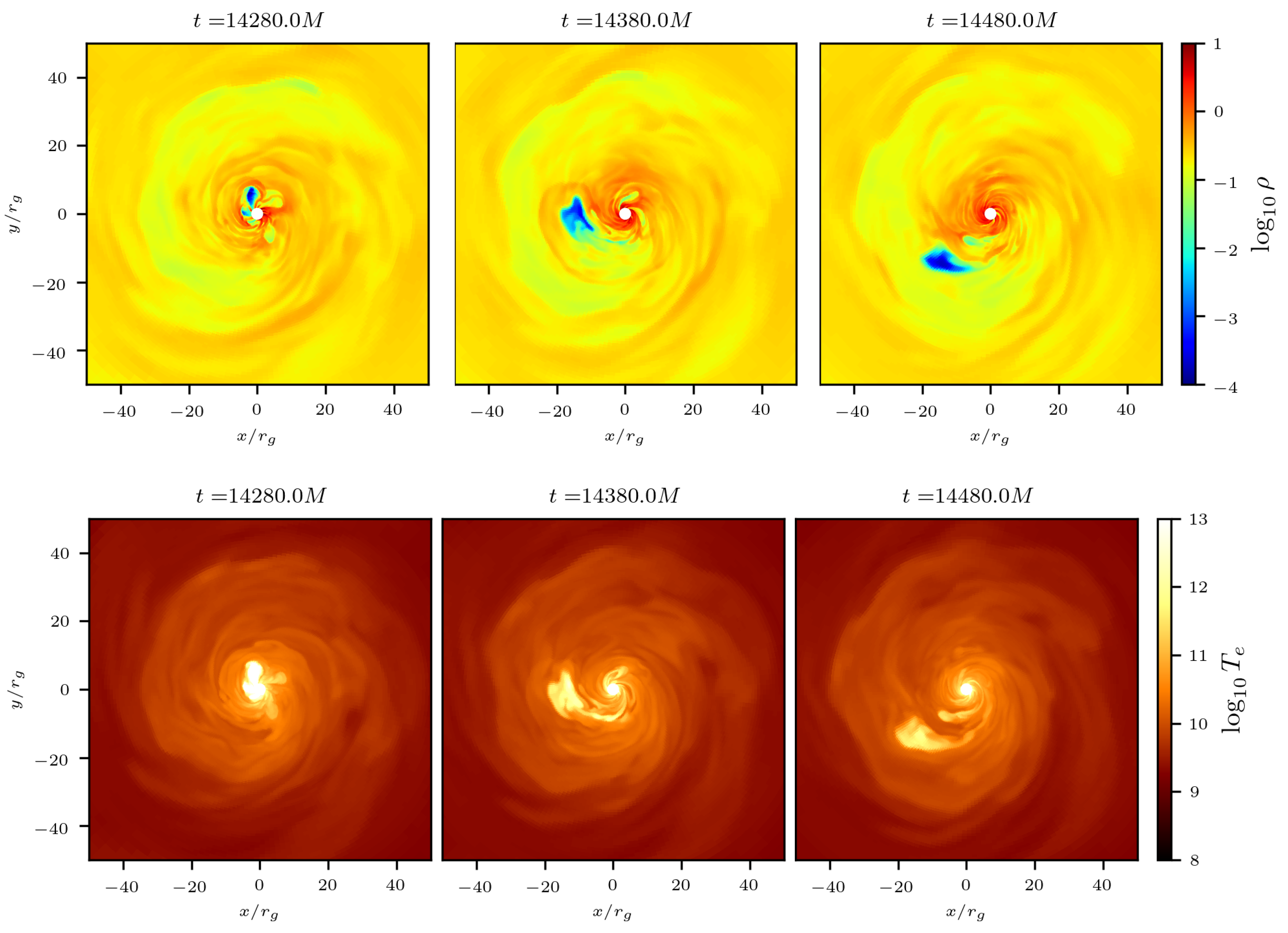
3. Results
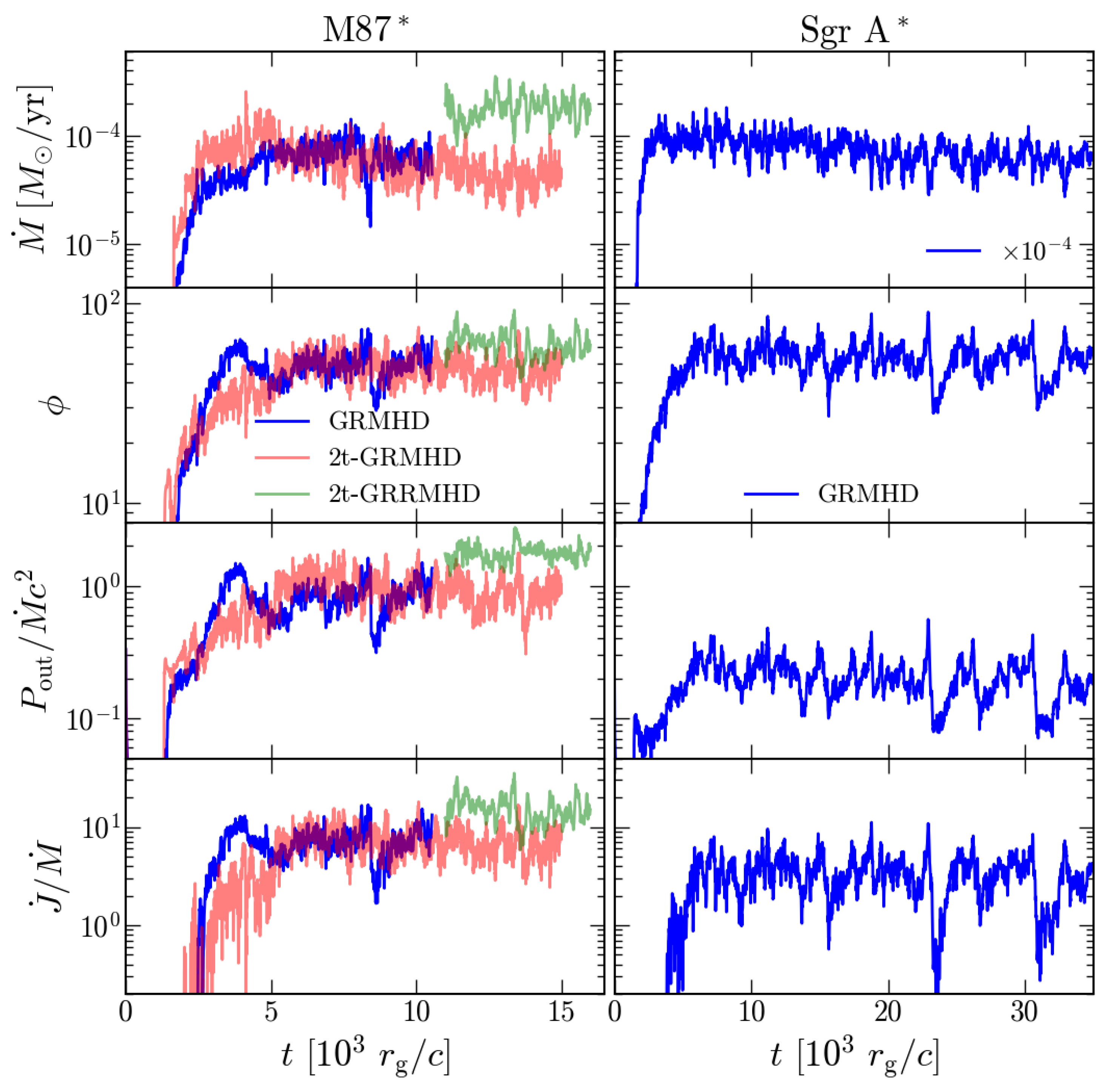
3.1. Temporal Behavior of Horizon Fluxes
3.2. Disk-Averaged Quantities
3.3. Jet Properties
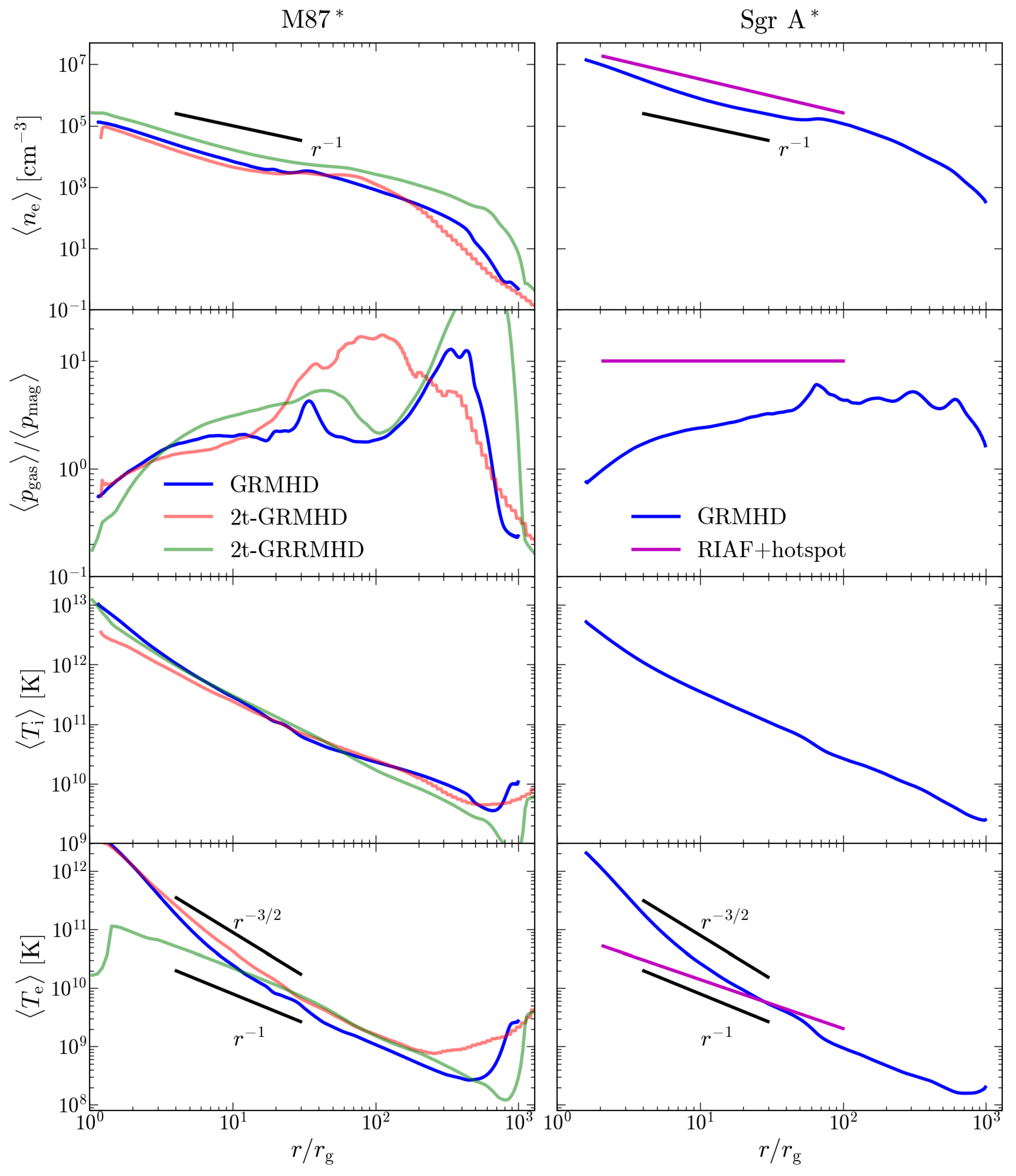
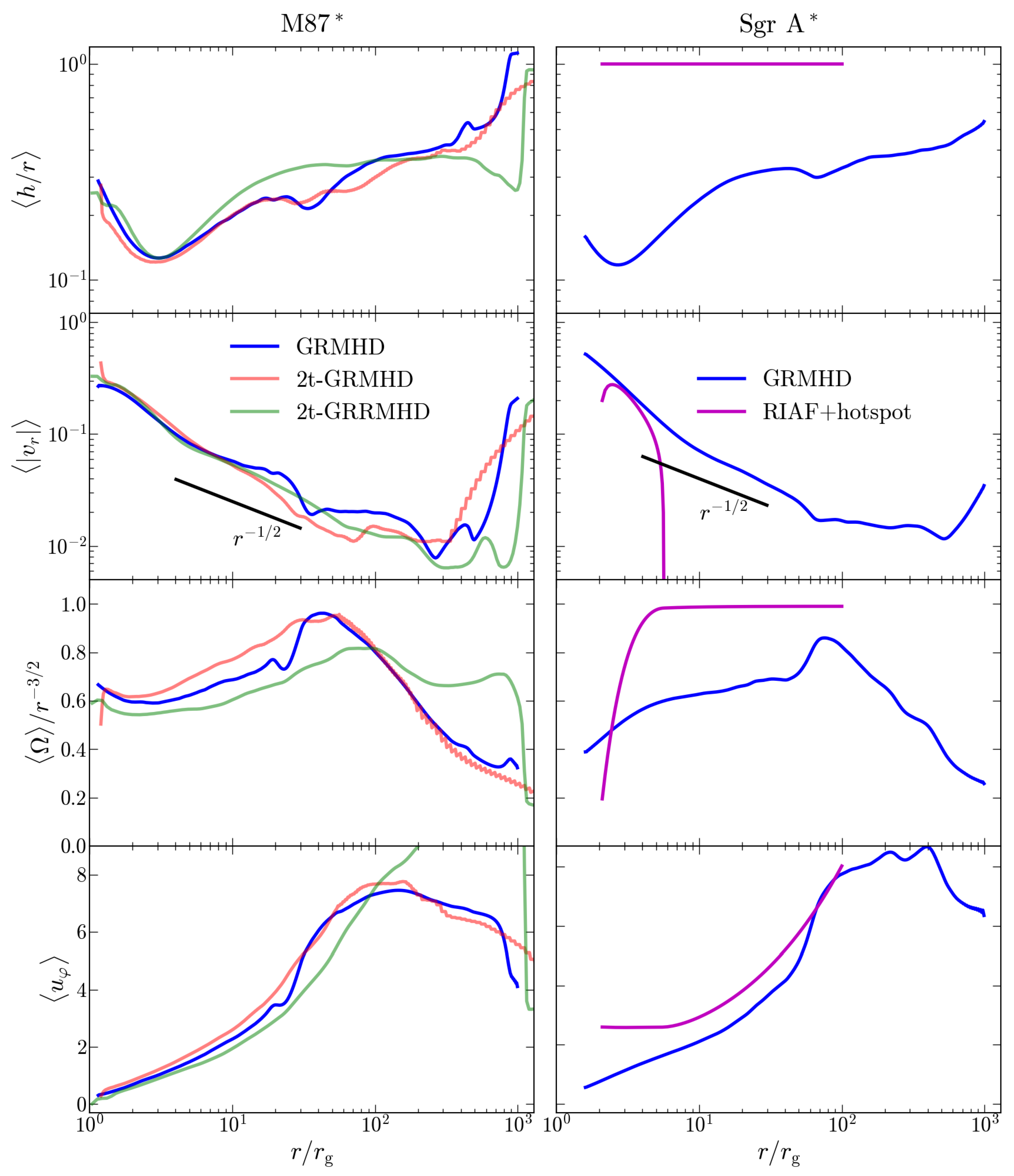
3.4. Axisymmetrized Profiles
3.5. Orbiting Hotspot in a RIAF Model
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | https://www.ngeht.org/ (accessed on 22 November 2022). |
| 2 | https://challenge.ngeht.org/ (accessed on 22 November 2022). |
| 3 | Specific enthalpy includes the rest-mass energy contribution in our definition from Section 2.3. |
References
- Akiyama, K. et al. [Event Horizon Telescope Collaboration]. First M87 Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole. Astrophys. J. Lett. 2019, 875, L1. [Google Scholar] [CrossRef]
- Akiyama, K. et al. [Event Horizon Telescope Collaboration]. First Sagittarius A* Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole in the Center of the Milky Way. Astrophys. J. Lett. 2022, 930, L12. [Google Scholar] [CrossRef]
- Doeleman, S.; Blackburn, L.; Dexter, J.; Gomez, J.L.; Johnson, M.D.; Palumbo, D.C.; Weintroub, J.; Farah, J.R.; Fish, V.; Loinard, L.; et al. Studying Black Holes on Horizon Scales with VLBI Ground Arrays. Bulletin of the AAS, 2019, Volume 51. Available online: https://baas.aas.org/pub/2020n7i256 (accessed on 22 November 2022).
- Roelofs, F.; Blackburn, L.; Lindahl, G.; Doeleman, S.S.; Johnson, M.D.; Arras, P.; Chatterjee, K.; Emami, R.; Fromm, C.; Fuentes, A.; et al. The ngEHT Analysis Challenges. Galaxies 2022, 11, 12. [Google Scholar] [CrossRef]
- Raymond, A.W.; Palumbo, D.; Paine, S.N.; Blackburn, L.; Córdova Rosado, R.; Doeleman, S.S.; Farah, J.R.; Johnson, M.D.; Roelofs, F.; Tilanus, R.P.J.; et al. Evaluation of New Submillimeter VLBI Sites for the Event Horizon Telescope. Astrophys. J. Suppl. Ser. 2021, 253, 5. [Google Scholar] [CrossRef]
- Shakura, N.I.; Sunyaev, R.A. Reprint of 1973A&A....24..337S. Black holes in binary systems. Observational appearance. Astron. Astrophys. 1973, 500, 33–51. [Google Scholar]
- Rees, M.J.; Phinney, E.S.; Begelman, M.C.; Blandford, R.D. Ion-supported tori and the origin of radio jets. Nature 1982, 295, 17–21. [Google Scholar] [CrossRef]
- Narayan, R.; Yi, I.; Mahadevan, R. Explaining the spectrum of Sagittarius A* with a model of an accreting black hole. Nature 1995, 374, 623–625. [Google Scholar] [CrossRef]
- Quataert, E.; Gruzinov, A. Convection-dominated Accretion Flows. Astrophys. J. 2000, 539, 809–814. [Google Scholar] [CrossRef] [Green Version]
- Narayan, R.; Igumenshchev, I.V.; Abramowicz, M.A. Magnetically Arrested Disk: An Energetically Efficient Accretion Flow. PASJ 2003, 55, L69–L72. [Google Scholar] [CrossRef] [Green Version]
- McKinney, J.C. General relativistic magnetohydrodynamic simulations of the jet formation and large-scale propagation from black hole accretion systems. Mon. Not. R. Astron. Soc. 2006, 368, 1561–1582. [Google Scholar] [CrossRef] [Green Version]
- Komissarov, S.S.; Barkov, M.V.; Vlahakis, N.; Königl, A. Magnetic acceleration of relativistic active galactic nucleus jets. Mon. Not. R. Astron. Soc. 2007, 380, 51–70. [Google Scholar] [CrossRef] [Green Version]
- Tchekhovskoy, A.; Narayan, R.; McKinney, J.C. Efficient generation of jets from magnetically arrested accretion on a rapidly spinning black hole. Mon. Not. R. Astron. Soc. 2011, 418, L79–L83. [Google Scholar] [CrossRef] [Green Version]
- Narayan, R.; Sa̧dowski, A.; Penna, R.F.; Kulkarni, A.K. GRMHD simulations of magnetized advection-dominated accretion on a non-spinning black hole: Role of outflows. Mon. Not. R. Astron. Soc. 2012, 426, 3241–3259. [Google Scholar] [CrossRef]
- Tchekhovskoy, A.; Bromberg, O. Three-dimensional relativistic MHD simulations of active galactic nuclei jets: Magnetic kink instability and Fanaroff–Riley dichotomy. Mon. Not. R. Astron. Soc. 2016, 461, L46–L50. [Google Scholar] [CrossRef] [Green Version]
- Yuan, F.; Quataert, E.; Narayan, R. Nonthermal Electrons in Radiatively Inefficient Accretion Flow Models of Sagittarius A*. Astrophys. J. 2003, 598, 301–312. [Google Scholar] [CrossRef]
- Lucchini, M.; Krauß, F.; Markoff, S. The unique case of the active galactic nucleus core of M87: A misaligned low-power blazar? Mon. Not. R. Astron. Soc. 2019, 489, 1633–1643. [Google Scholar] [CrossRef]
- Akiyama, K. et al. [Event Horizon Telescope Collaboration]. First M87 Event Horizon Telescope Results. V. Physical Origin of the Asymmetric Ring. Astrophys. J. Lett. 2019, 875, L5. [Google Scholar] [CrossRef]
- Akiyama, K. et al. [Event Horizon Telescope Collaboration]. First M87 Event Horizon Telescope Results. VIII. Magnetic Field Structure near The Event Horizon. Astrophys. J. Lett. 2021, 910, L13. [Google Scholar] [CrossRef]
- Akiyama, K. et al. [Event Horizon Telescope Collaboration]. First Sagittarius A* Event Horizon Telescope Results. V. Testing Astrophysical Models of the Galactic Center Black Hole. Astrophys. J. Lett. 2022, 930, L16. [Google Scholar] [CrossRef]
- Igumenshchev, I.V.; Narayan, R.; Abramowicz, M.A. Three-dimensional Magnetohydrodynamic Simulations of Radiatively Inefficient Accretion Flows. Astrophys. J. 2003, 592, 1042–1059. [Google Scholar] [CrossRef]
- Blandford, R.D.; Znajek, R.L. Electromagnetic extraction of energy from Kerr black holes. Mon. Not. R. Astron. Soc. 1977, 179, 433–456. [Google Scholar] [CrossRef]
- Gammie, C.F.; McKinney, J.C.; Tóth, G. HARM: A Numerical Scheme for General Relativistic Magnetohydrodynamics. Astrophys. J. 2003, 589, 444–457. [Google Scholar] [CrossRef]
- Fragile, P.C.; Blaes, O.M.; Anninos, P.; Salmonson, J.D. Global General Relativistic Magnetohydrodynamic Simulation of a Tilted Black Hole Accretion Disk. Astrophys. J. 2007, 668, 417–429. [Google Scholar] [CrossRef] [Green Version]
- Chael, A.; Narayan, R.; Johnson, M.D. Two-temperature, Magnetically Arrested Disc simulations of the jet from the supermassive black hole in M87. Mon. Not. R. Astron. Soc. 2019, 486, 2873–2895. [Google Scholar] [CrossRef]
- Porth, O.; Chatterjee, K.; Narayan, R.; Gammie, C.F.; Mizuno, Y.; Anninos, P.; Baker, J.G.; Bugli, M.; Chan, C.k.; Davelaar, J.; et al. The Event Horizon General Relativistic Magnetohydrodynamic Code Comparison Project. Astrophys. J. Suppl. Ser. 2019, 243, 26. [Google Scholar] [CrossRef] [Green Version]
- Narayan, R.; Chael, A.; Chatterjee, K.; Ricarte, A.; Curd, B. Jets in magnetically arrested hot accretion flows: Geometry, power, and black hole spin-down. Mon. Not. R. Astron. Soc. 2022, 511, 3795–3813. [Google Scholar] [CrossRef]
- Janssen, M.; Falcke, H.; Kadler, M.; Ros, E.; Wielgus, M.; Akiyama, K.; Baloković, M.; Blackburn, L.; Bouman, K.L.; Chael, A.; et al. Event Horizon Telescope observations of the jet launching and collimation in Centaurus A. Nat. Astron. 2021, 5, 1017–1028. [Google Scholar] [CrossRef]
- Broderick, A.E.; Loeb, A. Imaging optically thin hotspots near the black hole horizon of Sgr A* at radio and near-infrared wavelengths. Mon. Not. R. Astron. Soc. 2006, 367, 905–916. [Google Scholar] [CrossRef]
- Broderick, A.E.; McKinney, J.C. Parsec-scale Faraday Rotation Measures from General Relativistic Magnetohydrodynamic Simulations of Active Galactic Nucleus Jets. Astrophys. J. 2010, 725, 750–773. [Google Scholar] [CrossRef]
- Tiede, P.; Pu, H.Y.; Broderick, A.E.; Gold, R.; Karami, M.; Preciado-López, J.A. Spacetime Tomography Using the Event Horizon Telescope. Astrophys. J. 2020, 892, 132. [Google Scholar] [CrossRef]
- Broderick, A.E.; Fish, V.L.; Doeleman, S.S.; Loeb, A. Evidence for Low Black Hole Spin and Physically Motivated Accretion Models from Millimeter-VLBI Observations of Sagittarius A*. Astrophys. J. 2011, 735, 110. [Google Scholar] [CrossRef]
- Broderick, A.E.; Fish, V.L.; Johnson, M.D.; Rosenfeld, K.; Wang, C.; Doeleman, S.S.; Akiyama, K.; Johannsen, T.; Roy, A.L. Modeling Seven Years of Event Horizon Telescope Observations with Radiatively Inefficient Accretion Flow Models. Astrophys. J. 2016, 820, 137. [Google Scholar] [CrossRef]
- Pu, H.Y.; Akiyama, K.; Asada, K. The Effects of Accretion Flow Dynamics on the Black Hole Shadow of Sagittarius A*. Astrophys. J. 2016, 831, 4. [Google Scholar] [CrossRef]
- Broderick, A.; Blandford, R. Covariant magnetoionic theory—II. Radiative transfer. Mon. Not. R. Astron. Soc. 2004, 349, 994–1008. [Google Scholar] [CrossRef] [Green Version]
- Jones, T.W.; O’Dell, S.L. Transfer of polarized radiation in self-absorbed synchrotron sources. I. Results for a homogeneous source. Astrophys. J. 1977, 214, 522–539. [Google Scholar] [CrossRef]
- Liska, M.T.P.; Chatterjee, K.; Issa, D.; Yoon, D.; Kaaz, N.; Tchekhovskoy, A.; van Eijnatten, D.; Musoke, G.; Hesp, C.; Rohoza, V.; et al. H-AMR: A New GPU-accelerated GRMHD Code for Exascale Computing with 3D Adaptive Mesh Refinement and Local Adaptive Time Stepping. Astrophys. J. Suppl. Ser. 2022, 263, 26. [Google Scholar] [CrossRef]
- Porth, O.; Olivares, H.; Mizuno, Y.; Younsi, Z.; Rezzolla, L.; Moscibrodzka, M.; Falcke, H.; Kramer, M. The black hole accretion code. Comput. Astrophys. Cosmol. 2017, 4, 1. [Google Scholar] [CrossRef]
- Sądowski, A.; Narayan, R.; Tchekhovskoy, A.; Zhu, Y. Semi-implicit scheme for treating radiation under M1 closure in general relativistic conservative fluid dynamics codes. Mon. Not. R. Astron. Soc. 2013, 429, 3533–3550. [Google Scholar] [CrossRef]
- Liska, M.; Hesp, C.; Tchekhovskoy, A.; Ingram, A.; van der Klis, M.; Markoff, S. Formation of precessing jets by tilted black hole discs in 3D general relativistic MHD simulations. Mon. Not. R. Astron. Soc. 2018, 474, L81–L85. [Google Scholar] [CrossRef] [Green Version]
- Younsi, Z.; Porth, O.; Mizuno, Y.; Fromm, C.M.; Olivares, H. Modelling the polarised emission from black holes on event horizon-scales. Proc. IAU 2020, 14, 9–12. [Google Scholar] [CrossRef] [Green Version]
- Mościbrodzka, M.; Gammie, C.F. IPOLE - semi-analytic scheme for relativistic polarized radiative transport. Mon. Not. R. Astron. Soc. 2018, 475, 43–54. [Google Scholar] [CrossRef] [Green Version]
- Gebhardt, K.; Adams, J.; Richstone, D.; Lauer, T.R.; Faber, S.M.; Gültekin, K.; Murphy, J.; Tremaine, S. The Black Hole Mass in M87 from Gemini/NIFS Adaptive Optics Observations. Astrophys. J. 2011, 729, 119. [Google Scholar] [CrossRef]
- Do, T.; Witzel, G.; Gautam, A.K.; Chen, Z.; Ghez, A.M.; Morris, M.R.; Becklin, E.E.; Ciurlo, A.; Hosek, M., Jr.; Martinez, G.D.; et al. Unprecedented Near-infrared Brightness and Variability of Sgr A *. Astrophys. J. Lett. 2019, 882, L27. [Google Scholar] [CrossRef]
- Abuter, R. et al. [GRAVITY Collaboration]. A geometric distance measurement to the Galactic center black hole with 0.3% uncertainty. Astron. Astrophys. 2019, 625, L10. [Google Scholar] [CrossRef] [Green Version]
- Mościbrodzka, M.; Falcke, H.; Shiokawa, H. General relativistic magnetohydrodynamical simulations of the jet in M87. A&A 2016, 586, A38. [Google Scholar] [CrossRef] [Green Version]
- Xiao, F. Modelling energetic particles by a relativistic kappa-loss-cone distribution function in plasmas. Plasma Phys. Control. Fusion 2006, 48, 203–213. [Google Scholar] [CrossRef]
- Davelaar, J.; Mościbrodzka, M.; Bronzwaer, T.; Falcke, H. General relativistic magnetohydrodynamical κ-jet models for Sagittarius A*. Astron. Astrophys. 2018, 612, A34. [Google Scholar] [CrossRef] [Green Version]
- Ressler, S.M.; Tchekhovskoy, A.; Quataert, E.; Chandra, M.; Gammie, C.F. Electron thermodynamics in GRMHD simulations of low-luminosity black hole accretion. Mon. Not. R. Astron. Soc. 2015, 454, 1848–1870. [Google Scholar] [CrossRef]
- Dexter, J.; Jiménez-Rosales, A.; Ressler, S.M.; Tchekhovskoy, A.; Bauböck, M.; de Zeeuw, P.T.; Eisenhauer, F.; von Fellenberg, S.; Gao, F.; Genzel, R.; et al. A parameter survey of Sgr A * radiative models from GRMHD simulations with self-consistent electron heating. Mon. Not. R. Astron. Soc. 2020, 494, 4168–4186. [Google Scholar] [CrossRef] [Green Version]
- Howes, G.G. A prescription for the turbulent heating of astrophysical plasmas. Mon. Not. R. Astron. Soc. 2010, 409, L104–L108. [Google Scholar] [CrossRef] [Green Version]
- Kawazura, Y.; Barnes, M.; Schekochihin, A.A. Thermal disequilibration of ions and electrons by collisionless plasma turbulence. Proc. Natl. Acad. Sci. USA 2019, 116, 771–776. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Werner, G.R.; Uzdensky, D.A.; Begelman, M.C.; Cerutti, B.; Nalewajko, K. Non-thermal particle acceleration in collisionless relativistic electron-proton reconnection. Mon. Not. R. Astron. Soc. 2018, 473, 4840–4861. [Google Scholar] [CrossRef]
- Rowan, M.E.; Sironi, L.; Narayan, R. Electron and Proton Heating in Transrelativistic Magnetic Reconnection. Astrophys. J. 2017, 850, 29. [Google Scholar] [CrossRef] [Green Version]
- Mizuno, Y.; Fromm, C.M.; Younsi, Z.; Porth, O.; Olivares, H.; Rezzolla, L. Comparison of the ion-to-electron temperature ratio prescription: GRMHD simulations with electron thermodynamics. Mon. Not. R. Astron. Soc. 2021, 506, 741–758. [Google Scholar] [CrossRef]
- Sądowski, A.; Narayan, R. Photon-conserving Comptonization in simulations of accretion discs around black holes. Mon. Not. R. Astron. Soc. 2015, 454, 2372–2380. [Google Scholar] [CrossRef] [Green Version]
- Chael, A.; Rowan, M.; Narayan, R.; Johnson, M.; Sironi, L. The role of electron heating physics in images and variability of the Galactic Centre black hole Sagittarius A*. Mon. Not. R. Astron. Soc. 2018, 478, 5209–5229. [Google Scholar] [CrossRef]
- Sądowski, A.; Wielgus, M.; Narayan, R.; Abarca, D.; McKinney, J.C.; Chael, A. Radiative, two-temperature simulations of low-luminosity black hole accretion flows in general relativity. Mon. Not. R. Astron. Soc. 2017, 466, 705–725. [Google Scholar] [CrossRef] [Green Version]
- Doeleman, S.S.; Fish, V.L.; Schenck, D.E.; Beaudoin, C.; Blundell, R.; Bower, G.C.; Broderick, A.E.; Chamberlin, R.; Freund, R.; Friberg, P.; et al. Jet-Launching Structure Resolved Near the Supermassive Black Hole in M87. Science 2012, 338, 355. [Google Scholar] [CrossRef] [Green Version]
- Akiyama, K.; Lu, R.; Fish, V.L.; Doeleman, S.S.; Broderick, A.E.; Dexter, J.; Hada, K.; Kino, M.; Nagai, H.; Honma, M.; et al. 230 GHz VLBI Observations of M87: Event-horizon-scale Structure during an Enhanced Very-high-energy γ-Ray State in 2012. Astrophys. J. 2015, 807, 150. [Google Scholar] [CrossRef] [Green Version]
- Porth, O.; Mizuno, Y.; Younsi, Z.; Fromm, C.M. Flares in the Galactic Centre - I. Orbiting flux tubes in magnetically arrested black hole accretion discs. Mon. Not. R. Astron. Soc. 2021, 502, 2023–2032. [Google Scholar] [CrossRef]
- Begelman, M.C.; Scepi, N.; Dexter, J. What really makes an accretion disc MAD. Mon. Not. R. Astron. Soc. 2022, 511, 2040–2051. [Google Scholar] [CrossRef]
- Ripperda, B.; Liska, M.; Chatterjee, K.; Musoke, G.; Philippov, A.A.; Markoff, S.B.; Tchekhovskoy, A.; Younsi, Z. Black Hole Flares: Ejection of Accreted Magnetic Flux through 3D Plasmoid-mediated Reconnection. Astrophys. J. Lett. 2022, 924, L32. [Google Scholar] [CrossRef]
- Chatterjee, K.; Narayan, R. Flux Eruption Events Drive Angular Momentum Transport in Magnetically Arrested Accretion Flows. Astrophys. J. 2022, 941, 30. [Google Scholar] [CrossRef]
- Chatterjee, K.; Markoff, S.; Neilsen, J.; Younsi, Z.; Witzel, G.; Tchekhovskoy, A.; Yoon, D.; Ingram, A.; van der Klis, M.; Boyce, H.; et al. General relativistic MHD simulations of non-thermal flaring in Sagittarius A*. Mon. Not. R. Astron. Soc. 2021, 507, 5281–5302. [Google Scholar] [CrossRef]
- Wielgus, M.; Marchili, N.; Martí-Vidal, I.; Keating, G.K.; Ramakrishnan, V.; Tiede, P.; Fomalont, E.; Issaoun, S.; Neilsen, J.; Nowak, M.A.; et al. Millimeter Light Curves of Sagittarius A* Observed during the 2017 Event Horizon Telescope Campaign. Astrophys. J. Lett. 2022, 930, L19. [Google Scholar] [CrossRef]
- Narayan, R.; Yi, I. Advection-dominated accretion: A self-similar solution. Astrophys. J. Lett. 1994, 428, L13–L16. [Google Scholar] [CrossRef]
- Narayan, R.; Mahadevan, R.; Quataert, E. Advection-dominated accretion around black holes. In Theory of Black Hole Accretion Disks; Abramowicz, M.A., Bjornsson, G., Pringle, J.E., Eds.; Cambridge University Press: Cambridge, UK, 1998; pp. 138–182. [Google Scholar] [CrossRef]
- Liska, M.; Tchekhovskoy, A.; Quataert, E. Large-scale poloidal magnetic field dynamo leads to powerful jets in GRMHD simulations of black hole accretion with toroidal field. Mon. Not. R. Astron. Soc. 2020, 494, 3656–3662. [Google Scholar] [CrossRef]
- Nakamura, M.; Asada, K.; Hada, K.; Pu, H.Y.; Noble, S.; Tseng, C.; Toma, K.; Kino, M.; Nagai, H.; Takahashi, K.; et al. Parabolic Jets from the Spinning Black Hole in M87. Astrophys. J. 2018, 868, 146. [Google Scholar] [CrossRef] [Green Version]
- Asada, K.; Nakamura, M. The Structure of the M87 Jet: A Transition from Parabolic to Conical Streamlines. ApJL 2012, 745, 5. [Google Scholar] [CrossRef] [Green Version]
- Nokhrina, E.E.; Gurvits, L.I.; Beskin, V.S.; Nakamura, M.; Asada, K.; Hada, K. M87 black hole mass and spin estimate through the position of the jet boundary shape break. Mon. Not. R. Astron. Soc. 2019, 489, 1197–1205. [Google Scholar] [CrossRef] [Green Version]
- Chatterjee, K.; Liska, M.; Tchekhovskoy, A.; Markoff, S.B. Accelerating AGN jets to parsec scales using general relativistic MHD simulations. Mon. Not. R. Astron. Soc. 2019, 490, 2200–2218. [Google Scholar] [CrossRef] [Green Version]
- Issaoun, S.; Johnson, M.D.; Blackburn, L.; Brinkerink, C.D.; Mościbrodzka, M.; Chael, A.; Goddi1, C.; Martí-Vidal, I.; Wagner, J.; Doeleman, S.S. The Size, Shape, and Scattering of Sagittarius A* at 86 GHz: First VLBI with ALMA. Astrophys. J. 2019, 871, 30. [Google Scholar] [CrossRef] [Green Version]
- Markoff, S.; Bower, G.C.; Falcke, H. How to hide large-scale outflows: Size constraints on the jets of Sgr A *. Mon. Not. R. Astron. Soc. 2007, 379, 1519–1532. [Google Scholar] [CrossRef]
- Mertens, F.; Lobanov, A.P.; Walker, R.C.; Hardee, P.E. Kinematics of the jet in M87 on scales of 100–1000 Schwarzschild radii. Astron. Astrophys. 2016, 595, A54. [Google Scholar] [CrossRef] [Green Version]
- Hada, K.; Kino, M.; Doi, A.; Nagai, H.; Honma, M.; Hagiwara, Y.; Giroletti, M.; Giovannini, G.; Kawaguchi, N. The Innermost Collimation Structure of the M87 Jet Down to 10 Schwarzschild Radii. Astrophys. J. 2013, 775, 70. [Google Scholar] [CrossRef] [Green Version]
- Nakamura, M.; Asada, K. The Parabolic Jet Structure in M87 as a Magnetohydrodynamic Nozzle. Astrophys. J. 2013, 775, 118. [Google Scholar] [CrossRef]
- Hada, K.; Kino, M.; Doi, A.; Nagai, H.; Honma, M.; Akiyama, K.; Tazaki, F.; Lico, R.; Giroletti, M.; Giovannini, G.; et al. High-sensitivity 86 GHz (3.5 mm) VLBI Observations of M87: Deep Imaging of the Jet Base at a Resolution of 10 Schwarzschild Radii. Astrophys. J. 2016, 817, 131. [Google Scholar] [CrossRef]
- Fragile, P.C.; Meier, D.L. General Relativistic Magnetohydrodynamic Simulations of the Hard State as a Magnetically Dominated Accretion Flow. Astrophys. J. 2009, 693, 771–783. [Google Scholar] [CrossRef]
- Yoon, D.; Chatterjee, K.; Markoff, S.B.; van Eijnatten, D.; Younsi, Z.; Liska, M.; Tchekhovskoy, A. Spectral and imaging properties of Sgr A * from high-resolution 3D GRMHD simulations with radiative cooling. Mon. Not. R. Astron. Soc. 2020, 499, 3178–3192. [Google Scholar] [CrossRef]
- Sadowski, A. Magnetic flux stabilizing thin accretion discs. Mon. Not. R. Astron. Soc. 2016, 462, 960–965. [Google Scholar] [CrossRef] [Green Version]
- Liska, M.T.P.; Musoke, G.; Tchekhovskoy, A.; Porth, O.; Beloborodov, A.M. Formation of Magnetically Truncated Accretion Disks in 3D Radiation-Transport Two-Temperature GRMHD Simulations. arXiv 2022, arXiv:2201.03526. [Google Scholar] [CrossRef]
- Mishra, B.; Fragile, P.C.; Anderson, J.; Blankenship, A.; Li, H.; Nalewajko, K. The Role of Strong Magnetic Fields in Stabilizing Highly Luminous Thin Disks. Astrophys. J. 2022, 939, 31. [Google Scholar] [CrossRef]
- Wong, G.N.; Du, Y.; Prather, B.S.; Gammie, C.F. The Jet-disk Boundary Layer in Black Hole Accretion. Astrophys. J. 2021, 914, 55. [Google Scholar] [CrossRef]
- Aharonian, F.; Akhperjanian, A.G.; Bazer-Bachi, A.R.; Beilicke, M.; Benbow, W.; Berge, D.; Bernlöhr, K.; Boisson, C.; Bolz, O.; Borrel, V.; et al. Fast Variability of Tera-Electron Volt γ Rays from the Radio Galaxy M87. Science 2006, 314, 1424–1427. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Acciari, V.A.; Aliu, E.; Arlen, T.; Aune, T.; Beilicke, M.; Benbow, W.; Boltuch, D.; Bradbury, S.M.; Buckley, J.H.; Bugaev, V.; et al. Veritas 2008-2009 Monitoring of the Variable Gamma-ray Source M 87. Astrophys. J. 2010, 716, 819–824. [Google Scholar] [CrossRef] [Green Version]
- Baganoff, F.K.; Bautz, M.W.; Brandt, W.N.; Chartas, G.; Feigelson, E.D.; Garmire, G.P.; Maeda, Y.; Morris, M.; Ricker, G.R.; Townsley, L.K.; et al. Rapid X-ray flaring from the direction of the supermassive black hole at the Galactic Centre. Nature 2001, 413, 45–48. [Google Scholar] [CrossRef] [Green Version]
- Eckart, A.; Baganoff, F.K.; Schödel, R.; Morris, M.; Genzel, R.; Bower, G.C.; Marrone, D.; Moran, J.M.; Viehmann, T.; Bautz, M.W.; et al. The flare activity of Sagittarius A*. New coordinated mm to X-ray observations. Astron. Astrophys. 2006, 450, 535–555. [Google Scholar] [CrossRef] [Green Version]
- Hornstein, S.D.; Matthews, K.; Ghez, A.M.; Lu, J.R.; Morris, M.; Becklin, E.E.; Rafelski, M.; Baganoff, F.K. A Constant Spectral Index for Sagittarius A* during Infrared/X-ray Intensity Variations. Astrophys. J. 2007, 667, 900–910. [Google Scholar] [CrossRef] [Green Version]
- Nowak, M.A.; Neilsen, J.; Markoff, S.B.; Baganoff, F.K.; Porquet, D.; Grosso, N.; Levin, Y.; Houck, J.; Eckart, A.; Falcke, H.; et al. Chandra/HETGS Observations of the Brightest Flare Seen from Sgr A *. Astrophys. J. 2012, 759, 95. [Google Scholar] [CrossRef]
- Neilsen, J.; Nowak, M.A.; Gammie, C.; Dexter, J.; Markoff, S.; Haggard, D.; Nayakshin, S.; Wang, Q.D.; Grosso, N.; Porquet, D.; et al. A Chandra/HETGS Census of X-ray Variability from Sgr A * during 2012. Astrophys. J. 2013, 774, 42. [Google Scholar] [CrossRef] [Green Version]
- Witzel, G.; Martinez, G.; Hora, J.; Willner, S.P.; Morris, M.R.; Gammie, C.; Becklin, E.E.; Ashby, M.L.N.; Baganoff, F.; Carey, S.; et al. Variability Timescale and Spectral Index of Sgr A * in the Near Infrared: Approximate Bayesian Computation Analysis of the Variability of the Closest Supermassive Black Hole. Astrophys. J. 2018, 863, 15. [Google Scholar] [CrossRef]
- Haggard, D.; Nynka, M.; Mon, B.; de la Cruz Hernandez, N.; Nowak, M.; Heinke, C.; Neilsen, J.; Dexter, J.; Fragile, P.C.; Baganoff, F.; et al. Chandra Spectral and Timing Analysis of Sgr A *’s Brightest X-ray Flares. Astrophys. J. 2019, 886, 96. [Google Scholar] [CrossRef] [Green Version]
- Dodds-Eden, K.; Sharma, P.; Quataert, E.; Genzel, R.; Gillessen, S.; Eisenhauer, F.; Porquet, D. Time-Dependent Models of Flares from Sagittarius A*. Astrophys. J. 2010, 725, 450–465. [Google Scholar] [CrossRef]
- Dibi, S.; Markoff, S.; Belmont, R.; Malzac, J.; Barrière, N.M.; Tomsick, J.A. Exploring plasma evolution during Sagittarius A* flares. Mon. Not. R. Astron. Soc. 2014, 441, 1005–1016. [Google Scholar] [CrossRef] [Green Version]
- Nathanail, A.; Fromm, C.M.; Porth, O.; Olivares, H.; Younsi, Z.; Mizuno, Y.; Rezzolla, L. Plasmoid formation in global GRMHD simulations and AGN flares. Mon. Not. R. Astron. Soc. 2020, 495, 1549–1565. [Google Scholar] [CrossRef]
- Dexter, J.; Tchekhovskoy, A.; Jiménez-Rosales, A.; Ressler, S.M.; Bauböck, M.; Dallilar, Y.; de Zeeuw, P.T.; Eisenhauer, F.; von Fellenberg, S.; Gao, F.; et al. Sgr A * near-infrared flares from reconnection events in a magnetically arrested disc. Mon. Not. R. Astron. Soc. 2020, 497, 4999–5007. [Google Scholar] [CrossRef]
- Scepi, N.; Dexter, J.; Begelman, M.C. Sgr A * X-ray flares from non-thermal particle acceleration in a magnetically arrested disc. Mon. Not. R. Astron. Soc. 2022, 511, 3536–3547. [Google Scholar] [CrossRef]
- Markoff, S.; Falcke, H.; Yuan, F.; Biermann, P.L. The Nature of the 10 kilosecond X-ray flare in Sgr A *. Astron. Astrophys. 2001, 379, L13–L16. [Google Scholar] [CrossRef] [Green Version]
- Dibi, S.; Markoff, S.; Belmont, R.; Malzac, J.; Neilsen, J.; Witzel, G. Using infrared/X-ray flare statistics to probe the emission regions near the event horizon of Sgr A *. Mon. Not. R. Astron. Soc. 2016, 461, 552–559. [Google Scholar] [CrossRef] [Green Version]
- Gutiérrez, E.M.; Nemmen, R.; Cafardo, F. A Nonthermal Bomb Explains the Near-infrared Superflare of Sgr A *. Astrophys. J. Lett. 2020, 891, L36. [Google Scholar] [CrossRef]
- Bauböck, M. et al. [Gravity Collaboration]. Modeling the orbital motion of Sgr A *’s near-infrared flares. Astron. Astrophys. 2020, 635, A143. [Google Scholar] [CrossRef]
- Ball, D.; Özel, F.; Christian, P.; Chan, C.K.; Psaltis, D. A Plasmoid model for the Sgr A * Flares Observed With Gravity and CHANDRA. Astrophys. J. 2021, 917, 8. [Google Scholar] [CrossRef]
- Wielgus, M.; Moscibrodzka, M.; Vos, J.; Gelles, Z.; Martí-Vidal, I.; Farah, J.; Marchili, N.; Goddi, C.; Messias, H. Orbital motion near Sagittarius A*. Constraints from polarimetric ALMA observations. Astron. Astrophys. 2022, 665, L6. [Google Scholar] [CrossRef]
- Emami, R.; Tiede, P.; Doeleman, S.S.; Roelofs, F.; Wielgus, M.; Blackburn, L.; Liska, M.; Chatterjee, K.; Ripperda, B.; Fuentes, A.; et al. Tracing Hot Spot Motion in Sagittarius A* Using the Next-Generation Event Horizon Telescope (ngEHT). Galaxies 2023, 11, 23. [Google Scholar] [CrossRef]
- Gelles, Z.; Chatterjee, K.; Johnson, M.; Ripperda, B.; Liska, M. Relativistic Signatures of Flux Eruption Events near Black Holes. Galaxies 2022, 10, 107. [Google Scholar] [CrossRef]
- Ressler, S.M.; White, C.J.; Quataert, E.; Stone, J.M. Ab Initio Horizon-scale Simulations of Magnetically Arrested Accretion in Sagittarius A* Fed by Stellar Winds. Astrophys. J. Lett. 2020, 896, L6. [Google Scholar] [CrossRef]
- Lalakos, A.; Gottlieb, O.; Kaaz, N.; Chatterjee, K.; Liska, M.; Christie, I.M.; Tchekhovskoy, A.; Zhuravleva, I.; Nokhrina, E. Bridging the Bondi and Event Horizon Scales: 3D GRMHD Simulations Reveal X-shaped Radio Galaxy Morphology. Astrophys. J. Lett. 2022, 936, L5. [Google Scholar] [CrossRef]
- Cruz-Osorio, A.; Sánchez-Salcedo, F.J.; Lora-Clavijo, F.D. Relativistic Bondi-Hoyle-Lyttleton accretion in the presence of small rigid bodies around a black hole. Mon. Not. R. Astron. Soc. 2017, 471, 3127–3134. [Google Scholar] [CrossRef] [Green Version]
- Kaaz, N.; Murguia-Berthier, A.; Chatterjee, K.; Liska, M.; Tchekhovskoy, A. Jet Formation in 3D GRMHD Simulations of Bondi-Hoyle-Lyttleton Accretion. arXiv 2022, arXiv:2201.11753. [Google Scholar]
- Avara, M.J.; McKinney, J.C.; Reynolds, C.S. Efficiency of thin magnetically arrested discs around black holes. Mon. Not. R. Astron. Soc. 2016, 462, 636–648. [Google Scholar] [CrossRef] [Green Version]
- Sadowski, A.; Narayan, R. Three-dimensional simulations of supercritical black hole accretion discs - luminosities, photon trapping and variability. Mon. Not. R. Astron. Soc. 2016, 456, 3929–3947. [Google Scholar] [CrossRef] [Green Version]
- Curd, B.; Narayan, R. GRRMHD simulations of tidal disruption event accretion discs around supermassive black holes: Jet formation, spectra, and detectability. Mon. Not. R. Astron. Soc. 2019, 483, 565–592. [Google Scholar] [CrossRef] [Green Version]
- White, C.J.; Quataert, E.; Blaes, O. Tilted Disks around Black Holes: A Numerical Parameter Survey for Spin and Inclination Angle. Astrophys. J. 2019, 878, 51. [Google Scholar] [CrossRef] [Green Version]
- Chatterjee, K.; Younsi, Z.; Liska, M.; Tchekhovskoy, A.; Markoff, S.B.; Yoon, D.; van Eijnatten, D.; Hesp, C.; Ingram, A.; van der Klis, M.B.M. Observational signatures of disc and jet misalignment in images of accreting black holes. Mon. Not. R. Astron. Soc. 2020, 499, 362–378. [Google Scholar] [CrossRef]
- Akiyama, K. et al. [Event Horizon Telescope Collaboration]. First Sagittarius A* Event Horizon Telescope Results. VI. Testing the Black Hole Metric. Astrophys. J. Lett. 2022, 930, L17. [Google Scholar] [CrossRef]
- Mizuno, Y.; Younsi, Z.; Fromm, C.M.; Porth, O.; De Laurentis, M.; Olivares, H.; Falcke, H.; Kramer, M.; Rezzolla, L. The current ability to test theories of gravity with black hole shadows. Nat. Astron. 2018, 2, 585–590. [Google Scholar] [CrossRef] [Green Version]
- Olivares, H.; Younsi, Z.; Fromm, C.M.; De Laurentis, M.; Porth, O.; Mizuno, Y.; Falcke, H.; Kramer, M.; Rezzolla, L. How to tell an accreting boson star from a black hole. Mon. Not. R. Astron. Soc. 2020, 497, 521–535. [Google Scholar] [CrossRef]
- Nampalliwar, S.; Yfantis, A.I.; Kokkotas, K.D. Extending GRMHD for thin disks to non-Kerr spacetimes. Phys. Rev. D 2022, 106, 063009. [Google Scholar] [CrossRef]



| Model | Grid Resolution | Sim. Time | MI() | MI() | MI | MI |
|---|---|---|---|---|---|---|
| Name | () | () | () | () | ||
| M87 GRMHD | 5.6–10.6 | 0.27 | 0.15 | 0.26 | 0.33 | |
| M87 2t-GRMHD | 10–15 | 0.29 | 0.14 | 0.25 | 0.31 | |
| M87 2t-GRRMHD | 11–16 | 0.28 | 0.14 | 0.14 | 0.31 | |
| Sgr A GRMHD | 30–35 | 0.23 | 0.21 | 0.39 | 0.57 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chatterjee, K.; Chael, A.; Tiede, P.; Mizuno, Y.; Emami, R.; Fromm, C.; Ricarte, A.; Blackburn, L.; Roelofs, F.; Johnson, M.D.; et al. Accretion Flow Morphology in Numerical Simulations of Black Holes from the ngEHT Model Library: The Impact of Radiation Physics. Galaxies 2023, 11, 38. https://doi.org/10.3390/galaxies11020038
Chatterjee K, Chael A, Tiede P, Mizuno Y, Emami R, Fromm C, Ricarte A, Blackburn L, Roelofs F, Johnson MD, et al. Accretion Flow Morphology in Numerical Simulations of Black Holes from the ngEHT Model Library: The Impact of Radiation Physics. Galaxies. 2023; 11(2):38. https://doi.org/10.3390/galaxies11020038
Chicago/Turabian StyleChatterjee, Koushik, Andrew Chael, Paul Tiede, Yosuke Mizuno, Razieh Emami, Christian Fromm, Angelo Ricarte, Lindy Blackburn, Freek Roelofs, Michael D. Johnson, and et al. 2023. "Accretion Flow Morphology in Numerical Simulations of Black Holes from the ngEHT Model Library: The Impact of Radiation Physics" Galaxies 11, no. 2: 38. https://doi.org/10.3390/galaxies11020038
APA StyleChatterjee, K., Chael, A., Tiede, P., Mizuno, Y., Emami, R., Fromm, C., Ricarte, A., Blackburn, L., Roelofs, F., Johnson, M. D., Doeleman, S. S., Arras, P., Fuentes, A., Knollmüller, J., Kosogorov, N., Lindahl, G., Müller, H., Patel, N., Raymond, A., ... Vega, J. (2023). Accretion Flow Morphology in Numerical Simulations of Black Holes from the ngEHT Model Library: The Impact of Radiation Physics. Galaxies, 11(2), 38. https://doi.org/10.3390/galaxies11020038







