Study of the Influence of Temperature on Contact Pressures and Resource of Metal-Polymer Plain Bearings with Filled Polyamide PA6 Bushing
Abstract
1. Introduction
2. Materials and Methods
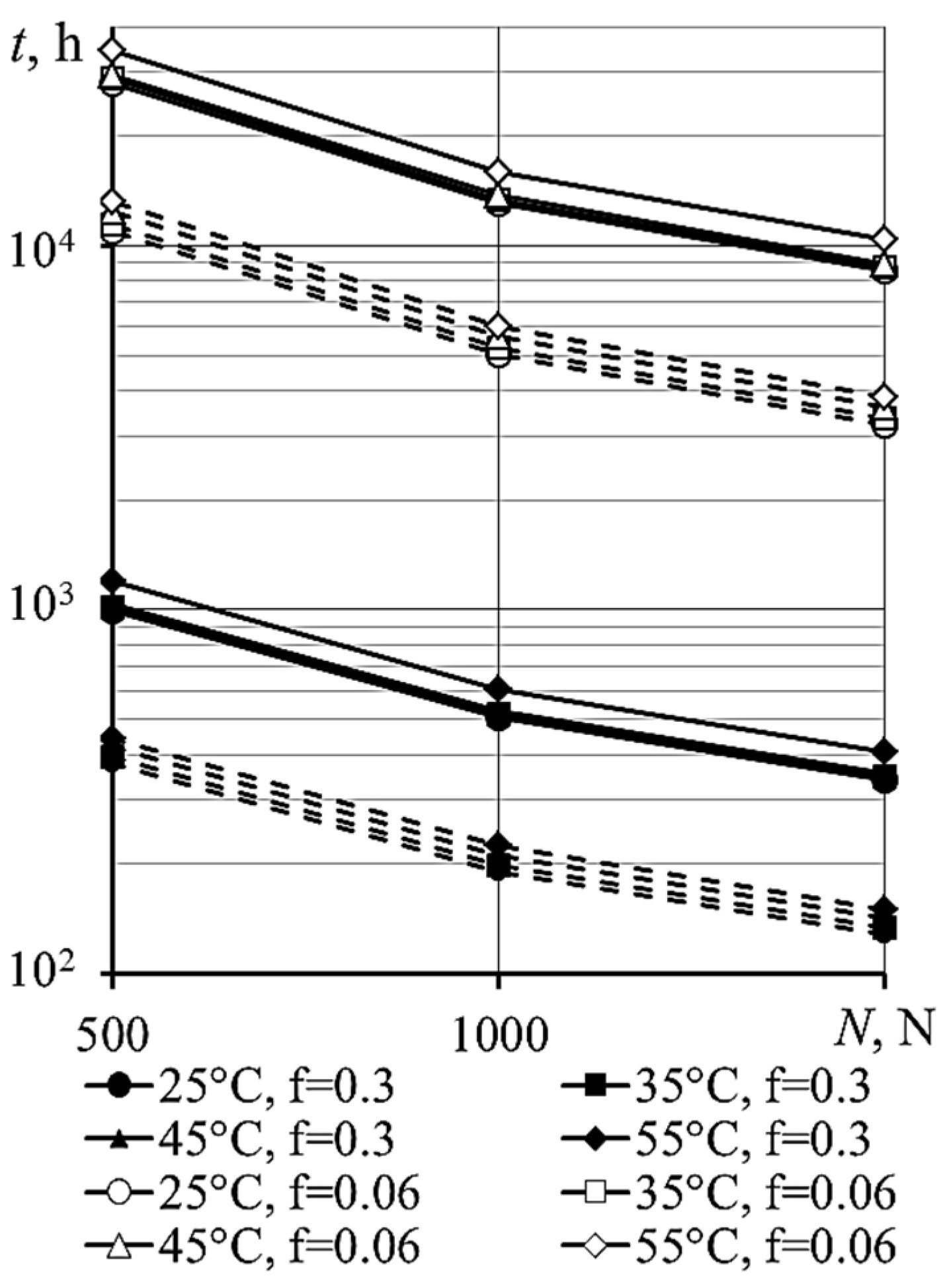
2.1. Influence of Temperature
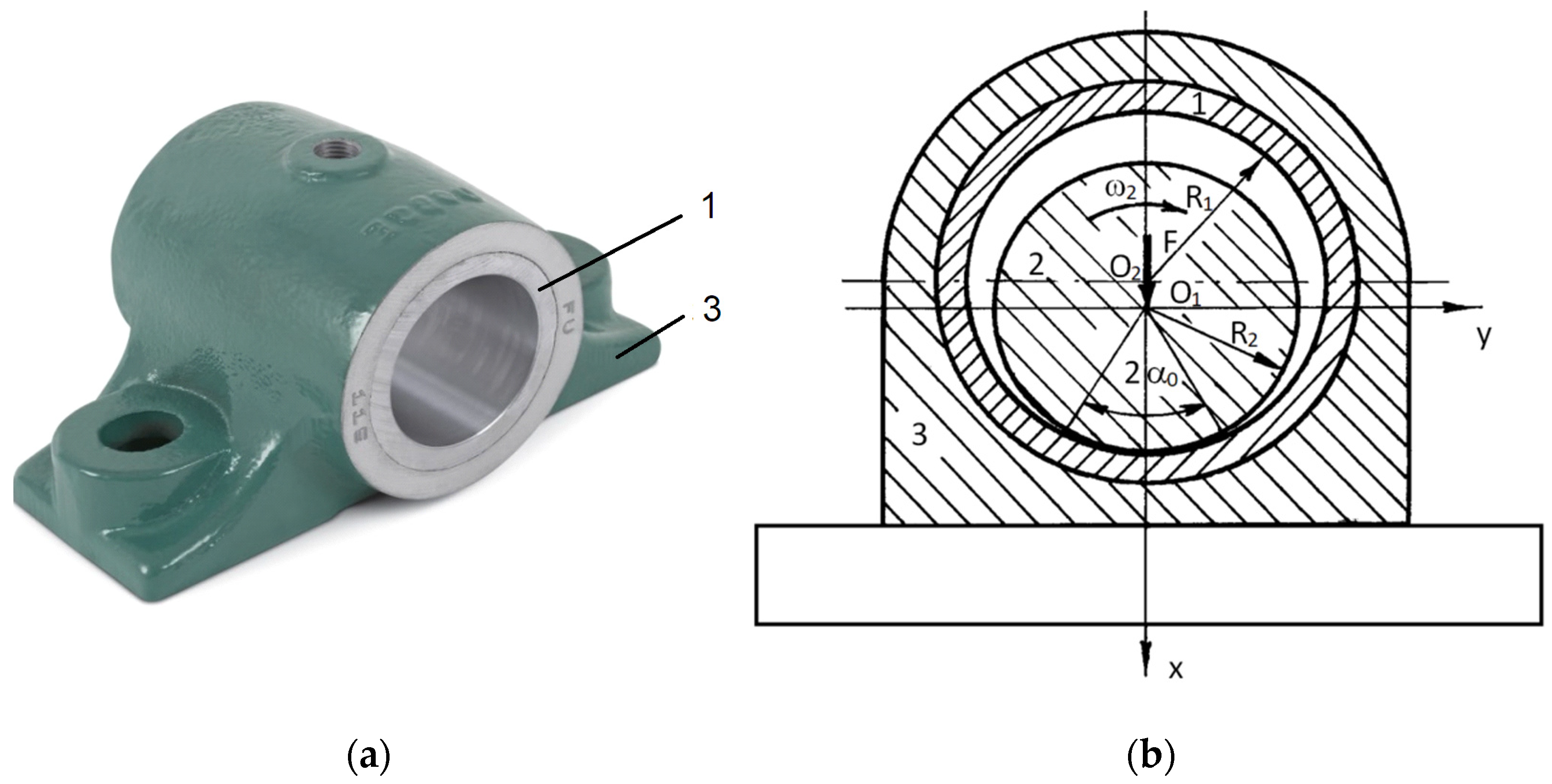
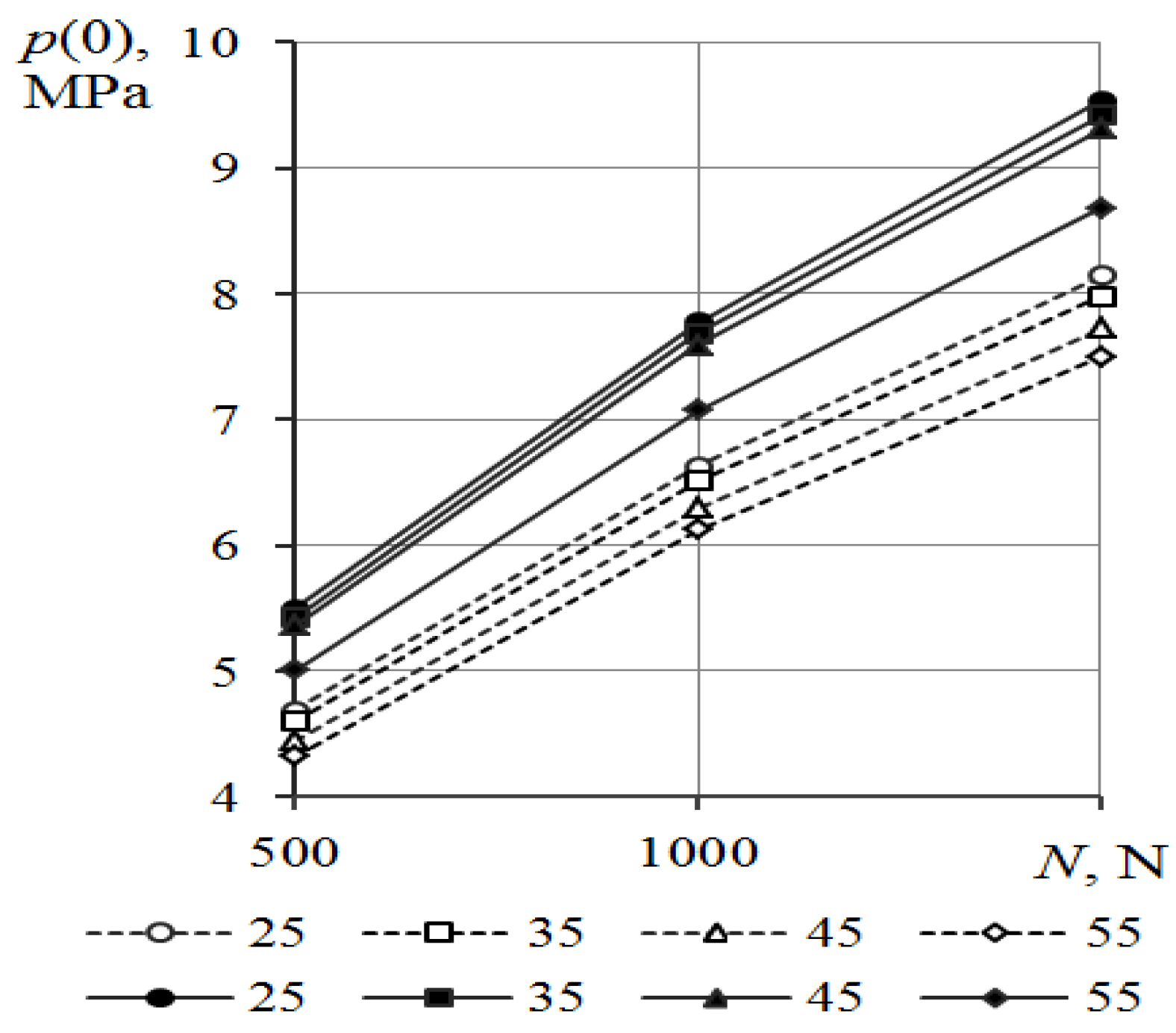
2.2. Calculation of Contact Pressures
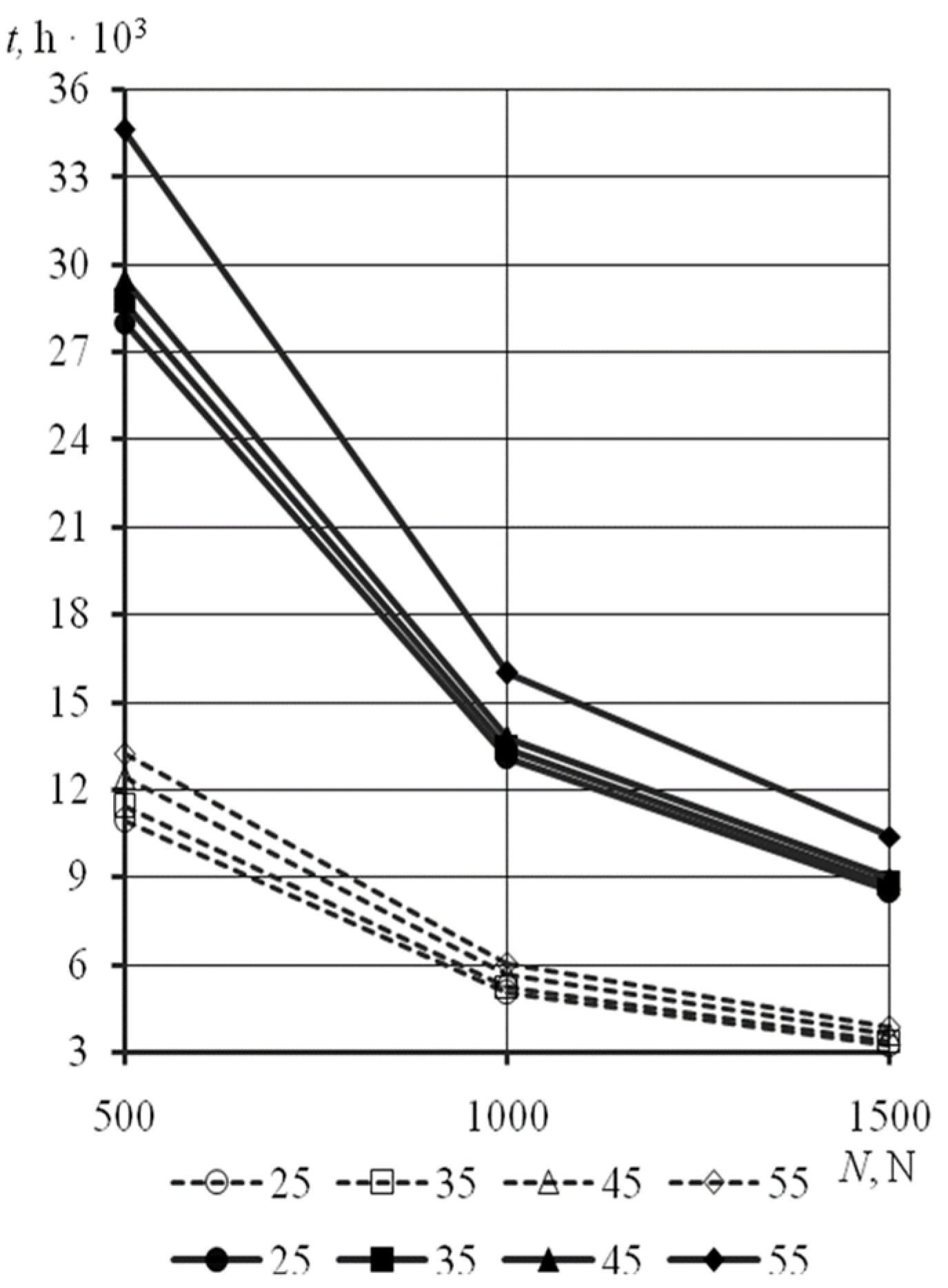
2.3. Calculation of Resource
2.4. Conditional Calculation of Bearing Capacity
3. Solution, Results, and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Wielieba, W. Maintenance-Free Plain Bearings Made of Thermoplastic Polymers; Wyd. Wrocław University of Science and Technology: Wrocław, Poland, 2013. [Google Scholar]
- Feyzullahoglu, E.; Saffak, Z. The tribological behavior of different engineering plastics under dry friction conditions. Mater. Des. 2008, 29, 205–211. [Google Scholar] [CrossRef]
- Demirci, M.T.; Düzcükoğlu, H. Wear behaviors of Polytetrafluoroethylene and glass fiber reinforced Polyamide 66 journal bearings. Mater. Des. 2014, 57, 560–567. [Google Scholar] [CrossRef]
- Miler, D.; Škec, S.; Katana, B.; Žeželj, D. An Experimental Study of Composite Plain Bearings: The Influence of Clearance on Friction Coefficient and Temperature. Stroj. Vestn. J. Mech. Eng. 2019, 65, 547–556. [Google Scholar] [CrossRef]
- Zhu, J.; Xie, F.; Dwyer-Joyce, R.S. PEEK Composites as Self-Lubricating Bush Materials for Articulating Revolute Pin Joints. Polymers 2020, 12, 665. [Google Scholar] [CrossRef]
- Kurdi, A.; Kan, W.H.; Chang, L. Tribological behaviour of high performance polymers and polymer composites at elevated temperature. Tribol. Ind. 2019, 130, 94–105. [Google Scholar] [CrossRef]
- Kindrachuk, M.; Volchenko, A.; Volchenko, D.; Volchenko, N.; Poliakov, P.; Tisov, O.; Kornienko, A. Polymers with enhanced energy capacity modified by semiconductor materials. Funct. Mater. 2019, 26, 629–634. [Google Scholar] [CrossRef]
- Goryacheva, I.G. Mechanics of Frictional Interaction; Nauka: Moscow, Russia, 2001. [Google Scholar]
- Kragelsky, I.V.; Dobychin, N.M.; Kombalov, V.S. Fundamentals of Friction and Wear Calculations; Mashinostroenie: Moscow, Russia, 1977. [Google Scholar]
- Kuzmenko, A.G. Development of Methods of Contact Tribomechanics; KhNU: Khmelnytsky, Ukraine, 2010. [Google Scholar]
- Teplyy, M.I. Determination of contact parameters and wear in cylindrical sliding bearings. Frict. Wear 1987, 6, 895–902. [Google Scholar]
- Usov, P.P. Internal contact of cylindrical bodies of close radii during wear of their surfaces. Frict. Wear 1985, 3, 404–414. [Google Scholar]
- Dykha, A.; Marchenko, D. Prediction the wear of sliding bearings. Int. J. Eng. Technol. 2018, 7, 4–8. [Google Scholar] [CrossRef][Green Version]
- Sorokatyi, R.; Chernets, M.; Dykha, A.; Mikosyanchyk, O. Phenomenological model of accumulation of fatigue tribological damage in the surface layer of materials. Mech. Mach. Sci. 2019, 73, 3761–3769. [Google Scholar] [CrossRef]
- Zwieżycki, W. Forecasting the Reliability of the Wearing Parts of Machines; ITE: Radom, Poland, 1999. [Google Scholar]
- Sorokatyi, R.V. Modeling the behavior of tribosystems using the method of triboelements. J. Frict. Wear 2002, 23, 16–22. [Google Scholar]
- Sorokatyi, R.V. Solution of the problem of wear of a fine elastic layer with a rigid bearing mounted on a rigid shaft using the method of triboelements. J. Frict. Wear 2003, 24, 35–41. [Google Scholar]
- Rezaei, A.; Ost, W.; Van Paepegem, W.; De Baets, P.; Degrieck, J. Experimental study and numerical simulation of the large-scale testing of polymeric composite journal bearings: Three-dimensional and dynamic modeling. Wear 2011, 270, 431–438. [Google Scholar] [CrossRef]
- Rezaei, A.; Ost, W.; Van Paepegem, W.; De Baets, P.; Degrieck, J. A study on the effect of the clearance on the contact stresses and kinematics of polymeric composite journal bearings under reciprocating sliding conditions. Tribol. Int. 2012, 48, 8–14. [Google Scholar] [CrossRef][Green Version]
- Rezaei, A.; Van Paepegem, W.; De Baets, P.; Ost, W.; Degrieck, J. Adaptive finite element simulation of wear evolution in radial sliding bearing. Wear 2012, 296, 660–671. [Google Scholar] [CrossRef]
- Andreikiv, M.V.; Chernets, M.V. Evaluation of the Contact Interaction of Rubbing Machine Elements; Naukova Dumka: Kiev, Ukraine, 1991. [Google Scholar]
- Chernets, M.; Pashechko, M.; Nevchas, A. Methods of Forecasting and Increasing the Wear Resistance of Tribotechnical Sliding Systems. In Vol.1. Research and Calculation of Sliding Tribosystems, Methods to Increase Durability and Wear Resistance; KOLO: Drogobich, Ukraine, 2001. [Google Scholar]
- Chernets, M.; Pashechko, M.; Nevchas, A. Methods of forecasting and increasing the wear resistance of tribotechnical sliding systems. In Vol. 2. Surface Reinforcement of Structural Materials of Sliding Tribosystems; KOLO: Drogobich, Ukraine, 2001. [Google Scholar]
- Chernets, M.V.; Andreikiv, O.E.; Liebiedieva, N.M.; Zhydyk, V.B. A model for evaluation of wear and durability of plain bearing with small out-of-roundness. Mater. Sci. 2009, 2, 279–290. [Google Scholar] [CrossRef]
- Chernets, M.V. Prediction of the life of a sliding bearing based on a cumulative wear model taking into account the lobing of shaft contour. J. Frict. Wear 2015, 36, 163–169. [Google Scholar] [CrossRef]
- Chernets, M.V. Contact problem for a cylindrical joint with technological faceting of the contours of its parts. Mater. Sci. 2009, 6, 859–868. [Google Scholar] [CrossRef]
- Chernets, M.; Chernets, J. Generalized method for calculating the durability of sliding bearings with technological out-of-roundness of details. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2015, 229, 216–226. [Google Scholar] [CrossRef]
- Chernets, M.V.; Shil’ko, S.V.; Pashechko, M.I.; Barshch, M. Wear resistance of glass- and carbon-filled polyamide composites for metal-polymer gears. J. Frict. Wear 2018, 39, 361–364. [Google Scholar] [CrossRef]
- Chernets, M.; Chernets, J.; Kindrachuk, M.; Kornienko, A. Methodology of calculation of metal-polymer sliding bearings for contact strength, durability and wear. Tribol. Ind. 2020, 42, 572–584. [Google Scholar] [CrossRef]
- Budynas, R.G.; Nisbett, J.K. Shigley’s Mechanical Engineering Design, 11th ed.; McGraw-Hill: New York, NY, USA, 2019. [Google Scholar]







| Materials/Temperature, T °C | 25 | 35 | 45 | 55 | Decrease E, Times | Poisson’s Ratio 1, μ | Increase μ, Times |
|---|---|---|---|---|---|---|---|
| PA6 | 2700 | 2550 | 2400 | 2050 | 1.32 | 0.39/0.416 | 1.067 |
| PA6 + 30%GF | 3900 | 3750 | 3500 | 3000 | 1.3 | 0.42/0.438 | 1.043 |
| PA6 + 30%CF | 5400 | 5270 | 5150 | 4450 | 1.21 | 0.4/0.418 | 1.045 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chernets, M.; Pashechko, M.; Kornienko, A.; Buketov, A. Study of the Influence of Temperature on Contact Pressures and Resource of Metal-Polymer Plain Bearings with Filled Polyamide PA6 Bushing. Lubricants 2022, 10, 13. https://doi.org/10.3390/lubricants10010013
Chernets M, Pashechko M, Kornienko A, Buketov A. Study of the Influence of Temperature on Contact Pressures and Resource of Metal-Polymer Plain Bearings with Filled Polyamide PA6 Bushing. Lubricants. 2022; 10(1):13. https://doi.org/10.3390/lubricants10010013
Chicago/Turabian StyleChernets, Myron, Mykhaylo Pashechko, Anatolii Kornienko, and Andriy Buketov. 2022. "Study of the Influence of Temperature on Contact Pressures and Resource of Metal-Polymer Plain Bearings with Filled Polyamide PA6 Bushing" Lubricants 10, no. 1: 13. https://doi.org/10.3390/lubricants10010013
APA StyleChernets, M., Pashechko, M., Kornienko, A., & Buketov, A. (2022). Study of the Influence of Temperature on Contact Pressures and Resource of Metal-Polymer Plain Bearings with Filled Polyamide PA6 Bushing. Lubricants, 10(1), 13. https://doi.org/10.3390/lubricants10010013





