Optimal Design of Boundary Angle for Gas Foil Thrust Bearing Thermal Performance
Abstract
:1. Introduction
2. Numerical Method
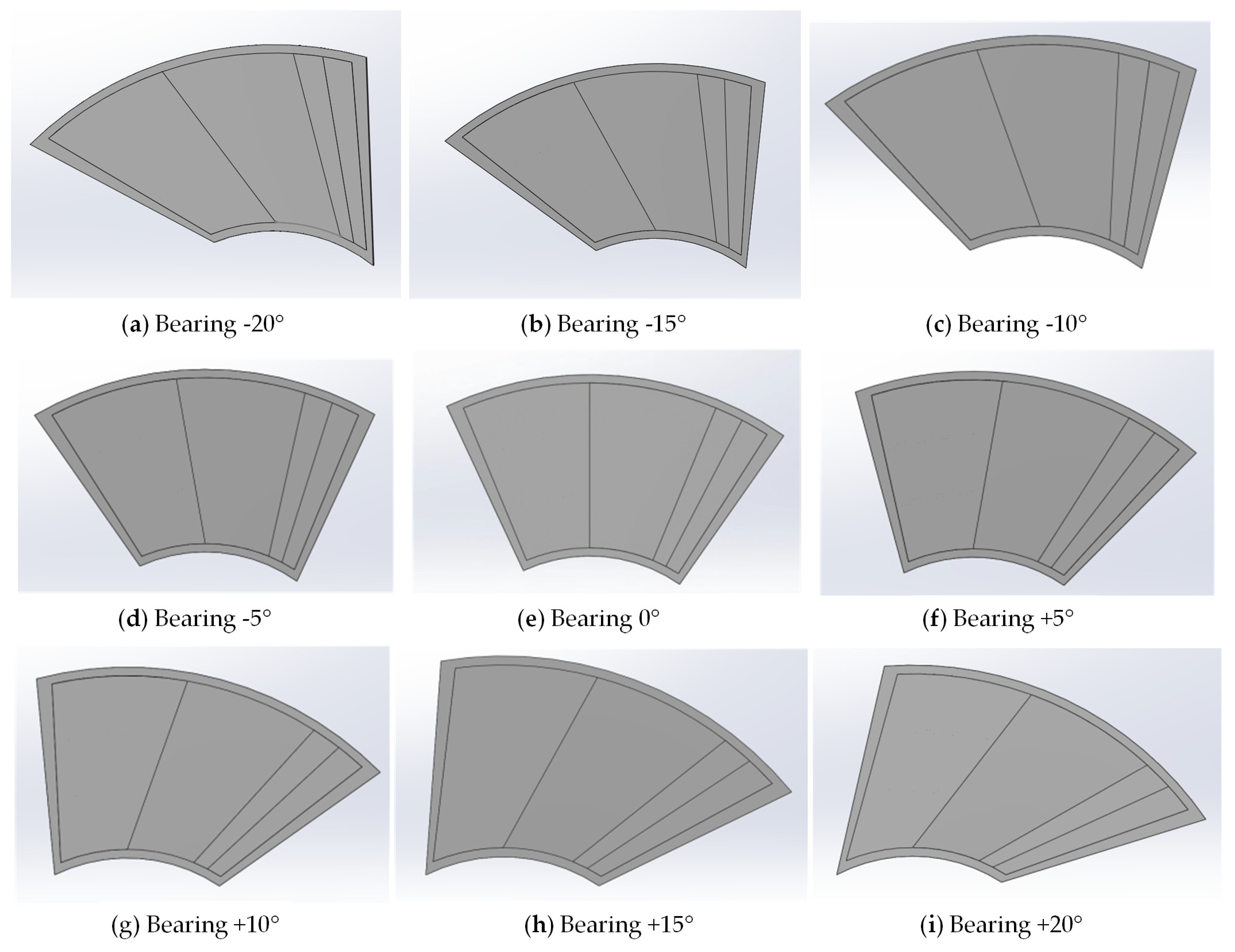
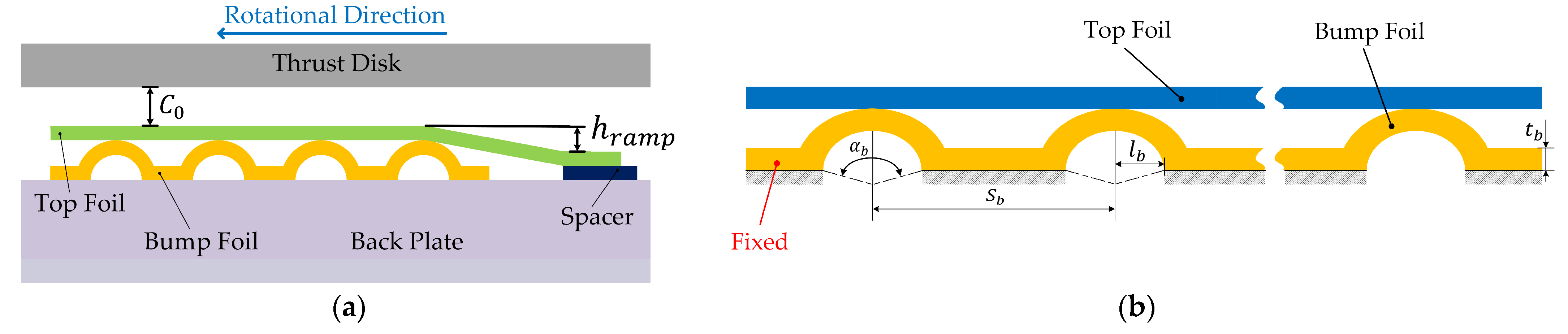
2.1. Description of the Novel GFTB
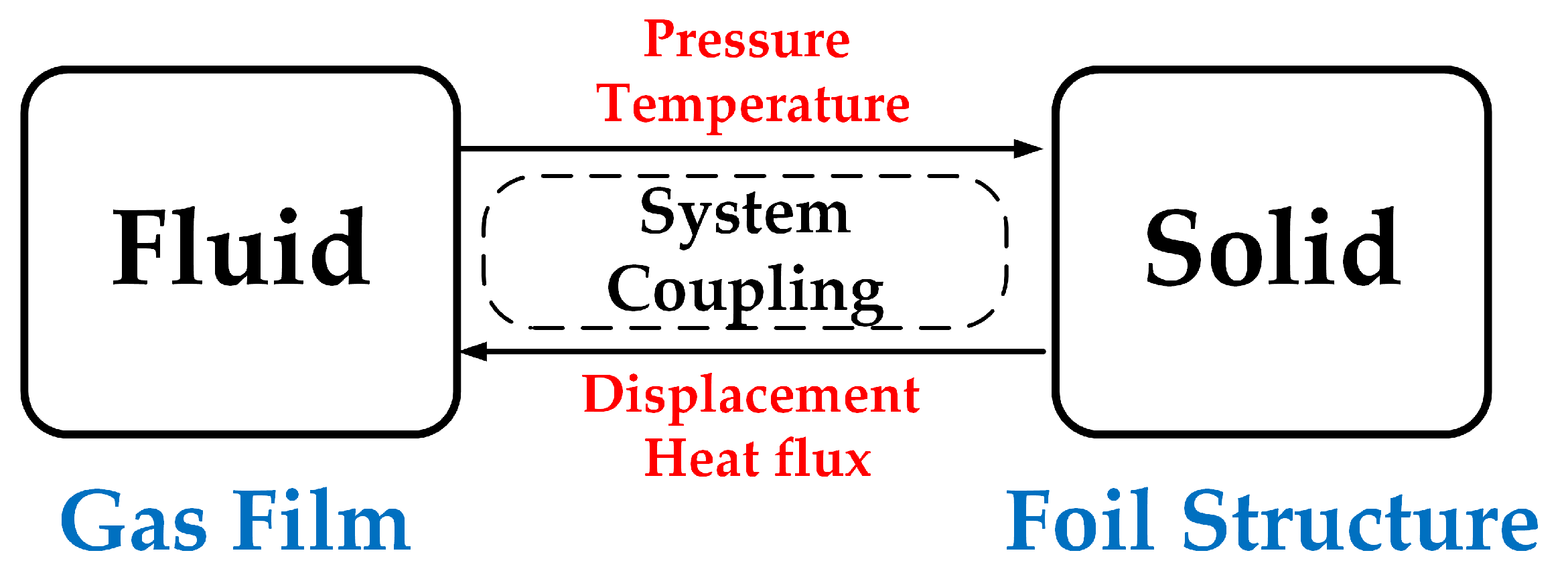
2.2. Computational Domains and Governing Equations
2.3. Boundary Condition
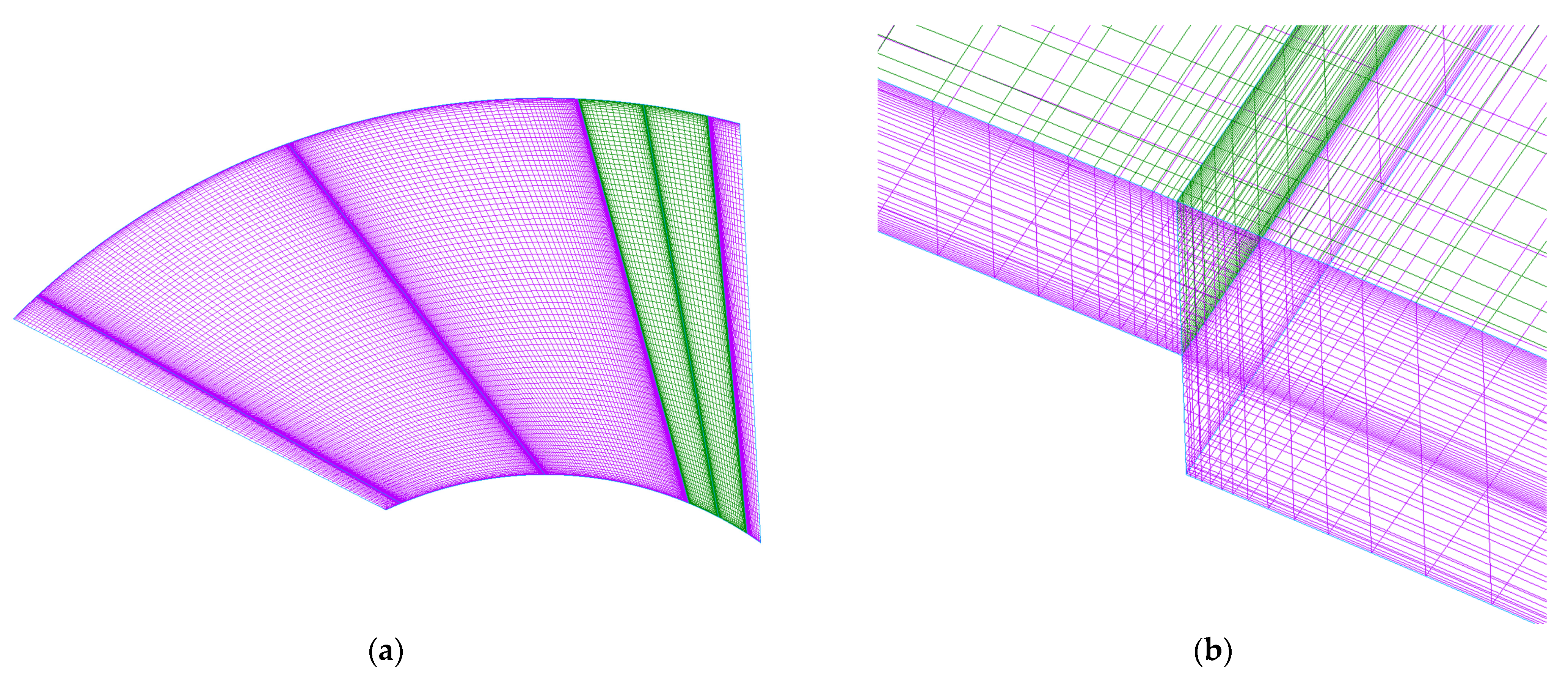
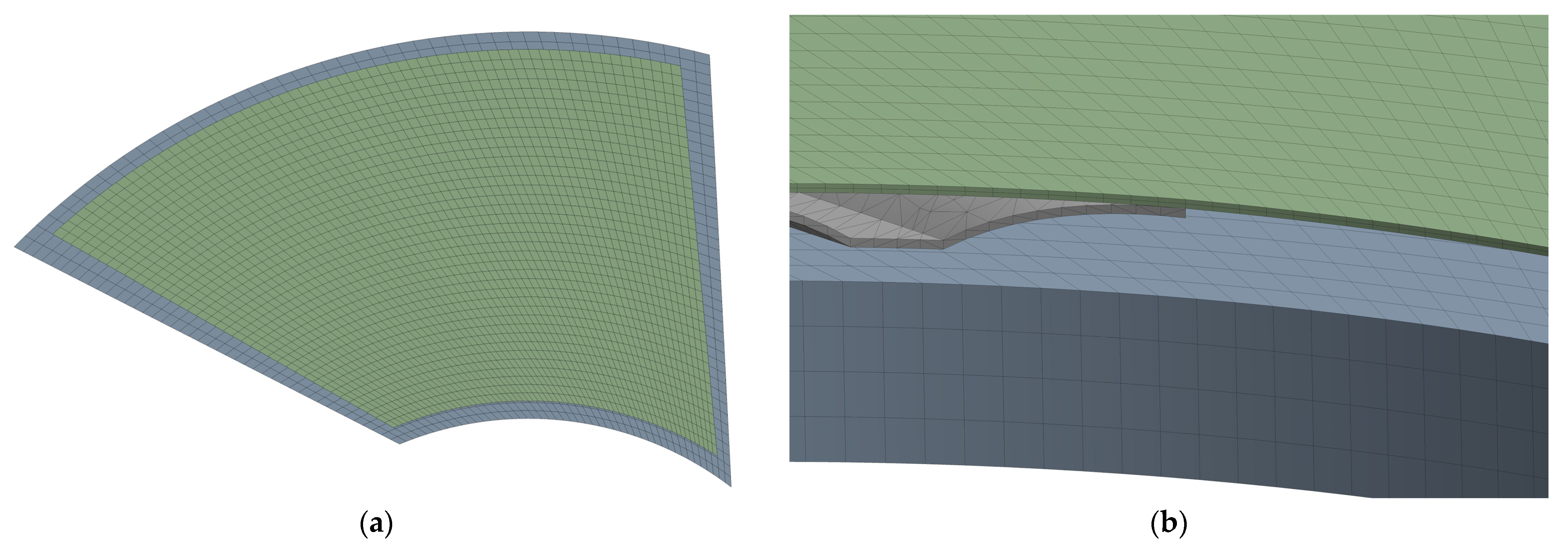
2.4. Mesh
3. Experimental Methodology
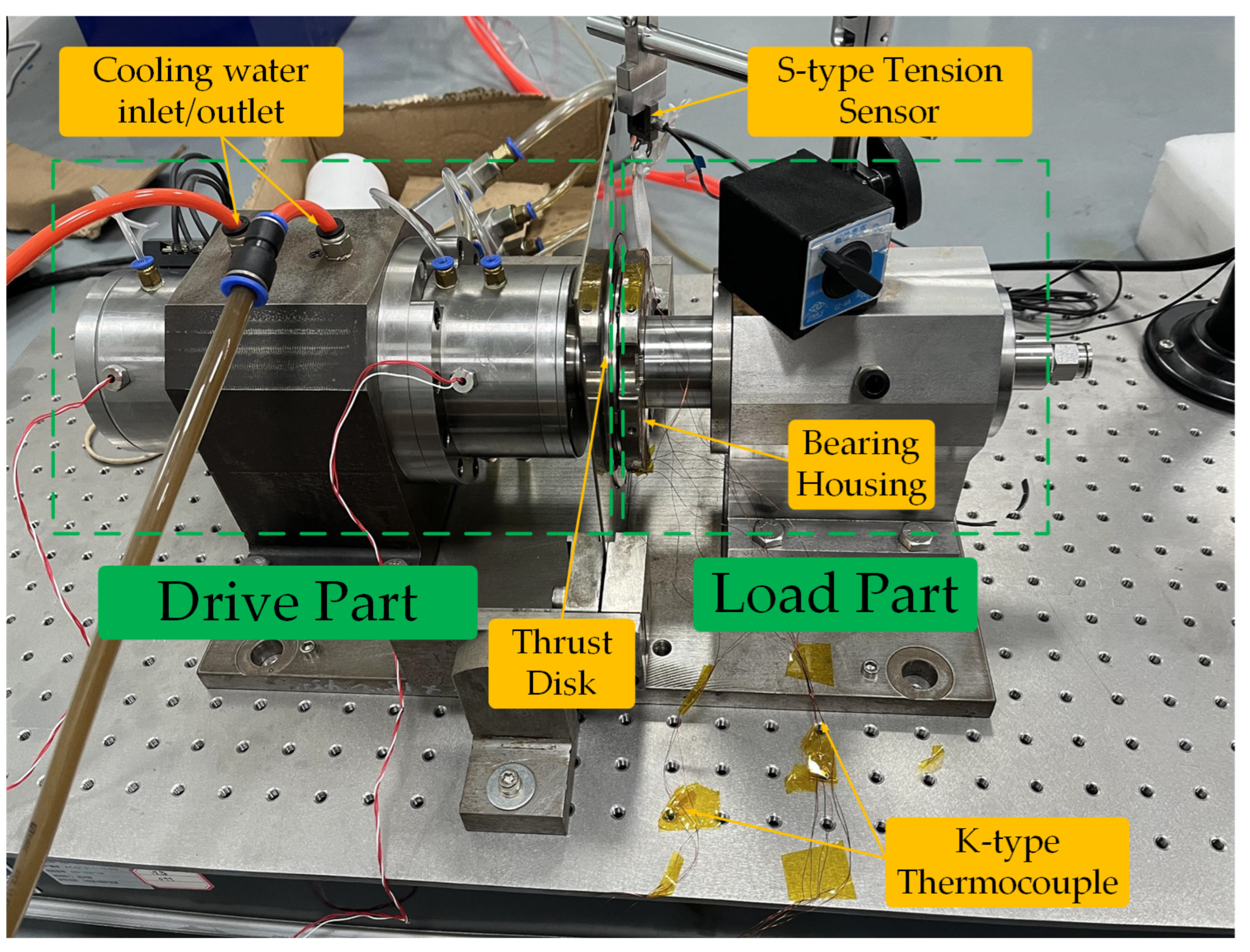
3.1. Test Rig
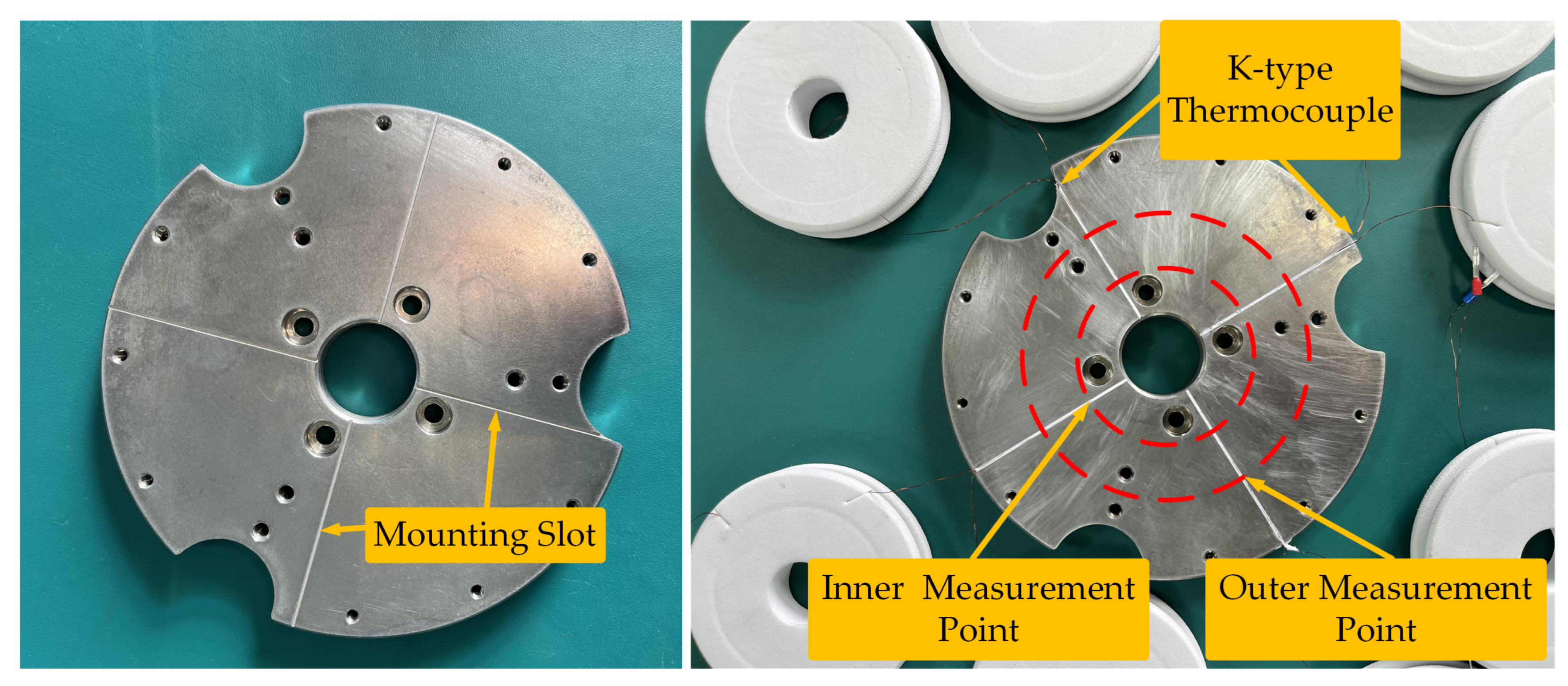
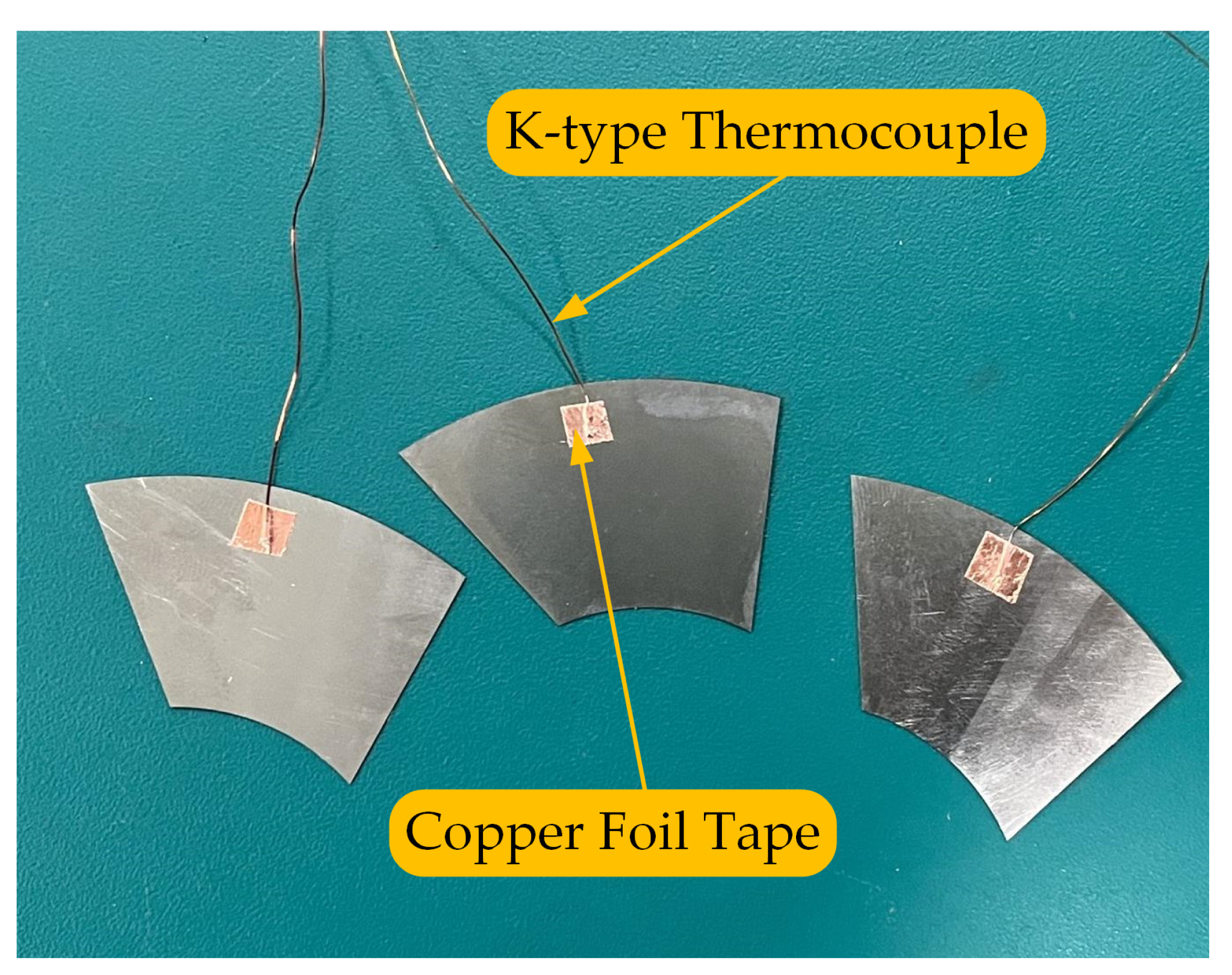
3.2. Test Measurement System
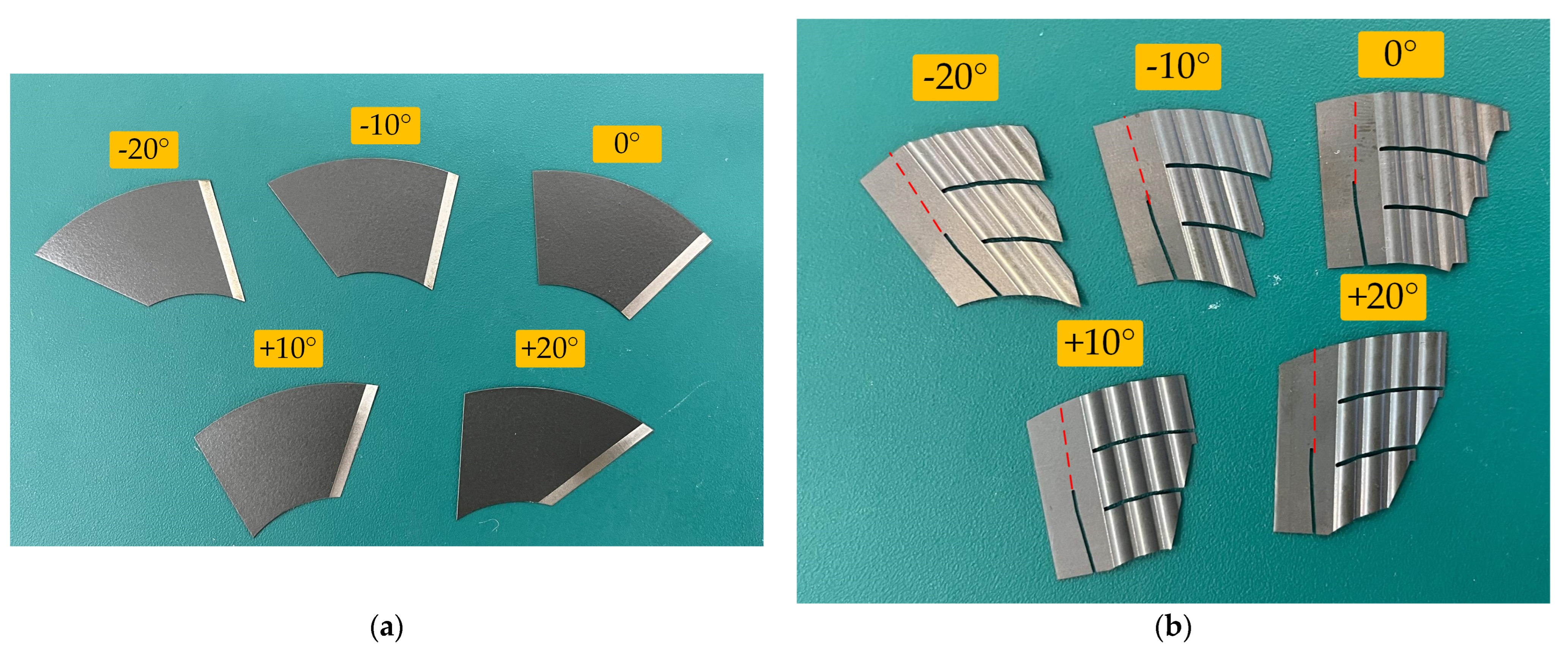
3.3. Test GFTBs
4. Results and Discussion
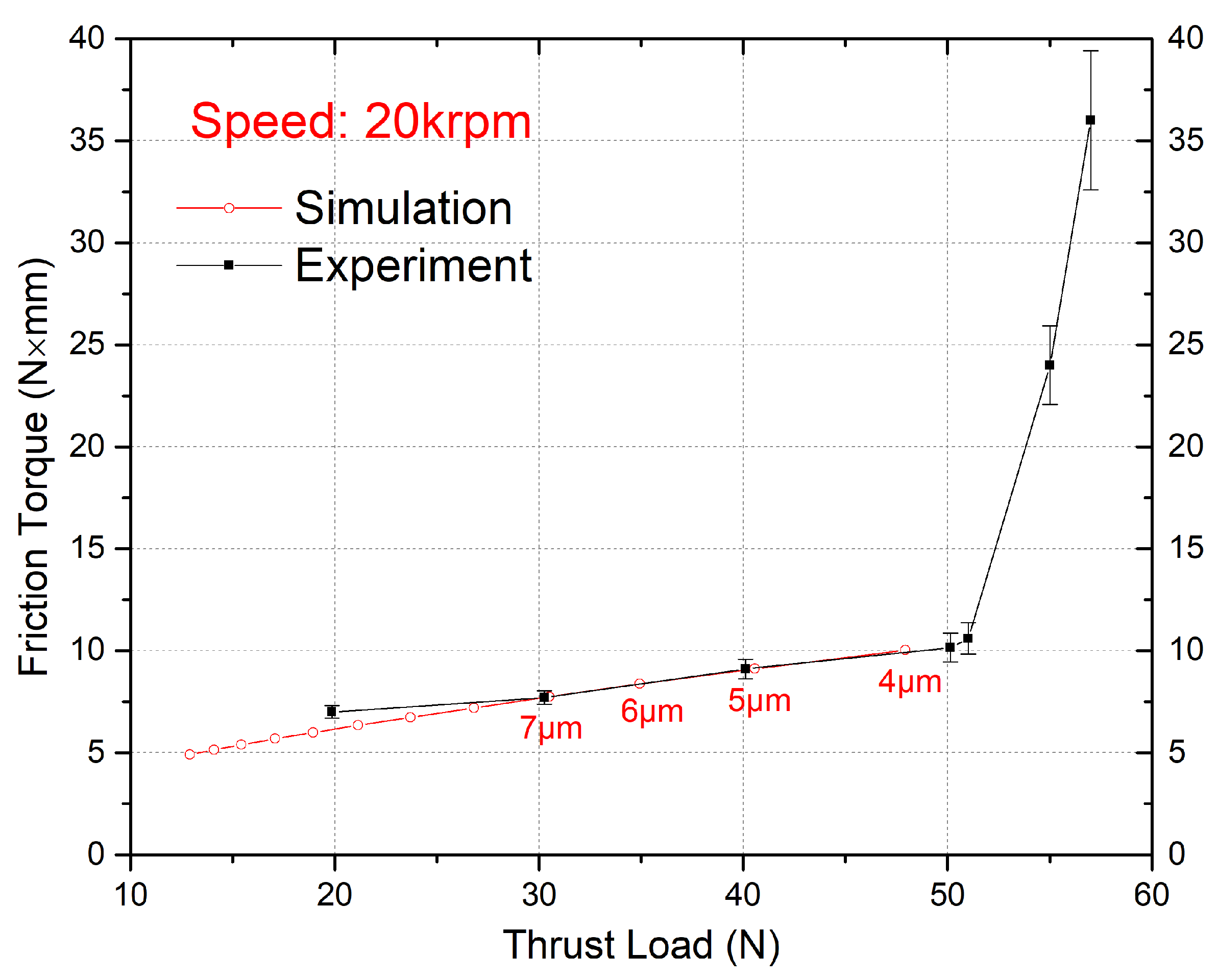
4.1. Load Capacity
4.2. Thermal Characteristics
5. Conclusions
- The load capacity increases as the nominal clearance decreases, with a more substantial capacity boost observed at smaller nominal clearance values. For the GFTBs examined in this study with a minimum nominal clearance of 4 μm, boundary angles do not impact the ultimate load capacity at varying rotational speeds.
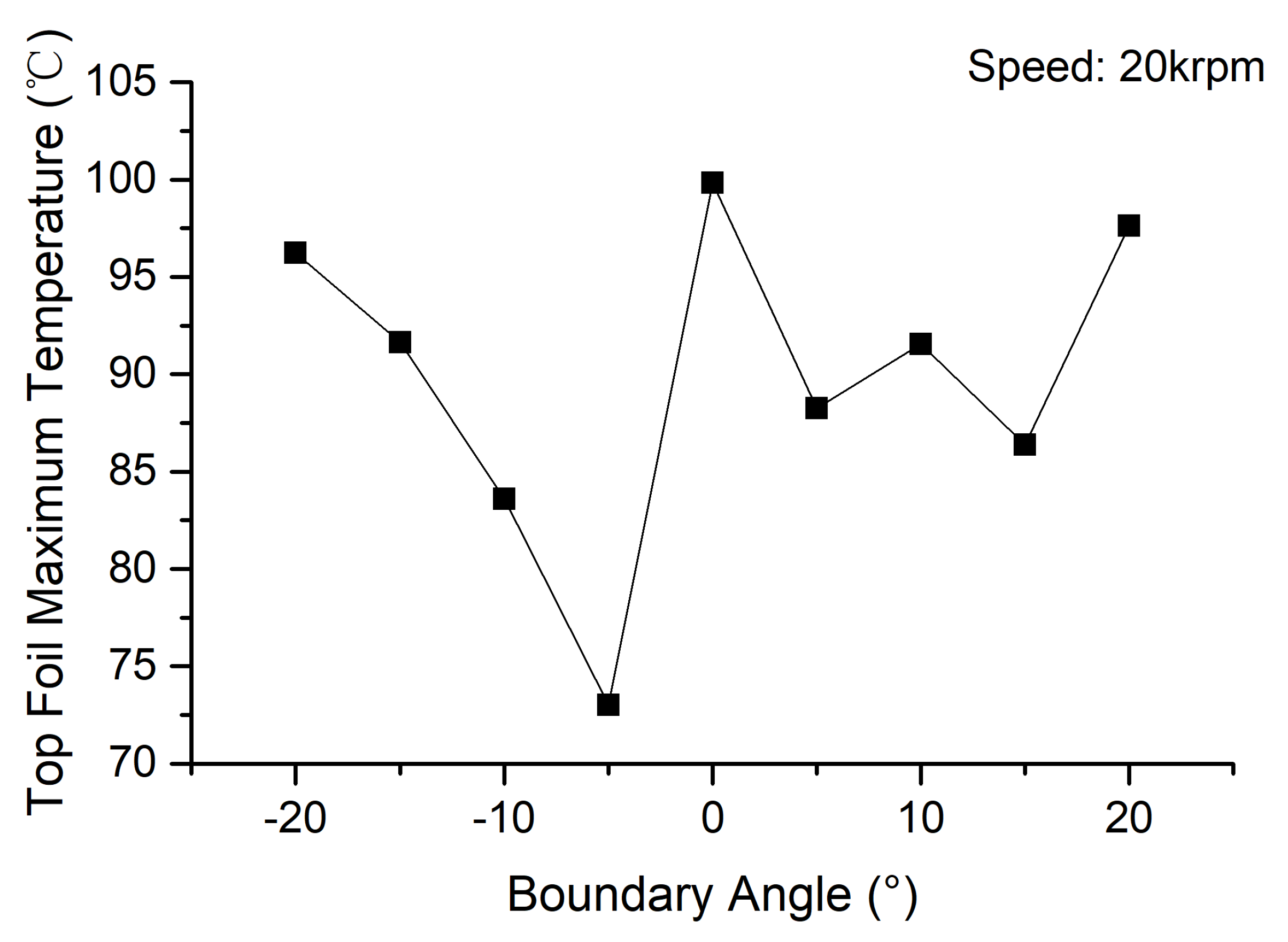
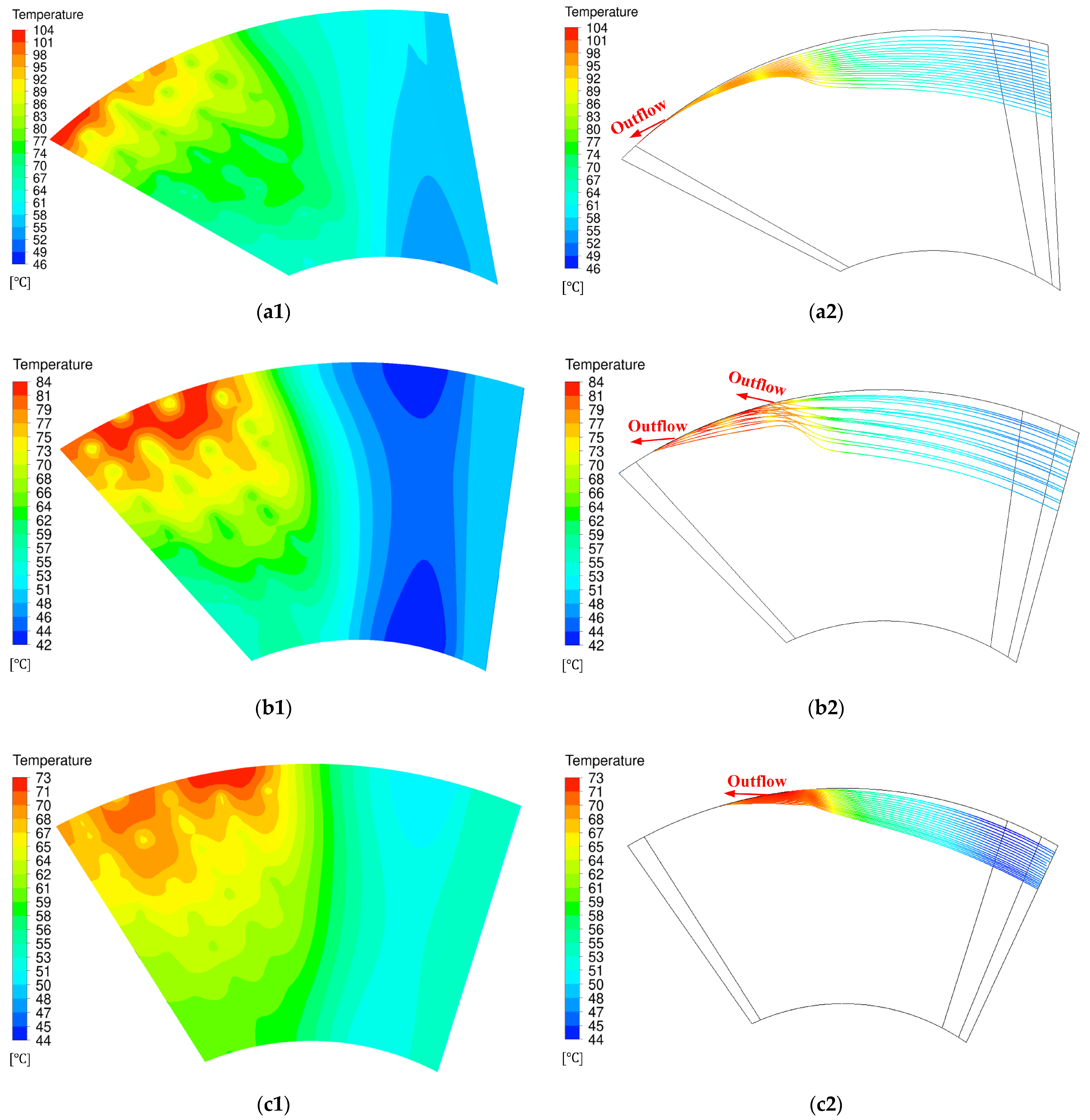
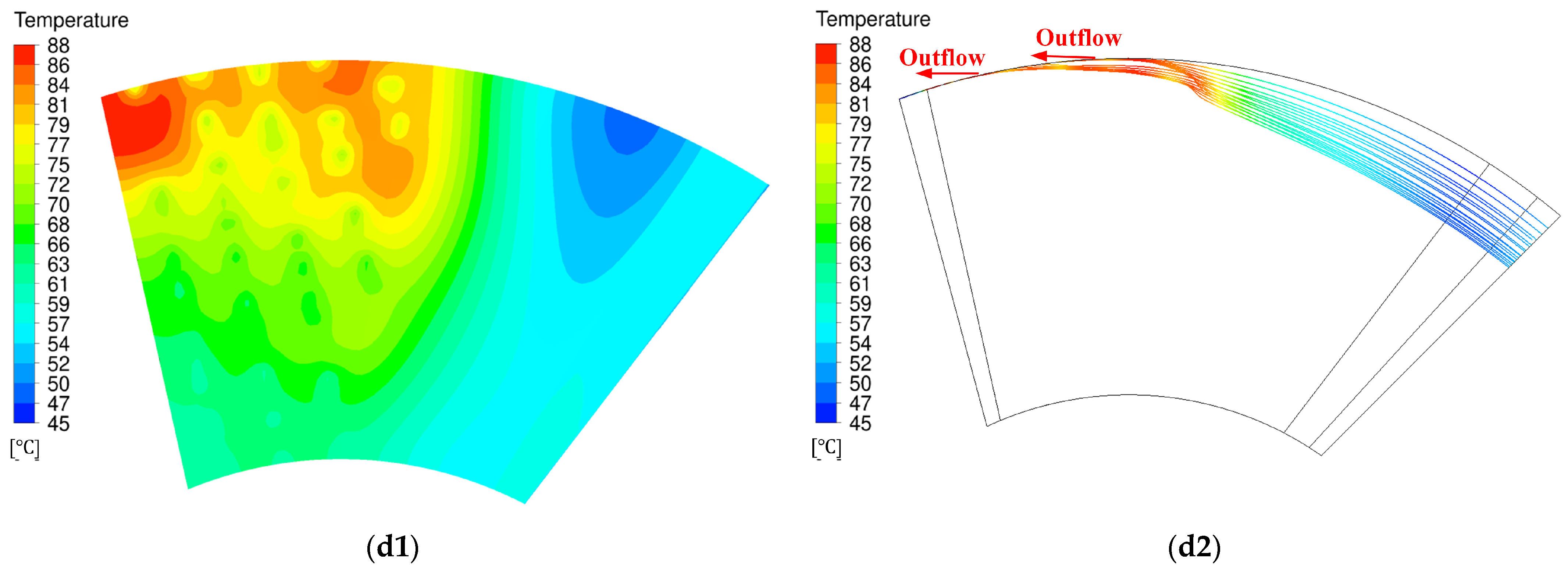
- Bearing −10° exhibits higher air film pressure and a larger high-pressure air film area, leading to increased top foil deformation and contact area between the top foil and bump foil. Consequently, it demonstrates superior heat dissipation capabilities, resulting in the lowest average temperature of its top foil.
- The high-pressure distribution area of Bearing −5° is situated near the boundary line and the bearing’s outer diameter region. As heated air from the high-pressure air film enters the top foil’s flat area, it flows from the bearing’s outer diameter to the ambient air after a brief heating period. This configuration contributes to the lowest maximum temperature of the top foil among the studied angles.
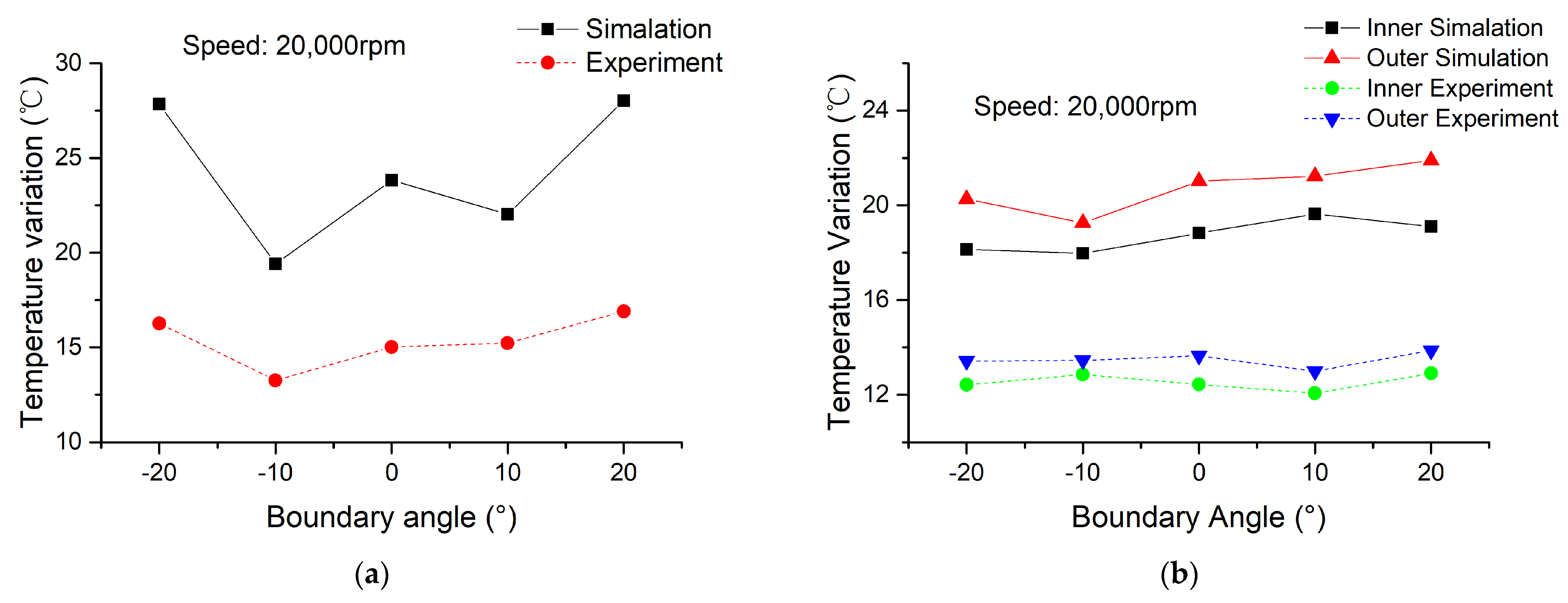
- Novel GFTBs with boundary angles ranging from −10° to −5°, as opposed to conventional GFTBs with a 0° boundary angle, not only maintain load capacity but also enhance thermal characteristics. This improvement is more pronounced at higher rotational speeds, highlighting the efficacy of the novel design.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lee, J.; Cho, H.; Kim, J. Techno-economic analysis of on-site blue hydrogen production based on vacuum pressure adsorption: Practical application to real-world hydrogen refueling stations. J. Environ. Chem. Eng. 2023, 11, 109549. [Google Scholar] [CrossRef]
- Chen, S.; Zuo, S.; Wu, Z. Aerodynamic Performance Modeling of the Centrifugal Compressor and Stability Analysis of the Compression System for Fuel Cell Vehicles. SAE Int. J. Adv. Curr. Pract. Mobil. 2021, 3, 2325–2336. [Google Scholar] [CrossRef]
- Zhang, G.; Huang, M.; Chen, G.; Li, J.; Liu, Y.; He, J.; Zheng, Y.; Tang, S.; Cui, H. Design and optimization of fluid lubricated bearings operated with extreme working performances—A comprehensive review. Int. J. Extrem. Manuf. 2024, 6, 022010. [Google Scholar] [CrossRef]
- Hou, Y.; Zhao, Q.; Guo, Y.; Ren, X.; Lai, T.; Chen, S. Application of Gas Foil Bearings in China. Appl. Sci. 2021, 11, 6210. [Google Scholar] [CrossRef]
- Ha, K.-K.; Lee, C.H.; Kim, C.M.; Kim, S.H.; Ahn, B.K. A Study on the Characteristics of an Oil-Free Centrifugal Compressor for Fuel Cell Vehicles. SAE Int. J. Alt. Power 2016, 5, 167–174. [Google Scholar] [CrossRef]
- Agrawal, G.L. Foil Air/Gas Bearing Technology—An Overview. In Proceedings of the ASME 1997 International Gas Turbine and Aeroengine Congress and Exhibition, Orlando, FL, USA, 2–5 June 1997. [Google Scholar]
- DellaCorte, C.; Bruckner, R.J. Remaining Technical Challenges and Future Plans for Oil-Free Turbomachinery. J. Eng. Gas Turbines Power 2011, 133, 042502. [Google Scholar] [CrossRef]
- Heshmat, H.; Walowit, J.A.; Pinkus, O. Analysis of Gas Lubricated Compliant Thrust Bearings. J. Lubr. Technol. 1983, 105, 638–646. [Google Scholar] [CrossRef]
- Heshmat, H. Analysis of compliant foil bearings with spatially variable stiffness. In Proceedings of the 27th Joint Propulsion Conference, Sacramento, CA, USA, 24–26 June 1991. [Google Scholar]
- Iordanoff, I. Analysis of an Aerodynamic Compliant Foil Thrust Bearing: Method for a Rapid Design. J. Tribol. 1999, 121, 816–822. [Google Scholar] [CrossRef]
- Park, D.-J.; Kim, C.-H.; Jang, G.-H.; Lee, Y.-B. Theoretical considerations of static and dynamic characteristics of air foil thrust bearing with tilt and slip flow. Tribol. Int. 2008, 41, 282–295. [Google Scholar] [CrossRef]
- Lee, Y.-B.; Kim, T.Y.; Kim, C.H.; Kim, T.H. Thrust Bump Air Foil Bearings with Variable Axial Load: Theoretical Predictions and Experiments. Tribol. Trans. 2011, 54, 902–910. [Google Scholar] [CrossRef]
- San Andrés, L.; Ryu, K.; Diemer, P. Prediction of Gas Thrust Foil Bearing Performance for Oil-Free Automotive Turbochargers. J. Eng. Gas Turbines Power 2015, 137, 032502. [Google Scholar] [CrossRef]
- Lehn, A.; Mahner, M.; Schweizer, B. Elasto-gasdynamic modeling of air foil thrust bearings with a two-dimensional shell model for top and bump foil. Tribol. Int. 2016, 100, 48–59. [Google Scholar] [CrossRef]
- Xu, Z.; Li, C.; Du, J.; Li, J.; Wang, Y. Load-carrying characteristics of bump-type gas foil thrust bearings. Int. J. Mech. Sci. 2023, 244, 108080. [Google Scholar] [CrossRef]
- Bruckner, R.J. Simulation and Modeling of the Hydrodynamic, Thermal, and Structural Behavior of Foil Thrust Bearings. Ph.D. Thesis, Case Western Reserve University, Cleveland, OH, USA, 2004. [Google Scholar]
- Bruckner, R.J.; Dellacorte, C.; Prahl, J.M. Analytic Modeling of the Hydrodynamic, Thermal, and Structural Behavior of Foil Thrust Bearings. In Proceedings of the 2005 Annual Meeting and Exhibition, 60th Society of Tribologists and Lubrication Engineers, Las Vegas, NV, USA, 15–19 May 2005. [Google Scholar]
- Lee, D.; Kim, D. Three-Dimensional Thermohydrodynamic Analyses of Rayleigh Step Air Foil Thrust Bearing with Radially Arranged Bump Foils. Tribol. Trans. 2011, 54, 432–448. [Google Scholar] [CrossRef]
- Mahner, M.; Lehn, A.; Schweizer, B. Thermogas- and thermohydrodynamic simulation of thrust and slider bearings: Convergence and efficiency of different reduction approaches. Tribol. Int. 2016, 93, 539–554. [Google Scholar] [CrossRef]
- Lehn, A.; Mahner, M.; Schweizer, B. A thermo-elasto-hydrodynamic model for air foil thrust bearings including self-induced convective cooling of the rotor disk and thermal runaway. Tribol. Int. 2018, 119, 281–298. [Google Scholar] [CrossRef]
- Kumar, J.; Khamari, D.S.; Behera, S.K.; Sahoo, R.K. Influence of slip-flow phenomenon on thermohydrodynamic behaviour of gas foil thrust bearings. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2022, 236, 15–30. [Google Scholar] [CrossRef]
- Qin, K.; Jacobs, P.A.; Keep, J.A.; Li, D.; Jahn, I.H. A fluid-structure-thermal model for bump-type foil thrust bearings. Tribol. Int. 2018, 121, 481–491. [Google Scholar] [CrossRef]
- Liu, X.; Li, C.; Du, J. The Fluid-Structure-Thermal Performance Analysis of Gas Foil Thrust Bearing by Using Computational Fluid Dynamics. Lubricants 2022, 10, 294. [Google Scholar] [CrossRef]
- Gao, Q.-h.; Sun, W.-j.; Zhang, J.-z. Optimal design of top-foil wedge shape for a specific multi-layer gas foil thrust bearing by considering aerodynamic and thermal performances. Therm. Sci. Eng. Prog. 2023, 44, 102060. [Google Scholar] [CrossRef]
- Xiong, C.; Xu, B.; Yu, H.; Huang, Z.; Chen, Z. A thermo-elastic-hydrodynamic model for air foil thrust bearings considering thermal seizure and failure analyses. Tribol. Int. 2023, 183, 108373. [Google Scholar] [CrossRef]
- Xiong, C.; Xu, B.; Yu, H.; Huang, Z.; Chen, Z. Static and dynamic characteristics of aerodynamic thrust bearings based on fluid-thermal-structural interaction approach. Therm. Sci. Eng. Prog. 2023, 42, 101901. [Google Scholar] [CrossRef]
- Kim, T.H.; Park, M.; Lee, T.W. Design Optimization of Gas Foil Thrust Bearings for Maximum Load Capacity1. J. Tribol. 2017, 139, 031705. [Google Scholar] [CrossRef]
- Qin, K.; Li, D.; Huang, C.; Luo, K. Comparative analysis of turbulence models for gas bearings flowfield simulations. Fluid Dyn. Res. 2019, 51, 045505. [Google Scholar] [CrossRef]
- Xiong, C.; Xu, B.; Yu, H.; Huang, Z.; Chen, Z. Thermal failure optimization of foil thrust bearings. Int. J. Mech. Sci. 2024, 267, 109026. [Google Scholar] [CrossRef]
- Hoffmann, R.; Liebich, R. Experimental and numerical analysis of the dynamic behaviour of a foil bearing structure affected by metal shims. Tribol. Int. 2017, 115, 378–388. [Google Scholar] [CrossRef]
- Zywica, G.; Baginski, P.; Bogulicz, M.; Martowicz, A.; Roemer, J.; Kantor, S. Numerical identification of the dynamic characteristics of a nonlinear foil bearing structure: Effect of the excitation force amplitude and the assembly preload. J. Sound Vib. 2022, 520, 116663. [Google Scholar] [CrossRef]
- Dykas, B. Factors Influencing the Performance of Foil Gas Thrust Bearings for Oil-Free Turbomachinery Applications. Ph.D. Thesis, Case Western Reserve University, Cleveland, OH, USA, 2006. [Google Scholar]
- Kim, T.H.; Lee, Y.-B.; Kim, T.Y.; Jeong, K.H. Rotordynamic Performance of an Oil-Free Turbo Blower Focusing on Load Capacity of Gas Foil Thrust Bearings. J. Eng. Gas Turbines Power 2011, 134, 022501. [Google Scholar] [CrossRef]
- Conboy, T.M. Real-Gas Effects in Foil Thrust Bearings Operating in the Turbulent Regime. J. Tribol. 2013, 135, 031703. [Google Scholar] [CrossRef]
- Gao, Q.; Sun, W.; Zhang, J. Thermo-elasto-hydrodynamic analysis of a specific multi-layer gas foil thrust bearing under thermal-fluid–solid coupling. Chin. J. Aeronaut. 2023, 36, 231–246. [Google Scholar] [CrossRef]
- Zhang, J.-Y.; Qiao, X.-Y.; Chen, W.-D.; Chen, B.-X.; Lyu, Y.-W.; Luo, X.-Y. Numerical investigation of Thermo-aerodynamic characteristics of gas foil thrust bearing. Therm. Sci. Eng. Prog. 2022, 31, 101296. [Google Scholar] [CrossRef]










| GFTB Parameters | Value |
|---|---|
| 40 mm | |
| 20 mm | |
| 0.51 mm | |
| 0.5 | |
| Boundary angle | |
| 0.10 mm | |
| 0.10 mm | |
| 1.25 mm | |
| 3.14 mm | |
| Foil Young’s modules | 186 GPa |
| Foil Poisson’s ratio | 0.29 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, B.; Hou, A.; Deng, R.; Yang, X.; Wu, Z.; Ni, Q.; Li, Z. Optimal Design of Boundary Angle for Gas Foil Thrust Bearing Thermal Performance. Lubricants 2024, 12, 143. https://doi.org/10.3390/lubricants12050143
Hu B, Hou A, Deng R, Yang X, Wu Z, Ni Q, Li Z. Optimal Design of Boundary Angle for Gas Foil Thrust Bearing Thermal Performance. Lubricants. 2024; 12(5):143. https://doi.org/10.3390/lubricants12050143
Chicago/Turabian StyleHu, Bin, Anping Hou, Rui Deng, Xiaodong Yang, Zhiyong Wu, Qifeng Ni, and Zhong Li. 2024. "Optimal Design of Boundary Angle for Gas Foil Thrust Bearing Thermal Performance" Lubricants 12, no. 5: 143. https://doi.org/10.3390/lubricants12050143
APA StyleHu, B., Hou, A., Deng, R., Yang, X., Wu, Z., Ni, Q., & Li, Z. (2024). Optimal Design of Boundary Angle for Gas Foil Thrust Bearing Thermal Performance. Lubricants, 12(5), 143. https://doi.org/10.3390/lubricants12050143





