Effect of Micro-Dimple Texture on the Lubrication Characteristics of Elliptical Sliding Pairs Between a Flex Spline and a Novel Forced Wave Generator
Abstract
:1. Introduction
2. Materials and Methods
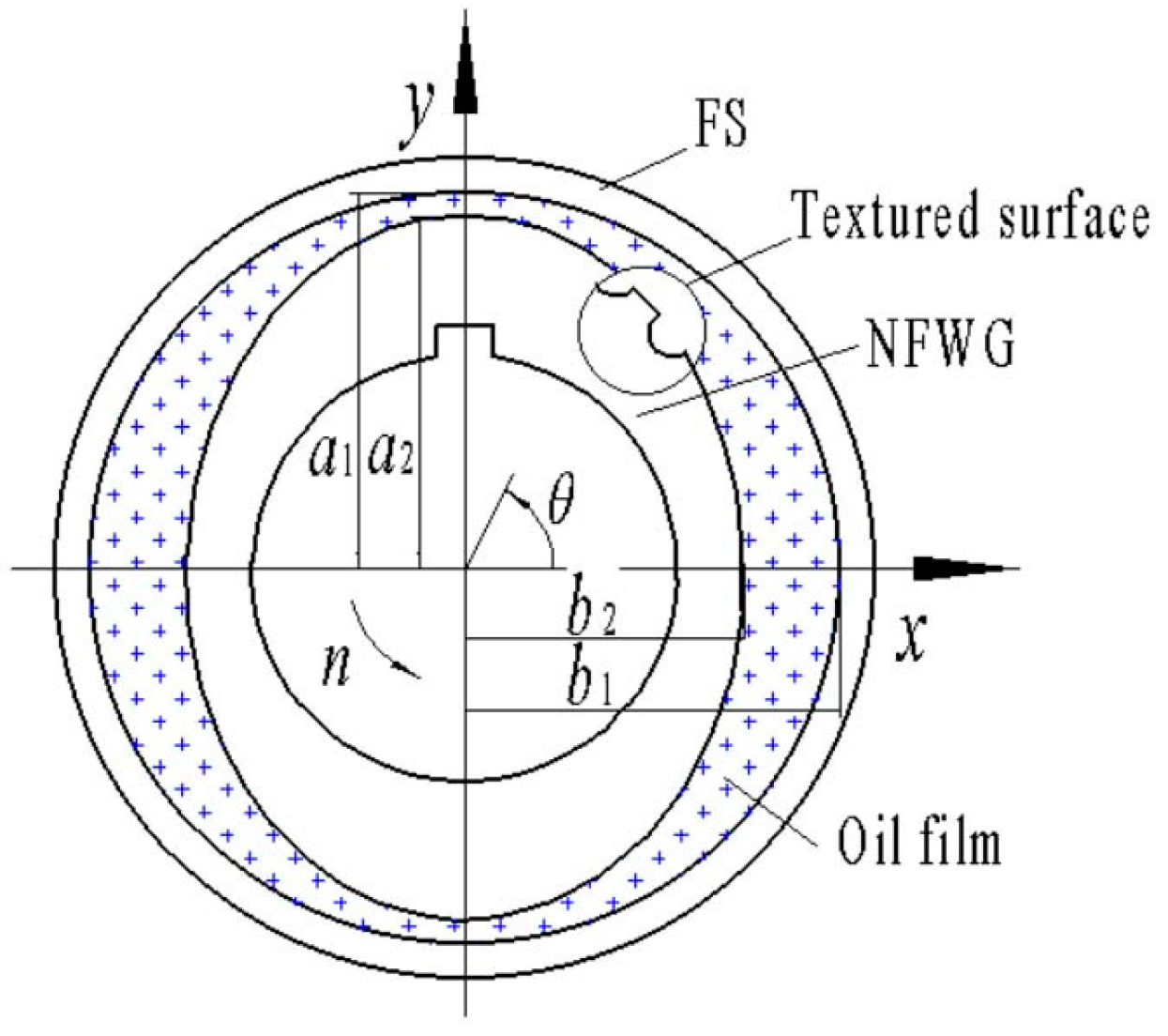
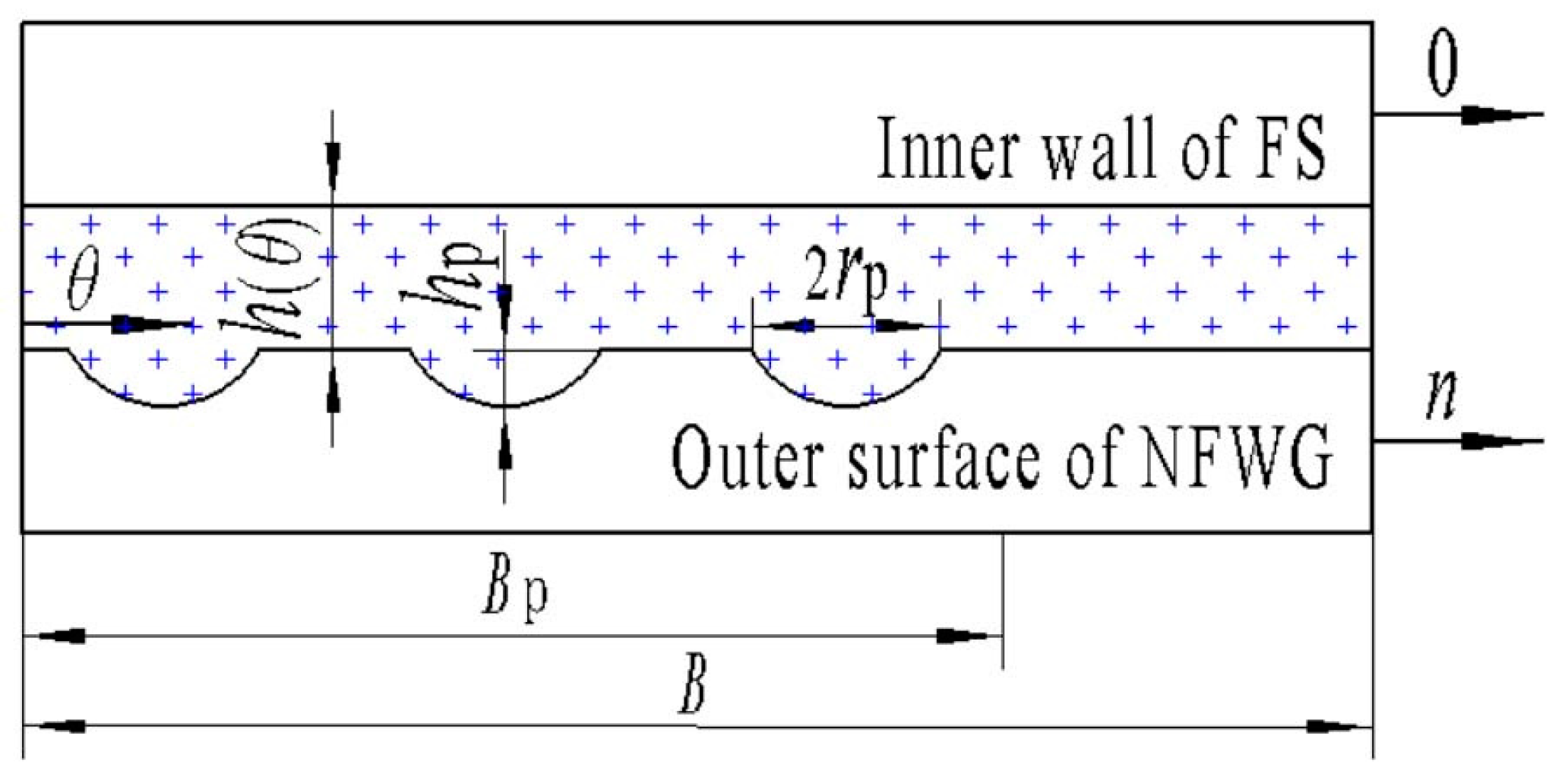
2.1. Mathematical Models
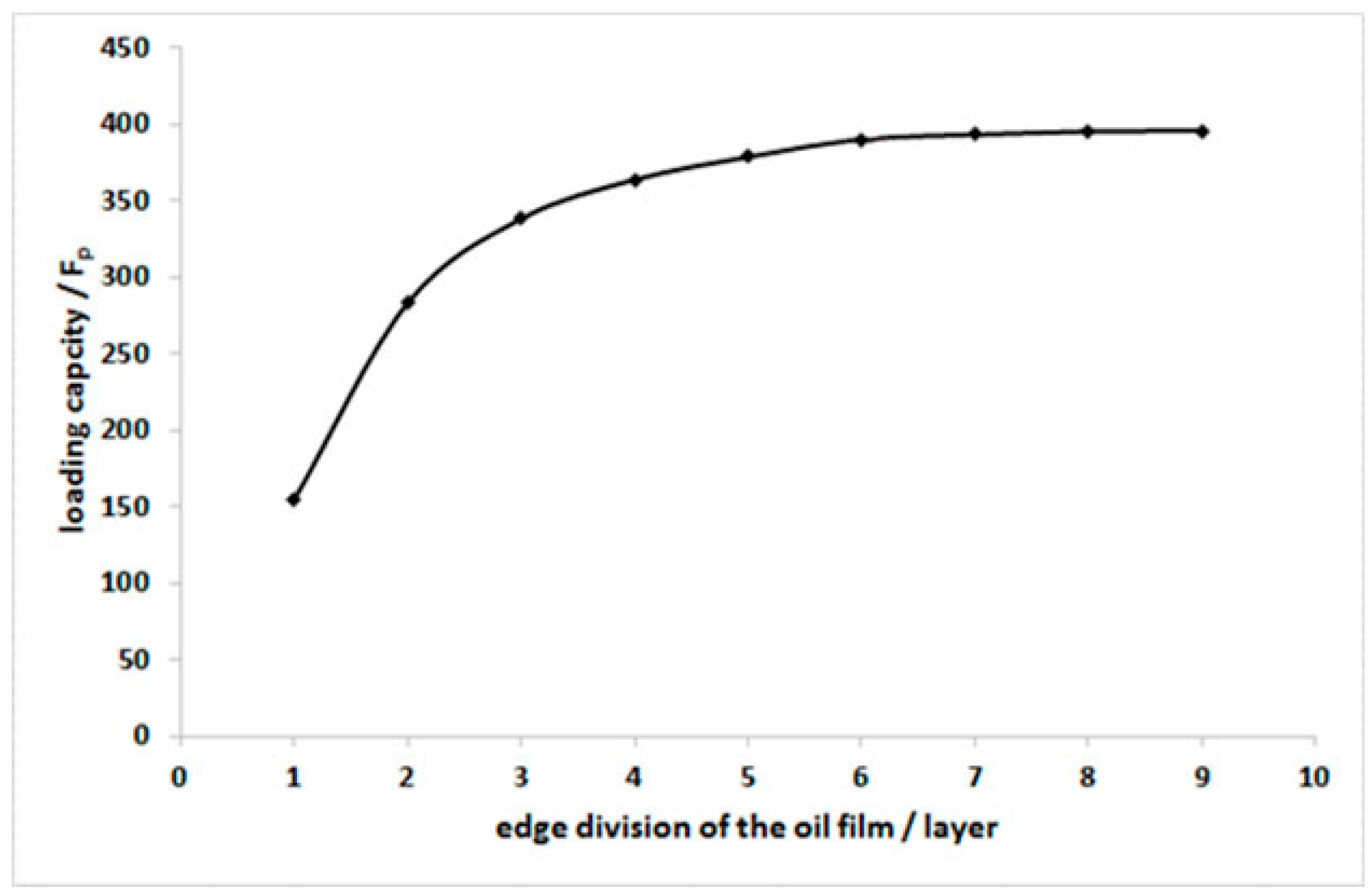
2.2. Numerical Models
3. Results
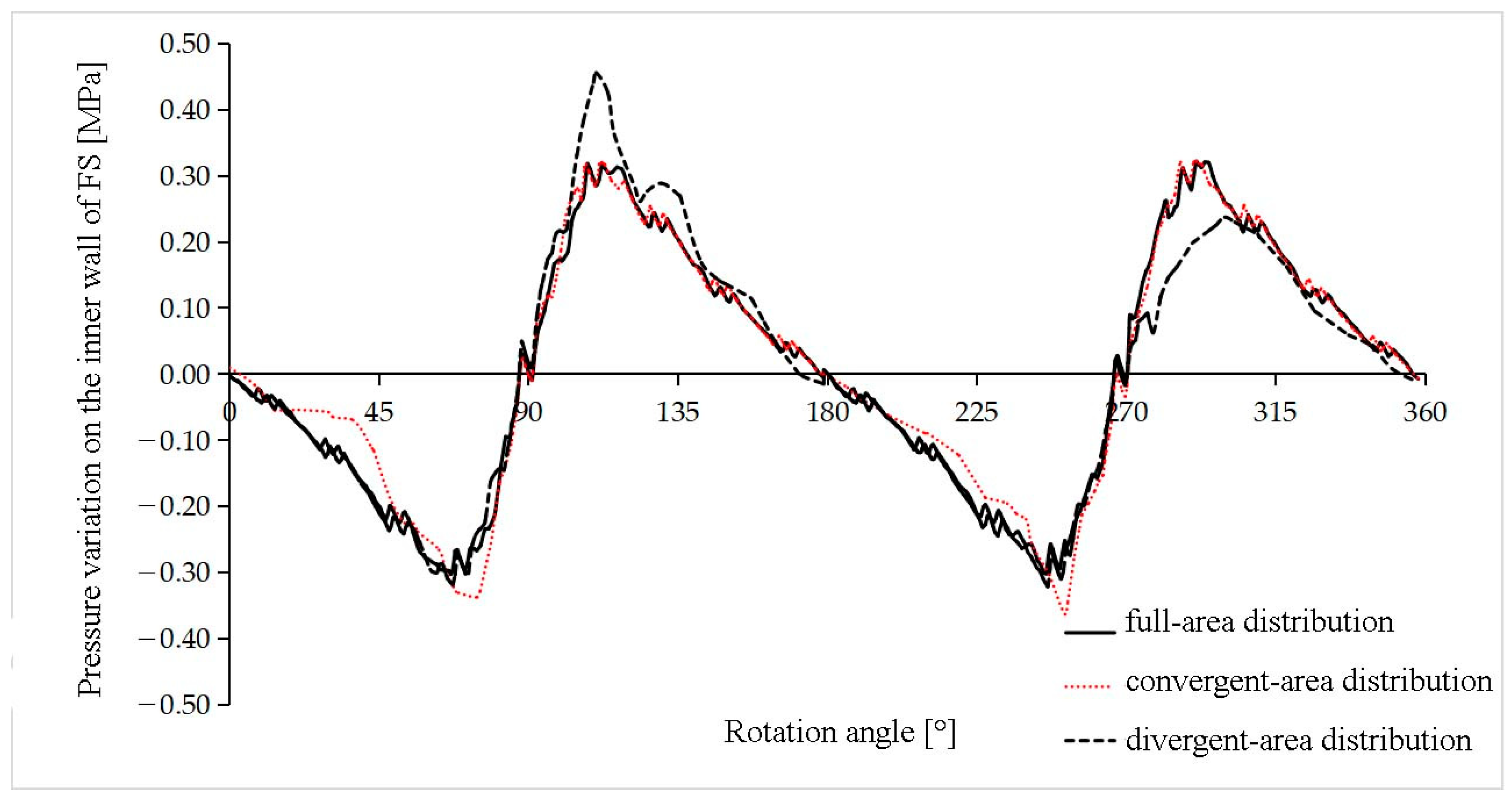
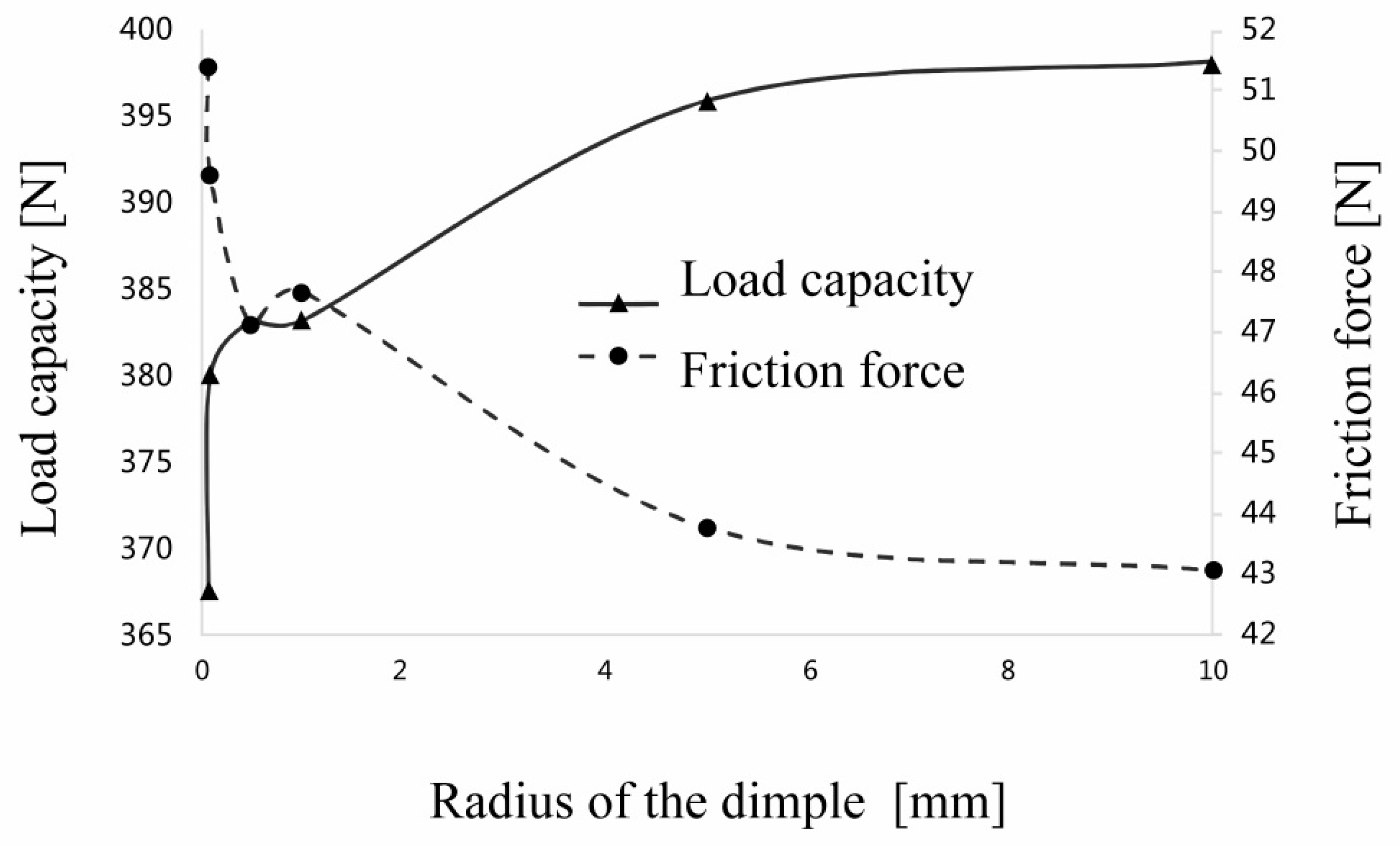
3.1. Effect of Texture Distribution Area
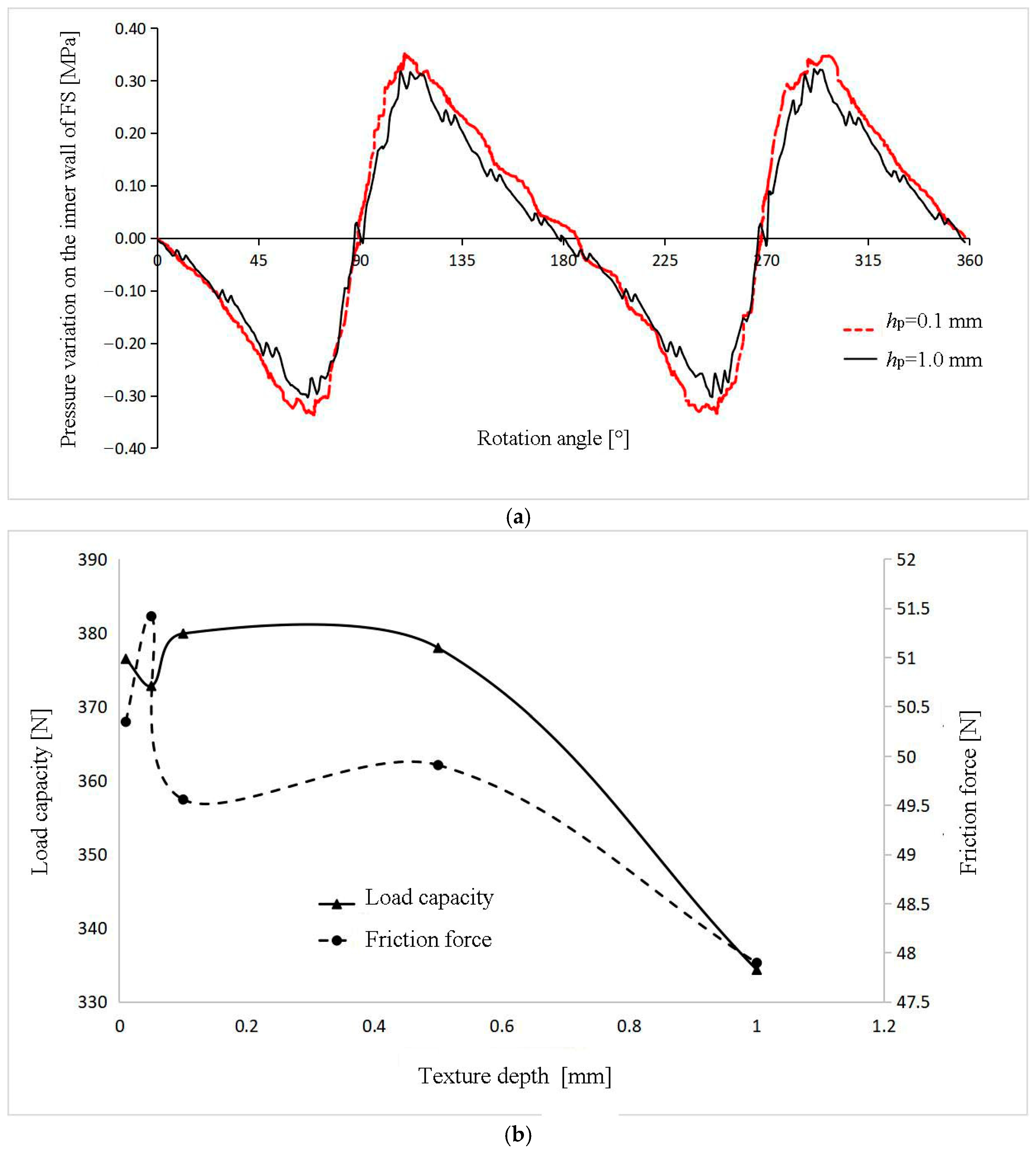
3.2. Effect of Texture Depth
3.3. Effect of Texture Shape
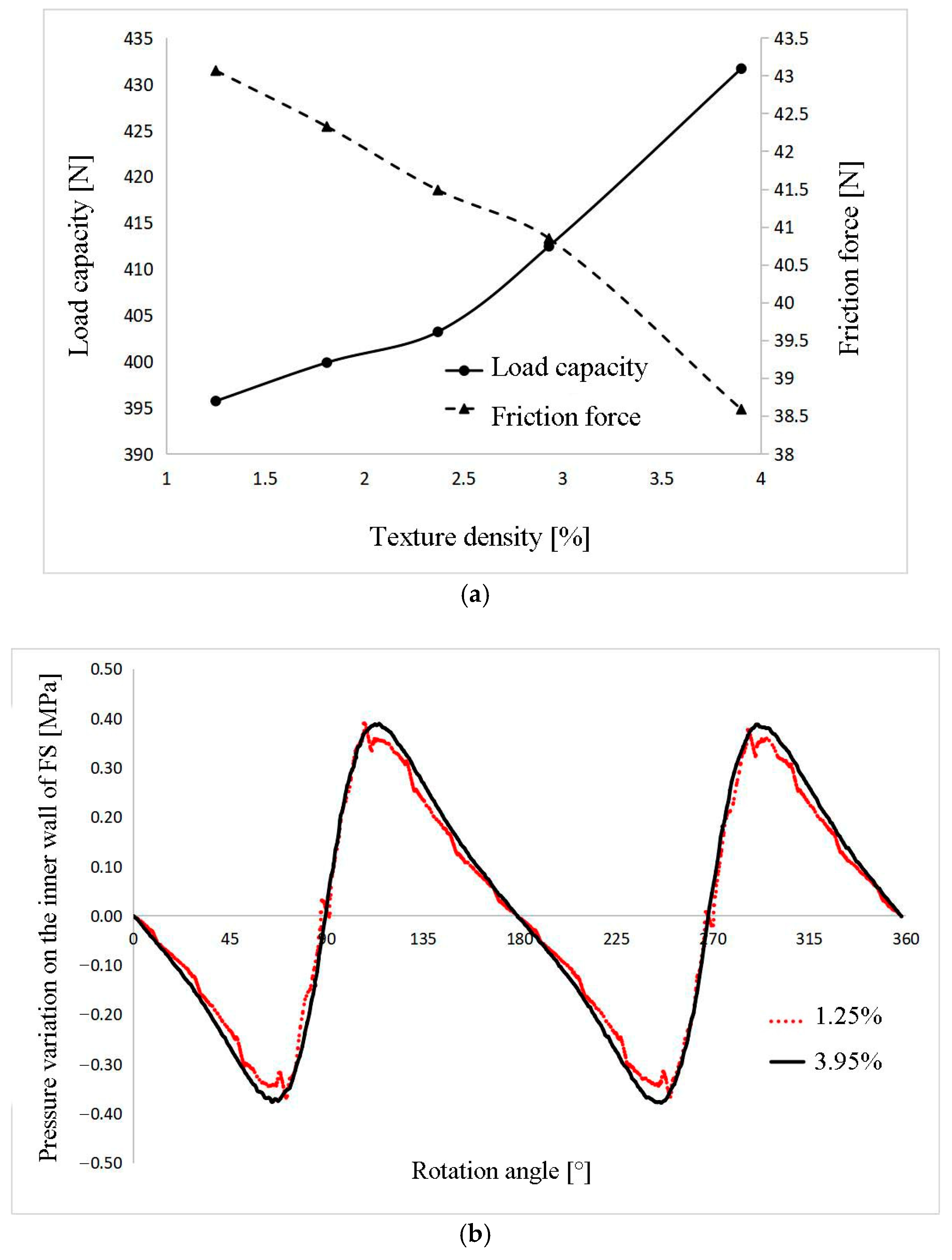
3.4. Effect of Texture Density
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gachot, C.; Rosenkranz, A.; Hsu, S.M.; Costa, H.L. A Critical Assessment of Surface Texturing for Friction and Wear Improvement. Wear 2017, 372–337, 21–41. [Google Scholar] [CrossRef]
- Etsion, I. Surface Texturing For In-cylinder Friction Reduction. In Tribology and Dynamics of Engine and Powertrain: Fundamentals, Applications and Future Trends; Rahnejat, H., Ed.; Woodhead Publishing: Sawston, UK, 2010; pp. 458–470e. [Google Scholar]
- Morris, N.J.; Shahmohamadi, H.; Rahmani, R.; Rahnejat, H.; Garner, C.P. Combined Experimental and Multiphase Computational Fluid Dynamics Analysis of Surface Textured Journal Bearings in Mixed Regime of Lubrication. Lubr. Sci. 2018, 30, 161–173. [Google Scholar] [CrossRef]
- Gu, C.X.; Meng, X.H.; Xie, Y.B.; Yang, Y.M. Effects of Surface Texturing on Ring/Liner Friction Under Starved Lubrication. Tribol. Int. 2016, 94, 591–605. [Google Scholar] [CrossRef]
- Ran, H.; Liu, D.; Wang, S. A Numerical Wear Simulation Method of Reciprocating Seals with a Textured Rod. Materials 2020, 13, 4458. [Google Scholar] [CrossRef]
- Tagawa, N.; Bogy, D.B. Air Film Dynamics for Micro-Textured Flying Head Slider Bearings in Magnetic Hard Disk Drives. J. Tribol. Trans. 2002, 124, 568–574. [Google Scholar] [CrossRef]
- Blatter, A.; Maillat, M.; Pimenov, S.M.; Shafeev, G.A.; Simakin, A.V.; Loubnin, E.N. Lubricated Sliding Performance of Laser-Patterned Sapphire. Wear 1999, 232, 226–230. [Google Scholar] [CrossRef]
- Nam, P.S.; Mohsen, M.; Phillip, S.H. Control of Friction. Wear 1994, 175, 151–158. [Google Scholar]
- Ranjan, R.; Lambeth, D.N.; Tromel, M.; Goglia, P.; Li, Y. Laser Texturing for Low-Flying-Height Media. J. Appl. Phys. 1991, 69, 5745–5747. [Google Scholar] [CrossRef]
- Wang, X.L.; Liu, W.; Zhou, F.; Zhu, D. Preliminary Investigation of the Effect of Dimple Size on Friction in Line Contacts. Tribol. Int. 2009, 42, 1118–1123. [Google Scholar] [CrossRef]
- Steinhoff, K.; Rasp, W.; Pawelski, O. Development of Deterministic-Stochastic Surface Structures to Improve the Tribological Conditions of Sheet Forming Processes. J. Mater. Process. Technol. 1996, 60, 355–361. [Google Scholar] [CrossRef]
- Yu, T.H.; Sadeghi, F. Groove Effects on Thrust Washer Lubrication. J. Tribol. 2001, 123, 295–304. [Google Scholar] [CrossRef]
- Scaraggi, M.; Mezzapesa, F.P.; Carbone, G.; Ancona, A.; Tricarico, L. Friction Properties of Lubricated Laser-MicroTextured-Surfaces: An Experimental Study from Boundary- to Hydrodynamic- Lubrication. Tribol. Lett. 2013, 49, 117–125. [Google Scholar] [CrossRef]
- Andersson, P.; Koskinen, J.; Varjus, S.; Gerbig, Y.; Haefke, H.; Georgiou, S.; Zhmud, B.; Buss, W. Microlubrication Effect by Laser-Textured Steel Surfaces. Wear 2007, 262, 369–379. [Google Scholar] [CrossRef]
- Lu, P.; Wood, R.J. Tribological Performance of Surface Texturing in Mechanical Applications-A Review. Surf. Topogr. Metrol. Prop. 2020, 8, 043001. [Google Scholar] [CrossRef]
- Rahmani, R.; Rahnejat, H. Enhanced Performance of Optimised Partially Textured Load Bearing Surfaces. Tribol. Int. 2018, 117, 272–282. [Google Scholar] [CrossRef]
- Meng, F.M.; Zhou, R.; Davis, T.; Cao, J.; Wang, Q.J.; Hua, D.; Liu, J. Study on Effect of Dimples on Friction of Parallel Surfaces under Different Sliding Conditions. Appl. Surf. Sci. 2010, 256, 2863–2875. [Google Scholar] [CrossRef]
- Vlădescu, S.C.; Medina, S.; Olver, A.V.; Pegg, I.G.; Reddyhoff, T. Lubricant Film Thickness and Friction Force Measurements in A Laser Surface Textured Reciprocating Line Contact Simulating the Piston Ring–Liner Pairing. Tribol. Int. 2016, 98, 317–329. [Google Scholar] [CrossRef]
- Walker, J.C.; Kamps, T.J.; Lam, J.W.; Mitchell-Smith, J.; Clare, A.T. Tribological Behaviour of An Electrochemical Jet Machined Textured Al-Si Automotive Cylinder Liner Material. Wear 2017, 376–377 Pt B, 1611–1621. [Google Scholar] [CrossRef]
- Qiu, M.; Delic, A.; Raeymaekers, B. The Effect of Texture Shape on the Load-Carrying Capacity of Gas-Lubricated Parallel Slider Bearings. Tribol. Lett. 2012, 48, 315–327. [Google Scholar] [CrossRef]
- Etsion, I. State of the Art in Laser Surface Texturing. J. Tribol. 2005, 127, 248–253. [Google Scholar] [CrossRef]
- Jia, H.; Xin, H. Study on Lubrication Characteristics of Novel Forced Wave Generator of Harmonic Drive without Flexible Bearing. Materials 2022, 15, 215. [Google Scholar] [CrossRef] [PubMed]
- Ivannov, M.N. Harmonic Drive; National Defence Press: Beijing, China, 1987. [Google Scholar]
- Xin, H.B.; He, H.Y.; Xi, J.R. Research on Meshing Stiffness of Harmonic Drive. Opt. Precision Eng. 1997, 5, 26–29. [Google Scholar]
- Shen, Y.W.; Ye, Q.T. Theory and Design of Harmonic Drive; China Machine Press: Beijing, China, 1985. [Google Scholar]
- Xin, H.B. Fracture Strength Analysis of Flexspline Materials Applied Frequently. J. Changchun Inst. Opt. and Fine Mech. 1998, 21, 65–67. [Google Scholar]
- Wang, X.; Shao, F.X.; Yuan, H.X. Structure and Mold Design of Plastic Flexible Wheels. China Plas. 2016, 30, 100–104. [Google Scholar]
- Wu, K.C.; Liu, J.W.; Zhang, Z.B.; Wang, S.B.; Hu, X.Q. Study on Harmonic Gear Reducer From Engineering Plastics. China Plas. 1993, 7, 30–35. [Google Scholar]
- Gertzos, K.P.; Nikolakopoulos, P.G.; Papadopoulos, C.A. CFD analysis of journal bearing hydrodynamic lubrication by Bingham lubricant. Tribol. Int. 2008, 41, 1190–1204. [Google Scholar] [CrossRef]
- Westerberg, L.G.; Sarkar, C.; Lladós, J.F.; Lundström, T.S.; Höglund, E. Lubricating Grease Flow in a Double Restriction Sealgeometry: A Computational Fluid dynamics Spproach. Tribol. Lett. 2017, 65, 82. [Google Scholar] [CrossRef]
- Li, J.X.; Westerberg, L.G.; Höglund, E.; Lugt, P.M.; Baart, P. Lubricating Grease Shear Flow and Boundary Layers in a Concentric Cylinder Configuration. Tribol. Trans. 2014, 57, 1106–1115. [Google Scholar] [CrossRef]
| = 0.05 mm | /MPa | Fp/N | /N | = 0.1 mm | /MPa | Fp/N | /N |
| Convergent | 0.45 | 367.84 | 66.41 | Convergent | 0.42 | 373.09 | 71.72 |
| Divergent | 0.35 | 359.59 | 68.6 | Divergent | 0.47 | 383.21 | 64.84 |
| Full | 0.364 | 372.84 | 51.42 | Full | 0.41 | 379.92 | 48.56 |
| = 0.5 mm | /MPa | Fp/N | /N | = 1 mm | /MPa | Fp/N | /N |
| Convergent | 0.35 | 374.26 | 72.79 | Convergent | 0.33 | 333.55 | 70.34 |
| Divergent | 0.44 | 391.32 | 64.64 | Divergent | 0.498 | 355.08 | 66.58 |
| Full | 0.37 | 377.98 | 49.91 | Full | 0.32 | 334.39 | 49.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yi, Z.; Xin, H. Effect of Micro-Dimple Texture on the Lubrication Characteristics of Elliptical Sliding Pairs Between a Flex Spline and a Novel Forced Wave Generator. Lubricants 2025, 13, 192. https://doi.org/10.3390/lubricants13050192
Yi Z, Xin H. Effect of Micro-Dimple Texture on the Lubrication Characteristics of Elliptical Sliding Pairs Between a Flex Spline and a Novel Forced Wave Generator. Lubricants. 2025; 13(5):192. https://doi.org/10.3390/lubricants13050192
Chicago/Turabian StyleYi, Zixiang, and Hongbing Xin. 2025. "Effect of Micro-Dimple Texture on the Lubrication Characteristics of Elliptical Sliding Pairs Between a Flex Spline and a Novel Forced Wave Generator" Lubricants 13, no. 5: 192. https://doi.org/10.3390/lubricants13050192
APA StyleYi, Z., & Xin, H. (2025). Effect of Micro-Dimple Texture on the Lubrication Characteristics of Elliptical Sliding Pairs Between a Flex Spline and a Novel Forced Wave Generator. Lubricants, 13(5), 192. https://doi.org/10.3390/lubricants13050192




