The Relationship between Change of Direction Tests in Elite Youth Soccer Players
Abstract
:1. Introduction
2. Materials and Methods
2.1. Subjects
2.2. Procedures
2.3. Statistical Analyses
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Nimphius, S.; Callaghan, S.J.; Spiteri, T.; Lockie, R.G. Change of Direction Deficit: A more isolated measure of change of direction performance than total 505 timeu. J. Srength Cond. Res. 2016, 30, 3024–3032. [Google Scholar] [CrossRef] [PubMed]
- Reilly, T.; Williams, M. Science and Soccer; Routledge: New York, NY, USA, 2003. [Google Scholar]
- Nimphius, S. Increasing Agility. High-Performance Training for Sports; Human Kinetics: Champaign, IL, USA, 2014. [Google Scholar]
- Pauole, K.; Madole, K.; Garhammer, J.; LaCourse, M.; Rozenek, R. Reliability and Validity of the T-Test as a Measure of Agility, Leg Power, and Leg Speed in College-Aged Men and Women. J. Srength Cond. Res. 2000, 14, 443–450. [Google Scholar]
- Gabbett, T.; Georgieff, B. Physiological and Anthropometric Characteristics of Australian Junior National, State, and Novice Volleyball Players. J. Srength Cond. Res. 2007, 21, 902. [Google Scholar]
- Lockie, R.G.; Jalilvand, F. Realiability and criterion validity of the arrowhead change-of-direction speed test for soccer. Phys. Educ. Sport 2017, 139–151. [Google Scholar] [CrossRef]
- Miller, M.G.; Herniman, J.J.; Ricard, M.D.; Cheatham, C.C.; Michael, T.J. The Effects of a 6-Week Plyometric Training Program on Agility. J. Sports Sci. Med. 2006, 5, 459–465. [Google Scholar]
- Keiner, M.; Sander, A.; Wirth, K.; Schmidtbleicher, D. Long-Term Strength Trainings Effects on Change-of-Direction Sprint Performance. J. Srength Cond. Res. 2013, 28, 223–231. [Google Scholar] [CrossRef] [PubMed]
- Desch, M.; Lottermann, S. DFB-Talentförderprogramm: Trainingswissenschaftliche Begleitung durch das Institut für Sportspiele der Deutschen Sporthochschule Köln; Fußball-Verband, B., Ed.; 2003. [Google Scholar]
- Nimphius, S.; Samuel, C.; Neil, B.; Robert, L. Change of Direction and Agility Tests: Challenging our current measures of Performance. Strength Cond. Res. 2017, 40, 26–38. [Google Scholar] [CrossRef]
- Vescovi, J.D.; McGuigan, M.R. Relationships between sprinting, agility, and jump ability in female athletes. J. Sports Sci. 2008, 26, 97–107. [Google Scholar] [CrossRef] [PubMed]
- Brughelli, M.; Cronin, J.; Levin, G.; Chaouachi, A. Understanding change of direction ability in sport. Sports Med. 2008, 38, 1045–1063. [Google Scholar] [CrossRef] [PubMed]
- Draper, J.A.; Lancaster, M.G. The 505 test: A test for agility in the horizontal plane. Aust. J. Sci. Med. Sport 1985, 17, 15–18. [Google Scholar]
- Emmonds, S.; Nicholson, G.; Beggs, C.; Jones, B.; Bissas, A. Importance of physical qualities for speed and change of direction ability in elite female soccer players. J. Srength Cond. Res. 2017. [Google Scholar] [CrossRef]
- Lockie, R.G.; Schultz, A.B.; Callaghan, S.J.; Jeffriess, M.D.; Berry, S.P. Reliability and Validity of a New Test of Change-of-Direction Speed for Field-Based Sports: The Change-of-Direction and Acceleration Test (CODAT). J. Sports Sci. Med. 2013, 12, 88–96. [Google Scholar]
- Cone, J.R. Soccer-Specific Performance Testing of Fitness and Athleticism. Srength Cond. J. 2012, 34, 11–19. [Google Scholar] [CrossRef]
- Buttifant, D.; Cross, K.; Graham, K. Agility and speed of soccer players are two different performance parameters. Paper presented at the Science and Football IV Conference, Sydney, NSW, Australia, 22–26 February 1999. [Google Scholar]
- Stewart, P.F.; Turner, A.N.; Miller, S.C. Reliability, factorial validity, and interrelationship of five commonly used change of direction speed tests. Scand. J. Med. Sci. Sports 2014, 24, 500–506. [Google Scholar] [CrossRef]
- Raya, M.A.; Gailey, R.S.; Gaunaurd, I.A.; Jayne, D.M.; Campbell, S.M.; Gagne, E.; Manrique, P.G.; Muller, D.G.; Tucker, C. Comparison of three agility tests with male servicemembers: Edgren Side Step Test, T-Test, and Illinois Agility Test. J. Rehabil. Res. Dev. 2013, 50, 951–960. [Google Scholar] [CrossRef]
- Çınarlı, F.S.; Kafkas, A.Ş.; Kafkas, M.E. Relationship Between Linear Running and Change of Direction Performances of Male Soccer Players. Turk. J. Sport Exerc. 2018, 20, 93–99. [Google Scholar]
- Hachana, Y.; Chaabene, H.; Nabli, M.A.; Attia, A.; Moualhi, J.; Farhat, N.; Elloumi, M. Test-Retest Reliability, Criterion-Related Validity, and Minimal Detectable Change of the Illinois Agility Test in Male Team Sport Athletes. J. Srength Cond. Res. 2013, 27, 2752–2759. [Google Scholar] [CrossRef]
- Semenick, D. Tests and Measurements: The T-test. Strength Cond. J. 1990, 12, 36. [Google Scholar] [CrossRef]
- Guarav, V.; Singh, S.; Singh, M.; Rathi, B. A comparative study of arm and Shoulder gridle strength and agility of college-lavel baseball pitchers and non-pothers. J. Phys. Educ. Sports Manag. 2011, 2, 17–20. [Google Scholar]
- Shrout, P.E.; Fleiss, J.L. Intraclass correlations: Uses in assessing rater reliability. Psychol. Bull. 1979, 86, 420–428. [Google Scholar] [CrossRef]
- Bortz, J.; Döring, N. Forschungsmethoden und Evaluation für Human- und Sozialwissenschaftler; Springer: Heidelberg, Germany, 2016. [Google Scholar]
- Benjamini, Y.; Hochberg, Y. Controlling the False Discovery Rate: A Practical and Powerful Approach to Multiple Testing. J. R. Stat. Soc. 1995, 57, 289–300. [Google Scholar] [CrossRef]
- Koo, T.K.; Li, M.Y. A Guideline of Selecting and Reporting Intraclass Correlation Coefficients for Reliability Research. J. Chiropr. Med. 2016, 15, 155–163. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Svensson, M.; Drust, B. Testing soccer players. J. Sports Sci. 2005, 23, 601–618. [Google Scholar] [CrossRef] [PubMed]
- Castillo-Rodríguez, A.; Fernández-García, J.C.; Chinchilla-Minguet, J.L.; Carnero, E. Álvarez Relationship Between Muscular Strength and Sprints with Changes of Direction. J. Srength Cond. Res. 2012, 26, 725–732. [Google Scholar] [CrossRef]
- Rouissi, M.; Chtara, M.; Owen, A.; Chaalali, A.; Chaouachi, A.; Gabbett, T.; Chamari, K. Effect of leg dominance on change of direction ability amongst young elite soccer players. J. Sports Sci. 2015, 34, 1–7. [Google Scholar] [CrossRef]
- Frick, U. Taktiktraining im Fußball. In Berichte und Analysen zum Fußballsport VIII: 11 Jahrentagung der dvs-Kommission Fußball vom 22-24. November (1995) in Mainz. (S.125-132); Augustin, D., Ed.; Czwalina Verlag: Hamburg, Germany, 1995. [Google Scholar]
- Oberbeck, H. Seitigkeitsphänomene und Seitigkeitstypologie im Sport. Schriftreihe des Bundesinstitutes für Sportwissenschaft; Hofmann: Schorndorf, Germany, 1989. [Google Scholar]
- Bühner, M. Einführung in Die Test- und Fragebogenkonstruktion; Pearson: München, Germany, 2010. [Google Scholar]
- Verheijin, R. Handbuch Fußballkondition; bfp Versand Anton Lindemann: Leer, Germany, 1997. [Google Scholar]
- Bloomfield, J.; Polman, R.; O’Donoghue, P. Physical demands of different positions in FA Premier League soccer. J. Sports Sci. Med. 2007, 6, 63–70. [Google Scholar]
| Test | Total Test Distance (m) | Number of CODs | Degree of COD | Approximate Total Time of the COD Test (s) | References |
|---|---|---|---|---|---|
| Triangle test | 10 | 2 | 60° | 2.9–3.5 | [8] |
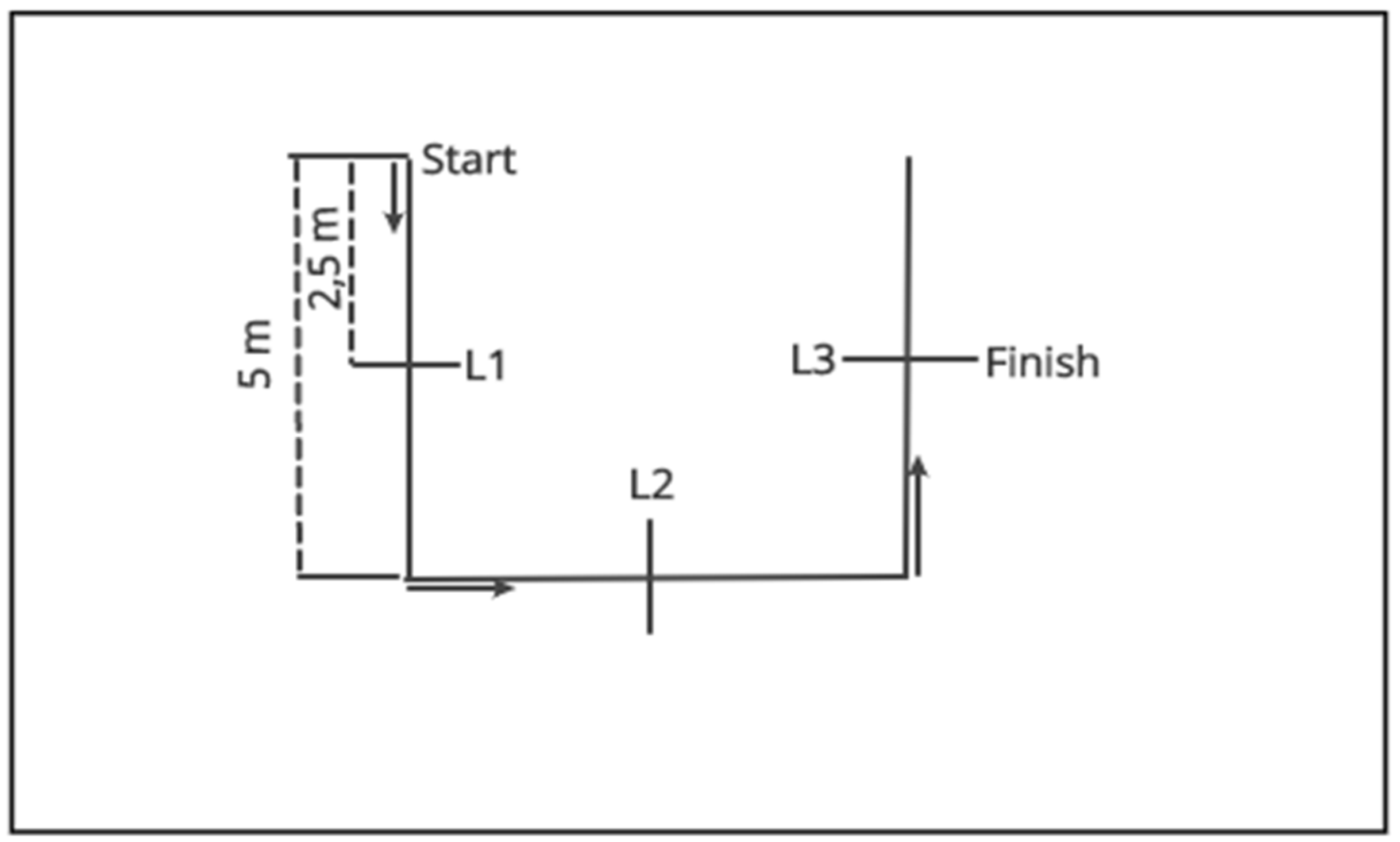
| Square test | 12.5 | 2 | 90° | 2.9–3.5 | Figure 1 |
| 505 agility test | 20 | 1 | 180° | 2.0–3.0 | [14] |
| Gewandtheitslauf | 20 | 6 | 90° | 7–8 | [11] |
| T agility test | 36.6 | 4 | 90° | 10–12 | [7,15] |
| Illinois agility test | 36.6 | 9 | 90°, 180° | 14–18 | [12,15,16] |
| Test | Mean ± SD (in s) |
|---|---|
| 505 | 2.17 ± 0.06 |
| IAT | 13.76 ± 0.31 |
| TT | 9.21 ± 0.30 |
| GewT | 7.50 ± 0.36 |
| Tri-t-L | 3.10 ± 0.12 |
| Tri-t-R | 3.14 ± 0.13 |
| SQT-L | 3.19 ± 0.15 |
| SQT-R | 3.21 ± 0.13 |
| Reliability Analyses | TT | 505 | IAT | GewT | Tri-t-L | Tri-t-R | SQT-L | SQT-R |
|---|---|---|---|---|---|---|---|---|
| ICC | 0.90 | 0.72 | 0.94 | 0.93 | 0.92 | 0.93 | 0.88 | 0.93 |
| 95% Cl | 0.81–0.95 | 0.48–0.87 | 0.88–0.97 | 0.88–0.96 | 0.88–0.95 | 0.9–0.96 | 0.82–0.93 | 0.89–0.95 |
| Tests | TT | 505 | IAT | GewT | Tri-t-L | Tri-t-R | SQT-L | SQT-R | |
|---|---|---|---|---|---|---|---|---|---|
| TT | / | ||||||||
| 505 | r | 0.38 | / | ||||||
| r2 | 0.14 | ||||||||
| IAT | r | 0.29 | 0.51 * | / | |||||
| r2 | 0.08 | 0.26 * | |||||||
| GewT | r | 0.31 | 0.36 # | 0.61 *# | / | ||||
| r2 | 0.10 | 0.13 # | 0.37 *# | ||||||
| Tri-t-L | r | 0.63 *# | 0.45 *# | 0.42 * | 0.51 *# | / | |||
| r2 | 0.40 *# | 0.20 *# | 0.18 * | 0.26 *# | |||||
| Tri-t-R | r | 0.63 *# | 0.43 | 0.23 | 0.37 | 0.51 *# | / | ||
| r2 | 0.40 *# | 0.18 | 0.05 | 0.14 | 0.26 *# | ||||
| SQT-L | r | 0.60 *# | 0.44 * | 0.32 | 0.53 * | 0.74 *# | 0.51 * | / | |
| r2 | 0.36 *# | 0.19 * | 0.10 | 0.28 * | 0.55 *# | 0.26 * | |||
| SQT-R | r | 0.61 *# | 0.45 * | 0.47 *# | 0.56 *# | 0.46 *# | 0.33 # | 0.52 *# | / |
| r2 | 0.37 *# | 0.20 * | 0.22 *# | 0.31 *# | 0.21 *# | 0.11 # | 0.27 *# |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kadlubowski, B.; Keiner, M.; Hartmann, H.; Wirth, K.; Frick, U. The Relationship between Change of Direction Tests in Elite Youth Soccer Players. Sports 2019, 7, 111. https://doi.org/10.3390/sports7050111
Kadlubowski B, Keiner M, Hartmann H, Wirth K, Frick U. The Relationship between Change of Direction Tests in Elite Youth Soccer Players. Sports. 2019; 7(5):111. https://doi.org/10.3390/sports7050111
Chicago/Turabian StyleKadlubowski, Björn, Michael Keiner, Hagen Hartmann, Klaus Wirth, and Ulrich Frick. 2019. "The Relationship between Change of Direction Tests in Elite Youth Soccer Players" Sports 7, no. 5: 111. https://doi.org/10.3390/sports7050111







