Interstitial Atom Engineering in Magnetic Materials
Abstract
:1. Introduction
2. Rare-Earth Fe-Based Compounds
2.1. Th2Zn17-Type
2.2. ThMn12-Type
2.3. BaCd11-Type
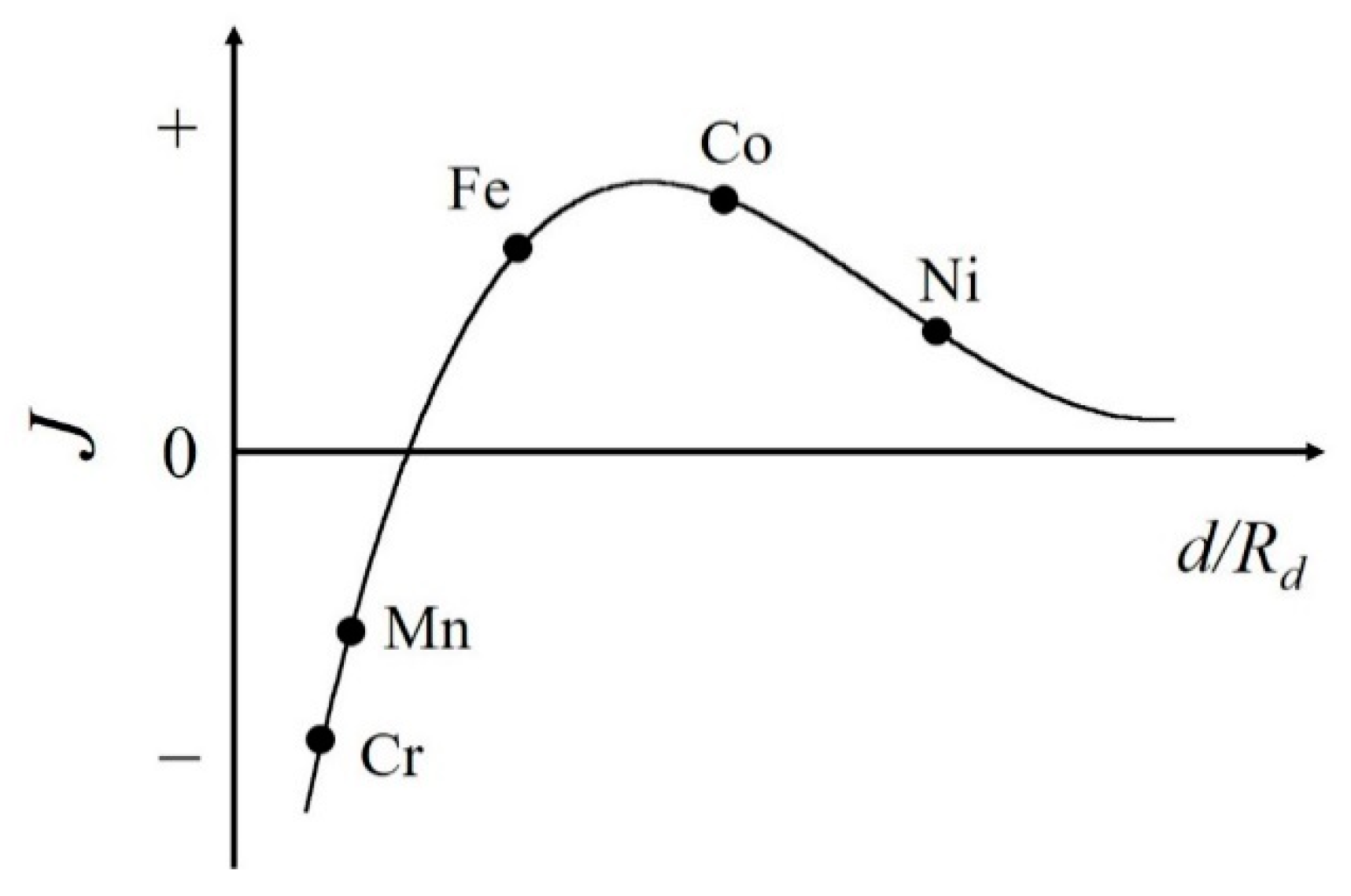
2.4. Remark on Hybridization Effect
3. Mn-Based Compounds
3.1. Hydrogen-Absorbed (R or Th)6Mn23
3.2. Hydrogen-Absorbed YMn2
3.3. Carbon-Added Mn5Si3
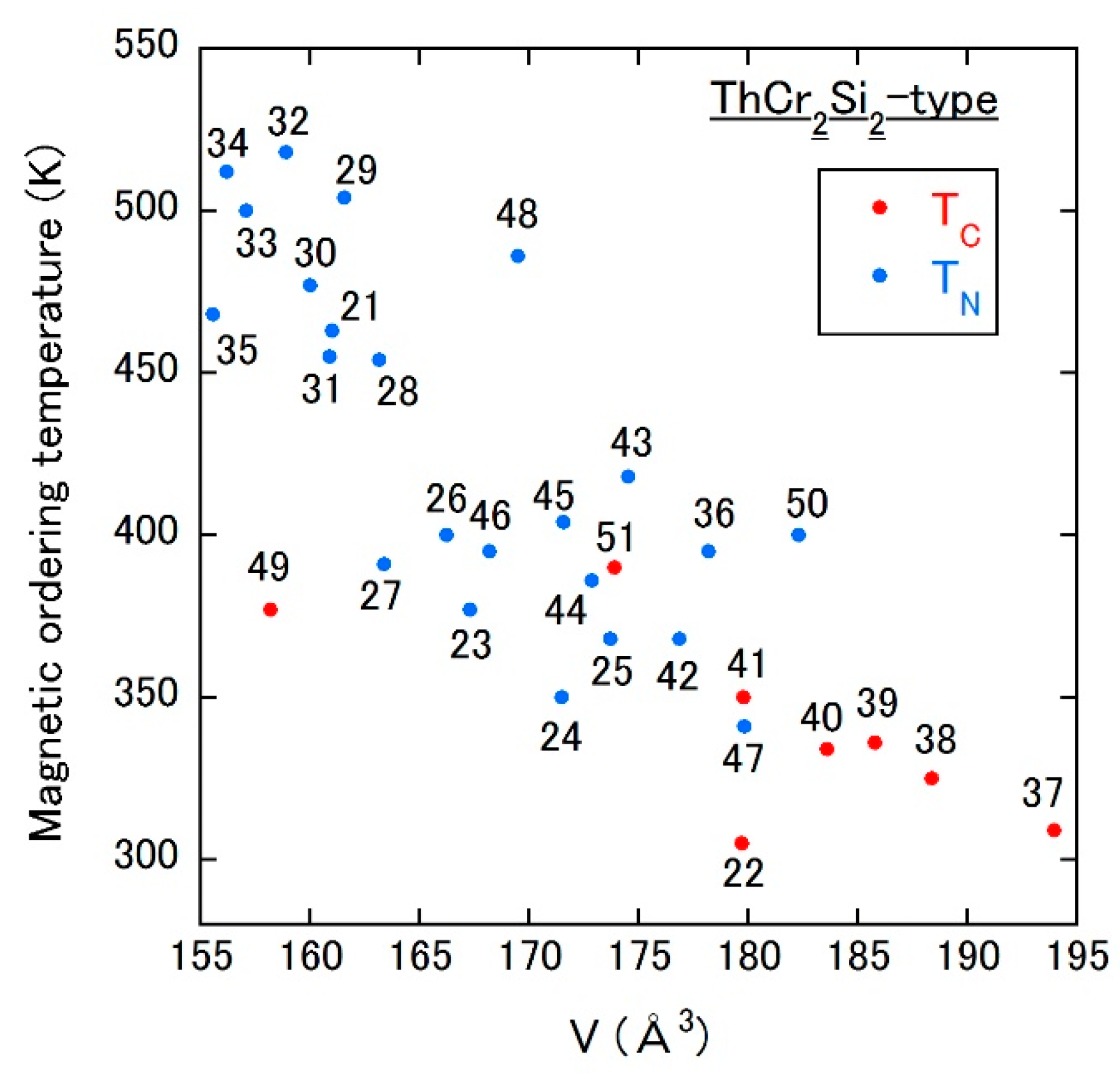
3.4. (R or Actinide)Mn2Si2 and Its Germanides
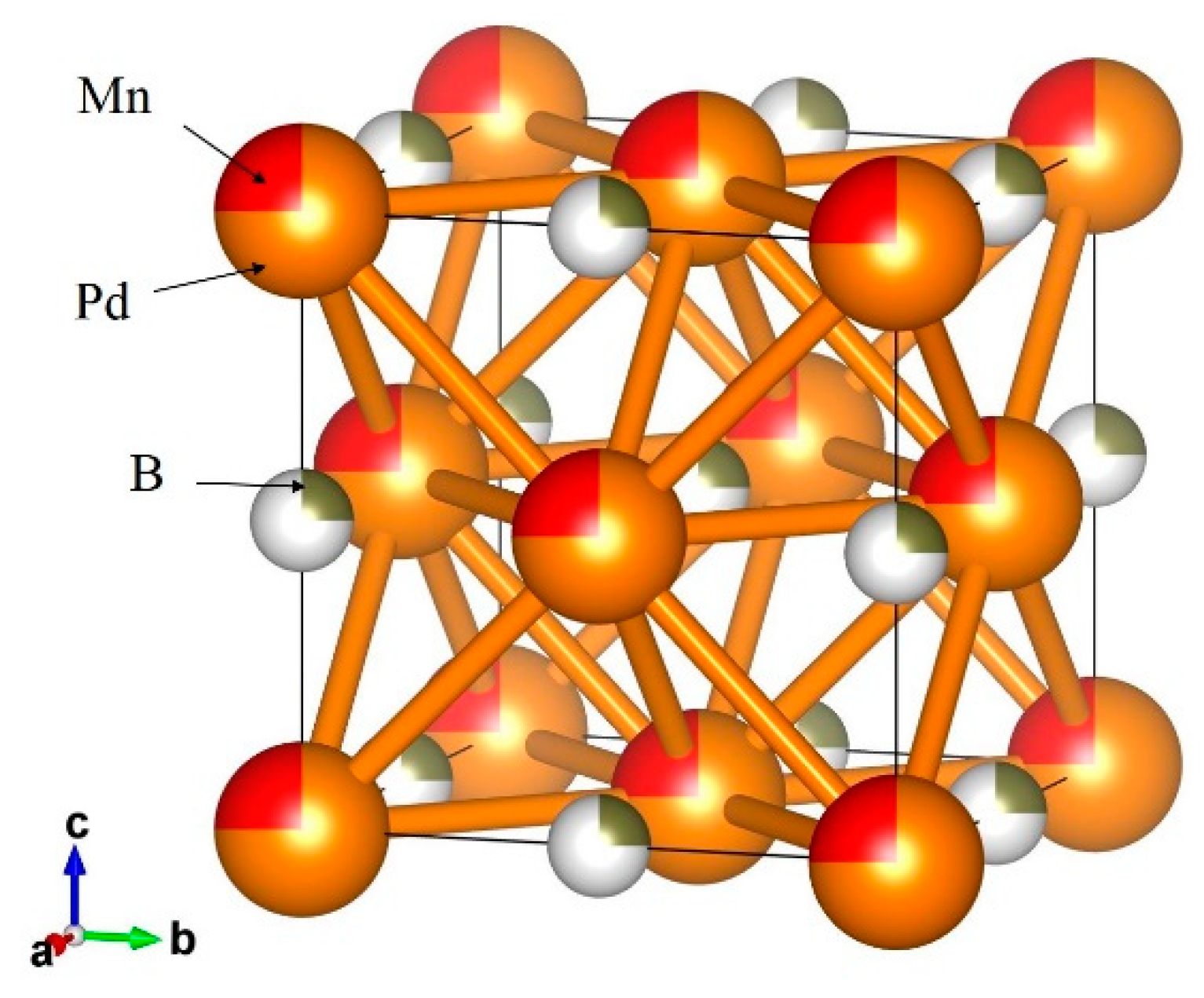
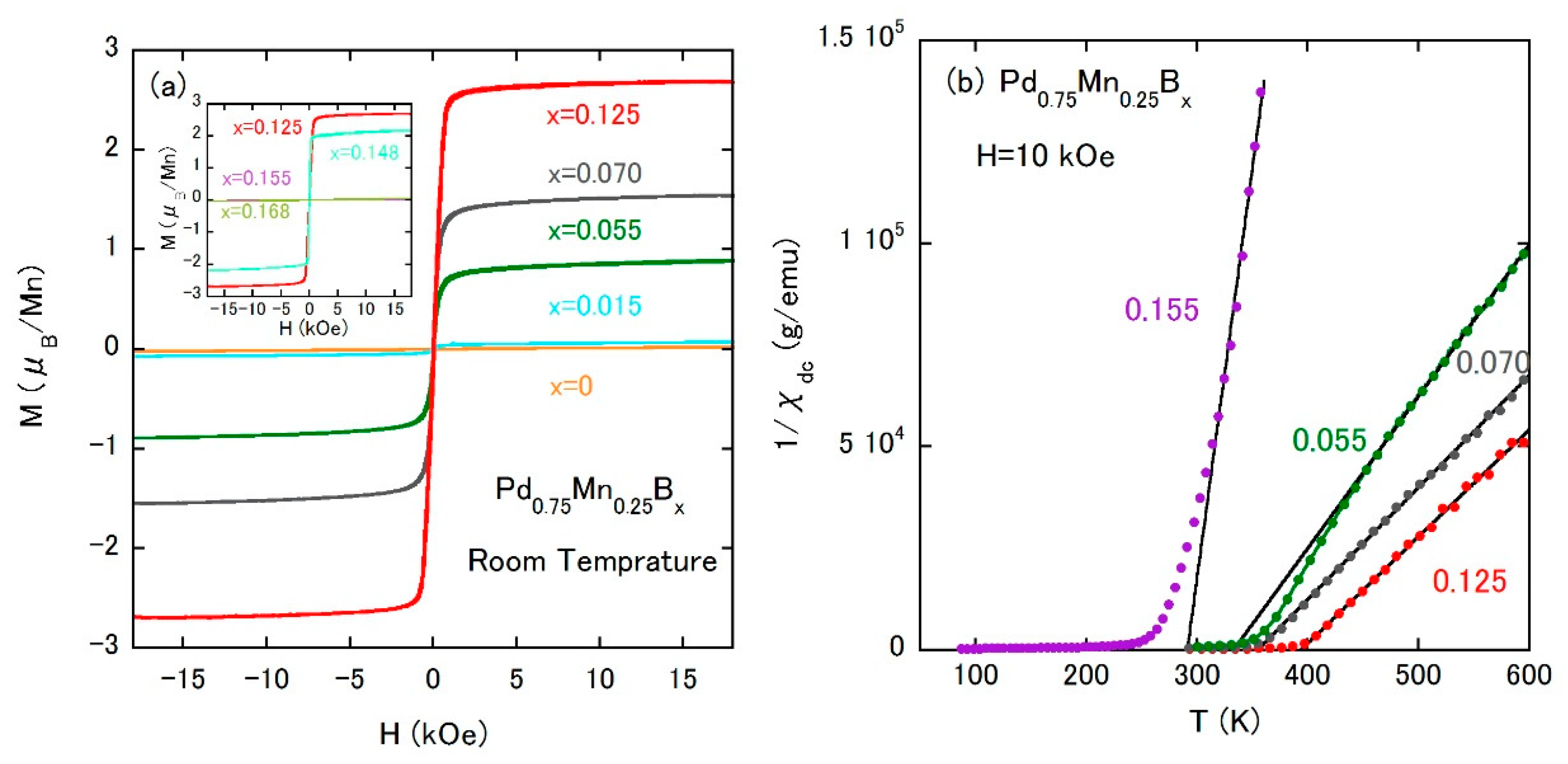
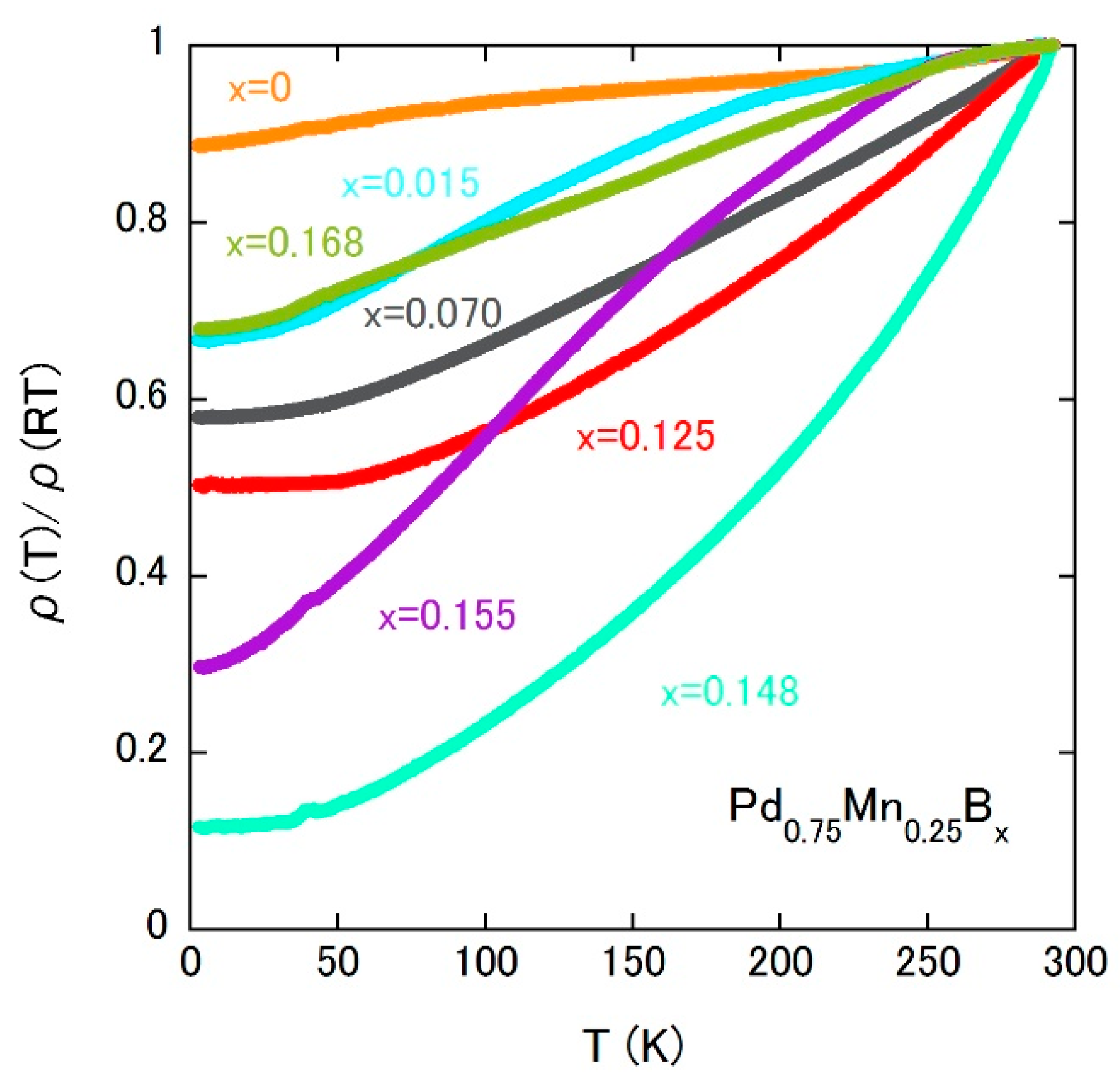
3.5. Boron-Added Pd0.75Mn0.25 Alloy
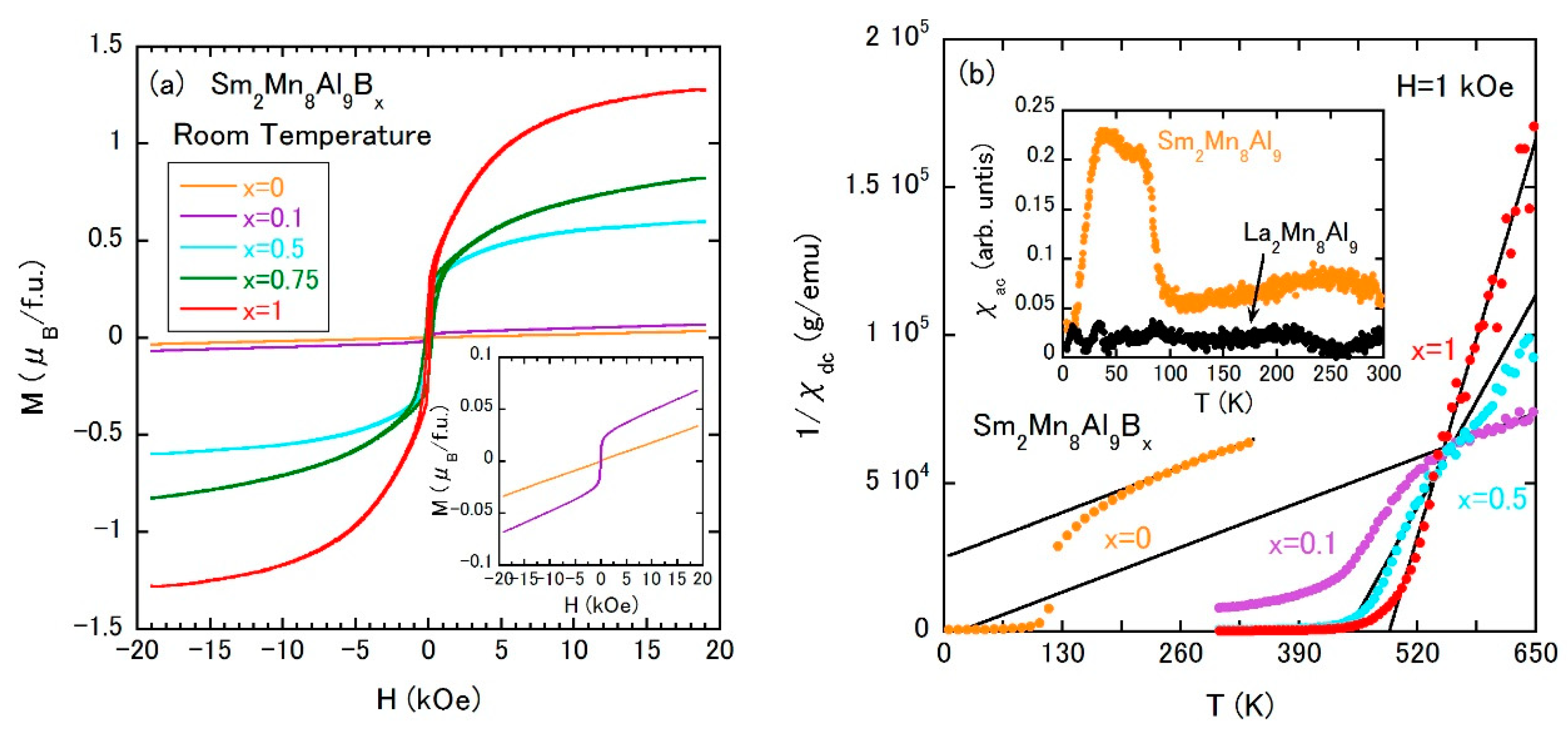
3.6. Boron-Added Sm2Mn8Al9
3.7. Brief Summary of Mn-Based Compounds
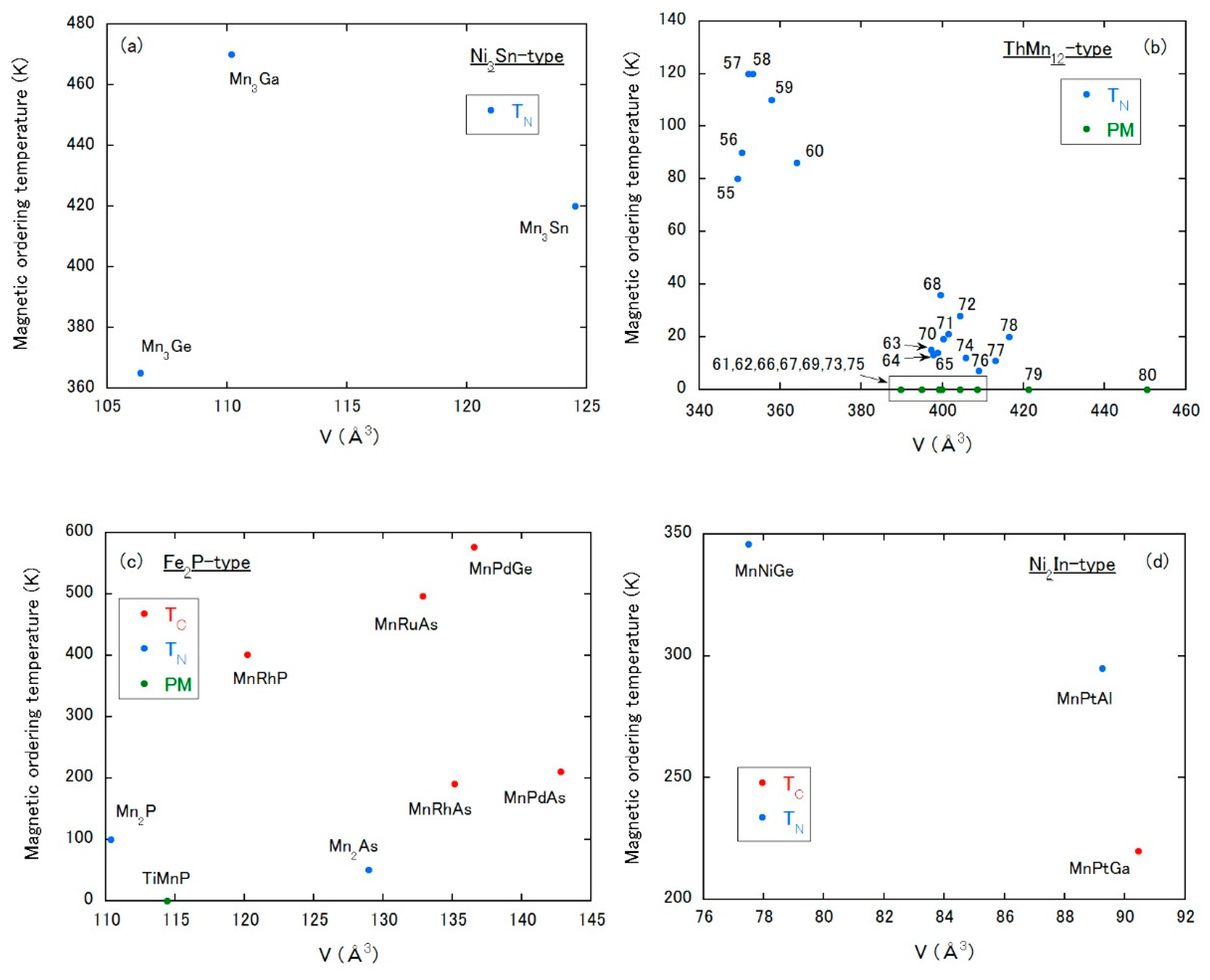
3.8. Candidate Showing a Change in Magnetism by Interstitial Atoms
3.8.1. Ni3Sn-Type Structure
3.8.2. ThMn12-Type Structure
3.8.3. Fe2P-Type Structure
3.8.4. Ni2In-Type Structure
3.8.5. LiAlSi-Type Structure
3.8.6. TiNiSi-Type Structure
3.8.7. AuCu-Type Structure
3.8.8. Cu2Sb-Type Structure
4. Rare-Earth-Based Compounds
4.1. R5Si3Bx
4.2. NdScSiCx
5. Perspectives
5.1. Application of Mn-Based Magnetic Materials
5.2. Towards Further on-Demand Control of Magnetism
5.3. Comments on Control of Magnetism by External Field
5.4. Comments on Critical Behavior
6. Summary
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Goldschmidt, H.J. Interstitial Alloys; Butterworths: London, UK, 1967. [Google Scholar]
- Grandjean, F.; Long, G.J.; Buschow, K.H.J. Interstitial Intermetallic Alloys; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1995. [Google Scholar]
- Yang, Y.; Yang, J.; Han, J.; Wang, C.; Liu, S.; Du, H. Researh and Development of Interstitial Compounds. IEEE Trans. Magn. 2015, 51, 2103806. [Google Scholar] [CrossRef]
- Nieves, P.; Arapan, S.; Maudes-Raedo, J.; Marticorena-Sánchez, R.; Del Brío, N.L.; Kovacs, A.; Echevarria-Bonet, C.; Salazar, D.; Weischenberg, J.; Zhang, H.; et al. Database of novel magnetic materials for high-performance permanent magnet development. Comput. Mater. Sci. 2019, 168, 188–202. [Google Scholar] [CrossRef] [Green Version]
- Coey, J.M.D. Perspective and Prospects for Rare Earth Permanent Magnets. Engineering 2020, 6, 119–131. [Google Scholar] [CrossRef]
- Hadjipanayis, G.C.; Gabay, A.M.; Schönhöbel, A.M.; Martín-Cid, A.; Barandiaran, J.M.; Niarchos, D. ThMn12-Type Alloys for Permanent Magnets. Engineering 2020, 6, 141–147. [Google Scholar] [CrossRef]
- Slater, J.C. Cohesion in Monovalent Metals. Phys. Rev. 1930, 35, 509–529. [Google Scholar] [CrossRef]
- Slater, J.C. Atomic Shielding Constants. Phys. Rev. 1930, 36, 57–64. [Google Scholar] [CrossRef]
- Sommerfeld, A.; Bethe, H. Handbuch der Physik; Springer: Berlin, Germany, 1933; Volume 24, p. 595. [Google Scholar]
- Cardias, R.; Szilva, A.; Bergman, A.; Marco, I.D.; Katsnelson, M.I.; Lichtenstein, A.I.; Nordström, L.; Klautau, A.B.; Eriksson, O.; Kvashnin, Y.O. The Bethe-Slater curve revisited; new insights from electronic structure theory. Sci. Rep. 2017, 7, 4058. [Google Scholar] [CrossRef] [Green Version]
- Givord, D.; Lemaire, R. Magnetic Transition and Anomalous Thermal Expansion in R2Fe17 Compounds. IEEE Trans. Magn. 1970, MAG-10, 109–113. [Google Scholar]
- Palstra, T.T.M.; Nieuwenhuys, G.J.; Mydosh, J.A.; Buschow, K.H.J. Mictomagnetic, ferromagnetic, and antiferromagnetic transitions in La(FexAl1−x)13 intermetallic compounds. Phys. Rev. B 1985, 31, 4622–4632. [Google Scholar] [CrossRef]
- Kitagawa, J.; Yakabe, G.; Nakayama, A.; Nishizaki, T.; Tsubota, M. Competition between ferromagnetic and antiferromagnetic states in Al8.5-xFe23Ge12.5+x (0 ≤ x ≤ 3). J. Solid State Chem. 2020, 284, 121188. [Google Scholar] [CrossRef]
- Chen, D.; Gondō, Y. Temperature Dependence of the Magneto-Optic Effect and Resonance Phenomena in Oriented MnBi Films. J. Appl. Phys. 1964, 35, 1024–1025. [Google Scholar] [CrossRef]
- Park, J.H.; Hong, Y.K.; Bae, S.; Lee, J.J.; Jalli, J.; Abo, G.S.; Neveu, N.; Kim, S.G.; Choi, C.J.; Lee, J.G. Saturation magnetization and crystalline anisotropy calculations for MnAl permanent magnet. J. Appl. Phys. 2010, 107, 09A731. [Google Scholar] [CrossRef]
- Gabay, A.M.; Hadjipanayis, G.C.; Cui, J. New anisotropic MnBi permanent magnets by field-annealing of compacted melt-spun alloys modified with Mg and Sb. J. Magn. Magn. Mater. 2020, 495, 165860. [Google Scholar] [CrossRef]
- Xiang, Z.; Xu, C.; Wang, T.; Song, Y.; Yang, H.; Lu, W. Enhanced magnetization and energy product in isotropic nanocrystalline Mn55Al45 alloys with boron doping. Intermetallics 2018, 101, 13–17. [Google Scholar] [CrossRef]
- Zhao, S.; Wu, Y.; Zhang, C.; Wang, J.; Fu, Z.; Zhang, R.; Jiang, C. Stabilization of τ-phase in carbon-doped MnAl magnetic alloys. J. Alloy. Compd. 2018, 755, 257–264. [Google Scholar] [CrossRef]
- Jonietz, F.; Mühlbauer, S.; Pfleiderer, C.; Neubauer, A.; Münzer, W.; Bauer, A.; Adams, T.; Georgii, R.; Böni, P.; Duine, R.A.; et al. Spin Transfer Torques in MnSi at Ultralow Current Densities. Science 2010, 330, 1648–1651. [Google Scholar] [CrossRef] [Green Version]
- Nakatsuji, S.; Kiyohara, N.; Higo, T. Large anomalous Hall effect in a non-collinear antiferromagnet at room temperature. Nature 2015, 527, 212–215. [Google Scholar] [CrossRef]
- Sun, H.; Coey, J.M.D.; Otani, Y.; Hurley, D.P.F. Magnetic properties of a new series of rare-earth iron nitrides: R2Fe17Ny (y approximately 2.6). J. Phys. Condens. Matter 1990, 2, 6465–6470. [Google Scholar] [CrossRef]
- Mukai, T.; Fujimoto, T. Kerr microscopy observation of nitrogenated Sm2Fe17 intermetallic compounds. J. Magn. Magn. Mater. 1992, 103, 165–173. [Google Scholar] [CrossRef]
- Coey, J.M.D. Novel Permanent Magnetic Materials. Phys. Scr. 1991, T39, 21–28. [Google Scholar] [CrossRef]
- Wei, Y.-N.; Sun, K.; Fen, Y.-B.; Zhang, J.-X.; Hu, B.-P.; Wang, Y.-Z.; Rao, X.-L.; Liu, G.-C. Structural and intrinsic magnetic properties of Sm2Fe17Ny (y = 2 − 8). J. Alloy. Compd. 1993, 194, 9–12. [Google Scholar] [CrossRef]
- Coey, J.M.D.; Hurley, D.P.F. New interstitial rare-earth iron intermetallics produced by gas phase reaction. J. Magn. Magn. Mater. 1992, 104–107, 1098–1101. [Google Scholar] [CrossRef]
- Christodoulou, C.N.; Takeshita, T. Interstitial carbonation of the Sm2Fe17 phase by reaction with hydrocarbons. J. Alloy. Compd. 1992, 190, 41–48. [Google Scholar] [CrossRef]
- Coey, J.M.D.; Otani, Y. Magnetic properties of R2Fe17N3-δ. J. Magn. Soc. Jpn. 1991, 15, 677–684. [Google Scholar]
- Li, H.-S.; Coey, J.M.D. Magnetic Properties of Ternary Rare-Earth Transition-Metal Compounds. In Handbook of Magnetic Materials VI; Buschow, K.H.J., Ed.; Elsevier: Amsterdam, The Netherlands, 1991. [Google Scholar]
- Coey, J.M.D.; Sun, H.; Hurley, D.P.F. Intrinsic magnetic properties of new rare-earth iron intermetallic series. J. Magn. Magn. Mater. 1991, 101, 310–316. [Google Scholar] [CrossRef]
- Harashima, Y.; Terakura, K.; Kino, H.; Ishibashi, S.; Miyake, T. First-Principles Study of Structural and Magnetic Properties of R(Fe, Ti)12 and R(Fe, Ti)12N (R = Nd, Sm, Y). JPS Conf. Proc. 2015, 5, 011021. [Google Scholar]
- Kong, L.-S.; Cao, L.; Shen, B.-G. Structures and magnetic properties of the nitrides (Nd1-xDyx)TiFe11Ny. J. Magn. Magn. Mater. 1993, 124, 301–304. [Google Scholar] [CrossRef]
- Hirayama, Y.; Miyake, T.; Hono, K. Potential and issues of permanent magnet candidate compound Nd(FeM)12N with ThMn12-type structure. Mater. Jpn. 2016, 55, 97–103. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.-C.; Zhang, X.-D.; Kong, L.-S.; Pan, Q. Magnetocrystalline anisotropies of RTiFe11Nx compounds. Appl. Phys. Lett. 1991, 58, 2042–2044. [Google Scholar] [CrossRef]
- Chang, H.; Zhang, X.; Yang, Y. A study on the effect of hydrogen in the compounds with BaCd11-type structure. Solid State Commun. 2001, 119, 403–407. [Google Scholar] [CrossRef]
- Miyake, T.; Terakura, K.; Harashima, Y.; Kino, H.; Ishibashi, S. First-Principles Study of Magnetocrystalline Anisotropy and Magnetization in NdFe12, NdFe11Ti, and NdFe11TiN. J. Phys. Soc. Jpn. 2014, 83, 043702. [Google Scholar] [CrossRef]
- Harashima, Y.; Terakura, K.; Kino, H.; Ishibashi, S.; Miyake, T. First-principles study on stability and magnetism of NdFe11M and NdFe11MN for M = Ti, V, Cr, Mn, Fe, Co, Ni, Cu, Zn. J. Appl. Phys. 2016, 120, 203904. [Google Scholar] [CrossRef] [Green Version]
- Fukazawa, T.; Akai, H.; Harashima, Y.; Miyake, T. First-principles study of intersite magnetic couplings in NdFe12 and NdFe12X (X = B, C, N, O, F). J. Appl. Phys. 2017, 122, 053901. [Google Scholar] [CrossRef] [Green Version]
- Sato, T.; Ohsuna, T.; Yano, M.; Kato, A.; Kaneko, Y. Permanent magnetic properties of NdFe12Nx sputtered films epitaxially grown on V buffer layer. J. Appl. Phys. 2017, 122, 053903. [Google Scholar] [CrossRef]
- Sepehri-Amina, H.; Tamazawa, Y.; Kambayashi, M.; Saito, G.; Takahashi, Y.K.; Ogawa, D.; Ohkubo, T.; Hirosawa, S.; Doi, M.; Shima, T.; et al. Achievement of high coercivity in Sm(Fe0.8Co0.2)12 anisotropic magnetic thin film by boron doping. Acta Mater. 2020, 194, 337–342. [Google Scholar] [CrossRef]
- Mao, W.; Zhang, X.; Ji, C.; Chang, H.; Cheng, B.; Yang, Y.; Du, H.; Xue, Y.; Zhang, B.; Wang, L.; et al. Structural and magnetic properties of PrFe12−xVx and their nitrides. Acta Mater. 2001, 49, 721–728. [Google Scholar] [CrossRef]
- Buschow, K.H.J.; Sherwood, R.C. Magnetic properties and hydrogen absorption in rare-earth intermetallics of the type RMn2 and R6Mn23. J. Appl. Phys. 1977, 48, 4643–4648. [Google Scholar] [CrossRef]
- Shiga, M. Magnetism and spin fluctuations of laves phase manganese compounds. Phys. B + C 1988, 149, 293–305. [Google Scholar] [CrossRef]
- Corbett, J.D.; Garcia, E.; Guloy, A.M.; Hurng, W.-M.; Kwon, Y.-U.; Leon-Escamilla, E.A. Widespread Interstitial Chemistry of Mn5Si3-Type and Related Phases. Hidden Impurities and Opportunities. Chem. Mater. 1998, 10, 2824–2836. [Google Scholar] [CrossRef]
- Gajdzik, M.; Sürgers, C.; Kelemen, M.; Löhneysen, H.v. Ferromagnetism in carbon-doped Mn5Si3 films. J. Appl. Phys. 2000, 87, 6013–6015. [Google Scholar] [CrossRef]
- Sürgers, C.; Gajdzik, M.; Fischer, G.; Löhneysen, H.V.; Welter, E.; Attenkofer, K. Preparation and structural characterization of ferromagnetic Mn5Si3Cx films. Phys. Rev. B 2003, 68, 174423. [Google Scholar] [CrossRef]
- Saha, D.K.; Ohshima, K.; Wey, M.Y.; Miida, R.; Kimoto, T. Structure and magnetism of fcc Pd-Mn alloys. Phys. Rev. B 1994, 49, 15715–15722. [Google Scholar] [CrossRef] [PubMed]
- Rashid, M.H.; Sellmyer, D.J. Spin-glass-like freezing in disordered MnPd3 and CrPd3 alloys. J. Appl. Phys. 1984, 55, 1735–1737. [Google Scholar] [CrossRef] [Green Version]
- Sakamoto, Y.; Takao, K.; Nagaoka, Y.; Kida, H.; Flanagan, T.B. Investigations of ordered structures in boron-containing Pd3Mn alloys. J. Alloy. Compd. 1993, 197, 35–41. [Google Scholar] [CrossRef]
- Kitagawa, J.; Sakaguchi, K. New room-temperature ferromagnet: B-added Pd0.75Mn0.25 alloy. J. Magn. Magn. Mater. 2018, 468, 115–122. [Google Scholar] [CrossRef] [Green Version]
- Worsham, J.E., Jr.; Wilkinson, M.K.; Shull, C.G. Neutron-diffraction observations on the palladium-hydrogen and palladium-deuterium systems. J. Phys. Chem. Solids 1957, 3, 303–310. [Google Scholar] [CrossRef]
- Kitagawa, J.; Hara, T.; Sakaguchi, K.; Shirakawa, N.; Tsubota, M. Emergence of room-temperature ferromagnetism in boron-added Mn compounds. In Proceedings of the Joint European Magnetic Symposia 2019, Uppsala, Sweden, 26–30 August 2019; p. 158. [Google Scholar]
- Izumi, F.; Momma, K. Three-dimensional visualization in powder diffraction. Solid State Phenom. 2007, 130, 15–20. [Google Scholar] [CrossRef]
- Kotur, B.Y.; Palasyuk, A.M.; Bauer, E.; Michor, H.; Hilscher, G. Uncommon conductivity of R–Mn–Al (R = Gd, Tb) ternary compounds. J. Phys. Condens. Matter 2001, 13, 9421–9431. [Google Scholar] [CrossRef]
- Graf, T.; Felser, C.; Parkin, S.S.P. Simple rules for the understanding of Heusler compounds. Prog. Solid State Chem. 2011, 39, 1–50. [Google Scholar] [CrossRef]
- Sen, S.; Singh, C.; Mukharjee, P.K.; Nath, R.; Nayak, A.K. Observation of the topological Hall effect and signature of room-temperature antiskyrmions in Mn-Ni-Ga D2d Heusler magnets. Phys. Rev. B 2019, 99, 134404. [Google Scholar] [CrossRef] [Green Version]
- Devi, P.; Zavareh, M.G.; Mejía, C.S.; Hofmann, K.; Albert, B.; Felser, C.; Nicklas, M.; Singh, S. Reversible adiabatic temperature change in the shape memory Heusler alloy Ni2.2Mn0.8Ga: An effect of structural compatibility. Phys. Rev. Mater. 2018, 2, 122401. [Google Scholar] [CrossRef] [Green Version]
- Lemoine, P.; Vernière, A.; Mazet, T.; Malaman, B. Magnetic and magnetocaloric properties of R6Mn23 (R = Y, Nd, Sm, Gd-Tm, Lu) compounds. J. Magn. Magn. Mater. 2011, 323, 2690–2695. [Google Scholar] [CrossRef]
- Tsvyashchenko, A.V.; Popova, S.V.; Makhotkin, V.E.; Fradkov, V.A.; Zaritskii, V.N. Investigations of the magnetic properties of the Yb6Mn23 intermetallic compound. J. Less-Common Met. 1984, 96, 99–102. [Google Scholar] [CrossRef]
- Malik, S.K.; Takeshita, T.; Wallace, W.E. Hydrogen induced magnetic ordering in Th6Mn23. Solid State Commun. 1977, 23, 599–602. [Google Scholar] [CrossRef]
- Bushow, K.H.J. Magnetic properties of the ternary hydrides of Nd6Mn23 and Sm6Mn23. Solid State Commun. 1981, 40, 207–210. [Google Scholar] [CrossRef]
- Hardman-Rhyne, K.; Rhyne, J.J.; Prince, E.; Crowder, C.; James, W.J. Magnetic and crystallographic structure of Y6Mn23D23. Phys. Rev. B 1984, 29, 416–422. [Google Scholar] [CrossRef]
- Hardman-Rhyne, K.; Kevin Smith, H.; Wallace, W.E. Magnetic and crystallographic structure of Th6Mn23Dx. J. Less-Common Met. 1984, 96, 201–211. [Google Scholar] [CrossRef]
- Pourarian, F.; Boltich, E.B.; Wallace, W.E.; Craig, R.S.; Malik, S.K. Magnetic characteristics of R6Mn23 hydrides (R = Gd, Ho or Er). J. Magn. Magn. Mater. 1980, 21, 128–132. [Google Scholar] [CrossRef]
- Pourarian, F.; Boltich, E.B.; Wallace, W.E.; Malik, S.K. Effect of absorbed hydrogen on magnetic ordering in Tb6Mn23 and Dy6Mn23. J. Less-Common Met. 1980, 74, 153–159. [Google Scholar] [CrossRef]
- Szytuła, A.; Szott, I. Magnetic properties of ternary RMn2Si2 and RMn2Ge2 compounds. Solid State Commun. 1981, 40, 199–202. [Google Scholar] [CrossRef]
- Hofmann, M.; Campbell, S.J.; Edge, A.V.J. EuMn2Ge2 and EuMn2Si2: Magnetic structures and valence transitions. Phys. Rev. B 2004, 69, 174432. [Google Scholar] [CrossRef]
- Szytuka, A.; Siek, S.; Leciejewicz, J.; Zygmunt, A.; Ban, Z. Neutron diffraction study of UT2X2 (T: Mn, Fe, X: Si, Ge) intermetallic systems. J. Phys. Chem. Solids 1988, 49, 1113–1118. [Google Scholar] [CrossRef]
- Fukamichi, K. Antiferromagnetic Materials; Kyoritsu: Tokyo, Japan, 2014. [Google Scholar]
- Liu, Z.H.; Zhang, Y.J.; Liu, G.D.; Ding, B.; Liu, E.K.; Jafri, H.M.; Hou, Z.P.; Wang, W.H.; Ma, X.Q.; Wu, G.H. Transition from Anomalous Hall Effect to Topological Hall Effect in Hexagonal Non-Collinear Magnet Mn3Ga. Sci. Rep. 2017, 7, 515. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Morales, M.; Artigas, M.; Bacmann, M.; Fruchart, D.; Skolozdra, R.; Soubeyroux, J.L.; Wolfers, P. Comparison of the magnetic properties of ErMn12−xFex series with their related hydrides and carbides. J. Magn. Magn. Mater. 1999, 196, 703–704. [Google Scholar] [CrossRef]
- Stankiewicz, J.; Bartolomé, J.; Fruchart, D. Spin Disorder Scattering in Magnetic Metallic Alloys. Phys. Rev. Lett. 2002, 89, 106602. [Google Scholar] [CrossRef]
- Yang, J.B.; Yelon, W.B.; James, W.J.; Cai, S.; Eckert, D.; Handstein, A.; Müller, K.H.; Yang, Y.C. Structural and magnetic properties of R(FexMn1−x)12 (R = Ho, Y). Phys. Rev. B 2002, 65, 064444. [Google Scholar] [CrossRef]
- Piqué, C.; Blanco, J.A.; Burriel, R.; Abad, E.; Artigas, M.; Fernández-Díaz, M.T. Influence of 3d−4f interactions in the magnetic phases of RFexMn12−x (R=Gd, Tb, and Dy) compounds: Coexistence of ferromagnetism and antiferromagnetism at different crystallographic sites. Phys. Rev. B 2007, 75, 224424. [Google Scholar] [CrossRef]
- Kirchmayr, H. Magnetic properties of rare earth--Manganese compounds. IEEE Trans. Magn. 1966, 2, 493–499. [Google Scholar] [CrossRef]
- Muro, Y.; Nakamura, H.; Kohara, T. Magnetism of ScMn4Al8. In Proceedings of the Physical Society of Japan Autumn Meeting 2005, Kyotanabe, Japan, 19–22 September 2005. [Google Scholar]
- Felner, I.; Nowik, I. Magnetism and hyperfine interactions of 57Fe, 151Eu, 155Gd, 161Dy, 166Er and 170Yb in RM4Al8 compounds (R = rare earth or Y, M = Cr, Mn, Fe, Cu). J. Phys. Chem. Solids 1979, 40, 1035–1044. [Google Scholar] [CrossRef]
- Bourée-Vigneron, F.; Pinot, M.; Olès, A.; Baran, A.; Suski, W. UCr4Al8 and UMn4Al8: Neutron diffraction study. Solid State Commun. 1990, 75, 929–933. [Google Scholar] [CrossRef]
- Duong, N.P.; Klaasse, J.C.P.; Brück, E.; de Boer, F.R.; Buschow, K.H.J. Magnetic properties of GdT4Al8 and GdT6Al6 compounds (T = Cr, Mn, Cu). J. Alloy. Compd. 2001, 315, 28–35. [Google Scholar] [CrossRef]
- Schobinger-Papamantellos, P.; Fischer, P.; de Groot, C.H.; de Boer, F.R.; Buschow, K.H.J. Magnetic properties and neutron diffraction of TbMn4Al8. J. Alloy. Compd. 1996, 232, 154–159. [Google Scholar] [CrossRef] [Green Version]
- Baran, A.; Suski, W.; Mydlarz, T. Magnetic properties of the (U, Th) (Cr, Mn)4Al8 compounds. J. Magn. Magn. Mater. 1987, 63, 196–198. [Google Scholar] [CrossRef]
- Fruchart, R.; Roger, A.; Senateur, J.P. Crystallographic and Magnetic Properties of Solid Solutions of the Phosphides M2P, M = Cr, Mn, Fe, Co, and Ni. J. Appl. Phys. 1969, 40, 1250–1257. [Google Scholar] [CrossRef]
- Srivastava, B.K.; Ericsson, T.; Haggstrom, L.; Verma, H.R.; Andersson, Y.; Rundqvist, S. A Mossbauer study of the (Fe1-xMnx)2P system. J. Phys. C 1987, 20, 463–472. [Google Scholar] [CrossRef]
- Suzuki, N.; Asahi, R.; Kishida, Y.; Masuoka, Y.; Sugiyama, J. Measurement and ab initio calculation of the structural parameters and physical properties of 3d transition intermetallics TiMP (M = Cr, Mn, Fe, Co, or Ni). Mater. Res. Express 2017, 4, 046505. [Google Scholar] [CrossRef]
- Kanomata, T.; Kawashima, T.; Utsugi, H.; Goto, T.; Hasegawa, H.; Kaneko, T. Magnetic properties of the intermetallic compounds MM’X (M = Cr, Mn, M’ = Ru, Rh, Pd, and X = P, As). J. Appl. Phys. 1991, 69, 4639–4641. [Google Scholar] [CrossRef]
- Jeitschko, W.; Johnson, V. High pressure Mn2As with Fe2P-type structure. Acta Crystallogr. Sect. B 1972, 28, 1971–1973. [Google Scholar] [CrossRef]
- Bartolomé, J.; García, J.; Floría, L.M.; Falo, F.; Navarro, R.; Fruchart, D.; Bacmann, M.; de Jongh, L.J. A magnetic Kosterlitz-Thouless transition in quasi 2-d MnRhAs? J. Magn. Magn. Mater. 1986, 54–57, 1547–1548. [Google Scholar] [CrossRef]
- Bażela, W. Magnetic properties of intermetallic compounds RhMnGe, PdMnGe, Pd1.5Mn0.5Si, Pd1.5Mn0.5Ge and RhMnSi. J. Less-Common Met. 1984, 100, 341–346. [Google Scholar] [CrossRef]
- Bazela, W.; Szytuła, A.; Todorović, J.; Tomkowicz, Z.; Zięba, A. Crystal and magnetic structure of NiMnGe. Phys. Status Solidi A 1976, 38, 721–729. [Google Scholar] [CrossRef]
- Brück, E.; van Streepen, T.; Duijn, H.G.M.; Haanappel, E.G.; de Boer, F.R.; Buschow, K.H. J Magnetic and transport properties of MnPtAl1-xGax compounds. J. Magn. Magn. Mater. 1988, 177–181, 767–768. [Google Scholar] [CrossRef]
- Buschow, K.H.J.; De Mooij, D.B. Crystal structure and magnetic properties of PtMnGa and PtMnAl. J. Less-Common Met. 1984, 99, 125–130. [Google Scholar] [CrossRef]
- Kübler, J. Ab initio estimates of the Curie temperature for magnetic compounds. J. Phys. Condens. Matter 2006, 18, 9795–9808. [Google Scholar] [CrossRef]
- Krishnamurthy, V.V.; Kawamura, N.; Suzuki, M.; Ishikawa, T.; Mankey, G.J.; Raj, P.; Sathyamoorthy, A.; Joshi, A.G.; Malik, S.K. Evidence for a magnetic moment on Ir in IrMnAl from x-ray magnetic circular dichroism. Phys. Rev. B 2003, 68, 214413. [Google Scholar] [CrossRef] [Green Version]
- Buschow, K.H.J.; van Engen, P.G.; Jongebreur, R. Magneto-optical properties of metallic ferromagnetic materials. J. Magn. Magn. Mater. 1983, 38, 1–22. [Google Scholar] [CrossRef]
- Masumoto, H.; Watanabe, K. New Compounds of the Clb, Cl Types of RhMnSb, IrMnSn and IrMnAl, New L21 (Heusler) Type of Ir2MnAl and Rh2MnAl Alloys, and Magnetic Properties. J. Phys. Soc. Jpn. 1972, 32, 281. [Google Scholar] [CrossRef]
- Chikazumi, S. Physics of Ferromagnetism; Oxford University Press: New York, NY, USA, 1997. [Google Scholar]
- Helmholdt, R.B.; Buschow, K.H.J. A neutron diffraction and magnetization study of PdMnTe. J. Less-Common Met. 1986, 123, 169–173. [Google Scholar] [CrossRef]
- Offernes, L.; Ravindran, P.; Kjekshus, A. Prediction of large polar Kerr rotation in the Heusler-related alloys AuMnSb and AuMnSn. Appl. Phys. Lett. 2003, 82, 2862–2864. [Google Scholar] [CrossRef] [Green Version]
- Beckman, O.; Lundgren, L. Compounds of transition elements with nonmetals. In Handbook of Magnetic Materials; Buschow, K.H.J., Ed.; Elsevier: New York, NY, USA, 1991; Volume 6, pp. 181–287. [Google Scholar]
- Lamichhane, T.N.; Taufour, V.; Masters, M.W.; Parker, D.S.; Kaluarachchi, U.S.; Thimmaiah, S.; Bud’ko, S.L.; Canfield, P.C. Discovery of ferromagnetism with large magnetic anisotropy in ZrMnP and HfMnP. Appl. Phys. Lett. 2016, 109, 092402. [Google Scholar] [CrossRef] [Green Version]
- Ventutini, G.; ljjaali, I.; Ressouche, E.; Malaman, B. Neutron diffraction study of the HoMnSi, LuMnSi and Sc0.9Lu0.1MnSi compounds. J. Alloy. Compd. 1997, 256, 65–75. [Google Scholar] [CrossRef]
- Hoffmann, R.-D.; Pöttgen, R.; Chevalier, B.; Gaudin, E.; Matar, S.F. The ternary germanides UMnGe and U2Mn3Ge. Solid State Sci. 2013, 21, 73–80. [Google Scholar] [CrossRef]
- Venturini, G.; Malaman, B.; Ressouche, E. Neutron diffraction study of the TbMnGe compound. J. Alloy. Compd. 1996, 243, 98–105. [Google Scholar] [CrossRef]
- Klosek, V.; Vernière, A.; Ouladdiaf, B.; Malaman, B. Crystal and magnetic structures of the R(=Y, Dy–Tm)MnGe compounds. J. Magn. Magn. Mater. 2003, 256, 69–92. [Google Scholar] [CrossRef]
- Umetsu, R.Y.; Fukamichi, K.; Sakuma, A. Electrical and magnetic properties of antiferromagnetic L10-type Mn alloys. Mater. Jpn. 2004, 43, 831–839. [Google Scholar] [CrossRef]
- Cui, B.Z.; Marinescu, M.; Liu, J.F. Ferromagnetic Tetragonal L10-Type MnGa Isotropic Nanocrystalline Microparticles. IEEE Trans. Magn. 2013, 49, 3322–3325. [Google Scholar] [CrossRef]
- Sakuma, A. Electronic Structure and Magnetocrystalline Anisotropy Energy of MnAl. J. Phys. Soc. Jpn. 1994, 63, 1422–1428. [Google Scholar] [CrossRef]
- Suits, J.C. New magnetic compounds with Heusler and Heusler-related structures. Phys. Rev. B 1976, 14, 4131–4135. [Google Scholar] [CrossRef]
- Umetsu, R.Y.; Mitsui, Y.; Yuito, I.; Takeuchi, T.; Kawarada, H. Substitution Effects of Cr or Fe on the Curie Temperature for Mn-Based Layered Compounds MnAlGe and MnGaGe With Cu2Sb-Type Structure. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Shibata, K.; Shinohara, T.; Watanabe, H. Magnetic Properties of A New Ferromagnetic Compound MnGaGe. J. Phys. Soc. Jpn. 1972, 32, 1431. [Google Scholar] [CrossRef]
- Matsuzaki, H.; Endo, S.; Notsu, Y.; Ono, F.; Kanomata, T.; Kaneko, T. Pressure Effect on Curie Temperature in MnZnSb. Jpn. J. Appl. Phys. 1993, 32, 271–272. [Google Scholar] [CrossRef] [Green Version]
- Beleanu, A.; Kiss, J.; Kreiner, G.; Köhler, C.; Müchler, L.; Schnelle, W.; Burkhardt, U.; Chadov, S.; Medvediev, S.; Ebke, D.; et al. Large resistivity change and phase transition in the antiferromagnetic semiconductors LiMnAs and LaOMnAs. Phys. Rev. B 2013, 88, 184429. [Google Scholar] [CrossRef] [Green Version]
- Kido, H.; Hoshikawa, T.; Shimada, M.; Koizumi, M. Preparation and magnetic properties of YMnSi. Phys. Status Solidi A 1985, 88, K39–K43. [Google Scholar] [CrossRef]
- Ovtchenkova, I.A.; Nikitin, S.A.; Ivanova, T.I.; Tskhadadze, G.A.; Chistyakov, O.D.; Badurski, D. Magnetocaloric effect and magnetoresistance in GdxLa1−xMnSi compounds. J. Magn. Magn. Mater. 2006, 300, e493–e496. [Google Scholar] [CrossRef]
- Welter, R.; Venturini, G.; Ressouche, E.; Malaman, B. Magnetic properties of TbMnSi, determined by susceptibility measurements and neutron diffraction study. J. Alloy. Compd. 1994, 210, 273–277. [Google Scholar] [CrossRef]
- Welter, R.; Venturini, G.; Ressouche, E.; Malaman, B. Neutron diffraction studies of the CeFeSi-type CaMnSi and CaMnGe compounds. Solid State Commun. 1996, 97, 503–507. [Google Scholar] [CrossRef]
- Welter, R.; Venturini, G.; Ressouche, E.; Malaman, B. Crystallographic data and magnetic properties of new CeFeSi-type RMnGe compounds (R = La Sm) studied by magnetization and neutron diffraction measurements. J. Alloy. Compd. 1995, 228, 59–74. [Google Scholar] [CrossRef]
- Dascoulidou, A.; Müller, P.; Bronger, W. Ternäre Mangan-Verbindungen AMnX (A ≙ Mg, Ca, Sr oder Ba; X ≙ Si, Ge oder Sn): Neutronenbeugungsuntersuchungen zur Charakterisierung der magnetischen Eigenschaften. Z. Anorg. Allg. Chem. 1998, 624, 124–128. [Google Scholar] [CrossRef]
- Roger, J.; Yahia, M.B.; Babizhetskyy, V.; Bauer, J.; Cordier, S.; Guérin, R.; Hiebl, K.; Rocquefelte, X.; Saillard, J.-Y.; Halet, J.-F. Mn5Si3-type host-interstitial boron rare-earth metal silicide compounds RE5Si3: Crystal structures, physical properties and theoretical considerations. J. Solid State Chem. 2006, 179, 2310–2328. [Google Scholar] [CrossRef]
- Mahon, T.; Gaudin, E.; Villesuzanne, A.; Chevalier, B.; Tencé, S. Effect of Carbon Insertion on the Structural and Magnetic Properties of NdScSi. Inorg. Chem. 2019, 58, 15255–15268. [Google Scholar] [CrossRef]
- Coey, J.M.D. New permanent magnets; manganese compounds. J. Phys. Condens. Matter 2014, 26, 064211. [Google Scholar] [CrossRef] [PubMed]
- Tanaka, S.; Terada, H.; Shirakawa, N.; Tsubota, M.; Kitagawa, J. The Impact of the Composition Effect on Ferromagnetic Properties of Tb2Co2Ga. Metals 2019, 9, 1242. [Google Scholar] [CrossRef] [Green Version]
- Kitagawa, J.; Takeda, N.; Sakai, F.; Ishikawa, M. Effect of composition in (RE)3Pd20Ge6 (RE = La, Ce and Nd). J. Phys. Soc. Jpn. 1999, 68, 3413–3416. [Google Scholar] [CrossRef]
- Kitagawa, J.; Terada, H.; Shirakawa, N.; Tsubota, M.; Nose, A.; Tanaka, S. Composition effect in ferromagnetic properties of Tb3Co3Ga. Results Phys. 2019, 15, 102591. [Google Scholar] [CrossRef]
- Lu, Q.M.; Yue, M.; Zhang, H.G.; Wang, M.L.; Yu, F.; Huang, Q.Z.; Ryan, D.H.; Altounian, Z. Intrinsic magnetic properties of single-phase Mn1+xGa (0 < x < 1) alloys. Sci. Rep. 2015, 5, 17086. [Google Scholar]
- Enkovaara, J.; Heczko, O.; Ayuela, A.; Nieminen, R.M. Coexistence of ferromagnetic and antiferromagnetic order in Mn-doped Ni2MnGa. Phys. Rev. B 2003, 67, 212405. [Google Scholar] [CrossRef] [Green Version]
- Larson, P.; Mazin, I.I.; Papaconstantopoulos, D.A. Effects of doping on the magnetic anisotropy energy in SmCo5-xFex and YCo5-xFex. Phys. Rev. B 2004, 69, 134408. [Google Scholar] [CrossRef]
- Sakuma, A.; Manabe, Y.; Kota, Y. First principles calculation of magnetocrystalline anisotropy energy of MnBi and MnBi1-xSnx. J. Phys. Soc. Jpn. 2013, 82, 073704. [Google Scholar] [CrossRef]
- Pandey, T.; Parker, D.S. Magnetic properties and magnetocrystalline anisotropy of Nd2Fe17, Nd2Fe17X3, and related compounds. Sci. Rep. 2018, 8, 3601. [Google Scholar] [CrossRef]
- Miyahara, J.; Shirakawa, N.; Setoguchi, Y.; Tsubota, M.; Kuroiwa, K.; Kitagawa, J. Hill plot focusing on Ce compounds with high magnetic ordering temperatures and consequent study of Ce2AuP3. J. Supercond. Nov. Magn. 2018, 31, 3559–3564. [Google Scholar] [CrossRef] [Green Version]
- Yamada, Y.; Ueno, K.; Fukumura, T.; Yuan, H.T.; Shimotani, H.; Iwasa, Y.; Gu, L.; Tsukimoto, S.; Ikuhara, Y.; Kawasaki, M. Electrically Induced Ferromagnetism at Room Temperature in Cobalt-Doped Titanium Dioxide. Science 2011, 332, 1065–1067. [Google Scholar] [CrossRef] [PubMed]
- Kitagawa, J.; Kitajima, D.; Shimokawa, K.; Takaki, H. Photoinduced Kondo effect in CeZn3P3. Phys. Rev. B 2016, 93, 035122. [Google Scholar] [CrossRef] [Green Version]
- Stewart, G.R. Non-Fermi-liquid behavior in d- and f-electron metals. Rev. Mod. Phys. 2001, 73, 797–855. [Google Scholar] [CrossRef]
- Eom, D.; Ishikawa, M.; Kitagawa, J.; Takeda, N. Suppression of Antiferromagnetism by Kondo Effect and Quantum Critical Behavior in CeCoGe 3-xSix (0 ≤ x ≤ 3). J. Phys. Soc. Jpn. 1998, 67, 2495–2500. [Google Scholar] [CrossRef]
- Custers, J.; Gegenwart, P.; Wilhelm, H.; Neumaier, K.; Tokiwa, Y.; Trovarelli, O.; Geibel, C.; Steglich, F.; Pépin, C.; Coleman, P. The break-up of heavy electrons at a quantum critical point. Nature 2003, 424, 524–527. [Google Scholar] [CrossRef] [Green Version]
- Löhneysen, H.V.; Rosch, A.; Vojta, M.; Wölfle, P. Fermi-liquid instabilities at magnetic quantum phase transitions. Rev. Mod. Phys. 2007, 79, 1015–1075. [Google Scholar] [CrossRef] [Green Version]
- Huang, C.-L.; Hallas, A.M.; Grube, K.; Kuntz, S.; Spieß, B.; Bayliff, K.; Besara, T.; Siegrist, T.; Cai, Y.; Beare, J.; et al. Quantum Critical Point in the Itinerant Ferromagnet Ni1−xRhx. Phys. Rev. Lett. 2020, 124, 117203. [Google Scholar] [CrossRef] [Green Version]
- Akayama, M.; Fujii, H.; Yamamoto, K.; Tatami, K. Physical properties of nitrogenated RFe11Ti intermetallic compounds (R = Ce, Pr and Nd) with ThMn12-type structure. J. Magn. Magn. Mater. 1994, 130, 99–107. [Google Scholar] [CrossRef]






| Compound | TC (K) | MS @RT (T) | Easy Magnetization Direction | a (Å) | c (Å) | V (Å3) | Ref. |
|---|---|---|---|---|---|---|---|
| Sm2Fe17 | 389 | 1.00 | in-plane | 8.55 | 12.45 | 788.2 | [21,22] |
| Sm2Fe17N3 | 749 | 1.54 | c axis | 8.74 | 12.70 | 840.2 | [23,24] |
| Sm2Fe17C3 | 668 | 1.43 | c axis | 8.744 | 12.572 | 832.4 | [25,26] |
| SmFe11Ti | 584 | 1.15 | c axis | 8.56 | 4.80 | 351.7 | [27,28] |
| SmFe11TiN | 769 | 1.28 | in-plane | 8.64 | 4.84 | 361.3 | [28,29] |
| NdFe11Ti | 547 | 1.38 | in-plane | 8.585 | 4.789 | 353.0 | [30,31] |
| NdFe11TiN | 729 | 1.48 | c axis | 8.701 | 4.844 | 366.7 | [32,33] |
| SmFe9Si2C1.0 | 462 | 0.88 | c axis | 10.057 | 6.512 | 658.7 | [34] |
| SmFe9Si2C1.5 | 492 | 0.90 | c axis | 10.082 | 6.554 | 666.1 | [34] |
| x | a (Å) | V (Å3) | µeff (µB/Mn) | θ (K) | TC (K) |
|---|---|---|---|---|---|
| 0 | 3.909 | 59.7 | 4.85 | −94 | - |
| 0.015 | 3.916 | 60.1 | 2.01 | 218 | 325 |
| 0.055 | 3.925 | 60.5 | 2.84 | 333 | 340 |
| 0.070 | 3.936 | 61.0 | 3.31 | 355 | 339 |
| 0.105 | 3.988 | 63.4 | 3.09 | 384 | 374 |
| 0.125 | 4.008 | 64.4 | 3.40 | 394 | 390 |
| 0.148 | 4.026 | 65.3 | 3.72 | 313 | 330 |
| 0.155 | 4.031 | 65.5 | 1.23 | 291 | 252 |
| 0.168 | 4.020 | 65.0 | 1.26 | 296 | 256 |
| x | a (Å) | c (Å) | V (Å3) | µeff (µB/Mn) | θ (K) | TC due to Mn (K) |
|---|---|---|---|---|---|---|
| 0 | 8.970 | 13.109 | 913.5 | 2.89 | −212 | - |
| 0.1 | 8.958 | 13.091 | 909.7 | 2.91 | 15 | 415(20) |
| 0.5 | 8.958 | 13.093 | 910.0 | 1.34 | 443 | 408(10) |
| 0.75 | 8.966 | 13.100 | 912.0 | 1.84 | 453 | 404(5) |
| 1 | 8.975 | 13.112 | 914.6 | 0.98 | 489 | 437 |
| No. | Compound | Structure Type | a (Å) | b (Å) | c (Å) | V(Å3) | Torder (K) | Type | Ref. |
|---|---|---|---|---|---|---|---|---|---|
| 1 | Lu6Mn23 | Th6Mn23 | 12.187 | 12.187 | 12.187 | 1810.0 | 398 | FM | [57] |
| 2 | Yb6Mn23 | Th6Mn23 | 12.189 | 12.189 | 12.189 | 1810.9 | 406 | FM | [58] |
| 3 | Tm6Mn23 | Th6Mn23 | 12.226 | 12.226 | 12.226 | 1827.5 | 411 | FM | [57] |
| 4 | Er6Mn23 | Th6Mn23 | 12.275 | 12.275 | 12.275 | 1849.5 | 430 | FM | [57] |
| 5 | Ho6Mn23 | Th6Mn23 | 12.324 | 12.324 | 12.324 | 1871.8 | 445 | FM | [57] |
| 6 | Dy6Mn23 | Th6Mn23 | 12.361 | 12.361 | 12.361 | 1888.7 | 447 | FM | [57] |
| 7 | Tb6Mn23 | Th6Mn23 | 12.396 | 12.396 | 12.396 | 1904.8 | 457 | FM | [57] |
| 8 | Y6Mn23 | Th6Mn23 | 12.438 | 12.438 | 12.438 | 1924.2 | 500 | FM | [59] |
| 9 | Gd6Mn23H24 | Th6Mn23 | 12.515 | 12.515 | 12.515 | 1960.2 | 260 | FM | [60] |
| 10 | Th6Mn23 | Th6Mn23 | 12.523 | 12.523 | 12.523 | 1963.9 | 0 | PM | [59] |
| 11 | Sm6Mn23 | Th6Mn23 | 12.558 | 12.558 | 12.558 | 1980.4 | 456 | FM | [57] |
| 12 | Gd6Mn23 | Th6Mn23 | 12.578 | 12.578 | 12.578 | 1989.9 | 489 | FM | [57] |
| 13 | Nd6Mn23 | Th6Mn23 | 12.657 | 12.657 | 12.657 | 2027.6 | 441 | FM | [57] |
| 14 | Y6Mn23H23 | Th6Mn23 | 12.805 | 12.805 | 12.805 | 2099.6 | 180 | AFM | [61] |
| 15 | Th6Mn23D16 | Th6Mn23 | 12.922 | 12.922 | 12.922 | 2157.7 | 329 | FM | [62] |
| 16 | Gd12Mn45H43 | Th6Mn23 | 12.97 | 12.97 | 12.97 | 2181.8 | 180 | FM | [63] |
| 17 | Tb6Mn23H23 | Th6Mn23 | 13.017 | 13.017 | 13.017 | 2205.6 | 220 | FM | [64] |
| 18 | Sm6Mn23H24 | Th6Mn23 | 13.04 | 13.04 | 13.04 | 2217.3 | 230 | FM | [60] |
| 19 | Nd6Mn23H24 | Th6Mn23 | 13.237 | 13.237 | 13.237 | 2319.4 | 220 | FM | [60] |
| 20 | Th6Mn23H30 | Th6Mn23 | 13.259 | 13.259 | 13.259 | 2330.9 | 329 | FM | [59] |
| 21 | YMn2Si2 | ThCr2Si2 | 3.924 | 3.924 | 10.457 | 161.0 | 463 | AFM | [65] |
| 22 | LaMn2Si2 | ThCr2Si2 | 4.1151 | 4.1151 | 10.612 | 179.7 | 305 | FM | [65] |
| 23 | CeMn2Si2 | ThCr2Si2 | 3.99 | 3.99 | 10.51 | 167.3 | 377 | AFM | [65] |
| 24 | PrMn2Si2 | ThCr2Si2 | 4.03 | 4.03 | 10.559 | 171.5 | 350 | AFM | [65] |
| 25 | NdMn2Si2 | ThCr2Si2 | 4.063 | 4.063 | 10.522 | 173.7 | 368 | AFM | [65] |
| 26 | SmMn2Si2 | ThCr2Si2 | 3.975 | 3.975 | 10.52 | 166.2 | 400 | AFM | [65] |
| 27 | EuMn2Si2 | ThCr2Si2 | 3.966 | 3.966 | 10.387 | 163.4 | 391 | AFM | [66] |
| 28 | GdMn2Si2 | ThCr2Si2 | 3.948 | 3.948 | 10.468 | 163.2 | 454 | AFM | [65] |
| 29 | TbMn2Si2 | ThCr2Si2 | 3.931 | 3.931 | 10.456 | 161.6 | 504 | AFM | [65] |
| 30 | DyMn2Si2 | ThCr2Si2 | 3.915 | 3.915 | 10.44 | 160.0 | 477 | AFM | [65] |
| 31 | HoMn2Si2 | ThCr2Si2 | 3.931 | 3.931 | 10.412 | 160.9 | 455 | AFM | [65] |
| 32 | ErMn2Si2 | ThCr2Si2 | 3.905 | 3.905 | 10.42 | 158.9 | 518 | AFM | [65] |
| 33 | TmMn2Si2 | ThCr2Si2 | 3.887 | 3.887 | 10.398 | 157.1 | 500 | AFM | [65] |
| 34 | YbMn2Si2 | ThCr2Si2 | 3.877 | 3.877 | 10.391 | 156.2 | 512 | AFM | [65] |
| 35 | LuMn2Si2 | ThCr2Si2 | 3.873 | 3.873 | 10.37 | 155.6 | 468 | AFM | [65] |
| 36 | YMn2Ge2 | ThCr2Si2 | 4.0516 | 4.0516 | 10.854 | 178.2 | 395 | AFM | [65] |
| 37 | LaMn2Ge2 | ThCr2Si2 | 4.195 | 4.195 | 11.022 | 194.0 | 309 | FM | [65] |
| 38 | CeMn2Ge2 | ThCr2Si2 | 4.144 | 4.144 | 10.97 | 188.4 | 325 | FM | [65] |
| 39 | PrMn2Ge2 | ThCr2Si2 | 4.123 | 4.123 | 10.929 | 185.8 | 336 | FM | [65] |
| 40 | NdMn2Ge2 | ThCr2Si2 | 4.1022 | 4.1022 | 10.909 | 183.6 | 334 | FM | [65] |
| 41 | SmMn2Ge2 | ThCr2Si2 | 4.062 | 4.062 | 10.896 | 179.8 | 350 | FM | [65] |
| 42 | GdMn2Ge2 | ThCr2Si2 | 4.029 | 4.029 | 10.895 | 176.9 | 368 | AFM | [65] |
| 43 | TbMn2Ge2 | ThCr2Si2 | 4.006 | 4.006 | 10.875 | 174.5 | 418 | AFM | [65] |
| 44 | DyMn2Ge2 | ThCr2Si2 | 3.989 | 3.989 | 10.863 | 172.9 | 386 | AFM | [65] |
| 45 | HoMn2Ge2 | ThCr2Si2 | 3.977 | 3.977 | 10.847 | 171.6 | 404 | AFM | [65] |
| 46 | ErMn2Ge2 | ThCr2Si2 | 3.948 | 3.948 | 10.791 | 168.2 | 395 | AFM | [65] |
| 47 | YbMn2Ge2 | ThCr2Si2 | 4.067 | 4.067 | 10.871 | 179.8 | 341 | AFM | [65] |
| 48 | ThMn2Si2 | ThCr2Si2 | 4.0225 | 4.0225 | 10.475 | 169.5 | 486 | AFM | [65] |
| 49 | UMn2Si2 | ThCr2Si2 | 3.922 | 3.922 | 10.284 | 158.2 | 377 | FM | [67] |
| 50 | ThMn2Ge2 | ThCr2Si2 | 4.084 | 4.084 | 10.93 | 182.3 | 400 | AFM | [65] |
| 51 | UMn2Ge2 | ThCr2Si2 | 4.012 | 4.012 | 10.803 | 173.9 | 390 | FM | [67] |
| 52 | Mn3Ge | Ni3Sn | 5.338 | 5.338 | 4.312 | 106.4 | 365 | AFM | [68] |
| 53 | Mn3Ga | Ni3Sn | 5.404 | 5.404 | 4.357 | 110.2 | 470 | AFM | [69] |
| 54 | Mn3Sn | Ni3Sn | 5.64 | 5.64 | 4.52 | 124.5 | 420 | AFM | [20] |
| 55 | ErMn12 | ThMn12 | 8.5719 | 8.5719 | 4.7553 | 349.4 | 80 | AFM | [70] |
| 56 | HoMn12 | ThMn12 | 8.5817 | 8.5817 | 4.7592 | 350.5 | 90 | AFM | [71] |
| 57 | YMn12 | ThMn12 | 8.597 | 8.597 | 4.7637 | 352.1 | 120 | AFM | [72] |
| 58 | TbMn12 | ThMn12 | 8.6076 | 8.6076 | 4.7666 | 353.2 | 120 | AFM | [73] |
| 59 | DyMn12 | ThMn12 | 8.67 | 8.67 | 4.76 | 357.8 | 110 | AFM | [74] |
| 60 | GdMn12 | ThMn12 | 8.673 | 8.673 | 4.839 | 364.0 | 86 | AFM | [74] |
| 61 | ScMn4Al8 | ThMn12 | 8.7734 | 8.7734 | 5.0629 | 389.7 | 0 | PM | [75] |
| 62 | LuMn4Al8 | ThMn12 | 8.814 | 8.814 | 5.083 | 394.9 | 0 | PM | [75] |
| 63 | ErMn4Al8 | ThMn12 | 8.829 | 8.829 | 5.096 | 397.2 | 15 | AFM | [76] |
| 64 | TmMn4Al8 | ThMn12 | 8.848 | 8.848 | 5.08 | 397.7 | 13 | AFM | [76] |
| 65 | HoMn4Al8 | ThMn12 | 8.845 | 8.845 | 5.097 | 398.8 | 14 | AFM | [76] |
| 66 | UMn4Al8 | ThMn12 | 8.8474 | 8.8474 | 5.0993 | 399.2 | 0 | PM | [77] |
| 67 | YMn4Al8 | ThMn12 | 8.86 | 8.86 | 5.09 | 399.6 | 0 | PM | [76] |
| 68 | GdMn6Al6 | ThMn12 | 8.845 | 8.845 | 5.108 | 399.6 | 36 | AFM | [78] |
| 69 | YbMn4Al8 | ThMn12 | 8.854 | 8.854 | 5.102 | 400.0 | 0 | PM | [76] |
| 70 | DyMn4Al8 | ThMn12 | 8.849 | 8.849 | 5.112 | 400.3 | 19 | AFM | [76] |
| 71 | TbMn4Al8 | ThMn12 | 8.865 | 8.865 | 5.108 | 401.4 | 21 | AFM | [76] |
| 72 | GdMn4Al8 | ThMn12 | 8.887 | 8.887 | 5.119 | 404.3 | 28 | AFM | [76] |
| 73 | TbMn4.04Al7.96 | ThMn12 | 8.8885 | 8.8885 | 5.1179 | 404.3 | 0 | PM | [79] |
| 74 | SmMn4Al8 | ThMn12 | 8.902 | 8.902 | 5.12 | 405.7 | 12 | AFM | [76] |
| 75 | CeMn4Al8 | ThMn12 | 8.89 | 8.89 | 5.17 | 408.6 | 0 | PM | [76] |
| 76 | NdMn4Al8 | ThMn12 | 8.925 | 8.925 | 5.133 | 408.9 | 7 | AFM | [76] |
| 77 | PrMn4Al8 | ThMn12 | 8.962 | 8.962 | 5.143 | 413.1 | 11 | AFM | [76] |
| 78 | EuMn4Al8 | ThMn12 | 8.982 | 8.982 | 5.161 | 416.4 | 20 | AFM | [76] |
| 79 | LaMn4Al8 | ThMn12 | 9.031 | 9.031 | 5.166 | 421.3 | 0 | PM | [76] |
| 80 | ThMn4Al8 | ThMn12 | 8.937 | 8.937 | 5.639 | 450.4 | 0 | PM | [80] |
| 81 | Mn2P | Fe2P | 6.074 | 6.074 | 3.454 | 110.4 | 100 | AFM | [81,82] |
| 82 | TiMnP | Fe2P | 6.188 | 6.188 | 3.451 | 114.4 | 0 | PM | [83] |
| 83 | MnRhP | Fe2P | 6.226 | 6.226 | 3.581 | 120.2 | 401 | FM | [84] |
| 84 | Mn2As | Fe2P | 6.3627 | 6.3627 | 3.6784 | 129.0 | 50 | AFM | [85] |
| 85 | MnRuAs | Fe2P | 6.5155 | 6.5155 | 3.614 | 132.9 | 496 | FM | [84] |
| 86 | MnRhAs | Fe2P | 6.482 | 6.482 | 3.714 | 135.1 | 190 | FM | [86] |
| 87 | MnPdGe | Fe2P | 6.639 | 6.639 | 3.577 | 136.5 | 576 | FM | [87] |
| 88 | MnPdAs | Fe2P | 6.626 | 6.626 | 3.756 | 142.8 | 210 | FM | [84] |
| 89 | MnNiGe | Ni2In | 4.078 | 4.078 | 5.381 | 77.50 | 346 | AFM | [88] |
| 90 | MnPtAl | Ni2In | 4.329 | 4.329 | 5.499 | 89.25 | 295 | AFM | [89] |
| 91 | MnPtGa | Ni2In | 4.328 | 4.328 | 5.576 | 90.45 | 220 | FM | [90] |
| 92 | NiMnSb | LiAlSi | 5.928 | 5.928 | 5.928 | 208.3 | 728 | FM | [91] |
| 93 | MnIrAl | LiAlSi | 5.981 | 5.981 | 5.981 | 214.0 | 379 | FM | [92] |
| 94 | MnCuSb | LiAlSi | 6.095 | 6.095 | 6.095 | 226.4 | 55 | AFM | [93] |
| 95 | MnRhSb | LiAlSi | 6.142 | 6.142 | 6.142 | 231.7 | 320 | FM | [93] |
| 96 | MnIrSn | LiAlSi | 6.182 | 6.182 | 6.182 | 236.3 | 265 | FM | [94] |
| 97 | MnPtSb | LiAlSi | 6.21 | 6.21 | 6.21 | 239.5 | 585 | FM | [93] |
| 98 | PdMnSb | LiAlSi | 6.231 | 6.231 | 6.231 | 241.9 | 500 | FM | [95] |
| 99 | MnPdTe | LiAlSi | 6.2605 | 6.2605 | 6.2605 | 245.4 | 17 | AFM | [96] |
| 100 | MnPtSn | LiAlSi | 6.264 | 6.264 | 6.264 | 245.8 | 330 | FM | [93] |
| 101 | MnAuSn | LiAlSi | 6.4313 | 6.4312 | 6.4312 | 266.0 | 740 | FM | [97] |
| 102 | MnRhSi | TiNiSi | 6.1994 | 3.7968 | 7.1387 | 168.0 | 367 | AFM | [98] |
| 103 | HfMnP | TiNiSi | 6.3257 | 3.6298 | 7.409 | 170.1 | 320 | FM | [99] |
| 104 | ZrMnP | TiNiSi | 6.41 | 3.657 | 7.515 | 176.2 | 370 | FM | [99] |
| 105 | LuMnSi | TiNiSi | 6.82 | 3.962 | 7.839 | 211.8 | 255 | AFM | [100] |
| 106 | UMnGe | TiNiSi | 6.866 | 4.2594 | 7.3618 | 215.3 | 240 | AFM | [101] |
| 107 | TbMnGe | TiNiSi | 7.077 | 4.132 | 8.166 | 238.8 | 510 | AFM | [102] |
| 108 | GdMnGe | TiNiSi | 7.138 | 4.1698 | 8.191 | 243.8 | 490 | AFM | [103] |
| 109 | MnNi | AuCu | 3.69 | 3.69 | 2.609 | 35.52 | 1070 | AFM | [104] |
| 110 | MnPt | AuCu | 3.855 | 3.855 | 2.726 | 40.51 | 970 | AFM | [104] |
| 111 | MnGa | AuCu | 3.889 | 3.889 | 2.75 | 41.59 | 629 | FM | [105] |
| 112 | MnRh | AuCu | 3.93 | 3.93 | 2.779 | 42.92 | 0 | PM | [104] |
| 113 | MnPd | AuCu | 4.069 | 4.069 | 2.877 | 47.63 | 780 | AFM | [104] |
| 114 | MnAl | AuCu | 3.9 | 3.9 | 3.54 | 53.84 | 578 | FM | [106] |
| 115 | MnRh2Sb | AuCu | 4.171 | 4.171 | 3.494 | 60.79 | 304 | FM | [107] |
| 116 | MnAlGe | Cu2Sb | 3.914 | 3.914 | 5.933 | 90.89 | 518 | FM | [108] |
| 117 | MnGaGe | Cu2Sb | 3.966 | 3.966 | 5.885 | 92.57 | 440 | FM | [109] |
| 118 | MnZnSb | Cu2Sb | 4.173 | 4.173 | 6.233 | 108.5 | 310 | FM | [110] |
| 119 | LiMnAs | Cu2Sb | 4.267 | 4.267 | 6.178 | 112.5 | 374 | AFM | [111] |
| 120 | YMnSi | Cu2Sb | 3.978 | 3.978 | 7.152 | 113.2 | 282 | FM | [112] |
| 121 | GdMnSi | Cu2Sb | 4.009 | 4.009 | 7.183 | 115.5 | 314 | FM | [113] |
| 122 | NdMnSi | Cu2Sb | 4.087 | 4.087 | 7.245 | 121.0 | 175 | AFM | [114] |
| 123 | CeMnSi | Cu2Sb | 4.13 | 4.13 | 7.279 | 124.2 | 240 | AFM | [114] |
| 124 | CaMnSi | Cu2Sb | 4.1814 | 4.1814 | 7.1429 | 124.9 | 360 | AFM | [115] |
| 125 | PrMnGe | Cu2Sb | 4.196 | 4.196 | 7.344 | 129.3 | 415 | AFM | [116] |
| 126 | LaMnSi | Cu2Sb | 4.189 | 4.189 | 7.378 | 129.5 | 310 | AFM | [114] |
| 127 | CaMnGe | Cu2Sb | 4.23 | 4.23 | 7.27 | 130.1 | 420 | AFM | [115] |
| 128 | CeMnGe | Cu2Sb | 4.226 | 4.226 | 7.386 | 131.9 | 415 | AFM | [116] |
| 129 | LaMnGe | Cu2Sb | 4.277 | 4.277 | 7.449 | 136.3 | 420 | AFM | [116] |
| 130 | SrMnGe | Cu2Sb | 4.4 | 4.4 | 7.52 | 145.6 | 254 | AFM | [117] |
| 131 | CaMnSn | Cu2Sb | 4.4839 | 4.4839 | 7.4988 | 150.8 | 250 | AFM | [117] |
| 132 | BaMnGe | Cu2Sb | 4.5 | 4.5 | 7.92 | 160.4 | 425 | AFM | [117] |
| 133 | SrMnSn | Cu2Sb | 4.662 | 4.662 | 7.749 | 168.4 | 252 | AFM | [117] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kitagawa, J.; Sakaguchi, K.; Hara, T.; Hirano, F.; Shirakawa, N.; Tsubota, M. Interstitial Atom Engineering in Magnetic Materials. Metals 2020, 10, 1644. https://doi.org/10.3390/met10121644
Kitagawa J, Sakaguchi K, Hara T, Hirano F, Shirakawa N, Tsubota M. Interstitial Atom Engineering in Magnetic Materials. Metals. 2020; 10(12):1644. https://doi.org/10.3390/met10121644
Chicago/Turabian StyleKitagawa, Jiro, Kohei Sakaguchi, Tomohiro Hara, Fumiaki Hirano, Naoki Shirakawa, and Masami Tsubota. 2020. "Interstitial Atom Engineering in Magnetic Materials" Metals 10, no. 12: 1644. https://doi.org/10.3390/met10121644
APA StyleKitagawa, J., Sakaguchi, K., Hara, T., Hirano, F., Shirakawa, N., & Tsubota, M. (2020). Interstitial Atom Engineering in Magnetic Materials. Metals, 10(12), 1644. https://doi.org/10.3390/met10121644







