An Overview of Major Experimental Methods and Apparatus for Measuring and Investigating Erosion-Corrosion of Ferrous-Based Steels
Abstract
:1. Introduction
- Erosion-dominated (Wc/We < 0.1);
- Erosion-corrosion-dominated (0.1 ≤ Wc/We < 1);
- Corrosion-erosion-dominated (1 ≤ Wc/We < 10);
- Corrosion-dominated (Wc/We ≥ 10).
2. Apparatus for Simulating Erosion-Corrosion of Steel
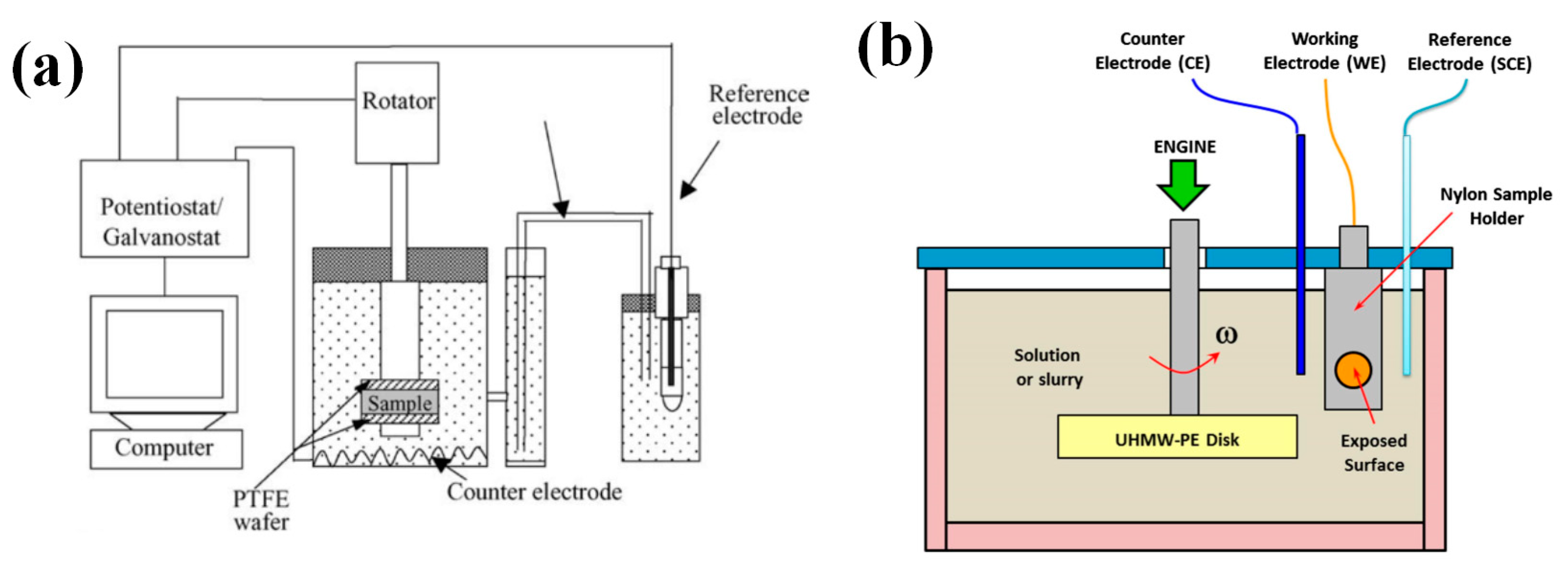
2.1. Rotating Disc/Cylinder Electrode System
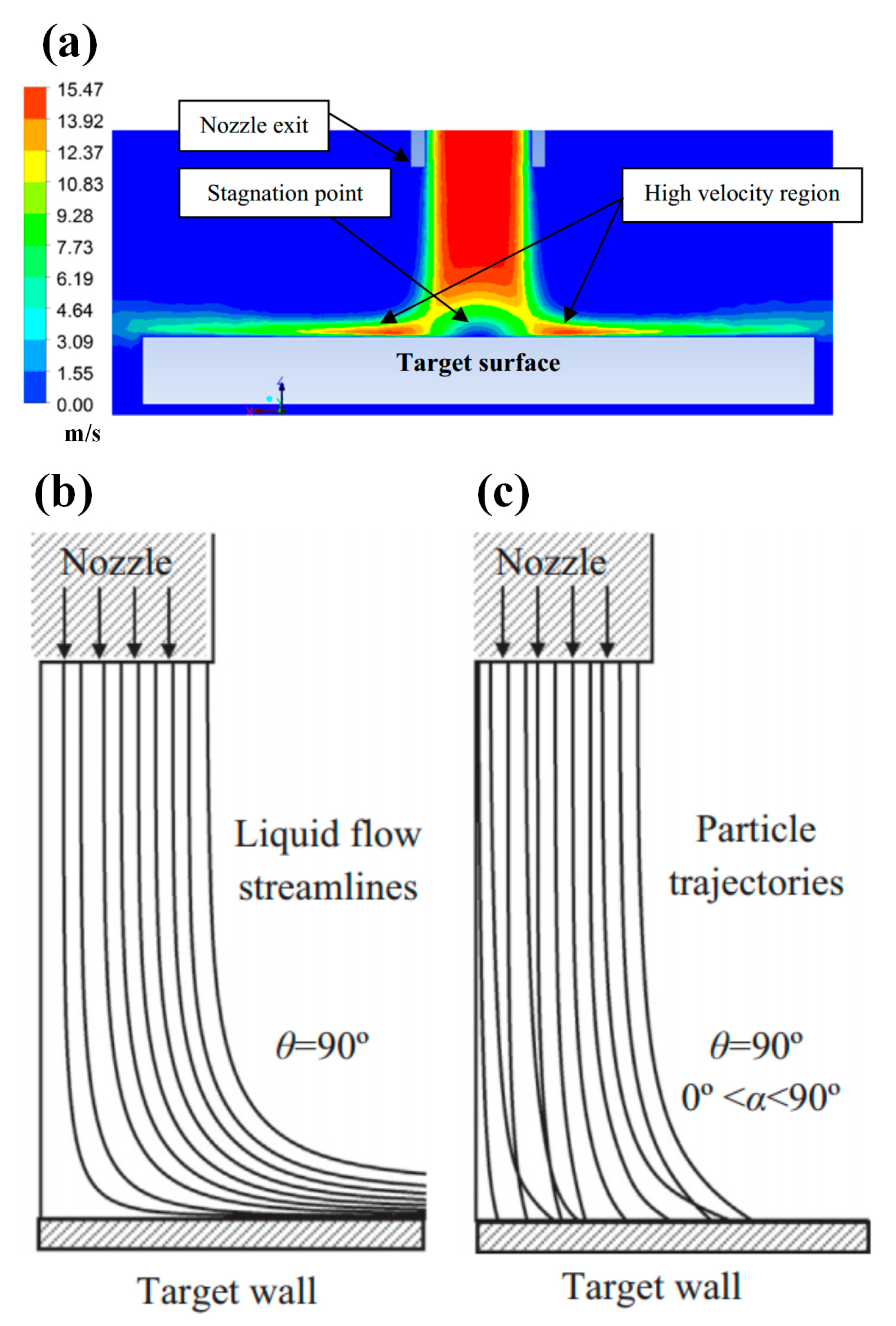
2.2. Slurry Jet Impingement Rig System
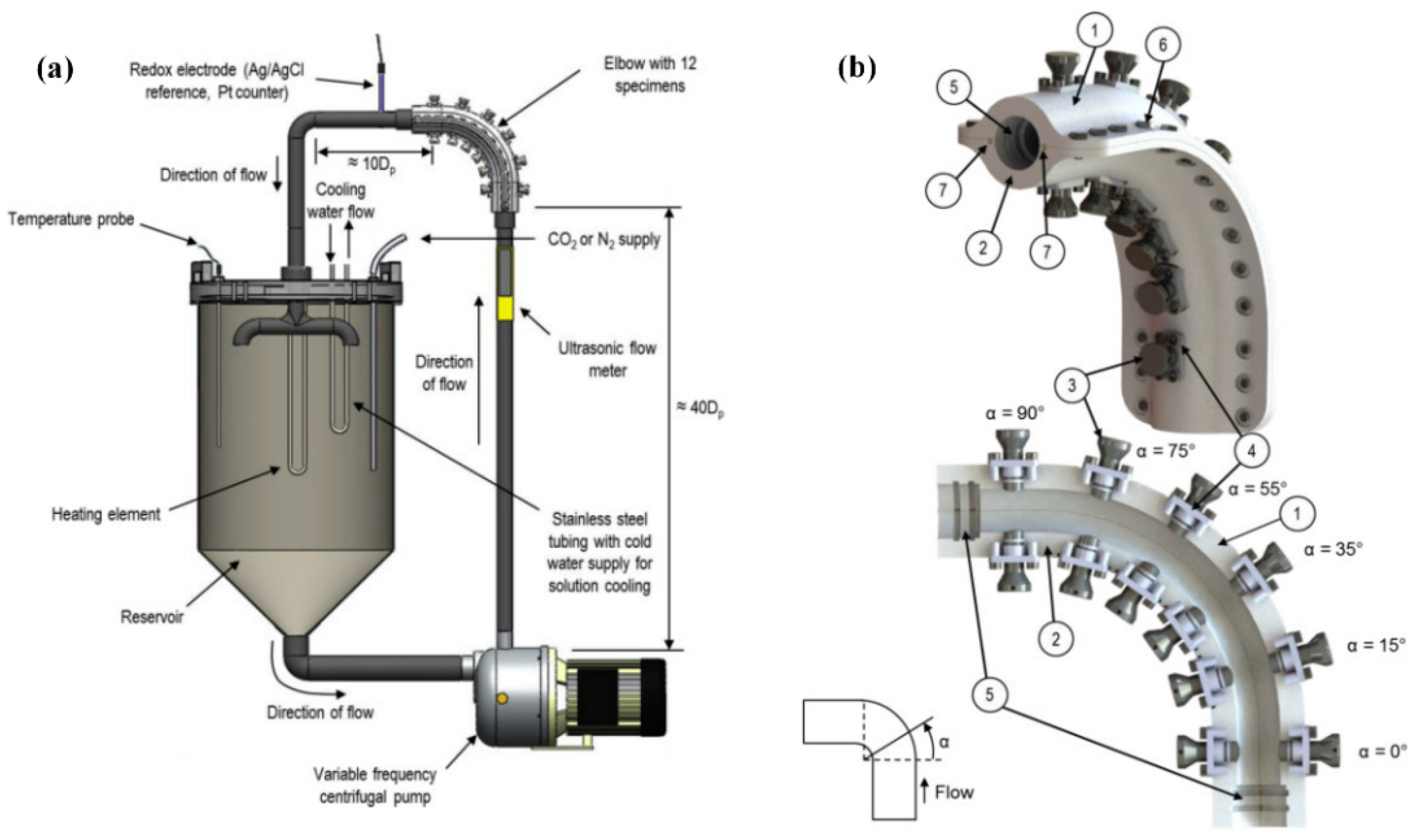
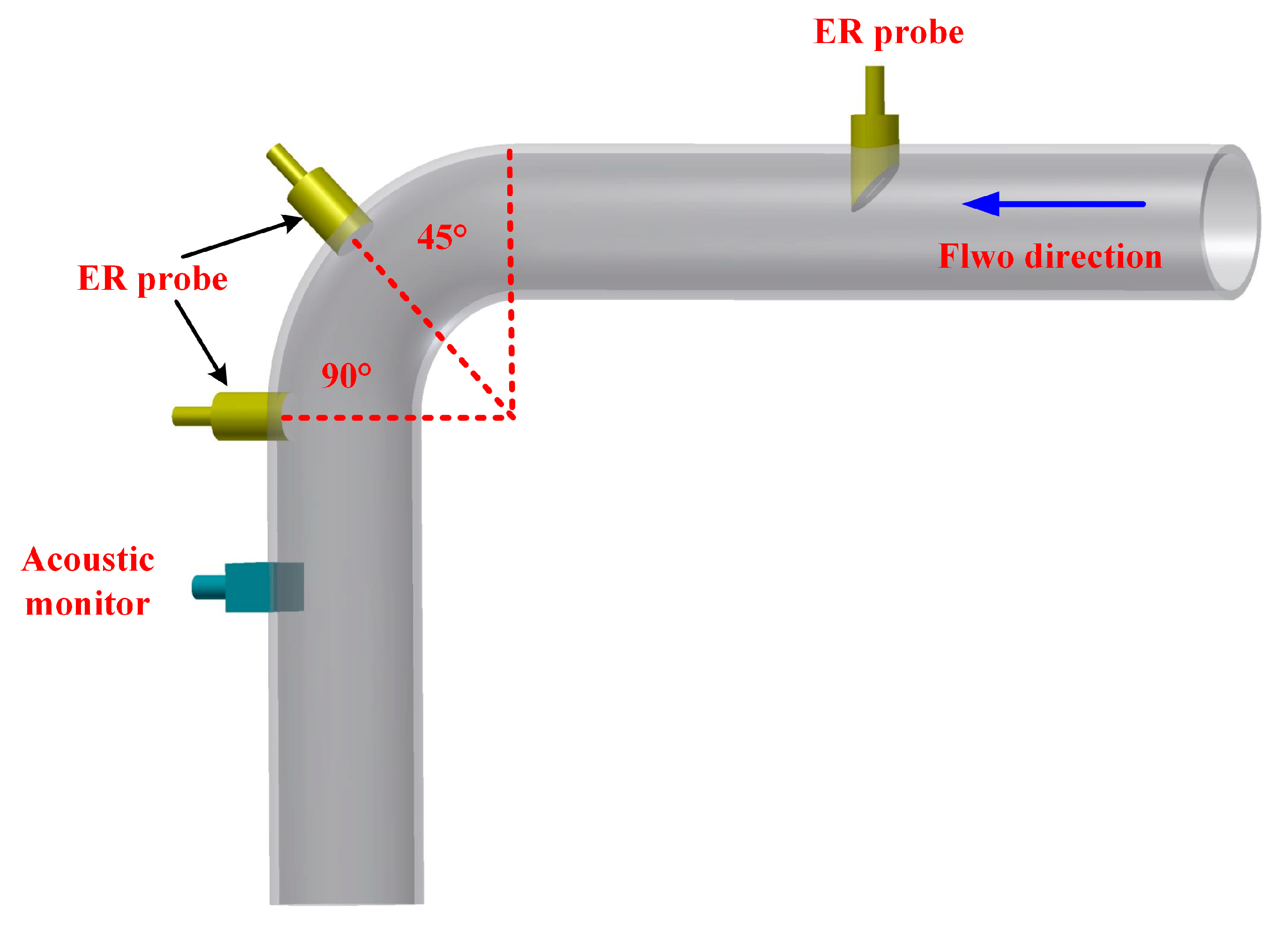
2.3. Test Flow Loop
3. The Main Measuring Techniques for Erosion-Corrosion
3.1. Non-Electrochemical Measurement Techniques
3.2. Electrochemical Techniques in Conjunction with Gravimetric Measurement
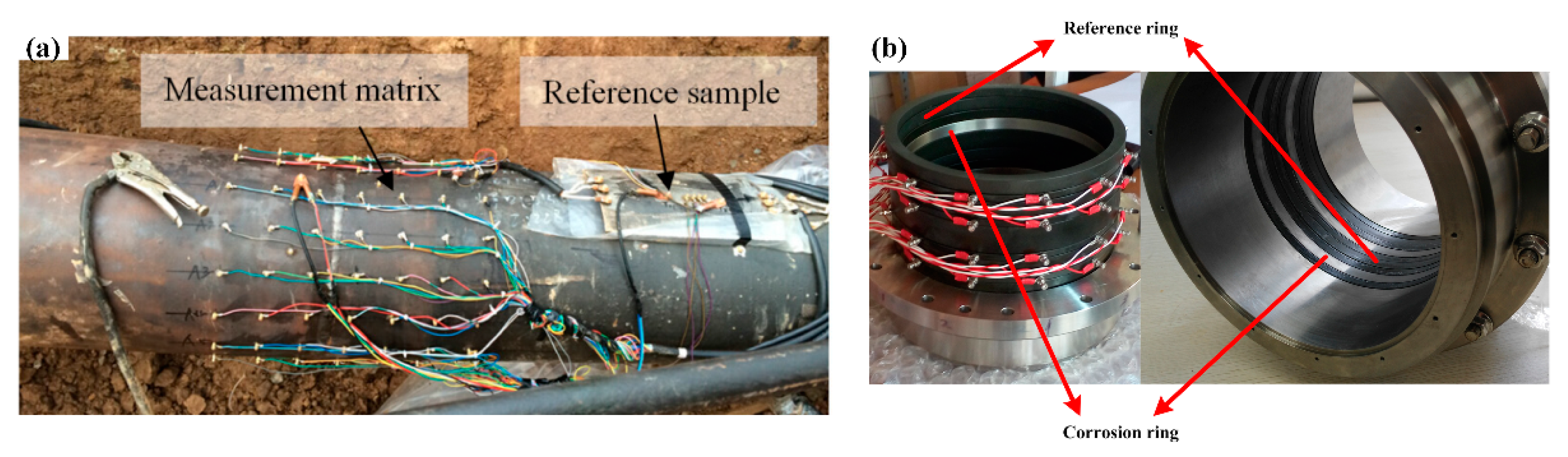
3.3. Advances in the Monitoring of Erosion-Corrosion Using Specially Designed Sensors
4. Advances in the Mechanism Study of Erosion-Corrosion of Steel
4.1. The Interaction between Erosion and Corrosion in Cyclic Erosion-Corrosion Tests
4.2. Understanding the Erosion-Corrosion Behavior of Steels under Passive State
4.3. Understanding the Erosion-Corrosion Behavior of Steels under Active Corrosion
5. Summary
Author Contributions
Funding
Conflicts of Interest
References
- Wu, J.; Graham, L.J.W.; Lester, D.; Wong, C.Y.; Kilpatrick, T.; Smith, S.; Nguyen, B. An effective modeling tool for studying erosion. Wear 2011, 270, 598–605. [Google Scholar] [CrossRef]
- Matsumura, M. Erosion-Corrosion: An Introduction to Flow Induced Macro-Cell Corrosion; Bentham Science Publishers, Hiroshima University: Hiroshima, Japan, 2012. [Google Scholar]
- Zhu, M.; Sun, L.; Ou, G.; Wang, K.; Wang, K.; Sun, Y. Erosion corrosion failure analysis of the elbow in sour water stripper overhead condensing reflux system. Eng. Fail. Anal. 2016, 26, 93–102. [Google Scholar] [CrossRef]
- Muthanna, B.G.N.; Amara, M.; Meliani, M.H.; Mettai, B.; Božić, Z.; Suleiman, R.; Sorour, A.A. Inspection of internal erosion-corrosion of elbow pipe in the desalination station. Eng. Fail. Anal. 2019, 102, 293–302. [Google Scholar] [CrossRef]
- Zhang, M.; Liu, Y.; Wang, W.; Wang, P.; Li, J. The fatigue of impellers and blades. Eng. Fail. Anal. 2016, 62, 208–231. [Google Scholar] [CrossRef]
- Zeng, L.; Zhang, G.A.; Guo, X.P. Erosion–corrosion at different locations of X65 carbon steel elbow. Corros. Sci. 2014, 85, 318–330. [Google Scholar] [CrossRef]
- Tian, B.R.; Cheng, Y.F. Electrochemical corrosion behavior of X-65 steel in the simulated oil sand slurry I: Effects of hydrodynamic condition. Corros. Sci. 2008, 50, 773–779. [Google Scholar] [CrossRef]
- Dong, C.F.; Xiao, K.; Li, X.G.; Cheng, Y.F. Erosion accelerated corrosion of a carbon steel–stainless steel galvanic couple in a chloride solution. Wear 2010, 270, 39–45. [Google Scholar] [CrossRef]
- Guo, H.X.; Lu, B.T.; Luo, J.L. Non-Faraday material loss in flowing corrosive solution. Electrochim. Acta 2006, 51, 5341–5348. [Google Scholar] [CrossRef]
- Guo, H.X.; Lu, B.T.; Luo, J.L. Interaction of mechanical and electrochemical factors in erosion–corrosion of carbon steel. Electrochim. Acta 2005, 51, 315–323. [Google Scholar] [CrossRef]
- Tang, X.; Xu, L.Y.; Cheng, Y.F. Electrochemical corrosion behavior of X-65 steel in the simulated oil–sand slurry II: Synergism of erosion and corrosion. Corros. Sci. 2008, 50, 1469–1474. [Google Scholar] [CrossRef]
- Li, Y.; Burstein, G.T.; Hutchings, I.M. The influence of corrosion on the erosion of aluminium by aqueous silica slurries. Wear 1995, 186, 515–522. [Google Scholar] [CrossRef]
- Zhang, G.A.; Xu, L.Y.; Cheng, Y.F. Investigation of erosion–corrosion of 3003 aluminum alloy in ethylene glycol–water solution by impingement jet system. Corros. Sci. 2009, 51, 283–290. [Google Scholar] [CrossRef]
- Malka, R.; Nešić, S.; Gulino, D.A. Erosion–corrosion and synergistic effects in disturbed liquid-particle flow. Wear 2007, 262, 791–799. [Google Scholar] [CrossRef] [Green Version]
- Lu, B.T.; Luo, J.L.; Mohammadi, F.; Wang, K.; Wan, X.M. Correlation between repassivation kinetics and corrosion rate over a passive surface in flowing slurry. Electrochim. Acta 2008, 53, 7022–7031. [Google Scholar] [CrossRef]
- Hu, X.; Neville, A. CO2 erosion–corrosion of pipeline steel (API X65) in oil and gas conditions—A systematic approach. Wear 2009, 267, 2027–2032. [Google Scholar] [CrossRef]
- Owen, J.; Ramsey, C.; Barker, R.; Neville, A. Erosion-corrosion interactions of X65 carbon steel in aqueous CO2 environments. Wear 2018, 414–415, 376–389. [Google Scholar] [CrossRef]
- Barik, R.C.; Wharton, J.A.; Wood, R.J.K.; Tan, K.S.; Stokes, K.R. Erosion and erosion–corrosion performance of cast and thermally sprayed nickel–aluminium bronze. Wear 2005, 259, 230–242. [Google Scholar] [CrossRef]
- Tan, K.S.; Wharton, J.A.; Wood, R.J.K. Solid particle erosion–corrosion behaviour of a novel HVOF nickel aluminium bronze coating for marine applications—Correlation between mass loss and electrochemical measurements. Wear 2005, 258, 629–640. [Google Scholar] [CrossRef]
- ASTM Standard Guide for Determining Synergism between Wear and Corrosion: G119-09; ASTM International: West Conshohocken, PA, USA, 2009.
- Lu, B.T.; Luo, J.L.; Ma, H.Y. A theoretical model on electrochemical response of passivated metals to solid particle impingement. J. Electrochem. Soc. 2007, 154, C159. [Google Scholar] [CrossRef]
- Stack, M.M.; Stott, F.H. An approach to modeling erosion-corrosion of alloys using erosion-corrosion maps. Corros. Sci. 1993, 35, 1027–1034. [Google Scholar] [CrossRef]
- Saha, G.C.; Khan, T.I.; Zhang, G.A. Erosion–corrosion resistance of microcrystalline and near-nanocrystalline WC–17Co high velocity oxy-fuel thermal spray coatings. Corros. Sci. 2011, 53, 2106–2114. [Google Scholar] [CrossRef]
- Stack, M.M.; Abdulrahman, G.H. Mapping erosion–corrosion of carbon steel in oil–water solutions: Effects of velocity and applied potential. Wear 2012, 274–275, 401–413. [Google Scholar] [CrossRef] [Green Version]
- Stack, M.M.; Chi, K. Mapping sliding wear of steels in aqueous conditions. Wear 2003, 255, 456–465. [Google Scholar] [CrossRef]
- Stack, M.M.; Corlett, N.; Zhou, S. Impact angle effects on the transition boundaries of the aqueous erosion–corrosion map. Wear 1999, 225, 190–198. [Google Scholar] [CrossRef]
- Stack, M.M.; Corlett, N.; Zhou, S. A methodology for the construction of the erosion-corrosion map in aqueous environments. Wear 1997, 203, 474–488. [Google Scholar] [CrossRef]
- Stack, M.M.; Peña, D. Mapping erosion of Ni–Cr/WC-based composites at elevated temperatures: Some recent advances. Wear 2001, 251, 1433–1443. [Google Scholar] [CrossRef]
- Stack, M.M.; Pungwiwat, N. Erosion–corrosion mapping of Fe in aqueous slurries: Some views on a new rationale for defining the erosion–corrosion interaction. Wear 2004, 256, 565–576. [Google Scholar] [CrossRef]
- Stack, M.M.; Wang, H.W. Simplifying the erosion–corrosion mechanism map for erosion of thin coatings in aqueous slurries. Wear 1999, 233, 542–551. [Google Scholar] [CrossRef]
- Finnie, I. Erosion of surfaces by solid particles. Wear 1960, 3, 87–103. [Google Scholar] [CrossRef]
- Bitter, J.G.A. A study of erosion phenomena Part I. Wear 1962, 6, 5–21. [Google Scholar] [CrossRef]
- Hutchings, I.M. A model for the erosion of metals by spherical particles at normal incidence. Wear 1981, 70, 269–281. [Google Scholar] [CrossRef] [Green Version]
- McLaury, B.S. A Model to Predict Solid Particle Erosion in Oilfield Geometries. Ph.D. Thesis, The University of Tulsa, Tulsa, OK, USA, 1993. [Google Scholar]
- Oka, Y.I.; Okamura, K.; Yoshida, T. Practical estimation of erosion damage caused by solid particle impact. Wear 2005, 259, 95–101. [Google Scholar] [CrossRef]
- Javaheri, V.; Porter, D.; Kuokkala, V. Slurry erosion of steel—Review of tests, mechanisms and materials. Wear 2018, 408–409, 248–273. [Google Scholar] [CrossRef]
- Lu, B.; Luo, J. A phenomenological model for non-Faradaic material loss in flowing electrolyte without solid particle. Electrochim. Acta 2010, 56, 559–565. [Google Scholar] [CrossRef]
- Lu, B.T.; Mao, L.C.; Luo, J.L. Hydrodynamic effects on erosion-enhanced corrosion of stainless steel in aqueous slurries. Electrochim. Acta 2010, 56, 85–92. [Google Scholar] [CrossRef]
- Xie, J.; Alpas, A.T.; Northwood, D.O. Mechano-electrochemical effect between erosion and corrosion. J. Mater. Sci. 2003, 38, 4849–4856. [Google Scholar] [CrossRef]
- Xie, J.; Alpas, A.T.; Northwood, D.O. The effect of erosion on the electrochemical properties of AISI 1020 steel. J. Mater. Eng. Perform. 2003, 12, 77–86. [Google Scholar] [CrossRef]
- Naz, M.Y.; Sulaiman, S.A.; Shukrullah, S.; Ghaffar, A.; Ibrahim, K.A.; AbdEl-Salam, N.M. Development of erosion-corrosion mechanisms for the study of steel surface behavior in a sand slurry. Measurement 2017, 106, 203–210. [Google Scholar] [CrossRef]
- Lu, B.T.; Luo, J.L.; Guo, H.X.; Mao, L.C. Erosion-enhanced corrosion of carbon steel at passive state. Corros. Sci. 2011, 53, 432–440. [Google Scholar] [CrossRef]
- Zeng, L.; Guo, X.P.; Zhang, G.A. Inhibition of the erosion-corrosion of a 90° low alloy steel bend. J. Alloy Compd. 2017, 724, 827–840. [Google Scholar] [CrossRef]
- Eisenberg, M.; Tobias, C.W.; Wilke, C.R. Ionic mass transfer and concentration polarization at rotating electrodes. J. Electrochem. Soc. 1954, 101, 306. [Google Scholar] [CrossRef]
- Walsh, F.C.; Kear, G.; Nahlé, A.H.; Wharton, J.A.; Arenas, L.F. The rotating cylinder electrode for studies of corrosion engineering and protection of metals—An illustrated review. Corros. Sci. 2017, 123, 1–20. [Google Scholar] [CrossRef] [Green Version]
- Rajahram, S.S.; Harvey, T.J.; Wood, R.J.K. Electrochemical investigation of erosion–corrosion using a slurry pot erosion tester. Tribol. Int. 2011, 44, 232–240. [Google Scholar] [CrossRef]
- Efird, K.D.; Wright, E.J.; Boros, J.A.; Hailey, T.G. Correlation of steel corrosion in pipe flow with jet impingement and rotating cylinder tests. Corrosion 1993, 49, 992–1003. [Google Scholar] [CrossRef]
- Jiang, X.; Zheng, Y.G.; Ke, W. Effect of flow velocity and entrained sand on inhibition performances of two inhibitors for CO2 corrosion of N80 steel in 3% NaCl solution. Corros. Sci. 2005, 47, 2636–2658. [Google Scholar] [CrossRef]
- Choi, Y.; Nesic, S.; Ling, S. Effect of H2S on the CO2 corrosion of carbon steel in acidic solutions. Electrochim. Acta 2011, 56, 1752–1760. [Google Scholar] [CrossRef]
- Zhang, G.A.; Cheng, Y.F. Electrochemical corrosion of X65 pipe steel in oil/water emulsion. Corros. Sci. 2009, 51, 901–907. [Google Scholar] [CrossRef]
- Paolinelli, L.D.; Brown, B.; Simison, S.N.; Nesic, S. Inhibition of CO2 corrosion of carbon steel with 1% Cr. Mater. Chem. Phys. 2012, 136, 1092–1102. [Google Scholar] [CrossRef]
- Lu, B.T.; Luo, J.L. Correlation between surface-hardness degradation and erosion resistance of carbon steel-Effects of slurry chemistry. Tribol. Int. 2015, 83, 146–155. [Google Scholar] [CrossRef]
- Xu, Y.; Tan, M.Y. Probing the initiation and propagation processes of flow accelerated corrosion and erosion corrosion under simulated turbulent flow conditions. Corros. Sci. 2019, 151, 163–174. [Google Scholar] [CrossRef]
- Ayyagari, A.; Hasannaeimi, V.; Grewal, H.S.; Arora, H.; Mukherjee, S. Corrosion, Erosion and wear behavior of complex concentrated alloys: A Review. Metals 2018, 8, 603. [Google Scholar] [CrossRef] [Green Version]
- Alegría-Ortega, J.A.; Ocampo-Carmona, L.M.; Suárez-Bustamante, F.A.; Olaya-Flórez, J.J. Erosion–corrosion wear of Cr/CrN multi-layer coating deposited on AISI-304 stainless steel using the unbalanced magnetron (UBM) sputtering system. Wear 2012, 290–291, 149–153. [Google Scholar] [CrossRef]
- Li, Y.Y.; Wang, Z.Z.; Guo, X.P.; Zhang, G.A. Galvanic corrosion between N80 carbon steel and 13Cr stainless steel under supercritical CO2 conditions. Corros. Sci. 2019, 147, 260–272. [Google Scholar] [CrossRef]
- Haider, G.; Arabnejad, H.; Shirazi, S.A.; Mclaury, B.S. A mechanistic model for stochastic rebound of solid particles with application to erosion predictions. Wear 2017, 376–377, 615–624. [Google Scholar] [CrossRef]
- Arabnejad, H.; Mansouri, A.; Shirazi, S.A.; McLaury, B.S. Development of mechanistic erosion equation for solid particles. Wear 2015, 332–333, 1044–1050. [Google Scholar] [CrossRef]
- Mansouri, A.; Arabnejad, H.; Shirazi, S.A.; McLaury, B.S. A combined CFD/experimental methodology for erosion prediction. Wear 2015, 332–333, 1090–1097. [Google Scholar] [CrossRef]
- Toor, I.U.; Irshad, H.M.; Badr, H.M.; Samad, M.A. The effect of impingement velocity and angle variation on the erosion corrosion performance of API 5L-X65 carbon steel in a flow loop. Metals 2018, 8, 402. [Google Scholar] [CrossRef] [Green Version]
- Zhao, W.; Wang, C.; Zhang, T.; Yang, M.; Han, B.; Neville, A. Effects of laser surface melting on erosion–corrosion of X65 steel in liquid–solid jet impingement conditions. Wear 2016, 362–363, 39–52. [Google Scholar] [CrossRef] [Green Version]
- Zhang, G.A.; Cheng, Y.F. Electrochemical characterization and computational fluid dynamics simulation of flow-accelerated corrosion of X65 steel in a CO2-saturated oilfield formation water. Corros. Sci. 2010, 52, 2716–2724. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhou, F.; Yao, J.; Dong, S.; Li, N. Erosion–corrosion behavior and corrosion resistance of AISI 316 stainless steel in flow jet impingement. Wear 2015, 328–329, 464–474. [Google Scholar] [CrossRef]
- Aribo, S.; Barker, R.; Hu, X.; Neville, A. Erosion–corrosion behaviour of lean duplex stainless steels in 3.5% NaCl solution. Wear 2013, 302, 1602–1608. [Google Scholar] [CrossRef]
- Ukpai, J.I.; Barker, R.; Hu, X.; Neville, A. Exploring the erosive wear of X65 carbon steel by acoustic emission method. Wear 2013, 301, 370–382. [Google Scholar] [CrossRef]
- Karimi, S.; Shirazi, S.A.; McLaury, B.S. Predicting fine particle erosion utilizing computational fluid dynamics. Wear 2017, 376–377, 1130–1137. [Google Scholar] [CrossRef]
- Pasha, A.; Ghasemi, H.M.; Neshati, J. Study of the pitting corrosion of superduplex stainless steel and X-65 carbon steel during erosion-corrosion by cyclic polarization technique. Corros. Eng. Sci. Technol. 2016, 51, 463–471. [Google Scholar] [CrossRef]
- Azarian, N.S.; Ghasemi, H.M.; Monshi, M.R. Synergistic erosion and corrosion behavior of AA5052 aluminum alloy in 3.5 wt% nacl solution under various impingement angles. J. Bio-Tribo-Corros. 2015, 1, 1–10. [Google Scholar] [CrossRef] [Green Version]
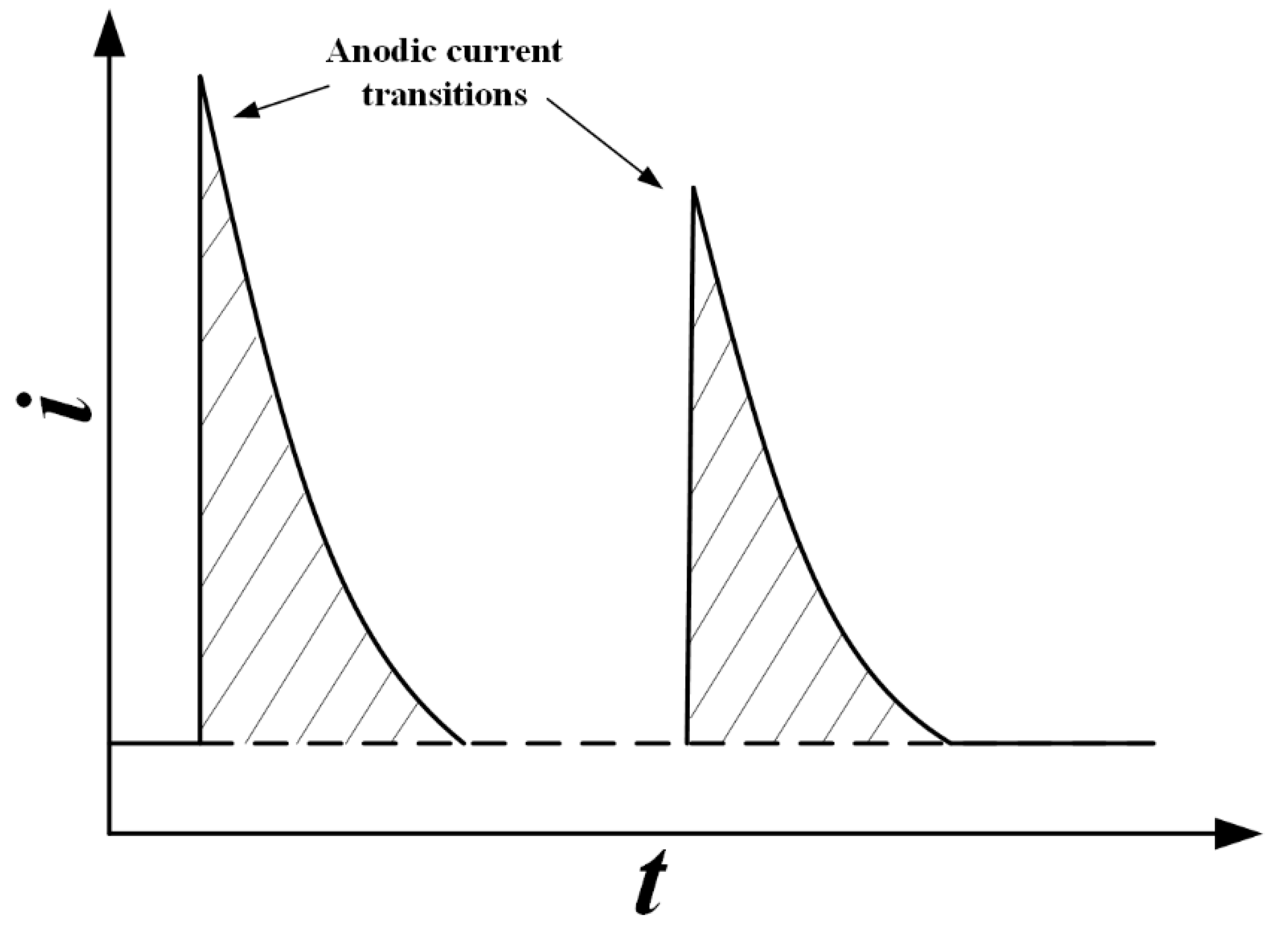
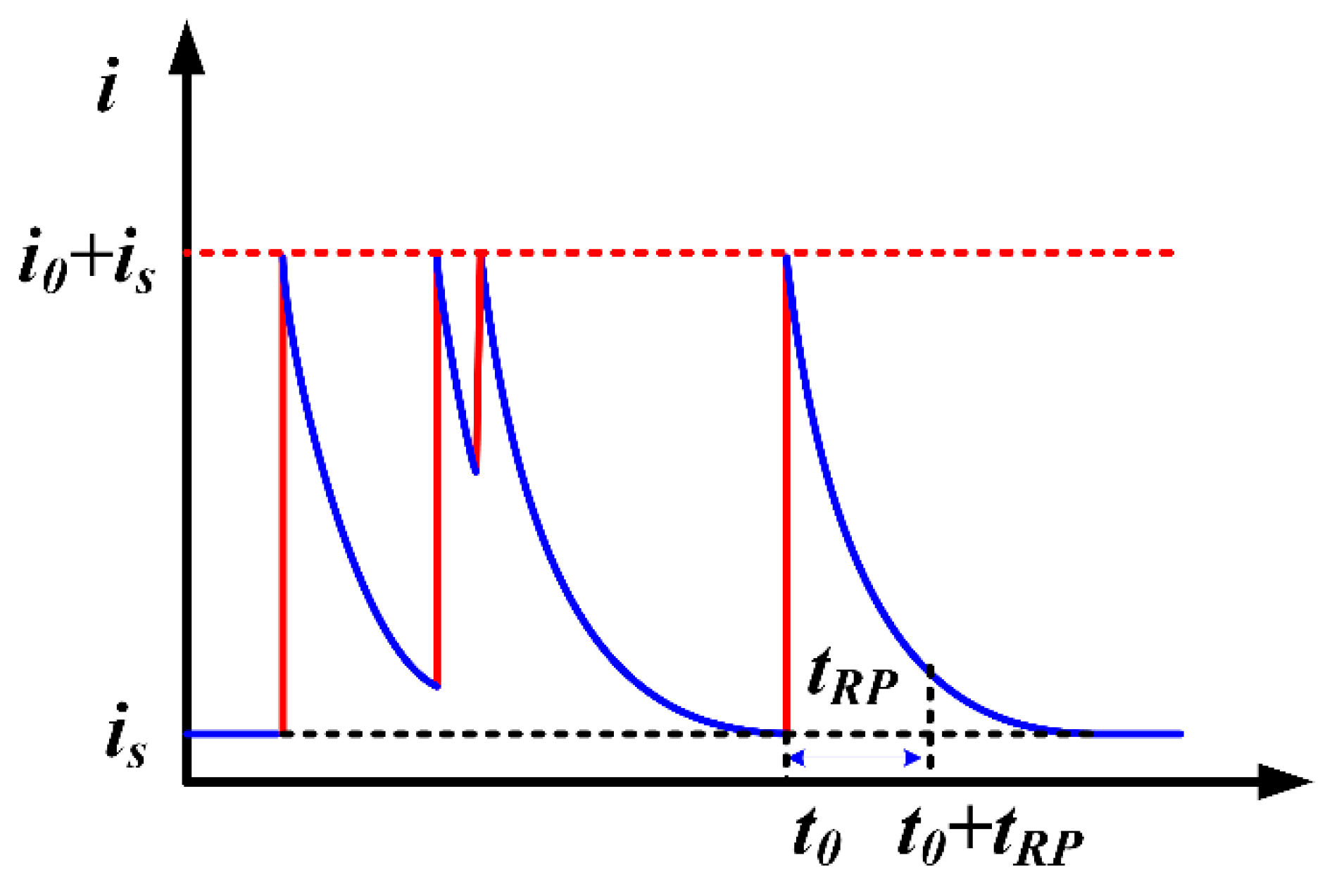
- Mohammadi, F.; Luo, J.; Lu, B.; Afacan, A. Single particle impingement current transients for prediction of erosion-enhanced corrosion on 304 stainless steel. Corros. Sci. 2010, 52, 2331–2340. [Google Scholar] [CrossRef]
- Yi, J.Z.; Hu, H.X.; Wang, Z.B.; Zheng, Y.G. On the critical flow velocity for erosion-corrosion in local eroded regions under liquid-solid jet impingement. Wear 2019, 422–423, 94–99. [Google Scholar] [CrossRef]
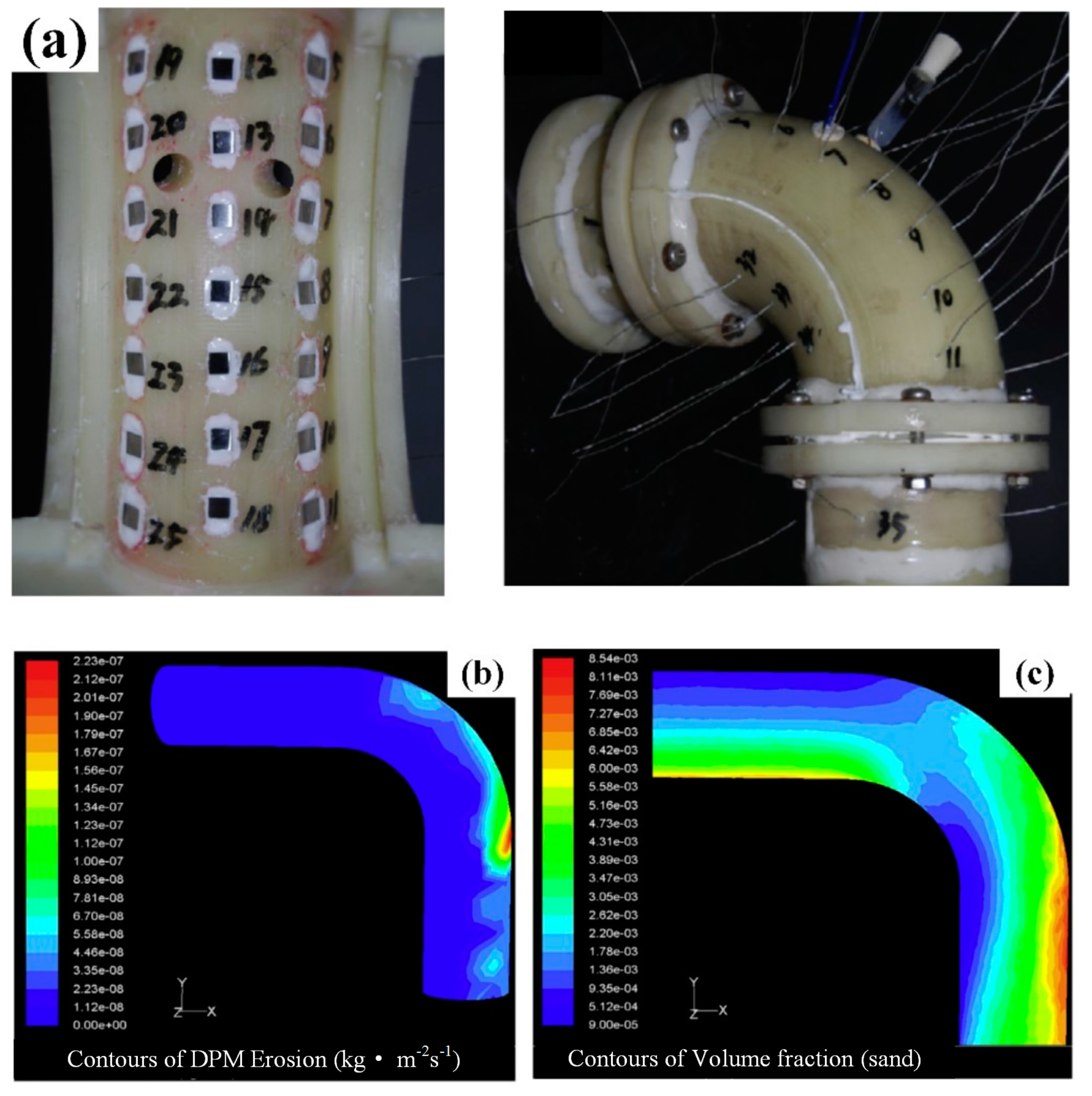
- Zhang, G.A.; Zeng, L.; Huang, H.L.; Guo, X.P. A study of flow accelerated corrosion at elbow of carbon steel pipeline by array electrode and computational fluid dynamics simulation. Corros. Sci. 2013, 77, 334–341. [Google Scholar] [CrossRef]
- Zeng, L.; Guo, X.P.; Zhang, G.A.; Chen, H.X. Semiconductivities of passive films formed on stainless steel bend under erosion-corrosion conditions. Corros. Sci. 2018, 144, 258–265. [Google Scholar] [CrossRef]
- Zeng, L.; Shuang, S.; Guo, X.P.; Zhang, G.A. Erosion-corrosion of stainless steel at different locations of a 90° elbow. Corros. Sci. 2016, 111, 72–83. [Google Scholar] [CrossRef]
- Li, Q.; Hu, H.; Cheng, Y.F. Corrosion of pipelines in CO2-saturated oil-water emulsion flow studied by electrochemical measurements and computational fluid dynamics modeling. J. Petrol Sci. Eng. 2016, 147, 408–415. [Google Scholar] [CrossRef]
- Owen, J.; Ducker, E.; Huggan, M.; Ramsey, C.; Neville, A.; Barker, R. Design of an elbow for integrated gravimetric, electrochemical and acoustic emission measurements in erosion-corrosion pipe flow environments. Wear 2019, 428–429, 76–84. [Google Scholar] [CrossRef]
- Liu, L.; Xu, Y.; Xu, C.; Wang, X.; Huang, Y. Detecting and monitoring erosion-corrosion using ring pair electrical resistance sensor in conjunction with electrochemical measurements. Wear 2019, 428–429, 328–339. [Google Scholar] [CrossRef]
- Orlikowski, J.; Darowicki, K.; Mikołajski, S. Multi-sensor monitoring of the corrosion rate and the assessment of the efficiency of a corrosion inhibitor in utility water installations. Sens. Actuators B: Chem. 2013, 181, 22–28. [Google Scholar] [CrossRef]
- Ryl, J.; Darowicki, K. Impedance monitoring of carbon steel cavitation erosion under the influence of corrosive factors. J. Electrochem. Soc. 2008, 155, 44. [Google Scholar] [CrossRef]
- Vieira, R.E.; Parsi, M.; Zahedi, P.; McLaury, B.S.; Shirazi, S.A. Electrical resistance probe measurements of solid particle erosion in multiphase annular flow. Wear 2017, 382–383, 15–28. [Google Scholar] [CrossRef]
- Fan, C.L.; Mclaury, B.S. Evaluation of electrical resistance probes to detect pipeline erosion and sand production in low liquid loading flow conditions. In Proceedings of the SPE International Conference, Columbus, OH, USA, 17–19 August 2011. [Google Scholar]
- Gan, F.; Tian, G.; Wan, Z.; Liao, J.; Li, W. Investigation of pitting corrosion monitoring using field signature method. Measurement 2016, 82, 46–54. [Google Scholar] [CrossRef]
- Huang, Y.; Xu, Y.; Li, B.; Ying, L.; Yang, F.; Wang, X. Novel electrical resistance method to measure underdeposit corrosion and its inhibition in pipeline steels. Corros. Eng. Sci. Technol. 2016, 51, 211–222. [Google Scholar] [CrossRef]
- Xu, Y.; Huang, Y.; Wang, X.; Lin, X. Experimental study on pipeline internal corrosion based on a new kind of electrical resistance sensor. Sens. Actuators B Chem. 2016, 224, 37–47. [Google Scholar] [CrossRef]
- Zhang, Y.; Reuterfors, E.P.; McLaury, B.S.; Shirazi, S.A.; Rybicki, E.F. Comparison of computed and measured particle velocities and erosion in water and air flows. Wear 2007, 263, 330–338. [Google Scholar] [CrossRef]
- Prosek, T.; Taube, M.; Dubois, F.; Thierry, D. Application of automated electrical resistance sensors for measurement of corrosion rate of copper, bronze and iron in model indoor atmospheres containing short-chain volatile carboxylic acids. Corros. Sci. 2014, 87, 376–382. [Google Scholar] [CrossRef]
- Xu, Y.; Li, K.; Liu, L.; Yang, L.; Wang, X.; Huang, Y. Experimental study on rebar corrosion using the galvanic sensor combined with the electronic resistance technique. Sensors 2016, 16, 1451. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Cheng, Y.F. Parametric effects on the erosion–corrosion rate and mechanism of carbon steel pipes in oil sands slurry. Wear 2012, 276–277, 141–148. [Google Scholar] [CrossRef]
- Birkin, P.R.; Barber, J.L. Particle induced surface erosion–Tumbling and direct impact; a high-speed electrochemical, acoustic and visual study. Wear 2019, 428–429, 147–153. [Google Scholar] [CrossRef] [Green Version]
- Droubi, M.G.; Reuben, R.L. Monitoring acoustic emission (AE) energy of abrasive particle impacts in a slurry flow loop using a statistical distribution model. Appl. Acoust. 2016, 113, 202–209. [Google Scholar] [CrossRef] [Green Version]
- Stern, M. Electrochemical polarization 2. Ferrous-Ferric electrode kinetics on stainless steel. J. Electrochem. Soc. 1957, 104, 559–563. [Google Scholar] [CrossRef]
- Sasaki, K.; Burstein, G.T. Erosion–corrosion of stainless steel under impingement by a fluid jet. Corros. Sci. 2007, 49, 92–102. [Google Scholar] [CrossRef]
- Burstein, G.T.; Organ, R.M. Repassivation and pitting of freshly generated aluminium surfaces in acidic nitrate solution. Corros. Sci. 2005, 47, 2932–2955. [Google Scholar] [CrossRef]
- Wang, Y.; Xing, Z.Z.; Luo, Q.; Rahman, A.; Jiao, J.; Qu, S.J.; Zheng, Y.G.; Shen, J. Corrosion and erosion–corrosion behaviour of activated combustion high-velocity air fuel sprayed Fe-based amorphous coatings in chloride-containing solutions. Corros. Sci. 2015, 98, 339–353. [Google Scholar] [CrossRef]
- Zheng, Z.B.; Zheng, Y.G.; Zhou, X.; He, S.Y.; Sun, W.H.; Wang, J.Q. Determination of the critical flow velocities for erosion–corrosion of passive materials under impingement by NaCl solution containing sand. Corros. Sci. 2014, 88, 187–196. [Google Scholar] [CrossRef]
- Zheng, Z.B.; Zheng, Y.G.; Sun, W.H.; Wang, J.Q. Effect of applied potential on passivation and erosion–corrosion of a Fe-based amorphous metallic coating under slurry impingement. Corros. Sci. 2014, 82, 115–124. [Google Scholar] [CrossRef]
- Zheng, Z.B.; Zheng, Y.G. Effects of surface treatments on the corrosion and erosion-corrosion of 304 stainless steel in 3.5% NaCl solution. Corros. Sci. 2016, 112, 657–668. [Google Scholar] [CrossRef]
- Wang, Z.B.; Zheng, Y.G.; Yi, J.Z. The role of surface film on the critical flow velocity for erosion-corrosion of pure titanium. Tribol. Int. 2019, 133, 67–72. [Google Scholar] [CrossRef]
- Yi, J.Z.; Hu, H.X.; Wang, Z.B.; Zheng, Y.G. Comparison of critical flow velocity for erosion-corrosion of six stainless steels in 3.5 wt% NaCl solution containing 2 wt% silica sand particles. Wear 2018, 416–417, 62–71. [Google Scholar] [CrossRef]
- Burstein, G.T.; Sasaki, K. The birth of corrosion pits as stimulated by slurry erosion. Corros. Sci. 2000, 42, 841–860. [Google Scholar] [CrossRef]
- Laleh, M.; Hughes, A.E.; Xu, W.; Gibson, I.; Tan, M.Y. Unexpected erosion-corrosion behaviour of 316L stainless steel produced by selective laser melting. Corros. Sci. 2019, 155, 67–74. [Google Scholar] [CrossRef]
- Poulson, B. Electrochemical measurements in flowing solutions. Corros. Sci. 1983, 23, 391–430. [Google Scholar] [CrossRef]
- Hoseinieh, S.M.; Homborg, A.M.; Shahrabi, T.; Mol, J.M.C.; Ramezanzadeh, B. A novel approach for the evaluation of under deposit corrosion in marine environments using combined analysis by electrochemical impedance spectroscopy and electrochemical noise. Electrochim. Acta 2016, 217, 226–241. [Google Scholar] [CrossRef]
- Wu, J.W.; Bai, D.; Baker, A.P.; Li, Z.H.; Liu, X.B. Electrochemical techniques correlation study of on-line corrosion monitoring probes. Mater. Corros. 2015, 66, 143–151. [Google Scholar] [CrossRef]
- Xu, Y.; Tan, M.Y. Visualising the dynamic processes of flow accelerated corrosion and erosion corrosion using an electrochemically integrated electrode array. Corros. Sci. 2018, 139, 438–443. [Google Scholar] [CrossRef]
- Xu, Y.Z.; Liu, L.; Zhou, Q.P.; Wang, X.N.; Huang, Y. Understanding the influences of pre-corrosion on the erosion-corrosion performance of pipeline steel. Wear 2020, 442–443, 203151. [Google Scholar] [CrossRef]
- Xie, J.; Alpas, A.T.; Northwood, D.O. The role of heat treatment on the erosion–corrosion behavior of AISI 52100 steel. Mater. Sci. Eng. A 2005, 393, 42–50. [Google Scholar] [CrossRef]
- Islam, M.A.; Farhat, Z.N. Effect of impact angle and velocity on erosion of API X42 pipeline steel under high abrasive feed rate. Wear 2014, 311, 180–190. [Google Scholar] [CrossRef]
- Islam, M.A.; Alam, T.; Farhat, Z.N.; Mohamed, A.; Alfantazi, A. Effect of microstructure on the erosion behavior of carbon steel. Wear 2015, 332–333, 1080–1089. [Google Scholar] [CrossRef]
- Xie, Y.; Jiang, J.J.; Islam, M.A. Elastomers and plastics for resisting erosion attack of abrasive/erosive slurries. Wear 2019, 426–427, 612–619. [Google Scholar] [CrossRef]
- Islam, M.A.; Farhat, Z. Erosion-corrosion mechanism and comparison of erosion-corrosion performance of API steels. Wear 2017, 376–377, 533–541. [Google Scholar] [CrossRef]
- Aminul Islam, M.; Farhat, Z.N.; Ahmed, E.M.; Alfantazi, A.M. Erosion enhanced corrosion and corrosion enhanced erosion of API X-70 pipeline steel. Wear 2013, 302, 1592–1601. [Google Scholar] [CrossRef]
- Islam, M.A.; Jiang, J.J.; Xie, Y.; Fiala, P. Investigation of erosion-corrosion behavior of (WTi)C based weld overlays. Wear 2017, 390–391, 155–165. [Google Scholar] [CrossRef]
- Islam, M.A.; Farhat, Z.N. The influence of porosity and hot isostatic pressing treatment on wear characteristics of cast and P/M aluminum alloys. Wear 2011, 271, 1594–1601. [Google Scholar] [CrossRef]
- Haseeb, A.S.M.A.; Islam, M.A.; Bepari, M.M.A. Tribological behaviour of quenched and tempered, and austempered ductile iron at the same hardness level. Wear 2000, 244, 15–19. [Google Scholar] [CrossRef]
- Islam, M.A. Erosion, Corrosion and Erosion-Corrosion of Oil and Gas Pipeline Steels. Ph.D. Thesis, Dalhousie University, Halifax, NS, Canada, 2015. [Google Scholar]
- Islam, M.A.; Farhat, Z.N. Mechanical and electrochemical synergism of API X42 pipeline steel during erosion–corrosion. J. Bio-Tribo-Corros. 2015, 1, 1–26. [Google Scholar]
- Burstein, G.T.; Liu, C. Depassivation current transients measured between identical twin microelectrodes in open circuit. Corros. Sci. 2008, 50, 2–7. [Google Scholar] [CrossRef]
- Wharton, J.A.; Wood, R.J.K. Influence of flow conditions on the corrosion of AISI 304L stainless steel. Wear 2004, 256, 525–536. [Google Scholar] [CrossRef]
- Xu, Y.Z.; He, L.M.; Yang, L.J.; Wang, X.N.; Huang, Y. Electrochemical study of steel corrosion in saturated calcium hydroxide solution with chloride ions and sulfate ions. Corrosion 2018, 74, 1063–1082. [Google Scholar] [CrossRef]
- Burstein, G.T.; Sasaki, K. Effect of impact angle on the slurry erosion–corrosion of 304L stainless steel. Wear 2000, 240, 80–94. [Google Scholar] [CrossRef]
- Wei, L.; Pang, X.; Gao, K. Effect of flow rate on localized corrosion of X70 steel in supercritical CO2 environments. Corros. Sci. 2018, 136, 339–351. [Google Scholar] [CrossRef]
- Song, G.D.; Jeon, S.; Son, Y.; Kim, J.G.; Hur, D.H. Galvanic effect of magnetite on the corrosion behavior of carbon steel in deaerated alkaline solutions under flowing conditions. Corros. Sci. 2018, 131, 71–80. [Google Scholar] [CrossRef]
- Sasaki, K.; Burstein, G.T. Observation of a threshold impact energy required to cause passive film rupture during slurry erosion of stainless steel. Phil. Mag. Lett. 2000, 80, 489–493. [Google Scholar] [CrossRef]
- Hu, X.; Neville, A. An examination of the electrochemical characteristics of two stainless steels (UNS S32654 and UNS S31603) under liquid–solid impingement. Wear 2004, 256, 537–544. [Google Scholar] [CrossRef]
- Lindsley, B.A.; Marder, A.R. The effect of velocity on the solid particle erosion rate of alloys. Wear 1999, 225, 510–516. [Google Scholar] [CrossRef]
- Guo, H.; Lu, B.; Luo, J. Response of surface mechanical properties to electrochemical dissolution determined by in situ nanoindentation technique. Electrochem. Commun. 2006, 8, 1092–1098. [Google Scholar] [CrossRef]










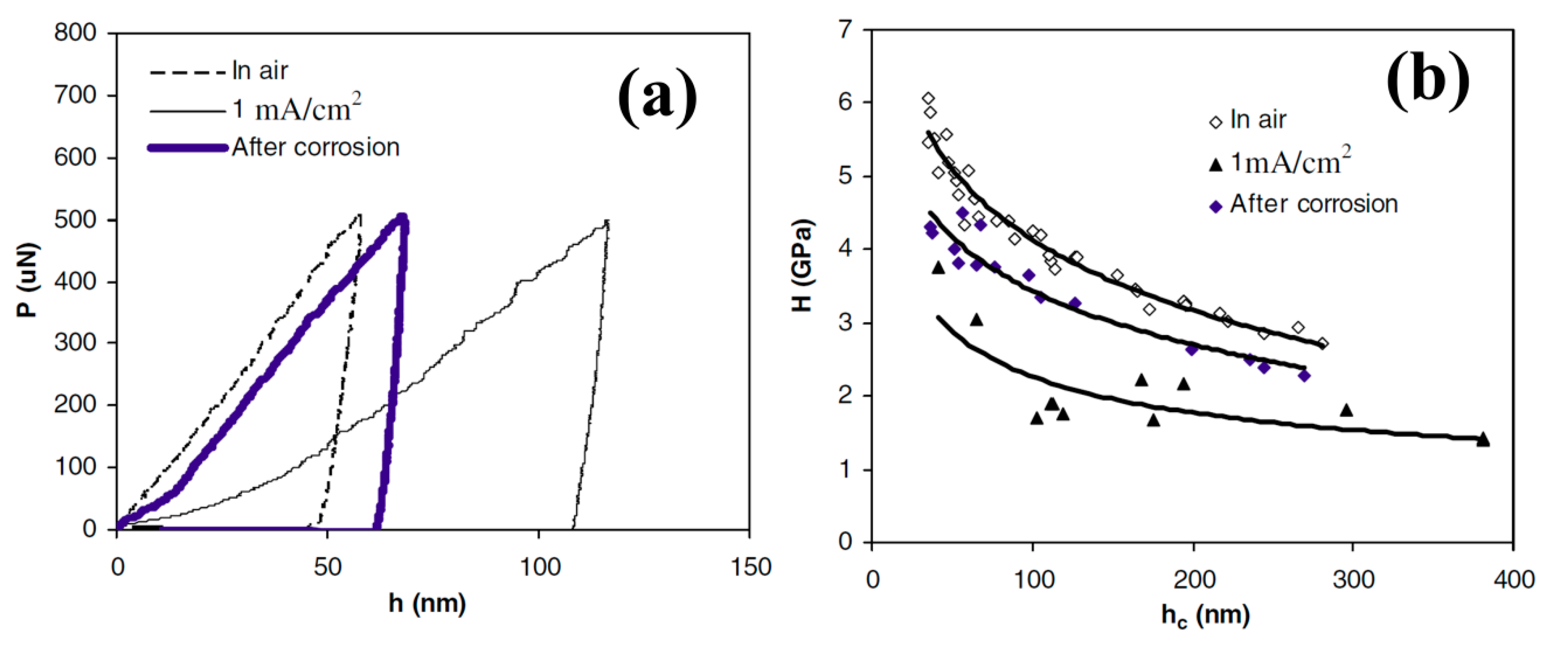
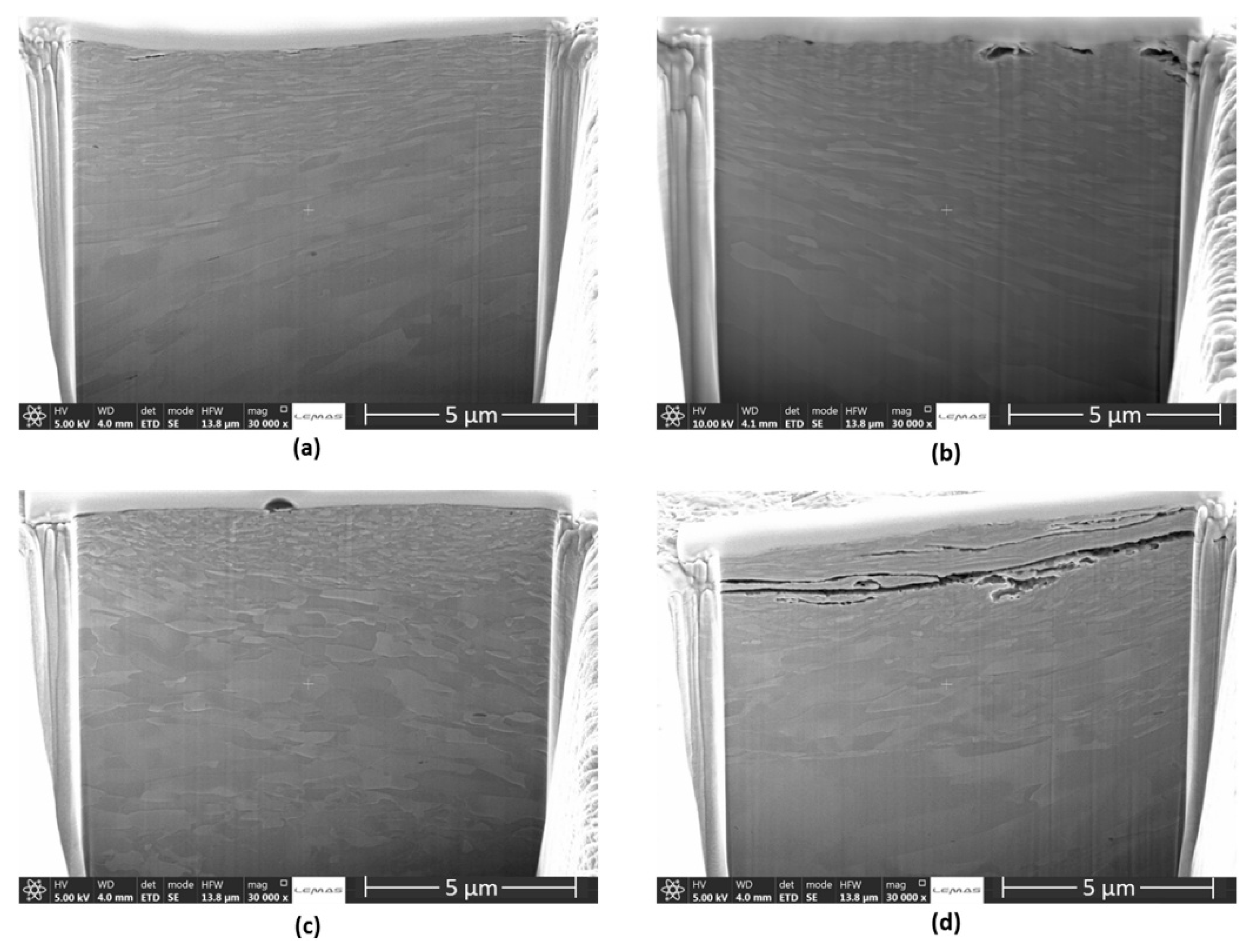
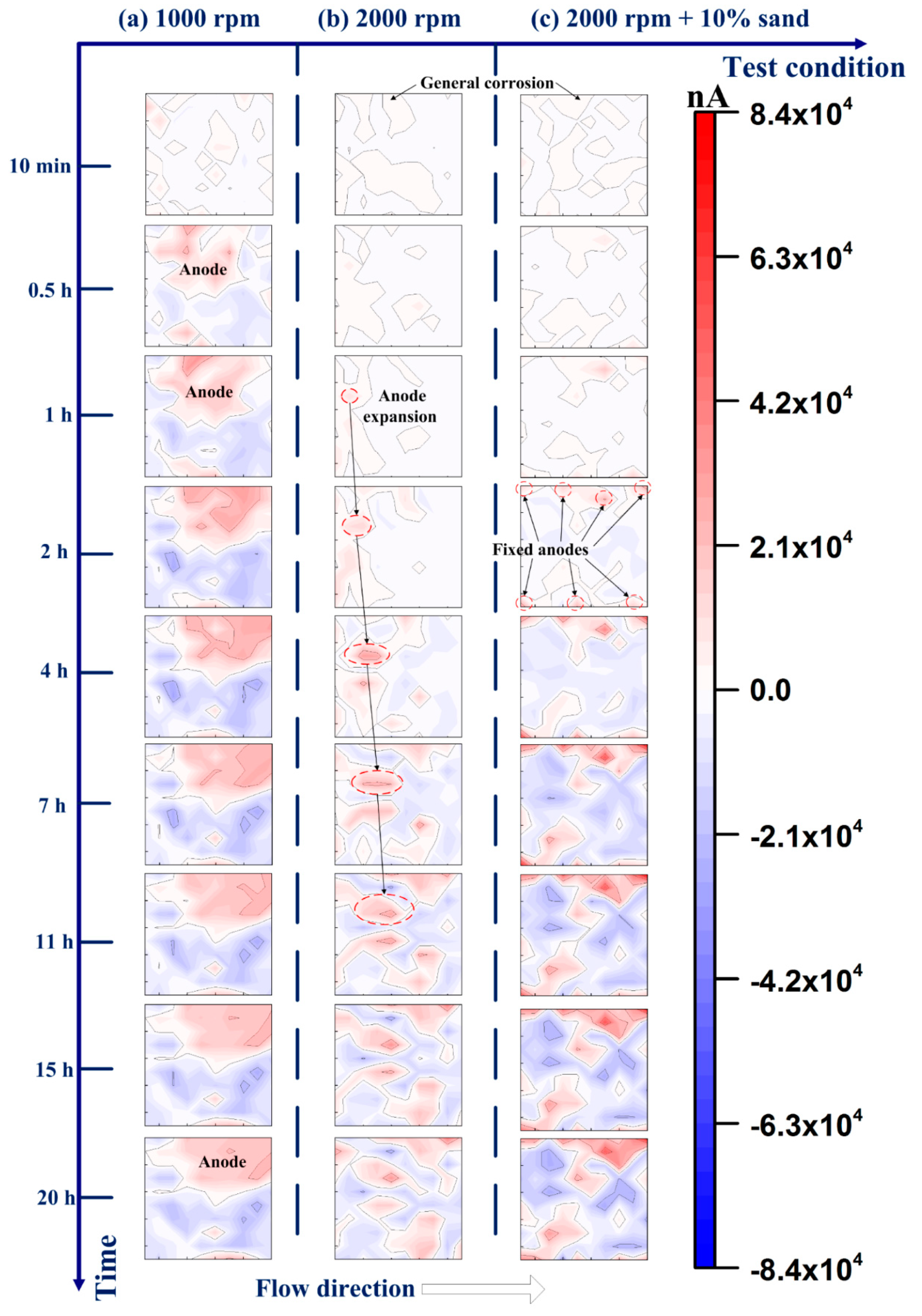
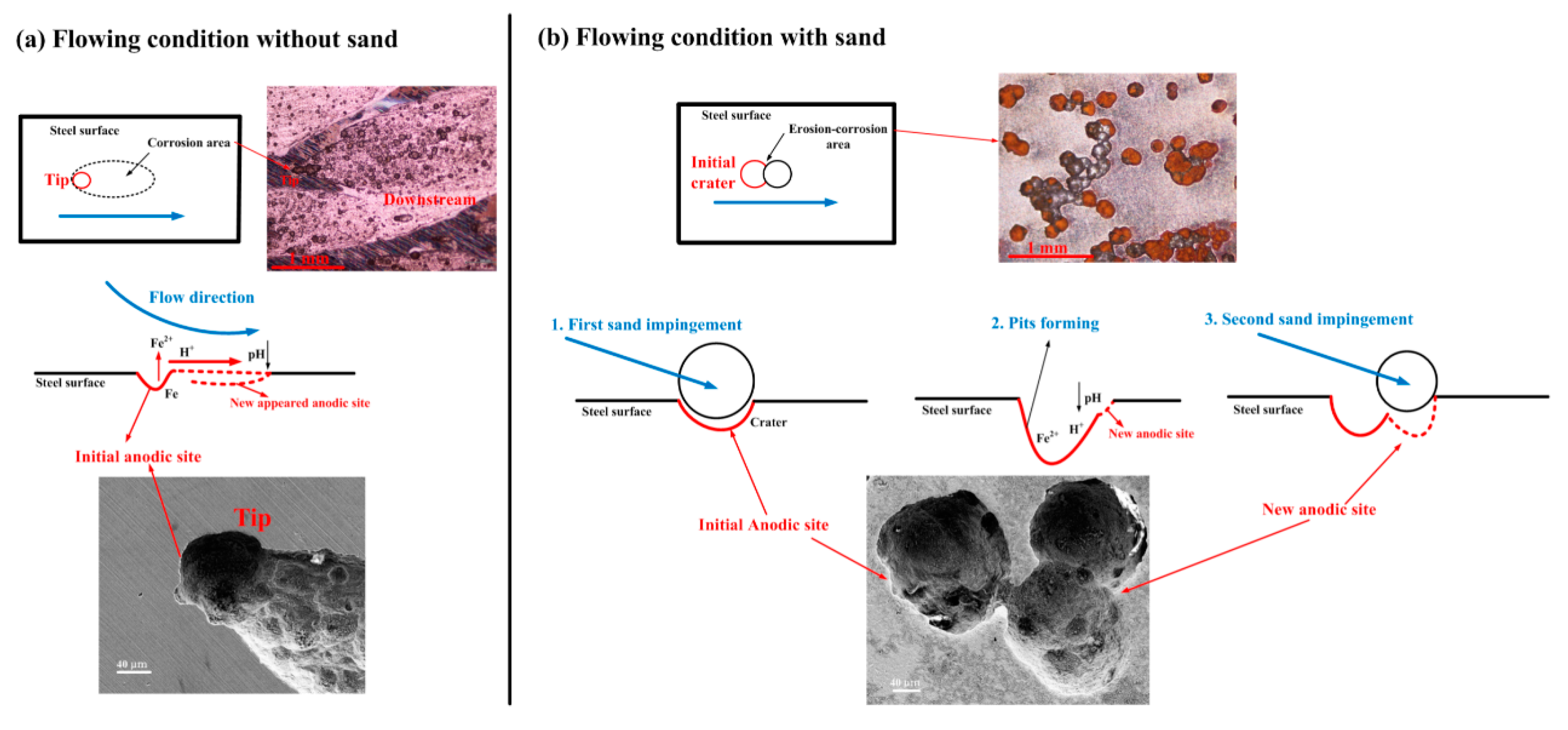
| Materials | Vp (m/s) | Vr (m/s) | Vm (m/s) |
|---|---|---|---|
| 2Cr13 SS | 12 | 11–13 | 11–13 |
| 304 SS | 12 | 11–13 | 11–13 |
| 316 SS | 12 | 12–14 | 12–14 |
| 2205 DSS | 13 | 12–14 | 12–14 |
| 2507 DSS | 13 | 12–14 | 12–14 |
| 254 SMO SS | 14 | 13–15 | 13–15 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, Y.; Liu, L.; Zhou, Q.; Wang, X.; Tan, M.Y.; Huang, Y. An Overview of Major Experimental Methods and Apparatus for Measuring and Investigating Erosion-Corrosion of Ferrous-Based Steels. Metals 2020, 10, 180. https://doi.org/10.3390/met10020180
Xu Y, Liu L, Zhou Q, Wang X, Tan MY, Huang Y. An Overview of Major Experimental Methods and Apparatus for Measuring and Investigating Erosion-Corrosion of Ferrous-Based Steels. Metals. 2020; 10(2):180. https://doi.org/10.3390/met10020180
Chicago/Turabian StyleXu, Yunze, Liang Liu, Qipiao Zhou, Xiaona Wang, Mike Yongjun Tan, and Yi Huang. 2020. "An Overview of Major Experimental Methods and Apparatus for Measuring and Investigating Erosion-Corrosion of Ferrous-Based Steels" Metals 10, no. 2: 180. https://doi.org/10.3390/met10020180
APA StyleXu, Y., Liu, L., Zhou, Q., Wang, X., Tan, M. Y., & Huang, Y. (2020). An Overview of Major Experimental Methods and Apparatus for Measuring and Investigating Erosion-Corrosion of Ferrous-Based Steels. Metals, 10(2), 180. https://doi.org/10.3390/met10020180







