Molten Steel Flow, Heat Transfer and Inclusion Distribution in a Single-Strand Continuous Casting Tundish with Induction Heating
Abstract
1. Introduction
2. Model Description
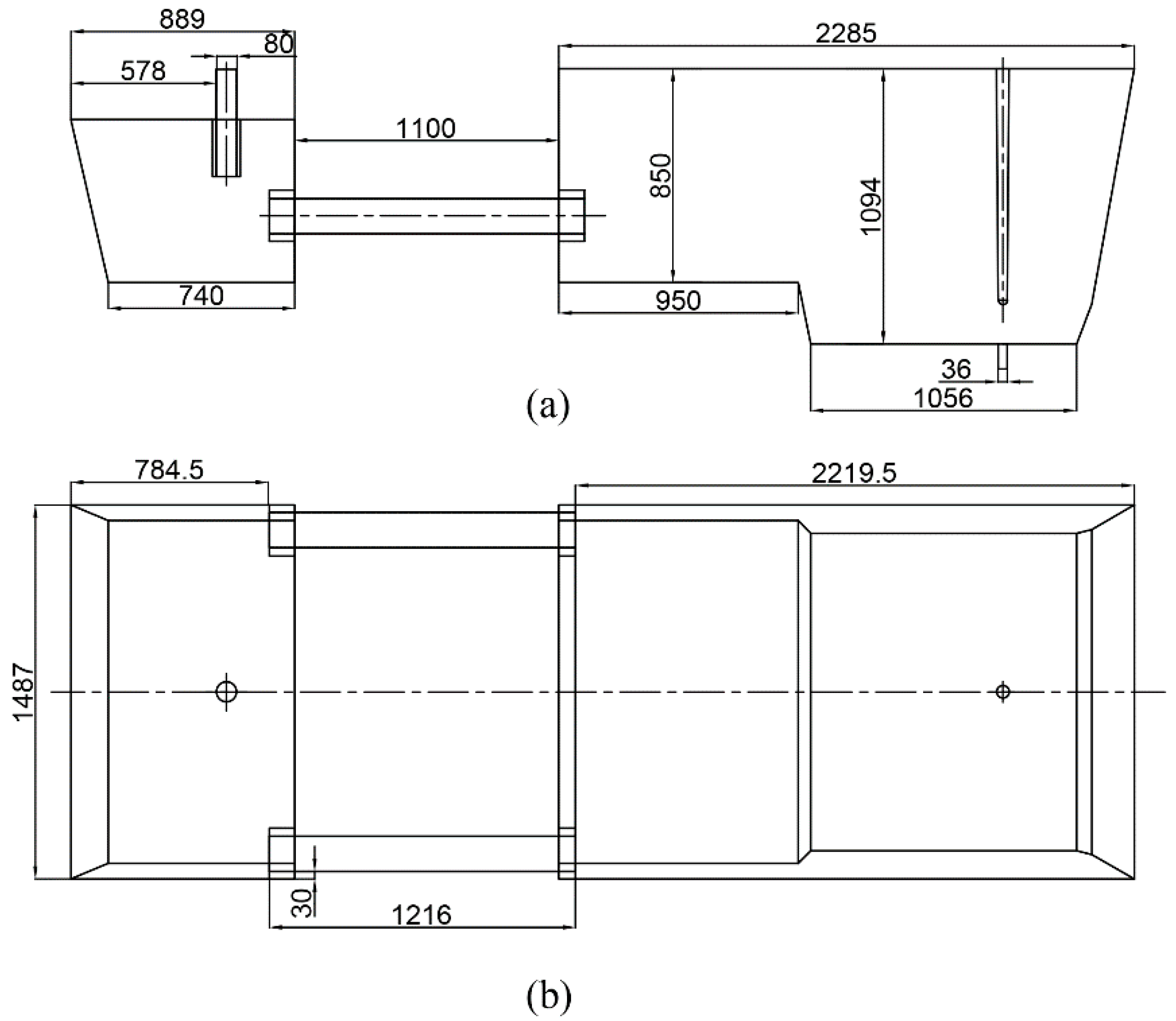
2.1. Main Dimensions of Model
2.2. Mathematical Modeling and Methodology
2.2.1. Fluid Flow and Heat Transfer Model
2.2.2. Heat Transfer Model
2.2.3. Boundary Condition and Parameters
- (1)
- The tundish inlet is at speed entry and the inlet turbulence intensity is 8%. The inlet boundary condition is set as velocity magnitude, the outlet is set as the outflow boundary condition, zero shear stress is applied to the free surface of the tundish and no-slip boundary conditions are set to the other walls.
- (2)
- The constant value of the inlet temperature of molten steel is 1800 K.
- (3)
- The molten steel dynamic viscosity is constant 0.0061 kg/(m·s), specific heat is 750 J/(kg·K), thermal conductivity is 41 W/(m·K) [19].
- (4)
2.2.4. Solution Method
2.2.5. Model Validation
3. Results and Discussion
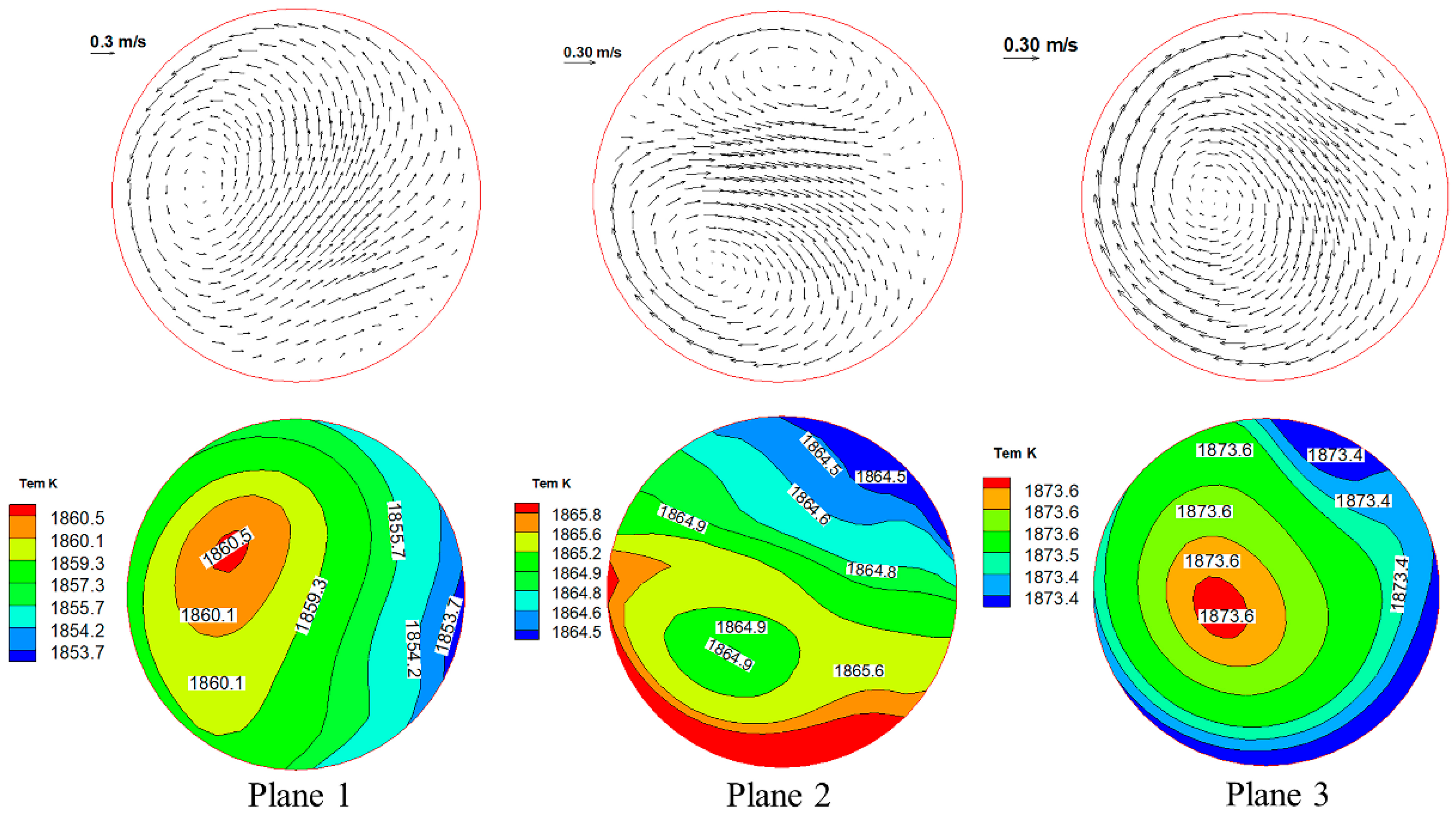
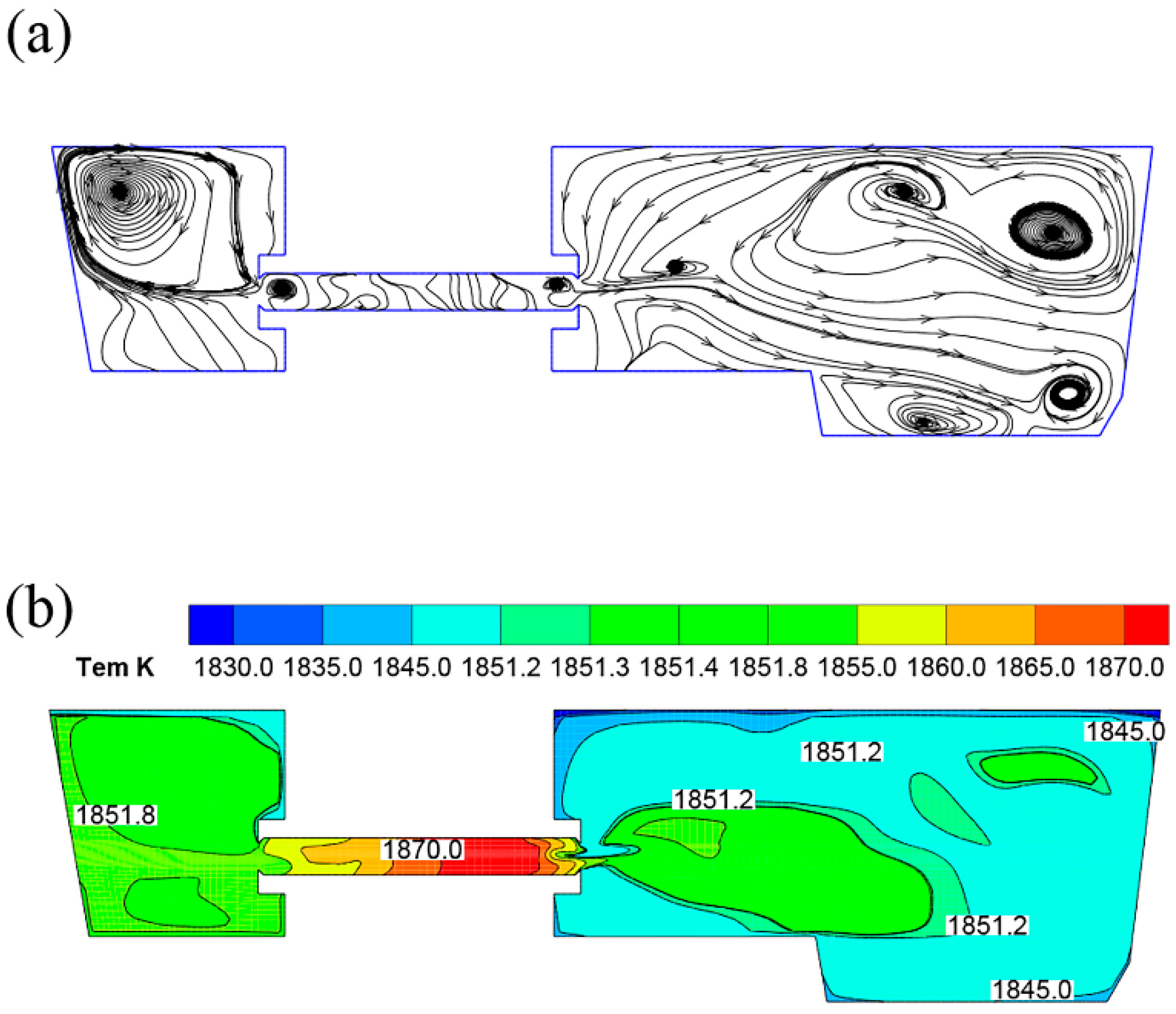
3.1. Velocity and Temperature Field
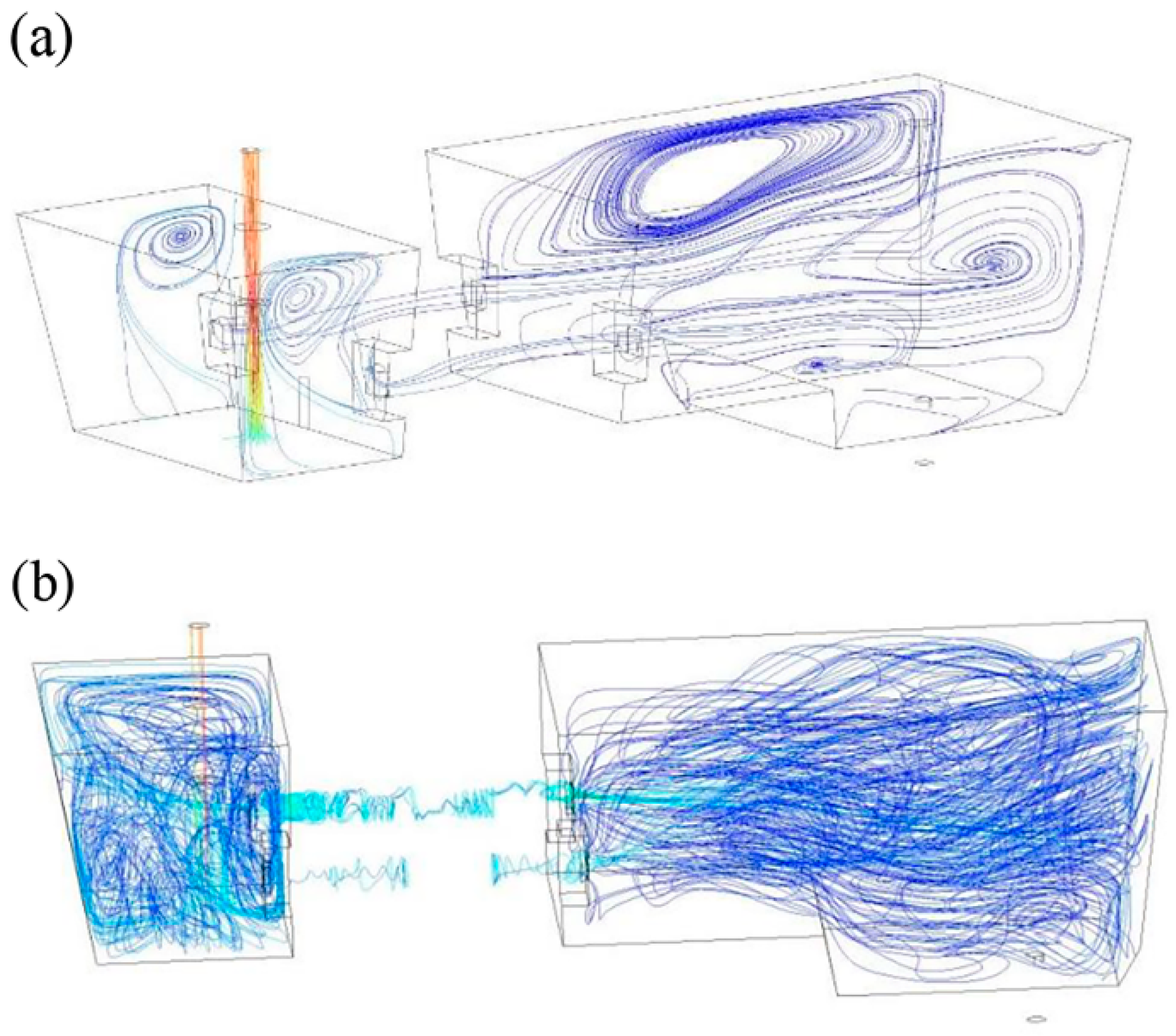
3.2. Path Lines of Flow Field
3.3. Industrial Trials
4. Conclusions
- (1)
- The molten steel in the channel changes from straight flow to spiral flow under the electromagnetic field. The flow characteristics of molten steel in the channel change with time, showing an alternating flow of single vortex and double vortex.
- (2)
- The heat loss of the molten steel in the tundish with IH can be compensated, and the temperature of the molten steel in the channel increases by 31.8 K after heating for 145 s. The temperature of molten steel in the tundish can keep the target value at 1813 ± 3 K after using the electromagnetic IH equipment in industrial trials.
- (3)
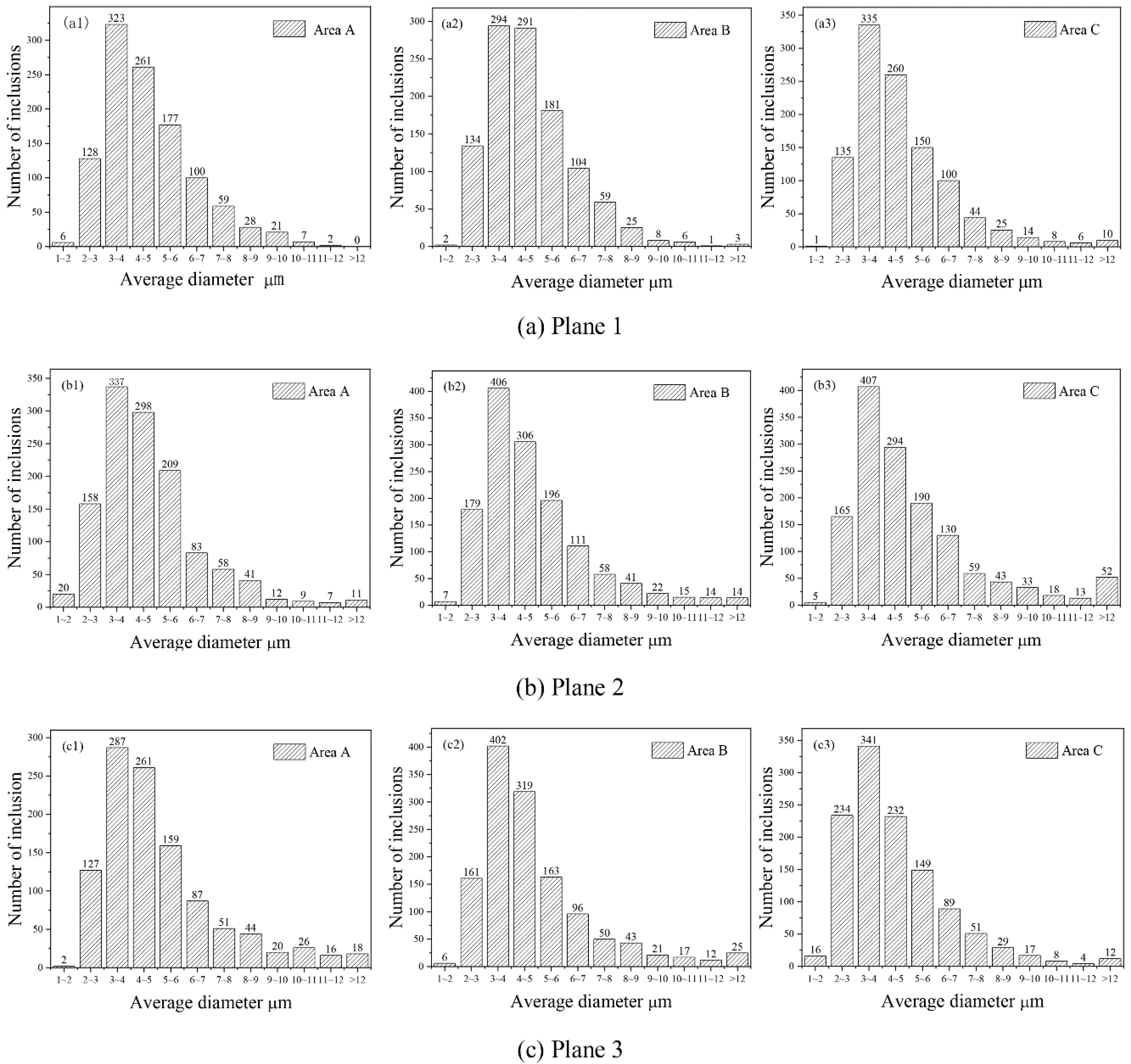
- The electromagnetic field has little effect on the inclusions with diameters less than 9 μm in the molten steel in the channel of the IH tundish. The number of inclusions with diameters greater than 9 μm at the edge of the channel is greater than that at the center and other positions of the IH tundish channel.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Morales, R.D.; Guarneros, J.; Chattopadhyay, K.; Nájera-Bastida, A.; Rodríguez, J. Fluid flow control in a billet tundish during steel filling operations. Metals 2019, 9, 394. [Google Scholar] [CrossRef]
- Cwudziński, A. Influence of subflux turbulence controller and ladle shroud asymmetric using on hydrodynamic conditions in one strand slab tundish. Metals 2019, 9, 68. [Google Scholar] [CrossRef]
- Zhang, L. Fluid flow, heat transfer and inclusion motion in a four-strand billet continuous casting tundish. Steel Res. Int. 2005, 76, 784–796. [Google Scholar] [CrossRef]
- Ilegbusi, O.J.; Szekely, J. Transport phenomena in tundishes—heat-transfer and the role of auxiliary heating. Steel Res. 1991, 62, 193–200. [Google Scholar] [CrossRef]
- Sheng, D.Y.; Zou, Z. Application of tanks-in-series model to characterize non-ideal flow regimes in continuous casting tundish. Metals 2021, 11, 208. [Google Scholar] [CrossRef]
- Huang, W.; Chang, S.; Zou, Z.; Shao, L.; Qu, Y.; Li, B. Modeling of flow behaviors in a swirling flow tundish for the deep cleaning of molten steel. Steel Res. Int. 2021. [Google Scholar] [CrossRef]
- Zhao, M.; Wang, Y.; Yang, S.; Li, J.; Song, Z. Flow behavior and heat transfer of molten steel in a two-strand tundish heated by plasma. J. Mater. Res. Technol. 2021, 13, 561–572. [Google Scholar] [CrossRef]
- Tang, H.; Wang, K.; Li, X.; Liu, J.; Zhang, J. Improved metallurgical effect of tundish through a novel induction heating channel for multistrand casting. Metals 2021, 11, 1075. [Google Scholar] [CrossRef]
- Cwudziński, A. New Insight on Liquid steel microalloying by pulse-step method in two-strand slab tundish by numerical simulations. Crystals 2021, 11, 448. [Google Scholar] [CrossRef]
- Vives, C. Electromagnetic refining of aluminum alloys by the CREM process: Part II. Specific practical problems and their solutions. Metall. Trans. B 1989, 20, 631–643. [Google Scholar] [CrossRef]
- Garnier, M. Electromagnetic processing of liquid materials in Europe. ISIJ Int. 1990, 30, 1–7. [Google Scholar] [CrossRef]
- Kishida, Y.; Takeda, K.; Miyoshino, I.; Takeuchi, E. Anisotropic effect of magnetohydrodynamics on metal solidification. ISIJ Int. 1990, 30, 34–40. [Google Scholar] [CrossRef][Green Version]
- Yue, Q.; Pei, X.; Zhang, C.; Wang, X. Magnetohydrodynamic calculation on double-loop channel induction tundish. Arch. Metall. Mater. 2018, 63, 329–336. [Google Scholar]
- Yang, B.; Lei, H.; Bi, Q.; Jiang, J.M.; Zhang, H.W.; Zhao, Y.; Zhou, J.A. Electromagnetic conditions in a tundish with channel type induction heating. Steel Res. Int. 2018, 89, 1800145. [Google Scholar] [CrossRef]
- Wang, Q.; He, M.; Zhu, X.W.; Li, X.L.; Wu, C.L.; Dong, S.L.; Liu, T. Study and development on numerical simulation for application of electromagnetic field technology in metallurgical processes. Acta Metall. Sin. 2017, 54, 228–246. [Google Scholar]
- Taniguchi, S.; Brimacombe, J.K. Application of pinch force to the separation of inclusion particles from liquid steel. ISIJ Int. 1994, 34, 722–731. [Google Scholar] [CrossRef]
- Ghojel, J.I. Thermal analysis of twin-channel induction furnaces. Metall. Mater. Trans. B 2003, 34, 679–684. [Google Scholar] [CrossRef]
- Pak, Y.A.; Filippov, G.; Yusupov, D.; Tyuftyaev, A.; Isakaev, M.K.; Sarychev, B. Two-strand tundish with chambers for plasma heating of liquid metal. Metallurgist 2014, 58, 672–676. [Google Scholar] [CrossRef]
- Wang, Q.; Li, B.K.; Tsukihashi, F. Modeling of a thermo-electromagneto-hydrodynamic problem in continuous casting tundish with channel type induction heating. ISIJ Int. 2014, 54, 311–320. [Google Scholar] [CrossRef]
- Barron-Meza, M.A.; Barreto-Sandoval, J.D.J.; Morales, R.D. Physical and mathematical models of steel flow and heat transfer in a tundish heated by plasma. Metall. Mater. Trans. B 2000, 31, 63–74. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Iwai, K.; Asai, S. Plasma behavior under imposition of alternating magnetic field perpendicular or parallel to the plasma arc current. ISIJ Int. 2007, 47, 960–964. [Google Scholar] [CrossRef][Green Version]
- Ghojel, J.I.; Ibrahim, R.N. Computer simulation of the thermal regime of double-loop channel induction furnaces. J. Mater. Process. Technol. 2004, 153–154, 386–391. [Google Scholar] [CrossRef]
- Ghojel, J.I. Modelling of electromagnetically excited turbulent flow of molten metal in a twin-channel induction furnace. Prog. Comput. Fluid Dyn. 2006, 6, 435–445. [Google Scholar] [CrossRef]
- Kirpo, M.; Jakovics, A.; Nacke, B.; Baake, E.; Langejurgen, M. LES of heat and mass exchange in induction channel furnaces. Prz. ElektroTech. 2008, 84, 154–158. [Google Scholar]
- Yue, Y.F.; Xu, Q.M.; Guo, P.; Luo, A. Constant temperature control of tundish induction heating power supply for metallurgical manufacturing. Front. Energy 2019, 13, 16–26. [Google Scholar] [CrossRef]
- Tang, H.Y.; Guo, L.Z.; Wu, G.H.; Xiao, H.; Yao, H.Y.; Zhang, J.Q. Hydrodynamic modeling and mathematical simulation on flow field and inclusion removal in a seven-strand continuous casting tundish with channel type induction heating. Metals 2018, 8, 374. [Google Scholar] [CrossRef]
- Yang, B.; Lei, H.; Bi, Q.; Jiang, J.M.; Zhang, H.W.; Zhao, Y.; Zhou, J.A. Fluid flow and heat transfer in a tundish with channel type induction heating. Steel Res. Int. 2018, 89, 1800173. [Google Scholar] [CrossRef]
- Felten, F.; Fautrelle, Y.; Du Terrail, Y.; Metais, O. Numerical modelling of electromagnetically-driven turbulent flows using LES methods. Appl. Math. Model. 2004, 28, 15–27. [Google Scholar] [CrossRef]
- Zhao, T.; Ou, S.D.; Zhou, J.M.; Xiong, J.Z. Numerical analysis on thermal regime in double-loop channel inductor. J. Cent. S. Univ. Technol. 2010, 17, 180–186. [Google Scholar] [CrossRef]
- Xing, F.; Zheng, S.G.; Zhu, M.Y. Motion and removal of inclusions in new induction heating tundish. Steel Res. Int. 2018, 89, 1700542. [Google Scholar] [CrossRef]
- Xing, F.; Zheng, S.; Liu, Z.; Zhu, M. Flow field, temperature field, and inclusion removal in a new induction heating tundish with bent channels. Metals 2019, 9, 561. [Google Scholar] [CrossRef]
- Yue, Q.; Zhang, C.B.; Pei, X.H. Magnetohydrodynamic flows and heat transfer in a twin-channel induction heating tundish. Ironmak. Steelmak. 2017, 44, 227–236. [Google Scholar] [CrossRef]
- Wang, P.; Xiao, H.; Chen, X.; He, H.; Tang, H.Y.; Zhang, J.Q. Influence of dual-channel induction heating coil parameters on the magnetic field and macroscopic transport behavior in T-type tundish. Metall. Mater. Trans B. 2021, 52, 3447–3467. [Google Scholar] [CrossRef]
- Wang, P.; Chen, X.; Xiao, H.; Li, X.; Zhang, J. Effect of flow control devices on the distribution of magnetic-flow-heat in the channel induction heating tundish. Ironmak. Steelmak. 2021, 1–11. [Google Scholar] [CrossRef]
- Zhang, Q.; Xu, G.; Iwai, K. Effective removal zone of inclusions in a horizontal channel under A.C. magnetic field imposition. ISIJ Int. 2021, 61, 42–48. [Google Scholar] [CrossRef]
- Wang, Q.; Qi, F.S.; Li, B.K.; Tsukihashi, F. Behavior of non-metallic inclusions in a continuous casting tundish with channel type induction heating. ISIJ Int. 2014, 54, 2796–2805. [Google Scholar] [CrossRef]





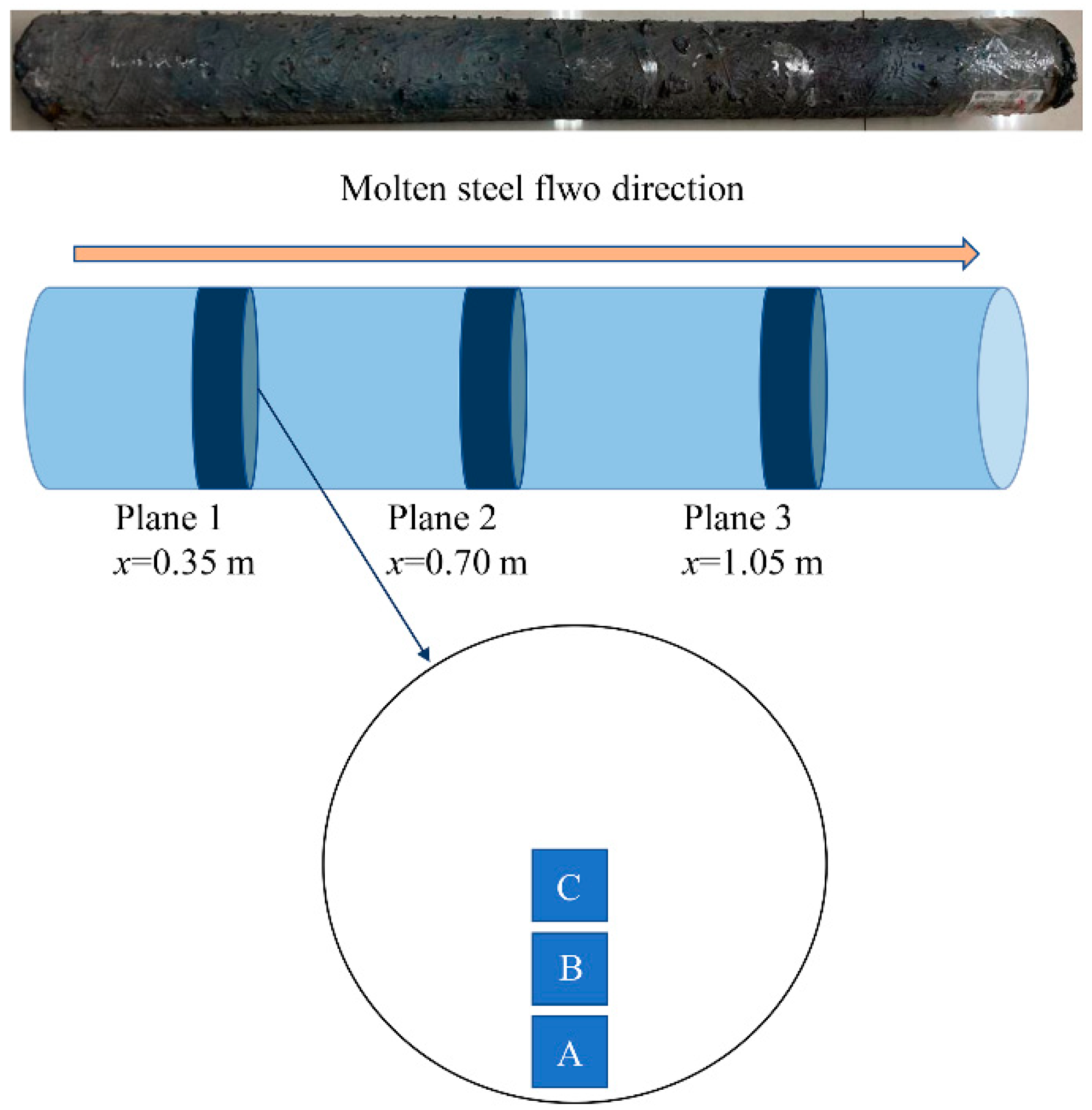
| Parameters | Value |
|---|---|
| Coefficient of thermal expansion of liquid steel, K−1 | 1.2 × 10−4 |
| Inlet velocity, m s−1 | 0.72 |
| Top wall heat flux, W m−2 | 15,000 |
| Bottom wall heat flux, W m−2 | 1800 |
| Longitudinal walls flux, W m−2 | 4600 |
| Transversal walls flux, W m−2 | 4000 |
| Channel walls flux, W m−2 | 1200 |
| Tundish IH power, kW | 900 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dou, W.; Yang, Z.; Wang, Z.; Yue, Q. Molten Steel Flow, Heat Transfer and Inclusion Distribution in a Single-Strand Continuous Casting Tundish with Induction Heating. Metals 2021, 11, 1536. https://doi.org/10.3390/met11101536
Dou W, Yang Z, Wang Z, Yue Q. Molten Steel Flow, Heat Transfer and Inclusion Distribution in a Single-Strand Continuous Casting Tundish with Induction Heating. Metals. 2021; 11(10):1536. https://doi.org/10.3390/met11101536
Chicago/Turabian StyleDou, Weixue, Zexi Yang, Ziming Wang, and Qiang Yue. 2021. "Molten Steel Flow, Heat Transfer and Inclusion Distribution in a Single-Strand Continuous Casting Tundish with Induction Heating" Metals 11, no. 10: 1536. https://doi.org/10.3390/met11101536
APA StyleDou, W., Yang, Z., Wang, Z., & Yue, Q. (2021). Molten Steel Flow, Heat Transfer and Inclusion Distribution in a Single-Strand Continuous Casting Tundish with Induction Heating. Metals, 11(10), 1536. https://doi.org/10.3390/met11101536






