Investigation of the Self-Heating of Q460 Butt Joints and an S-N Curve Modeling Method Based on Infrared Thermographic Data for High-Cycle Fatigue
Abstract
1. Introduction
- (1)
- Entropy production during HCF is mainly induced by recoverable motions (anelasticity) and irreversible microstructural changes (microplasticity);
- (2)
- Irreversible microstructural changes contribute to damage accumulation, whereas the anelasticity is regarded to be non-damaging.
2. Energy Dissipation Framework during HCF
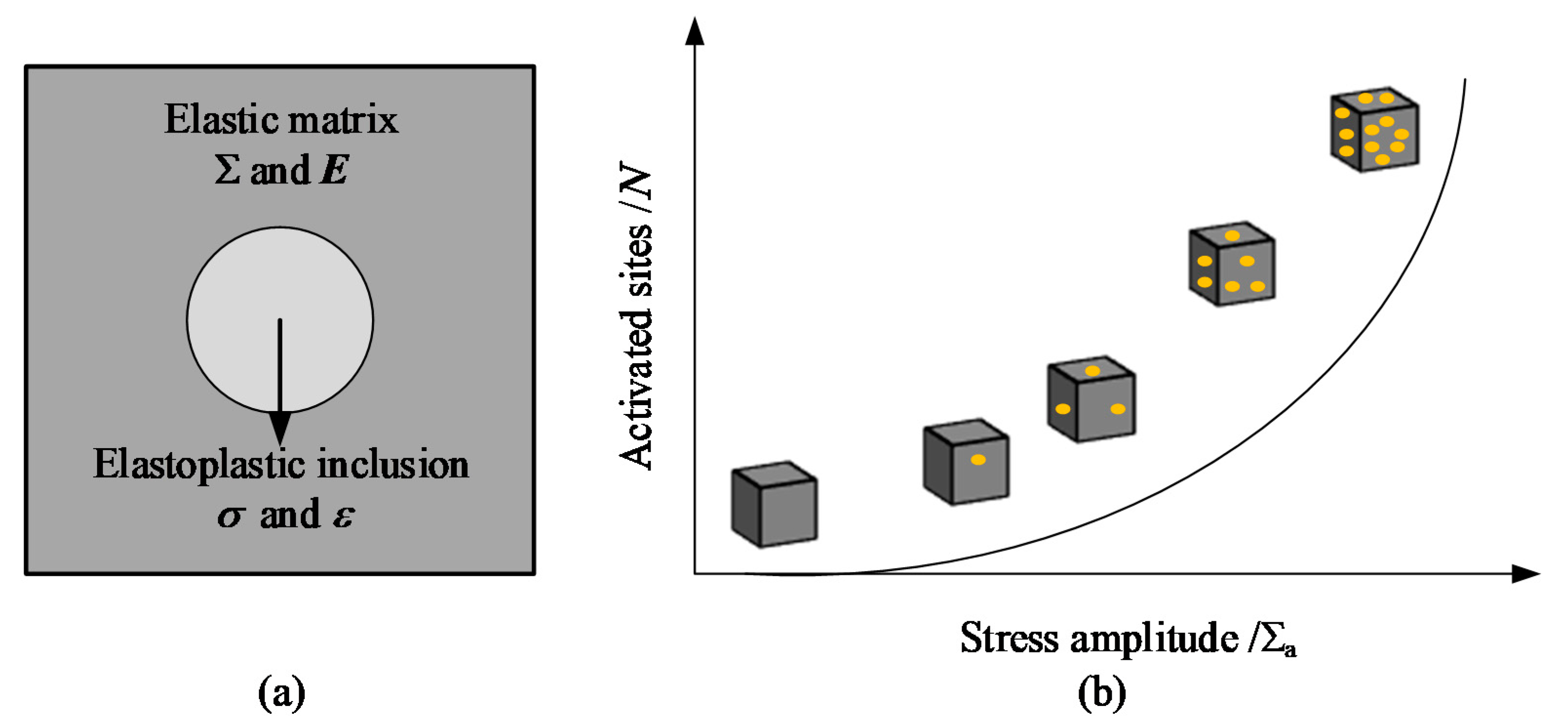
2.1. RVE Model
2.2. Intrinsic Dissipation
3. Thermodynamic Entropy
3.1. Cumulative Damage Entropy (CDE) Calculation
- (1)
- Both inelasticity/microplasticity and anelasticity evolutions are irreversible;
- (2)
- Irreversible inelastic behavior will cause energy dissipation and damage accumulation;
- (3)
- Anelastic evolution is irreversible and does not damage the material because of the recoverable microstructural motions, so the related entropy production is minimal.
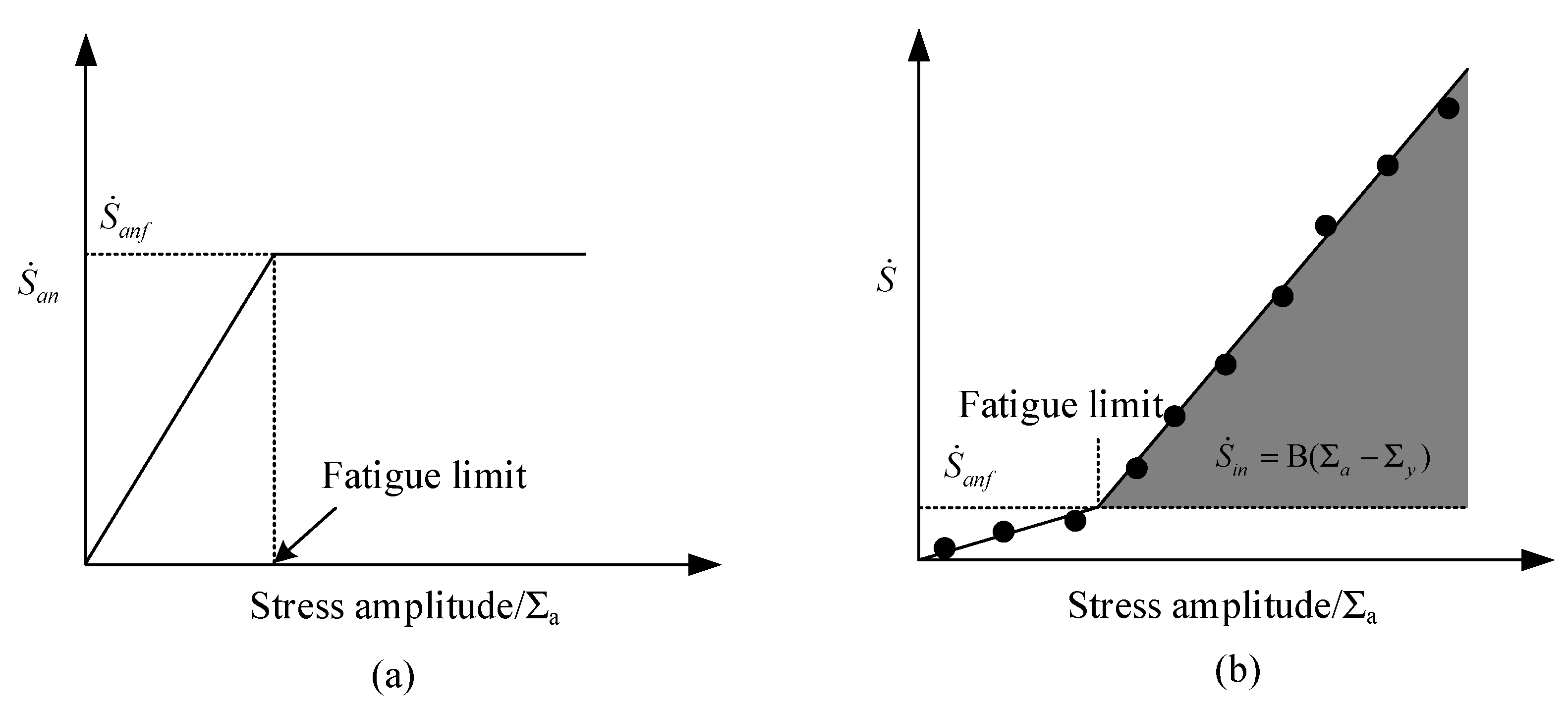
3.2. Fatigue Limit Prediction
3.3. S-N Curve Evaluation Using Thermography Data
3.4. P-S-N Curve Evaluation
- (1)
- Based on Equation (22), the predicted fatigue life lgNa(i) (i = 1, 2, …, n1) with a 50% survival probability rate under different stress levels Σa(i) can be obtained;
- (2)
- Then, the tested fatigue life lgNac(j) (j = 1, 2, …, n2) of three specimens under a certain stress amplitude Σac can be used, as presented in Section 4.3, Procedure (2), to obtain relevant parameters of the P-S-N curve.
- (1)
- Logarithmic fatigue life under different stress levels obey the normal distribution;
- (2)
- The relationship of the mean of logarithmic fatigue life μi and standard deviation σi, with any stress amplitude Σa(i), (above fatigue limit), is supposed linear.
4. Experimental Study
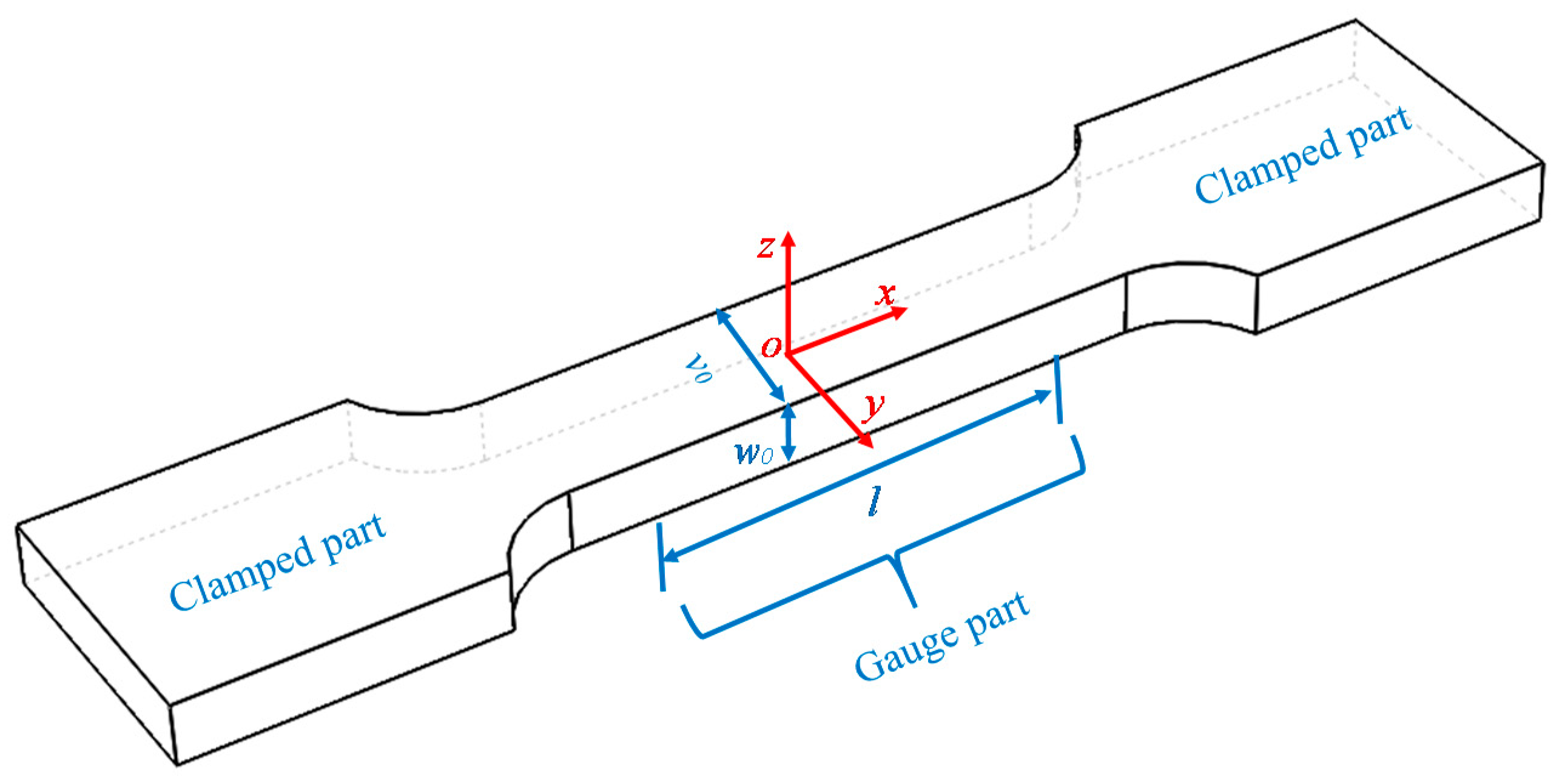
4.1. Materials and Specimens
4.2. Experimental Apparatus
4.3. Experimental Procedure
- (1)
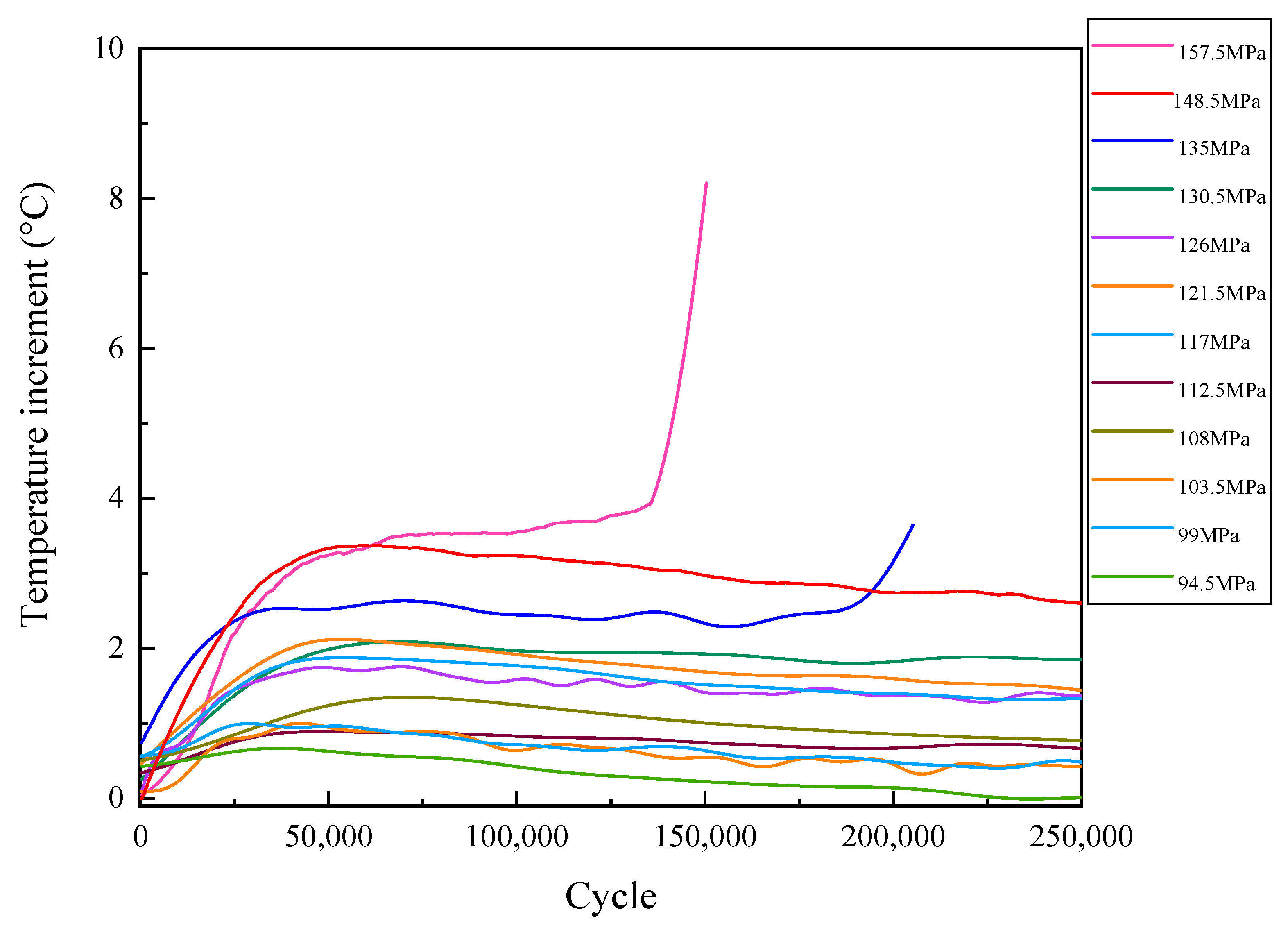
- Stress amplitudes of 157.5, 148.5, 135, 130.5, 126, 121.5, 117, 112.5, 108, 103.5, 99, and 94.5 MPa were selected to conduct fatigue tests until fracture or 2 × 106 cycles to obtain the entropy production rate in Phase II;
- (2)
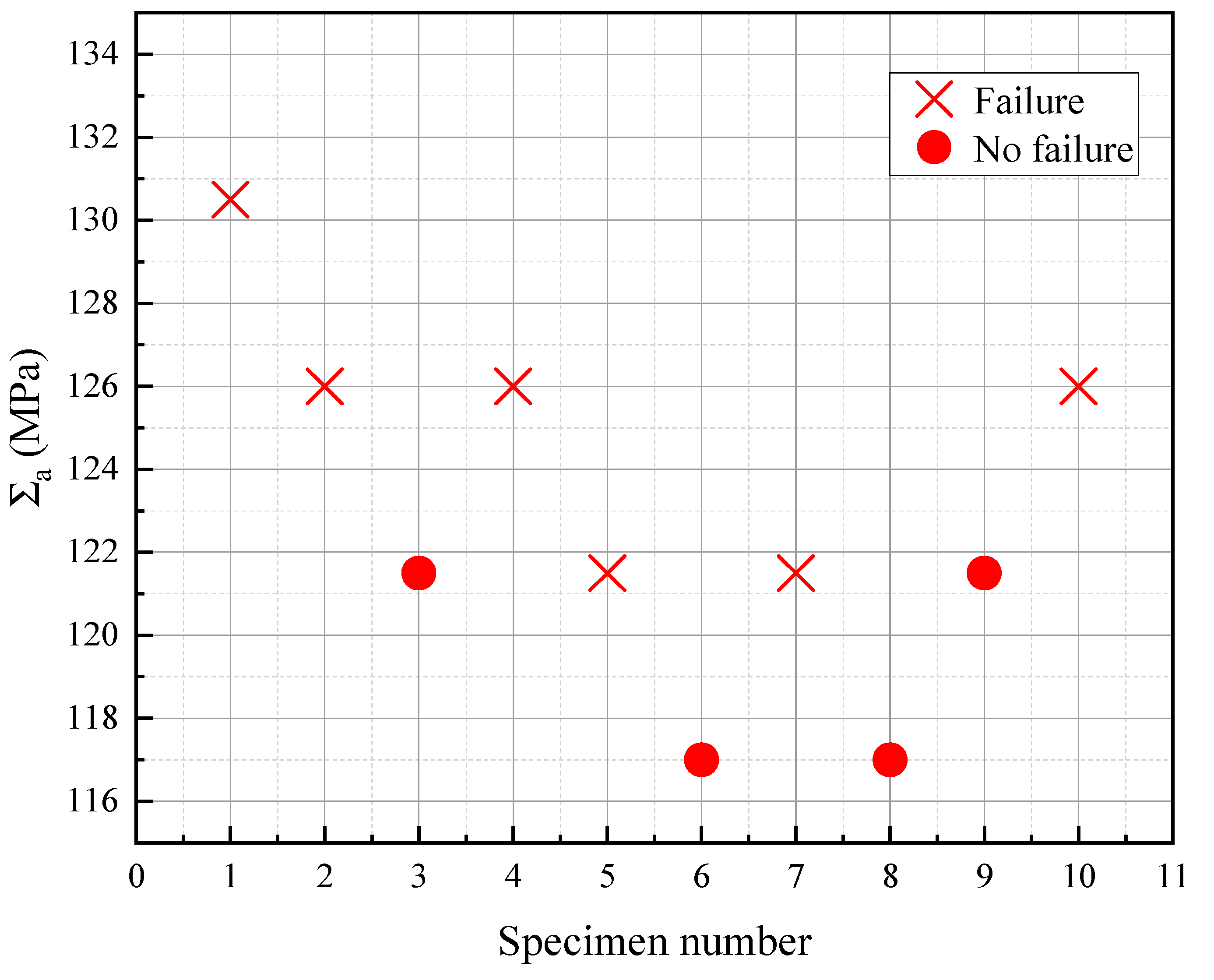
- After finishing Procedure (1), i.e., determining the fatigue limit using the method developed in Section 3.2, three specimens were tested under the stress amplitude above the fatigue limit to estimate the average CDE for S-N curve prediction;
- (3)
- The fatigue life of tested specimens in Procedure (1) and (2) was employed to verify the predicted S-N curve.
5. Results and Discussion
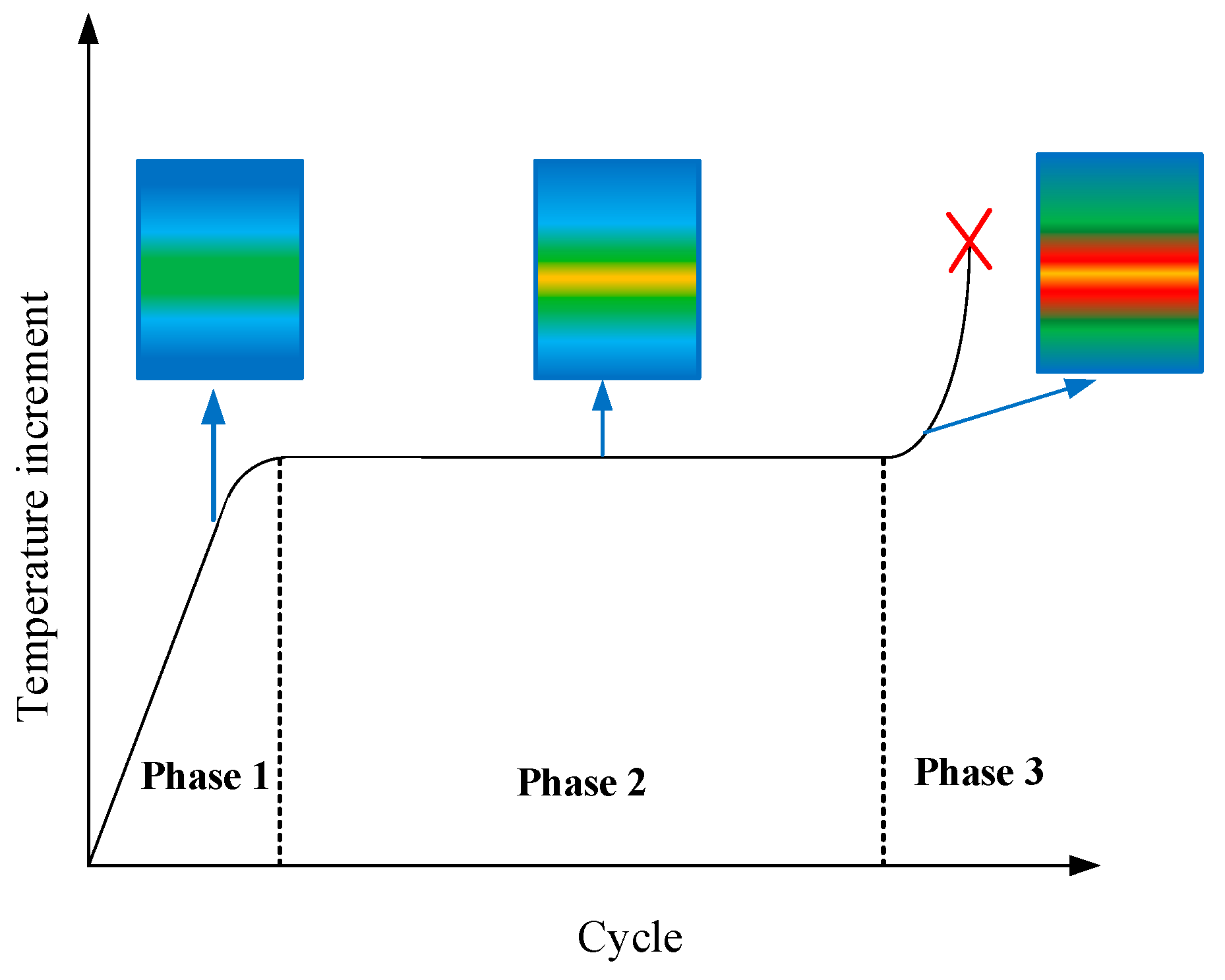
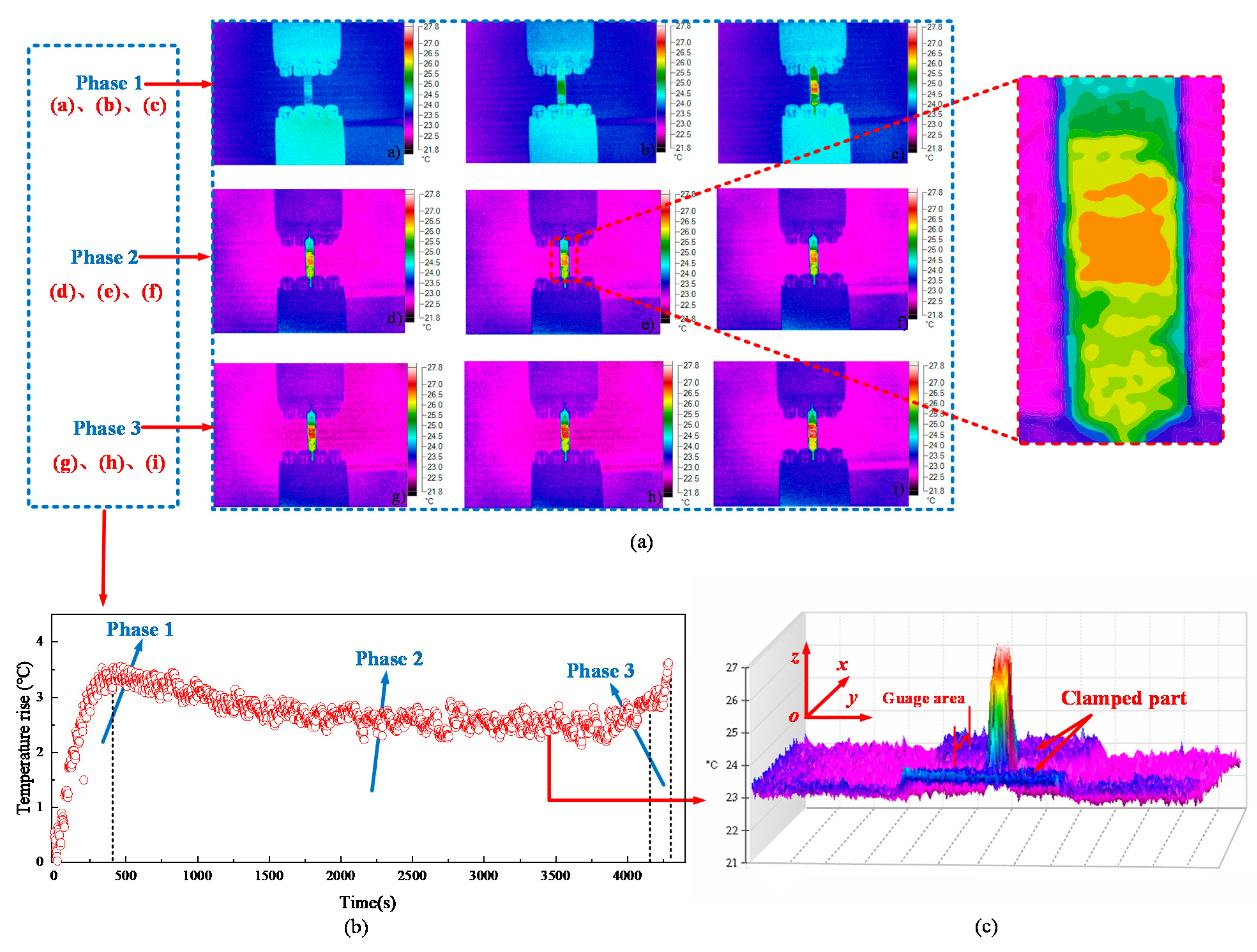
5.1. Fatigue Temperature Evolution by IR Techniques
5.2. Fatigue Limit Prediction
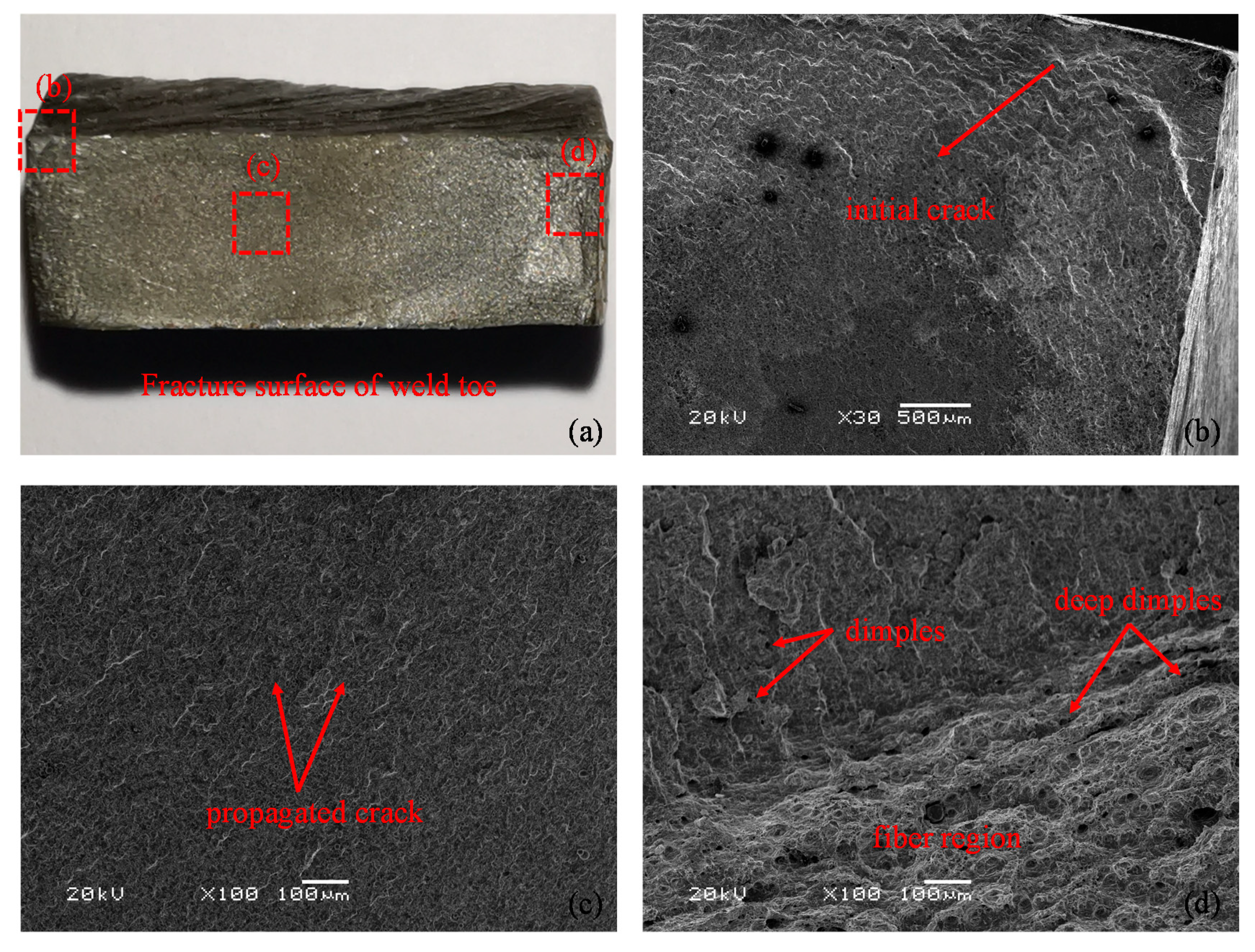
5.3. Analysis of Fatigue Fracture Mechanism
5.4. S-N Curve Estimation of Q460 Butt Joints
6. Conclusions
- (1)
- Perform fatigue tests of Q460 welded joints and simultaneously record the temperature field using an infrared camera;
- (2)
- Calculate the entropy production rate in Phase II under different stress amplitudes. Two datasets of the entropy generation rate are obtained. Fit them linearly to determine the fatigue limit;
- (3)
- Test three specimens to acquire the average CDE threshold and realize the P-S-N curve prediction combining with the maximum likelihood method. Finally, the P-S-N curve can be estimated quickly compared with the traditional method. This new method complements conventional methods toward achieving rapid S-N curve prediction of welded joints.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| B | Material coefficient |
| C | Specific heat capacity |
| CDE | Cumulative damage entropy |
| d1 | Intrinsic dissipation |
| dRVE | Microplastic energy dissipation |
| din | Inelastic dissipation |
| dan | Anelastic dissipation |
| e | Kinematic viscosity |
| F | Helmholtz free energy |
| f | Test frequency |
| g | Gravitational acceleration |
| h | Coefficient relates to radiation and convection |
| hc | The convection coefficient |
| hr | The radiation coefficient |
| HCF | High-cycle fatigue |
| Jq | Heat flux |
| k | Thermal conductivity |
| ka | Heat conduction coefficient |
| l | The gauge length |
| LCF | Low-cycle fatigue |
| n1 | The number of estimated fatigue life under different stress amplitude based on the proposed model |
| n2 | The total number of specimens under a certain stress amplitude |
| Nac | The fatigue life under a certain stress amplitude (n2) |
| Na(i) | The number of estimated fatigue life under different stress amplitude (n1) |
| re | External volumetric heat source |
| Ṡ | Entropy production rate |
| Ṡin | Entropy production rate contributed by inelastic behavior |
| Ṡan | Entropy production rate resulting from anelasticity |
| T | Real-time temperature |
| T0 | Initial temperature |
| Tb | Specimen boundary temperature |
| Tr | Room temperature |
| Tf | Film temperature |
| up | The standard normal deviation |
| v | Poisson ratio |
| v0 | The width of specimen’s gauge part |
| w0 | The thickness of specimen’s gauge part |
| α | Internal variables |
| β | Spherical inclusion based on Eshelby analysis |
| ε | Thermal emissivity |
| Strain rate | |
| η | volume friction fraction of plastic strain coefficient |
| θ | Temperature increment |
| λ | Volume expansion coefficient |
| μ | Shear modulus |
| μac | The mean of logarithmic fatigue life under Σac |
| μi | The mean of logarithmic fatigue life under Σa(i) |
| σ | Microscopic yield stress tensor |
| σ | Stefan–Boltzmann constant |
| σac | The standard deviation under Σac |
| σi | The standard deviation under Σa(i) |
| Σ | Macroscopic yield stress tensor |
| Σa | Macro stress amplitude |
| Σac | A certain stress amplitude |
| Σa(i) | Stress amplitude relates to Na(i) |
| Σy | Fatigue limit |
| ρ | Density |
| τeq | Time constant relates to thermal convection and radiation |
References
- Xiong, J.J.; Shenoi, R.A. Fatigue and Fracture Reliability Engineering; Springer Science & Business Media: London, UK, 2011. [Google Scholar]
- Gurney, T.R.; Saunders, H. Fatigue of Welded Structures (2nd Edition). Eng. Mater. Technol. 1979, 103, 185. [Google Scholar] [CrossRef][Green Version]
- Okagata, Y.; Kiriyama, K.; Kato, T. Fatigue strength evaluation of the Japanese railway wheel. Fatigue Fract. Eng. Mater. Struct. 2007, 30, 356–371. [Google Scholar] [CrossRef]
- Fricke, W.; Remes, H.; Feltz, O.; Lilleme, I.; Tchuindjang, D.; Reinert, T.; Nevierov, A.; Sichermann, W.; Brinkmann, M.; Kontkanen, T. Fatigue strength of laser-welded thin-plate ship structures based on nominal and structural hot-spot stress approach. Ships Offshore Struct. 2015, 10, 39–44. [Google Scholar] [CrossRef]
- Schijve, J. Fatigue of Structures and Materials; Kluwer Academic Publishers: AA Dordrecht, Netherlands, 2001. [Google Scholar]
- Luong, M.; Van, K.D. Metal fatigue limit evaluation using infrared thermography. In Proceedings of the Workshop Advanced Infrared Technology and Applications, Capri, Italy, 20–21 September 1993; pp. 245–253. [Google Scholar]
- Luong, M.P. Fatigue limit evaluation of metals using an infrared thermographic technique. Mech Mater 1998, 28, 155–163. [Google Scholar] [CrossRef]
- Geraci, A.; La Rosa, G.; Risitano, A. On the new methodology for the determination of the fatigue limit of materials using thermal infrared techniques. In Proceedings of the VDI IMEKO/GESA Symposium, Düsseldorf, Germany, 28–30 April 1992; pp. 183–190. [Google Scholar]
- Huang, J.; Pastor, M.-L.; Garnier, C.; Gong, X. Rapid evaluation of fatigue limit on thermographic data analysis. Int. J. Fatigue 2017, 104, 293–301. [Google Scholar] [CrossRef]
- Guo, S.; Liu, X.; Zhang, H.; Yan, Z.; Fang, H. Fatigue Limit Evaluation of AZ31B Magnesium Alloy Based on Temperature Distribution Analysis. Metals 2020, 10, 1331. [Google Scholar] [CrossRef]
- Amiri, M.; Khonsari, M.M. Life prediction of metals undergoing fatigue load based on temperature evolution. Mater. Sci. Eng. A 2010, 527, 1555–1559. [Google Scholar] [CrossRef]
- Xiao-qing, L.; Hong-xia, Z.; Zhi-feng, Y.; Wen-xian, W.; Ya-guo, Z.; Qian-ming, Z. Fatigue life prediction of AZ31B magnesium alloy and its welding joint through infrared thermography. Theor. Appl. Fract. Mech. 2013, 67-68, 46–52. [Google Scholar] [CrossRef]
- Zhang, L.; Liu, X.S.; Wu, S.H.; Ma, Z.Q.; Fang, H.Y. Rapid determination of fatigue life based on temperature evolution. Int. J. Fatigue 2013, 54, 1–6. [Google Scholar] [CrossRef]
- Acosta, R.; Wu, H.; Venkat, S.R.; Weber, F.; Tenkamp, J.; Walther, F.; Starke, P. SteBLife, a New Approach for the Accelerated Generation of Metallic Materials’ Fatigue Data. Metals 2020, 10, 798. [Google Scholar] [CrossRef]
- Amiri, M.; Khonsari, M.M. Rapid determination of fatigue failure based on temperature evolution: Fully reversed bending load. Int. J. Fatigue 2010, 32, 382–389. [Google Scholar] [CrossRef]
- Plekhov, O.; PALIN-LUC, T.; Saintier, N.; Uvarov, S.; Naimark, O. Fatigue crack initiation and growth in a 35CrMo4 steel investigated by infrared thermography. Fatigue Fract. Eng. Mater. Struct. 2010, 28, 169–178. [Google Scholar] [CrossRef]
- Wagner, D.; Ranc, N.; Bathias, C.; Paris, P.C. Fatigue crack initiation detection by an infrared thermography method. Fatigue Fract. Eng. Mater. Struct. 2009, 33, 12–21. [Google Scholar] [CrossRef]
- Yang, W.; Guo, X.; Guo, Q.; Fan, J. Rapid evaluation for high-cycle fatigue reliability of metallic materials through quantitative thermography methodology. Int. J. Fatigue 2019, 124, 461–472. [Google Scholar] [CrossRef]
- Guo, Q.; Guo, X.; Fan, J.; Wu, C. Research on high-cycle fatigue behavior of FV520B steel based on intrinsic dissipation (in chinese). Acta Metall. Sin. 2015, 51, 18–24. [Google Scholar]
- Guo, Q.; Guo, X. Research on high-cycle fatigue behavior of FV520B stainless steel based on intrinsic dissipation. Mater. Des. 2016, 90, 248–255. [Google Scholar] [CrossRef]
- Fan, J.; Zhao, Y.; Guo, X. A unifying energy approach for high cycle fatigue behavior evaluation. Mech. Mater. 2018, 120, 15–25. [Google Scholar] [CrossRef]
- Teng, Z.; Wu, H.; Boiler, C.; Starke, P. A unified fatigue life calculation based on intrinsic thermal dissipation and microplasticity evolution. Int. J. Fatigue 2020, 131, 105370. [Google Scholar] [CrossRef]
- Naderi, M.; Amiri, M.; Khonsari, M.M. On the thermodynamic entropy of fatigue fracture. Proc. R. Soc. A Math. Phys. Eng. Sci. 2010, 466, 423–438. [Google Scholar] [CrossRef]
- Naderi, M.; Khonsari, M. An experimental approach to low-cycle fatigue damage based on thermodynamic entropy. Int. J. Solids Struct. 2010, 47, 875–880. [Google Scholar] [CrossRef]
- Naderi, M.; Khonsari, M. Real-time fatigue life monitoring based on thermodynamic entropy. Struct. Health Monit. 2011, 10, 189–197. [Google Scholar] [CrossRef]
- Ribeiro, P.; Petit, J.; Gallimard, L. Experimental Determination of Entropy and Exergy in Low Cycle Fatigue. Int. J. Fatigue 2019, 136, 105333. [Google Scholar] [CrossRef]
- Salimi, H.; Pourgol-Mohammad, M.; Yazdani, M. Metal Fatigue Assessment Based on Temperature Evolution and Thermodynamic Entropy Generation. Int. J. Fatigue 2019, 127, 403–416. [Google Scholar] [CrossRef]
- Rongda, Z.; Dejun, X.; Tao, L.; Fangbin, W.; Zhutao, C. Thermodynamic Entropy Characteristics Analysis and Life Prediction Model of Metal Low Cycle Fatigue Processes (in chinese). China Mech. Eng. 2019, 30, 1904–1910. [Google Scholar]
- Wang, J.; Yao, Y. An Entropy Based Low-Cycle Fatigue Life Prediction Model for Solder Materials. Entropy 2017, 19, 503. [Google Scholar] [CrossRef]
- Mahmoudi, A.; Mohammadi, B. On the evaluation of damage-entropy model in cross-ply laminated composites. Eng. Fract. Mech. 2019, 219, 106626. [Google Scholar] [CrossRef]
- Teng, Z.; Wu, H.; Boller, C.; Starke, P. Thermodynamic entropy as a marker of high-cycle fatigue damage accumulation: Example for normalized SAE 1045 steel. Fatigue Fract. Eng. Mater. Struct. 2020, 1–13. [Google Scholar] [CrossRef]
- Huang, J.; Li, C.; Liu, W. Investigation of internal friction and fracture fatigue entropy of CFRP laminates with various stacking sequences subjected to fatigue loading. Thin-Walled Struct. 2020, 155, 106978. [Google Scholar] [CrossRef]
- Doudard, C.; Calloch, S.; Cugy, P.; Galtier, A.; Hild, F. A probabilistic two-scale model for high-cycle fatigue life predictions. Fatigue Fract. Eng. Mater. Struct. 2010, 28, 279–288. [Google Scholar] [CrossRef]
- Fan, J.L.; Guo, X.L.; Wu, C.W.; Zhao, Y.G. Research on fatigue behavior evaluation and fatigue fracture mechanisms of cruciform welded joints. Mater. Sci. Eng. A 2011, 528, 8417–8427. [Google Scholar] [CrossRef]
- Guo, Q.; Guo, X.; Fan, J.; Syed, R.; Wu, C. An energy method for rapid evaluation of high-cycle fatigue parameters based on intrinsic dissipation. Int. J. Fatigue 2015, 80, 136–144. [Google Scholar] [CrossRef]
- Corigliano, P.; Epasto, G.; Guglielmino, E.; Risitano, G. Fatigue analysis of marine welded joints by means of DIC and IR images during static and fatigue tests. Eng. Fract. Mech. 2017, 183, 26–38. [Google Scholar] [CrossRef]
- Chrysochoos, A.; Louche, H. An infrared image processing to analyse the calorific effects accompanying strain localisation. Int. J. Eng. Sci. 2000, 38, 1759–1788. [Google Scholar] [CrossRef]
- Yang, W.; Guo, X.; Guo, Q. A high-cycle fatigue energy dissipation accurate estimation method considering natural convection and radiation heat transfer. Int. J. Fatigue 2020, 138, 105717. [Google Scholar] [CrossRef]
- Holman, J.P. Heat Transfer, 10th ed.; McGraw-Hill: New York, NY, USA, 2010. [Google Scholar]
- Lemaitre, J.; Chaboche, J.-L. Mechanics of Solid Materials; Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Gao, Z. Fatigue Applied Statistics (in Chinese); National Defence Industry Press: Beijing, China, 1986. [Google Scholar]
- Gu, Y.J.; Jin, T.Z.; Zu, H.D.; Xu, J.; Chen, D.C. High-Cycle Fatigue P-S-N Curve Estimating Method Based on Maximum Likelihood Method for Turbine Coupling Bolt Materials. Appl. Mech. Mater. 2014, 541–542, 592–598. [Google Scholar] [CrossRef]
- Zhang, H.; Wu, G.; Yan, Z.; Guo, S.; Chen, P.; Wang, W. An experimental analysis of fatigue behavior of AZ31B magnesium alloy welded joint based on infrared thermography. Mater. Des. 2014, 55, 785–791. [Google Scholar] [CrossRef]
- Liu, Y. Research on Cumulative Damage Aseessment Method of Spot Welded Joints Based on Fatigue Damage Entropy; Dalian Jiaotong University: Dalian, China, 2018. [Google Scholar]
- Liu, Y.; Sun, Y.; Sun, Y.; Xu, H.; Yang, X. Rapid fatigue life prediction for spot-welded joint of SUS301 L-DLT stainless steel and Q235B carbon steel based on energy dissipation. Adv. Mech. Eng. 2018, 10, 1687814018811013. [Google Scholar] [CrossRef]
- Skibicki, D.; Lipski, A.; Pejkowski, Ł. Evaluation of plastic strain work and multiaxial fatigue life in CuZn37 alloy by means of thermography method and energy-based approaches of Ellyin and Garud. Fatigue Fract. Eng. Mater. Struct. 2018, 4, 2541–2556. [Google Scholar] [CrossRef]
- Huang, J.; Garnier, C.; Pastor, M.L.; Gong, X. Investigation of self-heating and life prediction in CFRP laminates under cyclic shear loading condition based on the infrared thermographic data. Eng. Fract. Mech. 2020, 229, 106971. [Google Scholar] [CrossRef]
- Dong, P. A Structural Stress Definition and Numerical Implementation for Fatigue Analysis of Welded Joints. Int. J. Fatigue 2001, 23, 865–876. [Google Scholar] [CrossRef]



| Material | C | Mn | Si | Ni | Mo | V | Ti | Al |
|---|---|---|---|---|---|---|---|---|
| Q460 | ≤0.16 | 1.7 | 0.6 | 0.5 | 0.2 | 0.1 | 0.05 | 0.02 ~ 0.04 |
| Material | Elastic Modulus (GPa) | Yield Strength (MPa) | Tensile Strength (MPa) | Elongation (%) |
|---|---|---|---|---|
| Q460 | 190 | 490 | 630 | 19 |
| Welding Voltage (V) | Welding Current (A) | Welding Speed (mm/s) | Welding Pass |
|---|---|---|---|
| 26 | 220 | 6.5 | 1 |
| Parameters | DM | SM | |Error| |
|---|---|---|---|
| Fatigue limit (MPa) | 111.03 | 121.5 | 8.62% |
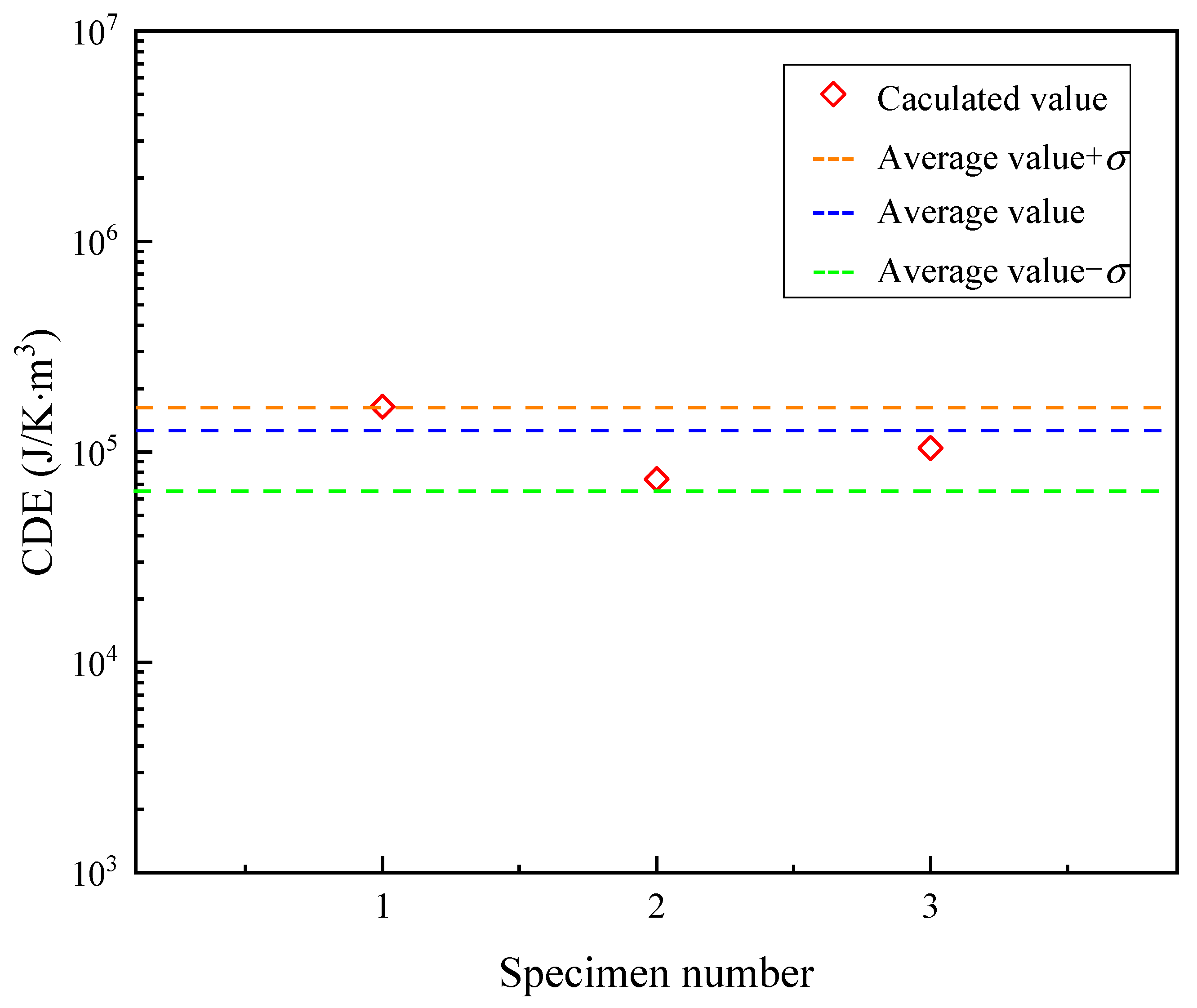
| Specimen Number | No.1 | No.2 | No.3 |
|---|---|---|---|
| CDE (J/K·m3) | 1.64 × 105 | 7.43 × 104 | 1.04 × 105 |
| (J/K·m3) | 1.14 × 105 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, W.; Li, C.; Sun, Y.; Xu, H.; Yang, X. Investigation of the Self-Heating of Q460 Butt Joints and an S-N Curve Modeling Method Based on Infrared Thermographic Data for High-Cycle Fatigue. Metals 2021, 11, 232. https://doi.org/10.3390/met11020232
Wei W, Li C, Sun Y, Xu H, Yang X. Investigation of the Self-Heating of Q460 Butt Joints and an S-N Curve Modeling Method Based on Infrared Thermographic Data for High-Cycle Fatigue. Metals. 2021; 11(2):232. https://doi.org/10.3390/met11020232
Chicago/Turabian StyleWei, Wei, Cheng Li, Yibo Sun, Hongji Xu, and Xinhua Yang. 2021. "Investigation of the Self-Heating of Q460 Butt Joints and an S-N Curve Modeling Method Based on Infrared Thermographic Data for High-Cycle Fatigue" Metals 11, no. 2: 232. https://doi.org/10.3390/met11020232
APA StyleWei, W., Li, C., Sun, Y., Xu, H., & Yang, X. (2021). Investigation of the Self-Heating of Q460 Butt Joints and an S-N Curve Modeling Method Based on Infrared Thermographic Data for High-Cycle Fatigue. Metals, 11(2), 232. https://doi.org/10.3390/met11020232







