A Review on Part Geometric Precision Improvement Strategies in Double-Sided Incremental Forming
Abstract
:1. Introduction
2. Process Mechanism of DSIF
2.1. Toolpath Generation for DSIF Process
2.2. The Role of Thickness Variations in DSIF Toolpath
2.3. Deformation and Fracture Mechanism in DSIF
3. Accuracy Improvement in DSIF Process
3.1. Geometry Accuracy Improvement by DSIF Toolpath Compensation
3.2. Geometry Accuracy Improvement by Toolpath Adaptation
3.3. Geometry Accuracy Improvement by Multi-Pass DSIF
3.4. Geometry Accuracy Improvement by Heat-Assisted DSIF
3.5. Forces Effects on Geometric Precision Improvement
4. Discussion and Future Work Recommendations
- In the DSIF process, the lost contact between the tool and the sheet due to the inaccurate tool gap predicted by sine law is one of the reasons for the part geometric error. The tool gap is usually calculated with sine law, which only considers the wall angle and is not enough to predict accurate wall thickness. Some researchers established modified sine law. They included part height, curvature, and wall angle, but these studies were limited to specific parts. A generalized formula or method should be derived, which could predict the wall thickness precisely for any geometric shape accurately.
- Apart from the sine law, the stiffness variation in metal during forming also plays a role in contact loss. Following the pre-defined toolpath, the reduced reaction force for the support tool can be observed during the forming process. Support tool deviations from the component should be considered an online error in the under-process part. The enhanced stiffness in the sheet during forming applies a load on the master tool. It deviates the master tool from the designed toolpath due to increasing stiffness. Some researchers compensated the master tool toolpath based on empirical formulas and obtained good geometric precision to accommodate such errors. It should be extended to other processes and material parameters. Besides, some methods for stiffness calculation or reduction can be beneficial. The ultrasonic-assisted DSIF process is still not trialed in DSIF, while it has successfully reduced the forming force in other ISF variants due to the acoustoplastic softening effect [100].
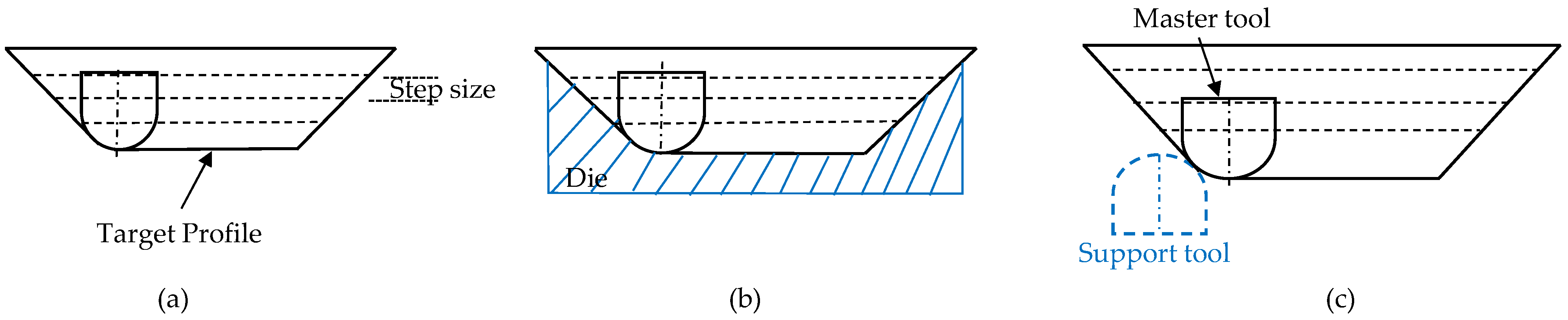
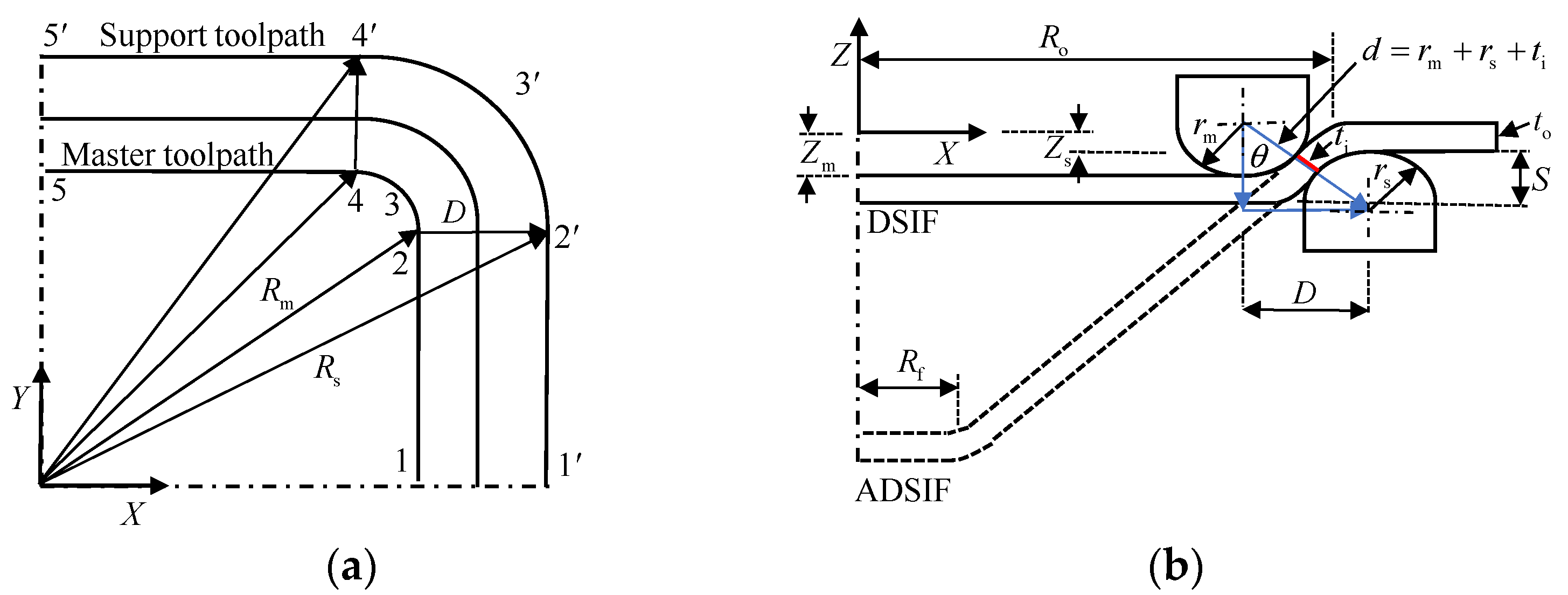
- The relative position between the master and support tool is adjustable due to the flexible support tool in DSIF, which gives more options for toolpath alteration than SPIF or TPIF. ADSIF results from such alterations, which resolves the tool–sheet lost contact problems effectively. It alters the conventional toolpath sequence used in DSIF. The gap between the two tools is not an issue as virgin material is always processed. The geometric accuracy obtained with ADSIF surpasses other strategies. However, ADSIF has some limitations as long processing time and complicated toolpath design. Support tool position determination is not as flexible as in the conventional-DSIF process and is determined for each profile by the trial-and-error procedure. Some researchers had trialed to introduce generalized methods for these shortcomings. However, to date, no generalized method established. It needs further improvements to take full benefits from it. The strategy which is dependent on ADSIF as MDSIF can be improved if ADSIF limitations are solved.
- The DSIF process should be expanded to larger, thicker, and asymmetric components to increase the DSIF process’s industrial viability. Researchers enhanced part precision and formability by successfully preserving tool–sheet contact while using sheet thickness of 1 mm or less for symmetric components in most circumstances. Besides this, the part size formed in most cases is less than 100 mm. Geometric precision in asymmetric parts is more difficult due to the transition from convex to straight and convex to concave regions, and it is the least studied area in DSIF. To obtain acceptability in the industrial sector, all of these constraints must be addressed efficiently.
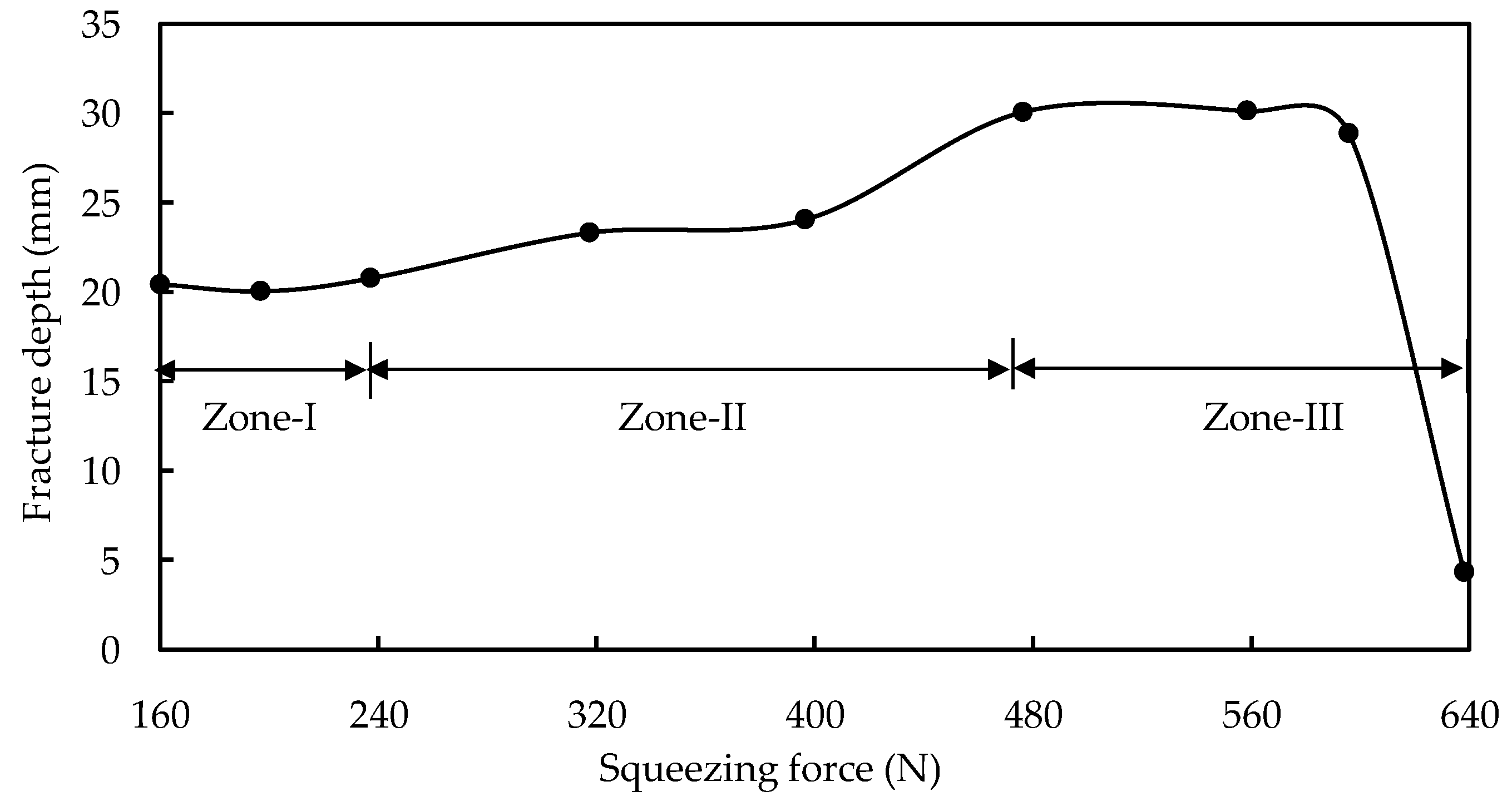
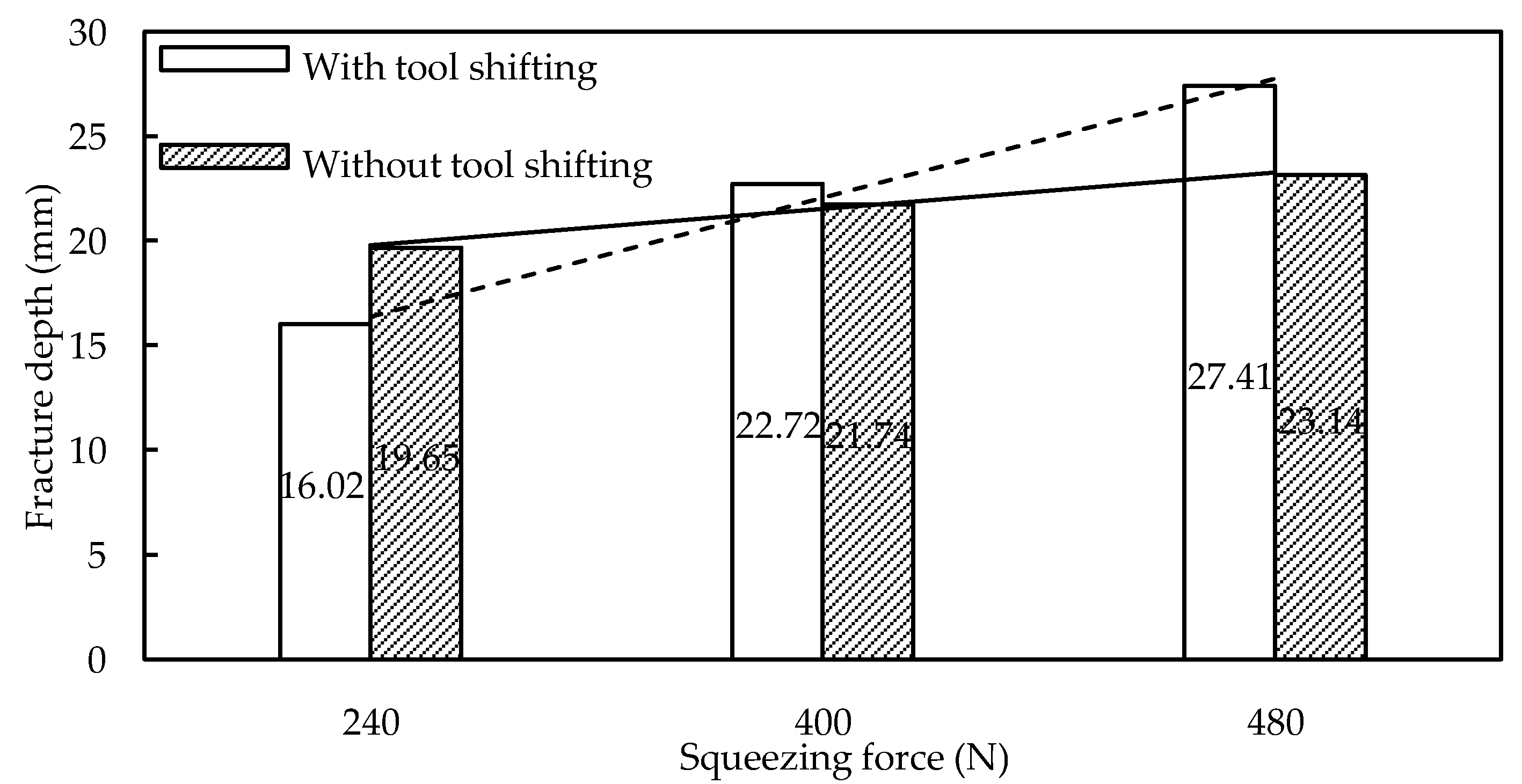
- TPIF gives good geometric accuracy if compared with DSIF and SPIF. To date, no direct comparison for TPIF and DSIF. In TPIF, the die provides essential support to the master tool wherever required. However, in DSIF, a limited region of the master tool is supported. Due to this limitation, researchers trialed DSIF-P, DSIF-L, squeezing, reverse bending, etc. There is a dire need to design a support tool that can provide simultaneous support to the master tool wherever required. Besides, the optimal support tool force required for geometric precision improvement needs investigations.
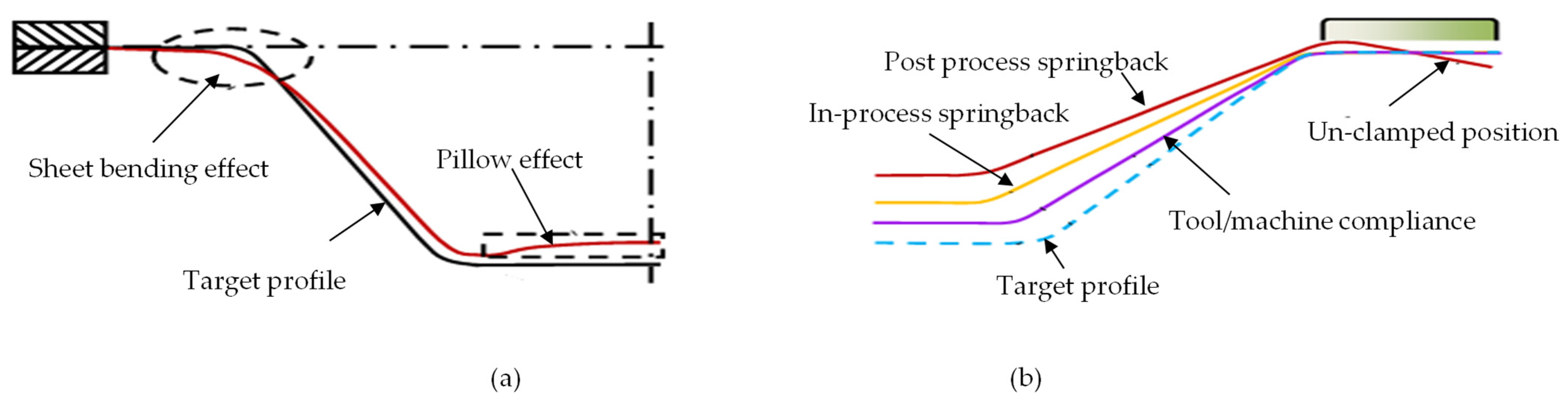
- Springback is the foremost geometric error source in ISF. DSIF can reduce springback if the sheet is squeezed between the tools tactically. In ISF, elastic deformation recovers back as tools leave the formed region. In individual steps, the error due to elastic recovery may be negligible. The accumulation of springback for each step, on the other hand, leads to significant inaccuracies. Elastic recovery compensation in each step size can reduce springback error in the form part; nevertheless, forming in two stages can increase the forming time. Numerical or analytical techniques for calculating elastic recovery for a single step size can simplify a two-stage forming process to a single-stage procedure. A numerical or analytical approach for calculating elastic recovery for a single step size needs investigation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Duflou, J.R.; Habraken, A.-M.; Cao, J.; Malhotra, R.; Bambach, M.; Adams, D.; Vanhove, H.; Mohammadi, A.; Jeswiet, J. Single point incremental forming: State-of-the-art and prospects. Int. J. Mater. Form. 2017, 11, 743–773. [Google Scholar] [CrossRef]
- Jeswiet, J.; Micari, F.; Hirt, G.; Bramley, A.; Duflou, J.; Allwood, J. Asymmetric Single Point Incremental Forming of Sheet Metal. CIRP Ann. 2005, 54, 88–114. [Google Scholar] [CrossRef]
- Allwood, J.; Music, O.; Raithathna, A.; Duncan, S.R. Closed-loop feedback control of product properties in flexible metal forming processes with mobile tools. CIRP Ann. 2009, 58, 287–290. [Google Scholar] [CrossRef]
- Allwood, J.M.; Braun, D.; Music, O. The effect of partially cut-out blanks on geometric accuracy in incremental sheet forming. J. Mater. Process. Technol. 2010, 210, 1501–1510. [Google Scholar] [CrossRef]
- Nasulea, D.; Oancea, G. Achieving Accuracy Improvements for Single-Point Incremental Forming Process Using a Circumferential Hammering Tool. Metals 2021, 11, 482. [Google Scholar] [CrossRef]
- Hussain, G.; Gao, L. A novel method to test the thinning limits of sheet metals in negative incremental forming. Int. J. Mach. Tools Manuf. 2007, 47, 419–435. [Google Scholar] [CrossRef]
- Li, X.; Han, K.; Song, X.; Wang, H.; Li, D.; Li, Y.; Li, Q. Experimental and numerical investigation on surface quality for two-point incremental sheet forming with interpolator. Chin. J. Aeronaut. 2020, 33, 2794–2806. [Google Scholar] [CrossRef]
- Wei, H.; Hussain, G.; Shi, X.; Isidore, B.B.L.; Alkahtani, M.; Abidi, M.H. Formability of Materials with Small Tools in Incremental Forming. Chin. J. Mech. Eng. 2020, 33, 1–9. [Google Scholar] [CrossRef]
- Behera, A.K.; Verbert, J.; Lauwers, B.; Duflou, J.R. Tool path compensation strategies for single point incremental sheet forming using multivariate adaptive regression splines. Comput. Des. 2012, 45, 575–590. [Google Scholar] [CrossRef]
- Lasunon, O.; Knight, W.A. Comparative investigation of single-point and double-point incremental sheet metal forming processes. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2007, 221, 1725–1732. [Google Scholar] [CrossRef]
- Park, J.-J.; Kim, Y.-H. Fundamental studies on the incremental sheet metal forming technique. J. Mater. Process. Technol. 2003, 140, 447–453. [Google Scholar] [CrossRef]
- Reddy, N.V.; Lingam, R.; Cao, J. Incremental metal forming processes in manufacturing. In Handbook of Manufacturing Engineering and Technology; Nee, A.Y.C., Ed.; Springer: London, UK, 2014; pp. 411–452. [Google Scholar] [CrossRef]
- Behera, A.K.; de Sousa, R.A.; Ingarao, G.; Oleksik, V. Single point incremental forming: An assessment of the progress and technology trends from 2005 to 2015. J. Manuf. Process. 2017, 27, 37–62. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Chen, X.; Liu, Z.; Sun, J.; Li, F.; Li, J.; Zhao, G. A review on the recent development of incremental sheet-forming process. Int. J. Adv. Manuf. Technol. 2017, 92, 2439–2462. [Google Scholar] [CrossRef]
- Ai, S.; Long, H. A review on material fracture mechanism in incremental sheet forming. Int. J. Adv. Manuf. Technol. 2019, 104, 33–61. [Google Scholar] [CrossRef] [Green Version]
- Lu, H.; Liu, H.; Wang, C. Review on strategies for geometric accuracy improvement in incremental sheet forming. Int. J. Adv. Manuf. Technol. 2019, 102, 3381–3417. [Google Scholar] [CrossRef]
- Peng, W.; Ou, H.; Becker, A. Double-Sided Incremental Forming: A Review. J. Manuf. Sci. Eng. 2019, 141, 1–42. [Google Scholar] [CrossRef] [Green Version]
- Gohil, A.; Modi, B. Review of the effect of process parameters on performance measures in the incremental sheet forming process. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2020, 235, 303–332. [Google Scholar] [CrossRef]
- Tomasz Trzepieci, N.; Oleksik, V.; Pepelnjak, T.; Mohammed Najm, S.; Paniti, I.; Kuntal, M. Emerging Trends in Single Point Incremental Sheet Forming of Lightweight Metals. Metals 2021, 11, 1188. [Google Scholar] [CrossRef]
- Smith, J.; Malhotra, R.; Liu, W.K.; Cao, J. Deformation mechanics in single-point and accumulative double-sided incremental forming. Int. J. Adv. Manuf. Technol. 2013, 69, 1185–1201. [Google Scholar] [CrossRef]
- Ren, H.; Moser, N.; Zhang, Z.; Ehmann, K.F.; Cao, J. Effects of tool deflection in accumulated double-sided incremental forming regarding part geometry. In Proceedings of the ASME 2016 11th International Manufacturing Science and Engineering Conference, Blacksburg, VA, USA, 27 June 2016; pp. 1–9. [Google Scholar] [CrossRef]
- Ullah, S.; Li, X.; Xu, P.; Li, Y.; Han, K.; Li, D. A toolpath strategy for improving geometric accuracy in double-sided incremental sheet forming. Chin. J. Aeronaut. 2021. [Google Scholar] [CrossRef]
- Lingam, R.; Bhattacharya, A.; Asghar, J.; Reddy, N.V. Compensations for tool path to enhance accuracy during double sided incremental forming. In Proceedings of the ASME 2015 International Manufacturing Science and Engineering Conference, Charlotte, NC, USA, 8–12 June 2015; pp. 1–8. [Google Scholar] [CrossRef]
- Meier, H.; Smukala, V.; Dewald, O.; Zhang, J. Two Point Incremental Forming with Two Moving Forming Tools. Key Eng. Mater. 2007, 344, 599–605. [Google Scholar] [CrossRef]
- Ndip-Agbor, E.; Smith, J.; Ren, H.; Jiang, Z.; Xu, J.; Moser, N.; Chen, W.; Xia, Z.C.; Cao, J. Optimization of relative tool position in accumulative double sided incremental forming using finite element analysis and model bias correction. Int. J. Mater. Form. 2015, 9, 371–382. [Google Scholar] [CrossRef]
- Malhotra, R.; Cao, J.; Ren, F.; Kiridena, V.; Xia, Z.C.; Reddy, N. Improvement of Geometric Accuracy in Incremental Forming by Using a Squeezing Toolpath Strategy with Two Forming Tools. J. Manuf. Sci. Eng. 2011, 133, 061019. [Google Scholar] [CrossRef]
- Moser, N.; Zhang, Z.; Ren, H.; Zhang, H.; Shi, Y.; Ndip-Agbor, E.E.; Lu, B.; Chen, J.; Ehmann, K.F.; Cao, J. Effective forming strategy for double-sided incremental forming considering in-plane curvature and tool direction. CIRP Ann. 2016, 65, 265–268. [Google Scholar] [CrossRef] [Green Version]
- Moser, N.; Ndip-Agbor, E.; Ren, H.Q.; Zhang, Z.X.; Ehmann, K.; Cao, J. Challenges and Process Strategies Concerning Multi-Pass Double Sided Incremental Forming. Key Eng. Mater. 2015, 651–653, 1122–1127. [Google Scholar] [CrossRef]
- Otsu, M.; Nagai, S.-Y.; Miura, T.; Okada, M.; Yoshimura, H.; Matsumoto, R.; Muranaka, T. Forming accuracy improvement by double-side incremental forming. Procedia Manuf. 2018, 15, 1177–1183. [Google Scholar] [CrossRef]
- Lu, B.; Xu, D.K.; Liu, R.Z.; Ou, H.A.; Long, H.; Chen, J. Cranial Reconstruction Using Double Side Incremental Forming. Key Eng. Mater. 2015, 639, 535–542. [Google Scholar] [CrossRef] [Green Version]
- Malhotra, R.; Cao, J.; Beltran, M.; Xu, D.; Magargee, J.; Kiridena, V.; Xia, Z.C. Accumulative-DSIF strategy for enhancing process capabilities in incremental forming. CIRP Ann. 2012, 61, 251–254. [Google Scholar] [CrossRef]
- Zhang, Z.; Ren, H.; Xu, R.; Moser, N.; Smith, J.; Ndip-Agbor, E.; Malhotra, R.; Xia, Z.C.; Ehmann, K.F.; Cao, J. A Mixed Double-Sided Incremental Forming Toolpath Strategy for Improved Geometric Accuracy. J. Manuf. Sci. Eng. 2015, 137, 051007. [Google Scholar] [CrossRef]
- Choi, H.; Lee, C. A mathematical model to predict thickness distribution and formability of incremental forming combined with stretch forming. Robot. Comput. Manuf. 2018, 55, 164–172. [Google Scholar] [CrossRef]
- Salem, E.; Shin, J.; Nath, M.; Banu, M.; Taub, A.I. Investigation of Thickness Variation in Single Point Incremental Forming. Procedia Manuf. 2016, 5, 828–837. [Google Scholar] [CrossRef] [Green Version]
- Cao, T.; Lu, B.; Xu, D.; Zhang, H.; Chen, J.; Long, H.; Cao, J. An efficient method for thickness prediction in multi-pass incremental sheet forming. Int. J. Adv. Manuf. Technol. 2014, 77, 469–483. [Google Scholar] [CrossRef] [Green Version]
- Mirnia, M.J.; Dariani, B.M.; Vanhove, H.; Duflou, J.R. An investigation into thickness distribution in single point incremental forming using sequential limit analysis. Int. J. Mater. Form. 2013, 7, 469–477. [Google Scholar] [CrossRef]
- Meier, H.; Magnus, C.; Smukala, V. Impact of superimposed pressure on dieless incremental sheet metal forming with two moving tools. CIRP Ann. 2011, 60, 327–330. [Google Scholar] [CrossRef]
- Lu, B.; Fang, Y.; Xu, D.; Chen, J.; Ai, S.; Long, H.; Ou, H.; Cao, J. Investigation of material deformation mechanism in double side incremental sheet forming. Int. J. Mach. Tools Manuf. 2015, 93, 37–48. [Google Scholar] [CrossRef]
- Valoppi, B.; Zhang, Z.; Deng, M.; Ghiotti, A.; Bruschi, S.; Ehmann, K.F.; Cao, J. On the Fracture Characterization in Double-Sided Incremental Forming of Ti6Al4V Sheets at Elevated Temperatures. Procedia Manuf. 2017, 10, 407–416. [Google Scholar] [CrossRef]
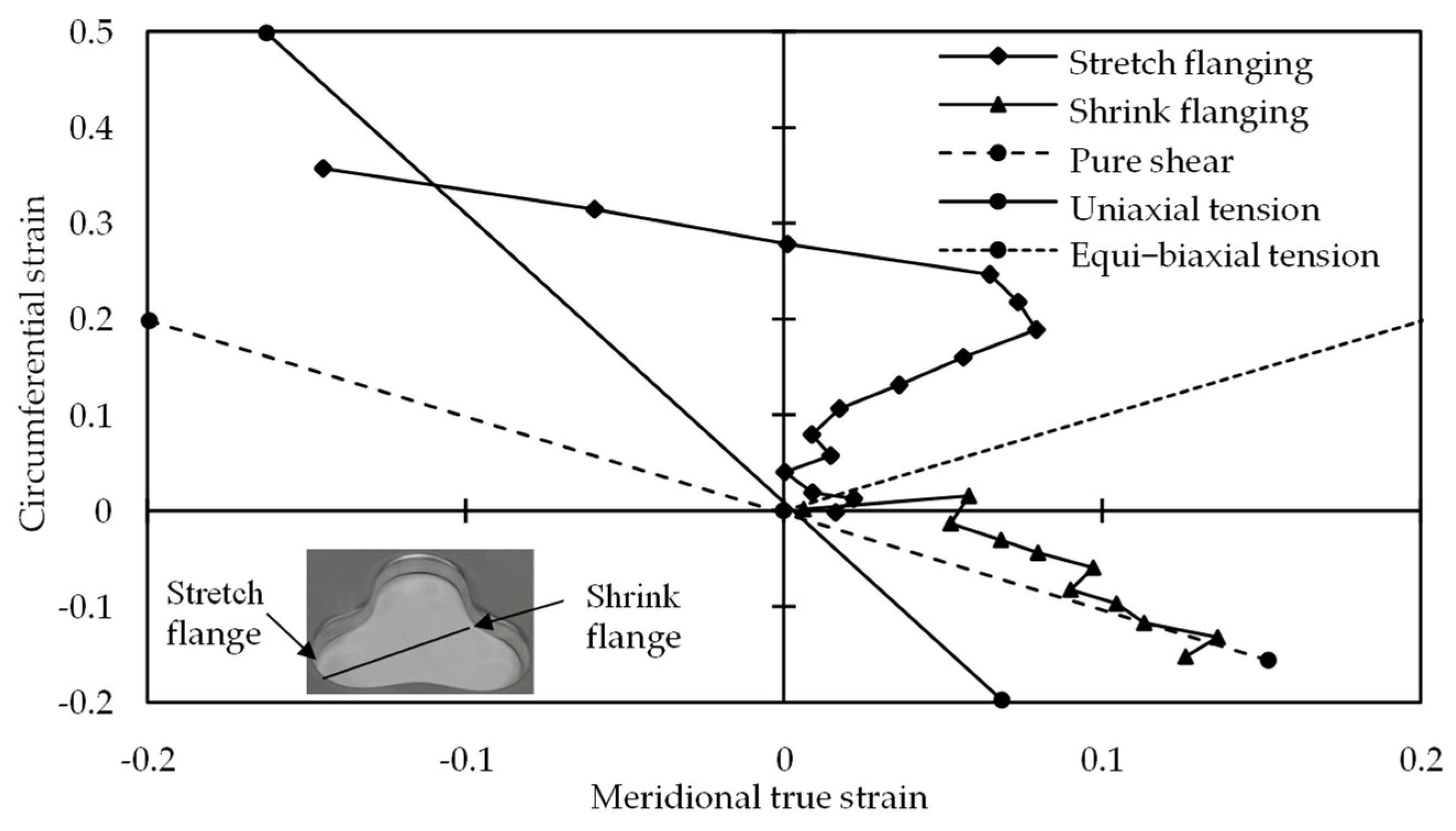
- Zhang, H.; Zhang, Z.; Ren, H.; Cao, J.; Chen, J. Deformation mechanics and failure mode in stretch and shrink flanging by double-sided incremental forming. Int. J. Mech. Sci. 2018, 144, 216–222. [Google Scholar] [CrossRef]
- Moser, N.; Zhang, Z.; Ren, H.; Ehmann, K.; Cao, J. An investigation into the mechanics of double-sided incremental forming using finite element methods. AIP Conf. Proc. 2016, 1769, 070021. [Google Scholar] [CrossRef]
- Malhotra, R.; Xue, L.; Belytschko, T.; Cao, J. Mechanics of fracture in single point incremental forming. J. Mater. Process. Technol. 2012, 212, 1573–1590. [Google Scholar] [CrossRef]
- Davarpanah, M.A.; Zhang, Z.; Bansal, S.; Cao, J.; Malhotra, R. Preliminary investigations on Double Sided Incremental Forming of thermoplastics. Manuf. Lett. 2016, 8, 21–26. [Google Scholar] [CrossRef] [Green Version]
- Micari, F.; Ambrogio, G.; Filice, L. Shape and dimensional accuracy in Single Point Incremental Forming: State of the art and future trends. J. Mater. Process. Technol. 2007, 191, 390–395. [Google Scholar] [CrossRef]
- Wang, Y.; Huang, Y.; Cao, J.; Reddy, N.V. Experimental study on a new method of double side incremental forming. In Proceedings of the ASME 2008 3rd International Manufacturing Science and Engineering Conference, Evanston, IL, USA, 7–10 October 2008. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, W.; Huang, Y.; Reddy, N.V.; Cao, J. Experimental and numerical analysis of double sided incremental forming. In Proceedings of the ASME 2009 International Manufacturing Science and Engineering Conference, West Lafayette, IN, USA, 4–7 October 2009. [Google Scholar] [CrossRef]
- Meier, H.; Buff, B.; Laurischkat, R.; Smukala, V. Increasing the part accuracy in dieless robot-based incremental sheet metal forming. CIRP Ann. 2009, 58, 233–238. [Google Scholar] [CrossRef]
- Meier, H.; Zhu, J.; Buff, B.; Laurischkat, R. CAx Process Chain for Two Robots Based Incremental Sheet Metal Forming. Procedia CIRP 2012, 3, 37–42. [Google Scholar] [CrossRef]
- Ren, H.; Li, F.; Moser, N.; Leem, D.; Li, T.; Ehmann, K.; Cao, J. General contact force control algorithm in double-sided incremental forming. CIRP Ann. 2018, 67, 381–384. [Google Scholar] [CrossRef]
- Ren, H.; Xie, J.; Liao, S.; Leem, D.; Ehmann, K.; Cao, J. In-situ springback compensation in incremental sheet forming. CIRP Ann. 2019, 68, 317–320. [Google Scholar] [CrossRef]
- Rakesh, L.; Amit, S.; Reddy, N.V. Deflection Compensations for Tool Path to Enhance Accuracy During Double-Sided Incremental Forming. J. Manuf. Sci. Eng. 2016, 138, 091008. [Google Scholar] [CrossRef]
- Praveen, K.; Lingam, R.; Reddy, N.V. Tool path design system to enhance accuracy during double sided incremental forming: An analytical model to predict compensations for small/large components. J. Manuf. Process. 2020, 58, 510–523. [Google Scholar] [CrossRef]
- Konka, P.; Lingam, R.; Singh, U.A.; Shivaprasad, C.; Reddy, N.V. Enhancement of accuracy in double sided incremental forming by compensating tool path for machine tool errors. Int. J. Adv. Manuf. Technol. 2020, 111, 1–13. [Google Scholar] [CrossRef]
- Allwood, J.M.; Childs, T.; Clare, A.; De Silva, A.K.; Dhokia, V.; Hutchings, I.M.; Leach, R.; Leal-Ayala, D.R.; Lowth, S.; Majewski, C.E.; et al. Manufacturing at double the speed. J. Mater. Process. Technol. 2015, 229, 729–757. [Google Scholar] [CrossRef] [Green Version]
- Störkle, D.; Altmann, P.; Möllensiep, D.; Thyssen, L.; Kuhlenkötter, B. Automated parameterization of local support at every toolpath point in robot-based incremental sheet forming. Procedia Manuf. 2019, 29, 67–73. [Google Scholar] [CrossRef]
- Wu, J.H.; Wang, Q.C. Comparison of the Geometric Accuracy by DSIF Toolpath with SPIF Toolpath. Appl. Mech. Mater. 2014, 494–495, 497–501. [Google Scholar] [CrossRef]
- Wang, H.; Zhang, R.; Zhang, H.; Hu, Q.; Chen, J. Novel strategies to reduce the springback for double-sided incremental forming. Int. J. Adv. Manuf. Technol. 2018, 96, 973–979. [Google Scholar] [CrossRef]
- Peng, W.; Li, M.; Lu, B.; Chen, J.; Becker, A.; Ou, H. Experimental and finite element investigation of over-bending phenomenon in Double-Sided Incremental Forming (DSIF) of aluminium sheets. Procedia Manuf. 2019, 29, 59–66. [Google Scholar] [CrossRef]
- Zhu, H.; Xiao, D.; Kang, J. Research on point-pressing based double-sided CNC incremental forming. J. Mech. Sci. Technol. 2019, 33, 4389–4400. [Google Scholar] [CrossRef]
- Lingam, R.; Harikrishnan, C.L.; Kishan, I.V.M.; Reddy, N.V. Importance of feature sequencing in incremental forming. ASME 2015 Int. Manuf. Sci. Eng. Conf. MSEC 2015, 1, 1–9. [Google Scholar] [CrossRef]
- Lingam, R.; Prakash, O.; Belk, J.H.; Reddy, N.V. Automatic feature recognition and tool path strategies for enhancing accuracy in double sided incremental forming. Int. J. Adv. Manuf. Technol. 2016, 88, 1639–1655. [Google Scholar] [CrossRef]
- Ndip-Agbor, E.; Ehmann, K.; Cao, J. Automated Flexible Forming Strategy for Geometries with Multiple Features in Double-Sided Incremental Forming. J. Manuf. Sci. Eng. 2017, 140, 031004. [Google Scholar] [CrossRef]
- Zhu, H.; Xiao, D.; Kang, J. Research on the double-sided incremental forming toolpath planning and generation based on STL model. Int. J. Adv. Manuf. Technol. 2019, 102, 839–856. [Google Scholar] [CrossRef]
- Araghi, B.T.; Manco, G.; Bambach, M.; Hirt, G. Investigation into a new hybrid forming process: Incremental sheet forming combined with stretch forming. CIRP Ann. 2009, 58, 225–228. [Google Scholar] [CrossRef]
- Sarraji, W.K.H.; Hussain, J.; Ren, W.-X. Experimental Investigations on Forming Time in Negative Incremental Sheet Metal Forming Process. Mater. Manuf. Process. 2011, 27, 499–506. [Google Scholar] [CrossRef]
- Li, J.-C.; Li, C.; Zhou, T.-G. Thickness distribution and mechanical property of sheet metal incremental forming based on numerical simulation. Trans. Nonferrous Met. Soc. China 2012, 22, s54–s60. [Google Scholar] [CrossRef]
- Otsu, M.; Yasunaga, M.; Matsuda, M.; Takashima, K. Friction Stir Incremental Forming of A2017 Aluminum Sheets. Procedia Eng. 2014, 81, 2318–2323. [Google Scholar] [CrossRef] [Green Version]
- Mohammadi, A.; Qin, L.; Vanhove, H.; Seefeldt, M.; Van Bael, A.; Duflou, J.R. Single Point Incremental Forming of an Aged AL-Cu-Mg Alloy: Influence of Pre-heat Treatment and Warm Forming. J. Mater. Eng. Perform. 2016, 25, 2478–2488. [Google Scholar] [CrossRef]
- Jeswiet, J.; Hagan, E.; Szekeres, A. Forming parameters for incremental forming of aluminium alloy sheet metal. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2002, 216, 1367–1371. [Google Scholar] [CrossRef]
- Hussain, G.; Ilyas, M.; Isidore, B.L.; Khan, W.A. Mechanical properties and microstructure evolution in incremental forming of AA5754 and AA6061 aluminum alloys. Trans. Nonferrous Met. Soc. China 2020, 30, 51–64. [Google Scholar] [CrossRef]
- Xu, R.; Shi, X.; Xu, D.; Malhotra, R.; Cao, J. A preliminary study on the fatigue behavior of sheet metal parts formed with accumulative-double-sided incremental forming. Manuf. Lett. 2014, 2, 8–11. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, Z.; Ren, H.; Moser, N.; Cao, J. Dieless double-sided incremental hole-flanging with different toolpath strategies. In Proceedings of the ASME 2016 11th International Manufacturing Science and Engineering Conference, Blacksburg, VA, USA, 27 June–1 July 2016. [Google Scholar]
- Asghar, J.; Reddy, N.V. Importance of tool configuration in incremental sheet metal forming of difficult to form materials using electro-plasticity. In Proceedings of the World Congress on Engineering 2013, London, UK, 3–5 July 2013; Volume III. WCE 2013. [Google Scholar]
- Duflou, J.; Callebaut, B.; Verbert, J.; De Baerdemaeker, H. Laser Assisted Incremental Forming: Formability and Accuracy Improvement. CIRP Ann. 2007, 56, 273–276. [Google Scholar] [CrossRef]
- Fan, G.; Sun, F.; Meng, X.; Gao, L.; Tong, G. Electric hot incremental forming of Ti-6Al-4V titanium sheet. Int. J. Adv. Manuf. Technol. 2009, 49, 941–947. [Google Scholar] [CrossRef]
- Ambrogio, G.; Filice, L.; Manco, G. Warm incremental forming of magnesium alloy AZ31. CIRP Ann. 2008, 57, 257–260. [Google Scholar] [CrossRef]
- Meier, H.; Magnus, C. Incremental Sheet Metal Forming with Direct Resistance Heating Using Two Moving Tools. Key Eng. Mater. 2013, 554, 1362–1367. [Google Scholar] [CrossRef]
- Xu, D.; Lu, B.; Cao, T.; Zhang, H.; Chen, J.; Long, H.; Cao, J. Enhancement of process capabilities in electrically-assisted double sided incremental forming. Mater. Des. 2015, 92, 268–280. [Google Scholar] [CrossRef]
- Valoppi, B.; Egea, A.J.S.; Zhang, Z.; Rojas, H.A.G.; Ghiotti, A.; Bruschi, S.; Cao, J. A hybrid mixed double-sided incremental forming method for forming Ti6Al4V alloy. CIRP Ann. 2016, 65, 309–312. [Google Scholar] [CrossRef] [Green Version]
- Kiridena, V.; Verma, R.; Gutowski, T.; Roth, J. Rapid freeform sheet metal forming: Technology development and system verification[R]; Office of Scientific and Technical Information (OSTI): Oak Ridge, TN, USA, 2018. [Google Scholar]
- Zhang, Z.; Zhang, H.; Shi, Y.; Moser, N.; Ren, H.; Ehmann, K.F.; Cao, J. Springback Reduction by Annealing for Incremental Sheet Forming. Procedia Manuf. 2016, 5, 696–706. [Google Scholar] [CrossRef] [Green Version]
- Xu, R.; Ren, H.; Zhang, Z.; Malhotra, R.; Cao, J. A mixed toolpath stratagy for improved geometric accuracy and higher throughput in double-sided incremental forming. In Proceedings of the ASME 2014 International Manufacturing Science and Engineering Conference, Detroit, MI, USA, 9–13 June 2014; pp. 1–8. [Google Scholar]
- Szekeres, A.; Ham, M.; Jeswiet, J. Force Measurement in Pyramid Shaped Parts with a Spindle Mounted Force Sensor. Key Eng. Mater. 2007, 344, 551–558. [Google Scholar] [CrossRef]
- Li, P.; He, J.; Liu, Q.; Yang, M.; Wang, Q.; Yuan, Q.; Li, Y. Evaluation of forming forces in ultrasonic incremental sheet metal forming. Aerosp. Sci. Technol. 2017, 63, 132–139. [Google Scholar] [CrossRef] [Green Version]
- Duflou, J.; Tunçkol, Y.; Szekeres, A.; Vanherck, P. Experimental study on force measurements for single point incremental forming. J. Mater. Process. Technol. 2007, 189, 65–72. [Google Scholar] [CrossRef]
- Aerens, R.; Eyckens, P.; Bael, A.V.; Duflou, J.R. Force prediction for single point incremental forming deduced from experimental and FEM observations. Int. J. Adv. Manuf. Technol. 2010, 46, 969–982. [Google Scholar] [CrossRef]
- Wang, J.; Nair, M.; Zhang, Y. An Efficient Force Prediction Strategy in Single Point Incremental Sheet Forming. Procedia Manuf 2016, 5, 761–771. [Google Scholar] [CrossRef] [Green Version]
- Henrard, C.; Bouffioux, C.; Eyckens, P.; Soly, H.; Duflou, J.; Van Houtte, P.; Van Bael, A.; Duchêne, L.; Habraken, A. Forming forces in single point incremental forming: Prediction by finite element simulations, validation and sensitivity. Comput. Mech. 2010, 47, 573–590. [Google Scholar] [CrossRef]
- Filice, L.; Ambrogio, G.; Micari, F. On-Line Control of Single Point Incremental Forming Operations through Punch Force Monitoring. CIRP Ann. 2006, 55, 245–248. [Google Scholar] [CrossRef]
- Hagan, E.; Jeswiet, J. Analysis of surface roughness for parts formed by computer numerical controlled incremental forming. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2004, 218, 1307–1312. [Google Scholar] [CrossRef]
- Powers, B.M.; Ham, M.; Wilkinson, M.G. Small data set analysis in surface metrology: An investigation using a single point incremental forming case study. Scanning 2010, 32, 199–211. [Google Scholar] [CrossRef]
- Bhattacharya, A.; Maneesh, K.; Reddy, N.V.; Cao, J. Formability and Surface Finish Studies in Single Point Incremental Forming. J. Manuf. Sci. Eng. 2011, 133, 061020. [Google Scholar] [CrossRef]
- Durante, M.; Formisano, A.; Langella, A. Comparison between analytical and experimental roughness values of components created by incremental forming. J. Mater. Process. Technol. 2010, 210, 1934–1941. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, S.; Li, Y.; Meehan, P.A. Modeling and Optimization of Surface Roughness in Incremental Sheet Forming using a Multi-objective Function. Mater. Manuf. Process. 2014, 29, 808–818. [Google Scholar] [CrossRef]
- Wei, H.; Hussain, G.; Iqbal, A.; Zhang, Z.P. Surface roughness as the function of friction indicator and an important parameters-combination having controlling influence on the roughness: Recent results in incremental forming. Int. J. Adv. Manuf. Technol. 2018, 101, 2533–2545. [Google Scholar] [CrossRef]
- Kumar, A.; Gulati, V. Experimental investigation and optimization of surface roughness in negative incremental forming. Measurement 2018, 131, 419–430. [Google Scholar] [CrossRef]
- Kim, Y.; Park, J. Effect of process parameters on formability in incremental forming of sheet metal. J. Mater. Process. Technol. 2002, 130–131, 42–46. [Google Scholar] [CrossRef]
- Lu, B.; Fang, Y.; Xu, D.; Chen, J.; Ou, H.; Moser, N.; Cao, J. Mechanism investigation of friction-related effects in single point incremental forming using a developed oblique roller-ball tool. Int. J. Mach. Tools Manuf. 2014, 85, 14–29. [Google Scholar] [CrossRef]
- Skjoedt, M.; Silva, M.B.; Bay, N.; Martins, P.A.F.; Lenau, T. Single point incremental forming using a dummy sheet. Int. Conf. New Form. Technol. 2007, 2, 267–276. [Google Scholar]
- Cheng, Z.; Li, Y.; Li, J.; Li, F.; Meehan, P.A. Ultrasonic assisted incremental sheet forming: Constitutive modeling and deformation analysis. J. Mater. Process. Technol. 2021, 299, 117365. [Google Scholar] [CrossRef]











| Method | Part Type | Error (mm) | Part Size (mm) (O × H × θ) | Thick (mm) | Material | Cause-and-Effect | Researchers |
|---|---|---|---|---|---|---|---|
| Springback and machine compliances | cone | ±0.25 | 100 × 40 × 53° | 0.8 | AA99.5 | Springback, sine law-undersize, compliance- oversize | Meier et al. (2009) |
| Squeezing (1.0,0.9,0.85) | cone | improved | 130 × 36 × 65° | 1.5 | AA5182 | Sine law-support tool lost contact | Malhotra et al. (2011) |
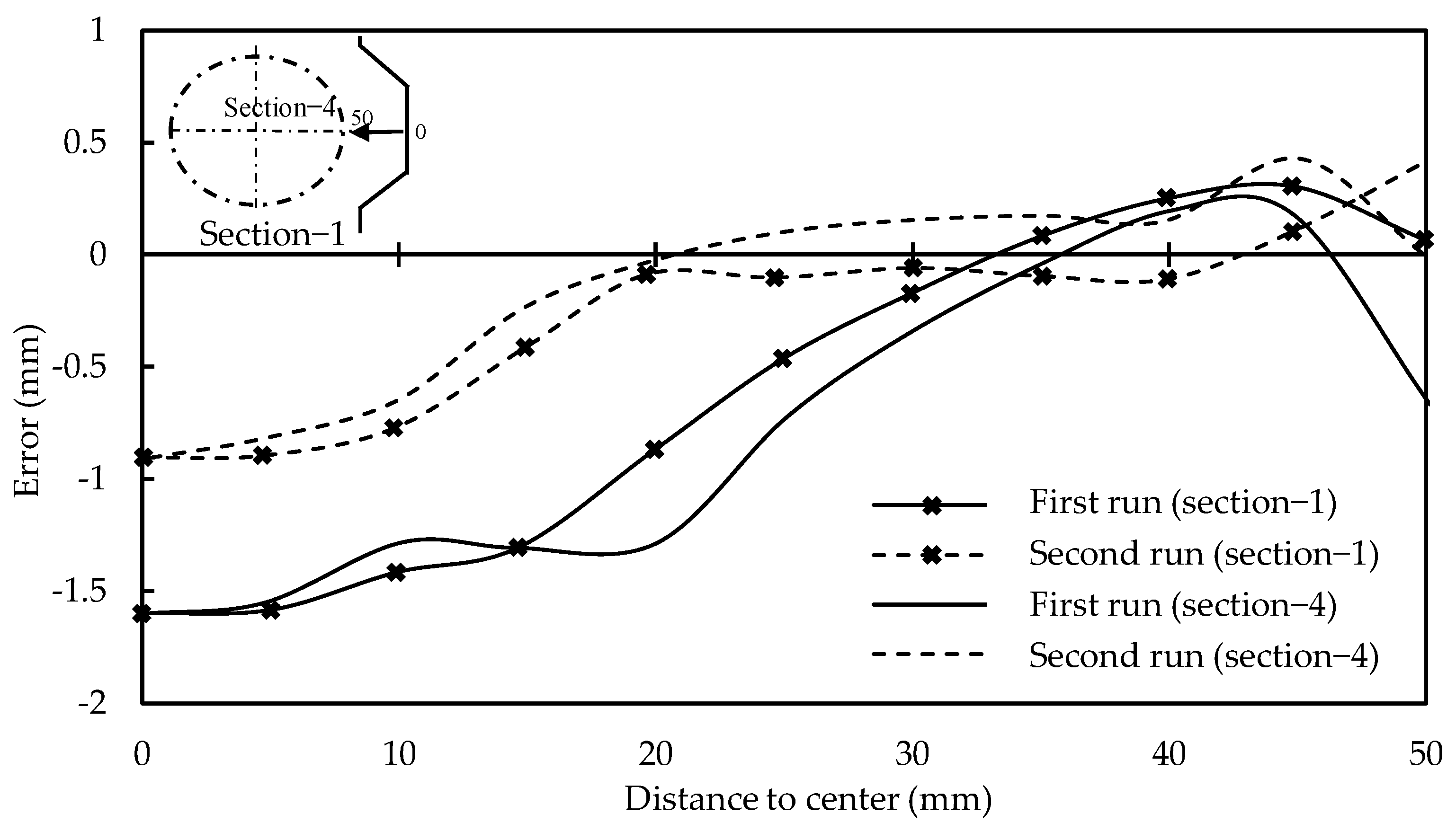
| Tool gap correction | shamrock | NR | 110 × 31.2 × 65° | 1.0 | AA5754 | Modified sine law-specific to shamrock | Moser et al. (2016) |
| Support tool force control | cone | 1.0 | 45 × 16 × 40° | 0.5 | AA2024-T3 | Complicated- Implementation issue | Ren et al. (2018) |
| funnel | NR | 45 × 22 × 65° | 1.0 | AA5754-O | |||
| In-situ springback | cone | 0.8 | 70 × 22 × 45° | 0.5 | AA2024-T3 | Simulation based-time consuming | Ren et al. (2019) |
| pyramid | 0.2 | 80 × 22 × 45° | 1.0 | AA5754-O | |||
| Tool and sheet deflection (small part) | cone | 0.40 | 78.6 × 20 × 60° | 0.88 | AA5052-O | Empirical formula-limited to small parts | Lingam et al. (2015, 2016) |
| funnel | 0.46 | 80 × 22.5 × 60° | |||||
| Tool and sheet deflection (large part) | Varying wall component | 1.25 | 260 × 72 × 60° | 0.8 | AA8011 | No machine compliance- support tool lost contact | Praveen et al. (2020) |
| Elliptical free-form | 1.52 | 640 × 110 × 55° | |||||
| Machine, tool, and sheet deflection (large part) | Varying wall component | 0.62 | 260 × 72 × 60° | Empirical formula: trialed for AA8011 only with a thin sheet | Konka et al. (2020) | ||
| Elliptical free-form | 0.93 | 640 × 110× 55° | |||||
| Incremental springback accommodation (D-DSIF) | Pyramid | 0.33 0.57 | 45 × 15 × 40° 100 × 30 × 45° | 1.0 | AA7075 DC-04 | Implementations of the second stage should be based on the formula | S. Ullah et al. (2021) |
| Method | Part Type | Error (mm) | Part Size (mm) (O × H × θ) | Thick (mm) | Material | Cause and Effect | Researchers |
|---|---|---|---|---|---|---|---|
| DSIF-L DSIF-P DSIF-L DSIF-P | Free-form | 1.0 | NR | NR | Al Mn 99.8 | Trial based and sine law-time consuming | Meier et al. (2011) |
| 2.5 | |||||||
| Skull | <5.0 | <(120 × 25) | 0.5 | Grade-1 Titanium | Insufficient support-increase in error | Bin Lu et al. (2015 | |
| 5.0 | |||||||
| ADSIF | Cone | 0.49 | 45 × 14 × 40° | 0.5 | AA2024-T3 | Low step size, trial based-time consuming | Malhotra et al. (2012) |
| 45 × 17 × 50° | |||||||
| MDSIF (ASIF+DSIF) | Pyramid with pocket | <0.49 | 45 × 15 × varied | 0.5 | AA2024-T3 | Based on ADSIF-time consuming | Zhang et al. (2015) |
| Reverse bending | Ellipsoidal | ±0.25 | Major:112 × 21 × 41.1° Minor:102 × 21 × 44.8° | 1.0 | AA7075 | Over bending-precision degradation | Wang et al. (2018) |
| Squeezing | 0.4 | Ineffective-springback reduction | |||||
| Automatic feature recognition | Conical profile with an inclined hump | 0.3 | 80 × 17 × NR | 0.88 | AA5052 | Lingam and Ndip-Agbor strategy recommended the sequence in the opposite direction | Lingam et al. (2017) |
| pyramid with an inclined base and a protrusion | 0.25 | 80 × 17 × 45° | |||||
| Multiple features with Z-based slicing | Freeform | 1.5 | <(80 × 18) | 1.0 | AA5754-O | Ndip-Agbor et al. (2018) | |
| Tool-path adaptation based on STL model | Bidirectional protruding feature | 3.2 | <(200 × 25) | 0.6 | AA1060 | Springback: precision degradation | Zhu et al. (2019b) |
| Method | Part Type | Error (mm) | Part Size (O × H × θ) (O and H in mm) | Thickness (mm) | Material | Researchers |
|---|---|---|---|---|---|---|
| Direct resistance heating | Cone | 1.0 | NR | 0.8 | DX54D | Meier and Magnus (2013) |
| High-density pulse | Pyramid | NR | NR × 25 × 45° | NR | Titanium | Asghar and Reddy (2013) |
| HE-DSIF | Cone | 1.0 | 80 × 25 × 45° | 1.41 | AZ3B1 | Xu et al. (2016) |
| E-DSIF | 2.2 | |||||
| E-SPIF | >3.0 | |||||
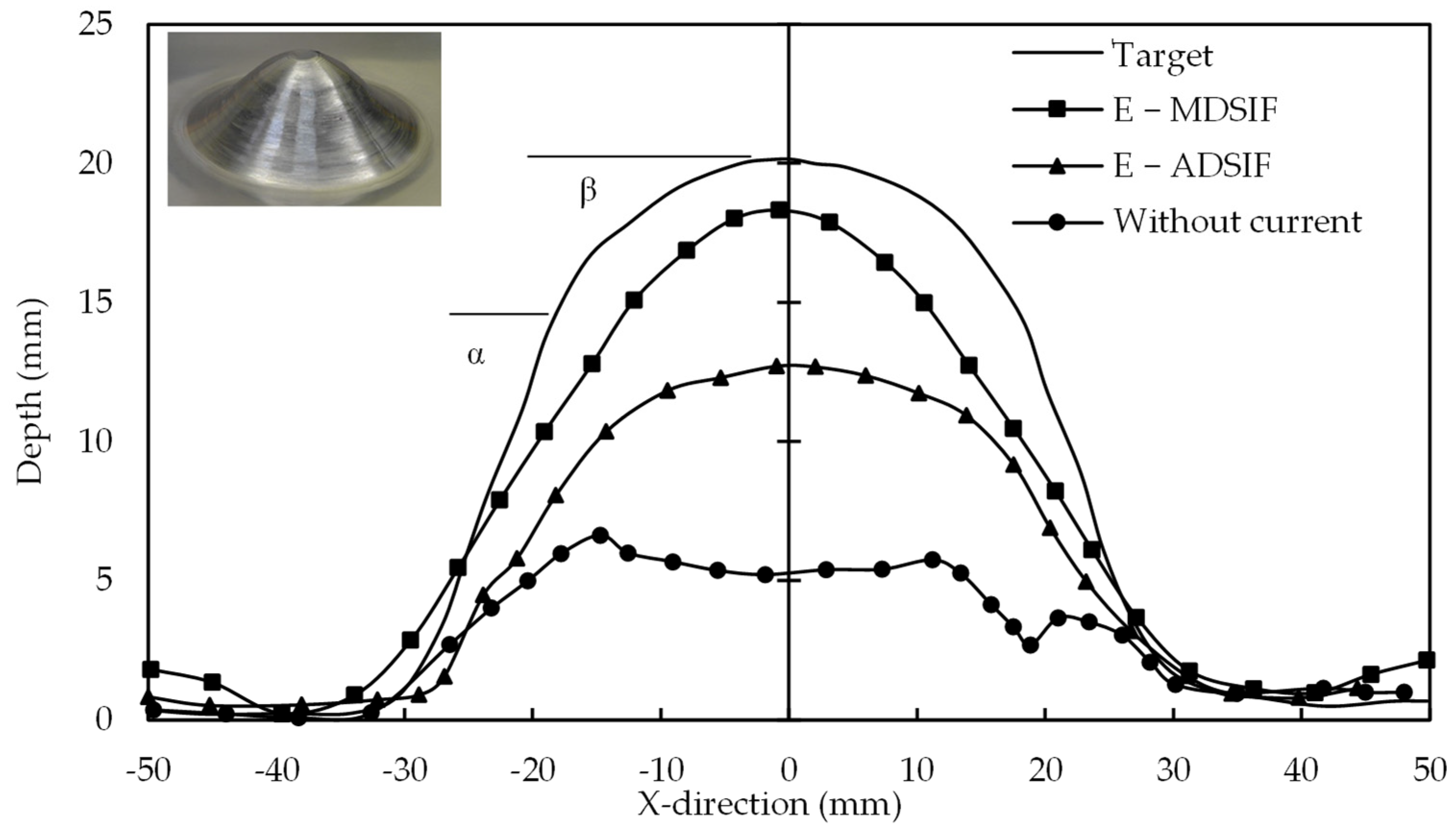
| E-MDSIF | Free-form | 1.98 | Figure 19 | 0.5 | Ti6Al4V | Valoppi et al. (2016) |
| E-ADSIF | 8.0 | |||||
| Without current | 15.42 |
| Toolpath | Step Size (mm) | Type | Feed Rate (mm/s) | Tool Diameter (mm) | Thickness (mm) | Forces (N) | Material | Researchers |
|---|---|---|---|---|---|---|---|---|
| DSIF | 0.5 | SBG | 10 | 6 | 1.5 | M:1200–1400 | AA5182 | Malhotra et al. (2011) |
| ADSIF | 0.1 | SBG | 5 | 5 | 0.5 | SR:180 | AA2024-T3 | Ren et al. (2018) |
| DSIF | 0.5 | SBG | 5 | 5 | 1 | SR:200 | AA5754-O | |
| DSIF | 0.5 | SBG | 9.5 | 0.88 | M:650, S:70 | AA5052-O | Lingam et al. (2015, 2016) | |
| DSIF | 0.5 | SBG | 12.7 | 0.8 | M: 400, S:35 | AA8011 | Konka et al. (2020) | |
| DSIF | SBG | M: 320, S:45 | ||||||
| DSIF | 0.2 | SBP | 12 | S: 125 | Al Mn 99.8 | Meier et al. (2011) | ||
| ADSIF | 0.025 | SBG | 5 | 0.5 | M:150–425, S:80–280 MR:220–274, SR:80–160 | AA2024-T3 | Malhotra et al. (2012) | |
| D-stage of MDSIF | 0.1 | SBG | 5 | 5 | 0.5 | M:240, S:200 | AA2024-T3 | Xu et al. (2014) |
| Squeezing | 0.02 | SBP | 20 | 10 | 1 | S:360 | AA7075 | Wang et al. (2018) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ullah, S.; Xu, P.; Li, X.; Li, Y.; Han, K.; Li, D. A Review on Part Geometric Precision Improvement Strategies in Double-Sided Incremental Forming. Metals 2022, 12, 103. https://doi.org/10.3390/met12010103
Ullah S, Xu P, Li X, Li Y, Han K, Li D. A Review on Part Geometric Precision Improvement Strategies in Double-Sided Incremental Forming. Metals. 2022; 12(1):103. https://doi.org/10.3390/met12010103
Chicago/Turabian StyleUllah, Sattar, Peng Xu, Xiaoqiang Li, Yanle Li, Kai Han, and Dongsheng Li. 2022. "A Review on Part Geometric Precision Improvement Strategies in Double-Sided Incremental Forming" Metals 12, no. 1: 103. https://doi.org/10.3390/met12010103
APA StyleUllah, S., Xu, P., Li, X., Li, Y., Han, K., & Li, D. (2022). A Review on Part Geometric Precision Improvement Strategies in Double-Sided Incremental Forming. Metals, 12(1), 103. https://doi.org/10.3390/met12010103








