Development of a Moving-Bed Ironmaking Process for Direct Gaseous Reduction of Iron Ore Concentrate
Abstract
:1. Introduction
2. Process Concept
3. Configuration of a Horizontal Moving-Bed Furnace
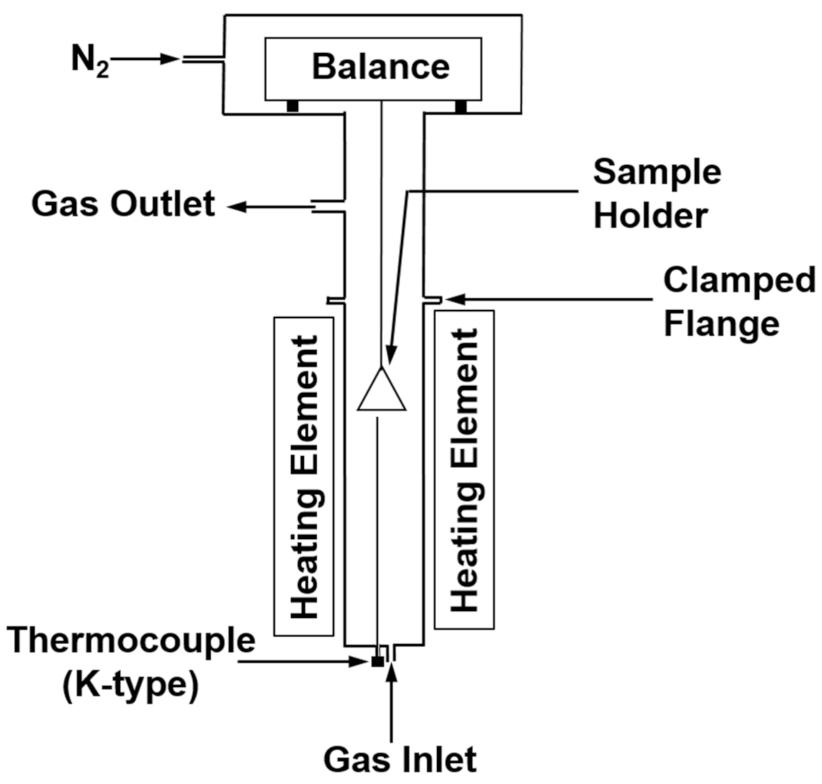
4. Hydrogen Reduction Kinetics of Concentrate Particles
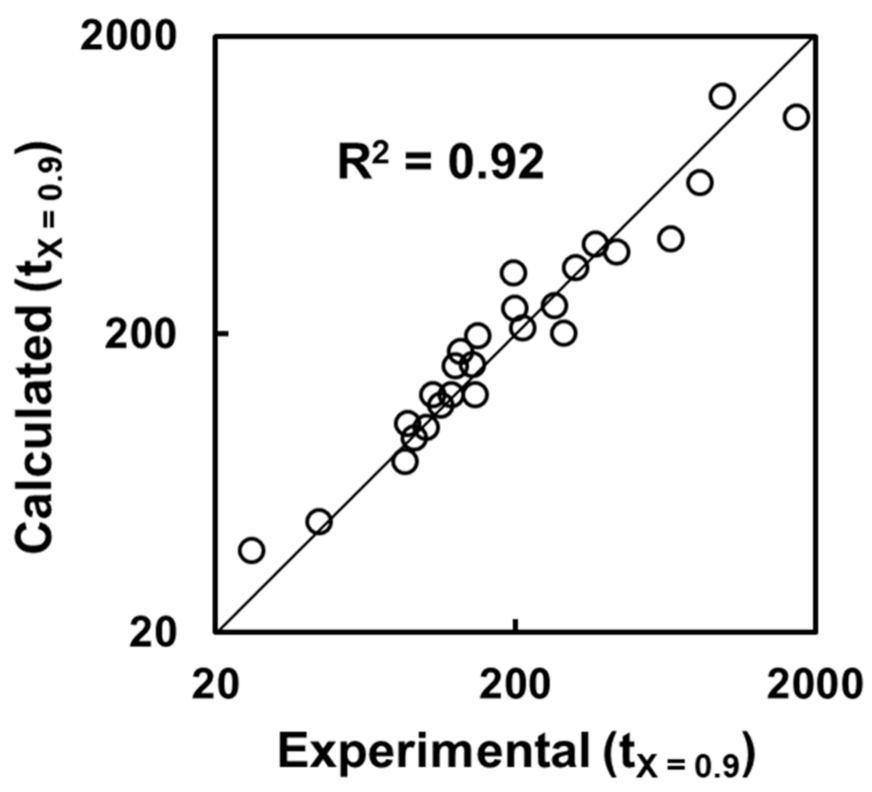
5. Incorporation of Interparticle Diffusion in the Rate Analysis
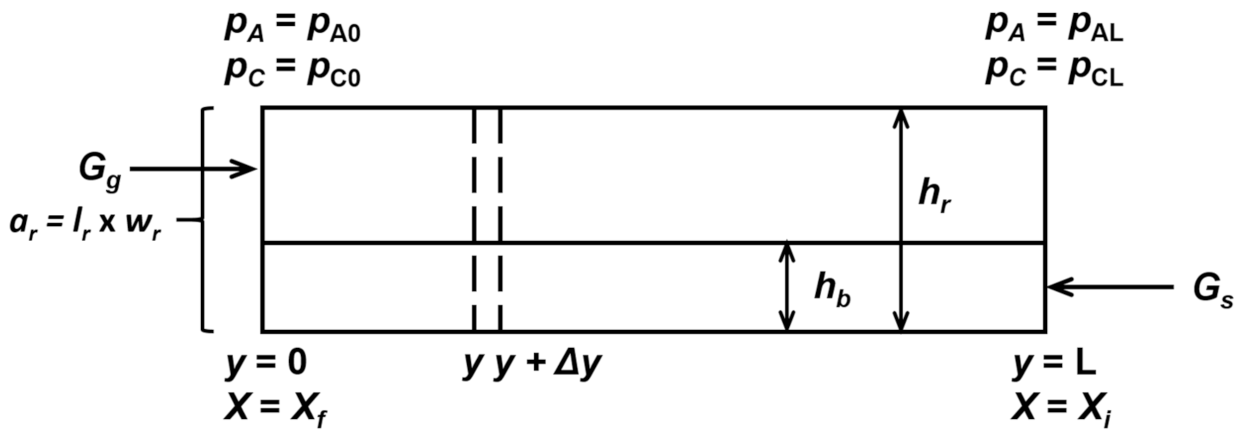
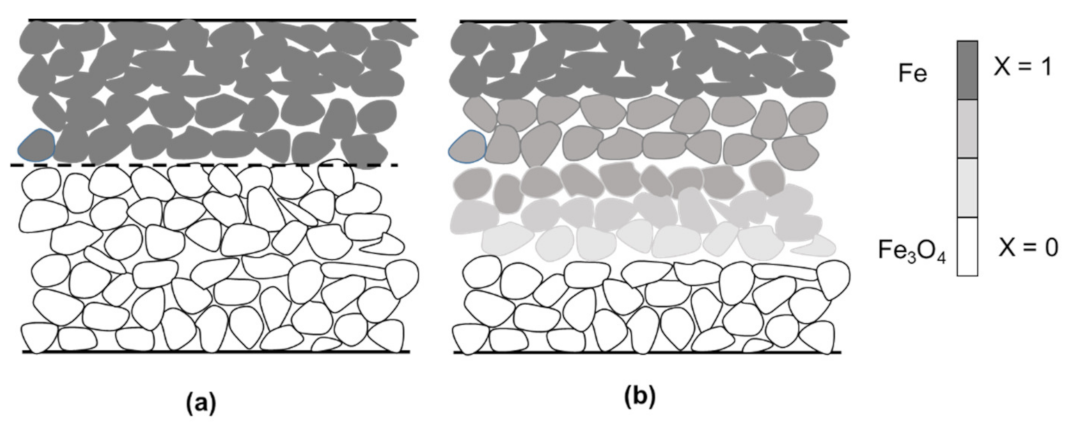
6. Design of a Horizontal Moving-Bed Furnace
6.1. Model Formulation
- (a)
- The overall reaction is , which in general notation is represented by .
- (b)
- The reduction occurs under isothermal conditions.
- (c)
- The solid and reducing gas are in plug flow and steady state.
- (d)
- The reactor has a uniform cross-sectional area.
- (e)
- Mass transfer between the gas and the top of the bed is fast.
6.2. Design of Industrial Reactors
7. Concluding Remarks
- (1)
- The proposed technology for a modest-scale ironmaking operation with a production rate of 0.1 Mtpy can be operated at temperatures between 650 and 1000 °C.
- (2)
- The design parameters and the operating conditions for the horizontal moving-bed reactor were established.
- (3)
- A simple model for a moving-bed reactor that indicated that the proposed ironmaking technology has industrial potential was formulated.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- WorldSteel Association. Steel Statistical Yearbook 2020. Available online: https://worldsteel.org/wp-content/uploads/Steel-Statistical-Yearbook-2020-concise-version.pdf (accessed on 25 October 2022).
- Hasanbeigi, A.; Arens, M.; Price, L. Alternative Emerging Ironmaking Technologies for Energy-Efficiency and Carbon Dioxide Emissions Reduction: A Technical Review. Renew. Sustain. Energy Rev. 2014, 33, 645–658. [Google Scholar] [CrossRef]
- Bellevrat, E.; Menanteau, P. Introducing Carbon constraint in the Steel Sector: ULCOS Scenarios and Economic Modeling. Revue de Métallurgie 2009, 106, 318–324. [Google Scholar] [CrossRef]
- Sohn, H.Y. Suspension Ironmaking Technology with Greatly Reduced Energy Requirement and CO2 Emissions. Steel Times Int. 2007, 31, 68–72. [Google Scholar]
- Sohn, H.Y.; Mohassab, Y. Development of a Novel Flash Ironmaking Technology with Greatly Reduced Energy Consumption and CO2 Emissions. J. Sustain. Metall. 2016, 2, 216–227. [Google Scholar] [CrossRef]
- Sohn, H.Y.; Fan, D.-Q.; Abdelghanyand, A. Design of Novel Flash Ironmaking Reactors for Greatly Reduced Energy Consumption and CO2 Emissions. Metals 2021, 11, 332. [Google Scholar] [CrossRef]
- Sohn, H.Y.; Elzohiery, M.; Fan, D.-Q. Development of the Flash Ironmaking Technology (FIT) for Green Ironmaking with Low Energy Consumption. J. Energy Power Technol. 2021, 3, 26. [Google Scholar] [CrossRef]
- Patisson, F.; Mirgaux, O. Hydrogen Ironmaking: How It Works. Metals 2020, 10, 922. [Google Scholar] [CrossRef]
- Pei, M.; Petäjäniemi, M.; Regnell, A.; Wijk, O. Toward a Fossil Free Future with HYBRIT: Development of Iron and Steelmaking Technology in Sweden and Finland. Metals 2020, 10, 972. [Google Scholar] [CrossRef]
- Ghosh, A.; Chatterjee, A. Ironmaking and Steelmaking Theory and Practice, 4th ed.; PHI Learning Private Ltd.: New Delhi, India, 2011; pp. 225–262. [Google Scholar]
- Sohn, H.Y.; Roy, S. Moving-Bed Reactor for Continuous Ironmaking with Gaseous Reduction of Iron Ore Concentrate. Provisional. U.S. Patent Application No. 63/389,795, 15 July 2022. [Google Scholar]
- Roy, S. Hydrogen Reduction of Iron Ore Concentrate in Loose Layers and Compacts. Ph.D. Thesis, University of Utah, Salt Lake City, UT, USA, 2022. [Google Scholar]
- Themelis, N.J.; Gauvin, W.H. Reduction of Iron Oxide in Gas-Conveyed Systems. AIChE J. 1962, 8, 437–444. [Google Scholar] [CrossRef]
- Wang, H.; Sohn, H.Y. Hydrogen Reduction Kinetics of Magnetite Concentrate Particles Relevant to a Novel Flash Ironmaking Process. Metall. Mater. Trans. B 2013, 44, 133–145. [Google Scholar] [CrossRef]
- Lien, H.O.; El-Mehairy, A.E.; Ross, H.U. A Two-Zone Theory of Iron-Oxide Reduction. J. Iron Steel Inst. 1971, 209, 541–545. [Google Scholar]
- Specht, O.G.; Zappfe, C.A. The Low-Temperature Gaseous Reduction of Magnetite Ore to Sponge Iron. Trans. Am. Inst. Min. Metall. Engrs. 1946, 167, 237–280. [Google Scholar]
- Sohn, H.Y.; Roy, S. Fluid–Solid Reaction Kinetics for Solids of Non-Basic Geometries: Application of The Law of Additive Times in Combination with the Shape-Factor Method. Metall. Mater. Trans. B 2020, 51, 601–610. [Google Scholar] [CrossRef]
- Sohn, H. Fluid–Solid Reactions; Elsevier: Cambridge, MA, USA, 2020; pp. 233–241. [Google Scholar]
- Sohn, H.Y. The Law of Additive Reaction Times in Fluid–Solid Reactions. Metall. Mater. Trans. B 1978, 9, 89–96. [Google Scholar] [CrossRef]
- Sohn, H.Y. Review of Fluid-Solid Reaction Analysis: Part 2. Single Porous Reactant Solid. Can. J. Chem. Eng. 2019, 97, 2068–2076. [Google Scholar] [CrossRef]
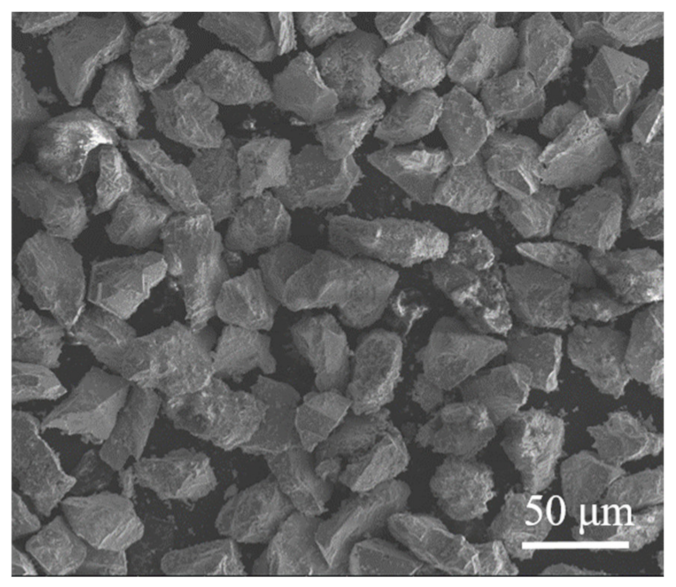
| Component | Wt.% |
|---|---|
| Total Iron | 70.65 |
| SiO2 | 1.87 |
| Al2O3 | 0.13 |
| CaO | 0.27 |
| MgO | 0.13 |
| MnO | 0.11 |
| Cr2O3 | 0.11 |
| K2O | 0.01 |
| Na2O | 0.1 |
| TiO2 | 0.01 |
| ZrO2 | 0.03 |
| P | 0.01 |
| S | 0.02 |
| C | 0.24 |
| Sr | 0.01 |
| Temperature (°C) | Bed Thickness (cm) | Residence Time (min) | Reactor Length (m) | Bed Speed (cm/min) | Gas Velocity (cm/s) |
|---|---|---|---|---|---|
| 1000 | 1 | 23.5 | 5.76 | 24.5 | 182 |
| 2 | 92 | 11.3 | 12.3 | 188 | |
| 5 | 571 | 28.1 | 4.91 | 211 | |
| 900 | 1 | 28.7 | 7.03 | 24.5 | 182 |
| 2 | 108 | 13.3 | 12.3 | 188 | |
| 5 | 661 | 32.4 | 4.91 | 211 | |
| 850 | 1 | 32.1 | 7.87 | 24.5 | 183 |
| 2 | 118 | 14.5 | 12.3 | 189 | |
| 5 | 718 | 35.3 | 4.91 | 212 | |
| 650 | 1 | 95 | 23.3 | 24.5 | 196 |
| 2 | 371 | 45.6 | 12.3 | 203 | |
| 5 | 2303 | 113.1 | 4.91 | 227 |
| Temperature (°C) | 1000 | 900 | 850 | 650 |
|---|---|---|---|---|
| Characteristic Length (cm) | 2 | 2 | 2 | 2 |
| Gas Flow Rate (Nm3/h) | 20,350 | 20,400 | 20,500 | 21,950 |
| Residence Time (min) | 92 | 108 | 118 | 371 |
| Speed of Grate (cm/min) | 12.3 | 12.3 | 12.3 | 12.3 |
| Reactor Length (m) | 11.3 | 13.3 | 14.5 | 45.6 |
| Reactor Volume (m3) | 170 | 200 | 218 | 684 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sohn, H.Y.; Roy, S. Development of a Moving-Bed Ironmaking Process for Direct Gaseous Reduction of Iron Ore Concentrate. Metals 2022, 12, 1889. https://doi.org/10.3390/met12111889
Sohn HY, Roy S. Development of a Moving-Bed Ironmaking Process for Direct Gaseous Reduction of Iron Ore Concentrate. Metals. 2022; 12(11):1889. https://doi.org/10.3390/met12111889
Chicago/Turabian StyleSohn, Hong Yong, and Syamantak Roy. 2022. "Development of a Moving-Bed Ironmaking Process for Direct Gaseous Reduction of Iron Ore Concentrate" Metals 12, no. 11: 1889. https://doi.org/10.3390/met12111889
APA StyleSohn, H. Y., & Roy, S. (2022). Development of a Moving-Bed Ironmaking Process for Direct Gaseous Reduction of Iron Ore Concentrate. Metals, 12(11), 1889. https://doi.org/10.3390/met12111889







