Acoustic Analysis of Slag Foaming in the BOF
Abstract
:1. Introduction
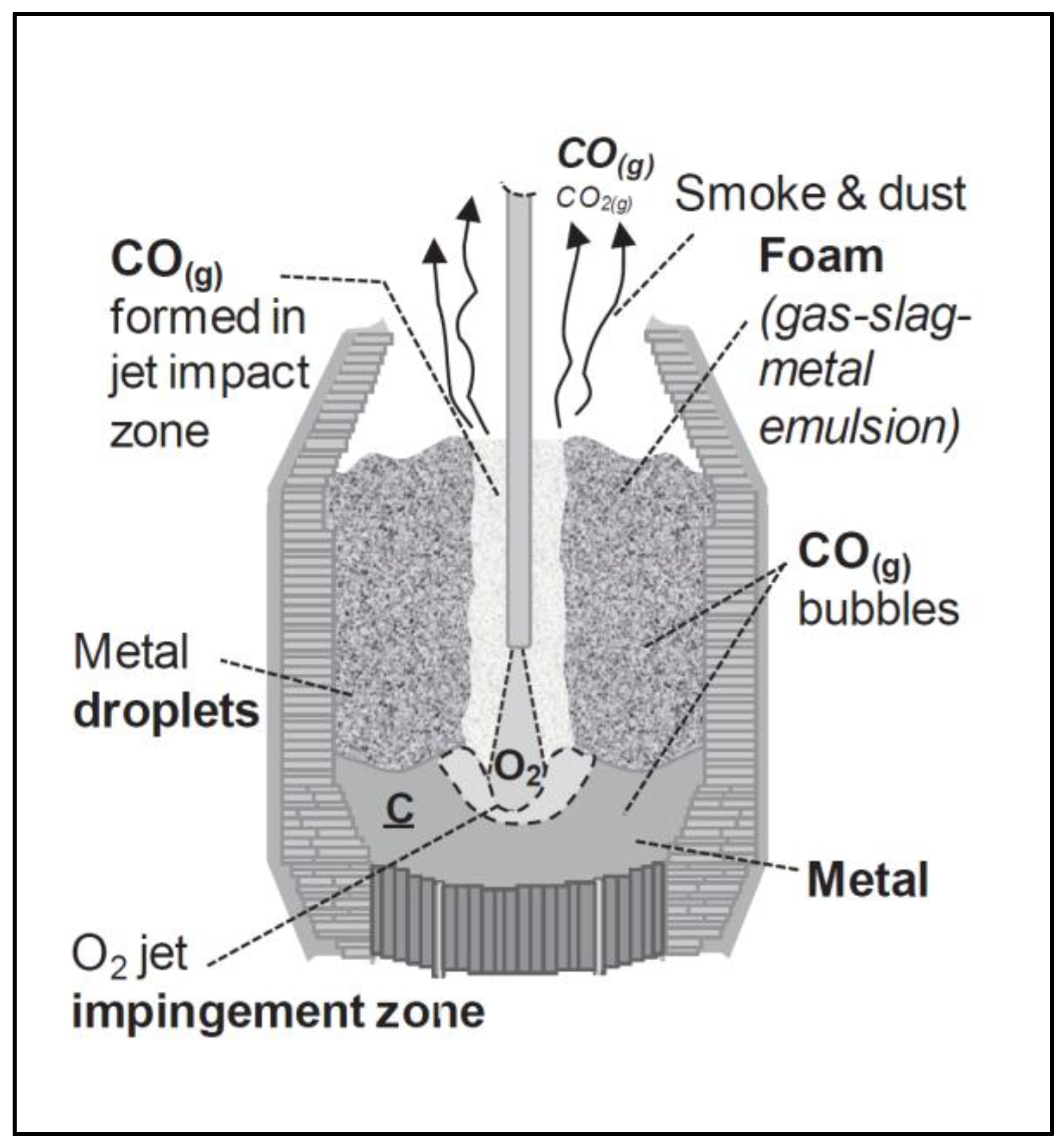
2. Foaming in the Basic Oxygen Furnace
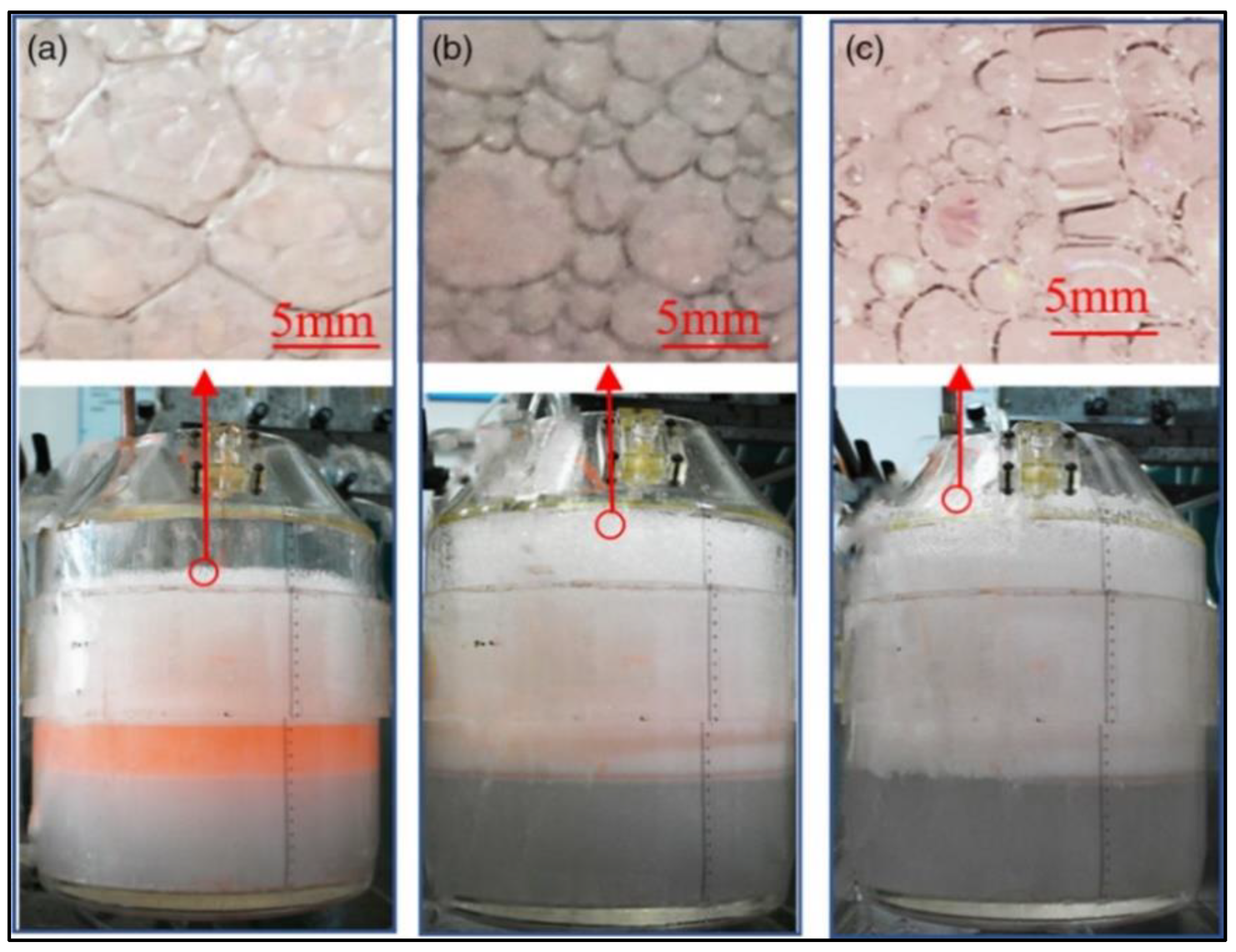
3. Sound in Liquid Foam
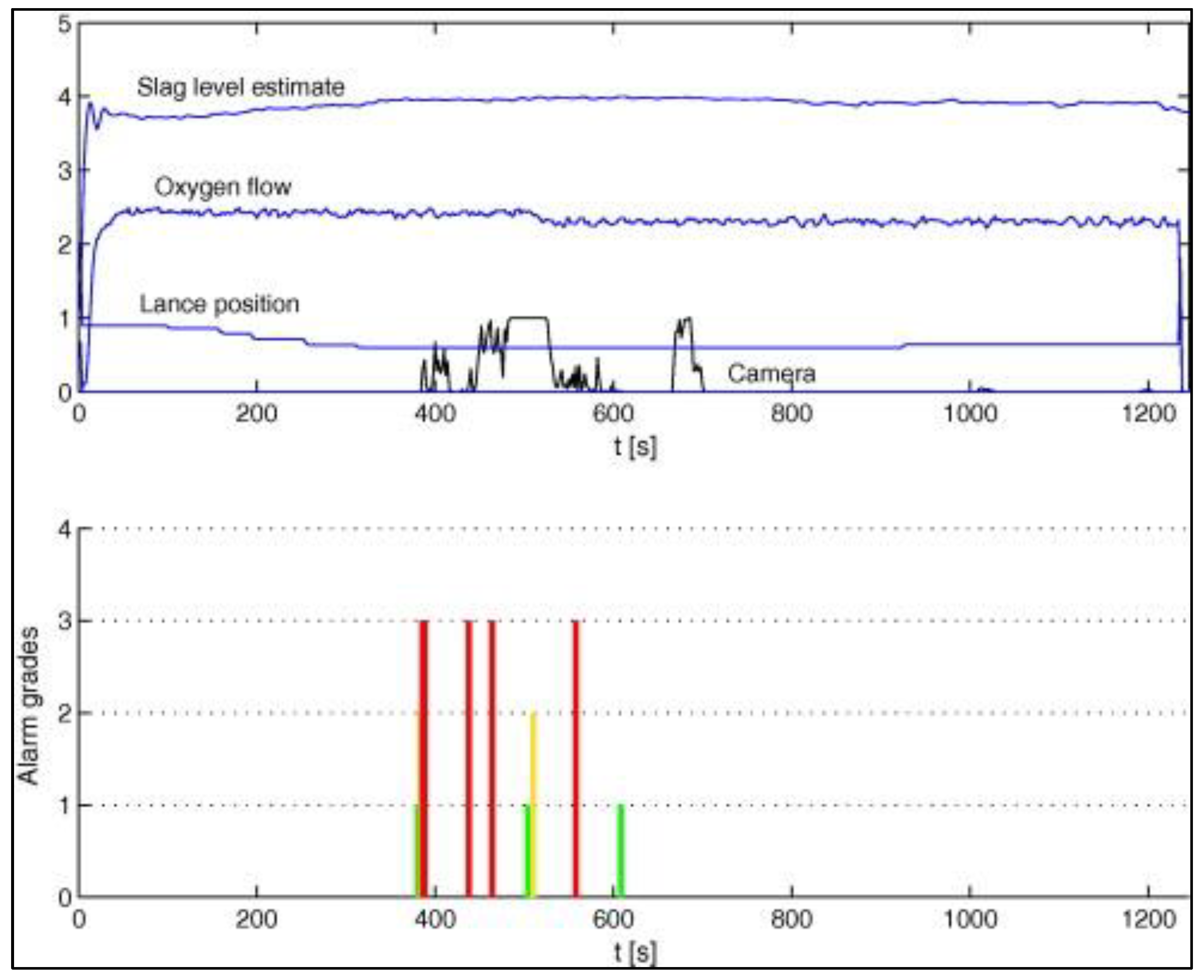
4. Cold Model Study on Control of Slag Foam Using Acoustic Analysis in the BOF
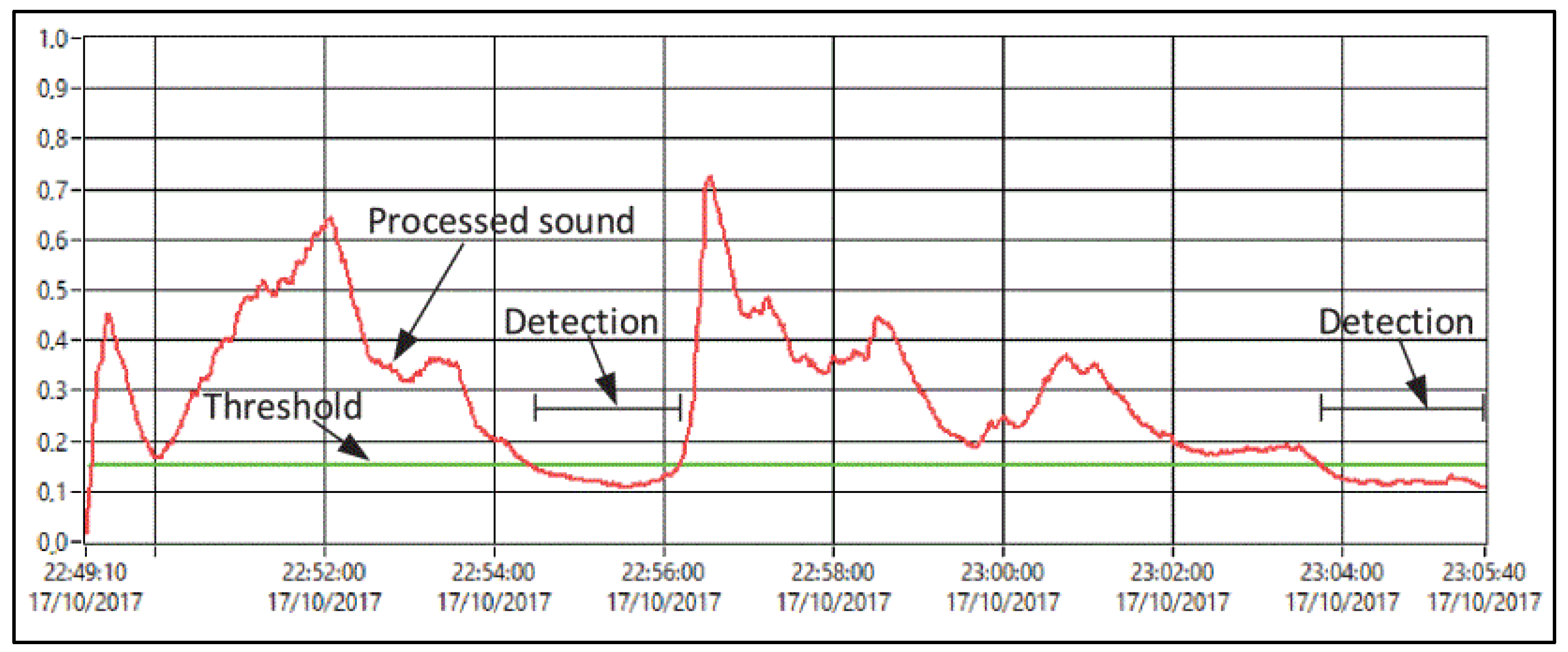
5. Plant-Based Studies on Dynamic Control of Slag Foam Using Acoustic Analysis
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Rout, B.K.; Brooks, G.; Rhamdhani, M.A.; Li, Z.; Schrama, F.N.H.; Overbosch, A. Dynamic Model of Basic Oxygen Steelmaking Process Based on Multizone Reaction Kinetics: Modeling of Decarburization. Metall. Mater. Trans. B 2018, 49, 1022–1033. [Google Scholar] [CrossRef]
- Dogan, N.; Brooks, G.A.; Rhamdhani, M.A. Comprehensive model of oxygen steelmaking part 2: Application of bloated droplet theory for decarburization in emulsion zone. ISIJ Int. 2011, 51, 1093–1101. [Google Scholar] [CrossRef] [Green Version]
- Snigdha, G.; Bharath, B.N.; Viswanathan, N.N. BOF process dynamics. Miner. Processing Extr. Metall. 2019, 128, 17–33. [Google Scholar] [CrossRef]
- Holappa, L. Historical overview on the development of converter steelmaking from Bessemer to modern practices and future outlook. Miner. Processing Extr. Metall. 2019, 128, 3–16. [Google Scholar] [CrossRef]
- Brämming, M.; Millman, S.; Overbosch, A.; Kapilashrami, A.; Malmberg, D.; Björkman, B. BOS vessel vibration measurement for foam level detection. ISIJ Int. 2011, 51, 71–79. [Google Scholar] [CrossRef] [Green Version]
- Brämming, M. Avoiding Slopping in Top-Blown BOS Vessels. Ph.D. Thesis, Luleå Tekniska Universitet, Luleå, Sweden, 2010. [Google Scholar]
- Evestedt, M.; Medvedev, A. Model-based slopping warning in the LD steel converter process. J. Process Control. 2009, 19, 1000–1010. [Google Scholar] [CrossRef]
- Birk, W.; Arvanitidis, I.; Jonsson, P.G.; Medvedev, A. Physical modeling and control of dynamic foaming in an LD-converter process. IEEE Trans. Ind. Appl. 2001, 37, 1067–1073. [Google Scholar] [CrossRef]
- Sandlöbes, S.; Senk, D.; Sancho, L.; Diaz, A. In-situ measurement of CO- and CO2-concentrations in bof off-gas. Steel Res. Int. 2011, 82, 632–637. [Google Scholar] [CrossRef]
- Pierre, J.; Dollet, B.; Leroy, V. Resonant Acoustic Propagation and Negative Density in Liquid Foams. Phys. Rev. Lett. 2014, 112, 148307. [Google Scholar] [CrossRef] [Green Version]
- Elias, F.; Crassous, J.; Derec, C.; Dollet, B.; Drenckhan, W.; Gay, C.; Leroya, V.; Noûse, C.; Pierref, J.; Saint-Jalmes, A. The Acoustics of Liquid Foams. Curr. Opin. Colloid Interface Sci. 2020, 50, 101391. [Google Scholar] [CrossRef]
- Pierre, J.; Guillermic, R.-M.; Elias, F.; Drenckhan, W.; Leroy, V. Acoustic characterisation of liquid foams with an impedance tube. Eur. Phys. J. E 2013, 36, 113. [Google Scholar] [CrossRef] [PubMed]
- Ingard, U. Notes on Sound Absorption Technology; Noise Control Foundation: Poughkeepsie, NY, USA, 1994.
- Dogan, N.; Brooks, G.A.; Rhamdhani, M.A. Comprehensive model of oxygen steelmaking part 1: Model development and validation. ISIJ Int. 2011, 51, 1086–1092. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Fruehan, R. Effect of the bubble size and chemical reactions on slag foaming. Metall. Mater. Trans. B 1995, 26, 803–812. [Google Scholar] [CrossRef]
- Kadrolkar, A.; Dogan, N. The Decarburization Kinetics of Metal Droplets in Emulsion Zone. Metall. Mater. Trans. B 2019, 50, 2912–2929. [Google Scholar] [CrossRef]
- He, Q.L.; Standish, N. A model study of droplet generation in the BOF steelmaking. ISIJ Int. 1990, 30, 305–309. [Google Scholar] [CrossRef]
- Molloy, N. Impinging jet flow in a 2-phase system-basic flow pattern. J. Iron Steel Inst. 1970, 208, 943. [Google Scholar]
- Subagyo, G.B.; Coley, K.; Irons, G. Generation of droplets in slag-metal emulsions through top gas blowing. ISIJ Int. 2003, 43, 983–989. [Google Scholar] [CrossRef] [Green Version]
- Miller, T.W.; Jimenez, J.; Sharan, A.; Goldstein, D.A. Oxygen steelmaking processes. Mak. Shap. Treat. Steel-Steelmak. Refin. Vol. 1998, 475–524. [Google Scholar]
- Ghag, S.S.; Hayes, P.C.; Lee, H.-G. Physical model studies on slag foaming. ISIJ Int. 1998, 38, 1201–1207. [Google Scholar] [CrossRef] [Green Version]
- De Vos, L.; Cnockaert, V.; Bellemans, I.; Vercruyssen, C.; Verbeken, K. Critical Assessment of the Applicability of the Foaming Index to the Industrial Basic Oxygen Steelmaking Process. Steel Res. Int. 2021, 92, 2000282. [Google Scholar] [CrossRef]
- Bikerman, J.J. Foams; Springer: Berlin/Heidelberg, Germany, 1973. [Google Scholar]
- Ito, K.; Fruehan, R. Study on the foaming of CaO-SiO2-FeO slags: Part I. Foaming parameters and experimental results. Metall. Trans. B 1989, 20, 509–514. [Google Scholar]
- Cooper, C.; Kitchener, J. The foaming of molten silicates. J. Iron Steel Inst. 1959, 193, 48–55. [Google Scholar]
- Swisher, J.; McCabe, C. Cr2O3 As Foaming Agent in CaO-SiO2 Slags. Trans. Metall. Soc. AIME 1964, 230, 1669. [Google Scholar]
- Bikerman, J.J. Surface Chemistry: Theory and Applications; Elsevier Science & Technology: Saint Louis, MO, USA, 1958. [Google Scholar]
- Ito, K.; Fruehan, R.J. Study on the foaming of CaO-SiO2-FeO slags: Part II. Dimensional analysis and foaming in iron and steelmaking processes. Metall. Trans. B 1989, 20, 515–521. [Google Scholar] [CrossRef]
- Jiang, R.; Fruehan, R. Slag foaming in bath smelting. Metall. Trans. B 1991, 22, 481–489. [Google Scholar] [CrossRef]
- Ghag, S.S.; Hayes, P.C.; Lee, H.-G. The prediction of gas residence times in foaming CaO–SiO2–FeO slags. ISIJ Int. 1998, 38, 1216–1224. [Google Scholar] [CrossRef]
- Lotun, D.; Pilon, L. Physical modeling of slag foaming for various operating conditions and slag compositions. ISIJ Int. 2005, 45, 835–840. [Google Scholar] [CrossRef]
- Gou, H.; Irons, G.A.; Lu, W.-K. A multiphase fluid mechanics approach to gas holdup in bath smelting processes. Metall. Mater. Trans. B 1996, 27, 195–201. [Google Scholar] [CrossRef]
- Boyd, J.W.; Varley, J. The uses of passive measurement of acoustic emissions from chemical engineering processes. Chem. Eng. Sci. 2001, 56, 1749–1767. [Google Scholar] [CrossRef]
- Roshid, M.M.; Manasseh, R. Extraction of bubble size and number data from an acoustically-excited bubble chain. J. Acoust. Soc. Am. 2020, 147, 921–940. [Google Scholar] [CrossRef]
- Ooi, A.; Manasseh, R. Coupled nonlinear oscillations of microbubbles. ANZIAM J. 2004, 46, C102–C116. [Google Scholar] [CrossRef]
- Nicholas, M.; Roy, R.A.; Crum, L.A.; Oguz, H.; Prosperetti, A. Sound emissions by a laboratory bubble cloud. J. Acoust. Soc. Am. 1994, 95, 3171–3182. [Google Scholar] [CrossRef]
- Guan YZhao, D.; Low, T.S. Experimental evaluation on acoustic impedance and sound absorption performances of porous foams with additives with Helmholtz number. Aerosp. Sci. Technol. 2021, 119, 107120. [Google Scholar] [CrossRef]
- Wood, A. A Textbook of Sound; George Bell & Sons Ltd.: London, UK, 1941. [Google Scholar]
- Moxon, N.; Torrance, A.; Richardson, S. The attenuation of acoustic signals by aqueous and particulate foams. Appl. Acoust. 1988, 24, 193–209. [Google Scholar] [CrossRef]
- Yenus, J. Vibration and Sound Signal Analysis in Gas Stirred Ladles. Ph.D. Thesis, Swinburne University of Technology, Hawthorn, Australia, 2017. [Google Scholar]
- Wang, R.; Zhang, B.; Hu, C.; Liu, C.; Jiang, M. Study on splashing mechanism in basic oxygen furnace based on slag foaming modeling. Steel Res. Int. 2022, 93, 2100318. [Google Scholar] [CrossRef]
- Choudhary, S.K.; Ajmani, S.K. Evaluation of bottom stirring system in BOF steelmaking vessel using cold model study and thermodynamic analysis. ISIJ Int. 2006, 46, 1171–1176. [Google Scholar] [CrossRef] [Green Version]
- Sabah, S.; Brooks, G. Study of cavity modes in BOF by analysis of sound. Ironmak. Steelmak. 2016, 43, 473–480. [Google Scholar] [CrossRef]
- Rao, K.; Kim, D.; Hwang, J.J. Fast Fourier Transform—Algorithms and Applications, 1st ed.; Signals and Communication Technology; Springer: Dordrecht, The Netherlands, 2010. [Google Scholar]
- Fronckova, K.; Slaby, A. Kalman Filter Employment in Image Processing; Springer International Publishing: Cham, Switzerland, 2020; pp. 833–844. [Google Scholar]
- Batista, L.G.; Salarolli, P.F.; de Menezes, R.P.; Ayres, L.M.; Pereira, R.P.D.A.; de Cuadros, S.L.M.A.; Furtado, H.S. Slopping detection system for LD converters using sound signal digital and image processing. In Proceedings of the 2018 13th IEEE International Conference on Industry Applications (INDUSCON), Sao Paulo, Brazil, 12–14 November 2018. [Google Scholar]
- De Menezes, R.P.; Salarolli, P.F.; Batista, L.G.; Furtado, H.S.; Cuadros, M.A.S.L. Slopping index for LD converters based on sound and image data fusion by fuzzy Kalman filter. Ironmak. Steelmak. 2021, 49, 178–188. [Google Scholar] [CrossRef]
- Deo, B.; Overbosch, A.; Snoeijer, B.; Das, D.; Srinivas, K. Control of slag formation, foaming, slopping, and chaos in BOF. Trans. Indian Inst. Met. 2013, 66, 543–554. [Google Scholar] [CrossRef]
- Brämming, M.; Parker, G.; Millman, S.; Kapilashrami, A.; Malmberg, D.; Björkman, B. Comparison between vessel vibration and audiometry for slopping control in the Top-Blown BOS Process. Steel Res. Int. 2011, 82, 683–692. [Google Scholar] [CrossRef]
- Ghosh, B. Opto-Acoustic Slopping Prediction System in Basic Oxygen Furnace Converters; TRITA-ICT-EX; Kth Royal Institute of Technology: Stockholm, Sweden, 2017; p. 56. [Google Scholar]
- Shreve, D.H. Signal Processing for Effective Vibration Analysis; IRD Mechanalysis, Inc.: Columbus, OH, USA, 1995; pp. 1–11. [Google Scholar]
- Rao, K.D.; Swamy, M.N.S. Spectral Analysis of Signals. In Digital Signal Processing: Theory and Practice; Springer: Singapore, 2018; pp. 721–751. [Google Scholar]
- Kuttruff, H. Room Acoustics; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]


| Measuring Method | Location | Process Information | Time Delay |
|---|---|---|---|
| Camera surveillance/image analysis | Below the LD converter | Monitors the amount of falling slag to quantify slopping | None |
| Off gas analysis | Inside off gas system | Calculation of per centage of CO and CO2 plus the measured off-gas flow rate. | 15–20 s |
| Sonic Meter | In the hood above the mouth | The sound level indicates changes in slag level | None |
| Oxygen flow meter | In the lance | Gives the actual oxygen flow rate through the lance. | None |
| Lance height | In the lance control system | Gives the lance position as calculated by the lance system | None |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Heenatimulla, J.; Brooks, G.A.; Dunn, M.; Sly, D.; Snashall, R.; Leung, W. Acoustic Analysis of Slag Foaming in the BOF. Metals 2022, 12, 1142. https://doi.org/10.3390/met12071142
Heenatimulla J, Brooks GA, Dunn M, Sly D, Snashall R, Leung W. Acoustic Analysis of Slag Foaming in the BOF. Metals. 2022; 12(7):1142. https://doi.org/10.3390/met12071142
Chicago/Turabian StyleHeenatimulla, Jason, Geoffrey A. Brooks, Michelle Dunn, David Sly, Rod Snashall, and Wang Leung. 2022. "Acoustic Analysis of Slag Foaming in the BOF" Metals 12, no. 7: 1142. https://doi.org/10.3390/met12071142
APA StyleHeenatimulla, J., Brooks, G. A., Dunn, M., Sly, D., Snashall, R., & Leung, W. (2022). Acoustic Analysis of Slag Foaming in the BOF. Metals, 12(7), 1142. https://doi.org/10.3390/met12071142







