Effect of Axial Normal Stress and Bending Moment between Contact and Non-Contact Zone on Forming Accuracy for Flexible Stretch Bending Formation
Abstract
:1. Introduction
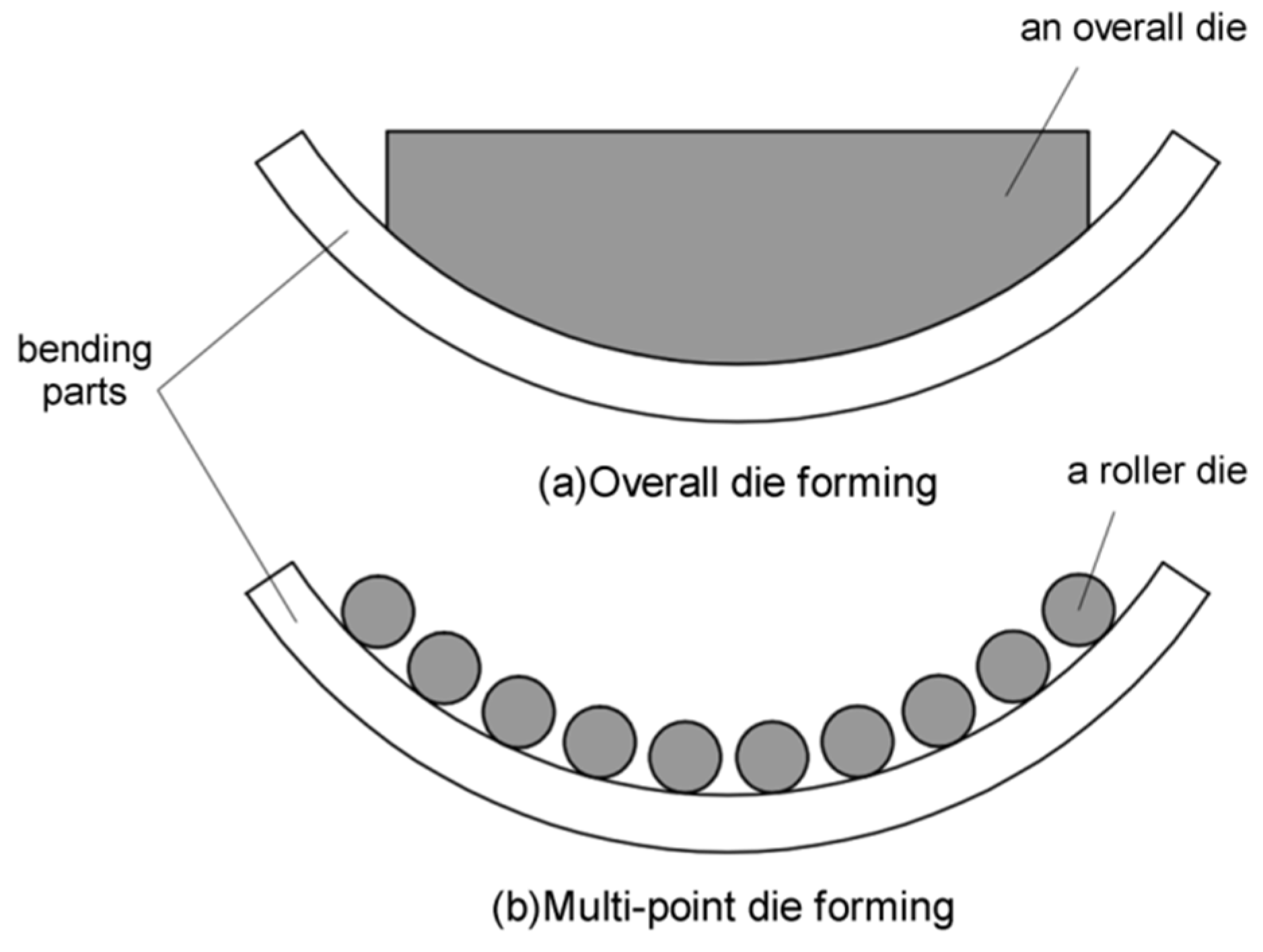
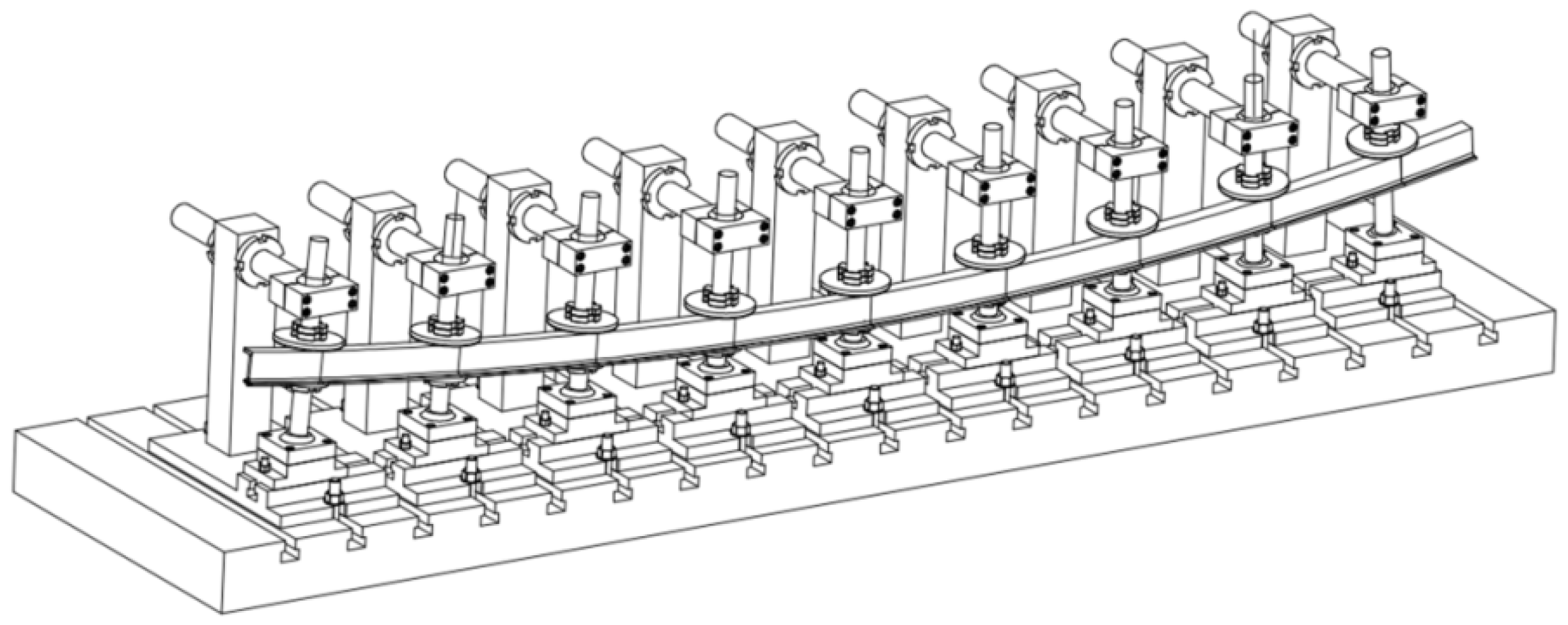
2. The Finite Element Simulation of FSB
2.1. The FSB Process
- (1)
- Adjust the position of the dies: In a multi-point die set, each die can be moved in vertical and horizontal positions. According to the shape of the target part, the position of each mold can be adjusted one by one. Thus, the whole set of multi-point molds can form the shape of the forming profile;
- (2)
- Torsion deformation: In order to adapt to the spatial position of the multi-point mold, before the profile is attached to the mold, the clamp drives the profile to rotate at an angle;
- (3)
- Stretch bending formation: One end of the profile is fixed by a clamp. At the same time, under the traction of the other end of the clamp, the profile is driven to complete the horizontal movement and vertical lifting. Make the profile fix the multi-point molds point by point to complete the space formation;
- (4)
- Clamp removing: After formation, the profile is removed from the mold to complete the preparation.
2.2. Model Parameters
2.3. Model Parameters
2.4. Design of Clamp Track
3. The Experiments and Numerical Simulation of FSB Process
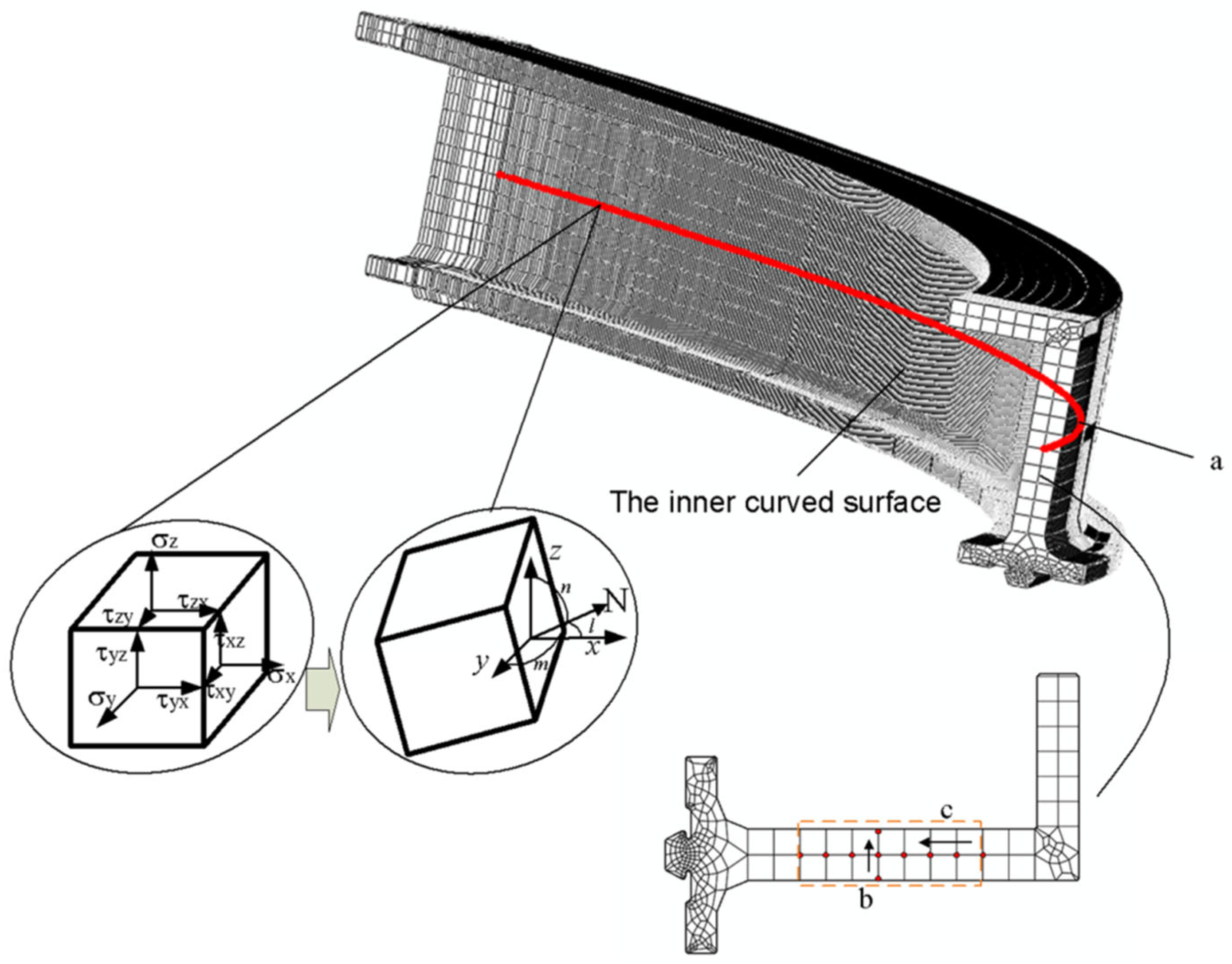
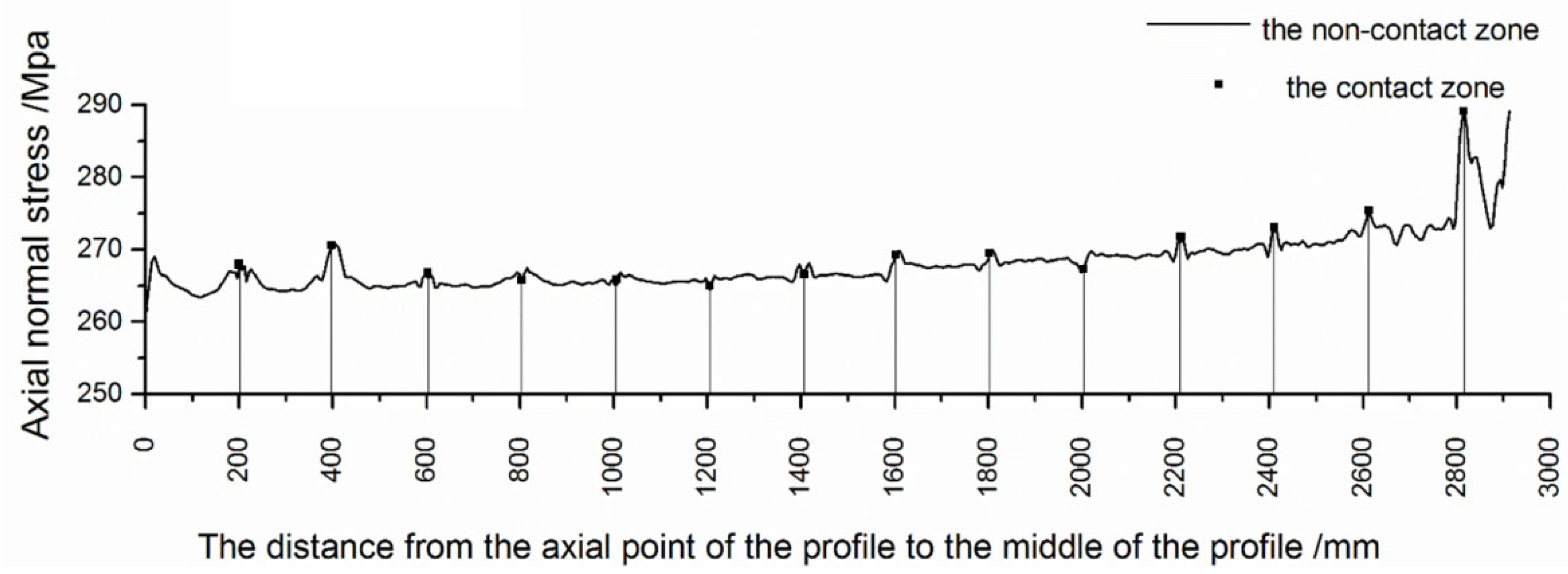
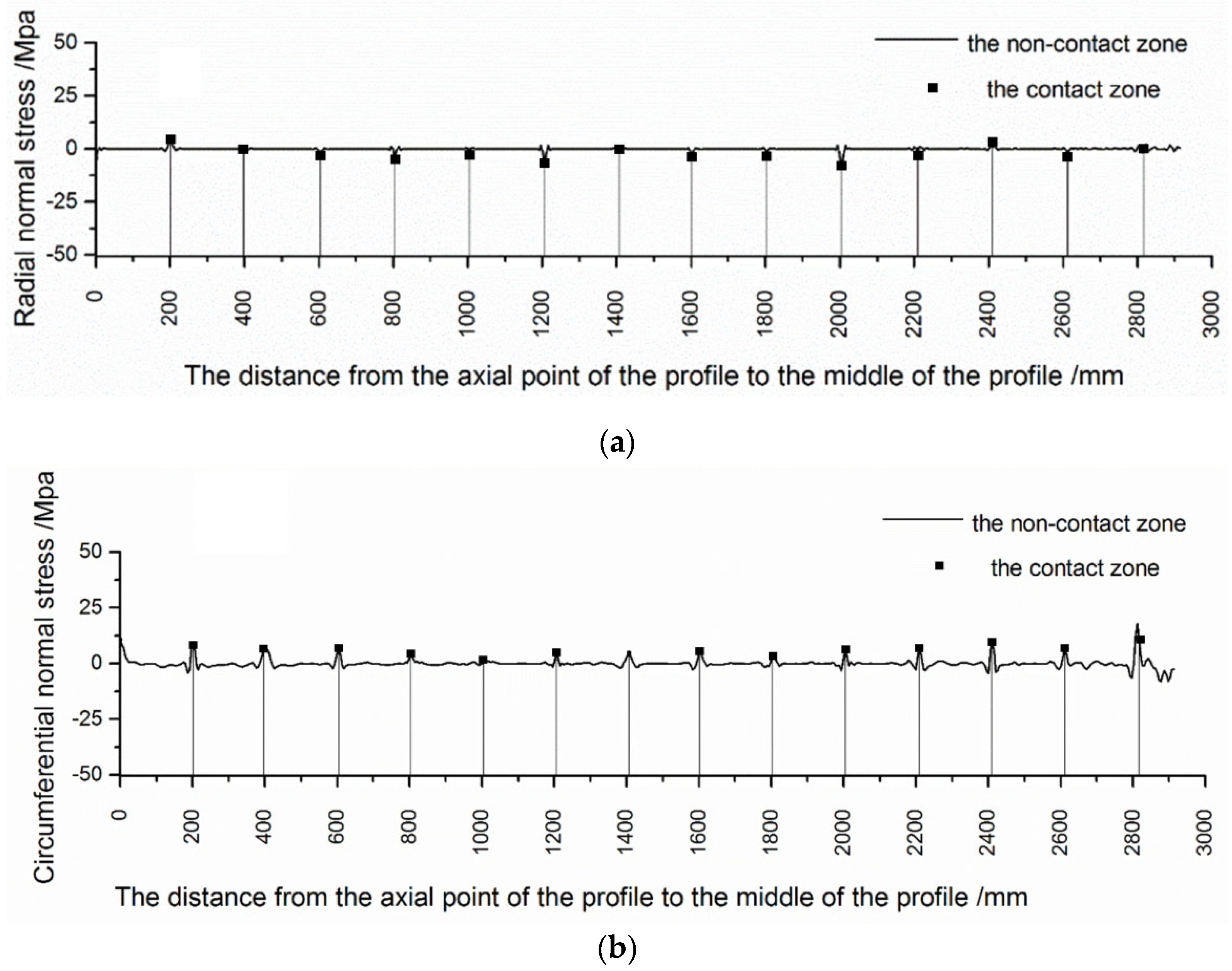
3.1. The Axial Normal Stress Analysis of Profile along Axial Direction
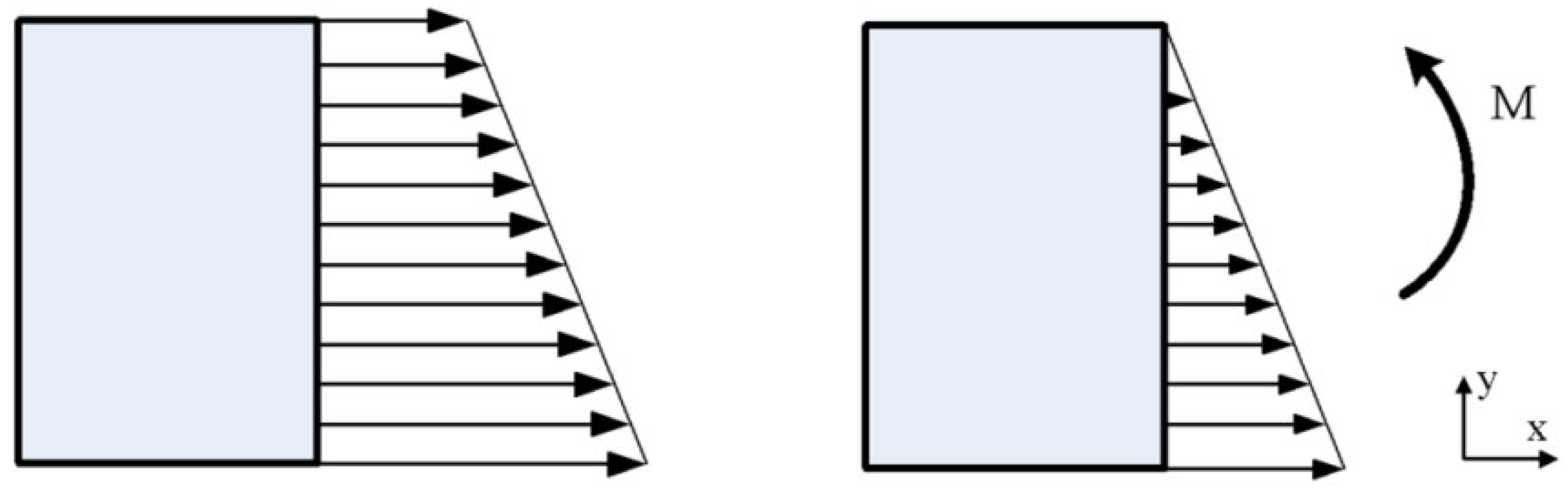
3.2. The Bending Moment Analysis of Profile along the Axial Direction
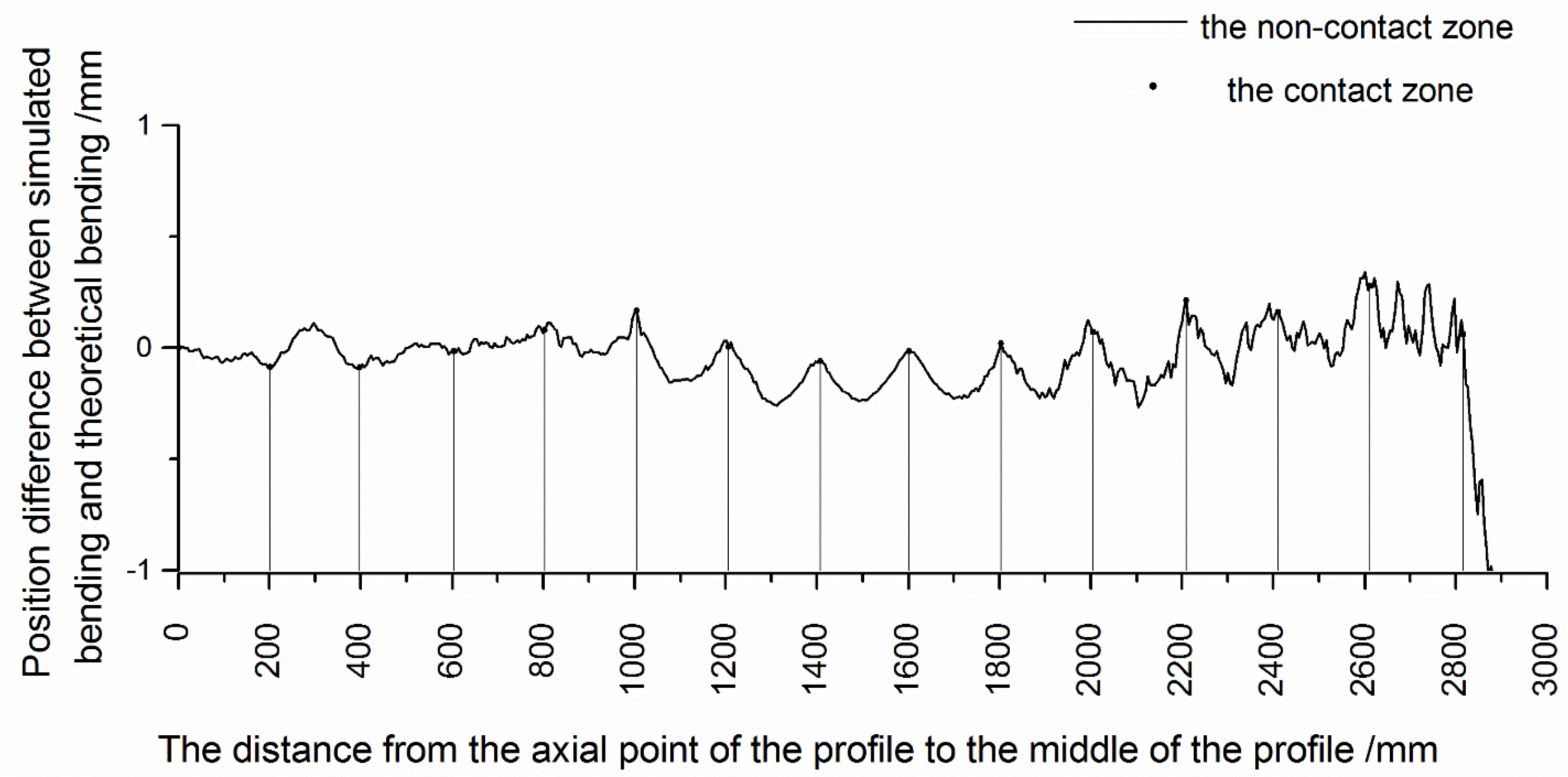
3.3. The Influence of Bending Moment Difference on Profile Deformation
3.4. The FSB Experiment
4. Conclusions
- (1)
- The axial normal stress in the contact zone was smaller than that in the non-contact zone, and the axial normal stress in the contact zone fluctuated slightly, which was slightly higher than that in the non-contact zone. The value of axial normal stress in the non-contact zone was relatively stable, and there was a small increase on the side near the clamp. Because of the support force and friction force given by the die, the longitudinal fibers of the profile produced normal stress, which made the cross-section slightly deformed and unable to keep the plane, meaning the axial normal stress distribution in the contact zone and non-contact zone was different.
- (2)
- By simulating the axial normal stress on different cross-sections in the middle of three groups of webs, it was found that the value of axial normal stress on the same cross-section had a linear relationship with the distance to the inner curved surface, but their normal stress parameters were different on different cross-sections.
- (3)
- The bending moment of the profile in the contact zone was obviously greater than that in the non-contact zone, and the bending moment gradually decreased to near zero from the contact zone to the non-contact zone. The bending deformation of the profile in the contact zone was obviously greater than that in the non-contact zone.
- (4)
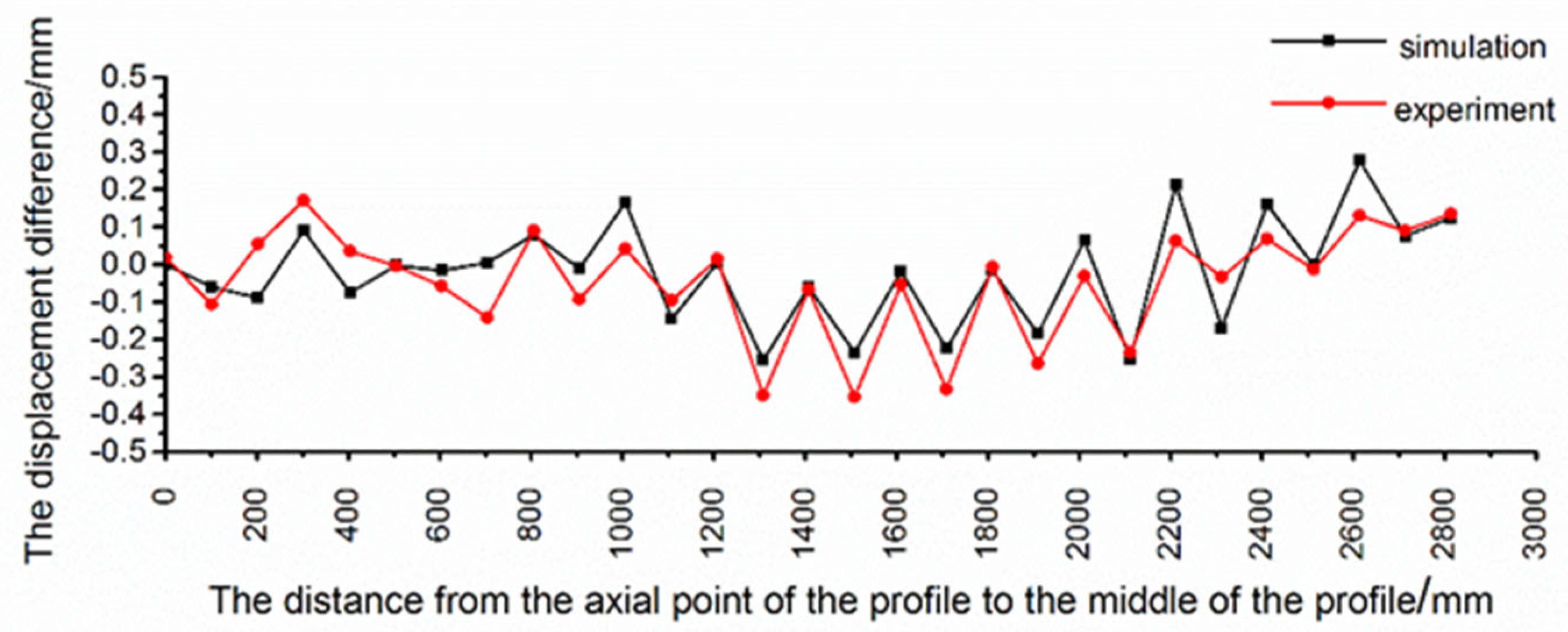
- The displacement of the profile in the contact area and the non-contact area was obviously different. In the non-contact area, the profile deviated from the theoretical bending position, while in the contact area, the offset of the profile decreased and was close to the theoretical bending position.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Corona, E. A simple analysis for bend-stretch forming of aluminum extrusion. Int. J. Mech. Sci. 2004, 46, 433–448. [Google Scholar] [CrossRef]
- Chatti, S.; Hermes, M.; Tekkaya, A.E.; Kleiner, M. The new TSS bending process: 3D bending of profiles with arbitrary cross-sections. CIRP Ann. Manuf. Technol. 2010, 59, 315–318. [Google Scholar] [CrossRef] [Green Version]
- Clausen, A.H.; Hopperstad, O.S.; Langseth, M. Stretch bending of aluminium extrusions for car bumpers. J. Mater. Process Technol. 2000, 102, 241–248. [Google Scholar] [CrossRef]
- Miller, J.E.; Kyriakides, S.; Bastard, A.H. On bend-stretch forming of aluminum extruded tubes—I: Experiments. Int. J. Mech. Sci. 2001, 43, 1283–1317. [Google Scholar] [CrossRef]
- Miller, J.E.; Kyriakides, S.; Corona, E. On bend-stretch forming of aluminum extruded tubes—II: Analysis. Int. J. Mech. Sci. 2001, 43, 1319–1338. [Google Scholar] [CrossRef]
- Li, M.Z.; Liu, Y.H.; Su, S.Z.; Li, G.Q. Multi-point forming: A flexible manufacturing method for a 3-d surface sheet. J. Mater. Process Technol. 1999, 87, 277–280. [Google Scholar] [CrossRef]
- Jicai, L.; Chengxiang, H.; Yi, L.; Kaifeng, Y.; Ce, L. Study on deformation difference between the contact zone and the non-contact zone of the flexible 3D stretch bending profile and roller dies based on pre-stretching amount. Int. J. Adv. Manuf. Technol. 2020, 108, 3579–3589. [Google Scholar]
- Yi, L.; Rui, L.; Ce, L.; Jicai, L.; Fei, T. Influence of the curvature of the multipoint die of flexible multipoint stretch bending on the quality of aluminum profile. Math. Probl. Eng. 2020, 5, 960–973. [Google Scholar]
- Liu, W.; Yang, Y.Y.; Li, M.Z. Numerical simulation of multi-point stretch forming and controlling on accuracy of formed workpiece. Int. J. Adv. Manuf. Technol. 2010, 50, 61–66. [Google Scholar] [CrossRef]
- Chen, J.; Fu, W.Z.; Li, M.Z. Local bending effect and its controlling methods in multi-point forming. Int. J. Adv. Manuf. Technol. 2017, 92, 4315–4322. [Google Scholar] [CrossRef]
- Yang, Z.; Cai, Z.Y.; Che, C.J.; Li, M.Z. Numerical simulation research on the loading trajectory in stretch forming process based on distributed displacement loading. Int. J. Adv. Manuf. Technol. 2016, 82, 1353–1362. [Google Scholar] [CrossRef]
- Zhou, Y.; Li, P.; Li, M.; Wang, L. Application and correction of L-shaped thin-wall aluminum in flexible-bending processing. Int. J. Adv. Manuf. Technol. 2017, 92, 981–988. [Google Scholar] [CrossRef]
- Jicai, L.; Yanfei, L.; Li, Y.I.; Ce, L. Study on the influence of bending angle of multipoint stretch-bending of profiles on section distortion of parts. Math. Probl. Eng. 2008, 2002, 1975805. [Google Scholar]
- Abosaf, M.; Essa, K.; Alghawail, A.; Tolipov, A.; Pham, D. Optimisation of multi-point forming process parameters. Int. J. Adv. Manuf. Technol. 2017, 92, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Miyazaki, K.; Kanno, S.; Ishiwata, M.; Hasegawa, K.; Ahn, S.H.; Ando, K. Fracture behavior of carbon steel pipe with local wall thinning subjected to bending load. Nucl. Eng. Des. 1999, 191, 195–204. [Google Scholar] [CrossRef]
- Tang, N.C. Plastic-deformation analysis in tube bending. Int. J. Press. Ves. Pip. 2000, 77, 751–759. [Google Scholar] [CrossRef]
- Daniel, I.M.; Gdoutos, E.E.; Wang, K.A.; Abot, J.L. Failure Modes of Composite Sandwich Beams. Int. J. Damage Mech. 2002, 11, 309–334. [Google Scholar] [CrossRef]
- Jicai, L.; Chuandong, C.; Yi, L.; Ce, L. Effect of roller dies on springback law of profile for flexible 3D multi-point stretch bending. Int. J. Adv. Manuf. Technol. 2020, 108, 3765–3777. [Google Scholar]
- Hopperstad, O.S.; Leira, B.J.; Remseth, S. Reliability-based analysis of a stretch-bending process for aluminum extrusions. Comput. Struct. 1999, 71, 63–75. [Google Scholar] [CrossRef]
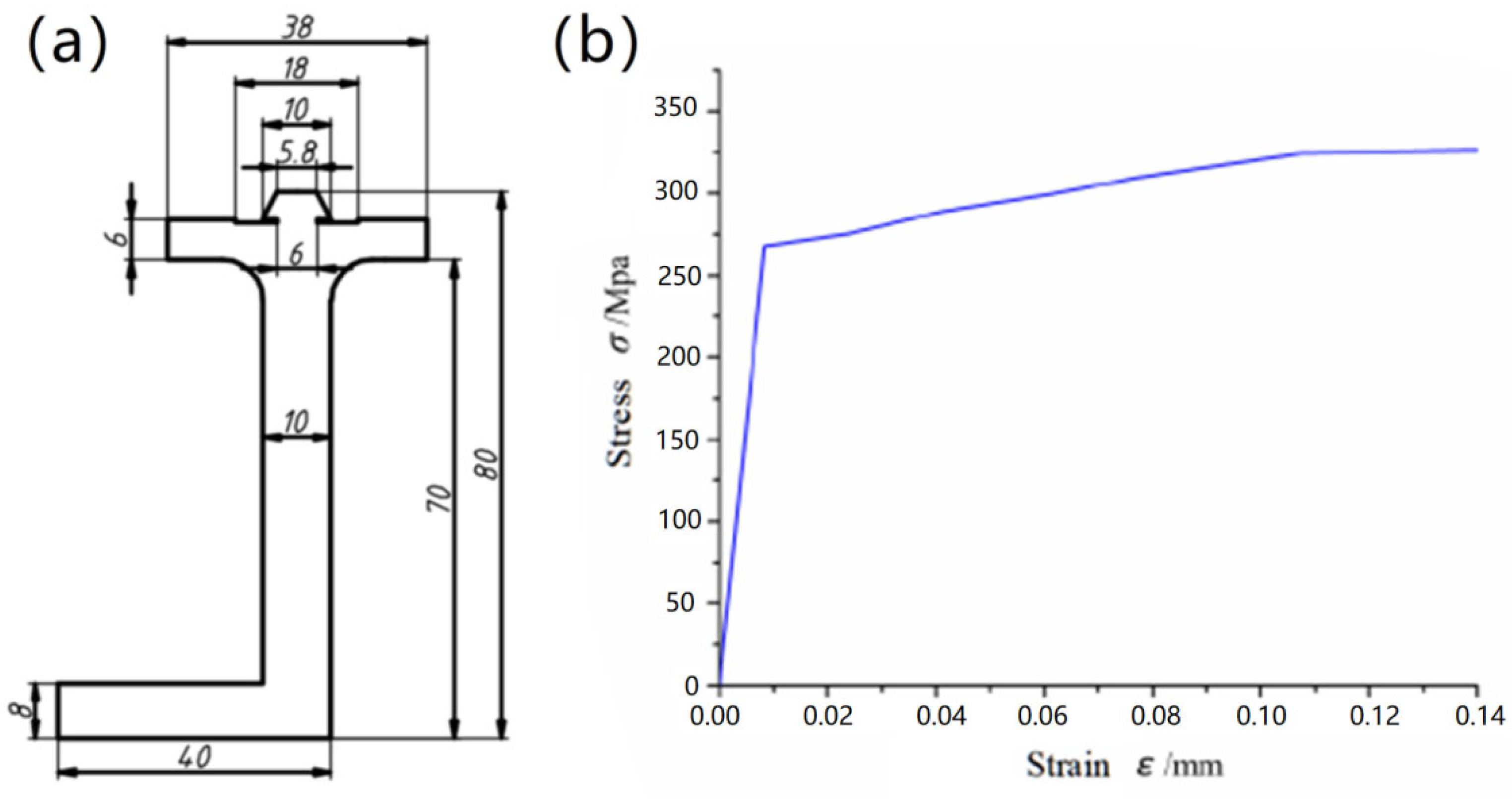








| E (MPa) | ν | σb (MPa) | Ρ (kg·m−3) |
|---|---|---|---|
| 71,000 | 0.33 | 264 | 2.71 |
| Finite Element Parts | Unit Type | Number of Units | Number of Nodes |
|---|---|---|---|
| Profile | C3D8R | 69,600 | 96,761 |
| Multi-point mold | R3D4 | 2733 | 2708 |
| Clamp | R3D4 | 138 | 180 |
| Bracket | R3D4 | 168 | 180 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Teng, F.; Liang, J.; Wang, S.; Han, Q. Effect of Axial Normal Stress and Bending Moment between Contact and Non-Contact Zone on Forming Accuracy for Flexible Stretch Bending Formation. Metals 2022, 12, 1168. https://doi.org/10.3390/met12071168
Teng F, Liang J, Wang S, Han Q. Effect of Axial Normal Stress and Bending Moment between Contact and Non-Contact Zone on Forming Accuracy for Flexible Stretch Bending Formation. Metals. 2022; 12(7):1168. https://doi.org/10.3390/met12071168
Chicago/Turabian StyleTeng, Fei, Jicai Liang, Shaoqiang Wang, and Qigang Han. 2022. "Effect of Axial Normal Stress and Bending Moment between Contact and Non-Contact Zone on Forming Accuracy for Flexible Stretch Bending Formation" Metals 12, no. 7: 1168. https://doi.org/10.3390/met12071168
APA StyleTeng, F., Liang, J., Wang, S., & Han, Q. (2022). Effect of Axial Normal Stress and Bending Moment between Contact and Non-Contact Zone on Forming Accuracy for Flexible Stretch Bending Formation. Metals, 12(7), 1168. https://doi.org/10.3390/met12071168






