Computational Analysis of the Influence of Residual Stress on the Strength of Composites with Different Aluminum Matrices and Carbide Particles
Abstract
:1. Introduction
2. Formulation of the Problem
2.1. Elastic–Plastic Response of the Matrix Material
2.2. Elastic–Brittle Behavior of the Particle Material
2.3. Numerical Details, Initial and Boundary Conditions
- Equations (1)–(5) are integrated into ABAQUS/Explicit software by the user-defined subroutine VUMAT, an example of which as a FORTRAN file is available in ABAQUS Documentation. This code calculates stress tensor components in every element of the computational domain and at each time step of the dynamic boundary value problem. The subroutine is modified to satisfy the plane stress conditions, in the case of which the third stress tensor component is equal to zero and the third strain tensor component depends on the first and second ones.
- An aluminum microvolume with a single particle (Figure 1a) is discretized by a regular rectilinear mesh containing 600 × 468 square finite elements of the CPS4R type available in ABAQUS/Explicit. To this end, the experimental image is reduced to a two-phase pixel map (Figure 1b). Using an in-house program, we transform the pixel map into an orphan voxel mesh (Figure 1c) which is written in an ABAQUS input file (*.inp) along with the matrix and particle material properties, calculation steps and boundary conditions (cooling and tension/compression).
- The *.inp file is exported to ABAQUS CAE, where the model can further be modified if necessary and then submitted for analysis by the JOB module attaching the user subroutine file developed. An explicit central difference time integration rule is used for calculating accelerations at the current time step to advance the displacement solution to the next step of time.
2.4. Validation of the Model Parameters
2.5. Verification of Simulation Results
3. Numerical Simulation Results
3.1. Effects of Thermomechanical Properties of the Matrix and Particle Materials
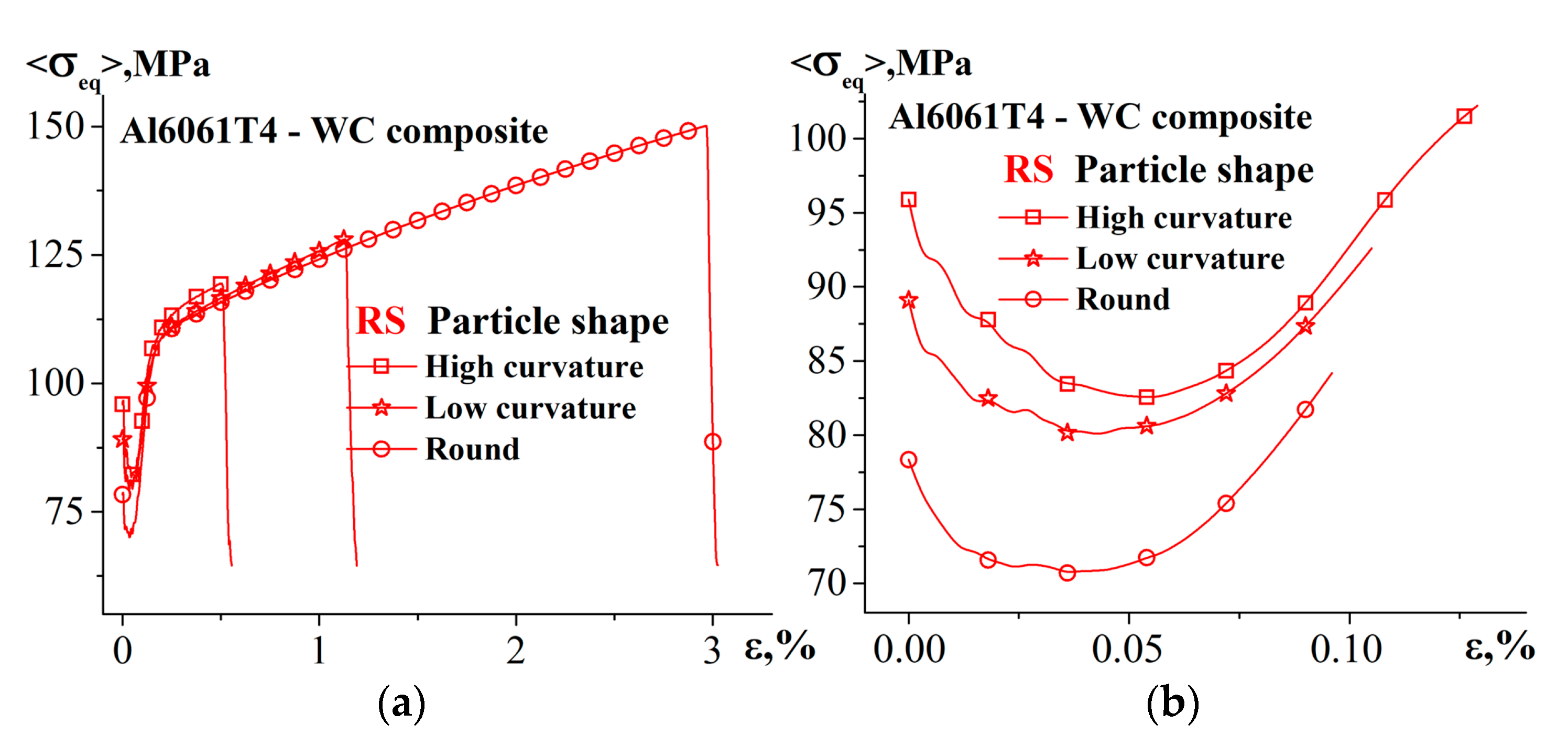
3.2. Particle Shape and Volume Fraction
4. Conclusions
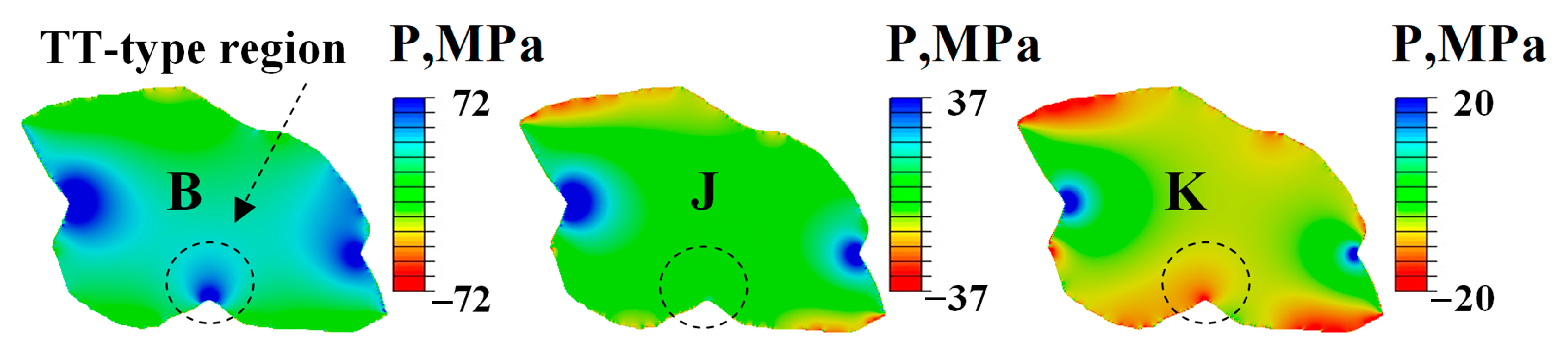
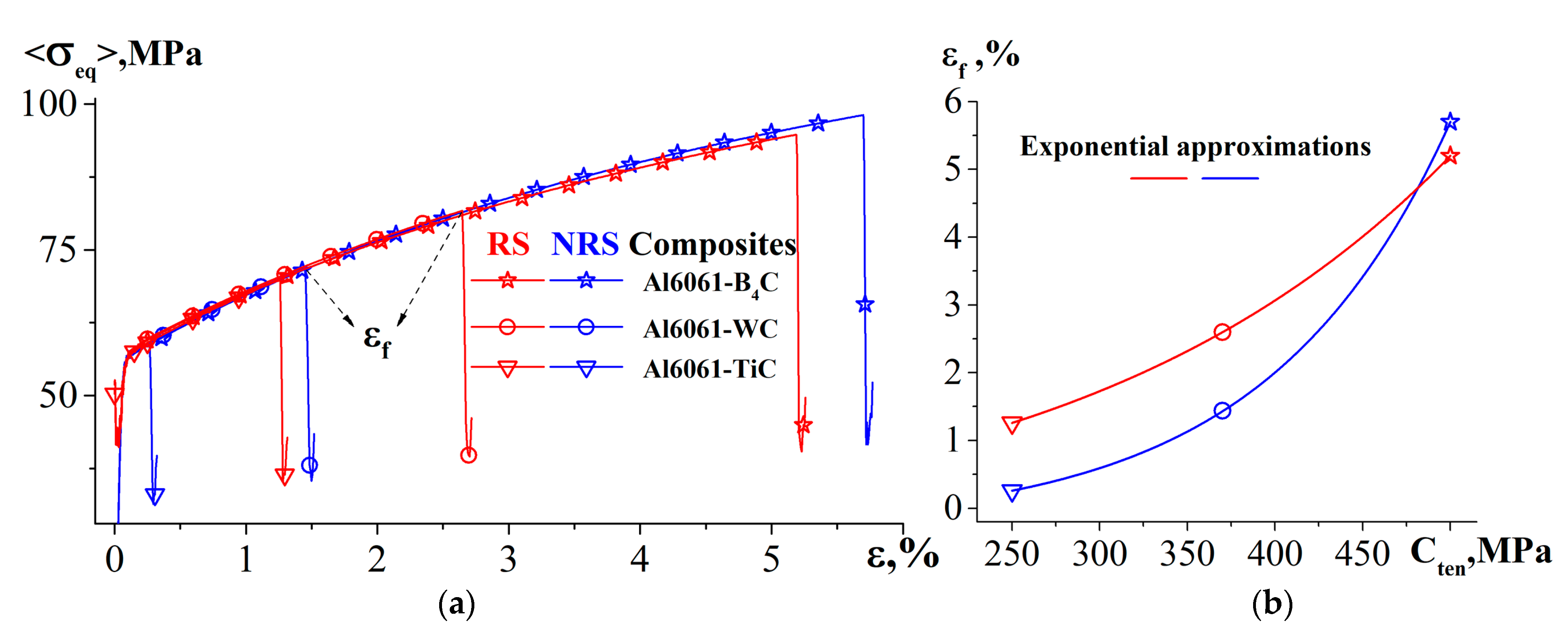
- Residual stresses and plastic strains develop after cooling the composites from the recrystallization temperature of 350 to 23 °C. For a variety of aluminum matrix and carbide particle materials, it is verified that the particle is volumetrically compressed and different regions of the matrix experience either tension or compression.
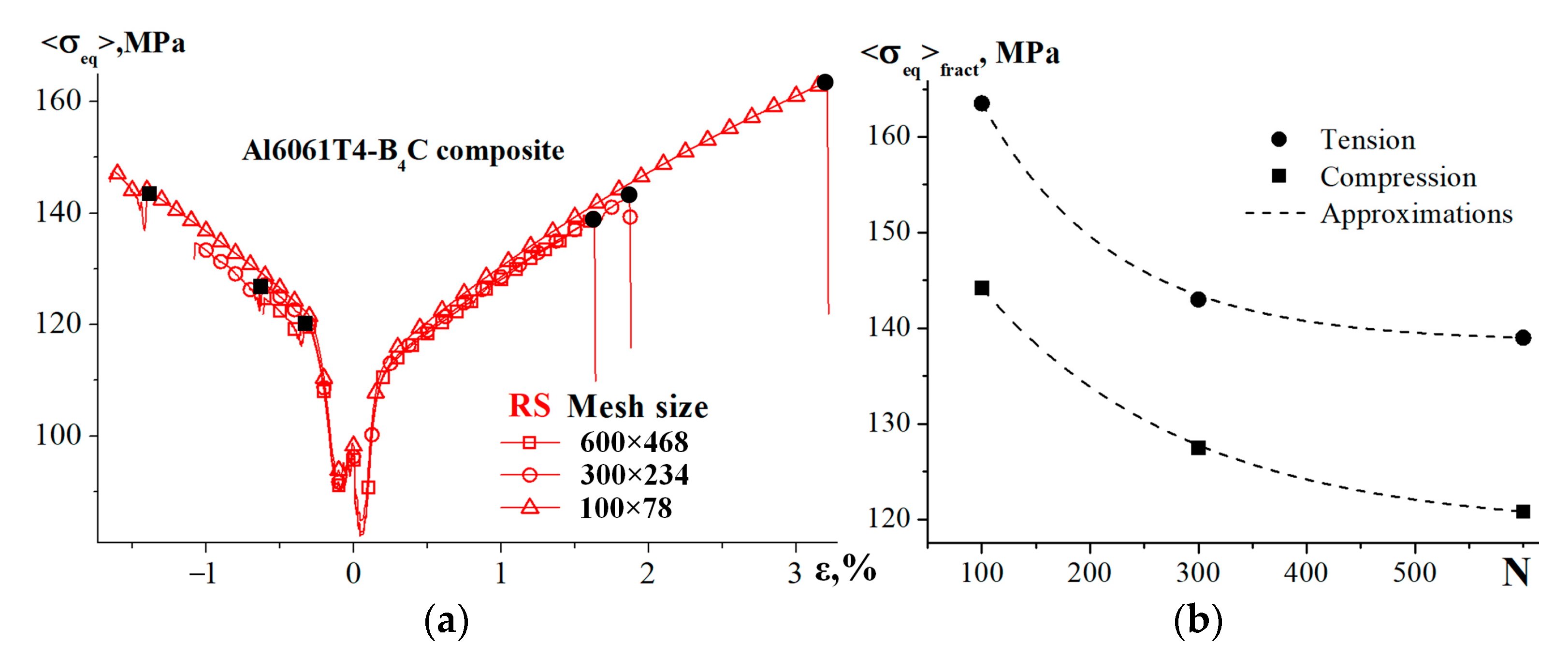
- Staging of the stress–strain curves of the composites subjected to cooling followed by tension was found. Stage 1 is characterized by a stress drop attributed to the evolution of a multiaxial stress state formed during the cooling of the composite into a uniaxial tension. A monotonic increase in the stress concentration and plastic strain localization in the particle and matrix is observed in stage 2. The composite fractures in stage 3.
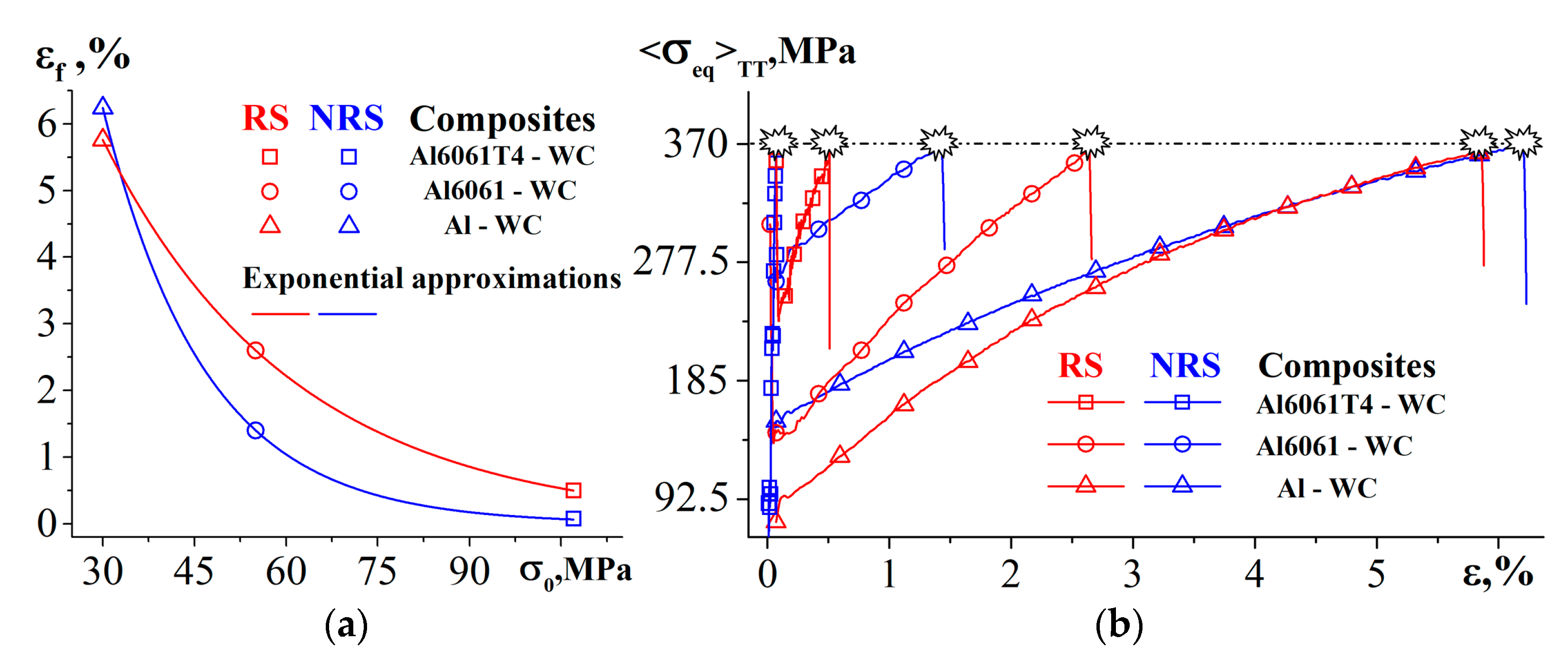
- The composite’s elongation to fracture is found to increase exponentially with the decreasing yield stress of the matrix and the increasing strength of the particles. The cooling-induced residual stresses have a positive effect on the strength of the composites with hard matrices and low-strength particles.
- The lower the curvature of the matrix–particle interface the more the particle tends to debonding rather than in-particle cracking. This results in an increase in the composite’s elongation to fracture with decreasing interfacial curvature.
- The higher the volume fraction of particles the lower the cooling-induced tensile residual stresses in the matrix and the higher the compressive stresses in the particle, causing a larger composite’s elongation to fracture.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Selvam, J.D.R.; Dinaharan, I.; Rai, R.S. Matrix and Reinforcement Materials for Metal Matrix Composites. In Encyclopedia of Materials: Composites, 1st ed.; Brabazon, D., Ed.; Elsevier Science Publishing Co Inc.: Amsterdam, The Netherlands, 2021; pp. 615–639. [Google Scholar]
- Panemangalore, D.B.; Shabadi, R. Microstructural Aspects of Metal-Matrix Composites. In Encyclopedia of Materials: Composites, 1st ed.; Brabazon, D., Ed.; Elsevier Science Publishing Co Inc.: Amsterdam, The Netherlands, 2021; pp. 274–297. [Google Scholar]
- Mertens, A.I. Metal Matrix Composites Processed by Laser Additive Manufacturing: Microstructure and Properties. Chapter 13. In Handbooks in Advanced Manufacturing, 1st ed.; Pou, J., Riveiro, A., Davim, J.P., Eds.; Elsevier: Amsterdam, The Netherlands, 2021; pp. 409–425. [Google Scholar]
- Kumar, J.; Singh, D.; Kalsi, N.S.; Sharma, S.; Pruncu, C.I.; Pimenov, D.Y.; Rao, K.V.; Kapłonek, W. Comparative study on the mechanical, tribological, morphological and structural properties of vortex casting processed, Al–SiC–Cr hybrid metal matrix composites for high strength wear-resistant applications: Fabrication and characterizations. J. Mater. Res. Technol. 2020, 9, 13607–13615. [Google Scholar] [CrossRef]
- Moktar, M.S.; Ghandvar, H.; Bakar, T.A.A. Development and Characterization of Aluminum Hybrid Metal Matrix Composites Used in Automotive Applications. In Encyclopedia of Renewable and Sustainable Materials, 1st ed.; Choudhury, I., Hashmi, S., Eds.; Elsevier: Amsterdam, The Netherlands, 2020; Volume 5, pp. 54–63. [Google Scholar]
- Samal, P.; Vundavilli, P.R.; Meher, A.; Mahapatra, M.M. Recent progress in aluminum metal matrix composites: A review on processing, mechanical and wear properties. J. Manuf. Process. 2020, 59, 131–152. [Google Scholar] [CrossRef]
- Ramanathan, A.; Krishnan, P.K.; Muraliraja, R. A review on the production of metal matrix composites through stir casting—Furnace design, properties, challenges, and research opportunities. J. Manuf. Process. 2019, 42, 213–245. [Google Scholar] [CrossRef]
- Al-Hamdani, K.S.; Murray, J.W.; Hussain, T.; Clare, A.T. Controlling ceramic-reinforcement distribution in laser cladding of MMCs. Surf. Coat. Technol. 2020, 381, 125128. [Google Scholar] [CrossRef]
- Wang, Q.; Shi, J.; Zhang, L.; Tsutsumi, S.; Feng, J.; Ma, N. Impacts of laser cladding residual stress and material properties of functionally graded layers on titanium alloy sheet. Addit. Manuf. 2020, 35, 101303. [Google Scholar] [CrossRef]
- Sankhla, A.M.; Patel, K.M.; Makhesana, M.A.; Giasin, K.; Pimenov, D.Y.; Wojciechowski, S.; Khanna, N. Effect of mixing method and particle size on hardness and compressive strength of aluminium based metal matrix composite prepared through powder metallurgy route. J. Mater. Res. Technol. 2022, 18, 282–292. [Google Scholar] [CrossRef]
- Sun, R.; Cao, Z.; Che, Z.; Zhang, H.; Zou, S.; Wu, J.; Guo, W. A combined experimental-numerical study of residual stress and its relaxation on laser shock peened SiC particle-reinforced 2009 aluminum metal matrix composites. Surf. Coat. Technol. 2022, 430, 127988. [Google Scholar] [CrossRef]
- Fomin, V.M.; Golyshev, A.A.; Kosarev, V.F.; Malikov, A.G.; Orishich, A.M.; Filippov, A.A. Deposition of Cermet Coatings on the Basis of Ti, Ni, WC, and B4C by Cold Gas Dynamic Spraying with Subsequent Laser Irradiation. Phys. Mesomech. 2020, 23, 291–300. [Google Scholar] [CrossRef]
- Suo, Y.; Wang, B.; Jia, P.; Gong, Y. Numerical analysis of mechanical properties and particle cracking probability of metal matrix composites. Mater. Today Commun. 2020, 24, 101082. [Google Scholar] [CrossRef]
- Wu, Q.; Xu, W.; Zhang, L. Microstructure-based modelling of fracture of particulate reinforced metal matrix composites. Compos. B Eng. 2019, 163, 384–392. [Google Scholar] [CrossRef]
- Balokhonov, R.R.; Romanova, V.A.; Schmauder, S.; Emelianova, E.S. A numerical study of plastic strain localization and fracture across multiple spatial scales in materials with metal-matrix composite coatings. Theor. Appl. Fract. Mech. 2019, 101, 342–355. [Google Scholar] [CrossRef]
- Balokhonov, R.; Romanova, V.; Kulkov, A. Microstructure-based analysis of deformation and fracture in metal-matrix composite materials. Eng. Fail Anal. 2020, 110, 104412. [Google Scholar] [CrossRef]
- Peng, P.; Gao, M.; Guo, E.; Kang, H.; Xie, H.; Chen, Z.; Wang, T. Deformation behavior and damage in B4Cp/6061Al composites: An actual 3D microstructure-based modeling. Mater. Sci. Eng. A 2020, 781, 139169. [Google Scholar] [CrossRef]
- Sun, Q.; Asqardoust, S.; Sarmah, A.; Jain, M.K. Elastoplastic analysis of AA7075-O aluminum sheet by hybrid micro-scale representative volume element modeling with really-distributed particles and in-situ SEM experimental testing. J. Mater. Sci. Technol. 2022, 123, 201–221. [Google Scholar] [CrossRef]
- Balokhonov, R.; Romanova, V.; Schwab, E.; Zemlianov, A.; Evtushenko, E. Computational Microstructure-Based Analysis of Residual Stress Evolution in Metal-Matrix Composite Materials during Thermomechanical Loading. Facta Univ. Mech. Eng. 2021, 19, 241–252. [Google Scholar] [CrossRef]
- Zhang, J.F.; Zhang, X.X.; Andrä, H.; Wang, Q.Z.; Xiao, B.L.; Ma, Z.Y. A fast numerical method of introducing the strengthening effect of residual stress and strain to tensile behavior of metal matrix composites. J. Mater. Sci. Technol. 2021, 87, 167–175. [Google Scholar] [CrossRef]
- González-Lezcano, R.A.; del Río-Campos, J.M.; Awad Parada, T. Influence of Thermal Residual Stresses on the Behaviour of Metal Matrix Composite Materials. Iran J. Sci. Technol. Trans. Mech. Eng. 2023. [Google Scholar] [CrossRef]
- Balokhonov, R.R.; Romanova, V.A.; Buyakova, S.P.; Kulkov, A.S.; Bakeev, R.A.; Evtushenko, E.P.; Zemlyanov, A.V. Deformation and Fracture Behavior of Particle-Reinforced Metal Matrix Composites and Coatings. Phys. Mesomech. 2022, 25, 492–504. [Google Scholar] [CrossRef]
- Shackelford, J.F.; Han, Y.-H.; Kim, S.; Kwon, S.-H. CRC Materials Science and Engineering Handbook, 4th ed.; CRC Press: Boca Raton, FL, USA, 2015; 634p. [Google Scholar]
- Domnich, V.; Reynaud, S.; Haber, R.A.; Chhowalla, M. Boron Carbide: Structure, Properties, and Stability under Stress. J. Am. Ceram. Soc. 2011, 94, 3605–3628. [Google Scholar] [CrossRef]
- Chang, R.; Graham, L.J. Low-Temperature Elastic Properties of ZrC and TiC. J. Appl. Phys. 1966, 37, 3778–3783. [Google Scholar] [CrossRef]
- ASM Handbook Committee. Properties of Wrought Aluminum and Aluminum Alloys. In ASM Handbook, Volume 2: Properties and Selection: Nonferrous Alloys and Special-Purpose Materials; ASM International: Almere, The Netherlands, 1990; pp. 62–122. [Google Scholar]
- Balasundaram, A.; Gokhale, A.M.; Graham, S.; Horstemeyer, M.F. Three-dimensional particle cracking damage development in an Al–Mg–base wrought alloy. Mater. Sci. Eng. A 2003, 355, 368–383. [Google Scholar] [CrossRef] [Green Version]
- Berasategi, J.; Gomez, A.; Bou-Ali, M.M.; Gutiérrez, J.; Barandiarán, J.M.; Beketov, I.V.; Safronov, A.P.; Kurlyandskaya, G.V. Fe nanoparticles produced by electric explosion of wire for new generation of magneto-rheological fluids. Smart Mater. Struct. 2018, 27, 045011. [Google Scholar] [CrossRef]


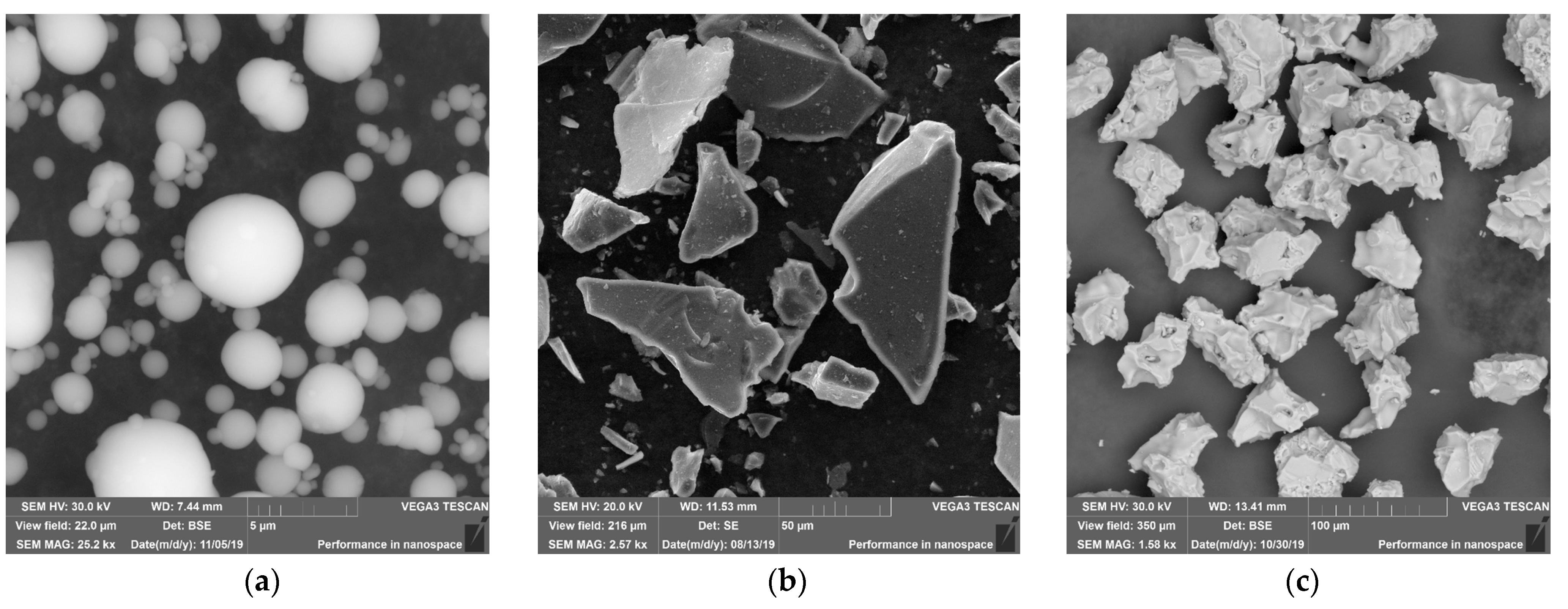










| Material | ρ, g/cm3 | K, GPa | µ, GPa | σS, MPa | σ0, MPa | εpr, % | Cten, MPa | α, 10−6 °C−1 |
|---|---|---|---|---|---|---|---|---|
| Matrices | ||||||||
| Al | 2.7 | 66 | 26 | 82 | 30 | 10 | - | 22 |
| Al6061 | 2.7 | 66 | 26 | 125 | 55 | 9.9 | - | 22 |
| Al6061T4 | 2.7 | 66 | 26 | 231 | 107 | 9.8 | - | 22 |
| Particles | ||||||||
| TiC | 4.9 | 234 | 185 | - | - | - | 250 | 9.5 |
| WC | 15.6 | 370 | 260 | - | - | - | 370 | 5 |
| B4C | 2.6 | 235 | 197 | - | - | - | 500 | 4.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Balokhonov, R.; Zemlianov, A.; Gatiyatullina, D.; Romanova, V. Computational Analysis of the Influence of Residual Stress on the Strength of Composites with Different Aluminum Matrices and Carbide Particles. Metals 2023, 13, 724. https://doi.org/10.3390/met13040724
Balokhonov R, Zemlianov A, Gatiyatullina D, Romanova V. Computational Analysis of the Influence of Residual Stress on the Strength of Composites with Different Aluminum Matrices and Carbide Particles. Metals. 2023; 13(4):724. https://doi.org/10.3390/met13040724
Chicago/Turabian StyleBalokhonov, Ruslan, Aleksandr Zemlianov, Diana Gatiyatullina, and Varvara Romanova. 2023. "Computational Analysis of the Influence of Residual Stress on the Strength of Composites with Different Aluminum Matrices and Carbide Particles" Metals 13, no. 4: 724. https://doi.org/10.3390/met13040724
APA StyleBalokhonov, R., Zemlianov, A., Gatiyatullina, D., & Romanova, V. (2023). Computational Analysis of the Influence of Residual Stress on the Strength of Composites with Different Aluminum Matrices and Carbide Particles. Metals, 13(4), 724. https://doi.org/10.3390/met13040724






