Numerical Simulation of the Flow Field in an Ultrahigh-Speed Continuous Casting Billet Mold
Abstract
:1. Introduction
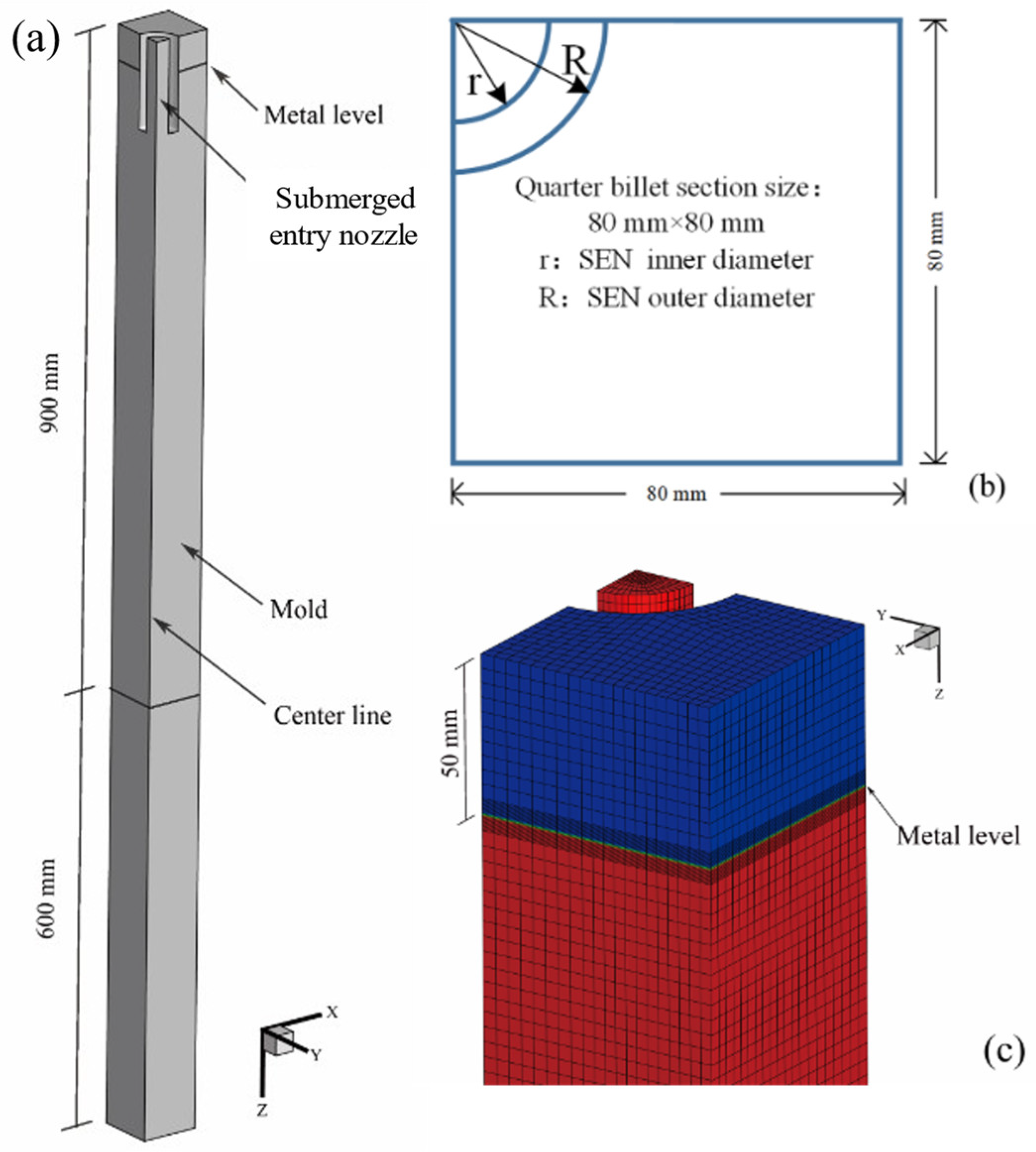
2. Model Development
2.1. Basic Assumptions
- The slag and molten steel in a mold are transient, incompressible, Newtonian fluids.
- The influence of external conditions, such as mold oscillation and argon blowing on the flow field in a mold, are ignored.
- Thermal buoyancy due to temperature differences is ignored.
- The molten steel in a mold is considered a homogeneous medium [27].
- The molten steel’s composition remains unchanged, and the slag’s solidification is ignored in continuous casting.
2.2. Governing Equations
2.3. Model Domain and Boundary Conditions
3. Results and Discussion
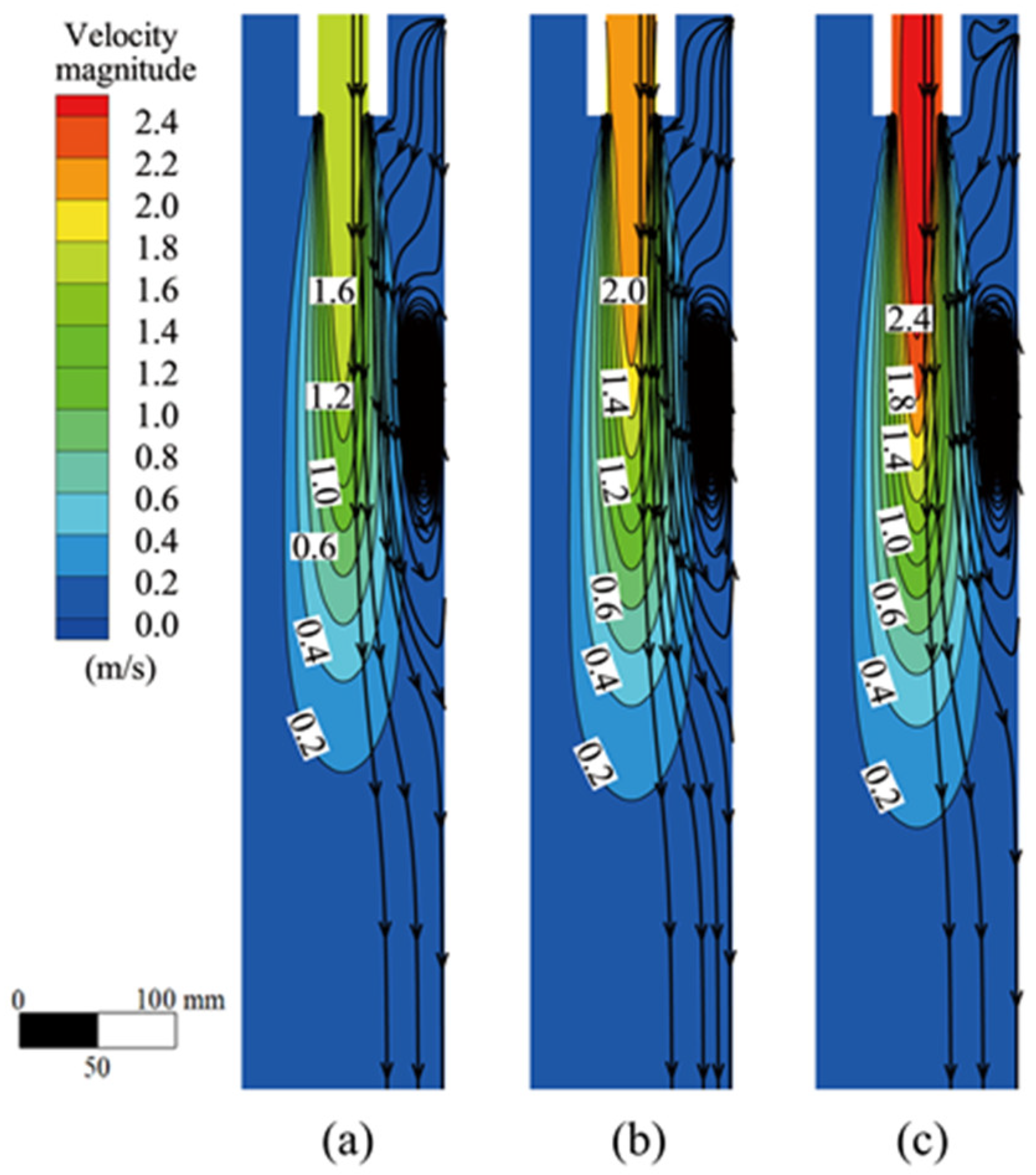
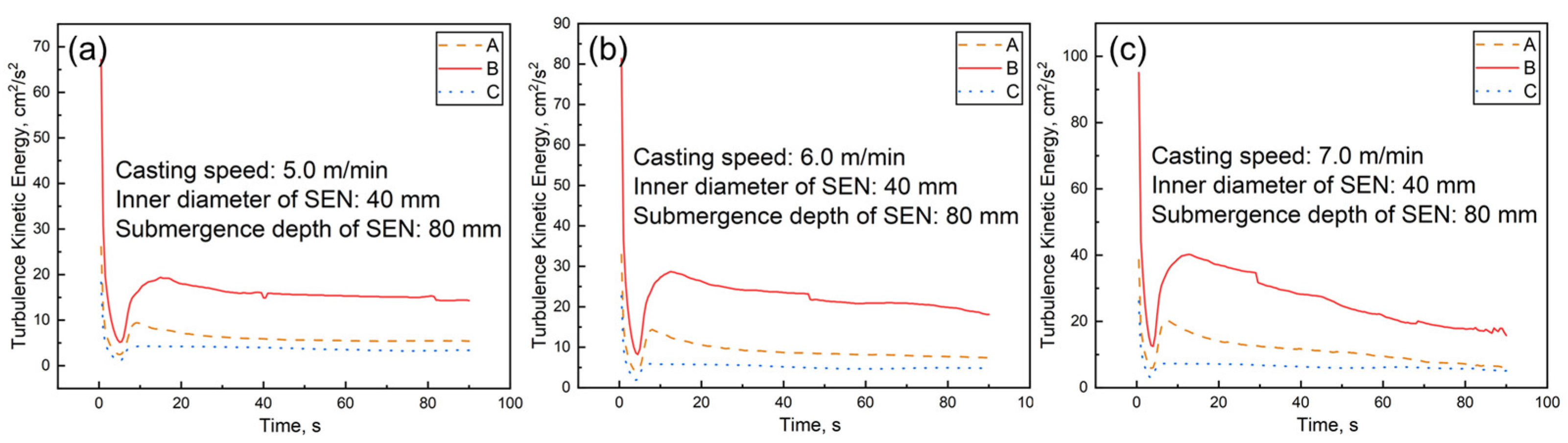
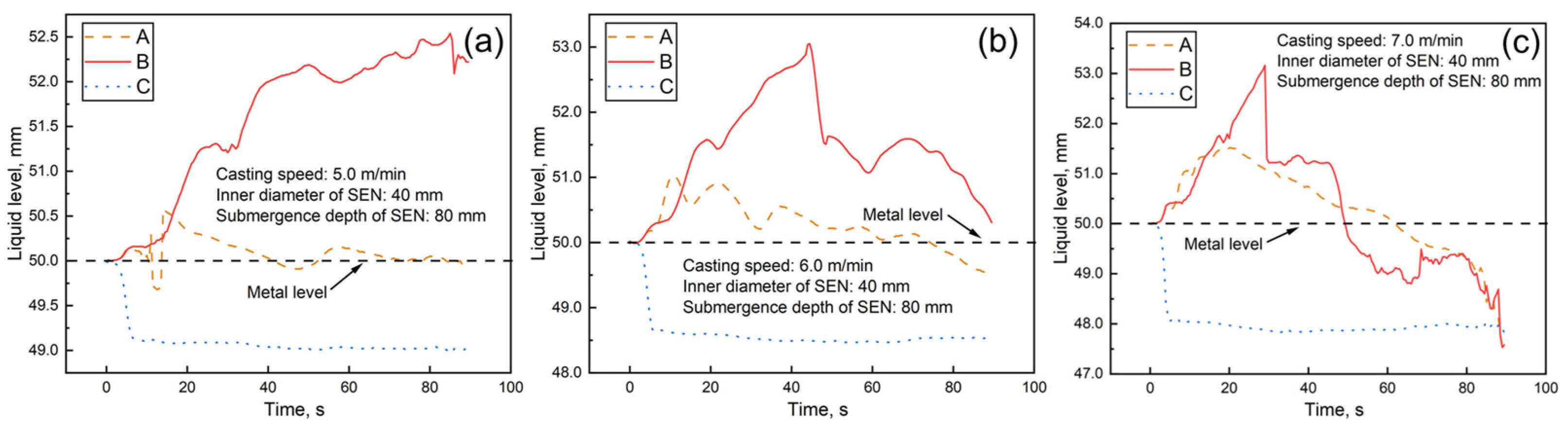
3.1. Effects of Casting Speeds
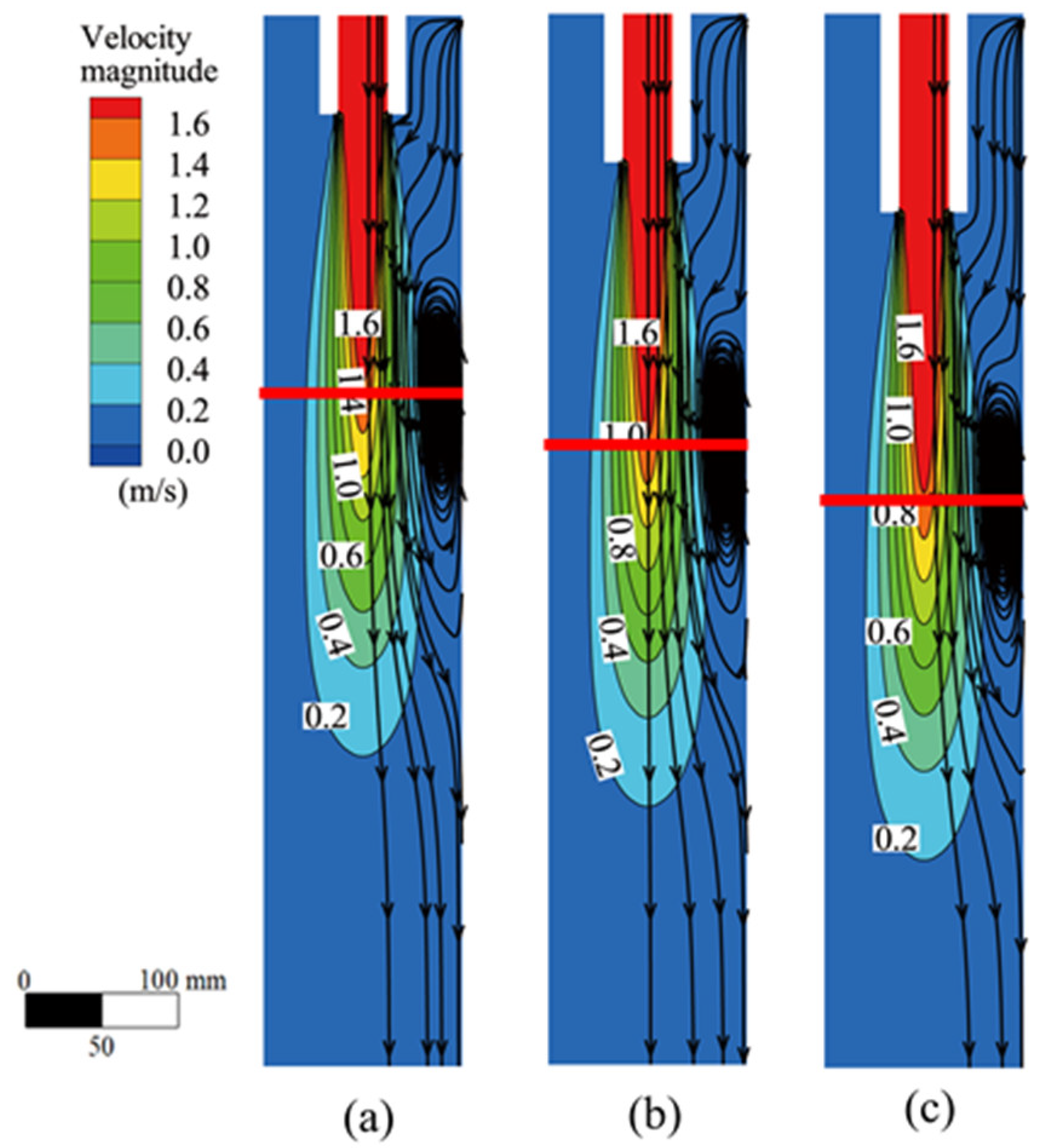
3.2. Effects of SEN Immersion Depths
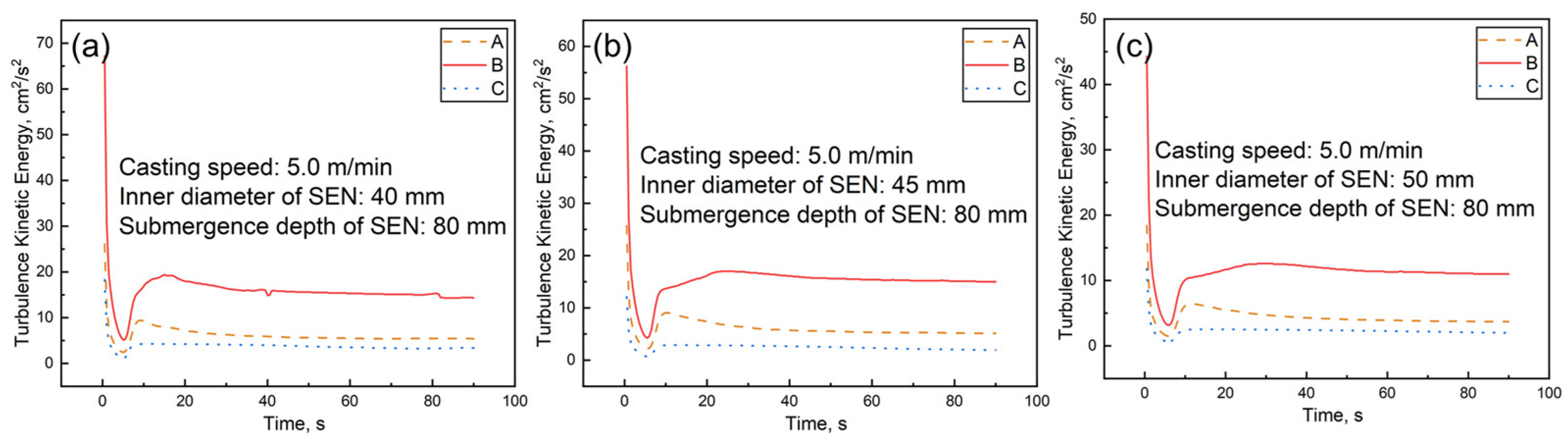
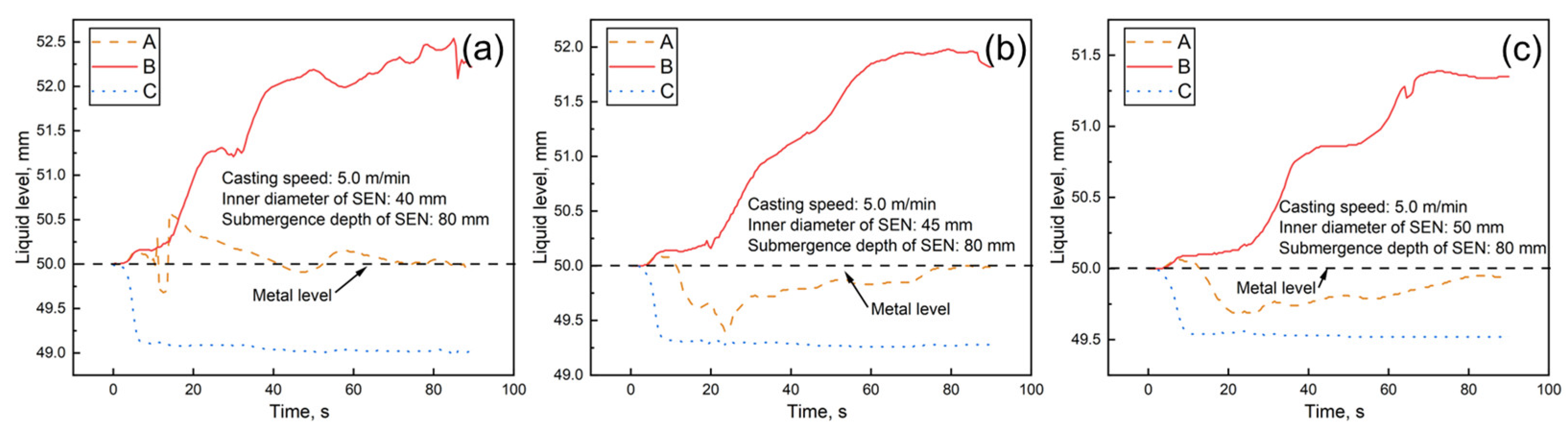
3.3. Effect of SEN Inner Diameters
4. Conclusions
- (1)
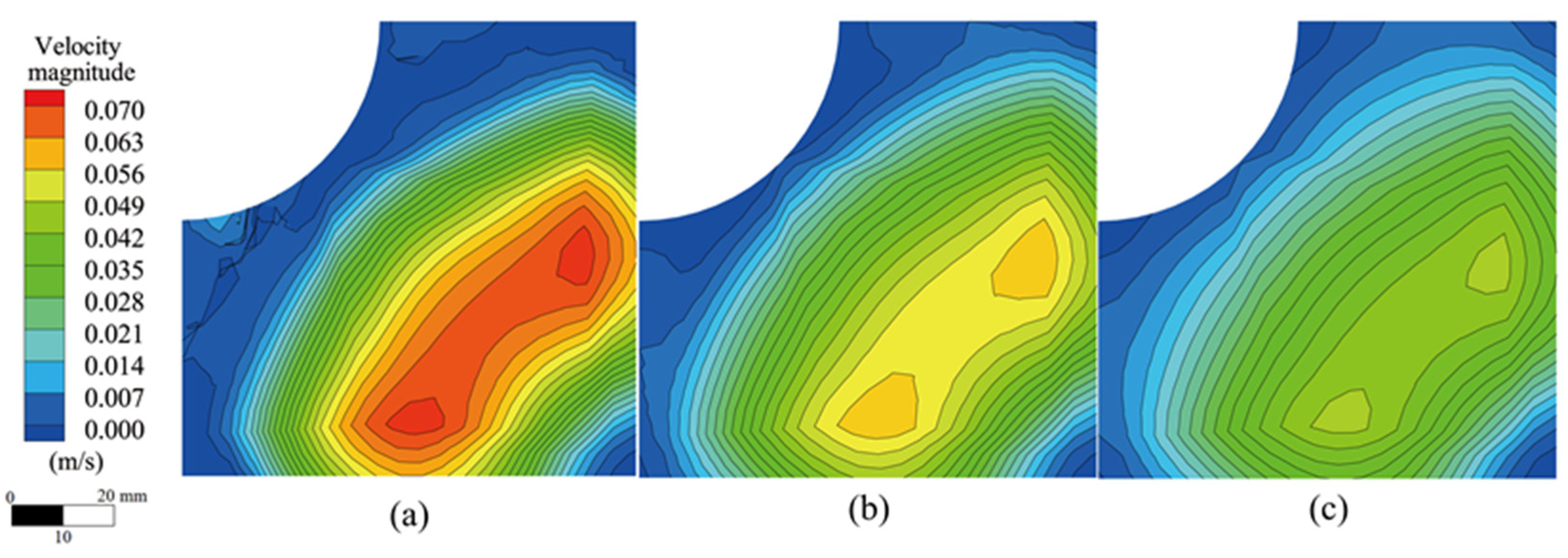
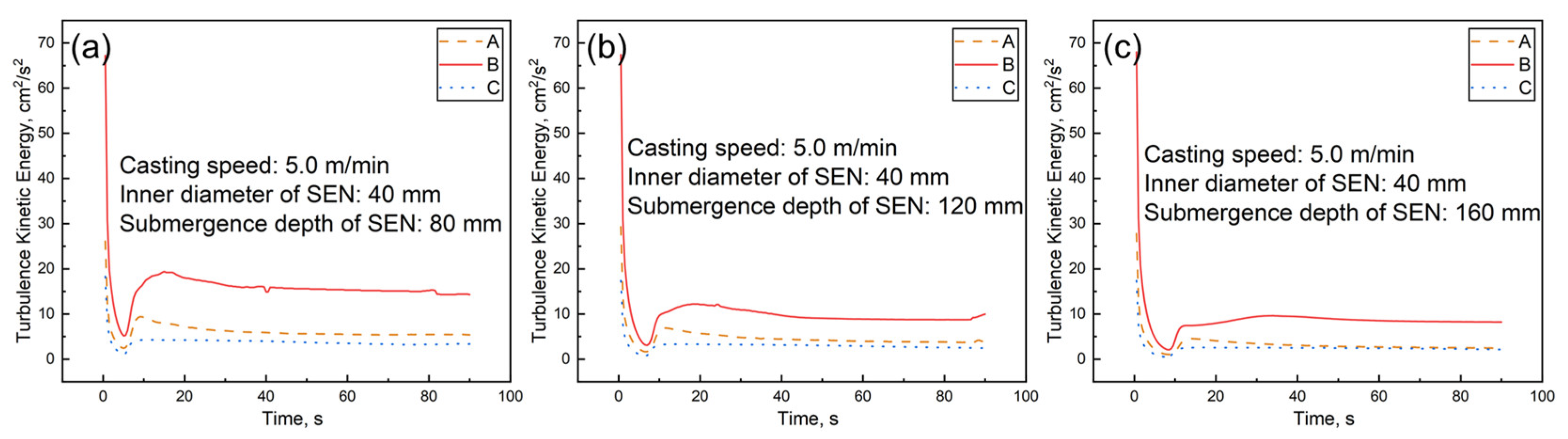
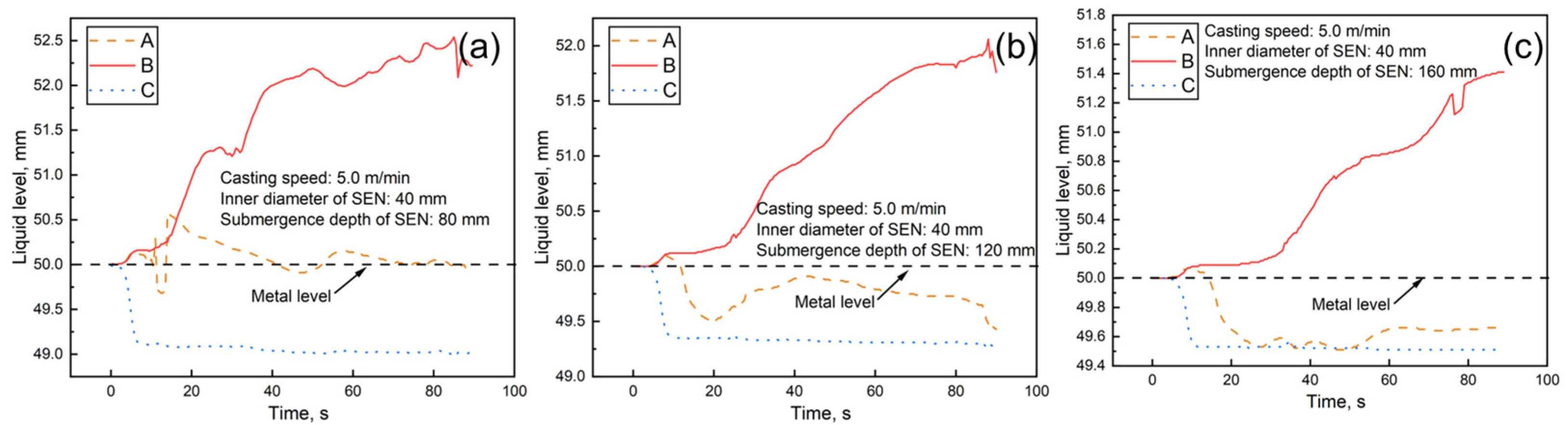
- The mold’s flow field patterns at different casting parameters were SRF, and there was no obvious change. The position where the liquid level flow velocity and fluctuation amplitude were the largest is concentrated in the middle of the liquid level. The turbulence of molten steel at the corner of the mold is difficult to develop, which can easily cause inclusion accumulation.
- (2)
- Changes to the casting speed had a minimal influence on the impact depth of the molten steel jet and the position of the raceway center. The velocity and fluctuation amplitude of the liquid level increased with the acceleration of the casting speed. When the casting speed was 7.0 m/min, the maximum velocity of the liquid level reached 0.1 m/s, and the maximum fluctuation value of the liquid level reached 5.64 mm.
- (3)
- When the SEN immersion depth deepened, the flow field in the mold moved downward as a whole, and changes to the impact depth and raceway center position were close to the shift of the immersion depth. When the SEN immersion depth was 160 mm, the liquid level flow velocity and liquid level fluctuation reached their minimum points of 0.066 m/s and 2.54 mm, respectively.
- (4)
- Although increasing the inner diameter of the SEN deepened the impact depth, it effectively weakened the initial velocity of the molten steel jet and reduced the scouring effect on the solidified shell. The main stream, after the inner diameter increased, also compressed the longitudinal space of the mold, reduced the overall area of the raceway, slowed down the liquid level disturbance, and facilitated high-speed casting. The velocity and fluctuation of molten steel at the liquid level also slowed down with the expansion of the inner diameter.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, E.G.; He, J.C. Finite element numerical simulation on thermo-mechanical behavior of steel billet in continuous casting mold. Sci. Technol. Adv. Mater. 2001, 2, 257–263. [Google Scholar] [CrossRef]
- Hong, K.H.; Kim, C.S.; Cha, P.R.; Yoon, J.K. A numerical analysis of fluid flow, heat transfer and solidification in the bending-type square billet continuous casting process. Met. Mater. Int. 2002, 8, 111–117. [Google Scholar] [CrossRef]
- Yang, J.; Chen, D.F.; Long, M.J.; Duan, H.M. Transient flow and mold flux behavior during ultra-high speed continuous casting of billet. J. Mater. Res. Technol. 2020, 9, 3984–3993. [Google Scholar] [CrossRef]
- Xu, P.; Chen, D.F.; Du, Y.Z.; Yu, H.S.; Long, M.J.; Liu, P.; Duan, H.M.; Yang, J. Hydraulic modeling on flow behavior in high-speed billet continuous casting mold considering hydrostatic pressure and solidified shell. Metals 2020, 10, 1226. [Google Scholar] [CrossRef]
- Abouelazayem, S.; Glavinić, I.; Wondrak, T.; Hlava, J. Flow control based on feature extraction in continuous casting process. Sensors 2020, 20, 6880. [Google Scholar] [CrossRef]
- Chow, C.; Samarasekera, I.; Walker, B.; Lockhart, G. High speed continuous casting of steel billets: Part 2: Mould heat transfer and mould design. Ironmak. Steelmak. 2002, 29, 61–69. [Google Scholar] [CrossRef]
- Ho, Y.H.; Chen, C.H.; Hwang, W.S. Analysis of molten steel flow in slab continuous caster mold. ISIJ Int. 1994, 34, 255–264. [Google Scholar] [CrossRef]
- Li, B.; Lu, H.B.; Zhong, Y.B.; Ren, Z.M.; Lei, Z.S. Numerical simulation for the influence of EMS position on fluid flow and inclusion removal in a slab continuous casting mold. ISIJ Int. 2020, 60, 1204–1212. [Google Scholar] [CrossRef]
- Guthrie, R.I.; Isac, M.M. Continuous casting practices for steel: Past, present and future. Metals 2022, 12, 862. [Google Scholar] [CrossRef]
- Chen, W.; Zhang, L.F. Effects of interphase forces on multiphase flow and bubble distribution in continuous casting strands. Metall. Mater. Trans. B 2021, 52, 528–547. [Google Scholar] [CrossRef]
- Wang, X.; Zheng, S.G.; Liu, Z.H.; Zhu, M.Y. Numerical simulation on multiple physical fields behaviors in billet continuous casting with different stirrer positions. Steel Res. Int. 2019, 91, 1900415. [Google Scholar] [CrossRef]
- Wu, H.D.; Chen, J.G.; Peng, Z. Simulation of the flow-heat transfer process in billet mold and analysis of the billet rhomboidity phenomenon. Appl. Therm. Eng. 2020, 173, 115235. [Google Scholar] [CrossRef]
- Hua, C.J.; Wang, M.; Bao, Y.P. Effect of nozzle clogging on the fluid flow pattern in a billet mold with particle image velocimetry technology. Metall. Mater. Trans. B 2020, 51, 2871–2881. [Google Scholar] [CrossRef]
- Ren, B.Z.; Chen, D.F.; Wang, H.D.; Long, M.J. Numerical analysis of coupled turbulent flow and macroscopic solidification in a round bloom continuous casting mold with electromagnetic stirring. Steel Res. Int. 2015, 86, 1104–1115. [Google Scholar] [CrossRef]
- Cho, S.M.; Thomas, B.G.; Kim, S.H. Effect of nozzle port angle on transient flow and surface slag behavior during continuous steel-slab casting. Metall. Mater. Trans. B 2019, 50, 52–76. [Google Scholar] [CrossRef]
- Lee, J.H.; Han, S.; Cho, H.J.; Park, I.S. Numerical and experimental study of the meniscus vortex core in a water model of continuous casting mold. Metall. Mater. Trans. B 2021, 52, 178–189. [Google Scholar] [CrossRef]
- Xu, P.; Zhou, Y.Z.; Chen, D.F.; Long, M.J.; Duan, H.M. Optimization of submerged entry nozzle parameters for ultra-high casting speed continuous casting mold of billet. J. Iron Steel Res. Int. 2022, 29, 44–52. [Google Scholar] [CrossRef]
- Michelic, S.K.; Bernhard, C. Significance of nonmetallic inclusions for the clogging phenomenon in continuous casting of steel—A review. Steel Res. Int. 2022, 93, 2200086. [Google Scholar] [CrossRef]
- Kholmatov, S.; Takagi, S.; Jonsson, L.; Jönsson, P.; Yokoya, S. Effect of nozzle angle on flow field and temperature distribution in a billet mould when using swirl flow. Steel Res. Int. 2008, 79, 31–39. [Google Scholar] [CrossRef]
- Gan, M.J.; Pan, W.J.; Wang, Q.Q.; Zhang, X.B.; He, S.P. Effect of exit shape of submerged entry nozzle on flow field and slag entrainment in continuous casting mold. Metall. Mater. Trans. B 2020, 51, 2862–2870. [Google Scholar] [CrossRef]
- Lee, W.H.; Yi, K.W. Relationship between fluid flow stability and submerged entry nozzle port angle in a conventional slab continuous-casting mold. Met. Mater. Int. 2021, 27, 4168–4181. [Google Scholar] [CrossRef]
- Vakhrushev, A.; Kharicha, A.; Liu, Z.; Wu, M.; Ludwig, A.; Nitzl, G.; Tang, Y.; Hackl, G.; Watzinger, J. Electric current distribution during electromagnetic braking in continuous casting. Metall. Mater. Trans. B 2020, 51, 2811–2828. [Google Scholar] [CrossRef]
- Fei, P.; Min, Y.; Liu, C.J.; Jiang, M.F. Effect of continuous casting speed on mold surface flow and the related near-surface distribution of non-metallic inclusions. Int. J. Miner. Metall. Mater. 2019, 26, 186–193. [Google Scholar] [CrossRef]
- Yu, Z.; Zhang, Z.Q.; Ren, Z.M. Effect of flow control mold on flow field during high-speed continuous casting. Adv. Manuf. 2017, 5, 271–278. [Google Scholar] [CrossRef]
- Li, X.T.; Zhang, Z.H.; Lv, M.; Fang, M.; Liu, K.L. Numerical simulation of the fluid flow, heat transfer, and solidification in ultrahigh speed continuous casting billet mold. Steel Res. Int. 2022, 93, 2100673. [Google Scholar] [CrossRef]
- Li, X.T.; Zhang, Z.H.; Lv, M.; Fang, M.; Ma, S.B.; Li, D.L.; Xi, X.F. Effect of mold electromagnetic stirring on metallurgical behavior in ultrahigh speed continuous casting billet mold. Steel Res. Int. 2023. [Google Scholar] [CrossRef]
- Li, Z.; Wang, E.G.; Zhang, L.T.; Xu, Y.; Deng, A.Y. Influence of vertical electromagnetic brake on the steel/slag interface behavior in a slab mold. Metall. Mater. Trans. B 2017, 48, 2389–2402. [Google Scholar] [CrossRef]
- Jiang, P.; Yang, J.; Zhang, T.; Xu, G.J.; Liu, H.J.; Zhou, J.J.; Qin, W. Optimization of flow field in slab continuous casting mold with medium width using high temperature measurement and numerical simulation for automobile exposed panel production. Metals 2019, 10, 9. [Google Scholar] [CrossRef]
- Wang, Y.; Fang, Q.; Zhang, H.; Zhou, J.A.; Liu, C.S.; Ni, H.W. Effect of argon blowing rate on multiphase flow and initial solidification in a slab mold. Metall. Mater. Trans. B 2020, 51, 1088–1100. [Google Scholar] [CrossRef]
- Sun, X.H.; Li, B.; Lu, H.B.; Zhong, Y.B.; Ren, Z.M.; Lei, Z.S. Steel/slag interface behavior under multifunction electromagnetic driving in a continuous casting slab mold. Metals 2019, 9, 983. [Google Scholar] [CrossRef]
- Cramb, A.W.; Jimbo, I. Calculation of the interfacial properties of liquid steel–slag systems. Steel Res. 1989, 60, 157–165. [Google Scholar] [CrossRef]




| Parameters | Values |
|---|---|
| Mold section (mm × mm) | 160 × 160 |
| Effective length of mold (mm) | 900 |
| Computational length of mold (mm) | 1500 |
| Steel density (kg/m3) | 7200 |
| Steel viscosity (Pa·s) | 0.0065 |
| Slag density (kg/m3) | 2600 |
| Slag viscosity (Pa·s) | 0.1 |
| Thickness of slag layer (mm) | 50 |
| Interfacial tension between molten steel and slag (N/m) | 1.35 |
| Casting Speeds (m/min) | Impact Depth (mm) | Distance from Raceway Center to Liquid Level (mm) |
|---|---|---|
| 5.0 | 315 | 351 |
| 6.0 | 316 | 352 |
| 7.0 | 317 | 353 |
| Immersion Depth (mm) | Impact Depth (mm) | Distance from Raceway Center to Liquid Level (mm) |
|---|---|---|
| 80 | 315 | 351 |
| 120 | 362 | 392 |
| 160 | 412 | 434 |
| Inner Diameters (mm) | Impact Depth (mm) | Distance from Raceway Center to Liquid Level (mm) |
|---|---|---|
| 40 | 315 | 351 |
| 45 | 343 | 346 |
| 50 | 362 | 337 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, D.; Zhang, Z.; Li, X.; Lv, M.; Mi, X.; Xi, X. Numerical Simulation of the Flow Field in an Ultrahigh-Speed Continuous Casting Billet Mold. Metals 2023, 13, 964. https://doi.org/10.3390/met13050964
Qiu D, Zhang Z, Li X, Lv M, Mi X, Xi X. Numerical Simulation of the Flow Field in an Ultrahigh-Speed Continuous Casting Billet Mold. Metals. 2023; 13(5):964. https://doi.org/10.3390/met13050964
Chicago/Turabian StyleQiu, Dejin, Zhaohui Zhang, Xintao Li, Ming Lv, Xiaoyu Mi, and Xiaofeng Xi. 2023. "Numerical Simulation of the Flow Field in an Ultrahigh-Speed Continuous Casting Billet Mold" Metals 13, no. 5: 964. https://doi.org/10.3390/met13050964
APA StyleQiu, D., Zhang, Z., Li, X., Lv, M., Mi, X., & Xi, X. (2023). Numerical Simulation of the Flow Field in an Ultrahigh-Speed Continuous Casting Billet Mold. Metals, 13(5), 964. https://doi.org/10.3390/met13050964







