Multi-Physical Field Coupling Simulation and Experiments with Pulse Electrochemical Machining of Large Size TiAl Intermetallic Blade
Abstract
1. Introduction
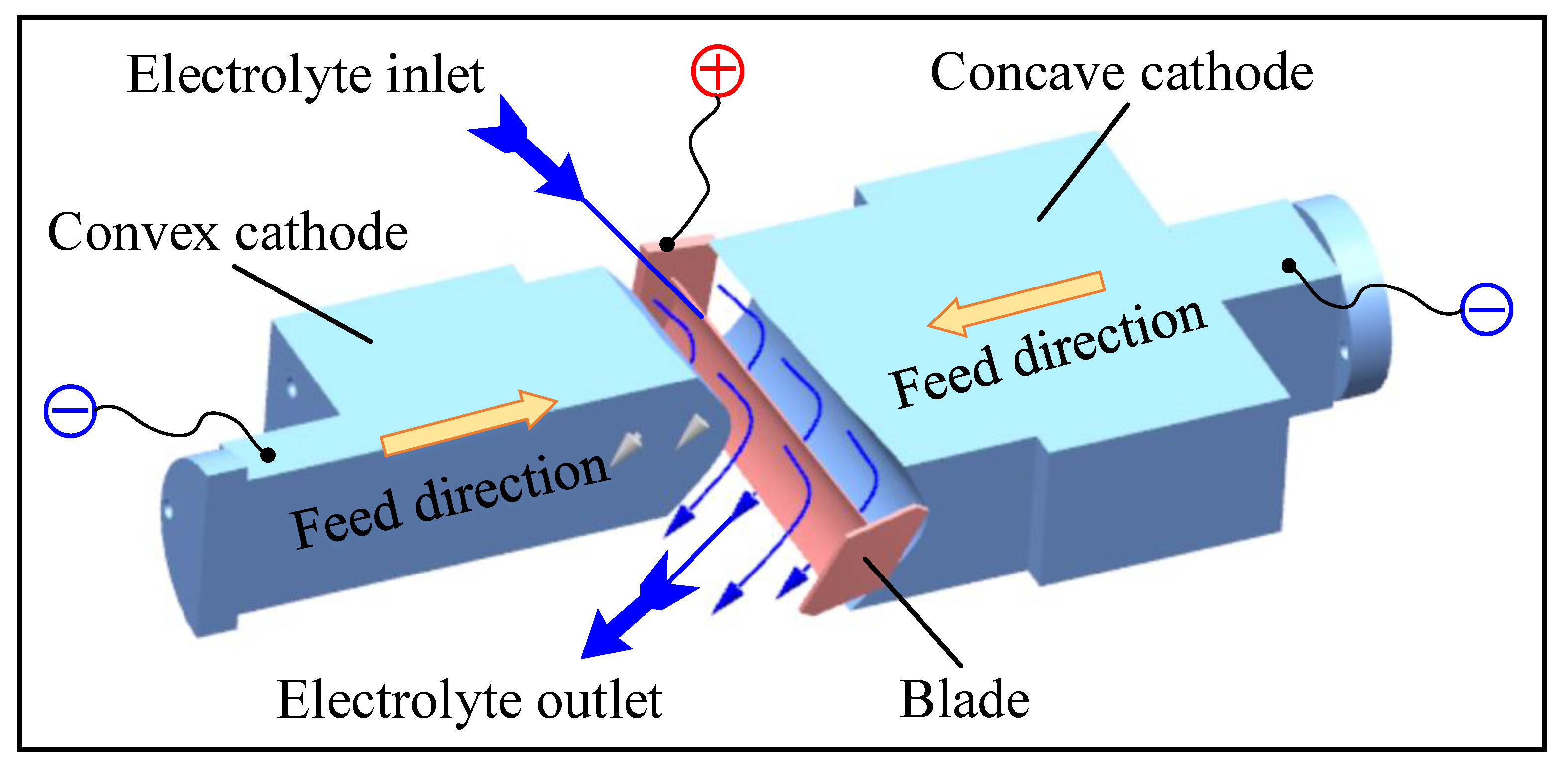
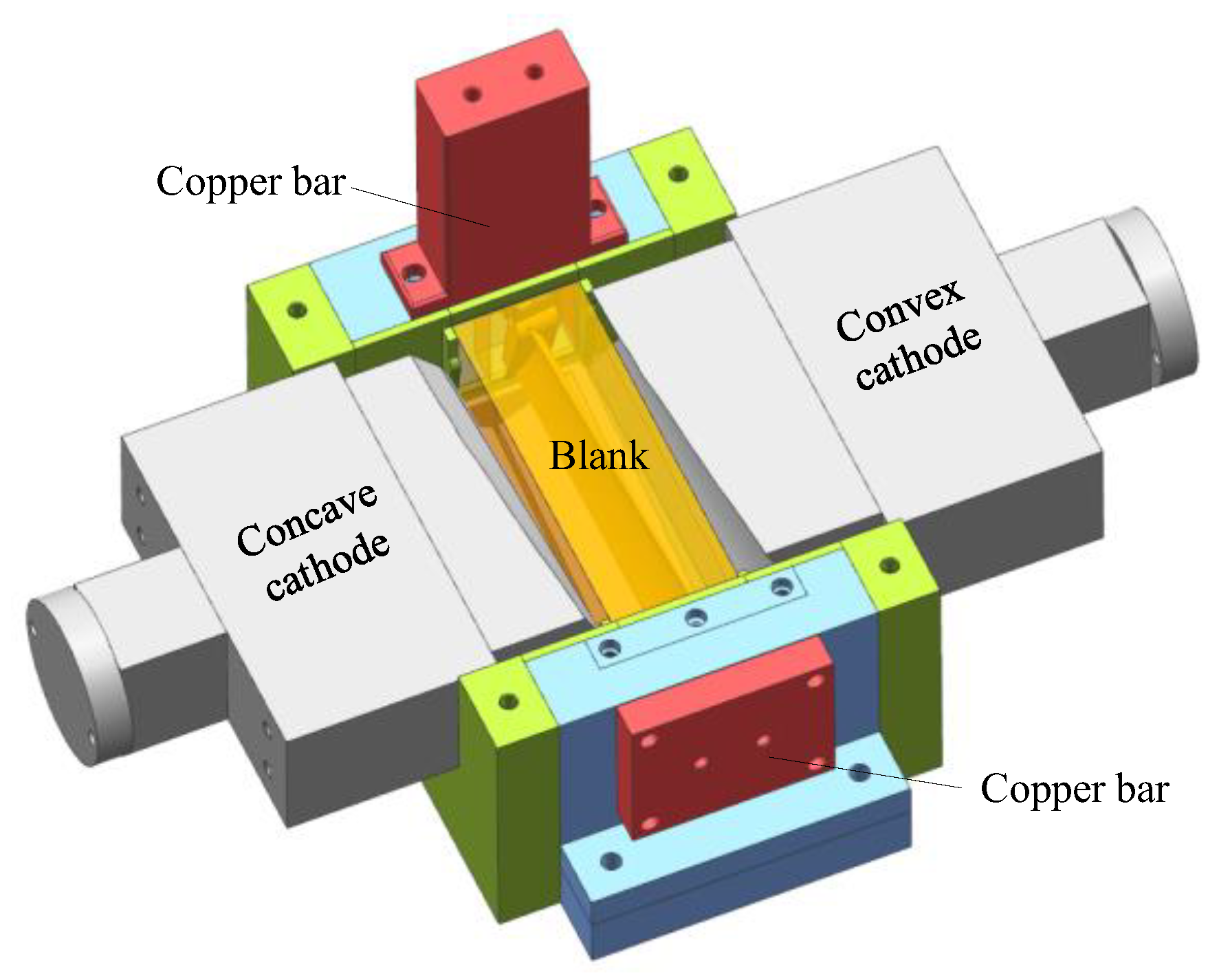
2. Principles of ECM for Large Blades
3. Model of Multi-Physical Field Coupling Simulation
3.1. Electric Field Model
3.2. Gas-Liquid Two-Phase Flow Field Model
3.3. Heat Transfer Model
3.4. Anodic Dissolution Model
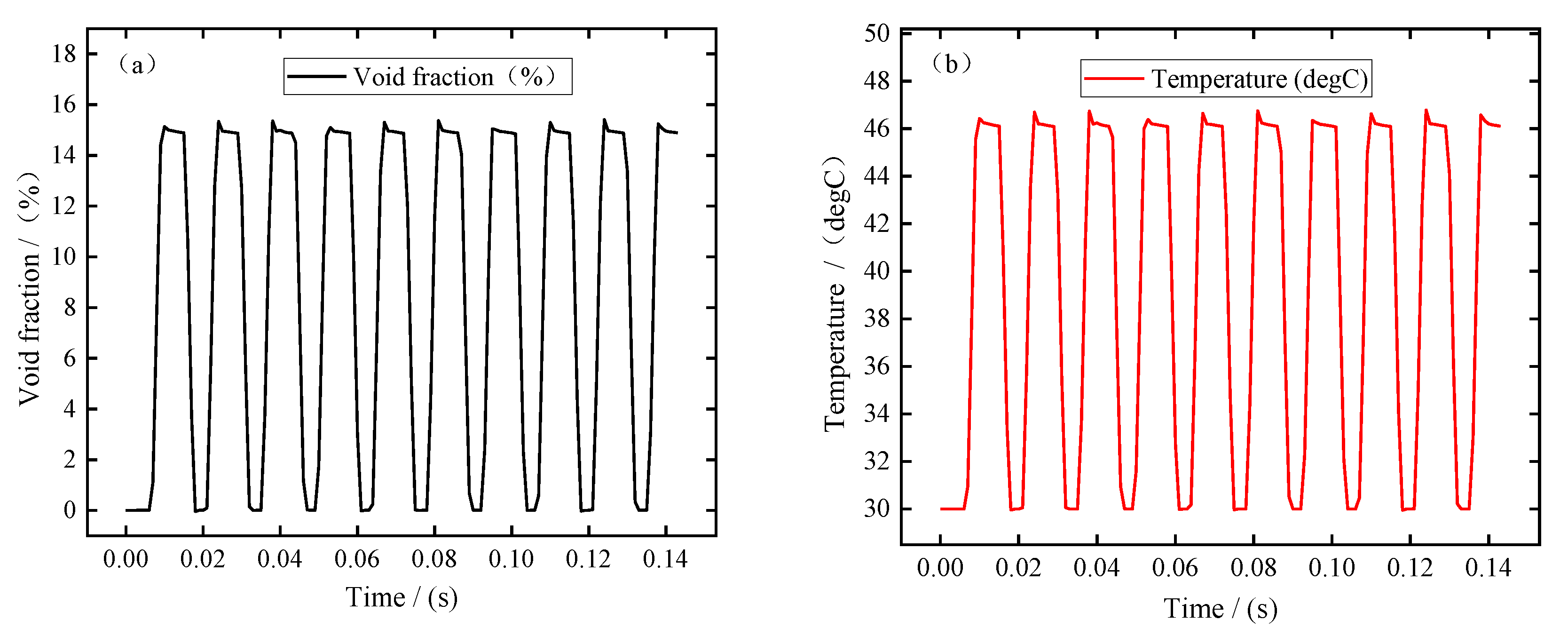
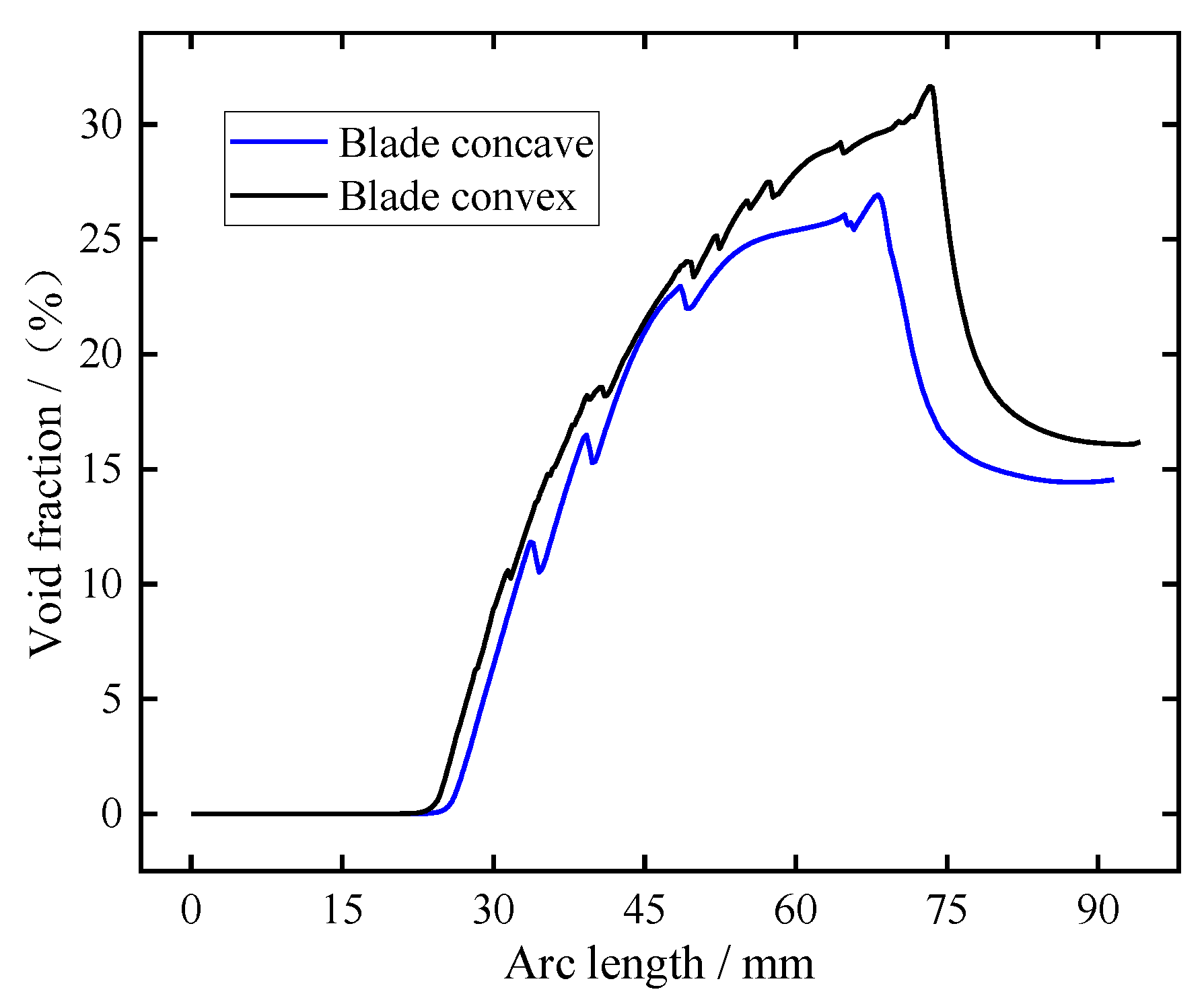
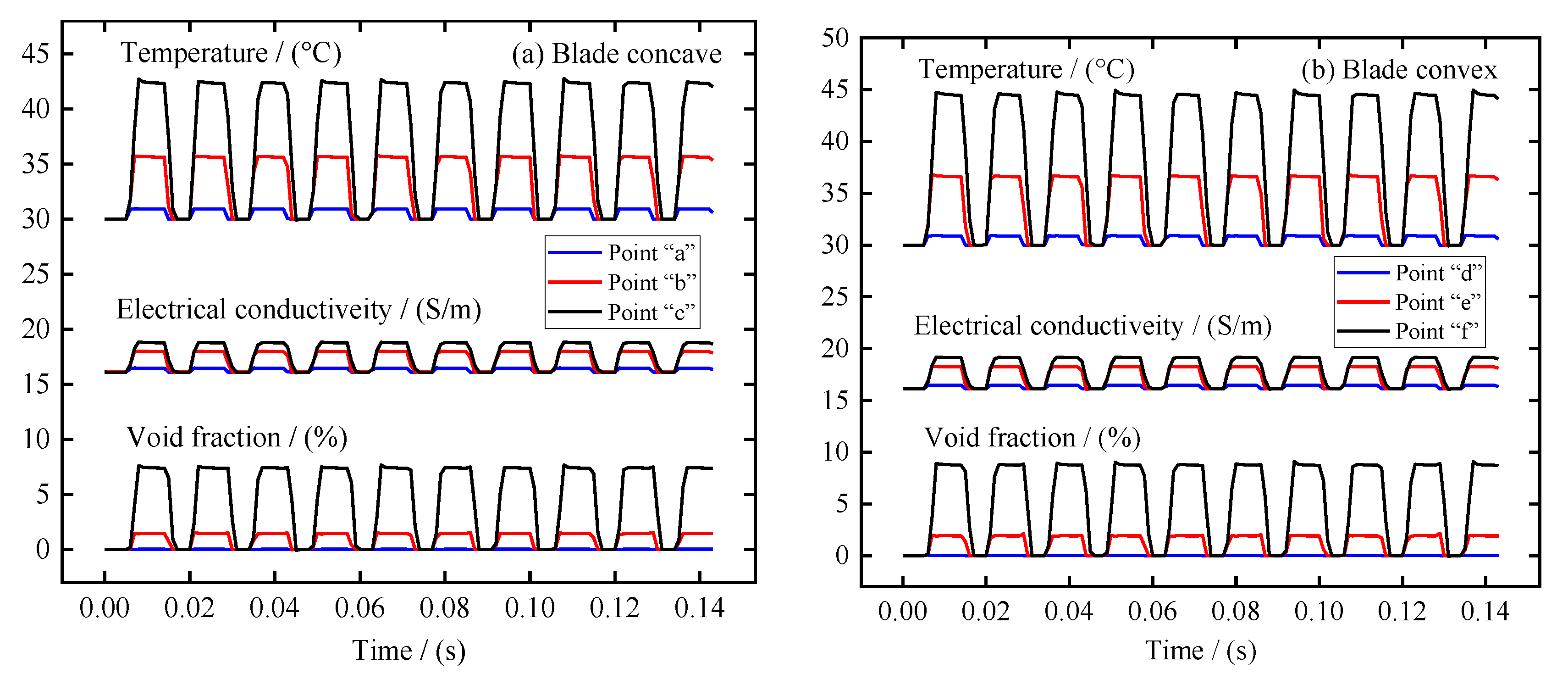
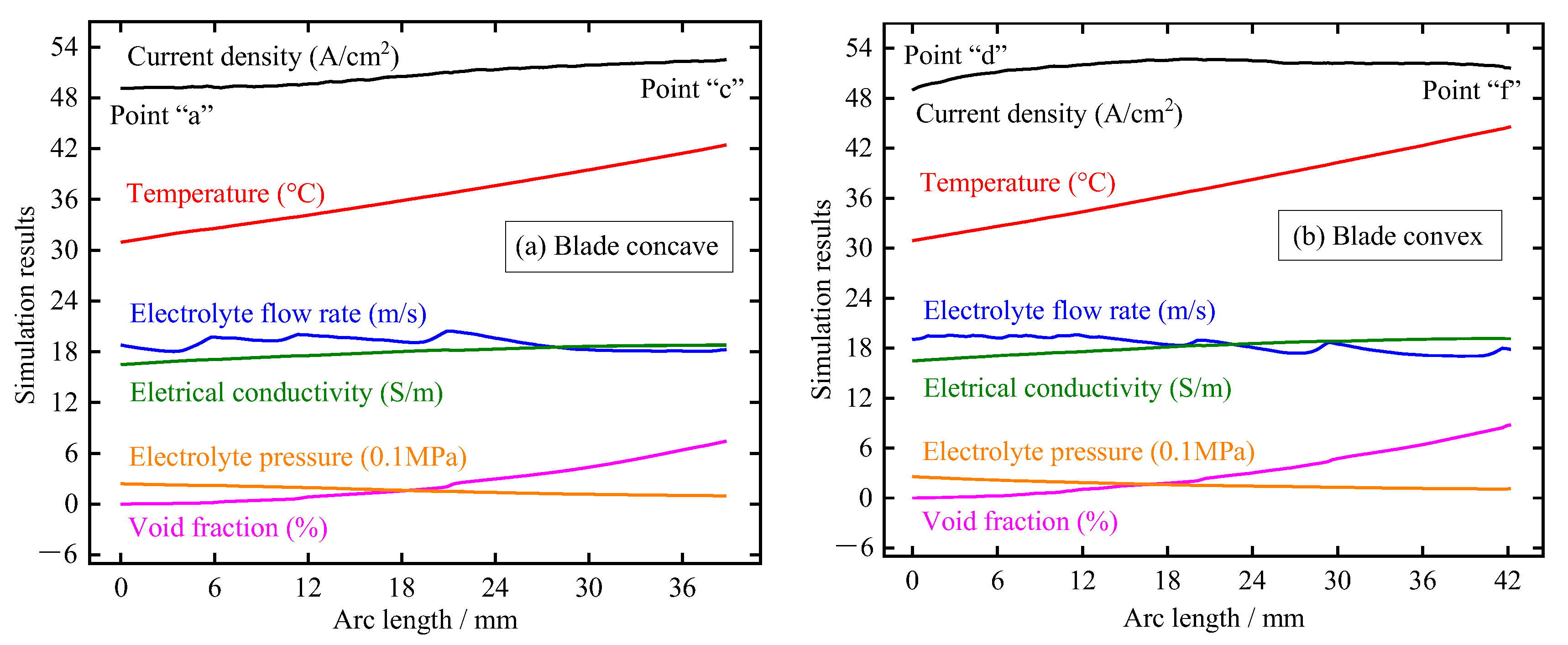
4. Results Analysis of Multi-Physical Field Coupling Simulation
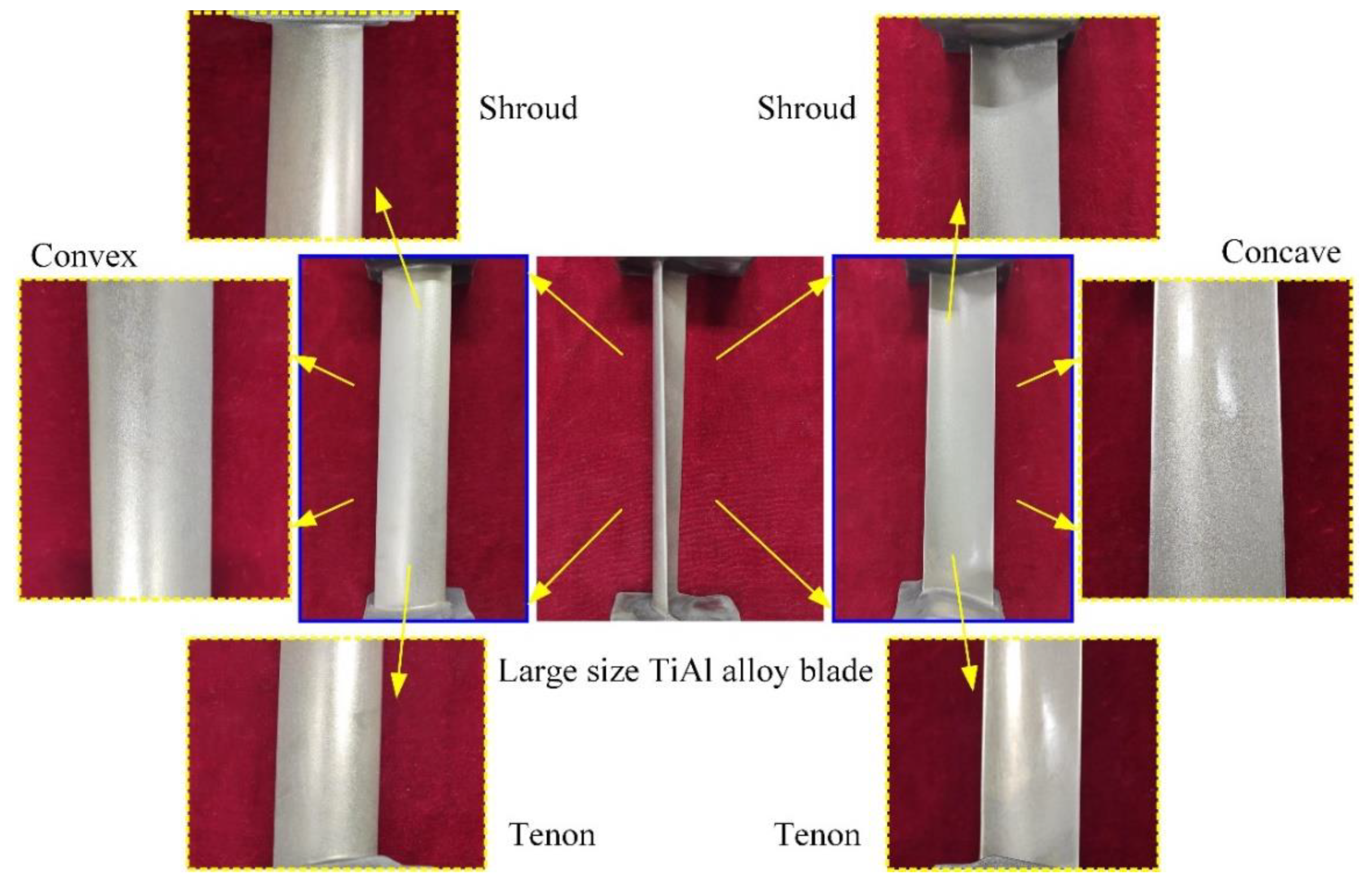
5. Experiments and Discussion
5.1. Pulse ECM of Large Size TiAl Alloy Blade
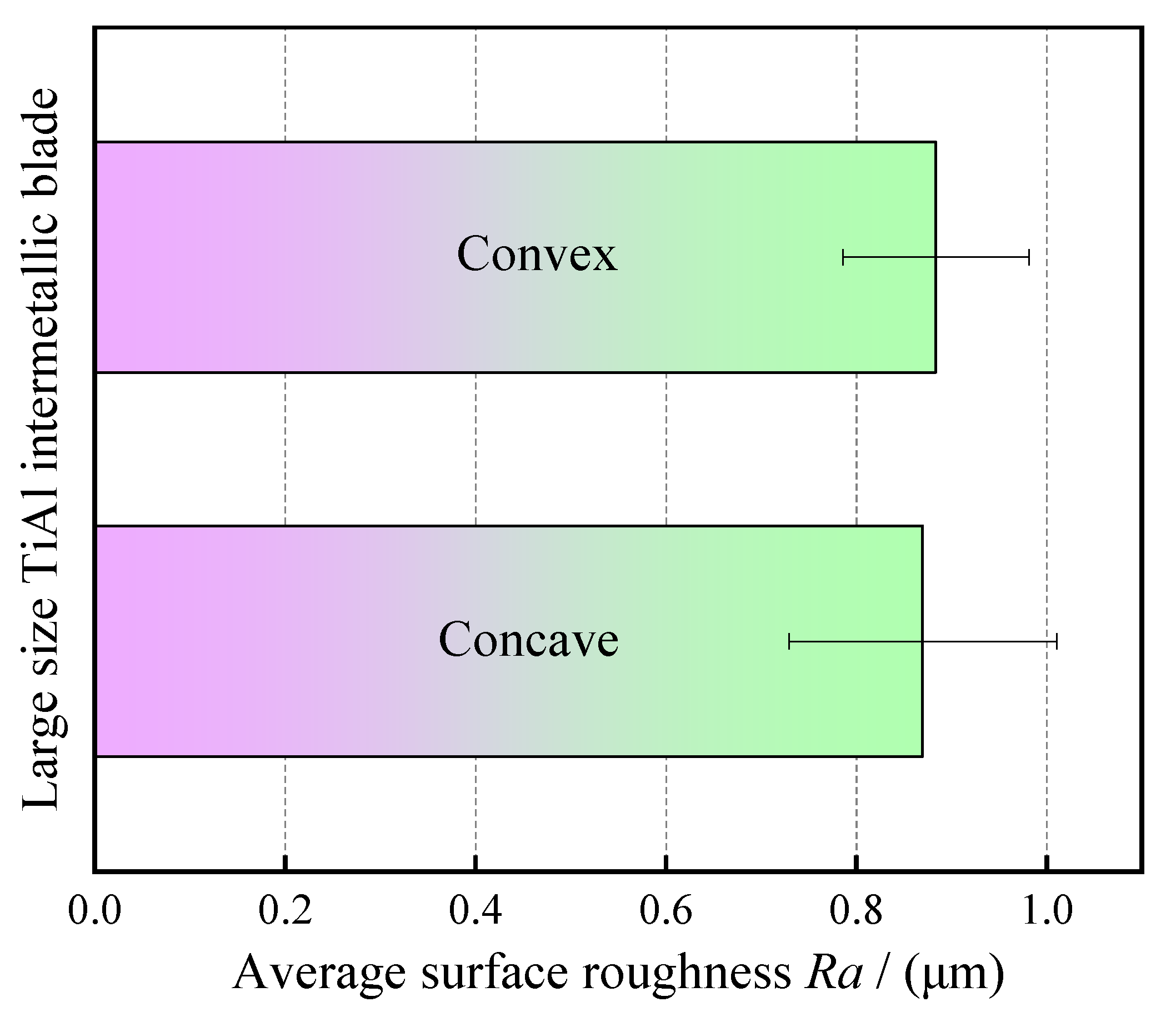
5.2. Analysis of Experimental Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kothari, K.; Radhakrishnan, R.; Wereley, N.M. Advances in gamma titanium aluminides and their manufacturing techniques. Prog. Aerosp. Sci. 2012, 55, 1–16. [Google Scholar] [CrossRef]
- Bewlay, B.P.; Weimer, M.; Kelly, T.; Suzuki, A.; Subramanian, P. The science, technology, and implementation of TiAl alloys in commercial aircraft engines. MRS Proc. 2013, 1516, 49–58. [Google Scholar] [CrossRef]
- Rajurkar, K.; Zhu, D.; McGeough, J.; Kozak, J.; De Silva, A. New developments in electro-chemical machining. CIRP Ann.-Manuf. Technol. 1999, 48, 567–579. [Google Scholar] [CrossRef]
- Xu, Z.; Wang, Y. Electrochemical machining of complex components of aero-engines: Developments, trends, and technological advances. Chin. J. Aeronaut. 2021, 34, 28–53. [Google Scholar] [CrossRef]
- Liu, J.; Hui, L.; Jia, D.; Liu, Y.; Zhu, D. An electrochemical machining method for aero-engine blades based on four-directional synchronous feeding of cathode tools. Chin. J. Aeronaut. 2022. [Google Scholar] [CrossRef]
- Schaarschmidt, I.; Luther, F.; Steinert, P.; Richter, M.; Schubert, A. Simulation of the magnetic field assisted electrochemical machining. Procedia CIRP 2023, 117, 249–256. [Google Scholar] [CrossRef]
- Wang, J.; Xu, Z.; Wang, J.; Xu, Z.; Zhu, D. Electrochemical machining of blisk channels with rotations of the cathode and the workpiece. Int. J. Mech. Sci. 2021, 208, 106655. [Google Scholar] [CrossRef]
- Clifton, D.; Mount, A.; Jardine, D.; Roth, R. Electrochemical machining of gamma titanium aluminide intermetallics. J. Mater. Process. Technol. 2001, 108, 338–348. [Google Scholar] [CrossRef]
- Liu, J.; Zhu, D.; Zhao, L.; Xu, Z. Experimental investigation on electrochemical machining of γ-TiAl intermetallic. Procedia CIRP 2015, 35, 20–24. [Google Scholar] [CrossRef]
- Baehre, D.; Ernst, A.; Weißhaar, K.; Natter, H.; Stolpe, M.; Busch, R. Electrochemical dissolution behavior of titanium and titanium-based alloys in different electrolytes. Procedia CIRP 2016, 42, 137–142. [Google Scholar] [CrossRef]
- Klocke, F.; Herrig, T.; Zeis, M.; Klink, A. Comparison of the electrochemical machinability of electron beam melted and casted gamma titanium aluminide TNB-V5. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2018, 232, 586–592. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, Z.; Zhang, A. Electrochemical dissolution behavior of Ti-45Al-2Mn-2Nb+0.8 vol% TiB2 XD alloy in NaCl and NaNO3 solutions. Corros. Sci. 2019, 157, 357–369. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, Z.; Zhang, A. Anodic characteristics and electrochemical machining of two typical γ-TiAl alloys and its quantitative dissolution model in NaNO3 solution. Electrochimica Acta 2020, 331, 135429. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, Z.; Zhang, A.; Xu, G.; Zhang, C. Surface morphology and electrochemical behaviour of Ti-48Al-2Cr-2Nb alloy in low-concentration salt solution. Sci. China Technol. Sci. 2021, 64, 283–296. [Google Scholar] [CrossRef]
- Klocke, F.; Zeis, M.; Klink, A. Interdisciplinary modelling of the electrochemical machining process for engine blades. CIRP Ann.-Manuf. Technol. 2015, 64, 217–220. [Google Scholar] [CrossRef]
- Klocke, F.; Zeis, M.; Harst, S.; Klink, A.; Veselovac, D.; Baumgärtner, M. Modeling and simulation of the electrochemical machining (ECM) material removal process for the manufacture of aero engine components. Procedia CIRP 2013, 8, 265–270. [Google Scholar] [CrossRef]
- Wang, H.; Zhu, D.; Liu, J. Improving the accuracy of the blade leading/trailing edges by electrochemical machining with tangential feeding. CIRP Ann.-Manuf. Technol. 2019, 68, 165–168. [Google Scholar] [CrossRef]
- Schaarschmidt, I.; Hackert-Oschätzchen, M.; Meichsner, G.; Zinecker, M.; Schubert, A. Implementation of the machine tool-specific current and voltage control characteristics in multiphysics simulation of electrochemical precision machining. Procedia CIRP 2019, 82, 237–242. [Google Scholar] [CrossRef]
- Chen, Y.; Zhou, X.; Chen, P.; Wang, Z. Electrochemical machining gap prediction with multi-physics coupling model based on two-phase turbulence flow. Chin. J. Aeronaut. 2020, 33, 1057–1063. [Google Scholar] [CrossRef]
- Wang, F.; Yao, J.; Kang, M. Electrochemical machining of a rhombus hole with synchronization of pulse current and low-frequency oscillations. J. Manuf. Process. 2020, 57, 91–104. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, Z.; Liu, J.; Zhang, A.; Xu, Z.; Meng, D.; Zhao, J. Study on flow field of electrochemical machining for large size blade. Int. J. Mech. Sci. 2021, 190, 106018. [Google Scholar] [CrossRef]
- Fujisawa, T.; Inaba, K.; Yamamoto, M.; Kato, D. Multiphysics simulation of electrochemical machining process for three-dimensional compressor blade. J. Fluids Eng. 2008, 130, 0816021–0816028. [Google Scholar] [CrossRef]
- Wang, M.; Liu, W.; Peng, W. Multiphysics research in electrochemical machining of internal spiral hole. Int. J. Adv. Manuf. Technol. 2014, 74, 749–756. [Google Scholar] [CrossRef]
- Fang, X.; Qu, N.; Zhang, Y.; Xu, Z.; Zhu, D. Effects of pulsating electrolyte flow in electrochemical machining. J. Mater. Process. Technol. 2014, 214, 36–43. [Google Scholar] [CrossRef]
- Zong, Y.; Liu, J.; Zhu, D. Improving blade accuracy via local electrochemical machining with partial insulated cathodes. Precis. Eng. 2022, 76, 284–293. [Google Scholar] [CrossRef]
- Wang, M.; Zhang, R.; Shang, Y.; Zheng, J.; Wang, X.; Xu, X. Micro-milling microstructures in air-shielding ultrasonic assisted electrochemical machining. J. Manuf. Process. 2023, 97, 171–184. [Google Scholar] [CrossRef]
- Jiao, E.; Zhu, D.; Zhang, C.; Wang, P.; Zuo, H. Research on the characteristics of multiphysics coupling fields in the electrochemical trepanning of an inward facing blisk. J. Manuf. Process. 2023, 93, 60–74. [Google Scholar] [CrossRef]
- He, C.Y. Multiphysics simulation of electrochemical machining process. Master’s Thesis, Guangdong University of Technology, Guangzhou, China, 2015. [Google Scholar]
- Jiang, C. Product distribution and parameter optimization in pulsating electrochemical machining. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2019. [Google Scholar]
- Wang, Y.; Xu, Z.; Meng, D.; Liu, L.; Fang, Z. Study on surface roughness of large size TiAl intermetallic blade in electrochemical machining. J. Manuf. Process. 2022, 76, 1–10. [Google Scholar] [CrossRef]







| Parameters | Values |
|---|---|
| Workpiece material | TiAl 45XD |
| Cathode material | 1Cr18Ni9Ti |
| Cathode feeding rate vc (mm/min) | 0.65 |
| Electrolyte concentration | 20% NaNO3 |
| Electrolyte initial conductivity κ (S/m) | 16.1 |
| Voltage U (V) | 26 |
| Pulse duty | 60% |
| Pulse frequency f (Hz) | 70 |
| Inlet pressure P1 (MPa) | 0.4 |
| Outlet pressure P2 (MPa) | 0.1 |
| Electrolyte temperature T (°C) | 30 |
| Parameters | Values | |
|---|---|---|
| First Step | Second Step | |
| Cathode feeding rate vc (mm/min) | 0.65 | 1.6 |
| Electrolyte concentration | 20% NaNO3 | |
| Voltage U (V) | 26 | 30 |
| Pulse duty | 60% | |
| Pulse frequency f (Hz) | 70 | 50 |
| Inlet pressure Pin (MPa) | 0.6, 0.4, 0.4 | 1.2, 1.0, 1.0 |
| Outlet pressure Pout (MPa) | 0.1 | |
| Electrolyte temperature T (°C) | 30 | |
| Cathode feeding depth (mm) | 11.4 | 0.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Xu, Z.; Meng, D.; Liu, L.; Fang, Z. Multi-Physical Field Coupling Simulation and Experiments with Pulse Electrochemical Machining of Large Size TiAl Intermetallic Blade. Metals 2023, 13, 985. https://doi.org/10.3390/met13050985
Wang Y, Xu Z, Meng D, Liu L, Fang Z. Multi-Physical Field Coupling Simulation and Experiments with Pulse Electrochemical Machining of Large Size TiAl Intermetallic Blade. Metals. 2023; 13(5):985. https://doi.org/10.3390/met13050985
Chicago/Turabian StyleWang, Yudi, Zhengyang Xu, Deman Meng, Lin Liu, and Zhongdong Fang. 2023. "Multi-Physical Field Coupling Simulation and Experiments with Pulse Electrochemical Machining of Large Size TiAl Intermetallic Blade" Metals 13, no. 5: 985. https://doi.org/10.3390/met13050985
APA StyleWang, Y., Xu, Z., Meng, D., Liu, L., & Fang, Z. (2023). Multi-Physical Field Coupling Simulation and Experiments with Pulse Electrochemical Machining of Large Size TiAl Intermetallic Blade. Metals, 13(5), 985. https://doi.org/10.3390/met13050985






