Numerical Simulation of Turbulence Intensity of an Acid Solution during the Strip Steel Pickling Process
Abstract
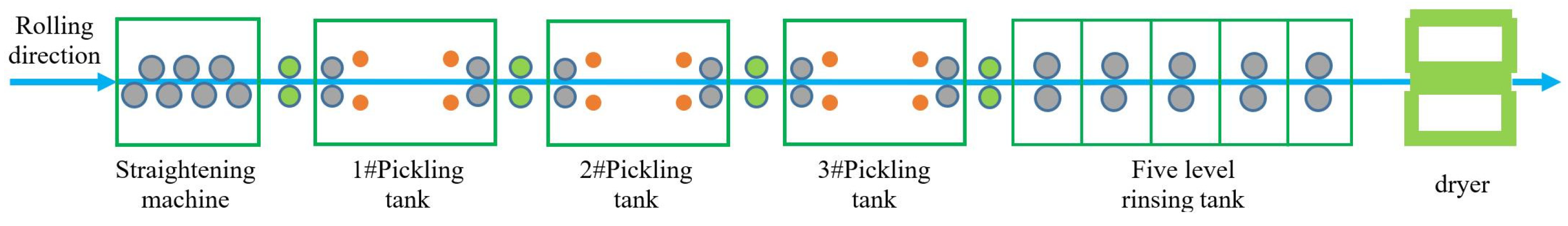
1. Introduction
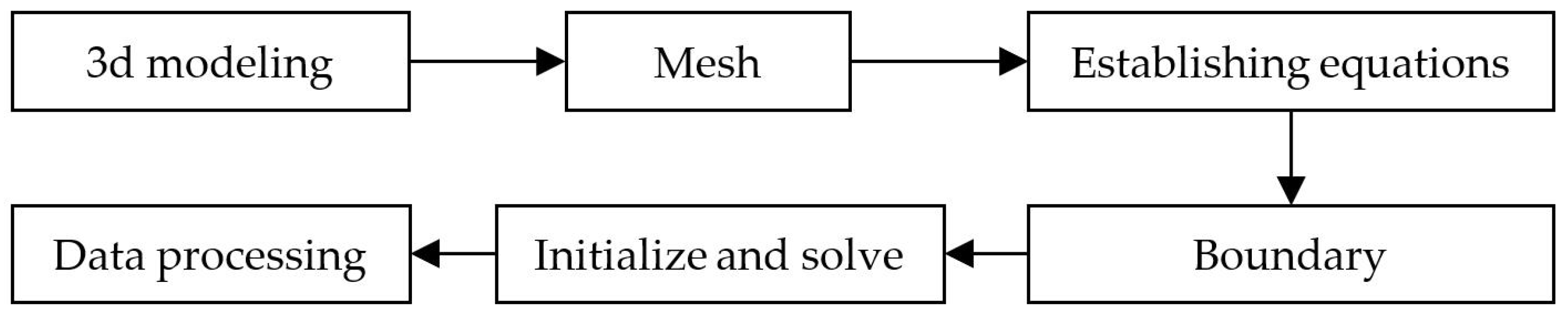
2. Materials and Methods
2.1. Model Parameter Settings
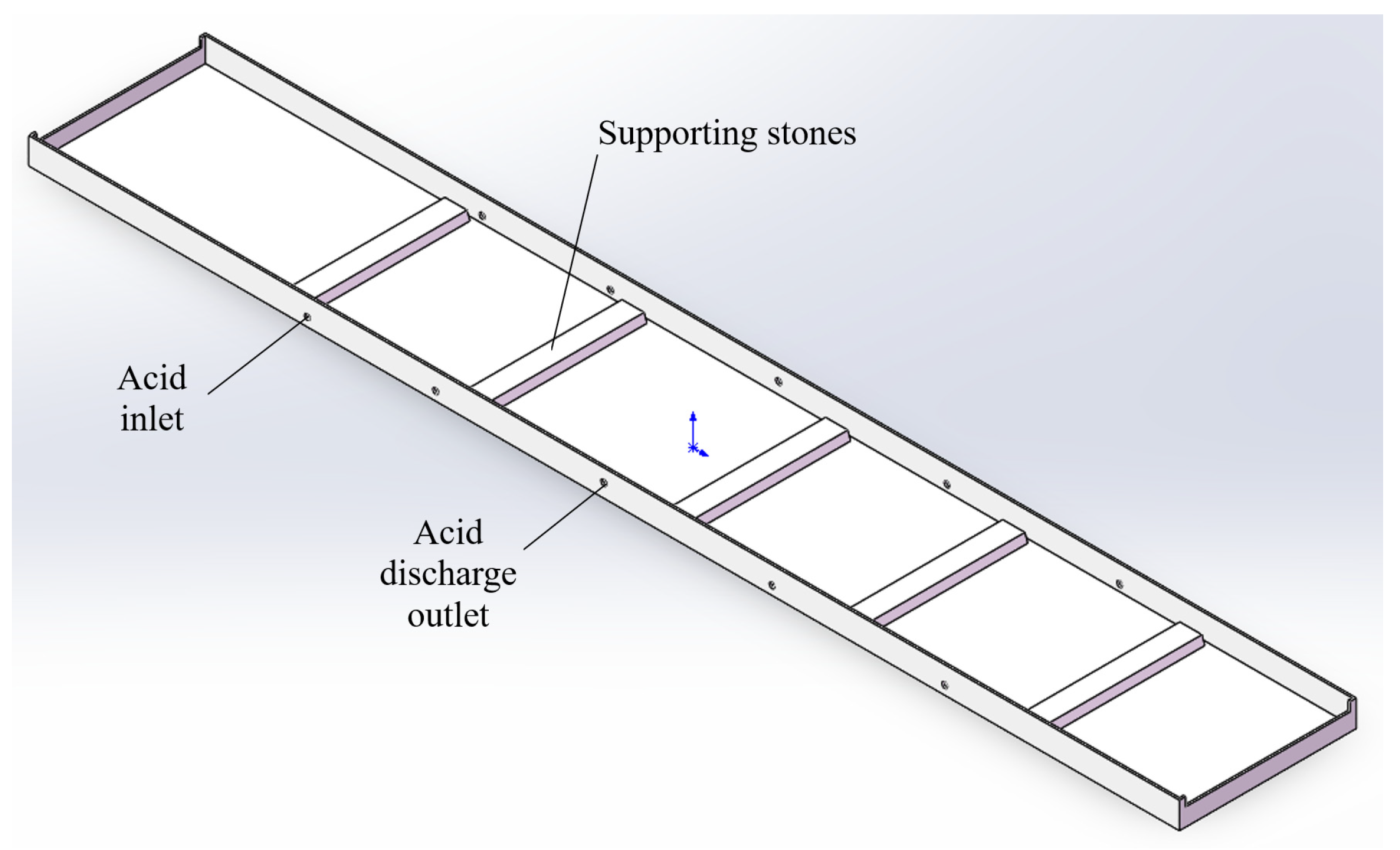
2.2. Establishment of Pickling Tank Model
2.3. Finite Element Meshing
3. Results
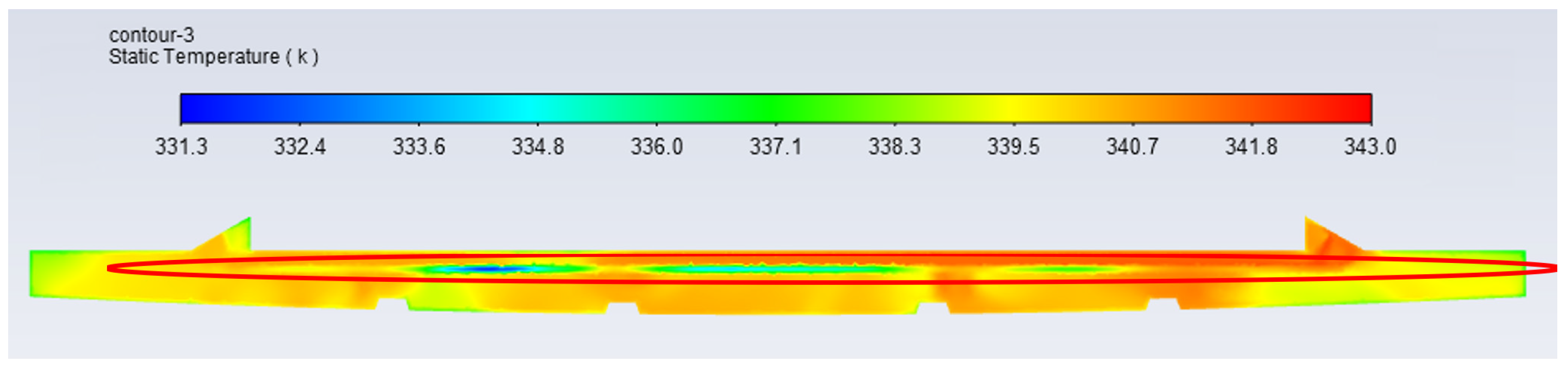
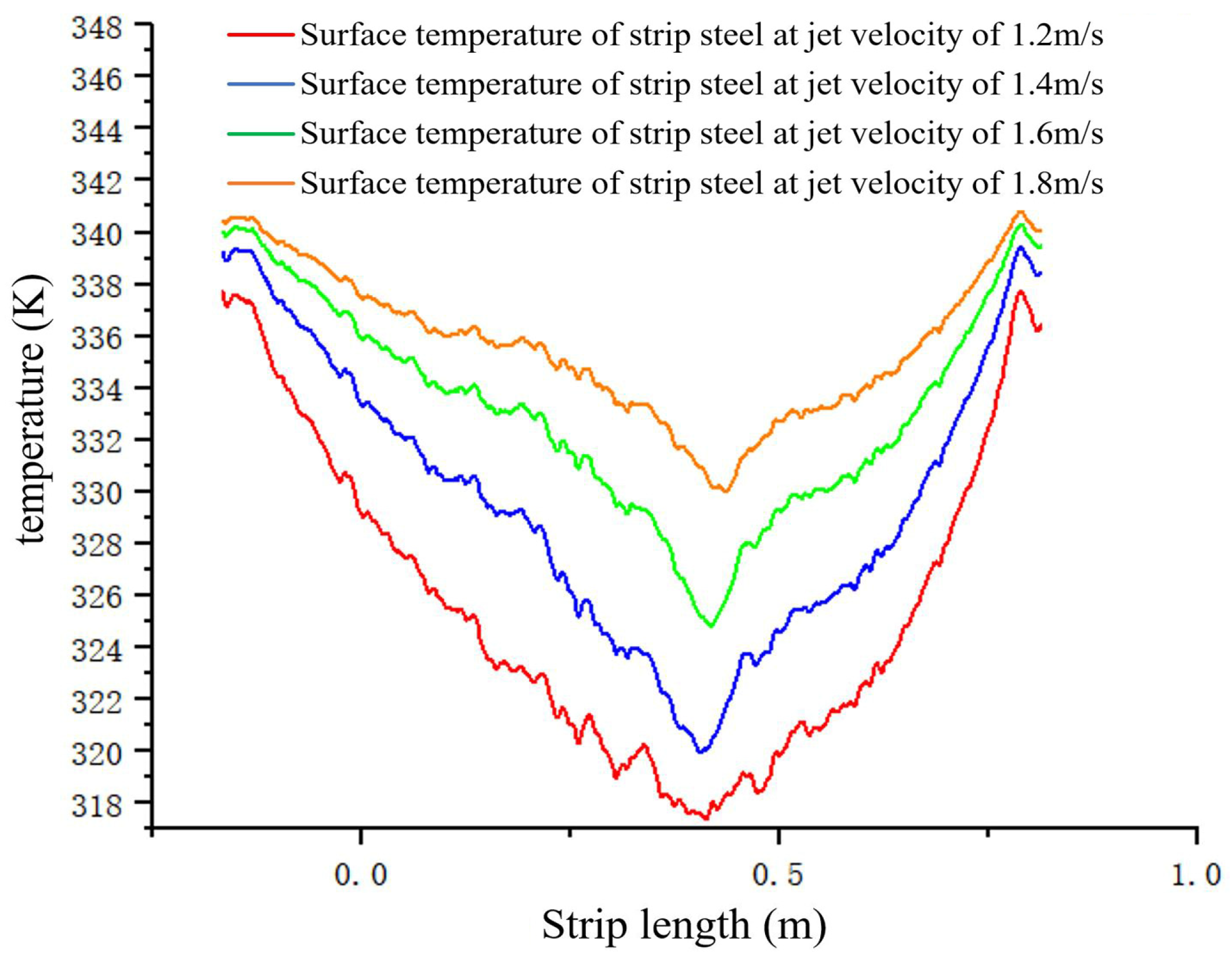
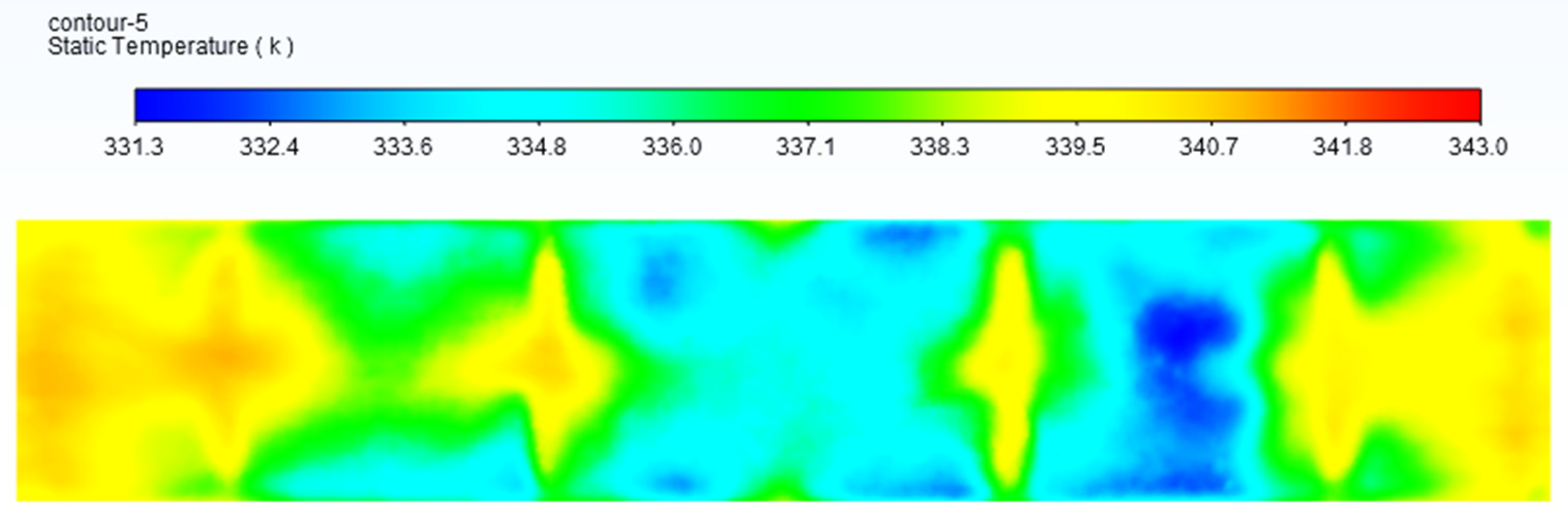
3.1. Flow Field and Heat Transfer Simulation Results at Different Injection Velocities
3.2. Flow Field and Heat Transfer Simulation Results at Different Strip Speeds
4. Discussion
4.1. Effect of Injection Velocity on Flow Field and Heat Transfer
4.2. Effect of Strip Movement Speed on Flow Field and Heat Transfer
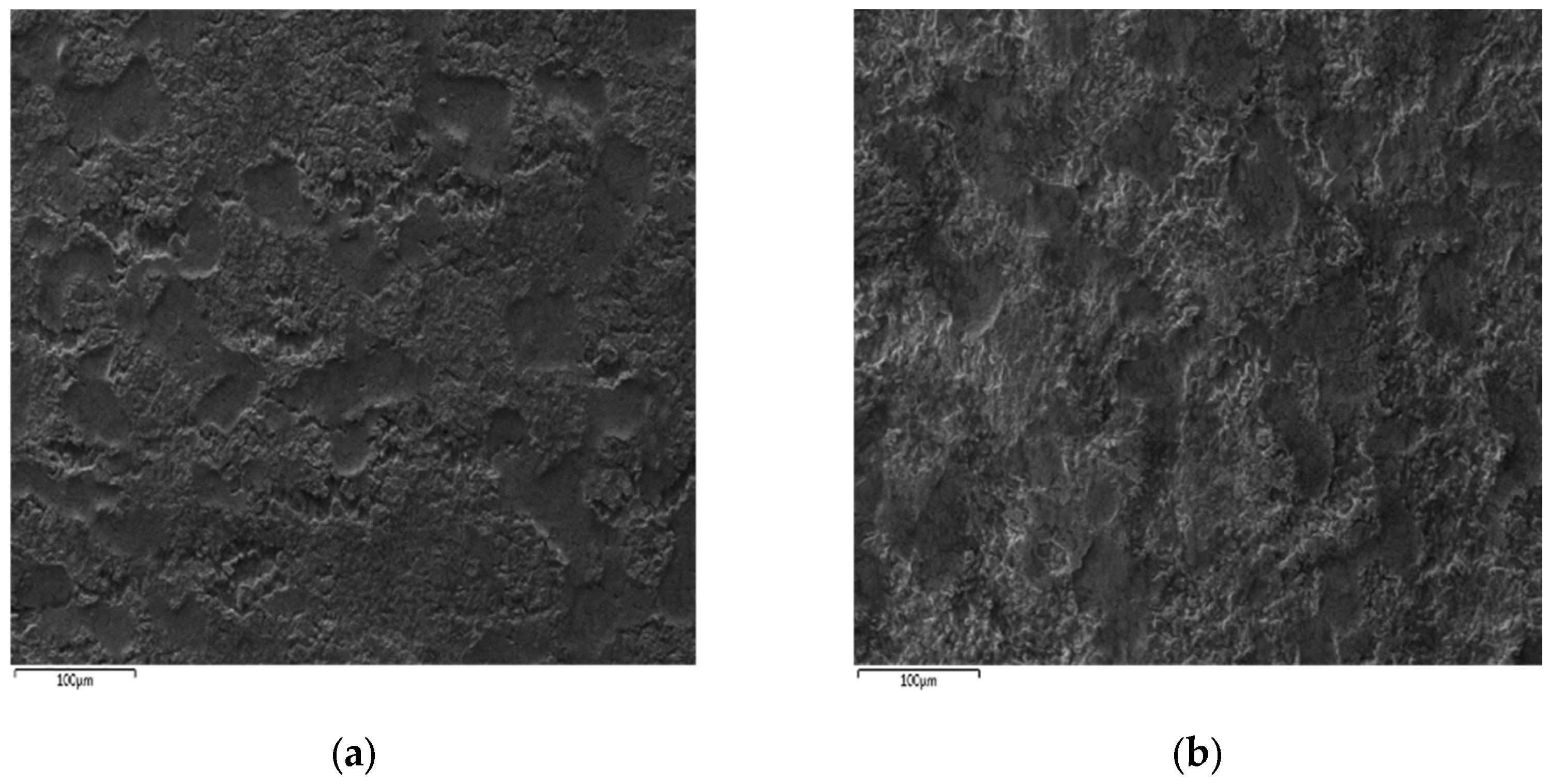
5. Application of Research Results
6. Conclusions
- (1)
- The turbulence intensity is influenced by the flow rate of the injection ports on the acid tank’s sides and the lower side of the strip. It is not significantly affected by increasing the incidence velocity. However, at high incidence velocities, the jet has a considerable impact on turbulence severity and temperature rise at the strip’s exit and entrance, while its impact inside the acid tank is relatively minor;
- (2)
- Enhancing the heat exchange between the acid and the strip can be achieved by increasing the incidence speed and the flow rate of the acid jet. This approach encourages the strip’s temperature to rise closer to the acid’s temperature, leading to accelerated chemical pickling reactions and improved pickling quality;
- (3)
- The velocity of the strip’s movement plays a crucial role in determining the turbulence formed on its surface within the acid bath. By increasing the strip’s motion speed, the rate of surface heat transfer can be enhanced, resulting in more uniform heating.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, Y.; Liao, W.; Liu, X. Online measurement system for acid concentration in cold rolling pickling line. J. Wuhan Eng. Vocat. Tech. Coll. 2022, 34, 41–44. [Google Scholar]
- Li, H.; Wu, S.; Lu, J.; Yue, C. Oxide Scales on Commercial Hot Rolled 1.5% Si Non-oriented Electrical Steel and the Pickling Behaviors. Metallogr. Microstruct. Anal. 2022, 11, 183–190. [Google Scholar] [CrossRef]
- Xingrun, C.; Jing, G.; Kai, H. Immersion pickling of oxide layer on hot-rolled 2205 duplex stainless steel in sulfuric acid with different additives. Metall. Res. Technol. 2022, 119, 210. [Google Scholar]
- Li, Y.; Xia, L.; Sun, R.-S. Study on the effect of element enrichment on the surface of high-strength pickling steel on the film-forming performance of phosphating film. J. Phys. Conf. Ser. 2023, 2454, 012020. [Google Scholar] [CrossRef]
- Hudson, R.M.; Warning, C.J. Effect of strip velocity on pickling rate of hot-rolled steel in hydrochloric acid. J. Miner. Met. Mater. Soc. 1982, 2, 65–70. [Google Scholar] [CrossRef]
- Zhaoning, Z.; Jie, Z.; Ning, K.; Hongbo, L. Strip Pickling Efficiency on the Basis of Combined Parameters Optimization during Tension Leveling Process. J. Mech. Eng. 2019, 55, 51–63. [Google Scholar] [CrossRef]
- Yamaguchi, S.; Yoshida, T.; Saito, T. Improvement in Descaling of Hot Strip by Hydrochloric Acid. ISIJ Int. 1994, 34, 670–678. [Google Scholar] [CrossRef]
- Peng, J.; Yang, Y.; Li, M. Pickling behavior of 2205 duplex stainless steel hot-rolled strips in mixed solutions of HNO3-HF. Mater. Res. Express 2022, 7, 046502. [Google Scholar] [CrossRef]
- Li, N.; Liu, C.; Zhao, Y. Improvement and quality control of turbulent flow pickling process for cold rolled silicon steel shallow grooves. Jiangxi Metall. 2018, 38, 23–26. [Google Scholar]
- Grube, N.E.; Martín, M.P. Compressibility effects on Reynolds stress amplification and shock structure in shock-isotropic turbulence interactions. J. Fluid Mech. 2023, 958, A1. [Google Scholar] [CrossRef]
- Cheng, C.; Fu, L. A scale-based study of the Reynolds number scaling for the near-wall streamwise turbulence intensity in wall turbulence. Int. J. Heat Fluid Flow 2023, 101, 109136. [Google Scholar] [CrossRef]
- Shao, S. A Study on the Mathematical Model of Turbulent Hydrochloric Acid Pickling for Hot Rolled Strip Steel. Master’s Thesis, East China University of Science and Technology, Shanghai, China, 2015; pp. 33–45. [Google Scholar]
- Noda, N.; Hendra, H.; Oosato, M.; Suzumoto, K.; Takase, Y.; Li, W.B. Strength Analysis for Shrink Fitting System Used for Ceramics Rolls in the Continuous Pickling Line. Key Eng. Mater. 2011, 462, 1140–1145. [Google Scholar]
- O’Donovan, T.S.; Murray, D.B. Fluctuating fluid flow and heat transfer of an obliquely impinging air jet. Int. J. Heat Mass Transf. 2008, 51, 25–26. [Google Scholar] [CrossRef]
- Yang, Y.T.; Wang, Y.X. Three-dimensional numerical simulation of an inclined jet with cross-flow. Int. J. Heat Mass Transf. 2005, 48, 19–20. [Google Scholar] [CrossRef]
- Pielsticker, K. Modern pickling lines with Vario technology. Metall. Plant Technol. Int. 1995, 18, 4. [Google Scholar]
- Dhamodaran, M.; Jegadeesan, S.; Kumar, R.P. Analysis and Calculation of the Fluid Flow and the Temperature Field by Finite Element Modeling. Meas. Sci. Rev. 2018, 18, 59–64. [Google Scholar] [CrossRef]
- Alves, M.A.; Oliveira, P.J.; Pinho, F.T. Numerical Methods for Viscoelastic Fluid Flows. Annu. Rev. Fluid Mech. 2021, 53, 509–541. [Google Scholar] [CrossRef]
- Ye, T.; Pan, D.Y.; Huang, C.; Liu, M.B. Smoothed particle hydrodynamics (SPH) for complex fluid flows: Recent developments in methodology and applications. Phys. Fluids 2019, 31, 011301. [Google Scholar] [CrossRef]
- Shadloo, M.S.; Oger, G.; Le Touze, D. Smoothed particle hydrodynamics method for fluid flows, towards industrial applications: Motivations, current state, and challenges. Comput. Fluids 2016, 136, 11–34. [Google Scholar] [CrossRef]
- Asbar; Mubarak, A.Z.; Supanji, M.; Irwansyah; Irwansyah. Stress Analysis of the LN2 Storage Container on Head and Nozzles Using Finite Element Method. In Proceedings of the 2nd International Conference on Experimental and Computational Mechanics in Engineering: ICECME 2020, Banda Aceh, Indonesia, 13–14 October 2020; 2020. [Google Scholar]
- Zhao, J.; Wu, G.; Zhang, J. Thermodynamics-CFD-DPM modeling of dross formation and diffusion in a Zn-0.3Al galvanizing bath. J. Mater. Res. Technol. 2023, 23, 3651–3664. [Google Scholar] [CrossRef]
- He, F.; Li, M.; Wang, B.J. Multi-mode acid concentration prediction models of cold-rolled strip steel pickling process. J. Process Control 2014, 24, 916–923. [Google Scholar]
- Gines, M.J.L.; Benitez, G.J.; Perez, T.; Merli, E.; Firpo, M.A.; Egli, W. Study of the picklability of 1.8 mm hot-rolled steel strip in hydrochloric acid. Lat. Am. Appl. Res. 2002, 32, 281–288. [Google Scholar]
- Kalasin, N.N.; Yenchum, S.; Nilsonthi, T. Adhesion behaviour of scales on hot-rolled steel strips produced from continuous casting slabs. Mater. Today-Proc. 2018, 5, 9359–9367. [Google Scholar] [CrossRef]
- Peng, J.G.; Li, M.C. Pickling Behaviour of 2205 Duplex Stainless Steel Hot-Rolled Strips in Sulfuric Acid Electrolytes. Adv. Mater. Sci. Eng. 2020, 2020, 4562418. [Google Scholar] [CrossRef]
- Liu, X.J.; Cao, G.M.; Nie, D.M.; Liu, Z.Y. Mechanism of Black Strips Generated on Surface of CSP Hot-Rolled Silicon Steel. J. Iron Steel Res. Int. 2013, 20, 54–59. [Google Scholar] [CrossRef]
- Khoo, B.C.; Xu, D. A computer analysis of turbulent flow of Acid in the pickling of steel strips. IC-SEC 2002, 1, 171–175. [Google Scholar]
- Mehrle, A.; Gittler, P.; Javurek, M.; Osterkorn, A. Numerical, Experimental and Analytical Investigation of the Mass Outflow from a Pickling Tank. In Proceedings of the ASME Pressure Vessels and Piping Conference, San Diego, CA, USA, 25–29 July 2004; American Society of Mechanical Engineers, Pressure Vessels and Piping Division: San Diego, CA, USA, 2004; Volume 46865, pp. 191–199. [Google Scholar]
- Wu, D.; Wang, Y.; Liao, Y.; Zhou, Y.; Tian, X.; Xu, L. Study on the pickling behavior and efficient pickling process of hot-rolled SPHC strip steel. Roll. Steel 2022, 39, 44–50. [Google Scholar]
- Zou, H.; Yang, Y.; Qin, X. Prediction of Acid Concentration in Continuous Pickling of Strip Steel Based on Deep Learning Optimized by Artificial Bee Colony. In Proceedings of the 34th Chinese Control and Decision Conference, Hefei, China, 15–17 August 2022. [Google Scholar]
- Wang, S. Research and Application of Cold Rolling Pickling Model. Shandong Metall. 2022, 44, 45–47. [Google Scholar]











| Parameter | Value |
|---|---|
| Hydrochloric acid density (kg/m3) | 1087 |
| Specific heat capacity of hydrochloric acid (kg·K) | 4180 |
| Hydrochloric acid viscosity (kg/m·s) | 0.001 |
| Hydrochloric acid thermal conductivity (m·K) | 0.6 |
| Incident angle of acid solution (°) | 60 |
| Strip initial temperature (K) | 300.15 |
| Acid temperature (K) | 348.15 |
| Strip speed (m/min) | 86 |
| Injection speed on the upper side of the strip (m/s) | 1.4 |
| Injection speed on the lower side of the strip (m/s) | 1.4 |
| Parameters | 1st Group | 2nd Group | 3rd Group | 4th Group |
|---|---|---|---|---|
| Incident velocity v (m/s) | 1.2 | 1.4 | 1.6 | 1.8 |
| Acid flow Q (m3/h) | 50.22 | 58.58 | 66.95 | 75.32 |
| Inlet Reynolds number Re | 25,000 | 30,000 | 35,000 | 40,000 |
| Surface average turbulence intensity (%) | 22.5 | 25.3 | 30.1 | 33.4 |
| Surface averaged turbulent kinetic energy (m2/s2) | 0.085 | 0.110 | 0.173 | 0.257 |
| Surface average turbulence intensity (%) | 8.4 | 8.4 | 8.5 | 8.7 |
| Surface-averaged turbulent kinetic energy (m2/s2) | 0.017 | 0.018 | 0.019 | 0.018 |
| Parameters | 1st Group | 2nd Group | 3rd Group | 4th Group |
|---|---|---|---|---|
| Incident velocity v (m/s) | 1.2 | 1.4 | 1.6 | 1.8 |
| Convective heat transfer coefficient (W/m2·K) | 368 | 443 | 545 | 582 |
| Average temperature of strip surface (K) | 318.95 | 320.55 | 323.45 | 325.35 |
| Convective heat transfer coefficient (W/m2·K) | 534 | 597 | 684 | 728 |
| Average temperature of strip surface (K) | 327.75 | 330.95 | 332.35 | 334.55 |
| Parameters | 1st Group | 2nd Group | 3rd Group | 4th Group |
|---|---|---|---|---|
| Strip speed R(m/min) | 60 | 90 | 120 | 150 |
| Surface average turbulence intensity (%) | 25.7 | 30.4 | 31.5 | 34.6 |
| Surface-averaged turbulent kinetic energy (m2/s2) | 0.106 | 0.135 | 0.152 | 0.175 |
| Surface average turbulence intensity (%) | 8.4 | 9.6 | 11.5 | 14.6 |
| Surface-averaged turbulent kinetic energy (m2/s2) | 0.013 | 0.016 | 0.020 | 0.028 |
| Convection heat transfer coefficient (W/m2·K) | 4.38 | 4.40 | 4.48 | 4.49 |
| Average strip surface temperature (℃) | 48.5 | 49.3 | 50.1 | 50.5 |
| Convection heat transfer coefficient (W/m2·K) | 5.49 | 5.64 | 5.70 | 5.74 |
| Average strip surface temperature (℃) | 57.4 | 58.9 | 61.5 | 63.3 |
| Parameters | C Content | Si Content | Mn Content | Coiling Temperature |
|---|---|---|---|---|
| Parameters value | 0.005 % | 0.4% | 1.85% | 500 °C |
| Parameters | Acid Injection Speed of Above Side (m/s) | Acid Injection Speed of Below Side (m/s) | Strip Speed (m/min) |
|---|---|---|---|
| Parameters value | 1.6 | 1.6 | 115 |
| Parameters | Before the Experiment | After the Experiment | Reduction Value of Color Difference Defect Occurrence Rate |
|---|---|---|---|
| Color difference defect occurrence rate (%) | 15.7 | 2.3 | 13.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cui, X.; Wang, J.; Sun, J.; Elmi, S.A.; Li, X.; Bai, Z. Numerical Simulation of Turbulence Intensity of an Acid Solution during the Strip Steel Pickling Process. Metals 2023, 13, 1293. https://doi.org/10.3390/met13071293
Cui X, Wang J, Sun J, Elmi SA, Li X, Bai Z. Numerical Simulation of Turbulence Intensity of an Acid Solution during the Strip Steel Pickling Process. Metals. 2023; 13(7):1293. https://doi.org/10.3390/met13071293
Chicago/Turabian StyleCui, Xiying, Jianhui Wang, Jiawei Sun, Sahal Ahmed Elmi, Xuetong Li, and Zhenhua Bai. 2023. "Numerical Simulation of Turbulence Intensity of an Acid Solution during the Strip Steel Pickling Process" Metals 13, no. 7: 1293. https://doi.org/10.3390/met13071293
APA StyleCui, X., Wang, J., Sun, J., Elmi, S. A., Li, X., & Bai, Z. (2023). Numerical Simulation of Turbulence Intensity of an Acid Solution during the Strip Steel Pickling Process. Metals, 13(7), 1293. https://doi.org/10.3390/met13071293






