Finite Element Simulation and Experimental Study of U-Bending Forming of High-Strength Mg-Gd-Y-Zn-Zr Alloy
Abstract
:1. Introduction
2. Finite Element Simulation of U-Bending of High-Strength Mg-Gd-Y-Zn-Zr Alloy
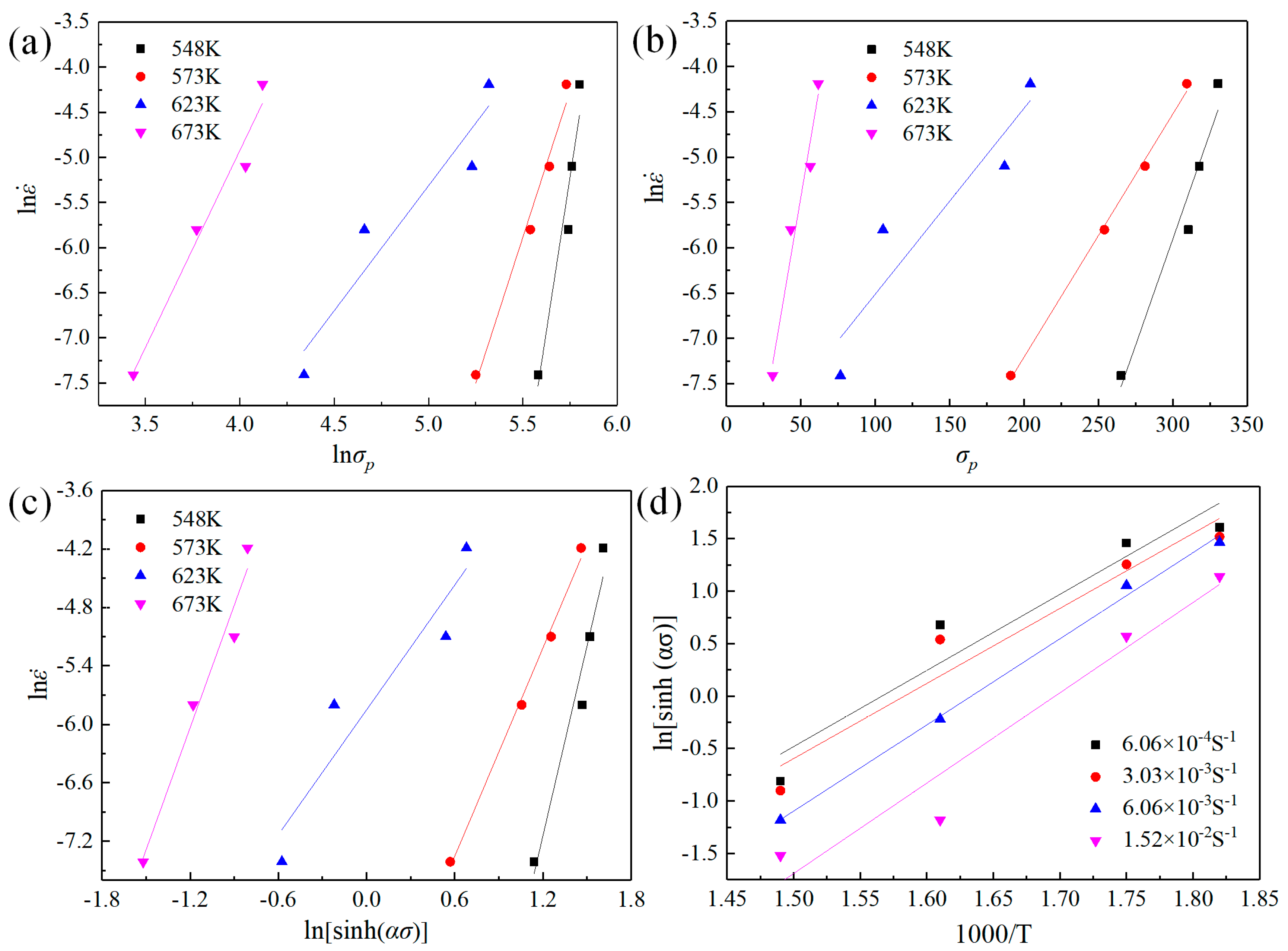
2.1. Construction of Constitutive Equation
2.2. Establishment of Finite Element Model
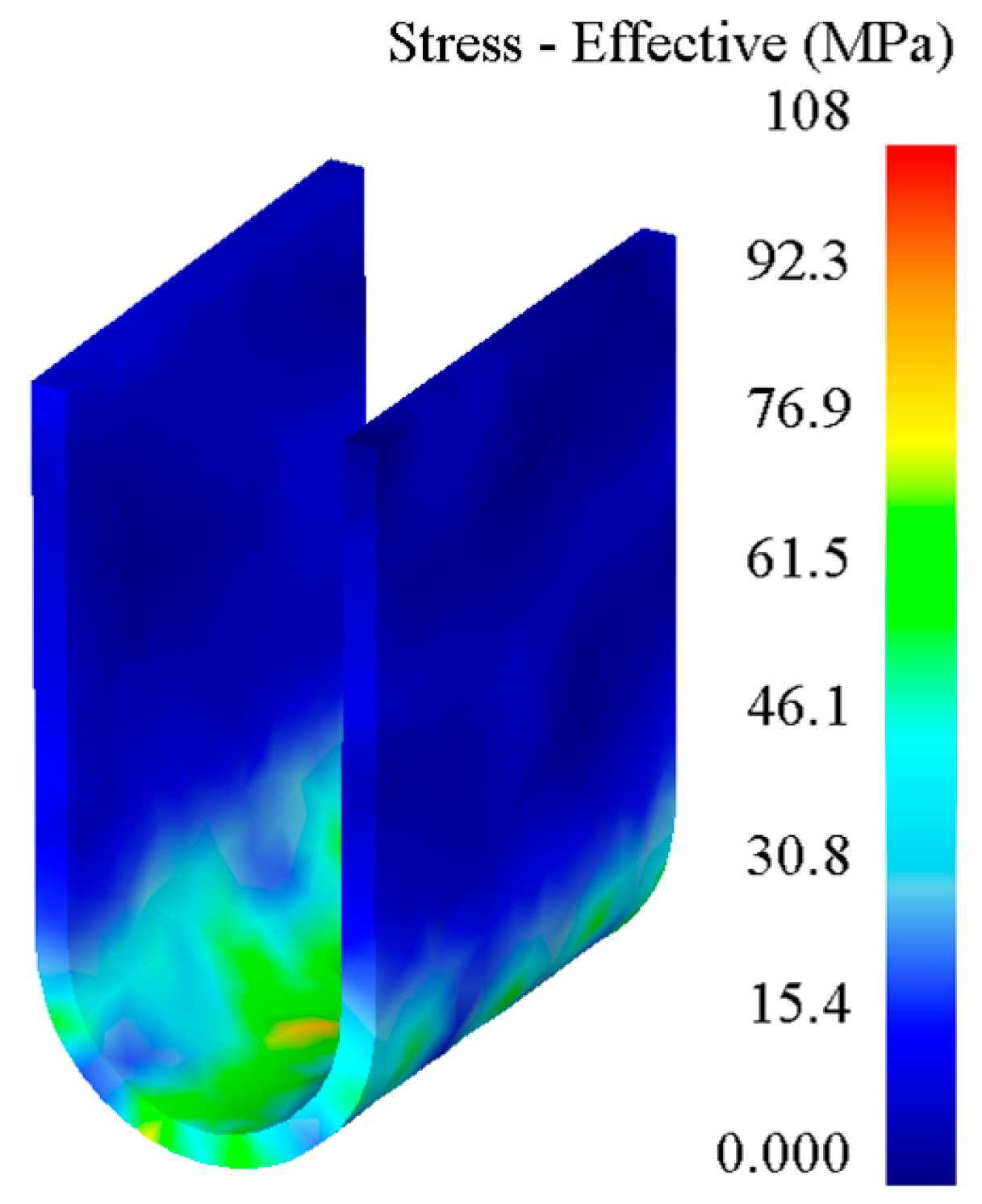
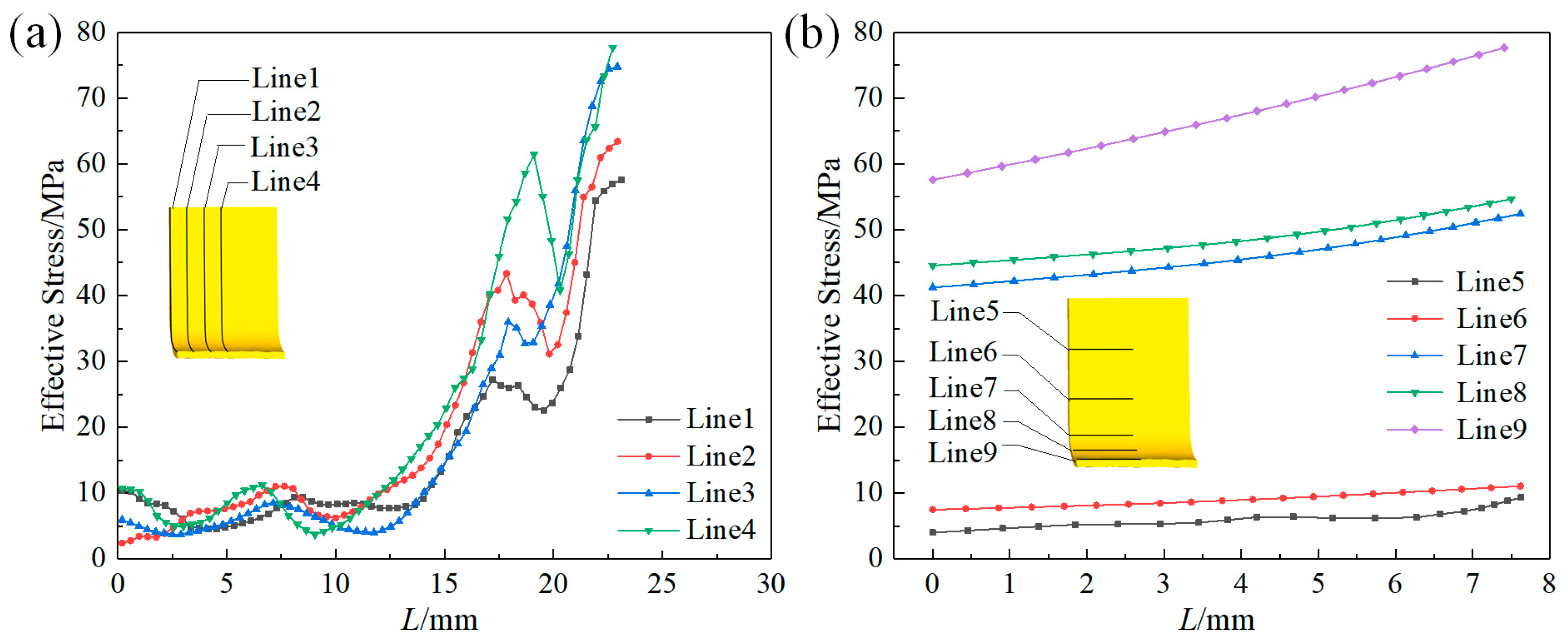
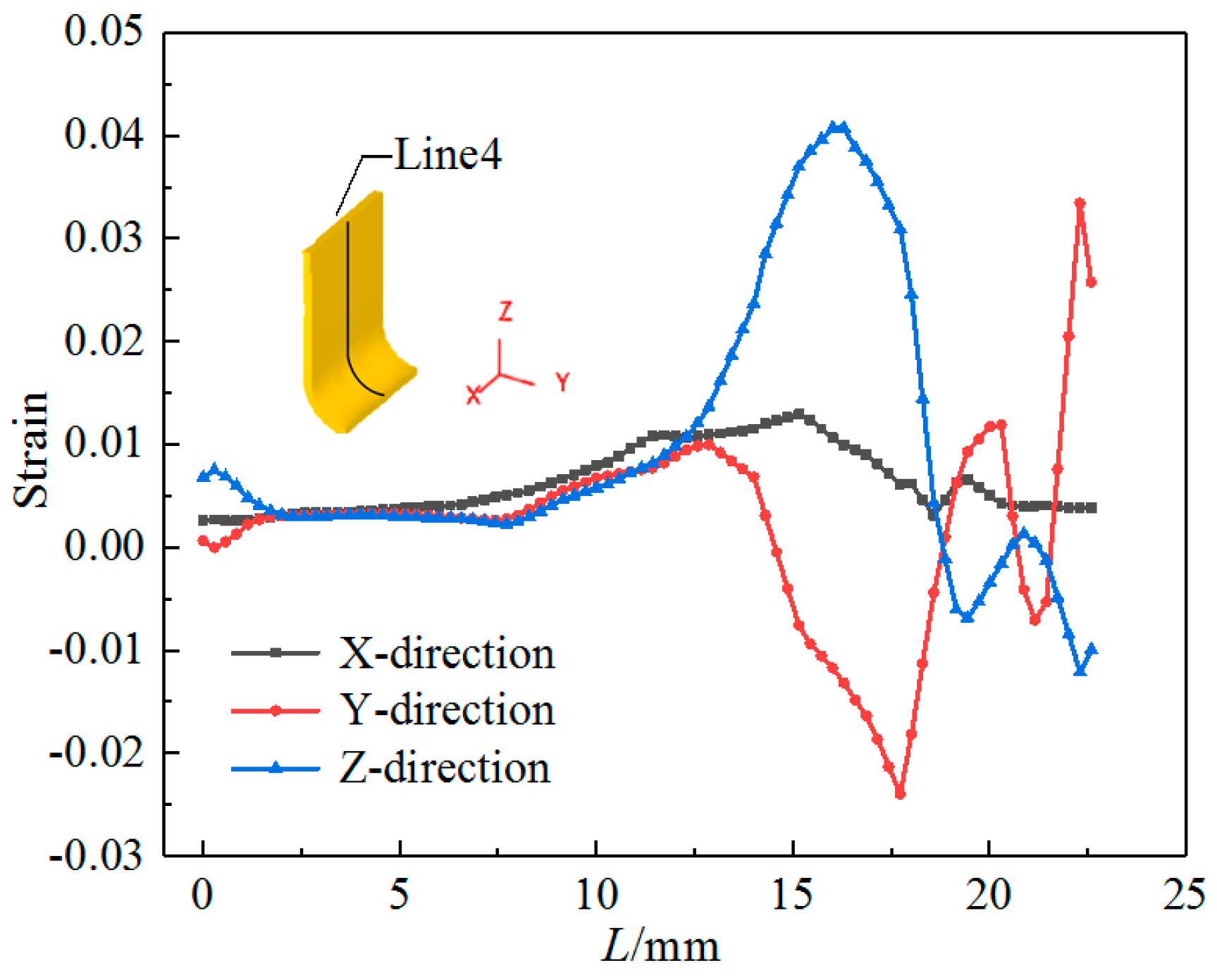
2.3. Analysis of Finite Element Results
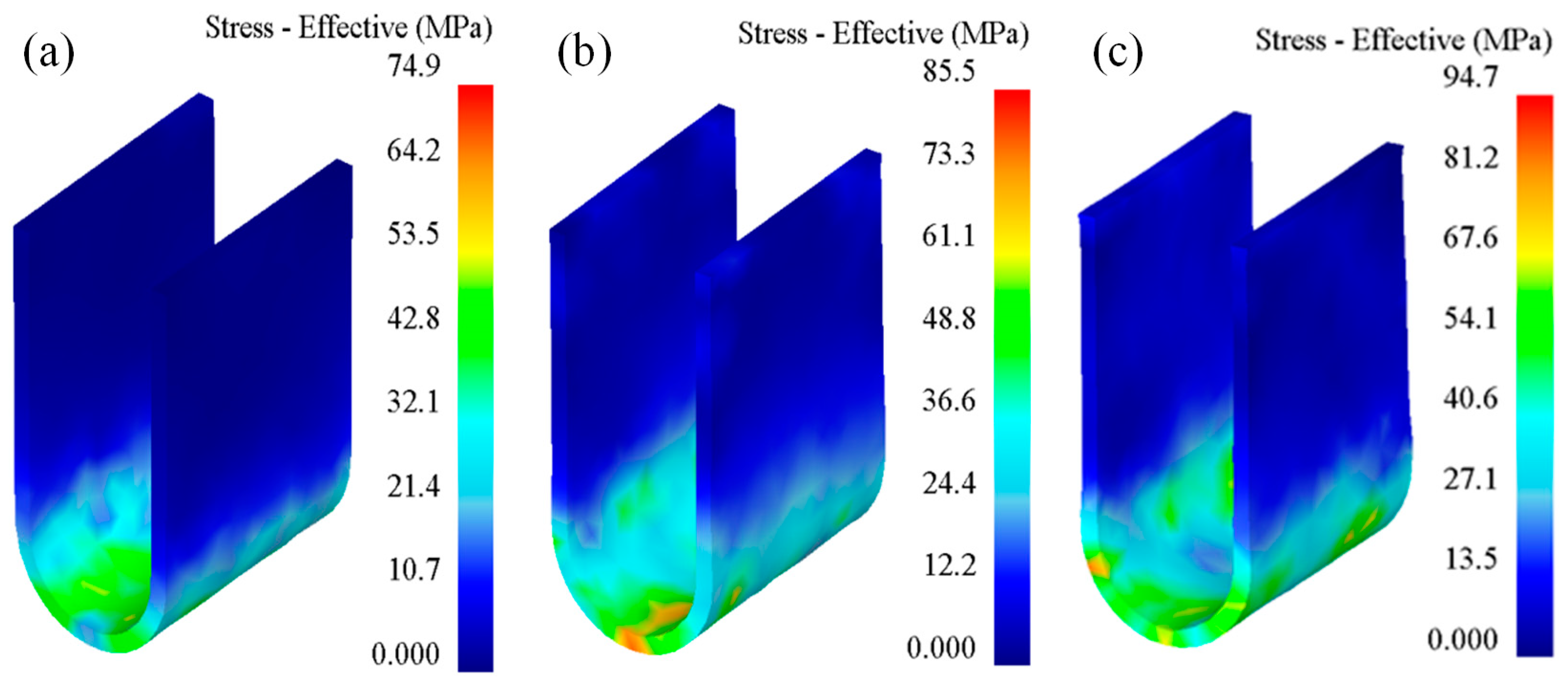
2.3.1. Stress–Strain Analysis
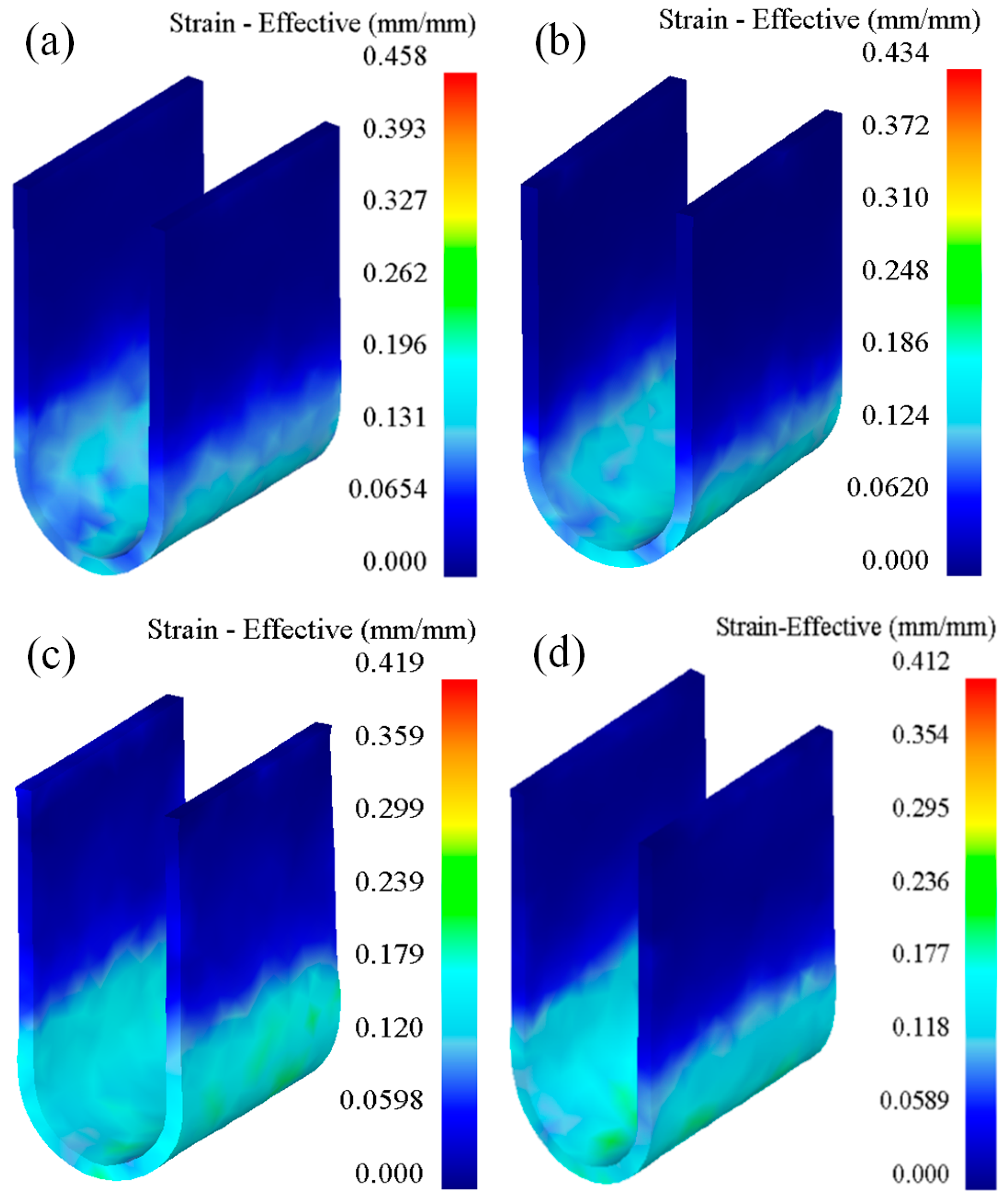
2.3.2. Effect of Temperature on Forming
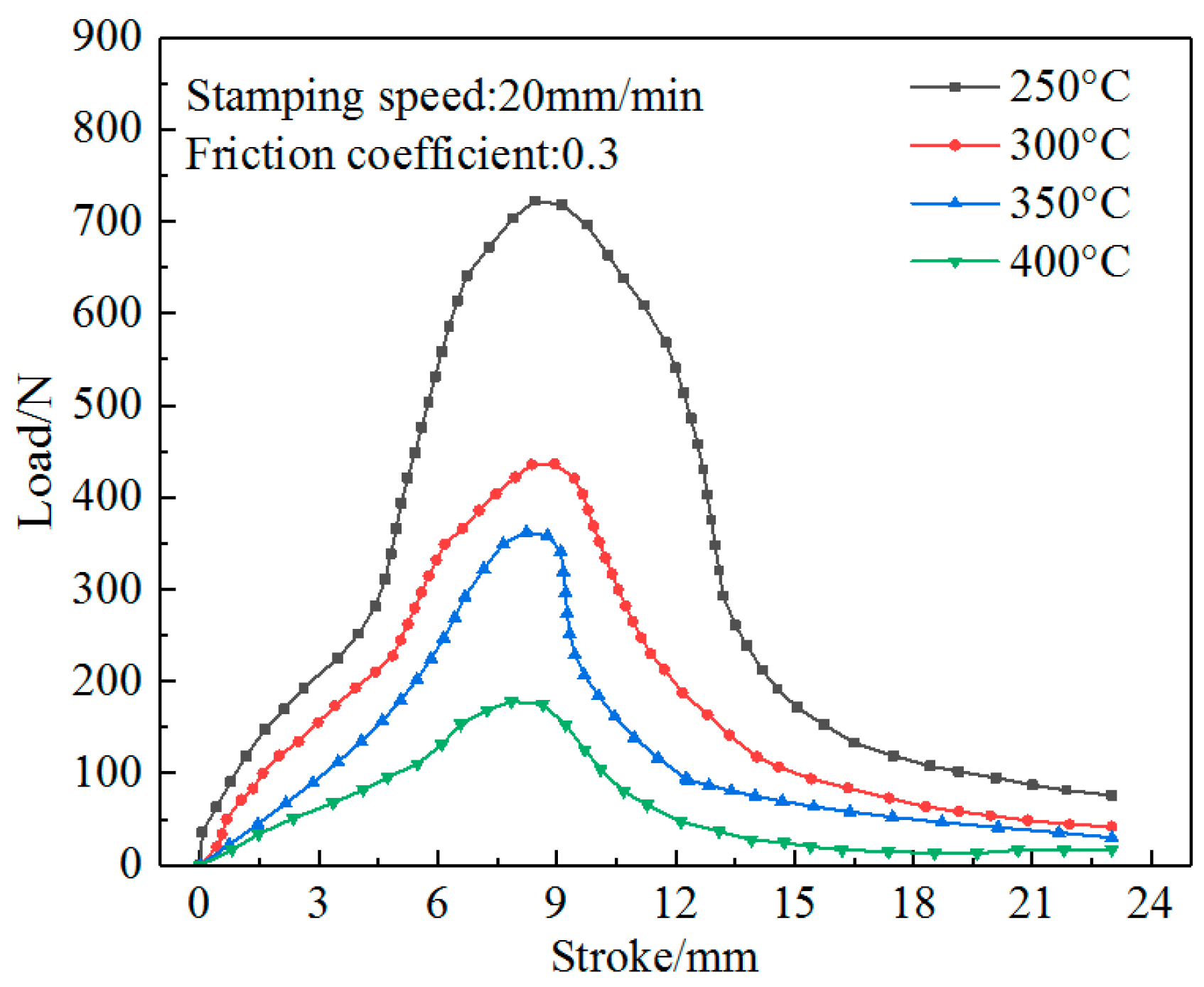
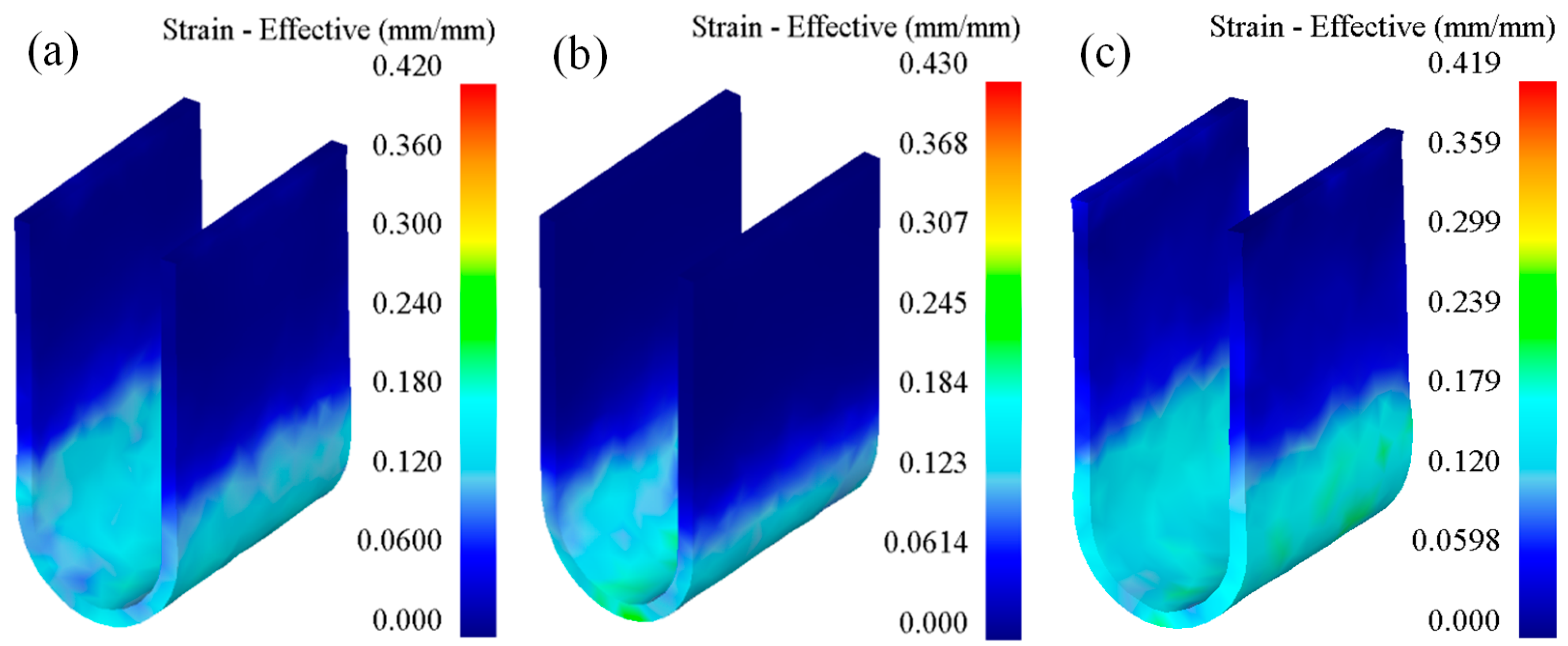
2.3.3. Effect of Stamping Speed on Forming
3. U-Bending Test
4. Conclusions
- (1)
- In this study, a constitutive model for the Mg-Gd-Y-Zn-Zr alloy sheet was established on the basis of tensile tests, and the U-shape bending forming of the sheet under different process conditions was simulated using the finite element software DEFORM. Variation rules of the equivalent stress, equivalent strain, and free bending force of the formed parts were obtained at different temperatures and stamping speeds.
- (2)
- In the bending forming of U-shaped parts, from the straight wall area to the curved corner area and from the edge to the core, the equivalent stress and equivalent strain of the formed part gradually increased. As the forming temperature increased, the equivalent stress, equivalent strain, and free bending force of the formed part decreased. With an increase in the stamping speed, the equivalent stress and free bending force of the formed parts increased, but the equivalent strain did not change significantly.
- (3)
- The error in the maximum free bending force between the test and simulation results was less than 10%. The established material constitutive model can accurately characterize the U-shape bending forming of high-strength Mg-Gd-Y-Zn-Zr alloys. The present research results can provide guidance for the stamping forming of high-strength Mg-Gd-Y-Zn-Zr alloy sheets. However, the influence mechanism between the forming process of U-shaped parts and the microstructure and mechanical properties of materials needs to be further studied.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wu, G.H.; Wang, C.L.; Sun, M.; Ding, W.J. Recent developments and applications on high-performance cast magnesium rare-earth alloys. J. Magnes. Alloys 2021, 9, 1–20. [Google Scholar] [CrossRef]
- Song, J.F.; Chen, J.; Xiong, X.M.; Peng, X.D.; Chen, D.L.; Pan, F.S. Research advances of magnesium and magnesium alloys worldwide in 2021. J. Magnes. Alloys 2022, 10, 863–898. [Google Scholar] [CrossRef]
- Tong, C.P.; Rong, Q.; Yardley, V.A.; Li, X.T.; Luo, J.M.; Zhu, G.S.; Shi, Z.S. New developments and future trends in low-temperature hot stamping technologies: A Review. Metals 2020, 10, 1652. [Google Scholar] [CrossRef]
- Zhang, R.Q.; Shi, Z.S.; Yardley, V.A.; Lin, J.G. Experimental studies of necking and fracture limits of boron steel sheet under hot stamping conditions. J. Mater. Process. Technol. 2022, 302, 117481. [Google Scholar] [CrossRef]
- Atxaga, G.; Arroyo, A.; Canflanca, B. Hot stamping of aerospace aluminium alloys: Automotive technologies for the aeronautics industry. J. Manuf. Process. 2022, 81, 817–827. [Google Scholar] [CrossRef]
- Zhao, P.J.; Wu, Q.; Yang, Y.L.; Chen, Z.H. Process Optimization of the Hot Stamping of AZ31 Magnesium Alloy Sheets Based on Response Surface Methodology. Materials 2023, 16, 1867. [Google Scholar] [CrossRef] [PubMed]
- Wang, H.T.; Ma, L.F.; Jia, W.T.; Xie, H.B.; Lu, L.W. Analysis of room-temperature stamping formability of complex features of AZ31 magnesium alloy variable-curvature plate shell. Int. J. Adv. Manuf. Technol. 2022, 123, 3159–3169. [Google Scholar] [CrossRef]
- Luo, A.A.; Shi, R.H.; Miao, J.S.; Avey, T. Review: Magnesium Sheet Alloy Development for Room Temperature Forming. JOM 2021, 73, 1403–1418. [Google Scholar] [CrossRef]
- Zhang, L.; Huang, G.S.; Zhang, H.; Song, B. Cold stamping formability of AZ31B magnesium alloy sheet undergoing repeated unidirectional bending process. J. Mater. Process. Technol. 2011, 211, 644–649. [Google Scholar] [CrossRef]
- Han, T.Z.; Huang, G.S.; Wang, Y.G.; Wang, G.G.; Zhao, Y.C.; Pan, F.S. Enhanced mechanical properties of AZ31 magnesium alloy sheets by continuous bending process after V-bending. Prog. Nat. Sci. 2016, 26, 97–102. [Google Scholar] [CrossRef]
- Wang, W.R.; Huang, L.; Tao, K.H.; Chen, S.C.; Wei, X.C. Formability and numerical simulation of AZ31B magnesium alloy sheet in warm stamping process. Mater. Des. 2015, 87, 835–844. [Google Scholar] [CrossRef]
- Chen, F.K.; Huang, T.B. Formability of stamping magnesium-alloy AZ31 sheets. J. Mater. Process. Technol. 2003, 142, 643–647. [Google Scholar] [CrossRef]
- Berge, F.; Winderlich, H.; Krbetschek, C.; Ullmann, M.; Kawalla, R. The effect of sheet thickness, loading rate and punch diameter on the deformation behaviour of AZ31 during 3-point bending. Mater. Sci. Forum 2016, 854, 65–72. [Google Scholar] [CrossRef]
- Wei, Y.H.; Lu, L.W.; Li, M.H.; Ma, M.; Huang, W.Y.; Zhao, X.; Wu, R.Z. Effect of 90° route on microstructure of AZ31 magnesium alloy sheets by forging-bending repeated deformation. J. Alloys Compd. 2023, 948, 169720. [Google Scholar] [CrossRef]
- Chen, Y.F.; Zhu, Z.Q.; Zhou, J.X. Study on the strengthening mechanism of rare earth yttrium on magnesium alloys. Mater. Sci. Eng. A 2022, 850, 143513. [Google Scholar] [CrossRef]
- Deng, J.F.; Tian, J.; Zhou, Y.C.; Chang, Y.Y.; Liang, W.; Ma, J.Y. Plastic deformation mechanism and hardening mechanism of rolled Rare-Earth magnesium alloy thin sheet. Mater. Des. 2022, 218, 110678. [Google Scholar] [CrossRef]
- Calado, L.M.; Carmezim, M.J.; Montermor, M.F. Rare Earth Based Magnesium Alloys -A Review on WE Series. Front. Mater. 2022, 9, 804906. [Google Scholar] [CrossRef]
- Wang, Y.J.; Jiang, H.T.; Liu, C.M.; Zhang, Y.; Guo, W.Q.; Kang, Q.; Xu, Z.; Wang, P.P. Effect of Ca-addition on hot deformation behavior and workability of Mg-Gd-Y-Zn-Zr alloy. Rare Metal Mat. Eng. 2020, 49, 1650–1656. [Google Scholar]
- Liu, H.L.; Meng, Y.Z.; Yu, H.S.; Xu, W.L.; Zhang, S.Y.; Jia, L.C.; Wu, G.Q. The role of long period stacking ordered phase in dynamic recrystallization of a Mg-Gd-Y-Zn-Zr Alloy during multi-directional forging process. Materials 2020, 13, 3290. [Google Scholar] [CrossRef]
- Zhou, X.J.; Liu, C.M.; Gao, Y.H.; Jiang, S.N.; Chen, Z.Y. Improved workability and ductility of the Mg-Gd-Y-Zn-Zr alloy via enhanced kinking and dynamic recrystallization. J. Alloys Compd. 2018, 749, 878–886. [Google Scholar] [CrossRef]
- Li, B.; Teng, B.G.; Chen, G.X. Microstructure evolution and mechanical properties of Mg-Gd-Y-Zn-Zr alloy during equal channel angular pressing. Mater. Sci. Eng. A 2019, 744, 396–405. [Google Scholar] [CrossRef]
- Ramezani, S.M.; Zarei-Hanzaki, A.; Anoushe, A.S.; Abedi, H.R.; Minarik, P.; Máthis, K.; Horváth, F.K. A new insight into LPSO transformation during multi-axial forging in Mg-Gd-Y-Zn-Zr alloy. Mater. Lett. 2020, 269, 127625. [Google Scholar] [CrossRef]








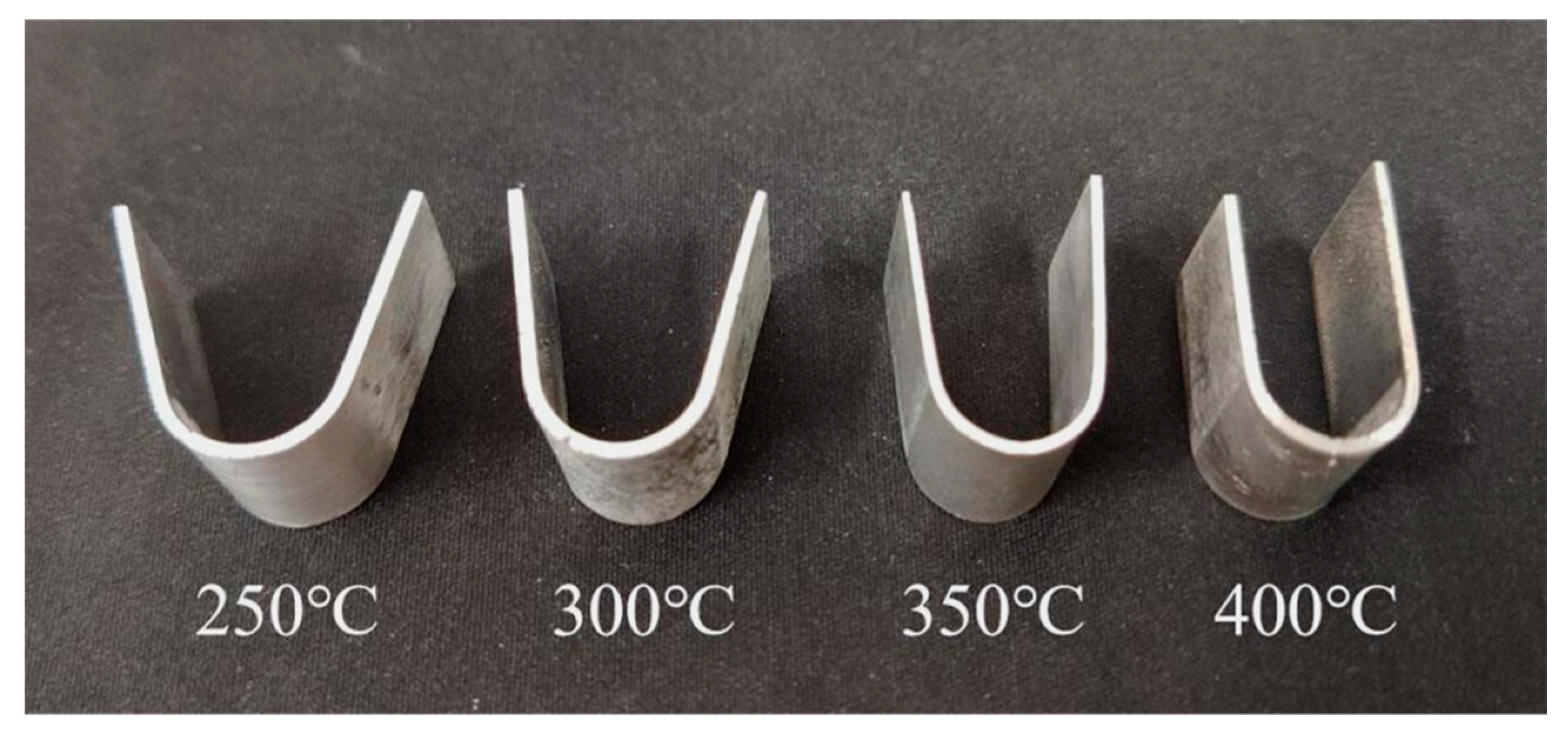
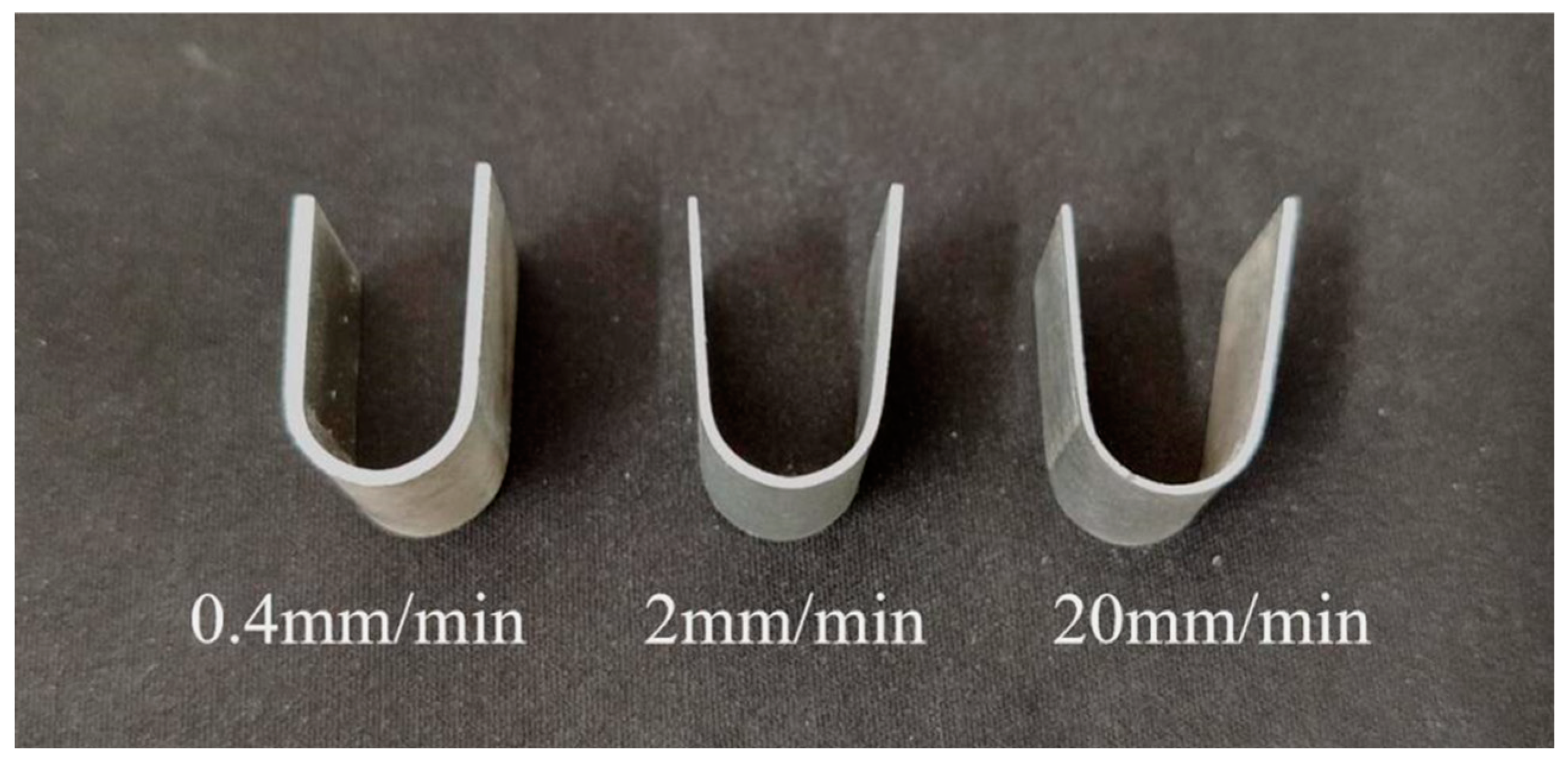

| Process Parameters | Forming Temperature/°C | |||
|---|---|---|---|---|
| 250 | 300 | 350 | 400 | |
| Stamping speed mm/min | -- | -- | 0.4 | -- |
| -- | -- | 2 | -- | |
| 20 | 20 | 20 | 20 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Huang, A.; Xing, S.; Zhang, C.; Luo, J. Finite Element Simulation and Experimental Study of U-Bending Forming of High-Strength Mg-Gd-Y-Zn-Zr Alloy. Metals 2023, 13, 1477. https://doi.org/10.3390/met13081477
Wang H, Huang A, Xing S, Zhang C, Luo J. Finite Element Simulation and Experimental Study of U-Bending Forming of High-Strength Mg-Gd-Y-Zn-Zr Alloy. Metals. 2023; 13(8):1477. https://doi.org/10.3390/met13081477
Chicago/Turabian StyleWang, Hao, Anqi Huang, Shiping Xing, Chunxiang Zhang, and Junting Luo. 2023. "Finite Element Simulation and Experimental Study of U-Bending Forming of High-Strength Mg-Gd-Y-Zn-Zr Alloy" Metals 13, no. 8: 1477. https://doi.org/10.3390/met13081477
APA StyleWang, H., Huang, A., Xing, S., Zhang, C., & Luo, J. (2023). Finite Element Simulation and Experimental Study of U-Bending Forming of High-Strength Mg-Gd-Y-Zn-Zr Alloy. Metals, 13(8), 1477. https://doi.org/10.3390/met13081477





