Melt–Vapor Phase Transition in the Aluminum–Selenium System in Vacuum
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Calculation Methodology
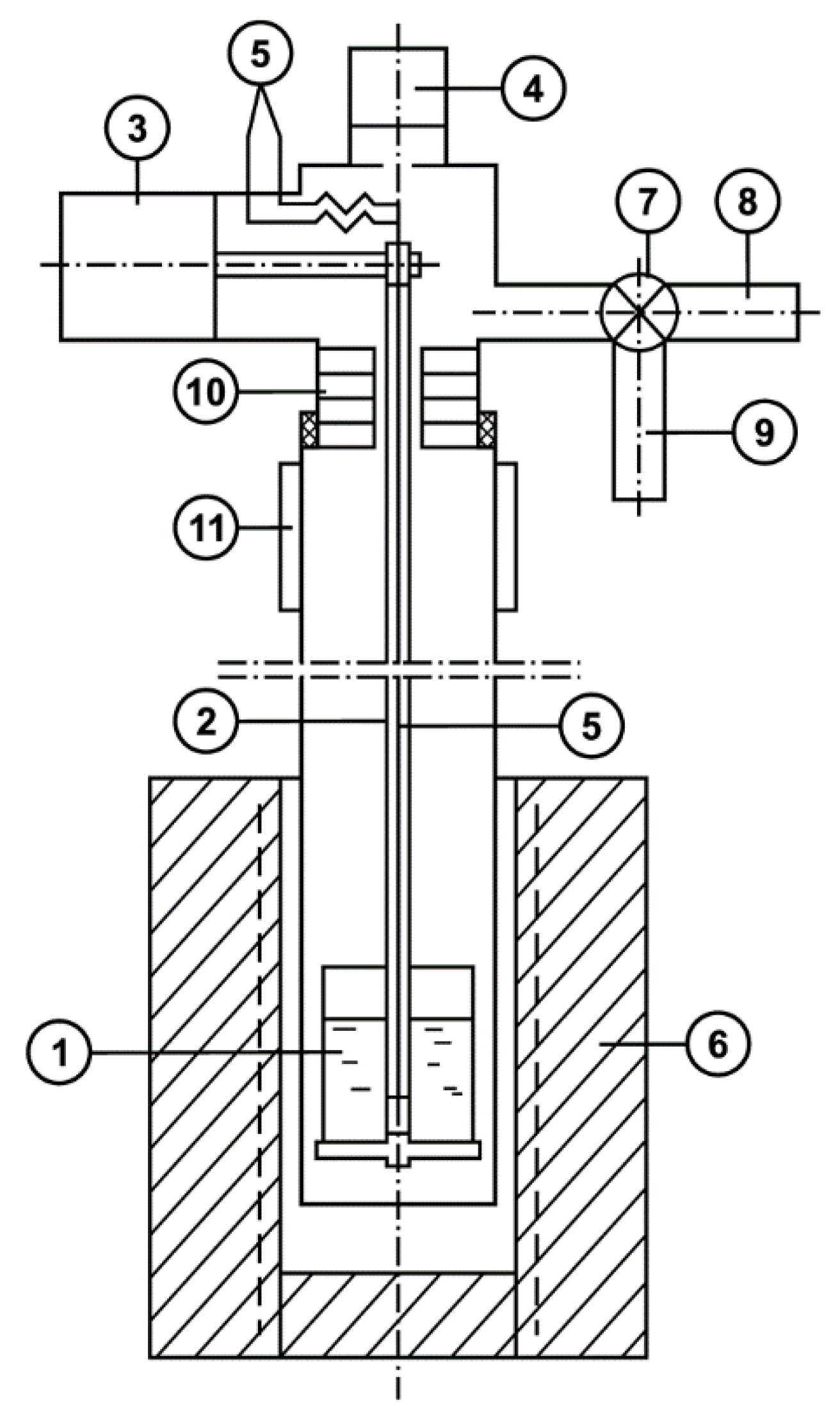
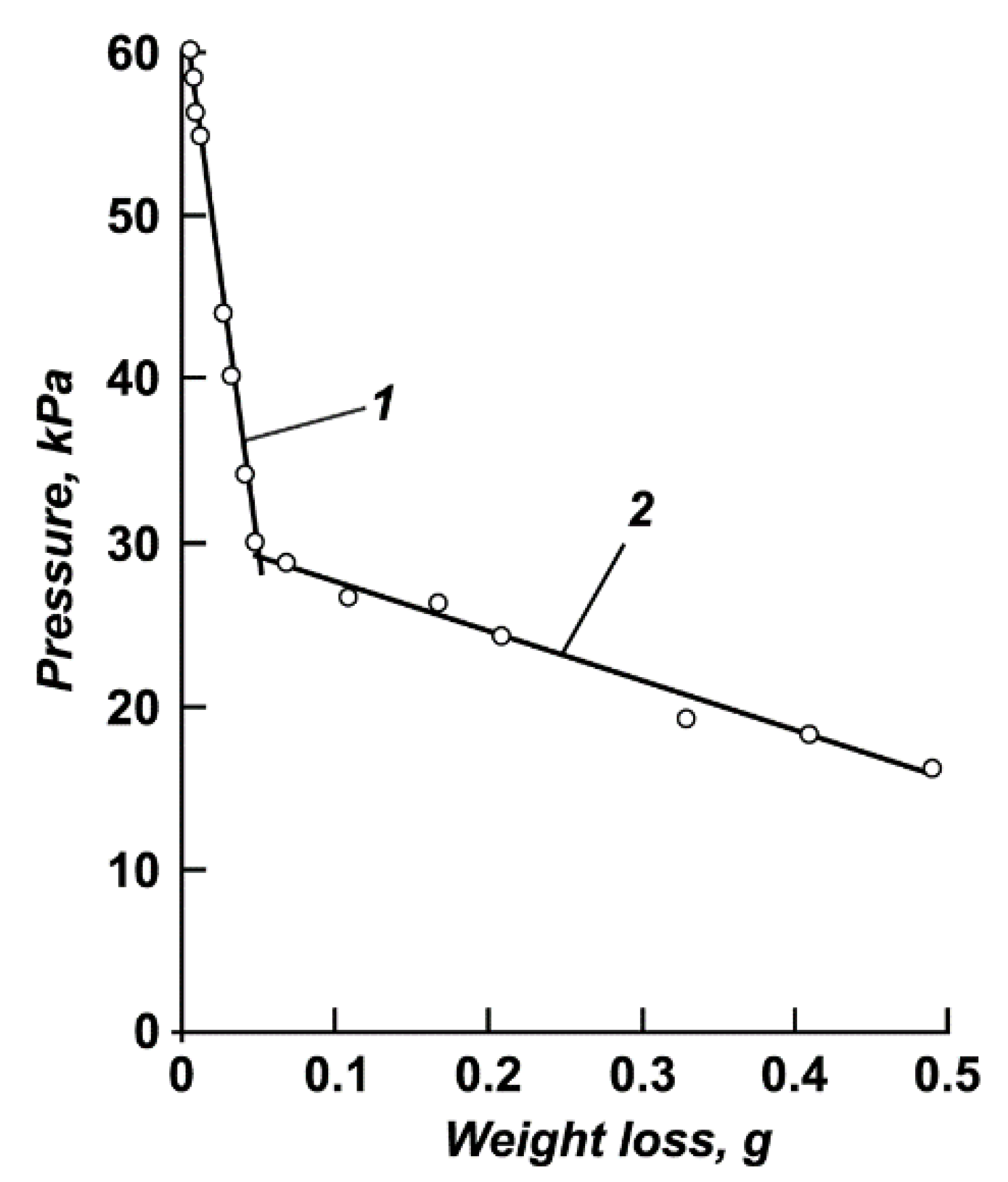
2.3. Method for Determination of the Saturated Vapor Pressure Value
3. Results and Discussion
4. Conclusions
- −
- Refining aluminum from the impurity of selenium, which will be in the melt in the form of aluminum selenide, will not cause technological difficulties since the vapor phase will be mainly represented by the compound Al2Se3;
- −
- Refining selenium from aluminum impurities by distillation from the melt will be accompanied by the accumulation of aluminum selenide in the distillation residue, and the vapor phase will be represented by selenium;
- −
- The field of coexistence of liquid solutions and the vapor phase in the aluminum selenide–selenium system on the state diagram, at both atmospheric and reduced pressure, is superimposed on the two-phase field of coexistence of liquid melts and crystalline aluminum selenide; however, this will not cause technological difficulties.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ding, X.; Li, M.; Chen, P.; Zhao, Y.; Zhao, M.; Leng, H.; Wang, Y.; Ali, S.; Raziq, F.; Wu, X.; et al. Bi2O2Se: A rising star for semiconductor devices. Matter 2022, 5, 4274–4431. [Google Scholar] [CrossRef]
- Ma, L.; Wu, J.; Li, Y.; Lv, Y.; Li, B.; Jin, Z. Rational design of carbon nanotube architectures for lithium–chalcogen batteries: Advances and perspectives. Energy Storage Mater 2021, 42, 723–752. [Google Scholar] [CrossRef]
- Long, C.; Liang, Y.; Jin, H.; Huang, B.; Dai, Y. PdSe2: Flexible twodimensional transition metal dichalcogenides monolayer for water splitting photocatalyst with extremely low recombination rate. ACS Appl. Energy Mater 2019, 2, 513–520. [Google Scholar] [CrossRef]
- Xiao, F.; Lei, W.; Wang, W.; Xu, L.; Zhang, S.; Ming, X. Pentagonal two-dimensional noble-metal dichalcogenide PdSSe for photocatalytic water splitting with pronounced optical absorption and ultrahigh anisotropic carrier mobility. J. Mater. Chem. C 2021, 9, 7753–7764. [Google Scholar] [CrossRef]
- Shi, X.; Zhang, F.; Dai, S.; Zeng, P.; Qu, J.; Song, J. Nanorod-textured Sb2(S,Se)3 bilayer with enhanced light harvesting and accelerated charge extraction for high-efficiency Sb2(S,Se)3 solar cells. Chem. Eng. J. 2022, 437, 135341. [Google Scholar] [CrossRef]
- Kalashnik, O.N.; Zhavoronkov, N.V.; Latyshonok, A.N.; Minazdinov, M.S.; Temiraev, S.O. Obtaining high-purity cadmium, tellurium and mercury by distillation methods. In Proceedings of the XXIV International Scientific and Technical Conference on Photoelectronics and Night Vision Devices, Moscow, Russia, 24–26 May 2016; pp. 143–146. [Google Scholar]
- Andreev, Y.V.; Petrov, G.V.; Belen’kiy, A.M. Pyrometallurgical refining of tellurium. Proc. Great Peter St. Petersburg Polytech. Univ. 2009, 510, 81–85. [Google Scholar]
- Zha, G.; Wang, Y.; Cheng, M.; Huang, D.; Jiang, W.; Xu, B.; Yang, B. Purification of crude selenium by vacuum distillation and analysis. Journal of materials research and technology. J. Mater. Res. Technol. 2020, 9, 2926–2933. [Google Scholar] [CrossRef]
- Zha, G.; Yang, B.; Luo, H.; Huang, D.; Jiang, W.; Xu, B.; Liu, D. Innovative green approach for the selective extraction of high-purity selenium from hazardous selenium sludge. Separ. Purif. Technol. 2021, 266, 118536. [Google Scholar] [CrossRef]
- Kenzhaliyev, B.K.; Trebukhov, S.A.; Nitsenko, A.V.; Burabayeva, N.M.; Trebukhov, A.A. Determination of technological parameters of selenium recovery from metallurgical production middlings in a vacuum distillation unit. Internat. J. Mech. Prod. Eng. Res. Dev. 2019, 9, 87–98. [Google Scholar] [CrossRef]
- Perez-Tello, M.; Sanchez-Corrales, V.M.; Prieto-Sanchez, M.R.; Rodríguez-Hoyos, O. A kinetic model for the oxidation of selenium and tellurium in an industrial kaldo furnace. JOM 2004, 56, 52–54. [Google Scholar] [CrossRef]
- Isakova, R.A.; Nesterov, V.N.; Chelokhsayev, L.S. Fundamentals of Vacuum Pyroseparation of Polymetallic Raw Materials; Nauka: Alma-Ata, Kazakhstan, 1973; 255p. [Google Scholar]
- Obolonchik, V.A. (Ed.) Chemistry and Physics of Chalcogenides; Naukova Dumka: Kiev, Ukraine, 1977; 140p. [Google Scholar]
- Vanyukov, A.V.; Isakova, R.A.; Bystrov, V.P. Thermal Dissociation of Metal Sulfides; Nauka: Alma-Ata, Kazakhstan, 1978; 272p. [Google Scholar]
- Novoselova, A.V.; Pashinkin, A.S. Vapor Pressure of Volatile Metal Chalcogenides; Nauka: Moscow, Russia, 1978; 112p. [Google Scholar]
- Weisburd, S.E. Physical and Chemical Properties and Peculiarities of the Structure of Sulfide Melts; Metallurgy: Moscow, Russia, 1996; 304p. [Google Scholar]
- Kopylov, N.I.; Lata, V.A.; Polyvyanyi, I.R.; Khegai, L.D. State Diagrams of Double and Triple Thiosystems; P&CE Zhuk LLC.: Komsomolsk-on-Amur, Russia, 1999; 177p. [Google Scholar]
- Volodin, V.N.; Trebukhov, S.A. Distillation Processes of Selenium Extraction and Refining; Tengri Ltd.: Karaganda, Kazakhstan, 2017; 220p. [Google Scholar]
- Shi, C.; Yang, B.; Hu, B.; Du, Y.; Yao, S. Thermodynamic description of the Al—X (X = S, Se, Te) systems. J. Phase Equilib. Diffus. 2019, 40, 392–402. [Google Scholar] [CrossRef]
- Bardi, G.; Trionfetti, G. The vapour pressure over Al2Se3. Thermochim. Acta 1988, 136, 313–314. [Google Scholar] [CrossRef]
- Lyakishev, N.P. (Ed.) State Diagrams of Dual Metallic Systems; Mashinostroenie: Moscow, Russia, 1996; Volume 1, 992p. [Google Scholar]
- Kapustinskiy, A.F.; Golutvin, Y.M. Thermochemistry and structure of atoms. Heat of formation of aluminum compounds with elements of Group VI of the periodic system of D.I. Mendeleev. Proc. Acad. Sci. USSR Dep. Chem. Sci. 1951, 2, 192–200. [Google Scholar]
- Joël, H.A.; Schneider, A. Die bildungsentalpie von aluminiumtellurid. Naturwissenschaften 1967, 54, 587. [Google Scholar] [CrossRef]
- Gattow, D.G. Orientierende berechnung der bildungwärmen von aluminium (I,II)–chalkogeniden. Angew. Chem. 1956, 68, 521. [Google Scholar] [CrossRef]
- Ficalora, P.J.; Hastie, J.W.; Margrave, J.L. Mass spectrometric studies at high temperatures. XXVII. The reactions of aluminum vapor with S2(g), Se2(g), and Te2(g). J. Phys. Chem. 1968, 72, 1660–1663. [Google Scholar] [CrossRef]
- Said, H.; Castanet, R.; Kehiaian, H.V. Étude calorimétrique du systéme binaire aluminium–tellure. J. Less-Comm. Met. 1976, 46, 209–215. [Google Scholar] [CrossRef]
- Said, H.; Chastel, R.; Bergman, C.; Castanet, R. Thermodynamic investigation on Al—Te alloys by differential thermal analysis and Knudsen-cell mass-spectrometry. Z. Met. 1981, 72, 360–365. [Google Scholar] [CrossRef]
- Ferro, D.; Nappi, B.M.; Balducci, G.; Placente, V. A vaporization study of Al2Te3. Thermochim. Acta 1980, 35, 35–41. [Google Scholar] [CrossRef]
- Blot, J.; Rogez, J.; Castanet, R. Étude potentiométrique des alliages liquides aluminium—Étain et al.uminium—Tellure. J. Less-Comm. Met. 1986, 118, 67–82. [Google Scholar] [CrossRef]
- Kniep, R.; Blees, P. Phasengleichgewichte und intermediäre phasen im System Al—Te. Z. Naturforsch 1988, 43b, 182–188. [Google Scholar] [CrossRef]
- Prabhu, N.; Howe, J.M. The Al—Te (Aluminium—Tellurium) system. Bull. Alloy Phase Diagr. 1990, 11, 202–206. [Google Scholar] [CrossRef]
- Oh, C.-S.; Lee, D.N. Thermodynamic assessments of the In—Te and Al—Te systems. CALPHAD 1993, 17, 175–187. [Google Scholar] [CrossRef]
- Burabaeva, N.; Volodin, V.; Trebukhov, S.; Nitsenko, A.; Linnik, X. On the distillation separation of Aluminum—Tellurium system melts under equilibrium condition. Metals 2022, 12, 2059. [Google Scholar] [CrossRef]
- Burabaeva, N.M.; Volodin, V.N.; Nitsenko, A.V.; Tuleutai, F.K. Evaporation thermodynamics and sublimation of aluminum telluride. Kompleksnoe Ispolzovanie Mineralnogo Syra. 2022, 323, 87–92. [Google Scholar] [CrossRef]
- Howe, J.M. The Al—Se (Aluminum—Selenium) system. Bull. Alloy Phase Diagr. 1989, 10, 650–652. [Google Scholar] [CrossRef]
- Steigmann, G.A.; Goodyear, J. The crystal structure of Al2Se3. Acta Cryst. 1966, 20, 617–619. [Google Scholar] [CrossRef]
- Uy, O.M.; Drowart, J. Determination by the mass spectrometric Knudsen cell method of the atomization energies of the gaseous aluminum chalcogenides, Al2, AlCu, AlCuS, and AlCuS2. Trans. Faraday Soc. 1971, 67, 1293–1301. [Google Scholar] [CrossRef]
- Kenzhaliyev, B.K.; Trebukhov, S.A.; Volodin, V.N.; Trebukhov, A.A.; Tuleutay, F.H. Selenium extraction out of metallurgical production middlings. Kompleks. Ispolz. Miner. Syra 2018, 307, 56–64. [Google Scholar] [CrossRef]
- Kenzhaliyev, B.K. Innovative technologies providing enhancement of non-ferrous, precious, rare and rare earth metals extraction. Kompleks. Ispolz. Miner. Syra 2019, 310, 64–75. [Google Scholar] [CrossRef]
- Volodin, V.N. Physical Chemistry and Technology of Cadmium Refining; Print-S: Almaty, Kazakhstan, 2011; 238p. [Google Scholar]
- Chizhikov, D.M.; Sevryukov, N.N. Continuous zinc refining by rectification. Proc. Acad. Sci. USSR 1941, 9, 89–97. [Google Scholar]
- Tsvetkov, Y.V.; Edelshtein, V.M. On the effect of pressure on the activity of components in boiling cadmium-zinc alloys. J. Appl. Chem. 1962, 35, 1927–1933. [Google Scholar]
- Volodin, V.N.; Tuleushev, Y.Z. The liquid–vapor phase transition in a copper–calcium system. Russ. J. Phys. Chem. A 2020, 94, 1300–1305. [Google Scholar] [CrossRef]

| Alloy Numbers | Alloy Composition, wt. % | Alloy Composition, at. % | ||
|---|---|---|---|---|
| Se | Al | Se | Al | |
| 1 | 36.13 | 63.87 | 16.20 | 83.80 |
| 2 | 56.58 | 43.42 | 30.81 | 69.19 |
| 3 | 69.95 | 30.15 | 44.18 | 55.82 |
| 4 | 81.45 | 18.55 | 60.00 | 40.00 |
| 5 | 84.66 | 15.34 | 65.35 | 34.65 |
| 6 | 88.17 | 11.83 | 71.80 | 28.20 |
| 7 | 93.98 | 6.02 | 84.21 | 15.79 |
| 8 | 97.23 | 2.77 | 92.31 | 7.69 |
| 9 | 99.07 | 0.93 | 97.33 | 2.67 |
| (Se), at. % | T, K | , kPa | Calculation, kPa | = B − A · T−1 | |Δ|, % | ||
|---|---|---|---|---|---|---|---|
| Experiment | Calculation | B | A | ||||
| 16.20 | 1473 | 1.20 | 1.28 | 9.12 × 10−4 | 24.455 | 25,480 | 6.47 |
| 1.20 | 6.47 | ||||||
| 1.47 | 14.58 | ||||||
| 1523 | 2.13 | 2.26 | 1.99 × 10−3 | 5.92 | |||
| 2.27 | 0.27 | ||||||
| 2.40 | 6.01 | ||||||
| 30.81 | 1423 | 1.13 | 1.12 | 2.70 × 10−4 | 25.730 | 26,627 | 1.16 |
| 1.47 | 31.60 | ||||||
| 0.84 | 24.80 | ||||||
| 1523 | 3.60 | 3.82 | 1.32 × 10−3 | 5.64 | |||
| 3.86 | 1.18 | ||||||
| 4.00 | 4.85 | ||||||
| 44.18 | 1423 | 1.33 | 1.44 | 1.90 × 10−4 | 26.505 | 27,365 | 7.83 |
| 1.33 | 7.83 | ||||||
| 1.69 | 17.12 | ||||||
| 1523 | 5.06 | 5.10 | 8.71 × 10−4 | 0.78 | |||
| 4.80 | 5.88 | ||||||
| 5.47 | 7.25 | ||||||
| 60.00 | 1373 | 0.93 | 0.89 | – | 27.209 | 29,069 | 4.83 |
| 1423 | 1.33 | 1.82 | – | 26.92 | |||
| 2.00 | 9.65 | ||||||
| 1.87 | 2.30 | ||||||
| 1473 | 4.13 | 3.56 | – | 16.06 | |||
| 4.27 | 19.80 | ||||||
| 3.33 | 6.40 | ||||||
| 1523 | 5.60 | 6.65 | – | 15.82 | |||
| 6.67 | 0.23 | ||||||
| 7.07 | 6.24 | ||||||
| |Δ|average = 9.42 | |||||||
| (Se), at. % | T, K | , kPa | Calculation, kPa | = B − A · T−1 | |Δ|, % | ||
|---|---|---|---|---|---|---|---|
| Experiment | Calculation | B | A | ||||
| 65.35 | 773 | 3.07 | 3.11 | 6.85 × 10−8 | 21.811 | 10,642 | 1.29 |
| 3.33 | 7.07 | ||||||
| 2.96 | 4.82 | ||||||
| 923 | 29.03 | 29.18 | 3.09 × 10−5 | 0.51 | |||
| 28.93 | 0.86 | ||||||
| 28.66 | 1.78 | ||||||
| 71.80 | 773 | 3.06 | 3.15 | 6.85 × 10−8 | 21.750 | 10,587 | 2.86 |
| 3.47 | 10.16 | ||||||
| 22.93 | 6.98 | ||||||
| 923 | 27.06 | 29.14 | 3.09 × 10−5 | 7.14 | |||
| 28.53 | 2.09 | ||||||
| 32.01 | 9.85 | ||||||
| 84.21 | 773 | 2.93 | 3.10 | 6.85 × 10−8 | 22.887 | 11,478 | 5.48 |
| 3.47 | 11.94 | ||||||
| 2.93 | 5.48 | ||||||
| 923 | 32.66 | 34.60 | 3.36 × 10−5 | 5.60 | |||
| 36.66 | 5.98 | ||||||
| 34.66 | 0.17 | ||||||
| 92.31 | 773 | 3.07 | 3.06 | 1.18 × 10−7 | 24.408 | 12,664 | 0.33 |
| 3.33 | 8.11 | ||||||
| 2.80 | 8.50 | ||||||
| 923 | 42.00 | 43.80 | 1.78 × 10−5 | 4.11 | |||
| 43.60 | 0.46 | ||||||
| 46.00 | 5.02 | ||||||
| 97.33 | 773 | 3.33 | 3.76 | 1.30 × 10−7 | 25.079 | 13,022 | 11.44 |
| 3.87 | 2.93 | ||||||
| 4.13 | 9.84 | ||||||
| 923 | 58.66 | 58.14 | 1.91 × 10−5 | 0.89 | |||
| 57.46 | 1.17 | ||||||
| 58.26 | 0.21 | ||||||
| |Δ| average = 4.78 | |||||||
| Alloy Composition, at. % | , kJ/mol | , kJ/mol | , kJ/mol | , kJ/mol | |
|---|---|---|---|---|---|
| Se | Al | ||||
| 0 | 100 | – | – | 0 | 0 |
| 10 | 90 | – | 25.79 ± 2.64 | 0.83 ± 0.09 | 5.23 ± 0.54 |
| 20 | 80 | – | 18.49 ± 1.90 | 3.43 ± 0.35 | 8.44 ± 0.87 |
| 30 | 70 | – | 11.96 ± 1.23 | 8.11 ± 0.83 | 10.04 ± 1.03 |
| 40 | 60 | – | 6.67 ± 0.68 | 15.55 ± 1.59 | 9.65 ± 0.99 |
| 50 | 50 | – | 2.73 ± 0.28 | 27.79 ± 2.85 | 6.91 ± 0.71 |
| 60 | 40 | – | 0 | – | 0 |
| 70 | 30 | 15.80 ± 1.62 | – | – | – |
| 80 | 20 | 15.75 ± 1.61 | – | – | – |
| 90 | 10 | 3.44 ± 0.35 | 10.05 ± 1.03 | – | 5.09 ± 0.52 |
| 100 | 0 | 0 | – | – | 0 |
| Alloy Composition, at. % | , J/(mol × K) | , J/(mol × K) | , J/(mol × K) | , J/(mol × K) | |
|---|---|---|---|---|---|
| Se | Al | ||||
| 0 | 100 | – | – | 0 | 0 |
| 10 | 90 | – | 29.06 ± 2.98 | 1.99 ± 0.20 | 6.77 ± 0.69 |
| 20 | 80 | – | 19.64 ± 2.01 | 5.30 ± 0.54 | 10.08 ± 1.03 |
| 30 | 70 | – | 12.57 ± 1.29 | 10.35 ± 1.08 | 11.47 ± 1.18 |
| 40 | 60 | – | 7.19 ± 0.74 | 17.94 ± 1.84 | 10.77 ± 1.10 |
| 50 | 50 | – | 3.09 ± 0.32 | 30.65 ± 3.14 | 7.69 ± 0.79 |
| 60 | 40 | – | 0 | – | 0 |
| 70 | 30 | 24.74 | – | – | – |
| 80 | 20 | 24.74 | – | – | – |
| 90 | 10 | 8.70 | 13.28 | – | 9.85 |
| 100 | 0 | 0 | – | – | 0 |
| Alloy Composition, at. % | , J/(mol × K) | , J/(mol × K) | , J/(mol × K) | , J/(mol × K) | |
|---|---|---|---|---|---|
| Se | Al | ||||
| 0 | 100 | – | – | 105.15 ± 10.78 | 105.15 ± 10.78 |
| 10 | 90 | – | 100.64 ± 10.32 | 103.16 ± 10.57 | 102.74 ± 10.53 |
| 20 | 80 | – | 110.75 ± 11.35 | 99.85 ± 10.23 | 103.48 ± 10.61 |
| 30 | 70 | – | 117.82 ± 12.08 | 94.80 ± 9.72 | 106.31 ± 10.90 |
| 40 | 60 | – | 123.20 ± 12.63 | 87.21 ± 8.94 | 111.21 ± 11.40 |
| 50 | 50 | – | 127.31 ± 13.05 | 74.50 ± 7.64 | 118.49 ± 12.14 |
| 60 | 40 | – | 130.39 ± 13.36 | – | 130.39 ± 13.36 |
| 70 | 30 | 94.96 ± 6.08 | – | – | – |
| 80 | 20 | 104.85 ± 6.71 | – | – | – |
| 90 | 10 | 107.95 ± 6.91 | 117.11 ± 7.50 | – | 100.50 ± 6.43 |
| 100 | 0 | 110.34 ± 7.06 | – | – | 110.34 ± 7.06 |
| Alloy Composition, at. % | , kJ/mol | , kJ/mol | , kJ/mol | , kJ/mol | |
|---|---|---|---|---|---|
| Se | Al | ||||
| 0 | 100 | – | – | 293.69 ± 30.10 | 293.69 ± 30.10 |
| 10 | 90 | – | 206.84 ± 21.20 | 292.86 ± 30.02 | 278.49 ± 28.55 |
| 20 | 80 | – | 214.59 ± 22.00 | 290.27 ± 29.75 | 265.07 ± 27.17 |
| 30 | 70 | – | 221.12 ± 22.66 | 285.58 ± 19.27 | 253.35 ± 25.97 |
| 40 | 60 | – | 226.38 ± 23.20 | 278.14 ± 28.51 | 243.62 ± 24.97 |
| 50 | 50 | – | 230.35 ± 23.61 | 265.91 ± 27.26 | 236.29 ± 24.22 |
| 60 | 40 | – | 233.08 ± 23.89 | – | 233.08 ± 23.89 |
| 70 | 30 | 85.60 ± 5.48 | – | – | – |
| 80 | 20 | 85.60 ± 5.48 | – | – | – |
| 90 | 10 | 94.96 ± 6.08 | 117.11 ± 7.50 | – | 100.50 ± 6.43 |
| 100 | 0 | 110.34 ± 7.06 | – | – | 110.34 ± 7.06 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nitsenko, A.; Volodin, V.; Linnik, X.; Burabayeva, N.; Trebukhov, S. Melt–Vapor Phase Transition in the Aluminum–Selenium System in Vacuum. Metals 2023, 13, 1297. https://doi.org/10.3390/met13071297
Nitsenko A, Volodin V, Linnik X, Burabayeva N, Trebukhov S. Melt–Vapor Phase Transition in the Aluminum–Selenium System in Vacuum. Metals. 2023; 13(7):1297. https://doi.org/10.3390/met13071297
Chicago/Turabian StyleNitsenko, Alina, Valeriy Volodin, Xeniya Linnik, Nurila Burabayeva, and Sergey Trebukhov. 2023. "Melt–Vapor Phase Transition in the Aluminum–Selenium System in Vacuum" Metals 13, no. 7: 1297. https://doi.org/10.3390/met13071297
APA StyleNitsenko, A., Volodin, V., Linnik, X., Burabayeva, N., & Trebukhov, S. (2023). Melt–Vapor Phase Transition in the Aluminum–Selenium System in Vacuum. Metals, 13(7), 1297. https://doi.org/10.3390/met13071297






