Study on Mechanical Properties and Deformation Mechanism of Fe-28Mn-10Al-C High-Strength Steel during Dynamic Deformation Process
Abstract
:1. Introduction
2. Materials and Methods
2.1. Annealed Test Steel
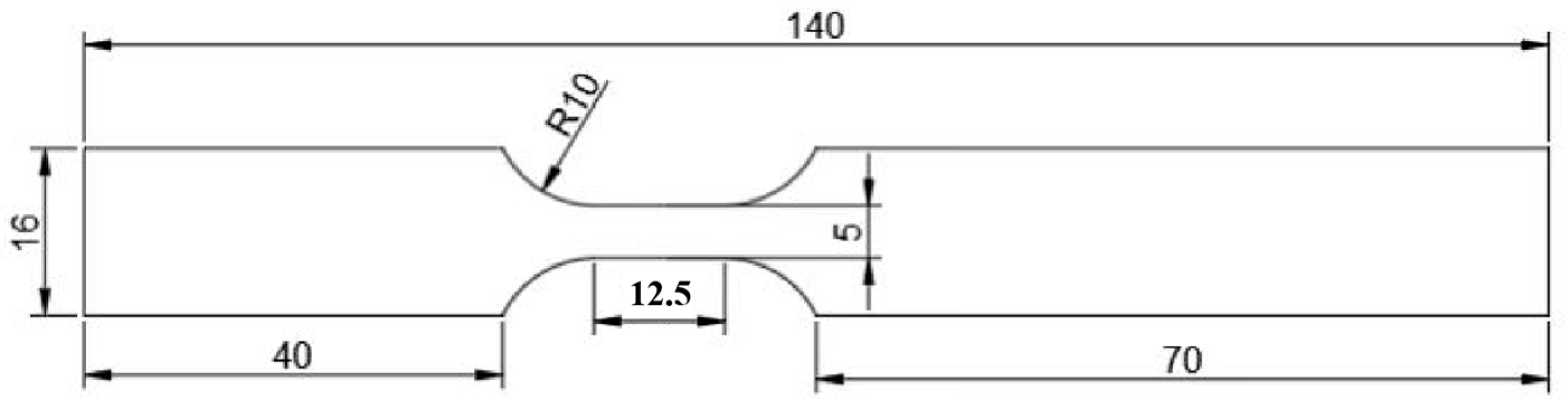
2.2. Tensile Specimen Treatment
3. Results
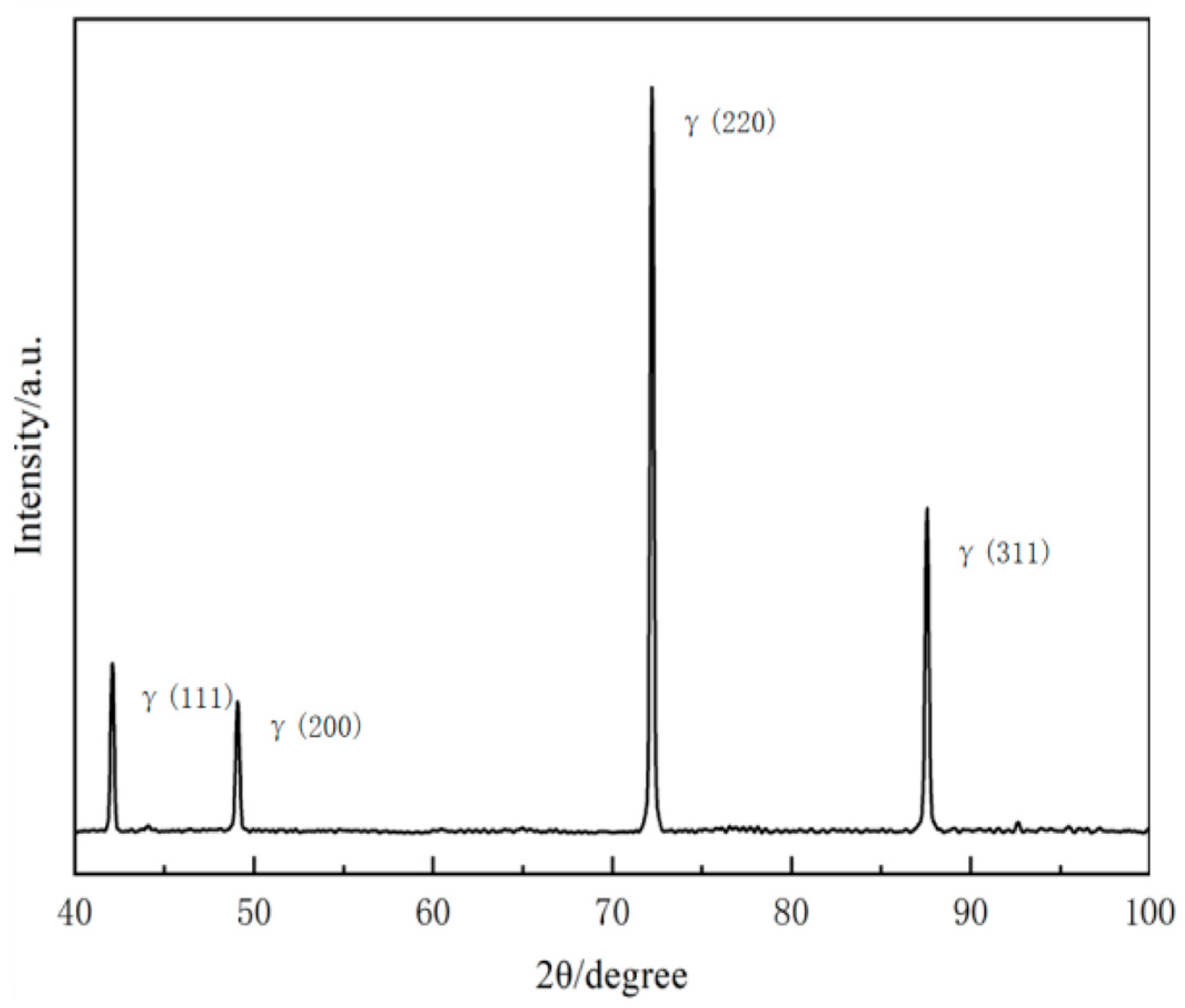
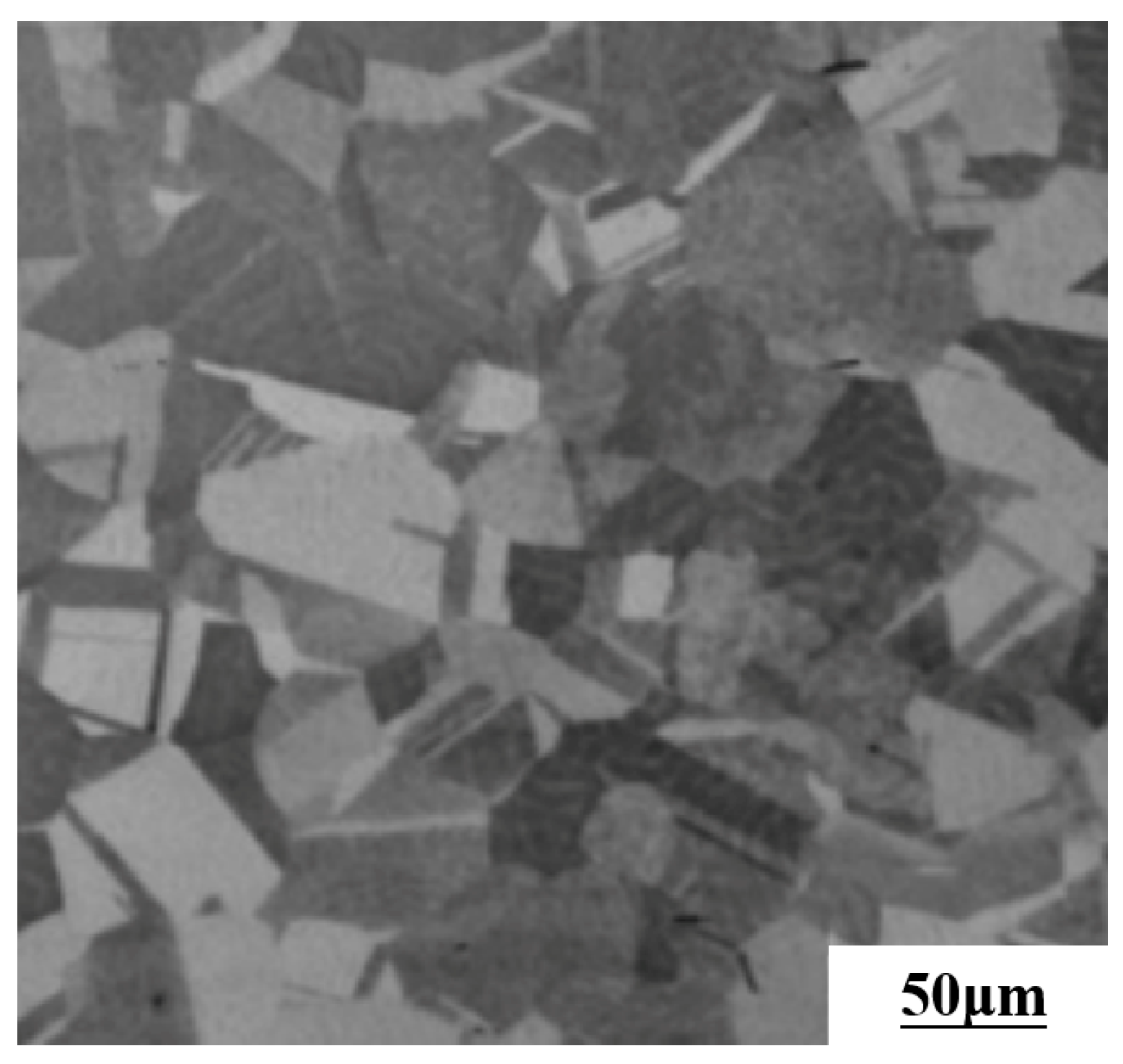
3.1. Metallographic Structure
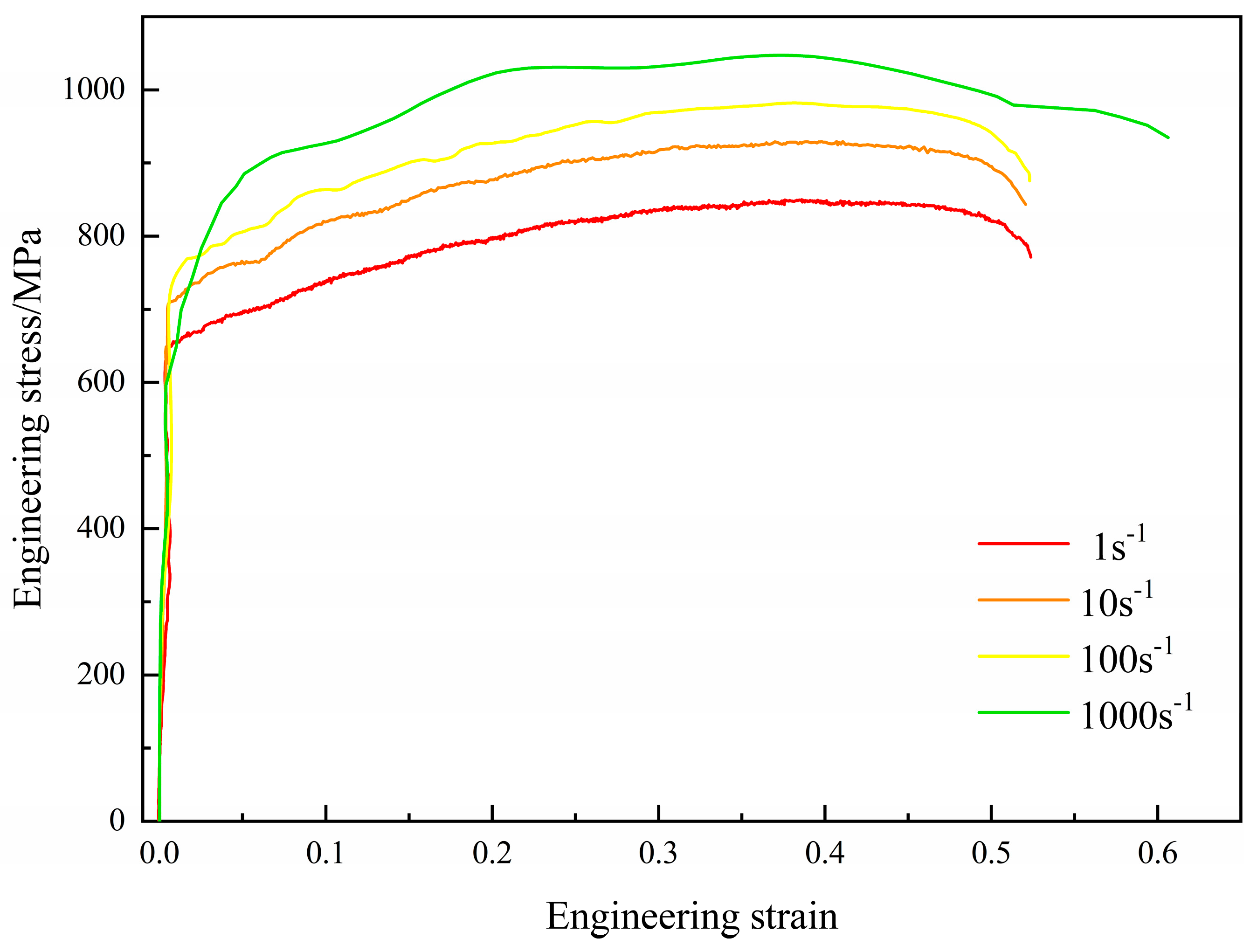
3.2. Analysis of Mechanical Properties
4. Discussion
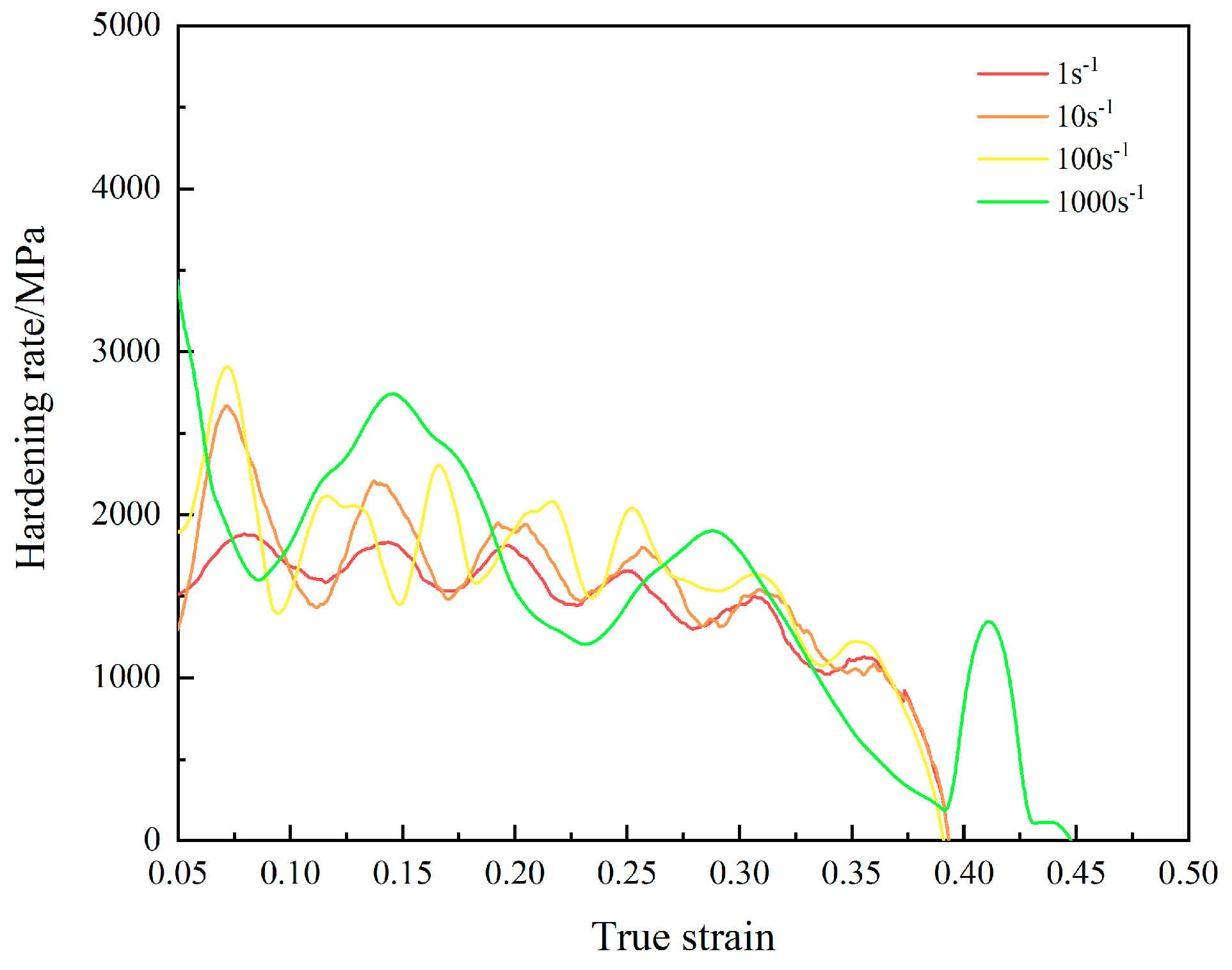
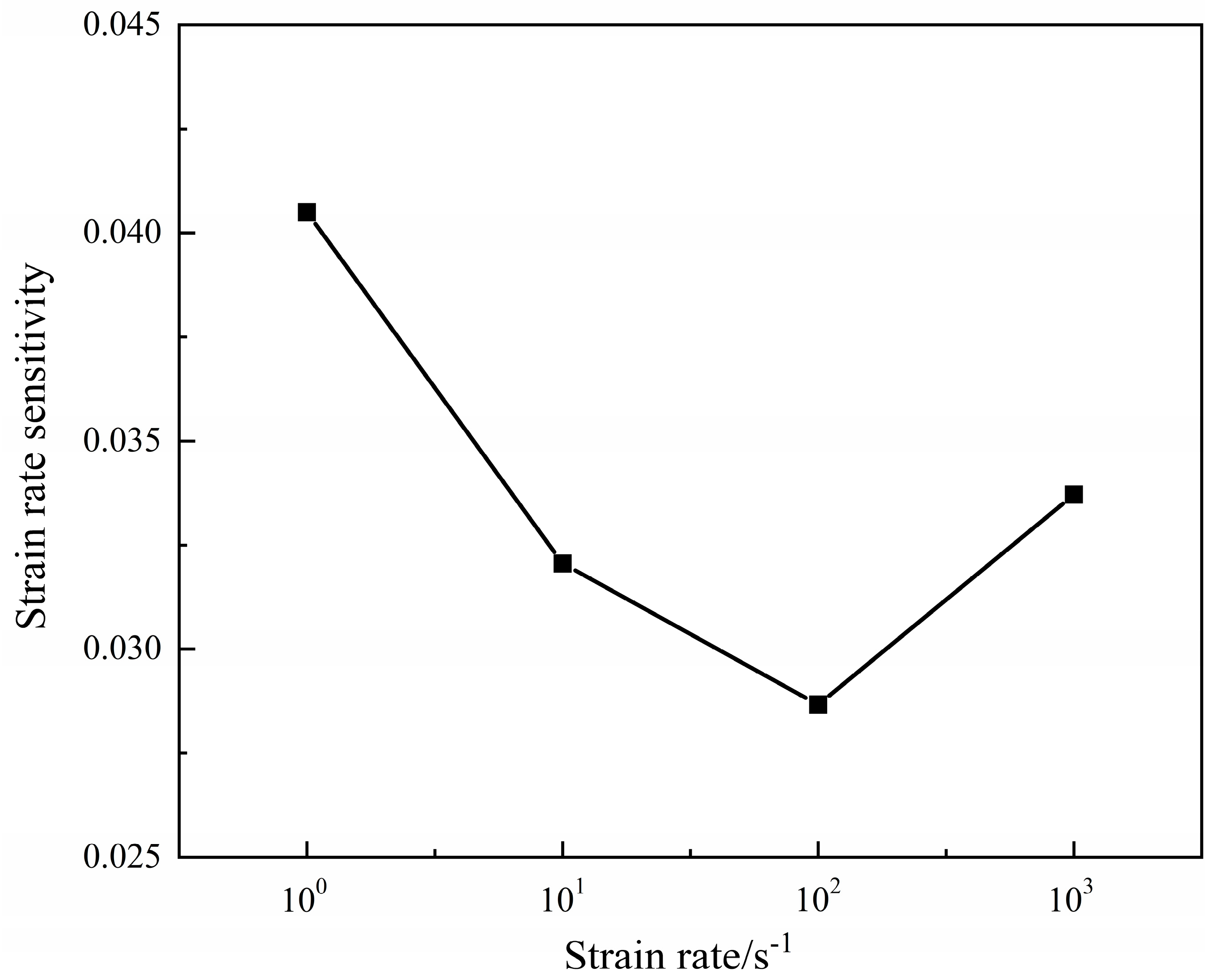
4.1. Analysis of Dynamic Properties
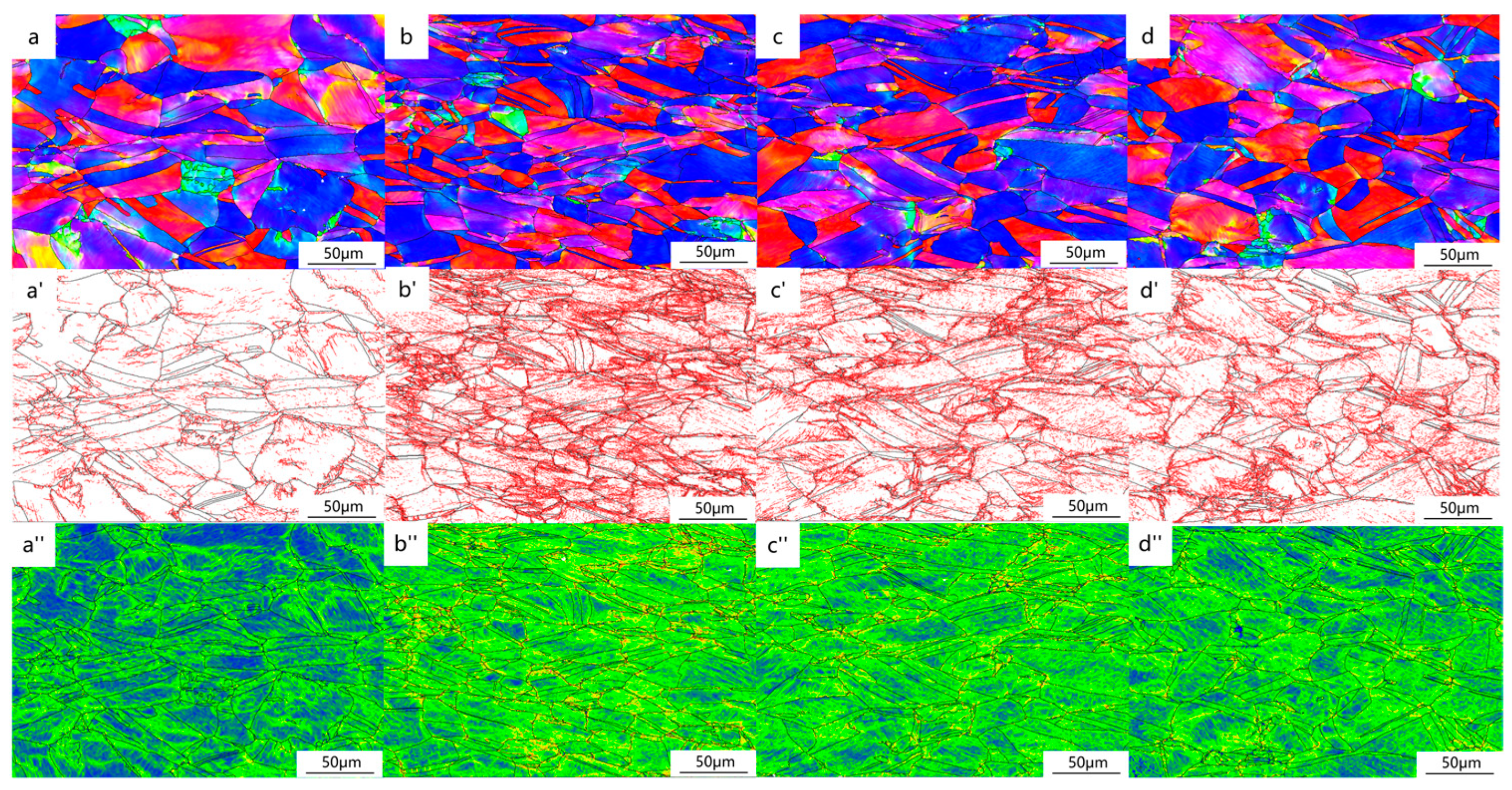
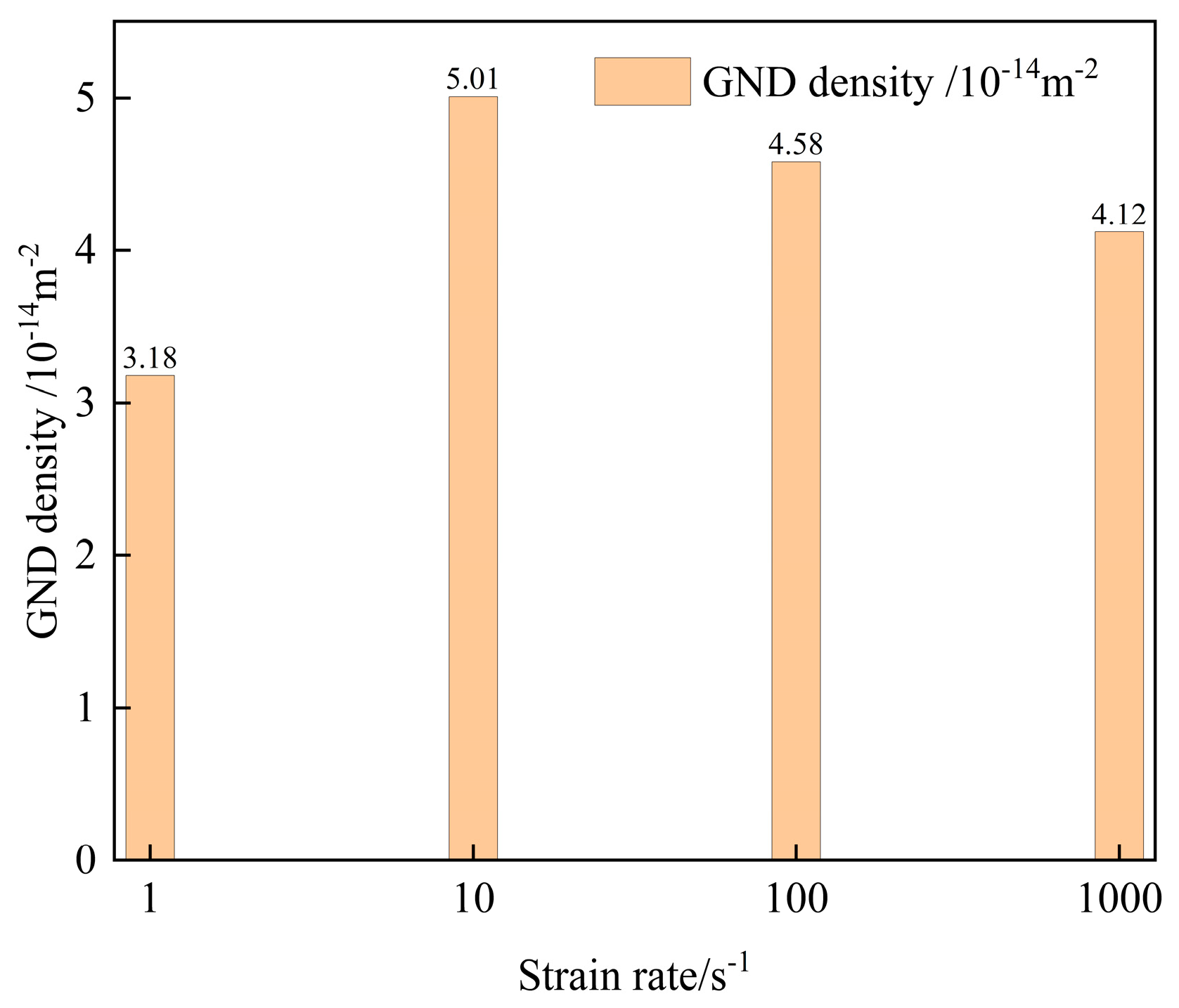
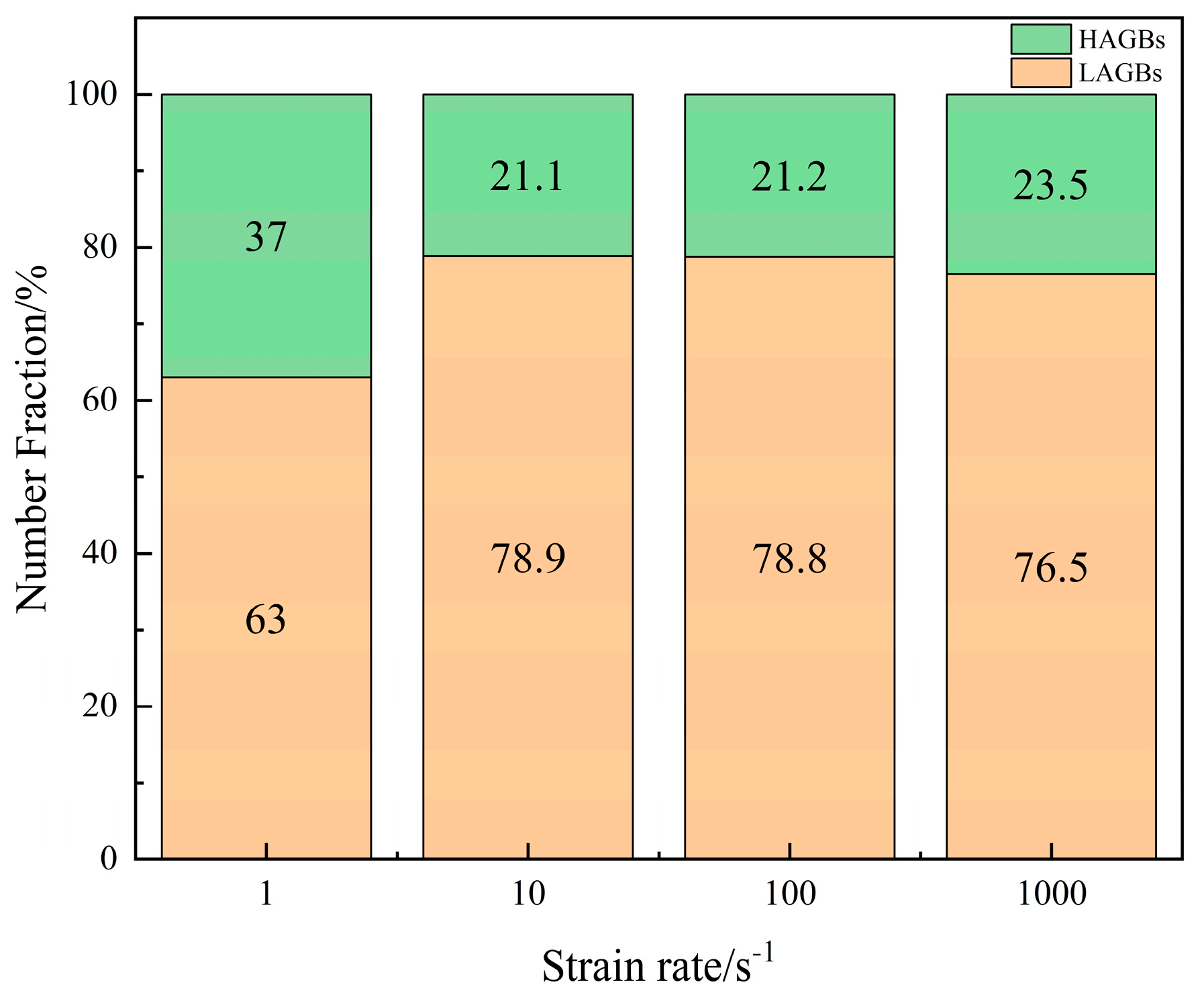
4.2. EBSD Analysis of Test Steel
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chen, P.; Li, X.; Yi, H. The κ-carbides in low-density Fe-Mn-Al-C steels: A review on their structure, precipitation and deformation mechanism. Metals 2020, 10, 1021. [Google Scholar] [CrossRef]
- Wang, Z.G.; Shen, C.L.; Zhang, J.L.; Song, C.J.; Zhai, Q.J. An accelerated aging assisted by electric current in a Fe-Mn-Al-C low-density steel. China Foundry 2022, 19, 395–402. [Google Scholar] [CrossRef]
- Ren, J.K.; Zhang, L.; Li, Z.H.; Chen, J.; Zhang, W.N.; Wu, S.W.; Liu, Z.Y. Fe-Mn-Al-C high-entropy steels with superior mechanical properties at 4.2 K. Mater. Des. 2023, 228, 111840. [Google Scholar] [CrossRef]
- Picak, S.; Vaughan, M.; El Atwani, O.; Mott, A.; Limmer, K.; Karaman, I. Effects of chemical segregation on ductility-anisotropy in high strength Fe-Mn-Al-C lightweight austenitic steels. Acta Mater. 2023, 245, 118589. [Google Scholar] [CrossRef]
- Ding, H.; Liu, D.; Cai, M.; Zhang, Y. Austenite-Based Fe-Mn-Al-C Lightweight Steels: Research and Prospective. Metals 2022, 12, 1572. [Google Scholar] [CrossRef]
- Riaz, T.; Das, S.R.; Sahu, P. Influence of Al Variation on the Mechanical Properties and Critical Shear Stress of Twinning in Fe-Mn-Al-C Steels. J. Mater. Eng. Perform. 2023, 32, 1636–1644. [Google Scholar] [CrossRef]
- Shyamal, S.; Das, S.R.; Jaskari, M.; Sahu, P. Evolution of deformation microstructure in a coarse-grained Fe-Mn-Al-C steel under bending fatigue. Mater. Lett. 2022, 327, 133006. [Google Scholar] [CrossRef]
- Zhang, X.-F.; Li, J.-X.; Yang, Y.; Zhang, K.; Wu, X.-J.; Huang, Z.-Y. Austenite Stability and Cryogenic Impact Toughness of a Lamellar Fe-Mn-Al-C Lightweight Structural Steel Subjected to Quenching and Tempering Process. J. Mater. Eng. Perform. 2022, 31, 5259–5268. [Google Scholar] [CrossRef]
- Li, Z.; Wang, Y.C.; Cheng, X.; Gao, C.; Li, Z.; Langdon, T.G. Microstructure and mechanical properties of an Fe–Mn–Al–C lightweight steel after dynamic plastic deformation processing and subsequent aging. Mater. Sci. Eng. A 2022, 833, 142566. [Google Scholar] [CrossRef]
- Jang, G.; Kim, J.N.; Lee, H.; Lee, T.; Enikeev, N.; Abramova, M.; Valiev, R.Z.; Kim, H.S.; Lee, C.S. Microstructural evolution and mechanical properties of nanocrystalline Fe–Mn–Al–C steel processed by high-pressure torsion. Mater. Sci. Eng. A 2021, 827, 142073. [Google Scholar] [CrossRef]
- Durán, J.F.; Pérez, G.A.; Rodríguez, J.S.; Aguilar, Y.; Logé, R.E.; Zambrano, O.A. Microstructural Evolution Study of Fe–Mn–Al–C Steels Through Variable Thermomechanical Treatments. Metall. Mater. Trans. A 2021, 52, 4785–4799. [Google Scholar] [CrossRef]
- Li, Z.; Wang, Y.; Cheng, X.; Li, Z.; Gao, C.; Li, S. The effect of rolling and subsequent aging on microstructures and tensile properties of a Fe–Mn–Al–C austenitic steel. Mater. Sci. Eng. A 2021, 822, 141683. [Google Scholar] [CrossRef]
- Ley, N.A.; Young, M.L.; Hornbuckle, B.C.; Field, D.M.; Limmer, K.R. Toughness enhancing mechanisms in age hardened Fe–Mn–Al–C steels. Mater. Sci. Eng. A 2021, 820, 141518. [Google Scholar] [CrossRef]
- Xing, M.; Lin, F.; Tang, L.; Wu, X.; Zhang, X.; Huang, Z. A review of the influence of Al element on Fe-Mn-Al-C low density steel. China Metall. 2022, 32, 15–26. (In Chinese) [Google Scholar]
- Ren, P. Deformation Mechanism and Micro-Structure Property Control of Fe-Mn-Al-C Austenitic Low Density Steel. Ph.D. Thesis, Chongqing University, Chongqing, China, 2021. (In Chinese). [Google Scholar]
- Sun, J.; Huang, Z.; Li, J.; Wang, P. Thermal deformation behavior of Fe-28 Mn-9Al-1C lightweight alloy steel. Ansactions Mater. Heat Treat. 2022, 43, 142–150. (In Chinese) [Google Scholar]
- Rahman, K.M.; Vorontsov, V.A.; Dye, D. The dynamic behaviour of a twinning induced plasticity steel. Mater. Sci. Eng. A 2014, 589, 252–261. [Google Scholar] [CrossRef]
- Tang, Z.Y.; Huang, J.N.; Ding, H.; Cai, Z.H.; Misra, R.D.K. On the dynamic behavior and relationship to mechanical properties of cold-rolled Fe-0.2C-15Mn-3Al steel at intermediate strain rate. Mater. Sci. Eng. A 2019, 742, 423–431. [Google Scholar] [CrossRef]
- Park, J.; Kang, M.; Sohn, S.S.; Kim, S.H.; Kim, H.S.; Kim, N.J.; Lee, S. Quasi-static and dynamic deformation mechanisms interpreted by microstructural evolution in TWinning Induced Plasticity (TWIP) steel. Mater. Sci. Eng. A 2017, 684, 54–63. [Google Scholar] [CrossRef]
- Yoo, J.D.; Park, K.T. Microband-induced plasticity in a high Mn-Al-C light steel. Mater. Sci. Eng. A 2008, 496, 417–424. [Google Scholar] [CrossRef]
- Hou, M.; Li, C.; Kong, W.; Bai, Y. Effect of Heat treatment on micro-structure and properties of Fe-Mn-Al-C steel. Spec. Steel 2023, 44, 96–100. (In Chinese) [Google Scholar]
- Wu, Z.; Zhao, J.; Zhou, N.; Luo, X. Effect of quench-tempering heat treatment on micro-structure and mechanical properties of ultra-high strength Fe-Mn-Al-C alloy steel. Angang Technol. 2023, 439, 23–27+62. (In Chinese) [Google Scholar]
- Li, X. Effect of Heat Treatment on Micro-Structure and Mechanical Properties of Rolled Fe-Mn-Al-C Steel. Master’s Thesis, North University of China, Taiyuan, China, 2022. (In Chinese). [Google Scholar]
- Meng, J.; Liu, R.; Guo, J.; Xu, R.; Wang, K.; Jin, X. Study on the effect of heat treatment temperature on the precipitation of aluminum series carbides in Fe-Mn-Al-C light steel. In The Chinese Society for Metals, Proceedings of the 13th China Steel Annual Conference-7. Advanced Steel Materials and Their Applications, Online, 23–24 November 2022; Metallurgical Industry Press: Beijing, China, 2022; Volume 6. (In Chinese) [Google Scholar]
- Hao, Y. Effect of Manganese Content and Heat Treatment on Micro-Structure and Mechanical Properties of Fe-Mn-Al-C Steel. Master’s Thesis, Xiangtan University, Xiangtan, China, 2020. (In Chinese). [Google Scholar]
- Yang, F.; Song, B.; Li, Y.; Sun, T.; Wang, K.; Kang, T. Effect of annealing temperature on properties of cold-rolled Fe-Mn-Al-C low-density steel. Chin. J. Mater. Res. 2015, 29, 108–114. (In Chinese) [Google Scholar]
- Mi, Z.L.; Tang, D.; Jiang, H.T.; Dai, Y.J.; Li, S.S. Effects of annealing temperature on the micro-structure and properties of the 25Mn-3Si-3Al TWIP steel. Int. J. Miner. Metall. Mater. 2009, 16, 154–158. [Google Scholar] [CrossRef]
- Seo, E.J.; Cho, L.; Estrin, Y.; De Cooman, B.C. Micro-structure-mechanical properties relationships for quenching and partitioning(Q&P) processed steel. Acta Mater. 2016, 113, 124–139. [Google Scholar]
- Sohn, S.S.; Lee, B.J.; Lee, S.; Kim, N.J.; Kwak, J.H. Effect of annealing temperature on microstructural modification and tensile properties in 0.35C-3.5Mn-5.8Al lightweight steel. Acta Mater. 2013, 61, 5050–5066. [Google Scholar] [CrossRef]
- Li, H.; Xia, P.; Xie, K.; Cao, M.; Tan, Y.; Jin, X. Effect of annealing temperature on micro-structure and tensile properties of Fe-Mn-Al-C steel. Heat Treat. Met. 2021, 46, 77–82. (In Chinese) [Google Scholar]
- Das, A.; Tarafder, S.; Sivaprasad, S.; Chakrabarti, D. Influence of microstructure and strain rate on the strain partitioning behaviour of dual phase steels. Mater. Sci. Eng. A 2019, 754, 348–360. [Google Scholar] [CrossRef]
- Daochun, H.; Minghe, C. Tensile Properties and Deformational Mechanism of C5191 Phosphor. Rare Met. Mater. Eng. 2017, 46, 1518–1523. [Google Scholar] [CrossRef]
- Tamuly, S.; Dixit, S.; Kombaiah, B.; Parameswaran, V.; Khanikar, P. High strain rate deformation behavior of Al0.65CoCrFe2Ni dual-phase high entropy alloy. Intermetallics 2023, 161, 107983. [Google Scholar] [CrossRef]
- El-Aty, A.A.; Xu, Y.; Zhang, S.-H.; Ha, S.; Ma, Y.; Chen, D. Impact of high strain rate deformation on the mechanical behavior, fracture mechanisms and anisotropic response of 2060 Al-Cu-Li alloy. J. Adv. Res. 2019, 18, 19–37. [Google Scholar] [CrossRef]
- Abd El-Aty, A.; Xu, Y.; Guo, X.; Zhang, S.H.; Ma, Y.; Chen, D. Strengthening mechanisms, deformation behavior, and anisotropic mechanical properties of Al-Li alloys: A review. J. Adv. Res. 2017, 10, 49–67. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Zhao, S.; Yang, Y. Discussion on automobile lightweight and application of aluminum alloy. Environ. Technol. 2021, 1, 62–66. (In Chinese) [Google Scholar]
- DIN EN ISO 26203-2-2011; Metallic Materials-Tensile Testing at High Strain Rates-Part 2: Servo-Hydraulic and Other Test Systems. German Institute for Standardization: Berlin, Germany, 2011.
- Coronado, J.J. Stacking Fault Energy Determination in Fe-Mn-Al-C Austenitic Steels by X-ray Diffraction. Metals 2021, 11, 1701. [Google Scholar]
- Zou, D.Q.; Li, S.H.; He, J. Temperature and strain rate dependent deformation induced martensitic transformation and flow behavior of quenching and partitioning steels. Mater. Sci. Eng. A 2016, 680, 54–63. [Google Scholar] [CrossRef]
- Curtze, S.; Kuokkala, V.-T. Dependence of tensile deformation behavior of TWIP steels on stacking fault energy, temperature and strain rate. Acta Mater. 2010, 58, 5129–5141. [Google Scholar] [CrossRef]
- Shen, Y.F.; Jia, N.; Misra, R.D.K.; Zuo, L. Softening behavior by excessive twinning and adiabatic heating at high strain rate in a Fe-20Mn-0.6C TWIP steel. Acta Mater. 2016, 103, 229–242. [Google Scholar] [CrossRef]
- Dong, Y.; Liu, Y.; Wang, L.; Su, L. Effect of strain rate on dynamic tensile deformation behavior of DP780 steel. Acta Metall. Sin. 2013, 49, 159–166. (In Chinese) [Google Scholar] [CrossRef]
- Meyers, M.A.; Zhang, Q.M.; Liu, Y.; Huang, F.L.; Lu, Z.J. Dynamic Behavior of Materials; National Defense Industry Press: Beijing, China, 2006; Volume 225. [Google Scholar]
- Kapoor, R.; Nemat-Nasser, S. Comparison between high and low strain-rate deformation of tantalum. Metall. Mater. Trans. A 2000, 31, 815–823. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, L.; He, S.; Feng, F.; Lv, X.; Zhang, B. Effect of long-term aging on dynamic tensile deformation behavior of GH4169 alloy. Acta Metall. Sin. 2012, 48, 49–55. (In Chinese) [Google Scholar] [CrossRef]
- Liu, J.; Wang, Z.; Shang, J. Deformation behavior of β -Sn single crystals of [110] and [112] orientations. Acta Metall. Sin. 2008, 44, 1409–1414. (In Chinese) [Google Scholar]
- Moussa, C.; Bernacki, M.; Besnard, R.; Bozzolo, N. Statistical analysis of dislocations and dislocation boundaries from EBSD data. Ultramicroscopy 2017, 8, 63–72. [Google Scholar] [CrossRef] [PubMed]
- Shi, H.; Zong, N.; Le, J.; Li, S.; Huang, G.; Li, J.; Mao, J.; Lu, W. Strain hardening versus softening: Anisotropic response of strain hardening-softening transition in a polycrystalline zirconium alloy at room temperature from dislocation viewpoint. Mater. Sci. Eng. A 2022, 847, 143334. [Google Scholar] [CrossRef]




| Element | Mn | Al | C | Fe |
|---|---|---|---|---|
| Percentage | 28.7 | 10.2 | 1.02 | Bal. |
| Strain Rate/s−1 | YS/MPa | UTS/MPa | EL/% | PSE/GPa·% |
|---|---|---|---|---|
| 1 | 668 | 849.2 | 52.4 | 44.5 |
| 10 | 736 | 929.2 | 52.1 | 48.4 |
| 100 | 775 | 982.1 | 52.3 | 51.4 |
| 1000 | 817 | 1047.4 | 60.6 | 63.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, S.; Zhang, H.; Tang, Z.; Yu, S. Study on Mechanical Properties and Deformation Mechanism of Fe-28Mn-10Al-C High-Strength Steel during Dynamic Deformation Process. Metals 2024, 14, 47. https://doi.org/10.3390/met14010047
Cao S, Zhang H, Tang Z, Yu S. Study on Mechanical Properties and Deformation Mechanism of Fe-28Mn-10Al-C High-Strength Steel during Dynamic Deformation Process. Metals. 2024; 14(1):47. https://doi.org/10.3390/met14010047
Chicago/Turabian StyleCao, Shanji, Hanwen Zhang, Zhengyou Tang, and Shuo Yu. 2024. "Study on Mechanical Properties and Deformation Mechanism of Fe-28Mn-10Al-C High-Strength Steel during Dynamic Deformation Process" Metals 14, no. 1: 47. https://doi.org/10.3390/met14010047
APA StyleCao, S., Zhang, H., Tang, Z., & Yu, S. (2024). Study on Mechanical Properties and Deformation Mechanism of Fe-28Mn-10Al-C High-Strength Steel during Dynamic Deformation Process. Metals, 14(1), 47. https://doi.org/10.3390/met14010047





