Suppression of Inhomogeneous Plastic Deformation in Medium-Carbon Tempered Martensite Steel
Abstract
1. Introduction
- (1)
- Inhomogeneous grain size distribution
- (2)
- Multiple phases
- (3)
- Macroscopic inhomogeneous structure
- (4)
- TRIP effect
2. Experimental Methods
2.1. Approach for Suppressing the Lüders Phenomenon
2.2. Sample Preparation and Tensile Test
3. Results and Discussion
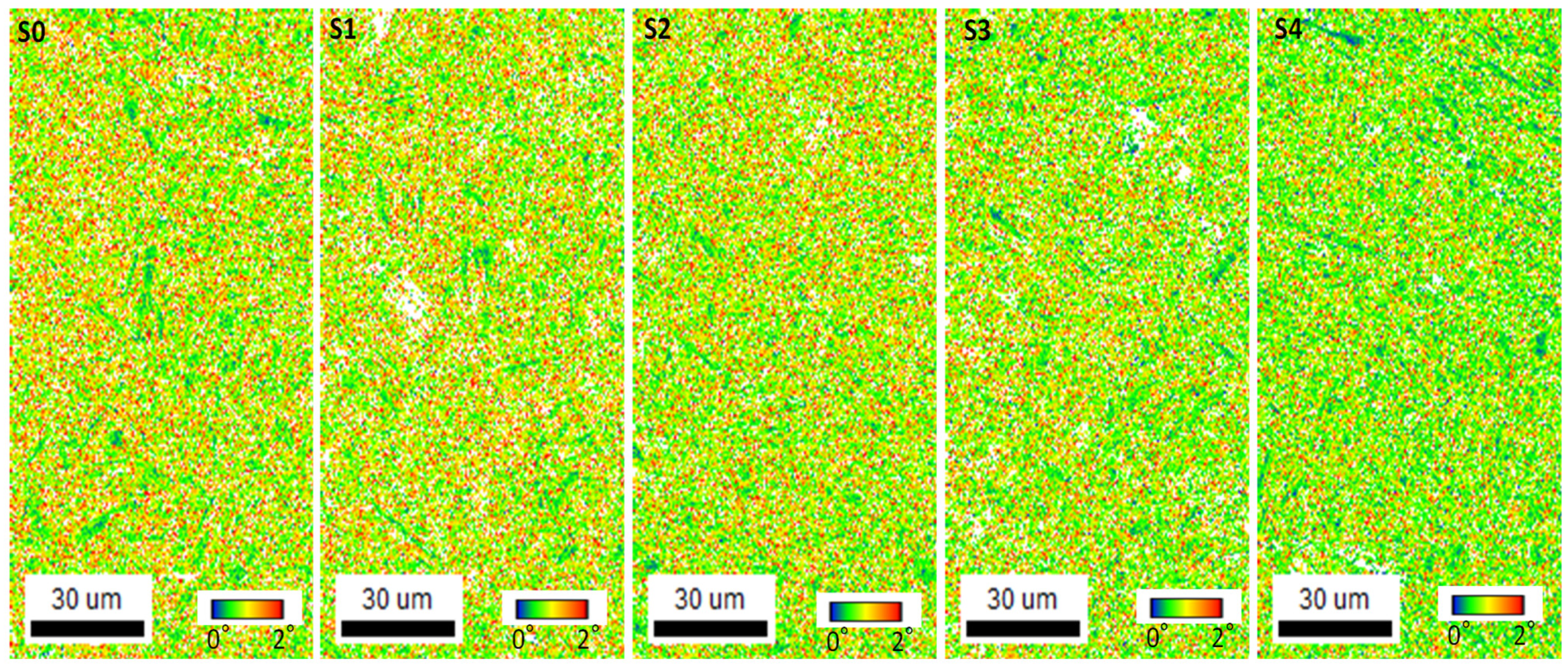
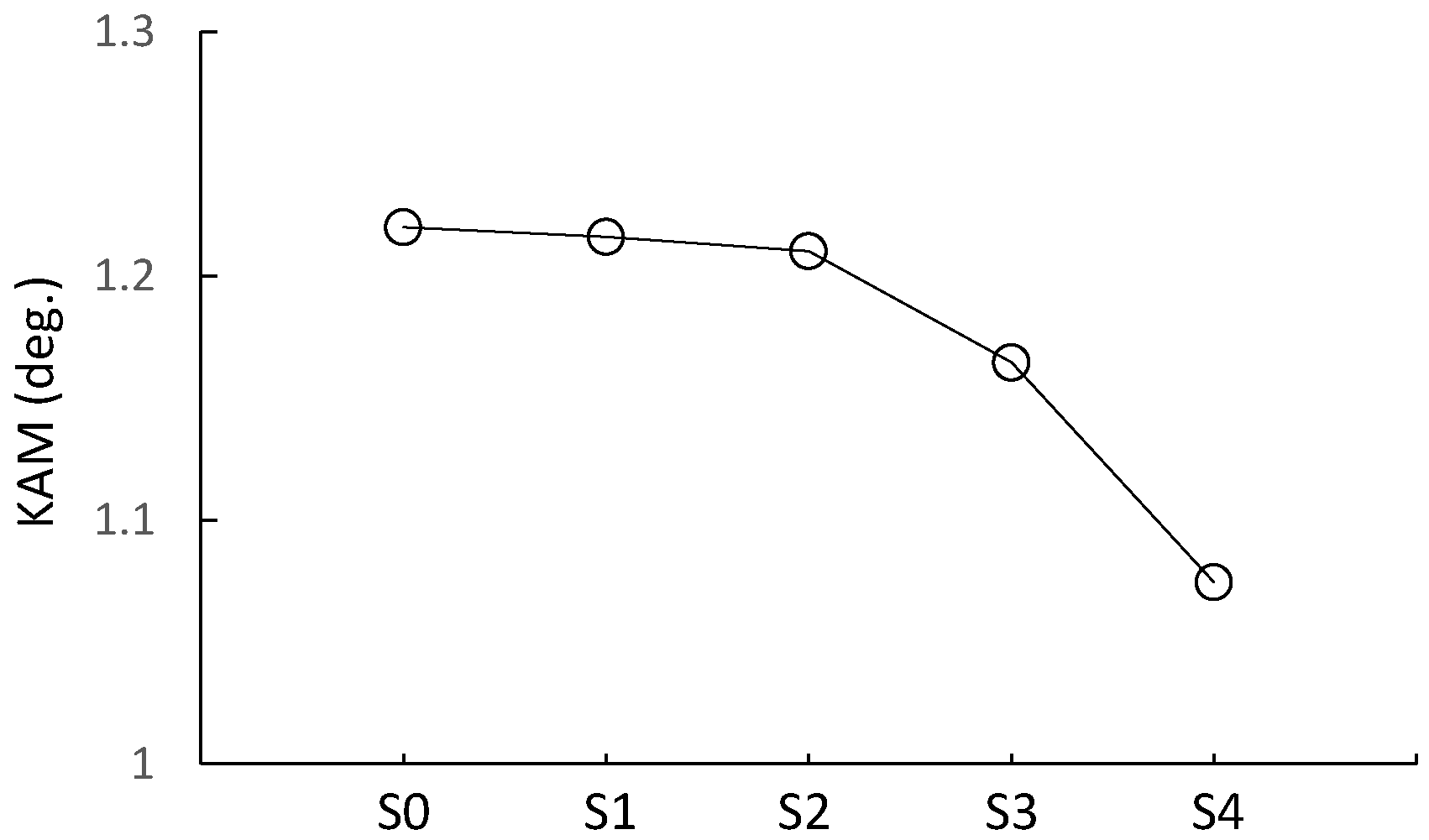
3.1. Microstructures
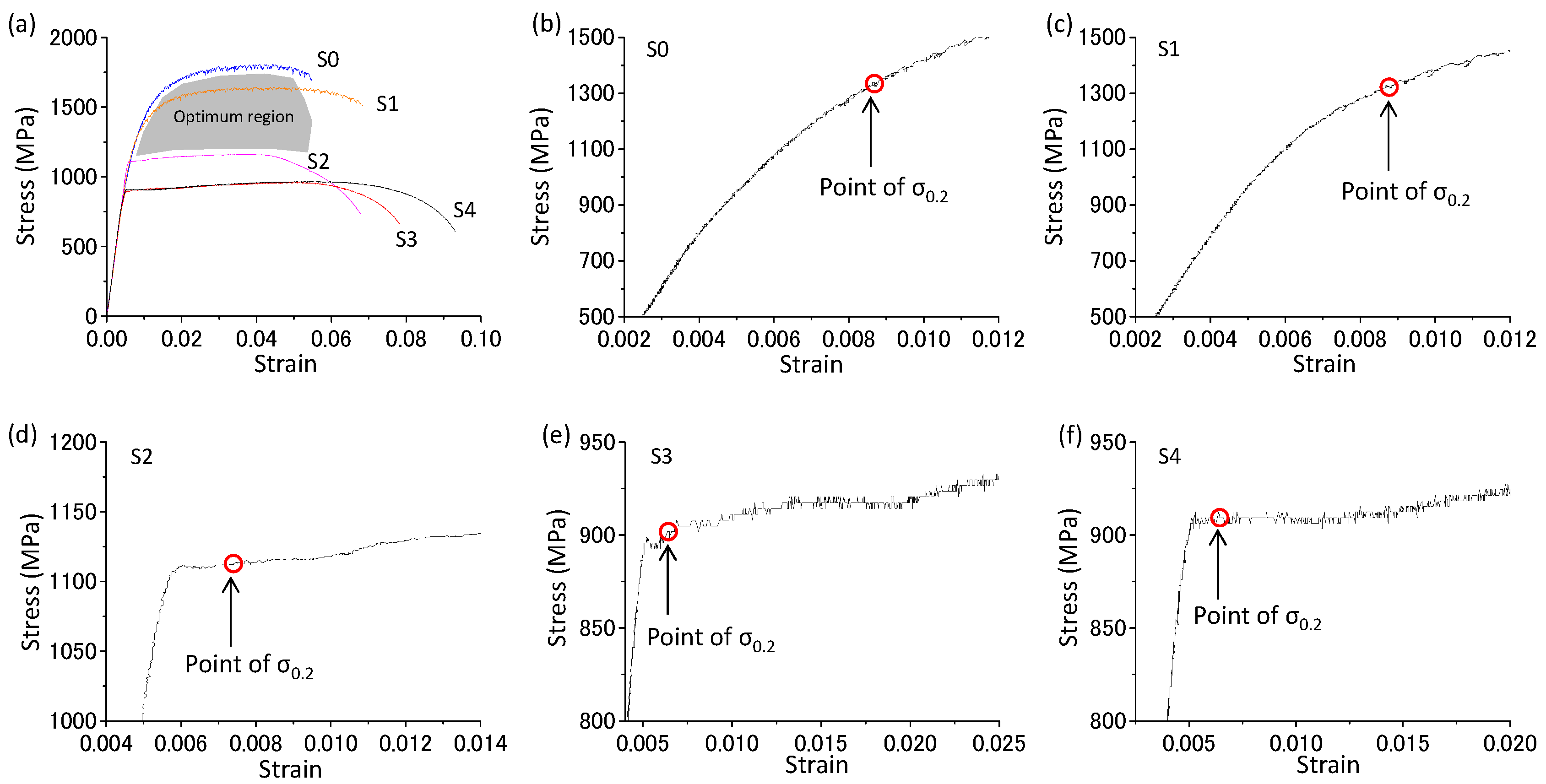
3.2. Stress–Strain Curves
3.3. Local Plastic Deformation
4. Conclusions
- (1)
- The amount of micro-yielding sites can be increased by increasing the initial dislocation density. When the initial dislocation density is high enough, the Lüders deformation can be suppressed.
- (2)
- Among the five samples, the S1 sample has the best behavior; it has a good balance of strength and ductility and no Lüders deformation phenomenon. The heat-treatment conditions between S0 and S2 are the optimal region for balancing the mechanical property and deformation behavior.
- (3)
- The Lüders deformation phenomenon is related to the work-hardening rate. Increasing the work-hardening rate is good for suppressing the Lüders deformation.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Piobert, A. Expériences sur la pénétration des projectiles dans le fer forgé. Mémoire L’artillerie 1842, 5, 502. [Google Scholar]
- Lüders, W. Uber die äeuerung der elasticität an stahlartigen eisenstäben und stahlstäben, und über eine beim biegen solcher stäbe beobachtete molecularbewegung. Dingler’s Polytech. J. 1860, 155, 18–22. [Google Scholar]
- Qiu, H.; Inoue, T.; Ueji, R. Experimental measurement of the variables of Lüders deformation in hot-rolled steel via digital image correlation. Mater. Sci. Eng. A 2020, 790, 139756. [Google Scholar] [CrossRef]
- Qiu, H.; Inoue, T.; Ueji, R. In-situ observation of Lüders band formation in hot-rolled steel via digital image correlation. Metals 2020, 10, 530. [Google Scholar] [CrossRef]
- Van Rooyen, G.T. Basic factors which influence the Lüders strain during discontinuous yielding. Mater. Sci. Eng. 1971, 7, 37–48. [Google Scholar] [CrossRef]
- Tsuchida, N.; Masuda, H.; Harada, Y.; Fukaura, K.; Tomota, Y.; Nagai, K. Effect of ferrite grain size on tensile deformation behavior of a ferrite-cementite low carbon steel. Mater. Sci. Eng. A 2008, 488, 446–452. [Google Scholar] [CrossRef]
- Zhang, Y.; Ding, H.; Zhu, H.K.; Zhou, X.D.; Zhang, Y.T.; Jia, N.; Zhang, N.X. Influence of microstructural morphology on the continuous/discontinuous yielding behavior in a medium manganese steel. Mater. Sci. Eng. A 2021, 824, 141746. [Google Scholar] [CrossRef]
- Hu, B.; Ding, F.L.; Tu, X.; Guo, Q.Y.; Ding, C.C.; Wang, Y.; Luo, H.W. Influence of lamellar and equiaxed microstructural morphologies on yielding behavior of a medium Mn steel. Materialia 2021, 20, 101252. [Google Scholar] [CrossRef]
- Lloyd, D.J.; Morris, L.R. Lüders band deformation in a fine grained aluminium alloy. Acta Metall. 1977, 25, 857–861. [Google Scholar] [CrossRef]
- Delwiche, D.E.; Moon, D.W. Strain profile of a propagating Lüders front. Mater. Sci. Eng. 1972, 9, 347–354. [Google Scholar] [CrossRef]
- Tsuchida, N.; Tomota, Y.; Nagai, K.; Fukaura, K. A simple relationship between Lüders elongation and work-hardening rate at lower yield stress. Scr. Mater. 2006, 54, 57–60. [Google Scholar] [CrossRef]
- El-Magd, E.; Scholles, H.; Weisshaupt, H. Influence of strain rate on the stress–strain curve in the range of Lüders strain. Steel Res. 1996, 67, 495–500. [Google Scholar] [CrossRef]
- VanRooyen, G.T. The stress and strain distribution in a propagation Lüders front accompanying the yield-point phenomenon in iron. Mater. Sci. Eng. 1968, 3, 105–117. [Google Scholar] [CrossRef]
- Cai, Y.L.; Yang, S.L.; Fu, S.H.; Zhang, Q.C. The influence of specimen thickness on the Lüders effect of a 5456 Al-based alloy: Experimental observations. Metals 2016, 6, 120. [Google Scholar] [CrossRef]
- Zhang, M.H.; Li, L.F.; Ding, J.; Wu, Q.B.; Wang, Y.D.; Almer, J.; Guo, F.M.; Ren, Y. Temperature-dependent micromechanical behavior of medium-Mn transformation-induced-plasticity steel studied by in situ synchrotron X-ray diffraction. Acta Mater. 2017, 141, 294–303. [Google Scholar] [CrossRef]
- Cottrell, A.H.; Bilby, B.A. Dislocation theory of yielding and strain ageing of iron. Phys. Soc. 1949, 62, 49–62. [Google Scholar] [CrossRef]
- Cottrell, A.H. A note on the Portevin-Le Chatelier effect. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1953, 44, 829–832. [Google Scholar] [CrossRef]
- Marais, A.; Mazière, M.; Forest, S.; Parrot, A.; Le Delliou, P. Identification of a strain-aging model accounting for Lüders behavior in a C-Mn steel. Philos. Mag. 2012, 92, 3589–3617. [Google Scholar] [CrossRef]
- Wang, X.G.; Wang, L.; Huang, M.X. Kinematic and thermal characteristics of Lüders and Portevin-Le Chatelier bands in a medium Mn transformation-induced plasticity steel. Acta Mater. 2017, 124, 17–29. [Google Scholar] [CrossRef]
- Tsukahara, H.; Iung, T. Finite element simulation of the Piobert–Lüders behavior in an uniaxial tensile test. Mater. Sci. Eng. A 1998, 248, 304–308. [Google Scholar] [CrossRef]
- Onodera, R.; Nonomura, M.; Aramaki, M. Stress drop, Lüders strain and strain rate during serrated flow. J. Jpn. Inst. Met. 2000, 64, 1162–1171. [Google Scholar] [CrossRef][Green Version]
- Hahn, G.T. A model for yielding with special reference to the yield-point phenomena of iron and related BCC metals. Acta Metall. 1962, 10, 727–738. [Google Scholar] [CrossRef]
- Ikeda, S.; Makii, K.; Shindo, Y.; Miura, M.; Hashimoto, S. The effect of precipitates on the plastic deformation behavior in high tensile strength IF sheet steels. Res. Dev. Kobe Steel Eng. Rep. 2002, 52, 39–42. [Google Scholar]
- Grassino, J.; Vedani, M.; Vimercati, G.; Zanella, G. Effects of skin pass rolling parameters on mechanical properties of steels. Int. J. Prec. Eng. Manufac. 2012, 13, 2017–2026. [Google Scholar] [CrossRef]
- Han, J.; Kang, S.-H.; Lee, S.-J.; Lee, Y.-K. Fabrication of bimodal-grained Al-free medium Mn steel by double intercritical annealing and its tensile properties. J. Alloys Compd. 2016, 681, 580–588. [Google Scholar] [CrossRef]
- Mohsenzadeh, M.S.; Mazinani, M. On the yield point phenomenon in low-carbon steels with ferrite-cementite microstructure. Mater. Sci. Eng. A 2016, 673, 193–203. [Google Scholar] [CrossRef]
- Liang, Z.Y.; Cao, Z.H.; Lu, J.; Huang, M.X.; Tasan, C.C. Influence of co-existing medium Mn and dual phase steel microstructures on ductility and Lüders band formation. Acta Mater. 2021, 221, 117418. [Google Scholar] [CrossRef]
- Mao, W.Q.; Gao, S.; Gong, W.; Harjo, S.; Kawasaki, T.; Tsuji, N. Quantitatively evaluating the huge Lüders band deformation in an ultrafine grain stainless steel by combining in situ neutron diffraction and digital image correlation analysis. Scripta Mater. 2023, 235, 115642. [Google Scholar] [CrossRef]
- Yin, W.J.; Briffod, F.; Hu, H.Y.; Yamazaki, K.; Shiraiwa, T.; Enoki, M. Quantitative investigation of strain partitioning and failure mechanism in ultrafine grained medium Mn steel through high resolution digital image correlation. Scripta Mater. 2023, 229, 115386. [Google Scholar] [CrossRef]
- Cai, Z.H.; Jing, S.Y.; Li, H.Y.; Zhang, K.M.; Misra, R.D.K.; Ding, H.; Tang, Z.Y. The influence of microstructural characteristics on yield point elongation phenomenon in Fe-0.2C-11Mn-2Al steel. Mater. Sci. Eng. A 2019, 739, 17–25. [Google Scholar] [CrossRef]
- Zhan, Z.D.; Shi, Z.G.; Wang, Z.M.; Lu, W.J.; Chen, Z.N.; Zhang, D.; Chai, F.; Luo, X.B. Effect of manganese on the strength–toughness relationship of low-carbon copper and nickel-containing hull steel. Materials 2024, 17, 1012. [Google Scholar] [CrossRef]
- Sugimoto, K.; Shioiri, S.; Kobayashi, J. Effects of mean normal stress on strain-hardening, strain-induced martensite transformation, and void-formation behaviors in high-strength TRIP-aided steels. Metals 2024, 14, 61. [Google Scholar] [CrossRef]
- Benzing, J.T.; Luecke, W.E.; Mates, S.P.; Ponge, D.; Raabe, D.; Wittig, J.E. Intercritical annealing to achieve a positive strain-rate sensitivity of mechanical properties and suppression of macroscopic plastic instabilities in multi-phase medium-Mn steels. Mater. Sci. Eng. A 2021, 803, 140469. [Google Scholar] [CrossRef]
- Liu, R.D.; Hu, Z.P.; Lin, C.Q.; Yang, D.P.; Gu, X.L.; Xu, X.; Guo, J.Y. A novel design to eliminate Lüders band in medium-Mn steel and its microstructure-property relationship. Crystals 2023, 13, 936. [Google Scholar] [CrossRef]
- Ma, J.W.; Lu, Q.; Sun, L.; Shen, Y. Two-step intercritical annealing to eliminate Lüders band in a strong and ductile medium Mn Steel. Metall. Mater. Trans. A 2018, 49, 4404–4408. [Google Scholar] [CrossRef]
- Qiu, H.; Ueji, R.; Inoue, T.; Kimura, Y. Plastic instability in medium-carbon tempered martensite steel. Materials 2021, 14, 4609. [Google Scholar] [CrossRef]
- Zhang, J.; Huang, M.X.; Sun, B.H.; Zhang, B.N.; Ding, R.; Luo, C.; Zeng, W.; Zhang, C.; Yang, Z.G.; van der Zwaag, S.; et al. Critical role of Lüders banding in hydrogen embrittlement susceptibility of medium Mn steels. Scr. Mater. 2021, 190, 32–37. [Google Scholar] [CrossRef]
- Ozerov, M.; Sokolovsky, V.; Yurchenko, N.; Astakhov, I.; Povolyaeva, E.; Plekhov, O.; Tagirov, D.; Stepanov, N.; Zherebtsov, S. Effect of cold rolling on microstructure and mechanical properties of a cast TiNbZr-based composite reinforced with borides. Metals 2024, 14, 104. [Google Scholar] [CrossRef]
- Yi, Y.X.; Yin, F.; Zhai, J.J.; Liu, Y.X. Microstructure evolution and numerical modeling of TC4 titanium alloy during ultrasonic shot peening process. Metals 2024, 14, 275. [Google Scholar] [CrossRef]
- Kato, M. Introduction to the Theory of Dislocations; Shokabo: Tokyo, Japan, 2003; p. 120. [Google Scholar]
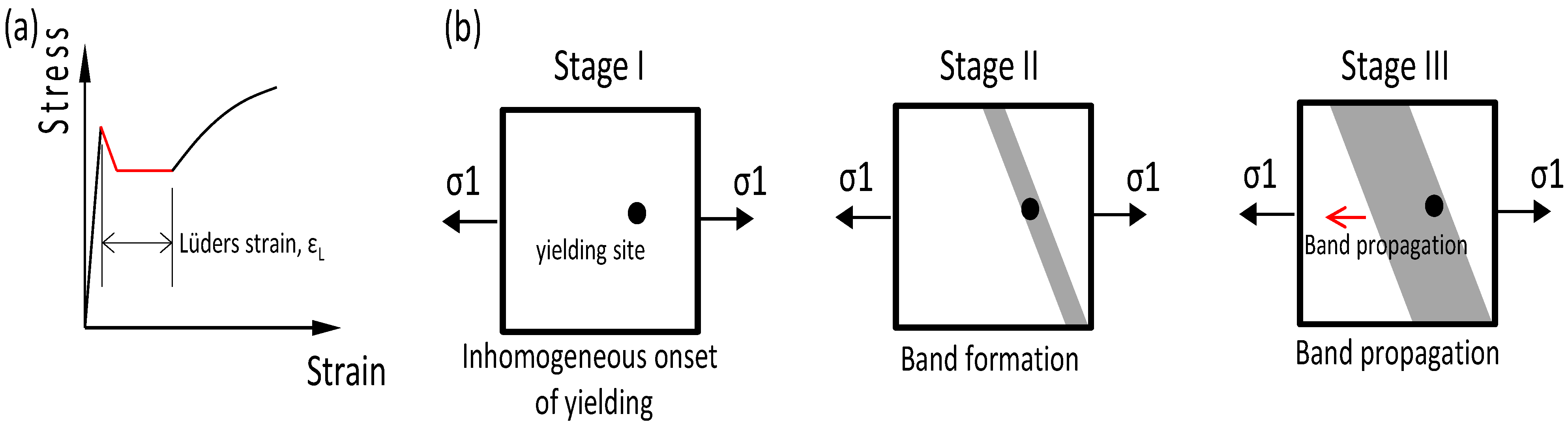
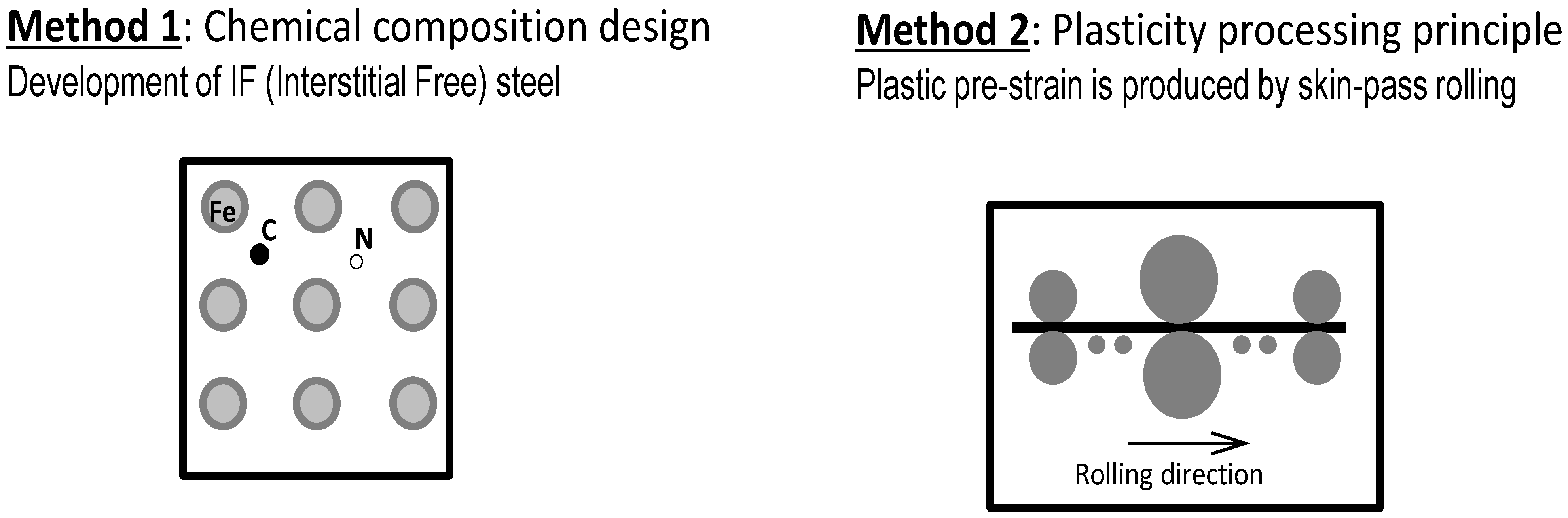

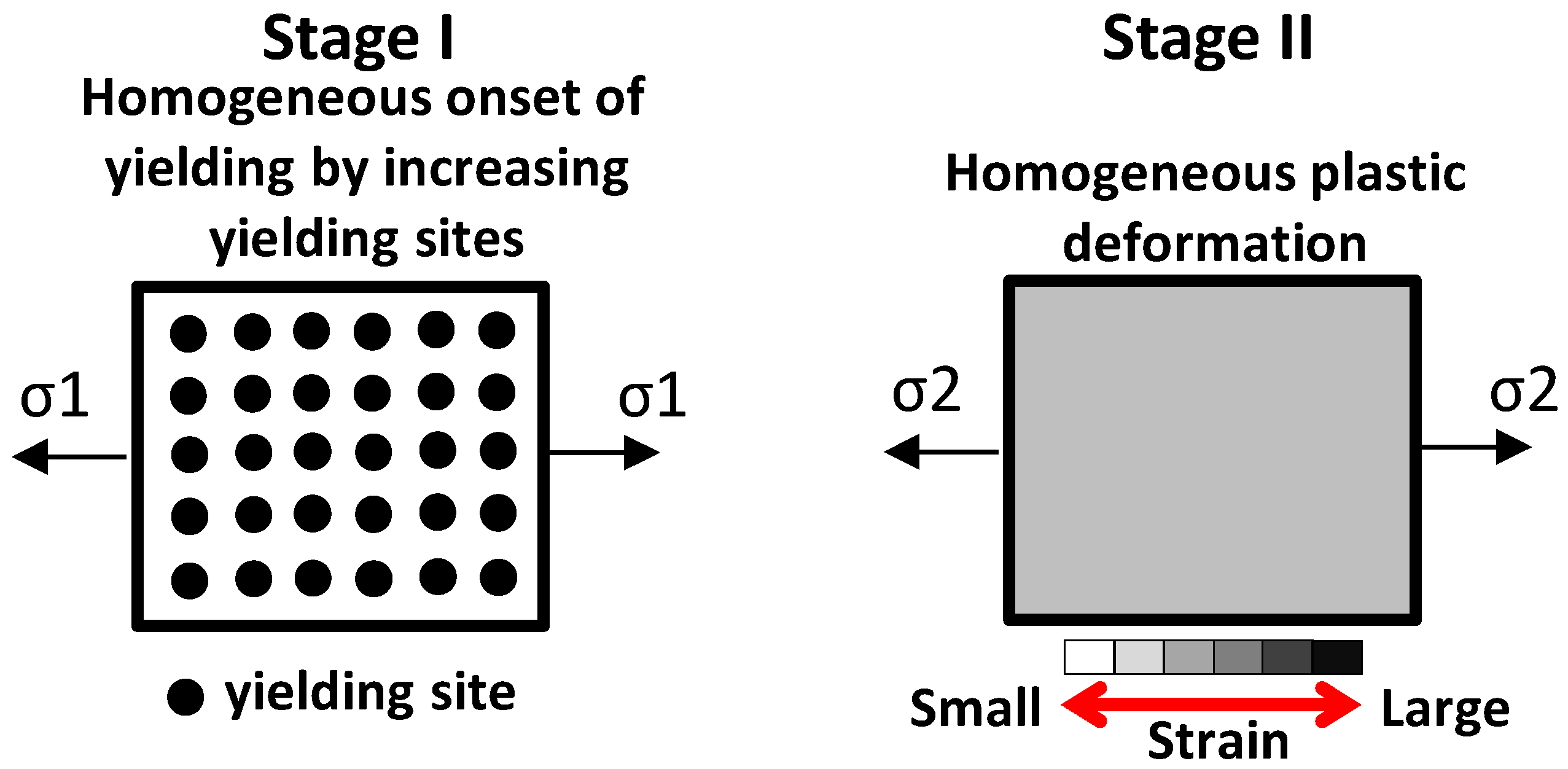

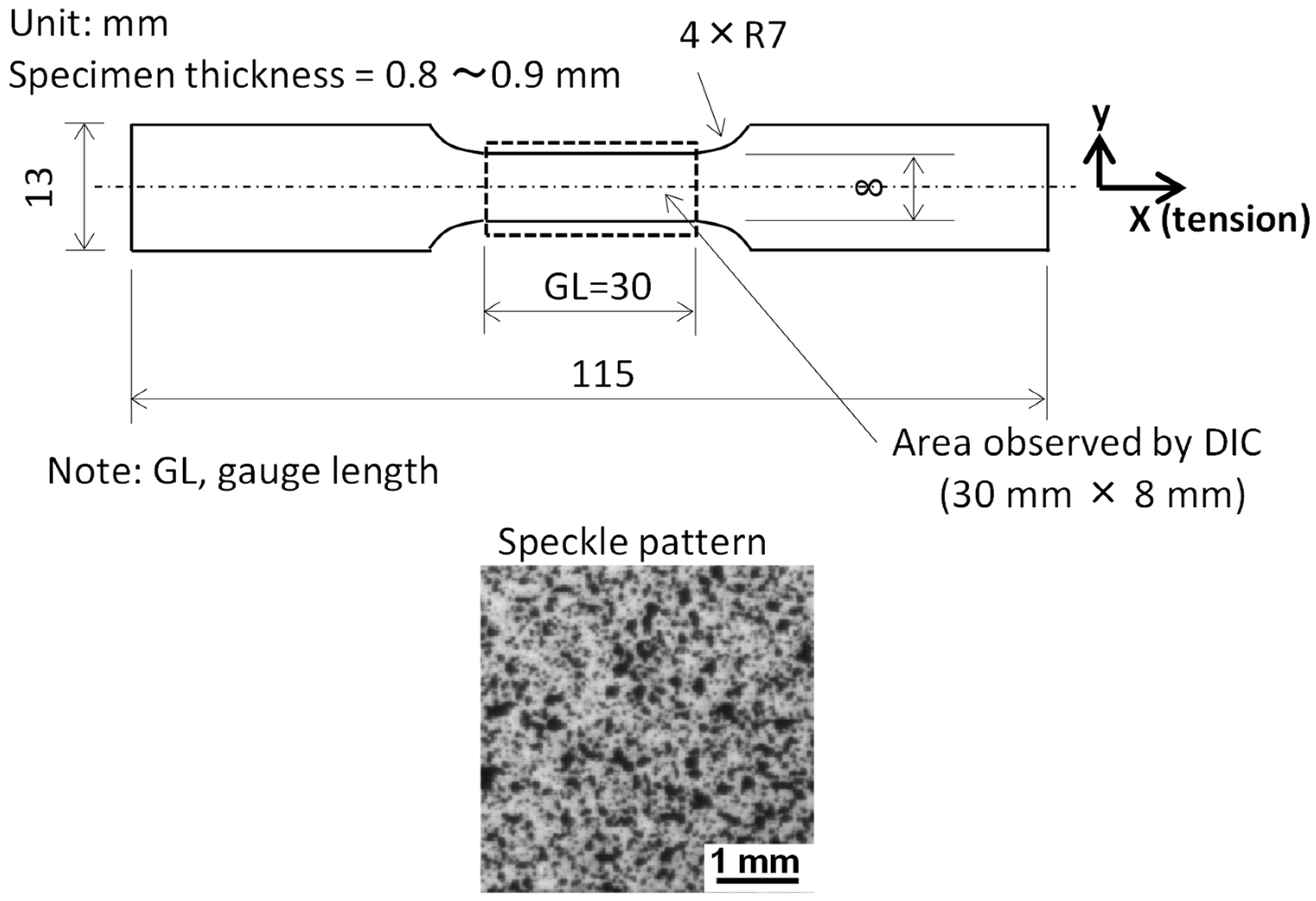


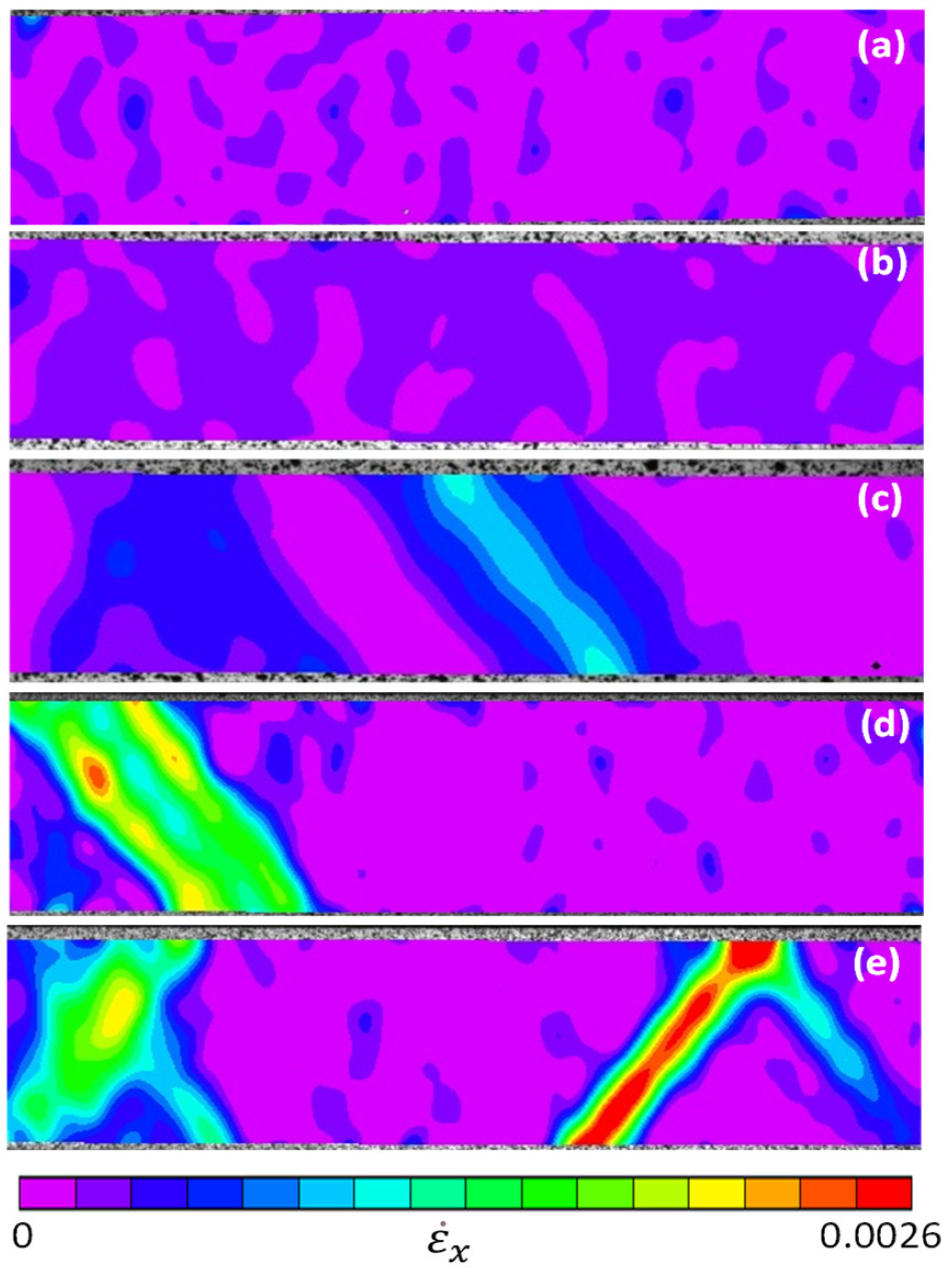
| Sample No. | Average Strain Rate (s−1) | Max. Strain Rate in the Band (s−1) |
|---|---|---|
| S0 | 1.02 × 10−4 | — |
| S1 | 1.83 × 10−4 | — |
| S2 | 2.61 × 10−4 | 1.09 × 10−3 |
| S3 | 3.21 × 10−4 | 2.37 × 10−3 |
| S4 | 4.62 × 10−4 | 3.33 × 10−3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, H.; Ueji, R.; Inoue, T. Suppression of Inhomogeneous Plastic Deformation in Medium-Carbon Tempered Martensite Steel. Metals 2024, 14, 306. https://doi.org/10.3390/met14030306
Qiu H, Ueji R, Inoue T. Suppression of Inhomogeneous Plastic Deformation in Medium-Carbon Tempered Martensite Steel. Metals. 2024; 14(3):306. https://doi.org/10.3390/met14030306
Chicago/Turabian StyleQiu, Hai, Rintaro Ueji, and Tadanobu Inoue. 2024. "Suppression of Inhomogeneous Plastic Deformation in Medium-Carbon Tempered Martensite Steel" Metals 14, no. 3: 306. https://doi.org/10.3390/met14030306
APA StyleQiu, H., Ueji, R., & Inoue, T. (2024). Suppression of Inhomogeneous Plastic Deformation in Medium-Carbon Tempered Martensite Steel. Metals, 14(3), 306. https://doi.org/10.3390/met14030306









