The Action Mechanism of Rolling Texture on the Anisotropic Behavior of a Pure Titanium Plate
Abstract
:1. Introduction
2. Materials and Methods
3. Results
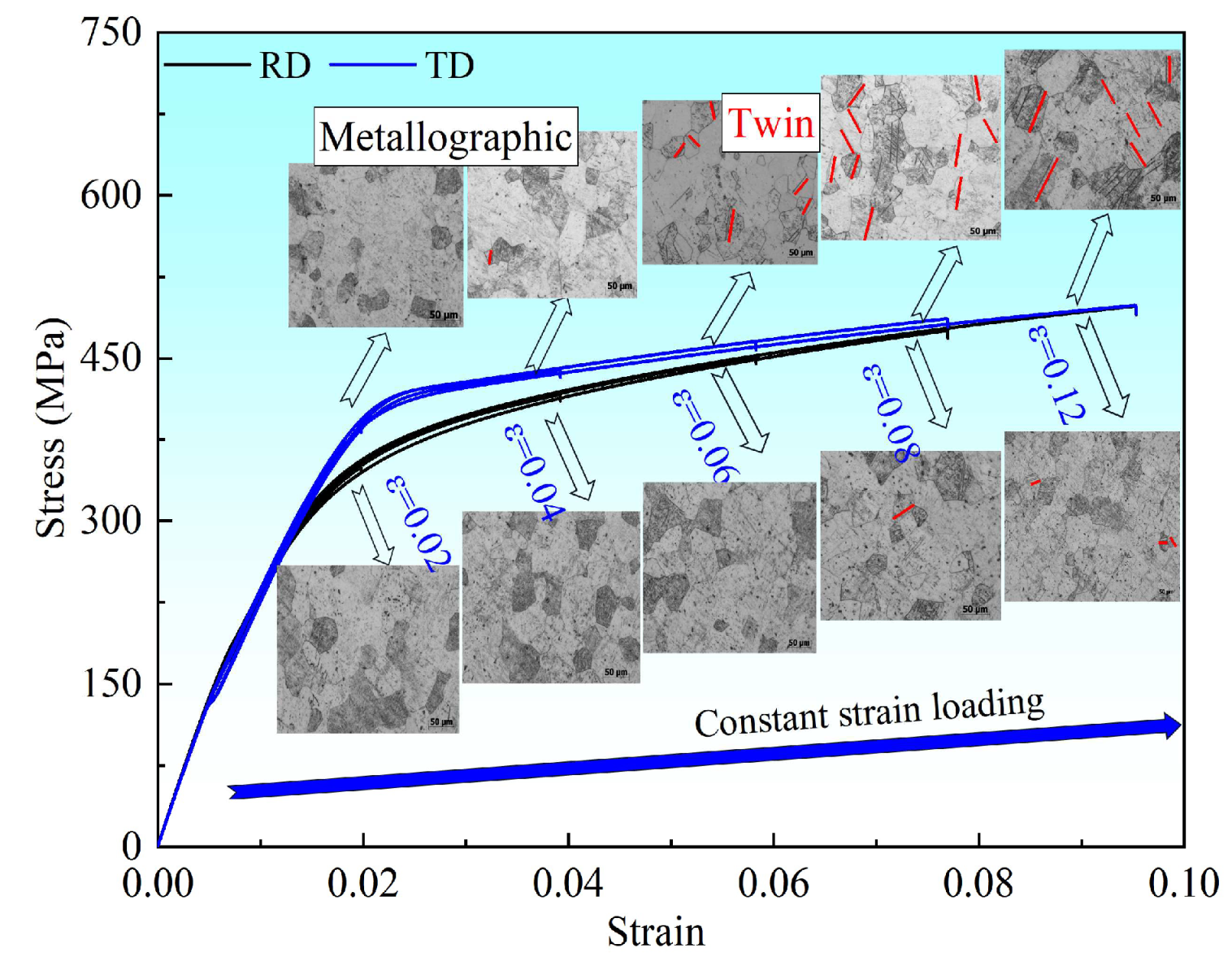
3.1. Macroscopic Stress–Strain Curves
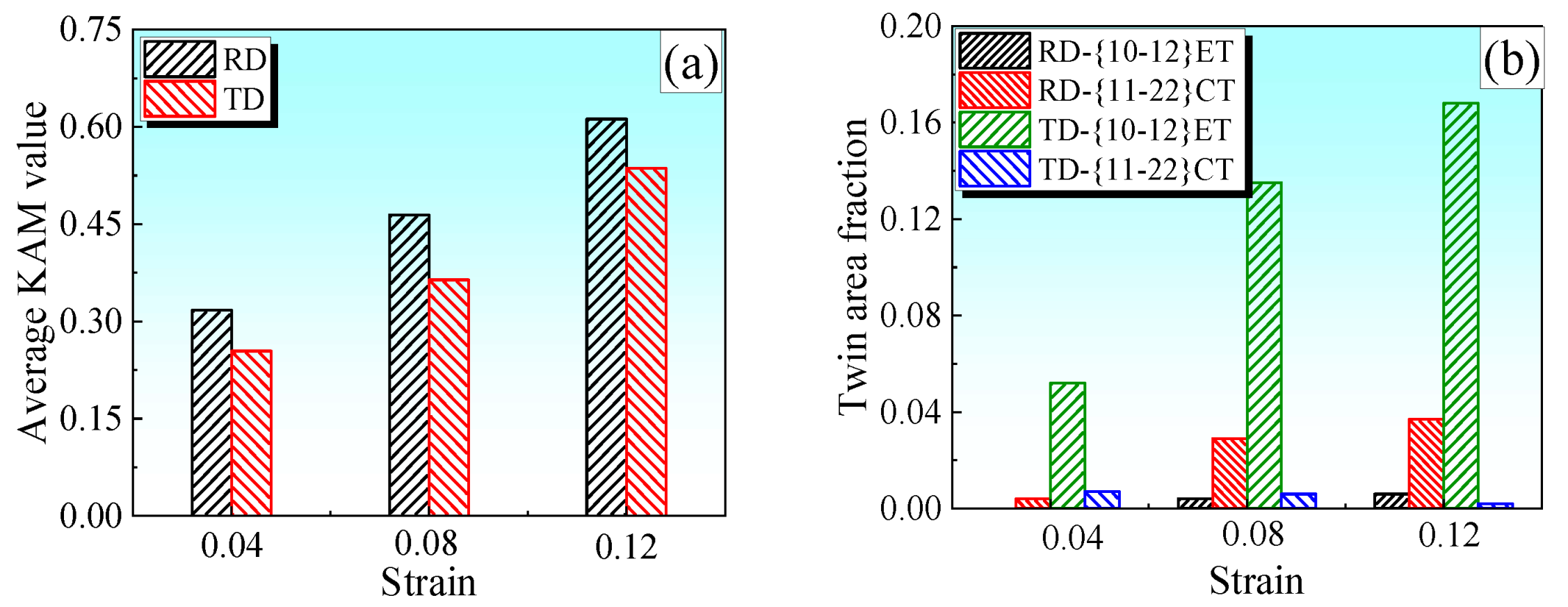
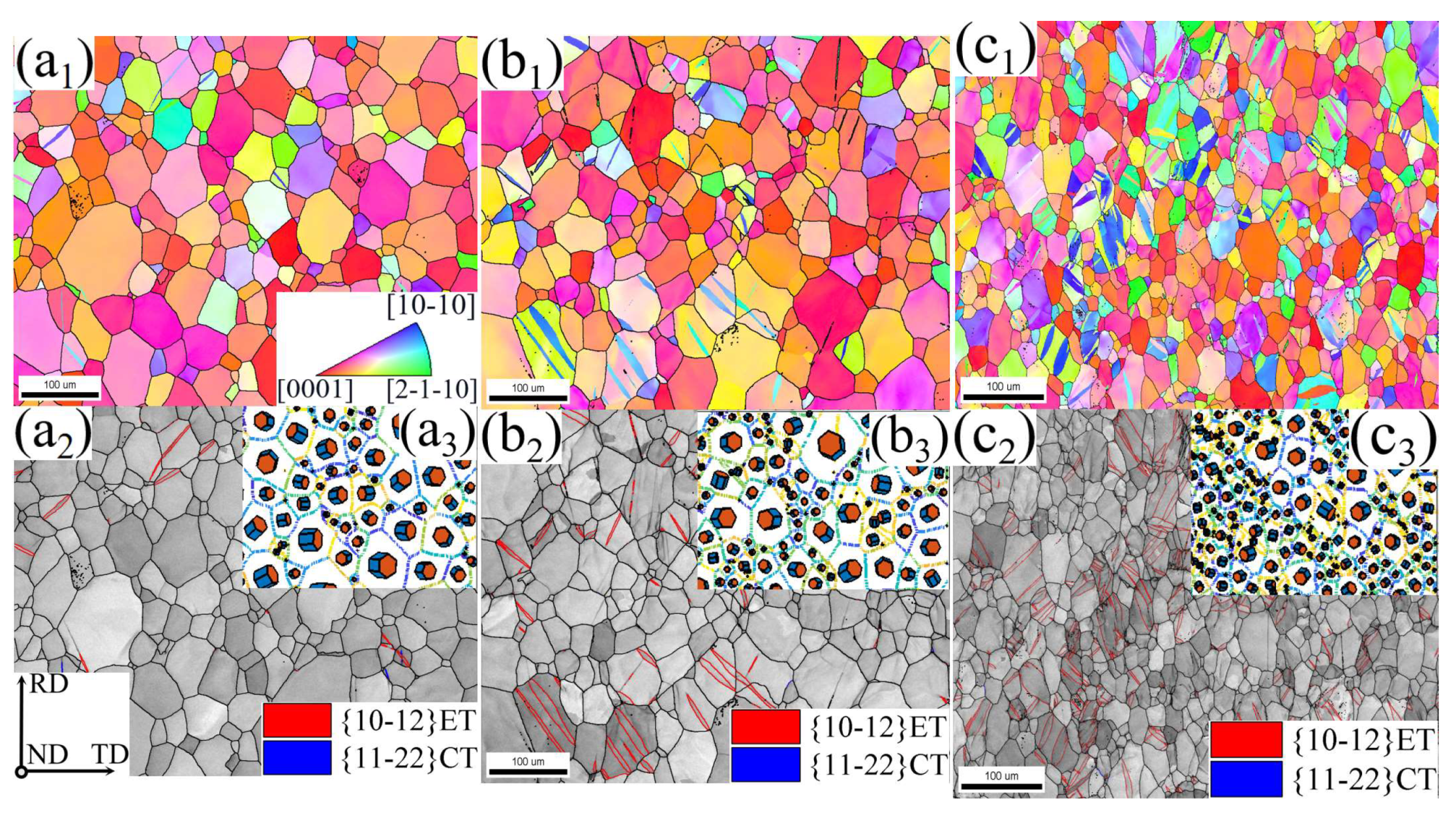
3.2. Microstructure Evolution
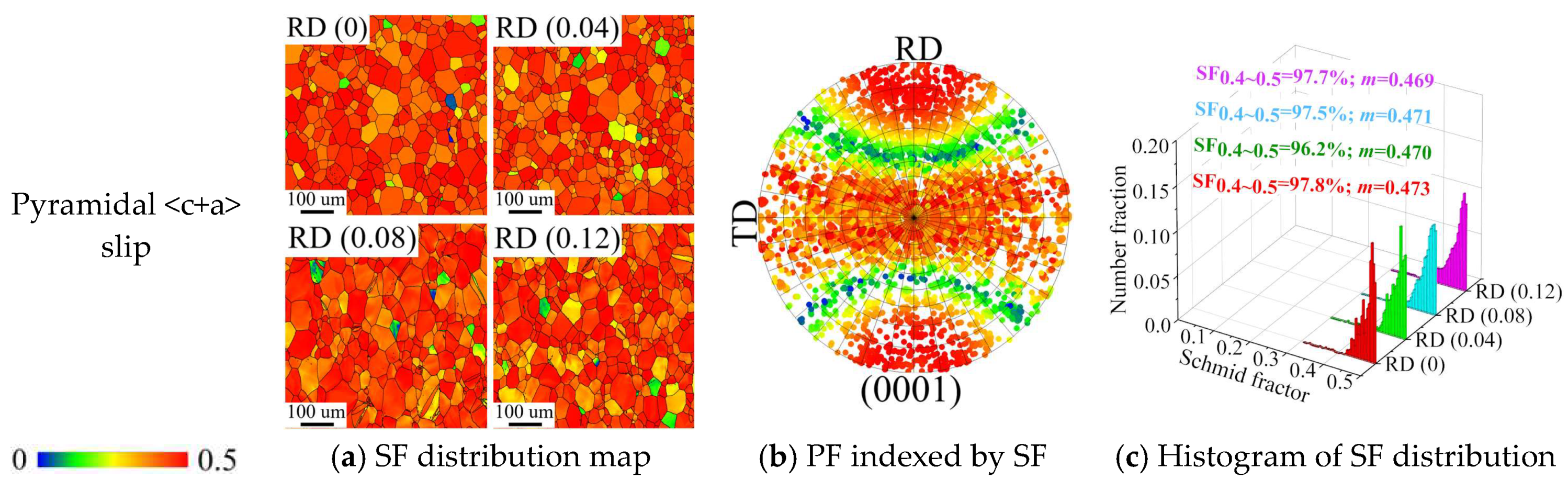
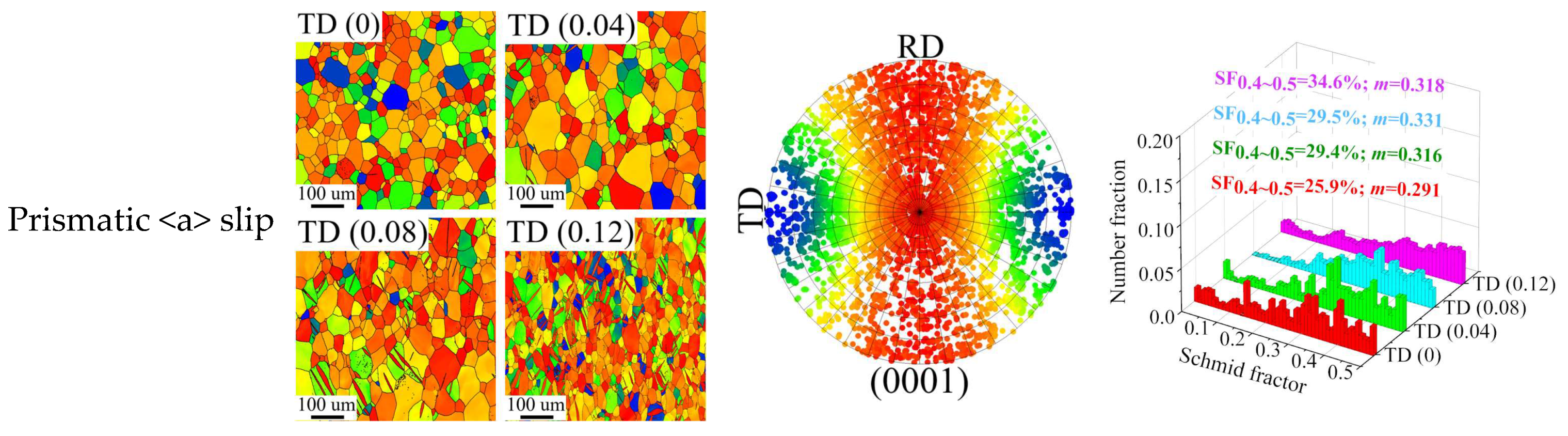
3.3. Texture Evolution
4. Discussion
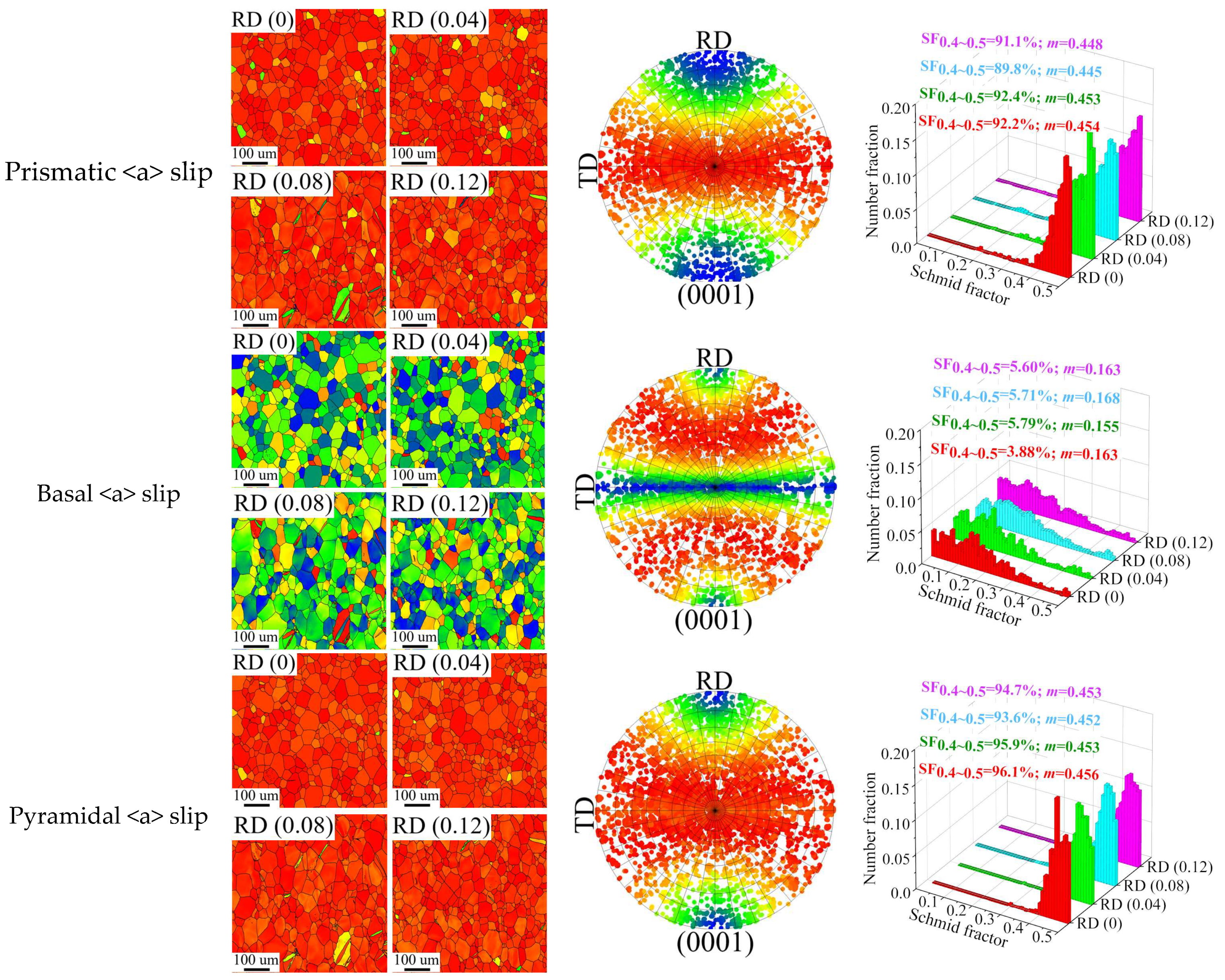
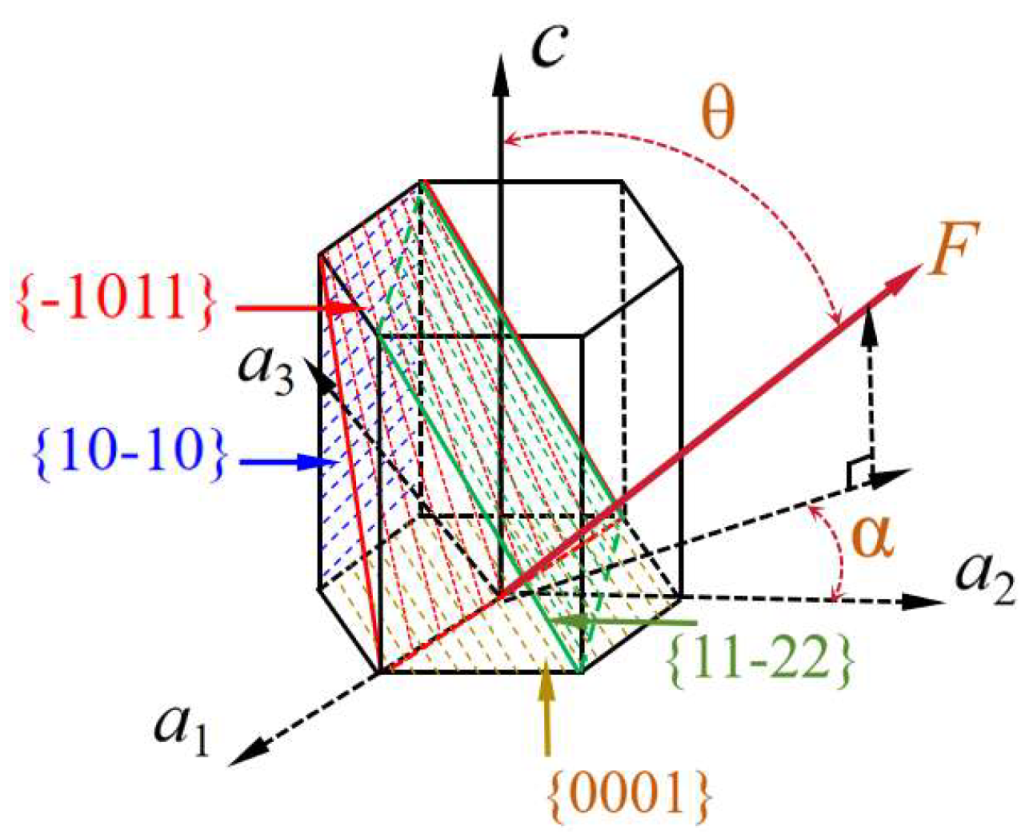
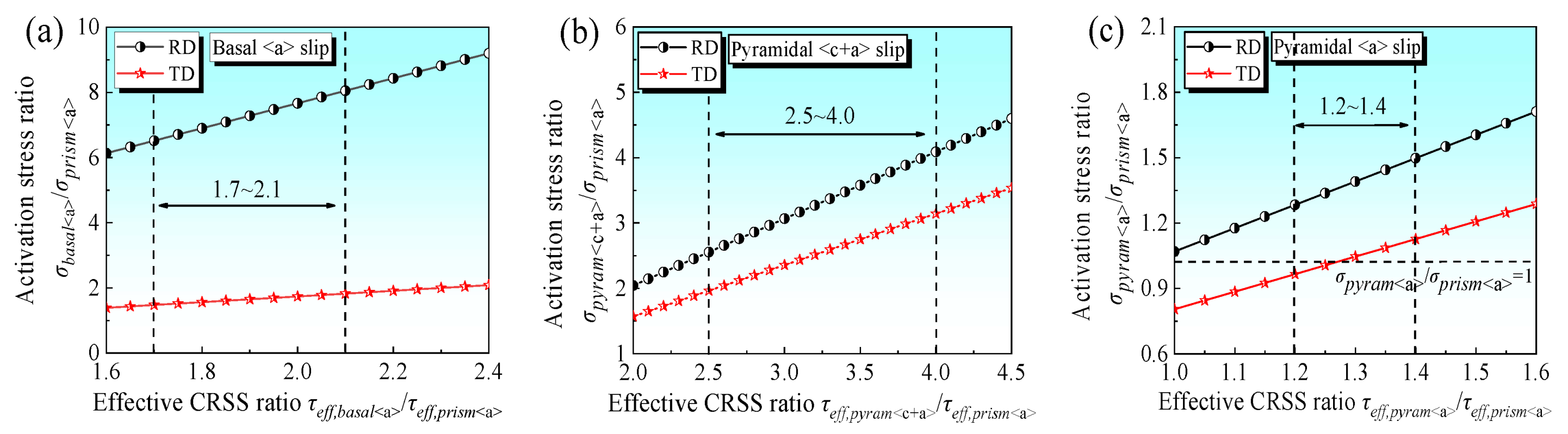
4.1. Effect of Texture on the Activability of Slip Systems
4.2. Quantitative Calculation of SF
4.3. Identification of the Dominant Deformation Mechanism
5. Conclusions
- In this work, the influence mechanism of rolling texture on the anisotropy of the plastic behavior of a pure titanium plate was studied by Schmid law in combination with a macroscopic tensile test and mesoscopic EBSD characterization. The conclusions are as follows:
- The special crystal orientation corresponding to the basal bimodal texture affects the activability of each slip system by changing the SF value, and the changes in the type and difficulty of the activated deformation mode lead to the obvious anisotropy of the macro- and micro- deformation characteristics of the rolled titanium plate. The angle θ between the loading axis and the c-axis mostly influences the variation trend of the SF of each slip system, whereas the angle α between the loading axis projection and the a-axis impacts the value of SF. The theoretical derivation results can well predict the SF value of each slip system under different loading conditions;
- The tilt angle θb of the grain c-axis from ND to TD becomes polarized due to dislocation slip during tensile along RD and TD, but the tilt angle θa of the grain c-axis from ND to RD stays approximately vertical. The SF value of each deformation mode changes with the change of θa during RD tensile, but the change of θa is minor, resulting in the ψ-m values of each deformation mode being almost constant. Since the SF values of each deformation mode change mainly with the change of θb during TD tensile, the discrete character of the SF values of each deformation mode results from the broad range of θb;
- The prismatic <a> slip essentially dominates the RD tensile deformation. The pyramidal <a> slip and pyramidal <c+a> slip will be activated during the subsequent hardening, but the basal <a> slip cannot. During TD tensile, the prismatic <a> slip is preferentially activated in the soft-oriented part of the grain. Prismatic <a> slip and pyramidal <a> slip become the dominant deformation modes during the subsequent hardening. The basal <a> slip will be activated in the soft-oriented grains, while the pyramidal <c+a> slip is more difficult to activate. {10-12} ET coordinates the c-axis deformation in the grains where the dislocation slip is limited.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Thomas, R. Titanium in the geothermal industry. Geothermics 2003, 32, 679–687. [Google Scholar] [CrossRef]
- Sathish, S.; Geetha, M.; Pandey, N.D.; Richard, C.; Asokamani, R. Studies on the corrosion and wear behavior of the laser nitrided biomedical titanium and its alloys. Mater. Sci. Eng. C 2010, 30, 376–382. [Google Scholar] [CrossRef]
- Shah, F.A.; Trobos, M.; Thomsen, P.; Palmquist, A. Commercially pure titanium (cp-Ti) versus titanium alloy (Ti6Al4V) materials as bone anchored implants—Is one truly better than the other? Mater. Sci. Eng. C 2016, 62, 960–966. [Google Scholar] [CrossRef] [PubMed]
- Lotfi, M.; Sajjady, S.A.; Amini, S. Wettability analysis of titanium alloy in 3D elliptical ultrasonic assisted turning. Int. J. Lightweight Mater. Manuf. 2019, 2, 235–240. [Google Scholar] [CrossRef]
- Battaini, M.; Pereloma, E.V.; Davies, C.H.J. Effect of orientation and temperature on the mechanical properties of commercially pure titanium. Adv. Mater. Res. 2007, 15, 941–946. [Google Scholar] [CrossRef]
- Merson, E.; Brydson, R.; Brown, A. The effect of crystallographic orientation on the mechanical properties of titanium. J. Phys. Conf. Ser. 2008, 126, 012120. [Google Scholar] [CrossRef]
- Nasiri, A.H.; Ekrami, A.; Ziaei, M.A.A. Effects of thickness and texture on mechanical properties anisotropy of commercially pure titanium thin sheets. Mater. Des. 2013, 44, 528–534. [Google Scholar] [CrossRef]
- Yoo, M.H. Slip, twinning, and fracture in hexagonal close-packed metals. Metall. Mater. Trans. A 1981, 12, 409–418. [Google Scholar] [CrossRef]
- Warwick, J.L.W.; Jones, N.G.; Rahman, K.M.; Dye, D. Lattice strain evolution during tensile and compressive loading of CP Ti. Acta Mater. 2012, 60, 6720–6731. [Google Scholar] [CrossRef]
- Li, H.; Mason, D.E.; Bieler, T.R. Methodology for estimating the critical resolved shear stress ratios of a-phase Ti using EBSD-based trace analysis. Acta Mater. 2013, 61, 7555–7567. [Google Scholar] [CrossRef]
- Wang, L.; Zheng, Z.; Phukan, H. Direct measurement of critical resolved shear stress of prismatic and basal slip in polycrystalline Ti using high energy X-Ray diffraction microscopy. Acta Mater. 2017, 132, 598–610. [Google Scholar] [CrossRef]
- Yoo, M.H.; Wei, C.T. Slip modes of hexagonal close packed metals. J. Appl. Phys. 1967, 38, 4317–4322. [Google Scholar] [CrossRef]
- Yoo, M.H.; Agnew, S.R.; Morris, J.R. Non-basal slip systems in HCP metals and alloys: Source mechanisms. Mater. Sci. Eng. A 2001, 319, 87–92. [Google Scholar] [CrossRef]
- Viswanathan, G.; Lee, E.; Maher, D.M. Direct observations and analyses of dislocation substructures in the α phase of an α/β Ti-alloy formed by nanoindentation. Acta Mater. 2005, 53, 5101–5115. [Google Scholar] [CrossRef]
- Williams, J.C.; Baggerly, R.G.; Paton, N.E. Deformation behavior of HCP Ti-Al alloy single crystals. Metall. Mater. Trans. A 2002, 33, 837–850. [Google Scholar] [CrossRef]
- Gong, J.C.; Wilkinson, A.J. Anisotropy in the plastic flow properties of single-crystal a titanium determined from micro-cantilever beams. Acta Mater. 2009, 57, 5693–5705. [Google Scholar] [CrossRef]
- Bertin, M.; Du, C.; Hoefnagels, J.P.M. Crystal plasticity parameter identification with 3D measurements and integrated digital image correlation. Acta Mater. 2016, 116, 321–331. [Google Scholar] [CrossRef]
- Salem, A.A.; Kalidindi, S.R.; Doherty, R.D. Strain hardening regimes and microstructure evolution during large strain compression of high purity titanium. Scr. Mater. 2002, 46, 419–423. [Google Scholar] [CrossRef]
- Song, S.G.; Gray, G.T. Structural interpretation of the nucleation and growth of deformation twins in Zr and Ti—I. application of the coincidence site lattice (CSL) theory to twinning problems in HCP structures. Acta Mater. 1995, 43, 2325–2337. [Google Scholar] [CrossRef]
- Song, S.G.; Gray, G.T. Structural interpretation of the nucleation and growth of deformation twins in Zr and Ti—II. Tem study of twin morphology and defect reactions during twinning. Acta Mater. 1995, 43, 2339–2350. [Google Scholar] [CrossRef]
- Murasawa, G.; Morimoto, T.; Yoneyama, S. Nucleation and growth behavior of twin region around yield point of polycrystalline pure Ti. Exp. Mech. 2012, 52, 503–512. [Google Scholar] [CrossRef]
- Chun, Y.B.; Yu, S.H.; Semiatin, S.L. Effect of deformation twinning on microstructure and texture evolution during cold rolling of CP-titanium. Mater. Sci. Eng. A 2005, 398, 209–219. [Google Scholar] [CrossRef]
- Wang, L.; Barabash, R.; Bieler, T. Study of {11-21} twinning in α-Ti by EBSD and laue microdiffraction. Metall. Mater. Trans. A 2013, 44, 3664–3674. [Google Scholar] [CrossRef]
- Deng, X.G.; Hui, S.X.; Ye, W.J. Analysis of twinning behavior of pure Ti compressed at different strain rates by schmid factor. Mater. Sci. Eng. A 2013, 575, 15–20. [Google Scholar] [CrossRef]
- Deng, X.G.; Zhang, D.L.; Jiao, Q.Y. Schmid factor analysis of twinning behavior in Ti-2Al alloy. Mater. Sci. Eng. A 2022, 844, 143201. [Google Scholar] [CrossRef]
- Chen, C.; Han, D.S.; Song, Y.T. Thermal stability of deformation twins in cryogenic rolled CP-Ti. Mater. Charact. 2023, 196, 112587. [Google Scholar] [CrossRef]
- Behnam, A.; Leyun, W.; Kumar, M.A. Grain boundary slip-twin transmission in titanium. Acta Mater. 2023, 244, 118556. [Google Scholar]
- Devesh, K.C.; Soumita, M.; Basudev, B. Role of slip and twinning on strain hardening, and correlation with geometric hardening, latent hardening, and grain boundary strengthening in titanium. Int. J. Plast. 2023, 161, 103516. [Google Scholar]
- Chun, Y.B.; Battaini, M.; Davies, C.H.J.; Hwang, S.K. Distribution characteristics of in-grain misorientation axes in cold-rolled commercially pure titanium and their correlation with active slip modes. Metall. Mater. Trans. A 2010, 41, 3473–3487. [Google Scholar] [CrossRef]
- Won, J.W.; Choi, S.W.; Yeom, J.T.; Hyun, Y.T.; Lee, C.S.; Park, S.H. Anisotropic twinning and slip behaviors and their relative activities in rolled alpha-phase titanium. Mater. Sci. Eng. A 2017, 698, 54–62. [Google Scholar] [CrossRef]
- Li, Q.X.; Liu, Y.F.; Si, C.Q.; Xu, Q.X.; Liu, D.X.; Le, Q.C.; Fu, L.; Jia, Z.; Ma, Z.B.; Yuan, R. The microstructure evolution, mechanical property and deformation mechanism of rolled Al3La/Mg-Li-Al-Zn composites prepared by in-situ synthesis. Mater. Sci. Eng. A 2024, 894, 146182. [Google Scholar] [CrossRef]
- Won, J.W.; Park, C.H.; Hong, S.G. Deformation anisotropy and associated mechanisms in rolling textured high purity titanium. J. Alloys Compd. 2015, 651, 245–254. [Google Scholar] [CrossRef]
- Kailas, S.V.; Prasad, Y.; Biswas, S.K. Influence of initial texture on the microstructural instabilities during compression of commercial α-titanium at 25°C to 400°C. Metall. Mater. Trans. A 1994, 25, 1425–1434. [Google Scholar] [CrossRef]
- Li, W.; Li, S.S.; Huang, Z.T.; Tang, G.Y.; Zhang, G.M.; Yu, H. Research on the anisotropic mechanism of plastic behavior during tensile process of textured pure titanium. Mater. Sci. Eng. A 2024, 894, 146153. [Google Scholar] [CrossRef]
- Wronski, M.; Kumar, M.A.; Capolungo, L.; Mccabe, R.J.; Wierzbanowski, K.; Tomé, C.N. Deformation behavior of cp-titanium: Experiment and crystal plasticity modeling. Mater. Sci. Eng. A 2018, 724, 289–297. [Google Scholar] [CrossRef]
- Roth, A.; Lebyodkin, M.A.; Lebedkina, T.A. Mechanisms of anisotropy of mechanical properties of α-titanium in tension conditions. Mater. Sci. Eng. A 2014, 596, 236–243. [Google Scholar] [CrossRef]
- Yi, N.; Hama, T.; Kobuki, A.; Fujimoto, H.; Takuda, H. Anisotropic deformation behavior under various strain paths in commercially pure titanium Grade 1 and Grade 2 sheets. Mater. Sci. Eng. A 2016, 655, 70–85. [Google Scholar] [CrossRef]
- Li, W.; Wang, S.Z.; Huang, Z.T.; Li, S.S.; Huang, Z.M.; Yu, H. Anisotropic mechanism of cold-rolled pure titanium plate during the two-step tensile process of the variable path. Mater. Sci. Eng. A 2024, 906, 146755. [Google Scholar] [CrossRef]
- Nasiri, A.H.; Ekrami, A.; Ziaei, M.A.A.; Shohani, M. Effects of rolling reduction on mechanical properties anisotropy of commercially pure titanium. Mater. Des. 2012, 34, 268–274. [Google Scholar] [CrossRef]
- Legrand, B. Influence De La Structure Electronique Sur La Facilit’e Relative Des Glissements Dans Les M´etaux De Structure Hexagonale Compacte. Ph.D. Thesis, Universite Pierre et Marie Curie, Paris, France, 1984. [Google Scholar]
- Amouzou, K.; Richeton, T.; Roth, A. Micromechanical modeling of hardening mechanisms in commercially pure α-titanium in tensile condition. Int. J. Plast. 2016, 80, 222–240. [Google Scholar] [CrossRef]
- Suh, B.C.; Kim, J.H.; Hwang, J.H. Twinning-mediated formability in Mg alloys. Sci. Rep. 2016, 6, 22364. [Google Scholar] [CrossRef] [PubMed]
- Park, S.H.; Hong, S.G.; Lee, C.S. Enhanced stretch formability of rolled Mg-3Al-1Zn Alloy at room temperature by initial {10–12} twins. Mater. Sci. Eng. A 2013, 578, 271–276. [Google Scholar] [CrossRef]
- Salem, A.A.; Kalidindi, S.R.; Doherty, R.D. Strain hardening of titanium: Role of deformation twinning. Acta Mater. 2003, 51, 4225–4237. [Google Scholar] [CrossRef]
- Salem, A.A.; Kalidindi, S.R.; Semiatin, S.L. Strain hardening due to deformation twinning in α-titanium: Constitutive relations and crystal-plasticity modeling. Acta Mater. 2005, 53, 3495–3502. [Google Scholar] [CrossRef]
- Salem, A.A.; Kalidindi, S.R.; Doherty, R.D. Strain hardening due to deformation twinning in -titanium: Mechanisms. Metall. Mater. Trans. A 2006, 37, 259–268. [Google Scholar] [CrossRef]
- Becker, H.; Pantleon, W. Work-hardening stages and deformation mechanism maps during tensile deformation of commercially pure titanium. Comput. Mater. Sci. 2013, 76, 52–59. [Google Scholar] [CrossRef]
- Gurao, N.P.; Sethuraman, S.; Suwas, S. Evolution of texture and microstructure in commercially pure titanium with change in strain path during rolling. Metall. Mater. Trans. A 2012, 44, 1497–1507. [Google Scholar] [CrossRef]
- Hutchinson, W.B.; Barnett, M.R. Effective values of critical resolved shear stress for slip in polycrystalline magnesium and other hcp metals. Scr. Mater. 2010, 63, 737–740. [Google Scholar] [CrossRef]
- Won, J.W.; Park, K.T.; Hong, S.G.; Lee, C.S. Anisotropic yielding behavior of rolling textured high purity titanium. Mater. Sci. Eng. A 2015, 637, 215–221. [Google Scholar] [CrossRef]
- Li, W.; Li, S.S.; Yu, H. Research on the anisotropy of plastic behavior and deformation mechanism of textured pure titanium. Mater. Charact. 2022, 194, 112483. [Google Scholar] [CrossRef]








| Elements | O | C | N | H | Fe | Ti |
|---|---|---|---|---|---|---|
| Composition (ppm) | 820 | 52 | 40 | 3 | 220 | Balance |
| Deformation Mode | RD (75~90°, 90°) | TD (50~70°, 60°) | ND (30~50°, 40°) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| mmin | mmax | m | mmin | mmax | m | mmin | mmax | m | |
| prismatic <a> slip | 0.41 | 0.50 | 0.46 | 0.25 | 0.44 | 0.33 | 0.11 | 0.29 | 0.17 |
| basal <a> slip | 0.00 | 0.24 | 0.12 | 0.28 | 0.49 | 0.38 | 0.37 | 0.50 | 0.42 |
| pyramidal <a> slip | 0.38 | 0.49 | 0.43 | 0.34 | 0.50 | 0.41 | 0.20 | 0.43 | 0.31 |
| pyramidal <c+a> slip | 0.34 | 0.50 | 0.45 | 0.19 | 0.48 | 0.42 | 0.19 | 0.41 | 0.40 |
| {10-12} ET | 0.00 | 0.03 | 0.00 | 0.00 | 0.20 | 0.18 | 0.17 | 0.38 | 0.30 |
| {11-22} CT | 0.34 | 0.50 | 0.40 | 0.20 | 0.48 | 0.30 | 0.00 | 0.29 | 0.09 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Li, W.; Huang, Z.; Li, S.; Zhang, G.; Yu, H. The Action Mechanism of Rolling Texture on the Anisotropic Behavior of a Pure Titanium Plate. Metals 2024, 14, 849. https://doi.org/10.3390/met14080849
Wang S, Li W, Huang Z, Li S, Zhang G, Yu H. The Action Mechanism of Rolling Texture on the Anisotropic Behavior of a Pure Titanium Plate. Metals. 2024; 14(8):849. https://doi.org/10.3390/met14080849
Chicago/Turabian StyleWang, Sanzhong, Wei Li, Ziteng Huang, Songsong Li, Genmao Zhang, and Hui Yu. 2024. "The Action Mechanism of Rolling Texture on the Anisotropic Behavior of a Pure Titanium Plate" Metals 14, no. 8: 849. https://doi.org/10.3390/met14080849
APA StyleWang, S., Li, W., Huang, Z., Li, S., Zhang, G., & Yu, H. (2024). The Action Mechanism of Rolling Texture on the Anisotropic Behavior of a Pure Titanium Plate. Metals, 14(8), 849. https://doi.org/10.3390/met14080849





