Study of the Thermomechanical Behavior of Single-Crystal and Polycrystal Copper
Abstract
:1. Introduction
2. Materials and Methods
2.1. Theory
2.1.1. Plasticity
2.1.2. Thermomechanics
2.1.3. Constitutive Model
2.2. Computation
2.2.1. Mechanical Tangent Operators
- Compute the fourth-rank tensor :
- Compute the elastic stiffness tensor ( denotes the elastic stiffness tensor in the crystal frame). To calculate the elastic stiffness tensor for the current grain orientation, the rotation tensor from the reference orientation to the current orientation is denoted by , and is used to calculate the elastic stiffness tensor using the expression .
- Use the quantities from steps 1 and 2 to calculate the fourth-rank tensor :
- For each slip system , use the Schmid tensor to compute the fourth-rank tensors and and the second-rank tensor :
- Compute the fourth-rank tensors and , which requires summing over all slip systems:
- Compute the following quantities:The appearing in the computation of represents the rotation components of the relative deformation gradient.
- As the final step, compute the Jacobian for the mechanical behavior of the material:
2.2.2. Thermal Tangent Operators
- : The portion of the mechanical work done on the material that is transformed to heat. It is calculated here as a portion of the plastic work done on the material. It is calculated as , where is the Taylor–Quinney factor, denotes the index for one of 12 slip systems in the metal, is the resolved shear stress on slip system , and is the slip rate on the same slip system.
- The variation of the Cauchy stress with respect to temperature: In this work, we assume that the thermal variation of the Cauchy stress arises from the thermal variation of the elastic moduli of the single crystal. This expression is derived from the definition of the second Piola–Kirchhoff stress:The temperature dependence of the elastic moduli, and consequently of the Cauchy stress, is obtained from Equation (5).
- The variation of with respect to temperature:
- The variation of with respect to the strain:The derivatives of and with respect to are denoted by the symbols and , respectively, and appear in the derivation of the mechanical Jacobian.
2.2.3. Mechanical and Thermal Quantities for the Taylor Polycrystal Model
- Cauchy stress.
- Tangent stiffness moduli in Equation (34).
- Rate of thermal energy generated.
- Derivative of the Cauchy stress with respect to the temperature.
- Derivative of the rate of thermal energy generation with respect to the temperature.
- Derivative of the rate of thermal energy generation with respect to the strain.
2.3. Experiment
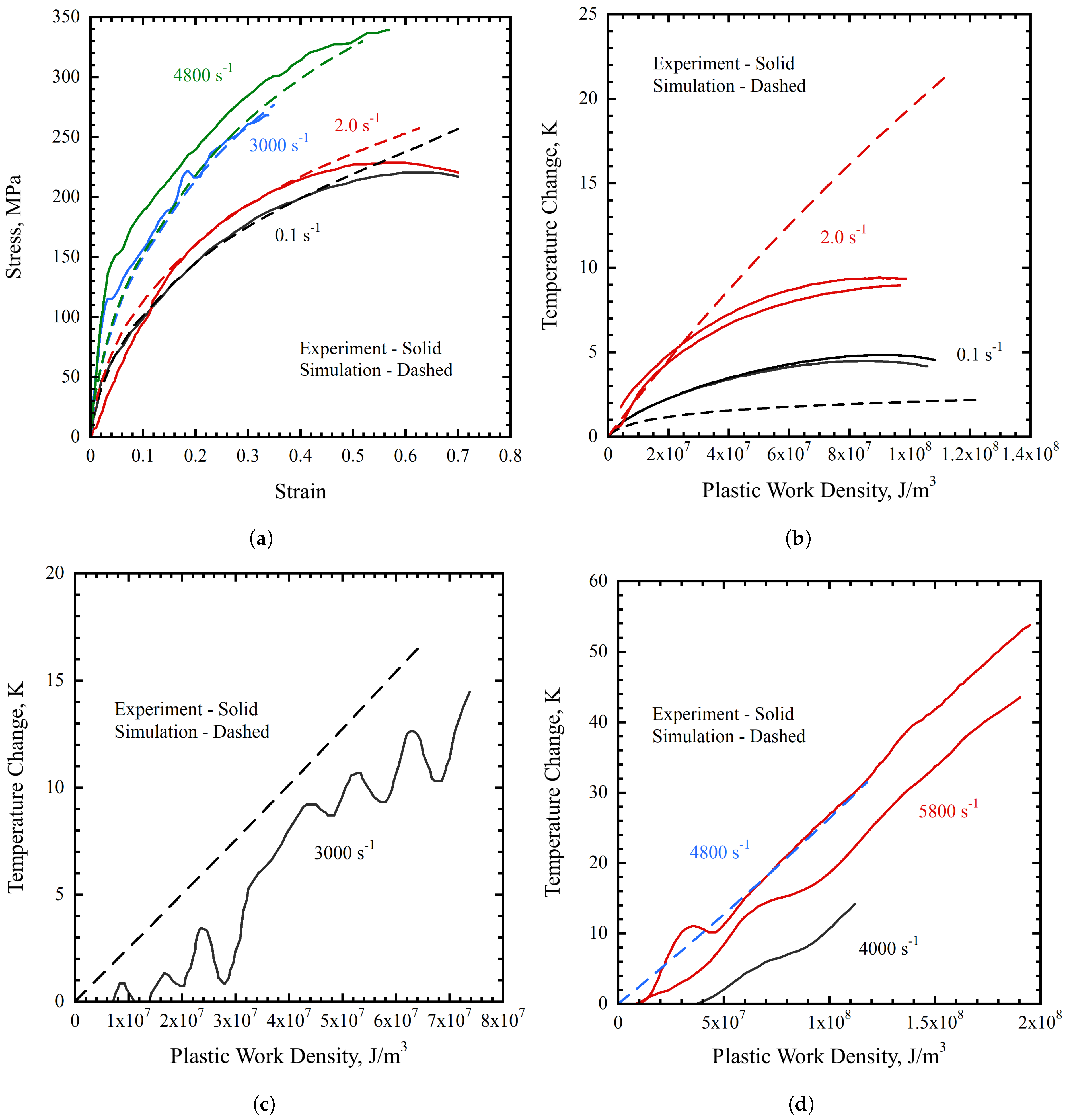
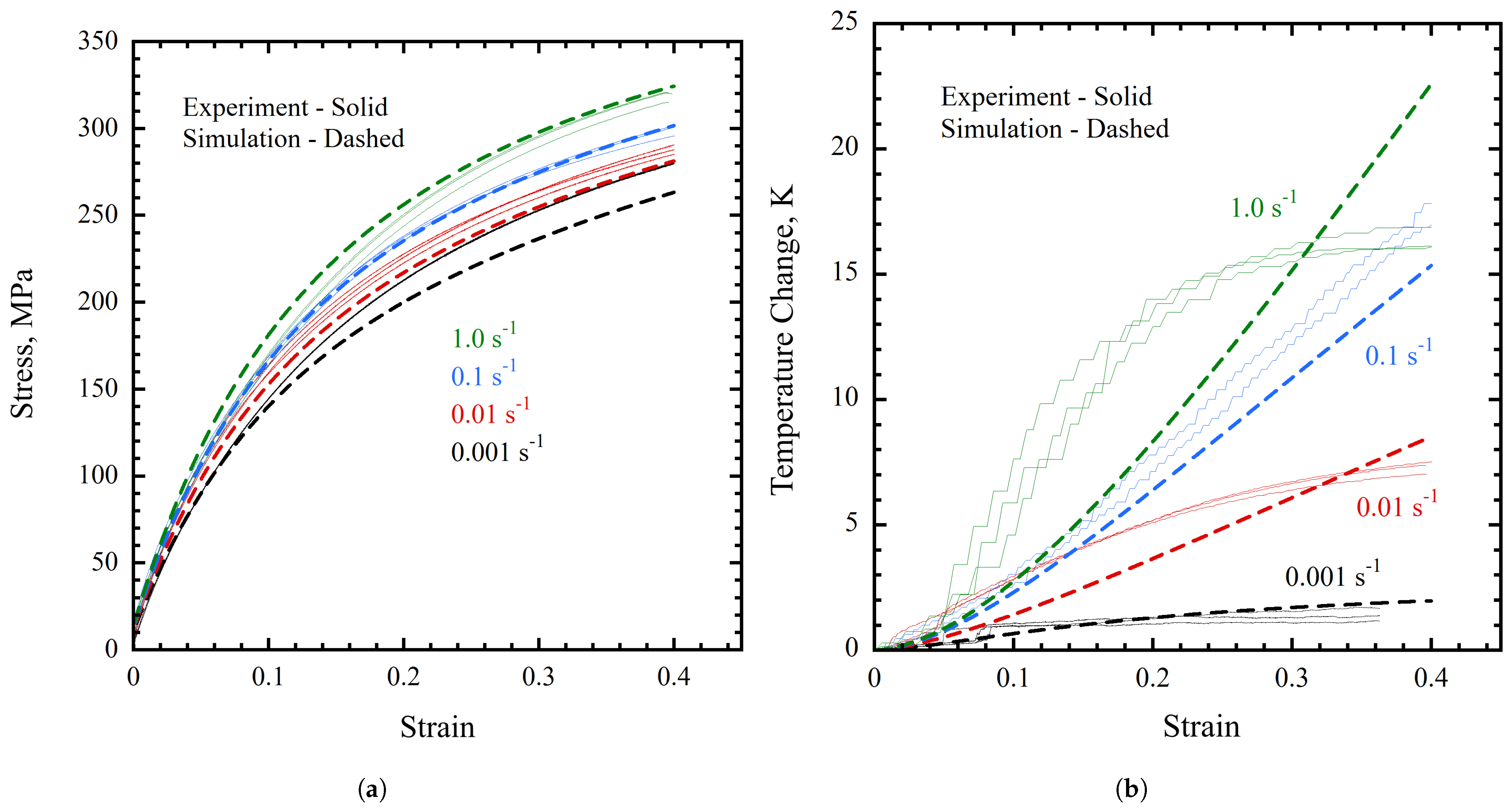
2.3.1. Single-Crystal Experiments
2.3.2. Polycrystal Experiments
2.4. Material Parameter Evaluation
- : Captures the interaction of a dislocation with other dislocations in the same slip system.
- , if and : This corresponds to the dislocation interactions that lead to the formation of dipoles.
- , if and : This corresponds to the formation of a Hirth lock.
- , if and : This corresponds to collinear interactions between dislocations.
- , if and : This corresponds to the formation of a glissile junction.
- for other configurations of slip normals and slip directions: Corresponds to the formation of a Lomer lock.
2.5. Free Surface Heat Transfer Coefficient Parameter Evaluation
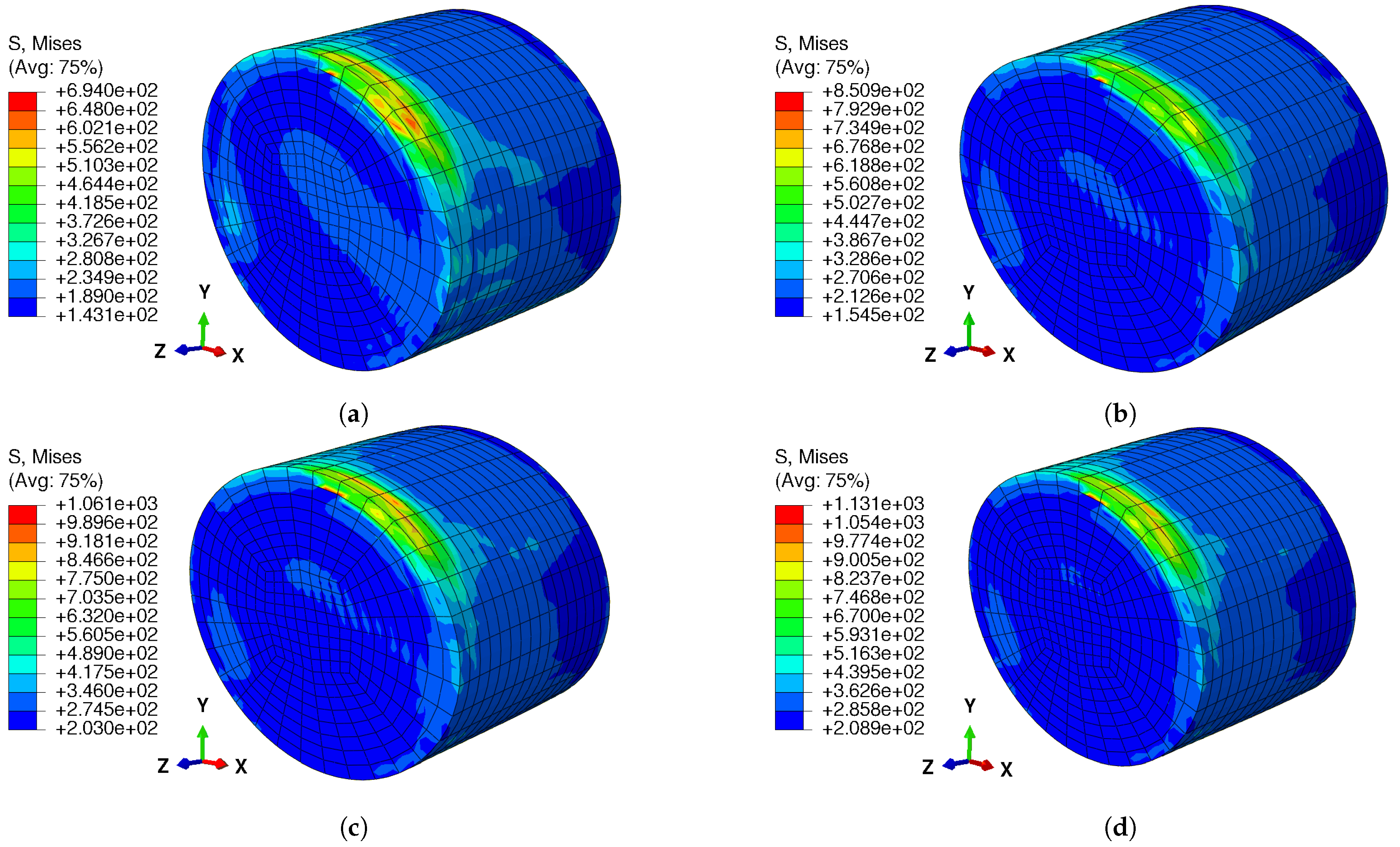
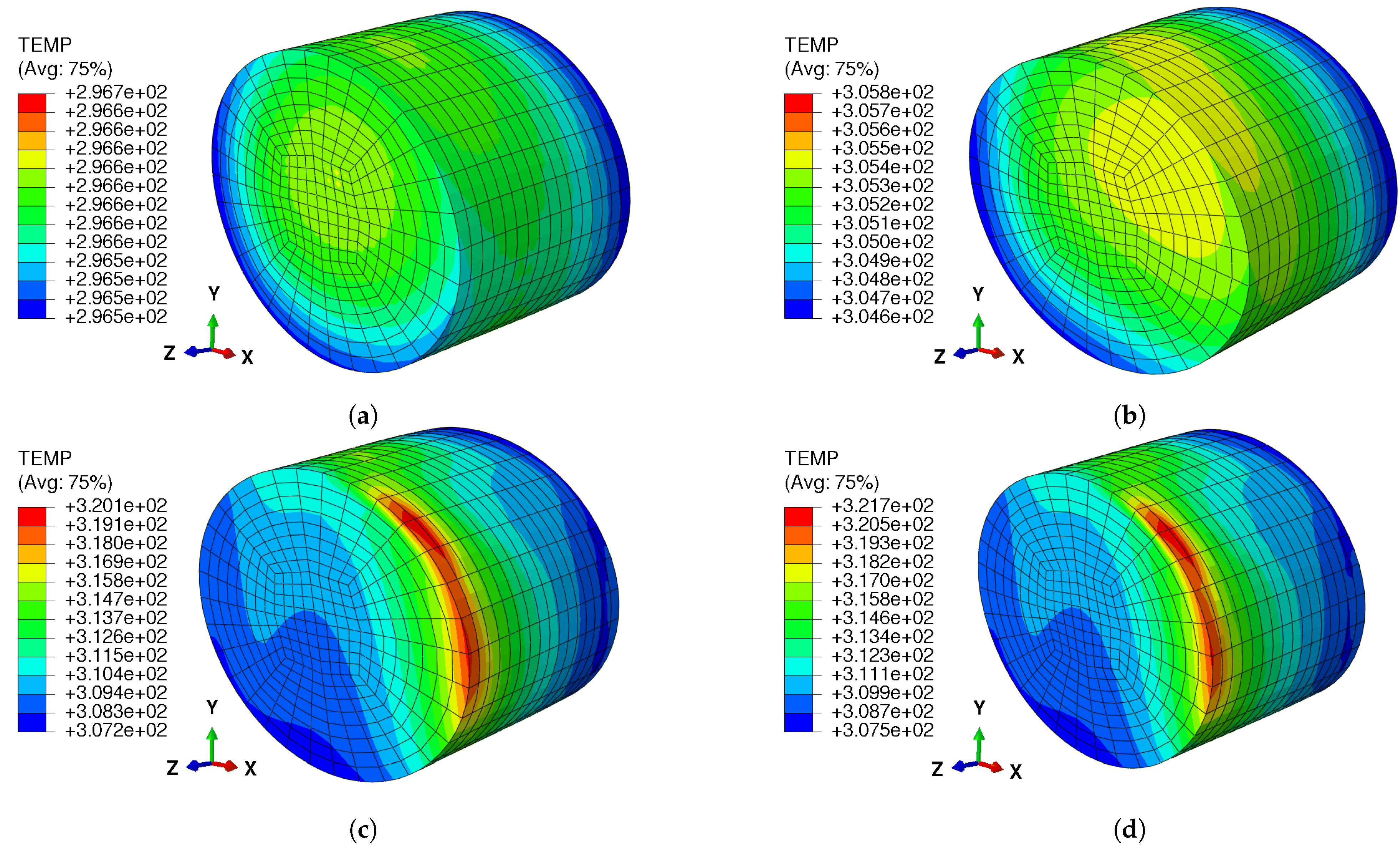
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Taylor, G.I.; Quinney, H. The latent energy remaining in a metal after cold working. Proc. R. Soc. Lond. Ser. A Contain. Pap. A Math. Phys. Character 1934, 143, 307–326. [Google Scholar]
- Farren, W.S.; Taylor, G.I. The heat developed during plastic extension of metals. Proc. R. Soc. Lond. Ser. A Contain. Pap. A Math. Phys. Character 1925, 107, 422–451. [Google Scholar]
- Barton, N.; Benson, D.; Becker, R. Crystal level continuum modelling of phase transformations: The α↔ϵ transformation in iron. Model. Simul. Mater. Sci. Eng. 2005, 13, 707. [Google Scholar] [CrossRef]
- Feng, B.; Bronkhorst, C.A.; Addessio, F.L.; Morrow, B.M.; Li, W.; Lookman, T.; Cerreta, E.K. Coupled nonlinear elasticity, plastic slip, twinning, and phase transformation in single crystal titanium for plate impact loading. J. Mech. Phys. Solids 2019, 127, 358–385. [Google Scholar] [CrossRef]
- Bronkhorst, C.A.; Gray, G.; Addessio, F.L.; Livescu, V.; Bourne, N.; McDonald, S.; Withers, P. Response and representation of ductile damage under varying shock loading conditions in tantalum. J. Appl. Phys. 2016, 119, 085103. [Google Scholar] [CrossRef]
- Mason, J.; Rosakis, A.; Ravichandran, G. On the strain and strain rate dependence of the fraction of plastic work converted to heat: An experimental study using high speed infrared detectors and the Kolsky bar. Mech. Mater. 1994, 17, 135–145. [Google Scholar] [CrossRef]
- Rittel, D.; Wang, Z. Thermo-mechanical aspects of adiabatic shear failure of AM50 and Ti6Al4V alloys. Mech. Mater. 2008, 40, 629–635. [Google Scholar] [CrossRef]
- Benzerga, A.; Bréchet, Y.; Needleman, A.; Van der Giessen, E. The stored energy of cold work: Predictions from discrete dislocation plasticity. Acta Mater. 2005, 53, 4765–4779. [Google Scholar] [CrossRef]
- Rittel, D.; Zhang, L.; Osovski, S. The dependence of the Taylor–Quinney coefficient on the dynamic loading mode. J. Mech. Phys. Solids 2017, 107, 96–114. [Google Scholar] [CrossRef]
- Rittel, D.; Ravichandran, G.; Venkert, A. The mechanical response of pure iron at high strain rates under dominant shear. Mater. Sci. Eng. A 2006, 432, 191–201. [Google Scholar] [CrossRef]
- Rittel, D.; Bhattacharyya, A.; Poon, B.; Zhao, J.; Ravichandran, G. Thermomechanical characterization of pure polycrystalline tantalum. Mater. Sci. Eng. A 2007, 447, 65–70. [Google Scholar] [CrossRef]
- Rittel, D.; Silva, M.; Poon, B.; Ravichandran, G. Thermomechanical behavior of single crystalline tantalum in the static and dynamic regime. Mech. Mater. 2009, 41, 1323–1329. [Google Scholar] [CrossRef]
- Rittel, D.; Kidane, A.; Alkhader, M.; Venkert, A.; Landau, P.; Ravichandran, G. On the dynamically stored energy of cold work in pure single crystal and polycrystalline copper. Acta Mater. 2012, 60, 3719–3728. [Google Scholar] [CrossRef]
- Asaro, R.J.; Rice, J. Strain localization in ductile single crystals. J. Mech. Phys. Solids 1977, 25, 309–338. [Google Scholar] [CrossRef]
- Asaro, R.J. Crystal plasticity. J. Appl. Mech. 1983, 50, 921–934. [Google Scholar] [CrossRef]
- Asaro, R.J. Micromechanics of crystals and polycrystals. Adv. Appl. Mech. 1983, 23, 1–115. [Google Scholar]
- Asaro, R.J.; Needleman, A. Overview no. 42 texture development and strain hardening in rate dependent polycrystals. Acta Metall. 1985, 33, 923–953. [Google Scholar] [CrossRef]
- Bassani, J.L. Plastic flow of crystals. Adv. Appl. Mech. 1993, 30, 191–258. [Google Scholar]
- Bassani, J.L.; Wu, T.Y. Latent hardening in single crystals. II. Analytical characterization and predictions. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1991, 435, 21–41. [Google Scholar]
- Wu, T.Y.; Bassani, J.L.; Laird, C. Latent hardening in single crystals-I. Theory and experiments. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1991, 435, 1–19. [Google Scholar]
- Kalidindi, S.R.; Bronkhorst, C.A.; Anand, L. Crystallographic texture evolution in bulk deformation processing of FCC metals. J. Mech. Phys. Solids 1992, 40, 537–569. [Google Scholar] [CrossRef]
- Bronkhorst, C.A.; Kalidindi, S.; Anand, L. Polycrystalline plasticity and the evolution of crystallographic texture in FCC metals. Philos. Trans. R. Soc. Lond. Ser. A Phys. Eng. Sci. 1992, 341, 443–477. [Google Scholar]
- Harren, S.; Asaro, R. Nonuniform deformations in polycrystals and aspects of the validity of the Taylor model. J. Mech. Phys. Solids 1989, 37, 191–232. [Google Scholar] [CrossRef]
- Harren, S.; Lowe, T.C.; Asaro, R.J.; Needleman, A. Analysis of large-strain shear in rate-dependent face-centred cubic polycrystals: Correlation of micro-and macromechanics. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1989, 328, 443–500. [Google Scholar]
- Acharya, A.; Tang, H.; Saigal, S.; Bassani, J.L. On boundary conditions and plastic strain-gradient discontinuity in lower-order gradient plasticity. J. Mech. Phys. Solids 2004, 52, 1793–1826. [Google Scholar] [CrossRef]
- Aifantis, E.C. On the role of gradients in the localization of deformation and fracture. Int. J. Eng. Sci. 1992, 30, 1279–1299. [Google Scholar] [CrossRef]
- Anand, L.; Gurtin, M.; Lele, S.; Gething, C. A one-dimensional theory of strain-gradient plasticity: Formulation, analysis, numerical results. J. Mech. Phys. Solids 2005, 53, 1789–1826. [Google Scholar] [CrossRef]
- Arsenlis, A.; Parks, D. Crystallographic aspects of geometrically-necessary and statistically-stored dislocation density. Acta Mater. 1999, 47, 1597–1611. [Google Scholar] [CrossRef]
- Busso, E.P.; McClintock, F.A. A dislocation mechanics-based crystallographic model of a B2-type intermetallic alloy. Int. J. Plast. 1996, 12, 1–28. [Google Scholar] [CrossRef]
- Busso, E.; Meissonnier, F.; O’Dowd, N. Gradient-dependent deformation of two-phase single crystals. J. Mech. Phys. Solids 2000, 48, 2333–2361. [Google Scholar] [CrossRef]
- Gerken, J.M.; Dawson, P.R. A crystal plasticity model that incorporates stresses and strains due to slip gradients. J. Mech. Phys. Solids 2008, 56, 1651–1672. [Google Scholar] [CrossRef]
- Gurtin, M.E. On the plasticity of single crystals: Free energy, microforces, plastic-strain gradients. J. Mech. Phys. Solids 2000, 48, 989–1036. [Google Scholar] [CrossRef]
- Gurtin, M.E.; Needleman, A. Boundary conditions in small-deformation, single-crystal plasticity that account for the Burgers vector. J. Mech. Phys. Solids 2005, 53, 1–31. [Google Scholar] [CrossRef]
- Mayeur, J.; Beyerlein, I.; Bronkhorst, C.; Mourad, H. Incorporating interface affected zones into crystal plasticity. Int. J. Plast. 2015, 65, 206–225. [Google Scholar] [CrossRef]
- Zhu, H.; Zbib, H. On the role of strain gradients in adiabatic shear banding. Acta Mech. 1995, 111, 111–124. [Google Scholar] [CrossRef]
- Dequiedt, J.; Denoual, C.; Madec, R. Heterogeneous deformation in ductile FCC single crystals in biaxial stretching: The influence of slip system interactions. J. Mech. Phys. Solids 2015, 83, 301–318. [Google Scholar] [CrossRef]
- Devincre, B.; Kubin, L.; Hoc, T. Physical analyses of crystal plasticity by DD simulations. Scr. Mater. 2006, 54, 741–746. [Google Scholar] [CrossRef]
- Devincre, B.; Hoc, T.; Kubin, L. Dislocation Mean Free Paths and Strain Hardening of Crystals. Science 2008, 320, 1745–1748. [Google Scholar] [CrossRef]
- Grilli, N.; Janssens, K.; Nellessen, J.; Sandlöbes, S.; Raabe, D. Multiple slip dislocation patterning in a dislocation-based crystal plasticity finite element method. Int. J. Plast. 2018, 100, 104–121. [Google Scholar] [CrossRef]
- Hansen, B.; Beyerlein, I.; Bronkhorst, C.; Cerreta, E.; Dennis-Koller, D. A dislocation-based multi-rate single crystal plasticity model. Int. J. Plast. 2013, 44, 129–146. [Google Scholar] [CrossRef]
- Hansen, B.; Bronkhorst, C.; Ortiz, M. Dislocation subgrain structures and modeling the plastic hardening of metallic single crystals. Model. Simul. Mater. Sci. Eng. 2010, 18, 055001. [Google Scholar] [CrossRef]
- Lee, S.; Cho, H.; Bronkhorst, C.A.; Pokharel, R.; Brown, D.W.; Clausen, B.; Vogel, S.C.; Anghel, V.; Gray, G.T.; Mayeur, J.R. Deformation, dislocation evolution and the non-Schmid effect in body-centered-cubic single- and polycrystal tantalum. Int. J. Plast. 2023, 163, 103529. [Google Scholar] [CrossRef]
- Madec, R.; Kubin, L.P. Dislocation strengthening in FCC metals and in BCC metals at high temperatures. Acta Mater. 2017, 126, 166–173. [Google Scholar] [CrossRef]
- Nguyen, T.; Fensin, S.J.; Luscher, D.J. Dynamic crystal plasticity modeling of single crystal tantalum and validation using Taylor cylinder impact tests. Int. J. Plast. 2021, 139, 102940. [Google Scholar] [CrossRef]
- Butler, B.G.; Paramore, J.D.; Ligda, J.P.; Ren, C.; Fang, Z.Z.; Middlemas, S.C.; Hemker, K.J. Mechanisms of deformation and ductility in tungsten—A review. Int. J. Refract. Met. Hard Mater. 2018, 75, 248–261. [Google Scholar] [CrossRef]
- Feng, B.; Bronkhorst, C.; Addessio, F.; Morrow, B.; Cerreta, E.; Lookman, T.; Lebensohn, R.; Low, T. Coupled elasticity, plastic slip, and twinning in single crystal titanium loaded by split-Hopkinson pressure bar. J. Mech. Phys. Solids 2018, 119, 274–297. [Google Scholar] [CrossRef]
- Acharya, A. New inroads in an old subject: Plasticity, from around the atomic to the macroscopic scale. J. Mech. Phys. Solids 2010, 58, 766–778. [Google Scholar] [CrossRef]
- Anand, L.; Gurtin, M.E.; Reddy, B.D. The stored energy of cold work, thermal annealing, and other thermodynamic issues in single crystal plasticity at small length scales. Int. J. Plast. 2015, 64, 1–25. [Google Scholar] [CrossRef]
- Arora, R.; Acharya, A. Dislocation pattern formation in finite deformation crystal plasticity. Int. J. Solids Struct. 2020, 184, 114–135. [Google Scholar] [CrossRef]
- Berdichevsky, V. Why is classical thermodynamics insufficient for solids? In Proceedings of the 2018 AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Kissimmee, FL, USA, 8–12 January 2018. [CrossRef]
- Berdichevsky, V. A continuum theory of edge dislocations. J. Mech. Phys. Solids 2017, 106, 95–132. [Google Scholar] [CrossRef]
- Berdichevsky, V. Entropy and temperature of microstructure in crystal plasticity. Int. J. Eng. Sci. 2018, 128, 24–30. [Google Scholar] [CrossRef]
- Berdichevsky, V. Beyond classical thermodynamics: Dislocation-mediated plasticity. J. Mech. Phys. Solids 2019, 129, 83–118. [Google Scholar] [CrossRef]
- del Castillo, P.R.D.; Huang, M. Dislocation annihilation in plastic deformation: I. Multiscale irreversible thermodynamics. Acta Mater. 2012, 60, 2606–2614. [Google Scholar] [CrossRef]
- Roy Chowdhury, S.; Roy, D. A non-equilibrium thermodynamic model for viscoplasticity and damage: Two temperatures and a generalized fluctuation relation. Int. J. Plast. 2019, 113, 158–184. [Google Scholar] [CrossRef]
- Hochrainer, T. Thermodynamically consistent continuum dislocation dynamics. J. Mech. Phys. Solids 2016, 88, 12–22. [Google Scholar] [CrossRef]
- Jafari, M.; Jamshidian, M.; Ziaei-Rad, S. A finite-deformation dislocation density-based crystal viscoplasticity constitutive model for calculating the stored deformation energy. Int. J. Mech. Sci. 2017, 128–129, 486–498. [Google Scholar] [CrossRef]
- Jiang, M.; Devincre, B.; Monnet, G. Effects of the grain size and shape on the flow stress: A dislocation dynamics study. Int. J. Plast. 2019, 113, 111–124. [Google Scholar] [CrossRef]
- Langer, J.; Bouchbinder, E.; Lookman, T. Thermodynamic theory of dislocation-mediated plasticity. Acta Mater. 2010, 58, 3718–3732. [Google Scholar] [CrossRef]
- Langer, J.S. Statistical thermodynamics of strain hardening in polycrystalline solids. Phys. Rev. E 2015, 92, 032125. [Google Scholar] [CrossRef]
- Le, K.; Tran, T.; Langer, J. Thermodynamic dislocation theory of adiabatic shear banding in steel. Scr. Mater. 2018, 149, 62–65. [Google Scholar] [CrossRef]
- Le, K. Thermodynamic dislocation theory for non-uniform plastic deformations. J. Mech. Phys. Solids 2018, 111, 157–169. [Google Scholar] [CrossRef]
- Le, K. Thermodynamic dislocation theory: Finite deformations. Int. J. Eng. Sci. 2019, 139, 1–10. [Google Scholar] [CrossRef]
- Le, K.C.; Tran, T.M.; Langer, J.S. Thermodynamic dislocation theory of high-temperature deformation in aluminum and steel. Phys. Rev. E 2017, 96, 013004. [Google Scholar] [CrossRef] [PubMed]
- Levitas, V.I.; Javanbakht, M. Thermodynamically consistent phase field approach to dislocation evolution at small and large strains. J. Mech. Phys. Solids 2015, 82, 345–366. [Google Scholar] [CrossRef]
- Nieto-Fuentes, J.; Rittel, D.; Osovski, S. On a dislocation-based constitutive model and dynamic thermomechanical considerations. Int. J. Plast. 2018, 108, 55–69. [Google Scholar] [CrossRef]
- Po, G.; Huang, Y.; Ghoniem, N. A continuum dislocation-based model of wedge microindentation of single crystals. Int. J. Plast. 2019, 114, 72–86. [Google Scholar] [CrossRef]
- Roy, A.; Acharya, A. Finite element approximation of field dislocation mechanics. J. Mech. Phys. Solids 2005, 53, 143–170. [Google Scholar] [CrossRef]
- Roy, A.; Acharya, A. Size effects and idealized dislocation microstructure at small scales: Predictions of a Phenomenological model of Mesoscopic Field Dislocation Mechanics: Part II. J. Mech. Phys. Solids 2006, 54, 1711–1743. [Google Scholar] [CrossRef]
- Shizawa, K.; Kikuchi, K.; Zbib, H.M. A strain-gradient thermodynamic theory of plasticity based on dislocation density and incompatibility tensors. Mater. Sci. Eng. A 2001, 309-310, 416–419. [Google Scholar] [CrossRef]
- Clayton, J. Nonlinear thermomechanics for analysis of weak shock profile data in ductile polycrystals. J. Mech. Phys. Solids 2019, 124, 714–757. [Google Scholar] [CrossRef]
- Clayton, J.D. Nonlinear Mechanics of Crystals; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010; Volume 177. [Google Scholar]
- Langer, J.S. Thermal effects in dislocation theory. Phys. Rev. E 2016, 94, 063004. [Google Scholar] [CrossRef] [PubMed]
- Langer, J. Statistical thermodynamics of dislocations in solids. Adv. Phys. 2021, 70, 445–467. [Google Scholar] [CrossRef]
- Lieou, C.K.; Bronkhorst, C.A. Dynamic recrystallization in adiabatic shear banding: Effective-temperature model and comparison to experiments in ultrafine-grained titanium. Int. J. Plast. 2018, 111, 107–121. [Google Scholar] [CrossRef]
- Lieou, C.K.; Mourad, H.M.; Bronkhorst, C.A. Strain localization and dynamic recrystallization in polycrystalline metals: Thermodynamic theory and simulation framework. Int. J. Plast. 2019, 119, 171–187. [Google Scholar] [CrossRef]
- Lieou, C.K.; Bronkhorst, C.A. Thermomechanical conversion in metals: Dislocation plasticity model evaluation of the Taylor-Quinney coefficient. Acta Mater. 2021, 202, 170–180. [Google Scholar] [CrossRef]
- Lieou, C.K.; Bronkhorst, C.A. Thermodynamic theory of crystal plasticity: Formulation and application to polycrystal fcc copper. J. Mech. Phys. Solids 2020, 138, 103905. [Google Scholar] [CrossRef]
- Noll, W.; Coleman, B.D.; Noll, W. The thermodynamics of elastic materials with heat conduction and viscosity. In The Foundations of Mechanics and Thermodynamics: Selected Papers; Springer: Berlin/Heidelberg, Germany, 1974; pp. 145–156. [Google Scholar]
- Kubin, L.; Devincre, B.; Hoc, T. Modeling dislocation storage rates and mean free paths in face-centered cubic crystals. Acta Mater. 2008, 56, 6040–6049. [Google Scholar] [CrossRef]
- Bronkhorst, C.; Hansen, B.; Cerreta, E.; Bingert, J. Modeling the microstructural evolution of metallic polycrystalline materials under localization conditions. J. Mech. Phys. Solids 2007, 55, 2351–2383. [Google Scholar] [CrossRef]
- Hansen, B.; Carpenter, J.; Sintay, S.; Bronkhorst, C.; McCabe, R.; Mayeur, J.; Mourad, H.; Beyerlein, I.; Mara, N.; Chen, S.; et al. Modeling the texture evolution of Cu/Nb layered composites during rolling. Int. J. Plast. 2013, 49, 71–84. [Google Scholar] [CrossRef]
- Mayeur, J.; Beyerlein, I.; Bronkhorst, C.; Mourad, H.; Hansen, B. A crystal plasticity study of heterophase interface character stability of Cu/Nb bicrystals. Int. J. Plast. 2013, 48, 72–91. [Google Scholar] [CrossRef]
- Kocks, U. Laws for work-hardening and low-temperature creep. J. Eng. Mater. Technol. 1976, 98, 76–85. [Google Scholar] [CrossRef]
- Balasubramanian, S. Polycrystalline Plasticity: Application to Deformation Processing of Lightweight Metals. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1998. [Google Scholar]
- Simmons, G.; Wang, H. Single Crystal Elastic Constants and Calculated Aggregate Properties: A Handbook; The M.I.T. Press: Cambridge, MA, USA, 1971. [Google Scholar]
- Madec, R.; Devincre, B.; Kubin, L.P. From dislocation junctions to forest hardening. Phys. Rev. Lett. 2002, 89, 255508. [Google Scholar] [CrossRef] [PubMed]
- Madec, R.; Devincre, B.; Kubin, L.; Hoc, T.; Rodney, D. The role of collinear interaction in dislocation-induced hardening. Science 2003, 301, 1879–1882. [Google Scholar] [CrossRef] [PubMed]
- Awbi, H.B. Calculation of convective heat transfer coefficients of room surfaces for natural convection. Energy Build. 1998, 28, 219–227. [Google Scholar] [CrossRef]
- Owen, C.J.; Naghdi, A.D.; Johansson, A.; Massa, D.; Papanikolaou, S.; Kozinsky, B. Unbiased Atomistic Predictions of Crystal Dislocation Dynamics using Bayesian Force Fields. arXiv 2024, arXiv:2401.04359. [Google Scholar]
- Fan, Y.; Kushima, A.; Yip, S.; Yildiz, B. Mechanism of Void Nucleation and Growth in bcc Fe: Atomistic Simulations at Experimental Time Scales. Phys. Rev. Lett. 2011, 106, 125501. [Google Scholar] [CrossRef]
- Fan, Y.; Yip, S.; Yildiz, B. Autonomous basin climbing method with sampling of multiple transition pathways: Application to anisotropic diffusion of point defects in hcp Zr. J. Phys. Condens. Matter 2014, 26, 365402. [Google Scholar] [CrossRef]
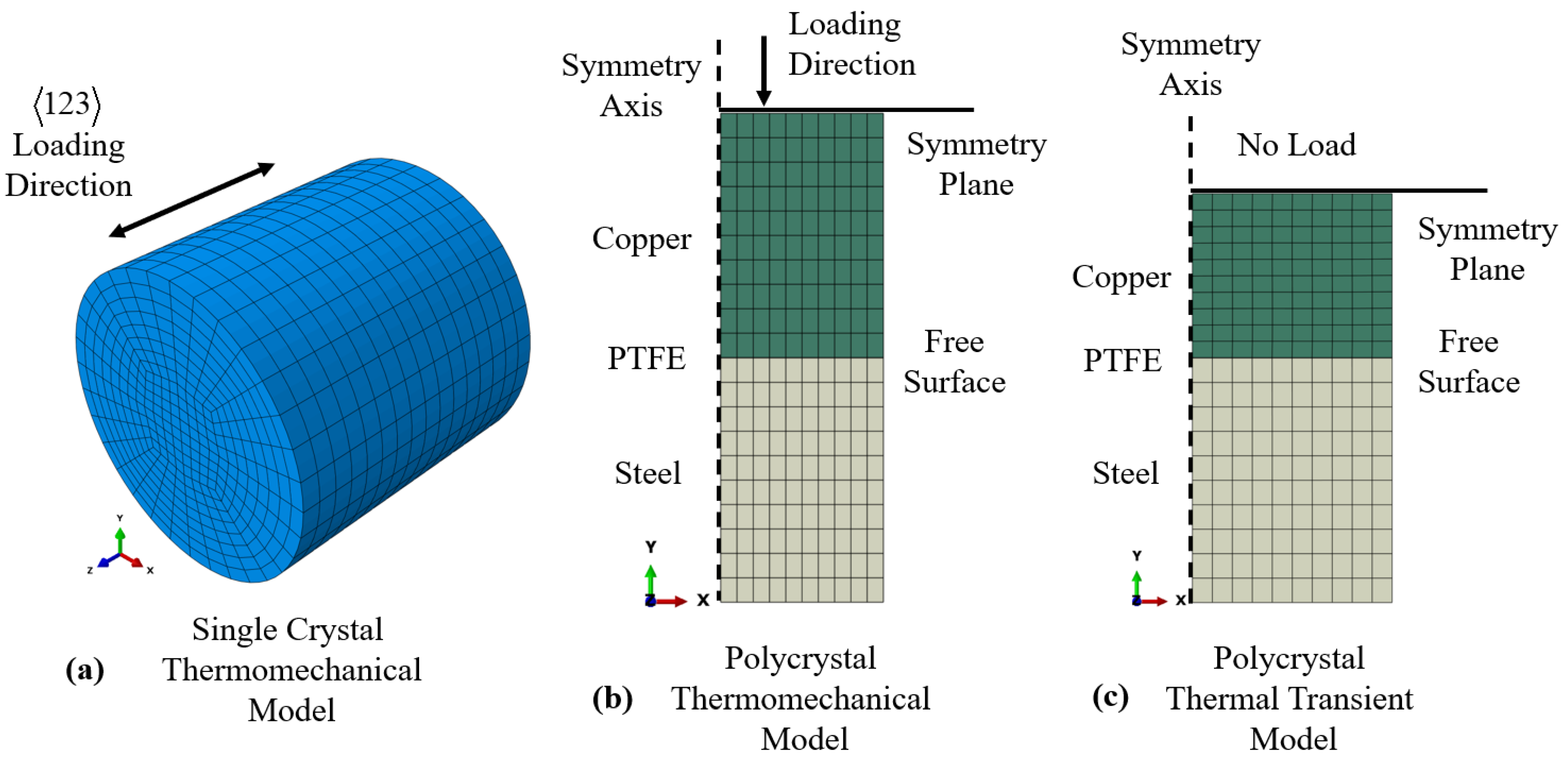
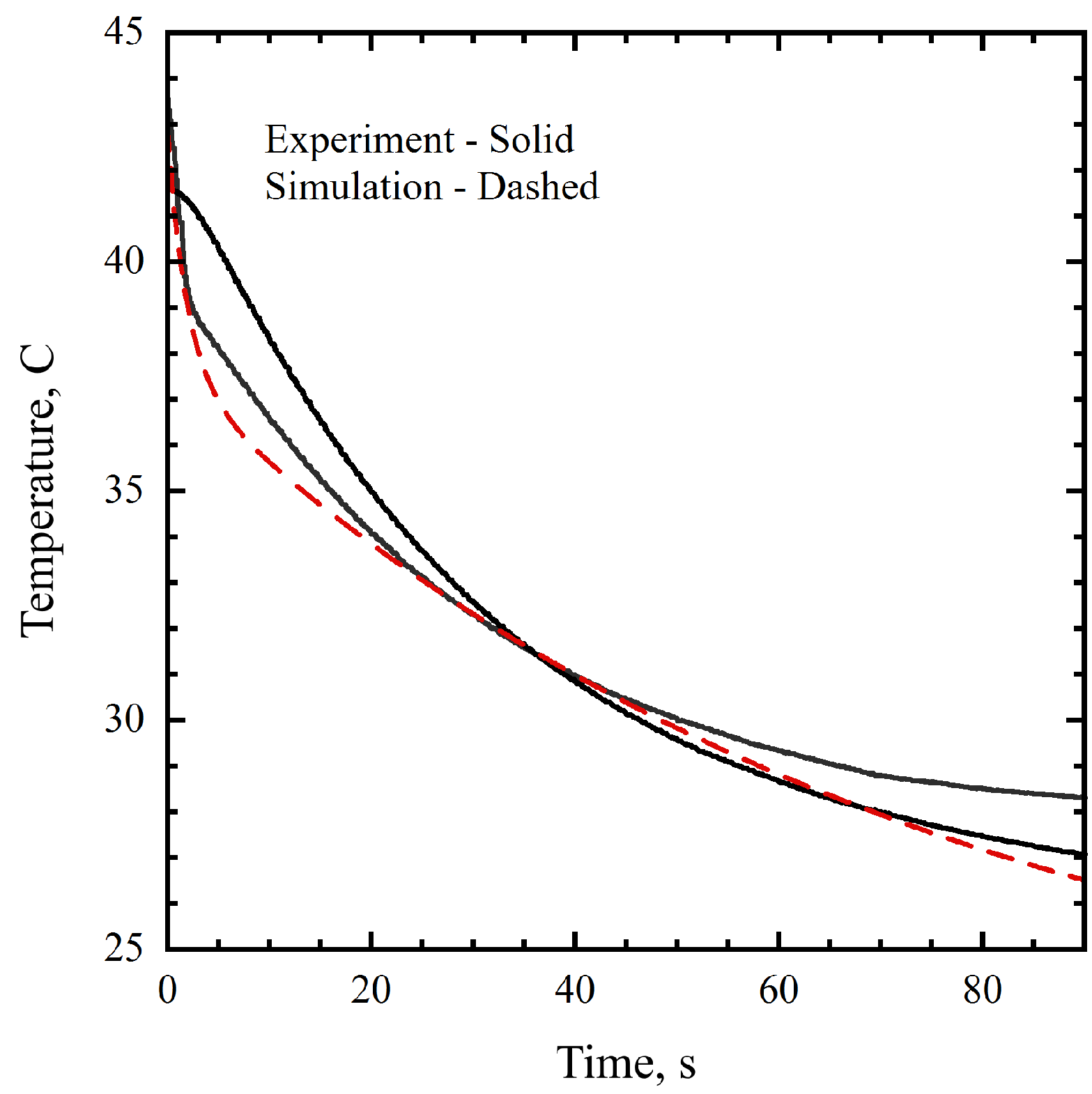

| Type of Quantity | Description of Symbol | Direct Notation | Indicial Notation |
|---|---|---|---|
| Scalars | Italicized small/cap letters without subscripts or superscripts | a, b, c, A, B, C | a, b, c, A, B, C |
| Matrices or Vectors | Bold upright letters, using capital letters for vectors in the reference configuration and small letters for vectors in the current configuration. | , | , |
| Second order tensors | Bold upright letters with underlines, with capital letters for objects in the reference configuration and and small letters for objects in the current configuration. | , | , |
| Fourth order tensors | Blackboard bold capital letters. |
| Variable Symbol | Definition or Meaning |
|---|---|
| , , | Total, elastic, and plastic deformation gradients |
| , , | Total, elastic, and plastic velocity gradients |
| Resolved plastic strain rate on slip system | |
| , | Unit slip direction vector and normal to slip system |
| Cauchy stress tensor | |
| Second Piola–Kirchhoff stress tensor | |
| Anisotropic fourth-order tensor of elastic constants | |
| , , | Independent crystallographic moduli for fcc lattice |
| Shear modulus | |
| Jacobian matrix of stress versus strain | |
| , | Kinetic–vibrational (thermal) energy and entropy density |
| , | Configurational energy and entropy density |
| , | Dislocation energy and entropy |
| , | Residual configurational energy and entropy density |
| Configurational free energy density | |
| Dislocation line energy | |
| , | Configurational and thermal fluxes |
| , | Effective temperature and initial effective temperature |
| , T | Thermal temperature (in units of energy and Kelvin) |
| Dislocation density on slip system | |
| Steady-state dislocation density on slip system | |
| Mean dislocation velocity on slip system | |
| Steady-state effective temperature (in units of ) | |
| Taylor–Quinney factor | |
| Specific heat capacity | |
| Dislocation storage rate | |
| Effective temperature increase rate | |
| a | Minimum separation between dislocations |
| b | Burgers vector |
| Atomic time scale | |
| Stress scale parameter | |
| Resolved shear stress on slip system | |
| Slip resistance due to dislocation interaction on slip system | |
| Intrinsic lattice resistance to dislocation motion | |
| Dislocation density corresponding to slip system | |
| Dislocation mean free path on slip system | |
| Dislocation depinning time on slip system | |
| Dislocation depinning barrier (in units of Kelvin) | |
| Dislocation interaction tensor | |
| Slip interaction tensor | |
| , | Mean free path parameters |
| Material Parameter Symbol | Value |
|---|---|
| 8960 Kg/m3 | |
| 380 J/Kg-K | |
| 394 W/m-K | |
| 179,500 MPa | |
| 126,400 MPa | |
| 82,500 MPa | |
| MPa/K | |
| MPa/K | |
| MPa/K | |
| b | mm |
| mm−2 ( mm−2) | |
| s | |
| 40,800 K | |
| J/K | |
| a | |
| 60 | |
| 15 | |
| 200 | |
| A | J |
| 0 MPa | |
| p | |
| q |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kunda, S.; Schmelzer, N.J.; Pedgaonkar, A.; Rees, J.E.; Dunham, S.D.; Lieou, C.K.C.; Langbaum, J.C.M.; Bronkhorst, C.A. Study of the Thermomechanical Behavior of Single-Crystal and Polycrystal Copper. Metals 2024, 14, 1086. https://doi.org/10.3390/met14091086
Kunda S, Schmelzer NJ, Pedgaonkar A, Rees JE, Dunham SD, Lieou CKC, Langbaum JCM, Bronkhorst CA. Study of the Thermomechanical Behavior of Single-Crystal and Polycrystal Copper. Metals. 2024; 14(9):1086. https://doi.org/10.3390/met14091086
Chicago/Turabian StyleKunda, Sudip, Noah J. Schmelzer, Akhilesh Pedgaonkar, Jack E. Rees, Samuel D. Dunham, Charles K. C. Lieou, Justin C. M. Langbaum, and Curt A. Bronkhorst. 2024. "Study of the Thermomechanical Behavior of Single-Crystal and Polycrystal Copper" Metals 14, no. 9: 1086. https://doi.org/10.3390/met14091086
APA StyleKunda, S., Schmelzer, N. J., Pedgaonkar, A., Rees, J. E., Dunham, S. D., Lieou, C. K. C., Langbaum, J. C. M., & Bronkhorst, C. A. (2024). Study of the Thermomechanical Behavior of Single-Crystal and Polycrystal Copper. Metals, 14(9), 1086. https://doi.org/10.3390/met14091086






