Effects of Interstitial Oxygen Content on Microstructures and Mechanical Properties of TiZrNb Refractory Medium-Entropy Alloy
Abstract
1. Introduction
2. Materials and Methods
2.1. Material Preparation
2.2. Microstructure Characterization
2.3. Mechanical Property Tests
2.4. Theoretical Strengthening Calculation
3. Results and Discussion
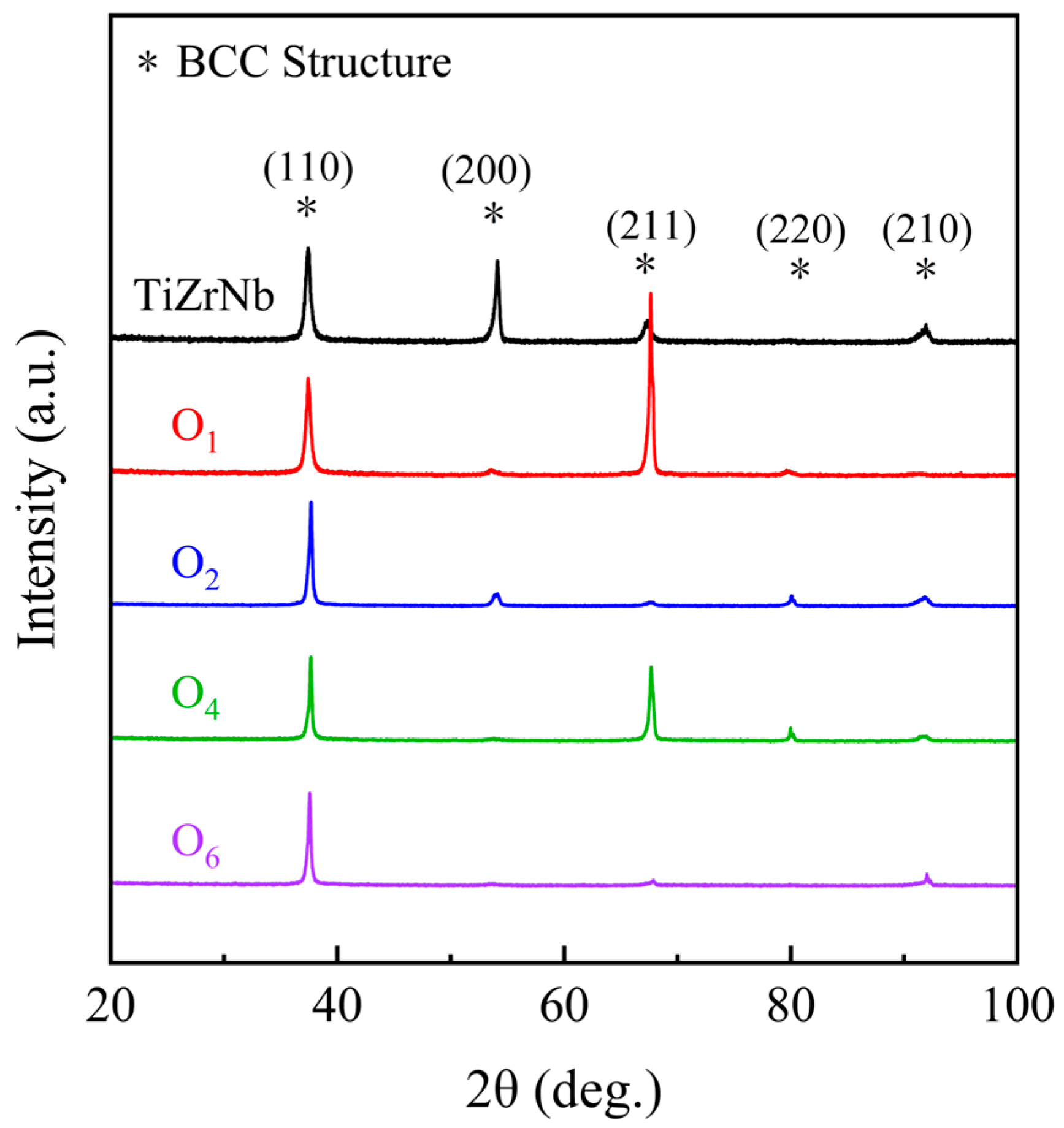
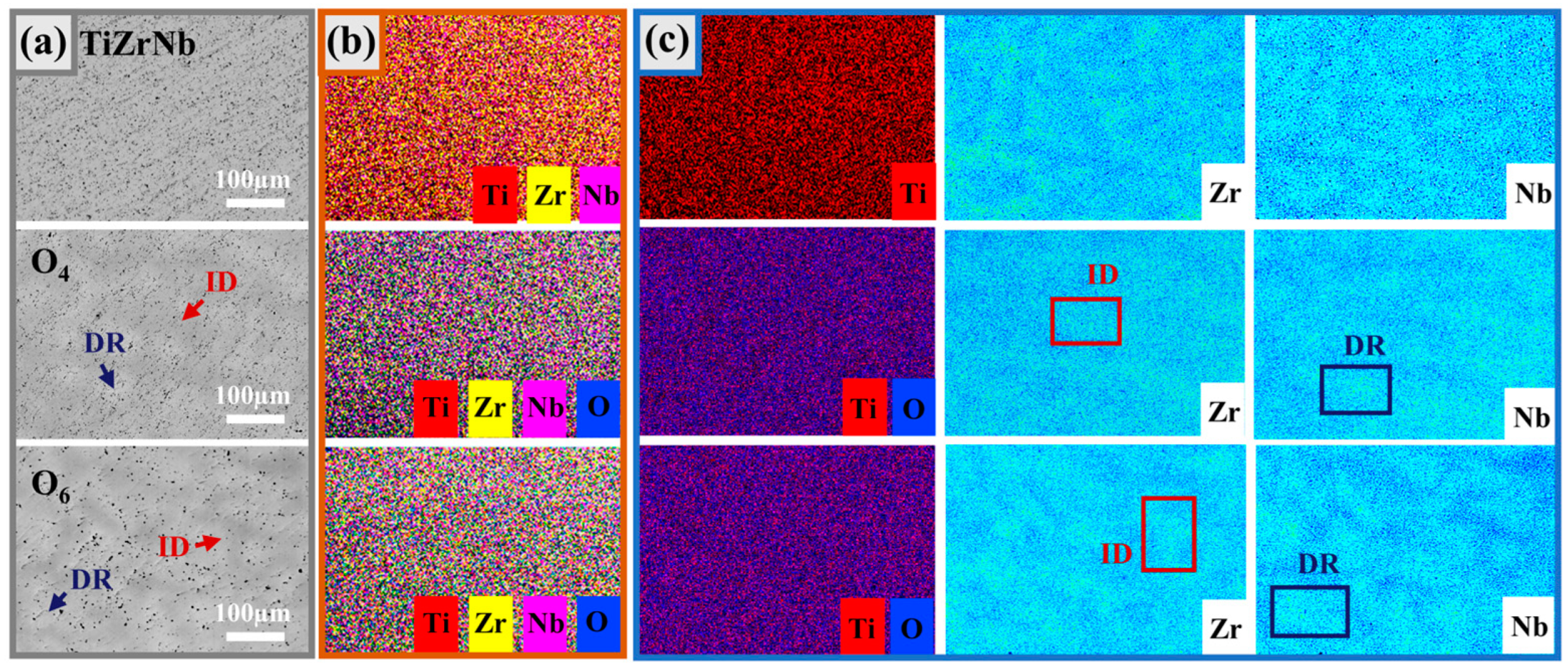
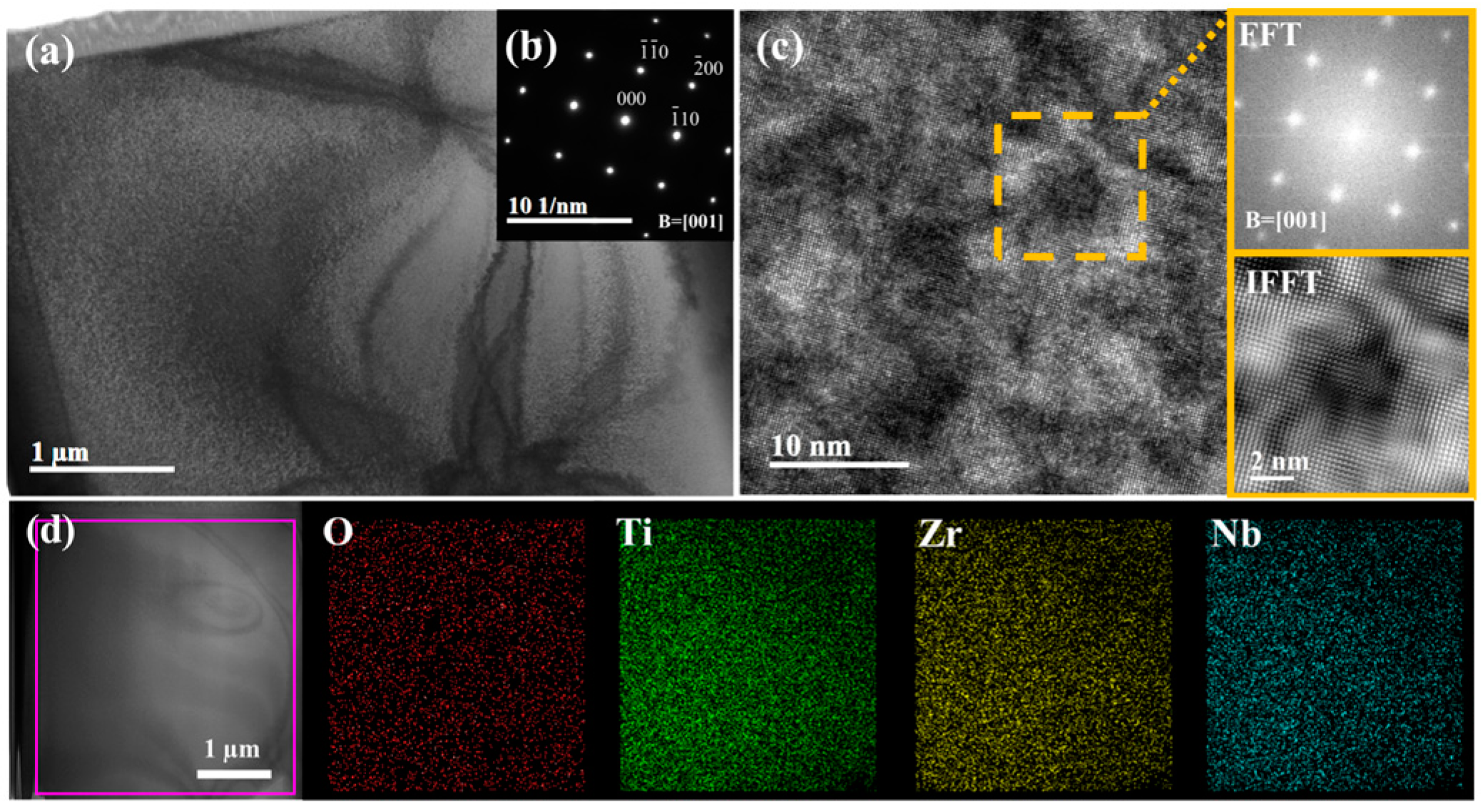
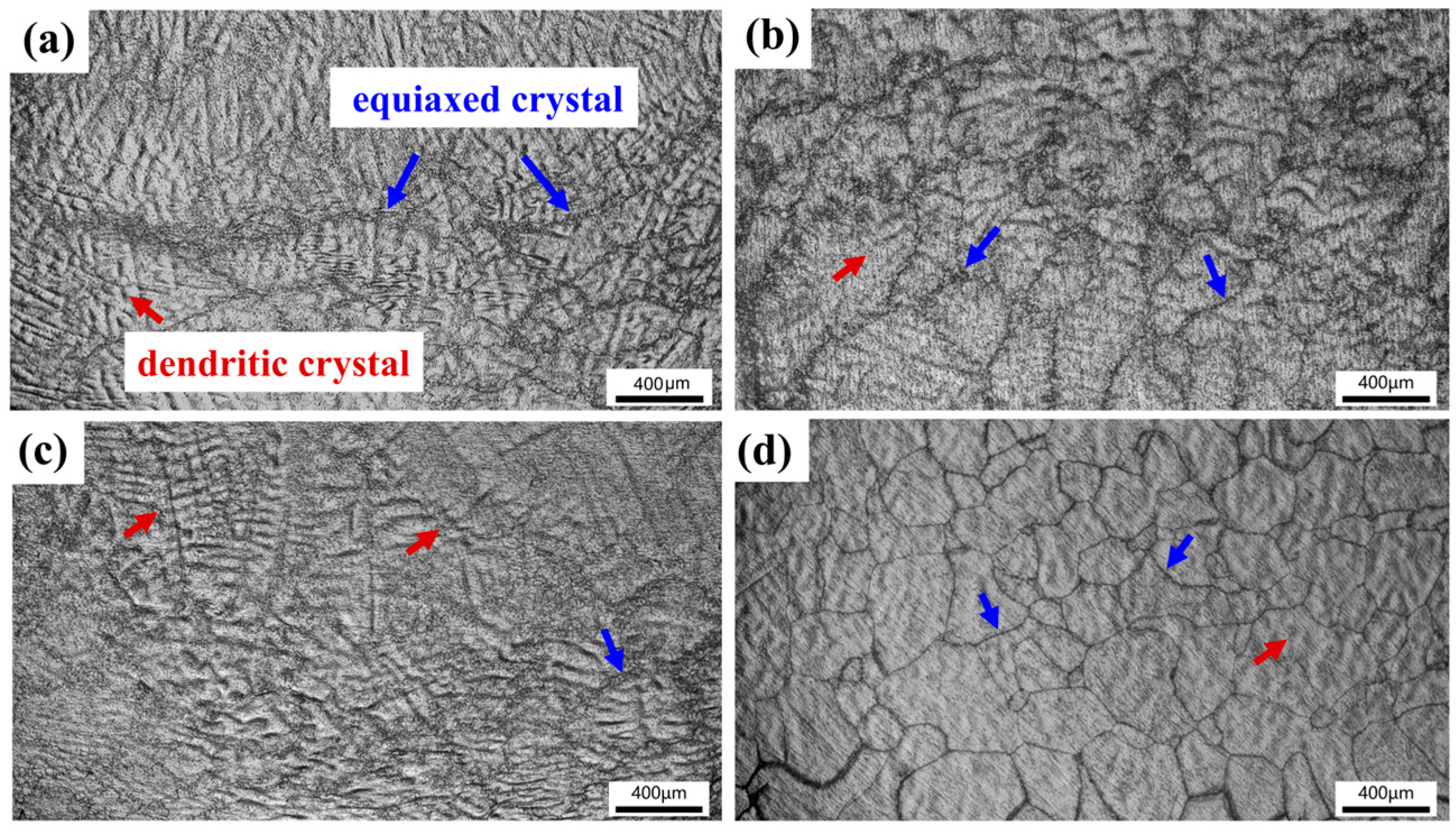
3.1. Microstructures
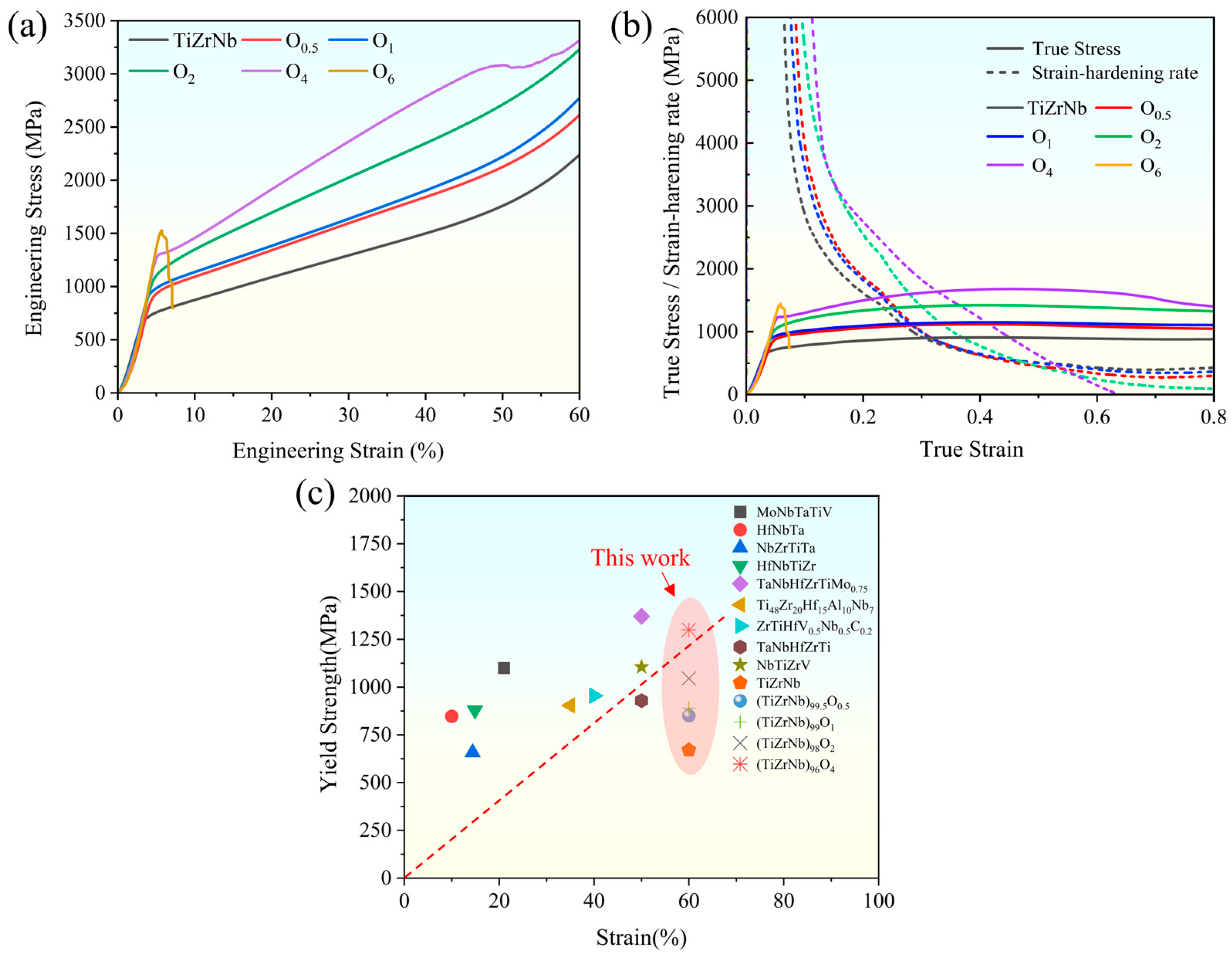
3.2. Room-Temperature Compressive Properties
3.3. High-Temperature Compressive Properties
3.4. Strengthening Mechanisms
4. Conclusions
- (1)
- The alloys display single BCC solid solution structures, the doped oxygen exists as interstitial atoms, and the increase in oxygen content does not induce the change in the crystal structures. The microstructure shows dendritic morphology, with Ti and oxygen elements uniformly distributed in the matrix, Zr slightly enriched in the interdendrites, and Nb slightly enriched in the dendrites. With increasing oxygen content, the average width of the dendritic structures decreases. For O6 alloy, it turns out to be an equiaxed crystal structure.
- (2)
- As oxygen content increases, the yield strength significantly increases from 670 MPa of TiZrNb to 1300 MPa of O4. Among them, O4 MEA shows the optimum mechanical properties, with a room-temperature yield strength of 1300 MPa, high-temperature yield strength of 545 MPa at 800 °C, and elongation at 60%. The enhancement of strength is attributed to the solid solution strengthening induced by interstitial oxygen. Meanwhile, the solid solution of oxygen atoms contributes to a new type of interstitial atom arrangement, which could effectively pin dislocations and alter the dislocation slip mechanism from planar slip to wave slip, thus facilitating more uniform deformation.
- (3)
- The Toda-Caraballo and Labusch models were used to evaluate the contributions of the strengthening effects, and the theoretical values are close to the experimental values. The results indicate that the strengthening mechanism of the alloy systems is dominated by solid solution strengthening, while grain boundary strengthening plays a secondary role. The solid solution of oxygen in the matrix increases lattice distortion, which hinders dislocation movement and effectively enhances the yield strength of the alloy. However, an excessive amount of oxygen can aggravate the internal stress, leading to brittle fracture during compression.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Senkov, O.N.; Wilks, G.B.; Miracle, D.B.; Chuang, C.P.; Liaw, P.K. Refractory High-Entropy Alloys. Intermetallics 2010, 18, 1758–1765. [Google Scholar] [CrossRef]
- Senkov, O.N.; Jensen, J.K.; Pilchak, A.L.; Miracle, D.B.; Fraser, H.L. Compositional Variation Effects on the Microstructure and Properties of a Refractory High-Entropy Superalloy AlMo0.5NbTa0.5TiZr. Mater. Des. 2018, 139, 498–511. [Google Scholar] [CrossRef]
- Chen, W.K.; Li, Y.K.; Chen, Y.W. High Compressibility ZrTiHfV0.5Nb0.5Cx Refractory High-Entropy Alloys. Mater. Sci. Forum 2019, 944, 163–168. [Google Scholar] [CrossRef]
- Maiti, S.; Steurer, W. Structural-Disorder and Its Effect on Mechanical Properties in Single-Phase TaNbHfZr High-Entropy Alloy. Acta Mater. 2016, 106, 87–97. [Google Scholar] [CrossRef]
- Han, Z.D.; Luan, H.W.; Liu, X.; Chen, N.; Li, X.Y.; Shao, Y.; Yao, K.F. Microstructures and Mechanical Properties of TiNbMoTaW Refractory High-Entropy Alloys. Mater. Sci. Eng. A 2018, 712, 380–385. [Google Scholar] [CrossRef]
- Huang, T.; Wu, S.; Jiang, H.; Lu, Y.; Wang, T.; Li, T. Effect of Ti Content on Microstructure and Properties of TixZrVNb Refractory High-Entropy Alloys. Int. J. Miner. Metall. Mater. 2020, 27, 1318–1325. [Google Scholar] [CrossRef]
- Ge, S.; Fu, H.; Zhang, L.; Mao, H.; Li, H.; Wang, A.; Li, W.; Zhang, H. Effects of Al Addition on the Microstructures and Properties of MoNbTaTiV Refractory High Entropy Alloy. Mater. Sci. Eng. A 2020, 784, 139275. [Google Scholar] [CrossRef]
- Senkov, O.N.; Wilks, G.B.; Scott, J.M.; Miracle, D.B. Mechanical Properties of Nb25Mo25Ta25W25 and V20Nb20Mo20Ta20W20 Refractory High Entropy Alloys. Intermetallics 2011, 19, 698–706. [Google Scholar] [CrossRef]
- Guo, N.N.; Wang, L.; Luo, L.S.; Li, X.Z.; Su, Y.Q.; Guo, J.J.; Fu, H.Z. Microstructure and Mechanical Properties of Refractory MoNbHfZrTi High-Entropy Alloy. Mater. Des. 2015, 81, 87–94. [Google Scholar] [CrossRef]
- Li, Q.; Zhang, H.; Li, D.; Chen, Z.; Huang, S.; Lu, Z.; Yan, H. WxNbMoTa Refractory High-Entropy Alloys Fabricated by Laser Cladding Deposition. Materials 2019, 12, 533. [Google Scholar] [CrossRef]
- Wang, S.-P.; Xu, J. TiZrNbTaMo High-Entropy Alloy Designed for Orthopedic Implants: As-Cast Microstructure and Mechanical Properties. Mater. Sci. Eng. C 2017, 73, 80–89. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.; Du, C.; Yu, Z.; Wang, R.; Ren, X. Effect of Cu Content on the Microstructure and Mechanical Properties of Fe20Co30Ni10Cr20Mn20 FCC-Typed HEAs. Mater. Sci. Eng. A 2024, 897, 146336. [Google Scholar] [CrossRef]
- Gao, L.; Wu, Y.; An, N.; Chen, J.; Liu, X.; Bai, R.; Hui, X. Nanoscale L12 Phase Precipitation Induced Superb Ambient and High Temperature Mechanical Properties in Ni–Co–Cr–Al System High-Entropy Superalloys. Mater. Sci. Eng. A 2024, 898, 145995. [Google Scholar] [CrossRef]
- Yuan, P.; Wang, L.; Liu, Y.; Hui, X. Abnormal Effect of Al on the Phase Stability and Deformation Mechanism of Ti-Zr-Hf-Al Medium-Entropy Alloys. Metals 2024, 14, 1035. [Google Scholar] [CrossRef]
- An, K.; Yang, T.; Feng, J.; Deng, H.; Zhang, X.; Zhao, Z.; Meng, Q.; Qi, J.; Wei, F.; Sui, Y. Influence of Al and Ti Alloying and Annealing on the Microstructure and Compressive Properties of Cr-Fe-Ni Multi-Principal Element Alloy. Metals 2024, 14, 1223. [Google Scholar] [CrossRef]
- Xiong, W.; Guo, A.X.Y.; Zhan, S.; Liu, C.T.; Cao, S.C. Refractory High-Entropy Alloys: A Focused Review of Preparation Methods and Properties. J. Mater. Sci. Technol. 2023, 142, 196–215. [Google Scholar] [CrossRef]
- Senkov, O.N.; Scott, J.M.; Senkova, S.V.; Miracle, D.B.; Woodward, C.F. Microstructure and Room Temperature Properties of a High-Entropy TaNbHfZrTi Alloy. J. Alloys Compd. 2011, 509, 6043–6048. [Google Scholar] [CrossRef]
- Lei, Z.; Liu, X.; Wu, Y.; Wang, H.; Jiang, S.; Wang, S.; Hui, X.; Wu, Y.; Gault, B.; Kontis, P.; et al. Enhanced Strength and Ductility in a High-Entropy Alloy via Ordered Oxygen Complexes. Nature 2018, 563, 546–550. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Li, Y.; Cheng, X.; Xu, Z.; Wu, C.; Cheng, B.; Wang, M. Interstitial Strengthening of Refractory ZrTiHfNb0.5Ta0.5Ox (x = 0.05, 0.1, 0.2) High-Entropy Alloys. Mater. Lett. 2018, 228, 145–147. [Google Scholar] [CrossRef]
- Wang, R.; Tang, Y.; Lei, Z.; Ai, Y.; Tong, Z.; Li, S.; Ye, Y.; Bai, S. Achieving High Strength and Ductility in Nitrogen-Doped Refractory High-Entropy Alloys. Mater. Des. 2022, 213, 110356. [Google Scholar] [CrossRef]
- Pande, C.S.; Cooper, K.P. Nanomechanics of Hall–Petch Relationship in Nanocrystalline Materials. Prog. Mater. Sci. 2009, 54, 689–706. [Google Scholar] [CrossRef]
- Lai, W.; Liu, H.; Yu, X.; Yi, Y.; Li, W.; Zhou, S.; Cui, S.; Wang, X. A Design of TiZr-Rich Body-Centered Cubic Structured Multi-Principal Element Alloys with Outstanding Tensile Strength and Ductility. Mater. Sci. Eng. A 2021, 813, 141135. [Google Scholar] [CrossRef]
- Jin, C.; Li, X.; Kang, J.; Li, H.; Wang, H. Effect of Interstitial Oxygen /Nitrogen on Mechanical and Wear Properties of TiZrHfNb Refractory High-Entropy Alloy. J. Alloys Compd. 2023, 960, 170863. [Google Scholar] [CrossRef]
- Cordero, Z.C.; Knight, B.E.; Schuh, C.A. Six Decades of the Hall–Petch Effect—A Survey of Grain-Size Strengthening Studies on Pure Metals. Int. Mater. Rev 2016, 61, 495–512. [Google Scholar] [CrossRef]
- Li, L.; Fang, Q.; Li, J.; Liu, B.; Liu, Y.; Liaw, P.K. Lattice-Distortion Dependent Yield Strength in High Entropy Alloys. Mater. Sci. Eng. A 2020, 784, 139323. [Google Scholar] [CrossRef]
- Yao, H.W.; Qiao, J.W.; Hawk, J.A.; Zhou, H.F.; Chen, M.W.; Gao, M.C. Mechanical Properties of Refractory High-Entropy Alloys: Experiments and Modeling. J. Alloys Compd. 2017, 696, 1139–1150. [Google Scholar] [CrossRef]
- Hu, S.; Li, T.; Su, Z.; Liu, D. Research on Suitable Strength, Elastic Modulus and Abrasion Resistance of Ti–Zr–Nb Medium Entropy Alloys (MEAs) for Implant Adaptation. Intermetallics 2022, 140, 107401. [Google Scholar] [CrossRef]
- Wei, Q.; Wang, L.; Fu, Y.; Qin, J.; Lu, W.; Zhang, D. Influence of Oxygen Content on Microstructure and Mechanical Properties of Ti–Nb–Ta–Zr Alloy. Mater. Des. 2011, 32, 2934–2939. [Google Scholar] [CrossRef]
- Bu, Y.; Wu, Y.; Lei, Z.; Yuan, X.; Wu, H.; Feng, X.; Liu, J.; Ding, J.; Lu, Y.; Wang, H.; et al. Local Chemical Fluctuation Mediated Ductility in Body-Centered-Cubic High-Entropy Alloys. Mater. Today 2021, 46, 28–34. [Google Scholar] [CrossRef]
- Ma, E.; Liu, C. Chemical Inhomogeneities in High-Entropy Alloys Help Mitigate the Strength-Ductility Trade-Off. Prog. Mater. Sci. 2024, 143, 101252. [Google Scholar] [CrossRef]
- Xun, K.; Zhang, B.; Wang, Q.; Zhang, Z.; Ding, J.; Ma, E. Local Chemical Inhomogeneities in TiZrNb-Based Refractory High-Entropy Alloys. J. Mater. Sci. Technol. 2023, 135, 221–230. [Google Scholar] [CrossRef]
- Gu, Z.-H.; Nong, Z.-S.; Wang, H.-Y.; Wang, J.-J. Microstructures, Mechanical and Electrochemical Properties of Monbta-Based Refractory Multi-Component Alloys. JOM 2022, 74, 4344–4351. [Google Scholar] [CrossRef]
- Wang, S.-P.; Ma, E.; Xu, J. New Ternary Equi-Atomic Refractory Medium-Entropy Alloys with Tensile Ductility: Hafnium versus Titanium into NbTa-Based Solution. Intermetallics 2019, 107, 15–23. [Google Scholar] [CrossRef]
- Wu, Y.D.; Cai, Y.H.; Wang, T.; Si, J.J.; Zhu, J.; Wang, Y.D.; Hui, X.D. A Refractory Hf25Nb25Ti25Zr25 High-Entropy Alloy with Excellent Structural Stability and Tensile Properties. Mater. Lett. 2014, 130, 277–280. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, Z.; Yang, H.J.; Qiao, J.W.; Wang, Z.H.; Wu, Y.C. Ultra-High Strain-Rate Strengthening in Ductile Refractory High Entropy Alloys upon Dynamic Loading. Intermetallics 2020, 121, 106699. [Google Scholar] [CrossRef]
- Wang, L.; Fu, C.; Wu, Y.; Li, R.; Wang, Y.; Hui, X. Ductile Ti-Rich High-Entropy Alloy Controlled by Stress Induced Martensitic Transformation and Mechanical Twinning. Mater. Sci. Eng. A 2019, 763, 138147. [Google Scholar] [CrossRef]
- Wang, X.; Hamasaki, H.; Yamamura, M.; Yamauchi, R.; Maeda, T.; Shirai, Y.; Yoshida, F. Yield-Point Phenomena of Ti-20V-4Al-1Sn at 1073 K and Its Constitutive Modelling. Mater. Trans. 2009, 50, 1576–1578. [Google Scholar] [CrossRef]
- Philippart, I.; Rack, H.J. High Temperature Dynamic Yielding in Metastable Ti–6.8Mo–4.5F–1.5Al. Mater. Sci. Eng. A 1998, 243, 196–200. [Google Scholar] [CrossRef]
- Momeni, A.; Abbasi, S.M.; Morakabati, M.; Akhondzadeh, A. Yield Point Phenomena in TIMETAL 125 Beta Ti Alloy. Mater. Sci. Eng. A 2015, 643, 142–148. [Google Scholar] [CrossRef]
- Shi, H.; Li, J.; Mao, J.; Lu, W. The Elimination of the Yield Point Phenomenon in a New Zirconium Alloy: Influence of Degree of Recrystallization on the Tensile Properties. Scr. Mater. 2019, 169, 28–32. [Google Scholar] [CrossRef]
- Senkov, O.N.; Scott, J.M.; Senkova, S.V.; Meisenkothen, F.; Miracle, D.B.; Woodward, C.F. Microstructure and Elevated Temperature Properties of a Refractory TaNbHfZrTi Alloy. J. Mater. Sci. 2012, 47, 4062–4074. [Google Scholar] [CrossRef]
- Xie, J.L.; Liu, Y.Z.; Wu, H.J.; Li, F.X. Hot compression behavior of semi-solid 7050 aluminum alloy. Spec. Cast. Nonferrous Alloys 2011, 31, 816–819. [Google Scholar]
- Hall, E.O. Iron and Its Alloys. In Yield Point Phenomena in Metals and Alloys; Springer: Boston, MA, USA, 1970; pp. 65–126. ISBN 978-1-4684-1860-6. [Google Scholar]
- Ravichandran, N.; Prasad, Y.V.R.K. Influence of Oxygen on Dynamic Recrystallization during Hot Working of Polycrystalline Copper. Mater. Sci. Eng. A 1992, 156, 195–204. [Google Scholar] [CrossRef]
- He, J.Y.; Wang, H.; Huang, H.L.; Xu, X.D.; Chen, M.W.; Wu, Y.; Liu, X.J.; Nieh, T.G.; An, K.; Lu, Z.P. A Precipitation-Hardened High-Entropy Alloy with Outstanding Tensile Properties. Acta Mater. 2016, 102, 187–196. [Google Scholar] [CrossRef]
- Labusch, R. A Statistical Theory of Solid Solution Hardening. Phys. Status Solidi B 1970, 41, 659–669. [Google Scholar] [CrossRef]
- Wu, L.; Yang, X.; Li, T.; Li, L.; Lu, Y. Achieving Superior Mechanical Properties and Biocompatibility in an O-Doping TiZrNb Medium Entropy Alloy. Intermetallics 2023, 161, 107991. [Google Scholar] [CrossRef]
- Li, T.; Miao, J.; Lu, Y.; Wang, T.; Li, T. Effect of Zr on the As-Cast Microstructure and Mechanical Properties of Lightweight Ti2VNbMoZrx Refractory High-Entropy Alloys. Int. J. Refract. Met. Hard Mater. 2022, 103, 105762. [Google Scholar] [CrossRef]


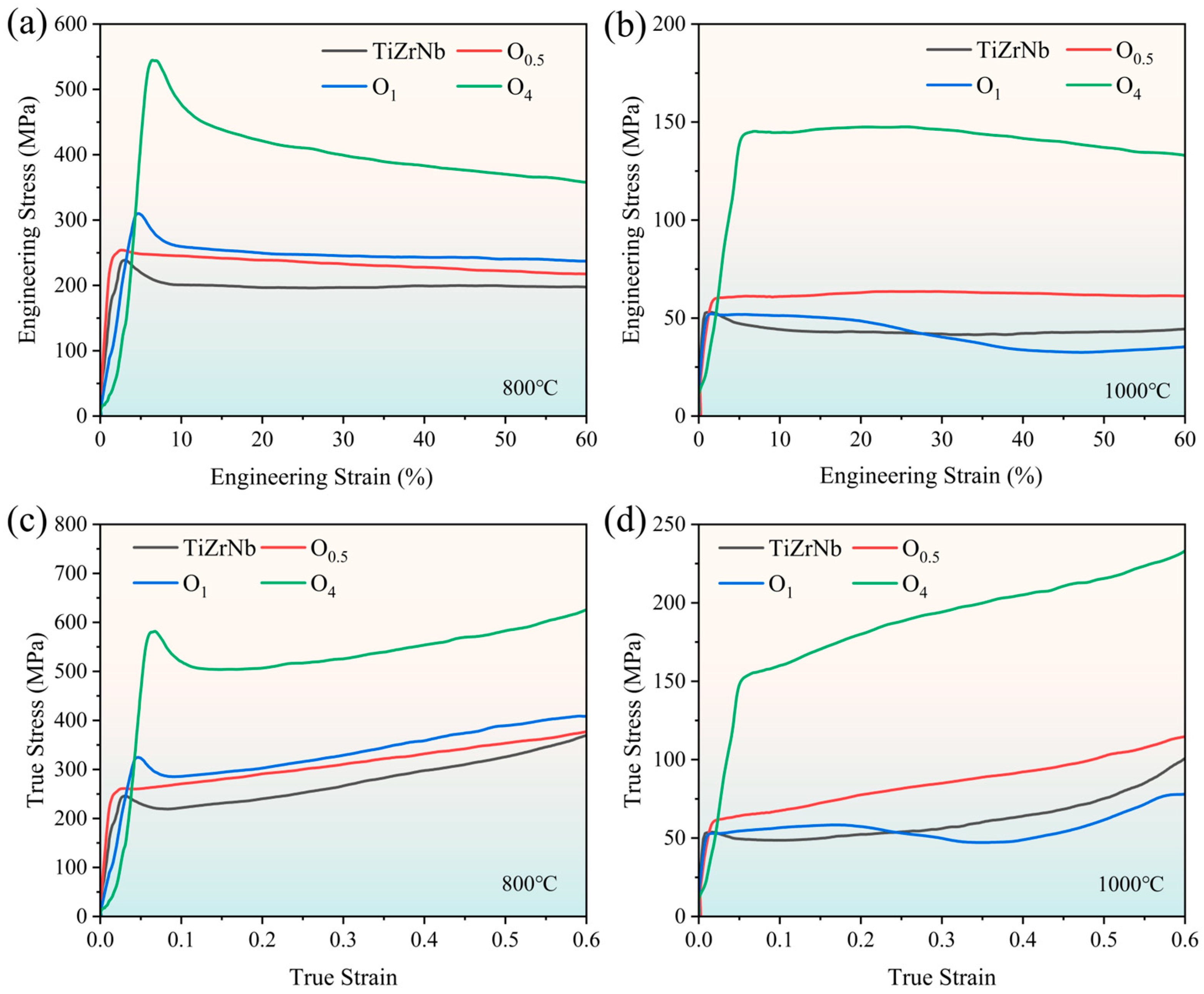

| Alloys | Temperature (°C) | Yield Strength , MPa) | Elongation of Fracture (%) |
|---|---|---|---|
| TiZrNb | 20 | 670 | >60 |
| 800 | 233 | >60 | |
| 1000 | 52 | >60 | |
| O0.5 | 20 | 850 | >60 |
| 800 | 245 | >60 | |
| 1000 | 52 | >60 | |
| O1 | 20 | 890 | >60 |
| 800 | 310 | >60 | |
| 1000 | 60 | >60 | |
| O2 | 20 | 1045 | >60 |
| O4 | 20 | 1300 | >60 |
| 800 | 545 | >60 | |
| 1000 | 143 | >60 | |
| O6 | 20 | 1520 (Ultimate strength) | 5 |
| Alloys | Grain Size , µm) | Grain Boundary Strengthening , MPa) |
|---|---|---|
| TiZrNb | 28.3 | 80.8 |
| O0.5 | 21.9 | 91.9 |
| O1 | 21.5 | 92.7 |
| O2 | 20.2 | 95.7 |
| O4 | 17.5 | 102.8 |
| Element | (GPa) | (pm) | (MPa) | |||
|---|---|---|---|---|---|---|
| Ti | 44 | 147 | 0.257 | 0.078 | 0.691 | 646 |
| Zr | 33 | 160 | 0.260 | 0.086 | 0.718 | 510 |
| Nb | 38 | 146 | 0.146 | 0.081 | 0.529 | 391 |
| Ti-Zr | Zr-Nb | Ti-Nb | |
|---|---|---|---|
| 0.286 | 0.147 | 0.143 | |
| 0.085 | 0.091 | 0.007 |
| Alloys | Experimental Yield Strength , MPa) | Grain Boundary Strengthening , MPa) | Solid Solution Strengthening , MPa) | Theoretical Yield Strength , MPa) |
|---|---|---|---|---|
| TiZrNb | 670 | 80.8 | 516 | 597 |
| O0.5 | 850 | 91.9 | 663 | 755 |
| O1 | 890 | 92.7 | 766 | 847 |
| O2 | 1045 | 95.7 | 913 | 994 |
| O4 | 1300 | 102.8 | 1146 | 1227 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, C.; Chen, C.; Jiang, L.; Li, Y.; Zhu, Z.; Chen, F.; Cao, Z.; Zhang, W. Effects of Interstitial Oxygen Content on Microstructures and Mechanical Properties of TiZrNb Refractory Medium-Entropy Alloy. Metals 2025, 15, 250. https://doi.org/10.3390/met15030250
Zhang C, Chen C, Jiang L, Li Y, Zhu Z, Chen F, Cao Z, Zhang W. Effects of Interstitial Oxygen Content on Microstructures and Mechanical Properties of TiZrNb Refractory Medium-Entropy Alloy. Metals. 2025; 15(3):250. https://doi.org/10.3390/met15030250
Chicago/Turabian StyleZhang, Chen, Caiying Chen, Li Jiang, Yanhui Li, Zhibin Zhu, Fei Chen, Zhiqiang Cao, and Wei Zhang. 2025. "Effects of Interstitial Oxygen Content on Microstructures and Mechanical Properties of TiZrNb Refractory Medium-Entropy Alloy" Metals 15, no. 3: 250. https://doi.org/10.3390/met15030250
APA StyleZhang, C., Chen, C., Jiang, L., Li, Y., Zhu, Z., Chen, F., Cao, Z., & Zhang, W. (2025). Effects of Interstitial Oxygen Content on Microstructures and Mechanical Properties of TiZrNb Refractory Medium-Entropy Alloy. Metals, 15(3), 250. https://doi.org/10.3390/met15030250







