Isothermal Austenite–Ferrite Phase Transformations and Microstructural Evolution during Annealing in Super Duplex Stainless Steels
Abstract
:1. Introduction
2. Experimental Procedure
3. Results
4. Discussion
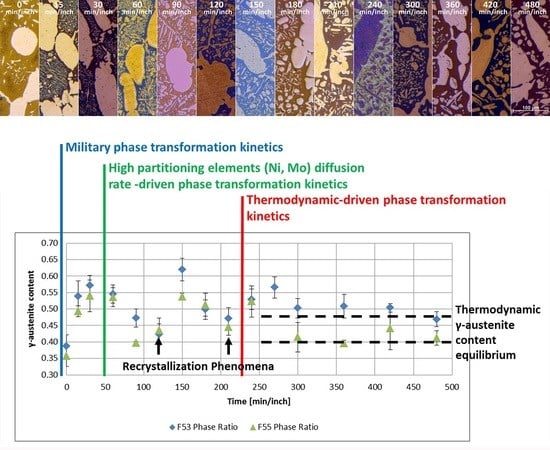
- The damping term is due to the loss of free-energy of the system near the thermodynamic equilibrium condition and it is associated with the thermodynamic aspects of the phase transformations induced by this thermal treatment.On the basis of the observations, the damping term of the phase fraction evolution follows an Arrhenius-type equation describing the driving force of the α-ferrite transformation into γ-austenite. The results confirm the lower activation energy of F53 (1.12 J/mol) if compared with F55 (2.25 J/mol), due to an initial wider deviation from the thermodynamic equilibrium as consequence of the higher concentration of γ-formers elements, as expected by the chemical composition standards [21].Because of the diffusive nature of this phase transformation, it is possible to define a diffusional relaxation time after which the transformation closely approaches the full thermodynamic equilibrium, defined as:where is the radius of the parent phase grains and is the diffusion coefficient of the substitutional solute atoms [22].The explicit formula of should be reported:where is a temperature-independent pre-exponential term of diffusivity, is referred to the Boltzmann constant and corresponds to the activation energy of the diffusion [21,23]. The values used for the calculations are those reported by F. Ruffini et al. [23].Calculating for the motion of Ni atoms in α-ferrite, the faster substitutional element ruling the studied system, and obtaining the radius of the parent γ-phase grains () from metallographic analysis (Figure 2), it is possible to calculate the diffusional relaxation time . The results are in good accordance with the experimental evidence for the longer soaking times of the annealing thermal treatment () (Figure 3 and Figure 10B).
- A robust deviation from the thermodynamic equilibrium and the Arrhenius-type trend of the phase ratio evolution occurs during the early stages of the annealing treatment. The explanation of this behavior can be ascribed to the military character of the ongoing γ-austenite nucleation. This assumes that local phase transformations would be much faster than the overall kinetics ruling the system, which is characterized by an intrinsically slower diffusion-controlled kinetics. As consequence of the military character of the ongoing γ-austenite nucleation processes, the transformation equilibrium is ruled by the local free-energies that exceed the overall reaction thermodynamic equilibrium (in the present case the supersaturation of α-matrix by γ-stabilizers elements). A clear picture of these phenomena can be provided by Widmanstätten precipitation processes: the nucleation is supported by a local supersaturation, generating grain boundaries’ saw-teeth structures but the transformation goes on until it is completed in the α-ferrite grains [24].This explanation can be supported by the observation of the local γ-nucleation processes, which are the cause of the fast increase of γ-austenite volume fraction at the early stages of the annealing thermal treatment (Figure 11).
- The minima of the evolution of the phase ratio with the increase of the soaking time of the annealing thermal treatment are related to recrystallization phenomena. In these cases, the γ-austenitic grains show a primary recrystallization as testified by CSL data and, after that the recrystallization, γ-austenite can grow again. Moreover, in the literature it is reported that while γ-austenite shows a primary recrystallization, α-ferrite undergoes recovery due to its high stacking fault energy (SFE). Indeed, α-ferrite grains resemble the annealing texture of single phase ferritic steels, without revealing any influence performed by the concurrent second phase. So, the retarded nucleation and the reduced dislocation density, due to recovery, essentially suppress recrystallization, which is not detected for α-ferritic grains (Figure 8) [17,18,19].
- The maxima of the phase ratio evolution related to the ongoing of the annealing thermal treatment are related to the local thermodynamic equilibrium at the moving interfaces. This can be described using the Local Equilibrium model and it has been already demonstrated experimentally. This model points out the differences in the diffusive phenomena of the slow diffusing substitutional elements within the Fe-C system. Nickel interactions with the moving interface are expected to be weak and can treated by the local equilibrium model associated with the negligible partitioning (LENP) and paraequilibrium (PE) models. On the other hand, the interactions between molybdenum and the moving interfaces are much stronger. This can be interpreted by a model including the solute drag effect [10]. This effect grows as a function of the interface motion and, therefore, of the soaking time of the annealing thermal treatment. However, the strong drag effect would result in a robust increase of the molybdenum concentration at the moving interfaces (Figure 12) [25]. This instance proceeds up to the complete loss of chemical energy, promoting the interface motion and, consequently, up to the inversion of the α/γ interface motion.
- The periodicity of the oscillating component of the phase fraction evolution can be associated with the diffusive competitive coarsening processes. As extrapolated by the SEM line-scans (Figure 6), the motion of the α/γ interfaces is the result of the movements of the strong partitioned elements, as Mo (acting as α-stabilizer) and Ni (behaving as γ-former). However, the strong partition of these elements (due to the generated concentration gradients) promotes their motion into the other phase, respectively Mo towards γ-austenitic grains and γ-nuclei and Ni into the α-ferritic matrix. If compared with the diffusing paths of atoms the sizes of the grains are much larger, so the motion direction can be always assumed as perpendicular to the α/γ interface. Thus, the motion direction of both the diffusing species is the same, while the direction of their diffusive paths is opposed [10].Provided the diffusion-controlled nature of the solid-state transformation of α-ferrite into γ-austenite and under the hypothesis of the competitive growth regime and the identification of Ni and Mo as the main actors involved in these phenomena, a complete description of the sinusoidal term of the phase evolution can be achieved. The diffusion-controlled competitive coarsening kinetic appears modeled through the Equation (9):where corresponds to the average grain size, is the chemical interdiffusivity, is the interface energy, is the atomic volume, is the solubility of the element in a phase for a system featured by a planar interface, is the Boltzmann constant, is the temperature and is the time. Under the hypothesis of the same value for the growth of the average particle size, the system can be solved and the difference in the diffusion rates between Mo atoms, moving into α-ferrite, and Ni atoms, diffusing into γ-austenite, results in a delay in time for Ni atoms to cover the same diffusion path length. This calculated delay () corresponds also to a period of the sinusoidal term of the phase fraction ratio evolution, which has been observed by the experimental observation () (Figure 3).The wide difference in the diffusion rates (100 times in α-ferrite than in γ-austenite) is related to the atomic packing factor of the b.c.c. α-ferritic structure that is lower than the f.c.c. γ-austenitic arrangement. Another consequence of this diffusive phenomenon, and according to the diffusion-controlled phase transformation models, is that the α-ferrite to γ-austenite transformation (α→γ) is approximately 1.9 times faster than the reverse austenite to ferrite transformation (γ→α) during the initial stages of the transformation. This occurs due to the absence of soft impingement phenomena (overlap of diffusion fields of the neighboring grains in the classic diffusion-controlled growth model, which slows the phase transformation kinetics) in the initial stages of the phase transformations [10].
5. Conclusions
- The precipitation-free zone surrounding the γ- grains is featured by a growth rate as a function of . This indicates the diffusion-limited competitive coarsening and the coupled action of the contemporary diffusions of both α-stabilizers and γ-formers elements.
- The evolution of the phase ratio α/γ results in a sinusoidal behavior damped to the equilibrium γ-austenite volume fraction. As consequence, the transformation process can be decomposed into two main contributions: the damping term (related to the thermodynamic aspects of the processes) due to the loss of free-energy of the system, and the sinusoidal oscillations associated to the diffusive processes in the alloys. This last aspect rules the kinetics of the reaction. The initial deviation from the thermodynamic equilibrium is caused by the military character of the α-intragranular γ nucleation.
- The thermodynamic factor is governed by an Arrhenius-type equation, resulting in lower activation energy for F53–S32750, as expected by the alloy composition. Discrepancies between the monotonic damping of the free-energy available for the phase transformation processes and the effective conversion of α-ferrite into γ-austenite are caused by different γ-grains aspect ratios. The sinusoidal kinetic component of the phase formation is ruled by the diffusive competitive coarsening processes. The periodicity is controlled by the diffusion rate of the high partitioning elements (i.e., nickel and molybdenum) in the different phases.
- The changes in the dominant transformation are due to the solute drag effect of Mo on the moving interfaces, as predicted by Local Equilibrium model, and to the recovery and recrystallization phenomena.
- The additivity character of the annealing thermal treatment has been proved. This result, coupled with the assessment of the phase fraction ratio evolution, represents a very powerful tool for the achievement of the desired and precise phase fraction ratio in the product realization.
Author Contributions
Conflicts of Interest
References
- Nilsson, J.-O. Super duplex stainless steels. Mater. Sci. Technol. 1992, 8, 685–700. [Google Scholar] [CrossRef]
- Charles, J.; Chemelle, P. The history of duplex developments, nowadays DSS properties and duplex market future trends. In Proceedings of the Duplex World Conference, Beaune, France, 13–15 October 2010. [Google Scholar]
- Tan, H.; Jiang, Y.; Deng, B.; Sun, T.; Xu, J.; Li, J. Effect of annealing temperature on the pitting corrosion resistance of super duplex stainless steel UNS S32750. Mater. Charact. 2009, 60, 1049–1054. [Google Scholar] [CrossRef]
- Baddoo, N.R. Stainless steel in construction: A review of research, applications, challenges and opportunities. J. Constr. Steel Res. 2008, 64, 1199–1206. [Google Scholar] [CrossRef]
- Olsson, J.; Snis, M. Duplex—A new generation of stainless steels for desalination plants. Desalination 2007, 205, 104–113. [Google Scholar] [CrossRef]
- Alvarez-Armas, I.; Degallaix-Moreuil, S. Duplex Stainless Steels; ISTE Ltd.: London, UK; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2009. [Google Scholar]
- Van der Ven, A.; Delaey, L. Models for precipitate growth during the γ → α + γ transformation in Fe-C and Fe-C-M alloys. Prog. Mater. Sci. 1996, 40, 181–264. [Google Scholar] [CrossRef]
- Southwick, P.D.; Honeycombe, R.W.K. Decomposition of ferrite to austenite in 26%Cr-5%Ni stainless steel. Met. Sci. 1980, 14, 253–261. [Google Scholar] [CrossRef]
- Chen, H.; Gamsjäger, E.; Schider, S.; Khanbareh, H.; van der Zwaag, S. In situ observation of austenite–ferrite interface migration in a lean Mn steel during cyclic partial phase transformations. Acta Mater. 2013, 61, 2414–2424. [Google Scholar] [CrossRef]
- Hutchinson, C.R.; Zurob, H.S.; Bréchet, Y. The Growth of Ferrite in Fe-C-X Alloys: The Role of Thermodynamics, Diffusion, and Interfacial Conditions. Metall. Mater. Trans. A 2006, 37, 1711–1720. [Google Scholar] [CrossRef]
- Gouné, M.; Danoix, F.; Ågren, J.; Bréchet, Y.; Hutchinson, C.R.; Militzer, M.; Purdy, G.; van der Zwaag, S.; Zurob, H. Overview of the current issues in austenite to ferrite transformation and the role of migrating interfaces therein for low alloyed steels. Mater. Sci. Eng. R 2015, 92, 1–38. [Google Scholar] [CrossRef]
- Liu, F.; Yang, C.; Yang, G.; Zhou, Y. Additivity rule, isothermal and non-isothermal transformations on the basis of an analytical transformation model. Acta Mater. 2007, 55, 5255–5267. [Google Scholar] [CrossRef]
- Grong, Ø.; Myhr, O.R. Additivity and isokinetic behavior in relation to diffusion controlled growth. Acta Mater. 2000, 48, 445–452. [Google Scholar] [CrossRef]
- Rios, P.R. Relationship between non-isothermal transformation curves and isothermal and non-isothermal kinetics. Acta Mater. 2005, 53, 4893–4901. [Google Scholar] [CrossRef]
- Nilsson, J.-O.; Huhtala, T.; Jonsson, P.; Karlsson, L.; Wilson, A. Structural stability of super duplex stainless weld metals and its dependence on tungsten and copper. Metall. Mater. Trans. A 1996, 27, 2196–2208. [Google Scholar] [CrossRef]
- Rutter, J.W.; Aust, K.T. Migration of <100> tilt grain boundaries in high purity lead. Acta Metall. 1965, 13, 181–186. [Google Scholar] [CrossRef]
- Zaid, M.; Bhattacharjee, P.P. Electron backscatter diffraction study of deformation and recrystallization textures of individual phases in a cross-rolled duplex steel. Mater. Charact. 2014, 96, 263–272. [Google Scholar] [CrossRef]
- Keichel, J.; Foct, J.; Gottstein, G. Deformation and Annealing Behavior of Nitrogen Alloyed Duplex Stainless Steels. Part II: Annealing. ISIJ Int. 2003, 43, 1788–1794. [Google Scholar] [CrossRef]
- Doherty, R.D.; Hughes, D.A.; Humphreys, F.J.; Jonas, J.J.; Juul Jensen, D.; Kassner, M.E.; King, W.E.; McNelley, T.R.; McQueen, H.J.; Rollett, A.D. Current issues in recrystallization: A review. Mater. Sci. Eng. A 1997, 238, 219–274. [Google Scholar] [CrossRef]
- Knyazeva, M.; Pohl, M. Duplex Steels: Part I: Genesis, Formation, Structure. Metallogr. Microstruct. Anal. 2013, 2, 113–121. [Google Scholar] [CrossRef]
- Balluffi, R.W.; Allen, S.M.; Carter, W.C. Kinetics of Materials; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2005. [Google Scholar]
- Rigsbee, J.M.; Aaronson, H.I. The interfacial structure of the broad faces of ferrite plates. Acta Metall. 1979, 27, 365–376. [Google Scholar] [CrossRef]
- Ruffini, F.; Tassa, O.; Carosi, A.; Arcobello, F.; Giambi, B. Thermodynamic modelling to support production of high nitrogen steels by different processes. Metall. Ital. 2007, 5, 13–20. [Google Scholar]
- Caballero, F.G.; Garcia-Mateo, C.; Santofimia, M.J.; Miller, M.K.; García de Andrés, C. New experimental evidence on the incomplete transformation phenomenon in steel. Acta Mater. 2009, 57, 8–17. [Google Scholar] [CrossRef] [Green Version]
- Gamsjäger, E.; Svoboda, J.; Fischer, F.D. Austenite-to-ferrite phase transformation in low-alloyed steels. Comput. Mater. Sci. 2005, 32, 360–369. [Google Scholar] [CrossRef]
- Okuda, H.; Ochiai, S. The Effects of Solute and Vacancy Depletion on the Formation of Precipitation-Free Zone in a Model Binary Alloy Examined by a Monte Carlo Simulation. Mater. Trans. JIM 2004, 45, 1455–1460. [Google Scholar] [CrossRef]
- Gandin, C.-A.; Jacot, A. Modeling of precipitate-free zone formed upon homogenization in a multi-component alloy. Acta Mater. 2007, 55, 2539–2553. [Google Scholar] [CrossRef]







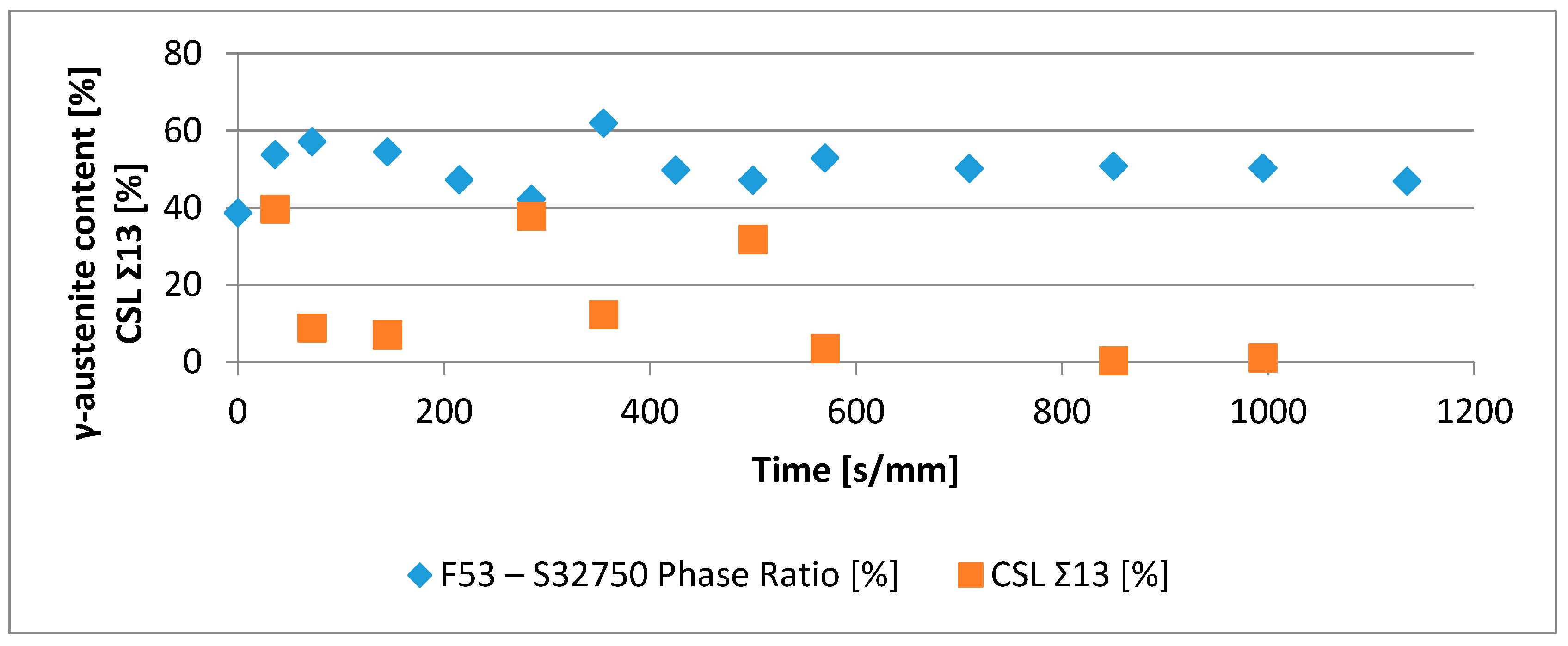




| Annealing Soaking Times [s/mm] | 0 | 36 | 72 | 145 | 215 | 285 | 355 | 425 | 500 | 570 | 710 | 850 | 995 | 1135 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| F53–S32750 | Phase Ratio | 0.39 | 0.54 | 0.57 | 0.55 | 0.47 | 0.42 | 0.62 | 0.50 | 0.47 | 0.53 | 0.50 | 0.51 | 0.50 | 0.47 |
| Error | 0.03 | 0.05 | 0.03 | 0.03 | 0.03 | 0.03 | 0.03 | 0.03 | 0.03 | 0.03 | 0.03 | 0.04 | 0.01 | 0.02 | |
| γ Aspect Ratio | 9.26 | 9.08 | 10.52 | 10.34 | 9.63 | 6.76 | 7.47 | 9.11 | 9.19 | 8.66 | 8.89 | 9.23 | 8.70 | 8.97 | |
| F55–S32760 | Phase Ratio | 0.36 | 0.49 | 0.54 | 0.54 | 0.40 | 0.44 | 0.54 | 0.51 | 0.45 | 0.52 | 0.41 | 0.40 | 0.44 | 0.41 |
| Error | 0.03 | 0.02 | 0.05 | 0.03 | 0.00 | 0.04 | 0.01 | 0.04 | 0.03 | 0.05 | 0.04 | 0.01 | 0.07 | 0.02 | |
| γ Aspect Ratio | 9.33 | 10.87 | 9.17 | 11.61 | 10.21 | 7.78 | 8.21 | 9.92 | 8.38 | 9.09 | 10.27 | 9.56 | 9.64 | 8.89 | |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ciuffini, A.F.; Barella, S.; Di Cecca, C.; Gruttadauria, A.; Mapelli, C.; Mombelli, D. Isothermal Austenite–Ferrite Phase Transformations and Microstructural Evolution during Annealing in Super Duplex Stainless Steels. Metals 2017, 7, 368. https://doi.org/10.3390/met7090368
Ciuffini AF, Barella S, Di Cecca C, Gruttadauria A, Mapelli C, Mombelli D. Isothermal Austenite–Ferrite Phase Transformations and Microstructural Evolution during Annealing in Super Duplex Stainless Steels. Metals. 2017; 7(9):368. https://doi.org/10.3390/met7090368
Chicago/Turabian StyleCiuffini, Andrea Francesco, Silvia Barella, Cosmo Di Cecca, Andrea Gruttadauria, Carlo Mapelli, and Davide Mombelli. 2017. "Isothermal Austenite–Ferrite Phase Transformations and Microstructural Evolution during Annealing in Super Duplex Stainless Steels" Metals 7, no. 9: 368. https://doi.org/10.3390/met7090368
APA StyleCiuffini, A. F., Barella, S., Di Cecca, C., Gruttadauria, A., Mapelli, C., & Mombelli, D. (2017). Isothermal Austenite–Ferrite Phase Transformations and Microstructural Evolution during Annealing in Super Duplex Stainless Steels. Metals, 7(9), 368. https://doi.org/10.3390/met7090368