Metallic Slit-Plate Dampers: Damage Evaluation with Metal Magnetic Memory Technique and Application to Structures with Rocking Columns
Abstract
:1. Introduction
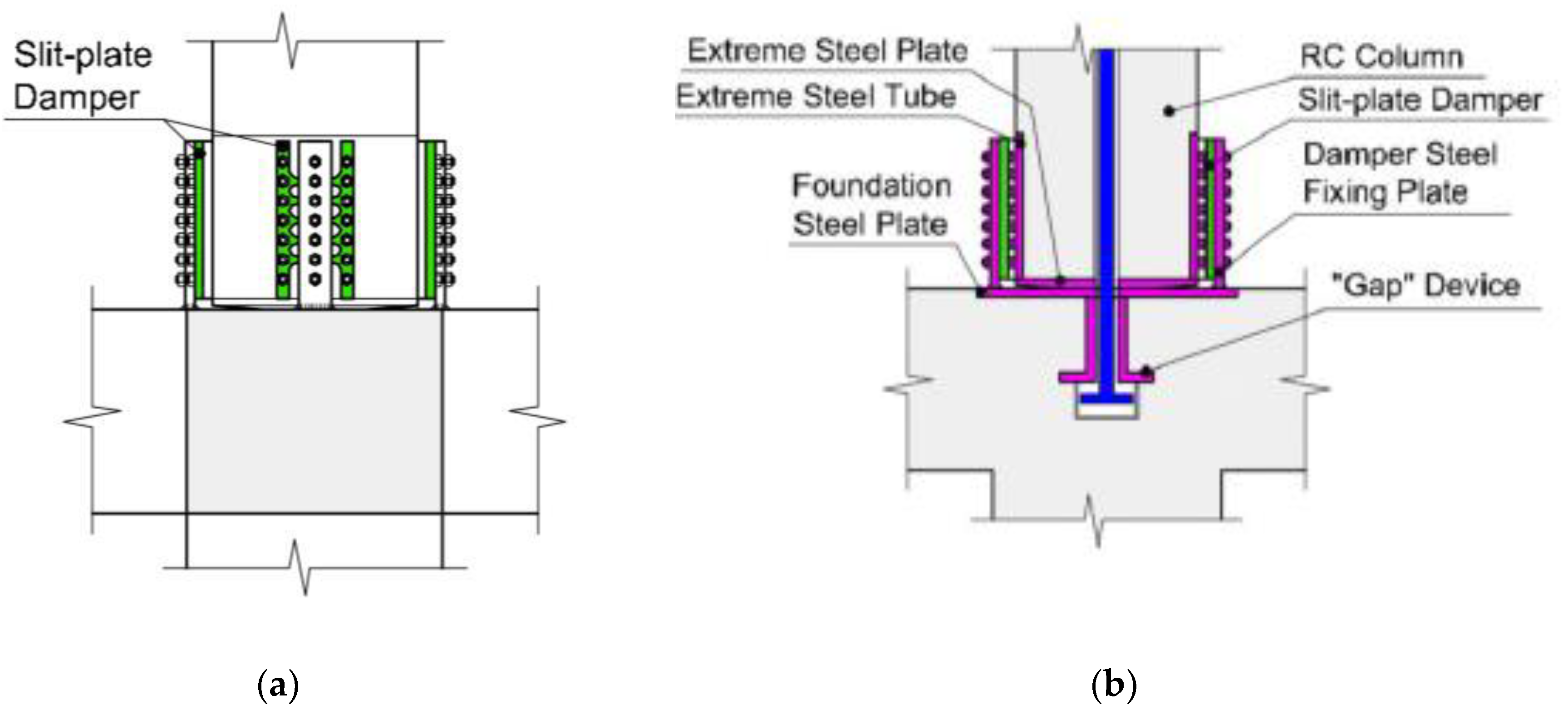
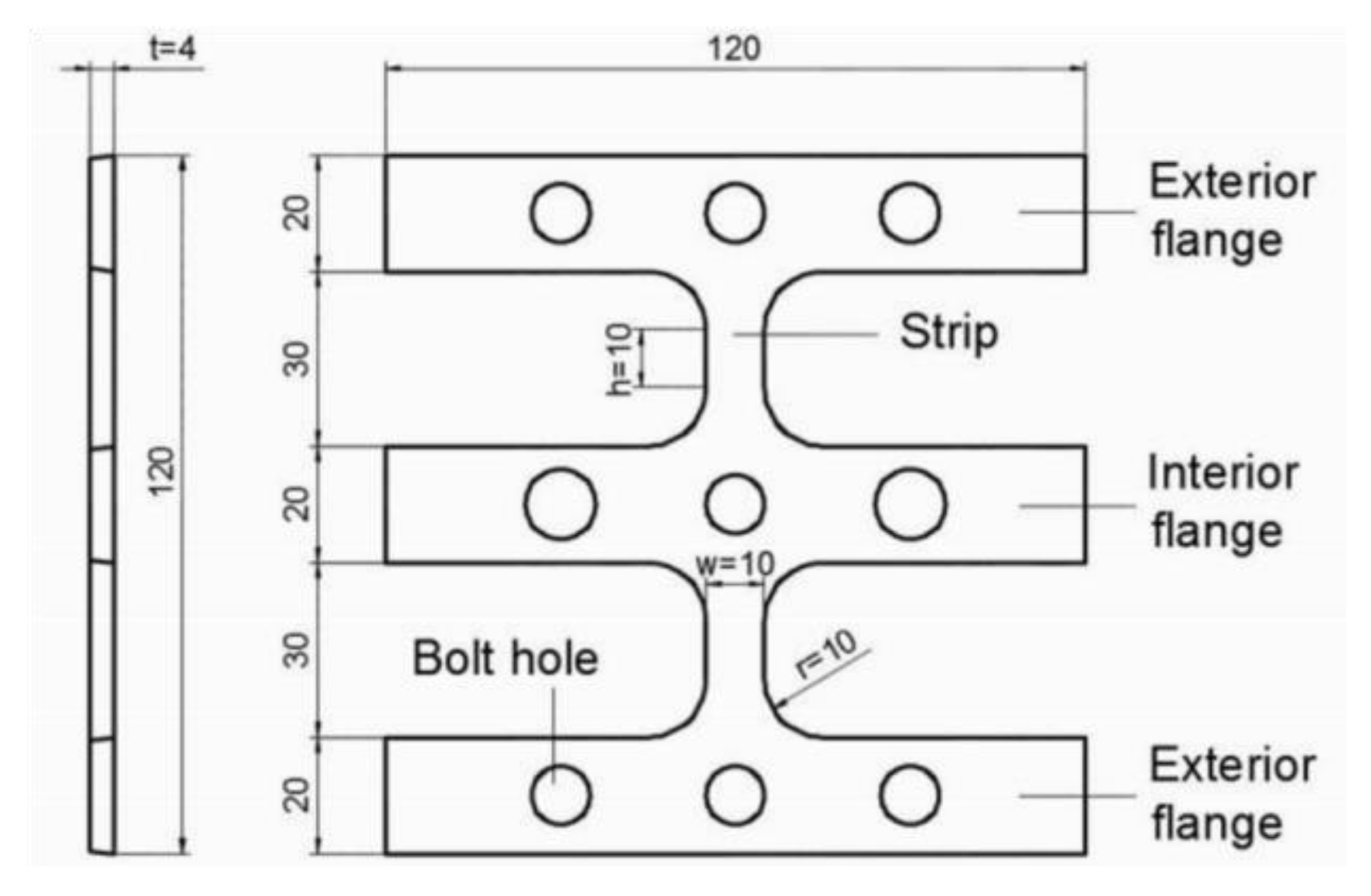
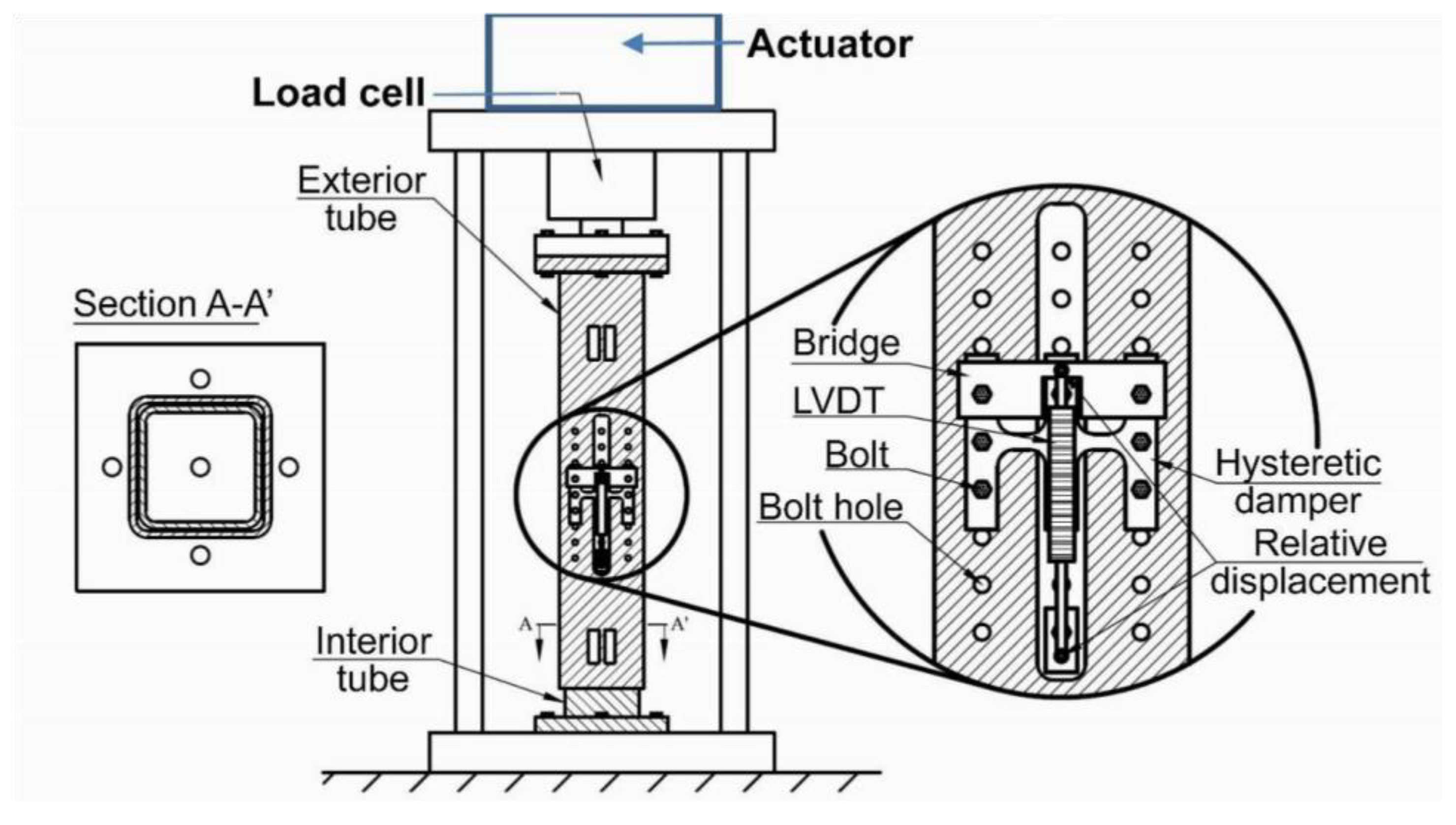
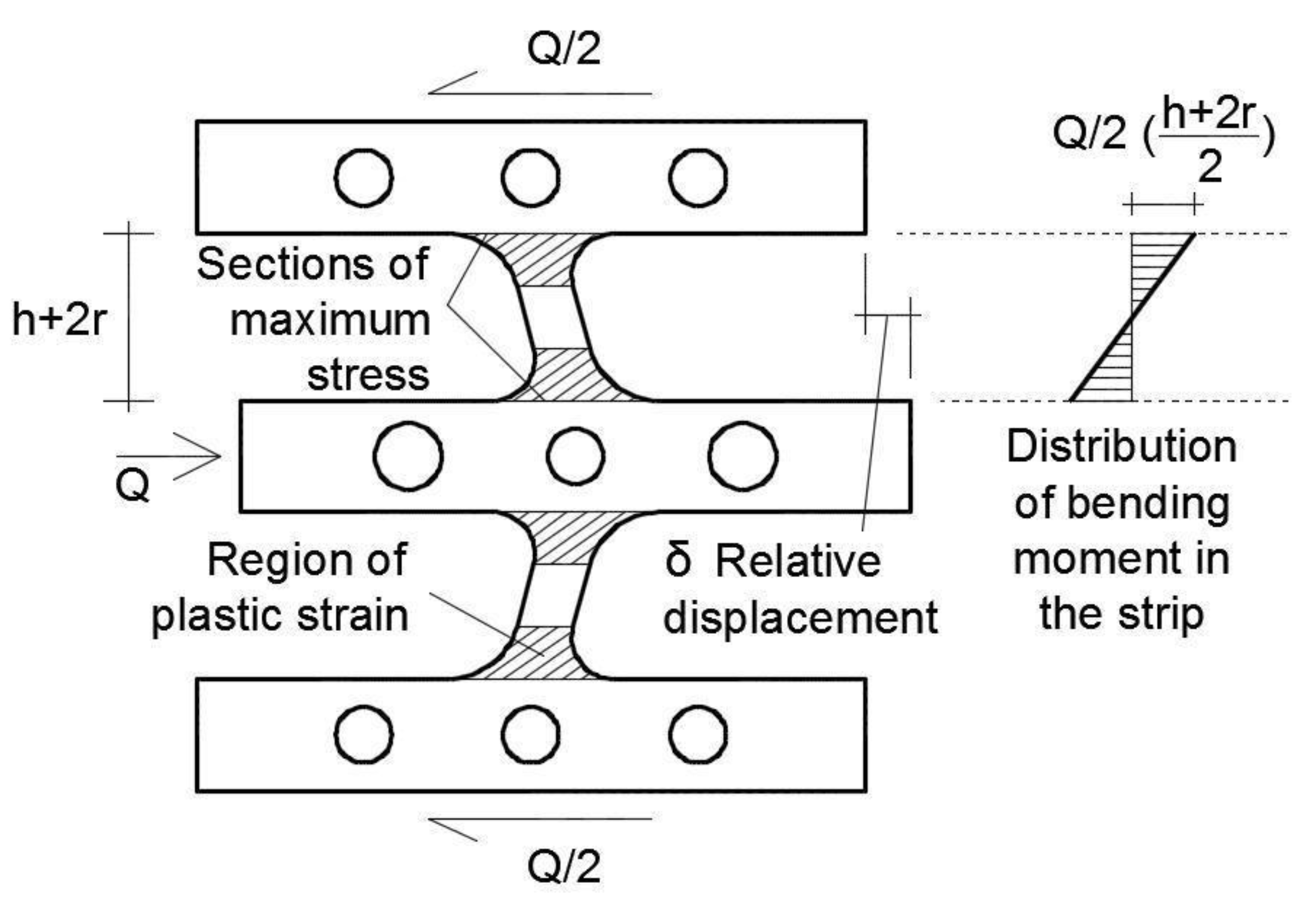
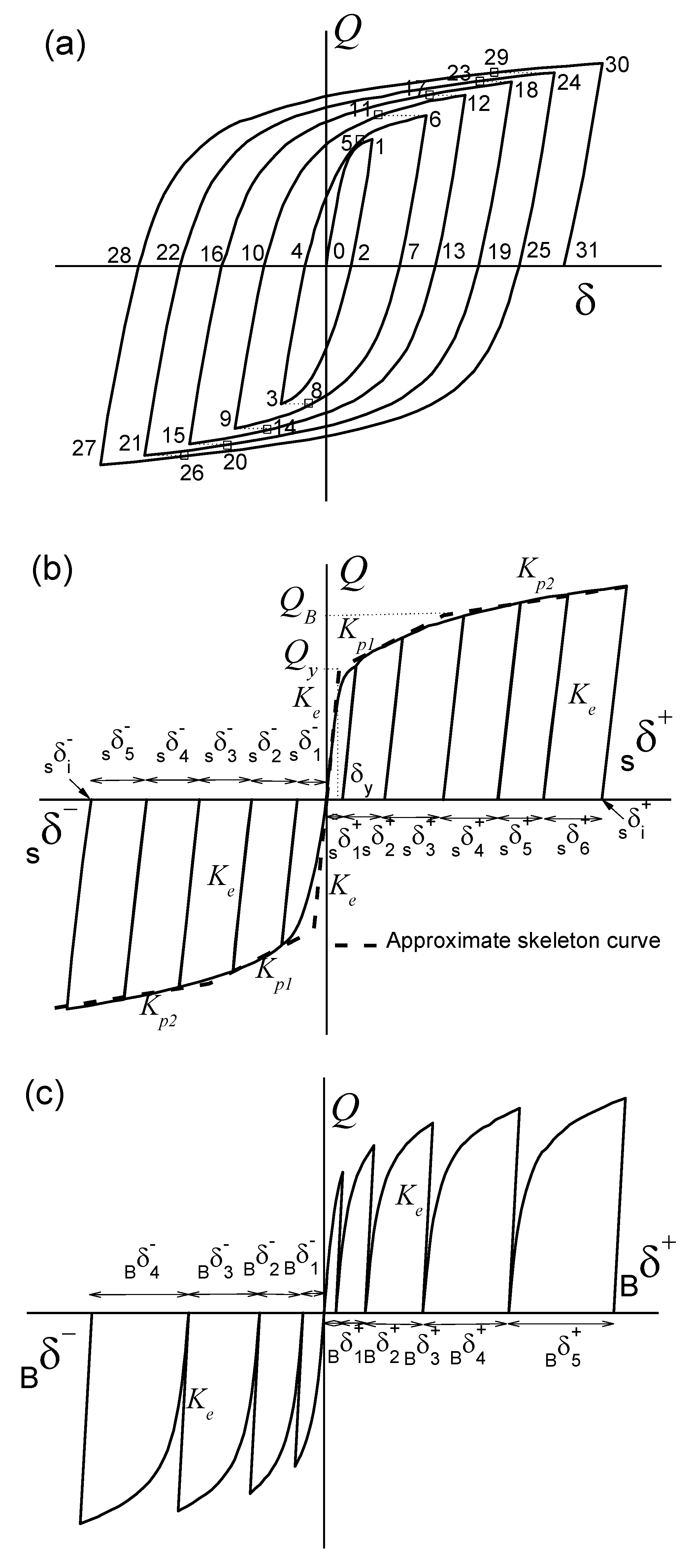
2. Description of the “Slit-Plate” Damper
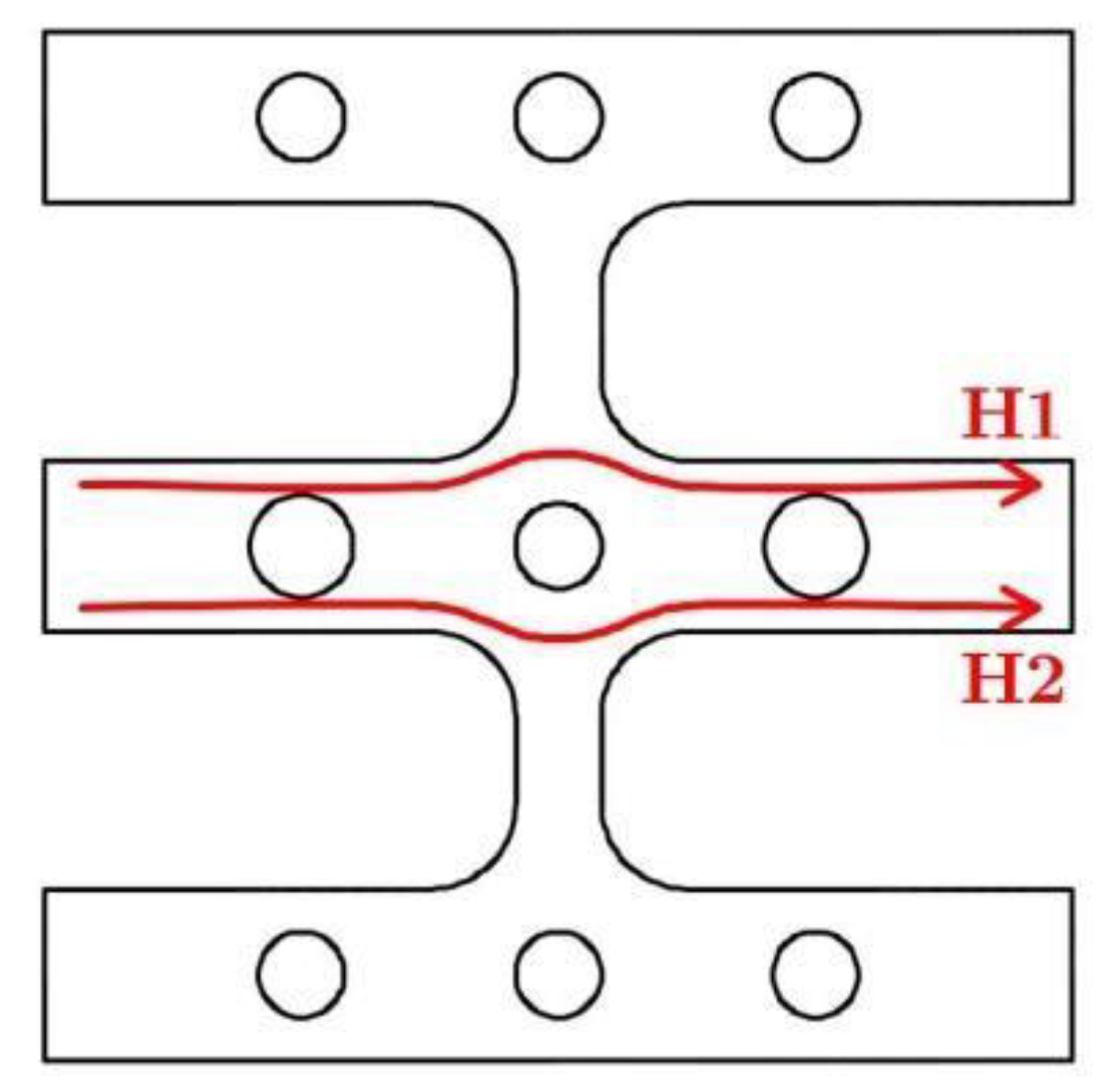
3. MMM Inspection of Specimens
- -
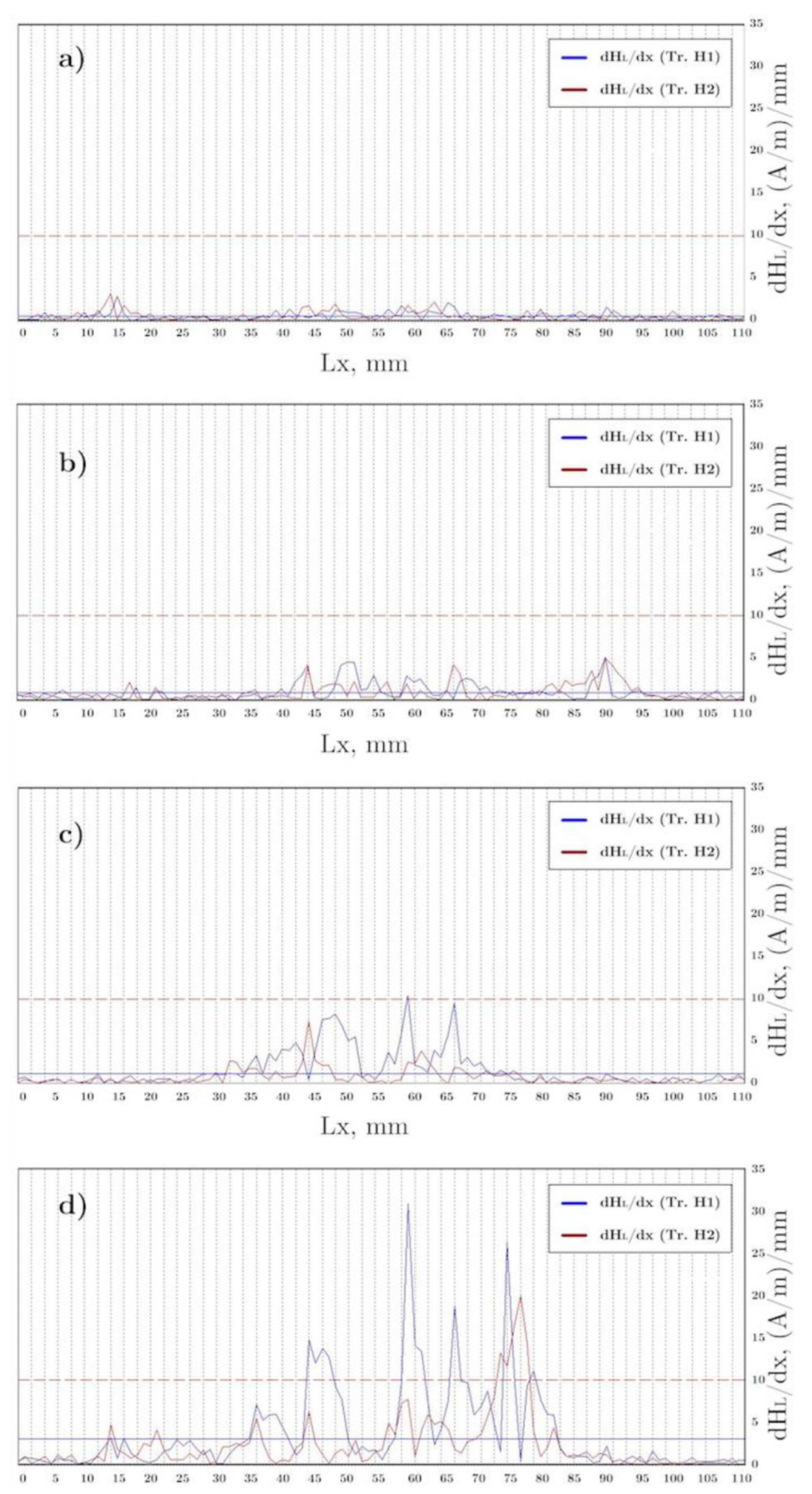
- HL, x: the normal component of the self-magnetic field intensity.
- -
- HL, x/dx: the gradient of the normal component of self-magnetic field intensity in the x-direction (scanning direction).
- -
- HL, y: the tangential component of the self-magnetic field intensity.
- -
- HL, y/dx: the gradient of the tangential component of self-magnetic field intensity in the x-direction (scanning direction).
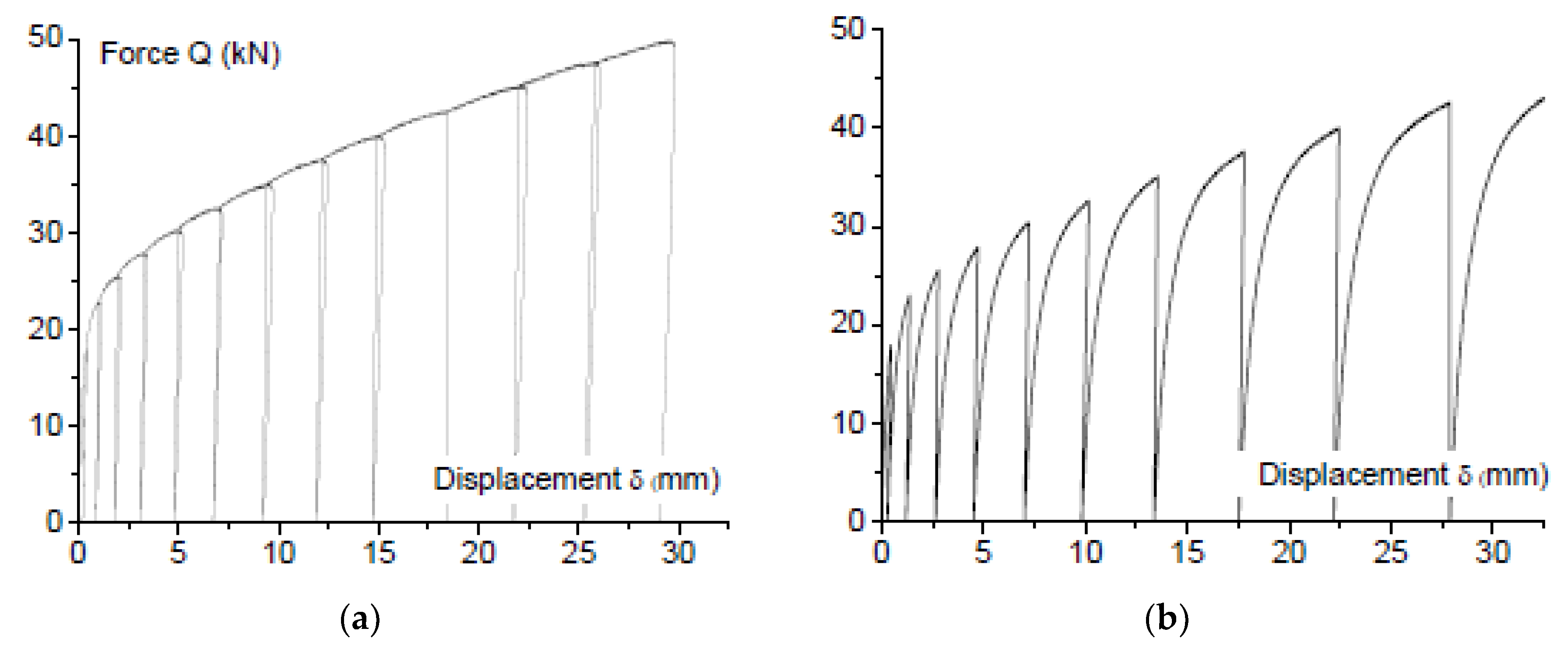
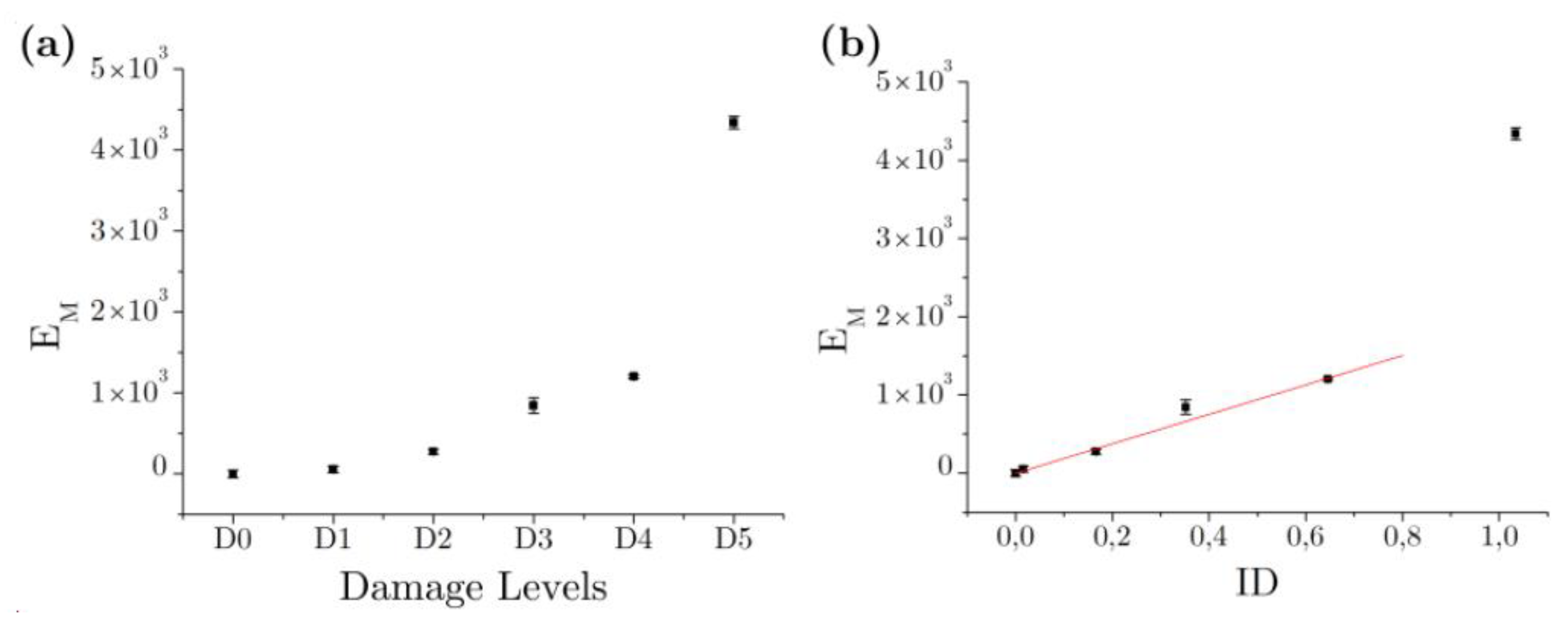
4. Ultimate Energy Dissipation Capacity and Mechanical Damage Index ID
5. Results
5.1. Evaluation of the Mechanical Index of Damage ID
5.2. Results and Interpretation of the MMM Inspection
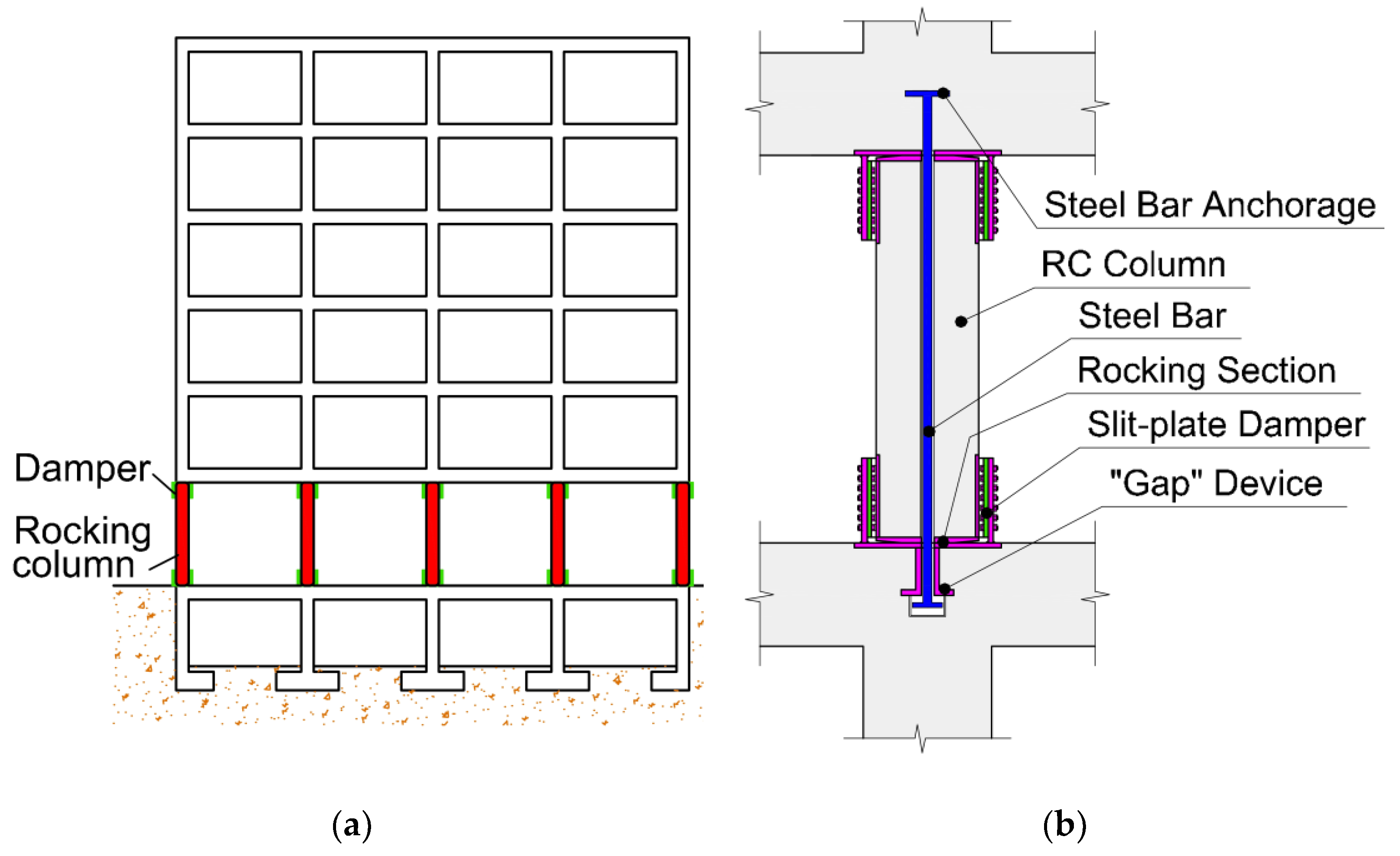
6. Seismic Response of RC Frames with Rocking Columns Equipped with Slit-Plate Dampers
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Benavent-Climent, A. An energy-based damage model for seismic response of steel structures. Earthquake Eng. Struct. Dyn. 2007, 36, 1049–1064. [Google Scholar] [CrossRef]
- Kouris, L.A.S.; Penna, A.; Magenes, G. Seismic damage diagnosis of a masonry building using short-term damping measurements. J. Sound Vib. 2017, 394, 366–391. [Google Scholar] [CrossRef]
- Kurashkin, K.V.; Mishakin, V.V. Ultrasonic estimation of residual stresses. Inorg. Mater. 2013, 4, 54–58. [Google Scholar] [CrossRef]
- Ma, S.; Yuan, K.; Zhang, H.; Wei, X. Evaluation of fracture damage of SUS306 structural steel using ultrasonic testing. J. Shanghai Univ. 2009, 13, 279. [Google Scholar] [CrossRef]
- Kikuchi, Y. Magnetostrictive materials and applications. IEEE Trans. Magn. 1968, 4, 107–117. [Google Scholar] [CrossRef]
- Hornreich, R.M.; Rubinstein, R.J. Magnetostrictive phenomena in metallic materials and some of their device applications. IEEE Trans. Magn. 1971, 7, 29–48. [Google Scholar] [CrossRef]
- Doubov, A.A. A study of metal properties using the method of magnetic memory. Met. Sci. Heat Treat. 1997, 39, 401–402. [Google Scholar] [CrossRef]
- ISO 24497-3:2007. Non-Destructive Testing–Metal Magnetic Memory–Part 3: Inspection of Welded Joints; International Organization for Standardization: Geneva, Switzerland, 2007. [Google Scholar]
- Doubov, A.A. Principal features of metal magnetic memory method and inspection tools as compared to known magnetic NDT methods. In Proceedings of the Sixteenth Annual World Conference on Non-Destructive Testing, Montreal, QC, Canada, 30 August–3 September 2004. [Google Scholar]
- Doubov, A.A. Screening of weld quality using the magnetic metal memory effect. Weld. World 1998, 41, 196–198. [Google Scholar]
- Mao, W.; Atherton, D.L. Effect of compressive stress on the reversible and irreversible differential magnetic susceptibility of a steel cube. J. Magn. Magn. Mater. 2000, 214, 69–77. [Google Scholar] [CrossRef]
- Doubov, A.A. A technique for monitoring the bends of boiler and steam-line tubes using the magnetic memory of metal. Therm. Eng. 2001, 48, 289–295. [Google Scholar]
- Doubov, A.A.; Demin, E.A.; Milayev, A.I.; Steklov, O.I. The experience of gas pipeline stress–strain state control with usage of the metal magnetic memory method as compared with conventional methods and stress control means. Weld. World 2002, 46, 29–33. [Google Scholar] [CrossRef]
- Li, Z.; Dixon, S.; Cawley, P.; Jarvis, R.; Nagy, P.B. Study of metal magnetic memory (MMM) technique using permanently installed magnetic sensor arrays. In Proceedings of the AIP Conference, Atlanta, GA, USA, 17–22 July 2016. [Google Scholar] [CrossRef]
- Stegemann, R.; Cabeza, S.; Pelkner, M.; Lyamkin, V.; Sonntag, N.; Bruno, G.; Skrotzki, B.; Kreutzbruck, M. Evaluation of high spatial resolution imaging of magnetic stray fields for early damage detection. In Proceedings of the AIP Conference, Atlanta, GA, USA, 17–22 July 2016. [Google Scholar] [CrossRef]
- Jarvis, R.; Cawley, P.; Nagy, P.B. Current deflection NDE for pipeline inspection and monitoring. In Proceedings of the AIP Conference, Minneapolis, MI, USA, 26–31 July 2016. [Google Scholar] [CrossRef]
- Huckelbridge, A.; Clough, R. Earthquake Simulation Tests of a Nine Story Steel Frame with Columns Allowed to Uplift; Report EERC-77/23; University of California at Berkeley: Berkeley, CA, USA, 1977. [Google Scholar]
- Beck, J.L.; Skinner, R.I. The seismic response of a reinforced concrete bridge pier designed to step. Earthquake Eng. Struct. Dyn. 1973, 2, 343–358. [Google Scholar] [CrossRef]
- Sharpe, R.D.; Skinner, R.I. The seismic design of an industrial chimney with rocking base. Bull. New Zealand 1983, 16, 98–106. [Google Scholar]
- Polyakov, S.V. Design of Earthquake Resistant Structures; MIR Publishers: Moscow, Russia, 1974. [Google Scholar]
- Smirnov, V.; Eisenberg, J.; Vasileva, A. Seismic Isolation of buildings and historical monuments. Recent developments in Russia. In Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, BC, Canada, 1–6 August 2004. [Google Scholar]
- Uzdin, A.M.; Doronin, F.A.; Davydova, G.V.; Avidon, G.E.; Karlina, E.A. Performance analysis of seismicinsulating elements with negative stiffness. Soil Mech. Found. Eng. 2009, 46, 15–21. [Google Scholar] [CrossRef]
- Eisenberg, J.M.; Smirnov, V.I. Development of Earthquake Protection Structural Systems Based on Response Control. In Proceedings of the 15 World Conference on Earthquake Engineering, Lisbon, Portugal, 24–28 September 2012. [Google Scholar]
- Bachmann, J.A.; Vassiliou, M.F.; Stojadinovic, B. Dynamics of rocking podium structures. Earthquake Eng. Struct. Dyn. 2017, 46, 2499–2517. [Google Scholar] [CrossRef]
- Mander, J.B.; Cheng, C.T. Seismic Resistance of Bridge Piers Based on Damage Avoidance Design; Technical Report for National Center for Earthquake Engineering Research; National Center for Earthquake Engineering Research, State University of New York at Buffalo: New York, NY, USA, 1997. [Google Scholar]
- Viti, S.; Cimellaro, G.P.; Reinhorn, A.M. Retrofit of a hospital through strength reduction and enhanced damping. Smart Struct. Syst. 2006, 2, 339–355. [Google Scholar] [CrossRef]
- Lavan, Q.; Cimellaro, G.P.; Reinhorn, A.M. Non-iterative optimization procedure for seismic weakening and damping of inelastic structures. ASCE J. Struct. Eng. 2008, 134, 1638–1648. [Google Scholar] [CrossRef]
- Benavent-Climent, A. A brace-type seismic damper based on yielding the walls of hollow structural sections. Eng. Struct. 2010, 32, 1113–1122. [Google Scholar] [CrossRef]
- European Committee for Standardization (CEN). Eurocode 2: Design of concrete structures–Part 1-1: General rules and rules for buildings. European Standard EN 1992–1:2004; European Committee for Standardization: Brussels, Belgium, 2004. [Google Scholar]
- European Committee for Standardization (CEN). Eurocode 8: Design of Structures for Earthquake Resistance, Part 1: General rules, Seismic Actions and Rules for Buildings. European Standard EN 19981:2004; European Committee for Standardization: Brussels, Belgium, 2004. [Google Scholar]
- McKenna, F.; Fenves, G.L.; Scott, M.H.; Jeremic, B. OpenSees: Open System for Earthquake Engineering Simulation [Internet]; Version 2.4.4; Pacific Earthquake Engineering Research Center, University of California: Berkeley, CA, USA, 2000; Available online: http://opensees.berkeley.edu (accessed on 1 March 2018).

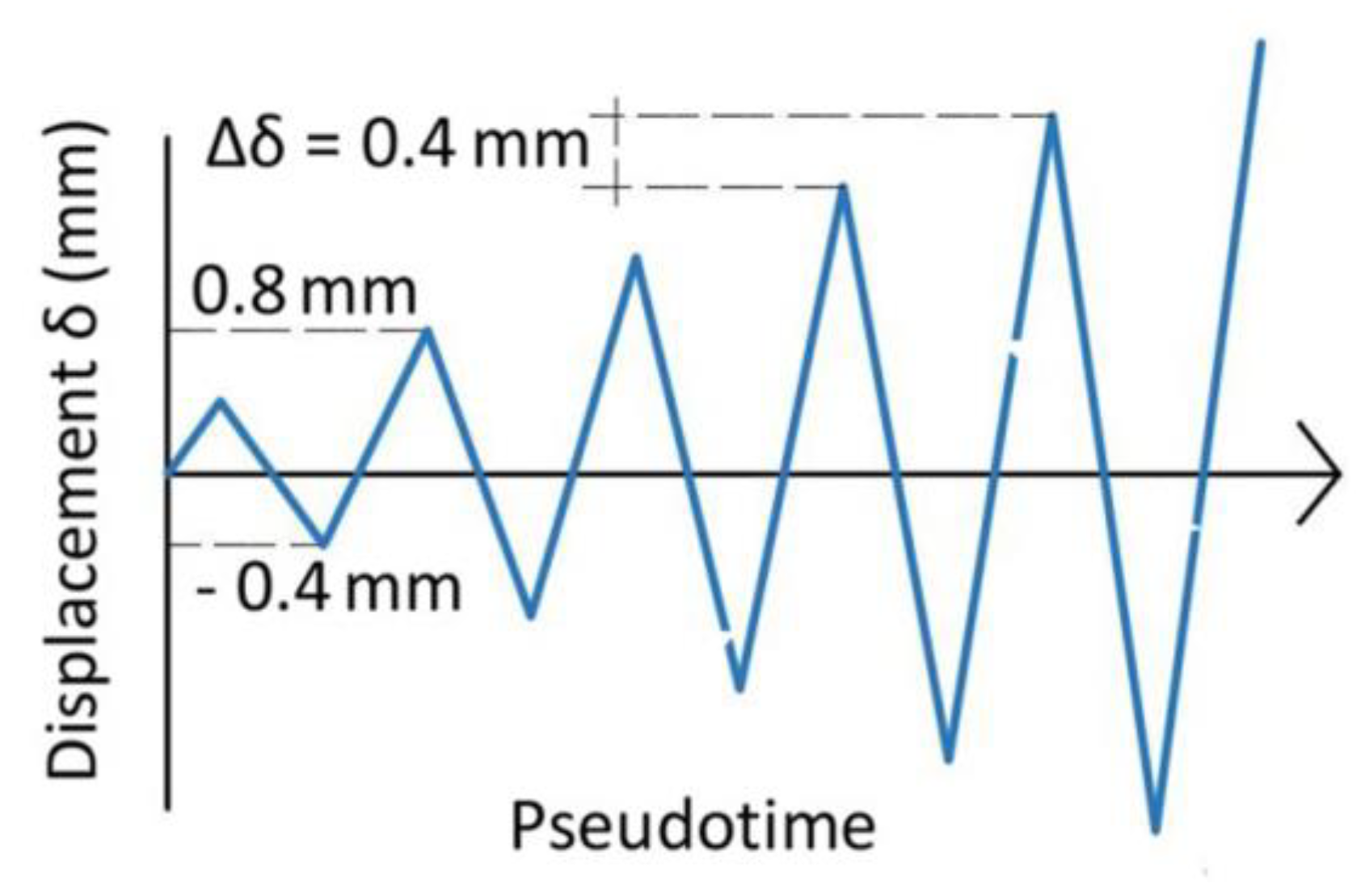





| Number of the Cycle | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Maximum displacement (mm) | 0.4 | 0.8 | 1.2 | 1.6 | 2 | 2.4 | 2.8 | 3.2 | 3.6 | 4 | 4.4 | 4.8 | 5.2 |
| DC1 (D1) | X | X | |||||||||||
| DC2 (D2) | X | X | X | X | X | X | |||||||
| DC3 (D3) | X | X | X | X | X | X | X | X | |||||
| DC4 (D4) | X | X | X | X | X | X | X | X | X | X | |||
| DC5 (D5) | X | X | X | X | X | X | X | X | X | X | X | X | X |
| Specimen | DC1 | DC2 | DC3 | DC4 | DC5 |
|---|---|---|---|---|---|
| Damage Level | D1 | D2 | D3 | D4 | D5 |
| ID Index | 0.016 | 0.166 | 0.352 | 0.646 | 1.034 |
| N° Record | Name of Earthquake | Year | Station | Mw | Rrup (km) | vs30 (m/s) | Peak Ground Acceleration (PGA (g)) |
|---|---|---|---|---|---|---|---|
| 1 | Hollister-01 | 1961 | Hollister City Hall | 5.6 | 19 | 199 | 0.11 |
| 2 | Friuly Italy | 1976 | Codroipo | 6.5 | 33 | 249 | 0.09 |
| 3 | Tabas Iran | 1978 | Boshrooyeh | 7.3 | 29 | 325 | 0.11 |
| 4 | Coyote Lake | 1979 | San Juan B. 24 Polk St | 5.7 | 20 | 335 | 0.11 |
| 5 | Imperial Valley-06 | 1979 | Compuertas | 6.5 | 15 | 260 | 0.19 |
| 6 | Victoria México | 1980 | SAHOP Casa Flores | 6.3 | 39 | 260 | 0.10 |
| 7 | Northern Calif 01 | 1941 | Fendhale City Hall | 6.4 | 45 | 219 | 0.12 |
| No Record | 1 | 2 | 3 | 4 | 5 | 6 | 7 | Average |
|---|---|---|---|---|---|---|---|---|
| Level 1 | 0.05 | 0.07 | 0.07 | 0.02 | 0.06 | 0.04 | 0.04 | 0.05 |
| Level 2 | 0.08 | 0.08 | 0.04 | 0.04 | 0.08 | 0.07 | 0.04 | 0.06 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abarkane, C.; Ríos-García, G.; Gale-Lamuela, D.; Rescalvo, F.; Gallego, A.; Benavent-Climent, A. Metallic Slit-Plate Dampers: Damage Evaluation with Metal Magnetic Memory Technique and Application to Structures with Rocking Columns. Metals 2019, 9, 953. https://doi.org/10.3390/met9090953
Abarkane C, Ríos-García G, Gale-Lamuela D, Rescalvo F, Gallego A, Benavent-Climent A. Metallic Slit-Plate Dampers: Damage Evaluation with Metal Magnetic Memory Technique and Application to Structures with Rocking Columns. Metals. 2019; 9(9):953. https://doi.org/10.3390/met9090953
Chicago/Turabian StyleAbarkane, Chihab, German Ríos-García, David Gale-Lamuela, Francisco Rescalvo, Antolino Gallego, and Amadeo Benavent-Climent. 2019. "Metallic Slit-Plate Dampers: Damage Evaluation with Metal Magnetic Memory Technique and Application to Structures with Rocking Columns" Metals 9, no. 9: 953. https://doi.org/10.3390/met9090953
APA StyleAbarkane, C., Ríos-García, G., Gale-Lamuela, D., Rescalvo, F., Gallego, A., & Benavent-Climent, A. (2019). Metallic Slit-Plate Dampers: Damage Evaluation with Metal Magnetic Memory Technique and Application to Structures with Rocking Columns. Metals, 9(9), 953. https://doi.org/10.3390/met9090953







