The Selection of Anchoring System for Floating Houses by Means of AHP Method

Abstract

This paper indicates and analyses the use of anchoring systems, such as mooring piles, booms, mooring cables, and deadweight anchors with additional elastic connectors, which are the most frequently applied by the producers of floating houses. The selection of the most advantageous anchoring system is complicated and requires the application of quantitative and qualitative data and methods. This publication presents the results of the calculations using one of the most common methods of multi-criteria analysis of decision-making, namely AHP (analytic hierarchy process). The anchoring system, which is the most beneficial for users, has been indicated with the use of the main criteria such as: cost, time, external risk factors, geospatial factors, and the sub-criteria of the first and second order. Due to the conducted analysis, it has been shown that the most significant factor of the anchoring system selection for the users of floating houses is the investment cost that needs to be borne during the usage, and the most favourable anchoring system is the use of mooring cables.

1. Introduction

The intensive economic development of European and Northern American countries at the beginning of the 1980s, the expansion of the urban structures and rapid urbanization, as well as the subsequent constant increase in land and property prices [1] underpin the search for an alternative method of modern construction, that includes developing buildings away from land, namely floating houses [2]. The Netherlands is the exception because the popularity of floating houses increased in manner forced by nature. Around 50% of the area of the Netherlands is located below sea level [3,4,5]. The consequence of the greenhouse effect is climate change, which causes the sea level to rise and storms to become more violent. Therefore, in the Netherlands it has been decided not to fight with the sea, but to use its strength and adjust to it [5]. A necessity soon turned into a lifestyle, fashion, a symbol and synonym of freedom, luxury, and a comfortable life. Today, with the awareness of the Dutch, floating houses certainly differ from traditional buildings only by the type of foundations [2].

In Poland, floating housing is slowly gaining in popularity. The first floating house was located in Wrocław in 2010. Since then, many changes have taken place and the awareness of the society has been raised enough for floating houses to be accepted and generate interest of growing group of people [5,6]. The consequence of the phenomenon is the dynamic market development and substantial growth in the number of producers offering floating houses, which are ready to move into or designed according to the needs and indications of an investor. Having selected a floating house, an adequate anchoring system should be customized. That requires the analysis of numerous factors i.e., the cost of a construction, the operation time, the external risk factors and geospatial aspects. Their impact should be considered on each stage of the construction process, beginning with the preparatory phase, designing, and gaining the required administrative decisions, then the implementation stage, and finally, the phase of handing over the investment for operation and usage.

2. Materials

Until recently, construction on water has been associated with mainly single-family houses or habited barges. However, due to technological progress of foundation structure production, it has soon expanded its scope to the concept of floating car parks, public buildings, sport and leisure facilities or hotels in cities such as Amsterdam, Hamburg, Berlin, or Copenhagen [2].

A floating house is a floating object used for residential purpose, the same as a house built on land, however, the main difference between these objects is how they are attached to the ground.

An object located on land is attached to the ground by its foundations, in case of floating objects, anchoring involves holding a vessel in one place. Anchoring systems serve a purpose of vessel immobilization.

Irrespectively of a country, among many available technical solutions on the market, producers of Floating Houses prefer four types of anchoring: mooring piles, booms, mooring ropes, deadweight anchors with or without additional elastic connectors.

2.1. Mooring Piles

The first of the presented anchoring systems consists of:

• a sea-bedded pile,
• a clamp surrounding a pile,
• a buffer,
• a guide—an element moving on a pile in the form of e.g., rollers [7,8].

A pile is sea-bedded to the depth providing adequate load bearing capacity, its height above the water table should be adjusted in such a way that in the highest possible level of the water table and with waving, there will be no sliding of the guides of a platform (a float) from the pile shank [7]. Joining the pile to the floating pier is ensured by a clamp, which surrounds a pile and is linked with the anchor bolts to the pier. The clamp should be constructed in the way that allows the adequate space between the pile and the guide, in a form of e.g., rollers. The guides (rollers) are placed in each side of the pile. Their function is to ensure the movement of the platform along the pile as a result of waving or a change of the water level and minimization of the impacts of the platform against the pile as a result of wind pressure. Anchoring by means of piles is possible with the use of minimum two piles [7,8].

The mooring piles can be wooden, steel, plastic or reinforced concrete pre-compressed. Anchoring by means of the piles requires establishing horizontal loads resulting from, among other things, the wind and ice pressure, the speed of the current, or the waves parameter.

Applying of the anchoring system by means of a pile is recommended at long-term stops, with significant differences in water table levels, when it is necessary to hold a vessel in a stable position against a particular location [7]. The disadvantage of such a solution is the necessity of obtaining many permits connected with the construction and the usage phase. Since connecting a vessel to a pile is not permanent, there is a possibility of towing it to a different place, however, leaving piles in a specific location turns them into a navigational obstacle. Having towed a vessel, it should be marked with the navigational light in a way which would guarantee safety to other vessels in accordance to waterway rules for navigation. An option of using a pile for another floating house is unlikely due to their large variety in shape and size and the fact that piles are designed for an individual vessel. The noticeable shortcoming of the anchoring system applying steel piles is their corrosion in a place of fitting a sliding element such as rollers and breaking-off of the rollers and clamps by the impact of the ice or excessive waving. The system of fitting and dismantling is relatively difficult, for example, by changing the location of a floating house.

2.2. Booms

The anchoring system consists of:

• a boom (e.g., a steel pipe),
• a steel cable (a pull cable),
• a loop or a mooring hinge.

Anchoring using piles is performed in the form of minimum 2 steel pipes of the recommended diameter over 10 cm and minimum 2 m in length.

Pipes connect a float with a quay by means of the following set: two mooring loops, one loop and one mooring hinge or two mooring hinges. In the absence of the hinges, a mooring post at the quay and a cleat on the vessel are necessary. The anchoring system in review involves a use of minimum 4 pull cables (steel cables) of minimum 10 mm thickness, in configuration where minimum 2 of them are fitted in transverse layout. Whilst booms are aimed at offsetting a vessel from the quay in order to keep the safe distance, the pull cables draw a vessel to the quay and block the possibility of moving horizontally along the quay [3].

Anchoring by means of the booms is possible during long-term and short-term stops. It is recommended to use the booms on waters of variable level of water table, but less often than the steel piles. It is advisable to frequently check the technical state of the pull cables, because they tend to become worn as a result of the vertical movements of water and waving.

2.3. MooringLines

Anchoring by means of the mooring lines is the easiest method in use and the cheapest out of the discussed solutions. It consists of a mooring line with a loop, often in a cover. There must be a mooring post at a quay and the line at the end must be attached to the vessel. In case of Floating Houses, their use is recommended during short-term stops, on waters with the minimal changes of water table levels and minimal waving. With the long-term stops, another form of anchoring is recommended due to a possibility of mechanical damage as a result of friction, intersection, or rupture.

The cost of constructing particular anchoring systems is varied, the cheapest in completion, usage and damage is the anchoring system using mooring ropes, despite the quite frequent necessity of changing the mooring rope.

2.4. Deadweight Anchors Using Elastic Connectors

The last analysed anchoring system is mooring using deadweight anchors, with a possibility of using elastic connectors.

The system consists of:

• deadweight anchors,
• rope (the pull cable),
• additional pulling element (e.g., elastic connector).

Two types of anchors can be distinguished—the active and deadweight anchors. The latter are made as iron or reinforced concrete and concrete blocks (boards), concave from underneath, which ensures their stronger attachment to the bottom of the basin [7]. The application of the deadweight anchors attached to a Floating House with the ropes is called the system of the pull cables.

The following 4 types of pull cables can be distinguished:

• homogenous pull cables-chains or lines,
• mixed pull cables—a line joined with a chain or a chain with a thin tape,
• pull cables with weights—e.g., a line with hanging weights,
• pull cables with flotation elements [7].

Homogenous pull cables in the form of chains, highly-resistant steel lines, or synthetic fibre ropes are predominantly applied whilst anchoring floating houses. The basic parameters of a pull cable are: the unit weight, the rupture resistance, the material extension, the diameter of lines and the gauge of a chain. The weight of one pull cable has a deciding impact on the movement of an anchored floating house and the longitudinal material indirectly affects the load on the pull cable and the possibility of its rupture [7].

In the system of the pull cables, they began to apply the system of connectors Seaflex (trademark of the producer who first used this solution) consisting of the rubber cables with a rubber core and also coated with a layer of rubber. These cables are very flexible and allow stretching equal to twice the length of the cable. It offers flexible impact of a Floating House on operation of the waves [7].

The right selection of the length of the pull cables, the weight of the anchor, the number of ropes, and their positioning on seabed makes it that using this system is recommended for mid- and long-term stops. Mooring exclusively by means of the deadweight anchors is very rare, usually it is an additional system for the spurs of floating platforms, where the main line of platforms is moored with piles. Application of Seaflex system is increasingly more popular. The right arrangement of the deadweight anchors and ropes under the sea level requires the assistance of a diver. The system can be applied in basins with a stable or low variability of water table level [7] at a safe distance from the waterway, having obtained the necessary permits required by the law.

3. Methods

3.1. Methodology

The analytic hierarchy process (AHP), developed by Thomas Saaty [9], is an effective method for dealing with complex decision-making from the elicited judgments from experts [10]. This method helps decision-makers set priorities between alternatives, sub-criteria and criteria in the decision-making process and also helps them to make the best decision [11,12,13,14,15,16,17].

The analytic hierarchy process (AHP) in short consists of 8 stages:

• Prioritizing a problem—the aim of this stage is the detailed description of the problem, identification of the participants, defining the main objective and expectations. Thereafter, a decomposition of the problem is undertaken in the form of the primary objective, the main and partial factors and variants considered, which generate some fulfillment of aims function on particular levels of the hierarchical model. The general structure of the hierarchy is presented in Figure 1.

• The assessment of the criteria by comparison with pairs—it is conducted by a decision maker, who compares the pairs with each other using the criteria and the criteria in relation to the primary objective on the basis of subjective decision as to which of the criterion and to what extent is more important than the other. The relations between the particular elements are established on the basis of the 9-point scale presented in

Table 1. Fundamental scale of absolute numbers [18,19,20].

Definition	Intensity of Importance
Equal Importance	1
Weak or slight	2
Moderate importance	3
Moderate plus	4
Strong importance	5
Strong plus	6
Very strong or demonstrated importance	7
Very, very strong	8
Extreme importance	9

[18,19,20].

• The next stage is to enter data received from the judges in the matrix of comparison with pairs A. The general record of matrix A is as follows:

$$\mathrm{A}=\left[\begin{array}{cccc}{a}_{11}& a_{12}& \dots & a_{1n}\\ a_{21}& a_{22}& \dots & a_{2n}\\ ⋮& ⋮& ⋮& ⋮\\ a_{\mathrm{n}1}& a_{\mathrm{n}2}& \dots & a_{\mathrm{nn}}\end{array}\right].$$

(1)

The attribute of matrix A is presented in the formula below:

$$a_{ij}=1, \mathrm{dla} i=j$$

(2)

and

$$a_{ij}=\frac{1}{a_{\mathrm{ji}}},$$

(3)

which indicates that the matrix of comparison with pairs A is the conversely symmetric matrix. As a result, the following record is produced:

$$\mathrm{A}=\left[\begin{array}{cccc}1& a_{12}& \dots & a_{1n}\\ \frac{1}{a_{12}}& 1& \dots & a_{2n}\\ ⋮& ⋮& ⋮& ⋮\\ \frac{1}{a_{1n}}& \frac{1}{a_{2n}}& \dots & 1\end{array}\right].$$

(4)

• As a result of the undertaken calculations, weights describing the meaning of a specific element are obtained. The scope of the values of weighting factors is defined by the formula below:

$$w_1+w_2+\dots +w_n=1 \wedge w_i\ge 0.$$

(5)

Matrix A is compatible when the following relation occurs:

$$a_{ij}=\frac{w_i}{w_j},$$

(6)

that is:

$$a_{ij}*\frac{w_j}{w_i}=1.$$

(7)

Applying relation (7) and the scalar notations, the following relation is obtained [21]:

$${\sum }_{j=1}^n{a}_{ij}*\frac{w_j}{w_i}=n\iff {\sum }_{j=1}^n{a}_{ij}*w_j=n*w_i,$$

(8)

or:

$$\mathrm{Aw}=\left[\begin{array}{cccc}\frac{w_1}{w_1}& \frac{w_1}{w_2}& \dots & \frac{w_1}{w_n}\\ \frac{w_2}{w_1}& \frac{w_2}{w_2}& \dots & \frac{w_2}{w_n}\\ ⋮& ⋮& ⋮& ⋮\\ \frac{w_{\mathrm{n}}}{w_1}& \frac{w_n}{w_1}& \dots & \frac{w_{\mathrm{n}}}{w_n}\end{array}\right]*\left[\begin{array}{c}{w}_1\\ w_2\\ ⋮\\ w_{\mathrm{n}}\end{array}\right]=n*\left[\begin{array}{c}{w}_1\\ w_2\\ ⋮\\ w_{\mathrm{n}}\end{array}\right]=nw.$$

(9)

In practice, a matrix is very rarely compatible. Thus, a vector of relative values of weighting factors is set, as a solution of the matrix equation, corresponding to the characteristic equation of the matrix with comparison of pairs A:

$$\mathrm{Aw}=\left[\begin{array}{cccc}{a}_{11}& a_{12}& \dots & a_{1n}\\ a_{21}& a_{22}& \dots & a_{2n}\\ ⋮& ⋮& ⋮& ⋮\\ a_{\mathrm{n}1}& a_{\mathrm{n}2}& \dots & a_{\mathrm{nn}}\end{array}\right]*\left[\begin{array}{c}{w}_1\\ w_2\\ ⋮\\ w_{\mathrm{n}}\end{array}\right]=cw,$$

(10)

where: c—certain variable, w—own vector of matrix A [22].

• Equation (10) has a solution if the fixed value c is the greatest value of the own vector [18] of matrix A. This value is denoted by the symbol ${\mathsf{\lambda }}_{\max }$ (principal eigenvalue) therefore, the Equation (10) takes the form of [22]:

$$\mathrm{Aw} ={ \mathsf{\lambda }}_{\max }w,$$

(11)

$$\mathrm{and} \mathrm{the} \mathrm{formula} \mathrm{for} {\mathsf{\lambda }}_{\max } \mathrm{can} \mathrm{be} \mathrm{written} \mathrm{in} \mathrm{the} \mathrm{following} \mathrm{way}:$$

$${\mathsf{\lambda }}_{\max }=\frac{1}{{\mathrm{w}}_{\mathrm{i}}} {{\displaystyle \sum }}_{\mathrm{j}=1}^{\mathrm{n}}{\mathrm{a}}_{\mathrm{ij}}{\mathrm{w}}_{\mathrm{j}}.$$

(12)

• The second factor necessary to obtain the AHP method is the CI (Consistency Index). It is the negative average of the other roots of the characteristic polynomial of A [23,24]:

$$\mathrm{CI}=\frac{{\mathsf{\lambda }}_{\max }-\mathrm{n}}{\mathrm{n}-1},$$

(13)

• The last factor is CR (consistency ratio). If the ratio of CI is significantly small, the estimate of w can be accepted. CR is determined by the formula [23]:

$$\mathrm{CR}=\frac{\mathrm{CI}}{\mathrm{RI}}<\mathrm{0,10},$$

(14)

where: RI–random index [23,25,26].

In the case where λ max = n and CI = 0, CI index is calculated with regard to the random index RI, which is the average value of CI for a large number of random generated matrix of comparisons. The value of RI is provided in the

Table 2. Value of index RI [27,28].

n	RI	n	RI
2 3 4 5 6	0 0.52 0.89 1.11 1.25	7 8 9 10 12	1.35 1.40 1.45 1.52 1.54

[23,27,28].

• The analysis of the selected results—choosing the best variant, which would address the main objective [23].

3.2. Group Decision Making

The AHP method is very frequently used as the group decision making (GDM). There are two approaches to this issue—the behavioural and the mathematical [29]. The most often used among the behavioral methods are: the debate and brainstorming [30], the six hats method [31], the focus group [32], and the Delphi method [33]. The mathematical methods of aggregation of experts’ opinions are: the arithmetic mean, the geometric mean, the weighted average (arithmetic and geometric), the median and the mode. Additionally, within the mathematical aggregation of assessments of the people participating in the decision making for the AHP method, two approaches can be identified [34]:

• Aggregating individual judgements (AIJ),
• Aggregating individual priorities (AIP).

In the context of the research carried out, the authors decided to apply the brainstorming method from the behavioural methods, also known as qualitative methods. Brainstorming is a process within the heuristic methods, which requires creativity and involves free access to new ideas and the exchange of opinions [22]. The correct progress of the brainstorming session should commence with appointing a leader, who has the following tasks:

• identifying a problem,
• the appropriate selection of the participants
• 5 to 10 is recommended,
• designation of a place and length of a session,
• setting a place to carry out a brainstorming session,
• presentation of the rules of the session to the participants—guidance of the session—recommended time—45 min,
• formulation of the results [35].

A hydrotechnical company was invited to participate in the session of brainstorming. Four engineers took part in the study. The session of brainstorming was organised in the head office of the hydrotechnical company, in the time suitable for the participants. The session was held in the main room of the office, and there were only the participants and the representative of the authors as a leader present. After the rules of the meeting had been presented, the session commenced and lasted longer than the recommended time. As a result of the meeting, a consensus was reached, that is the common position of the participants when filling in the questionnaire using the 9-grade scale of Saatie for the issue in question. The authors are aware that there is the group-thinking in brainstorming, that is a phenomenon [36] when one suppresses critical opinions against the way of thinking of the strong individuals in the group. Thereby, the input of the people who do not have the impetus is lessen, as they are not able to at least to counterbalance with other opinions. This phenomenon is based on the tendency of an individual to share the positions and views, which are perceived as favoured by the group [22]. This phenomenon has been minimalised by the selection of the judges participating in the study, who are the individuals of similar age and engeering experience, who have been co-operating professionally in the implementation of the shared visions and engeering projects.

4. Results

The subject of this research is a comparative analysis of the weights for the selection [37] of anchoring system for floating house by means of AHP method. The analysis using AHP method was conducted in SuperDecisions program [38] and using AHP-OS software [39].

4.1. Input Data

The first examined criterion is the cost, which appears in each phase of a life—cycle of an object (LCC Life Cycle Cost), namely in the phase of the construction/completion, the usage and the damage [40,41]. The construction/completion of the anchoring system is a serious financial strain taking place in the initial phase of an investment process. The expenditure incurred by an Investor is substantial, however, excessive thriftiness at this stage of the construction/completion results in the increased expenditure during the usage. The cost of the usage is fundamental because it takes place in the longer run. The financial expenditure, which is the consequence of a damage, is difficult to predict, but thanks to purchasing the products that are on offer on the insurance market, it can be fully or partially covered, which reduces the cost of the repairs significantly.

The next sub-criterion considered is the time criterion. Time is vital for an Investor during the designing and obtaining agreements, the constructing/completing system of anchoring and the expected life-cycle. Nowadays, time devoted to designing is relatively short with modern technological and technical possibilities, however, time devoted to obtaining permits connected with the local conditions, the ownership issues or the anchoring system specificity is vital and can prolong the investment process considerably. The time of completion/installation of the mooring system is also connected with the expected time of the usage and the adequate financial outlay. The more durable and complicated to complete anchoring system, the longer the expected time of usage.

The geospatial factors are connected with the location-based conditions and the scope of applying the particular anchoring systems. The parameters of the water area, such as its size (width), depth, current, type of shore or natural waving determine particular technical solutions. The anchoring system satisfying the requirements of the specific water area can turn out not necessarily sufficient elsewhere. The type of ground is vital because it determines the connection of a floating object with the ground covered with water or with the shore. Icing is connected directly with the safety of a vessel and potential emergency situations during winter.

The last criterion is the risk associated with the emergency situations, flood, draught or the intentional human activity. Flood and drought are two extreme sub-criteria differentiating the potential of applying certain anchoring solution, which will fully secure a house against flood, but if used in time of draught it can cause the opposite effect. The intentional human activity refers to the unpredictable situations where the main threat factor is a human, who caused damage to the anchoring system with his/her indirect or direct action. The situation threatens a vessel such as a floating house. The damage, which is the result of the incorrect usage, design and workmanship defects, is relatively rare. One can get insured against all the sub-criteria from the risk group and as a result, the relevance of the indicated factors for an Investor is minimal.

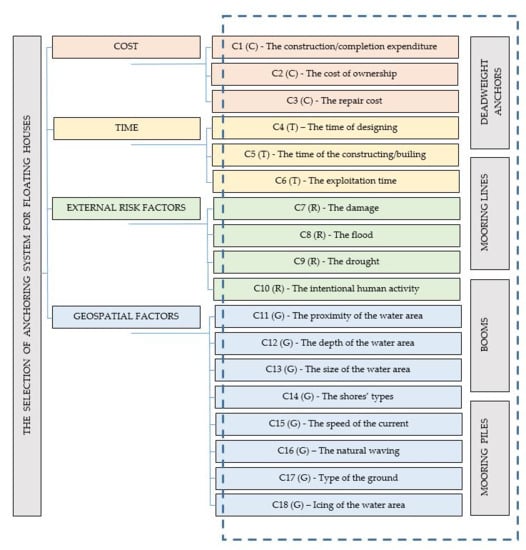
4.2. Hierarchy Structure Tree

The basis of the hierarchical structure tree (Figure 2) was a set of criteria proposed in the on assessment by the judges at the Brainstorm session. As a result of the AHP analysis of the most advantageous anchoring system for floating houses, four main criteria have been distinguished, i.e., cost, time, external risk factors, and geospatial factors. Each of the main criteria includes sub-criteria presented in Figure 2.

A hierarchical structure tree was developed, in which the following levels were distinguished:

• Goal: the selection of anchoring system for floating houses;
• Groups of criteria: time (T), cost (C), external risk factors (R), geospatial factors (E);
• Criteria: the construction/completion expenditure C1 (T), the cost of ownership C2 (T), the repair cost C3 (T), the time of designing C4 (C), the time of the constructing/builing C5 (C), the exploitation time C6 (C), the damage C7 (R), the flood C8 (R), the drought C9 (R), the intentional human activity C10 (R), ), the proximity of the water way C11 (G), the depth of the water area C12 (G), the size of the water area C13 (G), the shores’ types C14 (G), the speed of the current C15 (G), the natural waving C16 (G), a type of the ground C17 (G), icing of the water area C18 (G);
• Alternatives: mooring piles, booms, mooring lines, deadweight using elastic connectors [37].

4.3. Comparison Matrices

A series of pairwise comparisons of individual elements was subsequently made at each decision-making level (

Table 3. Comparison matrix for criteria groups.

	Cost	Time	External Risk f.	Geospatial f.	Priority Vector
Cost	1	4	7	5	0.61434
Time	$\frac{1}{4}$	1	3	2	0.19717
External risk f.	$\frac{1}{7}$	$\frac{1}{3}$	1	$\frac{1}{2}$	0.06988
Geospatial f.	$\frac{1}{5}$	$\frac{1}{2}$	2	1	0.11861
CR = 0.01696

Source: authors’ own work.

,

Table 4. Comparison matrix for Cost criteria group.

C—Group	C1	C2	C3	Priority Vector
C1	1	$\frac{1}{3}$	3	0.24264
C2	3	1	7	0.66942
C3	$\frac{1}{3}$	$\frac{1}{7}$	1	0.08795
CR = 0.00675

Source: authors’ own work.

,

Table 5. Comparison matrix for Time criteria group.

T—Group	C4	C5	C6	Priority Vector
C4	1	$\frac{1}{4}$	$\frac{1}{8}$	0.07325
C5	4	1	3	0.25596
C6	8	$\frac{1}{3}$	1	0.67079
CR = 0.01759

Source: authors’ own work.

,

Table 6. Comparison matrix for External Risk Factors criteria group.

R—Group	C7	C8	C9	C10	Priority Vector
C7	1	6	7	3	0.57255
C8	$\frac{1}{6}$	1	4	$\frac{1}{3}$	0.11776
C9	$\frac{1}{7}$	$\frac{1}{4}$	1	$\frac{1}{6}$	0.04844
C10	$\frac{1}{3}$	3	6	1	0.26126
CR = 0.07311

Source: authors’ own work.

,

Table 7. Comparison matrix for Geospatial Factors criteria group.

G—Group	C11	C12	C13	C14	C15	C16	C17	C18	Priority Vector
C11	1	$\frac{1}{5}$	$\frac{1}{6}$	2	$\frac{1}{2}$	$\frac{1}{4}$	4	3	0.06376
C12	5	1	$\frac{1}{2}$	4	3	2	7	6	0.22049
C13	6	2	1	7	5	4	9	8	0.36584
C14	$\frac{1}{2}$	$\frac{1}{4}$	$\frac{1}{7}$	1	$\frac{1}{2}$	$\frac{1}{3}$	3	2	0.04954
C15	2	$\frac{1}{3}$	$\frac{1}{5}$	2	1	$\frac{1}{3}$	5	4	0.08962
C16	4	$\frac{1}{2}$	$\frac{1}{4}$	3	3	1	6	5	0.15767
C17	$\frac{1}{4}$	$\frac{1}{7}$	$\frac{1}{9}$	$\frac{1}{3}$	$\frac{1}{5}$	$\frac{1}{6}$	1	$\frac{1}{2}$	0.02228
C18	$\frac{1}{3}$	$\frac{1}{6}$	$\frac{1}{8}$	$\frac{1}{2}$	$\frac{1}{4}$	$\frac{1}{5}$	2	1	0.03080
CR = 0.03771

Source: authors’ own work.

,

Table 8. Comparison matrix alternative for Cost criteria group.

C—Group	Mooring Piles	Booms	Mooring Lines	Deadweight Anchors	Priority Vector	Mooring Piles	Booms	Mooring Lines	Deadweight Anchors	Priority Vector
	C1—The Construction/Completion Expenditure					C2—The Cost Of Ownership
Mooring Piles	1	$\frac{1}{4}$	$\frac{1}{7}$	$\frac{1}{2}$	0.06288	1	2	$\frac{1}{5}$	3	0.17827
Booms	4	1	$\frac{1}{4}$	3	0.22956	$\frac{1}{2}$	1	$\frac{1}{6}$	3	0.12254
Mooring Lines	7	4	1	5	0.60370	5	6	1	6	0.63435
Deadweight Anchors	2	$\frac{1}{3}$	$\frac{1}{5}$	1	0.10386	$\frac{1}{3}$	$\frac{1}{3}$	$\frac{1}{6}$	1	0.06483
	CR = 0.04129					CR = 0.06644
	C3—The Repair Cost
Mooring Piles	1	$\frac{1}{5}$	$\frac{1}{6}$	3	0.09058
Booms	5	1	$\frac{1}{3}$	7	0.29963
Mooring Lines	6	3	1	8	0.56480
Deadweight Anchors	$\frac{1}{3}$	$\frac{1}{7}$	$\frac{1}{8}$	1	0.04500
	CR = 0.07159

Source: authors’ own work.

,

Table 9. Comparison matrix alternative for Time criteria group.

T—Group	Mooring Piles	Booms	Mooring Lines	Deadweight Anchors	Priority Vector	Mooring Piles	Booms	Mooring Lines	Deadweight Anchors	Priority Vector
	C4—The Time of Designing					C5—The Time of The Constructing/Building
Mooring Piles	1	$\frac{1}{6}$	$\frac{1}{7}$	$\frac{1}{3}$	0.04965	1	$\frac{1}{4}$	$\frac{1}{6}$	3	0.09461
Booms	6	1	$\frac{1}{4}$	4	0.26215	4	1	$\frac{1}{4}$	6	0.25207
Mooring Lines	7	4	1	5	0.58619	6	4	1	8	0.60679
Deadweight Anchors	3	$\frac{1}{4}$	$\frac{1}{5}$	1	0.10201	$\frac{1}{3}$	$\frac{1}{6}$	$\frac{1}{8}$	1	0.04653
	CR = 0.09430					CR = 0.07889
	C6—The Exploitation Time
Mooring Piles	1	8	7	4	0.61074
Booms	$\frac{1}{8}$	1	$\frac{1}{3}$	$\frac{1}{6}$	0.04531
Mooring Lines	$\frac{1}{7}$	3	1	$\frac{1}{5}$	0.08437
Deadweight Anchors	$\frac{1}{4}$	6	5	1	0.25958
	CR = 0.09759

Source: authors’ own work.

,

Table 10. Comparison matrix alternative for External risk factors criteria group.

R—Group	Mooring Piles	Booms	Mooring Lines	Deadweight Anchors	Priority Vector	Mooring Piles	Booms	Mooring Lines	Deadweight Anchors	Priority Vector
	C7—The Damage					C8—The Flood
Mooring Piles	1	7	7	4	0.61469	1	7	8	4	0.62046
Booms	$\frac{1}{7}$	1	1	$\frac{1}{5}$	0.06489	$\frac{1}{7}$	1	3	$\frac{1}{4}$	0.09186
Mooring Lines	$\frac{1}{7}$	1	1	$\frac{1}{5}$	0.06489	$\frac{1}{8}$	$\frac{1}{3}$	1	$\frac{1}{5}$	0.04867
Deadweight Anchors	$\frac{1}{4}$	5	5	1	0.25553	$\frac{1}{4}$	4	5	1	0.23900
	CR = 0.05274					CR = 0.07668
	C9—The Drought					C10—The Intentional Human Activity
Mooring Piles	1	6	6	4	0.60434	1	7	8	3	0.57445
Booms	$\frac{1}{6}$	1	1	$\frac{1}{4}$	0.07612	$\frac{1}{7}$	1	4	$\frac{1}{5}$	0.09609
Mooring Lines	$\frac{1}{6}$	1	1	$\frac{1}{4}$	0.07612	$\frac{1}{8}$	$\frac{1}{4}$	1	$\frac{1}{6}$	0.04382
Deadweight Anchors	$\frac{1}{4}$	4	4	1	0.24341	$\frac{1}{3}$	5	6	1	0.28564
	CR = 0.04591					CR = 0.10222

Source: authors’ own work.

and

Table 11. Comparison matrix alternative for External risk factors criteria group.

G—Group	Mooring Piles	Booms	Mooring Lines	Deadweight Anchors	Priority Vector	Mooring Piles	Booms	Mooring Lines	Deadweight Anchors	Priority Vector
	C11—The Proximity of the Water Area					C12—The Depth of the Water Area
Mooring Piles	1	3	$\frac{1}{4}$	4	0.23608	1	$\frac{1}{4}$	$\frac{1}{4}$	1	0.1
Booms	$\frac{1}{3}$	1	$\frac{1}{4}$	4	0.13521	4	1	1	4	0.4
Mooring Lines	4	4	1	7	0.57475	4	1	1	4	0.4
Deadweight Anchors	$\frac{1}{4}$	$\frac{1}{4}$	$\frac{1}{7}$	1	0.05397	1	$\frac{1}{4}$	$\frac{1}{4}$	1	0.1
	CR = 0.09037					CR = 0.00000
	C13—The Size of the Water Area					C14—The Shores’ Type
Mooring Piles	1	3	1	5	0.38986	1	6	8	4	0.60006
Booms	$\frac{1}{3}$	1	$\frac{1}{3}$	3	0.15235	$\frac{1}{6}$	1	3	$\frac{1}{5}$	0.08876
Mooring Lines	1	3	1	5	0.38986	$\frac{1}{8}$	$\frac{1}{3}$	1	$\frac{1}{6}$	0.04558
Deadweight Anchors	$\frac{1}{5}$	$\frac{1}{3}$	$\frac{1}{5}$	1	0.06792	$\frac{1}{4}$	5	6	1	0.26559
	CR = 0.01629					CR = 0.09951
	C15—The Speed of the Current					C16—The Natural Waving
Mooring Piles	1	4	2	6	0.48521	1	5	6	4	0.59122
Booms	$\frac{1}{4}$	1	$\frac{1}{4}$	3	0.12311	$\frac{1}{5}$	1	$\frac{1}{2}$	$\frac{1}{3}$	0.07614
Mooring Lines	$\frac{1}{2}$	4	1	5	0.33147	$\frac{1}{6}$	2	1	$\frac{1}{4}$	0.09625
Deadweight Anchors	$\frac{1}{6}$	$\frac{1}{3}$	$\frac{1}{5}$	1	0.06021	$\frac{1}{4}$	$3$	4	1	0.23640
	CR = 0.04708					CR = 0.08240
	C17—The Type of the Ground					C18—Icing of the Water Area
Mooring Piles	1	$\frac{1}{7}$	$\frac{1}{7}$	$\frac{1}{4}$	0.04721	1	3	$\frac{1}{6}$	$\frac{1}{4}$	0.09675
Booms	7	1	1	5	0.41961	$\frac{1}{3}$	1	$\frac{1}{8}$	$\frac{1}{5}$	0.04910
Mooring Lines	7	1	1	5	0.41961	6	8	1	4	0.60928
Deadweight Anchors	4	$\frac{1}{5}$	$\frac{1}{5}$	1	0.11357	4	5	$\frac{1}{4}$	1	0.24487
	CR = 0.05274					CR = 0.07681

Source: authors’ own work.

).

One of the basic criterion showing the reliability of the results obtained is the CR factor (see formula 13), which should be <0.1. It is possible to observe exceeding the allowable CR value for criteria C10 (CR = 0.10222) and C14 (CR = 0.9951). Exceeding this indicator may indicate inconsistency of the comparative matrix. In such a situation, a genetic algorithm (GA) can be used [42] or a three-step procedure lowering the CR value [43] based on the identification of the most incompatible matrix elements. However, such operations lead to a change in the original information obtained at the data collection stage [44], i.e., they change the information obtained from judges for the needs of the author of the study. Therefore, the CR factor value was not reduced in the publication, the more so that the results obtained in this study slightly exceed the allowable CR value <0.10 [22].

The global priorities indicate in the percentage terms, the significance of a particular factor for the criterion of the aim, which is the selection of the most advantageous anchoring system for the Investor. The percentage split of the global priorities is presented in detail in Figure 3.

The complete result set of the conducted analysis of analytical hierarchical process with the indication of the most beneficial system of mooring for an investor is shown in Figure 4.

The AHP method is time-consuming for a large number of main and partial criteria. It is also the subject of many studies and research, dividing the scientists into supporters and opponents of the method. Despite some critical opinions based on various proofs, the process of decision making using AHP can be applied in practice due to participation of experts whilst conducting the analysis. Both—the adequate selection of the group of experts, producing the correct mathematical calculations and critical analysis of the results, determine the sense of applying AHP method in the analyses of the different types of technological solutions and investments (based on [23]).

5. Conclusions

Although the AHP method is time-consuming, with a large number of main and sub-criteria, it is an excellent tool to analyze issues connected with the selection of the most advantageous technological solution, among others, the system of anchoring floating houses. As shown in the example of the criteria comparison aiming to present the most relevant factors for an investor and realistically occurring in the designing phase, the construction/completion and the operation, the AHP method allows a detailed analysis of the issue and real problems as well as the presentation of the problem in the hierarchical model. Therefore, the method allows the precise overview of the criteria, which are subject to expert assessment and appropriate mathematical analysis and indicate a hierarchy of the issues being considered in the achievement of the primary objective. The analysis of the hierarchical model can serve the educational purpose since it illustrates the complexity of the process and considers almost all its aspects.

In Poland there is a noticeable lack of regulations related to the functioning of houses on the water. This is mainly due to the fact that they are not subject to the provisions on floating objects or civil engineering, through no contact with the ground. Companies that sell ready-made units or design them to order leave the choice of anchoring system to the future user, who in most cases is not an expert in the field of floating objects.

Unfortunately, it is difficult to recall literature items referring to this issue in Poland, because in the scope of anchoring specific units, which are floating houses, there were no key publications dealing with this issue. The most frequently discussed directions of scientists’ interests are issues related to floating architecture [45], location [4], and legal issues [2,6,46].

The study has concluded that the cost factor in building on water like civil engineering is of key importance, especially the cost of ownership, then the main criteria include time, the geospatial factors, and the external risk factors. The sub-criterion that determines the selection of the anchoring system is the cost of the use, owing to which the most advantageous out of the most used systems of anchoring by the constructors of floating houses proved to be the mooring cables with as much as 47.6%, followed by the mooring piles with 25.8%, followed by the booms with 15.1%, and the deadweight anchors with 11.5% [23].

This publication used scientific tools to solve the problems faced by people unrelated to scientific or technical issues. The use of the AHP method guarantees that the indicated anchoring system would be chosen objectively and not subjectively.