Fiber-Reinforced Polymers in Freeform Structures: A Review
Abstract
:1. Introduction
1.1. What Are Freeform Structures?
1.2. FRP Composites
1.3. Applications of FRP Composites in Freeform Architecture
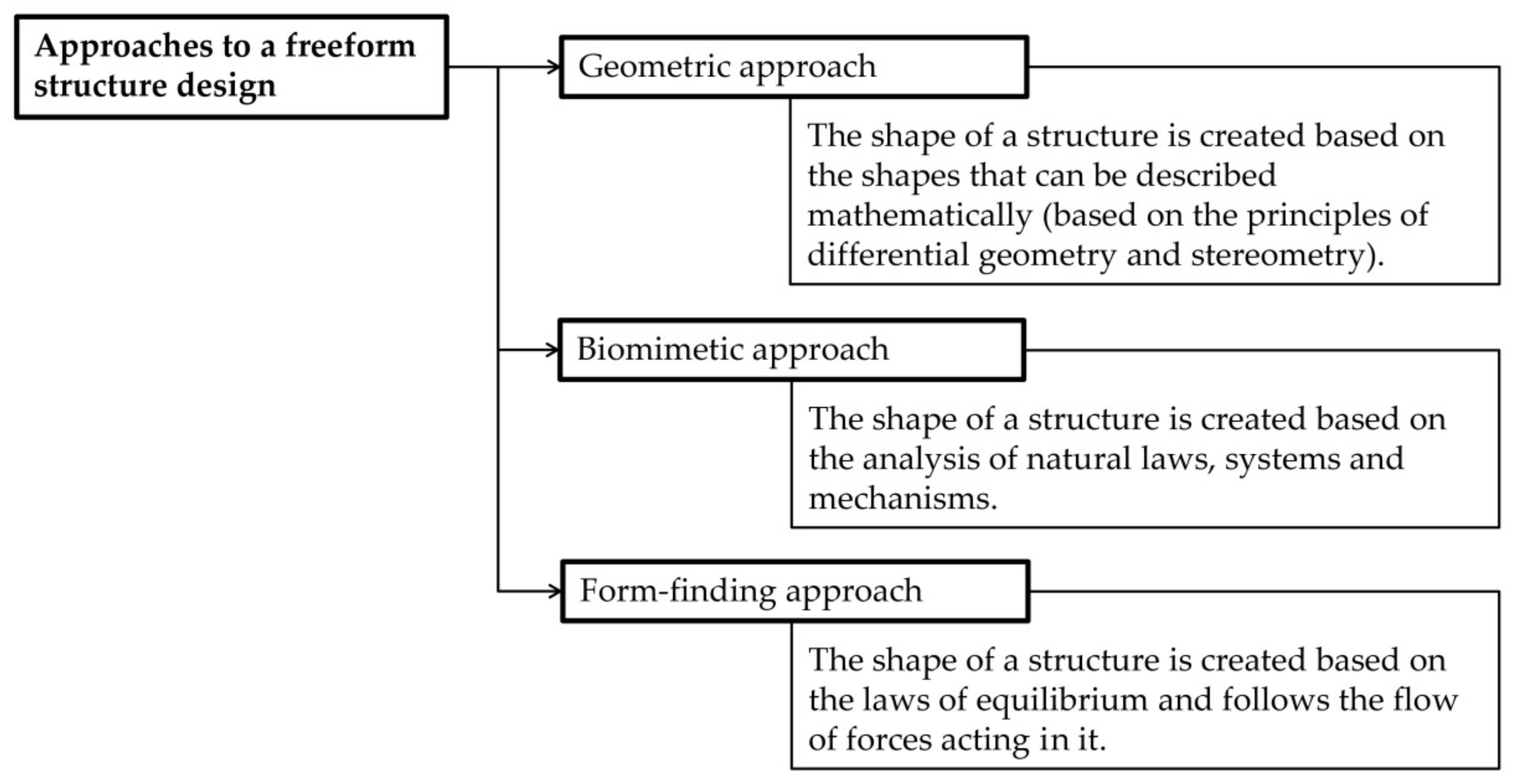
2. Freeform Structures Created by Using the Geometric Approach
2.1. What Is the Geometric Approach?
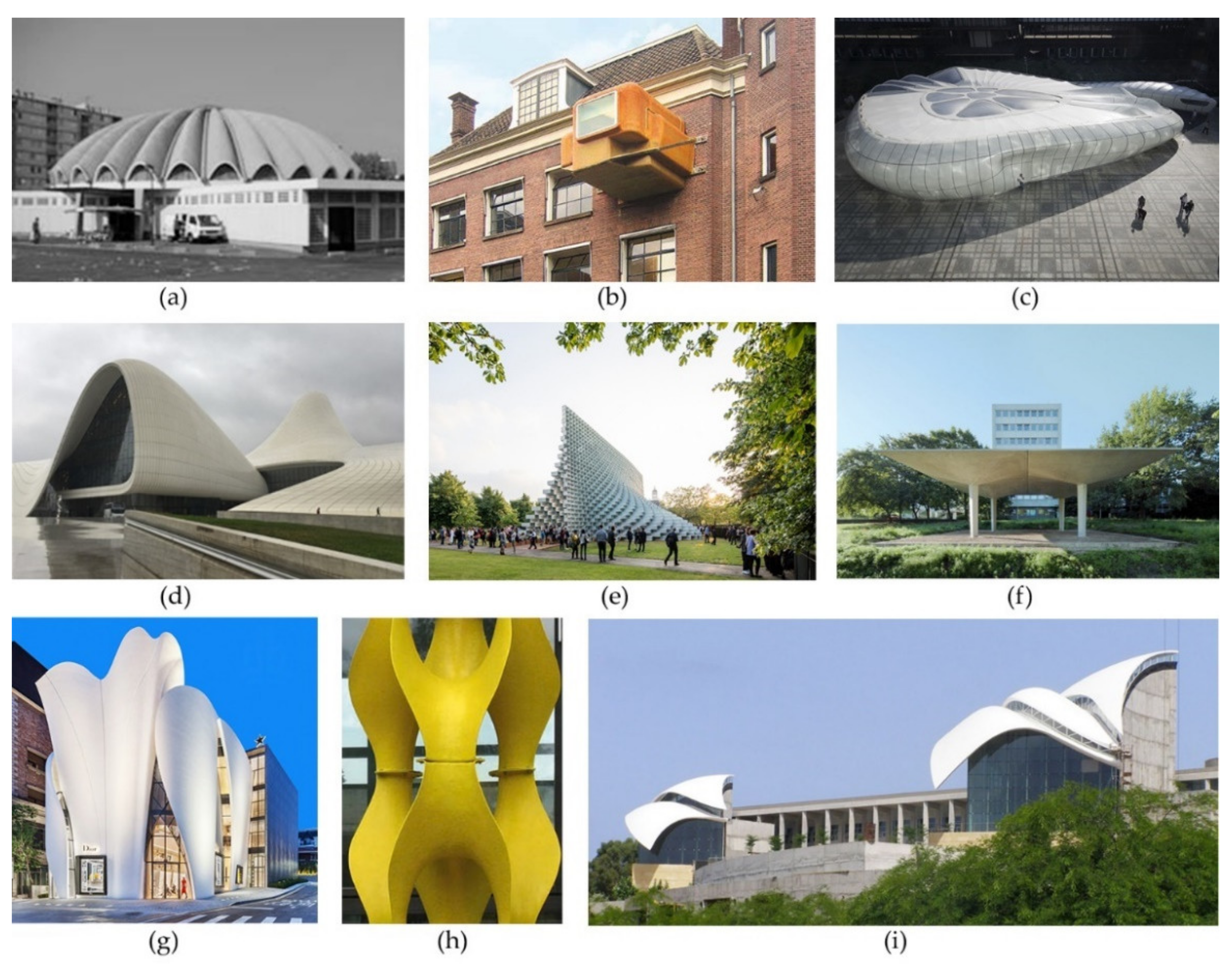
2.2. Geometrical Composite Freeform Structures: Case Studies
3. Freeform Structures Created by Using the Biomimetic Approach
3.1. What Is Biomimetics?
3.2. Biomimetic Composite Freeform Structures: Case Studies

4. Freeform Structures Created by Using the Form-Finding Approach
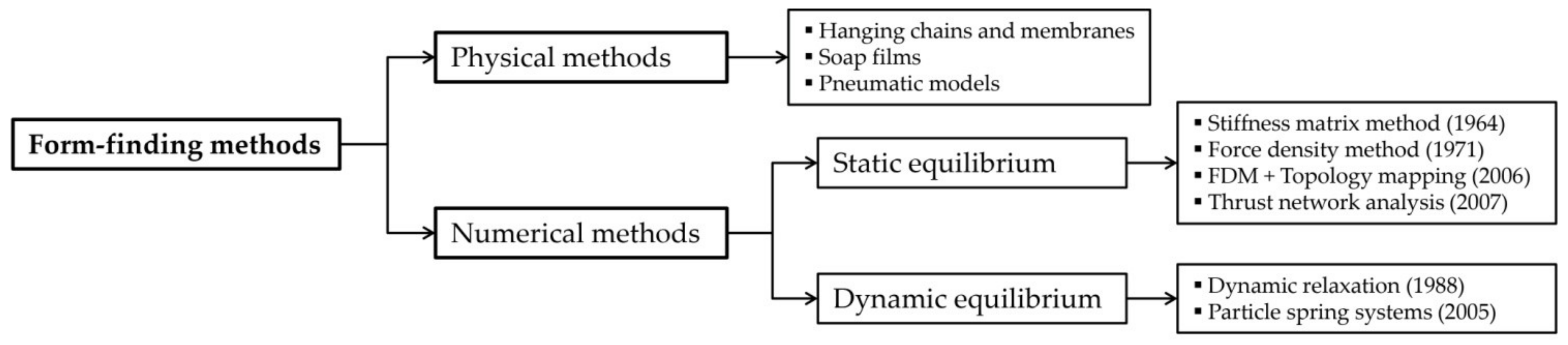
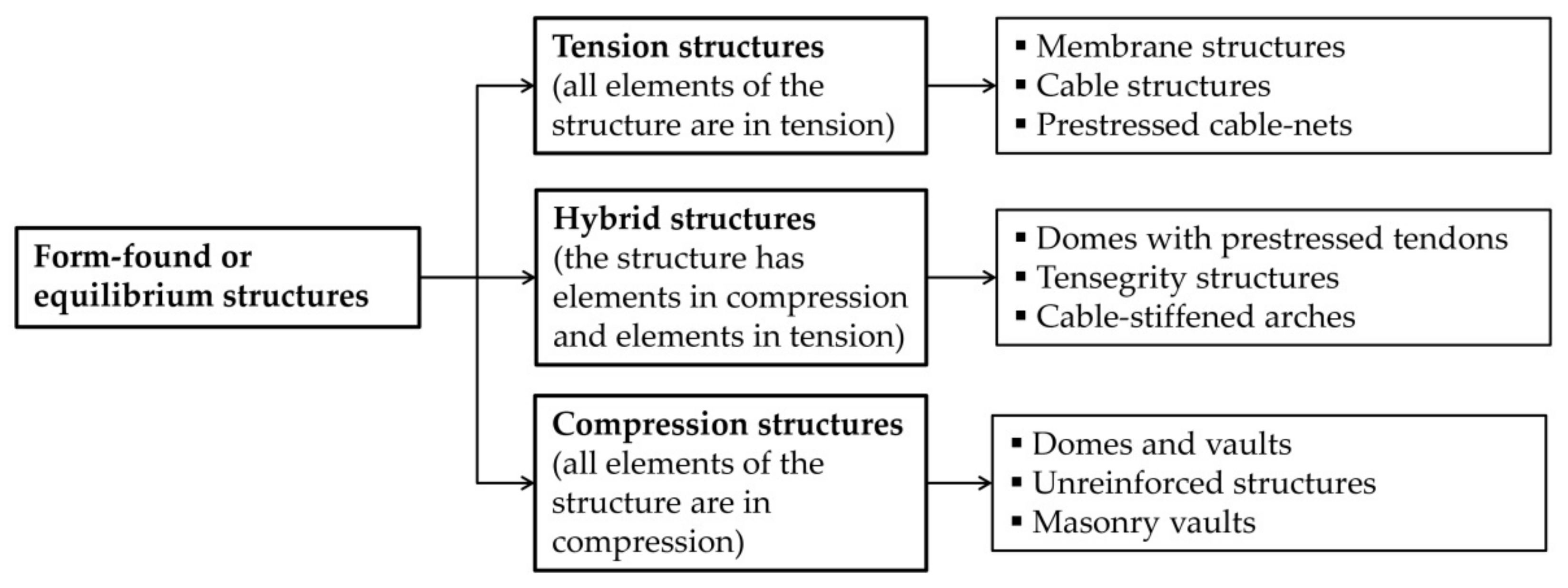
4.1. What Is Form-Finding?
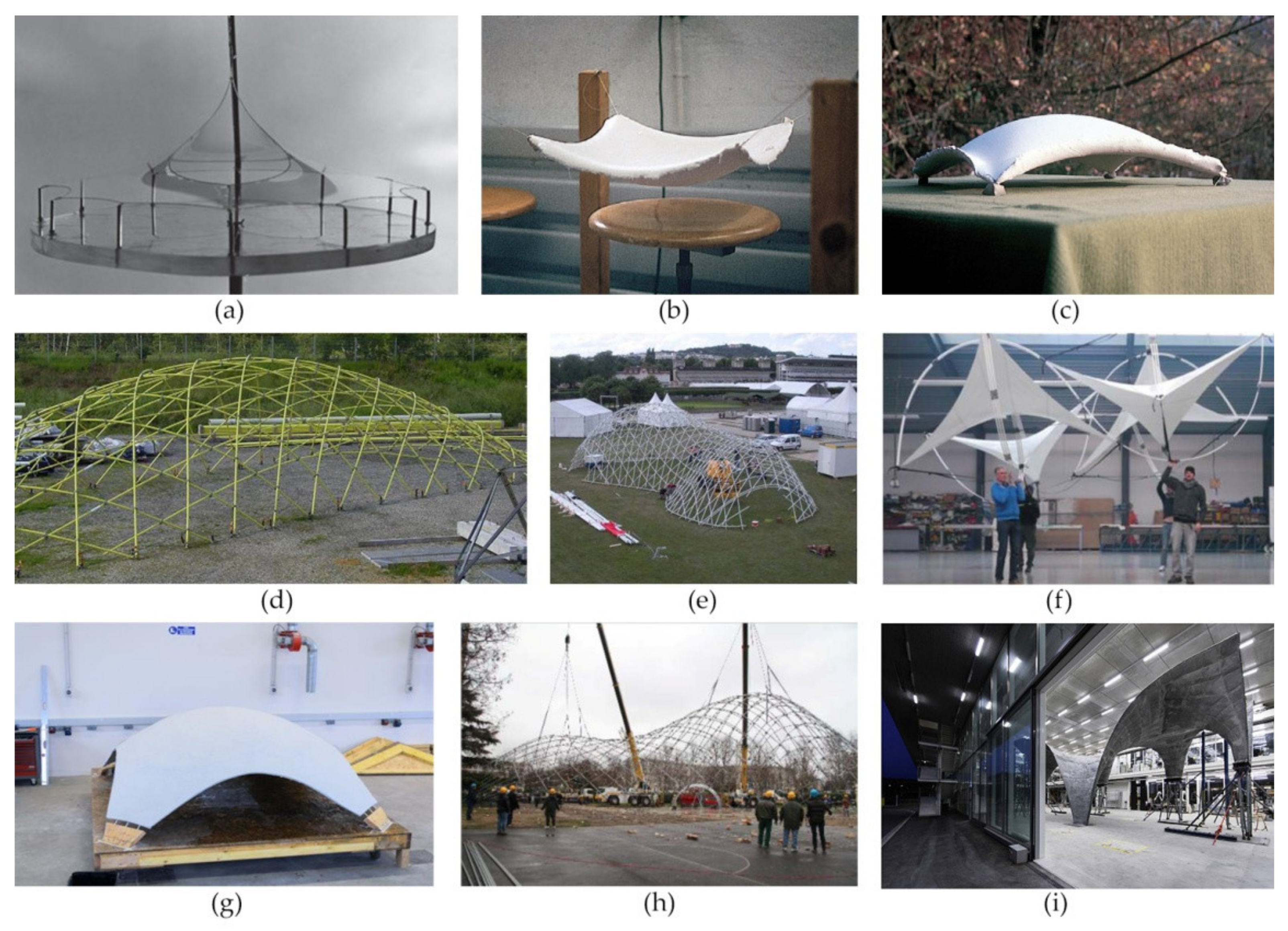
4.2. Form-Found Composite Freeform Structures: Case Studies
5. Future Trends
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Veltkamp, M. Free Form Structural Design: Schemes, Systems & Prototypes of Structures for Irregular Shaped Buildings; IOS Press BV: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Kim, K.; Son, K.; Kim, E.D.; Kim, S. Current trends and future directions of free-form building technology. Archit. Sci. Rev. 2015, 58, 230–243. [Google Scholar] [CrossRef]
- Eekhout, M.; Gelder, B. Case Study E Management of Complex Free Form Design and Engineering Processes. In Architectural Management: International Research and Practice; Wiley-Blackwell: Singapore, 2009; pp. 244–267. ISBN 9781405177863. [Google Scholar]
- Li, Q. Form Follows Force: A theoretical framework for Structural Morphology, and Form-Finding research on shell structures. A+BE Archit. Built Environ. 2018, 8, 1–278. [Google Scholar] [CrossRef]
- Kim, S.; Son, S.; Lee, D. Development of Sustainable Production Technology of Free-Form Concrete Panels Using a Multi-Point Press CNC Machine. Sustainability 2021, 13, 1990. [Google Scholar] [CrossRef]
- Waimer, F.; Noack, T.; Schmid, A.; Bechmann, R. The Railway Station Stuttgart 21—Computational Design and Digital Fabrication of Freeform Concrete Shells, Proceedings of IASS Annual Symposia, IASS 2019 Barcelona Symposium: Realizations, Case Studies, Barcelona, Spain, 7–9 October 2019; International Association for Shell and Spatial Structures (IASS): Barcelona, Spain, 2019; pp. 1–8. [Google Scholar]
- Poirriez, C.; Franceschi, M.; Bouzida, Y. Parametrization, integration and buildability: Design and construction of a 50M span freeform roof in Bangkok. J. Int. Assoc. Shell Spat. Struct. 2019, 60, 287–293. [Google Scholar] [CrossRef]
- Schleicher, S.; Herrmann, M. Constructing hybrid gridshells using bending-active formwork. Int. J. Sp. Struct. 2020, 35, 80–89. [Google Scholar] [CrossRef]
- San, B.; Feng, D.; Qiu, Y. Shape optimization of concrete free-form shells considering material damage. Eng. Optim. 2021, 53, 1–18. [Google Scholar] [CrossRef]
- Abramczyk, J. Symmetric Free Form Building Structures Arranged Regularly on Smooth Surfaces with Polyhedral Nets. Symmetry 2020, 12, 763. [Google Scholar] [CrossRef]
- Kavuma, A.; Ock, J.; Jang, H. Factors influencing Time and Cost Overruns on Freeform Construction Projects. KSCE J. Civ. Eng. 2019, 23, 1442–1450. [Google Scholar] [CrossRef]
- Wang, X.; Zhu, S.; Zeng, Q.; Guo, X. Improved multi-objective Hybrid Genetic Algorithm for Shape and Size Optimization of Free-form latticed structures. J. Build. Eng. 2021, 43, 102902. [Google Scholar] [CrossRef]
- Wang, Z.; Cao, Z.; Fan, F.; Sun, Y. Shape optimization of free-form grid structures based on the sensitivity hybrid multi-objective evolutionary algorithm. J. Build. Eng. 2021, 44, 102538. [Google Scholar] [CrossRef]
- Tellier, X.; Douthe, C.; Hauswirth, L.; Baverel, O. Fabrication-Aware Design of Architectural Envelopes Using Surfaces with Planar Curvature Lines, Proceedings of IASS Annual Symposia, IASS 2019 Barcelona Symposium: Realizations, Case Studies, Barcelona, Spain, 7–9 October 2019; International Association for Shell and Spatial Structures (IASS): Barcelona, Spain, 2019. [Google Scholar]
- Wakasa, M.; Shimoda, M. Free-form optimization for controlling time-dependent responses of shell structures. Struct. Multidiscip. Optim. 2019, 60, 1545–1560. [Google Scholar] [CrossRef]
- Hameed, A.; Al Alwan, H.; Oukaili, N. Architectural Potentiality of Free-Form Structures, Proceedings of the 3rd European and Mediterranean Structural Engineering and Construction Conference 2020, Limassol, Cyprus, 3–8 August 2020; ISEC Press: Fargo, ND, USA, 2020. [Google Scholar]
- Eekhout, M. Form as a Bridge between Architectural, Structural and Industrial Design. In Proceedings of the 4th International colloqium on Structural Morphology IASS: Spatial Lattice and Tension Structures, Delft, The Netherlands, 17–19 August 2000; pp. 1000–1010. [Google Scholar]
- Wester, T. Nature of Structural Morphology and Some Interdisciplinary Examples, Proceedings of the IASS-ASCE International Symposium 1994 on Spatial, Lattice and Tension Structures, Atlanta, GA, USA, 24–28 April 1994; ASCE: Reston, VA, USA, 1994; pp. 1000–1009. [Google Scholar]
- Li, Q.; Borgart, A.; Wu, Y. How to understand “structural morphology”? J. Int. Assoc. Shell Spat. Struct. 2016, 57, 145–158. [Google Scholar] [CrossRef]
- Abramczyk, J. Transformed Corrugated Shell Units Used as a Material Determining Unconventional Forms of Complex Building Structures. Materials 2021, 14, 2402. [Google Scholar] [CrossRef] [PubMed]
- Pantazis, E.; Gerber, D.J. Beyond geometric complexity: A critical review of complexity theory and how it relates to architecture engineering and construction. Archit. Sci. Rev. 2019, 62, 371–388. [Google Scholar] [CrossRef]
- Gero, J.S. Computational Models of Innovative and Creative Design Processes. Technol. Forecast. Soc. Change 2000, 64, 183–196. [Google Scholar] [CrossRef]
- Williams, C. What is a shell? In Shell Structures for Architecture: Form Finding and Optimization; Routledge: New York, NY, USA, 2014; pp. 21–31. [Google Scholar]
- Linkwitz, K. About formfinding of double-curved structures. Eng. Struct. 1999, 21, 709–718. [Google Scholar] [CrossRef]
- Abramczyk, J. Folded Sheets as a Universal Material for Shaping Transformed Shell Roofs. Materials 2021, 14, 2051. [Google Scholar] [CrossRef] [PubMed]
- Abramczyk, J. Transformed Shell Structures Determined by Regular Networks as a Complex Material for Roofing. Materials 2021, 14, 3582. [Google Scholar] [CrossRef]
- Minchenkov, K.; Vedernikov, A.; Safonov, A.; Akhatov, I. Thermoplastic pultrusion: A review. Polymers 2021, 13, 180. [Google Scholar] [CrossRef]
- Knippers, J.; Cremers, J.; Gabler, M.; Lienhard, J. Construction Manual for Polymers + Membranes; Walter de Gruyter: Berlin, Germany, 2012. [Google Scholar]
- Vedernikov, A.; Nasonov, Y.; Korotkov, R.; Gusev, S.; Akhatov, I.; Safonov, A. Effects of additives on the cure kinetics of vinyl ester pultrusion resins. J. Compos. Mater. 2021, 00219983211001528. [Google Scholar] [CrossRef]
- Engelsmann, S.; Spalding, V.; Peters, S. Plastics in Architecture and Construction; Walter de Gruyter: Berlin, Germany, 2012. [Google Scholar]
- Vedernikov, A.; Tucci, F.; Carlone, P.; Gusev, S.; Konev, S.; Firsov, D.; Akhatov, I.; Safonov, A. Effects of pulling speed on structural performance of L-shaped pultruded profiles. Compos. Struct. 2021, 255, 112967. [Google Scholar] [CrossRef]
- Safonov, A.; Gusev, M.; Saratov, A.; Konstantinov, A.; Sergeichev, I.; Konev, S.; Gusev, S.; Akhatov, I. Modeling of cracking during pultrusion of large-size profiles. Compos. Struct. 2020, 235, 111801. [Google Scholar] [CrossRef]
- Vedernikov, A.; Safonov, A.; Tucci, F.; Carlone, P.; Akhatov, I. Modeling spring-in of l-shaped structural profiles pultruded at different pulling speeds. Polymers 2021, 13, 2748. [Google Scholar] [CrossRef]
- Alajarmeh, O.; Zeng, X.; Aravinthan, T.; Shelley, T.; Alhawamdeh, M.; Mohammed, A.; Nicol, L.; Vedernikov, A.; Safonov, A.; Schubel, P. Compressive behaviour of hollow box pultruded FRP columns with continuous-wound fibres. Thin-Walled Struct. 2021, 168, 108300. [Google Scholar] [CrossRef]
- Vedernikov, A.; Safonov, A.; Tucci, F.; Carlone, P.; Akhatov, I. Pultruded materials and structures: A review. J. Compos. Mater. 2020, 54, 4081–4117. [Google Scholar] [CrossRef]
- Tinkov, D.V.; Safonov, A.A. Design optimization of truss bridge structures of composite materials. J. Mach. Manuf. Reliab. 2017, 46, 46–52. [Google Scholar] [CrossRef]
- Sorina, T.G.; Safonov, A.A.; Khairetdinov, A.K. Pecularities of using carbon glass-reinforced plastic in pultrusion composite profiles for bridge engineering. J. Mach. Manuf. Reliab. 2010, 39, 47–51. [Google Scholar] [CrossRef]
- Bakis, C.E.; Bank, L.C.; Asce, F.; Brown, V.L.; Asce, M.; Cosenza, E.; Davalos, J.F.; Asce, A.M.; Lesko, J.J.; Machida, A.; et al. Fibre Reinforced Polymer Composites for Construction—State-of-the-Art Review. J. Compos. Constr. 2002, 6, 73–87. [Google Scholar] [CrossRef] [Green Version]
- Starr, T.F. Pultrusion for Engineers; Woodhead Publishing: Sawston, UK, 2000; ISBN 9781855734258. [Google Scholar]
- Bank, L.C. Composites for Construction: Structural Design with FRP Materials; John Wiley and Sons: Hoboken, NJ, USA, 2007; ISBN 0471681261. [Google Scholar]
- Raupach, M.; Morales Cruz, C. Textile-Reinforced Concrete: Selected Case Studies. In Textile Fibre Composites in Civil Engineering; Elsevier Inc.: Amsterdam, The Netherlands, 2016; pp. 275–299. ISBN 9781782424697. [Google Scholar]
- Ehlig, D.; Schladitz, F.; Frenzel, M.; Curbach, M. Textilbeton—Ausgeführte Projekte im Überblick. Beton Stahlbetonbau 2012, 107, 777–785. [Google Scholar] [CrossRef]
- Tysmans, T.; Adriaenssens, S.; Wastiels, J. Form finding methodology for force-modelled anticlastic shells in glass fibre textile reinforced cement composites. Eng. Struct. 2011, 33, 2603–2611. [Google Scholar] [CrossRef]
- Solico. Benefits of Composite Materials. Available online: https://www.solico.nl/about-us/benefits-of-composite-materials (accessed on 25 January 2021).
- Bedon, C. Review on the use of FRP composites for façades and building skins. Am. J. Eng. Appl. Sci. 2016, 9, 713–723. [Google Scholar] [CrossRef] [Green Version]
- Gates, P.; Ibell, T.; Darby, A.; Evernden, M.; Kragh, M. Advanced composites for façade retrofit. Struct. Eng. 2012, 90, 46–48. [Google Scholar]
- Bedon, C.; Agullo, C.P.; Luna-Navarro, A.; Overend, M.; Favoino, F. Thermo-Mechanical Investigation of Novel GFRP-Glass Sandwich Facade Components, Proceedings of the Challenging Glass 6: Conference on Architectural and Structural Applications of Glass, CGC 2018—Proceedings, Delft, The Netherlands, 17–18 May 2018; TU Delft Open: Delft, The Netherlands, 2018; pp. 501–512. [Google Scholar]
- Nguyen, K.T.Q.; Navaratnam, S.; Mendis, P.; Zhang, K.; Barnett, J.; Wang, H. Fire safety of composites in prefabricated buildings: From fibre reinforced polymer to textile reinforced concrete. Compos. Part B Eng. 2020, 187, 107815. [Google Scholar] [CrossRef]
- Henriksen, T.; Lo, S.; Knaack, U. The impact of a new mould system as part of a novel manufacturing process for complex geometry thin-walled GFRC. Archit. Eng. Des. Manag. 2016, 12, 231–249. [Google Scholar] [CrossRef] [Green Version]
- Schipper, R.; Eigenraam, P.; Grünewald, S.; Soru, M.; Nap, P.; Overveld, B.V.A.N.; Vermeulen, J. Kine-Mould: Manufacturing Technology for Curved Architectural Elements in Concrete, Proceedings of the IASS Annual Symposia, IASS 2015 Amsterdam Symposium: Future Visions—Symposium on Flexible Formwork, Amsterdam, The Netherlands, 16–20 August; International Association for Shell and Spatial Structures: Amsterdam, The Netherlands, 2015. [Google Scholar]
- Adapa. Adaptive Mould D300—Adaptive Moulds. Available online: https://adapa.dk/portfolio-item/adaptive-mould-d300-2/ (accessed on 23 March 2021).
- Henriksen, T.; Lo, S.; Knaack, U. A new method to advance complex geometry thin-walled glass fibre reinforced concrete elements. J. Build. Eng. 2016, 6, 243–251. [Google Scholar] [CrossRef] [Green Version]
- Krivoshapko, S.N.; Ivanov, V.N. Encyclopedia of Analytical Surfaces; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Henriksson, V.; Hult, M. Rationalizing Freeform Architecture—Surface Discretization and Multi-Objective Optimization. Master’s Thesis, Chalmers University of Technology, Göteborg, Sweden, 2015. [Google Scholar]
- Rogers, D.F. An Introduction to NURBS: With Historical Perspective; Morgan Kaufmann Publishers: Burlington, NJ, USA, 2001. [Google Scholar]
- Farin, G. From Conics to NURBS: A Tutorial and Survey. IEEE Comput. Graph. Appl. 1992, 12, 78–86. [Google Scholar] [CrossRef]
- Caetano, I.; Leitão, A. Architecture meets computation: An overview of the evolution of computational design approaches in architecture. Archit. Sci. Rev. 2020, 63, 165–174. [Google Scholar] [CrossRef]
- Pottmann, H.; Wallner, J. Freeform architecture and discrete differential geometry. In Discrete Geometry for Computer Imagery—DGCI 2017; Lecture Notes in Computer Science (Including Subseries Lecture Notes in Artificial Intelligence and Lecture Notes in Bioinformatics); Springer: Berlin/Heidelberg, Germany, 2017; Volume 10502, pp. 3–8. [Google Scholar]
- Genzel, E. Zur Geschichte der Konstruktion und der Bemessung von Tragwerken des Hochbaus aus faserverstärkten Kunststoffen 1950–1980. Ph.D. Thesis, Bauhaus-Universität Weimar, Weimar, Germany, 2006. [Google Scholar]
- Hollaway, L.C. The evolution of and the way forward for advanced polymer composites in the civil infrastructure. In Proceedings of the Construction and Building Materials; Elsevier: Amsterdam, The Netherlands, 2003; Volume 17, pp. 365–378. [Google Scholar]
- Harries, K.A.; Chen, J.-F.; Presidents, V.; Bakis, C.E.; Kotynia, R.; Wu, Z.-S.; Smith, S.T.; Bisby, L.A.; Dai, J.-G.; El-Hacha, R.; et al. FRP International; International Institute for FRP in Construction: Kingston, ON, Canada, 2011; Volume 8. [Google Scholar]
- Eekhout, M. Composite stressed skin roofs for liquid design architecture. Int. J. Struct. Eng. 2010, 1, 255–279. [Google Scholar] [CrossRef]
- Eekhout, A.J.C.M.; Wichers, S. Lord of the Wings: The Making of Free Form Architecture; IOS Press—Delft University Press: Amsterdam, The Netherlands, 2016. [Google Scholar]
- Zaha Hadid Architects. Mobile Art Chanel Contemporary Art Container. Available online: https://www.zaha-hadid.com/architecture/chanel-art-pavilion/ (accessed on 7 December 2020).
- Pazdur-Czarnowska, A. Ecological Properties of Glass Fibre Reinforced Materials Based on Architecture of Zaha Hadid. In Proceedings of the IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2019; Volume 471. [Google Scholar]
- ArchDaily. Heydar Aliyev Center/Zaha Hadid Architects. Available online: https://www.archdaily.com/448774/heydar-aliyev-center-zaha-hadid-architects?ad_medium=gallery (accessed on 7 December 2020).
- ArchDaily. BIG’s 2016 Serpentine Pavilion Opens Alongside 4 Summerhouses. Available online: https://www.archdaily.com/789018/bigs-2016-serpentine-pavilion-opens-alongside-4-summerhouses (accessed on 7 December 2020).
- Scholzen, A.; Chudoba, R.; Hegger, J. Thin-walled shell structures made of textile-reinforced concrete: Part I: Structural design and construction. Struct. Concr. 2015, 16, 106–114. [Google Scholar] [CrossRef]
- Scholzen, A.; Chudoba, R.; Hegger, J. Thin-walled shell structures made of textile-reinforced concrete: Part II: Experimental characterization, ultimate limit state assessment and numerical simulation. Struct. Concr. 2015, 16, 115–124. [Google Scholar] [CrossRef]
- Hawkins, W.; Orr, J.; Shepherd, P.; Ibell, T. Design, Construction and Testing of a Low Carbon Thin-Shell Concrete Flooring System. Structures 2019, 18, 60–71. [Google Scholar] [CrossRef]
- Sharei, E.; Scholzen, A.; Hegger, J.; Chudoba, R. Structural behavior of a lightweight, textile-reinforced concrete barrel vault shell. Compos. Struct. 2017, 171, 505–514. [Google Scholar] [CrossRef]
- Verwimp, E.; Tysmans, T.; Mollaert, M.; Berg, S. Experimental and numerical buckling analysis of a thin TRC dome. Thin-Walled Struct. 2015, 94, 89–97. [Google Scholar] [CrossRef]
- Voigt, P. Die Pionierphase des Bauens mit Glasfaserverstärkten Kunststoffen (GFK) 1942 bis 1980. Ph.D. Thesis, Bauhaus-Universität Weimar, Weimar, Germany, 2007. [Google Scholar]
- ArchDaily. Chanel Mobile Art Pavilion/Zaha Hadid Architects. Available online: https://www.archdaily.com/144378/chanel-mobile-art-pavilion-zaha-hadid-architects (accessed on 11 March 2021).
- New Christian Dior Flagship Store in Seoul Reflects Dior’s Soft And Flowing Style. Available online: https://worldarchitecture.org/articles/ccmpc/new-christian-dior-flagship-store-in-seoul-reflects-diors-soft-and-flowing-style.html (accessed on 14 July 2021).
- McCulloch, W.S.; Kelly, P.M. Living Prototypes—The Key to New Technology, Proceedings of the Bionics-Symposium, Dayton, OH, USA, 13–15 September 1961; Directorate of Advanced Systems Technology, Wright Air Development Division, Air Research and Development Command, U.S. Air Force: Dayton, OH, USA, 1961.
- Pohl, G.; Nachtigall, W. Biomimetics for Architecture & Design; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Torgal, F.P.; Labrincha, J.A.; Diamanti, M.V.; Yu, C.P.; Lee, H.K. Biotechnologies and Biomimetics for Civil Engineering; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Pohl, G.; Speck, T.; Speck, O.; Pohl, J. The role of textiles in providing biomimetic solutions for construction. In Textiles, Polymers and Composites for Buildings; Elsevier: Amsterdam, The Netherlands, 2010. [Google Scholar]
- Speck, T.; Speck, O. Process sequences in biomimetic research. WIT Trans. Ecol. Environ. 2008, 114, 3–11. [Google Scholar] [CrossRef] [Green Version]
- Knippers, J.; Speck, T. Design and construction principles in nature and architecture. Bioinspir. Biomim. 2012, 7, 015002. [Google Scholar] [CrossRef]
- Speck, T.; Burgert, I. Plant stems: Functional design and mechanics. Annu. Rev. Mater. Res. 2011, 41, 169–193. [Google Scholar] [CrossRef]
- Badarnah, L.; Kadri, U. A methodology for the generation of biomimetic design concepts. Archit. Sci. Rev. 2015, 58, 120–133. [Google Scholar] [CrossRef]
- Milwich, M.; Speck, T.; Speck, O.; Stegmaier, T.; Planck, H. Biomimetics and technical textiles: Solving engineering problems with the help of nature’s wisdom. Am. J. Bot. 2006, 93, 1455–1466. [Google Scholar] [CrossRef] [PubMed]
- Baumeister, D. Biomimicry Resource Handbook: A Seed Bank of Knowledge and Best Practices; Biomimicry 3.8: Missoula, MT, USA, 2013. [Google Scholar]
- Gebeshuber, I.C.; Drack, M. An attempt to reveal synergies between biology and mechanical engineering. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2008, 222, 1281–1287. [Google Scholar] [CrossRef]
- Vattam, S.S.; Helms, M.E.; Goel, A.K. Nature of creative analogies in biologically inspired innovative design. In Proceedings of the 7th Conference on Creativity & Cognition, Berkeley, CA, USA, 26–30 October 2009. [Google Scholar]
- Palombini, F.L.; de Araujo Mariath, J.E.; de Oliveira, B.F. Bionic design of thin-walled structure based on the geometry of the vascular bundles of bamboo. Thin-Walled Struct. 2020, 155, 106936. [Google Scholar] [CrossRef]
- Chen, J.; Ni, Q.Q.; Xu, Y.; Iwamoto, M. Lightweight composite structures in the forewings of beetles. Compos. Struct. 2007, 79, 331–337. [Google Scholar] [CrossRef]
- Libonati, F.; Vergani, L. Understanding the structure-property relationship in cortical bone to design a biomimetic composite. Compos. Struct. 2016, 139, 188–198. [Google Scholar] [CrossRef] [Green Version]
- Hao, R.; Li, D.X.; Liu, W. A novel three-dimensional structure model of biomimetic staggered composites. Compos. Struct. 2020, 239, 112042. [Google Scholar] [CrossRef]
- Arjangpay, A.; Darvizeh, A.; Yarmohammad Tooski, M.; Ansari, R. An experimental and numerical investigation on low velocity impact response of a composite structure inspired by dragonfly wing configuration. Compos. Struct. 2018, 184, 327–336. [Google Scholar] [CrossRef]
- Al-Obaidi, K.M.; Azzam Ismail, M.; Hussein, H.; Abdul Rahman, A.M. Biomimetic building skins: An adaptive approach. Renew. Sustain. Energy Rev. 2017, 79, 1472–1491. [Google Scholar] [CrossRef]
- López, M.; Rubio, R.; Martín, S.; Croxford, B. How plants inspire façades. From plants to architecture: Biomimetic principles for the development of adaptive architectural envelopes. Renew. Sustain. Energy Rev. 2017, 67, 692–703. [Google Scholar] [CrossRef]
- Hamm, C. Evolution of Lightweight Structures: Analyses and Technical Applications; Springer: Berlin/Heidelberg, Germany, 2015; ISBN 9789401793971. [Google Scholar]
- Fischer, J.-R. Optimizing Digital Organic Freeform Modelling for Fabrication by Using Parameterization with Glass Fibre Reinforced Plastics, Proceedings of the 30th International Conference on Education and Research in Computer Aided Architectural Design in Europe, Prague, Czech Republic, 12–14 September 2012; eCAADe: Prague, Czech Republic, 2012; Volume 2, pp. 181–190. [Google Scholar]
- Pohl, G. Fibre Reinforced Building Envelopes Inspired by Nature: Pavilion COCOON_FS. In Evolution of Lightweight Structures; Springer: Dordrecht, The Netherlands, 2015. [Google Scholar]
- Reichert, S.; Schwinn, T.; La Magna, R.; Waimer, F.; Knippers, J.; Menges, A. Fibrous structures: An integrative approach to design computation, simulation and fabrication for lightweight, glass and carbon fibre composite structures in architecture based on biomimetic design principles. CAD Comput. Aided Des. 2014, 52, 27–39. [Google Scholar] [CrossRef]
- Weigele, J.; Schloz, M.; Schwinn, T.; Reichert, S.; La Magna, R.; Waimer, F.; Knippers, J.; Menges, A. Fibrous Morphologies, Proceedings of the Computation and Performance—Proceedings of the 31st eCAADe Conference, Delft, The Netherlands, 18–20 September 2013; Stouffs, R., Sariyildiz, S., Eds.; Faculty of Architecture, Delft University of Technology: Delft, The Netherlands, 2013; pp. 549–558. [Google Scholar]
- Waimer, F.; La Magna, R.; Reichert, S.; Schwinn, T.; Menges, A.; Knippers, J. Integrated design methods for the simulation of fibre-based structures. In Proceedings of the Rethinking Prototyping—Proceedings of the Design Modelling Symposium Berlin 2013; University of the Arts Berlin: Berlin, Germany, 2013; pp. 1–9. [Google Scholar]
- Vasey, L.; Baharlou, E.; Dörstelmann, M.; Koslowski, V.; Prado, M.; Schieber, G.; Menges, A.; Knippers, J. Behavioral Design and Adaptive Robotic Fabrication of a Fiber Composite Compression Shell with Pneumatic Formwork, Proceedings of the ACADIA 2015—Computational Ecologies: Design in the Anthropocene: Proceedings of the 35th Annual Conference of the Association for Computer Aided Design in Architecture, Cincinnati, OH, USA, 19–25 October; ACADIA: Pittsburgh, PA, USA, 2015. [Google Scholar]
- Doerstelmann, M.; Knippers, J.; Koslowski, V.; Menges, A.; Prado, M.; Schieber, G.; Vasey, L. ICD/ITKE Research Pavilion 2014–15: Fibre placement on a pneumatic body based on a water spider web. Archit. Des. 2015, 85, 60–65. [Google Scholar] [CrossRef]
- Solly, J.; Früh, N.; Saffarian, S.; Aldinger, L.; Margariti, G.; Knippers, J. Structural design of a lattice composite cantilever. Structures 2019, 18, 28–40. [Google Scholar] [CrossRef]
- Gil Pérez, M.; Rongen, B.; Koslowski, V.; Knippers, J. Structural design, optimization and detailing of the BUGA fibre pavilion. Int. J. Sp. Struct. 2020, 35, 147–159. [Google Scholar] [CrossRef]
- Dambrosio, N.; Zechmeister, C.; Bodea, S.; Koslowski, V.; Gil-Pérez, M.; Rongen, B.; Knippers, J.; Menges, A. Buga Fibre Pavilion Towards an Architectural Application of Novel Fiber Composite Building Systems, Proceedings of the Ubiquity and Autonomy—Paper Proceedings of the 39th Annual Conference of the Association for Computer Aided Design in Architecture, Austin, TX, USA, 18 September 2019; ACADIA: Pittsburgh, PA, USA, 2019. [Google Scholar]
- Schleicher, S.; Lienhard, J.; Knippers, J.; Poppinga, S.; Masselter, T.; Speck, T. Bio-inspired Kinematics for Adaptive Shading Systems on Free Form Facades. In Taller, Longer, Lighter: Meeting Growing Demand with Limited Resources, Proceedings of IABSE—IASS 2011 London Symposium, London, UK, 20–23 September 2011; Hemming Group: London, UK, 2011. [Google Scholar]
- Schleicher, S.; Lienhard, J.; Poppinga, S.; Masselter, T.; Speck, T. Adaptive façade shading systems inspired by natural elastic kinematics. In Proceedings of the International Adaptive Architecture Conference, Klagenfurt, Austria, 6–8 September 2011; Building Centre: London, UK, 2011. [Google Scholar]
- Lienhard, J.; Schleicher, S.; Poppinga, S.; Masselter, T.; Milwich, M.; Speck, T.; Knippers, J. Flectofin: A hingeless flapping mechanism inspired by nature. Bioinspir. Biomim. 2011, 6, 045001. [Google Scholar] [CrossRef]
- Poppinga, S.; Lienhard, J.; Masselter, T.; Schleicher, S.; Knippers, J.; Speck, T. Biomimetic Deployable Systems in Architecture, Proceedings of the 6th World Congress on Biomechanics, Singapore, 1–6 August 2010; Springer: Cham, Switzerland, 2010; Volume 31. [Google Scholar]
- Poppinga, S.; Masselter, T.; Lienhard, J.; Schleicher, S.; Knippers, J.; Speck, T. Plant movements as concept generators for deployable systems in architecture. WIT Trans. Ecol. Environ. 2010, 138, 403–409. [Google Scholar] [CrossRef] [Green Version]
- Institute for Computational Design and Construction, University of Stuttgart. ICD/ITKE Research Pavilion 2012. Available online: https://www.icd.uni-stuttgart.de/projects/icditke-research-pavilion-2012/ (accessed on 20 May 2021).
- ICD and ITKE’s New Lightweight Pavilion Mimics the Structure of Water Spider’s Underwater Nests. Available online: https://inhabitat.com/icd-and-itkes-lightweight-pavilion-mimics-the-structure-of-water-spiders-underwater-nests/ (accessed on 7 December 2020).
- Poppinga, S.; Körner, A.; Sachse, R.; Born, L.; Westermeier, A.; Hesse, L.; Knippers, J.; Bischoff, M.; Gresser, G.T.; Speck, T. Compliant Mechanisms in Plants and Architecture. In Biomimetic Research for Architecture and Building Construction; Springer: Cham, Switzerland, 2016. [Google Scholar]
- ArchDaily. One Ocean, Thematic Pavilion EXPO 2012/Soma. Available online: https://www.archdaily.com/236979/one-ocean-thematic-pavilion-expo-2012-soma?ad_medium=gallery (accessed on 7 December 2020).
- Pottmann, H.; Eigensatz, M.; Vaxman, A.; Wallner, J. Architectural geometry. Comput. Graph. 2015, 47, 145–164. [Google Scholar] [CrossRef] [Green Version]
- Bletzinger, K.U.; Ramm, E. Computational form finding and optimization. In Shell Structures for Architecture: Form Finding and Optimization; Routledge: New York, NY, USA, 2014; pp. 45–55. [Google Scholar]
- Hernández-Montes, E.; Fernández-Ruiz, M.A.; Aschheim, M.; Gil-Martín, L.M. Structural Knowledge within the 6th Century AD Arch of Taq-iKisra. Int. J. Archit. Herit. 2017, 11, 891–900. [Google Scholar] [CrossRef]
- Cleary, R. Review: Seven Structural Engineers: The Felix Candela Lectures by Guy Nordenson, editor. J. Soc. Archit. Hist. 2011, 70, 393–394. [Google Scholar] [CrossRef]
- Isler, H. Concrete Shells Derived from Experimental Shapes. Struct. Eng. Int. 1994, 4, 142–147. [Google Scholar] [CrossRef]
- Linkwitz, K. New methods for the determination of cutting pattern of prestressed cable nets and their application to the Olympic Roofs Munich. In Tension Structures and Space Frames, Proceedings of the IASS Pacific Symposium, Tokyo, Japan, 17–23 October 1971; Architectural Institute of Japan: Tokyo, Japan, 1971. [Google Scholar]
- Linkwitz, K.; Schek, H.J. Einige Bemerkungen zur Berechnung von vorgespannten Seilnetzkonstruktionen. Ingenieur-Archiv 1971, 40, 145–158. [Google Scholar] [CrossRef]
- Schek, H.J. The force density method for form finding and computation of general networks. Comput. Methods Appl. Mech. Eng. 1974, 3, 115–134. [Google Scholar] [CrossRef]
- Haber, R.B.; Abel, J.F. Initial equilibrium solution methods for cable reinforced membranes part I—Formulations. Comput. Methods Appl. Mech. Eng. 1982, 30, 263–284. [Google Scholar] [CrossRef]
- Block, P.; Ochsendorf, J. Thrust network analysis: A new methodology for three-dimensional equilibrium. J. Int. Assoc. Shell Spat. Struct. 2007, 48, 167–173. [Google Scholar]
- Block, P. Thrust Network Analysis: Exploring Three-Dimensional Equilibrium. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2009. [Google Scholar]
- Hernández-Montes, E.; Jurado-Piña, R.; Bayo, E. Topological Mapping for Tension Structures. J. Struct. Eng. 2006, 132, 970–977. [Google Scholar] [CrossRef] [Green Version]
- Jurado-Piña, R.; Gil-Martín, L.M.; Hernández-Montes, E. Topological mesh for shell structures. Appl. Math. Model. 2009, 33, 948–958. [Google Scholar] [CrossRef]
- Carbonell-Márquez, J.F.; Gil-Martín, L.M.; Fernández-Ruíz, M.A.; Hernández-Montes, E. Topological design of compression structures. Arch. Appl. Mech. 2016, 86, 1495–1508. [Google Scholar] [CrossRef]
- Fernández-Ruiz, M.A.; Moskaleva, A.; Gil-Martín, L.M.; Palomares, A.; Hernández-Montes, E. Design and form-finding of compression structures with prestressing tendons. Eng. Struct. 2019, 197, 109394. [Google Scholar] [CrossRef]
- Barnes, M.R. Form-finding and analysis of prestressed nets and membranes. Comput. Struct. 1988, 30, 685–695. [Google Scholar] [CrossRef]
- Barnes, M.R. Form finding and analysis of tension structures by dynamic relaxation. Int. J. Sp. Struct. 1999, 14, 89–104. [Google Scholar] [CrossRef]
- Kilian, A.; Ochsendorf, J. Particle-spring systems for structural form finding. J. Int. Assoc. Shell Spat. Struct. 2005, 46, 77–84. [Google Scholar]
- Veenendaal, D.; Block, P. An overview and comparison of structural form finding methods for general networks. Int. J. Solids Struct. 2012, 49, 3741–3753. [Google Scholar] [CrossRef] [Green Version]
- López López, D.; Domènech Rodríguez, M.; Palumbo Fernández, M. “Brick-topia”, the thin-tile vaulted pavilion. Case Stud. Struct. Eng. 2014, 2, 33–40. [Google Scholar] [CrossRef] [Green Version]
- Douthe, C.; Baverel, O.; Caron, J.F. Form-finding of a grid shell in composite materials. J. Int. Assoc. Shell Spat. Struct. 2006, 47, 53–62. [Google Scholar]
- Douthe, C.; Caron, J.F.; Baverel, O. Gridshell structures in glass fibre reinforced polymers. Constr. Build. Mater. 2010, 24, 1580–1589. [Google Scholar] [CrossRef]
- Douthe, C. Étude de Structures Élancées Précontraintes en Matériaux Composites: Application à la Conception des Gridshells. Ph.D. Thesis, Ecole Nationale des Ponts et Chaussées, Champs-sur-Marne, France, 2007. [Google Scholar]
- Tayeb, F.; Lefevre, B.; Baverel, O.; Caron, J.F.; Du Peloux, L. Design and realisation of composite gridshell structures. J. Int. Assoc. Shell Spat. Struct. 2015, 56, 49–59. [Google Scholar]
- Tayeb, F.; Caron, J.F.; Baverel, O.; Du Peloux, L. Stability and robustness of a 300 m2 composite gridshell structure. Constr. Build. Mater. 2013, 49, 926–938. [Google Scholar] [CrossRef] [Green Version]
- Lefevre, B.; Douthe, C.; Baverel, O. Buckling of elastic gridshells. J. Int. Assoc. Shell Spat. Struct. 2015, 56, 153–171. [Google Scholar]
- Cuvilliers, P.; Douthe, C.; Du Peloux, L.; Le Roy, R. Hybrid structural skin: Prototype of a gfrp elastic gridshell braced by a fiber-reinforced concrete envelope. J. Int. Assoc. Shell Spat. Struct. 2017, 58, 65–78. [Google Scholar] [CrossRef]
- Wenzel, F.; Meissner, I.; Otto, F.; Möller, E. Frei Otto: Forschen, Bauen, Inspirieren/a Life of Research, Construction and Inspiration; DETAIL—Institut für Internationale Architektur Dokumentation: Munich, Germany, 2019. [Google Scholar]
- Schling, E.; Barthel, R.; Ihde, A.; Tutsch, J.; Huth, S. Bending-activated tensegrity. In Proceedings of the International Association for Shell and Spatial Structures Symposium, Amsterdam, The Netherlands, 17–20 August 2015; IASS: Madrid, Spain, 2015. [Google Scholar]
- Du Peloux, L.; Tayeb, F.; Baverel, O.; Caron, J.F. Construction of a large composite gridshell structure: A lightweight structure made with pultruded glass fibre reinforced polymer tubes. Struct. Eng. Int. 2016, 26, 160–167. [Google Scholar] [CrossRef] [Green Version]
- Méndez Echenagucia, T.; Pigram, D.; Liew, A.; Van Mele, T.; Block, P. A Cable-Net and Fabric Formwork System for the Construction of Concrete Shells: Design, Fabrication and Construction of a Full Scale Prototype. Structures 2019, 18, 72–82. [Google Scholar] [CrossRef] [Green Version]
- Du Peloux, L. Modeling of Bending-Torsion Couplings in Active-Bending Structures: Application to the Design of Elastic Gridshells. Ph.D. Thesis, Ecole Nationale des Ponts et Chaussées, Champs-sur-Marne, France, 2017. [Google Scholar]
- Schling, E.; Barthel, R.; Tutsch, J. Freie form—Experimentelle tragstruktur—entwurf und umsetzung von modularen flächentragwerken. Bautechnik 2014, 91, 859–868. [Google Scholar] [CrossRef] [Green Version]
- Block, P.; Schlueter, A.; Veenendaal, D.; Bakker, J.; Begle, M.; Hischier, I.; Hofer, J.; Jayathissa, P.; Maxwell, I.; Echenagucia, T.M.; et al. NEST HiLo: Investigating lightweight construction and adaptive energy systems. J. Build. Eng. 2017, 12, 332–341. [Google Scholar] [CrossRef]
- (PDF) Filigree Shell Slabs Material and Fabrication-Aware Shape Optimisation for CFRP Coreless-Wound Slab Components. Available online: https://www.researchgate.net/publication/351225612_Filigree_Shell_Slabs_Material_and_Fabrication-aware_Shape_Optimisation_for_CFRP_Coreless-_wound_Slab_Components (accessed on 3 June 2021).
- ArchDaily. Restoration of Buckminster Fuller’s Iconic Fly’s Eye Dome at America’s Cup. Available online: https://www.archdaily.com/139238/restoration-of-buckminster-fuller%25e2%2580%2599s-iconic-fly%25e2%2580%2599s-eye-dome-at-america%25e2%2580%2599s-cup (accessed on 7 December 2020).
- ArchDaily. Lincoln Park Zoo South Pond/Studio Gang Architects. Available online: https://www.archdaily.com/83676/lincoln-park-zoo-south-pond-studio-gang-architects (accessed on 11 May 2021).
- Ljusglober. Available online: https://www.octatube.nl/en_GB/project-item/projectitem/37-ljusglober.html (accessed on 11 May 2021).
- Archello. Fiberline Factory—Lay Light by Fiberline. Available online: https://archello.com/project/fiberline-factory (accessed on 11 May 2021).
- Engelsmann, S.; Spalding, V. Eine Geometrisch Komplexe Plastik-skulptur mit Modularem Tragwerk. Bautechnik 2008, 85, 345–348. [Google Scholar] [CrossRef]
- ArchDaily. Stedelijk Museum Amsterdam/Benthem Crouwel Architects. Available online: https://www.archdaily.com/350843/stedelijk-museum-amsterdam-benthem-crouwel-architects (accessed on 11 May 2021).
- ArchDaily. Fletcher Hotel/Benthem Crouwel Architects. Available online: https://www.archdaily.com/350690/fletcher-hotel-benthem-crouwel-architects (accessed on 11 May 2021).
- Atelier PRO. Part 1. Enexis Regional Offices: In Search of an Intelligent Facade. Available online: https://www.atelierpro.nl/en/blog/58/40 (accessed on 26 January 2021).
- Atelier PRO. Part 2. Enexis Regional Offices: In Search of an Intelligent Facade. Available online: https://www.atelierpro.nl/en/blog/59/41 (accessed on 20 May 2021).
- ArchDaily. House of Dior Seoul/Christian de Portzamparc. Available online: https://www.archdaily.com/775902/house-of-dior-seoul-christian-de-portzamparc (accessed on 7 December 2020).
- ArchDaily. BBVA Headquarters/Herzog & de Meuron. Available online: https://www.archdaily.com/893996/bbva-headquarters-herzog-and-de-meuron (accessed on 7 December 2020).
- Jiang, C.; Wang, C.; Schling, E.; Pottmann, H.; Jiang, C.; Wang, C.; Schling, E.; Pottmann, H. Computational design and optimization of quad meshes based on diagonal meshes. In Proceedings of Advances in Architectural Geometry; Ecole des Ponts Paristech: Paris, France, 2020; pp. 38–61. [Google Scholar]
- Schikore, J.; Schling, E.; Oberbichler, T.; Bauer, A.M. Kinetics and Design of Semi-Compliant Grid Mechanisms. Adv. Archit. Geom. 2021, 108–129. [Google Scholar]
- Beites, S. Morphological behavior of shape memory polymers toward a deployable, adaptive architecture. In ACADIA 2013: Adaptive Architecture, Proceedings of the 33rd Annual Conference of the Association for Computer Aided Design in Architecture, Cambridge, UK, 24–26 October 2013; ACADIA: Pittsburgh, PA, USA, 2013. [Google Scholar]
- Yoon, J. Design-to-fabrication with thermo-responsive shape memory polymer applications for building skins. Archit. Sci. Rev. 2021, 64, 72–86. [Google Scholar] [CrossRef]
- Korotkov, R.; Vedernikov, A.; Gusev, S.; Alajarmeh, O.; Akhatov, I.; Safonov, A. Shape memory behavior of unidirectional pultruded laminate. Compos. Part A Appl. Sci. Manuf. 2021, 150, 106609. [Google Scholar] [CrossRef]
- Naaman, A.E. Thin TRC Products: Status, Outlook, and Future Directions. In Textile Fibre Composites in Civil Engineering; Woodhead Publishing: Duxford, UK, 2016. [Google Scholar]
- Früh, N.; Knippers, J. Multi-stage filament winding: Integrative design and fabrication method for fibre-reinforced composite components of complex geometries. Compos. Struct. 2021, 268, 113969. [Google Scholar] [CrossRef]
- Safonov, A.A. 3D topology optimization of continuous fiber-reinforced structures via natural evolution method. Compos. Struct. 2019, 215, 289–297. [Google Scholar] [CrossRef]
- Kendall, D. The business case for composites in construction. Reinf. Plast. 2008, 52, 20–27. [Google Scholar] [CrossRef]
- Yang, Y.; Boom, R.; Irion, B.; van Heerden, D.J.; Kuiper, P.; de Wit, H. Recycling of composite materials. Chem. Eng. Process. Process Intensif. 2012, 51, 53–68. [Google Scholar] [CrossRef]


| Mold Type | Labor Intensity | Material Cost and Labor Costs * | Mold Production Time | Reusability ** | Comments |
|---|---|---|---|---|---|
| Wooden molds (limited to single-curved and large radii (r > 0.5 m) double curvature) | medium | Material 25 €/m2; Labor 40 €/h | 2–4 h/m2 | 1–20 times | Sometimes a structural calc. for a timber mold is also required (concrete-pressure and weight). This adds additional cost. |
| Steel molds | High | Material approx. 50 €/m2 | 5–8 h/m2 | 20–500 times | Steel molds are used when it is required to have a seamless appearance, largest size 400 × 600 cm. |
| Rubber molds | High | Material 80–200 €/m2 | 3–5 h | 10–50 times | Must be applied to a timber mold. Limited sizes. |
| Polystyrene foam molds, wire cut | Low | Material will be calc. in m³ foam; approx. 30 €/m³ | 1 h | 5–30 times | Standard Polystyrene foam-block is 120 × 120 × 500 cm. Significant waste. |
| 3D computer numerical controlled (CNC) milled molds (foam, plastic) | Low | 300–400 €/m2 | 5–10 h | 5–10 times | The molds are typically made from foam or plastic. Timber or metal alternatives can also be used. The quality of the mold depends on the quality of the foam or plastic. Limited sizes. |
| Flexible tables with pistons | Low | High machine cost | 20 min | Motors 10,000 times Surface 100–500 times | Limited sizes, approx. 1 m × 2 m. |
| Flexible tables with actuators and membranes | Low | High machine cost | 5 min | Motors 10,000 times Surface 500 times | Limited sizes, currently approx. 1.2 m × 1.2 m. |
| Name of the Structure | Year | Location | Architect | Type of Structure, Manufacturing and Construction Method | Function in the Building | Type of Design Approach | Source |
|---|---|---|---|---|---|---|---|
| Petrol station | 1962 | Thun, Switzerland | Heinz Isler | GFRP coffers (boxes) | Roof structure | Geometric approach | [28] |
| Fly’s Eye Dome | 1965 | Three prototypes in different parts of the world; Miami, USA (present) | Richard Buckminster Fuller | GFRP pre-cast module elements | Supporting structure + envelope | Biomimetic approach | [150] |
| Market Hall | 1967 | Argenteuil, France | Stephane DuChateau | 30 pre-fabricated 6 mm thick GFRP shell elements mounted on a supporting tubular steel construction | Roof covering | Geometric approach | [30] |
| The yard of the Realschule | 1967 | Geisslingen, Baden Wuerttemberg, Germany | Heinz Isler | GFRP coffers | Roof structure | Geometric approach | [61] |
| Futuro | 1968 | Different locations worldwide | Matti Suuronen | Modulus CFRP sandwich elements, which form the envelope structure | Supporting structure + envelope | Geometric approach | [30] |
| Clip-On | 1997 | Utrecht, Netherlands | Atelier van Lieshout with Klaar van der Lippe | Continuous sandwich envelope, composed of rigid PUR panels with GFRP facing layers on both sides | Supporting structure + envelope | Geometric approach | [30] |
| Hoofddorp bus station | 2003 | Hoofddorp, Netherlands | NIO architecten | Solid PS foam structure covered by GFRP skin by spraying method | Supporting structure + envelope | Geometric approach | [30] |
| Yitzhak Rabin Center | 2005 | Tel Aviv, Israel | Moshe Safdie Architects | Double-curved shell roof structures, made as sandwich structures with PUR core and GFRP facing layers | Roof structure | Geometric approach | [62,63] |
| Lincoln Park Zoo South Pond | 2005 | Chicago, USA | Studio Gang Architects | Fiberglass pod-shape 3D panels | Envelope | Geometric approach | [151] |
| Ljusglober | 2005 | Sweden, Östersund | Monika Gora | GFRP double-curved slices | Supporting structure + envelope | Biomimetic approach | [152] |
| Fiberline composites factory and offices | 2006 | Middelfart, Denmark | KHR Arkitekter | GFRP pultruded profiles and plates for façade and window structure | Envelope | Geometric approach | [153] |
| Badajoz Congress Centre and Auditorium | 2006 | Badajoz, Spain | SelgasCano | Pultruded GFRP elliptical profiles, which form a fence-like woven structure | Envelope | Geometric approach | [30] |
| Plastic Tower Sculpture | 2007 | Stuttgart, Germany | Research group of Stuttgart State Academy of Art and Design | Double-curved GFRP module elements, made with hand lay-up process, connected together with bolts | Supporting structure | Geometric approach | [30,154] |
| Novartis campus reception building | 2007 | Basel, Switzerland | Marco Serra | Seamless sandwich slab structure with GFRP facing layers and a rigid PUR foam core | Roof structure | Geometric approach | [30] |
| Chanel Mobile Art Pavilion | 2010 | Paris, France | Zaha Hadid Architects | GFRP double-curved thermoformed panels | Envelope | Geometric approach | [64] |
| Pavilion COCOON_FS | 2011 | Jena + international, Germany | Pohl Architects | GFRP modulus elements | Supporting structure + envelope | Biomimetic approach | [96] |
| One Ocean, Thematic Pavilion EXPO 2012 | 2012 | Yeosu-si, South Korea | SOMA Lima | Curved GFRP laminas | Façade shading structure | Biomimetic approach | [114] |
| Stedelijk Museum Amsterdam | 2012 | Amsterdam, Netherlands | Benthem Crouwel Architects | FRP seamless envelope structure with aramid and carbon fibers | Envelope | Geometric approach | [155] |
| Heydar Aliyev Center | 2013 | Baku, Azerbaijan | Zaha Hadid Architects | GFRC and GFRP double-curved façade panels | Envelope | Geometric approach | [66] |
| Fletcher Hotel | 2013 | Amsterdam, Netherlands | Benthem Crouwel Architects | Composite curved façade elements 7 × 3.5 m, thickness 150 mm, finishing on the outside with vinyl with built-in windows | Envelope | Geometric approach | [156] |
| Enexis office buildings | 2013 | Maastricht, Netherlands | Atelier PRO | Façade composite elements 16 × 2.8 m, with a thickness of 200 mm | Envelope | Geometric approach | [157,158] |
| House of Dior Seoul | 2015 | Seoul, South Korea | Christian de Portzamparc | Sculptural free-shape façade of GFRP façade panels | Envelope | Geometric approach | [159] |
| ICD/ITKE Research Pavilion 2014/2015 | 2015 | Stuttgart, Germany | ICD/ITKE research group | Shell structure made by using coreless filament winding method with pneumatic formwork | Supporting structure + envelope | Biomimetic approach | [112] |
| BBVA Headquarters | 2015 | Madrid, Spain | Herzog & de Meuron | FRP solar protection fins | Façade shading structure | Geometric approach | [160] |
| BIG’s Serpentine Gallery Pavilion | 2016 | London, Great Britain (in 2016)Vancouver, Canada (present) | BIG | GRFP pultruded boxes | Supporting structure | Geometric approach | [67] |
| ICD/ITKE Research Pavilion 2016/2017 | 2017 | Stuttgart, Germany | ICD/ITKE research group | Cantilever shell structure made by using a multimachine fabrication system using coreless filament winding method | Supporting structure + envelope | Biomimetic approach | [103] |
| BUGA Fibre Pavilion | 2019 | Heilbronn, Germany | ICD/ITKE research group | Coreless filament winding | Supporting structure | Biomimetic approach | [104,105] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moskaleva, A.; Safonov, A.; Hernández-Montes, E. Fiber-Reinforced Polymers in Freeform Structures: A Review. Buildings 2021, 11, 481. https://doi.org/10.3390/buildings11100481
Moskaleva A, Safonov A, Hernández-Montes E. Fiber-Reinforced Polymers in Freeform Structures: A Review. Buildings. 2021; 11(10):481. https://doi.org/10.3390/buildings11100481
Chicago/Turabian StyleMoskaleva, Anastasiia, Alexander Safonov, and Enrique Hernández-Montes. 2021. "Fiber-Reinforced Polymers in Freeform Structures: A Review" Buildings 11, no. 10: 481. https://doi.org/10.3390/buildings11100481
APA StyleMoskaleva, A., Safonov, A., & Hernández-Montes, E. (2021). Fiber-Reinforced Polymers in Freeform Structures: A Review. Buildings, 11(10), 481. https://doi.org/10.3390/buildings11100481