Effect of Shape, Number, and Location of Openings on Punching Shear Capacity of Flat Slabs
Abstract
:1. Introduction
2. Experimental Program
2.1. Test Matrix
2.2. Specimen Design
- = concrete cylinder compressive strength (MPa)
- = effective slab thickness for shear (mm)
- = perimeter of shear critical section 0.5d from loading area periphery (mm)
- = 40 for interior columns, 30 for edge columns, and 20 for corner columns.
- = The ratio of long to short sides of the columns, concentrated load, or reaction area.
- = 1

- is in MPa
- k = 2.0 (d in mm)
- = 0.02
- related to the bonded tension steel in y- and z-direction respectively.
- is 0.18.
- = Flexural capacity
- L = Length of supported slab
- c = Loading plate side length
- = Flexural reinforcement ratio
2.3. Material Properties
2.4. Load Setup
3. Experimental Results
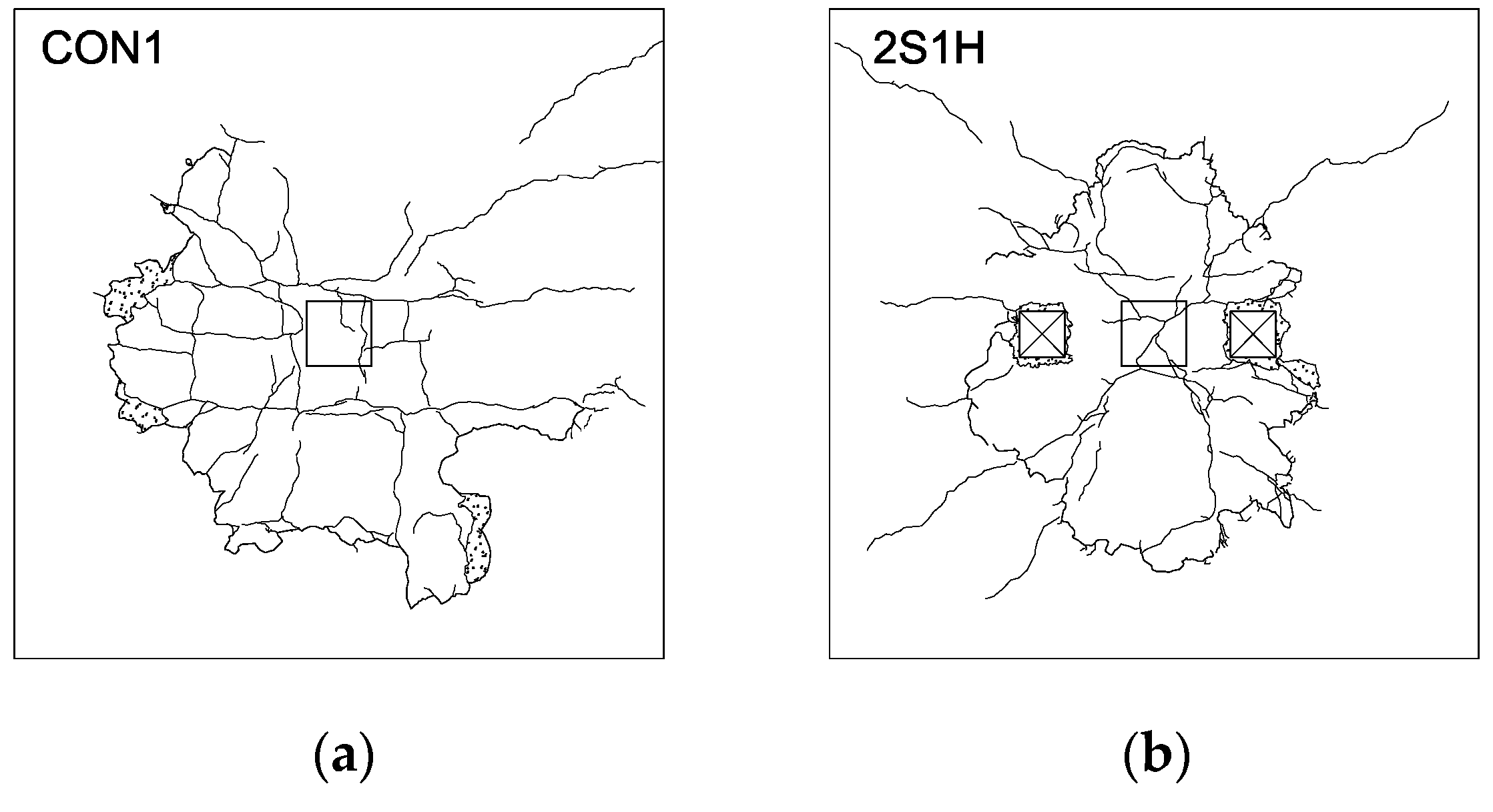
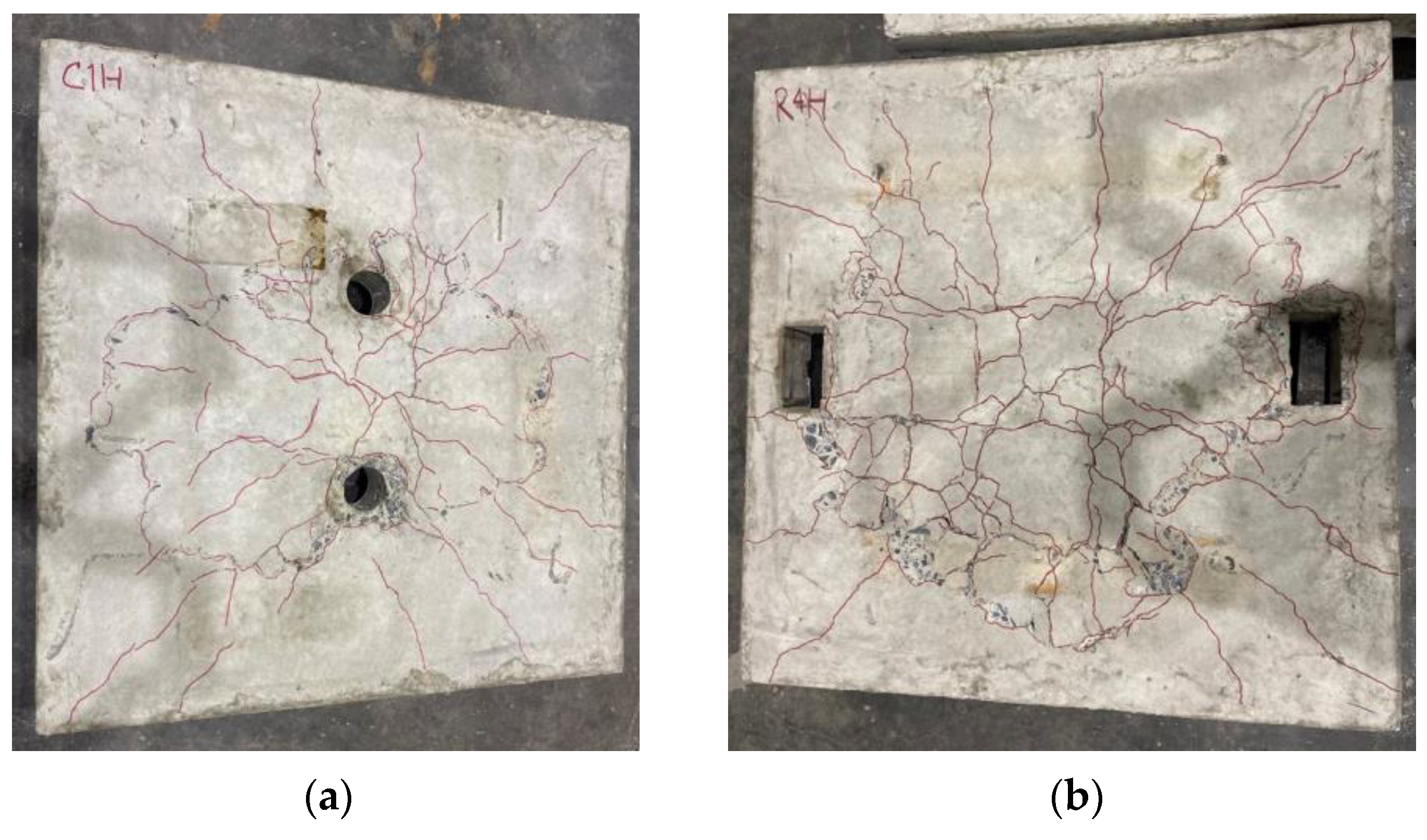
3.1. Failure Modes and Observations
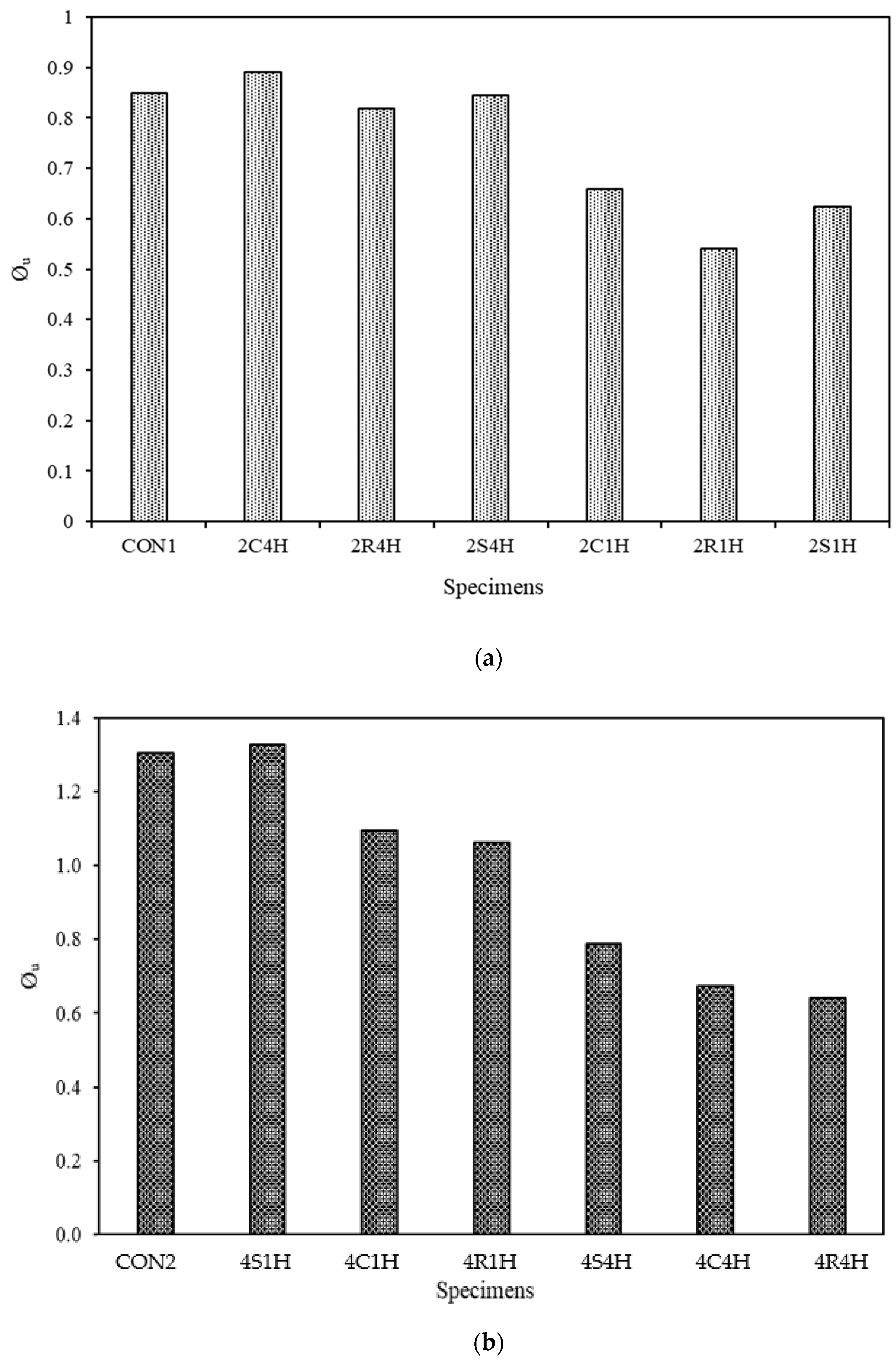
3.2. Ultimate Rotation
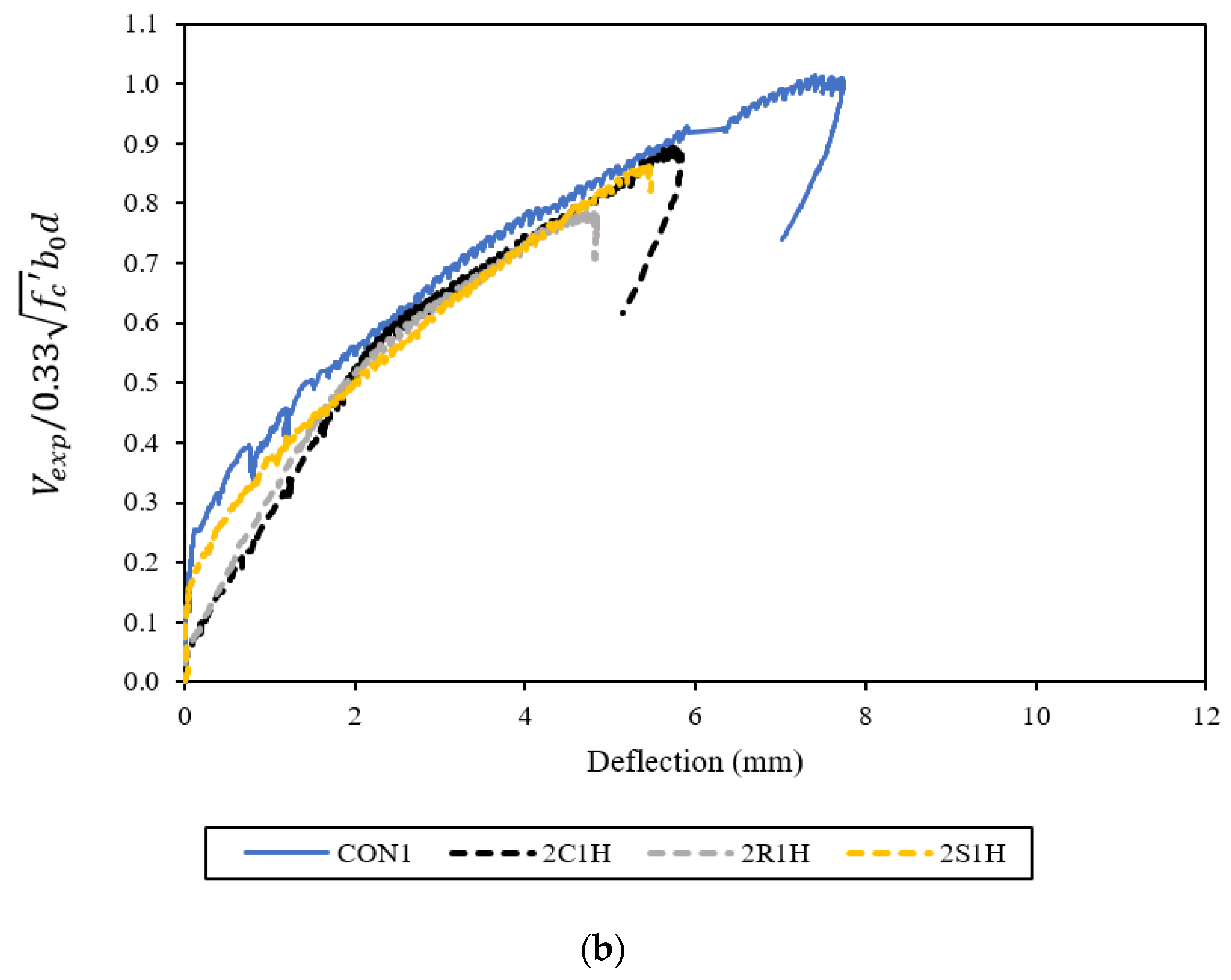
3.3. Load vs. Deflection
3.3.1. Location of Openings
3.3.2. Shape of Openings
3.3.3. Number of Openings
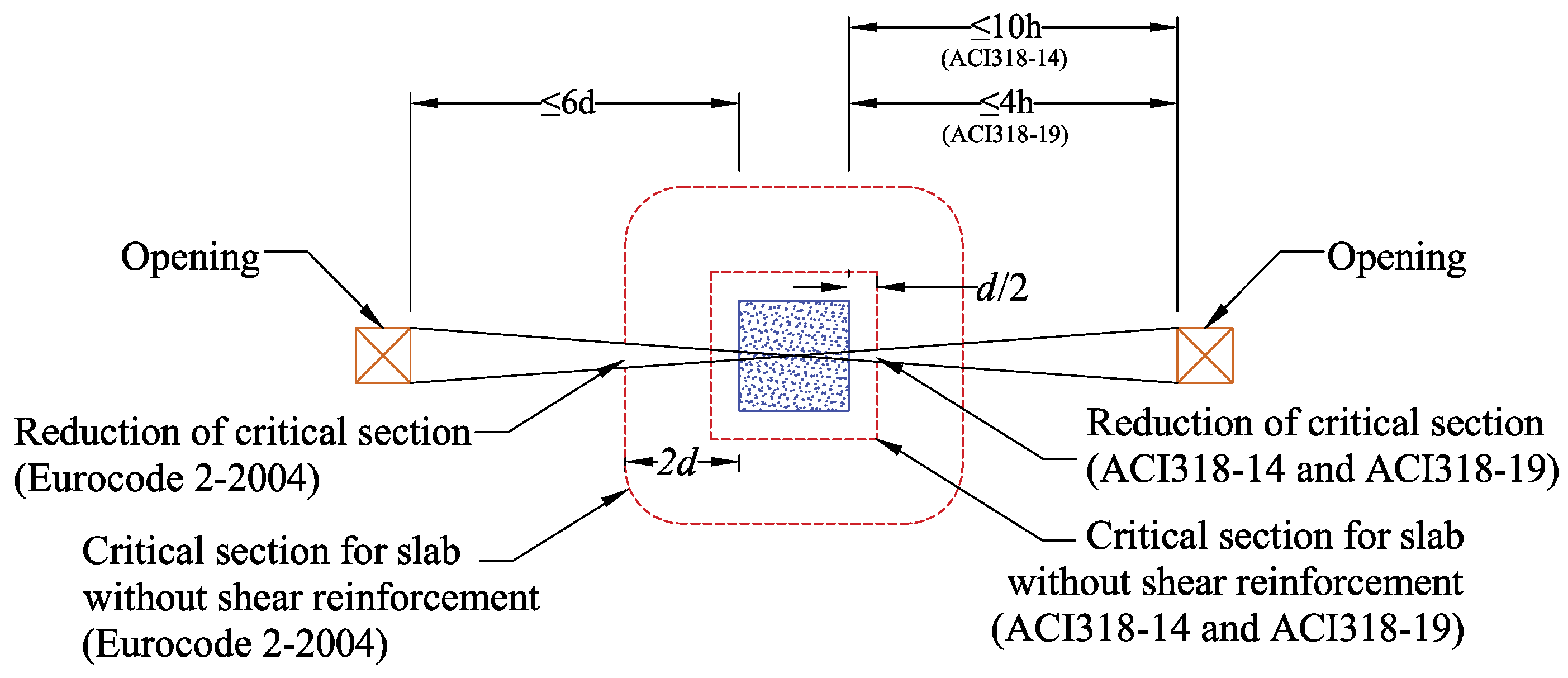
4. Comparison between Experimental Results & Analytical Predictions
5. Discussion
6. Conclusions
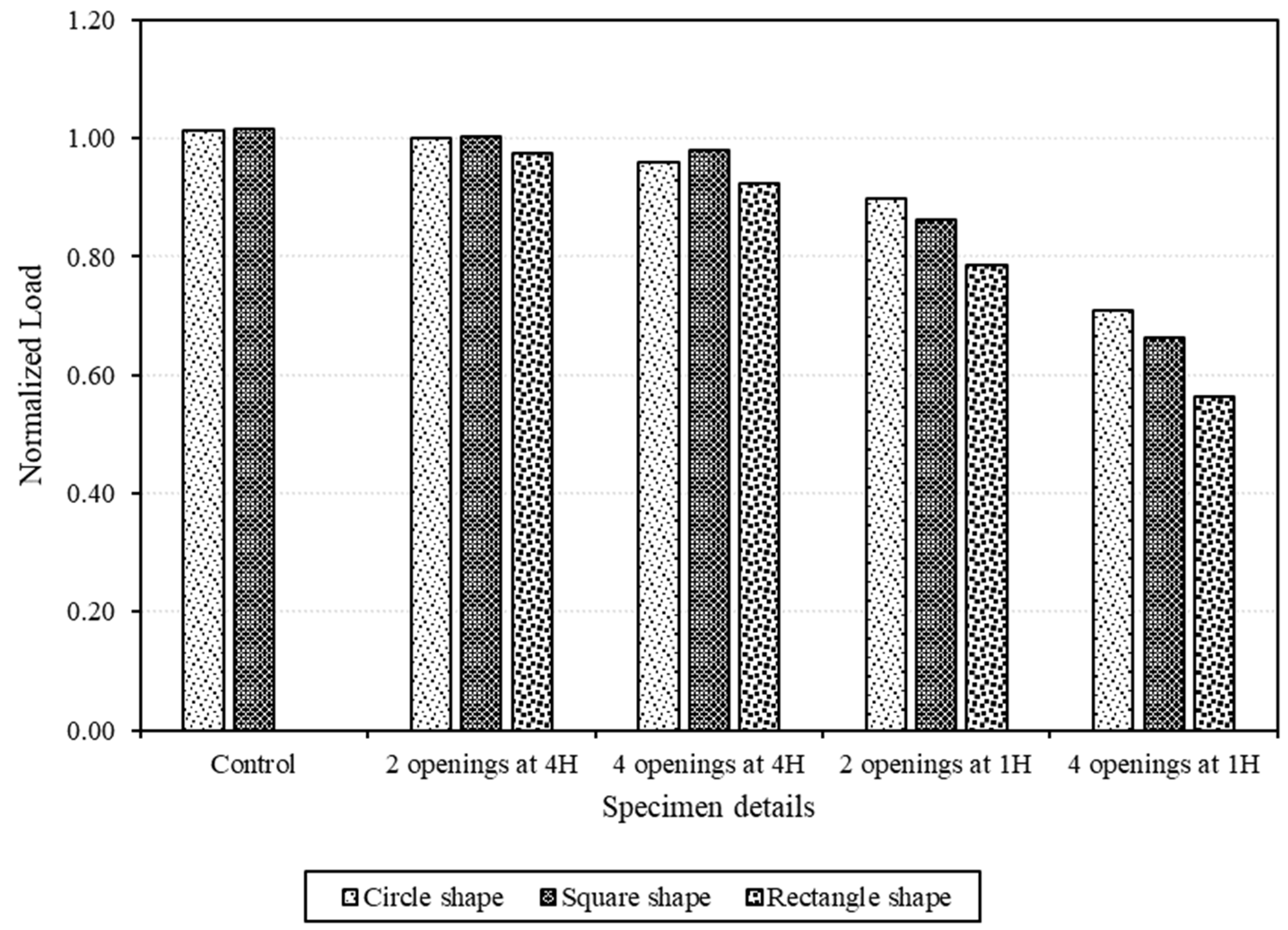
- In terms of the shape of openings, punching capacity of flat slabs were least affected by circular openings followed by square and rectangular openings, respectively.
- Openings provided at 1H from column’s face drastically reduced the punching shear capacity of flat slabs. On the contrary, openings provided at 4H from column’s face had little impact on slab’s shear capacity. Rectangular openings had the worst effect on punching capacity for all opening shapes at 4H from column’s face. Rectangular openings at 4H reduced punching capacity by 3.86 and 8.98% for 2 and 4 openings, respectively. Reduction caused by circular and square and circular openings was comparable at 4H from column’s face and irrespective of their number.
- Irrespective of the shape of the openings, increasing the number of openings from 2 to 4 substantially reduced the punching capacity of slabs.
- Ultimate rotation of slabs with openings at 1H from column’s face were significantly lower than their counterpart slabs with openings at 4H from column’s face.
- Punching shear capacities were analytically predicted following the descriptive equations of ACI 318-19 and Eurocode 2. Ratio of experimental to analytical predictions of ACI 318-19 and Eurocode 2 were 1.04 and 0.93 suggesting that ACI 318-19 recommendations are slightly closer to the real case scenarios. This reason can be attributed to the different locations of critical punching shear perimeter from column’s face as stated by the two codes.
- ACI 318-19 consistently predicted conservative and more closer punching capacities than those predicted by Eurocode 2. For openings located at same distance and of same number, the variation of code predictions from experimental results was marginal for square and circular openings. For instance, ACI 318-19 predicted punching strengths for specimens 2S4H and 2C4H to be −5 and −4% lower than their respective experimental values. However, this was not true for rectangular openings as this error increased to −8% for the specimen 2R4H. Similarly, the predictions of ACI 318-19 for 2S1H and 2C1H resulted in a variation of about −0.4% from their experimental results. Corresponding prediction for the rectangular openings (i.e., for specimen 2R1H) resulted in a variation of −7.5%. A similar trend in the predictions of Eurocode 2 was also observed. Therefore, further research is desired in area to account for the shape of openings in determining their deteriorating effect on punching capacities of flat slabs.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Silva, J.A.; Marques, M.G.; Trautwein, L.M.; Gomes, R.B.; Guimarães, G.N. Punching of Reinforced Concrete Flat Slabs with Holes and Shear Reinforcement. REM Int. Eng. J. 2017, 70, 407–413. [Google Scholar] [CrossRef] [Green Version]
- Santos, G.S.; Nicácio, W.G.; Lima, A.W.; Melo, G.S.S.A. Punching Strengthening in Flat Plates of Reinforced Concrete with Carbon Fiber Reinforced Polymer (CFRP). Rev. Ibracon Estrut. Mater. 2014, 7, 592–625. [Google Scholar] [CrossRef] [Green Version]
- Son, K.-S.; Pilakoutas, K.; Neocleous, K. Behaviour of Concrete Columns with Drilled Holes. Mag. Concr. Res. 2015, 58, 411–419. [Google Scholar] [CrossRef] [Green Version]
- King, S.; Delatte, N.J. Collapse of 2000 Commonwealth Avenue: Punching Shear Case Study. J. Perform. Constr. Facil. 2004, 18, 54–61. [Google Scholar] [CrossRef] [Green Version]
- Gardner, N.J.; Huh, J.; Chung, L. Lessons from the Sampoong Department Store Collapse. Cem. Concr. Compos. 2002, 24, 523–529. [Google Scholar] [CrossRef]
- El-Salakawy, E.F.; Polak, M.A.; Soliman, M.H. Reinforced Concrete Slab-Column Edge Connections with Shear Studs. Can. J. Civ. Eng. 2011, 27, 338–348. [Google Scholar] [CrossRef]
- Teng, S.; Cheong, H.K.; Kuang, K.L.; Geng, J.Z. Punching Shear Strength of Slabs with Openings and Supported on Rectangular Columns. Struct. J. 2004, 101, 678–687. [Google Scholar] [CrossRef]
- Borges, L.L.J.; Melo, G.S.; Gomes, R.B. Punching Shear of Reinforced Concrete Flat Plates with Openings. ACI Struct. J. 2013, 110, 547–556. [Google Scholar] [CrossRef] [Green Version]
- Anil, Ö.; Kina, T.; Salmani, V. Effect of Opening Size and Location on Punching Shear Behaviour of Two-Way RC Slabs. Mag. Concr. Res. 2015, 66, 955–966. [Google Scholar] [CrossRef]
- Ha, T.; Lee, M.-H.; Park, J.; Kim, D.-J. Effects of Openings on the Punching Shear Strength of RC Flat-Plate Slabs without Shear Reinforcement. Struct. Des. Tall Spec. Build. 2015, 24, 895–911. [Google Scholar] [CrossRef]
- Balomenos, G.P.; Genikomsou, A.S.; Polak, M.A. Investigation of the Effect of Openings of Interior Reinforced Concrete Flat Slabs. Struct. Concr. 2018, 19, 1672–1681. [Google Scholar] [CrossRef]
- Liberati, E.A.P.; Marques, M.G.; Leonel, E.D.; Almeida, L.C.; Trautwein, L.M. Failure Analysis of Punching in Reinforced Concrete Flat Slabs with Openings Adjacent to the Column. Eng. Struct. 2019, 182, 331–343. [Google Scholar] [CrossRef]
- Genikomsou, A.S.; Polak, M.A. Effect of Openings on Punching Shear Strength of Reinforced Concrete Slabs—Finite Element Investigation. Struct. J. 2017, 114, 1249–1261. [Google Scholar] [CrossRef]
- Marques, M.G.; Liberati, E.A.P.; Gomes, R.B.; Almeida, L.C.; Trautwein, L.M. Study of Failure Mode of Reinforced Concrete Flat Slabs with Openings and Studs. Struct. J. 2020, 117, 39–48. [Google Scholar] [CrossRef]
- Anıl, Ö.; Ulusoy, B. Nonlinear FEA of Two-Way RC Slabs’ Punching Behavior with Openings. Iran. J. Sci. Technol. Trans. Civ. Eng. 2020, 44, 1109–1124. [Google Scholar] [CrossRef]
- da Silva Lourenço, D.; Liberati, E.A.P.; Marques, M.G.; de Almeida, L.C.; Trautwein, L.M. Reinforced Concrete Flat Slabs with Openings at Different Distances from the Column. Rev. Ibracon Estrut. Mater. 2020, 14, e14111. [Google Scholar] [CrossRef]
- Bora, A.S.; Gaidhankar, D.G.; Kulkarni, M. Analysis of Flat Slab With And Without Opening. Int. J. Sci. Technol. Res. 2019, 8, 1455–1459. [Google Scholar]
- ACI Committee 318 Aci 318-19; American Concrete Institute: Farmington Hills, MI, USA, 2019; ISBN 9781641950565.
- EN 1992-1-1: Eurocode 2: Design of Concrete Structures-Part 1-1: General Rules and Rules for Buildings; British Standard Institution: London, UK, 2004.
- Genikomsou, A.; Polak, M.A. Finite Element Simulation of Concrete Slabs with various Placement and Amount of Shear Bolts. Procedia Eng. 2017, 193, 313–320. [Google Scholar] [CrossRef]
- Johansen, K. Yield-Line Formulae for Slabs; CRC Press: London, UK, 1972. [Google Scholar]
- ASTM C39/C39M-14, Standard Test Method for Compressive Strength of Cylindrical Concrete Specimens; ASTM International: West Conshohocken, PA, USA, 2014.

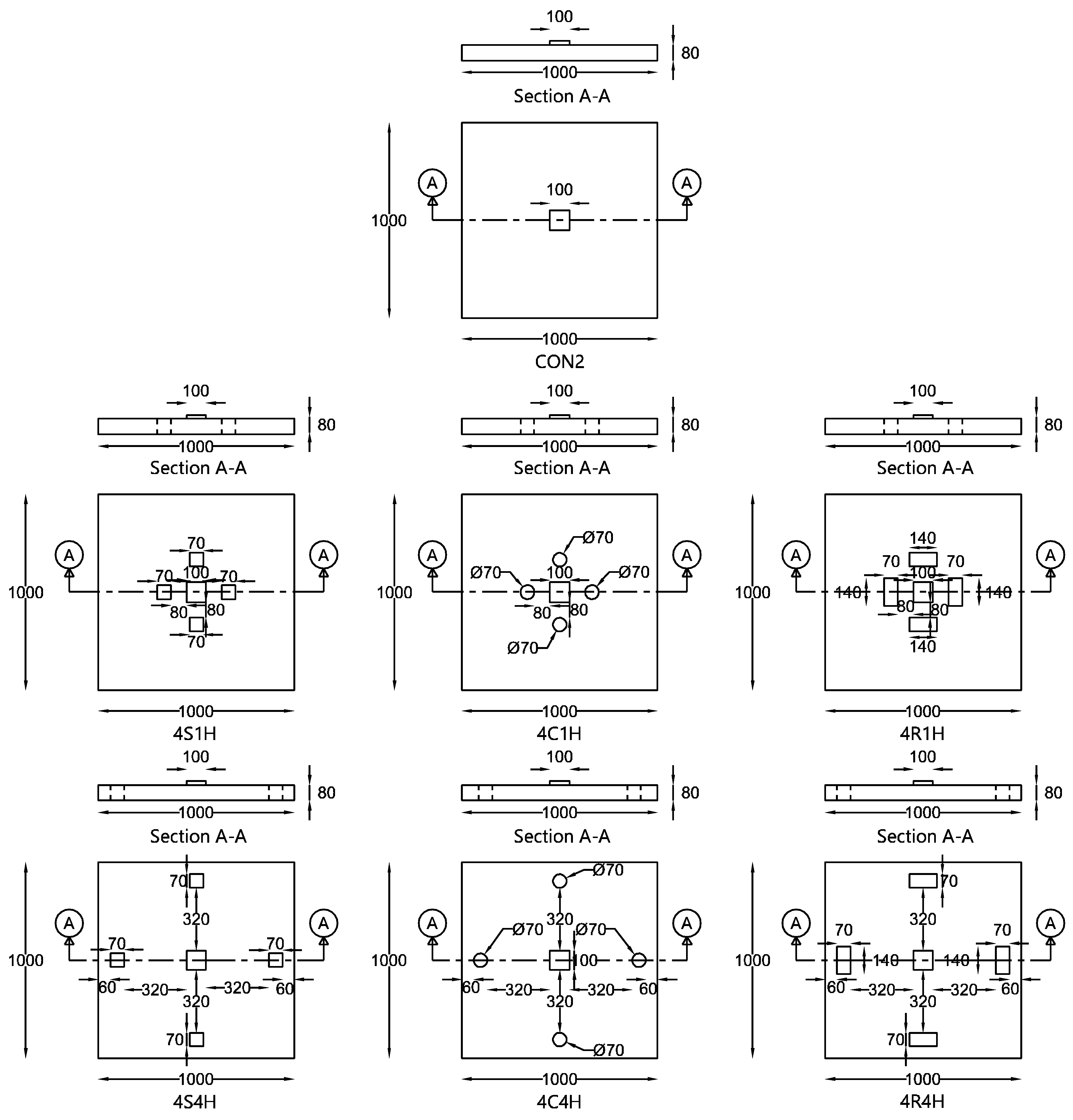




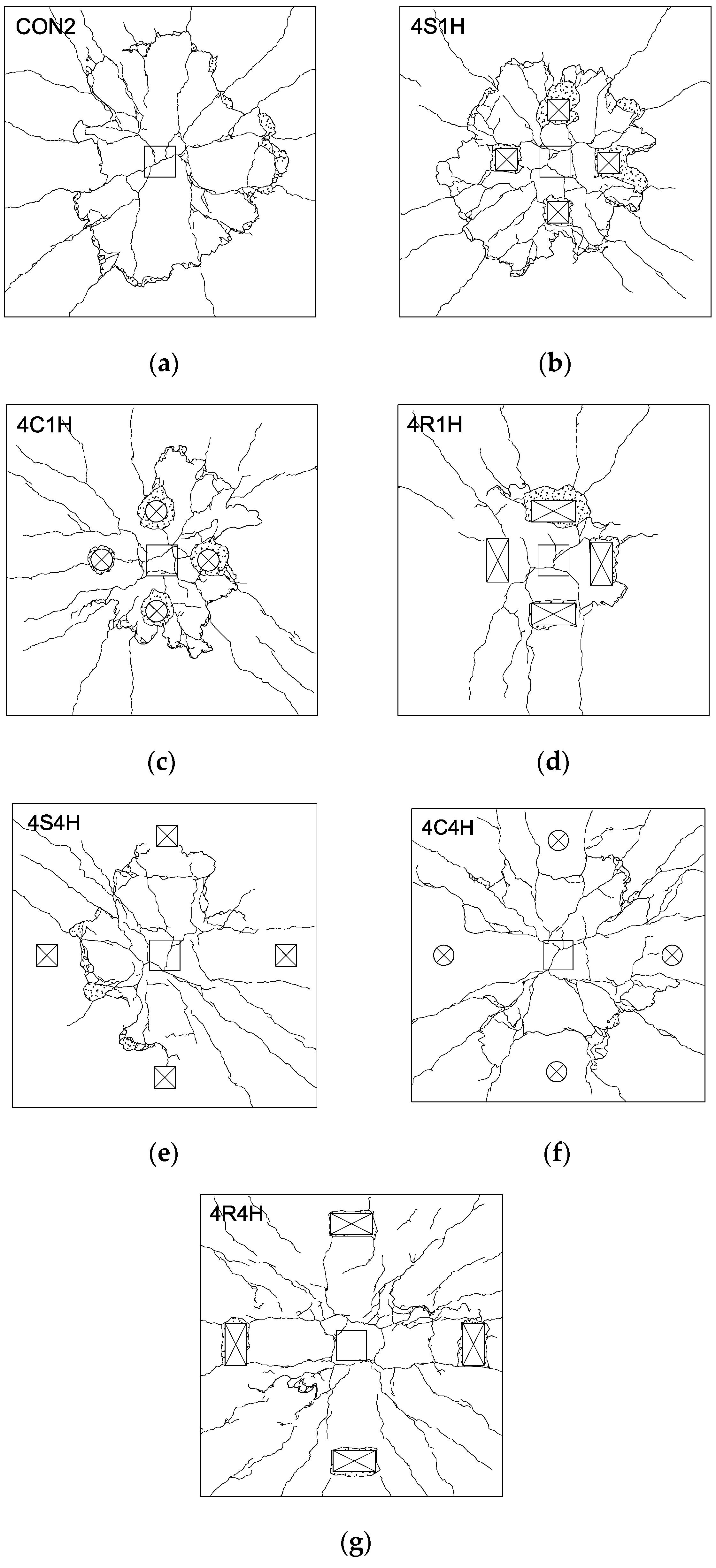





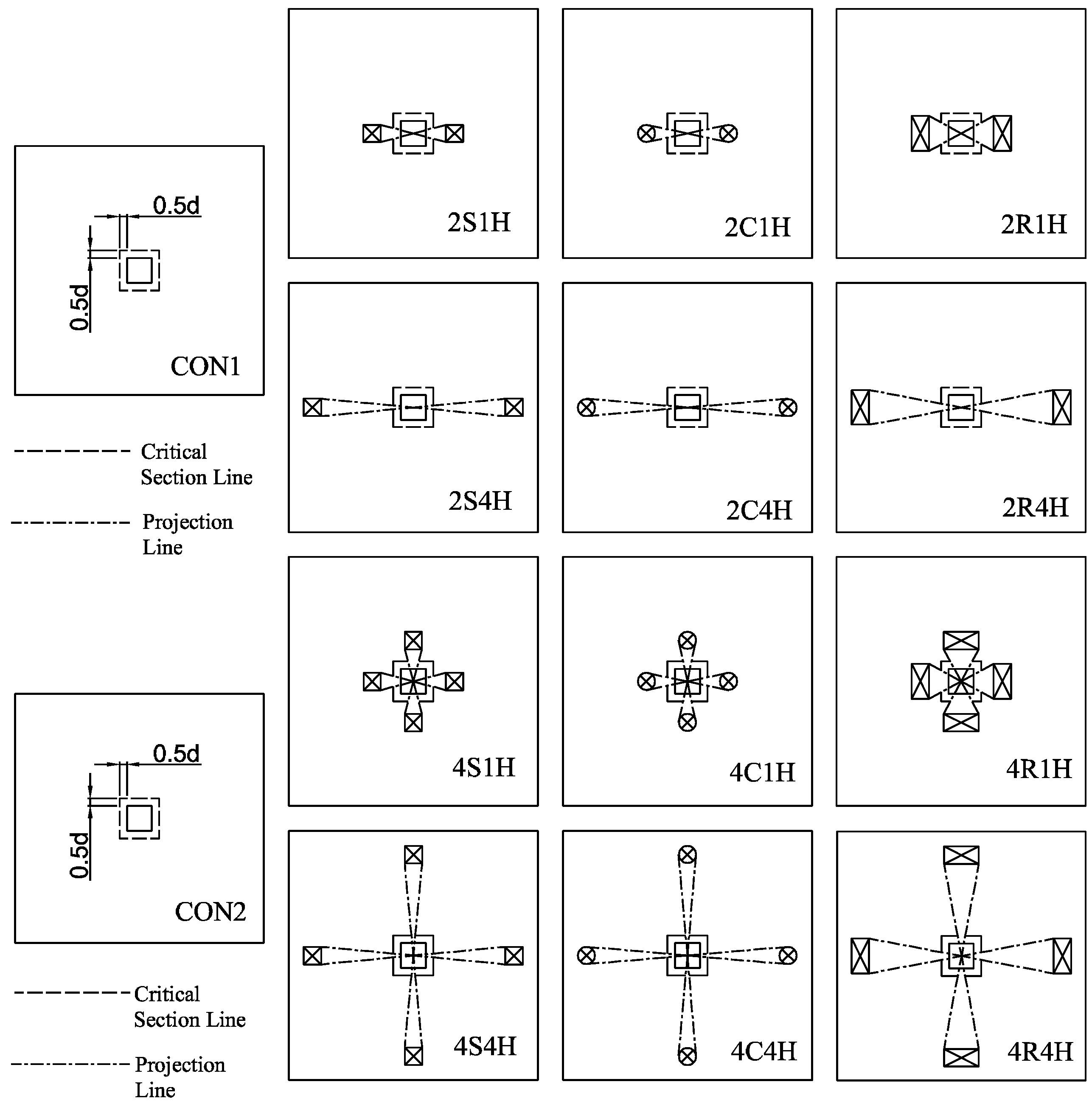
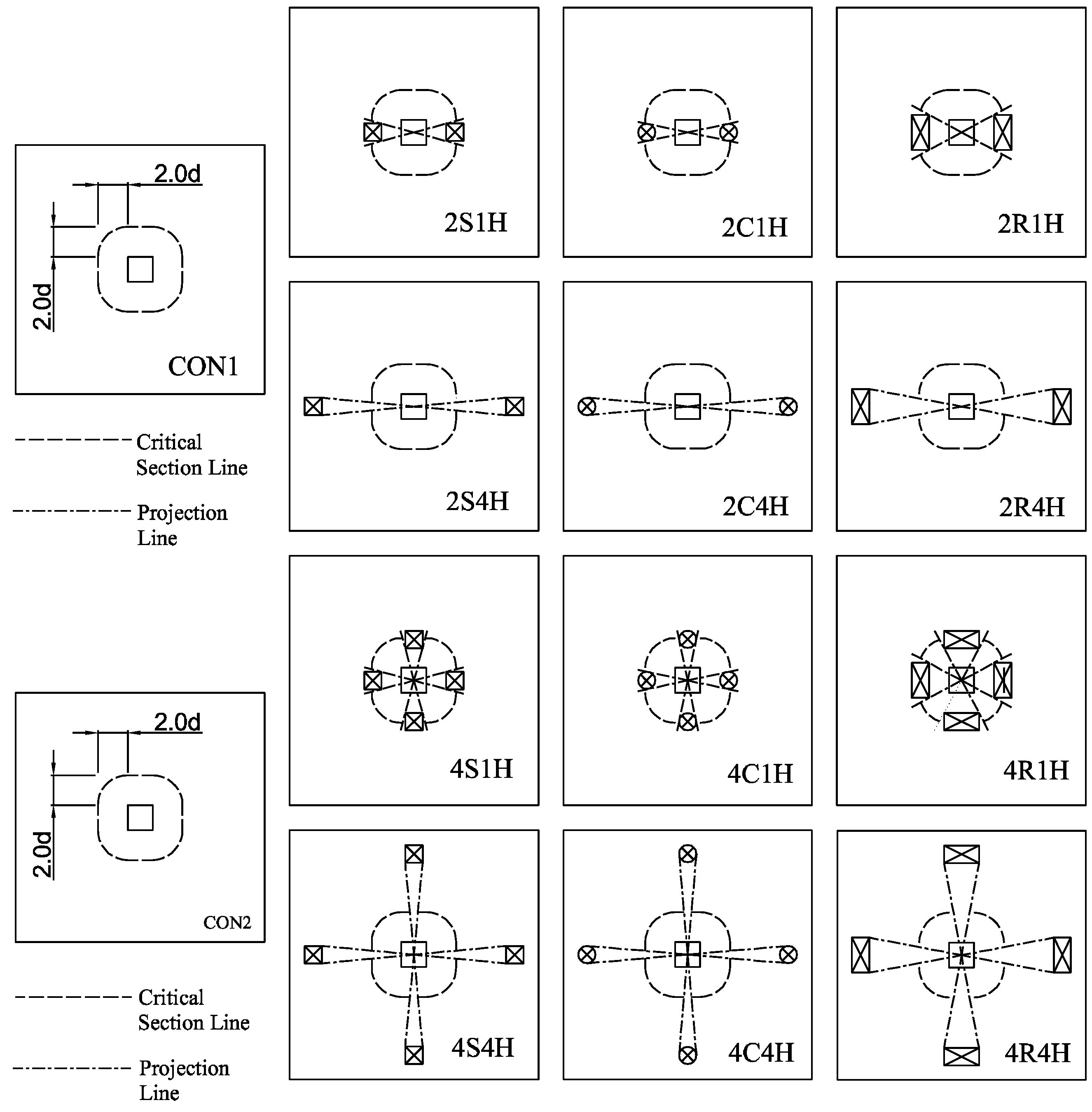

| Specimen No. | Specimen Name | Compressive Strength of Concrete (MPa) | Number of Opening | Opening Shape | Opening Size (mm) | Location (mm) |
|---|---|---|---|---|---|---|
| 1 | CON1 | 20.18 | - | Control | Control | Control |
| 2 | 2S1H | 20.18 | 2 | Square | 70 × 70 | 80 (1 h) |
| 3 | 2C1H | 20.18 | 2 | Circular | Diameter 70 | 80 (1 h) |
| 4 | 2R1H | 20.18 | 2 | Rectangular | 70 × 140 | 80 (1 h) |
| 5 | 2S4H | 20.18 | 2 | Square | 70 × 70 | 320 (4 h) |
| 6 | 2C4H | 20.18 | 2 | Circular | Diameter 70 | 320 (4 h) |
| 7 | 2R4H | 20.18 | 2 | Rectangular | 70 × 140 | 320 (4 h) |
| 8 | CON2 | 29.71 | - | Control | Control | Control |
| 9 | 4S1H | 29.71 | 4 | Square | 70 × 70 | 80 (1 h) |
| 10 | 4C1H | 29.71 | 4 | Circular | Diameter 70 | 80 (1 h) |
| 11 | 4R1H | 29.71 | 4 | Rectangular | 70 × 140 | 80 (1 h) |
| 12 | 4S4H | 29.71 | 4 | Square | 70 × 70 | 320 (4 h) |
| 13 | 4C4H | 29.71 | 4 | Circular | Diameter 70 | 320 (4 h) |
| 14 | 4R4H | 29.71 | 4 | Rectangular | 70 × 140 | 320 (4 h) |
| Specimen No. | Specimen Name | Flexural Capacity (kN) | Shear Capacity (kN) | |
|---|---|---|---|---|
| ACI 318-19 | Eurocode 2 | |||
| 1 | CON1 | 109.34 | 56.93 | 66.54 |
| 2 | 2S1H | 109.34 | 49.26 | 56.00 |
| 3 | 2C1H | 109.34 | 50.89 | 58.22 |
| 4 | 2R1H | 109.34 | 41.59 | 46.04 |
| 5 | 2S4H | 109.34 | 54.24 | 66.18 |
| 6 | 2C4H | 109.34 | 54.47 | 66.21 |
| 7 | 2R4H | 109.34 | 51.55 | 65.81 |
| 8 | CON2 | 115.15 | 69.07 | 75.70 |
| 9 | 4S1H | 115.15 | 50.47 | 51.72 |
| 10 | 4C1H | 115.15 | 54.44 | 50.20 |
| 11 | 4R1H | 115.15 | 31.89 | 29.07 |
| 12 | 4S4H | 115.15 | 62.94 | 74.86 |
| 13 | 4C4H | 115.15 | 63.11 | 74.94 |
| 14 | 4R4H | 115.15 | 56.03 | 74.02 |
| Specimen No. | Specimen Name | Compressive Strength of Concrete (MPa) | Peak | Failure Mode | |
|---|---|---|---|---|---|
| Load (kN) | Deflection (mm) | ||||
| 1 | CON1 | 20.18 | 57.73 | 7.745 | Punching Shear |
| 2 | 2S1H | 20.18 | 49.09 | 5.479 | Punching Shear |
| 3 | 2C1H | 20.18 | 51.10 | 5.835 | Punching Shear |
| 4 | 2R1H | 20.18 | 44.71 | 4.845 | Punching Shear |
| 5 | 2S4H | 20.18 | 57.04 | 7.616 | Punching Shear |
| 6 | 2C4H | 20.18 | 56.92 | 7.802 | Punching Shear |
| 7 | 2R4H | 20.18 | 55.50 | 7.236 | Punching Shear |
| 8 | CON2 | 29.71 | 70.15 | 11.829 | Punching Shear |
| 9 | 4S1H | 29.71 | 45.84 | 5.677 | Punching Shear |
| 10 | 4C1H | 29.71 | 49.06 | 7.244 | Punching Shear |
| 11 | 4R1H | 29.71 | 38.89 | 6.029 | Punching Shear |
| 12 | 4S4H | 29.71 | 67.61 | 9.299 | Punching Shear |
| 13 | 4C4H | 29.71 | 66.34 | 12.195 | Punching Shear |
| 14 | 4R4H | 29.71 | 63.85 | 9.847 | Punching Shear |
| No. | Name | (MPa) | Experimental (kN) | Reduction (%) | Analytical Punching Capacity (kN) | Ratio of Experimental to Analytical | ||
|---|---|---|---|---|---|---|---|---|
| ACI318 | Eurocode 2 | ACI318 | Eurocode 2 | |||||
| 1 | CON1 | 20.18 | 57.73 | - | 56.93 | 66.54 | 1.01 | 0.87 |
| 2 | 2S1H | 20.18 | 49.09 | 14.97 | 49.26 | 56.00 | 1.00 | 0.88 |
| 3 | 2C1H | 20.18 | 51.10 | 11.48 | 50.89 | 58.22 | 1.00 | 0.88 |
| 4 | 2R1H | 20.18 | 44.71 | 22.55 | 41.59 | 46.04 | 1.08 | 0.97 |
| 5 | 2S4H | 20.18 | 57.04 | 1.20 | 54.24 | 66.18 | 1.05 | 0.86 |
| 6 | 2C4H | 20.18 | 56.92 | 1.40 | 54.47 | 66.21 | 1.04 | 0.86 |
| 7 | 2R4H | 20.18 | 55.50 | 3.86 | 51.55 | 65.81 | 1.08 | 0.84 |
| 8 | CON2 | 29.71 | 70.15 | - | 69.07 | 75.70 | 1.02 | 0.93 |
| 9 | 4S1H | 29.71 | 45.84 | 34.65 | 50.47 | 51.72 | 0.91 | 0.89 |
| 10 | 4C1H | 29.71 | 49.06 | 30.06 | 54.44 | 50.20 | 0.90 | 0.98 |
| 11 | 4R1H | 29.71 | 38.89 | 44.56 | 31.86 | 29.07 | 1.22 | 1.34 |
| 12 | 4S4H | 29.71 | 67.61 | 3.62 | 62.94 | 74.86 | 1.07 | 0.9 |
| 13 | 4C4H | 29.71 | 66.34 | 5.43 | 63.11 | 74.94 | 1.05 | 0.89 |
| 14 | 4R4H | 29.71 | 63.85 | 8.98 | 56.03 | 74.02 | 1.14 | 0.86 |
| Average | 1.04 | 0.93 | ||||||
| Standard Deviation (SD) | 0.08 | 0.12 | ||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yooprasertchai, E.; Tiawilai, Y.; Wittayawanitchai, T.; Angsumalee, J.; Joyklad, P.; Hussain, Q. Effect of Shape, Number, and Location of Openings on Punching Shear Capacity of Flat Slabs. Buildings 2021, 11, 484. https://doi.org/10.3390/buildings11100484
Yooprasertchai E, Tiawilai Y, Wittayawanitchai T, Angsumalee J, Joyklad P, Hussain Q. Effect of Shape, Number, and Location of Openings on Punching Shear Capacity of Flat Slabs. Buildings. 2021; 11(10):484. https://doi.org/10.3390/buildings11100484
Chicago/Turabian StyleYooprasertchai, Ekkachai, Yonlada Tiawilai, Theerawee Wittayawanitchai, Jiranuwat Angsumalee, Panuwat Joyklad, and Qudeer Hussain. 2021. "Effect of Shape, Number, and Location of Openings on Punching Shear Capacity of Flat Slabs" Buildings 11, no. 10: 484. https://doi.org/10.3390/buildings11100484
APA StyleYooprasertchai, E., Tiawilai, Y., Wittayawanitchai, T., Angsumalee, J., Joyklad, P., & Hussain, Q. (2021). Effect of Shape, Number, and Location of Openings on Punching Shear Capacity of Flat Slabs. Buildings, 11(10), 484. https://doi.org/10.3390/buildings11100484






