Residual Strength of Reinforced Concrete Beams under Sequential Small Impact Loads
Abstract
:1. Introduction
2. Materials and Methods
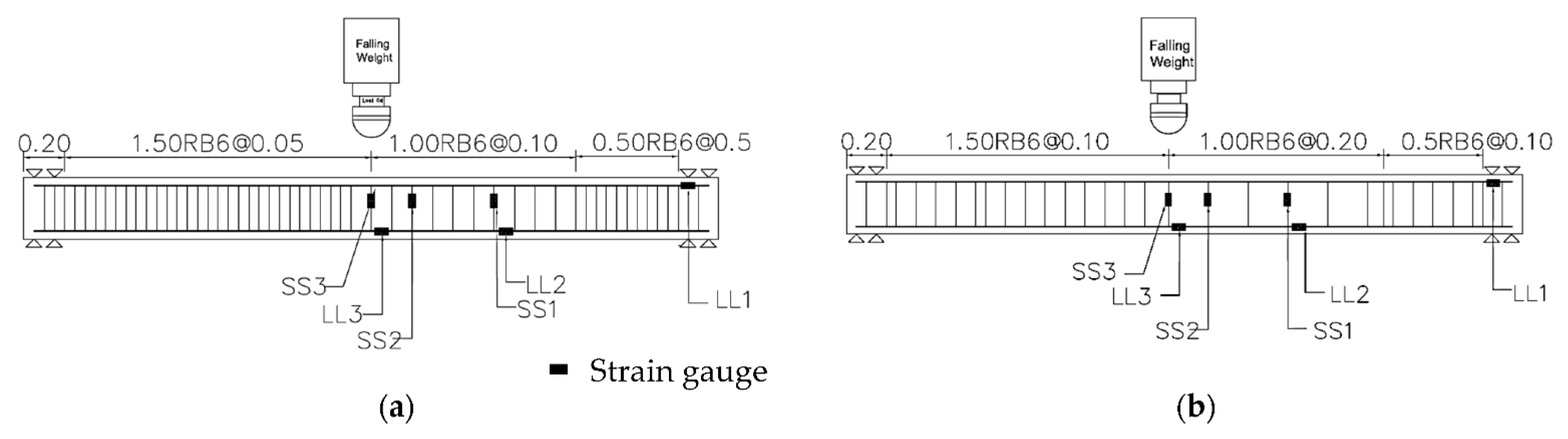
2.1. Test Specimens
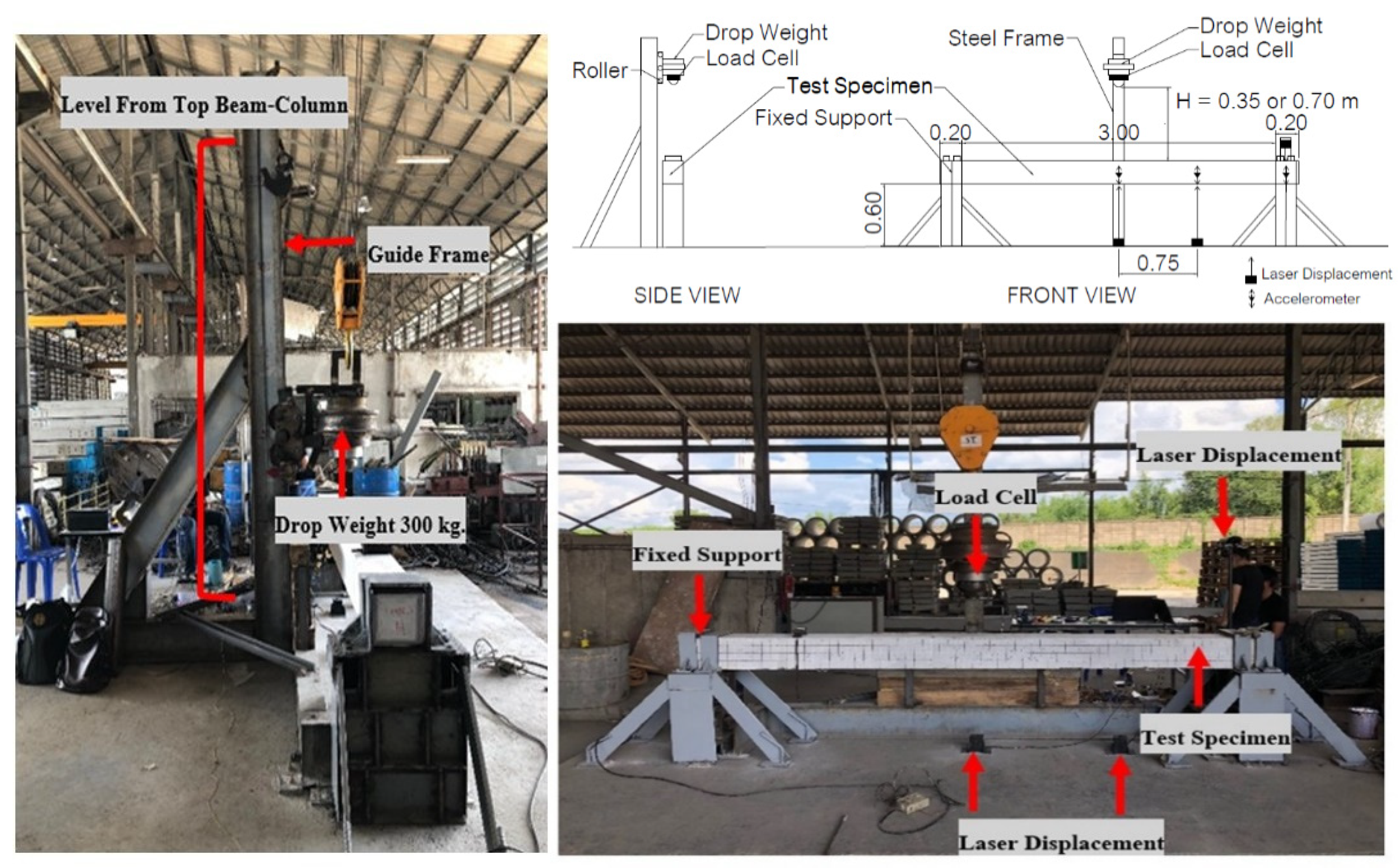
2.2. Sequential Small Impact Loading
3. Sequential Impact Test Results
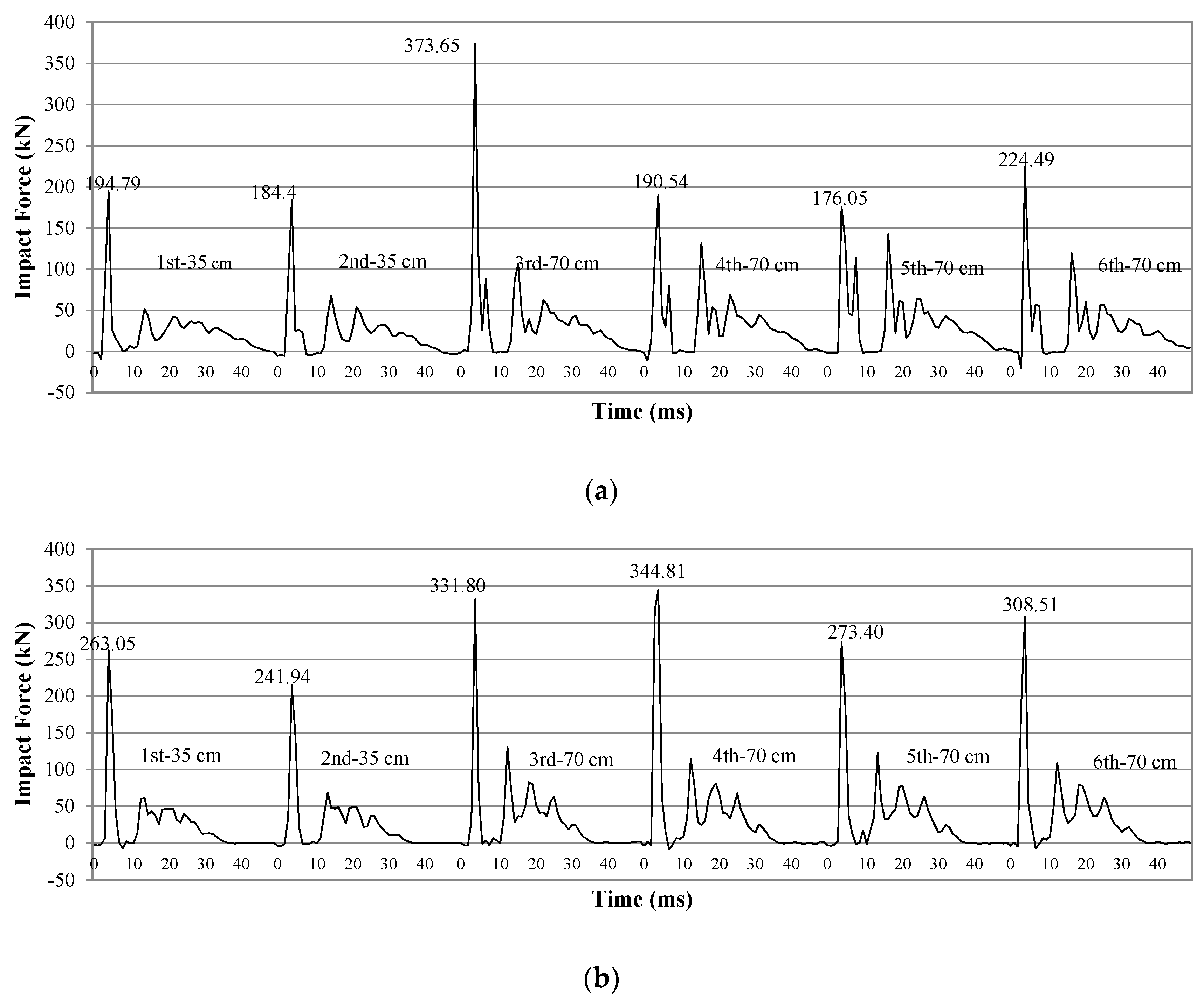
3.1. Impact Force and Midspan Deflection Time History
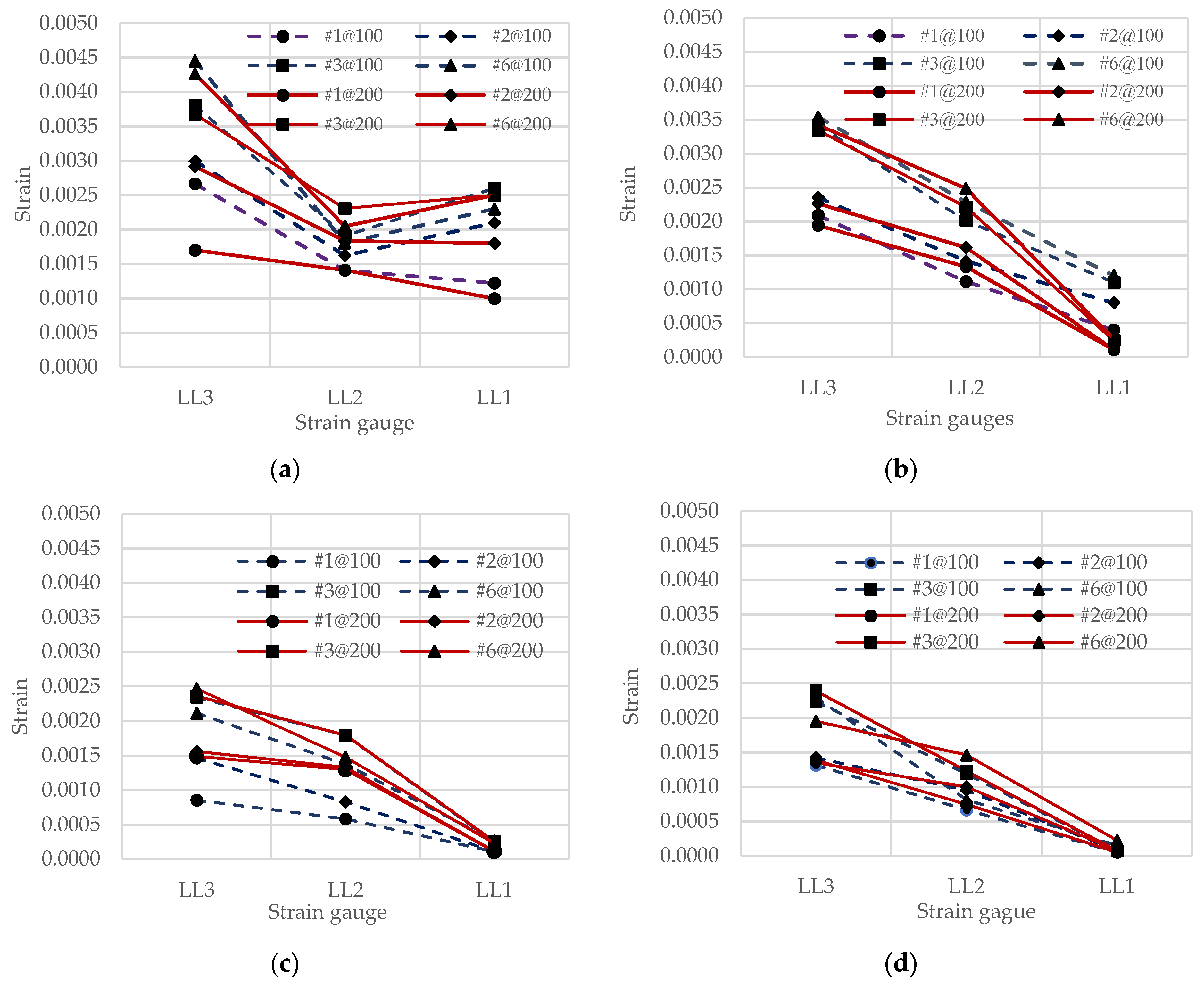
3.2. Strains of Longitudinal Reinforcement
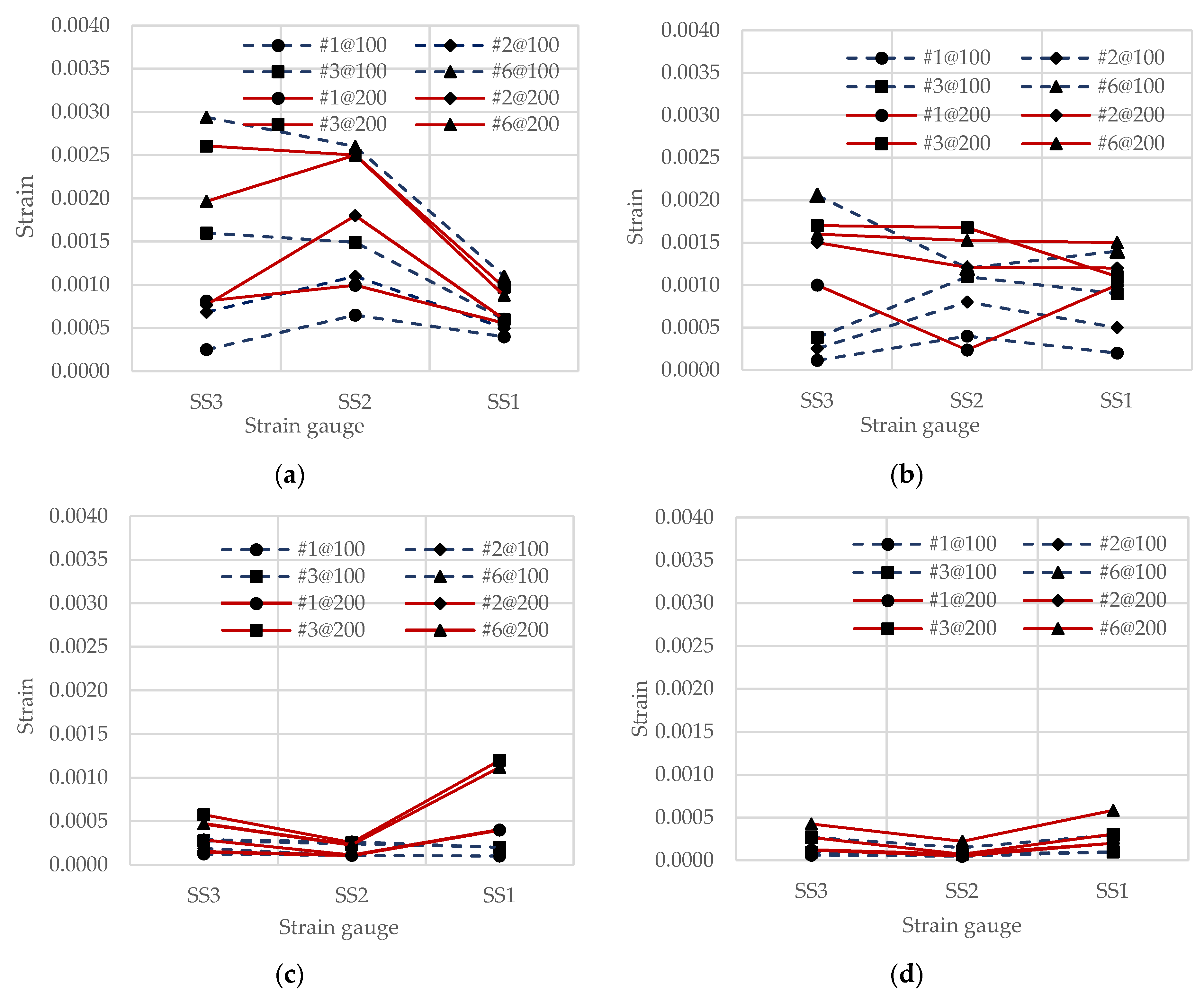
3.3. Strains of Stirrups
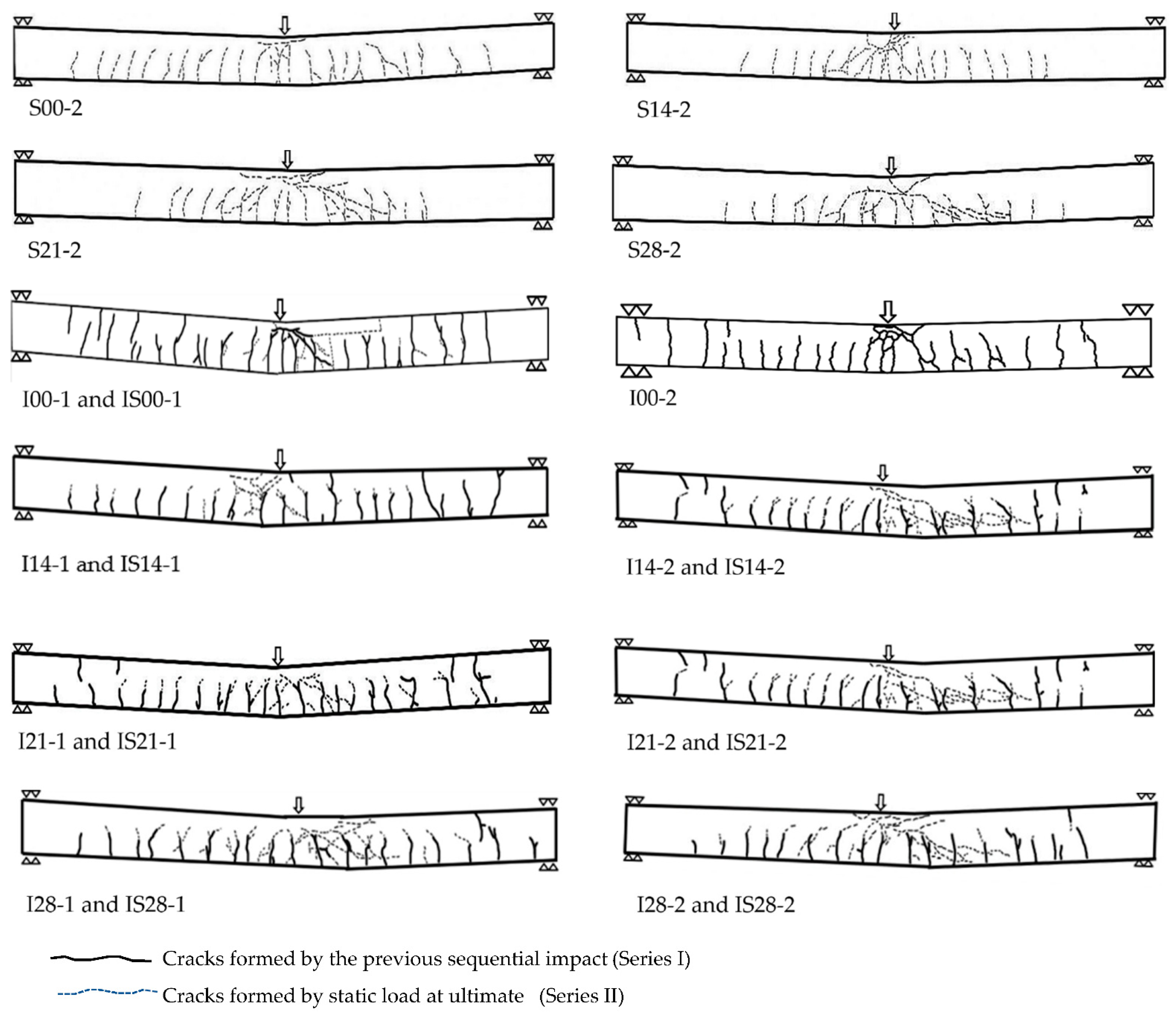
3.4. Crack Pattern
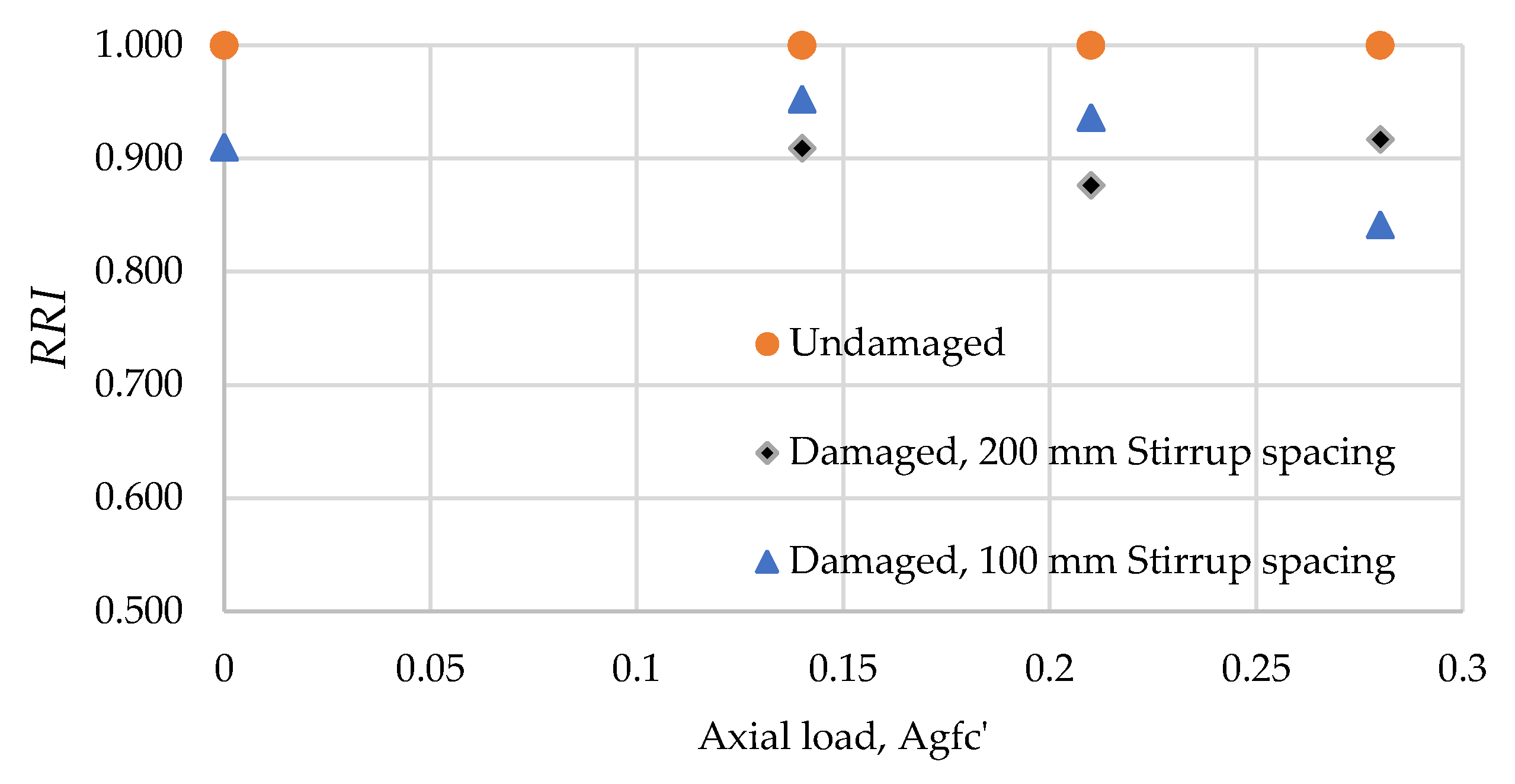
4. Static Reserved Strength
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hansapinyo, C.; Wongmatar, P.; Vimonsatit, V.; Chen, W. Pounding of Seismically Designed Low-rise Reinforced Concrete Frames. Proc. Inst. Civ. Eng. Struct. Build. 2018, 11, 172. [Google Scholar] [CrossRef]
- Do, T.V.; Pham, T.M.; Hao, H. Numerical Investigation of the Behavior of Precast Concrete Segmental Columns Subjected Vehicle Collision. Eng. Struct. 2018, 156, 375–393. [Google Scholar] [CrossRef] [Green Version]
- Jahami, A.; Temsah, Y.; Khatib, J. The efficiency of using CFRP as a strengthening technique for reinforced concrete beams subjected to blast loading. Int. J. Adv. Struct. Eng. 2019, 11, 411–420. [Google Scholar] [CrossRef] [Green Version]
- Jahami, A.; Temsah, Y.; Khatib, J.; Baalbaki, O.; Kenai, S. The behavior of CFRP strengthened RC beams subjected to blast loading. Mag. Civ. Eng. 2021, 103, 10309. [Google Scholar] [CrossRef]
- American Association of State Highway & Transportation Officials. AASHTO LRFD Bridge Design Specifications, 8th ed.; AASHTO: Washington, DC, USA, 2017. [Google Scholar]
- European Committee for Standardization. Eurocode 1: Actions on Structures—Part. 1-1: General Actions, Densities, Self-Weight, Imposed Loads for Buildings, (ANNEX B) BS EN 1991-1-1:2002; European Committee for Standardization: Brussels, Belgium, 2004. [Google Scholar]
- Wongmatar, P.; Hansapinyo, C.; Vimonsatit, V.; Chen, W. Recommendations for Designing Reinforced Concrete Beams Against Low Velocity Impact Loads. Int. J. Struct. Stab. Dyn. 2018, 18, 1850104. [Google Scholar] [CrossRef]
- Zhao, D.B.; Yi, W.J.; Kunnath, S.K. Shear Mechanism in Reinforced Concrete Beams under Impact Loading. J. Struct. Eng. 2017, 143, 04017089. [Google Scholar] [CrossRef]
- Saatci, S.; Vecchio, F.J. Effects of Shear Mechanisms on Impact Behavior of Reinforced Concrete Beams. ACI Struct. J. 2009, 106, 78–86. [Google Scholar]
- Kishi, N.; Mikami, H.; Matsuoka, K.G.; Ando, T. Impact Behavior of Shear-Failure-Type RC Beams Without Shear Rebars. Int. J. Impact Eng. 2002, 27, 955–968. [Google Scholar] [CrossRef]
- Fujikake, K.; Li, B.; Soeun, S. Impact Response of Reinforced Concrete Beam and Its Analytical Evaluation. J. Struct. Eng. 2009, 135, 938–950. [Google Scholar] [CrossRef]
- Tantrapongsaton, W.; Hansapinyo, C.; Wongmatar, P.; Chaisomphob, T. Flexural Reinforced Concrete Members with Minimum Reinforcement Under Low-Velocity Impact Load. Int. J. 2018, 14, 129–136. [Google Scholar] [CrossRef]
- Zhao, W.; Qian, J. Dynamic Response and Shear Demand of Reinforced Concrete Beams Subjected to Impact Loading. Int. J. Struct. Stab. Dyn. 2019, 19, 1950091. [Google Scholar] [CrossRef]
- Tantrapongsaton, W.; Hansapinyo, C. Impact Response of Reinforced Concrete Columns with Different Axial Load under Low-Velocity Impact Loading. Key Eng. Mater. 2019, 803, 322–330. [Google Scholar] [CrossRef]
- Chen, W.; Hao, H.; Chen, S. Numerical Analysis of Prestressed Reinforced Concrete Beam Subjected to Blast Loading. Mater. Des. 2015, 65, 662–674. [Google Scholar] [CrossRef]
- Li, H.; Chen, W.; Pham, T.M.; Hao, H. Analytical and Numerical Studies on Impact Force Profile of RC beam Under Drop Weight Impact. Int. J. Impact Eng. 2021, 147, 103743. [Google Scholar] [CrossRef]
- Lui, B.; Fan, W.; Guo, W.; Chen, B.; Liu, R. Experimental Investigation and Improved FE Modeling of Axially-Loaded Circular RC Columns Under Lateral Impact Loading. Eng. Struct. 2017, 152, 619–642. [Google Scholar] [CrossRef]
- Adhikary, S.D.; Li, B.; Fujikake, K. Residual Resistance of Impact Damaged Reinforced Concrete Beams. Mag. Concr. Res. 2015, 67, 364–378. [Google Scholar] [CrossRef]
- Huang, Z.; Chen, W.; Hao, H.; Chen, Z.; Pham, T.M.; Tran, T.T.; Elchalakani, M. Flexural Behaviour of Ambient Cured Geopolymer Concrete Beams Reinforced with BFRP Bars under Static and Impact Loads. Compos. Struct. 2020, 261, 113282. [Google Scholar] [CrossRef]
- Huang, Z.; Chen, W.; Tran, T.T.; Pham, T.M.; Hao, H.; Chen, Z.; Elchalakani, M. Experimental and Numerical Study on Concrete Beams Reinforced with Basalt FRP Bars under Static and Impact Loads. Compos. Struct. 2021, 263, 113648. [Google Scholar] [CrossRef]
- Tran, T.T.; Pham, T.M.; Huang, Z.; Chen, W.; Hao, H.; Elchalakani, M. Impact Response of Fibre Reinforced Geopolymer Concrete Beams with BFRP Bars and Stirrups. Eng. Struct. 2021, 231, 111785. [Google Scholar] [CrossRef]
- Tamai, H.; Sonoda, Y.; Bolander, J.E. Impact resistance of RC beams with reinforcement corrosion: Experimental observations. Constr. Build. Mater. 2020, 263, 120638. [Google Scholar] [CrossRef]
- ACI Committee 318. Building Code Requirements for Reinforced Concrete; American Concrete Institute: Detroit, MI, USA, 2014. [Google Scholar]
- Norton, R.L. Machine Design: An Integrated Approach, 4th ed.; Prentice Hall: Hoboken, NJ, USA, 2010. [Google Scholar]
- Comité Euro-International du Béton. Concrete Structures under Impact and Impulsive Loading; Bulletin d’Information CEB; No. 187; CEB Bulletins: Lausanne, Switzerland, 1988. [Google Scholar]
- Malvar, L.J.; Ross, C.A. Review of strain rate effects for concrete in tension. ACI Mater. J. 1998, 95, 735–739. [Google Scholar]
- Yan, D.; Lin, G. Dynamic properties of concrete in direct tension. Cem. Concr. Res. 2006, 36, 1371–1378. [Google Scholar] [CrossRef]
- Malvar, L.J. Review of static and dynamic properties of steel reinforcing bars. ACI Mater. J. 1998, 95, 609–616. [Google Scholar]
- Hao, Y.; Hao, H. Influence of the concrete DIF model on the numerical predictions of RC wall responses to blast loadings. Eng. Struct. 2014, 73, 24–38. [Google Scholar] [CrossRef]



| Test No. | Test Specimen | Axial Load (% ) | Stirrup Spacing (s, mm) | Calculated Static Capacity (P at Midspan) | ||
|---|---|---|---|---|---|---|
| Bending Capacity, Pf (kN) | Shear Capacity *, Pv (kN) | Capacity Ratio (Pf/Pv) | ||||
| Series I: Sequential impact load at midspan | ||||||
| 1 | I00-1 | 0.00 | 100 | 67.86 | (83.13 + 64.10) 147.23 | 0.461 |
| 2 | I00-2 | 0.00 | 200 | 67.86 | (83.13 + 32.05) 115.18 | 0.589 |
| 3 | I14-1 | 0.14 | 100 | 89.30 | (112.40 + 64.10) 176.50 | 0.506 |
| 4 | I14-2 | 0.14 | 200 | 89.30 | (112.40 + 32.05) 144.45 | 0.618 |
| 5 | I21-1 | 0.21 | 100 | 98.84 | (127.03 + 64.10) 191.13 | 0.517 |
| 6 | I21-2 | 0.21 | 200 | 98.84 | (127.03 + 32.05) 159.08 | 0.621 |
| 7 | I28-1 | 0.28 | 100 | 107.34 | (141.67 + 64.10) 205.77 | 0.522 |
| 8 | I28-2 | 0.28 | 200 | 107.34 | (141.67 + 32.05) 173.72 | 0.618 |
| Series II: Ultimate static load at midspan | ||||||
| 9 | S00-2 | 0.00 | 200 | 67.86 | (83.13 + 32.05) 115.18 | 0.589 |
| 10 | S14-2 | 0.14 | 200 | 89.30 | (112.40 + 32.05) 144.45 | 0.618 |
| 11 | S21-2 | 0.21 | 200 | 98.84 | (127.03 + 32.05) 159.08 | 0.621 |
| 12 | S28-2 | 0.28 | 200 | 107.34 | (141.67 + 32.05) 173.72 | 0.618 |
| 13 | IS00-1 | 0.00 | 100 | Damaged specimens from Series I and the calculated static capacity is not included. | ||
| 14 | IS14-1 | 0.14 | 100 | |||
| 15 | IS14-2 | 0.14 | 200 | |||
| 16 | IS21-1 | 0.21 | 100 | |||
| 17 | IS21-2 | 0.21 | 200 | |||
| 18 | IS28-1 | 0.28 | 100 | |||
| 19 | IS28-2 | 0.28 | 200 | |||
| Test | Peak Impact Force, kN | |||||
|---|---|---|---|---|---|---|
| 1st | 2nd | 3rd | 4th | 5th | 6th | |
| I00-1 | 194.8 | 184.4 | 373.7 | 190.5 | 176.1 | 224.5 |
| I00-2 | 207.8 | 174.9 | 221.5 | 340.3 | 336.1 | 247.5 |
| I14-1 | 276.0 | 172.3 | 263.4 | 293.6 | 314.2 | 268.3 |
| I14-2 | 199.3 | 183.7 | 325.6 | 219.3 | 238.9 | 318.0 |
| I21-1 | 186.4 | 178.4 | 303.3 | 352.0 | 291.0 | 323.4 |
| I21-2 | 190.6 | 211.4 | 202.4 | 284.3 | 292.9 | 316.7 |
| I28-1 | 232.3 | 235.7 | 312.3 | 205.4 | 279.0 | 245.0 |
| I28-2 | 263.0 | 214.9 | 331.8 | 344.8 | 273.4 | 308.5 |
| Specimen | Axial Load | Ultimate Static Load (kN) | RRI |
|---|---|---|---|
| S00-2 | 0 | 74.90 | 1.000 |
| IS00-1 | 0 | 68.17 | 0.910 |
| S14-2 | 0.14 | 114.10 | 1.000 |
| IS14-1 | 0.14 | 108.68 | 0.952 |
| IS14-2 | 0.14 | 103.75 | 0.909 |
| S21-2 | 0.21 | 121.00 | 1.000 |
| IS21-1 | 0.21 | 113.28 | 0.936 |
| IS21-2 | 0.21 | 106.07 | 0.877 |
| S28-2 | 0.28 | 130.00 | 1.000 |
| IS28-1 | 0.28 | 109.39 | 0.841 |
| IS28-2 | 0.28 | 119.22 | 0.917 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hansapinyo, C.; Limkatanyu, S.; Zhang, H.; Imjai, T. Residual Strength of Reinforced Concrete Beams under Sequential Small Impact Loads. Buildings 2021, 11, 518. https://doi.org/10.3390/buildings11110518
Hansapinyo C, Limkatanyu S, Zhang H, Imjai T. Residual Strength of Reinforced Concrete Beams under Sequential Small Impact Loads. Buildings. 2021; 11(11):518. https://doi.org/10.3390/buildings11110518
Chicago/Turabian StyleHansapinyo, Chayanon, Suchart Limkatanyu, Hexin Zhang, and Thanongsak Imjai. 2021. "Residual Strength of Reinforced Concrete Beams under Sequential Small Impact Loads" Buildings 11, no. 11: 518. https://doi.org/10.3390/buildings11110518
APA StyleHansapinyo, C., Limkatanyu, S., Zhang, H., & Imjai, T. (2021). Residual Strength of Reinforced Concrete Beams under Sequential Small Impact Loads. Buildings, 11(11), 518. https://doi.org/10.3390/buildings11110518







