Effects of Coarse Aggregate Maximum Size on Synthetic/Steel Fiber Reinforced Concrete Performance with Different Fiber Parameters
Abstract
:1. Introduction
2. Objectives
3. Experimental Program
3.1. Materials
3.2. Mix Design
3.3. Specimen Preparation
3.4. Experimental Methods
4. Results and Discussions
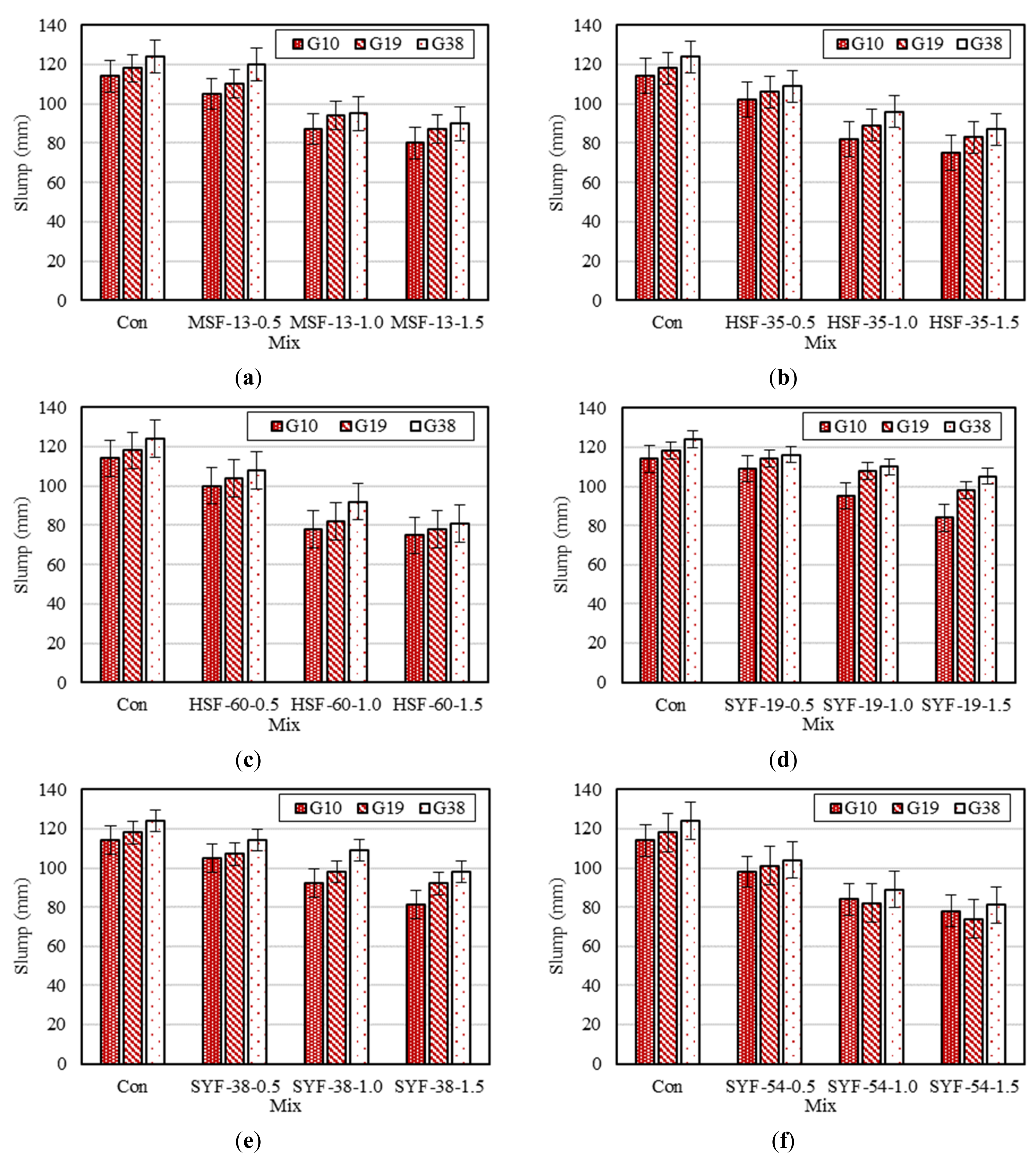
4.1. Effect of Fiber Properties on Flowability
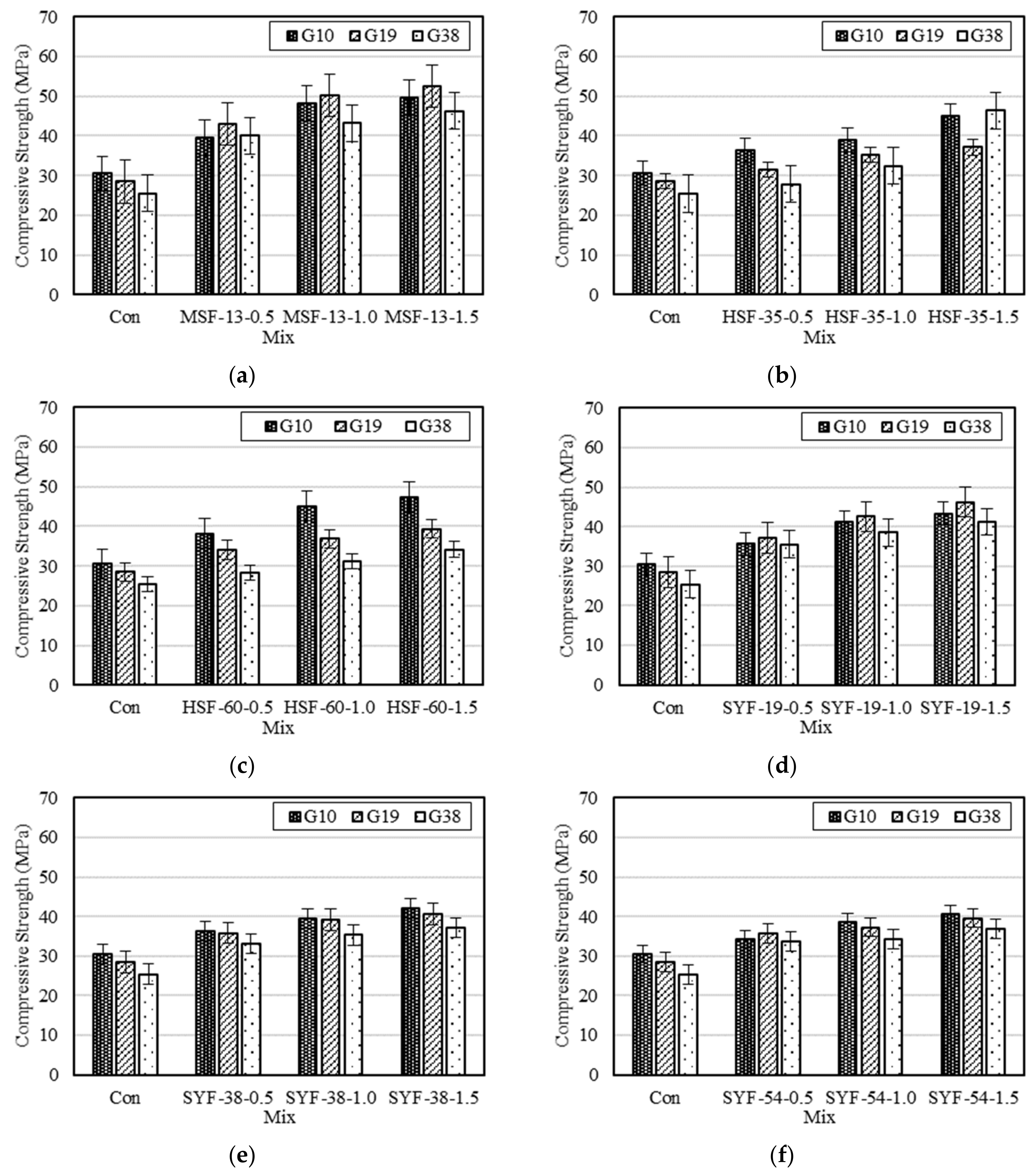
4.2. Effect of Fiber Properties on the Compressive Strength
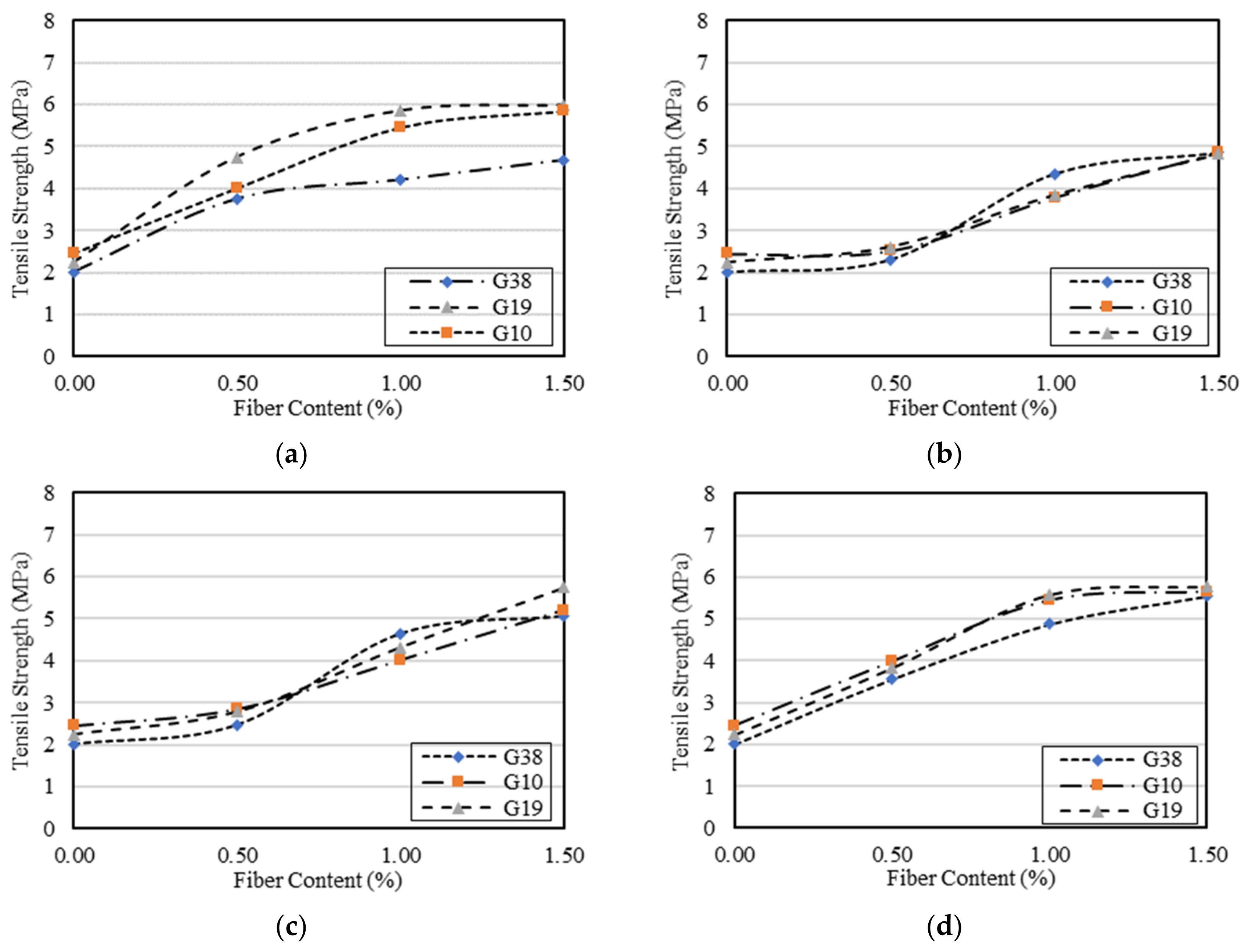
4.3. Effect of Fiber Properties on the Tensile Strength
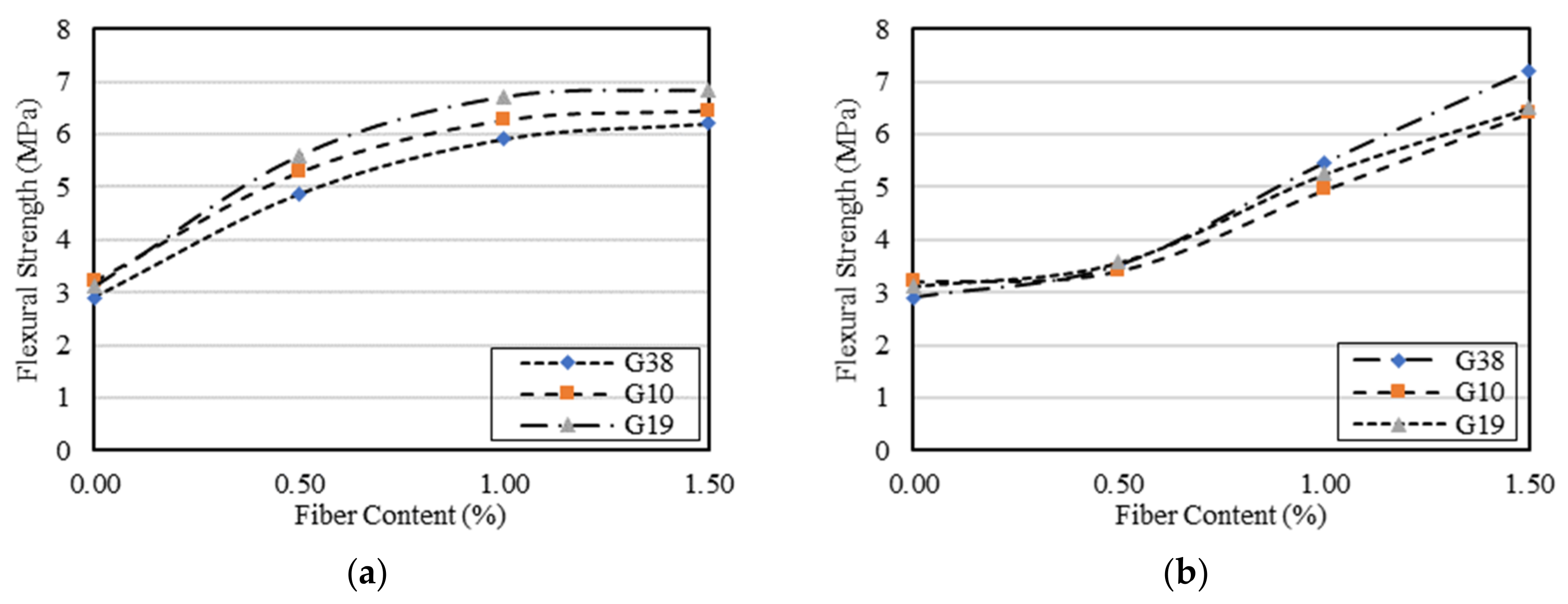
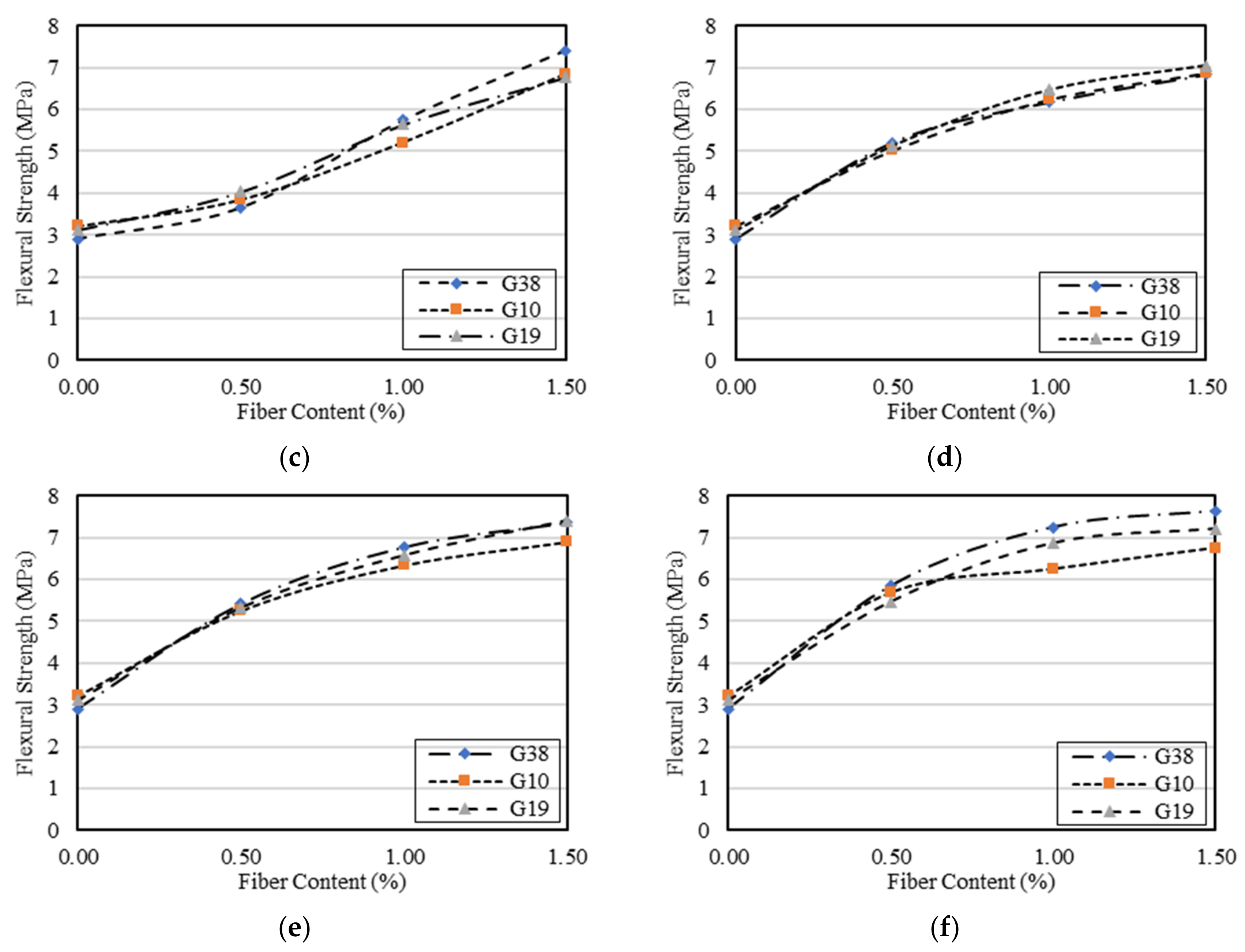
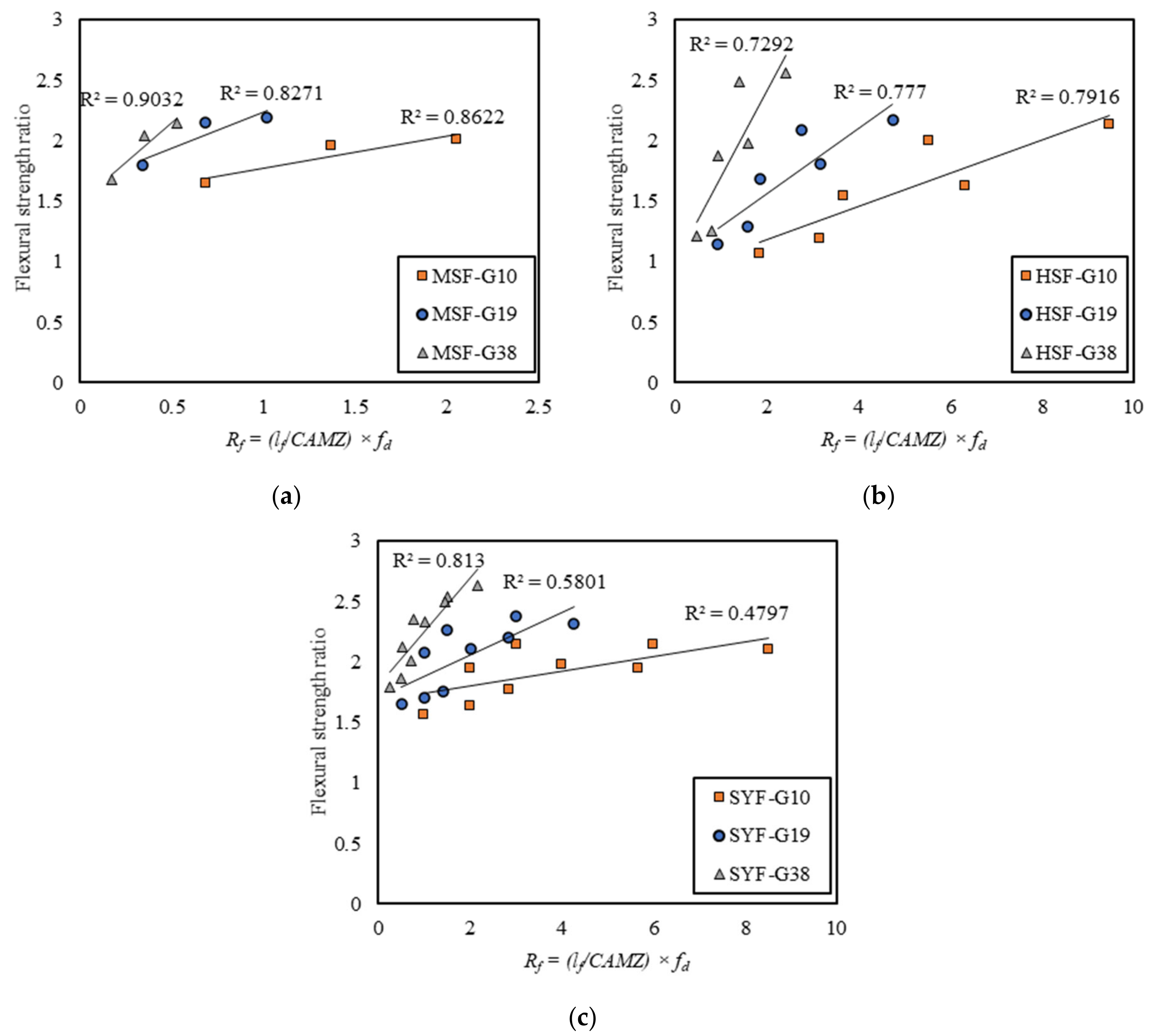
4.4. Effect of Fiber Properties on the Flexural Strength
4.5. Correlations among FRC Properties
4.5.1. Compressive and Splitting Tensile Strengths of FRC
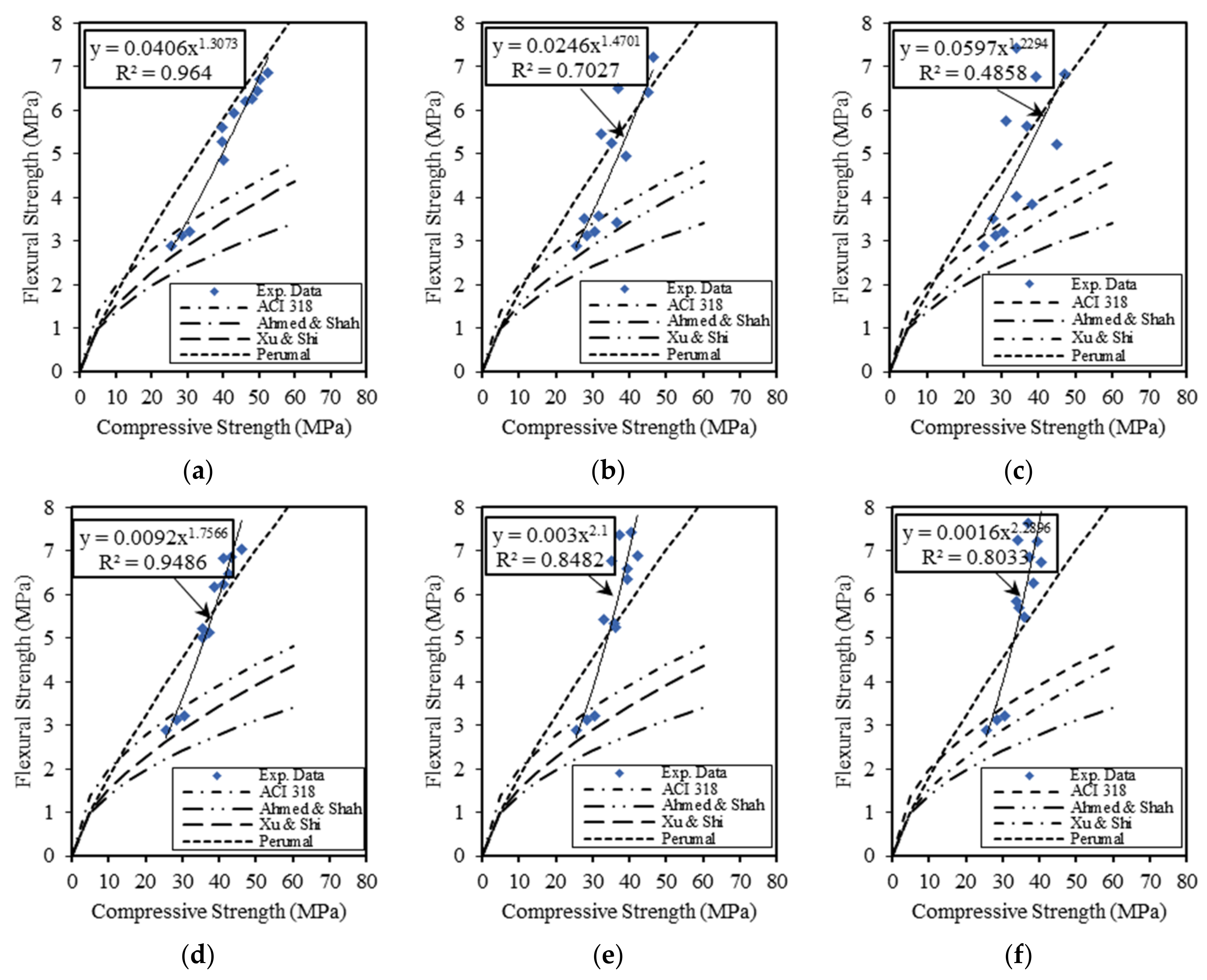
4.5.2. Compressive and Flexural Strengths of FRC
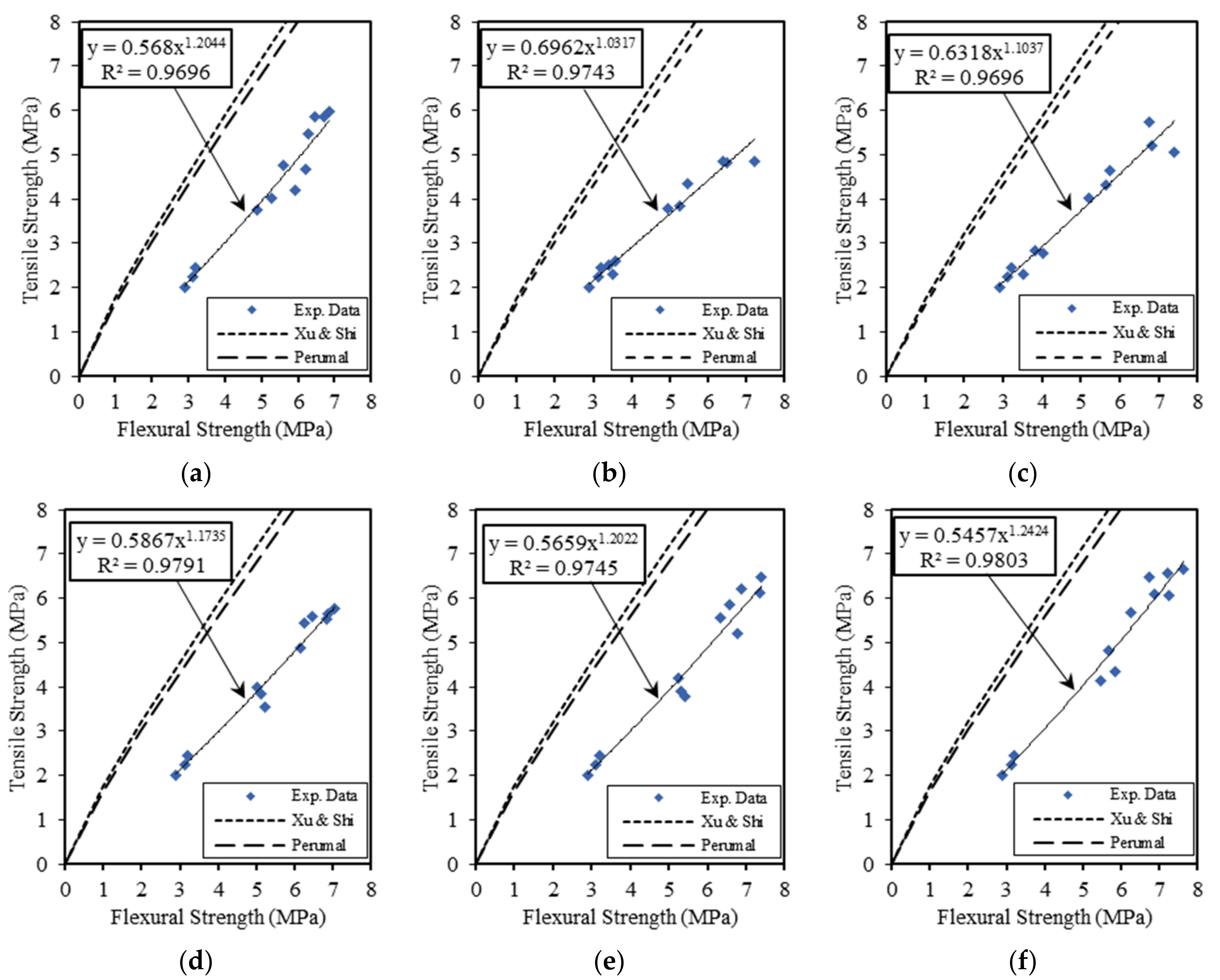
4.5.3. Splitting Tensile and Flexural Strengths of FRC
5. Conclusions
- The slump test revealed that the slump of fresh synthetic/steel fiber mix produced a decreasing tendency with the synthetic/steel fiber length’s increase. This decline in the fresh concrete flowability might have been due to the fiber length increase, which raised the overlap between fibers. Thus, the impact of the fiber–concrete matrix took the lead for the decrease of the mix slump, even though the number of fibers was lower in the same fiber dosage with the same volume of concrete;
- The compressive strength was only marginally affected by the CAMZ compared with the fiber dosage and geometrical properties, such as the length and diameter, which had an insignificant influence on the compressive strength;
- The splitting tensile strength of the FRC specimens improved with a CAMZ up to 19 mm and then declined with a CAMZ of 37.5 mm. With the rise of the CAMZ, the total surface area of the coarse aggregate reduced, which decreased the concrete matrix quantity covering the aggregate. Therefore, the large CAMZ might have had a disadvantage in the fiber distribution of fiber in the concrete that reduced the strengthening impact of the fiber on the concrete strength;
- There was a significant relationship between the tensile strength ratio and ratio (Rt) for all CAMZs. The bridging effect developed by fibers crossing cracks was improved with the increase of the Rt factor, which enhanced the FRC machinal performance. The results demonstrate that the fiber reinforcing effect on the tensile strength was substantial with the ratio (Rt) of the fiber length to the CAMZ multiplied by the fiber dosage (Rt = (lf/CAMZ) × fd);
- The synthetic fibers had a larger surface area than the hooked end fibers, causing a higher bond strength between the synthetic fiber and concrete matrix. In addition, the same explanations drawn for the differences in tensile strength with the rise of the fiber dosage, length, and CAMZ were primarily accountable for the differences in flexural strength;
- All mixtures were intended to exhibit similar compressive strengths. However, the synthetic/steel fiber advanced the brittleness ratios of the specimens with G10, G19, and G38 to be approximately 36.8%, 40.7%, and 47.4% greater than the Con specimens, respectively. Due to the fiber’s ability to bridge macro and microcracks, the enhancement amount of flexural strength depended on the fiber dosage and length;
- The flexural strength ratio significantly rose with the ratio (Rf) increase with good correlation. Additionally, there was a significant relationship between the flexural strength ratio and ratio (Rf) for all CAMZs. It is evident that the fiber reinforcing effect on the flexural strength was substantial with the ratio (Rt) of the fiber length to the CAMZ multiplied by the fiber dosage (Rf);
- The mechanical properties of synthetic and steel FRC could be influenced by many factors, such as the fiber type, steel fiber geometry, fiber parameters, aspect ratio, and fiber dosage. In addition, it should be highlighted that the regression analysis investigation was conducted for all compressive strengths, splitting tensile strengths, and flexural strengths of the synthetic and steel FRC with the consideration of these factors. These factors were a fiber length from 13 mm to 60 mm, CAMZ from 9.5 mm to 37.5 mm, and ratio of the fiber length to the CAMZ in the range of 0.35–5.68. Therefore, it can be noted that there were strong correlations from the regression analysis of the mechanical property results of synthetic and steel FRC.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Thomas, J.; Ramaswamy, A. Mechanical properties of steel fiber- reinforced concrete. J. Mater. Civ. Eng. 2007, 19, 385–392. [Google Scholar] [CrossRef]
- Mohammadi, Y.; Singh, S.P.; Kaushik, S.K. Properties of steel fibrous concrete containing mixed fibers in fresh and hardened state. Constr. Build. Mater. 2008, 22, 956–965. [Google Scholar] [CrossRef]
- Song, P.S.; Hwang, S. Mechanical properties of high-strength steel fiber- reinforced concrete. Constr. Build. Mater. 2004, 18, 669–673. [Google Scholar] [CrossRef]
- Sahin, Y.; Köksal, F. The influences of matrix and steel fiber tensile strengths on the fracture energy of high-strength concrete. Constr. Build. Mater. 2011, 25, 1801–1806. [Google Scholar] [CrossRef]
- Grünewald, S.; Walraven, J.C. Parameter-study on the influence of steel fibers and coarse aggregate content on the fresh properties of self- compacting concrete. Cem. Concr. Res. 2001, 31, 1793–1798. [Google Scholar] [CrossRef]
- Wu, Z.; Shi, C.; He, W.; Wu, L. Effects of steel fiber content and shape on mechanical properties of ultra high performance concrete. Constr. Build. Mater. 2016, 103, 8–14. [Google Scholar] [CrossRef]
- Ding, X.; Li, C.; Zhao, M.; Li, J.; Geng, H.; Lian, L. Tensile Behavior of Self-Compacting Steel Fiber Reinforced Concrete Evaluated by Different Test Methods. Crystals 2021, 11, 251. [Google Scholar] [CrossRef]
- Hassan, R.F.; Jaber, M.H.; Al-Salim, N.H.; Hussein, H.H. Experimental research on torsional strength of synthetic/steel fiber-reinforced hollow concrete beam. Eng. Struct. 2020, 220, 110948. [Google Scholar] [CrossRef]
- Elices, M.; Rocco, C.G. Effect of aggregate size on the fracture and mechanical properties of a simple concrete. Eng. Fract. Mech. 2008, 75, 3839–3851. [Google Scholar] [CrossRef]
- Appa, G.; Rao, B.K. Raghu Prasad, Fracture energy and softening behavior of high-strength concrete. Cem. Concr. Res. 2002, 32, 247–252. [Google Scholar]
- Khan, M.I.; Abbass, W.; Alrubaidi, M.; Alqahtani, F.K. Optimization of the Fine to Coarse Aggregate Ratio for the Workability and Mechanical Properties of High Strength Steel Fiber Reinforced Concretes. Materials 2020, 13, 5202. [Google Scholar] [CrossRef] [PubMed]
- Beygi, M.H.A.; Kazemi, M.T.; Nikbin, M.I.; Amiri, J.V.; Rabbanifar, S.; Rahmani, E. The influence of coarse aggregate size and volume on the fracture behavior and brittleness of self-compacting concret. Cem. Concr. Res. 2014, 66, 75–90. [Google Scholar] [CrossRef]
- Beygi, M.H.A.; Kazemi, M.T.; Nikbin, M.I.; Amiri, J.V.; Rabbanifar, S.; Rahmani, E. Evaluation of the effect of maximum aggregate size on fracture behavior of self compacting concrete. Constr. Build. Mater. 2014, 55, 202–211. [Google Scholar] [CrossRef]
- Jang, S.J.; Yun, Y.J.; Yun, H.D. Influence of fiber volume fraction and aggregate size on flexural behavior of high strength steel fiber-reinforced concrete (SFRC). Appl. Mech. Mater. 2013, 372, 223–226. [Google Scholar] [CrossRef]
- Olivito, R.S.; Zuccarell, F.A. An experimental study on the tensile strength of steel fiber reinforced concrete. Composites 2010, 41, 246–255. [Google Scholar] [CrossRef]
- Yao, W.; Cai, J.; Keru, W.; Zhou, Z. Study of flexural toughness of steel fiber reinforced concrete. Concrete 2002, 6, 30–33. [Google Scholar]
- Uygunog˘lu, T. Investigation of microstructure and flexural behavior of steel-fiber reinforced concrete. Mater. Struct. 2008, 41, 1441–1449. [Google Scholar] [CrossRef]
- Bayramov, F.; Tasdemir, C.; Tasdemir, M.A. Optimisation of steel fiber reinforced concretes by means of statistical response surface method. Cem. Concr. Compos. 2004, 26, 665–675. [Google Scholar] [CrossRef]
- Yoo, D.-Y.; Kang, S.-T.; Yoon, Y.-S. Effect of fiber length and placement method on flexural behavior, tension-softening curve, and fiber distribution characteristics of UHPFRC. Constr. Build. Mater. 2014, 64, 67–81. [Google Scholar] [CrossRef]
- Yoo, D.-Y.; Zi, G.; Kang, S.-T.; Yoon, Y.-S. Biaxial flexural behavior of ultra-high-performance fiber-reinforced concrete with different fiber lengths and placement methods. Cem. Concr. Compos. 2015, 63, 51–66. [Google Scholar] [CrossRef]
- Al-Masoodi, A.H.H.; Kawan, A.; Kasmuri, M.; Hamid, R.; Khan, M.N.N. Static and dynamic properties of concrete with different types and shapes of fibrous reinforcement. Constr. Build. Mater. 2016, 104, 247–262. [Google Scholar] [CrossRef]
- Blanco, A.; Pujadas, P.; De la Fuente, A.; Cavalaro, S.H.P.; Aguado, A. Assessment of the fiber orientation factor in SFRC slabs. Compos. Part B 2015, 68, 343–354. [Google Scholar] [CrossRef] [Green Version]
- Sergio, H.; Cavalaro, P.; López, R.; Torrents, J.M.; Aguado, A. Improved assessment of fiber content and orientation with inductive method in SFRC. Mater. Struct. 2015, 48, 1859–1873. [Google Scholar]
- Faris, M.A.; Abdullah, M.M.A.B.; Muniandy, R.; Abu Hashim, M.F.; Błoch, K.; Jeż, B.; Ghazali, M.F. Comparison of Hook and Straight Steel Fibers Addition on Malaysian Fly Ash-Based Geopolymer Concrete on the Slump, Density, Water Absorption and Mechanical Properties. Materials 2021, 14, 1310. [Google Scholar] [CrossRef] [PubMed]
- Zhang, P.; Yang, Y.; Wang, J.; Hu, S.; Jiao, M.; Ling, Y. Mechanical Properties and Durability of Polypropylene and Steel Fiber-Reinforced Recycled Aggregates Concrete (FRRAC): A Review. Sustainability 2020, 12, 9509. [Google Scholar] [CrossRef]
- Yardimci, M.Y.; Baradan, B.; Tasdemir, M.A. Effect of Fine to Coarse Aggregate Ratio on the Rheology and Fracture Energy of Steel Fiber Reinforced Self-Compacting Concretes; Indian Academy of Sciences: Bengaluru, India, 2014; Volume 39, pp. 1447–1469. [Google Scholar]
- Lee, C.; Kim, H. Orientation factor and number of fibers at failure plane in ring- type steel fiber reinforced concrete. Cem. Concr. Res. 2010, 40, 810–819. [Google Scholar] [CrossRef]
- Kang, S.T.; Lee, B.Y.; Kim, J.K.; Kim, Y.K. The effect of fiber distribution characteristics on the flexural strength of steel fiber-reinforced ultra high strength concrete. Constr. Build. Mater. 2011, 25, 2450–2457. [Google Scholar] [CrossRef]
- ASTM. C150-04a. Standard Specification for Portland Cement; ASTM International: West Conshohocken, PA, USA, 2004. [Google Scholar]
- ASTM. C33-03. Standard Specification for Concrete Aggregates; ASTM International: West Conshohocken, PA, USA, 2003. [Google Scholar]
- BSI. BS EN 12390-3. Testing Hardened Concrete. Compressive Strength of Test Specimen; BSI: London, UK, 2009. [Google Scholar]
- ASTM. C496/C496M-17. Standard Test Method for Splitting Tensile Strength of Cylindrical Concrete Specimens; ASTM International: West Conshohocken, PA, USA, 2017. [Google Scholar]
- ASTM. C78/C78M-10, Standard Test Method for Flexural Strength of Concrete (Using Simple Beam with Third-Point Loading; ASTM International: West Conshohocken, PA, USA, 2010. [Google Scholar]
- ASTM. C31/C31M-12. Standard Practice for Making and Curing Concrete Test Specimens in the Field; ASTM International: West Conshohocken, PA, USA, 2012. [Google Scholar]
- ASTM. C143/C143M-20. Standard Test Method for Slump of Hydraulic-Cement Concrete; ASTM International: West Conshohocken, PA, USA, 2020. [Google Scholar]
- Yap, S.P.; Khaw, K.R.; Alengaram, U.J.; Jumaat, M.Z. Effect of fiber aspect ratio on the torsional behavior of steel fiber-reinforced normal weight concrete and lightweight concrete. Eng. Struct. 2015, 101, 24–33. [Google Scholar] [CrossRef]
- Han, J.; Zhao, M.; Chen, J.; Lan, X. Effects of steel fiber length and coarse aggregate maximum size on mechanical properties of steel fiber reinforced concrete. Constr. Build. Mater. 2019, 209, 577–591. [Google Scholar] [CrossRef]
- Zhao, S.; Qian, X.; Chen, J.; He, R. Experimental study on the effect of coarse aggregate on the performance of steel fiber reinforced concrete. China Concr. Cem. Prod. 2006, 5, 45–48. [Google Scholar]
- Banthia, N.; Sappakittipakorn, M. Toughness enhancement in steel fiber reinforced concrete through fiber hybridization. Cem. Concr. Res. 2007, 37, 1366–1372. [Google Scholar] [CrossRef]
- Al Rikabi, F.T.; Sargand, S.M.; Khoury, I.; Hussein, H.H. Material properties of synthetic fiber–reinforced concrete under freeze-thaw conditions. J. Mater. Civ. Eng. 2018, 30, 04018090. [Google Scholar] [CrossRef]
- Al Rikabi, F.T.; Hussein, H.H.; Khoury, I. Experimental study on shear strength of synthetic fiber reinforced high strength concrete containing slag aggregate. In Structures Congress 2019: Buildings and Natural Disasters; American Society of Civil Engineers: Reston, VA, USA, 2019; pp. 179–187. [Google Scholar]
- Al Rikabi, F.T.; Sargand, S.M.; Kurdziel, J.; Hussein, H.H. Experimental investigation of thin-wall synthetic fiber-reinforced concrete pipes. ACI Struct. J. 2018, 115, 1671–1681. [Google Scholar] [CrossRef]
- Choi, O.C.; Lee, C. Flexural performance of ring-type steel fiber-reinforced concrete. Cem. Concr. Res. 2003, 33, 841–849. [Google Scholar] [CrossRef]
- Choi, Y.; Yuan, R.L. Experimental relationship between splitting tensile strength and compressive strength of GFRC and PFRC. Cem. Concr. Res. 2006, 35, 1587–1591. [Google Scholar] [CrossRef]
- Ramadoss, P.; Nagamani, K. Investigation on the tensile strength of high- performance fiber reinforced concrete using statistical methods. Comput. Concr. 2006, 3, 389–400. [Google Scholar] [CrossRef]
- Nataraja, M.C.; Dhang, N.; Gupta, A.P. Splitting tensile strength of SFRC. Indian Concr. J. 2001, 75, 287–290. [Google Scholar]
- ACI Committee 318. Building Code Requirements for Structural Concrete and Commentary; American Concrete Institute: Farmington Hills, MI, USA, 1999. [Google Scholar]
- CEB-FIP Model Code for Concrete Structures. Evaluation of the Time Dependent Behavior of Concrete. Bulletin Information No. 199; Comite Europe du Beton/Federation Internationale de Precontrainte: Lausanne, Switzerland, 1991. [Google Scholar]
- Xu, B.W.; Shi, H.S. Correlations among mechanical properties of steel fiber reinforced concrete. Con. Build. Mat. 2009, 23, 3468–3474. [Google Scholar] [CrossRef]
- Perumal, R. Correlation of compressive strength and other engineering properties of high-performance steel fiber-reinforced concrete. J. Mater. Civ. Eng. 2015, 27, 1–8. [Google Scholar] [CrossRef]
- Ahmad, S.H.; Shah, S.P. Structural properties of high strength concrete and its applications for precast prestressed concrete. PCI J. 1985, 30, 97–123. [Google Scholar]
- Wang, Z.L.; Wu, J.; Wang, J.G. Experimental and numerical analysis on effect of fibre aspect ratio on mechanical properties of SRFC. Constr. Build. Mater. 2010, 24, 559–565. [Google Scholar] [CrossRef]
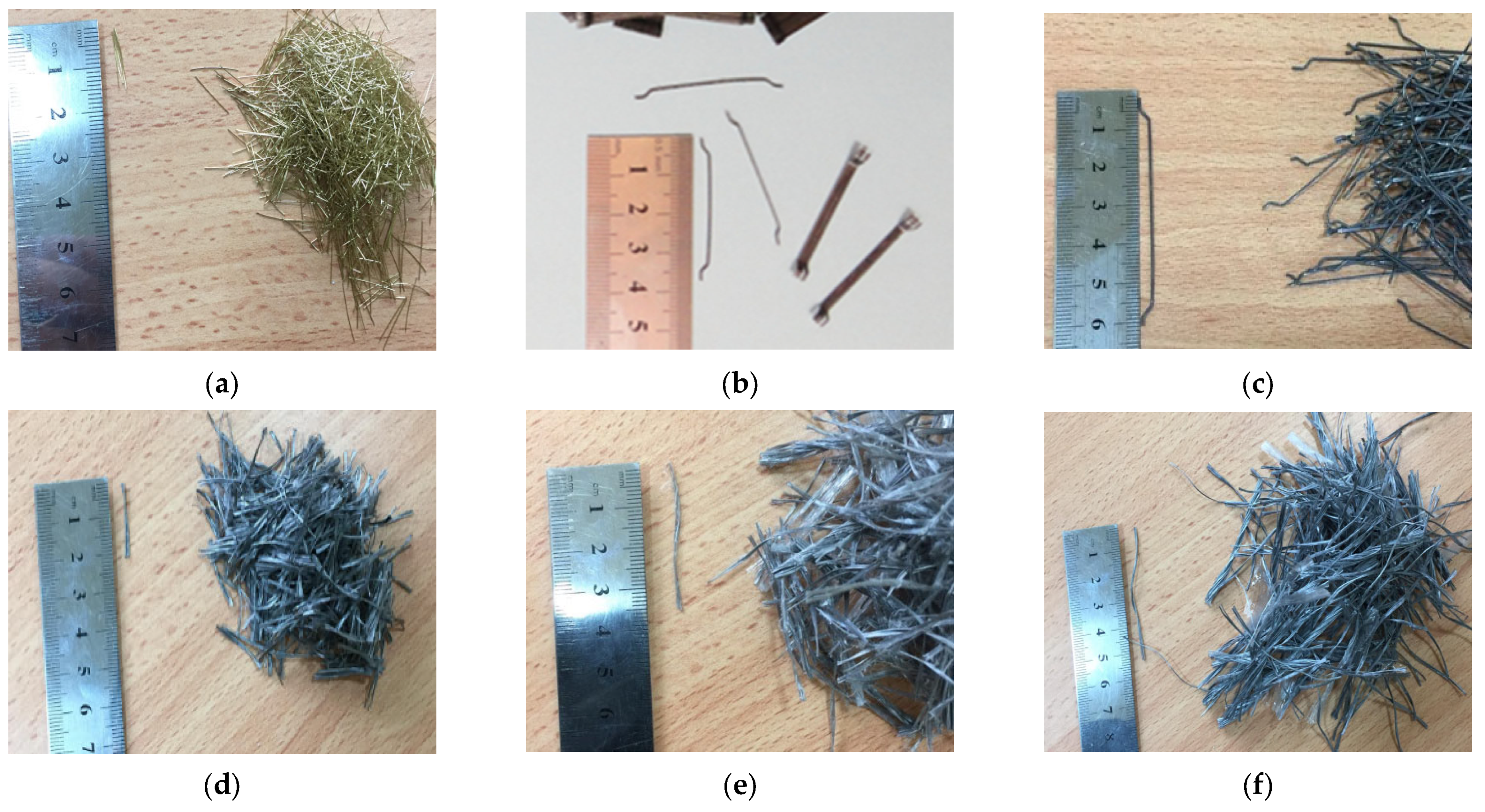



| Constituent | Chemical Composition | Cement (Type I) % by Weight ** | ASTM C150-04a [29] |
|---|---|---|---|
| Aluminum oxide | Al2O3 | 5.45 | − |
| Iron oxide | Fe2O3 | 3.41 | − |
| Magnesia | MgO | 3.7 | 6.0 * |
| Sulfate | SO3 | 2.25 | 3.0 * |
| Loss on ignition | L.O.I | 1.68 | 3.0 * |
| Insoluble residue | I.R | 0.42 | 0.75 * |
| Tricalcium aluminates | C3A | 9.85 | − |
| Tricalcium silicate | C3S | 40.43 | − |
| Diacalcium silicate | C2S | 28.1 | − |
| Tricalcium alumina ferrite | C4AF | 8.12 | − |
| Physical Properties | ASTM C150-04a [29] | Test Results *** |
|---|---|---|
| Finesses, specific surface (m2/kg) | ||
| Turbidimeter test | 160 ** | 190 |
| Air permeability | 280 ** | 310 |
| Soundness using autoclave method | 0.8% * | 0.12% |
| Setting time at which Vicat’s instrument was used | ||
| Initial (min) | 45 ** | 120 |
| Final (min) | 375 * | 280 |
| Compressive strength for the cement paste cube | ||
| 3 days (MPa) | 12 ** | 16 |
| 7 days (MPa) | 19 ** | 25 |
| Sieve Size (mm) | Cumulative Passing % * | % Passing of the Overall Limit of ASTM C33-03 [30] |
|---|---|---|
| 9.5 | 100 | 100–100 |
| 4.75 | 97 | 95–100 |
| 2.36 | 92 | 80–100 |
| 1.18 | 72 | 50–85 |
| 0.6 | 41 | 25–60 |
| 0.3 | 14 | 5–30 |
| 0.15 | 4 | 0–10 |
| Aggregate ID | Sieve Size (mm) | Cumulative Passing % * | % Passing of the Overall Limit of ASTM C33-03 [30] |
|---|---|---|---|
| G10 | 9.5 | 96 | 85–100 |
| 4.75 | 25 | 10–30 | |
| 2.36 | 8 | 0–10 | |
| 1.18 | 0 | 0–5 | |
| G19 | 19 | 98 | 90–100 |
| 9.5 | 51 | 20–55 | |
| 4.75 | 4 | 0–10 | |
| 2.36 | 0 | 0–5 | |
| G38 | 37.5 | 96 | 95–100 |
| 19 | 45 | 35–70 | |
| 9.5 | 21 | 10–30 | |
| 4.75 | 3 | 0–5 |
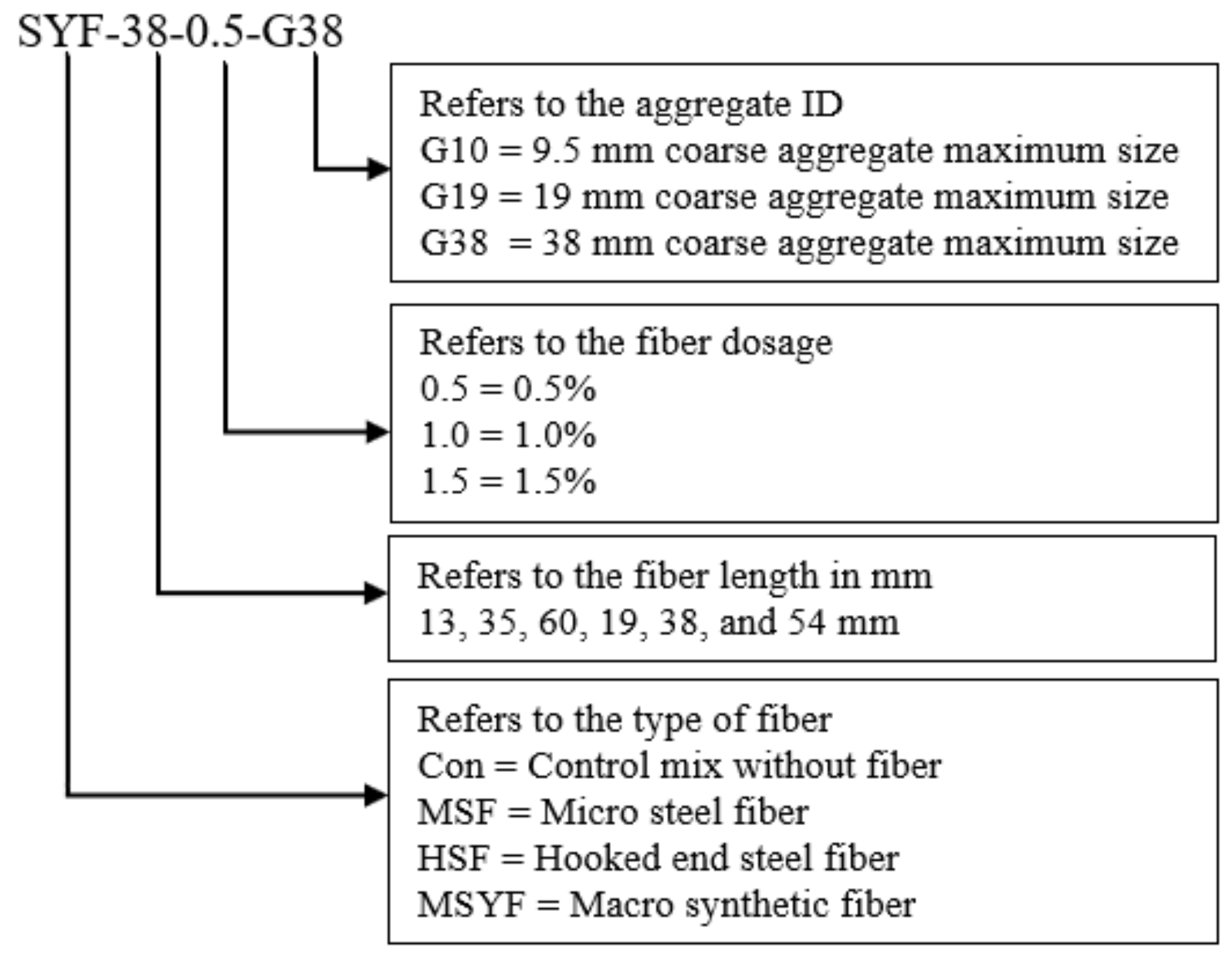
| Fiber ID | Fiber Type | Material | Length (lf) (mm) | Diameter (df) (mm) | Aspect Ratio (lf/df) | Tensile Strength MPa |
|---|---|---|---|---|---|---|
| MSF-13 | Micro steel fiber | Steel | 13 | 0.2 | 65 | >2100 |
| HSF-35 | Hooked end steel fiber | Steel | 35 | 0.55 | 64 | 900–2200 |
| HSF-60 | Hooked end steel fiber | Steel | 60 | 0.75 | 80 | 900–2200 |
| SYF-19 | Macro synthetic fiber | Copolymer/Polypropylene | 19 | 0.34 | 56 | 570–660 |
| SYF-38 | Macro synthetic fiber | Copolymer/Polypropylene | 38 | 0.34 | 112 | 570–660 |
| SYF-54 | Macro synthetic fiber | Copolymer/Polypropylene | 54 | 0.34 | 168 | 570–660 |
| Constituents | Mix Number | ||
|---|---|---|---|
| Mix 1 | Mix 2 | Mix 3 | |
| Cement (Kg/m3) | 450 | 450 | 450 |
| Water (Kg/m3) | 203 | 203 | 203 |
| Water/cement ratio (w/c) | 0.45 | 0.45 | 0.45 |
| Sand (Kg/m3) | 723 | 723 | 723 |
| Coarse aggregate (Kg/m3) | 1010 | 1010 | 1010 |
| CAMZ (mm) | 9.5 | 19 | 37.5 |
| Aggregate ID | G10 | G19 | G38 |
| Fiber content (%) by volume | 0/0.5/1.0/1.5 | 0/0.5/1.0/1.5 | 0/0.5/1.0/1.5 |
| Mix with CAMZ of 9.5 mm (G10) | Mix with CAMZ of 19 mm (G19) | Mix with CAMZ of 37.5 mm (G38) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Mix ID | Fiber ID | Dosage | lf/CAMZ | Mix ID | Fiber ID | Dosage | lf/CAMZ | Mix ID | Fiber ID | Dosage | lf/CAMZ |
| Con-G10 | − | 0.0% | − | Con-G19 | − | 0.0% | − | Con-G38 | − | 0.0% | − |
| MSF-13-0.5-G10 | MSF-13 | 0.5% | 1.37 | MSF-13-0.5-G19 | MSF-13 | 0.5% | 0.68 | MSF-13-0.5-G38 | MSF-13 | 0.5% | 0.35 |
| MSF-13-1.0-G10 | MSF-13 | 1.0% | 1.37 | MSF-13-1.0-G19 | MSF-13 | 1.0% | 0.68 | MSF-13-1.0-G38 | MSF-13 | 1.0% | 0.35 |
| MSF-13-1.5-G10 | MSF-13 | 1.5% | 1.37 | MSF-13-1.5-G19 | MSF-13 | 1.5% | 0.68 | MSF-13-1.5-G38 | MSF-13 | 1.5% | 0.35 |
| HSF-35-0.5-G10 | HSF-35 | 0.5% | 3.68 | HSF-35-0.5-G19 | HSF-35 | 0.5% | 1.84 | HSF-35-0.5-G38 | HSF-35 | 0.5% | 0.93 |
| HSF-35-1.0-G10 | HSF-35 | 1.0% | 3.68 | HSF-35-1.0-G19 | HSF-35 | 1.0% | 1.84 | HSF-35-1.0-G38 | HSF-35 | 1.0% | 0.93 |
| HSF-35-1.5-G10 | HSF-35 | 1.5% | 3.68 | HSF-35-1.5-G19 | HSF-35 | 1.5% | 1.84 | HSF-35-1.5-G38 | HSF-35 | 1.5% | 0.93 |
| HSF-60-0.5-G10 | HSF-60 | 0.5% | 6.32 | HSF-60-0.5-G19 | HSF-60 | 0.5% | 3.16 | HSF-60-0.5-G38 | HSF-60 | 0.5% | 1.60 |
| HSF-60-1.0-G10 | HSF-60 | 1.0% | 6.32 | HSF-60-1.0-G19 | HSF-60 | 1.0% | 3.16 | HSF-60-1.0-G38 | HSF-60 | 1.0% | 1.60 |
| HSF-60-1.5-G10 | HSF-60 | 1.5% | 6.32 | HSF-60-1.5-G19 | HSF-60 | 1.5% | 3.16 | HSF-60-1.5-G38 | HSF-60 | 1.5% | 1.60 |
| SYF-19-0.5-G10 | SYF-19 | 0.5% | 2.00 | SYF-19-0.5-G19 | SYF-19 | 0.5% | 1.00 | SYF-19-0.5-G38 | SYF-19 | 0.5% | 0.51 |
| SYF-19-1.0-G10 | SYF-19 | 1.0% | 2.00 | SYF-19-1.0-G19 | SYF-19 | 1.0% | 1.00 | SYF-19-1.0-G38 | SYF-19 | 1.0% | 0.51 |
| SYF-19-1.5-G10 | SYF-19 | 1.5% | 2.00 | SYF-19-1.5-G19 | SYF-19 | 1.5% | 1.00 | SYF-19-1.5-G38 | SYF-19 | 1.5% | 0.51 |
| SYF-38-0.5-G10 | SYF-38 | 0.5% | 4.00 | SYF-38-0.5-G19 | SYF-38 | 0.5% | 2.00 | SYF-38-0.5-G38 | SYF-38 | 0.5% | 1.01 |
| SYF-38-1.0-G10 | SYF-38 | 1.0% | 4.00 | SYF-38-1.0-G19 | SYF-38 | 1.0% | 2.00 | SYF-38-1.0-G38 | SYF-38 | 1.0% | 1.01 |
| SYF-38-1.5-G10 | SYF-38 | 1.5% | 4.00 | SYF-38-1.5-G19 | SYF-38 | 1.5% | 2.00 | SYF-38-1.5-G38 | SYF-38 | 1.5% | 1.01 |
| SYF-54-0.5-G10 | SYF-54 | 0.5% | 5.68 | SYF-54-0.5-G19 | SYF-54 | 0.5% | 2.84 | SYF-54-0.5-G38 | SYF-54 | 0.5% | 1.44 |
| SYF-54-1.0-G10 | SYF-54 | 1.0% | 5.68 | SYF-54-1.0-G19 | SYF-54 | 1.0% | 2.84 | SYF-54-1.0-G38 | SYF-54 | 1.0% | 1.44 |
| SYF-54-1.5-G10 | SYF-54 | 1.5% | 5.68 | SYF-54-1.5-G19 | SYF-54 | 1.5% | 2.84 | SYF-54-1.5-G38 | SYF-54 | 1.5% | 1.44 |
| Specimen ID | Brittleness Ratio | Diff. % | Specimen ID | Brittleness Ratio | Diff. % | Specimen ID | Brittleness Ratio | Diff. % |
|---|---|---|---|---|---|---|---|---|
| Con-G10 | 9.50 | 0.0% | Con-G19 | 9.14 | 0.0% | Con-G38 | 8.78 | 0.0% |
| MSF-13-0.5-G10 | 7.49 | −21.2% | MSF-13-0.5-G19 | 7.67 | −16.0% | MSF-13-0.5-G38 | 8.22 | −6.4% |
| MSF-13-1.0-G10 | 7.67 | −19.3% | MSF-13-1.0-G19 | 7.46 | −18.3% | MSF-13-1.0-G38 | 7.29 | −16.9% |
| MSF-13-1.5-G10 | 7.68 | −19.2% | MSF-13-1.5-G19 | 7.67 | −16.1% | MSF-13-1.5-G38 | 7.45 | −15.1% |
| HSF-35-0.5-G10 | 10.71 | 12.7% | HSF-35-0.5-G19 | 8.83 | −3.4% | HSF-35-0.5-G38 | 7.93 | −9.6% |
| HSF-35-1.0-G10 | 7.90 | −16.9% | HSF-35-1.0-G19 | 6.69 | −26.8% | HSF-35-1.0-G38 | 5.95 | −32.2% |
| HSF-35-1.5-G10 | 7.04 | −26.0% | HSF-35-1.5-G19 | 5.70 | −37.6% | HSF-35-1.5-G38 | 6.43 | −26.7% |
| HSF-60-0.5-G10 | 9.96 | 4.8% | HSF-60-0.5-G19 | 8.46 | −7.4% | HSF-60-0.5-G38 | 7.75 | −11.7% |
| HSF-60-1.0-G10 | 8.66 | −8.9% | HSF-60-1.0-G19 | 6.53 | −28.5% | HSF-60-1.0-G38 | 5.44 | −38.0% |
| HSF-60-1.5-G10 | 6.92 | −27.2% | HSF-60-1.5-G19 | 5.81 | −36.4% | HSF-60-1.5-G38 | 4.62 | −47.4% |
| SYF-19-0.5-G10 | 7.10 | −25.3% | SYF-19-0.5-G19 | 7.24 | −20.7% | SYF-19-0.5-G38 | 6.81 | −22.4% |
| SYF-19-1.0-G10 | 6.60 | −30.5% | SYF-19-1.0-G19 | 6.57 | −28.1% | SYF-19-1.0-G38 | 6.25 | −28.8% |
| SYF-19-1.5-G10 | 6.30 | −33.8% | SYF-19-1.5-G19 | 6.56 | −28.2% | SYF-19-1.5-G38 | 6.03 | −31.3% |
| SYF-38-0.5-G10 | 6.90 | −27.4% | SYF-38-0.5-G19 | 6.72 | −26.4% | SYF-38-0.5-G38 | 6.12 | −30.2% |
| SYF-38-1.0-G10 | 6.23 | −34.4% | SYF-38-1.0-G19 | 5.97 | −34.7% | SYF-38-1.0-G38 | 5.22 | −40.5% |
| SYF-38-1.5-G10 | 6.10 | −35.8% | SYF-38-1.5-G19 | 5.47 | −40.1% | SYF-38-1.5-G38 | 5.05 | −42.4% |
| SYF-54-0.5-G10 | 6.04 | −36.4% | SYF-54-0.5-G19 | 6.53 | −28.5% | SYF-54-0.5-G38 | 5.78 | −34.1% |
| SYF-54-1.0-G10 | 6.16 | −35.2% | SYF-54-1.0-G19 | 5.42 | −40.7% | SYF-54-1.0-G38 | 4.72 | −46.2% |
| SYF-54-1.5-G10 | 6.01 | −36.8% | SYF-54-1.5-G19 | 5.48 | −40.0% | SYF-54-1.5-G38 | 4.82 | −45.1% |
| Compressive Strength and Tensile Strength Relations | |
| Source | Equation |
| ACI 318 [47] | |
| CEB-FIP [48] | |
| Choi and Yuan [44] | |
| Xu and Shi [49] | |
| Perumal [50] | |
| Flexural Strength and Tensile Strength Relations | |
| Source | Equation |
| Xu and Shi [49] | |
| Perumal [50] | |
| Compressive Strength and Flexural Strength Relations | |
| Source | Equation |
| ACI 318 [47] | |
| Ahmed and Shah [51] | |
| Xu and Shi [49] | |
| Perumal [50] | |
| Fiber Type | Equation |
|---|---|
| MSF-13 | |
| HSF-35 | |
| HSF-60 | |
| SYF-19 | |
| SYF-38 | |
| SYF-54 |
| Fiber Type | Equation |
|---|---|
| MSF-13 | |
| HSF-35 | |
| HSF-60 | |
| SYF-19 | |
| SYF-38 | |
| SYF-54 |
| Fiber Type | Equation |
|---|---|
| MSF-13 | |
| HSF-35 | |
| HSF-60 | |
| SYF-19 | |
| SYF-38 | |
| SYF-54 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Baghdadi, H.M.; Al-Merib, F.H.; Ibrahim, A.A.; Hassan, R.F.; Hussein, H.H. Effects of Coarse Aggregate Maximum Size on Synthetic/Steel Fiber Reinforced Concrete Performance with Different Fiber Parameters. Buildings 2021, 11, 158. https://doi.org/10.3390/buildings11040158
Al-Baghdadi HM, Al-Merib FH, Ibrahim AA, Hassan RF, Hussein HH. Effects of Coarse Aggregate Maximum Size on Synthetic/Steel Fiber Reinforced Concrete Performance with Different Fiber Parameters. Buildings. 2021; 11(4):158. https://doi.org/10.3390/buildings11040158
Chicago/Turabian StyleAl-Baghdadi, Haider M., Faiz H. Al-Merib, Ayoob A. Ibrahim, Rafea F. Hassan, and Husam H. Hussein. 2021. "Effects of Coarse Aggregate Maximum Size on Synthetic/Steel Fiber Reinforced Concrete Performance with Different Fiber Parameters" Buildings 11, no. 4: 158. https://doi.org/10.3390/buildings11040158
APA StyleAl-Baghdadi, H. M., Al-Merib, F. H., Ibrahim, A. A., Hassan, R. F., & Hussein, H. H. (2021). Effects of Coarse Aggregate Maximum Size on Synthetic/Steel Fiber Reinforced Concrete Performance with Different Fiber Parameters. Buildings, 11(4), 158. https://doi.org/10.3390/buildings11040158








