Analytical Pushover Curves for X-Concentric Braced Steel Frames
Abstract
:1. Introduction
2. Trilinear Proposed Curves
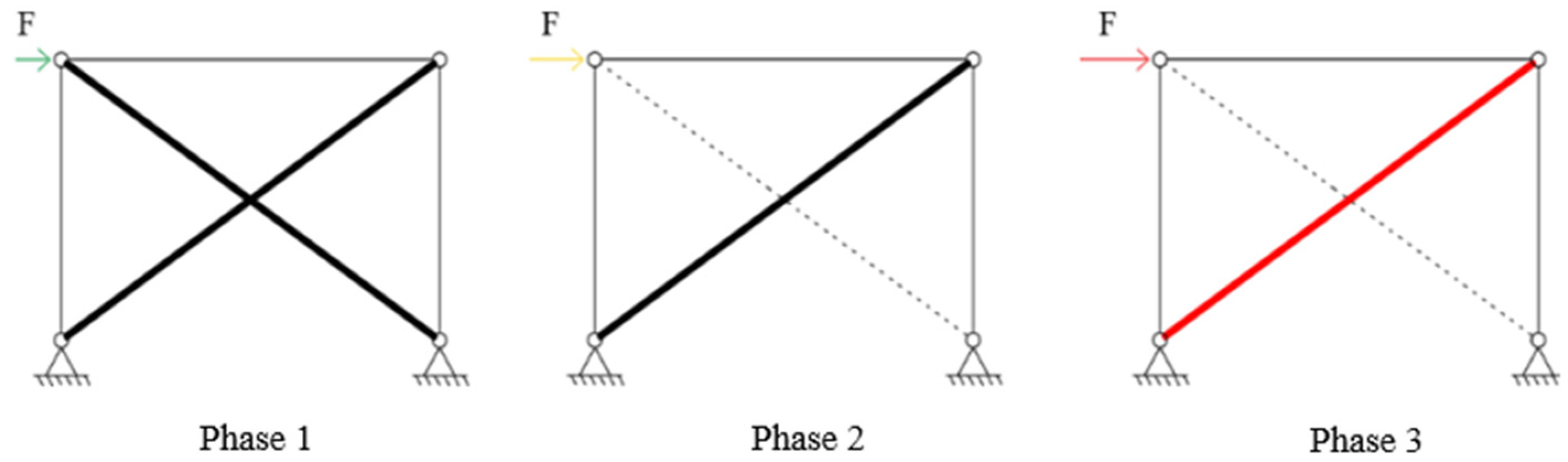
- In phase 1, the X-CBF has a high lateral stiffness ( ), everything remains elastic, and there are no buckling phenomena;
- In phase 2, the structure loses about half of its lateral stiffness because of the buckling of the compressed diagonal; by neglecting the contribution of this one, the lateral stiffness becomes ;
- In phase 3, the system loses all its stiffness () because of the yielding of the diagonal in tension.
- : shear base force;
- : top displacement;
- : Young’s modulus;
- : diagonal cross-section;
- : beam–column angle;
- : diagonal length.
- : reduction coefficient for stability problems;
- : normalized slenderness of the diagonal;
- : imperfection coefficient;
- : reduction coefficient for local buckling.
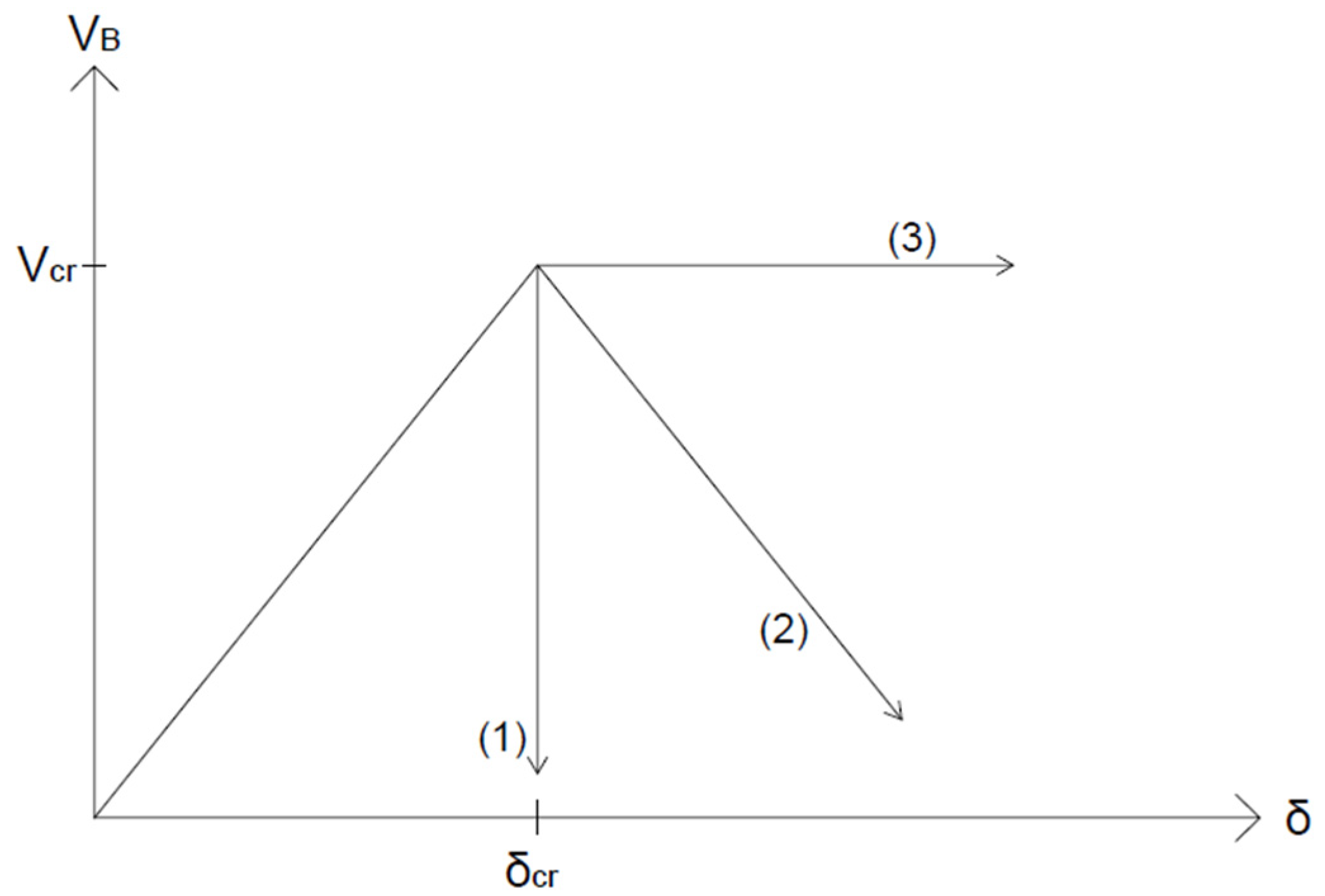
- (1)
- Instantaneous loss of load;
- (2)
- Relevant softening after the elastic phase;
- (3)
- Plastic behavior caused by relevant post-critical resources of the compressed diagonal.
- A squat diagonal () will show a behavior similar to that of curve (3);
- A slender diagonal () will show a behavior like curve (1), typical of cables, for example;
- A medium-slender diagonal () will show an intermediate behavior, like curve (2).
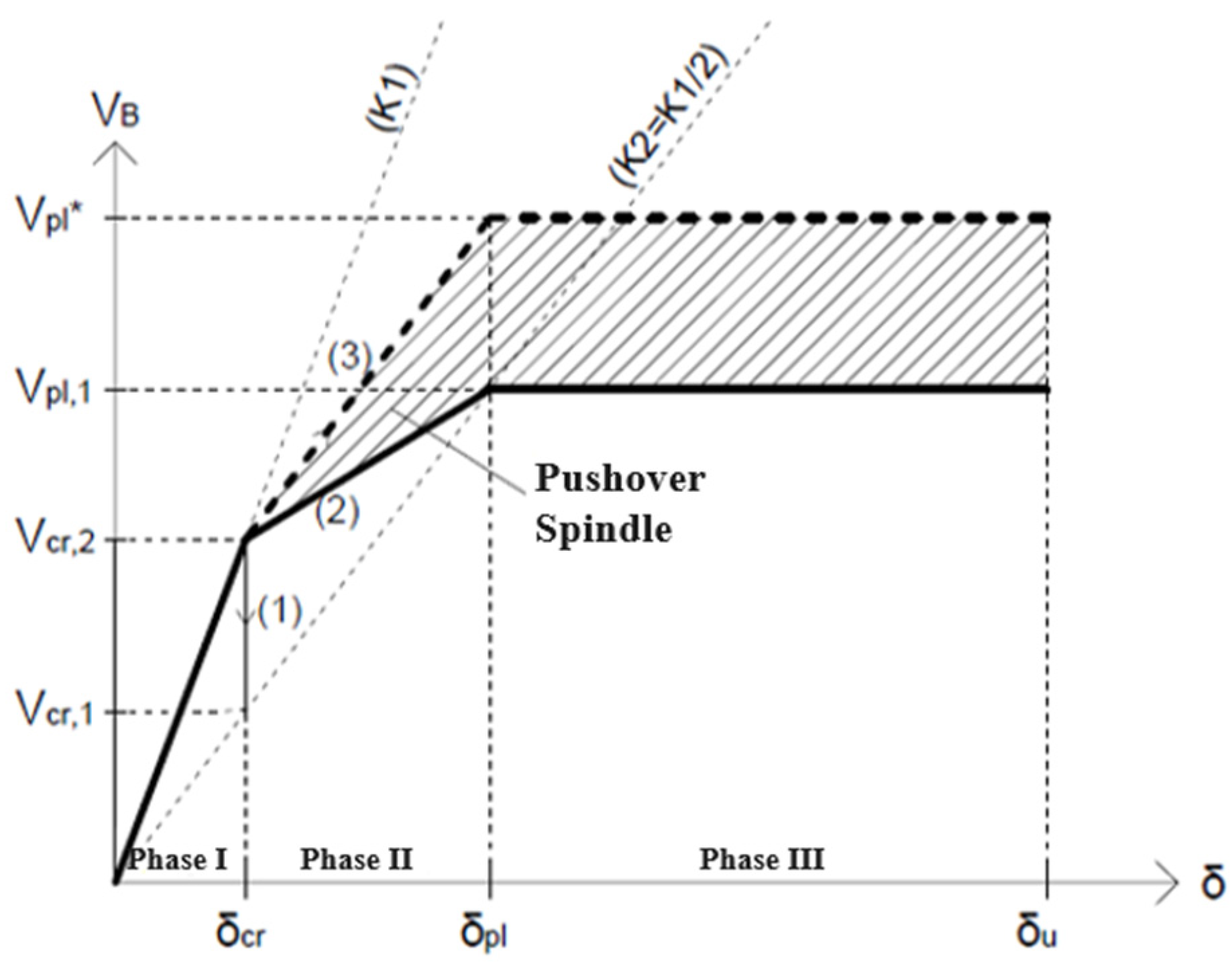
- If the load discharge is instantaneous (line (1) in Figure 4), a vertical line is followed, until the intersection with the line with stiffness in correspondence to the shear value ;
- If the compressed diagonal has a pseudo-plastic behavior, line (3) is followed, with a slope equal to =/2, reaching the third phase in correspondence to shear value and displacement;
- If the compressed diagonal has medium slenderness (), it is possible to reach the third phase with curve (2) characterized by a plastic shear lower than .
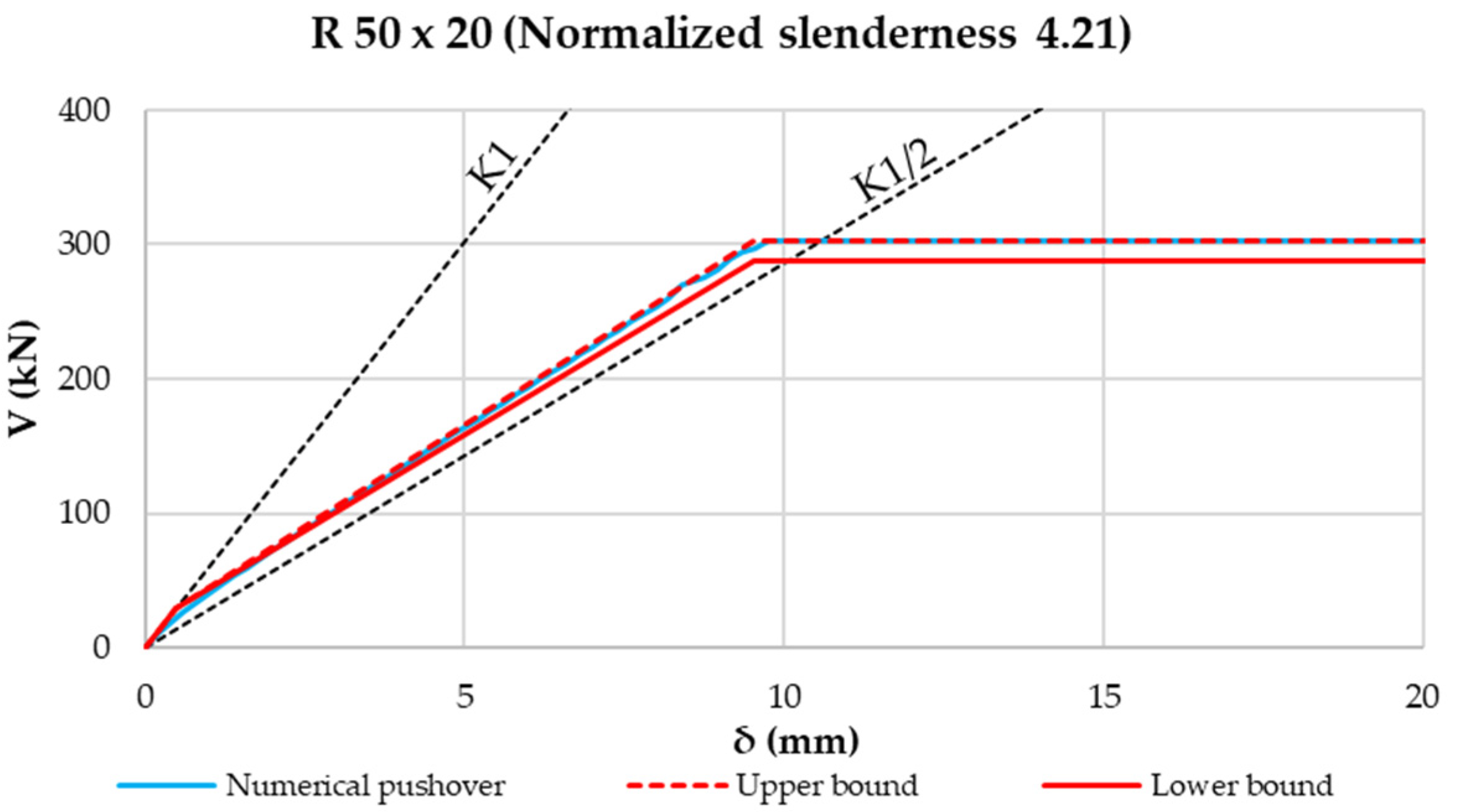
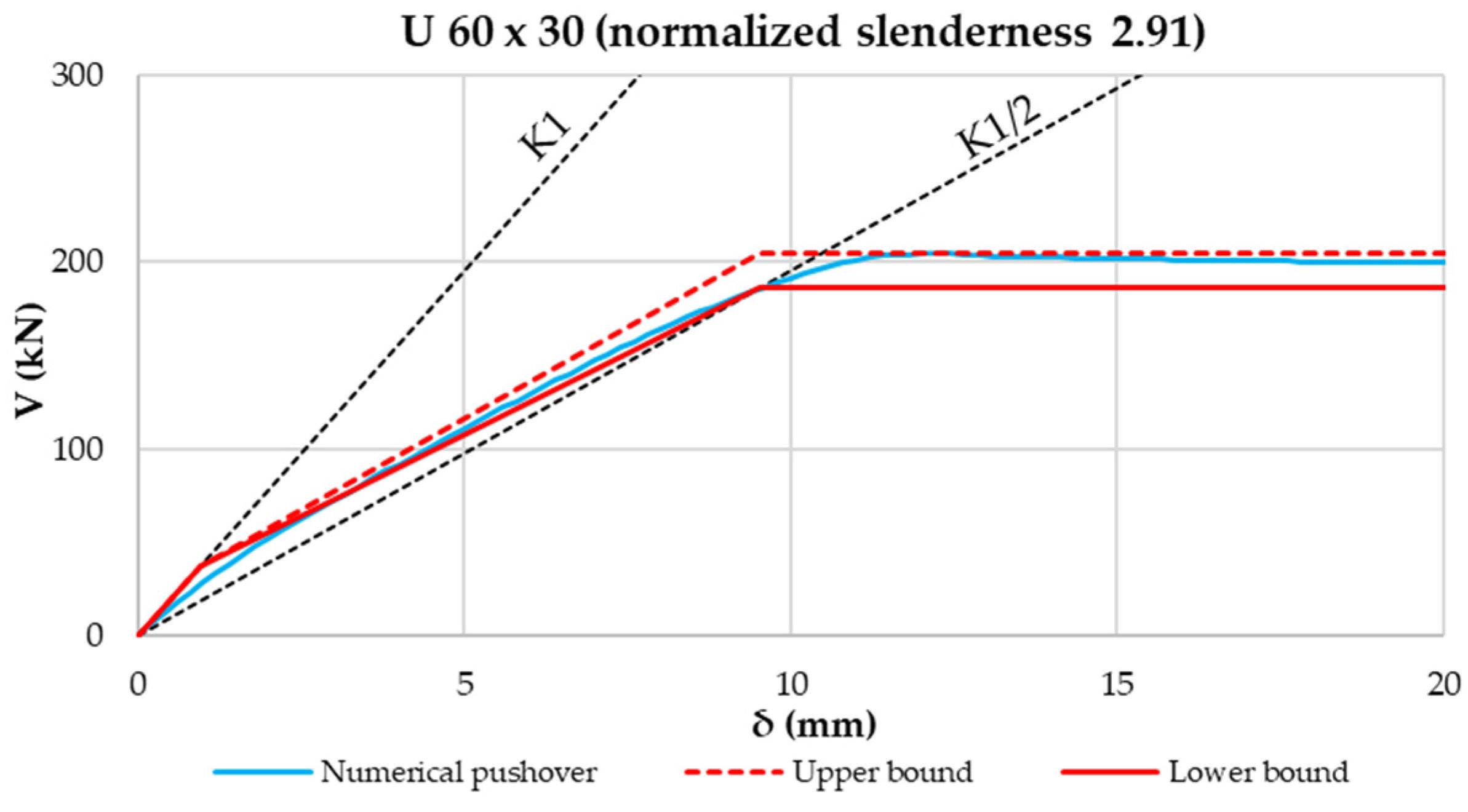
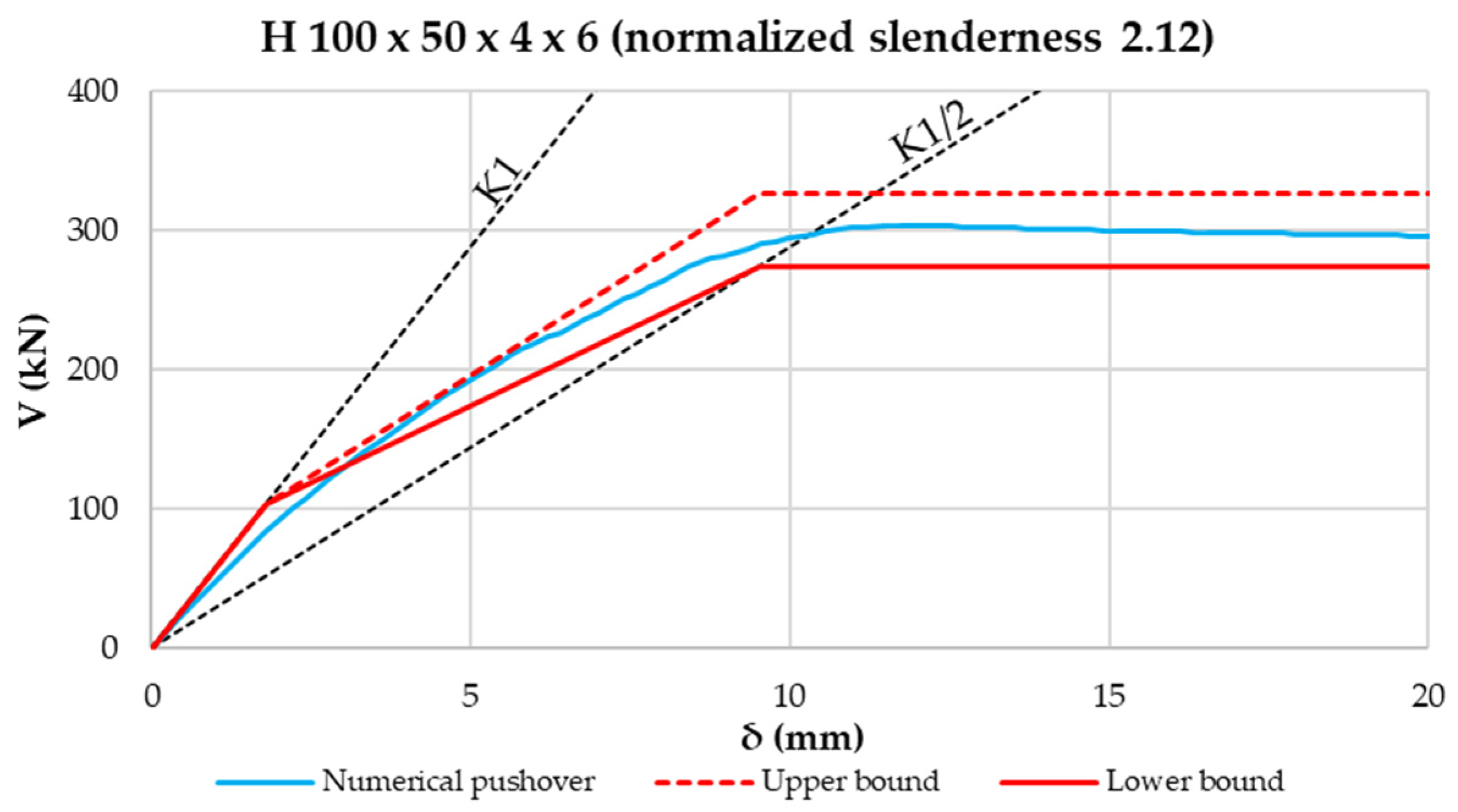
- The “Lower-bound” curve, characterized by a second phase with a stiffness less than half of , and achievable by connecting the point in correspondence to with the one with
- The “Upper-bound” curve, characterized by a higher second-phase stiffness, equal to , and with a plastic shear value equal to .
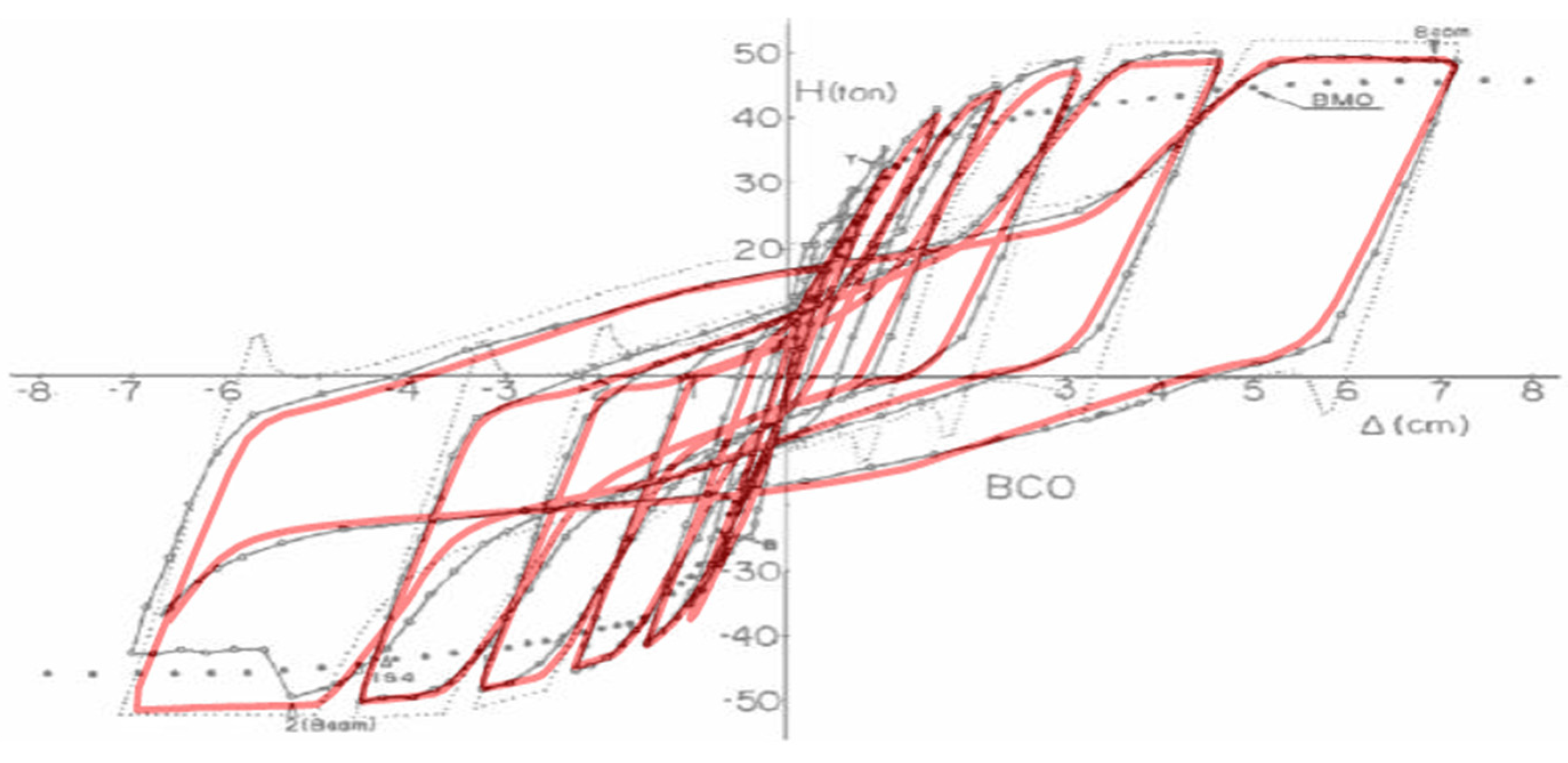
3. Numerical Validation
4. Numerical Comparisons
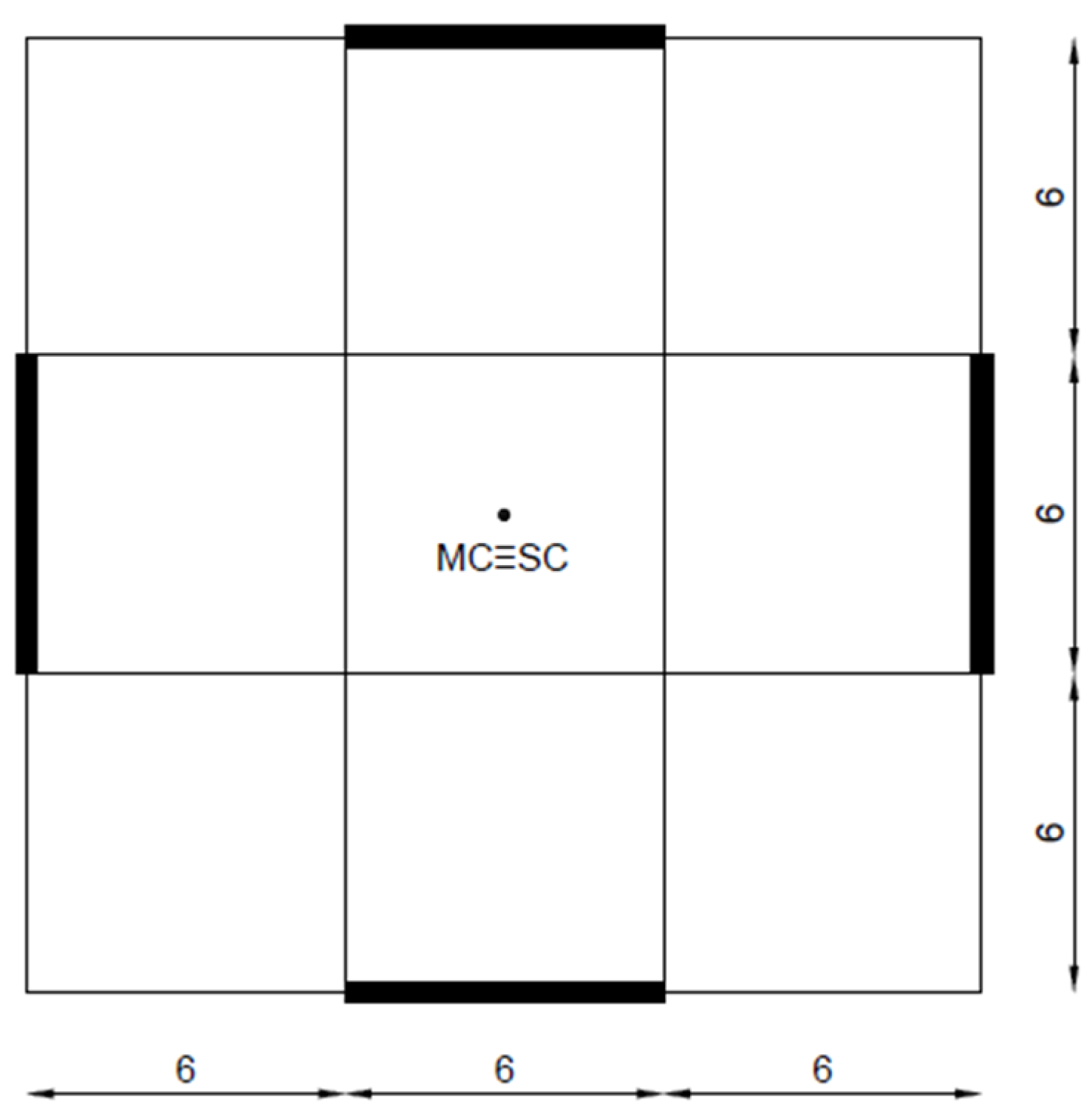
5. Application to Mono- and Multi-Storey Buildings
6. Case Studies
- Mono- or multi-storey buildings;
- Behavior factor equal to 1 or 4.
6.1. One-Floor Case Studies
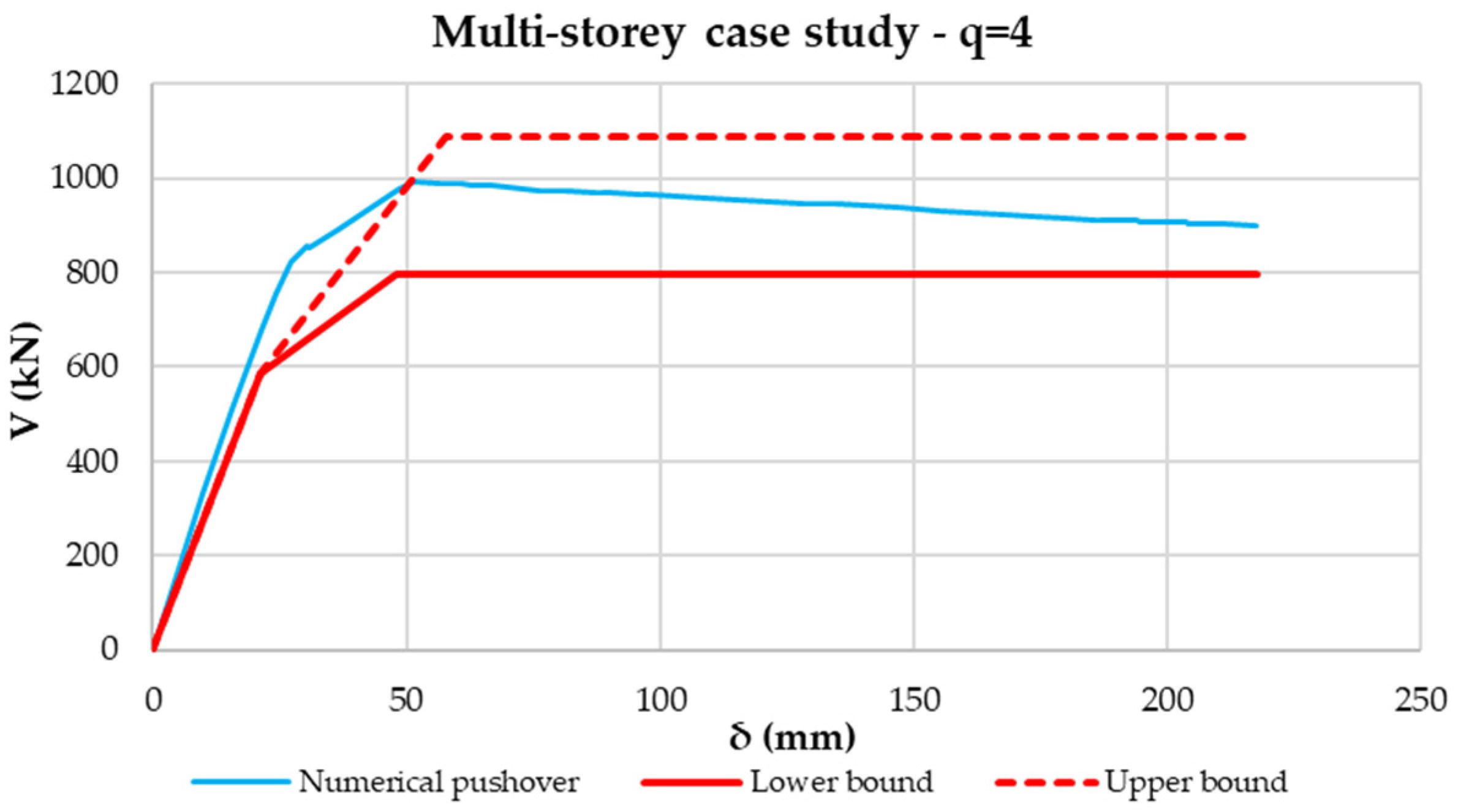
6.2. Multi-Story Case Studies
7. Conclusions
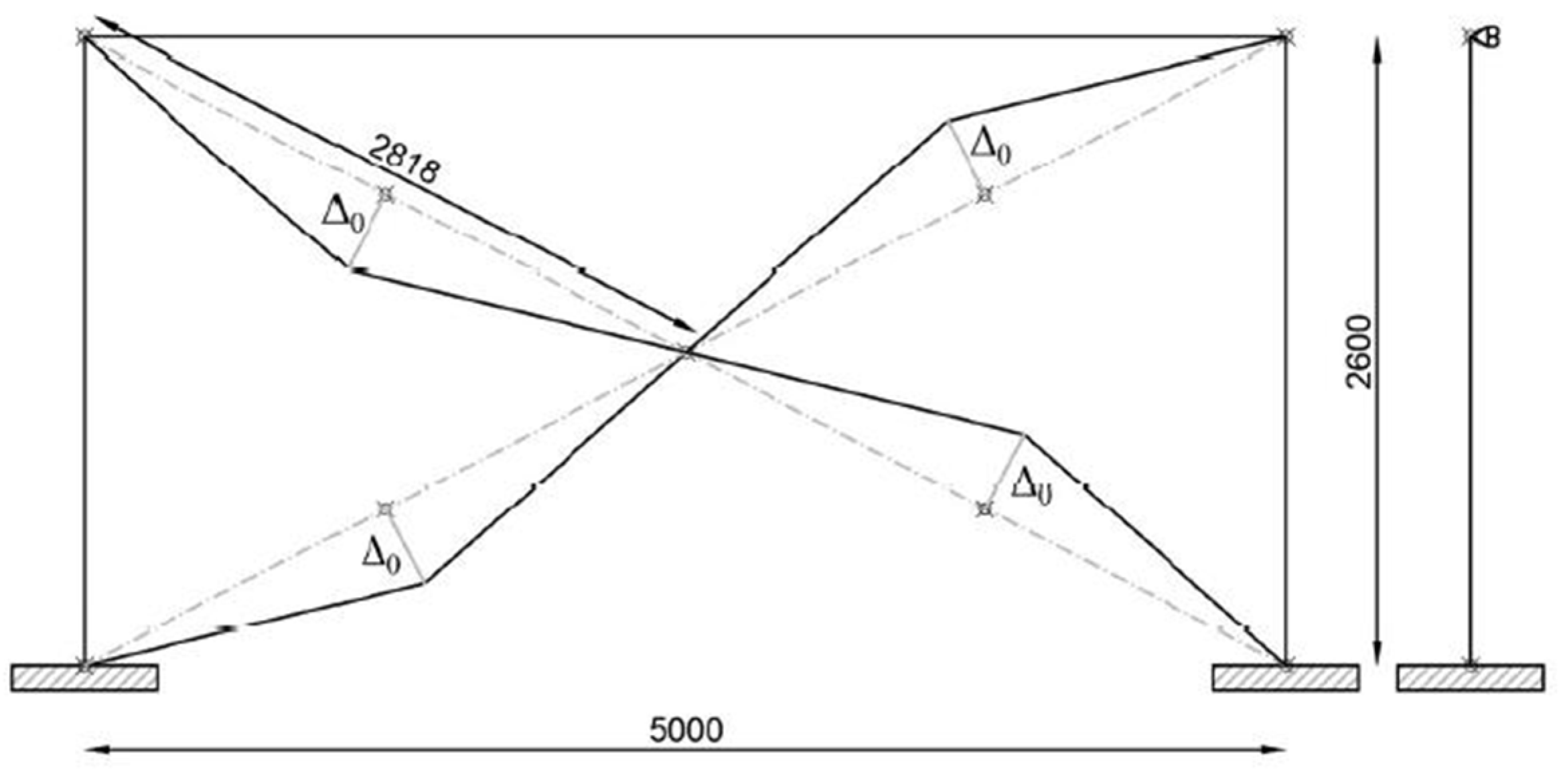
- It was first observed that the lateral stiffness of the X-CBFs in the Post-Buckling phase is not equal to half of the Pre-Buckling phase stiffness but is smaller and variable with the normalized slenderness of the diagonal.
- Taking these aspects into account, the construction of an analytical pushover “spindle” was proposed, characterized by lower- and upper-bound curves. Such limit curves form a field in which numerical pushover curves have to fall. This was proven by using a model validated through experimental tests. By varying the diagonal profiles with different normalized slenderness, it was shown that numerical curves fall always inside the proposed pushover spindle. In addition, the higher the normalized slenderness of the diagonal is, the tighter the analytical spindle is.
- The proposal was then extended to whole structures by analyzing mono- and multi-storey buildings. The numerical response of all the analyzed case studies falls back inside the spindle, which represents a strict strength domain and allows correctly evaluating the behavior of these types of structures.
- The proposed approach can be directly used in the design phase within the pushover method and allows a possible analytical control on the results obtained with the numerical model.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- British Standards Institution, European Committee for Standardization, British Standards Institution, and Standards Policy and Strategy Committee. Eurocode 8, Design of Structures for Earthquake Resistance; British Standards Institution: London, UK, 2005. [Google Scholar]
- American Institute of Steel Construction. Seismic Provisions for Structural Steel Buildings; American Institute of Steel Construction: Chicago, IL, USA, 2016. [Google Scholar]
- Goggins, J.; Salawdeh, S. Validation of nonlinear time history analysis models for single-storey concentrically braced frames using full-scale shake table tests. Earthq. Eng. Struct. Dyn. 2013, 42, 1151–1170. [Google Scholar] [CrossRef]
- Costanzo, S.; D’Aniello, M.; Landolfo, R. Proposal of design rules for ductile X-CBFS in the framework of EUROCODE 8. Earthq. Eng. Struct. Dyn. 2019, 48, 124–151. [Google Scholar] [CrossRef] [Green Version]
- Elghazouli, A.Y. Assessment of European seismic design procedures for steel framed structures. Bull. Earthq. Eng. 2010, 8, 65–89. [Google Scholar] [CrossRef]
- Costanzo, S.; Raffaele, L. Concentrically Braced Frames: European vs. North American Seismic Design Provisions. TOCIEJ Open Civ. Eng. J. 2017, 11, 453–463. [Google Scholar] [CrossRef] [Green Version]
- D’Aniello, M.; Ambrosino, G.l.; Portioli, F.; Landolfo, R. Modelling aspects of the seismic response of steel concentric braced frames. Steel Compos. Struct. 2013, 15, 539–566. [Google Scholar] [CrossRef]
- Uang, C.-M.; Bruneau, M. State-of-the-Art Review on Seismic Design of Steel Structures. J. Struct. Eng. 2018, 144, 03118002. [Google Scholar] [CrossRef]
- Canadian Standards Association; Standards Council of Canada. Design of Steel Structures; Canadian Standards Association: Toronto, ON, Canada; Standards Council of Canada: Toronto, ON, Canada, 2019. [Google Scholar]
- Marino, E.M.; Nakashima, M.; Mosalam, K.M. Comparison of European and Japanese Seismic Design of Steel Building Structures. Eng. Struct. 2005, 27, 827–840. [Google Scholar] [CrossRef]
- Amadio, C.; Bomben, L.; Noè, S. Design of X-Concentric Braced Steel Frame Systems Using an Equivalent Stiffness in a Modal Elastic Analysis. Buildings 2022, 12, 359. [Google Scholar] [CrossRef]
- Wakabayashi, M.; Matsui, C.; Minami, K.; Mitani, I. Inelastic Behavior of Full-Scale Steel Frames with and without Bracings. Bull. Disaster Prev. Res. Inst. 1974, 24, 1–23. [Google Scholar]
- Black, R.G.; Wenger, W.A.; Popov, E.P. Inelastic Buckling of Steel Struts under Cyclic load Reversals; Report No. UCB/EERC-80/40; Earthquake Engineering Research Center: Berkeley, CA, USA, 1980. [Google Scholar]
- Kanyilmaz, A. Role of Compression Diagonals in Concentrically Braced Frames in Moderate Seismicity: A Full Scale Experimental Study. J Constr. Steel Res. 2017, 133, 1–18. [Google Scholar] [CrossRef]
- Zhao, B.; Zhou, T.; Chen, Z.; Yu, J. Experimental seismic behavior of SCFRT column chevron concentrically braced frames. J Constr. Steel Res. 2017, 133, 141–155. [Google Scholar] [CrossRef]
- Zhao, Z.; Liu, H.; Liang, B. Novel numerical method for the analysis of semi-rigid jointed lattice shell structures considering plasticity. ADES Adv. Eng. Softw. 2017, 114, 208–214. [Google Scholar] [CrossRef]
- Uriz, P.; Filippou, F.C.; Mahin, S.A. Model for Cyclic Inelastic Buckling of Steel Braces. J. Struct. Eng. 2008, 134, 619–628. [Google Scholar] [CrossRef]
- Applied Technology Council, United States, Federal Emergency Management Agency, and Building Seismic Safety Council (U.S.). NEHRP Guidelines for the Seismic Rehabilitation of Buildings; Applied Technology Council, United States, Federal Emergency Management Agency, and Building Seismic Safety Council (U.S.): Washington DC, USA, 1997. [Google Scholar]
- Faggiano, B.; Formisano, A.; Vaiano, G.; Mazzolani, F.M. Numerical Study on Concentric Braced X Frames under Monotonic and Cyclic Loads. Key Eng. Mater. 2018, 763, 633–641. [Google Scholar] [CrossRef]
- I. Computers and Structures. In SAP2000: Integrated Software for Structural Analysis and Design; CSI: Berkeley, CA, USA, 2006.
- Dicleli, M.; Calik, E.E. Physical Theory Hysteretic Model for Steel Braces. J. Struct. Eng. 2008, 134, 1215. [Google Scholar] [CrossRef] [Green Version]
- Ministero delle Infrastrutture e dei Trasporti, Italia. Norme Tecniche per le Costruzioni 2018: Decreto 17 Gennaio 2018 ‘Aggiornamento delle Norme Tecniche per le Costruzioni’; Ministero delle Infrastrutture e dei Trasporti, Italia: Rome, Italy, 2018.










| Drift | Performance Level | ||
|---|---|---|---|
| S5 | S3 | S1 | |
| Transitional | 2% | 1.5% | 0.7% |
| Permanent | 2% | 0.5% | negligible |
| Geometrical Characteristics | Mechanical Characteristics | |||||||
|---|---|---|---|---|---|---|---|---|
| Element | (mm) | (mm) | (mm) | (mm) | (mm) | (MPa) | ||
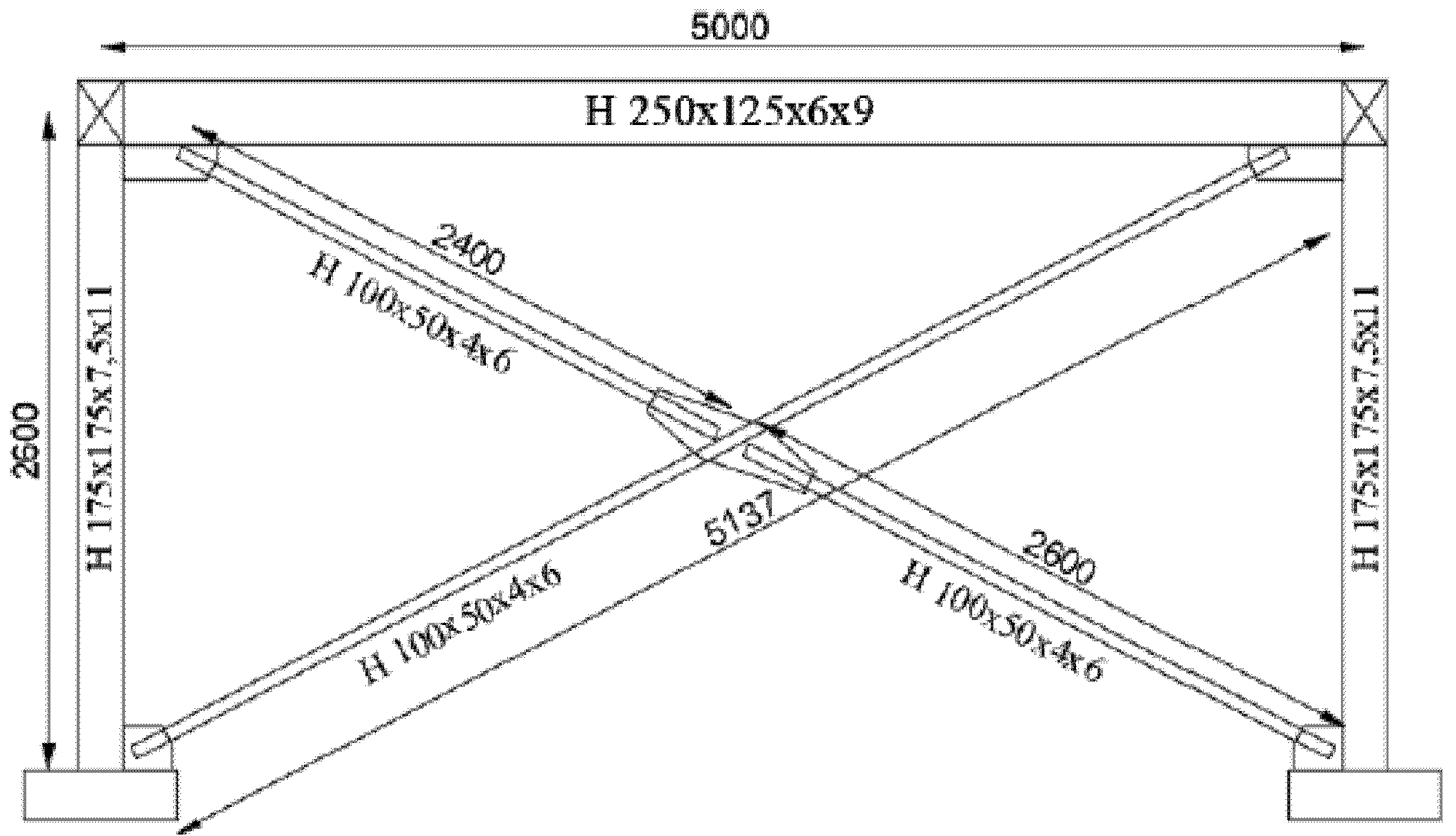
| Beam | 250 | 125 | 6 | 9 | 286 | 204,286 | 0.012 | 0.247 |
| Column | 175 | 175 | 7.5 | 11 | 266 | 204,615 | 0.0015 | 0.306 |
| Diagonal | 100 | 50 | 4 | 6 | 325 | 216,667 | 0.011 | 0.231 |
| Typology | Profile | ||
|---|---|---|---|
| Fully rectangular | R 50 × 20 | 4.21 | 1000 |
| U | U 60 × 30 | 2.91 | 646 |
| Double T | H 100 × 50 × 4 × 6 | 2.12 | 952 |
| Hollow rectangular | RHS 120 × 40 × 4 | 1.47 | 1216 |
| Hollow rectangular | RHS 100 × 50 × 4 | 1.19 | 1136 |
| Summary of Analytical Approach | |||
|---|---|---|---|
| Buckling Point | Plasticization Point | ||
| Lower-Bound Curve | Upper-Bound Curve | ||
Single X-CBF | |||
Mono-floor building | |||
Multi-storey building | |||
| 2.50 | kN/m2 | |
| 1.20 | kN/m2 | |
| 1.33 | kN/m2 |
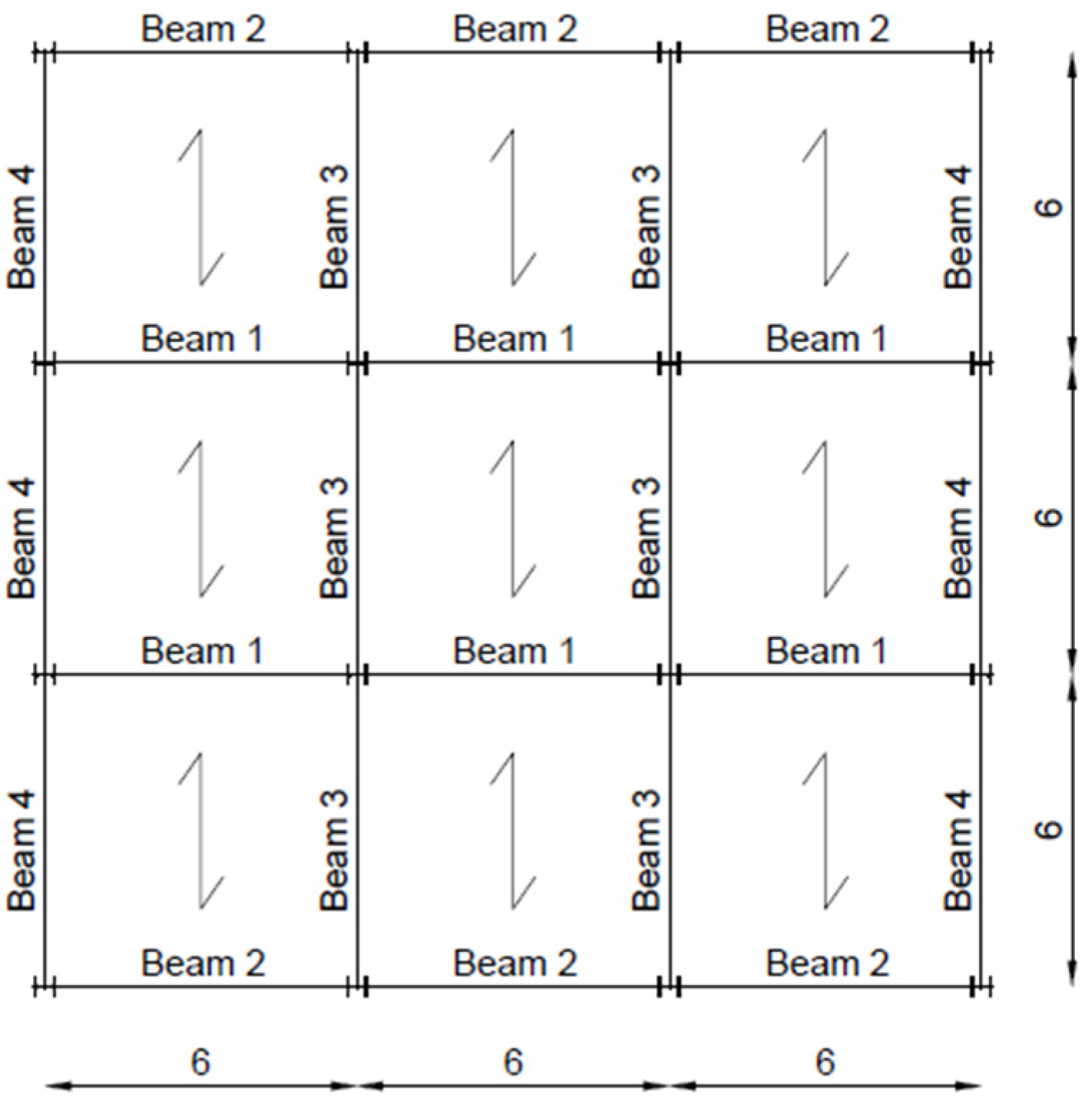
| Element | Profile |
|---|---|
| Beam 1 | IPE 400 |
| Beam 2 | IPE 330 |
| Beam 3 | IPE 300 |
| Beam 4 | IPE 240 |
| Column | HEB 200 |
| Diagonal − q = 1 | RHS 10 × 6 × 8 |
| Diagonal − q = 4 | RHS 8 × 4 × 2.5 |
| 3.00 | kN/m2 | |
| 3.00 | kN/m2 | |
| 1.20 | kN/m2 | |
| 1.33 | kN/m2 |
| Element | Profile |
|---|---|
| Beam 1 | IPE 450 |
| Beam 2 | IPE 360 |
| Beam 3 | IPE 300 |
| Beam 4 | IPE 240 |
| Column | HEB 240 |
| Diagonal Profiles − q = 1 | ||
|---|---|---|
| Level 1: RHS 300 × 50 × 8 | 5344 mm2 | |
| Level 2: RHS 250 × 50 × 8.5 | 4811 mm2 | |
| Level 3: RHS 250 × 50 × 6.5 | 3731 mm2 | |
| Level 4: RHS 180 × 40 × 4.5 | 1899 mm2 | |
| Diagonal Profiles − q = 4 | ||
|---|---|---|
| Level 1: RHS 100 × 60 × 3 | 914 mm2 | |
| Level 2: RHS 100 × 50 × 3 | 854 mm2 | |
| Level 3: RHS 80 × 40 × 3 | 674 mm2 | |
| Level 4: RHS 60 × 40 × 2 | 374 mm2 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amadio, C.; Bomben, L.; Noè, S. Analytical Pushover Curves for X-Concentric Braced Steel Frames. Buildings 2022, 12, 413. https://doi.org/10.3390/buildings12040413
Amadio C, Bomben L, Noè S. Analytical Pushover Curves for X-Concentric Braced Steel Frames. Buildings. 2022; 12(4):413. https://doi.org/10.3390/buildings12040413
Chicago/Turabian StyleAmadio, Claudio, Luca Bomben, and Salvatore Noè. 2022. "Analytical Pushover Curves for X-Concentric Braced Steel Frames" Buildings 12, no. 4: 413. https://doi.org/10.3390/buildings12040413







