Force Transfer Mechanism and Component-Based Model of Cast-Steel-Stiffened Circular-Tube-Column Frames for Progressive Collapse Analysis
Abstract
:1. Introduction
2. Prototype Frame for Progressive Collapse Analysis
3. Detailed Solid-Element Model and the Force Transfer Mechanism of CSS Joint Frame in Progressive Collapse
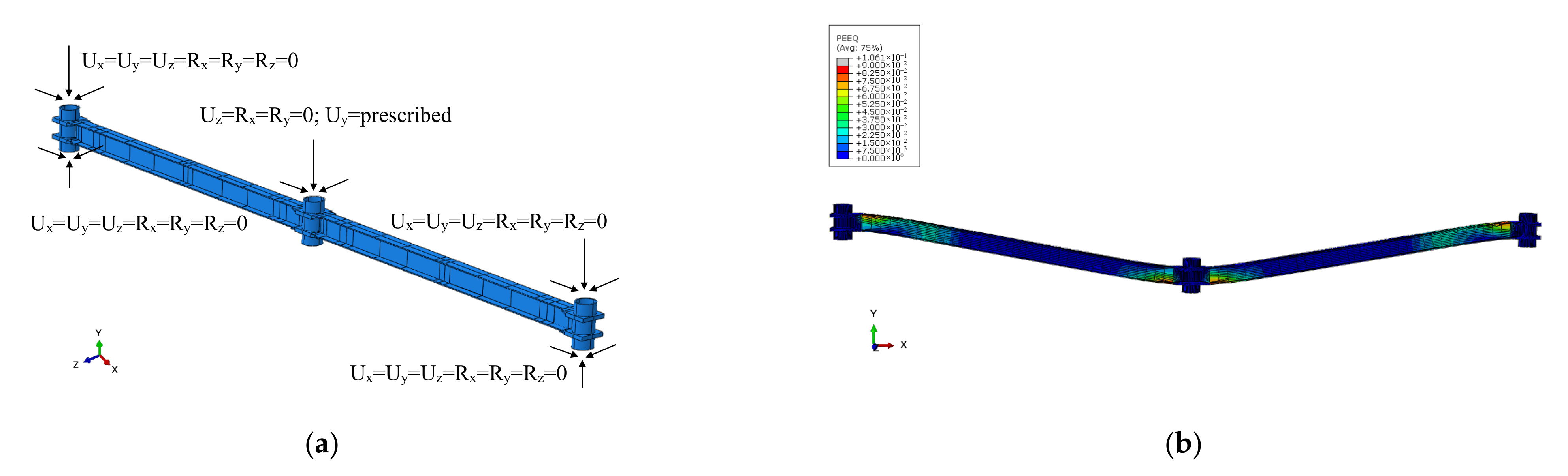
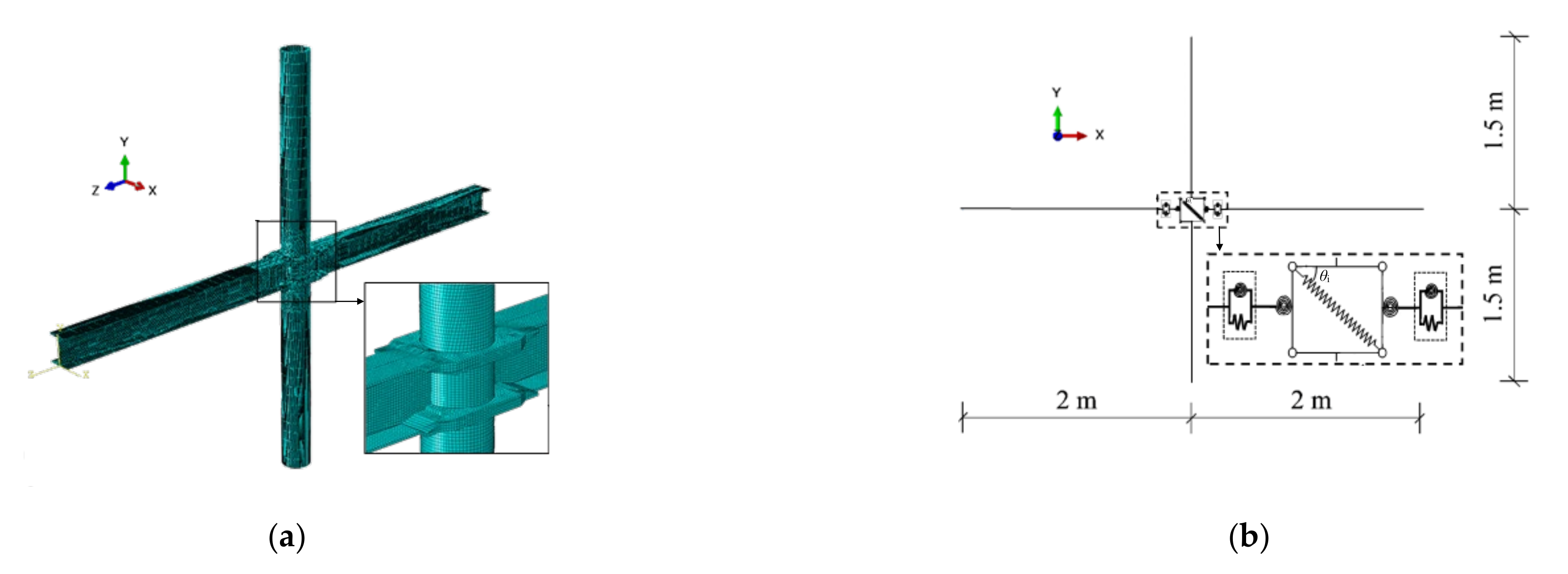
3.1. Detailed Solid-Element Model for Collapse
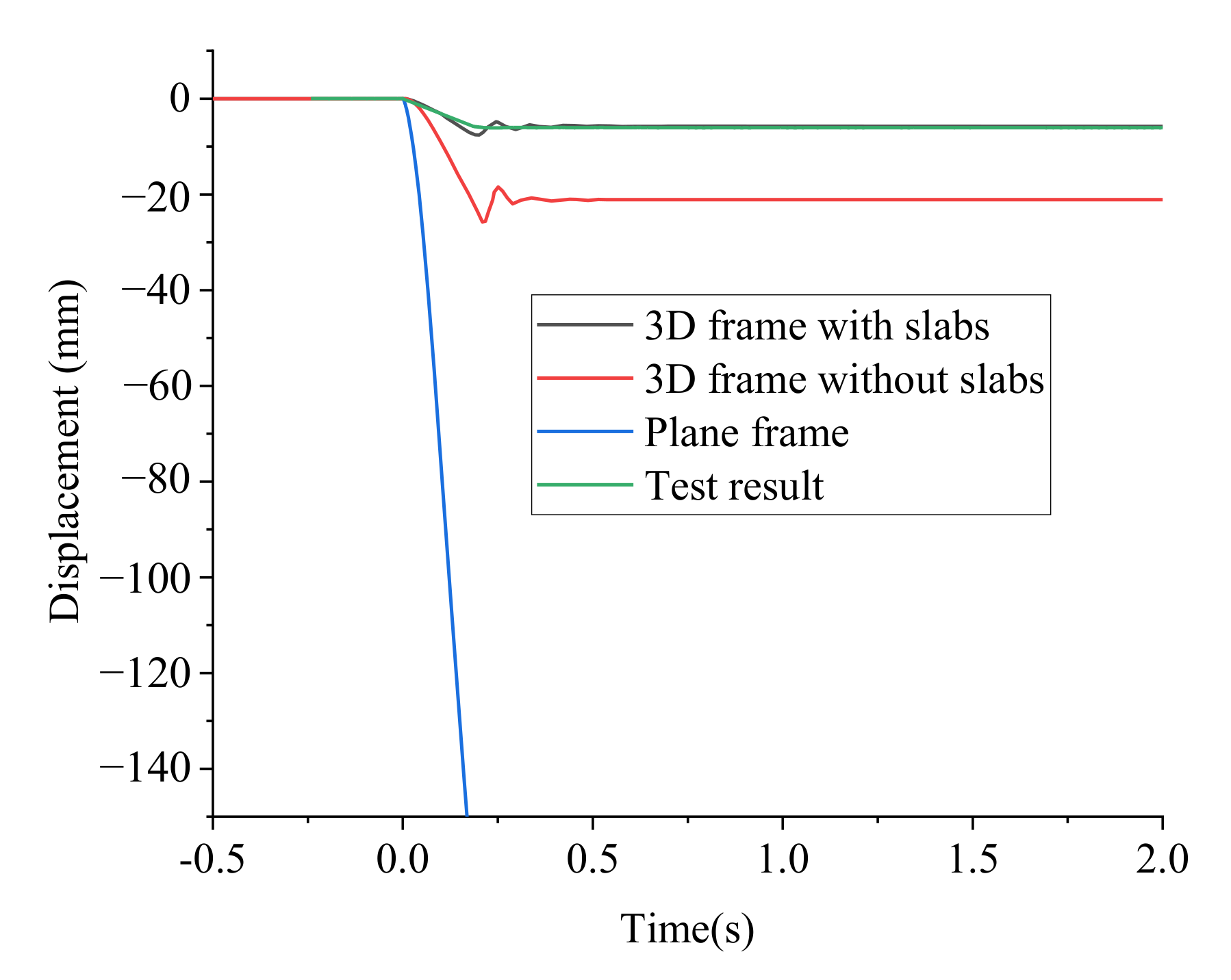
3.2. System Response under Different Load Cases
3.3. Force Transfer Mechanism
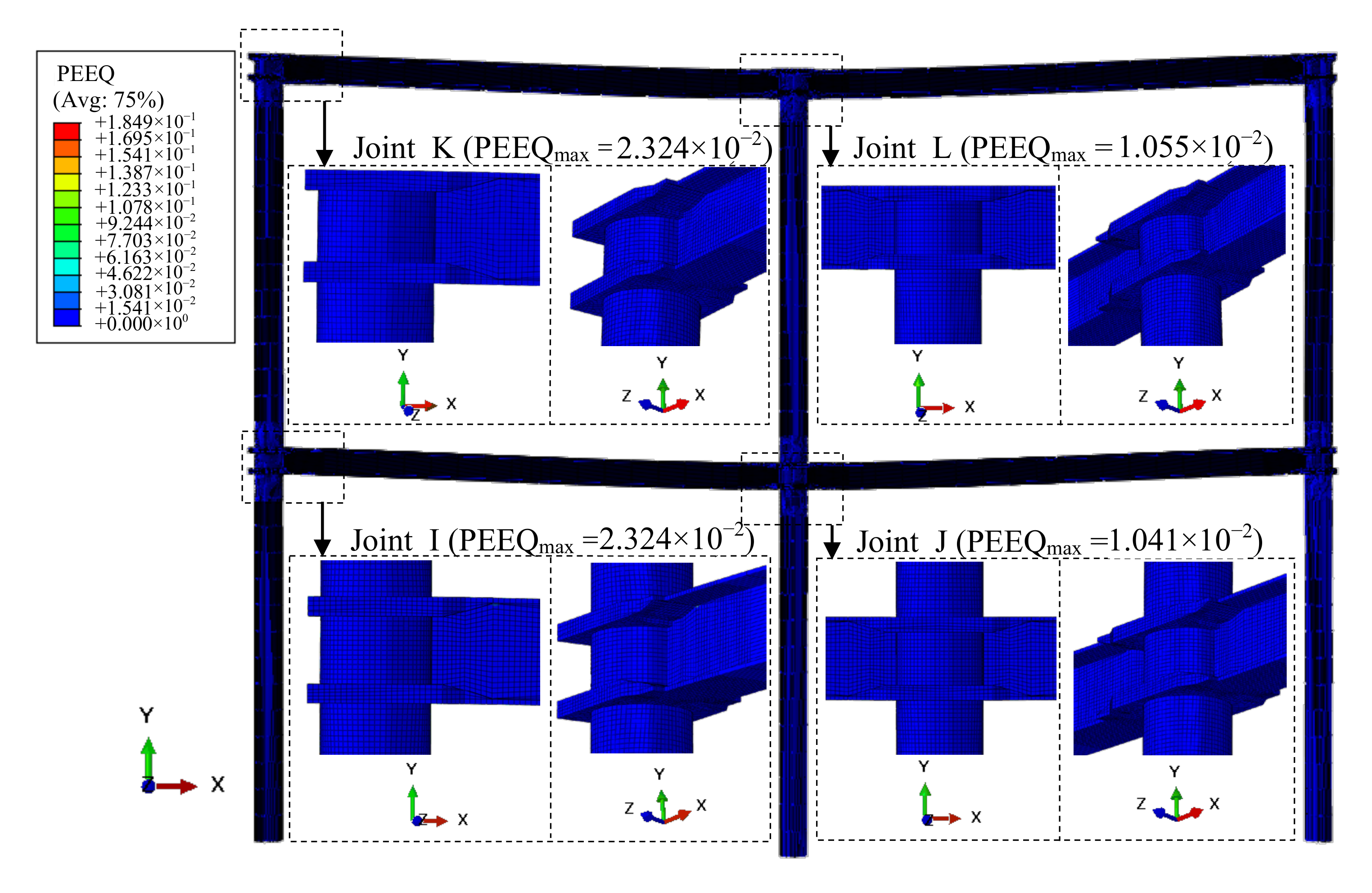
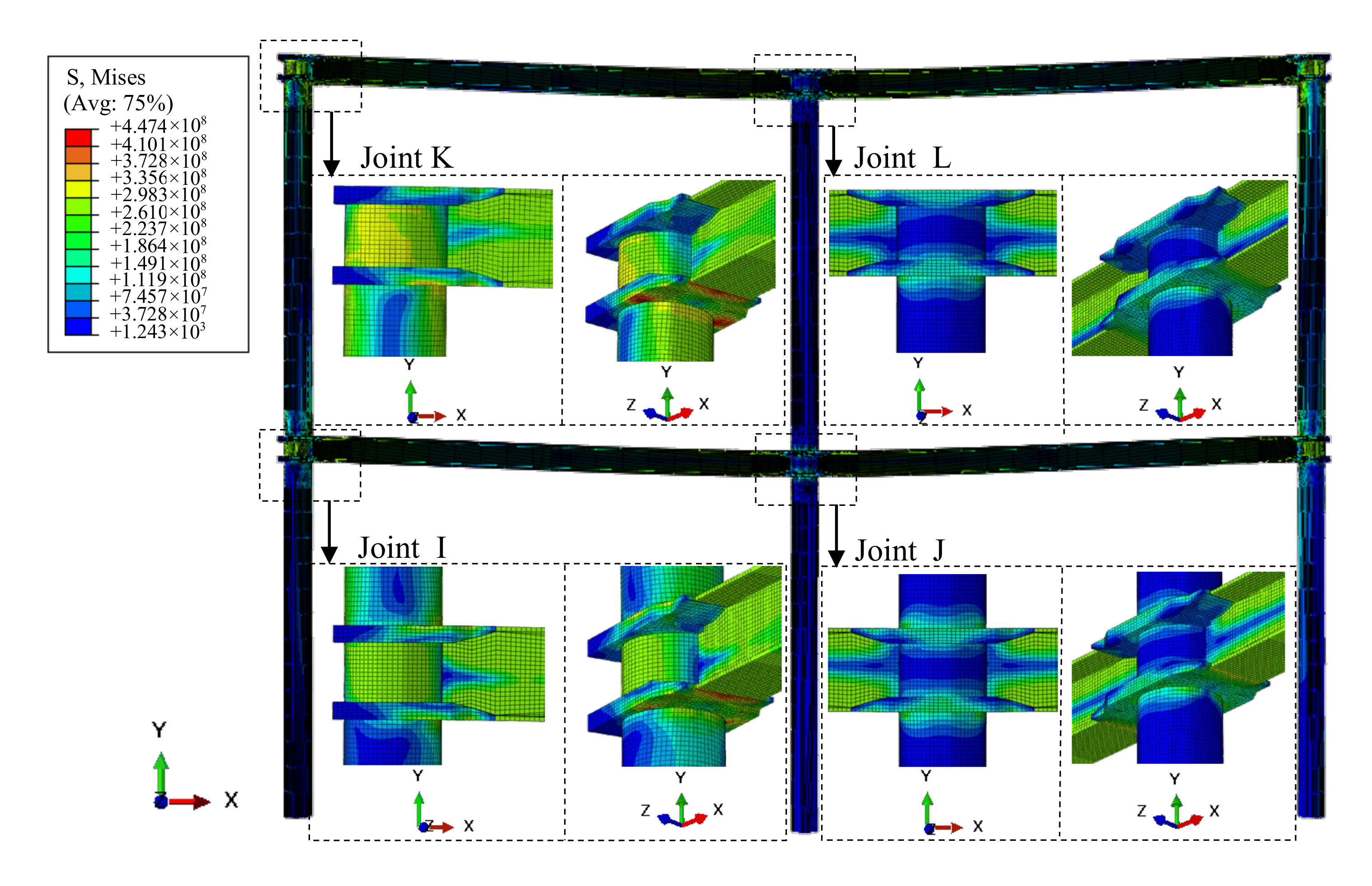
3.3.1. Stress Redistribution
3.3.2. Failure Mode
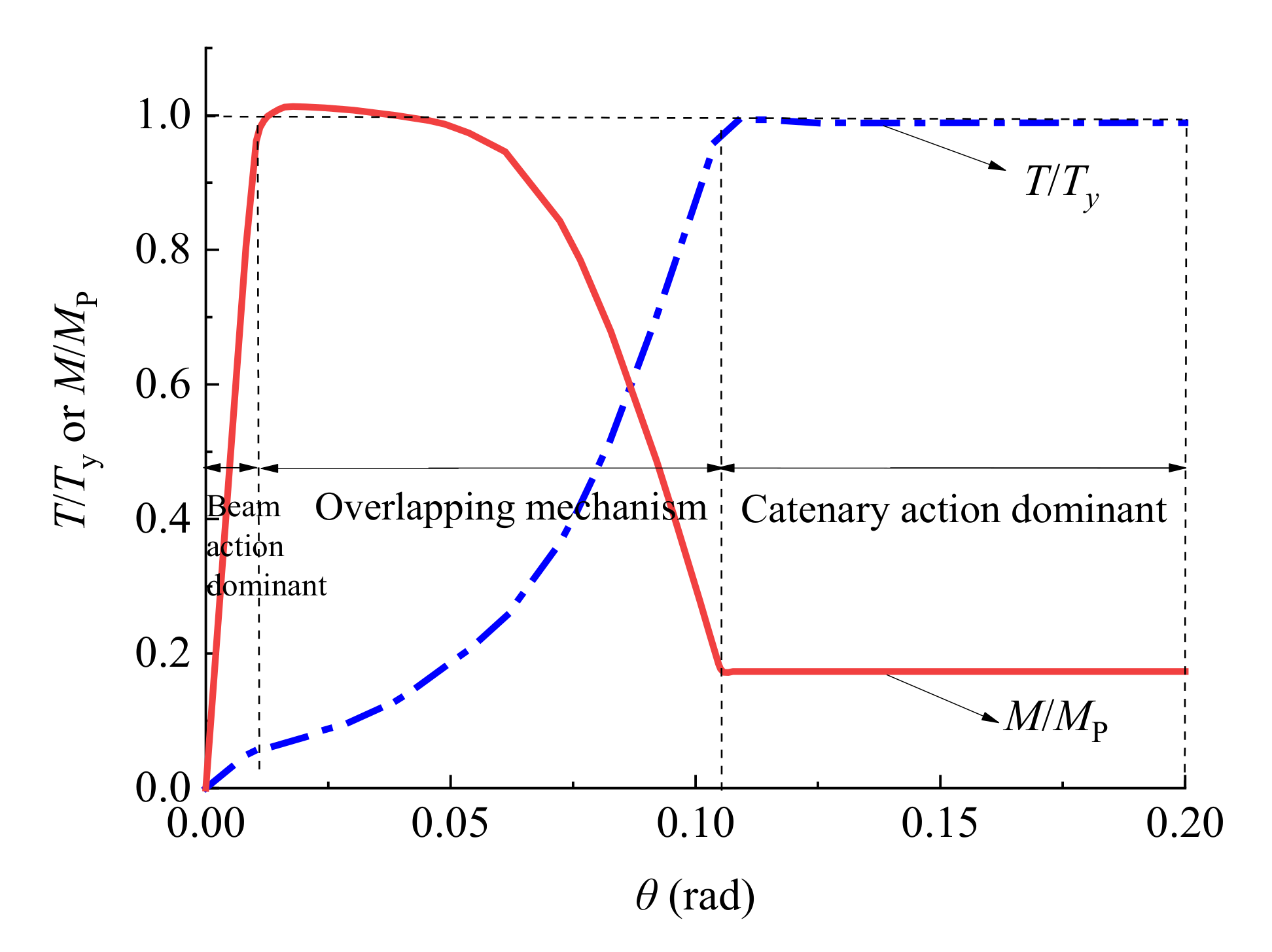
3.3.3. Axial Tension–Bending Moment Interaction at Beam Ends
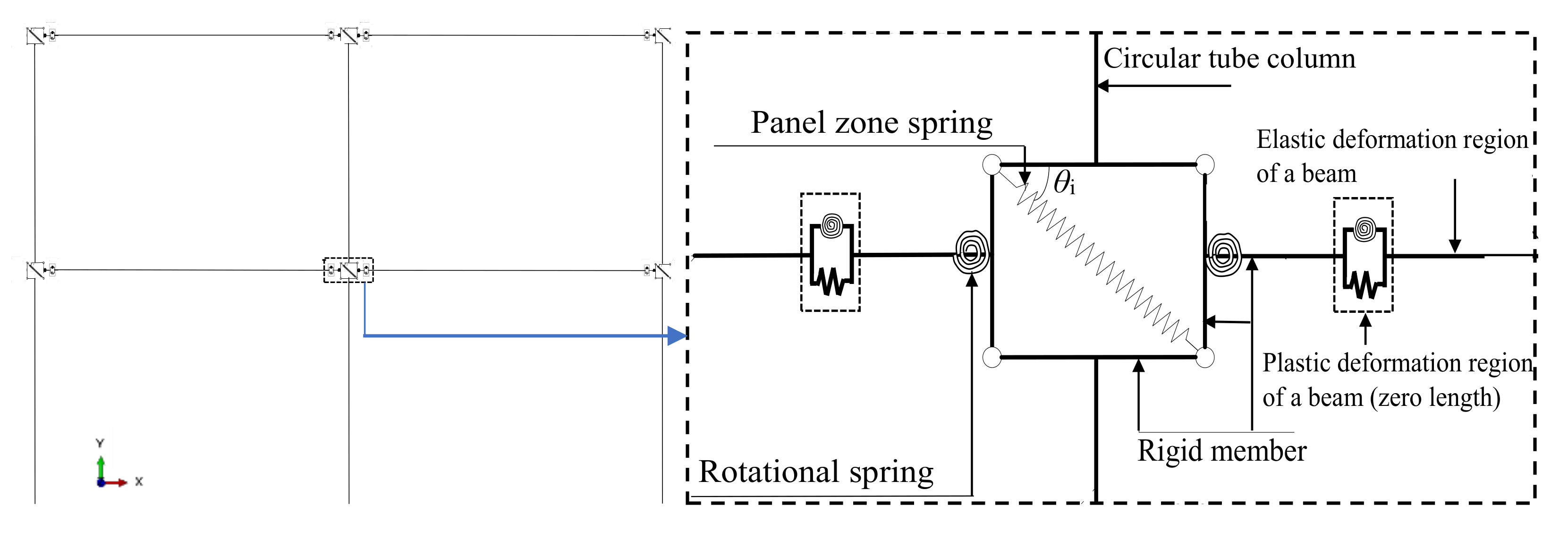
4. Component-Based Model for Frames with Circular Tube Columns and CSS Joints
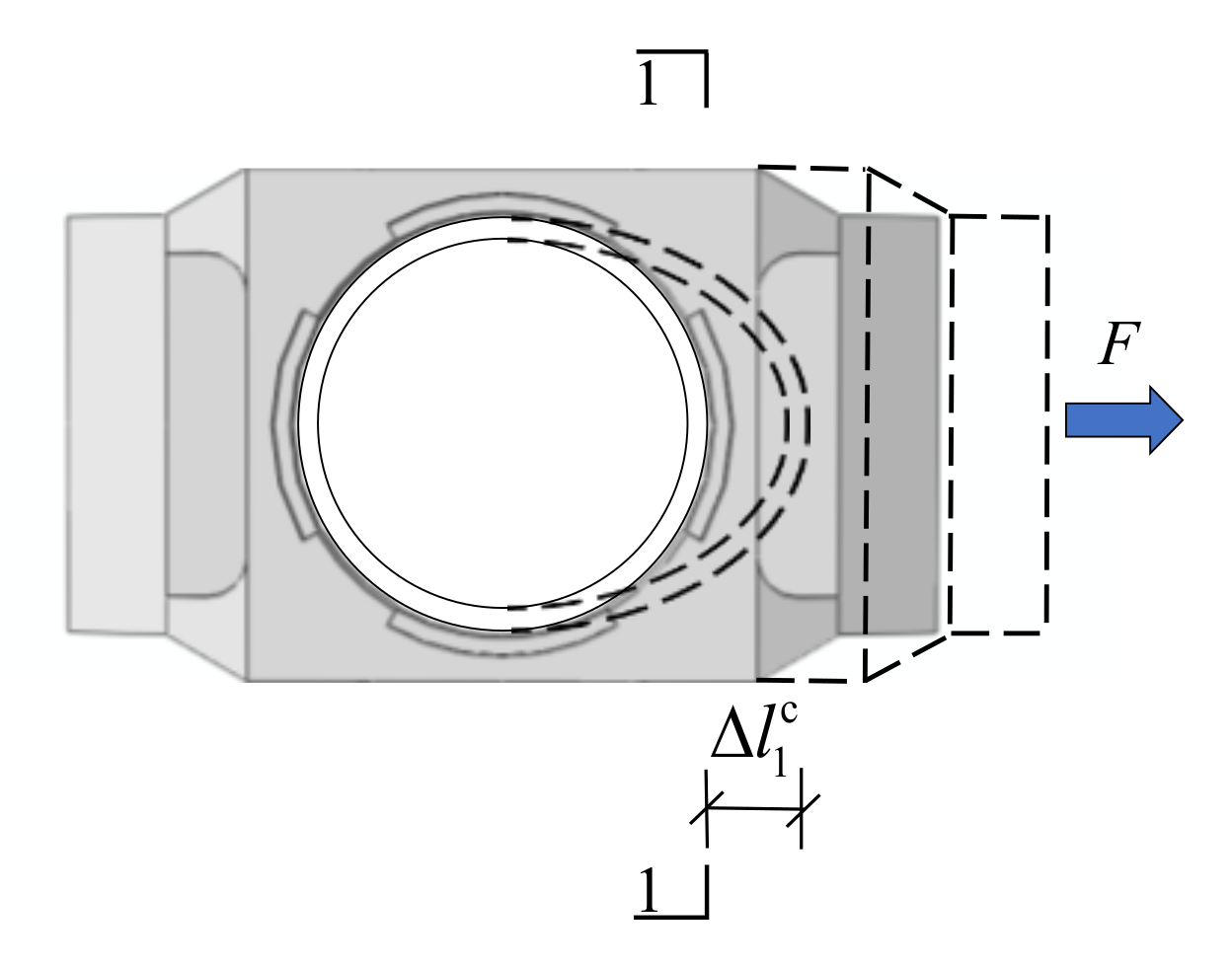
4.1. Plastic Deformation Region Properties at Beam End
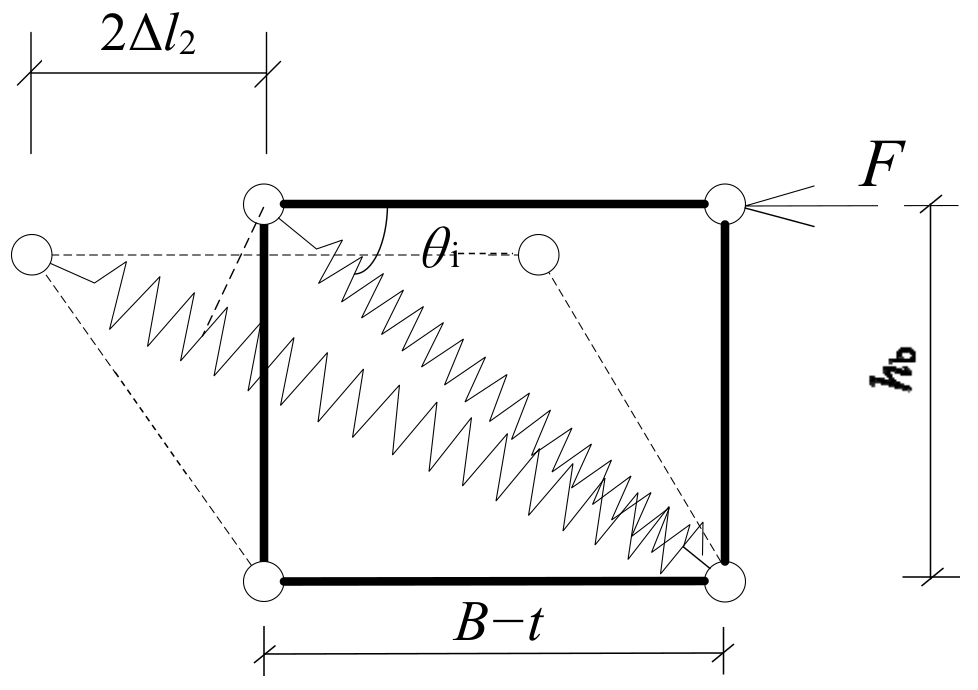
4.2. Force–Displacement Interaction Relationship of Joint Springs
4.2.1. Initial Stiffness of Joint Springs
- Rotational spring
- b.
- Panel zone spring
4.2.2. The Stiffness of the Joint Springs after Yielding
- (1)
- Bearing capacity
- a.
- Rotational spring
- b.
- Panel zone spring
- (2)
- Post-yield stiffness
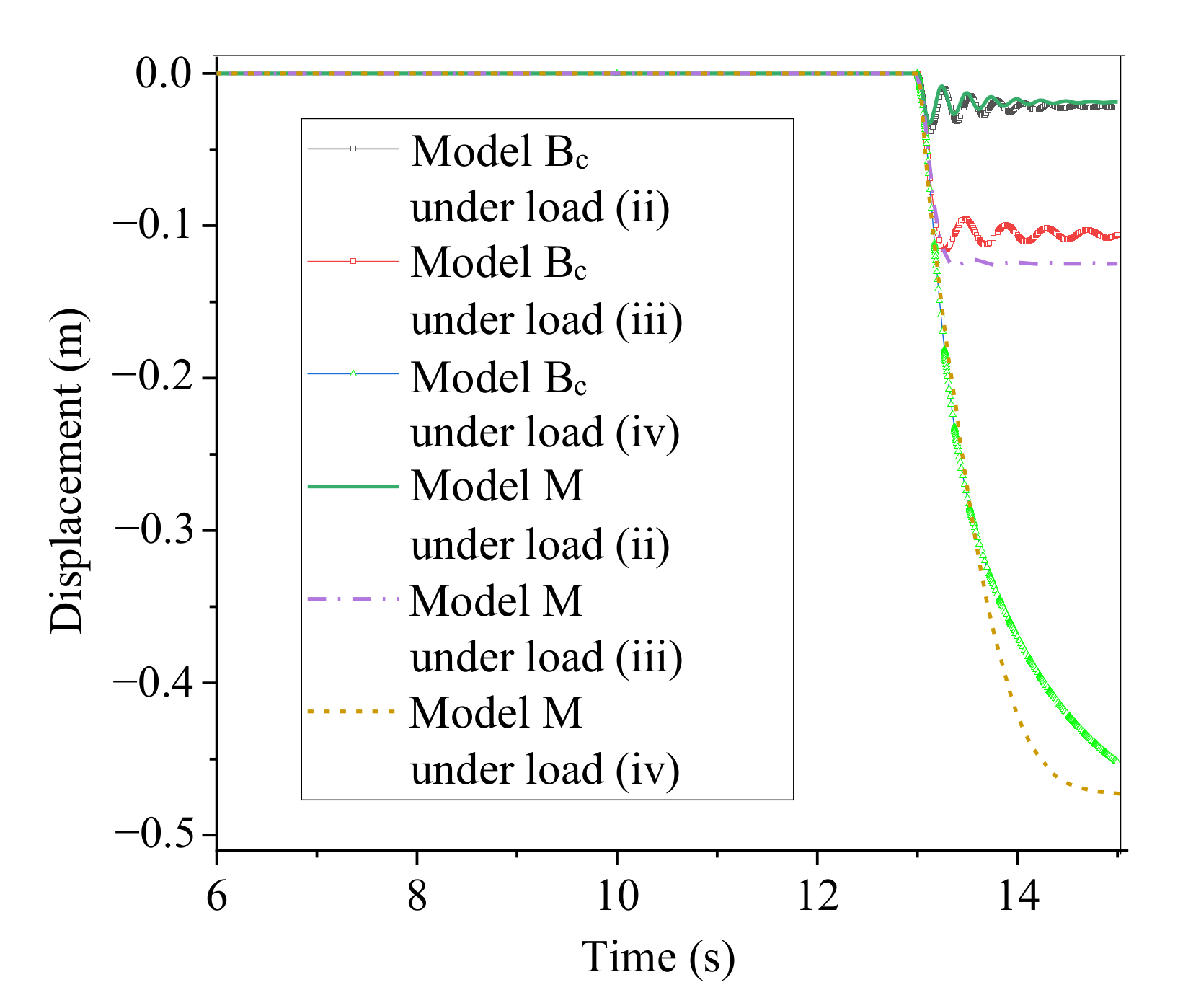
4.3. Component-Based Model Response under a Sudden Column Removal Scenario
5. Dynamic Response Increase Factor
6. Conclusions
- (1)
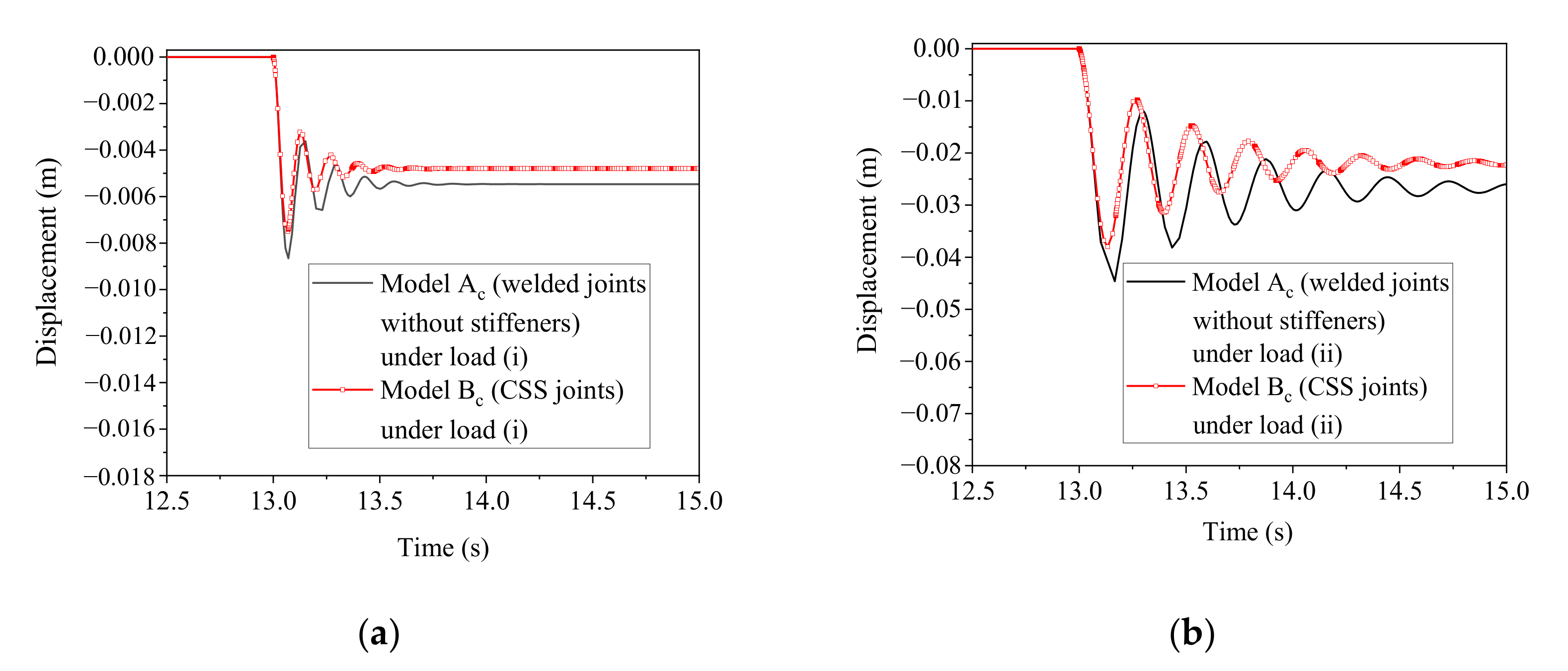
- The performance of the CSS joint frame for progressive collapse prevention is better than that of the fully welded joint frame. The CSS can delay the occurrence of plastic deformation of the CSS structures; the maximum and final values of the vertical displacement of the interior column in the CSS joint frame can be reduced, whether the frame is elastic, plastic, or collapsed after the sudden failure of the interior column.
- (2)
- In the case of sudden column removal, the stress distribution and the deformation mode of the CSS frame are revealed. Due to the relatively high rigidity and strength, the CSS can effectively bear the tensile loads from the flanges at beam ends and reduce the tensile loads borne by the front shell of the column, so the large strain of the column front shell is avoided. At the same time, due to the large strength and thickness of the cast steel stiffeners, the strain of the cast steel stiffeners is small. Therefore, the overall strain of the CSS joint is small and the stress distribution is relatively uniform. Moreover, plastic hinges at beam ends appear at locations farther away from columns in CSS joint frames, so the joints and the beam ends present relatively smooth-shaped curves after the sudden failure of the interior column.
- (3)
- Based on the stress distribution and the deformation mode of the circular-tube-column frames with CSS joints, a component-based model is proposed for the progressive collapse analysis. This efficient component-based model can simulate both the catenary effect and the construction details of the CSS joints for an overall steel frame under a column removal scenario. The equations of all the parameters of the springs in the component-based model are deduced based on the mechanical properties of the CSS joint frame, and the availability of the component-based model to simulate the dynamic nonlinear response of the CSS joint frame under a sudden column removal scenario is validated.
- (4)
- In order to further simplify the analysis process, dynamic effects can be incorporated into static analysis by using a dynamic increase factor for CSS joint frames. After plenty of static and dynamic analyses are conducted by using the component-based model, a dynamic increase factor of 1.7 suitable for the circular-tube-column frame with CSS is proposed. This dynamic increase factor is smaller than the 2.0 suggested in the DOD guidelines because the CSS joints reduce the maximum deformation of the frame during the progressive collapse.
Author Contributions
Funding
Conflicts of Interest
References
- DoD. Unified Facilities Criteria (UFC): Design of Buildings to Resist Progressive Collapse, Unified Facilities Criteria (UFC) 4-023-03; Department of Defense: Washington, DC, USA, 2010.
- Pearson, C.; Delatte, N. Ronan point apartment tower collapse and its effect on building codes. J. Perform. Constr. Fac. 2005, 19, 172–177. [Google Scholar] [CrossRef] [Green Version]
- Pantidis, P.; Gerasimidis, S. Progressive collapse of 3D steel composite buildings under interior gravity column loss. J. Constr. Steel Res. 2018, 150, 60–75. [Google Scholar] [CrossRef]
- Zhou, H.; Li, J.; Spencer, B.F., Jr. Multiscale random fields-based damage modeling and analysis of concrete structures. J. Eng. Mech. 2019, 145, 04019045. [Google Scholar] [CrossRef]
- Shabani, M.J.; Moghadam, A.S.; Aziminejad, A.; Razzaghi, M.S. Energy-based criteria for assessment of box-section steel columns against progressive collapse. Structures 2021, 34, 2580–2591. [Google Scholar] [CrossRef]
- Khandelwal, K.; El-Tawil, S. Pushdown resistance as a measure of robustness in progressive collapse analysis. Eng. Struct. 2011, 33, 2653–2661. [Google Scholar] [CrossRef]
- Gerasimidis, S.; Sideri, T. A new partial distributed damage method for progressive collapse analysis of buildings. J. Constr. Steel Res. 2016, 119, 233–245. [Google Scholar] [CrossRef]
- Sideri, J.; Mullen, C.L.; Gerasimidis, S.; Deodatis, G. Distributed Column damage effect on progressive collapse vulnerability in steel buildings exposed to an external blast event. J. Perform. Constr. Fac. 2017, 31, 04017077. [Google Scholar] [CrossRef]
- Wang, J.; Wang, W. Theoretical evaluation method for the progressive collapse resistance of steel frame buildings. J. Constr. Steel Res. 2021, 179, 106576. [Google Scholar] [CrossRef]
- Alashker, Y.; Li, H.; El-Tawil, S. Approximations in progressive collapse modeling. J. Struct. Eng. 2011, 137, 914–924. [Google Scholar] [CrossRef]
- Elsanadedy, H.M.; Abadel, A.A. High-fidelity FE models for assessing progressive collapse robustness of RC ordinary moment frame (OMF) buildings. Eng. Fail. Anal. 2022, 136, 106228. [Google Scholar] [CrossRef]
- Li, L.; Wang, W.; Chen, Y.; Lu, Y. Experimental investigation of beam-to-tubular column moment connections under column removal scenario. J. Constr. Steel Res. 2013, 88, 244–255. [Google Scholar] [CrossRef]
- Elsanadedy, H.; Alrubaidi, M.; Abbas, H.; Almusallam, T.; Al-Salloum, Y. Progressive collapse risk of 2D and 3D steel-frame assemblies having shear connections. J. Constr. Steel Res. 2021, 179, 106533. [Google Scholar] [CrossRef]
- Mageirou, G.E.; Lemonis, M.E. Influence of imperfections on the progressive collapse of steel moment resisting frames. J. Constr. Steel Res. 2021, 183, 106744. [Google Scholar] [CrossRef]
- Subki, N.E.A.; Mansor, H.; Hamid, Y.S.; Parke, G.A.R. The development of a moment-rotation model for progressive collapse analysis under the influence of tensile catenary action. J. Constr. Steel Res. 2021, 187, 106960. [Google Scholar] [CrossRef]
- Daneshvar, H.; Driver, R.G. Performance evaluation of WT connections in progressive collapse. Eng. Struct. 2018, 167, 376–392. [Google Scholar] [CrossRef]
- Zhong, W.; Meng, B.; Hao, J. Performance of different stiffness connections against progressive collapse. J. Constr. Steel Res. 2017, 135, 162–175. [Google Scholar] [CrossRef]
- Qian, K.; Lan, X.; Li, Z.; Li, Y.; Fu, F. Progressive collapse resistance of two-storey seismic configured steel sub-frames using welded connections. J. Constr. Steel Res. 2020, 170, 106117. [Google Scholar] [CrossRef]
- Li, H.; Cai, X.; Zhang, L.; Zhang, B.; Wang, W. Progressive collapse of steel moment-resisting frame subjected to loss of interior column: Experimental tests. Eng. Struct. 2017, 150, 203–220. [Google Scholar] [CrossRef]
- Alrubaidi, M.; Elsanadedy, H.; Abbas, H.; Almusallam, T. Investigation of different steel intermediate moment frame connections under column-loss scenario. Thin Walled Struct. 2020, 154, 106875. [Google Scholar] [CrossRef]
- Cortés, G.; Liu, J. Behavior of conventional and enhanced gravity connections subjected to column loss. J. Constr. Steel Res. 2017, 133, 475–484. [Google Scholar] [CrossRef]
- Meng, B.; Zhong, W.; Hao, J.; Tan, Z. Improved steel frame performance against progressive collapse with infill panels. J. Constr. Steel Res. 2019, 158, 201–212. [Google Scholar] [CrossRef]
- Chen, C.; Qiao, H.; Wang, J.; Chen, Y. Progressive collapse behavior of joints in steel moment frames involving reduced beam section. Eng. Struct. 2020, 225, 111297. [Google Scholar] [CrossRef]
- Yu, Y.; Lan, L.; Chen, Z.; Huang, J. Mechanical and seismic behaviors of bottom-flange-bolted upper-flange-welded through-diaphragm connections. J. Constr. Steel Res. 2019, 156, 86–95. [Google Scholar] [CrossRef]
- Han, Q.; Li, X.; Liu, M.; Spencer, B.F., Jr. Performance analysis and macromodel simulation of steel frame structures with beam-column joints using cast steel stiffeners for progressive collapse prevention. Thin Walled Struct. 2019, 140, 404–415. [Google Scholar] [CrossRef]
- Feng, D.C.; Shi, H.R.; Parisi, F.; Brunesi, E.; Wang, C. Efficient numerical model for progressive collapse analysis of prestressed concrete frame structures. Eng. Fail. Anal. 2021, 129, 105683. [Google Scholar] [CrossRef]
- Wang, F.; Yang, J.; Pan, Z. Progressive collapse behaviour of steel framed substructures with various beam-column connections. Eng. Fail. Anal. 2020, 109, 104399. [Google Scholar] [CrossRef]
- Lin, S.C.; Yang, B.; Kang, S.B.; Xu, S.Q. A new method for progressive collapse analysis of steel frames. J. Constr. Steel Res. 2019, 153, 71–84. [Google Scholar] [CrossRef]
- Ding, Y.; Song, X.; Zhu, H.T. Probabilistic progressive collapse analysis of steel frame structures against blast loads. Eng. Struct. 2017, 147, 679–691. [Google Scholar] [CrossRef]
- Liu, M. Progressive collapse design of seismic steel frames using structural optimization. J. Constr. Steel Res. 2011, 67, 322–332. [Google Scholar] [CrossRef]
- Dinu, F.; Marginean, I.; Dubina, D. Experimental testing and numerical modelling of steel moment-frame connections under column loss. Eng. Struct. 2017, 151, 861–878. [Google Scholar] [CrossRef]
- Jiang, Z.Q.; Chen, M.L.; Yang, Z.S.; Li, X.Y.; Cai, C. Cyclic loading tests of self-centering prestressed prefabricated steel beam-column joint with weakened FCP. Eng. Struct. 2022, 252, 113578. [Google Scholar] [CrossRef]
- Cheng, G.; Zhou, X.; Liu, J.; Chen, Y.F. Seismic behavior of circular tubed steel-reinforced concrete column to steel beam connections. Thin Walled Struct. 2019, 138, 485–495. [Google Scholar] [CrossRef]
- Khanouki, M.M.A.; Sulong, N.H.R.; Shariati, M.; Tahir, M.M. Investigation of through beam connection to concrete filled circular steel tube (CFCST) column. J. Constr. Steel Res. 2016, 121, 144–162. [Google Scholar] [CrossRef]
- Zhou, X.; Cheng, G.; Liu, J.; Yang, Y.; Chen, Y.F. Shear transfer behavior at the circular tubed column-steel beam interface. Thin Walled Struct. 2019, 137, 40–52. [Google Scholar] [CrossRef]
- Mou, B.; Li, Y.; Wang, F.; Pan, W.; Zhao, Y. Flexural behavior of a novel high-strength RCFST column-to-column connection. Thin Walled Struct. 2021, 159, 107274. [Google Scholar] [CrossRef]
- Yang, Z.; Deng, H.; Hu, X. Strength of longitudinal X-type plate-to-circular hollow section (CHS) connections reinforced by external ring stiffeners. Thin Walled Struct. 2018, 131, 500–518. [Google Scholar] [CrossRef]
- Liu, M.; Li, X.; Han, Q.; Lu, Y. Theoretical research on force transmission mechanism and bearing capacity of round steel tube column-H-shaped steel beam cast-steel connection joint. J. Build. Struct. 2019, 40, 200–209. (In Chinese) [Google Scholar]
- Khandelwal, K.; El Tawil, S.; Kunnath, S.K.; Lew, H.S. Macromodel-Based Simulation of Progressive Collapse: Steel Frame Structures. J. Struct. Eng. 2008, 134, 1070–1078. [Google Scholar] [CrossRef]
- Lee, C.; Kim, S.; Lee, K. Parallel axial-flexural hinge model for nonlinear dynamic progressive collapse analysis of welded steel moment frames. J. Struct. Eng. 2010, 136, 165–173. [Google Scholar] [CrossRef]
- Yu, J.; Tan, K.H. Experimental and numerical investigation on progressive collapse resistance of reinforced concrete beam column sub-assemblages. Eng. Struct. 2013, 55, 90–106. [Google Scholar] [CrossRef]
- Bao, Y.; Kunnath, S.K.; El-Tawil, S.; Lew, H.S. Macromodel-based simulation of progressive collapse: RC frame structures. J. Struct. Eng. 2008, 134, 1079–1091. [Google Scholar] [CrossRef]
- Fu, Q.; Yang, B.; Hu, Y.; Xiong, G.; Nie, S.; Zhang, W.; Dai, G. Dynamic analyses of bolted-angle steel joints against progressive collapse based on component-based model. J. Constr. Steel Res. 2016, 117, 161–174. [Google Scholar] [CrossRef]
- Zhao, Z.; Jian, X.; Liang, B.; Liu, H. Progressive collapse assessment of friction damped post-tensioned steel frames based on a simplified model. Structures 2020, 23, 447–458. [Google Scholar] [CrossRef]
- Liu, C.; Tan, K.H.; Fung, T.C. Component-based steel beam–column connections modelling for dynamic progressive collapse analysis. J. Constr. Steel Res. 2015, 107, 24–36. [Google Scholar] [CrossRef]
- Stylianidis, P.M.; Nethercot, D.A. Modelling of connection behaviour for progressive collapse analysis. J. Constr. Steel Res. 2015, 113, 169–184. [Google Scholar] [CrossRef]
- GB50017-2003; Code for Design of Steel Structures. Ministry of Housing and Urban–Rural Development of the People’s Republic of China: Beijing, China, 2003.
- GB50009-2012; Load Code for the Design of Building Structures. Ministry of Housing and Urban–Rural Development of the People’s Republic of China: Beijing, China, 2012.
- Khandelwal, K.; El-Tawil, S. Collapse behavior of steel special moment resisting frame connections. J. Struct. Eng. 2007, 133, 646–655. [Google Scholar] [CrossRef]
- Han, Q.; Li, X.; Liu, M.; Spencer, B.F., Jr. Experimental investigation of beam-column joints with cast steel stiffeners for progressive collapse prevention. J. Struct. Eng. 2019, 145, 04019020. [Google Scholar] [CrossRef]
- Wang, K.; Li, G.; Yang, T. The performance of restrained steel beams considering catenary effect under distributed loads (I)-Theoretical model. Chin. Civ. Eng. J. 2010, 43, 8–12. (In Chinese) [Google Scholar]
- EN1993-1-1; Eurocode 3: Design of Steel Structures, Part 1–3: General Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2005.
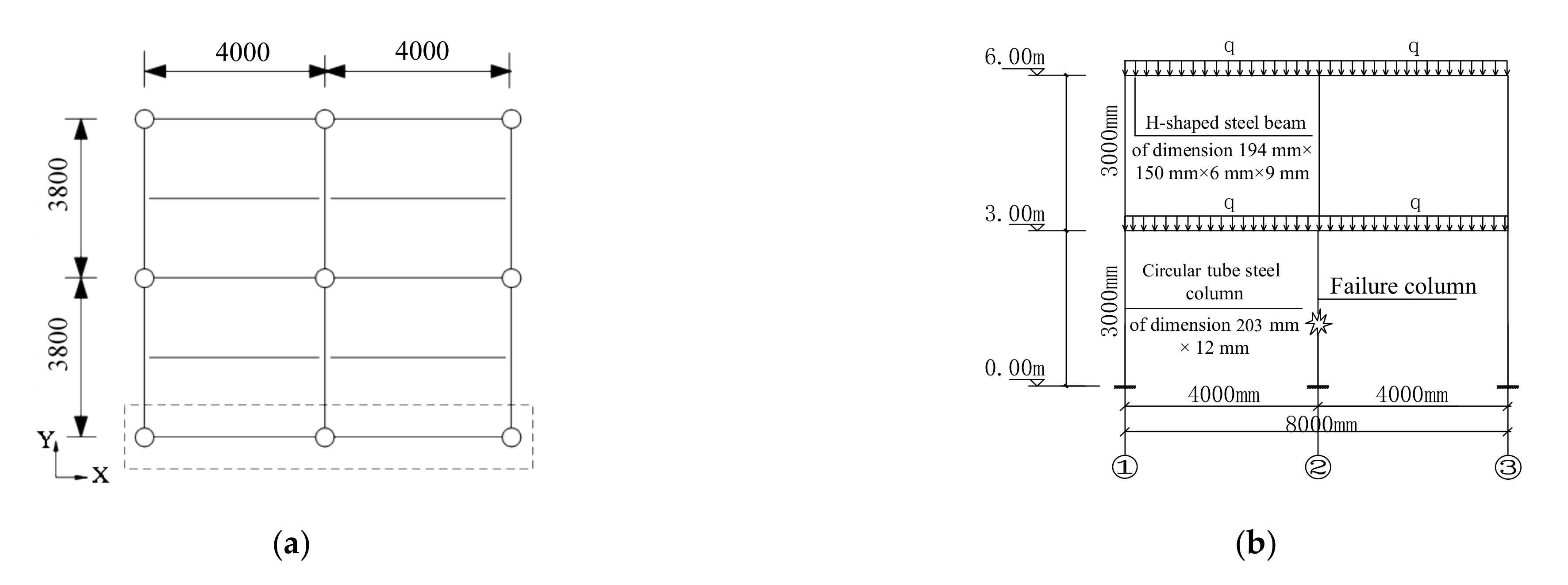

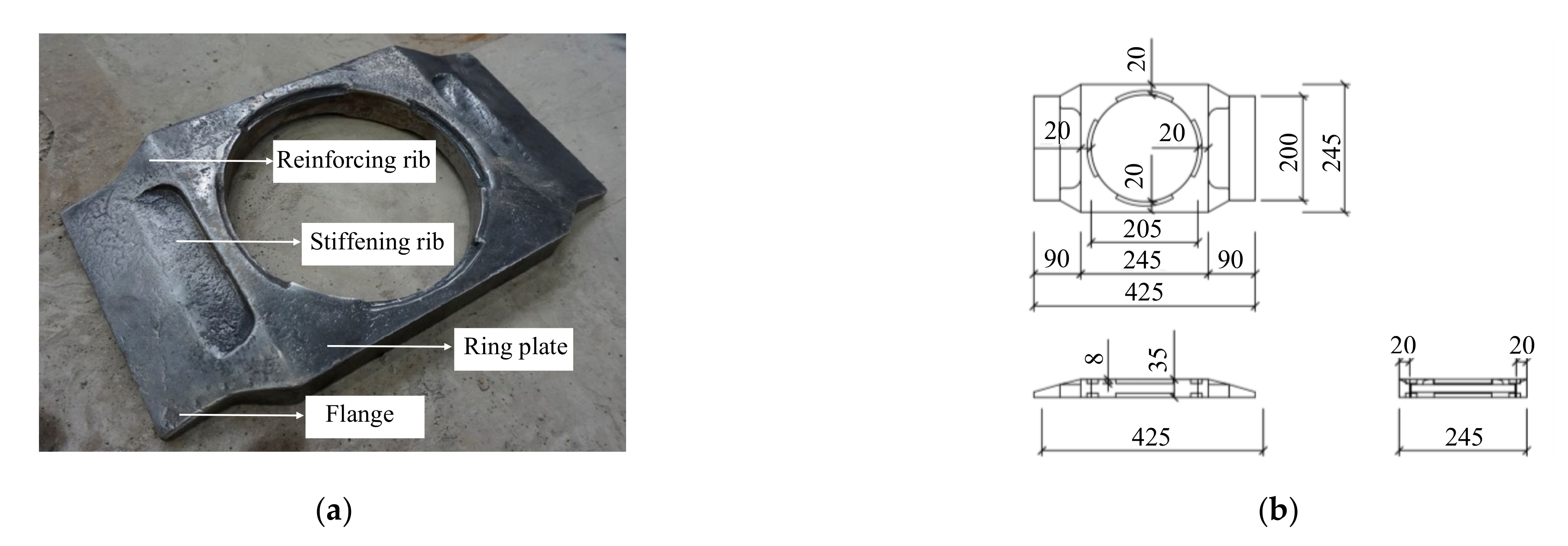









| Load | Dead Load (kN·m−2) | Live Load (kN·m−2) |
|---|---|---|
| i | 1.0 | 0.5 |
| ii | 3.2 | 2.0 |
| iii | 5.1 | 12.0 |
| iv | 5.1 | 20.0 |
| Load | Maximum Displacement (mm) | Final Displacement (mm) | ||
|---|---|---|---|---|
| Model Ac | Model Bc | Model Ac | Model Bc | |
| i | −8.65 | −7.49 | −5.47 | −4.80 |
| ii | −44.61 | −38.02 | −25.96 | −22.34 |
| iii | −202.04 | −115.99 | −202.04 | −105.97 |
| iv | −1016.92 | −451.97 | −1016.92 | −451.97 |
| Load | The Time When Plastic Strain Occurred (s) | |
|---|---|---|
| Model Ac | Model Bc | |
| i | - | - |
| ii | 13.059 | 13.071 |
| iii | 13.052 | 13.054 |
| iv | 13.052 | 13.054 |
| Specimen | Column Thickness t (mm) | Test Results K1 (kN·m·rad−1) | Detailed Solid-Element Model Results K2 (kN·m·rad−1) | Component-Based Model Results K3 (kN·m·rad−1) | K2/K1 | K3/K1 |
|---|---|---|---|---|---|---|
| 1 | 8 | 15,467 | 13,794 | 14,021 | 0.892 | 0.907 |
| 2 | 10 | 16,022 | 16,011 | 16,132 | 0.999 | 1.007 |
| 3 | 12 | 19,893 | 18,068 | 18,763 | 0.908 | 0.943 |
| Specimen | hb (mm) | B (mm) | t (mm) | hr (mm) | l1 (mm) | l2 (mm) | Detailed Solid-Element Model K2 (kN·m·rad−1) | Component-Based Model K3 (kN·m·rad−1) | K3/K2 |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 185 | 203 | 8 | 35 | 1407.5 | 1592.5 | 13,800 | 13,016 | 0.943 |
| 2 | 210 | 15,034 | 13,893 | 0.924 | |||||
| 3 | 235 | 16,150 | 15,932 | 0.987 | |||||
| 1 | 185 | 153 | 10 | 35 | 1407.5 | 1592.5 | 8926 | 9123 | 1.022 |
| 4 | 178 | 13,003 | 13,976 | 1.075 | |||||
| 5 | 203 | 16,035 | 16,923 | 1.055 | |||||
| 1 | 185 | 203 | 8 | 35 | 1407.5 | 1592.5 | 13,794 | 14,021 | 1.016 |
| 6 | 10 | 16,011 | 16,132 | 1.008 | |||||
| 7 | 12 | 18,068 | 18,763 | 1.038 | |||||
| 1 | 185 | 203 | 8 | 35 | 1407.5 | 1592.5 | 13,800 | 13,016 | 0.943 |
| 8 | 40 | 14,069 | 13,475 | 0.958 | |||||
| 9 | 45 | 14,296 | 13,972 | 0.977 | |||||
| 1 | 185 | 203 | 8 | 35 | 1407.5 | 1592.5 | 13,800 | 13,016 | 0.943 |
| 10 | 1842.5 | 11,917 | 10,334 | 0.867 | |||||
| 11 | 2092.5 | 10,048 | 9452 | 0.941 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Tao, L.; Liu, M. Force Transfer Mechanism and Component-Based Model of Cast-Steel-Stiffened Circular-Tube-Column Frames for Progressive Collapse Analysis. Buildings 2022, 12, 1049. https://doi.org/10.3390/buildings12071049
Li X, Tao L, Liu M. Force Transfer Mechanism and Component-Based Model of Cast-Steel-Stiffened Circular-Tube-Column Frames for Progressive Collapse Analysis. Buildings. 2022; 12(7):1049. https://doi.org/10.3390/buildings12071049
Chicago/Turabian StyleLi, Xinxia, Lan Tao, and Mingjie Liu. 2022. "Force Transfer Mechanism and Component-Based Model of Cast-Steel-Stiffened Circular-Tube-Column Frames for Progressive Collapse Analysis" Buildings 12, no. 7: 1049. https://doi.org/10.3390/buildings12071049
APA StyleLi, X., Tao, L., & Liu, M. (2022). Force Transfer Mechanism and Component-Based Model of Cast-Steel-Stiffened Circular-Tube-Column Frames for Progressive Collapse Analysis. Buildings, 12(7), 1049. https://doi.org/10.3390/buildings12071049





