Evaluation of Inverted-Pendulum-with-Rigid-Legs Walking Locomotion Models for Civil Engineering Applications
Abstract
:1. Introduction
2. Characteristics of Walking Locomotion
2.1. Kinematics and Kinetics of a Walking Gait Cycle

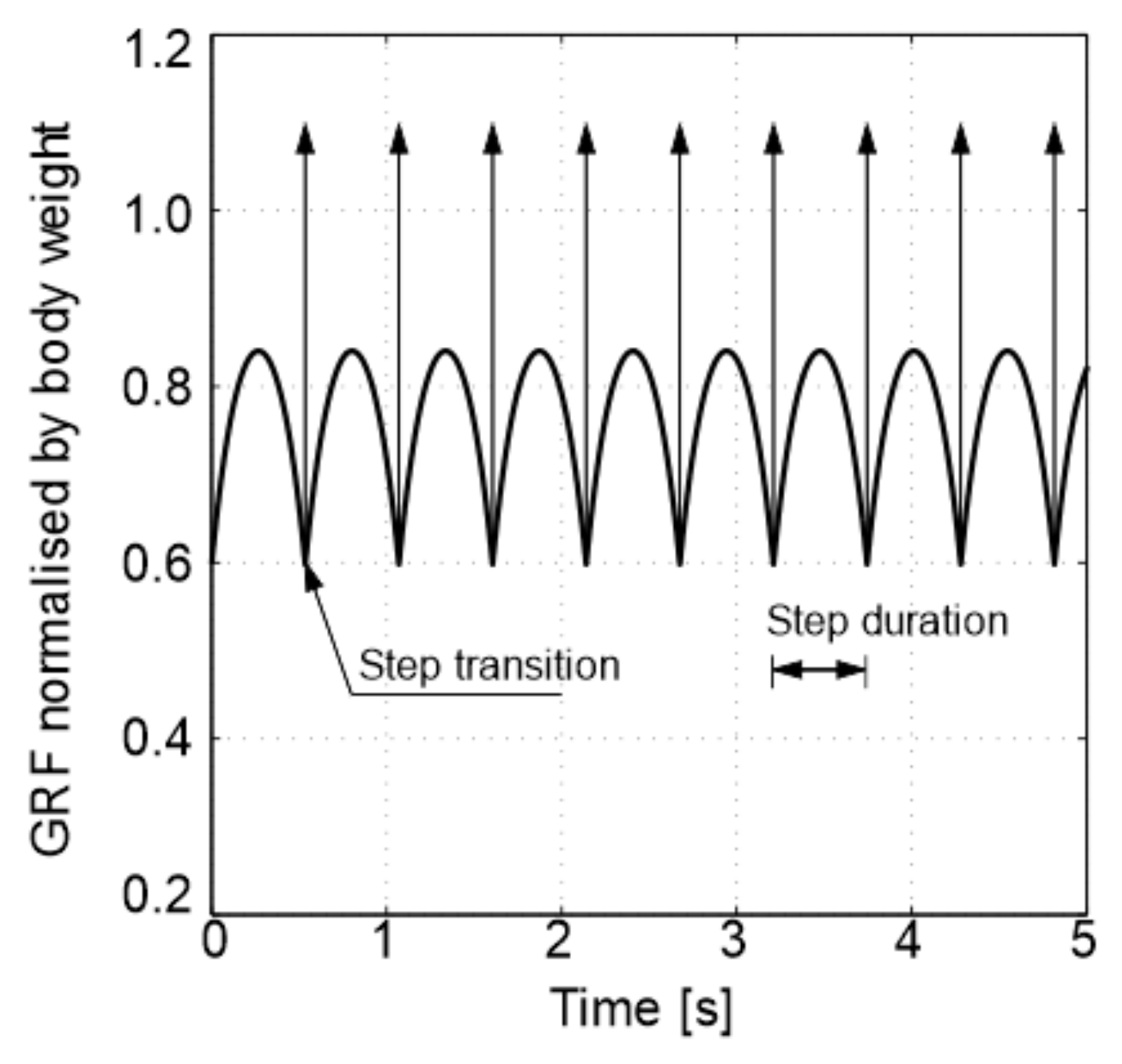
2.2. Frequency Content of Ground Reaction Force
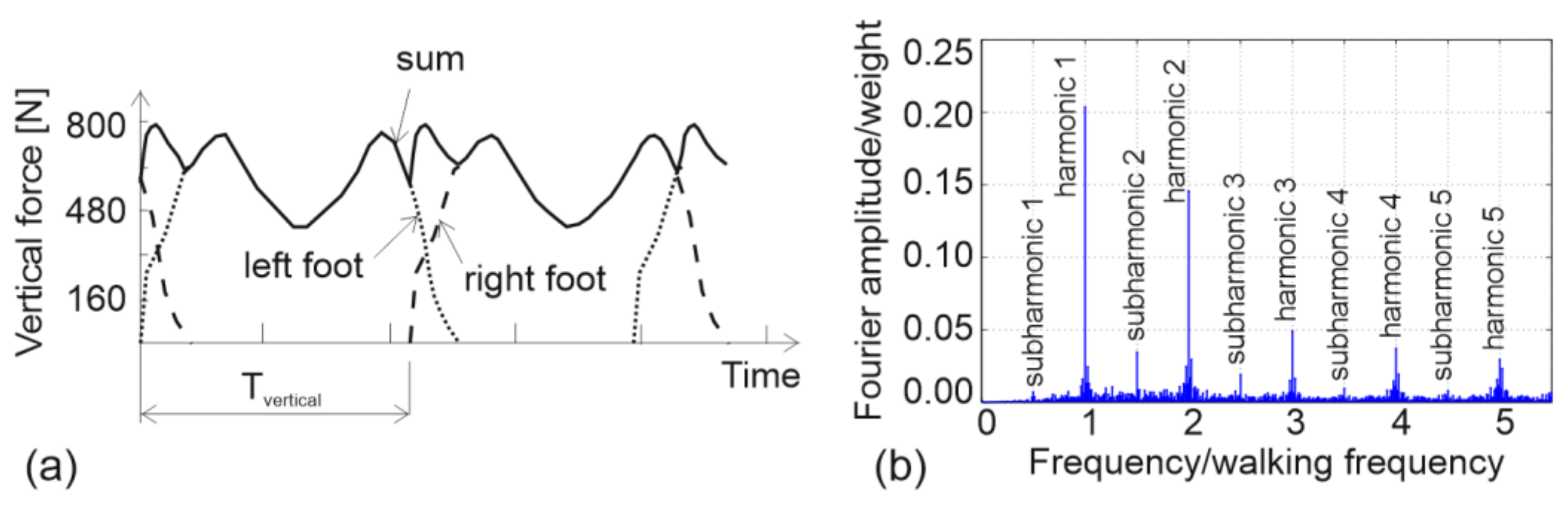
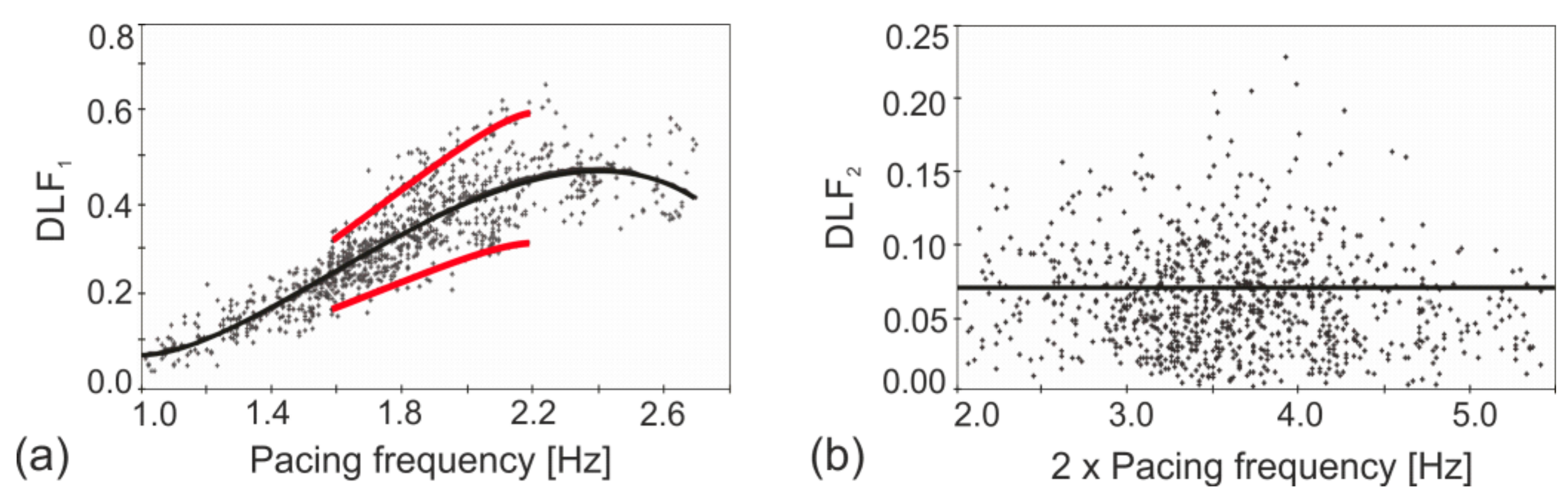
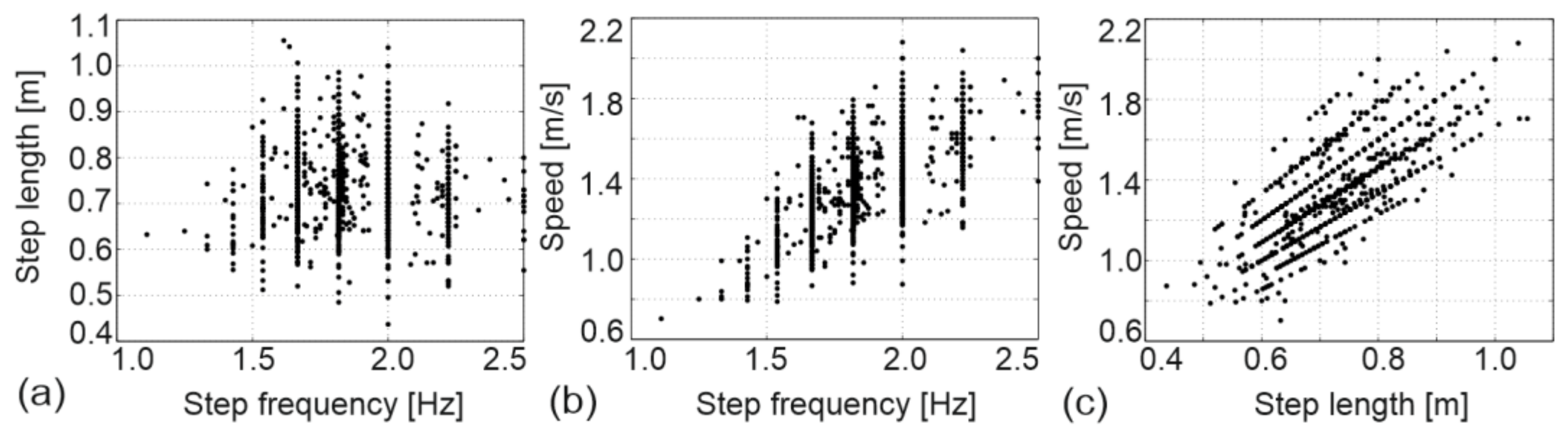
2.3. Pacing Frequency and Pedestrian’s Forward Speed
| Structure | Country | Sample | Pacing Rate (Hz) | Walking Speed (m/s) | ||
|---|---|---|---|---|---|---|
| [Reference] | Size | Mean | STD | Mean | STD | |
| Road [41] | Japan | 505 | 1.99 | 0.17 | - | - |
| Footbridge 1 [42] | UK | 200 | 1.86 | 0.11 | 1.38 | 0.13 |
| Footbridge 2 [42] | UK | 200 | 1.80 | 0.10 | 1.23 | 0.09 |
| Two shopping floors [42] | UK | 400 | 2.00 | 0.13 | 1.41 | 0.13 |
| Footbridge [43] | Germany | 251 | 1.82 | 0.12 | 1.37 | 0.15 |
| Walkway [44] | Italy | 116 | 1.84 | 0.17 | 1.41 | 0.22 |
| Indoor footbridge [45] | UK | 939 | 1.94 | 0.19 | 1.47 | 0.23 |
| Footbridge [46] | Montenegro | 2019 | 1.87 | 0.19 | 1.39 | 0.20 |
2.4. Criteria for Evaluation of Bipedal Models
3. Inverted Pendulum Models with Rigid Legs

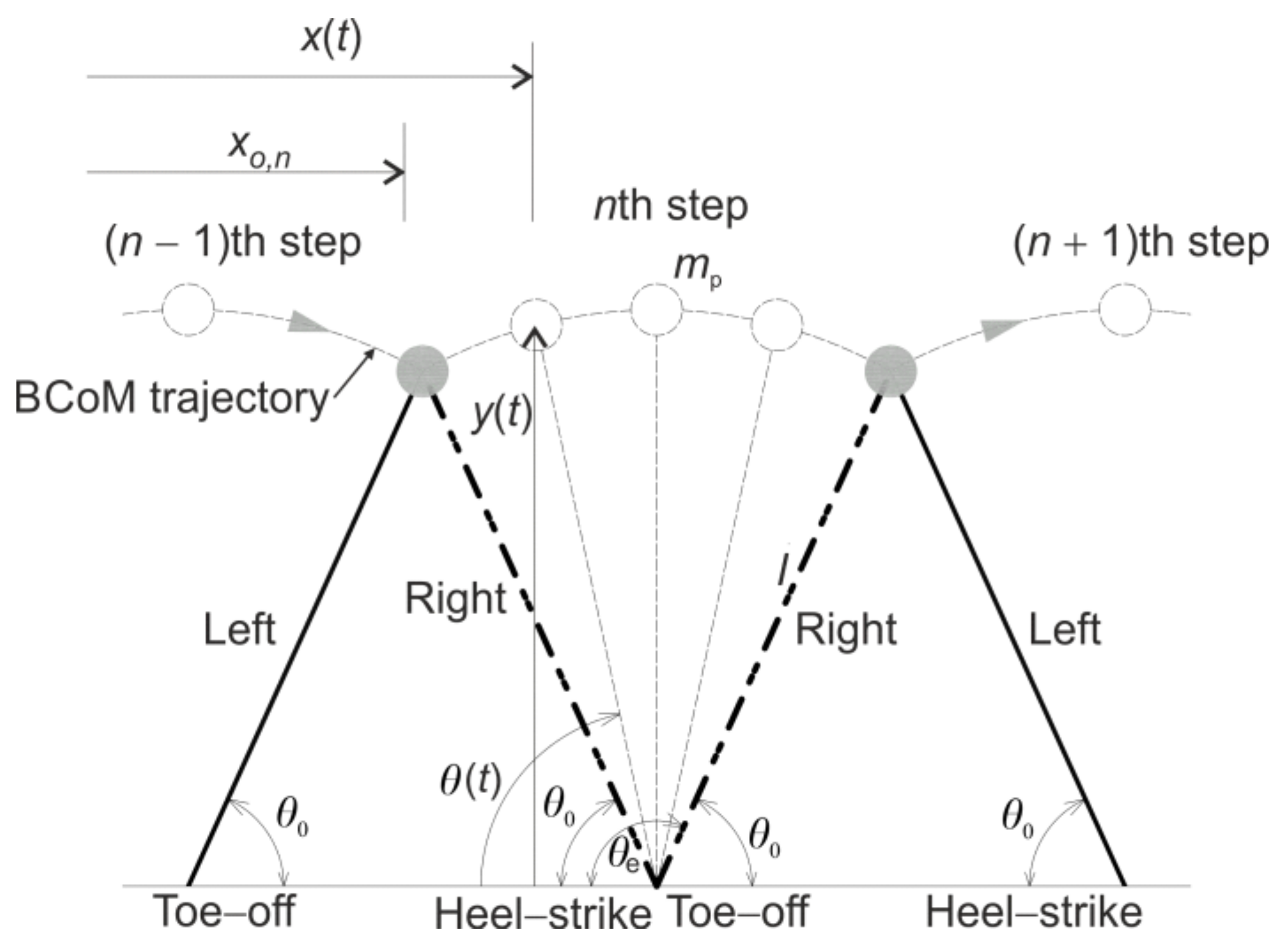
3.1. Inverted Pendulum Model
3.1.1. Model Inputs
3.1.2. Simulation Results
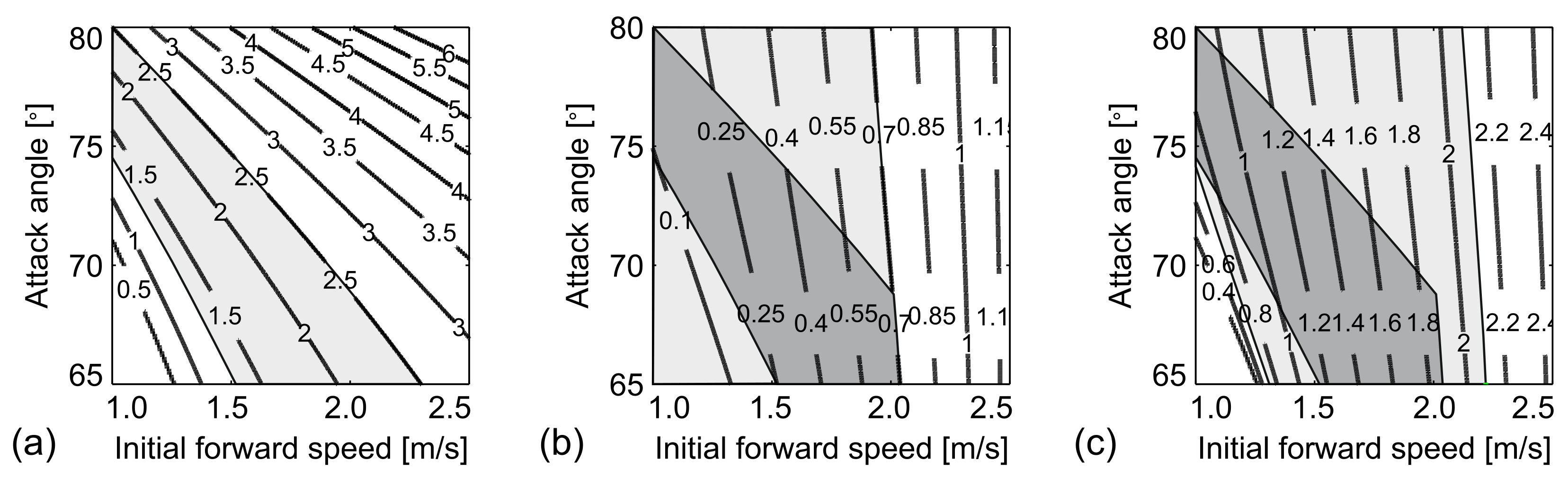

3.1.3. Dimensional Analysis
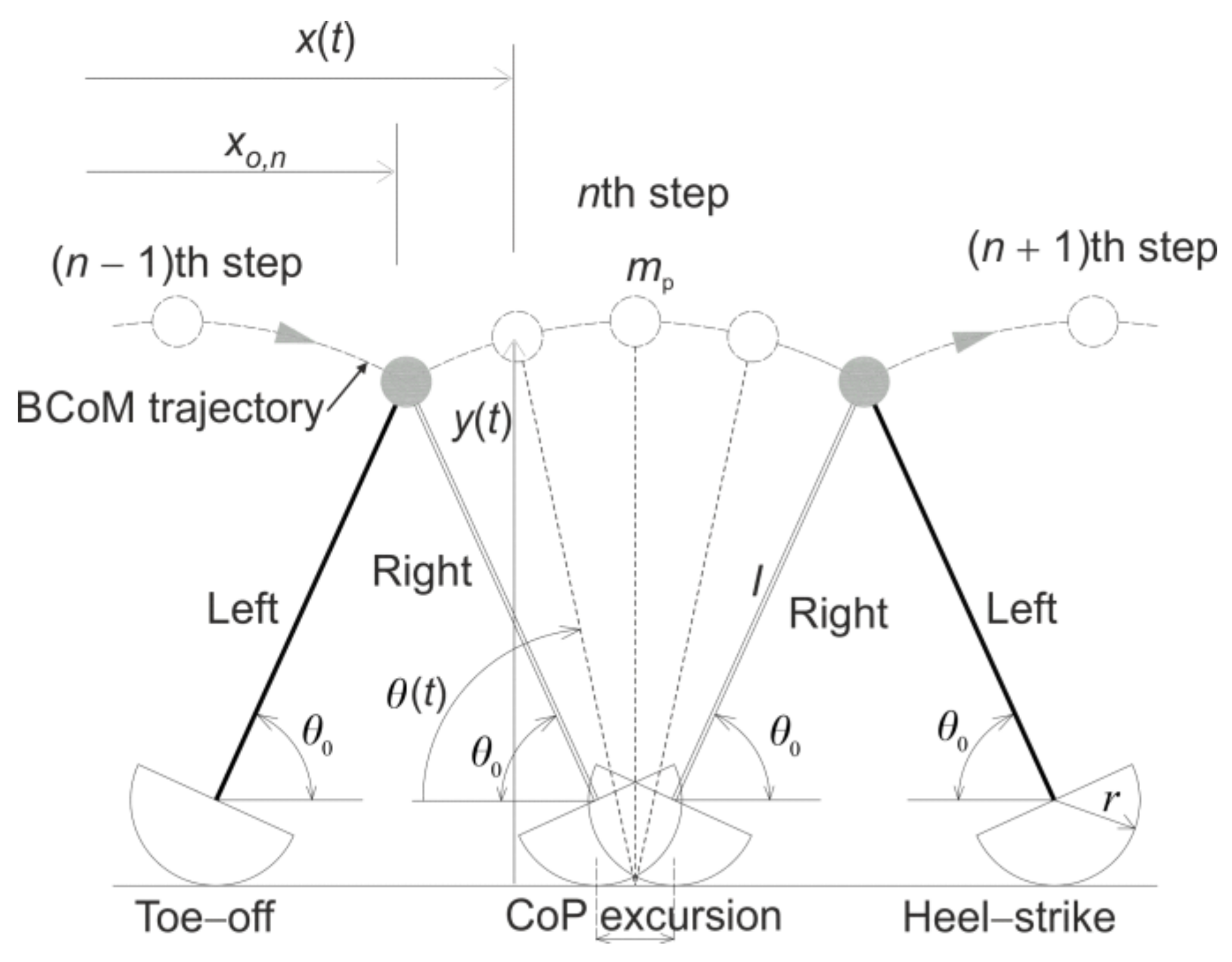
3.2. Inverted Pendulum with Rocker Foot Model
3.2.1. Model Inputs
3.2.2. Simulation Results

3.2.3. Dimensional Analysis
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- BS 5400; Steel, Concrete and Composite Bridges-Part 2: Specification for Loads; Appendix C: Vibration Serviceability Requirements for Foot and Cycle Track Bridges. British Standards Association: London, UK, 1978.
- Sétra. Footbridges: Assessment of Vibrational Behaviour of Footbridges under Pedestrian Loading: Technical Guide; Service d’Études Techniques des Routes et Autoroutes: Paris, France, 2006. [Google Scholar]
- NA to BS EN 1991-2:2003; UK National Annex to Eurocode 1: Actions on Structures-Part 2: Traffic Loads on Bridges. British Standards Institution: London, UK, 2008.
- Research Fund for Coal and Steel. Human Induced Vibrations of Steel Structures: Design of Footbridges—Guideline. RFS2-CT-2007-00033. 2009. Available online: https://www.coursehero.com/file/59133890/Footbridge-Backgroundpdf/ (accessed on 5 April 2013).
- Brownjohn, J.M.W.; Pavic, A.; Omenzetter, P. A spectral density approach for modelling continuous vertical forces on pedestrian structures due to walking. Can. J. Civ. Eng. 2004, 31, 65–77. [Google Scholar] [CrossRef]
- Živanović, S.; Pavic, A.; Reynolds, P. Probability based prediction of multi-mode vibration response to walking excitation. Eng. Struct. 2007, 29, 942–954. [Google Scholar] [CrossRef]
- Caprani, C.C.; Keogh, J.; Archbold, P.; Fanning, P. Enhancement factors for the vertical response of footbridges subjected to stochastic crowd loading. Comput. Struct. 2012, 102–103, 87–96. [Google Scholar] [CrossRef]
- Piccardo, G.; Tubino, F. Equivalent spectral model and maximum dynamic response for the serviceability analysis of footbridges. Eng. Struct. 2012, 40, 445–456. [Google Scholar] [CrossRef]
- Racic, V.; Brownjohn, J.M.W. Stochastic model of near periodic vertical loads due to humans walking. Adv. Eng. Inform. 2011, 25, 259–275. [Google Scholar] [CrossRef]
- Dallard, P.; Fitzpatrick, A.J.; Flint, A.; Le Bourva, S.; Low, A.; Ridsdill-Smith, R.M.; Willford, M. The London Millennium Footbridge. Struct. Eng. 2001, 79, 17–33. [Google Scholar]
- Macdonald, J.H.G. Lateral excitation of bridges by balancing pedestrians. Proc. R. Soc. Lond. Ser. A 2009, 465, 1055–1073. [Google Scholar] [CrossRef]
- Ingólfsson, E.T.; Georgakis, C.T.; Ricciardelli, F.; Jönsson, J. Experimental identification of pedestrian-induced lateral forces on footbridges. J. Sound Vib. 2011, 330, 1265–1284. [Google Scholar] [CrossRef]
- Carroll, S.P.; Owen, J.S.; Hussein, M.F.M. Experimental identification of the lateral human-structure interaction mechanism and assessment of the inverted-pendulum biomechanical model. J. Sound Vib. 2014, 333, 5865–5884. [Google Scholar] [CrossRef]
- McRobie, A.; Morghental, G.; Lasenby, J.; Ringer, M. Section model tests on human-structure lock-in. Proc. ICE-Bridge Eng. 2003, 156, 71–79. [Google Scholar] [CrossRef]
- Ricciardelli, F.; Mafrici MIngólfsson, E.T. Lateral Pedestrian-Induced Vibrations of Footbridges: Characteristics of Walking Forces. J. Bridge Eng. 2014, 19, 04014035. [Google Scholar] [CrossRef]
- Bocian, M.; Macdonald, J.H.G.; Burn, J.F. Biomechanically-inspired modelling of pedestrian-induced vertical self-excited forces. J. Bridge Eng. 2013, 18, 1336–1346. [Google Scholar] [CrossRef]
- Dang, H.V. Experimental and Numerical Modelling of Walking Locomotion on Vertically Vibrating Low-Frequency Structures. Ph.D. Thesis, University of Warwick, Coventry, UK, 2014. [Google Scholar]
- Ahmadi, E.; Caprani, C.; Živanović, S.; Heidarpour, A. Vertical ground reaction forces on rigid and vibrating surfaces for vibration serviceability assessment of structures. Eng. Struct. 2018, 172, 723–738. [Google Scholar] [CrossRef]
- Ahmadi, E.; Caprani, C.; Živanović, S.; Heidarpour, A. Assessment of human-structure interaction on a lively lightweight GFRP footbridge. Eng. Struct. 2019, 199, 109687. [Google Scholar] [CrossRef]
- Caprani, C.C.; Ahmadi, E. Formulation of human-structure interaction system models for vertical vibration. J. Sound Vib. 2016, 377, 346–367. [Google Scholar] [CrossRef]
- Bocian, M.; Macdonald, J.H.G.; Burn, J.F. Biomechanically inspired modelling of pedestrian-induced forces on laterally oscillating structures. J. Sound Vib. 2012, 331, 3914–3929. [Google Scholar] [CrossRef]
- Qin, J.W.; Law, S.S.; Yang, Q.S.; Yang, N. Pedestrian-bridge dynamic interaction, including human participation. J. Sound Vib. 2013, 332, 1107–1124. [Google Scholar] [CrossRef]
- Lin, B.; Zhang, Q.; Fan, F.; Shen, S. A damped bipedal inverted pendulum for human-structure interaction analysis. Appl. Math. Model. 2020, 87, 606–624. [Google Scholar] [CrossRef]
- Vega Ruiz, D.; Magluta, C.; Roitman, N. Experimental verification of biomechanical model of bipedal walking to simulate vertical loads induced by humans. Mech. Syst. Signal Process. 2022, 167, 108513. [Google Scholar] [CrossRef]
- Carroll, S.P.; Owen, J.S.; Hussein, M.F.M. Modelling crowd-bridge dynamic interaction with a discretely defined crowd. J. Sound Vib. 2012, 331, 2685–2709. [Google Scholar] [CrossRef]
- Whittle, M.W. Gait Analysis: An Introduction, 3rd ed.; Butterworth-Heinemann Elsevier: Oxford, UK, 2001. [Google Scholar]
- Inman, V.T.; Ralston, H.; Todd, F. Human Walking; Edwin Mellen Press Ltd.: Lewiston, NY, USA, 1989. [Google Scholar]
- Perry, J.; Burnfield, J.M. Gait Analysis: Normal and Pathological Function, 2nd ed.; SLACK Incorporated: Thorofare, NI, USA, 2010. [Google Scholar]
- Ayyappa, E. Normal human locomotion, Part 2: Motion, ground-reaction force and muscle activity. Prosthet. Orthot. 1997, 9, 4957. [Google Scholar] [CrossRef]
- Gard, S.A.; Childress, D.S. What determines the vertical displacement of the body during normal walking? J. Prosthet. Orthot. 2001, 13, 6467. [Google Scholar] [CrossRef]
- Saunders, J.B.D.M.; Inman, V.T.; Eberhart, H.D. The major determinants in normal and pathological gait. J. Bone Jt. Surg. 1953, 53, 543–558. [Google Scholar] [CrossRef]
- Cavagna, G.A.; Thys, H.; Zamboni, A. The sources of external work in level walking and running. J. Physiol. 1976, 262, 639657. [Google Scholar] [CrossRef]
- Whittle, M.W. Three-dimensional motion of the center of gravity of the body during walking. Hum. Mov. Sci. 1997, 16, 347–355. [Google Scholar] [CrossRef]
- Gard, S.A.; Miff, S.C.; Kuo, A.D. Comparison of kinematic and kinetic methods for computing the vertical motion of the body center of mass during walking. Hum. Mov. Sci. 2004, 22, 597610. [Google Scholar] [CrossRef]
- Blanchard, J.; Davies, B.L.; Smith, J.W. Design criteria and analysis for dynamic loading of footbridges. In Proceedings of the Symposium on Dynamic Behaviour of Bridges at the Transport and Road Research Laboratory, Crowthorne, UK, 19 May 1977; pp. 90–100. [Google Scholar]
- Rainer, J.H.; Pernica, G. Vertical dynamic forces from footsteps. Can. Acoust. 1986, 14, 12–21. [Google Scholar]
- Racic, V.; Pavic, A.; Brownjohn, J.M.W. Experimental identification and analytical modelling of human walking forces: Literature review. J. Sound Vib. 2009, 326, 1–49. [Google Scholar] [CrossRef]
- Kerr, S.C. Human Induced Loading on Staircases. Ph.D. Thesis, University College London, London, UK, 1998. [Google Scholar]
- Pedersen, L.; Frier, C. Sensitivity of footbridge vibrations to stochastic walking parameters. J. Sound Vib. 2010, 29, 2683–2701. [Google Scholar] [CrossRef]
- Živanović, S.; Pavic, A.; Reynolds, P. Vibration serviceability of footbridges under human-induced excitation: A literature review. J. Sound Vib. 2005, 279, 1–74. [Google Scholar] [CrossRef]
- Matsumoto, Y.; Nishioka, T.; Shiojiri, H.; Matsuzaki, K. Dynamic design of footbridges. IABSE Proc. 1978, P-17/78, 1–15. [Google Scholar]
- Pachi, A.; Ji, T. Frequency and velocity of people walking. Struct. Eng. 2005, 83, 36–40. [Google Scholar]
- Kasperski, K.; Sahnaci, C. Serviceability of Pedestrian Structures. In Proceedings of the IMAC-XXV, Orlando, FL, USA, 19–22 February 2007. [Google Scholar]
- Ricciardelli, F.; Briatico, C.; Ingólfsson, E.T.; Georgakis, C.T. Experimental validation and calibration of pedestrian loading models for footbridges. In Proceedings of the International Conference of Experimental Vibration Analysis for Civil Engineering Structures, Porto, Portugal, 24–26 October 2007. [Google Scholar]
- Živanović, S.; Racic, V.; El-Bahnasy, I.; Pavic, A. Statistical characterization of parameters defining human walking as observed on an indoor passerelle. In Proceedings of the International Conference of Experimental Vibration Analysis for Civil Engineering Structures, Porto, Portugal, 24–26 October 2007. [Google Scholar]
- Živanović, S. Benchmark footbridge for vibration serviceability assessment under vertical component of pedestrian load. J. Struct. Eng. 2012, 138, 1193–1202. [Google Scholar] [CrossRef]
- McGeer, T. Passive dynamic walking. Int. J. Robot. Res. 1990, 9, 6282. [Google Scholar] [CrossRef]
- Geyer, H. Simple Models of Legged Locomotion Based on Compliant Limb Behavior. Ph.D. Thesis, Friedrich-Schiller-Universität, Jena, Germany, 2005. [Google Scholar]
- Whittington, B.R.; Thelen, D.G. A simple mass-spring model with roller feet can induce the ground reactions observed in human walking. J. Biomech. Eng. 2009, 131, 011013. [Google Scholar] [CrossRef]
- Kim, S.; Park, S. Leg stiffness increases with speed to modulate gait frequency and propulsion energy. J. Biomech. 2011, 44, 1253–1258. [Google Scholar] [CrossRef]
- Goldstein, H. Classical Mechanics, 2nd edition; Addison-Wesley: San Francisco, CA, USA, 1980. [Google Scholar]
- National Health Service (NHS). Health Survey for England, United Kingdom. 2010. Available online: https://digital.nhs.uk/data-and-information/publications/statistical/health-survey-for-england/health-survey-for-england-2009-trend-tables (accessed on 5 April 2013).
- Pheasant, S.T. Anthropometric estimates for British civilian adults. Ergonomics 1982, 25, 993–1001. [Google Scholar] [CrossRef]
- Hof, A.L.; Gazendam, M.G.J.; Sinke, W.E. The condition for dynamic stability. J. Biomech. 2005, 38, 1–8. [Google Scholar] [CrossRef]
- Mathworks Inc. MATLAB, version 9.11.0.1769968 (R2021b); Matworks: Natick, MA, USA, 2021. [Google Scholar]
- Lee, C.R.; Farley, C.T. Determinants of the center of mass trajectory in human walking and running. J. Exp. Biol. 1998, 201, 2935–2944. [Google Scholar] [CrossRef]
- Gard, S.A.; Childress, D.S. The effect of pelvic list on the vertical displacement of the trunk during normal walking. Gait Posture 1997, 5, 233–238. [Google Scholar] [CrossRef]
- Gard, S.A.; Childress, D.S. The influence of stance-phase knee flexion on the vertical displacement of the trunk during normal walking. Arch. Phys. Med. Rehabil. 1999, 80, 26–32. [Google Scholar] [CrossRef]
- Hansen, A.; Gard, S.; Childress, D. The determination of foot/ankle roll-over shape: Clinical and research applications. In Proceedings of the Pediatric Gait: A New Millennium in Clinical Care and Motion Analysis Technology, Chicago, IL, USA, 22 July 2000; pp. 159–165. [Google Scholar] [CrossRef]
- Adamczyk, P.G.; Collins, S.H.; Kuo, A.D. The advantages of a rolling foot in human walking. J. Exp. Biol. 2006, 209, 3953–3963. [Google Scholar] [CrossRef]
- Gao, Y.; Wang, J.; Liu, M. The Vertical Dynamic Properties of Flexible Footbridges under Bipedal Crowd Induced Excitation. Appl. Sci. 2017, 7, 677. [Google Scholar] [CrossRef]


| Parameter | Dimensionless Parameter |
|---|---|
| Step frequency | |
| Step length | |
| Average speed | |
| Dynamic load factor DLF1 | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Živanović, S.; Lin, B.; Dang, H.V.; Zhang, S.; Ćosić, M.; Caprani, C.; Zhang, Q. Evaluation of Inverted-Pendulum-with-Rigid-Legs Walking Locomotion Models for Civil Engineering Applications. Buildings 2022, 12, 1216. https://doi.org/10.3390/buildings12081216
Živanović S, Lin B, Dang HV, Zhang S, Ćosić M, Caprani C, Zhang Q. Evaluation of Inverted-Pendulum-with-Rigid-Legs Walking Locomotion Models for Civil Engineering Applications. Buildings. 2022; 12(8):1216. https://doi.org/10.3390/buildings12081216
Chicago/Turabian StyleŽivanović, Stana, Bintian Lin, Hiep Vu Dang, Sigong Zhang, Mladen Ćosić, Colin Caprani, and Qingwen Zhang. 2022. "Evaluation of Inverted-Pendulum-with-Rigid-Legs Walking Locomotion Models for Civil Engineering Applications" Buildings 12, no. 8: 1216. https://doi.org/10.3390/buildings12081216
APA StyleŽivanović, S., Lin, B., Dang, H. V., Zhang, S., Ćosić, M., Caprani, C., & Zhang, Q. (2022). Evaluation of Inverted-Pendulum-with-Rigid-Legs Walking Locomotion Models for Civil Engineering Applications. Buildings, 12(8), 1216. https://doi.org/10.3390/buildings12081216






