Experimental and Numerical Study on an Innovative Trapezoidal-Shaped Damper to Improve the Behavior of CBF Braces
Abstract
1. Introduction
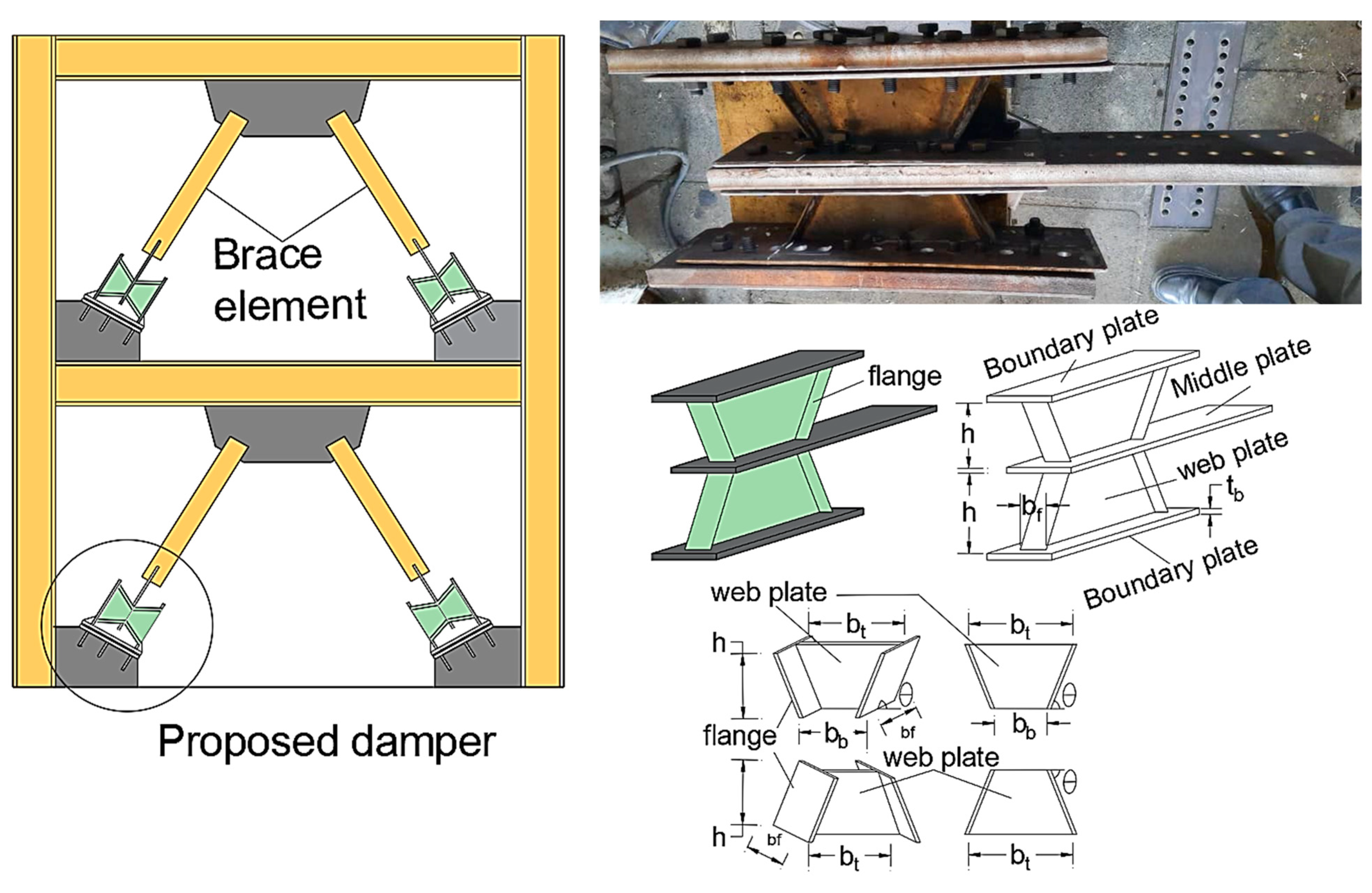
2. The Trapezoidal Damper
2.1. Construction Detailing
2.2. The Behavior of the Proposed Damper
2.3. Design of the Damper and Elements Outside the Damper
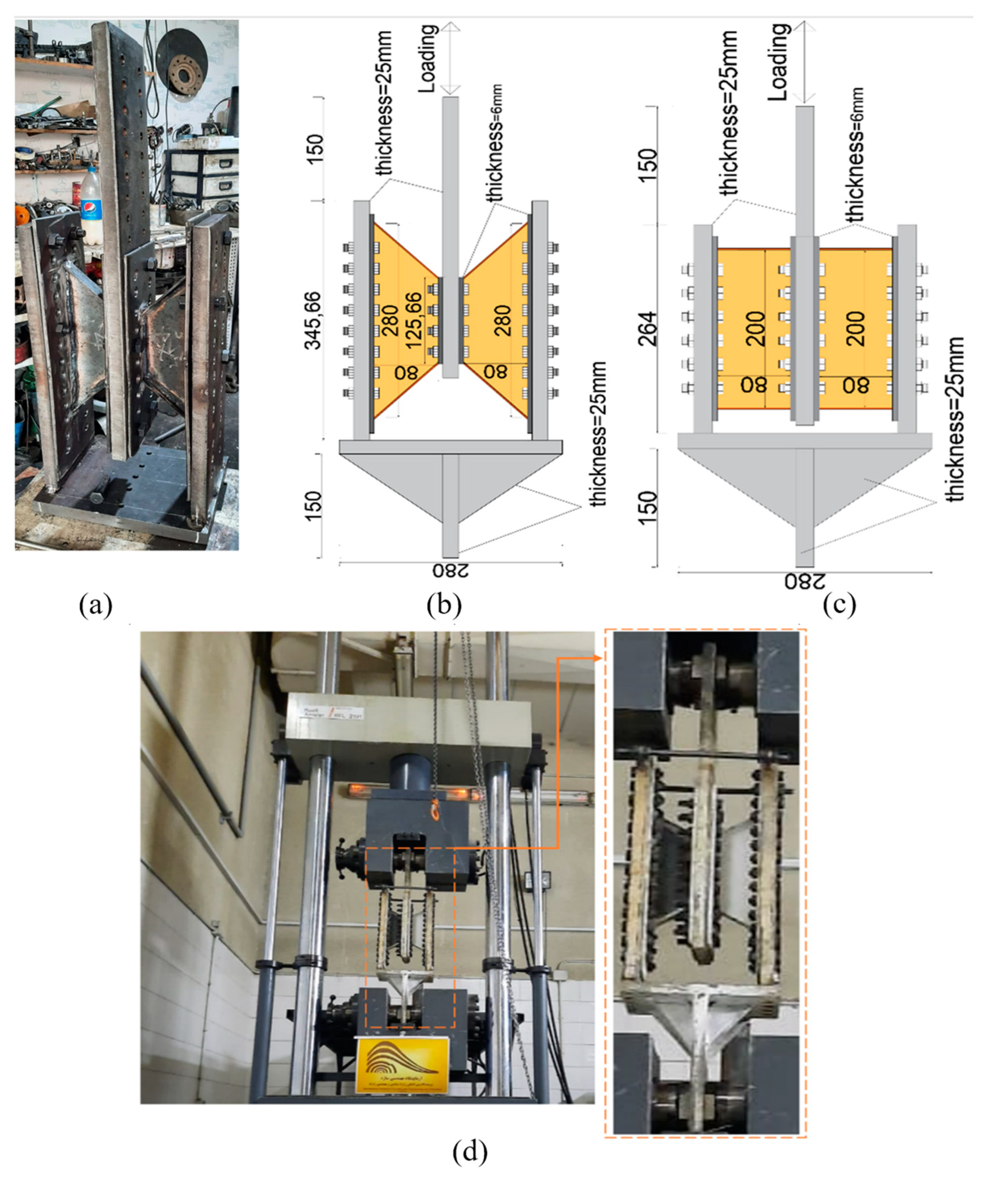
3. Experimental Study
3.1. Preparing the Specimens
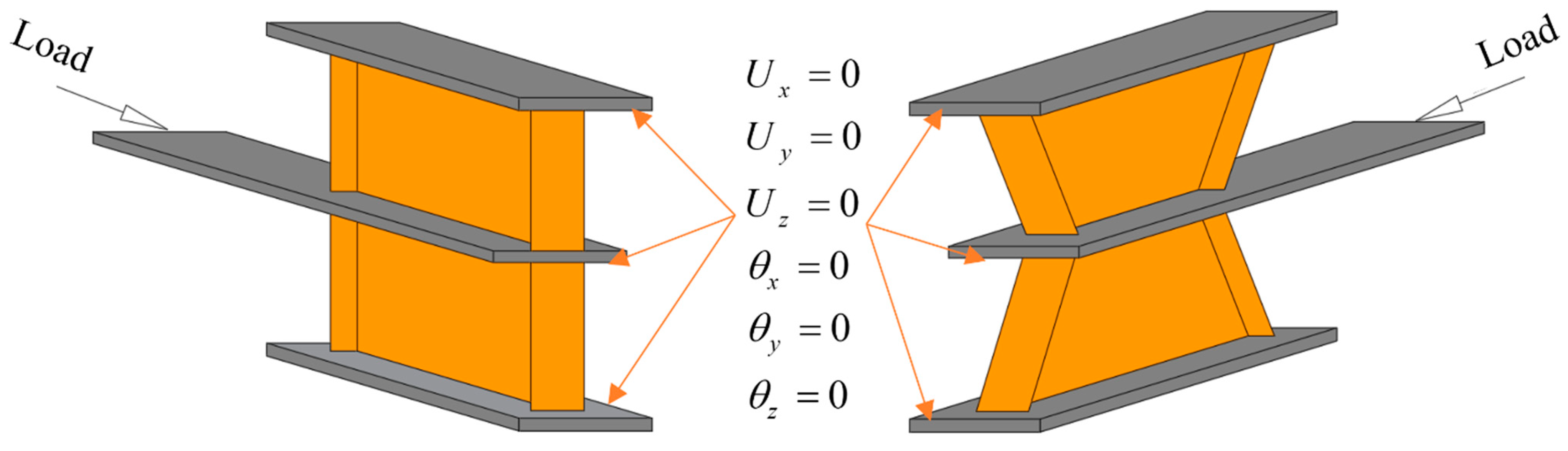
3.2. Boundary Condition
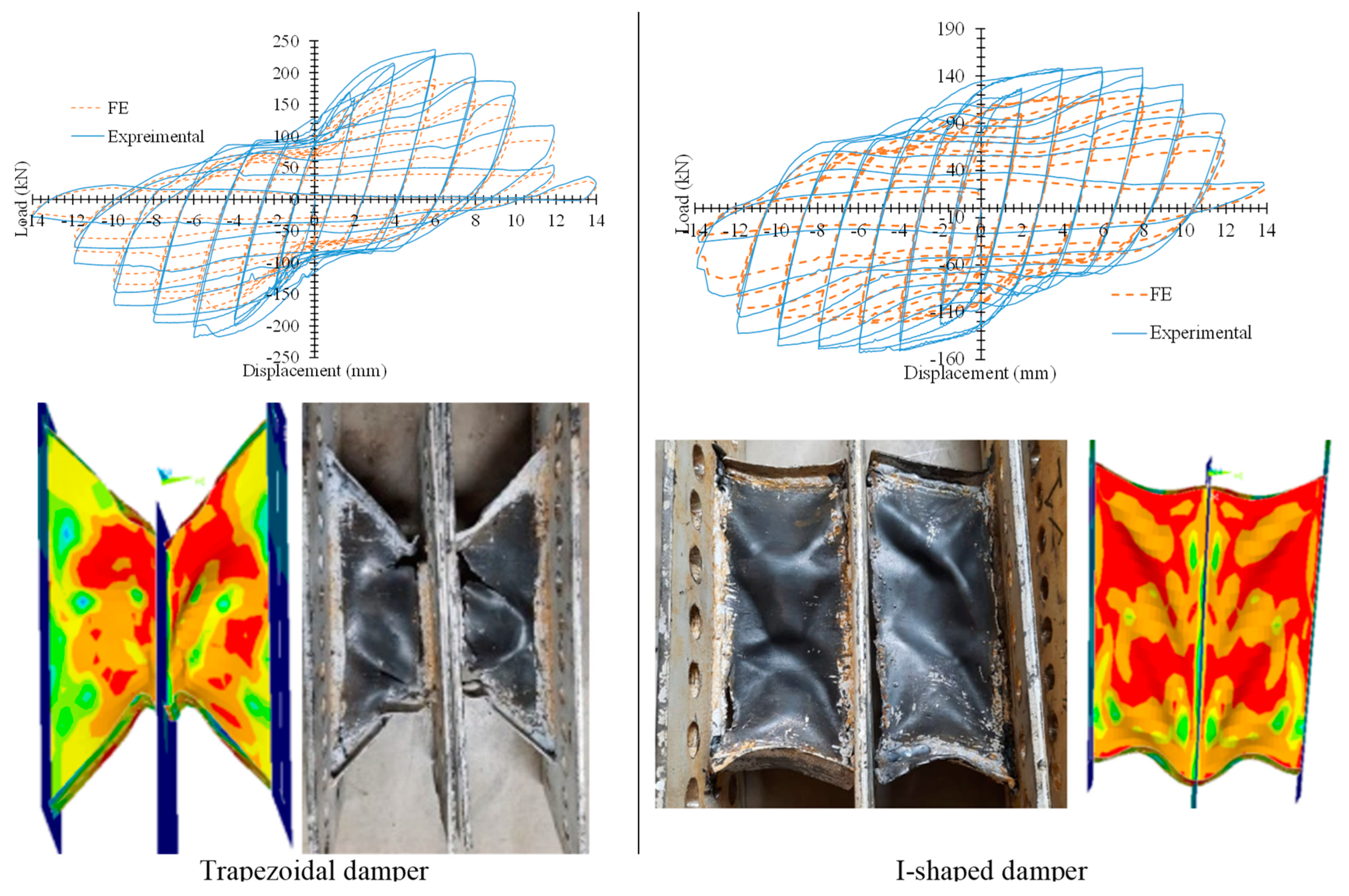
3.3. Experimental Results
3.3.1. Specimen Performance
3.3.2. Structural Parameters
4. Numerical Study
4.1. Finite Element (FE) Modeling
4.2. Verification of FE Results
5. Numerical Results
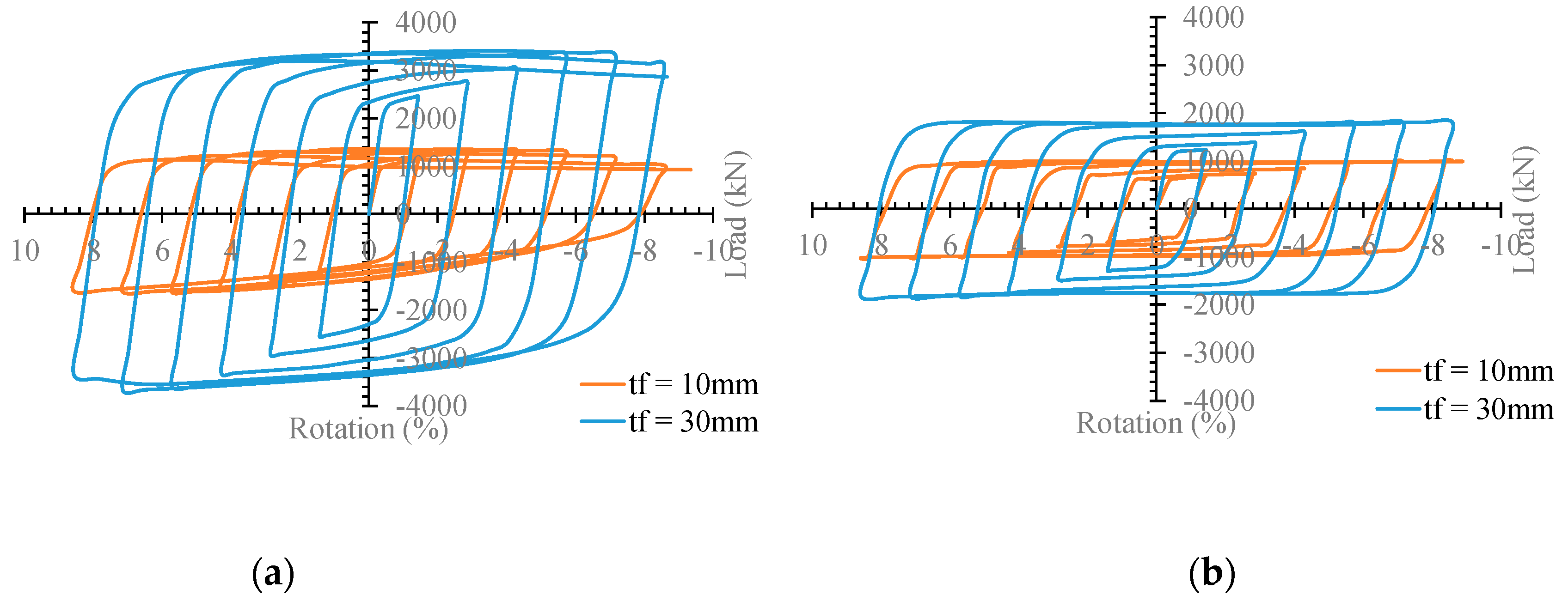
5.1. Hysteresis Curves
5.2. Comparing the I-Shaped Damper with the Trapezoidal Damper
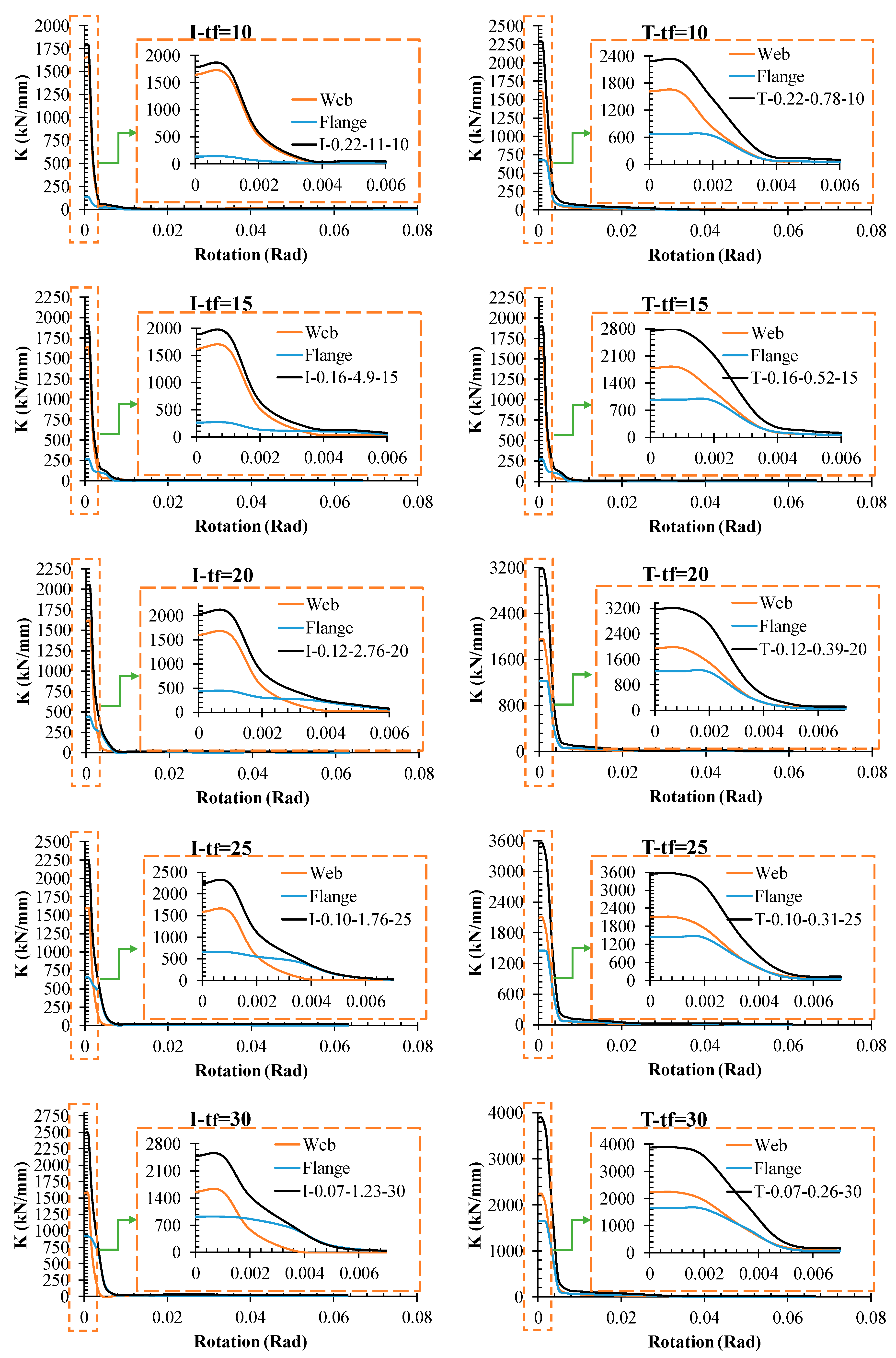
5.3. Stiffness
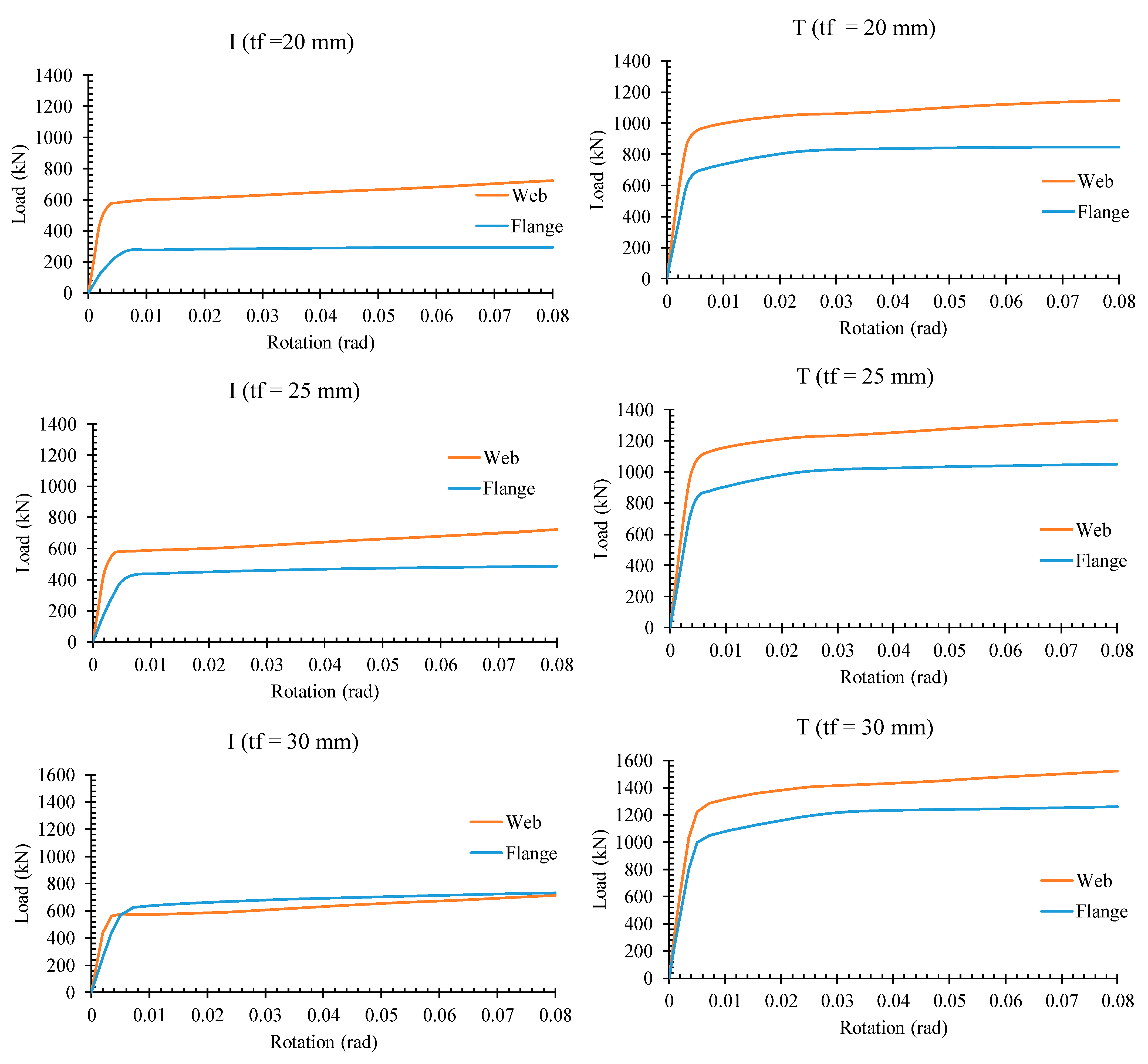
5.4. Share of Flanges on the Applied Load
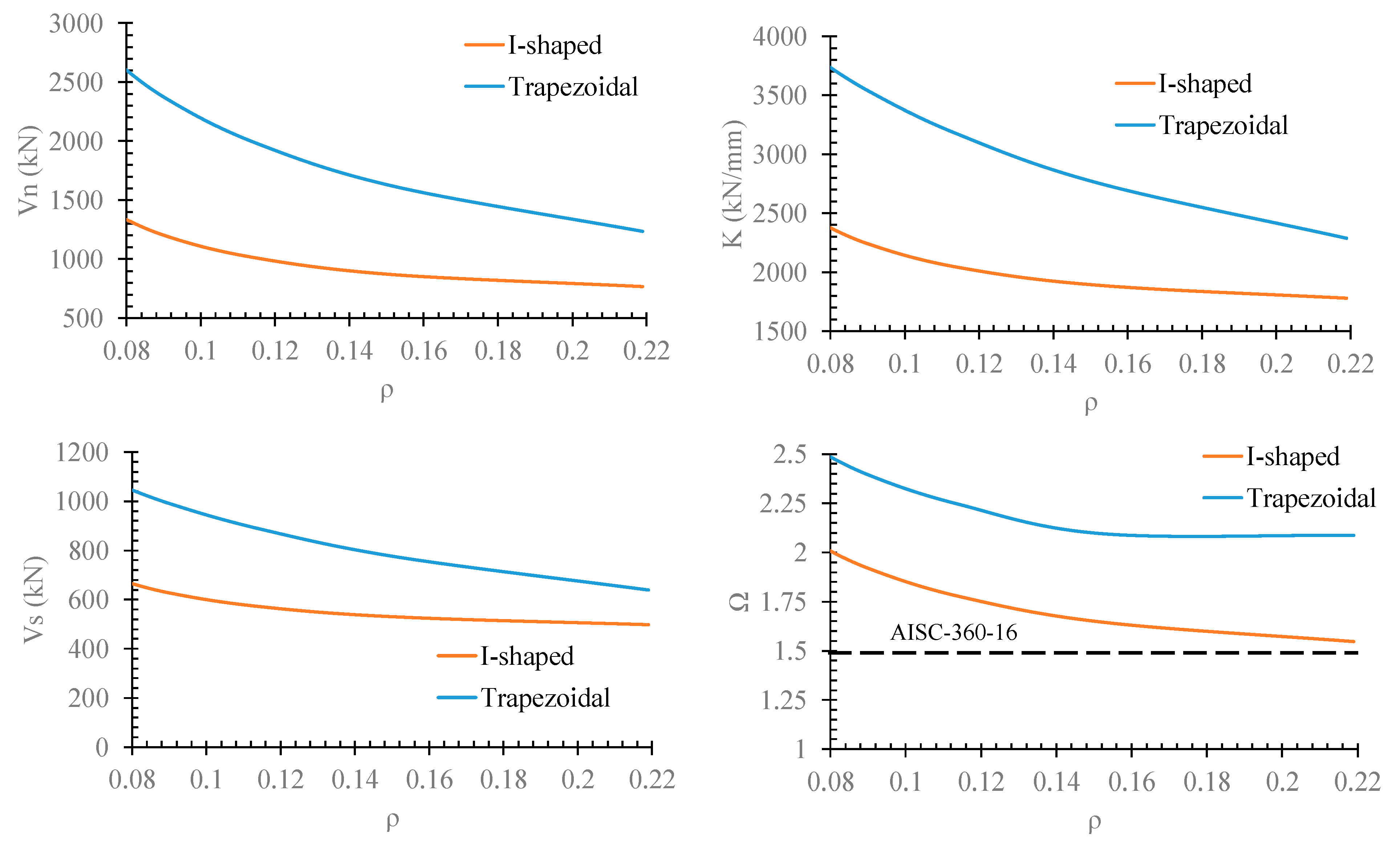
5.5. Effect of ρ
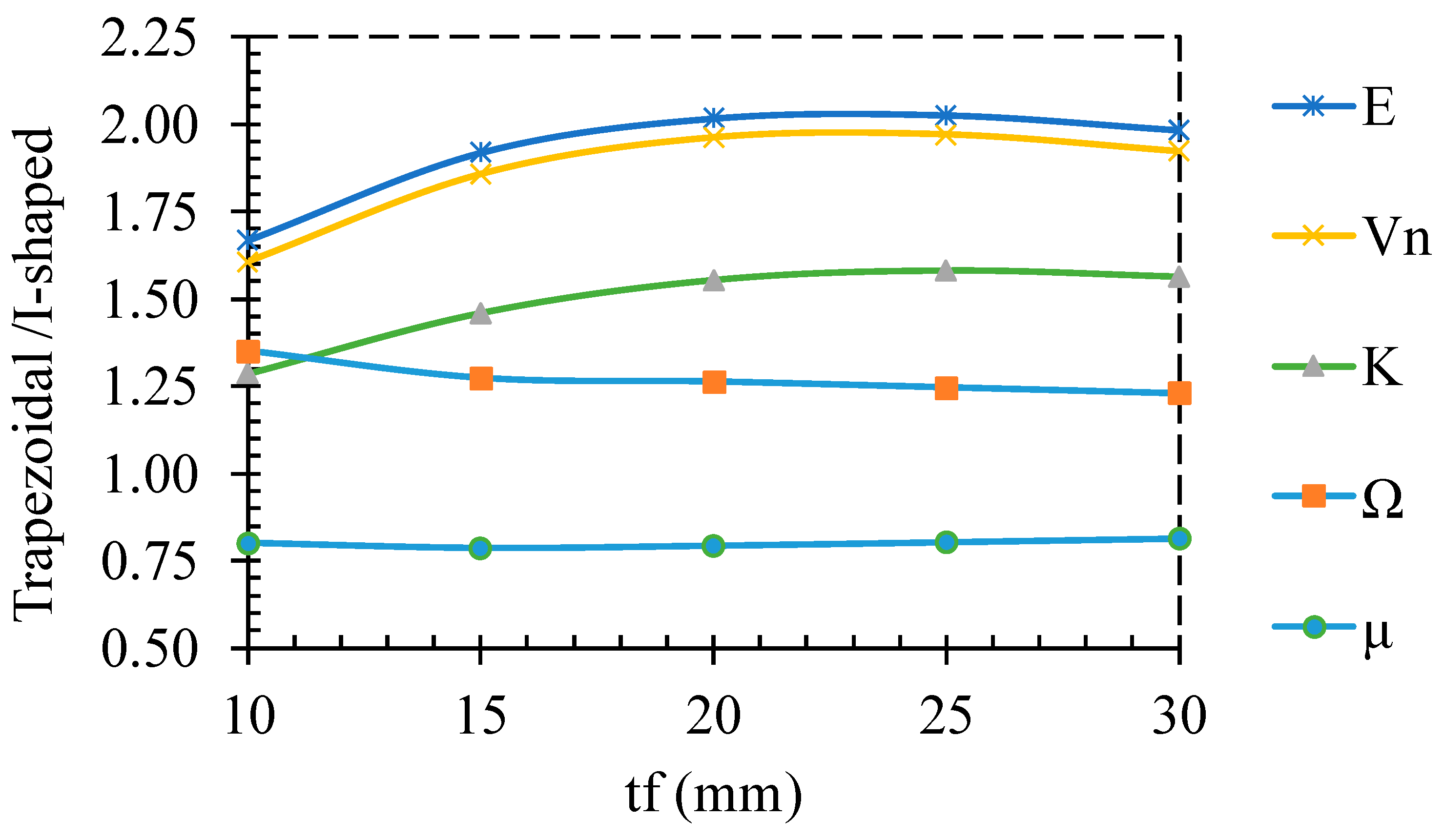
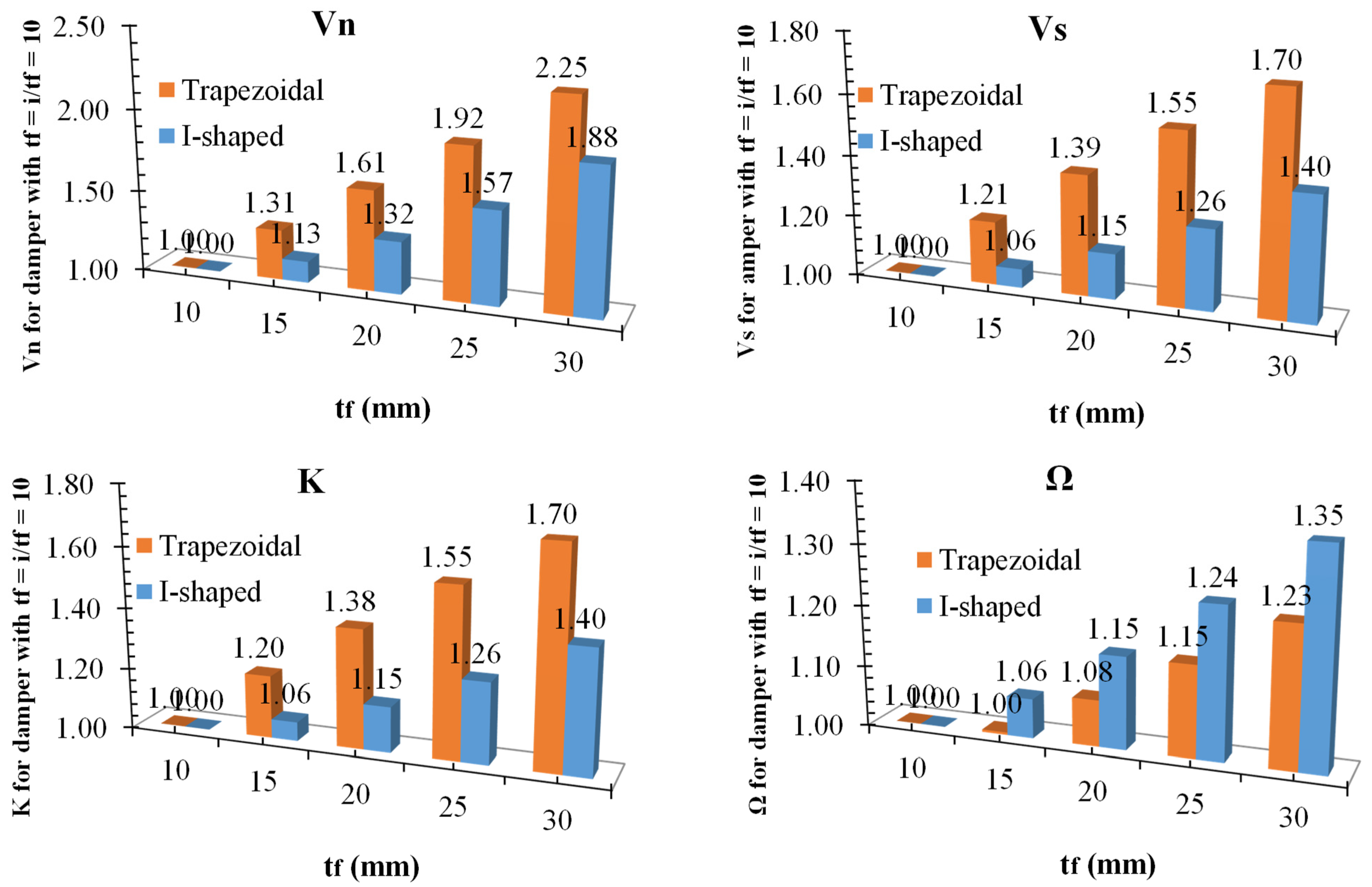
5.6. Effect of tf
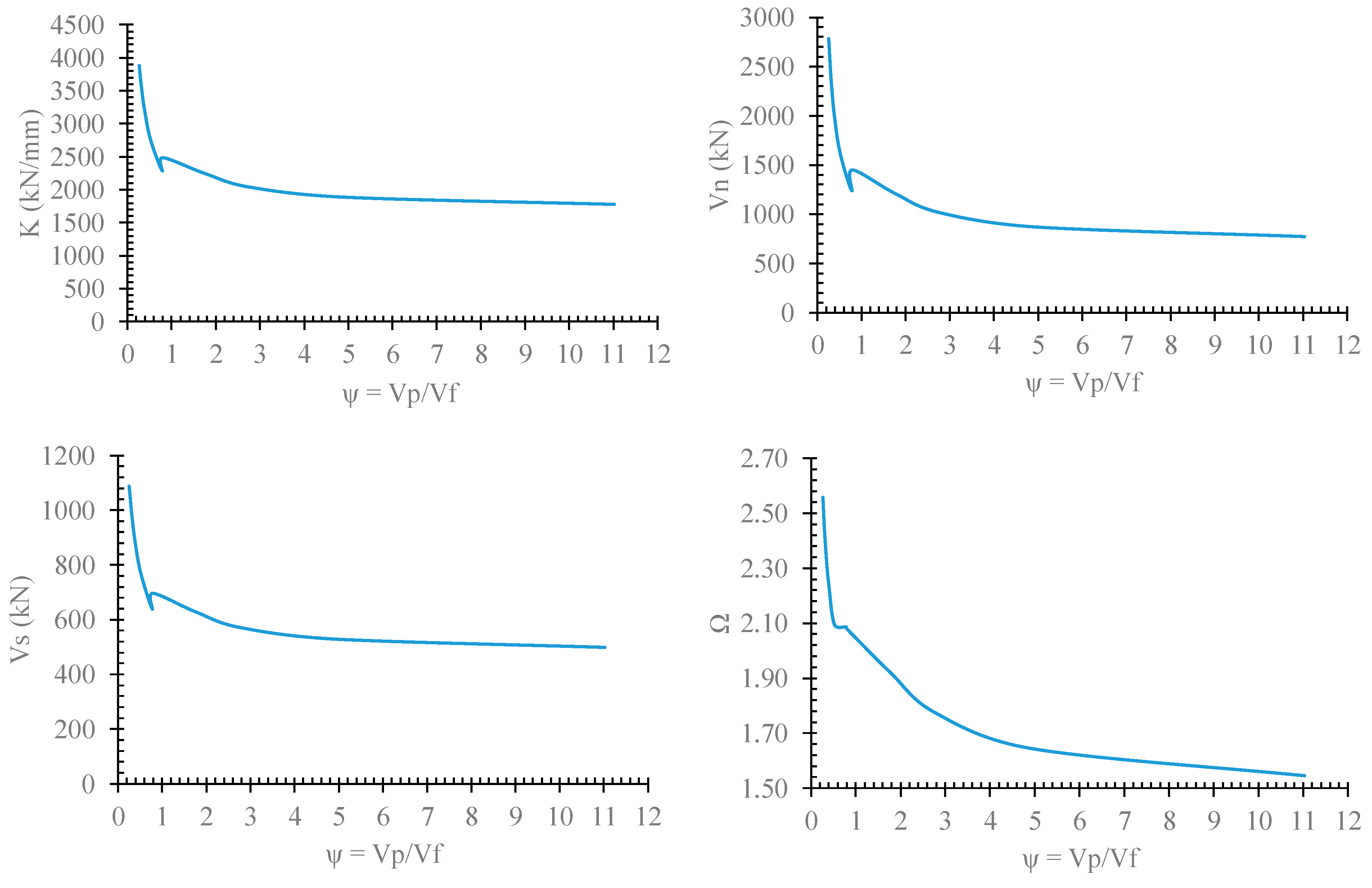
5.7. Effect of ψ
5.8. Overstrength
5.9. State of Yielding
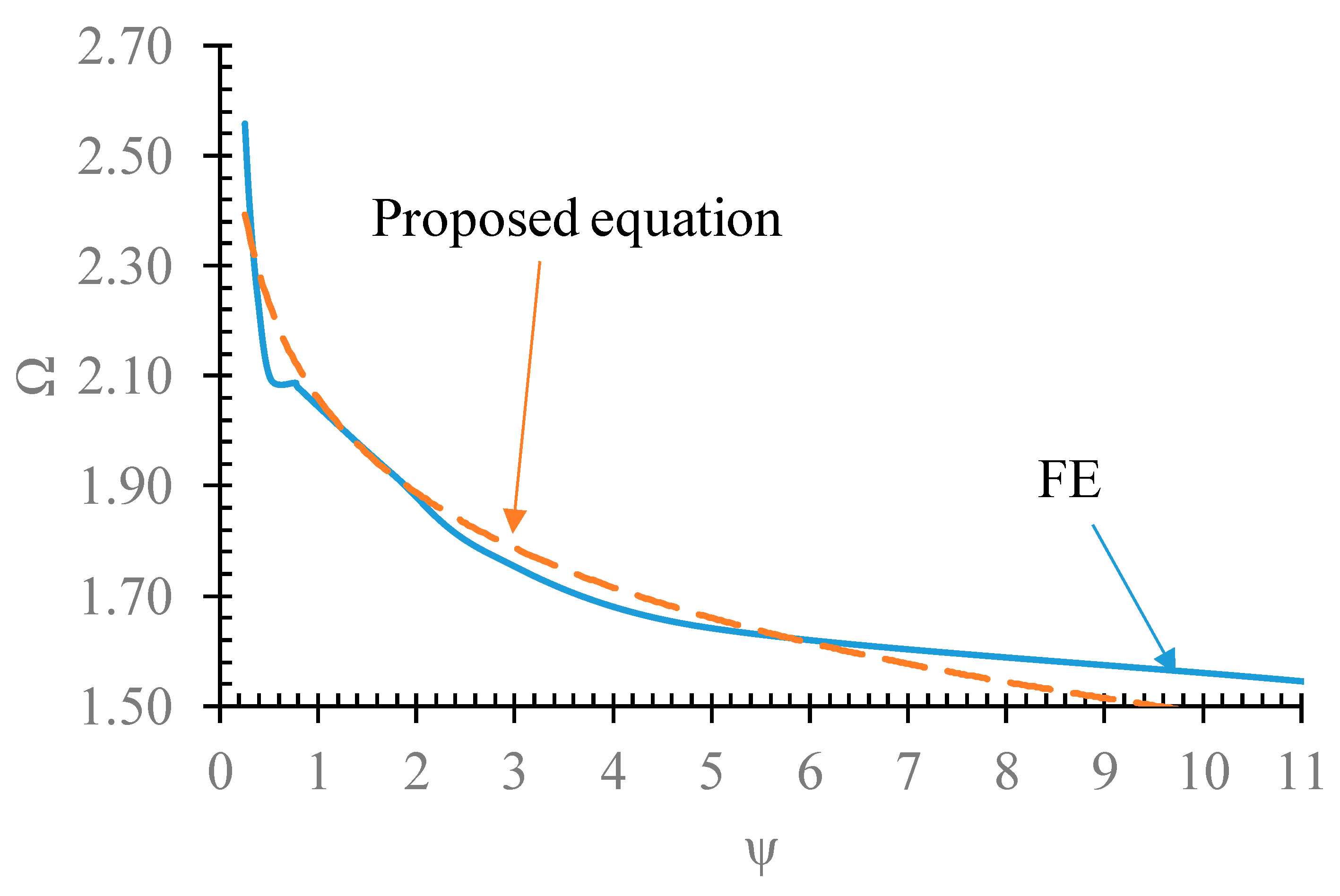
5.10. Accuracy of the Proposed Damper Equations
6. Conclusions
- -
- The experimental and numerical study indicated that the trapezoidal dampers have greater ultimate strength, elastic stiffness, dissipating energy, and overstrength than I-shaped.
- -
- Although the flange plate in both dampers affected their behavior, the thicknesses of the flange plate in the trapezoidal damper have more effect on the behavior of the damper than the I-shaped damper.
- -
- The amplified factor, 1.25 Ry, to the design of the elements outside the damper is less than the ultimate strength. Therefore, to design elements outside the damper, using is suggested. In this relation, the overstrength is proposed as .
- -
- Numerical results indicated that when using a trapezoidal damper instead of an I-shaped damper, the ultimate strength is between 61% and 92%, elastic stiffness (K) between 29% and 56%, and the energy dissipating (E) between 1.67 and 2.03 times are improved.
- -
- The stiffness of the damper coincides together around the rotation of 0.006 Rad. In the other words, although the types of damper (I-shaped or trapezoidal) and thickness of the flange plate are effective on the stiffness, they do not have a considerable effect on the stiffness after rotation more than 0.006 Rad.
- -
- For an I-shaped damper, 92.5% of the capacity of the damper is provided by a web plate at the beginning of the loading when a thin flange is used (7.5% by flange plates). By increasing the rotation, it is reduced to 89% (11% by flange plates). However, this is 70% at the beginning of the applied load and 62% after hinge formation in the web plate for the trapezoidal damper.
- -
- By increasing the tf from 10 mm to 30 mm, the share of web plate in load bearing at the beginning and after hinge formation in the damper, respectively, change from 70% and 62% (30% and 38% for flange plates) to 57% and 55% (43% and 45% for flange plates).
- -
- Comparing the effect of tf on the parameters Vn, K, and Ω reveals that the tf has the most effect on the Vn for both dampers. However, it has the same effect on K and Ω for the trapezoidal damper. In an I-shaped damper, the effect of tf on the Ω is more than K.
- -
- Recommendations for future work: To complete the research in this field, it is recommended to investigate the effect of the proposed damper to improve the behavior of reinforced concrete (RC) systems, especially for existing structures. Since the damper improves the behavior of the CBF system, can be built easily, and does not impose much cost on a structure, a comprehensive study is needed to achieve an optimum configuration between the damper and RC frame.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ghamari, A.; Jeong, S.H. A proposal for improving the behavior of CBF braces using an innovative flexural mechanism damper, an experimental and numerical study. Steel Compos. Struct. 2022, 45, 455–466. [Google Scholar] [CrossRef]
- Park, H.-Y.; Oh, S.-H. Structural performance of beam system with T-stub type slit damper. Eng. Struct. 2020, 205, 109858. [Google Scholar] [CrossRef]
- Heidari, P.S.; Jazany, R.A.; Kayhani, H. An Investigation on Bracing Configuration Effects on Behavior of Concentrically Braced Steel Frames. World Appl. Sci. J. 2012, 17, 1095–1108. [Google Scholar]
- Yang, T.Y.; Sheikh, H.; Tobber, L. Influence of the Brace Configurations on the Seismic Performance of Steel Concentrically Braced Frames. Front. Built Environ. 2019, 5, 27. [Google Scholar] [CrossRef]
- Jaisee, S.; Yue, F.; Ooi, Y.H. A state-of-the-art review on passive friction dampers and their applications. Eng. Struct. 2021, 235, 112022. [Google Scholar] [CrossRef]
- De Domenico, D.; Ricciardi, G.; Takewaki, I. Design strategies of viscous dampers for seismic protection of building structures: A review. Soil Dyn. Earthq. Eng. 2019, 118, 144–165. [Google Scholar] [CrossRef]
- Vasdravellis, G.; Valente, M.; Castiglioni, C. Dynamic response of composite frames with different shear connection degree. J. Constr. Steel Res. 2009, 65, 2050–2061. [Google Scholar] [CrossRef]
- Symans, M.D.; Charney, F.A.; Whittaker, A.S.; Constantinou, M.C.; Kircher, C.A.; Johnson, M.W.; McNamara, R.J. Energy Dissipation Systems for Seismic Applications: Current Practice and Recent Developments. Eng. Struct. 2008, 134, 3–21. [Google Scholar] [CrossRef]
- Hu, S.; Wang, W.; Qu, B. Seismic evaluation of low-rise steel building frames with self-centering energy-absorbing rigid cores designed using a force-based approach. Eng. Struct. 2020, 204, 110038. [Google Scholar] [CrossRef]
- Ghamari, A.; Almasi, B.; Kim, C.-H.; Jeong, S.-H.; Hong, K.-J. An Innovative Steel Damper with a Flexural and Shear–Flexural Mechanism to Enhance the CBF System Behavior: An Experimental and Numerical Study. Appl. Sci. 2021, 11, 11454. [Google Scholar] [CrossRef]
- Xia, C.; Hanson, R.D. Influence of ADAS Element Parameters on Building Seismic Response. Eng. Struct. 1992, 118, 1903–1918. [Google Scholar] [CrossRef]
- Khazaei, M. Investigation on Dynamics Nonlinear Analysis of Steel Frames with Steel Dampers. Procedia Eng. 2013, 54, 401–412. [Google Scholar] [CrossRef][Green Version]
- Tsai, K.C.; Chen, H.W.; Hong, C.P.; Su, Y.F. Design of steel triangular plate energy absorbers for seis-mic-resistant construction. Earthq. Spectra 1993, 9, 505–528. [Google Scholar] [CrossRef]
- Gray, M.G.; Christopoulos, C.; Packer, J.A. Design and Full-Scale Testing of a Cast Steel Yielding Brace System in a Braced Frame. J. Struct. Eng. 2017, 143, 04016210. [Google Scholar] [CrossRef]
- Han, Q.; Jia, J.; Xu, Z.; Bai, Y.; Song, N. Experimental evaluation of hysteretic behavior of rhombic steel plate dampers. Adv. Mech. Eng. 2014, 9, 99. [Google Scholar] [CrossRef]
- Guo, W.; Wang, X.; Yu, Y.; Chen, X.; Li, S.; Fang, W.; Zeng, C.; Wang, Y.; Bu, D. Experimental study of a steel damper with X-shaped welded pipe halves. J. Constr. Steel Res. 2020, 170, 106087. [Google Scholar] [CrossRef]
- Oh, S.-H.; Kim, Y.-J.; Ryu, H.-S. Seismic performance of steel structures with slit dampers. Eng. Struct. 2009, 31, 1997–2008. [Google Scholar] [CrossRef]
- Lee, J.; Kim, J. Development of box-shaped steel slit dampers for seismic retrofit of building structures. Eng. Struct. 2017, 150, 934–946. [Google Scholar] [CrossRef]
- Wang, Y.-P.; Chien, C.-S.C. A study on using pre-bent steel strips as seismic energy-dissipative devices. Earthq. Eng. Struct. Dyn. 2009, 38, 1009–1026. [Google Scholar] [CrossRef]
- Hsu, H.L.; Halim, H. Improving seismic performance of framed structures with steel curved dampers. Eng. Struct. 2017, 130, 99–111. [Google Scholar] [CrossRef]
- Okazaki, T.; Engelhardt, M.D.; Hong, J.-K.; Uang, C.-M.; Drolias, A. Improved Link-to-Column Connections for Steel Eccentrically Braced Frames. J. Struct. Eng. 2015, 141, 04014201. [Google Scholar] [CrossRef]
- Ghamari, A.; Haeri, H.; Khaloo, A.; Zhu, Z. Improving the hysteretic behavior of Concentrically Braced Frame (CBF) by a proposed shear damper. Steel Compos. Struct. 2019, 30, 383–392. [Google Scholar]
- Roeder, C.W.; Popov, E.P. Inelastic behavior of eccentrically braced steel frames under cyclic load-ings. STIN 1977, 78, 20375. [Google Scholar]
- Roeder, C.W.; Popov, E.P. Eccentrically Braced Steel Frames for Earthquakes. J. Struct. Div. 1978, 104, 391–412. [Google Scholar] [CrossRef]
- Richards, P.W.; Uang, C.-M. Effect of Flange Width-Thickness Ratio on Eccentrically Braced Frames Link Cyclic Rotation Capacity. J. Struct. Eng. 2005, 131, 1546–1552. [Google Scholar] [CrossRef]
- Zahrai, S.M. Cyclic testing of chevron braced steel frames with IPE shear panels. Steel Compos. Struct. 2015, 19, 1167–1184. [Google Scholar] [CrossRef]
- Ghadami, A.; Pourmoosavi, G.; Ghamari, A. Seismic design of elements outside of the short low-yield-point steel shear links. J. Constr. Steel Res. 2021, 178, 106489. [Google Scholar] [CrossRef]
- Deng, K.; Pan, P.; Sun, J.; Liu, J.; Xue, Y. Shape optimization design of steel shear panel dampers. J. Constr. Steel Res. 2014, 99, 187–193. [Google Scholar] [CrossRef]
- Ghamari, A.; Kim, C.; Jeong, S. Development of an innovative metallic damper for concentrically braced frame systems based on experimental and analytical studies. Struct. Des. Tall Spéc. Build. 2022, 31, e1927. [Google Scholar] [CrossRef]
- Giannuzzi, D.; Ballarini, R.; Huckelbridge, A.; Pollino, M.; Valente, M. Braced Ductile Shear Panel: New Seismic-Resistant Framing System. Eng. Struct. 2014, 140, 04013050. [Google Scholar] [CrossRef]
- Taiyari, F.; Mazzolani, F.M.; Bagheri, S. A proposal for energy dissipative braces with U-shaped steel strips. J. Constr. Steel Res. 2019, 154, 110–122. [Google Scholar] [CrossRef]
- Mahyari, S.L.; Riahi, H.T.; Hashemi, M. Investigating the analytical and experimental performance of a pure torsional yielding damper. J. Constr. Steel Res. 2019, 161, 385–399. [Google Scholar] [CrossRef]
- He, Z.; Chen, Q. Upgrading the seismic performance of underground structures by introducing lead-filled steel tube dampers. Tunn. Undergr. Space Technol. 2021, 108, 103727. [Google Scholar] [CrossRef]
- Zkaynak, H. Model proposal for steel cushions for usein reinforced concrete frames. KSCE J. Civ. Eng. 2017, 21, 2717–2727. [Google Scholar] [CrossRef]
- Watanabe, A.; Hitomi, Y.; Saeki, E.; Wada, A.; Fujimoto, M. Properties of brace encased in buck-ling-restraining concrete and steel tube. In Proceedings of the Ninth World Conference on Earthquake Engineering, Tokyo, Japan, 2–9 August 1988; Volume 4, pp. 719–724. [Google Scholar]
- Wang, C.-L.; Qing, Y.; Wu, J.; Wang, J.; Gu, Z. Analytical and experimental studies on buckling-restrained brace with gap-supported tendon protection. J. Constr. Steel Res. 2020, 164, 105807. [Google Scholar] [CrossRef]
- Cao, X.-Y.; Feng, D.-C.; Wang, Z.; Wu, G. Parametric investigation of the assembled bolt-connected buckling-restrained brace and performance evaluation of its application into structural retrofit. J. Build. Eng. 2022, 48, 103988. [Google Scholar] [CrossRef]
- Cao, X.-Y.; Shen, D.; Feng, D.-C.; Wang, C.-L.; Qu, Z.; Wu, G. Seismic retrofitting of existing frame buildings through externally attached sub-structures: State of the art review and future perspectives. J. Build. Eng. 2022, 57, 104904. [Google Scholar] [CrossRef]
- Cao, X.-Y.; Feng, D.-C.; Wu, G.; Wang, Z. Experimental and theoretical investigations of the existing reinforced concrete frames retrofitted with the novel external SC-PBSPC BRBF sub-structures. Eng. Struct. 2022, 256, 113982. [Google Scholar] [CrossRef]
- Qu, B.; Dai, C.; Qiu, J.; Hou, H.; Qiu, C. Testing of seismic dampers with replaceable U-shaped steel plates. Eng. Struct. 2019, 179, 625–639. [Google Scholar] [CrossRef]
- Thongchom, C.; Bahrami, A.; Ghamari, A.; Benjeddou, O. Performance Improvement of Innovative Shear Damper Using Diagonal Stiffeners for Concentrically Braced Frame Systems. Buildings 2022, 12, 1794. [Google Scholar] [CrossRef]
- Miao, F.; Nejati, F.; Zubair, S.A.M.; Yassin, M.E. Seismic Performance of Eccentrical Braced Frame Retrofitted by Box Damper in Vertical Links. Buildings 2022, 12, 1506. [Google Scholar] [CrossRef]
- Ghamari, A.; Kim, Y.-J.; Bae, J. Utilizing an I-shaped shear link as a damper to improve the behaviour of a concentrically braced frame. J. Constr. Steel Res. 2021, 186, 106915. [Google Scholar] [CrossRef]
- AISC. AISC 341-16, Seismic Provisions for Structural Steel Buildings; American Institute of Steel Construction: Chicago, IL, USA, 2016. [Google Scholar]
- Basler, K. Strength of plate girders in shear. J. Struct. Div. 1961, 87, 151–180. [Google Scholar] [CrossRef]
- Hjelmstad, K.D.; Popov, E.P. Seismic Behavior of Active Beam Link in Eccentrically Braced Frames. Rep. No. UCB/EERC-83/15; Earthquake Engineering Research Center, University of California, Berkeley: Berkeley, CA, USA, 1983. [Google Scholar]
- McDaniel, C.C.; Uang, C.-M.; Seible, F. Cyclic Testing of Built-Up Steel Shear Links for the New Bay Bridge. Eng. Struct. 2003, 129, 801–809. [Google Scholar] [CrossRef]












| Specimen | Vn (kN) | K (kN/mm) | Δy (mm) | μ | Ω | |||
|---|---|---|---|---|---|---|---|---|
| + | − | + | − | + | − | |||
| Trapezoidal | 235.76 | 217.3 | 141.32 | 1.67 | 1.54 | 7.19 | 4.68 | 2.05 |
| I-shaped | 149.22 | 152.8 | 130.58 | 1.14 | 1.17 | 8.75 | 7.48 | 1.31 |
| T/I | 1.58 | 1.42 | 1.08 | 1.46 | 1.32 | 0.82 | 0.63 | 1.56 |
| Model | Vn (kN) | K (kN/mm) | Vs (kN) | Ω | E (kN.mm) | μ | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Vn | K | Vs | Ω | E | μ | |||||||
| I-0.22-11-10 | 770.02 | 1779.74 | 498.26 | 1.55 | 7813.62 | 25.89 | ||||||
| I-0.16-4.9-15 | 869.93 | 1889.04 | 528.86 | 1.64 | 8811.98 | 24.32 | ||||||
| I-0.12-2.76-20 | 1015.11 | 2041.52 | 571.56 | 1.78 | 10,238.44 | 22.52 | ||||||
| I-0.10-1.76-25 | 1206.48 | 2242.91 | 627.94 | 1.92 | 12,100.72 | 20.82 | ||||||
| I-0.07-1.23-30 | 1447.04 | 2486.40 | 696.11 | 2.08 | 14,407.72 | 19.24 | ||||||
| T-0.22-0.78-10 | 1237.57 | 2289.51 | 639.04 | 2.09 | 13,029.37 | 20.72 | 1.61 | 1.29 | 1.28 | 1.35 | 1.67 | 0.80 |
| T-0.16-0.52-15 | 1615.99 | 2756.09 | 771.25 | 2.10 | 16,906.77 | 19.10 | 1.86 | 1.46 | 1.46 | 1.27 | 1.92 | 0.79 |
| T-0.12-0.39-20 | 1991.61 | 3170.87 | 887.70 | 2.24 | 20,648.02 | 17.83 | 1.96 | 1.55 | 1.55 | 1.26 | 2.02 | 0.79 |
| T-0.10-0.31-25 | 2377.29 | 3544.10 | 992.23 | 2.40 | 24,511.24 | 16.70 | 1.97 | 1.58 | 1.58 | 1.25 | 2.03 | 0.80 |
| T-0.07-0.26-30 | 2781.74 | 3885.19 | 1087.75 | 2.56 | 28,572.31 | 15.64 | 1.92 | 1.56 | 1.56 | 1.23 | 1.98 | 0.81 |
| Model | tf (mm) | ρ | ψ | Ω |
|---|---|---|---|---|
| I-0.22-11-10 | 10 | 0.22 | 11.03 | 1.55 |
| I-0.16-4.9-15 | 15 | 0.17 | 4.90 | 1.64 |
| I-0.12-2.76-20 | 20 | 0.13 | 2.76 | 1.78 |
| I-0.10-1.76-25 | 25 | 0.1 | 1.76 | 1.92 |
| I-0.07-1.23-30 | 30 | 0.07 | 1.23 | 2.08 |
| T-0.22-0.78-10 | 10 | 0.22 | 0.78 | 2.09 |
| T-0.16-0.52-15 | 15 | 0.16 | 0.52 | 2.10 |
| T-0.12-0.39-20 | 20 | 0.12 | 0.39 | 2.24 |
| T-0.10-0.31-25 | 25 | 0.10 | 0.31 | 2.40 |
| T-0.07-0.26-30 | 30 | 0.07 | 0.26 | 2.56 |
| Vpmin (kN) | Vf (kN) | Vmin (kN) | FE (kN) | Error | |
|---|---|---|---|---|---|
| T-0.22-0.78-10 | 1260 | 12.93 | 1915.86 | 1237.57 | +55 |
| T-0.16-0.52-15 | 1260 | 29.09 | 1948.18 | 1615.99 | +21 |
| T-0.12-0.39-20 | 1260 | 51.71 | 1993.42 | 1991.61 | 0.00 |
| T-0.10-0.31-25 | 1260 | 80.80 | 2051.60 | 2377.29 | −14 |
| T-0.07-0.26-30 | 1260 | 116.35 | 2122.70 | 2781.74 | −24 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thongchom, C.; Ghamari, A.; Putra Jaya, R.; Benjeddoud, O. Experimental and Numerical Study on an Innovative Trapezoidal-Shaped Damper to Improve the Behavior of CBF Braces. Buildings 2023, 13, 140. https://doi.org/10.3390/buildings13010140
Thongchom C, Ghamari A, Putra Jaya R, Benjeddoud O. Experimental and Numerical Study on an Innovative Trapezoidal-Shaped Damper to Improve the Behavior of CBF Braces. Buildings. 2023; 13(1):140. https://doi.org/10.3390/buildings13010140
Chicago/Turabian StyleThongchom, Chanachai, Ali Ghamari, Ramadhansyah Putra Jaya, and Omrane Benjeddoud. 2023. "Experimental and Numerical Study on an Innovative Trapezoidal-Shaped Damper to Improve the Behavior of CBF Braces" Buildings 13, no. 1: 140. https://doi.org/10.3390/buildings13010140
APA StyleThongchom, C., Ghamari, A., Putra Jaya, R., & Benjeddoud, O. (2023). Experimental and Numerical Study on an Innovative Trapezoidal-Shaped Damper to Improve the Behavior of CBF Braces. Buildings, 13(1), 140. https://doi.org/10.3390/buildings13010140









