Safety Risk Evaluation of Metro Shield Construction When Undercrossing a Bridge
Abstract
:1. Introduction
2. Literature Review
2.1. Identifying Safety Risk Factors of Metro Shield Construction
2.2. Evaluating Safety Risks of Metro Shield Construction
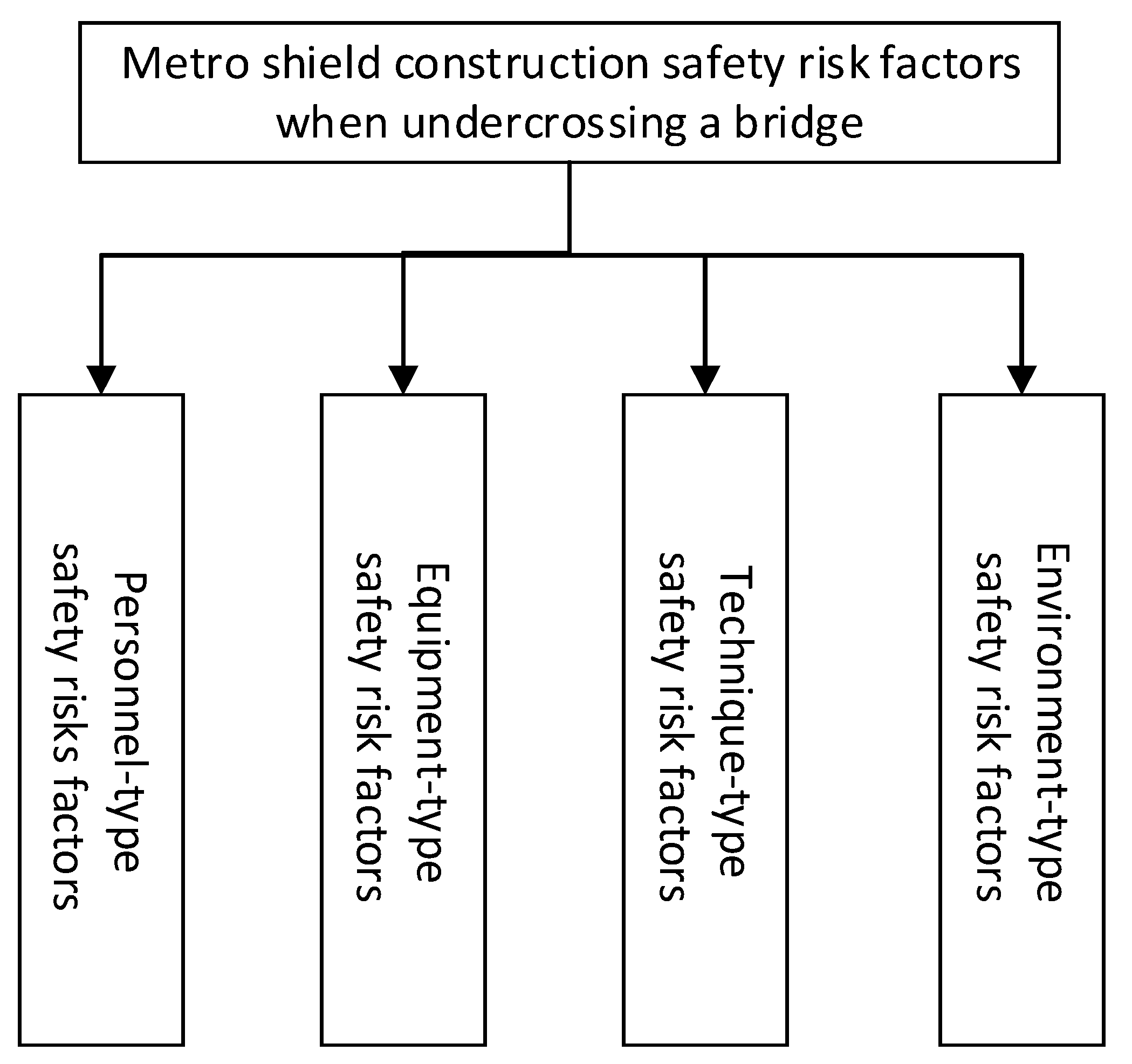
3. Identification of Safety Risk Factors of MSCUB
3.1. The Framework for Identification of Safety Risk Factors
3.2. The Identification Process of the Safety Risk Factors
4. Evaluation Model for Safety Risks of MSCUB
4.1. Weights Calculation Based on Confirmatory Factor Analysis
4.2. Measuring the Safety Risk (Factors) Using FER
- (1)
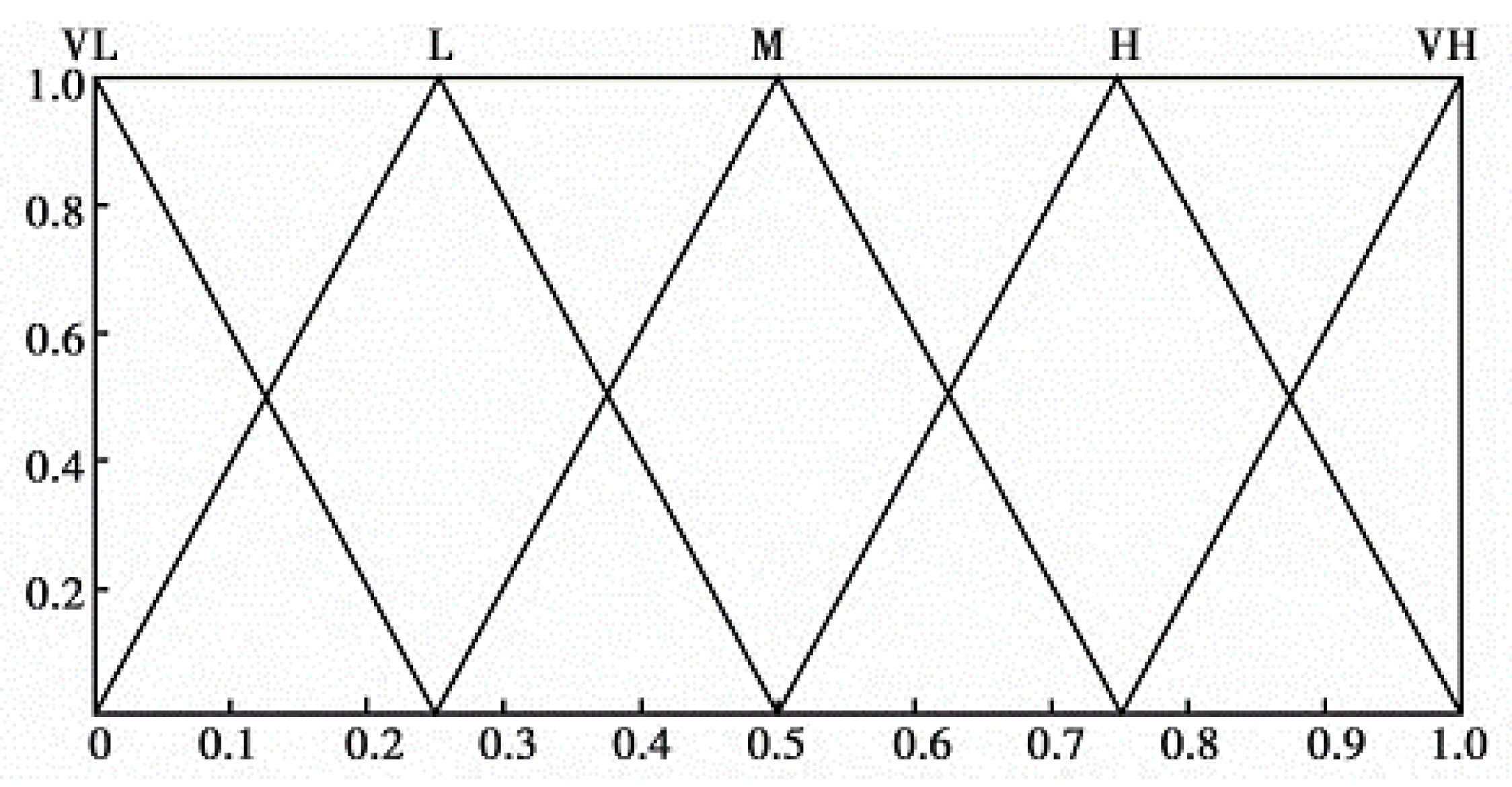
- Create the membership curve based on .
- (2)
- Locate the points where the membership curve of and the five-level membership curves in Figure 3 cross.
- (3)
- Compute the ordinates of the intersection points to express the belief values of the corresponding fuzzy evaluation level. If there are no intersection points, the belief value of this fuzzy evaluation level is zero. If there are two intersection points, the larger ordinate is selected as the belief value of this fuzzy evaluation level.
- (4)
- Next, standardize the five belief values after sequentially determining the belief values of a safety risk factor. As a result, the belief structure of a safety risk factor can be gained, which is expressed by Formula (4).
5. Case Validation
5.1. Project Overview
5.2. Identifying the Safety Risk Factors Based on the Expert Group
5.3. Calculating the Risk Values of the Safety Risk Factors Based on CFA and ER
6. Discussion and Management Implications
7. Conclusions
- (1)
- A practically feasible list of safety risk factors for MSCUB is established and consists of four first-level safety risks and thirty-seven second-level safety risk factors. The first-level safety risks include personnel-type, equipment-type, technique-type, and management-type safety risks.
- (2)
- An integrated safety risks assessment model was proposed to quantitatively assess the safety risks of MSCUB, and the model was validated as feasible in evaluating the risk values of the safety risk factors, first-level safety risks, and the overall worksite safety risk.
- (3)
- A case study showed that the overall worksite safety risk is at the medium level, and that environment-type safety risk factors and personnel-type safety risk factors have higher risk values when constructing a shield to undercross a bridge. Additionally, manager-type safety risk factors, as a whole, are higher than worker-type safety risk factors.
- (4)
- Two limitations to this research exist. Firstly, the paper neglects the relationships among the second-level safety risk factors. Follow-up research can establish new methods to evaluate the safety risks, considering the causality and coupling relationships among the safety risk factors. Secondly, the paper only selects one case to apply the proposed approach to; thus, we suggest more project cases can be analyzed in the future to validate its generality.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Safety Risks | Not Important | Slightly Important | Important | Relatively Important | Extremely Important |
|---|---|---|---|---|---|
| W1: Physical and psychological unhealthy | |||||
| W2: Poor safety awareness | |||||
| W3: Weak safety ability | |||||
| M1: Lower safety management awareness | |||||
| M2: Weaker safety management competency | |||||
| M3: Lower safety management intentions | |||||
| M4: Insufficient safety communication | |||||
| M5: Inadequate safety inspection | |||||
| EQ1: Malfunction of cutter head equipment | |||||
| EQ2: Malfunction of thrust cylinder equipment | |||||
| EQ3: Malfunction of screw conveyor | |||||
| EQ4: Malfunction of segment erector | |||||
| EQ5: Malfunction of grouting equipment | |||||
| EQ6: Malfunction of electrical equipment | |||||
| TE1: Improper bridge pier reinforcement technical scheme | |||||
| TE2: Inadequate geological and hydrological investigation scheme | |||||
| TE3: Improper construction monitoring technical scheme | |||||
| TE4: Improper excavation technical scheme | |||||
| TE5: Improper grouting and reinforcement technical scheme | |||||
| TE6: Sealed water-proof technical scheme | |||||
| TE7: Improper emergency plan | |||||
| NE1: Soft clay layer | |||||
| NE2: Silt soil layer | |||||
| NE3: Complex soil layer | |||||
| NE4: High-pressure underground water | |||||
| NE5: Subterranean boulders | |||||
| NE6: Subterranean voids | |||||
| BC1: Relatively close position of bridge piles and tunnel | |||||
| BC2: Friction bridge pile | |||||
| BC3: Large bridge pile diameter | |||||
| BC4: Poor bridge pile integrity | |||||
| BC5: Poor bridge safety condition | |||||
| ME1: Poor safety climate | |||||
| ME2: Incomplete safety institutions | |||||
| ME3: Incomplete safety organization | |||||
| ME4: Unclear safety rights and responsibility | |||||
| ME5: Inadequate safety training and education |
Appendix B
| Safety Risk Factors | Occurrence Probability Grade | Consequences Severity Grade |
|---|---|---|
| W1: Physical and psychological unhealthy | ||
| W2: Poor safety awareness | ||
| W3: Weak safety ability | ||
| M1: Lower safety management awareness | ||
| M2: Weaker safety management competency | ||
| M3: Lower safety management intentions | ||
| M4: Insufficient safety communication | ||
| M5: Inadequate safety inspection | ||
| EQ1: Malfunction of cutter head equipment | ||
| EQ2: Malfunction of thrust cylinder equipment | ||
| EQ3: Malfunction of screw conveyor | ||
| EQ4: Malfunction of segment erector | ||
| EQ5: Malfunction of grouting equipment | ||
| EQ6: Malfunction of electrical equipment | ||
| TE1: Improper bridge pier reinforcement technic scheme | ||
| TE2: Inadequate geological and hydrological investigation scheme | ||
| TE3: Improper construction monitoring technical scheme | ||
| TE4: Improper excavation technical scheme | ||
| TE5: Improper grouting and reinforcement technical scheme | ||
| TE6: Sealed water-proof technical scheme | ||
| TE7: Improper emergency plan | ||
| NE1: Soft clay layer | ||
| NE2: Silt soil layer | ||
| NE3: Complex soil layer | ||
| NE4: High-pressure underground water | ||
| NE5: Subterranean boulders | ||
| NE6: Subterranean voids | ||
| BC1: Relatively close position of bridge piles and tunnel | ||
| BC2: Friction bridge pile | ||
| BC3: Large bridge pile diameter | ||
| BC4: Poor bridge pile integrity | ||
| BC5: Poor bridge safety condition | ||
| ME1: Poor safety climate | ||
| ME2: Incomplete safety institutions | ||
| ME3: Incomplete safety organization | ||
| ME4: Unclear safety rights and responsibility | ||
| ME5: Inadequate safety training and education |
References
- Cheng, J.; Yang, X.; Wang, H.; Li, H.; Lin, X.; Guo, Y. Evaluation of the Emergency Capability of Subway Shield Construction Based on Cloud Model. Sustainability 2022, 14, 13309. [Google Scholar] [CrossRef]
- Zhang, C.; Qiao, M.; Li, H.; Chen, H.; Yang, Z. A review on blockchain applications in the construction area. J. Railw. Sci. Eng. 2023, 20, 1105–1115. [Google Scholar]
- Hu, X.; Zhang, X.; Dong, L.; Li, H.; He, Z.; Chen, H. Carbon Emission Factors Identification and Measurement Model Construction for Railway Construction Projects. Int. J. Environ. Res. Public Health 2022, 19, 11379. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Li, H.; Wang, Y.; Cheng, B. A comprehensive assessment approach for water-soil environmental risk during railway construction in ecological fragile region based on AHP and MEA. Sustainability 2020, 12, 7910. [Google Scholar] [CrossRef]
- Chen, H.; Li, H.; Hu, X. Safety Influential Factors and Accident Causation Model of Subway Shield Construction. J. Railw. Eng. Soc. 2020, 37, 87–92. [Google Scholar]
- Liu, T.; Chen, H.; Li, H. Research on safety impact factors and safety accident causation mechanism of subway shield construction in mix-ground. J. Railw. Sci. Eng. 2020, 17, 266–272. [Google Scholar]
- Zhou, Z.; Su, J. Study on active protection technology for an existing bridge adjacent to a subway project. Urban Rapid Rail Transit 2012, 25, 68–71. [Google Scholar]
- Liu, C.; Wang, B.; Song, F.; Xu, J.; Han, X. Influence of shield tunneling on a Large-Ang gle skewed railway box culvert. Chin. J. Undergr. Space Eng. 2022, 18, 318–325. [Google Scholar]
- Xia, Q. Research on the safety influence of subway shield method crossing existing high-speed railway frame bridge. J. Railw. Eng. Soc. 2021, 38, 82–86. [Google Scholar]
- Li, Z.; Wang, T.; Xiang, Y.; Zhang, M. A study on risk grade classification method and disposal measures for adjacent bridge piles in Beijing metro engineering. Rock Soil Mech. 2008, 2008, 1837–1842. [Google Scholar]
- Tao, Y.; Liu, S.; Zhao, H.; Li, X. Study on the restraint control of an isolation pile on an existing high-speed railway during the close passing of a shield machine. Front. Earth Sci. 2023, 11, 1142864. [Google Scholar] [CrossRef]
- Geng, D.; Tan, C.; Wang, N.; Jiang, Y. In Influence of Shield Tunnel and Train Load on Existing Bridge Piles. In ISMR 2020, Proceedings of the 7th International Symposium on Innovation & Sustainability of Modern Railway, Nanchang, China; IOS Press: Amsterdam, The Netherlands, 2020; pp. 48–63. [Google Scholar]
- Wu, X.; Li, T.; Lin, J.; Ma, J.; Zhang, L.; Liu, W. Safety risk assessment of metro shield tunnel-induced adjacent bridge damage based on rough set and bayesian network. J. Civ. Eng. Manag. 2016, 33, 9–15+29. [Google Scholar]
- Pan, H.; Gou, J.; Wan, Z.; Ren, C.; Chen, M.; Gou, T.; Luo, Z. Research on Coupling Degree Model of Safety Risk System for Tunnel Construction in Subway Shield Zone. Math. Probl. Eng. 2019, 2019, 5783938. [Google Scholar] [CrossRef]
- Liu, W.; Zhao, T.; Zhou, W.; Tang, J. Safety risk factors of metro tunnel construction in China: An integrated study with EFA and SEM. Saf. Sci. 2018, 105, 98–113.1. [Google Scholar] [CrossRef]
- Wang, X.; Li, S.; Xu, Z.; Li, X.; Lin, P.; Lin, C. An interval risk assessment method and management of water inflow and inrush in course of karst tunnel excavation. Tunn. Undergr. Space Technol. 2019, 92, 103033. [Google Scholar] [CrossRef]
- Hyun, K.C.; Min, S.; Choi, H.; Park, J.; Lee, I.M. Risk analysis using fault-tree analysis (FTA) and analytic hierarchy process (AHP) applicable to shield TBM tunnels. Tunn. Undergr. Space Technol. 2015, 49, 121–129. [Google Scholar] [CrossRef]
- Wu, X.; Feng, Z.; Liu, Y.; Qin, Y.; Yang, T.; Duan, J. Enhanced safety prediction of vault settlement in urban tunnels using the pair-copula and Bayesian network. Appl. Soft Comput. 2023, 132, 109711. [Google Scholar] [CrossRef]
- Liu, W.; Liu, W.; Zhai, S. Predictive Analysis of Settlement Risk in Tunnel Construction: A Bow-Tie-Bayesian Network Approach. Adv. Civ. Eng. 2019, 2019, 2045125. [Google Scholar] [CrossRef]
- Li, Z.; Wang, Y.; Olgun, C.G.; Yang, S.; Jiao, Q.; Wang, M. Risk assessment of water inrush caused by karst cave in tunnels based on reliability and GA-BP neural network. Geomatics. Nat. Hazards Risk 2020, 11, 1212–1232. [Google Scholar] [CrossRef]
- Huang, L.; Yang, J.; Zhang, C.; Jiang, C.; Su, B. Prediction and analysis of shield tunneling parameters in underwater karst stratum based on BP neural network. China Civ. Eng. J. 2020, 53, 75–80+98. [Google Scholar]
- Wu, Z.; Zou, S. A static risk assessment model for underwater shield tunnel construction. Sadhana 2020, 45, 215. [Google Scholar] [CrossRef]
- Chung, H.; Lee, I.-M.; Jung, J.-H.; Park, J. Bayesian networks-based shield TBM risk management system: Methodology development and application. KSCE J. Civ. Eng. 2019, 23, 452–465. [Google Scholar] [CrossRef]
- Li, H.; Chen, H.; Cheng, B.; Hu, X.; Cai, X. Study on formation model of subway construction safety climate based on fuzzy ISM-DEMATEL. J. Railw. Sci. Eng. 2021, 18, 2200–2208. [Google Scholar]
- Hu, C.; Lu, Z.; Mei, Y.; Zhang, W.; Zhang, Y. On the risk evaluation of the metro shield construction in the soft soil condition background with the extension method. J. Saf. Environ. 2017, 17, 21–26. [Google Scholar]
- Chen, H.; Yang, S.; Feng, Z.; Liu, Y.; Qin, Y. Safety evaluation of buildings adjacent to shield construction in karst areas: An improved extension cloud approach. Eng. Appl. Artif. Intell. 2023, 124, 106386. [Google Scholar]
- Hu, C.; Zhang, W.; Lu, Z.; Mei, Y. On the risk evaluation and control method of the shield tunneling under the hazardous buildings and rivers based on the catastrophe-forecasting theory. J. Saf. Environ. 2017, 17, 1221–1227. [Google Scholar]
- Wang, X. Safety risk assessment of subway tunnel shield construction undercrossing an existing building. Build. Struct. 2023, 53, 156. [Google Scholar]
- Qian, S.; Dong, J.; Li, L. Analysis on the safety influence of shield tunnel construction on its above metrostation. China Saf. Sci. J. 2009, 19, 172–176. [Google Scholar]
- Wu, X.; Liu, Q.; Chen, H.; Zeng, T.; Wang, J.; Tao, W. Preassessment of safety risk of shield tunneling underneath existing tunnel based on fuzzy Bayesian networks and evidence theory. Tunn. Constr. 2021, 41, 713–720. [Google Scholar]
- Lü, X.; Zeng, S.; Zhao, Y.; Huang, M.; Ma, S.; Zhang, Z. Physical model tests and discrete element simulation of shield tunnel face stability in anisotropic granular media. Acta Geotech. 2020, 15, 3017–3026. [Google Scholar] [CrossRef]
- Liu, C.; Su, Y. Pipeline deformation laws and safety risk assessments caused by shield construction. J. Railw. Sci. Eng. 2020, 17, 2882–2891. [Google Scholar]
- Zhai, Q.; Gu, H.; Jie, F. Safety evaluation of adjacent bridge in shield tunnel construction based on SPA method. J. Saf. Sci. Technol. 2021, 17, 129–135. [Google Scholar]
- Wu, H.; Wang, J.; Wang, M. In Shield construction safety risk assessment of metro tunnels based on cloud model. In Proceedings of the 2020 International Conference on Urban Engineering and Management Science, ICUEMS, Zhuhai, China, 24–26 April 2020; Volume 2020, pp. 584–587. [Google Scholar]
- Li, K.; Xiahou, X.; Huang, H.; Tang, L.; Huang, J.; Li, Q.; Feng, P. AHP-FSE-Based Risk Assessment and Mitigation for Slurry Balancing Shield Tunnel Construction. J. Environ. Public Health 2022, 2022, 1666950. [Google Scholar] [PubMed]
- Fan, Y.; Wang, R. Application of matter-element extension method in safety risk assessment of subway shield construction. J. Saf. Environ. 2023, 23, 1779–1790. [Google Scholar]
- Ren, J.; Yang, F.; Zhu, Y. The Risk Assessment of Shield Construction under the Condition of Adjacent Buildings in Xi’an Metro. J. Railw. Eng. Soc. 2016, 33, 88–93. [Google Scholar]
- Zhang, L.; Liu, S.; Li, K.; Xu, J.; Wang, S.; Li, Q.; Mei, Y.; Li, C.; Yang, B. Prediction of shield construction risks in subway tunnelling based on fault tree and Bayesian network. Mod. Tunneling Technol. 2021, 58, 21–29+55. [Google Scholar]
- Li, H.; Chen, H.; Zeng, X.; Hu, X.; Cheng, B.; Tang, X. Determining the factor structure of construction safety climate in building engineering: A case study in Changsha. J. Railw. Sci. Eng. 2021, 18, 1935–1942. [Google Scholar]
- Xu, Y.; Li, L.; Chen, H.; Cai, X.; Li, H. Research on the influence of supervisor-worker guanxi on construction workers’ safety behavior. J. Railw. Sci. Eng. 2022, 20, 3138–3150. [Google Scholar]
- Wei, D.; Zhang, Y. Fuzzy witness reasoning-based approach to the risk assessment of the deep foundation pit construction. J. Saf. Environ. 2021, 21, 512–520. [Google Scholar]
- He, R.; Zhang, L.; Tiong, R.L.K. Flood risk assessment and mitigation for metro stations: An evidential-reasoning-based optimality approach considering uncertainty of subjective parameters. Reliab. Eng. Syst. Saf. 2023, 238, 109453. [Google Scholar]
- He, X. Multivariate Statistic Analysis, 2nd ed.; China Remin University Press: Beijing, China, 2008; pp. 192–206. [Google Scholar]
- Johnson, R.; Wichern, D. Applied Multivariate Statistical Analysis, 5th ed.; Prentice Hall, Inc.: Upper Saddle River, NJ, USA, 2002; pp. 477–516. [Google Scholar]
- Brown, T.A. Confirmatory Factor Analysis for Applied Research, 2nd ed.; The Guilford Press: New York, NY, USA, 2015; pp. 35–75. [Google Scholar]
- Wenjuanxing. Wenjuanxing Website. Available online: https://www.wjx.cn/ (accessed on 30 July 2023).
- Zhang, W. Advanced Courses on SPSS Statistical Analysis; Higher Education Press: Beijing, China, 2004; pp. 218–227. [Google Scholar]
- Pallant, J. SPSS Survival Manual: A Step by Step Guide to Data Analysis Using IBM Spss, 6th ed.; Open University Press: Maidenhead, UK, 2016; pp. 202–224. [Google Scholar]
- Xia, N.; Ding, S.; Yuan, J. The impact of a challenging work environment: Do job stressors benefit citizenship behavior of project managers? Int. J. Proj. Manag. 2022, 40, 205–217. [Google Scholar]
- Xie, Q.; Xia, N.; Yang, G. Do Family Affairs Matter? Work-Family Conflict and Safety Behavior of Construction Workers. J. Manag. Eng. 2022, 38, 04021074. [Google Scholar]
- Xia, N.; Tang, Y.; Li, D.; Pan, A. Safety Behavior among Construction Workers: Influences of Personality and Leadership. J. Constr. Eng. Manag. 2021, 147, 04021019. [Google Scholar] [CrossRef]
- Qiu, H.; Lin, B. Principle and Application of Structural Equation Model; China Light Industry Press: Beijing, China, 2009; pp. 69–89. [Google Scholar]
- Wu, M. Structural Equation Model–Operation and Application of AMOS; Chongqing University Press: Chongqing, China, 2009; pp. 37–59. [Google Scholar]
- Qin, Y.; Zhang, J.; Jia, X.; Hui, Y.; Cui, C. Safety evaluation for the overtaking behavior on the two-lane highway based on the structural equation model. J. Saf. Environ. 2017, 17, 1359–1364. [Google Scholar]
- Sun, K.; Meng, Y.; Yan, X.; Zhou, X. PLS-SEM-based analysis on contruction safety of water conservancy project. Water Resour. Hydropower Eng. 2019, 50, 115–119. [Google Scholar]
- Duan, Y.; Zhou, S.; Guo, Y.; Wang, X. Safety risk and strategy of prefabricated building construction based on SEM. J. Civ. Eng. Manag. 2020, 37, 70–75+121. [Google Scholar]
- He, Y.; Fan, Z. Research on double-weight risk assessment method of coal mine safety management. J. Xi’an Univ. Sci. Technol. 2022, 42, 600–606. [Google Scholar]
- Jiang, J.; Li, X.; Xing, L.; Chen, Y. System risk analysis and evaluation approach based on fuzzy evidential reasoning. Syst. Eng.–Theory Pract. 2013, 33, 529–537. [Google Scholar]
- Gao, J.; Zhang, H.; Men, G.; Wang, Q. A fuzzy evidence reasoning method for aviation safety risk evaluation. Electron. Opt. Control 2022, 29, 81–85+113. [Google Scholar]
- Zhang, Z.; Wang, B.; Wang, X.; He, Y.; Wang, H.; Zhao, S. Safety-Risk Assessment for TBM Construction of Hydraulic Tunnel Based on Fuzzy Evidence Reasoning. Processes 2022, 10, 2597. [Google Scholar] [CrossRef]
- Yang, J.; Wang, Y.; Xu, D.; Chin, K.S. The evidential reasoning approach for MADA under both probabilistic and fuzzy uncertainties. Eur. J. Oper. Res. 2006, 171, 309–343. [Google Scholar] [CrossRef]
- Lu, H.; Qi, J.; Li, J.; Xie, Y.; Xu, G.; Wang, H. Multi-agent based safety computational experiment system for shield tunneling projects. Eng. Constr. Archit. Manag. 2020, 27, 1963–1991. [Google Scholar]
- Zhou, H.; Zhao, Y.; Shen, Q.; Yang, L.; Cai, H. Risk assessment and management via multi-source information fusion for undersea tunnel construction. Autom. Constr. 2020, 111, 103050. [Google Scholar] [CrossRef]
- Yiu, N.S.N.; Chan, D.W.M.; Shan, M.; Sze, N.N. Implementation of safety management system in managing construction projects: Benefits and obstacles. Saf. Sci. 2019, 117, 23–32. [Google Scholar] [CrossRef]
- Wang, Q.; Xiong, Z.; Zhu, K.; Guo, P. Construction Safety Risks of Metro Tunnels Constructed by the Mining Method in Wuhan City, China: A Structural Equation Model-Fuzzy Cognitive Map Hybrid Method. Buildings 2023, 13, 1335. [Google Scholar] [CrossRef]
- Zhang, L.; Wu, X.; Zhu, H.; AbouRizk, S.M. Perceiving safety risk of buildings adjacent to tunneling excavation: An information fusion approach. Autom. Constr. 2017, 73, 88–101. [Google Scholar] [CrossRef]
- Cai, Q.; Hu, Q.; Ma, G. Improved Hybrid Reasoning Approach to Safety Risk Perception under Uncertainty for Mountain Tunnel Construction. J. Constr. Eng. Manag. 2021, 147, 04021105. [Google Scholar] [CrossRef]
- Zhang, L.; Ding, L.; Wu, X.; Skibniewski, M.J. An improved Dempster–Shafer approach to construction safety risk perception. Knowl.-Based Syst. 2017, 132, 30–46. [Google Scholar] [CrossRef]
- Zheng, X.; Qi, J.; Chen, W.; Yang, K.; Chen, Q.; Xue, R. Analysis on influence of shield tunnel excavation in Nantong water-rich sand stratum on adjacent pile foundations. Sci. Technol. Work Saf. China 2022, 18, 146–151. [Google Scholar]
- Xia, N.; Xie, Q.; Hu, X.; Wang, X.; Meng, H. A dual perspective on risk perception and its effect on safety behavior: A moderated mediation model of safety motivation, and supervisor’s and coworkers’ safety climate. Accid. Anal. Prev. 2020, 134, 105350. [Google Scholar] [CrossRef]
- Xia, N.; Xie, Q.; Griffin, M.A.; Ye, G.; Yuan, J. Antecedents of safety behavior in construction: A literature review and an integrated conceptual framework. Accid. Anal. Prev. 2020, 148, 105834. [Google Scholar] [PubMed]
- Chen, H.; Gong, W.; Li, H.; Shi, S. Co-workers’ guanxi and construction workers’ safety behavior: The mediating role of group identification. Front. Public Health 2022, 10, 964514. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Li, H.; Goh, Y.M. A review of construction safety climate: Definitions, factors, relationship with safety behavior and research agenda. Saf. Sci. 2021, 142, 105391. [Google Scholar]
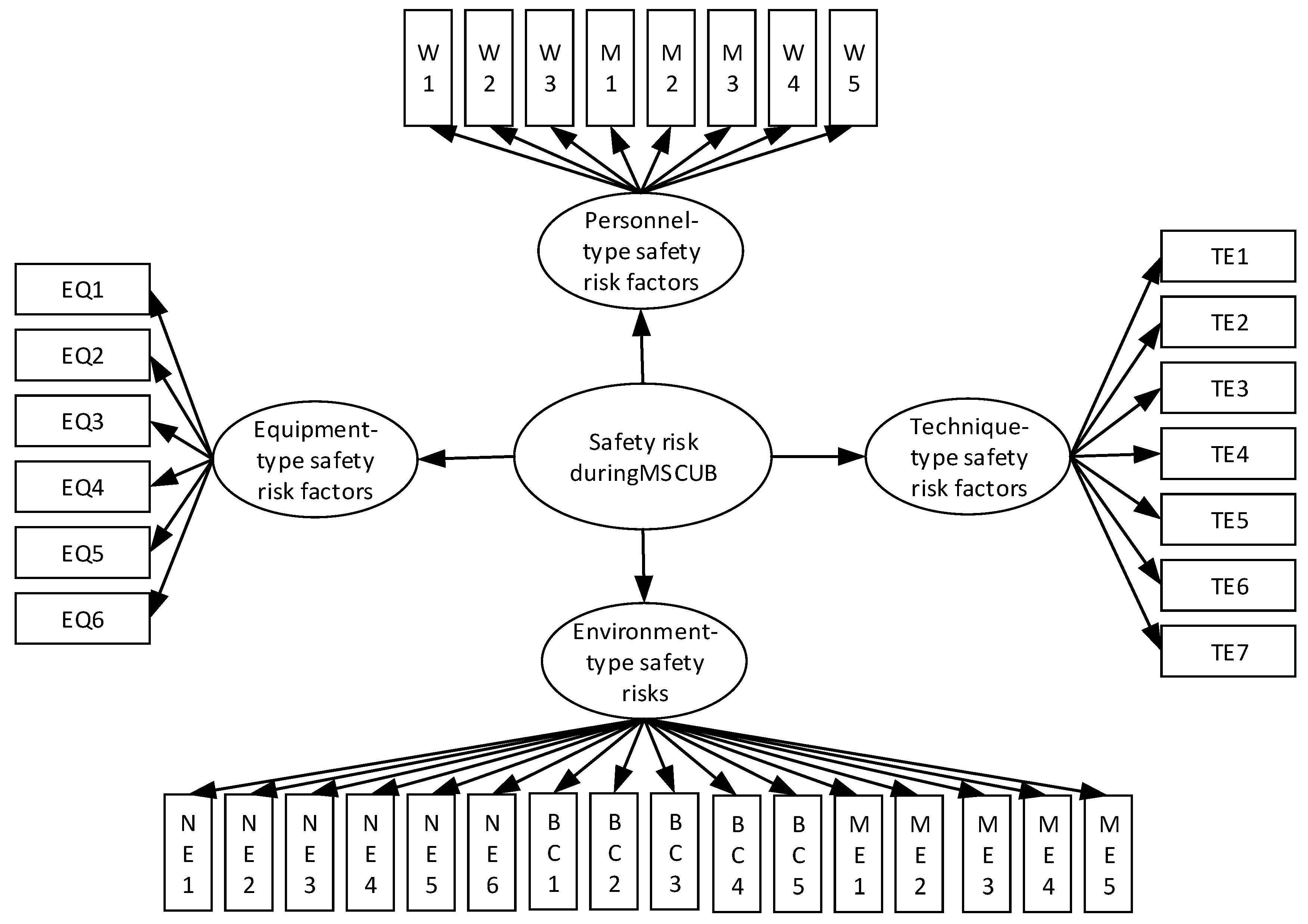
| First-Level Safety Risk Factors | Second-Level Safety Risk Factors | |
|---|---|---|
| Personnel-type | Worker-type | W1: Physical and psychological unhealth; W2: Poor safety awareness; W3: Weak safety ability. |
| Manager-type | M1: Lower safety management awareness; M2: Weaker safety management competency; M3: Lower safety management intentions; M4: Insufficient safety communication; M5: Inadequate safety inspection. | |
| Equipment-type | EQ1: Malfunction of cutter head equipment; EQ2: Malfunction of thrust cylinder equipment; EQ3: Malfunction of screw conveyor; EQ4: Malfunction of segment erector; EQ5: Malfunction of grouting equipment: EQ6: Malfunction of electrical equipment. | |
| Technique-type | TE1: Improper bridge pier reinforcement technical scheme; TE2: Inadequate geological and hydrological investigation scheme; TE3: Improper construction monitoring technical scheme; TE4: Improper excavation technical scheme; TE5: Improper grouting and reinforcement technical scheme; TE6: Sealed water-proof technical scheme; TE7: Improper emergency plan. | |
| Environment-type | Natural environment-type | NE1: Soft clay layer; NE2: Silt soil layer; NE3: Complex soil layer; NE4: High-pressure underground water; NE5: Subterranean boulders; NE6: Subterranean voids. |
| Bridge condition | BC1: Relatively close position of bridge piles and tunnel; BC2: Friction bridge pile type; BC3: Large bridge pile diameter; BC4: Poor bridge pile integrity; BC5: Poor bridge safety condition. | |
| Management environment-type | ME1: Poor safety climate; ME2: Incomplete safety institutions; ME3: Incomplete safety organization; ME4: Unclear safety rights and responsibility; ME5: Inadequate safety training and education. | |
| RP | SPC | p | RP | SPC | p | RP | SPC | p |
|---|---|---|---|---|---|---|---|---|
| PTSRF→W1 | 0.406 | *** | TTSRF→TE1 | 0.699 | *** | ETSRF→BC2 | 0.674 | *** |
| PTSRF→W2 | 0.711 | *** | TTSRF→TE2 | 0.531 | ** | ETSRF→BC3 | 0.642 | *** |
| PTSRF→W3 | 0.592 | *** | TTSRF→TE3 | 0.627 | *** | ETSRF→BC4 | 0.732 | *** |
| PTSRF→M1 | 0.570 | *** | TTSRF→TE4 | 0.779 | ** | ETSRF→BC5 | 0.755 | *** |
| PTSRF→M2 | 0.605 | *** | TTSRF→TE5 | 0.756 | ** | ETSRF→ME1 | 0.732 | *** |
| PTSRF→M3 | 0.739 | *** | TTSRF→TE6 | 0.587 | *** | ETSRF→ME1 | 0.835 | *** |
| PTSRF→M4 | 0.706 | *** | TTSRF→TE7 | 0.473 | *** | ETSRF→ME1 | 0.732 | *** |
| PTSRF→M5 | 0.727 | *** | ETSRF→NE1 | 0.747 | *** | ETSRF→ME1 | 0.813 | *** |
| ETSRT→EQ1 | 0.625 | *** | ETSRF→NE2 | 0.741 | *** | ETSRF→ME1 | 0.625 | *** |
| ETSRT→EQ2 | 0.682 | ** | ETSRF→NE3 | 0.673 | *** | SRMSCUB→PTSRF | 0.785 | *** |
| ETSRT→EQ3 | 0.409 | *** | ETSRF→NE4 | 0.723 | *** | SRMSCUB→ETSRF | 0.564 | *** |
| ETSRT→EQ4 | 0.622 | ** | ETSRF→NE5 | 0.547 | *** | SRMSCUB→TTSRF | 0.648 | ** |
| ETSRT→EQ5 | 0.768 | ** | ETSRF→NE6 | 0.543 | *** | SRMSCUB→ETSRF | 0.946 | ** |
| ETSRT→EQ6 | 0.594 | *** | ETSRF→BC1 | 0.769 | *** | - | - |
| Safety Risks Factor | Weight | Safety Risks Factor | Weight | Safety Risks Factor | Weight |
|---|---|---|---|---|---|
| W1 | 0.238 | TE1 | 0.157 | BC2 | 0.189 |
| W2 | 0.416 | TE2 | 0.119 | BC3 | 0.180 |
| W3 | 0.346 | TE3 | 0.141 | BC4 | 0.205 |
| M1 | 0.170 | TE4 | 0.175 | BC5 | 0.211 |
| M2 | 0.181 | TE5 | 0.170 | ME1 | 0.196 |
| M3 | 0.221 | TE6 | 0.132 | ME2 | 0.223 |
| M4 | 0.211 | TE7 | 0.106 | ME3 | 0.196 |
| M5 | 0.217 | NE1 | 0.188 | ME4 | 0.218 |
| EQ1 | 0.169 | NE2 | 0.186 | ME5 | 0.167 |
| EQ2 | 0.184 | NE3 | 0.169 | PTSRF | 0.267 |
| EQ3 | 0.111 | NE4 | 0.182 | ETSRF | 0.192 |
| EQ4 | 0.168 | NE5 | 0.138 | TTSRF | 0.220 |
| EQ5 | 0.208 | NE6 | 0.137 | ETSRF | 0.321 |
| EQ6 | 0.161 | BC1 | 0.215 | - | - |
| Level | Occurrence Probability | Consequence Severity | The Triangular Fuzzy Number |
|---|---|---|---|
| 1 | Extremely low | No impact | (0.00, 0.00, 0.25) |
| 2 | Low | Minor impact | (0.00, 0.25, 0.50) |
| 3 | Relatively high | Large impact | (0.25, 0.50, 0.75) |
| 4 | High | Dangerous | (0.50, 0.75, 1.00) |
| 5 | Extremely high | Catastrophic | (0.75, 1.00, 1.00) |
| No. | Level of Safety Risk | Definition | Membership Functions |
|---|---|---|---|
| 1 | Extremely low (EL) | The safety risk is acceptable. | (0.00, 0.00, 0.25) |
| 2 | Low (L) | The safety risk is acceptable, and if the safety risk cost is acceptable, measures should be taken to reduce the risk. | (0.00, 0.25, 0.50) |
| 3 | Medium (M) | If technology is feasible, measures must be taken to reduce the risk. | (0.25, 0.50, 0.75) |
| 4 | High (H) | Measures must be taken to reduce the risk. | (0.50, 0.75, 1.00) |
| 5 | Extremely high (EH) | Measures must be taken to reduce and control the risk. | (0.75, 1.00, 1.00) |
| Safety Risk Factor Category | Safety Risk Factors | |
|---|---|---|
| Personnel-type | Worker-type | W2: Poor safety awareness; W3: Weak safety ability. |
| Manager-type | M2: Weaker safety management competency; M4: Insufficient safety communication; M5: Inadequate safety inspection. | |
| Equipment-type | EQ2: Malfunction of thrust cylinder equipment; EQ3: Malfunction of screw conveyor; EQ5: Malfunction of grouting equipment: EQ6: Malfunction of electrical equipment. | |
| Technique-type | TE1: Improper bridge pier reinforcement technical scheme; TE3: Improper construction monitoring technical scheme; TE4: Improper excavation technical scheme; TE5: Improper grouting and reinforcement technical scheme; TE6: Sealed water-proof technical scheme. | |
| Environment-type | Natural environment-type | NE1: Soft clay layer; NE4: High-pressure underground water; NE5: Subterranean boulders. |
| Bridge condition | BC1: Relatively close position of bridge piles and tunnel; BC2: Friction bridge pile; BC3: Large bridge pile diameter; BC5: Poor bridge safety condition. | |
| Management environment-type | ME2: Incomplete safety institutions; ME4: Unclear safety rights and responsibility; ME5: Inadequate safety training and education. | |
| Safety Risk Factor | Occurrence Probability Level | Consequences Severity Level |
|---|---|---|
| W2: Poor safety awareness | 3 (Relatively high) | 4 (Dangerous) |
| W3: Weak safety ability | 3 (Relatively high) | 3 (Large impact) |
| M2: Weaker safety management competency | 4 (High) | 3 (Large impact) |
| M4: Insufficient safety communication | 3 (Relatively high) | 5 (Catastrophic) |
| M5: Inadequate safety inspection | 3 (Relatively high) | 4 (Dangerous) |
| EQ2: Malfunction of thrust cylinder equipment | 3 (Relatively high) | 4 (Dangerous) |
| EQ3: Malfunction of screw conveyor | 2 (Low) | 4 (Dangerous) |
| EQ5: Malfunction of grouting equipment | 3 (Relatively high) | 4 (Dangerous) |
| EQ6: Malfunction of electrical equipment | 3 (Relatively high) | 3 (Large impact) |
| TE1: Improper bridge pier reinforcement technic scheme | 3 (Relatively high) | 5 (Catastrophic) |
| TE3: Improper construction monitoring technical scheme | 3 (Relatively high) | 3 (Large impact) |
| TE4: Improper excavation technical scheme | 3 (Relatively high) | 3 (Large impact) |
| TE5: Improper grouting and reinforcement technical scheme | 3 (Relatively high) | 4 (Dangerous) |
| TE6: Sealed water-proof technical scheme | 3 (Relatively high) | 5 (Catastrophic) |
| NE1: Soft clay layer | 3 (Relatively high) | 4 (Dangerous) |
| NE4: High-pressure underground water | 4 (High) | 4 (Dangerous) |
| NE5: Subterranean boulders | 3 (Relatively high) | 3 (Large impact) |
| BC1: Relatively close position of bridge piles and tunnel; | 3 (Relatively high) | 4 (Dangerous) |
| BC2: Friction bridge pile | 4 (High) | 4 (Dangerous) |
| BC3: Large bridge pile diameter | 3 (Relatively high) | 4 (Dangerous) |
| BC5: Poor bridge safety condition | 3 (Relatively high) | 3 (Large impact) |
| ME2: Incomplete safety institutions | 3 (Relatively high) | 5 (Catastrophic) |
| ME4: Unclear safety rights and responsibility | 3 (Relatively high) | 4 (Dangerous) |
| ME5: Inadequate safety training and education | 3 (Relatively high) | 3 (Large impact) |
| Safety Risk Factor | Fuzzy Occurrence Probability | Fuzzy Consequences Severity Level | Fuzzy Values of Safety Risks |
|---|---|---|---|
| W2: Poor safety awareness | (0.25, 0.50, 0.75) | (0.50, 0.75, 1.00) | (0.125, 0.375, 0.750) |
| W3: Weak safety ability | (0.25, 0.50, 0.75) | (0.25, 0.50, 0.75) | (0.063, 0.250, 0.563) |
| M2: Weaker safety management competency | (0.50, 0.75, 1.00) | (0.25, 0.50, 0.75) | (0.125, 0.375, 0.750) |
| M4: Insufficient safety communication | (0.25, 0.50, 0.75) | (0.75, 1.00, 1.00) | (0.188, 0.500, 0.750) |
| M5: Inadequate safety inspection | (0.25, 0.50, 0.75) | (0.50, 0.75, 1.00) | (0.125, 0.375, 0.750) |
| EQ2: Malfunction of thrust cylinder equipment | (0.25, 0.50, 0.75) | (0.50, 0.75, 1.00) | (0.125, 0.375, 0.750) |
| EQ3: Malfunction of screw conveyor | (0.00, 0.25, 0.50) | (0.50, 0.75, 1.00) | (0.00, 0.188, 0.500) |
| EQ5: Malfunction of grouting equipment | (0.25, 0.50, 0.75) | (0.50, 0.75, 1.00) | (0.125, 0.375, 0.750) |
| EQ6: Malfunction of electrical equipment | (0.25, 0.50, 0.75) | (0.25, 0.50, 0.75) | (0.063, 0.250, 0.563) |
| TE1: Improper bridge pier reinforcement technical scheme | (0.25, 0.50, 0.75) | (0.75, 1.00, 1.00) | (0.188, 0.500, 0.750) |
| TE3: Improper construction monitoring technical scheme | (0.25, 0.50, 0.75) | (0.25, 0.50, 0.75) | (0.063, 0.250, 0.563) |
| TE4: Improper excavation technical scheme | (0.25, 0.50, 0.75) | (0.25, 0.50, 0.75) | (0.063, 0.250, 0.563) |
| TE5: Improper grouting and reinforcement technical scheme | (0.25, 0.50, 0.75) | (0.50, 0.75, 1.00) | (0.125, 0.375, 0.750) |
| TE6: Sealed water-proof technical scheme | (0.25, 0.50, 0.75) | (0.75, 1.00, 1.00) | (0.188, 0.500, 0.750) |
| NE1: Soft clay layer | (0.25, 0.50, 0.75) | (0.50, 0.75, 1.00) | (0.125, 0.375, 0.750) |
| NE4: High-pressure underground water | (0.50, 0.75, 1.00) | (0.50, 0.75, 1.00) | (0.250, 0.563, 1.000) |
| NE5: Subterranean boulders | (0.25, 0.50, 0.75) | (0.25, 0.50, 0.75) | (0.063, 0.250, 0.563) |
| BC1: Relatively close position of bridge piles and tunnel | (0.25, 0.50, 0.75) | (0.50, 0.75, 1.00) | (0.125, 0.375, 0.750) |
| BC2: Friction bridge pile | (0.50, 0.75, 1.00) | (0.50, 0.75, 1.00) | (0.250, 0.563, 1.000) |
| BC3: Large bridge pile diameter | (0.25, 0.50, 0.75) | (0.50, 0.75, 1.00) | (0.125, 0.375, 0.750) |
| BC5: Poor bridge safety condition | (0.25, 0.50, 0.75) | (0.25, 0.50, 0.75) | (0.063, 0.250, 0.563) |
| ME2: Incomplete safety institutions | (0.25, 0.50, 0.75) | (0.75, 1.00, 1.00) | (0.188, 0.500, 0.750) |
| ME4: Unclear safety rights and responsibility | (0.25, 0.50, 0.75) | (0.50, 0.75, 1.00) | (0.125, 0.375, 0.750) |
| ME5: Inadequate safety training and education | (0.25, 0.50, 0.75) | (0.25, 0.50, 0.75) | (0.063, 0.250, 0.563) |
| Safety Risk Factor | Belief Structure | Risk Level | ||||
|---|---|---|---|---|---|---|
| EL | L | M | H | EH | ||
| W2: Poor safety awareness | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| W3: Weak safety ability | 0.205 | 0.477 | 0.265 | 0.053 | 0.000 | L |
| M2: Weaker safety management competency | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| M4: Insufficient safety communication | 0.051 | 0.257 | 0.461 | 0.231 | 0.000 | M |
| M5: Inadequate safety inspection | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| EQ2: Malfunction of thrust cylinder equipment | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| EQ3: Malfunction of screw conveyor | 0.300 | 0.467 | 0.233 | 0.000 | 0.000 | L |
| EQ5: Malfunction of grouting equipment | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| EQ6: Malfunction of electrical equipment | 0.205 | 0.477 | 0.265 | 0.053 | 0.000 | L |
| TE1: Improper bridge pier reinforcement technical scheme | 0.051 | 0.257 | 0.461 | 0.231 | 0.000 | M |
| TE3: Improper construction monitoring technical scheme | 0.205 | 0.477 | 0.265 | 0.053 | 0.000 | L |
| TE4: Improper excavation technical scheme | 0.205 | 0.477 | 0.265 | 0.053 | 0.000 | L |
| TE5: Improper grouting and reinforcement technical scheme | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| TE6: Sealed water-proof technical scheme | 0.051 | 0.257 | 0.461 | 0.231 | 0.000 | M |
| NE1: Soft clay layer | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| NE4: High-pressure underground water | 0.000 | 0.184 | 0.366 | 0.300 | 0.15 | M |
| NE5: Subterranean boulders | 0.205 | 0.477 | 0.265 | 0.053 | 0.000 | L |
| BC1: Relatively close position of bridge piles and tunnel | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| BC2: Friction bridge pile | 0.000 | 0.184 | 0.366 | 0.300 | 0.150 | M |
| BC3: Large bridge pile diameter | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| BC5: Poor bridge safety condition | 0.205 | 0.477 | 0.265 | 0.053 | 0.000 | L |
| ME2: Incomplete safety institutions | 0.051 | 0.257 | 0.461 | 0.231 | 0.000 | M |
| ME4: Unclear safety rights and responsibility | 0.114 | 0.341 | 0.363 | 0.183 | 0.000 | M |
| ME5: Inadequate safety training and education | 0.205 | 0.477 | 0.265 | 0.053 | 0.000 | L |
| First-Level Safety Risk Factors/Overall Worksite Safety Risk | NC ) | Risk Level | |||||
|---|---|---|---|---|---|---|---|
| EL | L | M | H | EH | |||
| Worker-type safety risk factor | 0.138 | 0.080 | 0.560 | 0.330 | 0.030 | 0.000 | L |
| Manager-type safety risk factor | 0.276 | 0.007 | 0.300 | 0.615 | 0.078 | 0.000 | M |
| Personnel-type safety risk factor | 0.102 | 0.001 | 0.451 | 0.541 | 0.007 | 0.000 | M |
| Equipment-type safety risk factor | 0.820 | 0.023 | 0.740 | 0.233 | 0.004 | 0.000 | L |
| Technique-type safety risk factor | 3.040 | 0.001 | 0.480 | 0.516 | 0.003 | 0.000 | M |
| Natural environment-type safety risk factor | 0.595 | 0.012 | 0.464 | 0.500 | 0.024 | 0.000 | M |
| Bridge condition | 1.105 | 0.000 | 0.433 | 0.543 | 0.024 | 0.000 | M |
| Management environment-type safety risk factor | 0.406 | 0.000 | 0.440 | 0.532 | 0.028 | 0.000 | M |
| Environment-type safety risk factor | 0.302 | 0.000 | 0.394 | 0.604 | 0.002 | 0.000 | M |
| Overall worksite safety risk | 0.269 | 0.000 | 0.475 | 0.524 | 0.001 | 0.000 | M |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, K.; Zhu, J.; Wang, H.; Huang, Y.; Li, H.; Dai, Z.; Zhang, J. Safety Risk Evaluation of Metro Shield Construction When Undercrossing a Bridge. Buildings 2023, 13, 2540. https://doi.org/10.3390/buildings13102540
He K, Zhu J, Wang H, Huang Y, Li H, Dai Z, Zhang J. Safety Risk Evaluation of Metro Shield Construction When Undercrossing a Bridge. Buildings. 2023; 13(10):2540. https://doi.org/10.3390/buildings13102540
Chicago/Turabian StyleHe, Kuang, Jun Zhu, Hui Wang, Yanlong Huang, Hujun Li, Zishuang Dai, and Jingxiao Zhang. 2023. "Safety Risk Evaluation of Metro Shield Construction When Undercrossing a Bridge" Buildings 13, no. 10: 2540. https://doi.org/10.3390/buildings13102540
APA StyleHe, K., Zhu, J., Wang, H., Huang, Y., Li, H., Dai, Z., & Zhang, J. (2023). Safety Risk Evaluation of Metro Shield Construction When Undercrossing a Bridge. Buildings, 13(10), 2540. https://doi.org/10.3390/buildings13102540







