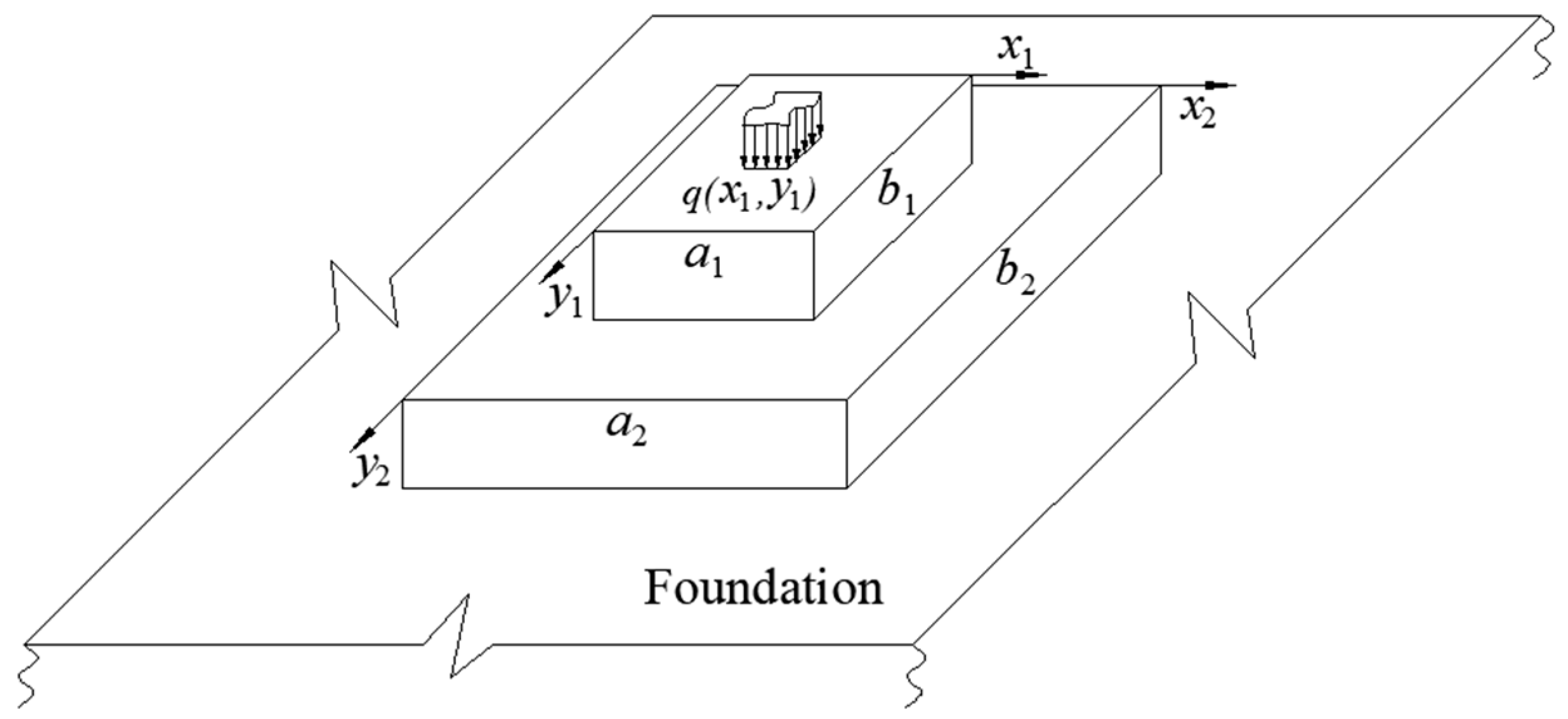
1. Introduction
Plate-foundation systems have become a research hotspot in civil engineering. Much practical engineering, such as foundation plates in civil engineering [
1], piezoelectric laminated plates in electronic engineering [
2], and pavement plates supporting the traffic load [
3], can be simplified into a mechanical model for analysis [
4]. In order to provide necessary parameters for the model of these structures, two methods are usually used. One is to provide accurate models for various types of plates and the interaction between the plate and foundation. The other one is to develop a numerical analysis method and computer codes for the solution of practical engineering problems.
In the past few centuries, much work has been done to develop simplified models of foundations to promote applications in engineering design. The Winkler foundation model, in which the foundation is assumed as a single layer of vertical springs, was first proposed in the 1860s [
5]. However, the accuracy of this model cannot be guaranteed in the analysis of nonlinear conditions of the foundation. Thus, the linear spring is replaced by a nonlinear type of model to overcome this shortage [
6]; this model can be used in static analysis [
7] and dynamic analysis [
8], but it still cannot accurately analyze the frictional foundation due to the reason that the analysis model of the Winkler foundation eliminates the interfacial shear stress. Thus, researchers have proposed two-parameter and multi-parameter models to solve this problem. Compared to the Winkler model, the two-parameter Pasternak foundation model has shear and vertical stiffness with nonlinear properties, which is helpful to improve the efficiency of the model, without resulting in significant loss of the accuracy of calculation results [
9,
10]. In addition, the three-parameter model [
11] and the frictional model [
7] were also proposed by researchers. Although the refinement of the parameters could improve the applicability of the foundation model, the increase of the number of the parameters will enhance the complication of the calculation process.
The plate theory also plays an important role in the simulation of numerical models and the structure design of the plate-foundation system. The Kirchhoff plate theory [
12] and the Mindlin plate theory [
13] are the two types of classical plate theories widely used in the simulation of plate foundations. The in-plane deformation of the plate is assumed to be linearly changed in the classical plate theories, and these theories could only be used in the analysis of thin and moderately thick plates. Many studies have been carried out using the classical plate theories. Özdemir [
14,
15] investigated the static performance, free vibration properties, and transient response of thick plates resting on the elastic Winkler foundation with the finite element method. Farida et al. [
16] presented the efficient analysis of plates resting on an elastic half-space foundation using the boundary element method. Ragba et al. [
17] used convolution and indirect meshless techniques to obtain the vibration performance of irregular composite plates on the linear and parabolic Winkler foundations. Ferreira et al. [
18] investigated the free vibration problem of the plate resting on the Winkler foundation using the wavelet collocation method. Investigations of the Kirchhoff plate on foundations are also reported in the literature. Yue et al. [
19] analyzed the influence of soil heterogeneity on the bending of a circular thin plate using two modified Vlasov foundation models. Mohammadimehr et al. [
20] obtained the buckling and free vibration response of functionally graded materials resting on a Pasternak foundation. To promote the development of the plate theory, higher-order shear deformation theory and quasi-3D theory have attracted the attention of researchers in recent years, and these theories take into account the nonlinear deformation of the plates. Singh et al. [
21] used the stress-function Galerkin method to investigate the dynamic response of a sandwich functionally graded plate resting on a Pasternak elastic foundation. Huang et al. [
22] studied the nonlinear dynamic performance of functionally graded material plates using an improved perturbation technique. Rachid et al. [
23] and Vu et al. [
24] used different quasi-3D theories to analyze the static response of the plate. Kumar et al. [
25] investigated the vibration performance of stepped FGM plates using the dynamic stiffness method.
Based on the foundation models and the different plate theories, analytical models can be used to obtain the accurate solution for the plate-foundation systems. Compared with the literature using numerical methods, analytical methods are not commonly used due to the difficulties in the partial differential equations and various boundary conditions [
26]. Yan [
27] introduced the usage of the Fourier series in the analysis of the bending, stability, and vibration of the plate. The deflection of the plate was expressed as a double Fourier series, and the various boundary conditions were used to obtain the analytical solution of the plate resting on the Winkler foundation. Wang [
28] used the method mentioned in [
27] to investigate the interaction and deflection of the thin plate resting on the elastic half-space foundation. The foundation models eliminated the assumptions of the Winkler foundation to overcome the shortages of the Winkler model to obtain a more accurate and reasonable analytical solution. Li et al. [
29] presented the analytic bending solution of a thin plate resting on the Winkler foundation. The governing differential equations of the plate were transferred into Hamilton canonical equations, and the analytic solution could be obtained with all edges slidingly supported. Bai et al. [
30] studied the bending problem of the free orthotropic rectangular thin plate (RTP) on a two-parameter elastic foundation under a concentrated load by using the symplectic superposition method. Tenenbaum et al. [
31] gave the analytical solutions for the buckling loads of thin rectangular plates with internal supports and different boundary conditions. The solution had a series form, and the coefficients were solved to match the edge conditions. With the development of economy and technology, multilayer structures under static loading or dynamic loading have been delt with in recent years. Multilayer structures, whose material properties are discontinuous at each interface of the plate, are different from traditional laminated composites. Functionally graded material (FGM) is one of the multilayer structures that is attracting tremendous research interest. The analytical solution for the bending performance of the FGM plate was first introduced in the 1990s [
32]. Afterwards, FGM plates were widely used in engineering structures, and the buckling and dynamic response [
33,
34,
35], post buckling behavior [
36,
37], and elastoplastic mechanical performances [
38,
39,
40] of the FGM plates were introduced.
As far as the authors know, there are few studies about the kinematical equations suitable for a stepped rectangular plate. It also seems to be a fact that there are few research papers on the static bending analysis of stepped rectangular plates resting on the elastic half-space foundation. To bridge this gap, this paper used traditional plate theory that is common in the analysis of single plates resting on an elastic foundation. In order to promote the application of traditional plate theories, the stepped rectangular plate is considered to be composed of two plates with different dimensions and properties (upper and lower plates), and taking into account the thickness of the upper and lower plates, the analytical method is divided into three cases: (1) the upper and lower plates are both thin plates; (2) one plate is a thin plate, while the other one is a moderately thick plate; (3) the upper and lower plates are both moderately thick plates. A Fourier series is used to establish the basic equations and the coordination equation of the plate-foundation system, and the boundary conditions are also used to obtain the analytical solution. In addition, the influence of plate theory, the elastic modulus of the plate-foundation system and dimensions of the plate on the bending performance of the stepped rectangular plate are analyzed.