An Open Database to Evaluate the Fundamental Frequency of Historical Masonry Towers through Empirical and Physics-Based Formulations
Abstract
:1. Introduction
2. Masonry Towers Database
2.1. Parameters Describing the Towers
2.2. Compilation of Data Collection
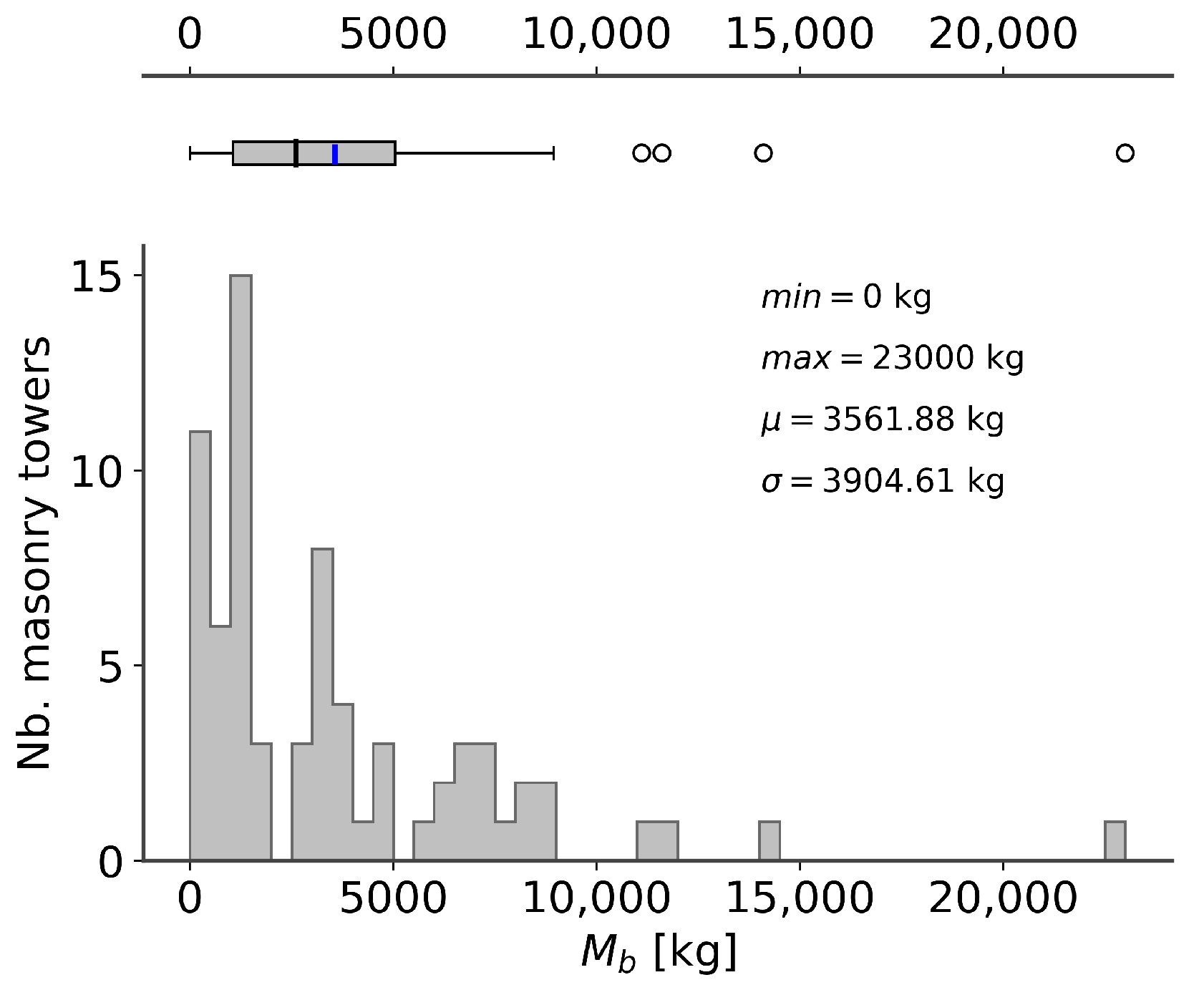
2.3. Statistical Description
3. Model for the Evaluation of the Fundamental Frequency of the Tower
3.1. Empirical Models
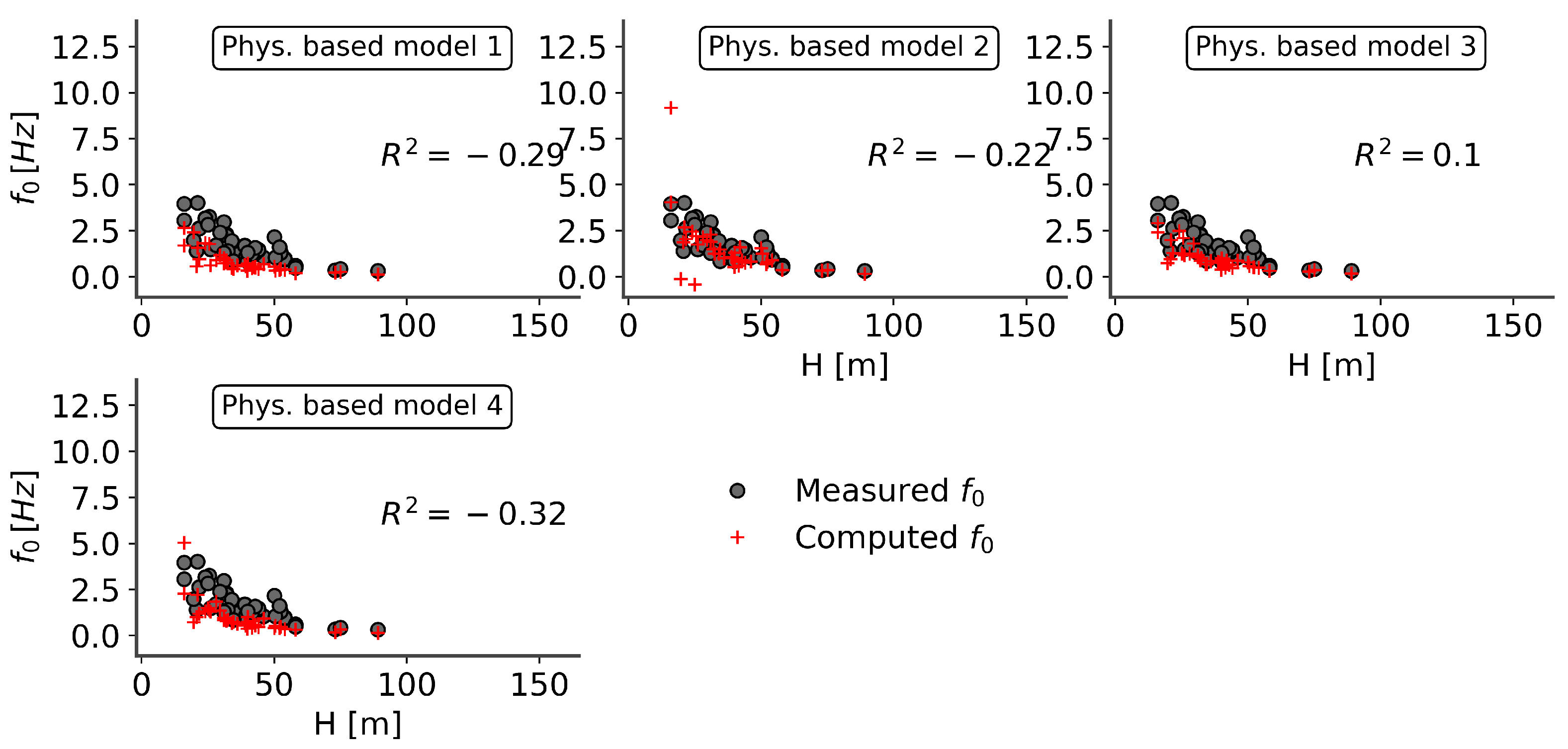
3.2. Physics Based Models
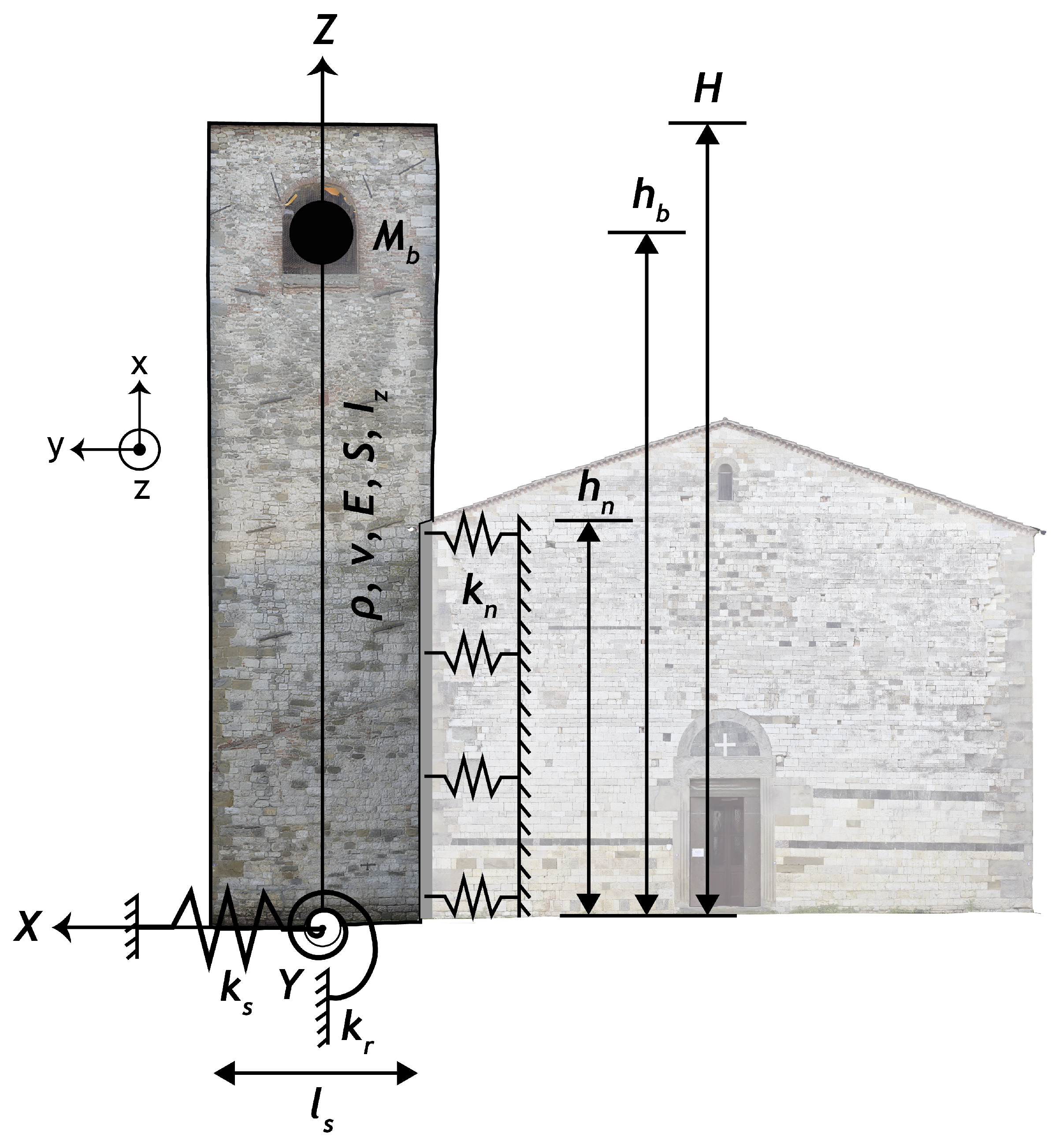
3.3. Description of the Timoshenko Beam
3.4. Rayleigh–Ritz Method
3.5. Model Validation
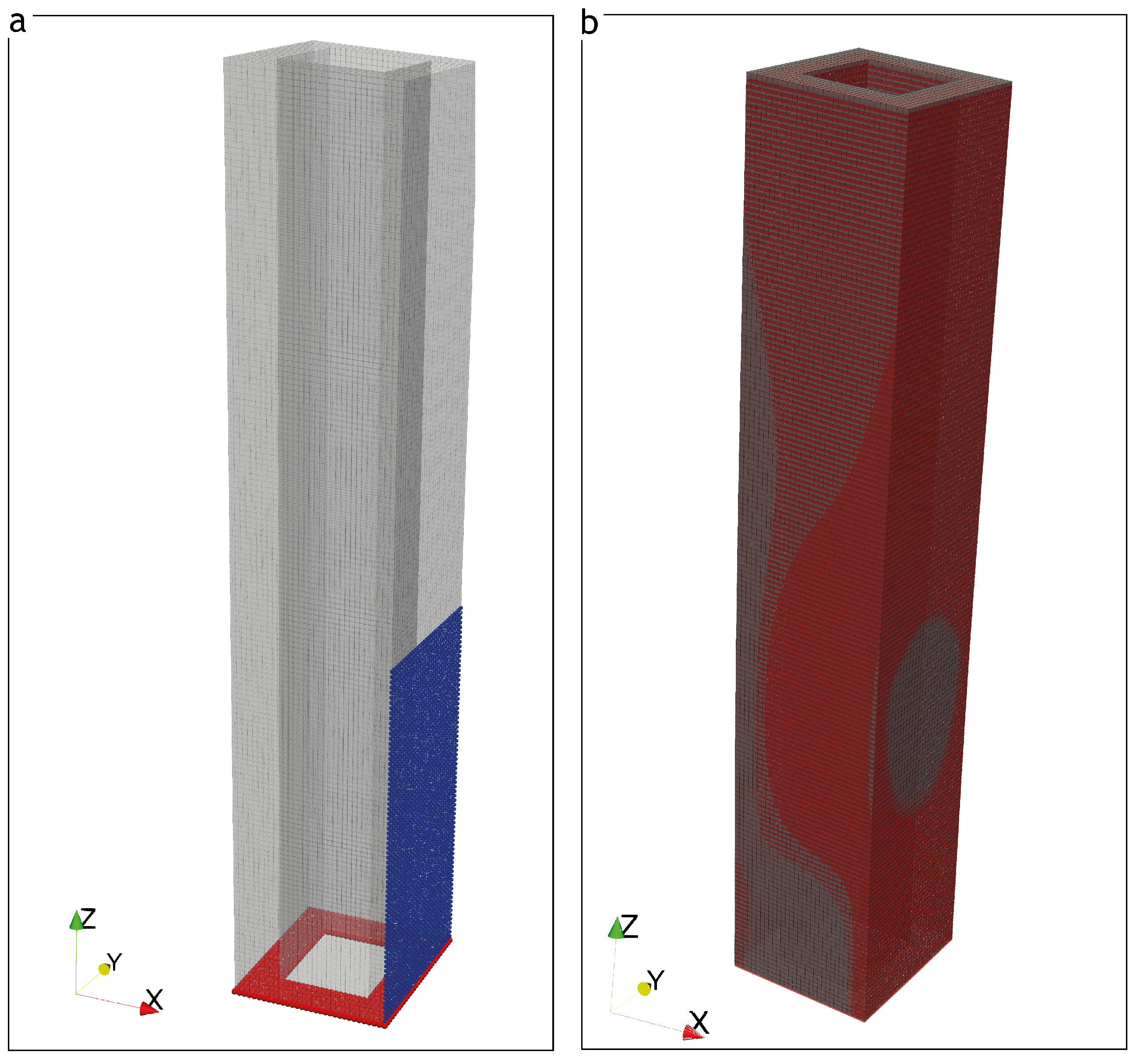
3.5.1. Characteristics of the Reference Tower
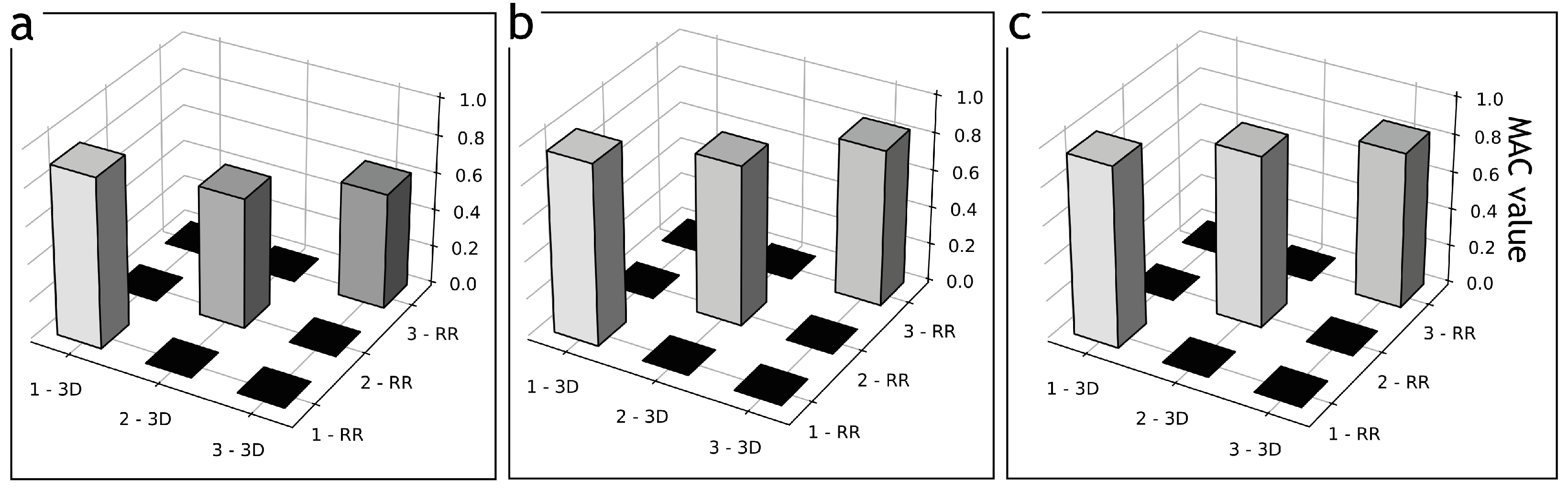
3.5.2. Evaluation of the Error
3.5.3. Results
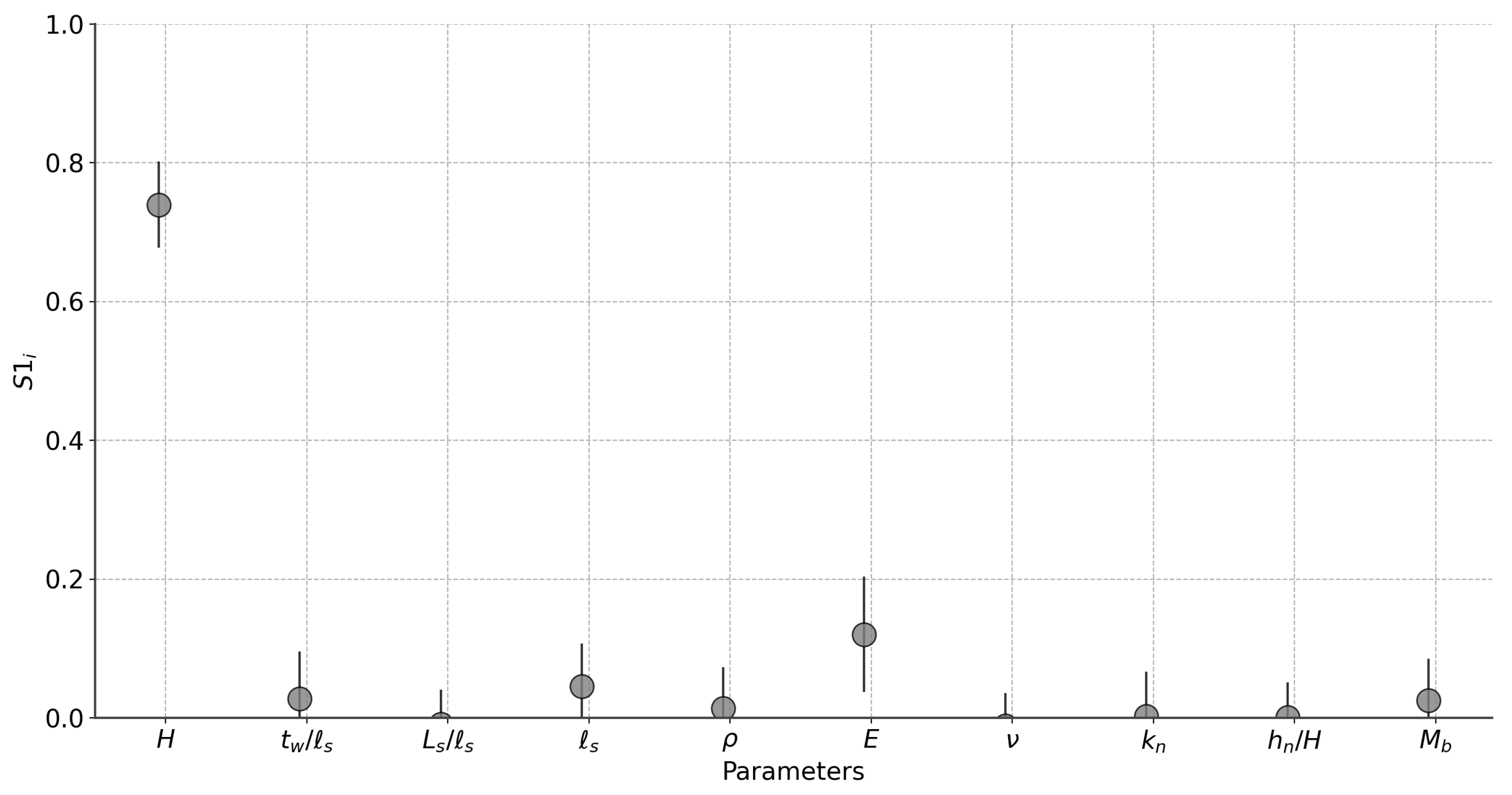
3.6. Sensitivity Analysis
3.6.1. The FAST Method
- Randomly permutes the set of samples for each input variable;
- Run the model using those permuted sets of input variables;
- Reorder the model output according to the input permutation for each input variable.
3.6.2. Sensitivity Analysis of the Rayleigh–Ritz Model
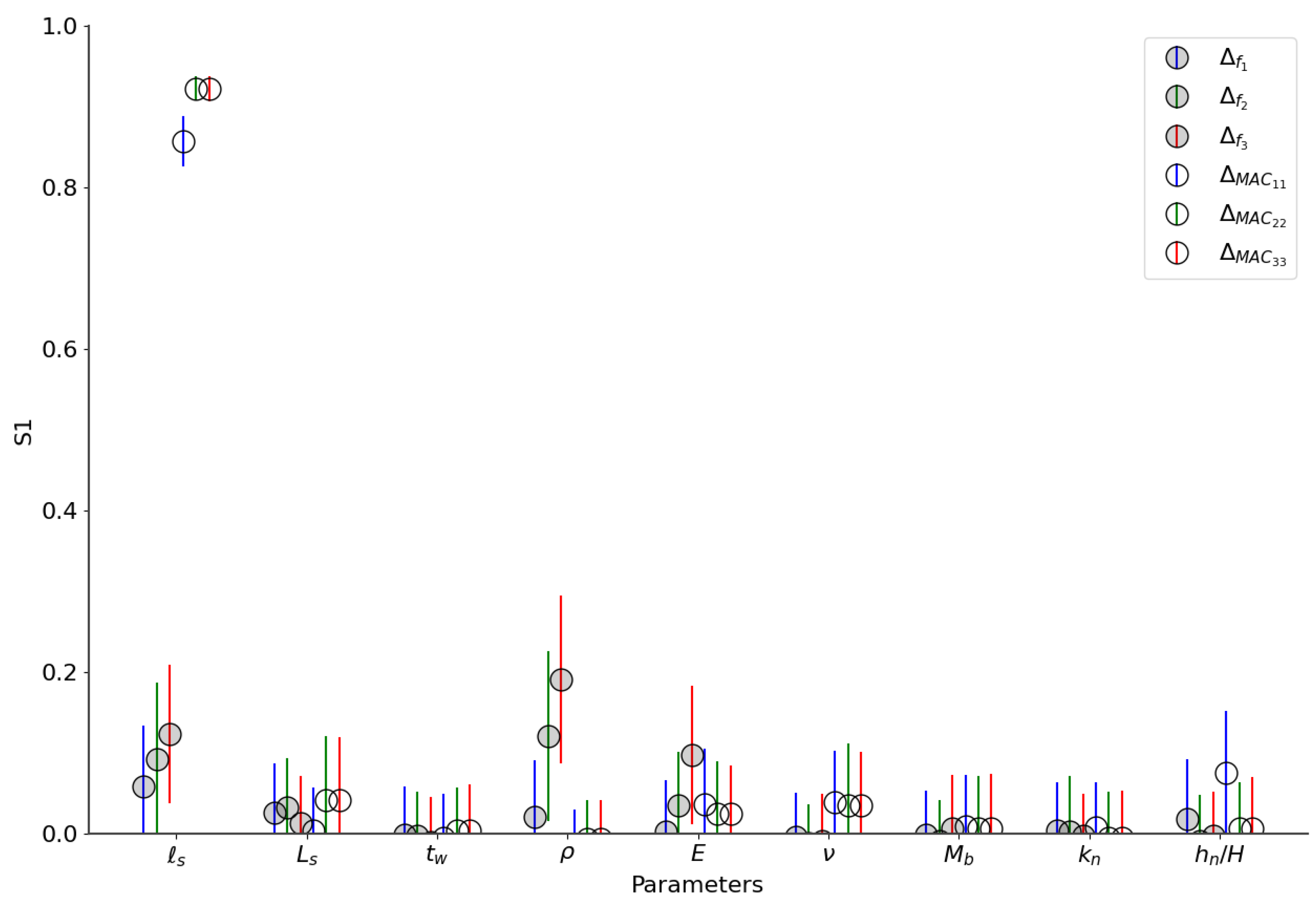
3.6.3. Sensitivity Analysis for Key Parameter Identification
3.7. Comparing Empirical, Physics-Based, and Rayleigh–Ritz Approach for the Evaluation of the Fundamental Frequency
4. Conclusions
- Compiling 244 instrumented masonry towers assembled from an extensive literature review. Worldwide masonry towers are described in terms of geometric, material features, interaction with adjacent buildings, aging, construction phase, repairs, and instrumentation condition;
- Describing the range of each parameter essential for the sensitivity analysis;
- Proposing a generic formulation for empirical and physical models summarizing the ones from the literature (available in the Python script);
- Expressing each feature contribution through a Rayleigh–Ritz formulation (available in the Python script);
- Conducting a sensitivity analysis to quantify how much each feature’s tower impacts the fundamental frequency.
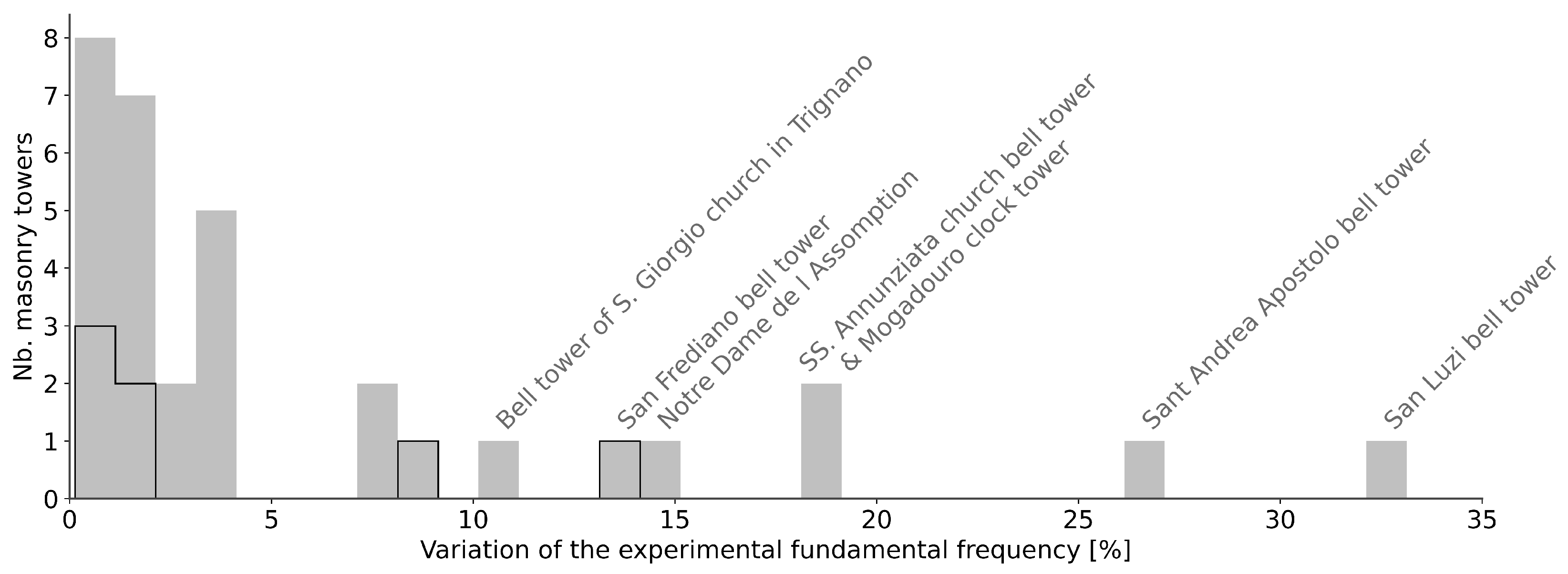
- The variability of the identified experimentally for the same historic tower. When available, most of the repeated OMA surveys highlight a discrepancy of up to 0.05 Hz. This difference is in the range of the contribution of tower features, inviting us to reduce in the uncertainties when evaluating both the fundamental frequency and the tower’s features;
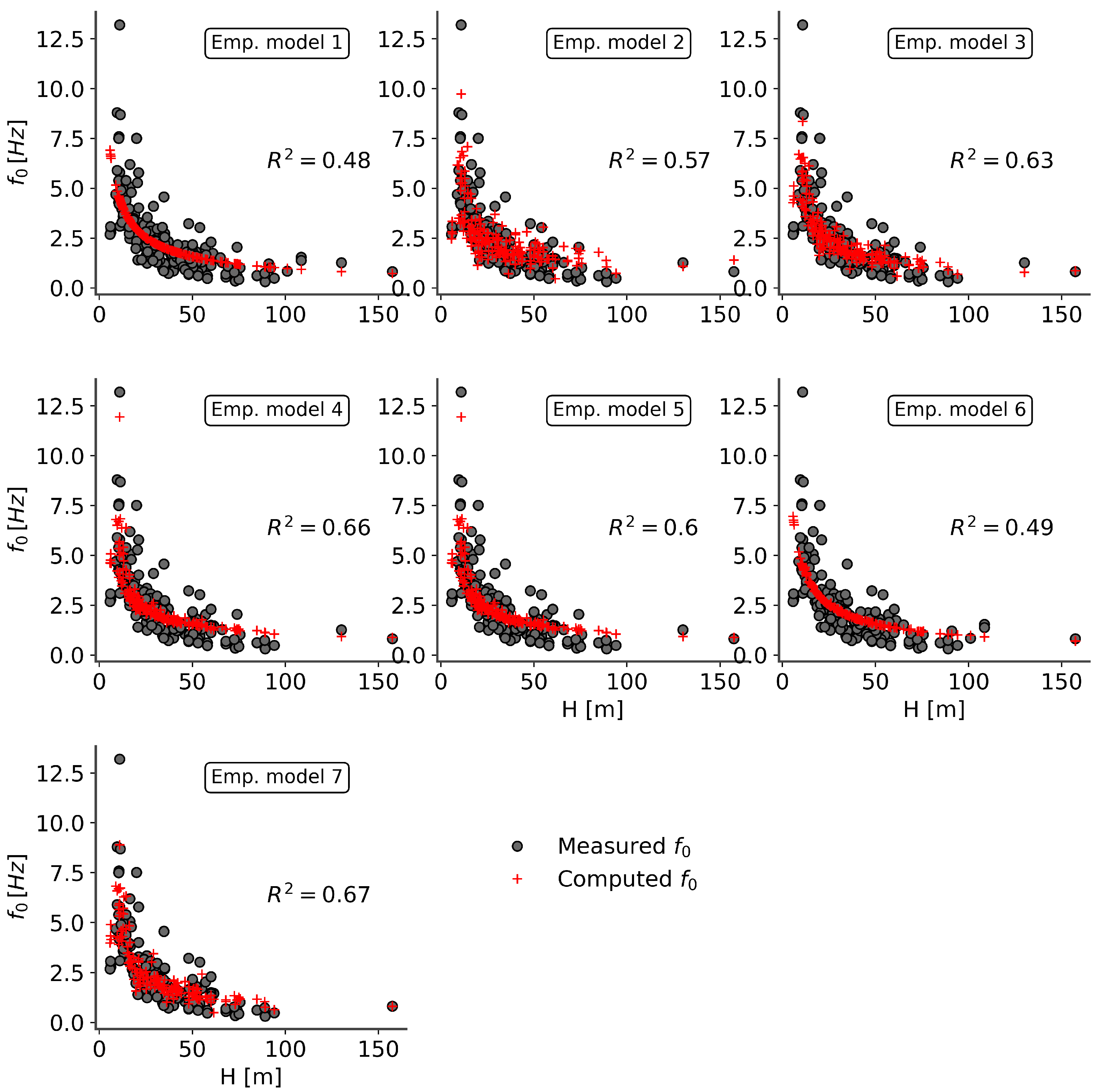
- Empirical relations provide a suitable evaluation of the fundamental frequency compared to physics-based formulations regarding a small number of parameters;
- The Rayleigh–Ritz formulation allows the best fit between experimental and computed fundamental frequency when all information about the towers’ features are available;
- The height of the tower is the critical parameter to evaluate the fundamental frequency. It invites us to take some precautions when evaluating the height of the building. Moreover, the impact of the interaction between the slender structure and the adjacent structure on the fundamental frequency increase with the tower’s height, although as a second-order parameter;
- The width significantly impacts the mode shapes of the three first bending modes. The density and Young modulus impact the frequencies of the second and higher modes. The impact of the height interaction is limited to the first bending modes. The tower’s other features play a second-order role. These values are generally taken as known in model updating processes, which prefer to focus on calibrating unknown material properties. They are measured by visual inspection or more advanced techniques (laser measurements, etc.). We recommend particular attention to minimizing the uncertainty associated with measuring these two parameters.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shakya, M.; Varum, H.; Vicente, R.; Costa, A. Empirical formulation for estimating the fundamental frequency of slender masonry structures. Int. J. Archit. Herit. 2016, 10, 55–66. [Google Scholar] [CrossRef]
- Bartoli, G.; Betti, M.; Marra, A.M.; Monchetti, S. On the role played by the openings on the first frequency of historic masonry towers. Bull. Earthq. Eng. 2020, 18, 427–451. [Google Scholar] [CrossRef]
- Pallarés, F.J.; Betti, M.; Bartoli, G.; Pallarés, L. Structural health monitoring (SHM) and Nondestructive testing (NDT) of slender masonry structures: A practical review. Constr. Build. Mater. 2021, 297, 123768. [Google Scholar] [CrossRef]
- Milani, G.; Casolo, S.; Naliato, A.; Tralli, A. Seismic Assessment of a Medieval Masonry Tower in Northern Italy by Limit, Nonlinear Static, and Full Dynamic Analyses. Int. J. Archit. Herit. 2012, 6, 489–524. [Google Scholar] [CrossRef]
- DPCM. Direttiva del Presidente del Consiglio dei Ministri per Valutazione e Riduzione del Rischio Sismico del Patrimonio Culturale con Riferimento alle Norme Tecniche per le Costruzioni, G.U. n. 47; DPCM: Roma, Italy, 2011. [Google Scholar]
- Brincker, R.; Ventura, C. Introduction to Operational Modal Analysis; John Wiley & Sons: Hoboken, NJ, USA, 2015. [Google Scholar]
- Lund, J.; Selby, A.; Wilson, J. The dynamics of bell towers—A survey in northeast England. WIT Trans. Built Environ. 1995, 17, 8. [Google Scholar]
- Bartoli, G.; Betti, M.; Marra, A.M.; Monchetti, S. Semiempirical Formulations for Estimating the Main Frequency of Slender Masonry Towers. J. Perform. Constr. Facil. 2017, 31. [Google Scholar] [CrossRef]
- Sanchez-Silva, M.; Klutke, G.A.; Rosowsky, D.V. Life-cycle performance of structures subject to multiple deterioration mechanisms. Struct. Saf. 2011, 33, 206–217. [Google Scholar] [CrossRef]
- Price Code. Eurocode 8: Design of Structures for Earthquake Resistance—Part 1: General Rules, Seismic Actions and Rules for Buildings; European Committee for Standardization: Brussels, Belgium, 2005. [Google Scholar]
- Faccio, P.; Podestà, S.; Saetta, A. Venezia, Campanile della Chiesa di Sant’Antonin, Esempio 5. In Linee Guida per la Valutazione e Riduzione del Rischio Sismico del Patrimonio Culturale Allineate alle Nuove Norme Tecniche per le Costruzioni (DM 14/01/2008), Circolare; Ministero della Cultura: Rome, Italy, 2011; Volume 26. [Google Scholar]
- Rainieri, C.; Fabbrocino, G. Estimating the Elastic Period of Masonry Towers. In Topics in Modal Analysis I; Springer: New York, NY, USA, 2012; Volume 5, pp. 243–248. [Google Scholar] [CrossRef]
- Diaferio, M.; Foti, D.; Potenza, F. Prediction of the fundamental frequencies and modal shapes of historic masonry towers by empirical equations based on experimental data. Eng. Struct. 2018, 156, 433–442. [Google Scholar] [CrossRef]
- Ministerio de Fomento. Real Decreto 997/2002, de 27 de septiembre, por el que se aprueba la norma de construcción sismorresistente: Parte general y edificación (NCSR-02). Boletín Oficial del Estado, 11 October 2002. [Google Scholar]
- Formisano, A.; Vituat, R.; Milani, G.; Sarhosis, V. Parametric Seismic Analysis on Masonry Bell Towers; Pisa University Press: Pisa, Italy, 2017; pp. 108–116. [Google Scholar]
- Montabert, A.; Giry, C.; Limoge Schraen, C.; Lépine, J.; Choueiri, C.; Mercerat, E.D.; Guéguen, P. TURRIS: An open source database and Python tools to compute the fundamental frequency of historic masonry towers. Zenodo. 2023. Available online: https://zenodo.org/record/8283641 (accessed on 18 August 2023).
- Schmidt, T. Dynamic behaviour of twin bell towers. In Proceedings of the 2nd International Operational Modal Analysis Conference, Copenhagen, Denmark, 30 April–2 May 2007. [Google Scholar]
- Schmidt, T. FE Comparison of the dynamic behavior of 16 historical twin bell towers. In Proceedings of the 3th International Operational Modal Analysis Conference, Portonovo, Italy, 4–6 May 2009; pp. 483–490. [Google Scholar]
- Rainieri, C.; Fabbrocino, G. Output-only modal identification for prediction of the elastic period of masonry towers. In Proceedings of the 4th International Operational Modal Analysis Conference, Istanbul, Turkey, 9–11 May 2011. [Google Scholar]
- Limoge, C. Méthode de Diagnostic à Grande Échelle de la Vulnérabilité Sismique des Monuments Historiques: Chapelles et Églises Baroques des Hautes Vallées de Savoie: Large-Scale Seismic Vulnerability Assesment Method for the Masonry Architectural Heritage: Baroque Chapels and Churches of the French Savoye. Ph.D. Thesis, Université Paris-Saclay (ComUE), Paris, France, 2016. [Google Scholar]
- Ziegler, A. Dynamik der Glockentürme. In Bauwerksdynamik und Erschütterungsmessungen; Springer Fachmedien: Wiesbaden, Germany, 2017; pp. 153–165. [Google Scholar] [CrossRef]
- Ruiz-Jaramillo, J.; Montiel-Vega, L.; García-Pulido, L.J.; Muñoz-González, C.; Blanca-Hoyos, Á. Ambient Vibration as a Basis for Determining the Structural Behaviour of Watchtowers against Horizontal Loads in Southeast Spain. Appl. Sci. 2020, 10, 6114. [Google Scholar] [CrossRef]
- Mercerat, D.; Montabert, A.; Giry, C.; Lancieri, M.; Arrighetti, A. Operational Modal Analysis of five historical bell towers in the Mugello basin (Tuscany, Italy). In Proceedings of the 3rd European Conference on Earthquake Engineering & Seismology (3ECEES), Bucharest, Romania, 4–9 September, 2022; pp. 4106–4111. [Google Scholar]
- Wimmer, H.; Majer, J. Dynamic behaviour and numerical simulation of old bell towers. In Structural Repair and Maintenance of Historical Buildings; Computational Mechanics Publications: Berlin, Germany, 1989; pp. 349–358. [Google Scholar]
- Modena, C.; Valluzzi, M.; Folli, R.T.; Binda, L. Design choices and intervention techniques for repairing and strengthening of the Monza cathedral bell-tower. Constr. Build. Mater. 2002, 16, 385–395. [Google Scholar] [CrossRef]
- Ivorra, S.; Foti, D.; Diaferio, M.; Carabellese, I. Preliminary OMA results on a soft calcarenite stone bell-tower in Mola di Bari (Italy). In Proceedings of the 7th International Operational Modal Analysis Conference, IOMAC, Ingolstadt, Germany, 10–12 May 2017. [Google Scholar]
- Combey, A.; Mercerat, D.E.; Gueguen, P.; Langlais, M.; Audin, L. Postseismic Survey of a Historic Masonry Tower and Monitoring of Its Dynamic Behavior in the Aftermath of Le Teil Earthquake (Ardèche, France). Bull. Seismol. Soc. Am. 2022, 112, 1101–1119. [Google Scholar] [CrossRef]
- Kohan, P.H.; Nallim, L.G.; Gea, S.B. Dynamic characterization of beam type structures: Analytical, numerical and experimental applications. Appl. Acoust. 2011, 72, 975–981. [Google Scholar] [CrossRef]
- Carone, A.S.; Foti, D.; Giannoccaro, N.I.; Nobile, R. Non-destructive characterization and dynamic identification of an historical bell tower. In Proceedings of the 4th International Conference on Integrity, Reliability and Failure, Funchal, Portugal, 23–27 June 2013; pp. 1–16. [Google Scholar]
- Diaferio, M.; Foti, D.; Giannoccaro, N. Modal parameters identification on environmental tests of an ancient tower and validation of its FE model. Int. J. Mech 2016, 10, 80–89. [Google Scholar]
- Mariella, D.; Dora, F.; Gentile, C.; Ivan Giannoccaro, N.; Saisi, A.E. Dynamic testing of a historical slender building using accelerometers and radar. In Proceedings of the 6th International Operational Modal Analysis Conference, Gijón, Spain, 12–14 May 2015; pp. 1–10. [Google Scholar]
- Stefano, A.D.; Ceravolo, R. Assessing the Health State of Ancient Structures: The Role of Vibrational Tests. J. Intell. Mater. Syst. Struct. 2007, 18, 793–807. [Google Scholar] [CrossRef]
- Lucidi, A.; Giordano, E.; Clementi, F.; Quattrini, R. Point cloud exploitation for structural modeling and analysis: A reliable workflow. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2021, XLIII-B2-2021, 891–898. [Google Scholar] [CrossRef]
- Gentile, C.; Saisi, A. Operational modal testing of historic structures at different levels of excitation. Constr. Build. Mater. 2013, 48, 1273–1285. [Google Scholar] [CrossRef]
- Gentile, C.; Saisi, A.; Cabboi, A. Structural Identification of a Masonry Tower Based on Operational Modal Analysis. Int. J. Archit. Herit. 2014, 9, 98–110. [Google Scholar] [CrossRef]
- Cabboi, A.; Gentile, C.; Saisi, A. From continuous vibration monitoring to FEM-based damage assessment: Application on a stone-masonry tower. Constr. Build. Mater. 2017, 156, 252–265. [Google Scholar] [CrossRef]
- Ferraioli, M.; Mandara, A.; Abruzzese, D.; Miccoli, L. Dynamic identification and seismic safety of masonry bell towers. In Proceedings of the 14th Conference of Associazione Nazionale Italiana di Ingegneria Sismica (ANIDIS), Bari, Italy, 18–22 September 2011; pp. 18–22. [Google Scholar]
- Angelis, A.D.; Lourenço, P.B.; Sica, S.; Pecce, M.R. Influence of the ground on the structural identification of a bell-tower by ambient vibration testing. Soil Dyn. Earthq. Eng. 2022, 155, 107102. [Google Scholar] [CrossRef]
- Pesci, A.; Teza, G.; Bonali, E.; Casula, G.; Boschi, E. A laser scanning-based method for fast estimation of seismic-induced building deformations. ISPRS J. Photogramm. Remote Sens. 2013, 79, 185–198. [Google Scholar] [CrossRef]
- Palermo, M.; Silvestri, S.; Gasparini, G.; Baraccani, S.; Trombetti, T. An approach for the mechanical characterisation of the Asinelli Tower (Bologna) in presence of insufficient experimental data. J. Cult. Herit. 2015, 16, 536–543. [Google Scholar] [CrossRef]
- Carpinteri, A.; Lacidogna, G.; Manuello, A.; Niccolini, G. A study on the structural stability of the Asinelli Tower in Bologna. Struct. Control. Health Monit. 2015, 23, 659–667. [Google Scholar] [CrossRef]
- Invernizzi, S.; Lacidogna, G.; Lozano-Ramírez, N.E.; Carpinteri, A. Structural monitoring and assessment of an ancient masonry tower. Eng. Fract. Mech. 2019, 210, 429–443. [Google Scholar] [CrossRef]
- Milani, G.; Clementi, F. Advanced Seismic Assessment of Four Masonry Bell Towers in Italy after Operational Modal Analysis (OMA) Identification. Int. J. Archit. Herit. 2019, 15, 157–186. [Google Scholar] [CrossRef]
- Standoli, G.; Giordano, E.; Milani, G.; Clementi, F. Model Updating of Historical Belfries Based on Oma Identification Techniques. Int. J. Archit. Herit. 2020, 15, 132–156. [Google Scholar] [CrossRef]
- Colapietro, D.; Fatiguso, F.; Pinto, M.; Bianco, F. Techniques for improving assessment of the seismic vulnerability of a masonry bell tower. Am. J. Eng. Res. (AJER) 2017, 6, 147–155. [Google Scholar]
- Azzara, R.M.; Girardi, M.; Padovani, C.; Pellegrini, D. Dynamic Behaviour of the Carillon Tower in Castel San Pietro, Italy; Technical Report; ISTI: Rome, Italy, 2022. [Google Scholar]
- Gazzani, V.; Poiani, M.; Clementi, F.; Milani, G.; Lenci, S. Modal parameters identification with environmental tests and advanced numerical analyses for masonry bell towers: A meaningful case study. Procedia Struct. Integr. 2018, 11, 306–313. [Google Scholar] [CrossRef]
- Balduzzi, B.; Mazza, D.; Papis, D.; Rossi, C.; Rossi, P.P. Experimental and numerical analysis for the strengthening intervention of the bell tower of St. Sistós Church in Bergamo. In Proceedings of the 5th International Conference on Structural Analysis of Historical Constructions (SAHC), Delhi, India, 6–8 November 2006; pp. 6–8. [Google Scholar]
- Girardi, M.; Padovani, C.; Pellegrini, D.; Robol, L. Model updating procedure to enhance structural analysis in FE code NOSA-ITACA. J. Perform. Constr. Facil. 2019, 33, 04019041. [Google Scholar] [CrossRef]
- Casciati, S.; Al-Saleh, R. Dynamic behavior of a masonry civic belfry under operational conditions. Acta Mech. 2010, 215, 211–224. [Google Scholar] [CrossRef]
- Casciati, S.; Faravelli, L. Vulnerability assessment for medieval civic towers. Struct. Infrastruct. Eng. 2010, 6, 193–203. [Google Scholar] [CrossRef]
- D’Ambrisi, A.; Mariani, V.; Mezzi, M. Seismic assessment of a historical masonry tower with nonlinear static and dynamic analyses tuned on ambient vibration tests. Eng. Struct. 2012, 36, 210–219. [Google Scholar] [CrossRef]
- Casciati, S.; Tento, A.; Marcellini, A.; Daminelli, R. Long run ambient noise recording for a masonry medieval tower. Smart Struct. Syst. 2014, 14, 367–376. [Google Scholar] [CrossRef]
- Bianconi, F.; Salachoris, G.P.; Clementi, F.; Lenci, S. A Genetic Algorithm Procedure for the Automatic Updating of FEM Based on Ambient Vibration Tests. Sensors 2020, 20, 3315. [Google Scholar] [CrossRef] [PubMed]
- Bassoli, E.; Vincenzi, L.; Bovo, M.; Mazzotti, C. Dynamic identification of an ancient masonry bell tower using a MEMS-based acquisition system. In Proceedings of the 2015 IEEE Workshop on Environmental, Energy, and Structural Monitoring Systems (EESMS), Trento, Italy, 9–10 July 2015. [Google Scholar] [CrossRef]
- Bru, D.; Ivorra, S.; Betti, M.; Adam, J.M.; Bartoli, G. Parametric dynamic interaction assessment between bells and supporting slender masonry tower. Mech. Syst. Signal Process. 2019, 129, 235–249. [Google Scholar] [CrossRef]
- Pieraccini, M.; Fratini, M.; Dei, D.; Atzeni, C. Structural testing of Historical Heritage Site Towers by microwave remote sensing. J. Cult. Herit. 2009, 10, 174–182. [Google Scholar] [CrossRef]
- Pieraccini, M.; Dei, D.; Mecatti, D.; Parrini, F. Dynamic Testing of Historic Towers Using an Interferometric Radar from an Unstable Measurement Position. J. Nondestruct. Eval. 2013, 32, 398–404. [Google Scholar] [CrossRef]
- Lacanna, G.; Ripepe, M.; Coli, M.; Genco, R.; Marchetti, E. Full structural dynamic response from ambient vibration of Giotto’s bell tower in Firenze (Italy), using modal analysis and seismic interferometry. NDT&E Int. 2019, 102, 9–15. [Google Scholar] [CrossRef]
- Ceravolo, R.; Pistone, G.; Fragonara, L.Z.; Massetto, S.; Abbiati, G. Vibration-Based Monitoring and Diagnosis of Cultural Heritage: A Methodological Discussion in Three Examples. Int. J. Archit. Herit. 2014, 10, 375–395. [Google Scholar] [CrossRef]
- Camata, G.; Cifelli, L.; Spacone, E.; Conte, J.; Loi, M.; Torrese, P. Seismic Safety Assessment of the Tower of the S. Maria Maggiore Cathedral in Guardiagrele, Italy. In Proceedings of the Ninth International Conference on Computational Structures Technology, Athens, Greece, 2–5 September 2008. [Google Scholar] [CrossRef]
- Capanna, I.; Cirella, R.; Aloisio, A.; Alaggio, R.; Fabio, F.D.; Fragiacomo, M. Operational Modal Analysis, Model Update and Fragility Curves Estimation, through Truncated Incremental Dynamic Analysis, of a Masonry Belfry. Buildings 2021, 11, 120. [Google Scholar] [CrossRef]
- Buffarini, G.P.; Cimellaro, C.G.P.; Stefano, A.D. Experimental dynamic analysis of Palazzo Margherita in L’Aquila after the April 6th, 2009, Earthquake. In Proceedings of the Experimental Vibration Analysis for Civil Engineering Structures (EVACES), Varenna, Italy, 3–5 October 2011; pp. 247–254. [Google Scholar]
- Peeters, B.; Sforza, G.; Sbaraglia, L.; Germano, F. Efficient operational modal testing and analysis for design verification and restoration baseline assessment: Italian case studies. In Proceedings of the Experimental Vibration Analysis for Civil Engineering Structures (EVACES), Varenna, Italy, 3–5 October 2011; pp. 3–5. [Google Scholar]
- Barsocchi, P.; Bartoli, G.; Betti, M.; Girardi, M.; Mammolito, S.; Pellegrini, D.; Zini, G. Wireless Sensor Networks for Continuous Structural Health Monitoring of Historic Masonry Towers. Int. J. Archit. Herit. 2020, 15, 22–44. [Google Scholar] [CrossRef]
- Zonta, D.; Pozzi, M.; Zanon, P.; Anese, G.; Busetto, A. Real-time probabilistic health monitoring of the Portogruaro Civic Tower. In Structural Analysis of Historic Construction: Preserving Safety and Significance, Two Volume Set; CRC Press: Boca Raton, FL, USA, 2008; pp. 743–752. [Google Scholar]
- Azzara, R.M.; Roeck, G.D.; Girardi, M.; Padovani, C.; Pellegrini, D.; Reynders, E. The influence of environmental parameters on the dynamic behaviour of the San Frediano bell tower in Lucca. Eng. Struct. 2018, 156, 175–187. [Google Scholar] [CrossRef]
- Barsocchi, P.; Cassara, P.; Mavilia, F.; Pellegrini, D. Sensing a city’s state of health: Structural monitoring system by internet-of-things wireless sensing devices. IEEE Consum. Electron. Mag. 2018, 7, 22–31. [Google Scholar] [CrossRef]
- Azzara, R.M.; Girardi, M.; Iafolla, V.; Padovani, C.; Pellegrini, D. Long-Term Dynamic Monitoring of Medieval Masonry Towers. Front. Built Environ. 2020, 6, 9. [Google Scholar] [CrossRef]
- Saisi, A.; Gentile, C.; Guidobaldi, M. Post-earthquake continuous dynamic monitoring of the Gabbia Tower in Mantua, Italy. Constr. Build. Mater. 2015, 81, 101–112. [Google Scholar] [CrossRef]
- Saisi, A.; Gentile, C. Post-earthquake diagnostic investigation of a historic masonry tower. J. Cult. Herit. 2015, 16, 602–609. [Google Scholar] [CrossRef]
- Saisi, A.; Gentile, C.; Ruccolo, A. Pre-diagnostic prompt investigation and static monitoring of a historic bell-tower. Constr. Build. Mater. 2016, 122, 833–844. [Google Scholar] [CrossRef]
- Gentile, C.; Guidobaldi, M.; Saisi, A. One-year dynamic monitoring of a historic tower: Damage detection under changing environment. Meccanica 2016, 51, 2873–2889. [Google Scholar] [CrossRef]
- Cavalagli, N.; Comanducci, G.; Gentile, C.; Guidobaldi, M.; Saisi, A.; Ubertini, F. Detecting earthquake-induced damage in historic masonry towers using continuously monitored dynamic response-only data. Procedia Eng. 2017, 199, 3416–3421. [Google Scholar] [CrossRef]
- Magrinelli, E.; Acito, M.; Bocciarelli, M. Numerical insight on the interaction effects of a confined masonry tower. Eng. Struct. 2021, 237, 112195. [Google Scholar] [CrossRef]
- Gentile, C.; Saisi, A. Dynamic Testing of Masonry Towers Using the Microwave Interferometry. Key Eng. Mater. 2014, 628, 198–203. [Google Scholar] [CrossRef]
- Saisi, A.; Terenzoni, S.; Ruccolo, A.; Gentile, C. Safety of the Architectural Heritage: Structural Assessment of the Zuccaro’s Tower in Mantua. In RILEM Bookseries; Springer International Publishing: Berlin/Heidelberg, Germany, 2019; pp. 2422–2430. [Google Scholar] [CrossRef]
- Fragonara, L.Z.; Boscato, G.; Ceravolo, R.; Russo, S.; Ientile, S.; Pecorelli, M.L.; Quattrone, A. Dynamic investigation on the Mirandola bell tower in post-earthquake scenarios. Bull. Earthq. Eng. 2016, 15, 313–337. [Google Scholar] [CrossRef]
- Lancellotta, R.; Sabia, D. Identification Technique for Soil-Structure Analysis of the Ghirlandina Tower. Int. J. Archit. Herit. 2014, 9, 391–407. [Google Scholar] [CrossRef]
- Foti, D.; Ivorra, S.; Sabbà, M.F. Dynamic Investigation of an Ancient Masonry Bell Tower with Operational Modal Analysis—Non-Destructive Experimental Technique to Obtain the Dynamic Characteristics of a Structure. Open Constr. Build. Technol. J. 2012, 6, 384–391. [Google Scholar] [CrossRef]
- Clementi, F.; Pierdicca, A.; Formisano, A.; Catinari, F.; Lenci, S. Numerical model upgrading of a historical masonry building damaged during the 2016 Italian earthquakes: The case study of the Podestà palace in Montelupone (Italy). J. Civ. Struct. Health Monit. 2017, 7, 703–717. [Google Scholar] [CrossRef]
- Saisi, A.; Gentile, C.; Ruccolo, A. Continuous monitoring of a challenging heritage tower in Monza, Italy. J. Civ. Struct. Health Monit. 2017, 8, 77–90. [Google Scholar] [CrossRef]
- Lorenzoni, F.; Modena, C.; Caldon, M.; Cohen, M.; Kislev, R.; Schaffer, Y. Structural health monitoring of heritage sites: The tower of David in Jerusalem. In Structural Analysis of Historical Constructions: Anamnesis, Diagnosis, Therapy, Controls; CRC Press: Boca Raton, FL, USA, 2016; pp. 745–751. [Google Scholar] [CrossRef]
- Montabert, A.; Mercerat, E.D.; Clément, J.; Langlaude, P.; Lyon-Caen, H.; Lancieri, M. High resolution operational modal analysis of Sant’Agata del Mugello in light of its building history. Eng. Struct. 2022, 254, 113767. [Google Scholar] [CrossRef]
- Osmancikli, G.; Uçak, Ş.; Turan, F.N.; Türker, T.; Bayraktar, A. Investigation of restoration effects on the dynamic characteristics of the Hagia Sophia bell-tower by ambient vibration test. Constr. Build. Mater. 2012, 29, 564–572. [Google Scholar] [CrossRef]
- Bayraktar, A.; Türker, T.; Sevım, B.; Altunişik, A.C.; Yildirim, F. Modal Parameter Identification of Hagia Sophia Bell-Tower via Ambient Vibration Test. J. Nondestruct. Eval. 2009, 28, 37–47. [Google Scholar] [CrossRef]
- Cantieni, R. One-Year Monitoring of a Historic Bell Tower. Key Eng. Mater. 2014, 628, 73–78. [Google Scholar] [CrossRef]
- Ivorra, S.; Pallarés, F.J.; Adam, J.M. Experimental and Numerical Results from the Seismic Study of a Masonry Bell Tower. Adv. Struct. Eng. 2009, 12, 287–293. [Google Scholar] [CrossRef]
- Ivorra, S.; Pallarés, F.J. Dynamic investigations on a masonry bell tower. Eng. Struct. 2006, 28, 660–667. [Google Scholar] [CrossRef]
- Ivorra, S.; Pallarés, F.J.; Adam, J.M.; Tomás, R. An evaluation of the incidence of soil subsidence on the dynamic behaviour of a Gothic bell tower. Eng. Struct. 2010, 32, 2318–2325. [Google Scholar] [CrossRef]
- Ivorra, S.; Cervera, J.R. Analysis of the dynamic actions when bells are swinging on the bell tower of Bonreposi Mirambell Church (Valencia, Spain). In Proceedings of the 3rd international Seminar of Historical Constructions, Guimarães, Portugal, 7–9 November 2001; Volume 413, p. 19. [Google Scholar]
- Cunha, Á.; Ramos, L.F.; Magalhães, F.; Lourenço, P.B. Dynamic identification and modelling of Clérigos Tower: Initial studies. In Proceedings of the EURODYN 2014—9th International Conference on Structural Dynamics, Porto, Portugal, 30 June–2 July 2014. [Google Scholar]
- Ramos, L.; Marques, L.; Lourenço, P.; Roeck, G.D.; Campos-Costa, A.; Roque, J. Monitoring historical masonry structures with operational modal analysis: Two case studies. Mech. Syst. Signal Process. 2010, 24, 1291–1305. [Google Scholar] [CrossRef]
- Guerreiro, L.; Azevedo, J. Análise e reforço da torres do relógio da Horta, Faial. In Proceedings of the 5th Encontro National de Sismologia e Engenharia Sismica, Azores, Portugal, 24–27 October 2001; pp. 639–650. [Google Scholar]
- Júlio, E.N.B.S.; da Silva Rebelo, C.A.; da Costa, D.A.S.G.D. Structural assessment of the tower of the University of Coimbra by modal identification. Eng. Struct. 2008, 30, 3468–3477. [Google Scholar] [CrossRef]
- Tomaszewska, A. Influence of statistical errors on damage detection based on structural flexibility and mode shape curvature. Comput. Struct. 2010, 88, 154–164. [Google Scholar] [CrossRef]
- Tomaszewska, A.; Szymczak, C. Identification of the Vistula Mounting tower model using measured modal data. Eng. Struct. 2012, 42, 342–348. [Google Scholar] [CrossRef]
- Shabani, A.; Ademi, A.; Kioumarsi, M. Structural Model Updating of a Historical Stone Masonry Tower in Tønsberg, Norway. In Lecture Notes in Civil Engineering; Springer International Publishing: Berlin/Heidelberg, Germany, 2021; pp. 576–585. [Google Scholar] [CrossRef]
- Jaras, A.; Kliukas, R.; Kačianauskas, R. The dynamic loading of Vilnius archcathedral belfry–Investigation and analysis. In Proceedings of the 10th International Conference Modern Building Materials, Structures and Techniques, Vilnius, Lithuania, 19–21 May 2010. [Google Scholar]
- Ribilotta, E.; Giordano, E.; Ferrante, A.; Clementi, F.; Lenci, S. Tracking Modal Parameter Evolution of Different Cultural Heritage Structure Damaged by Central Italy Earthquake of 2016. Key Eng. Mater. 2019, 817, 334–341. [Google Scholar] [CrossRef]
- Pavlovic, M.; Trevisani, S.; Cecchi, A. A Procedure for the Structural Identification of Masonry Towers. J. Nondestruct. Eval. 2019, 38. [Google Scholar] [CrossRef]
- Russo, G.; Bergamo, O.; Damiani, L.; Lugato, D. Experimental analysis of the “Saint Andrea” Masonry Bell Tower in Venice. A new method for the determination of “Tower Global Young’s Modulus E”. Eng. Struct. 2010, 32, 353–360. [Google Scholar] [CrossRef]
- Bergamo, O.; Campione, G.; Russo, G. Testing of “Global Young’s Modulus E” on a rehabilitated masonry bell tower in Venice. Eng. Fail. Anal. 2017, 74, 202–217. [Google Scholar] [CrossRef]
- Rosa Valluzzi, M.; Da Porto, F.; Casarin, F.; Monteforte, N.; Modena, C. A contribution to the characterization of masonry typologies by using sonic waves investigations. Actes J. Sci. LCPC 2009, 1, 713–718. [Google Scholar]
- Colapietro, D.; Fiore, A.; Netti, A.; Fatiguso, F.; Marano, G.; de Fino, M.; Cascella, D.; Ancona, A. Dynamic Identification and evaluation of the seismic safety of a masonry bell tower in the osuth of Italy. In Proceedings of the 4th International Conference on Computational Methods in Structural Dynamics and Earthquake Engineering (COMPDYN 2013), Athens, Greece, 12–14 June 2013. [Google Scholar] [CrossRef]
- Bongiovanni, G.; Clemente, P.; Buffarini, G. Analysis of the seismic response of a damaged masonry bell tower. In Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand, 30 January–4 February 2000; Volume 30. [Google Scholar]
- Ceriotti, M.; Mottola, L.; Picco, G.P.; Murphy, A.L.; Guna, S.; Corra, M.; Pozzi, M.; Zonta, D.; Zanon, P. Monitoring heritage buildings with wireless sensor networks: The Torre Aquila deployment. In Proceedings of the 2009 International Conference on Information Processing in Sensor Networks, San Francisco, CA, USA, 13–16 April 2009; pp. 277–288. [Google Scholar]
- Sepe, V.; Speranza, E.; Viskovic, A. A method for large-scale vulnerability assessment of historic towers. Struct. Control. Health Monit. 2008, 15, 389–415. [Google Scholar] [CrossRef]
- Ivorra, S.; Giannoccaro, N.I.; Foti, D. Simple model for predicting the vibration transmission of a squat masonry tower by base forced vibrations. Struct. Control. Health Monit. 2019, 26, e2360. [Google Scholar] [CrossRef]
- Diaferio, M.; Foti, D.; Giannoccaro, N.I.; Ivorra, S. Identification of the modal properties of an historic masonry clock tower. In Proceedings of the SAHC2014—9th International Conference on Structural Analysis of Historical Constructions, Mexico City, Mexico, 14–17 October 2014; Volume 6. [Google Scholar]
- Diaferio, M.; Foti, D.; Giannoccaro, N.; Vitti, M. On the use of modal analysis and ground penetrating radar test for the physical parameter identification of an historical bell tower. In Proceedings of the Vienna Congress on Recent Advances in Earthquake Engineering and structural Dynamics, Vienna, Austria, 28–30 August 2013; pp. 28–30. [Google Scholar]
- Foti, D.; Diaferio, M.; Venerito, M. Non-Destructive Damage Detection and Retrofitting Techniques on a Historical Masonry Tower. In Proceedings of the 3rd International Balkans Conference on Challenges of Civil Engineering, Epoka, Albania, 19–21 May 2016. [Google Scholar]
- Diaferio, M.; Foti, D. Seismic risk assessment of Trani’s Cathedral bell tower in Apulia, Italy. Int. J. Adv. Struct. Eng. 2017, 9, 259–267. [Google Scholar] [CrossRef]
- Pieraccini, M.; Dei, D.; Betti, M.; Bartoli, G.; Tucci, G.; Guardini, N. Dynamic identification of historic masonry towers through an expeditious and no-contact approach: Application to the “Torre del Mangia” in Siena (Italy). J. Cult. Herit. 2014, 15, 275–282. [Google Scholar] [CrossRef]
- Pelella, T.; Mannara, G.; Cosenza, E.; Iervolino, I.; Lecce, L. Structural dynamic investigations on the bell tower from the S. Lucia’s church–Serra S. Quirico, Ancona. In Proceedings of the 7th International Seminar on Seismic Isolation, Passive Energy Dissipation and Active Control of Vibrations of Structures, Assisi, Italy, 2–5 October 2001; pp. 2–5. [Google Scholar]
- Cosenza, E.; Iervolino, I. Case Study: Seismic Retrofitting of a Medieval Bell Tower with FRP. J. Compos. Constr. 2007, 11, 319–327. [Google Scholar] [CrossRef]
- Ferraioli, M.; Miccoli, L.; Abruzzese, D. Dynamic characterisation of a historic bell-tower using a sensitivity-based technique for model tuning. J. Civ. Struct. Health Monit. 2018, 8, 253–269. [Google Scholar] [CrossRef]
- Beconcini, M.L.; Bennati, S.; Salvatore, W. Structural characterisation of a medieval bell tower: First historical, experimental and numerical investigations. HIstor. Constr. 2001, 431–444. [Google Scholar]
- Bennati, S.; Nardini, L.; Salvatore, W. Dynamic Behavior of a Medieval Masonry Bell Tower. II: Measurement and Modeling of the Tower Motion. J. Struct. Eng. 2005, 131, 1656–1664. [Google Scholar] [CrossRef]
- Pieraccini, M. Extensive Measurement Campaign Using Interferometric Radar. J. Perform. Constr. Facil. 2017, 31. [Google Scholar] [CrossRef]
- Bartoli, G.; Betti, M.; Giordano, S. In situ static and dynamic investigations on the “Torre Grossa” masonry tower. Eng. Struct. 2013, 52, 718–733. [Google Scholar] [CrossRef]
- Zini, G.; Betti, M.; Bartoli, G.; Chiostrini, S. Frequency vs. time domain identification of heritage structures. Procedia Struct. Integr. 2018, 11, 460–469. [Google Scholar] [CrossRef]
- Bassoli, E.; Vincenzi, L.; Altri, A.M.D.; de Miranda, S.; Forghieri, M.; Castellazzi, G. Ambient vibration-based finite element model updating of an earthquake-damaged masonry tower. Struct. Control. Health Monit. 2018, 25, e2150. [Google Scholar] [CrossRef]
- Castellano, A.; Fraddosio, A.; Martorano, F.; Mininno, G.; Paparella, F.; Piccioni, M.D. Structural health monitoring of a historic masonry bell tower by radar interferometric measurements. In Proceedings of the 2018 IEEE Workshop on Environmental, Energy, and Structural Monitoring Systems (EESMS), Salerno, Italy, 21–21 June 2018. [Google Scholar] [CrossRef]
- Clementi, F.; Ferrante, A.; Ribilotta, E.; Milani, G.; Lenci, S. On the Dynamics of the Civic Clock Tower of Rotella (Ascoli Piceno) Severly Damaged by the Central Italy Seismic Sequence of 2016; Pisa University Press: Pisa, Italy, 2019; pp. 81–89. [Google Scholar]
- Abruzzese, D.; Vari, A. Vulnerabilità sismica di torri medievali in muratura. In Proceedings of the XI ANIDIS Conference, Genova, Italy, 25–29 June 2004. [Google Scholar]
- Bonato, P.; Ceravolo, R.; Stephano, A.D.; Molinari, F. Cross-time frequency techniques for the identification of masonry buildings. Mech. Syst. Signal Process. 2000, 14, 91–109. [Google Scholar] [CrossRef]
- Castagnetti, C.; Bassoli, E.; Vincenzi, L.; Mancini, F. Dynamic Assessment of Masonry Towers Based on Terrestrial Radar Interferometer and Accelerometers. Sensors 2019, 19, 1319. [Google Scholar] [CrossRef] [PubMed]
- Vincenzi, L.; Bassoli, E.; Ponsi, F.; Castagnetti, C.; Mancini, F. Dynamic monitoring and evaluation of bell ringing effects for the structural assessment of a masonry bell tower. J. Civ. Struct. Health Monit. 2019, 9, 439–458. [Google Scholar] [CrossRef]
- Pieraccini, M.; Parrini, F.; Dei, D.; Fratini, M.; Atzeni, C.; Spinelli, P. Dynamic characterization of a bell tower by interferometric sensor. NDT&E Int. 2007, 40, 390–396. [Google Scholar] [CrossRef]
- Castellacci, I.; Spinelli, P.; Vignoli, A.; Galano, L. Caratterizzazione dinamica del campanile della pieve di San Cresci a Macioli nei pressi di Pratolino, comune di Vaglia, e progetto di miglioramento sismico. Boll. Ing. 2007, 10, 21–23. [Google Scholar]
- Monchetti, S. On the Role of Uncertainties in the Seismic Risk Assessment of Historic Masonry Towers. Ph.D. Thesis, Technische Universität Braunschweig, Braunschweig, Germany, 2018. [Google Scholar]
- Zonta, D.; Pozzi, M. The remarkable story of Portogruaro Civic Tower’s probabilistic health monitoring. Struct. Monit. Maint. 2015, 2, 301–318. [Google Scholar] [CrossRef]
- Lorenzoni, F.; Valluzzi, M.; Salvalaggio, M.; Minello, A.; Modena, C. Operational modal analysis for the characterization of ancient water towers in Pompeii. Procedia Eng. 2017, 199, 3374–3379. [Google Scholar] [CrossRef]
- Atzeni, C.; Bicci, A.; Dei, D.; Fratini, M.; Pieraccini, M. Remote Survey of the Leaning Tower of Pisa by Interferometric Sensing. IEEE Geosci. Remote Sens. Lett. 2010, 7, 185–189. [Google Scholar] [CrossRef]
- García-Macías, E.; Ierimonti, L.; Venanzi, I.; Ubertini, F. An Innovative Methodology for Online Surrogate-Based Model Updating of Historic Buildings Using Monitoring Data. Int. J. Archit. Herit. 2019, 15, 92–112. [Google Scholar] [CrossRef]
- García-Macías, E.; Venanzi, I.; Ubertini, F. Metamodel-based pattern recognition approach for real-time identification of earthquake-induced damage in historic masonry structures. Autom. Constr. 2020, 120, 103389. [Google Scholar] [CrossRef]
- García-Macías, E.; Kita, A.; Ubertini, F. Synergistic application of operational modal analysis and ambient noise deconvolution interferometry for structural and damage identification in historic masonry structures: Three case studies of Italian architectural heritage. Struct. Health Monit. 2019, 19, 1250–1272. [Google Scholar] [CrossRef]
- García-Macías, E.; Ubertini, F. Automated operational modal analysis and ambient noise deconvolution interferometry for the full structural identification of historic towers: A case study of the Sciri Tower in Perugia, Italy. Eng. Struct. 2020, 215, 110615. [Google Scholar] [CrossRef]
- Gentile, C.; Ruccolo, A.; Saisi, A. Long-Term Vibration Measurements to Enhance the Knowledge of a Historic Bell-Tower. In RILEM Bookseries; Springer International Publishing: Berlin/Heidelberg, Germany, 2019; pp. 2236–2244. [Google Scholar] [CrossRef]
- Gentile, C.; Saisi, A. Ambient vibration testing of historic masonry towers for structural identification and damage assessment. Constr. Build. Mater. 2007, 21, 1311–1321. [Google Scholar] [CrossRef]
- Ceroni, F.; Pecce, M.; Manfredi, G. Seismic Assessment of the Bell Tower of Santa Maria Del Carmine: Problems and Solutions. J. Earthq. Eng. 2009, 14, 30–56. [Google Scholar] [CrossRef]
- Cavaleri, L.; Ferrotto, M.F.; Trapani, F.D.; Vicentini, A. Vibration Tests and Structural Identification of the Bell Tower of Palermo Cathedral. Open Constr. Build. Technol. J. 2019, 13, 319–330. [Google Scholar] [CrossRef]
- Ubertini, F.; Comanducci, G.; Cavalagli, N.; Pisello, A.L.; Materazzi, A.L.; Cotana, F. Environmental effects on natural frequencies of the San Pietro bell tower in Perugia, Italy, and their removal for structural performance assessment. Mech. Syst. Signal Process. 2017, 82, 307–322. [Google Scholar] [CrossRef]
- Tsogka, C.; Daskalakis, E.; Comanducci, G.; Ubertini, F. The Stretching Method for Vibration-Based Structural Health Monitoring of Civil Structures. Comput.-Aided Civ. Infrastruct. Eng. 2017, 32, 288–303. [Google Scholar] [CrossRef]
- Hinzen, K.G.; Fleischer, C.; Schock-Werner, B.; Schweppe, G. Seismic Surveillance of Cologne Cathedral. Seismol. Res. Lett. 2012, 83, 9–22. [Google Scholar] [CrossRef]
- Kuhlmann, W.; Butenweg, C.; López, M.; Fernández, S. Seismic Vulnerability Assessment of the Historic Aachen Cathedral Germany. In Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, BC, Canada, 1–6 August 2004. [Google Scholar]
- Uglešić, D.; Uglešić, A. Case Studies of structural natural frequencies assessment and application in SHM and the calibration of FEM models. In Proceedings of the 1st Croatian Conference on Earthquake Engineering, Zagreb, Croatia, 22–24 March 2021. [Google Scholar] [CrossRef]
- Gentile, C.; Canali, F. Continuous Monitoring the Cathedral of Milan: Design, Installation and Preliminary Results. In Proceedings of the the 18th International Conference on Experimental Mechanics, Brussels, Belgium, 1–5 June 2018. [Google Scholar] [CrossRef]
- Busca, G.; Cappellini, A.; Cigada, A.; Vanali, M. Operational modal Analysis of the “Guglia Maggiore” of the “Duomo” in Milano. In Proceedings of the 4th International Operational Modal Analysis Conference IOMAC 2011, Istanbul, Turkey, 9–11 May 2011; pp. 1–8. [Google Scholar]
- Wilson, J.M.; Selby, A.R. Durham Cathedral tower vibrations during bell-ringing. In Engineering a Cathedral; Thomas Telford Publishing: London, UK, 1993; pp. 77–100. [Google Scholar] [CrossRef]
- Rebelo, C.; Júlio, E.; Costa, D. Modal identification of the Coimbra University tower. In Proceedings of the 2nd International Operational Modal Analysis Conference, Copenhagen, Denmark, 30 April–2 May 2007; pp. 177–184. [Google Scholar]
- Foti, D.; Diaferio, M.; Giannoccaro, N.I.; Mongelli, M. Ambient vibration testing, dynamic identification and model updating of a historic tower. NDT&E Int. 2012, 47, 88–95. [Google Scholar] [CrossRef]
- Garcia Garcia, I.; Pardo, A.; Pelayo, F.; Martin, A.; Aenlle Lopez, M. Modal analysis of the tower of the Laboral City of Culture (Conference Paper). In Proceedings of the 6th International Operational Modal Analysis Conference, Gijon, Spain, 12–14 May 2015. [Google Scholar]
- Rainieri, C.; Gargaro, D.; Fabbrocino, G. The role of operational modal analysis in the non-destructive assessment of an Italian Monument. In Proceedings of the 6th International Operational Modal Analysis Conference, Gijon, Spain, 12–14 May 2015. [Google Scholar]
- Jardim, C.M.; Mendes, L.A.; Gonçalve, A.M. Dynamic characterization of the Funchal’s cathedral bell tower. In Proceedings of the 5th International Operational Modal Analysis Conference, Guimaraes, Portugal, 13–15 May 2013. [Google Scholar]
- Salachoris, G.P.; Standoli, G.; Betti, M.; Milani, G.; Clementi, F. Evolutionary numerical model for cultural heritage structures via genetic algorithms: A case study in central Italy. Bull. Earthq. Eng. 2023. [Google Scholar] [CrossRef]
- Patron-Solares, A.; Cremona, C.; Bottineau, C.; Leconte, R.; Goepfer, F. Study of bell swinging induced vibrations of bell tower of Metz cathedral (France). Actes J. Sci. LCPC 2005, 1, 529–536. [Google Scholar]
- Ditommaso, R.; Mucciarelli, M.; Parolai, S.; Picozzi, M. Monitoring the structural dynamic response of a masonry tower: Comparing classical and time-frequency analyses. Bull. Earthq. Eng. 2012, 10, 1221–1235. [Google Scholar] [CrossRef]
- Kolaj, M.; Adams, J. Dynamic characteristics of Canada’s Parliament Hill towers from ambient vibrations and recorded earthquake data. Can. J. Civ. Eng. 2021, 48, 16–25. [Google Scholar] [CrossRef]
- Peña, F.; Manzano, J. Dynamical Characterization of Typical Mexican Colonial Churches. In Computational Methods in Applied Sciences; Springer International Publishing: Berlin/Heidelberg, Germany, 2015; pp. 297–319. [Google Scholar] [CrossRef]
- Manos, G.; Kozikopoulos, E. In-situ measured dynamic response of the bell tower of Agios Gerasimos in Lixouri Kefalonia, Greece and its utilization in the numerical predictions of its earthquake response. In Proceedings of the 5th International Conference on Computational Methods in Structural Dynamics and Earthquake Engineering (COMPDYN 2015), Athens, Greece, 25–27 May 2015. [Google Scholar] [CrossRef]
- Ubertini, F.; Comanducci, G.; Cavalagli, N. Vibration-based structural health monitoring of a historic bell-tower using output-only measurements and multivariate statistical analysis. Struct. Health Monit. 2016, 15, 438–457. [Google Scholar] [CrossRef]
- Ribilotta, E.; Clementi, F.; Pellegrino, M.; Poiani, M.; Gazzani, V.; Santilli, G.; Lenci, S. Monitoring cultural heritage buildings: The San Ciriaco bell-tower in Ancona. AIP Conf. Proc. 2018, 2040, 090005. [Google Scholar] [CrossRef]
- Baraccani, S.; Azzara, R.M.; Palermo, M.; Gasparini, G.; Trombetti, T. Long-Term Seismometric Monitoring of the Two Towers of Bologna (Italy): Modal Frequencies Identification and Effects Due to Traffic Induced Vibrations. Front. Built Environ. 2020, 6, 85. [Google Scholar] [CrossRef]
- Sánchez-Aparicio, L.J.; Riveiro, B.; González-Aguilera, D.; Ramos, L.F. The combination of geomatic approaches and operational modal analysis to improve calibration of finite element models: A case of study in Saint Torcato Church (Guimarães, Portugal). Constr. Build. Mater. 2014, 70, 118–129. [Google Scholar] [CrossRef]
- Scamardo, M.; Zucca, M.; Crespi, P.; Longarini, N.; Cattaneo, S. Seismic Vulnerability Evaluation of a Historical Masonry Tower: Comparison between Different Approaches. Appl. Sci. 2022, 12, 11254. [Google Scholar] [CrossRef]
- Casarin, F.; Modena, C. Seismic Assessment of Complex Historical Buildings: Application to Reggio Emilia Cathedral, Italy. Int. J. Archit. Herit. 2008, 2, 304–327. [Google Scholar] [CrossRef]
- Spinelli, P.; Salvatori, L.; Lancellotta, R.; Betti, M. Preliminary Assessment Of The Seismic Behaviour Of Giotto’s Bell Tower In Florence. Int. J. Archit. Herit. 2022, 17, 23–45. [Google Scholar] [CrossRef]
- Ramírez, E.; Lourenço, P.B.; D’Amato, M. Seismic Assessment of the Matera Cathedral. In RILEM Bookseries; Springer International Publishing: Berlin/Heidelberg, Germany, 2019; pp. 1346–1354. [Google Scholar] [CrossRef]
- Mendes, P.; Baptista, M.; Agostinho, L.; Lagomarsino, S.; Costav, J. Structural and dynamic analysis of N. Sra. do Carmo church, Lagos Portugal. In Proceedings of the EURODYN 2005, Structural Dynamics, Paris, France, 4–7 September 2005; pp. 311–318. [Google Scholar]
- Baptista, M.; Mendes, P.; Afilhado, A.; Agostinho, L.; Lagomarsino, A.; Victor, L.M. Ambient vibration testing at N. Sra. do Carmo Church, preliminary results. In Proceedings of the 4th International Seminar on Structural analysis of Historical Constructions, Bath, UK, 2–4 July 2004; pp. 483–488. [Google Scholar]
- Ramos, L.F.; Aguilar, R.; Lourenço, P.B.; Moreira, S. Dynamic structural health monitoring of Saint Torcato church. Mech. Syst. Signal Process. 2013, 35, 1–15. [Google Scholar] [CrossRef]
- Aguilar, R.; Noel, M.F.; Ramos, L.F. Integration of reverse engineering and non-linear numerical analysis for the seismic assessment of historical adobe buildings. Autom. Constr. 2019, 98, 1–15. [Google Scholar] [CrossRef]
- Ivancic, S.R.; Briceno, C.; Marques, R.; Aguilar, R.; Perucchio, R.; Vargas, J. Seismic assessment of the St. Peter apostle church of Andahuaylillas in Cusco, Peru. In Proceedings of the SAHC2014–9th International Conference on Structural Analysis of Historical Constructions, Mexico City, Mexico, 14–17 October 2014. [Google Scholar]
- Zonno, G.; Aguilar, R.; Castañeda, B.; Boroschek, R.; Lourenço, P.B. Environmental and Dynamic Remote Monitoring of Historical Adobe Buildings: The Case Study of the Andahuaylillas Church in Cusco, Peru. In RILEM Bookseries; Springer International Publishing: Berlin/Heidelberg, Germany, 2019; pp. 2216–2224. [Google Scholar] [CrossRef]
- Peña, F.; Lourenço, P.B.; Mendes, N. Seismic assessment of the Qutb minar in Delhi, India. In Proceedings of the 14th World Conference on Earthquake Engineering, Beijing, China, 12–17 October 2008; pp. 483–488. [Google Scholar]
- Masciotta, M.G.; Ramos, L.F. Dynamic identification of historic masonry structures. In Long-Term Performance and Durability of Masonry Structures; Elsevier: Amsterdam, The Netherlands, 2019; pp. 241–264. [Google Scholar]
- Pineda, P.; Sáez, A. Assessment of ancient masonry slender towers under seismic loading: Dynamic characterization of the Cuatrovitas tower. In Heritage Masonry; WIT Press: Billerica, MA, USA, 2013; pp. 143–157. [Google Scholar] [CrossRef]
- Buachart, C.; Hansapinyo, C.; Tantisukhuman, N.; Miyamoto, M.; Matsushima, M.; Limkatanyu, S.; Imjai, T.; Zhang, H. Real time vibration measurement and inverse analysis for dynamic properties of an axisymmetric masonry structure. J. Asian Archit. Build. Eng. 2022, 1–10. [Google Scholar] [CrossRef]
- Francisca, S.L. Dynamic Characterisation of the Bell Tower of Sant Cugat Monastery. Master’s Thesis, Universitat Politècnica de Catalunya, Barcelona, Spain, 2020. [Google Scholar]
- Italian Ministry of Infrastructure. Norme Tecniche per le Costruzioni—DM 14 Gennaio 2008; Supplemento Ordinario; Italian Ministry of Infrastructure: Rome, Italy, 2008. [Google Scholar]
- Rodrigues, J.M.V.B.L. Identificação modal estocástica: Métodos de análise e aplicações em estruturas de engenharia civil; FEUP: Porto, Portugal, 2005. [Google Scholar]
- Cantieni, R. Experimental methods used in system identification of civil engineering structures. In Proceedings of the International Operational Modal Analysis Conference (IOMAC), Copenhagen, Denmark, 26–27 April 2005; pp. 249–260. [Google Scholar]
- Masjedian, M.; Keshmiri, M. A review on operational modal analysis researches: Classification of methods and applications. In Proceedings of the 3rd IOMAC, Portonovo, Italy, 4–6 May 2009; pp. 707–718. [Google Scholar]
- Zhang, L.; Wang, T.; Tamura, Y. A frequency–spatial domain decomposition (FSDD) method for operational modal analysis. Mech. Syst. Signal Process. 2010, 24, 1227–1239. [Google Scholar] [CrossRef]
- Brincker, R.; Zhang, L.; Andersen, P. Modal identification from ambient responses using frequency domain decomposition. In Proceedings of the 18th international Modal Analysis Conference (IMAC), San Antonio, TX, USA, 7–10 February 2000; Volume 1, pp. 625–630. [Google Scholar]
- Overchee, P.; Moor, B. Subspace Identification for Linear System; Atlantis Press: Amsterdam, The Netherlands, 1996. [Google Scholar]
- Casolo, S.; Diana, V.; Uva, G. Influence of soil deformability on the seismic response of a masonry tower. Bull. Earthq. Eng. 2017, 15, 1991–2014. [Google Scholar] [CrossRef]
- de Silva, F.; Pitilakis, D.; Ceroni, F.; Sica, S.; Silvestri, F. Experimental and numerical dynamic identification of a historic masonry bell tower accounting for different types of interaction. Soil Dyn. Earthq. Eng. 2018, 109, 235–250. [Google Scholar] [CrossRef]
- Traill-Nash, R.; Collar, A. The effects of shear flexibility and rotatory inertia on the bending vibrations of beams. Q. J. Mech. Appl. Math. 1953, 6, 186–222. [Google Scholar] [CrossRef]
- Nallim, L.G.; Grossi, R.O. A general algorithm for the study of the dynamical behaviour of beams. Appl. Acoust. 1999, 57, 345–356. [Google Scholar] [CrossRef]
- Cowper, G. The shear coefficient in Timoshenko’s beam theory. J. Appl. Mech. 1966, 33, 335–340. [Google Scholar] [CrossRef]
- Meirovitch, L. Analytical Methods in Vibrations; McMillan: New York, NY, USA, 1967. [Google Scholar]
- Mazanoglu, K. Natural frequency analyses of segmented Timoshenko–Euler beams using the Rayleigh–Ritz method. J. Vib. Control. 2017, 23, 2135–2154. [Google Scholar] [CrossRef]
- Combescure, A.; Hoffmann, A.; Pasquet, P. The CASTEM finite element system. In Finite Element Systems: A Handbook; Springer: Berlin/Heidelberg, Germany, 1982; pp. 115–125. [Google Scholar]
- Gatelli, D.; Kucherenko, S.; Ratto, M.; Tarantola, S. Calculating first-order sensitivity measures: A benchmark of some recent methodologies. Reliab. Eng. Syst. Saf. 2009, 94, 1212–1219. [Google Scholar] [CrossRef]
- Saltelli, A.; Tarantola, S.; Chan, K.S. A quantitative model-independent method for global sensitivity analysis of model output. Technometrics 1999, 41, 39–56. [Google Scholar] [CrossRef]
- Cukier, R.; Fortuin, C.; Shuler, K.E.; Petschek, A.; Schaibly, J.H. Study of the sensitivity of coupled reaction systems to uncertainties in rate coefficients. I Theory. J. Chem. Phys. 1973, 59, 3873–3878. [Google Scholar] [CrossRef]
- Tarantola, S.; Gatelli, D.; Mara, T.A. Random balance designs for the estimation of first order global sensitivity indices. Reliab. Eng. Syst. Saf. 2006, 91, 717–727. [Google Scholar] [CrossRef]
- Mara, T.A. Extension of the RBD-FAST method to the computation of global sensitivity indices. Reliab. Eng. Syst. Saf. 2009, 94, 1274–1281. [Google Scholar] [CrossRef]
- Jacques, J. Contributions to Sensitivity Analysis and Generalized Discriminant Analysis; Technical Report; University Joseph Fourier: Grenoble, France, 2005. [Google Scholar]
- Helton, J.C.; Johnson, J.D.; Sallaberry, C.J.; Storlie, C.B. Survey of sampling-based methods for uncertainty and sensitivity analysis. Reliab. Eng. Syst. Saf. 2006, 91, 1175–1209. [Google Scholar] [CrossRef]
- Xu, C.; Gertner, G.Z. Uncertainty and sensitivity analysis for models with correlated parameters. Reliab. Eng. Syst. Saf. 2008, 93, 1563–1573. [Google Scholar] [CrossRef]
- Fernandes, V.A.; Lopez-Caballero, F.; d’Aguiar, S.C. Probabilistic analysis of numerical simulated railway track global stiffness. Comput. Geotech. 2014, 55, 267–276. [Google Scholar] [CrossRef]
- Gaspar, A.; Lopez-Caballero, F.; Modaressi-Farahmand-Razavi, A.; Gomes-Correia, A. Methodology for a probabilistic analysis of an RCC gravity dam construction. Modelling of temperature, hydration degree and ageing degree fields. Eng. Struct. 2014, 65, 99–110. [Google Scholar] [CrossRef]
- Sobol, I.M. Global sensitivity indices for nonlinear mathematical models and their Monte Carlo estimates. Math. Comput. Simul. 2001, 55, 271–280. [Google Scholar] [CrossRef]
- Herman, J.; Usher, W. SALib: An open-source Python library for sensitivity analysis. J. Open Source Softw. 2017, 2, 97. [Google Scholar] [CrossRef]






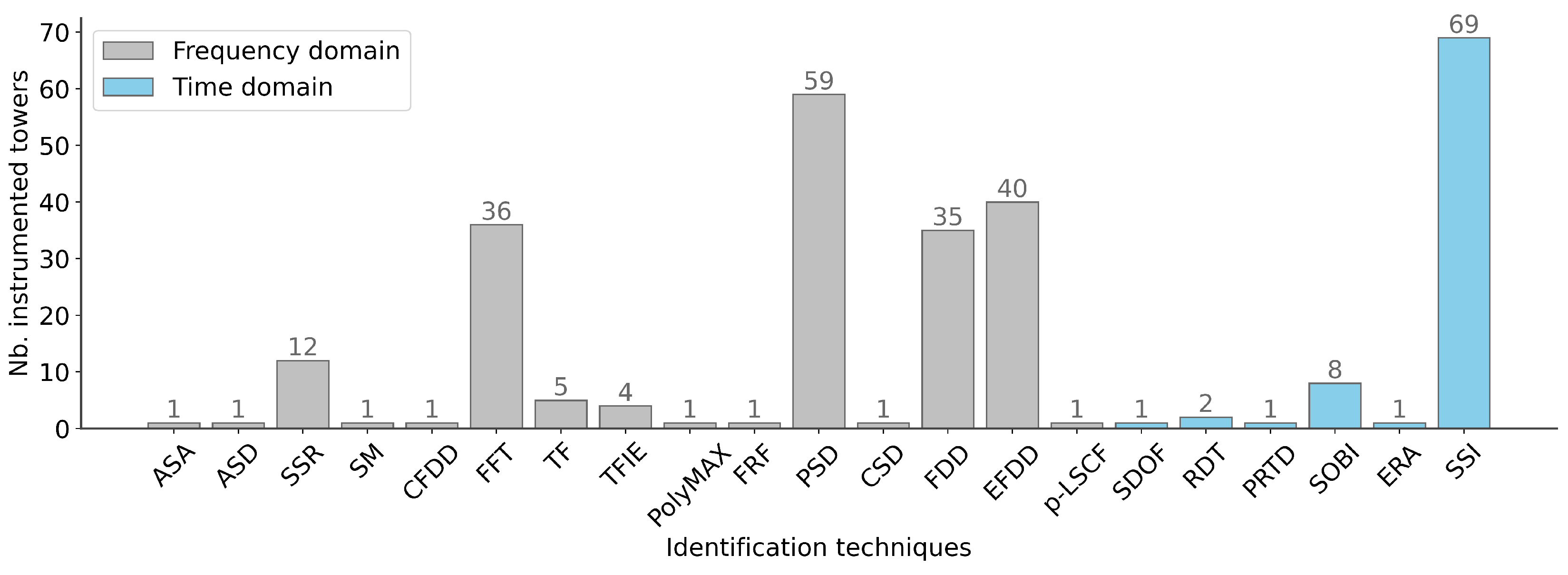



| Glossary | |
|---|---|
| TFIE | Time Frequency Instantaneous Estimators |
| PRTD | Polyreference time domain |
| DSPI | Direct system parameter identification |
| SSI | Stochastic Subspace Identification |
| CC-SSI | Crystal Clear Stochastic Subspace Identification method |
| SSI-COV-PC | Principal Component Covariance-Driven Stochastic Subspace Identification |
| SSI-DATA | Data-Driven Stochastic Subspace Identification |
| SSI-DATA-UPC | Unweighted Principal Component Stochastic Subspace Identification |
| SSI-DATA-CVA | Canonical Variate Analysis |
| ASA | Acceleration Spectral Amplitudes |
| ASD | Auto-Spectrum Displacement |
| ERA | Eigensystem realization algorithm |
| SM | stretching method |
| TF | Transfer function |
| SOBI | Second Order Blind Identification |
| PSD | Power Spectral Density |
| SSR | Standard Spectral Ratio |
| SDOF | Single Degree of Freedom technique |
| p-LSCF | Poly-reference Least Squares Complex Frequency-domain |
| Nomenclature | ||
|---|---|---|
| Symbol | Unit | Description |
| Geometric parameters | ||
| Set of global geometric parameters | ||
| H | [m] | Height of the tower |
| [m] | Width, lowest size of the tower’s section | |
| [m] | Height of interaction between the tower and any adjacent structures | |
| system | ||
| [Hz] | Eigenfrequency | |
| Dimensionless parameters | ||
| [-] | Slenderness | |
| [-] | Interaction factor | |
| Regression coefficients | ||
| [-] | Regression coefficient related to the height | |
| , , , , | [-] | Regression coefficient related to the section geometry |
| [-] | Regression coefficient related to lateral interaction | |
| Id. Model | Ref. | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| 1 | [10] | 20 | −3/4 | 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | [11] | 1/0.0187 | −1 | 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | [12] | 1/0.01137 | −1.138 | 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | [1] | 1/0.0151 | −1.08 | 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | [13] | 28.35 | −0.83 | 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | [13] | 135.343 | −1.32 | 0 | 0 | 0 | 0 | 0 | 0 |
| Empirical model 1 | 22.55 | 2.818 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 2 | [1] | 3.58 | 0 | 0 | 0.57 | 0 | 0 | 0 | 0 |
| Empirical model 2 | 7.608 | 0 | 0 | 0.817 | 0 | 0 | 0 | 0 | |
| 3 | [13] | 208.54 | −1.18 | 0 | 0.55 | 0 | 0 | 0 | 0 |
| Empirical model 3 | 17.113 | −0.369 | 0.538 | 0 | 0 | 0 | 0 | 0 | |
| 4 | [14] | 1/0.06 | −0.5 | 2 | 0.5 | 0.5 | 0 | 0 | 0 |
| 4 | [1] | 1/0.03 | −0.83 | 1 | 0.17 | 0.5 | 0 | 0 | 0 |
| Empirical model 4 | 7.361 | −0.46 | −0.03 | 0 | 3053.821 | 0 | 0 | 0 | |
| 5 | [15] | 1/0.0117 | 0 | −9.632 | 3 | −1 | 94.786 | 144.461 | 0 |
| Empirical model 5 | 0.1 | 0 | −26.78 | 188.47 | 29.40 | −34.47 | 13.93 | 0 | |
| 6 | [13] | 12.96 | −0.686 | 0 | 0 | 0 | 0 | 0 | −0.686 |
| Empirical model 6 | 23.322 | −0.695 | 0 | 0 | 0 | 0 | 0 | −0.028 | |
| 7 | [13] | 14.61 | −0.811 | 0 | −0.254 | 0 | 0 | 0 | −0.341 |
| Empirical model 7 | 17.619 | −0.365 | 0.616 | 0 | 0 | 0 | 0 | −0.171 | |
| Nomenclature | ||
|---|---|---|
| Symbol | Unit | Description |
| Geometrical and material parameters | ||
| Set of global geometric parameters | ||
| H | [m] | Height of the tower |
| [m] | Width, lowest size of the tower’s section | |
| [m] | Height of interaction between the tower and any adjacent structures | |
| [Hz] | Fundamental frequency | |
| [m] | Length, largest size of the tower’s section | |
| [m] | Wall thickness | |
| [m] | Altitude of the bell system | |
| S | [m2] | Surface area |
| , | [m4] | Second moment of area |
| r | [m] | Radius of inertia |
| E | [MPa] | Young modulus |
| [kg·m−3] | Volumetric mass density | |
| Dimensionless parameters | ||
| [-] | Slenderness | |
| [-] | Interaction factor | |
| [-] | Thickness factor | |
| [-] | Length factor | |
| , , , | [-] | Section factor |
| [rad.] | Angle of bending direction with respect to x axis | |
| Regression coefficients | ||
| , , | [-] | Regression coefficient |
| Parameters | SQ | REC | CIR |
|---|---|---|---|
| 1 | 1 | /4 | |
| 1/12 | 1/12 | /64 | |
| 1 | >1 | 1 |
| Id Model | Ref. | ||||
|---|---|---|---|---|---|
| 1 | [1] | 0 | 1 | ||
| 2 | [8] (Equation (22)) | 0.8 | 1 | 1 | |
| 3 | [8] (Equation (23)) | 0.8 | 0 | 1 | |
| 4 | [8] (Equation (24)) | 800 | 0 | 0 |
| Nomenclature | ||
|---|---|---|
| Symbol | Unit | Description |
| Set of global geometric parameters | ||
| H | [m] | Height of the tower |
| [m] | Width, lowest size of the tower’s section | |
| [m] | Height of interaction between the tower and any adjacent structures | |
| [Hz] | Eigenfrequency | |
| [m] | Length, largest size of the tower’s section | |
| [m] | Wall thickness | |
| [m] | Altitude of the bell system | |
| S | Surface area | |
| E | [MPa] | Young modulus |
| Volumetric mass density | ||
| [-] | Poisson ratio | |
| Second moment of inertia | ||
| Soil/structure translational stiffness | ||
| [N] | Soil/structure rotational stiffness | |
| Nave/structure translational stiffness | ||
| [kg] | Mass of the bell system | |
| G | [Pa] | Shear modulus |
| k | [-] | Shear coefficient |
| [m] | Transversal deflection | |
| [rad.] | Normal rotation | |
| [J] | Potential energy | |
| [J] | Kinetic energy | |
| [J] | Potential energy associated with the beam system | |
| [J] | Potential energy associated with the soil–structure interaction | |
| [J] | Potential energy associated with the tower-nave interaction system | |
| [J] | Kinetic energy associated with the beam system | |
| , | [-] | Polynomial functions to approximate the displacement and rotation field |
| [-] | Generalized coordinates system | |
| , | Mass and Stiffness matrix | |
| Case 1 | Case 2 | Case 3 | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Mode | |||||||||
| [Hz] | [Hz] | [%] | [Hz] | [Hz] | [%] | [Hz] | [Hz] | [%] | |
| 1 | 2.40 | 2.39 | 0.42 | 4.88 | 4.87 | 0.2 | 4.49 | 4.40 | 2.00 |
| 2 | 12.26 | 12.27 | 0.08 | 15.83 | 16.00 | 1.07 | 11.77 | 11.74 | 0.25 |
| 3 | 28.27 | 28.34 | 0.02 | 29.76 | 29.92 | 0.54 | 16.80 | 16.31 | 2.92 |
| Input Factors | ||
|---|---|---|
| 5 | 626 | |
| 6 | 786 | |
| 7 | 1394 | |
| Parameters [Unit] | Range |
|---|---|
| H [m] | 13.1–56.8 |
| [m] | 3.2–10.2 |
| [%] | 100–130 |
| [%] | 25–36 |
| E [GPa] | 0.2–5.3 |
| [-] | 0.13–0.27 |
| [kg·m−3] | 1500–2100 |
| [%] | 25–59 |
| [N·m2] | 10–10 |
| [kg] | 0–6500 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Montabert, A.; Giry, C.; Limoge Schraen, C.; Lépine, J.; Choueiri, C.; Mercerat, E.D.; Guéguen, P. An Open Database to Evaluate the Fundamental Frequency of Historical Masonry Towers through Empirical and Physics-Based Formulations. Buildings 2023, 13, 2168. https://doi.org/10.3390/buildings13092168
Montabert A, Giry C, Limoge Schraen C, Lépine J, Choueiri C, Mercerat ED, Guéguen P. An Open Database to Evaluate the Fundamental Frequency of Historical Masonry Towers through Empirical and Physics-Based Formulations. Buildings. 2023; 13(9):2168. https://doi.org/10.3390/buildings13092168
Chicago/Turabian StyleMontabert, Arnaud, Cédric Giry, Claire Limoge Schraen, Jade Lépine, Clarisse Choueiri, E. Diego Mercerat, and Philippe Guéguen. 2023. "An Open Database to Evaluate the Fundamental Frequency of Historical Masonry Towers through Empirical and Physics-Based Formulations" Buildings 13, no. 9: 2168. https://doi.org/10.3390/buildings13092168
APA StyleMontabert, A., Giry, C., Limoge Schraen, C., Lépine, J., Choueiri, C., Mercerat, E. D., & Guéguen, P. (2023). An Open Database to Evaluate the Fundamental Frequency of Historical Masonry Towers through Empirical and Physics-Based Formulations. Buildings, 13(9), 2168. https://doi.org/10.3390/buildings13092168







