Crack Resistance of Pre-Stressed Steel-Reinforced Concrete Composite Simple Supported Beams
Abstract
1. Introduction
2. Materials and Methods
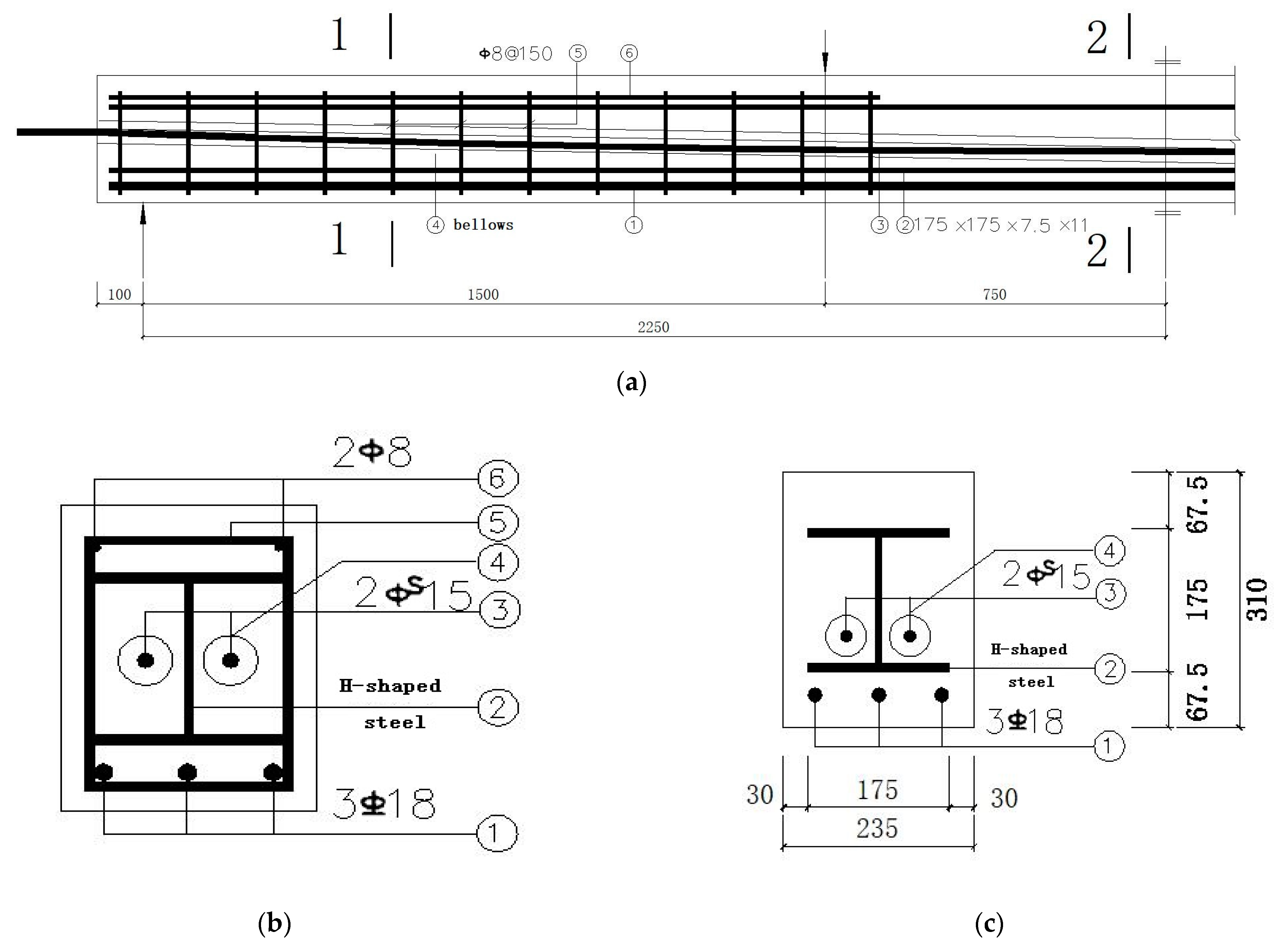
2.1. Design and Preparation of PSRC Beam Specimen
2.2. Experimental Loading
2.3. Experimental Process
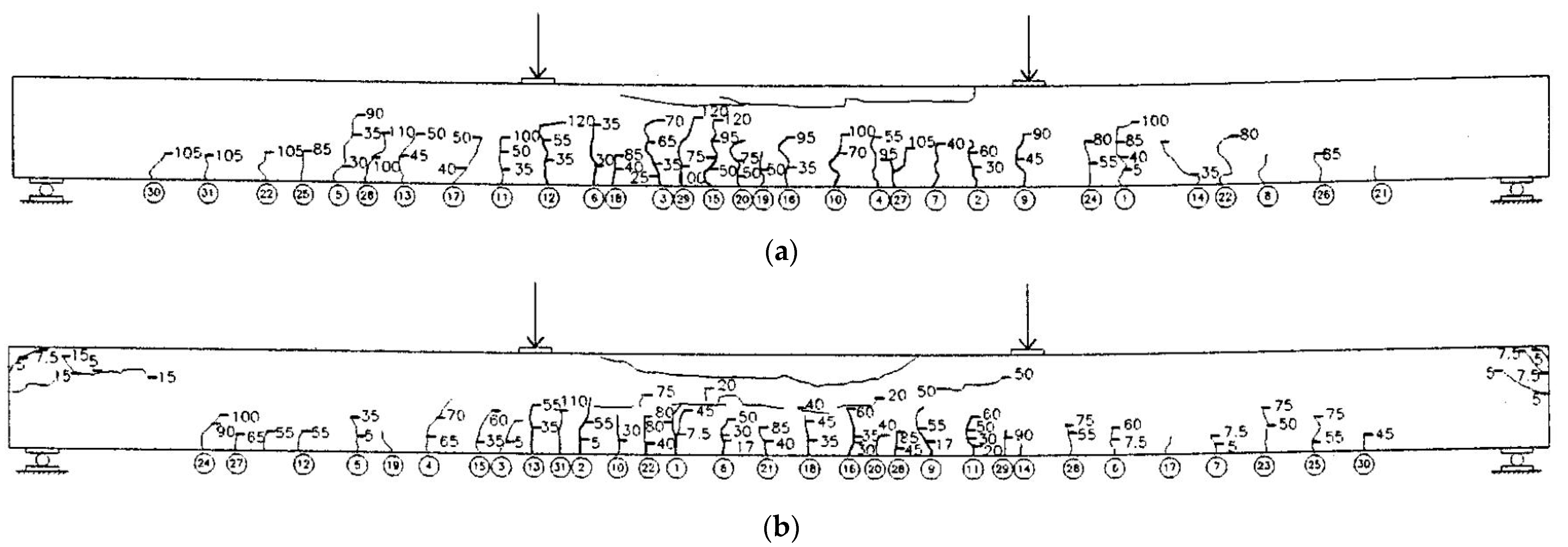
2.3.1. Test Beam Cracks
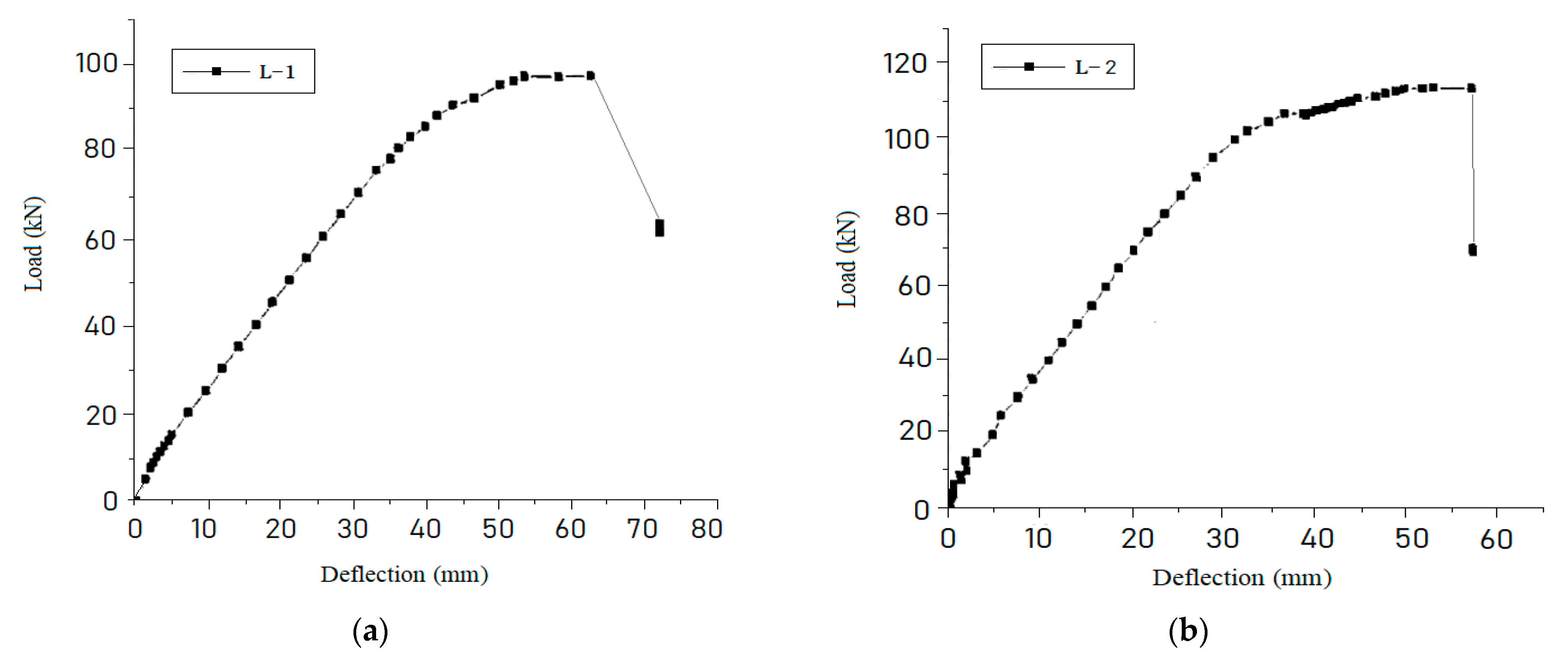
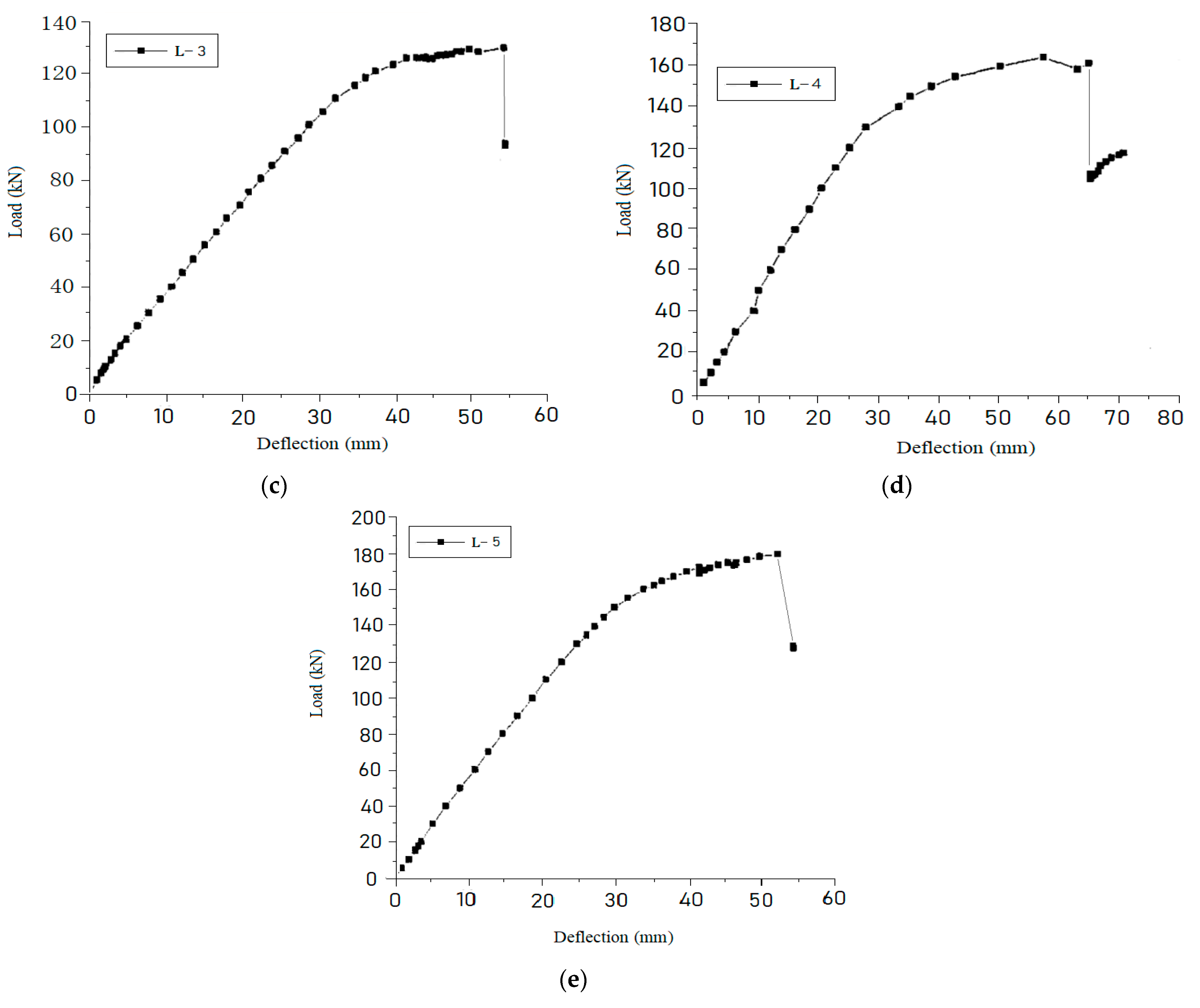
2.3.2. Beam Mid-Span Deflection Curves
3. Calculation of Crack Width of PSRC Simply Supported Composite Beams
3.1. Calculation of Average Crack Spacing
3.2. Calculation of Average Crack Width
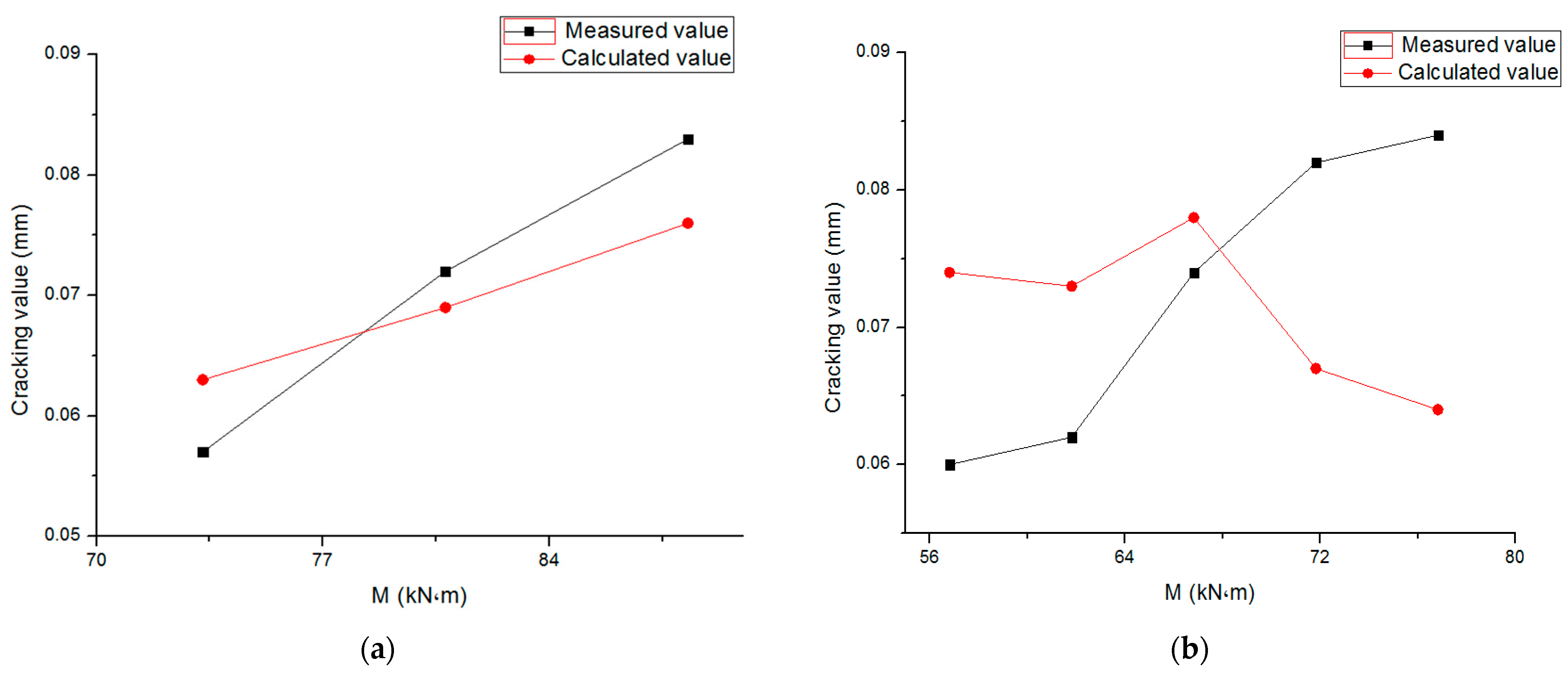
3.3. Calculation of Maximum Crack Width
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xue, J.; Briseghella, B.; Huang, F.; Nuti, C.; Tabatabai, H.; Chen, B. Review of ultra-high performance concrete and its application in bridge engineering. Constr. Build. Mater. 2020, 260, 119844. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, Y.; Hussein, H.H.; Chen, G. Flexural strengthening of reinforced concrete beams or slabs using ultra-high performance concrete (UHPC): A state of the art review. Eng. Struct. 2020, 205, 110035. [Google Scholar] [CrossRef]
- Bae, B.; Choi, H.; Choi, C. Flexural Strength Evaluation of Reinforced Concrete Members with Ultra High Performance Concrete. Adv. Mater. Sci. Eng. 2016, 2016, 2815247. [Google Scholar] [CrossRef]
- Singh, M.; Sheikh, A.; Ali, M.M.; Visintin, P.; Griffith, M. Experimental and numerical study of the flexural behaviour of ultra-high performance fibre reinforced concrete beams. Constr. Build. Mater. 2017, 138, 12–25. [Google Scholar] [CrossRef]
- Chen, S.; Zhang, R.; Jia, L.-J.; Wang, J.-Y.; Hu, A.; Liang, X.; Yu, J.; Shi, Q.; Yang, I.-H.; Joh, C.; et al. Flexural behaviour of rebar-reinforced ultra-high-performance concrete beams. Mag. Concr. Res. 2018, 70, 997–1015. [Google Scholar] [CrossRef]
- Liu, C.; Zhang, Y.; Yao, Y.; Huang, Y. Calculation Method for Flexural Capacity of High Strain-hardening Ultra-high Performance Concrete T-beams. Struct. Concr. 2019, 20, 405–419. [Google Scholar] [CrossRef]
- Qiu, M.; Shao, X.; Wille, K.; Yan, B.; Wu, J. Experimental Investigation on Flexural Behavior of Reinforced Ultra High Performance Concrete Low-Profile T-Beams. Int. J. Concr. Struct. Mater. 2020, 14, 5. [Google Scholar] [CrossRef]
- Deng, N.; Deng, Y.; Duan, J.; Xue, W. Experimental and Numerical Study on the Flexural Behaviors of Unbonded pre-stressed I-Shaped Steel Encased in Ultra-High-Performance Concrete Beams. Buildings 2023, 13, 2901. [Google Scholar] [CrossRef]
- Rossi, A.; de Carvalho, A.S.; de Oliveira, V.M.; de Souza, A.S.C.; Martins, C.H. A Parametric Study on the LDB Strength of Steel-Concrete Composite Beams. Eng 2023, 4, 2226–2253. [Google Scholar] [CrossRef]
- Nie, J.; Tao, M.; Cai, C.; Li, S. Analytical and numerical modeling of pre-stressed continuous steel-concrete composite beams. J. Struct. Eng. 2011, 137, 1405–1418. [Google Scholar] [CrossRef]
- Jia, J.; Meng, G.; Zhu, W. Experimental study on flexural performance and load-carrying capacity analysis of pre-stressed steel ultra-high-strength concrete composite beams. J. Build. Struct. 2014, 35, 1–10. [Google Scholar]
- T/CECS 203-2021; Technical Specification for Application of Self-Compacting Concrete. Architecture and Building Press: Beijing, China, 2021.
- JGJ 138-2001; Technical Specification of Steel Concrete Composite Structure. Architecture and Building Press: Beijing, China, 2001.
- YB9082-2006; Technical Specification of Steel-Reinforced Concrete Structures. Architecture and Building Press: Beijing, China, 2006.
- Wu, D. Experimental Study on Normal Section Mechanical Performance of Pre-Stressed Built-in H-Beam with Simple Support of Concrete. Master’s Thesis, Northeast Forestry University, Harbin, China, 2009. [Google Scholar]
- GB 50010-2010; Chinese Standard for Design of Concrete Structures. Architecture and Building Press: Beijing, China, 2010.
- Johnson, R. Composite Structures of Steel and Concrete: Beams, Slabs, Columns, and Frames for Buildings, 3rd ed.; Blackwell Publishing Inc.: Oxford, UK, 2004. [Google Scholar]
- Wang, L.; Cheng, D.; Qu, E.; Zhang, D.; Lv, C. Calculation of Additional Internal Forces in Post-Tensioned pre-stressed Concrete Frame Columns Based on Equivalent Lateral Stiffness. Buildings 2022, 12, 644. [Google Scholar] [CrossRef]


| Volume /m3 | Material Composition/kg | |||||
|---|---|---|---|---|---|---|
| Cement | Fly Ash | Sand | Stone | Water-Cement Ratio | Water Reducing Agent | |
| 1 | 400 | 100 | 798 | 848.6 | 0.42 | 3.2 |
| 0.6 1 | 24 | 6 | 47.88 | 50.9 | 0.42 | 0.192 |
| Index | Φ8 | ϕ18 | H-Shaped Steel | ||
|---|---|---|---|---|---|
| 150 | 175 | 200 | |||
| Yield strength | 295 | 357.5 | 275 | 250 | 290 |
| Ultimate strength | 395 | 587.5 | 440 | 415 | 425 |
| Yield strain (10−6) | 1405 | 1788 | 1335 | 1213 | 1408 |
| Beam Number | Section Size | H-Shaped Steel | Tension Reinforcement | Pre-Stressed Reinforcement |
|---|---|---|---|---|
| L-1 | 210 × 280 | 150 × 150 × 7 × 10 | 2 Φ 18 | 2 ϕs 5 |
| L-2 | 235 × 310 | 175 × 175 × 7.5 × 11 | 2 Φ 18 | 2 ϕs 15 |
| L-3 | 235 × 310 | 175 × 175 × 7.5 × 11 | 3 Φ 18 | 2 ϕs 15 |
| L-4 | 260 × 330 | 200 × 200 × 8 × 12 | 2 Φ 18 | 2 ϕs 15 |
| L-5 | 260 × 330 | 200 × 200 × 8 × 12 | 4 Φ 18 | 2 ϕs 15 |
| Beam Number | L-1 | L-2 | L-3 | L-4 | L-5 |
|---|---|---|---|---|---|
| Crack spacing | 111.78 | 106.20 | 101.45 | 103.15 | 108.26 |
| Beam Number | M (kN·m) | M/Mu | Mean Crack Width (mm) |
|---|---|---|---|
| L-1 | 43.287 | 0.285 | 0.020 |
| 50.787 | 0.334 | 0.020 | |
| 73.287 | 0.482 | 0.057 | |
| 80.787 | 0.531 | 0.072 | |
| 88.287 | 0.581 | 0.083 | |
| L-2 | 56.819 | 0.320 | 0.060 |
| 61.819 | 0.348 | 0.062 | |
| 66.819 | 0.376 | 0.074 | |
| 71.819 | 0.405 | 0.082 | |
| 76.819 | 0.433 | 0.084 | |
| L-3 | 74.319 | 0.371 | 0.043 |
| 89.319 | 0.446 | 0.059 | |
| 104.319 | 0.521 | 0.077 | |
| 119.319 | 0.596 | 0.096 | |
| L-4 | 97.814 | 0.383 | 0.107 |
| 107.814 | 0.442 | 0.105 | |
| 117.814 | 0.461 | 0.114 | |
| 137.817 | 0.540 | 0.141 | |
| L-5 | 97.814 | 0.352 | 0.020 |
| 157.814 | 0.567 | 0.068 | |
| 187.814 | 0.675 | 0.074 | |
| 217.814 | 0.783 | 0.115 |
| Load | Beam Number | ||||
|---|---|---|---|---|---|
| L-1 | L-2 | L-3 | L-4 | L-5 | |
| Cracking load | 13.75 | 5 | 7.5 | 10 | 15 |
| Yield load | 65 | 75 | 85 | 140 | 150 |
| Ultimate load | 97.5 | 113.8 | 129 | 165 | 180.2 |
| Crack | Beam Number | ||||
|---|---|---|---|---|---|
| L-1 | L-2 | L-3 | L-4 | L-5 | |
| Measured value | 111.78 | 106.2 | 101.45 | 103.15 | 108.26 |
| Calculated value | 93.472 | 98.886 | 93.482 | 103.26 | 93.099 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, D.; Wang, H.; Wang, R.; Lv, C. Crack Resistance of Pre-Stressed Steel-Reinforced Concrete Composite Simple Supported Beams. Buildings 2024, 14, 201. https://doi.org/10.3390/buildings14010201
Wu D, Wang H, Wang R, Lv C. Crack Resistance of Pre-Stressed Steel-Reinforced Concrete Composite Simple Supported Beams. Buildings. 2024; 14(1):201. https://doi.org/10.3390/buildings14010201
Chicago/Turabian StyleWu, Dan, Hongzhi Wang, Rui Wang, and Chun Lv. 2024. "Crack Resistance of Pre-Stressed Steel-Reinforced Concrete Composite Simple Supported Beams" Buildings 14, no. 1: 201. https://doi.org/10.3390/buildings14010201
APA StyleWu, D., Wang, H., Wang, R., & Lv, C. (2024). Crack Resistance of Pre-Stressed Steel-Reinforced Concrete Composite Simple Supported Beams. Buildings, 14(1), 201. https://doi.org/10.3390/buildings14010201





