A Study on the Influence of Hydraulic Compactor Reinforcement on the Force Law of an Independent Foundation Under a Column and Its Safety Standard
Abstract
:1. Introduction
2. Numerical Calculation
2.1. Numerical Models and Simulation Schemes
2.2. Calculation Results and Analysis
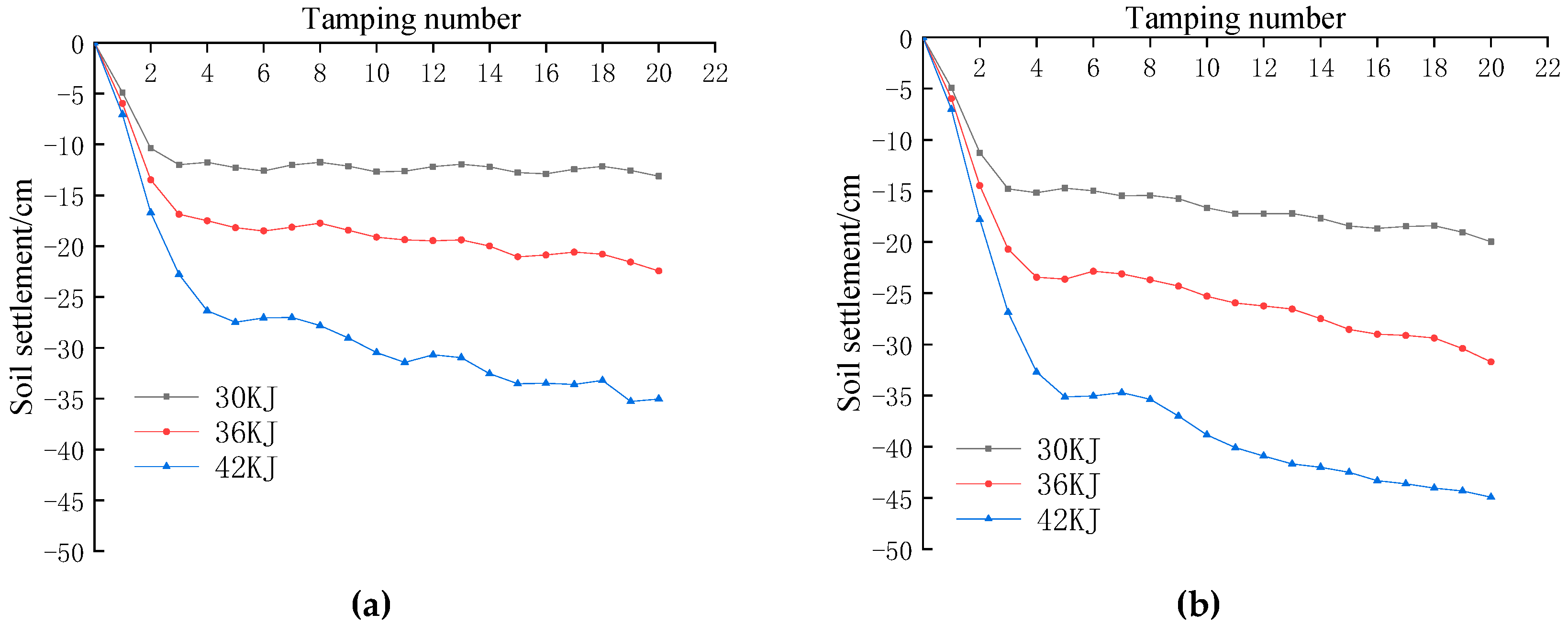
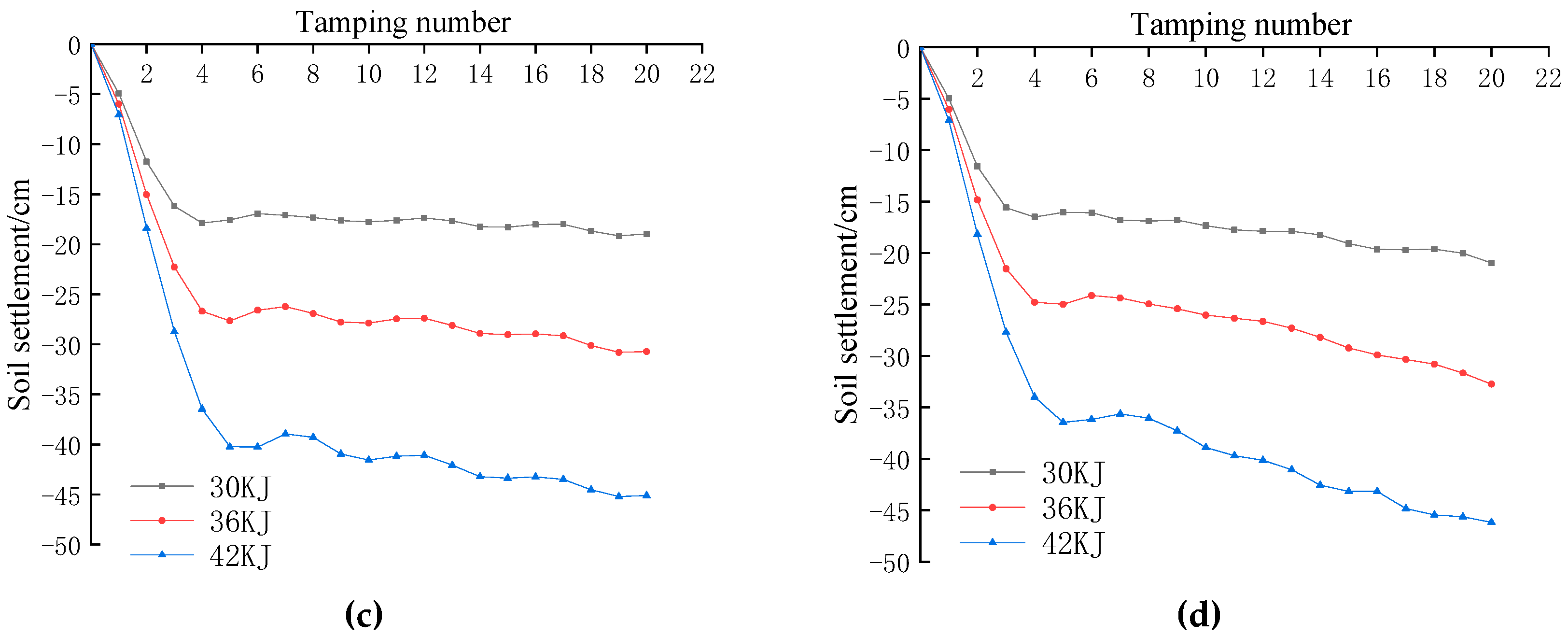
2.2.1. Analysis of the Optimal Number of Ramming Strokes
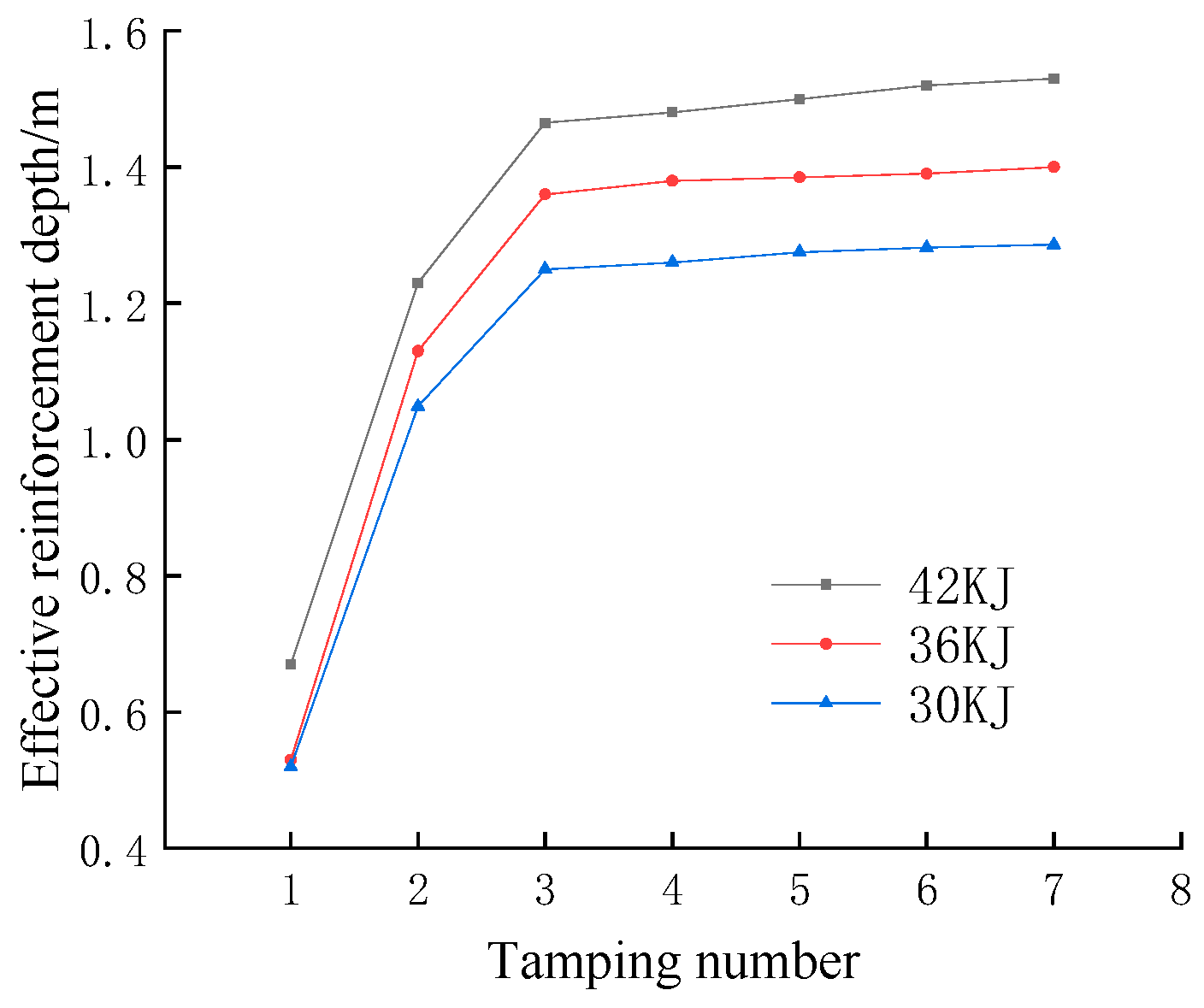
2.2.2. Analysis of Effective Reinforcement Depth
2.2.3. Structural Stress Analysis
3. Project Cases
3.1. Project Overview
3.2. On-Site Monitoring
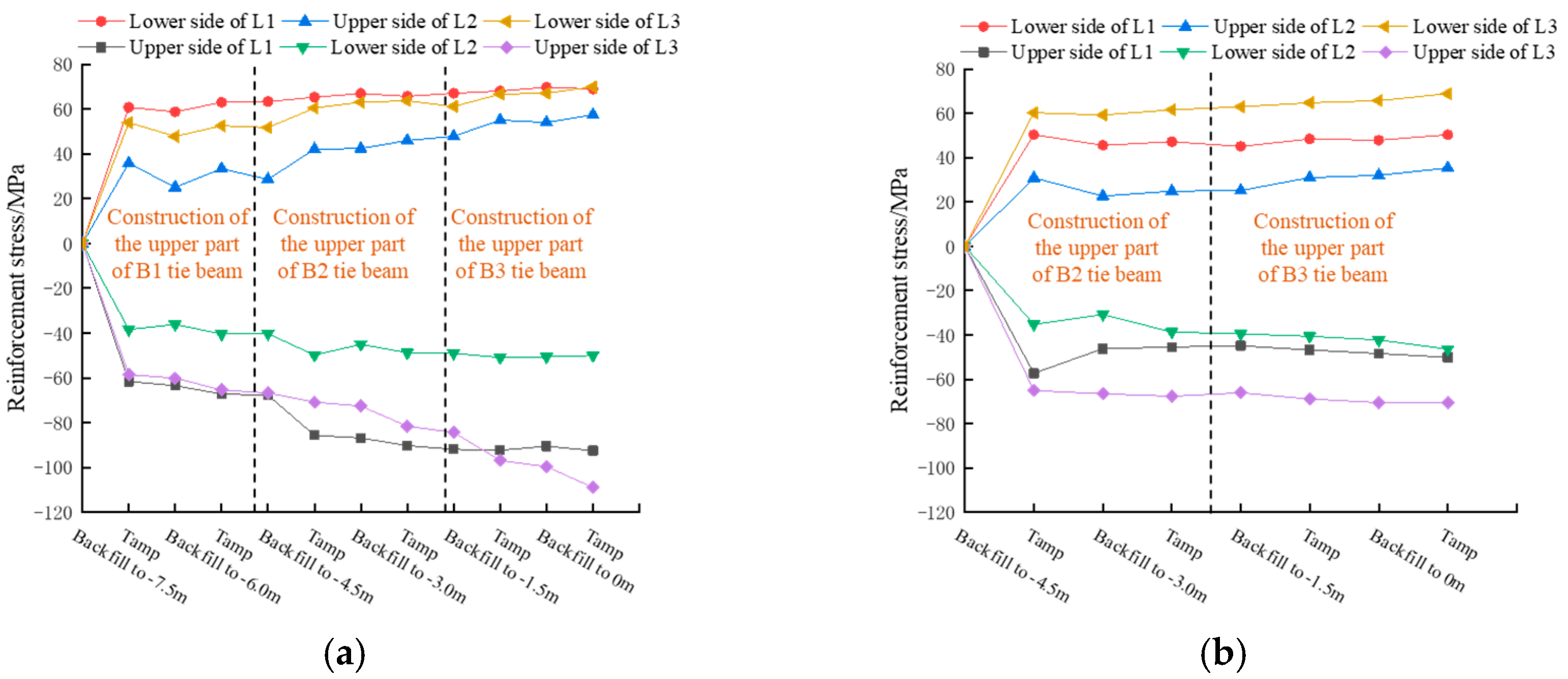
3.3. Analysis of Monitoring Data
4. Conclusions
- Hydraulic tamping has an effect on tie beams when used to tamp the upper backfills of independent foundations under columns. By properly controlling the tamping energy, the number of tamps and the thickness of the fill, it is possible to ensure that the structure is in a safe condition. Similarly, the hydraulic compactor is also applicable to the treatment of independent foundations under columns.
- In the case of tamping energy of 30 KJ, 36 KJ and 42 KJ, the recommended thickness of a single backfill is the effective reinforcement depth of tamping (1.26 m, 1.38 m and 1.48 m); for hydraulic tamping of 30 KJ and 36 KJ, the optimum number of tamping is three times, while for hydraulic tamping of 42 KJ, the optimum number of tamping is four times. In addition, the depth of reinforcement calculated according to the modified formula is in general agreement with the simulation results, providing a supplement to the relevant safety standards.
- The tamping action of the compactor has a significant effect on the structural force within the effective reinforcement area, and the structural force is negatively correlated with the backfill thickness. At the same time, the engineering numerical simulation and on-site test results meet its safety standards; at 42 KJ compactor energy and 1.5 m single filling thickness, the tie beam reinforcement stress reaches the specification of the warning value of 18.5~55.5%.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, W.X.; Liu, R.; Xie, H.T.; Yi, W.J. Experimental research on seismic behavior of reinforced concrete frame substructure considering soil-structure interaction. J. Build. Struct. 2021, 42, 72–81. [Google Scholar]
- Zhang, M.; Zhang, S.H.; Zhong, Z.L.; Hou, B.W.; Du, X.L. Robust geotechnical design of spread foundations. Rock Soil Mech. 2019, 40, 4506–4514. [Google Scholar]
- Zhang, J. Study on design method of single-footing with water-proof slab under column. Build. Struct. 2018, 48, 775–778. [Google Scholar]
- Haido, J.H. Prediction of the shear strength of RC beam-column joints using new ANN formulations. Structures 2022, 38, 1191–1209. [Google Scholar] [CrossRef]
- Xiong, W.; Qi, C.J.; Wu, X.L.; Li, H.T. Case study on treatment of large thickness miscellaneous fill soil foundation. J. Ground Improv. 2021, 43, 165–169. [Google Scholar]
- Zhu, J.J.; Zhang, H.M. Foundation treatment and pile foundation design for large-area filling. Build. Struct. 2017, 47, 454–457. [Google Scholar]
- Wang, J.; Zhang, Y.M.; Wang, K.; Li, L.P.; Cheng, S.; Sun, S.Q. Development of similar materials with different tension-compression ratios and evaluation of TBM excavation. Bull. Eng. Geol. Environ. 2024, 83, 190. [Google Scholar] [CrossRef]
- Gu, Q.; Lee, F.H. Ground response to dynamic compaction of dry sand. Geotechnique 2002, 52, 481–493. [Google Scholar] [CrossRef]
- Ji, M.; Zhang, Z.P.; Chen, C.C.; Zhou, Z.J. Research on Model Test of Hydraulic Compaction Method for Strengthening Subgrade on Abutment Subgrade. Highway 2020, 65, 1–9. [Google Scholar]
- Miao, X.Y.; Liu, H.Y.; Qu, Y.H.; Yang, H.; Li, K.C. Research on Application of Hydraulic Compaction Process on the Backfill of the Culvert. Highway 2017, 62, 30–35. [Google Scholar]
- Liu, J.Q.; Si, G.M.; Zhang, M.Q.; Shao, X.T.; Zhang, Q.L. Safety Evaluation of Culvert Structure in Construction Process of Lightweight Tamper. Road Mach. Constr. Mech. 2017, 34, 118–121. [Google Scholar]
- Hu, J.Y.; Xu, T.Y.; Yan, R.P.; Han, D.D.; Zhou, Z.J. Quality control method of hydraulic dynamic compaction reinforcing loess subgrade. J. Chang’an Univ. S.-Cent. 2020, 40, 15–26. [Google Scholar]
- Han, D.D.; Zhou, Z.J.; Lei, J.T.; Lin, M.G.; Zhan, H.C. Field experimental study for layered compactness of subgrade based on dimensional analysis. Geomech. Eng. 2022, 29, 583–598. [Google Scholar]
- Feng, X.H.; Wan, Z. Field test and numerical simulation study on treatment of expressway retailing backwall by hydraulic compaction. J. Railw. Sci. Eng. 2013, 10, 49–54. [Google Scholar]
- Zhang, Y.Z.; Yin, K.; Huang, J.H.; Liu, Y.S.; Li, C.C. Study on Shearing Resistance Design of Single Column Spread Footing on Rock Foundation. Chin. J. Undergr. Space Eng. 2019, 15, 1511–1518+1540. [Google Scholar]
- Li, X.C.; Yin, K.; Huang, J.H. Bending-expansion tensile failure of single-column footing on rock foundation. China Sci. Pap. 2017, 12, 1520–1525+1542. [Google Scholar]
- Zhou, S.; Chen, L.S.; Zhang, H.L.; Wang, J.; Zhang, P.Y.; Wu, G.; Zhang, Z.W.; Zhang, F. Study on the influence of hydraulic compaction of backfill on the stress of independent foundation structure under column. Build. Struct. 2023, 53, 2539–2544. [Google Scholar]
- Zhang, Z.K.; Chen, C.X.; Hou, Z.C.; Liang, Q.K.; Ou, Y.S. Rapid hydraulic compaction technology and determination method of its construction parameters. J. Ground. Improv. 2023, 5, 48–53. [Google Scholar]
- Li, B.P. Numerical analysis on pore pressure of clay under shock load. Combust. Explos. Shock. Waves 2005, 3, 281–284. [Google Scholar]
- Mohammad, M.N.; Elif, A.; Zeynep, Y. Experimental and numerical analysis of impactor geometric shape effects on steel beams under impact loading. Structures 2020, 27, 1118–1138. [Google Scholar]
- JGJ 79-2012; Technical Code for Ground Treatment of Buildings. The Standardization Administration of the People’s Republic of China: Beijing, China, 2012.
- Zhao, Y.L.; Gong, Y.L.; Zhang, Z.Y. Influencing factors of effective reinforcement depth by dynamic compaction on deep replacement of sand soil foundation. J. Heilongjiang Univ. Sci. Technol. 2023, 33, 710–717. [Google Scholar]
- Xie, W.D.; Chen, J.Y.; He, H.Y. Effect of dynamic compaction on hydraulic filled silty to fine sand with high fines content. Port Waters Eng. 2023, 9, 223–228. [Google Scholar]
- TB10092-2017; Code for Design of Concrete Structures of Railway Bridges and Culverts. National Railway Administration: Beijing, China, 2017.
- GB 55003-2021; General Code for Foundation Engineering of Building and Municipal Projects. Ministry of Housing and Urban-Rural Development: Beijing, China, 2021.
- GB 50497-2019; Technical Standard for Monitoring of Building Excavation Engineering. Ministry of Housing and Urban-Rural Development: Beijing, China, 2019.
- GB/T 1499.2-2018; Steel for the Reinforcement of Concrete—Part 2: Hot Rolled Ribbed Bars. National Standards of the People’s Republic of China: Beijing, China, 2018.
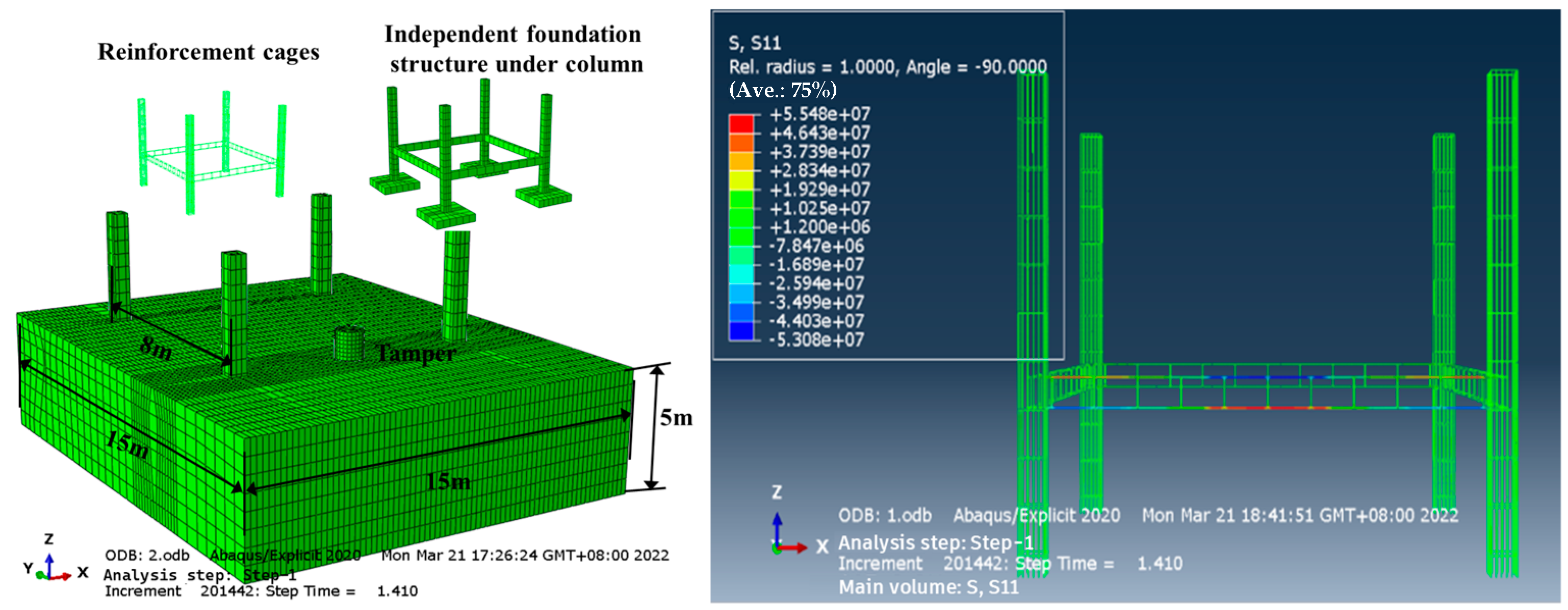


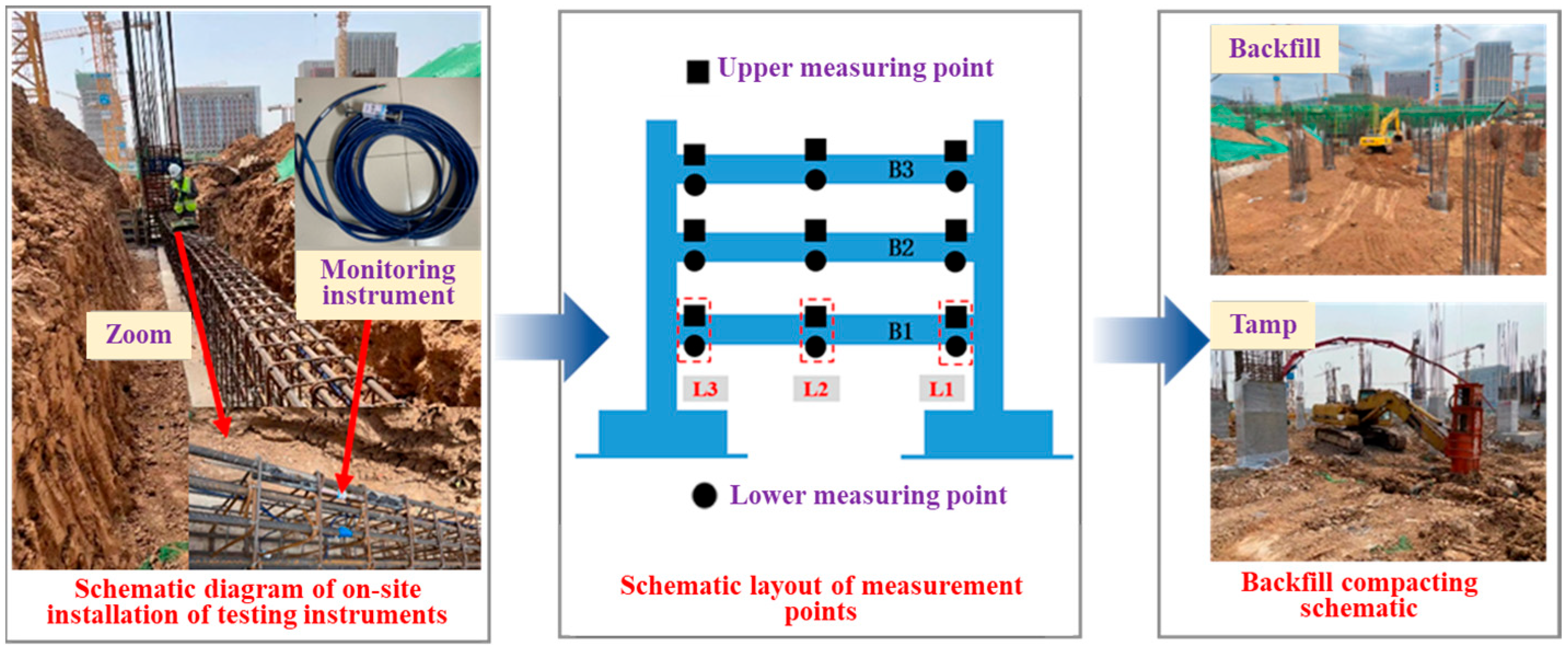


| Structural Category | Densities/(g/cm3) | Modulus of Elasticity/MPa | Poisson’s Ratio | Friction Angle/° | Cohesion/KPa |
|---|---|---|---|---|---|
| Upper soil | 1.260 | 2.5 | 0.38 | 24.80 | 23.78 |
| Underlying soil | 1.960 | 5.0 | 0.35 | 24.80 | 23.78 |
| Concrete structure | 2.500 | 3.25 × 104 | 0.20 | — | — |
| Reinforcing cage | 7.850 | 20.6 × 106 | 0.35 | — | — |
| Thickness of Backfill at Top of Tie Beams/m | 0.5 | 1.0 | 1.5 | 2.5 | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Tamping energy/KJ | 30 | 36 | 42 | 30 | 36 | 42 | 30 | 36 | 42 | 30 | 36 | 42 |
| Soil settlement/cm | 11.7 | 17.5 | 26.3 | 15.1 | 23.4 | 32.7 | 17.8 | 26.6 | 36.4 | 16.5 | 24.7 | 33.9 |
| Thickness of Backfill at Top of Tie Beams/m | 1.5 | 2.5 | ||||
|---|---|---|---|---|---|---|
| Tamping energy/KJ | 30 | 36 | 42 | 30 | 36 | 42 |
| Effective reinforcement depth/m | 1.20 | 1.34 | 1.45 | 1.26 | 1.38 | 1.48 |
| Calculation of reinforcement depth/m | 1.24 | 1.36 | 1.47 | 1.35 | 1.48 | 1.60 |
| Monitoring Project | Control Value | Warning Value | Gauge |
|---|---|---|---|
| Reinforcement stress | 270 MPa | 162 MPa | General Code for Foundation Engineering of Building and Municipal Projects (GB 55003-2021) [25] |
| Technical Standard for Monitoring of Building Excavation Engineering (GB 50497-2019) [26] | |||
| Code for Design of Concrete Structures of Railway Bridges and Culverts (TB10092-2017) [24] | |||
| Steel for the Reinforcement of Concrete—Part 2: Hot Rolled Ribbed Bars (GB/T 1499.2-2018) [27] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bu, S.; Chen, L.; Zhang, H.; Qin, Z. A Study on the Influence of Hydraulic Compactor Reinforcement on the Force Law of an Independent Foundation Under a Column and Its Safety Standard. Buildings 2024, 14, 3331. https://doi.org/10.3390/buildings14113331
Bu S, Chen L, Zhang H, Qin Z. A Study on the Influence of Hydraulic Compactor Reinforcement on the Force Law of an Independent Foundation Under a Column and Its Safety Standard. Buildings. 2024; 14(11):3331. https://doi.org/10.3390/buildings14113331
Chicago/Turabian StyleBu, Su, Lishan Chen, Hailin Zhang, and Zhe Qin. 2024. "A Study on the Influence of Hydraulic Compactor Reinforcement on the Force Law of an Independent Foundation Under a Column and Its Safety Standard" Buildings 14, no. 11: 3331. https://doi.org/10.3390/buildings14113331
APA StyleBu, S., Chen, L., Zhang, H., & Qin, Z. (2024). A Study on the Influence of Hydraulic Compactor Reinforcement on the Force Law of an Independent Foundation Under a Column and Its Safety Standard. Buildings, 14(11), 3331. https://doi.org/10.3390/buildings14113331






