Experimental Study on the Effect of the Displacement Rate on the Shear Strength of Coastal Soft Soil
Abstract
:1. Introduction
2. Experimental Research Scheme
2.1. Test Material and Sample Preparation
2.2. Test Scheme
3. Test Results and Analysis
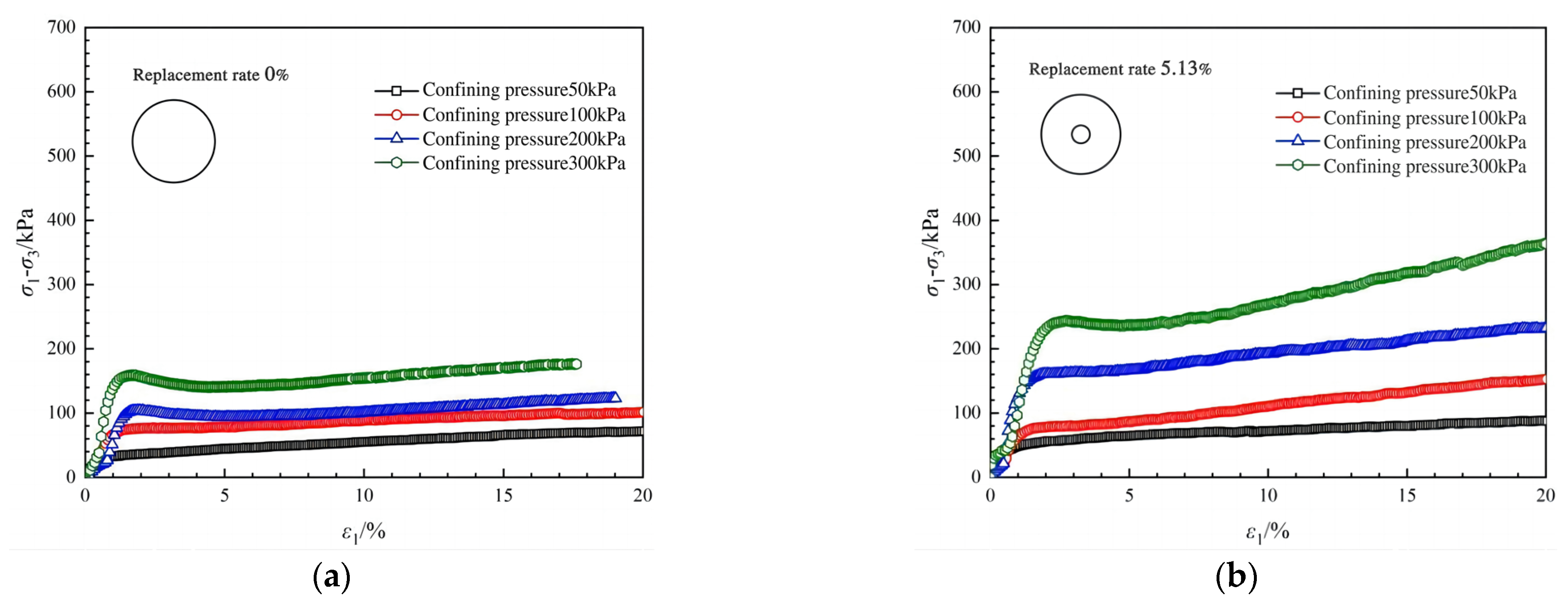
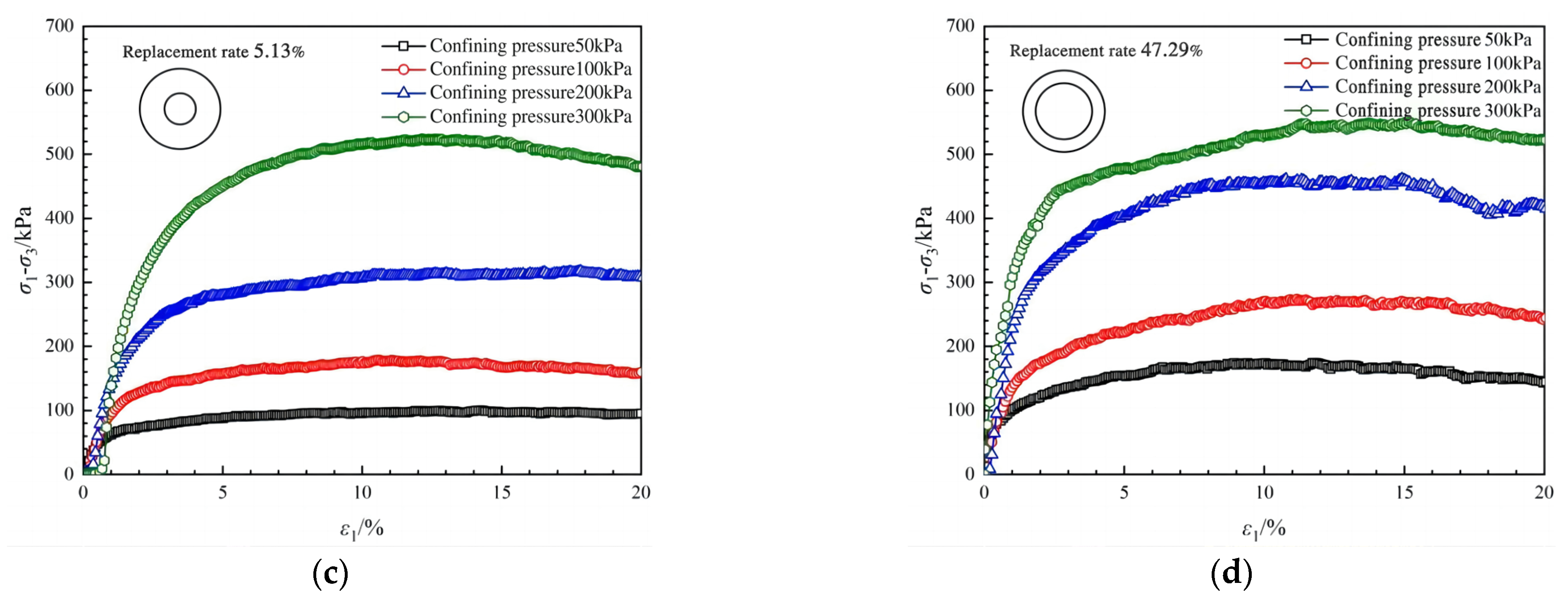
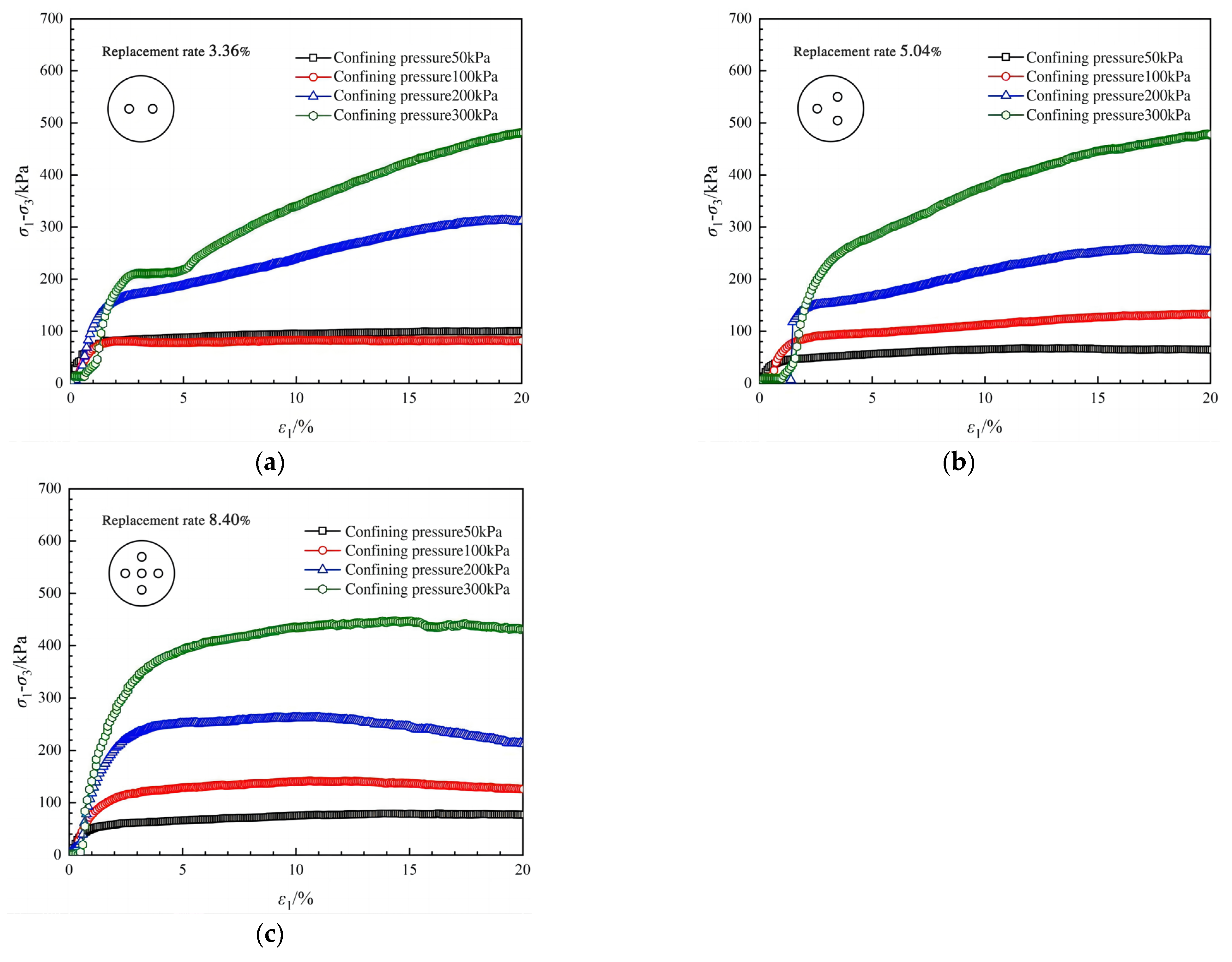
3.1. The Stress–Strain Relationship of Samples
3.2. Evaluation of Shear Strength Parameters in Experimental Samples
4. Effects of the Single-Pile Displacement Rate and Number of Piles on Shear Strength Parameters of Composite Soil
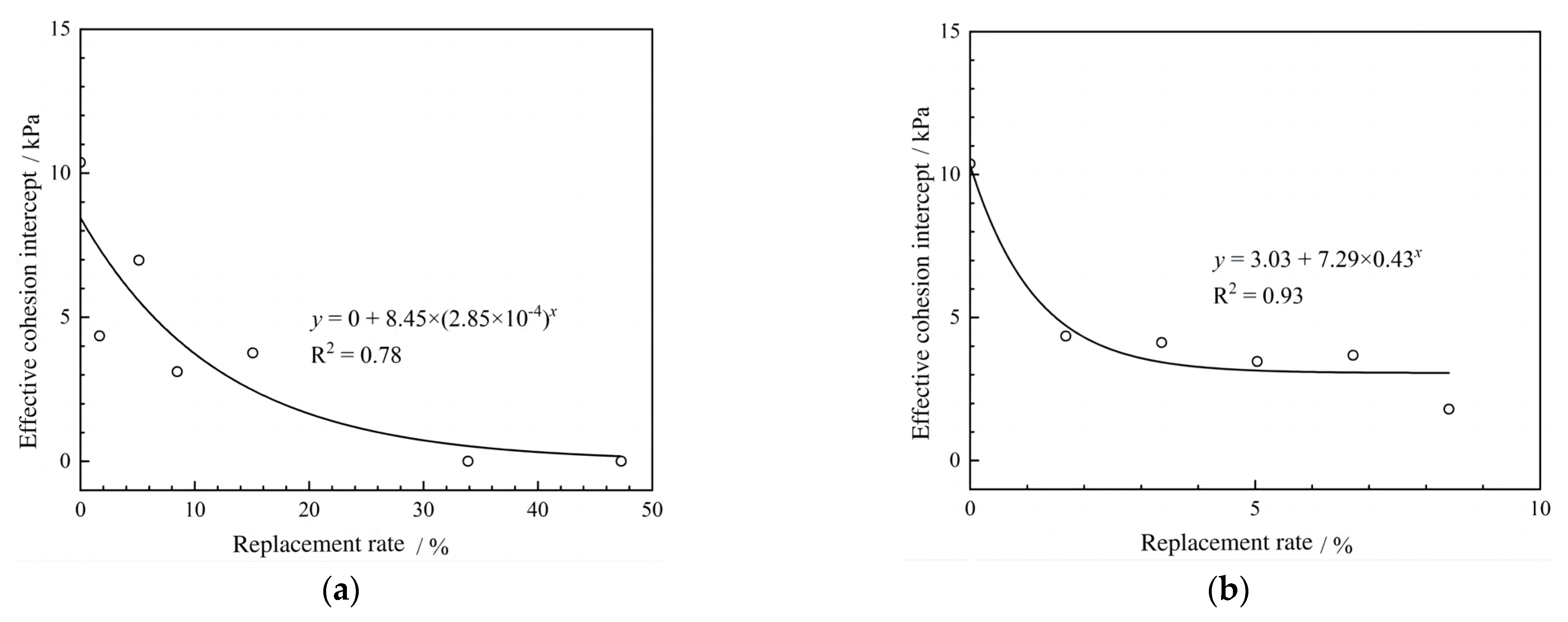
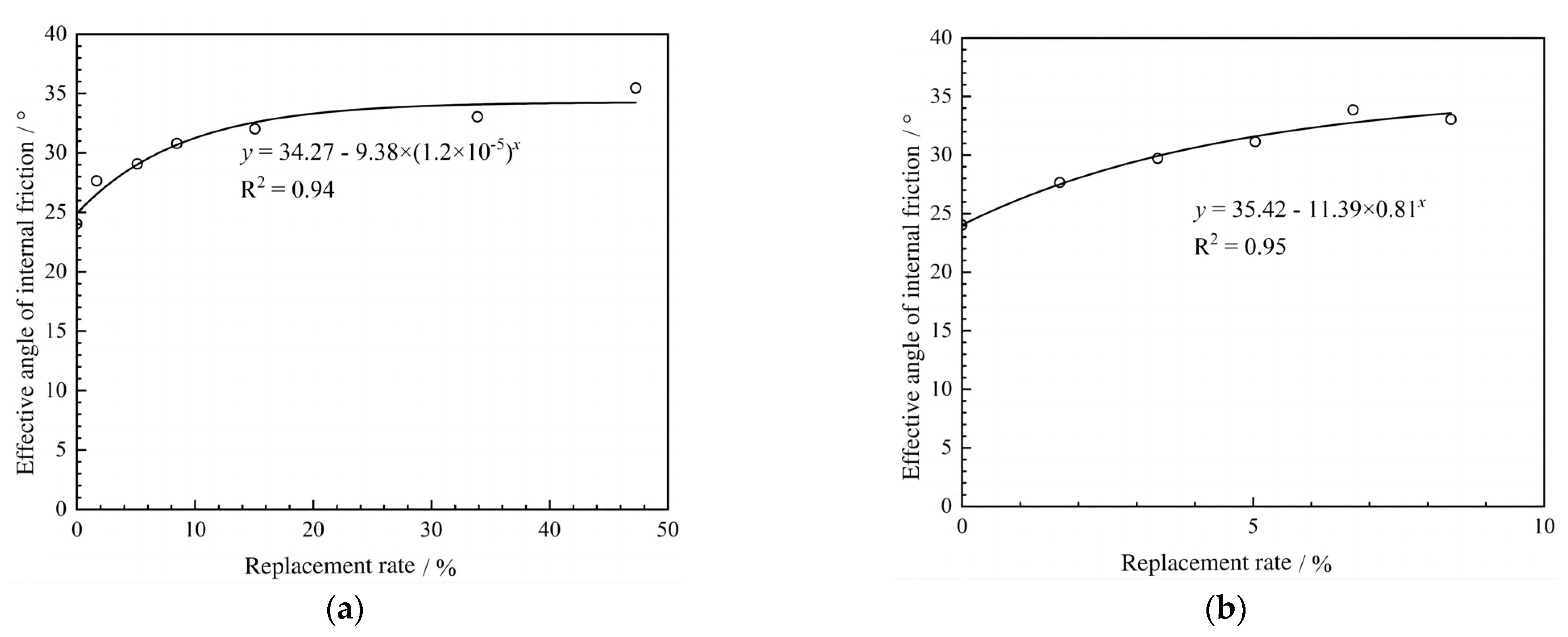
5. Analysis and Correction of Shear Strength Parameters
5.1. Correction of the Shear Strength Parameters
5.2. Comparison and Application of Research Findings
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yan, Z.L.; Chen, X.L.; Gao, M.T.; Li, T. Experimental study on dynamic characteristics of muddy soil in Qingdao Jiaozhou Bay. World Earthq. Eng. 2014, 30, 261–268. [Google Scholar]
- Sakr, M.; El-Sawwaf, M.; Azzam, W.; El-Disouky, E. Improvement of shear strength and compressibility of soft clay stabilized with lime columns. Innov. Infrastruct. Solut. 2021, 6, 133. [Google Scholar] [CrossRef]
- Al-Khafaji, K.R.; Fattah, M.Y.; Al-Recaby, M.K. Assessment of creep improvement of organic soil improved by stone columns. Geomech. Eng. 2024, 38, 191–203. [Google Scholar]
- Feng, S.J.; Tan, K.; Shui, W.H. Dynamic Compaction of Ultra-High Energy in Combination with Ground Replacement in Coastal Reclamation Areas. Mar. Georesour. Geotechnol. 2014, 33, 109–121. [Google Scholar] [CrossRef]
- Piotr, K.; Sławomir, K.; Jerzy, S. Soil Overconsolidation Changes Caused by Dynamic Replacement. IOP Conf. Ser. Mater. Sci. Eng. 2017, 245, 022062. [Google Scholar] [CrossRef]
- Lee, K.H.; Kim, S.K.; Yea, G.G.; Kim, H.Y. Assessment of Dynamic Characteristics for Compaction Piles with Geophysical Logging in Soft Ground. Mar. Georesour. Geotechnol. 2013, 32, 62–75. [Google Scholar] [CrossRef]
- Ammari, K.A.; Clarke, B.G. Effect of Vibro Stone-Column Installation on the Performance of Reinforced Soil. J. Geotech. Geoenviron. Eng. 2018, 144, 04018056. [Google Scholar] [CrossRef]
- Tarawneh, B.; Al Bodour, W.; Shatnawi, A.; Al Ajmi, K. Field evaluation and behavior of the soil improved using dynamic replacement. Case Stud. Constr. Mater. 2019, 10, e00214. [Google Scholar] [CrossRef]
- Cheng, X.S.; Jing, W. Calculation models and stability of composite foundation treated with compaction piles. Geomech. Eng. 2017, 13, 929–946. [Google Scholar]
- Gou, D.Z.; Li, Y.Y.; An, X.Z.; Yang, R. DEM modelling of particle fragmentation during compaction of particles. Powder Technol. 2022, 398, 117073. [Google Scholar] [CrossRef]
- Zhou, Y.D.; Zheng, Q.D.; Guo, S.J. Nonlinear consolidation model of composie foundation with partially penetrated stone column. Comput. Geotech. 2023, 161, 105621. [Google Scholar]
- Chen, P.; Lyu, W.; Liang, X.; Deng, J.; Li, C.; Yuan, Y. Multi-Factor Influence Analysis on the Liquefaction Mitigation of Stone Columns Composite Foundation. Appl. Sci. 2022, 12, 7308. [Google Scholar] [CrossRef]
- Etezad, M.; Hanna, A.M.; Ayadat, T. Bearing Capacity of a Group of Stone Columns in Soft Soil. Int. J. Geomech. 2015, 15, 04014043. [Google Scholar] [CrossRef]
- Mohamed, M.K.; Sakr, M.A.; Azzam, W.R. Geotechnical behavior of encased stone columns in soft clay soil. Innov. Infrastruct. Solut. 2023, 8, 80. [Google Scholar] [CrossRef]
- Guo, W.; Wang, X.; Ren, Y.X. Experimental study on underwater rammed stone column. Appl. Ocean Res. 2023, 140, 103740. [Google Scholar] [CrossRef]
- Wang, X.; Guo, W.; Ren, Y.X.; Xu, H.; Kang, Y.-F. Installation behavior of an underwater rammed stone column in clay. Acta Geotech. 2024, 19, 3213–3228. [Google Scholar] [CrossRef]
- Sakr, M.A.; Nazir, A.K.; Azzam, W.R.; Ali, N.O. Improving the Soft Clay using Seashell and Eggshell for Road Construction. J. Eng. Res. 2022, 6, 8. [Google Scholar]
- Sakr, M.A.; Nazir, A.K.; Azzam, W.R.; Ali, N.O. Soft clay improvement using eggshell powder as a sustainable material. Int. J. Geotech. Eng. 2023, 18, 377–391. [Google Scholar] [CrossRef]
- Zhang, H.N.; Guan, Y.; Dou, Y.G.; Wang, Q.; Xu, R.; Qin, S.; Wang, R.; Xia, W. Clay mineral assemblages and provenance in the surface sediment of Jiaozhou Bay. Mar. Geol. Front. 2023, 39, 20–28. [Google Scholar]
- Zhuang, H.H.; Xu, S.H.; Gao, M.S.; Hou, G.H.; Bai, E.T.; Huang, X.Y. Grain size characteristics of surface sediments in Jiaozhou Bay and their depositional environments. Mar. Geol. Front. 2018, 34, 24–31. [Google Scholar]
- Frikha, W.; Tounekti, F.; Kaffel, W.; Bouassida, M. Experimental study for the mechanical characterization of Tunis soft soil reinforced by a group of sand columns. Soils Found. 2015, 55, 181–191. [Google Scholar] [CrossRef]
- Nazariafshar, J.; Aslani, M. Effect of Stress Concentration Ratio on Shear Strength of Soft Soils Improved with Stone Columns. Iran J. Sci. Technol. Trans. Civ. Eng. 2021, 45, 315–334. [Google Scholar] [CrossRef]
- Ney, M.; Rackwitz, F. Baugrundverbesserung mit Sandsäulen in weichen organischen Böden. Bautechnik 2020, 97, 615–625. [Google Scholar] [CrossRef]
- Chen, S.Y.; Guan, Y.F.; Dai, J.Q. Field Experimental and Numerical Studies on Performance of Concrete–Cored Gravel Column-Supported Embankments. Int. J. Geomech. 2023, 23, 04023026. [Google Scholar] [CrossRef]
- Zaini, M.S.I.; Hasan, M.; Jusoh, W.N.B.W. Utilization of bottom ash waste as a granular column to enhance the lateral load capacity of soft kaolin clay soil. Environ. Sci. Pollut. Res. 2023. [Google Scholar] [CrossRef]
- Zaini, M.S.I.; Hasan, M.; Almuaythir, S. Experimental study on the use of polyoxymethylene plastic waste as a granular column to improve the strength of soft clay soil. Sci. Rep. 2024, 14, 22558. [Google Scholar] [CrossRef]
- Liu, Q.; Gao, L.Q.; Zhao, M.; Li, X.C.; Peng, Z.H. Study on the influence of dynamic compaction replacement on the stability of soft soil foundation pit slope excavation. Yangtze River 2024, 55, 226–233. [Google Scholar]
- Zhao, M. The Reinforcement Mechanism and Stability Evaluation of The Dynamic Replacement Method on Marine Soft Soil Foundation. Qingdao Univ. Technol. 2022, 115–130. [Google Scholar]







| Soil Sample | Moisture Content/% | Density/g·cm−3 | Specific Gravity | Void Ratio | Saturation Ratio/% | Liquid Limit/% | Plastic Limit/% | Internal Friction Angle/° | Cohesion/kPa |
|---|---|---|---|---|---|---|---|---|---|
| Silty clay | 35 | 1.91 | 2.75 | 2.49 | 96.8 | 30.3 | 16.4 | 4.6 | 3.6 |
| Number | Displacement Pile Diameter/mm | Displacement Pile Number | Displacement Rate/% |
|---|---|---|---|
| a | 0 | 0 | 0 |
| b | 8 | 1 | 1.68 |
| c | 14 | 1 | 5.13 |
| d | 18 | 1 | 8.48 |
| e | 24 | 1 | 15.08 |
| f | 36 | 1 | 33.90 |
| g | 42.5 | 1 | 47.29 |
| h | 8 | 2 | 3.36 |
| i | 8 | 3 | 5.04 |
| j | 8 | 4 | 6.72 |
| k | 8 | 5 | 8.40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, K.; Sun, Y.; Zhao, M.; Chen, X.; Jiao, A.; Sun, L.; Wu, J.; Li, J. Experimental Study on the Effect of the Displacement Rate on the Shear Strength of Coastal Soft Soil. Buildings 2024, 14, 3491. https://doi.org/10.3390/buildings14113491
He K, Sun Y, Zhao M, Chen X, Jiao A, Sun L, Wu J, Li J. Experimental Study on the Effect of the Displacement Rate on the Shear Strength of Coastal Soft Soil. Buildings. 2024; 14(11):3491. https://doi.org/10.3390/buildings14113491
Chicago/Turabian StyleHe, Keqiang, Yanlong Sun, Min Zhao, Xiaoyang Chen, Anliang Jiao, Linna Sun, Jingjiang Wu, and Jingkun Li. 2024. "Experimental Study on the Effect of the Displacement Rate on the Shear Strength of Coastal Soft Soil" Buildings 14, no. 11: 3491. https://doi.org/10.3390/buildings14113491
APA StyleHe, K., Sun, Y., Zhao, M., Chen, X., Jiao, A., Sun, L., Wu, J., & Li, J. (2024). Experimental Study on the Effect of the Displacement Rate on the Shear Strength of Coastal Soft Soil. Buildings, 14(11), 3491. https://doi.org/10.3390/buildings14113491




