Numerical Simulation Study of Bearing Characteristics of Large-Diameter Flexible Piles Under Complex Loads
Abstract
:1. Introduction
2. Numerical Simulation Method
2.1. Model Development and Parameter Selection
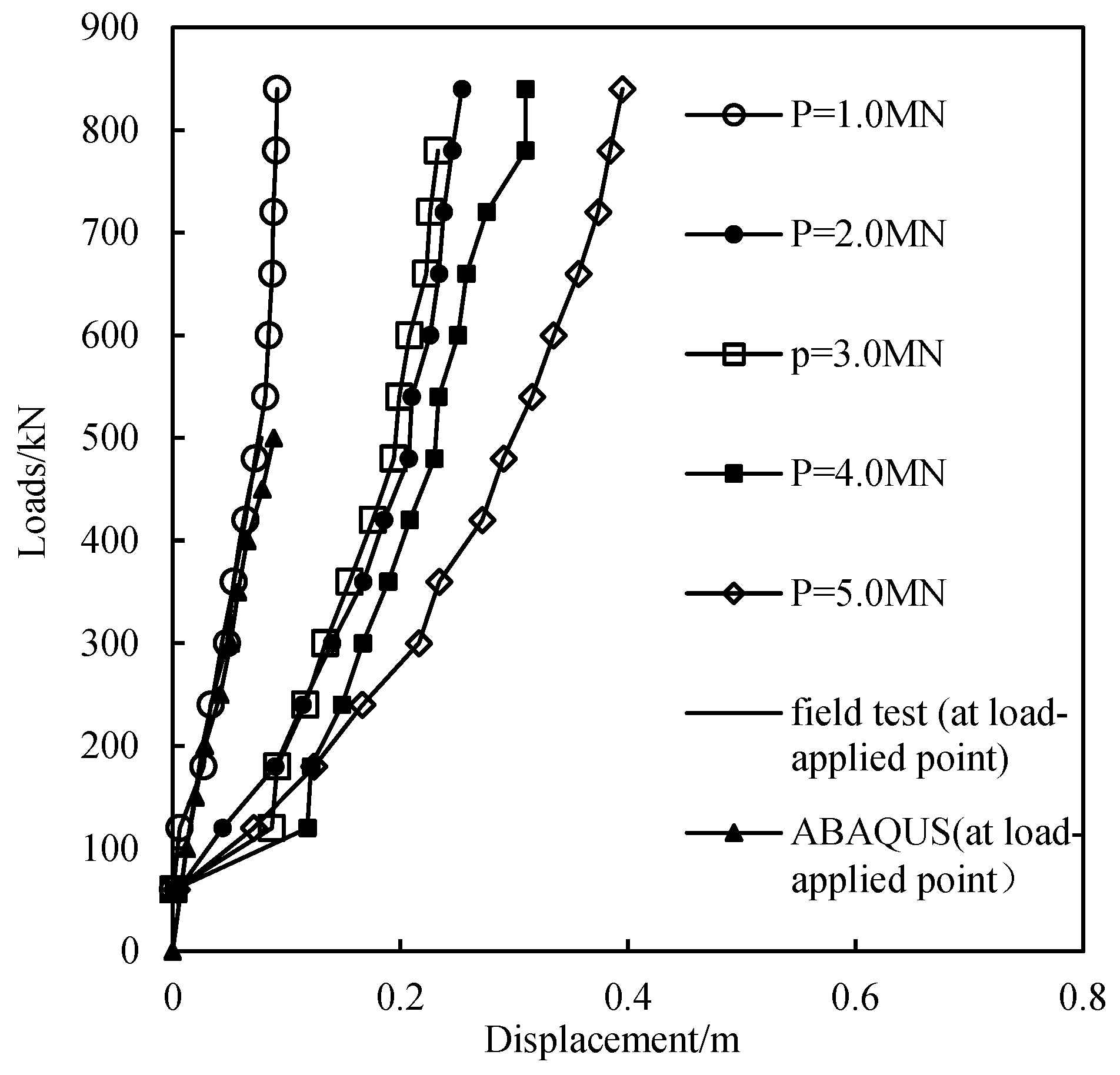
2.2. Comparison and Validation of Simulation Results
2.3. Loading Strategies
3. Results and Discussion
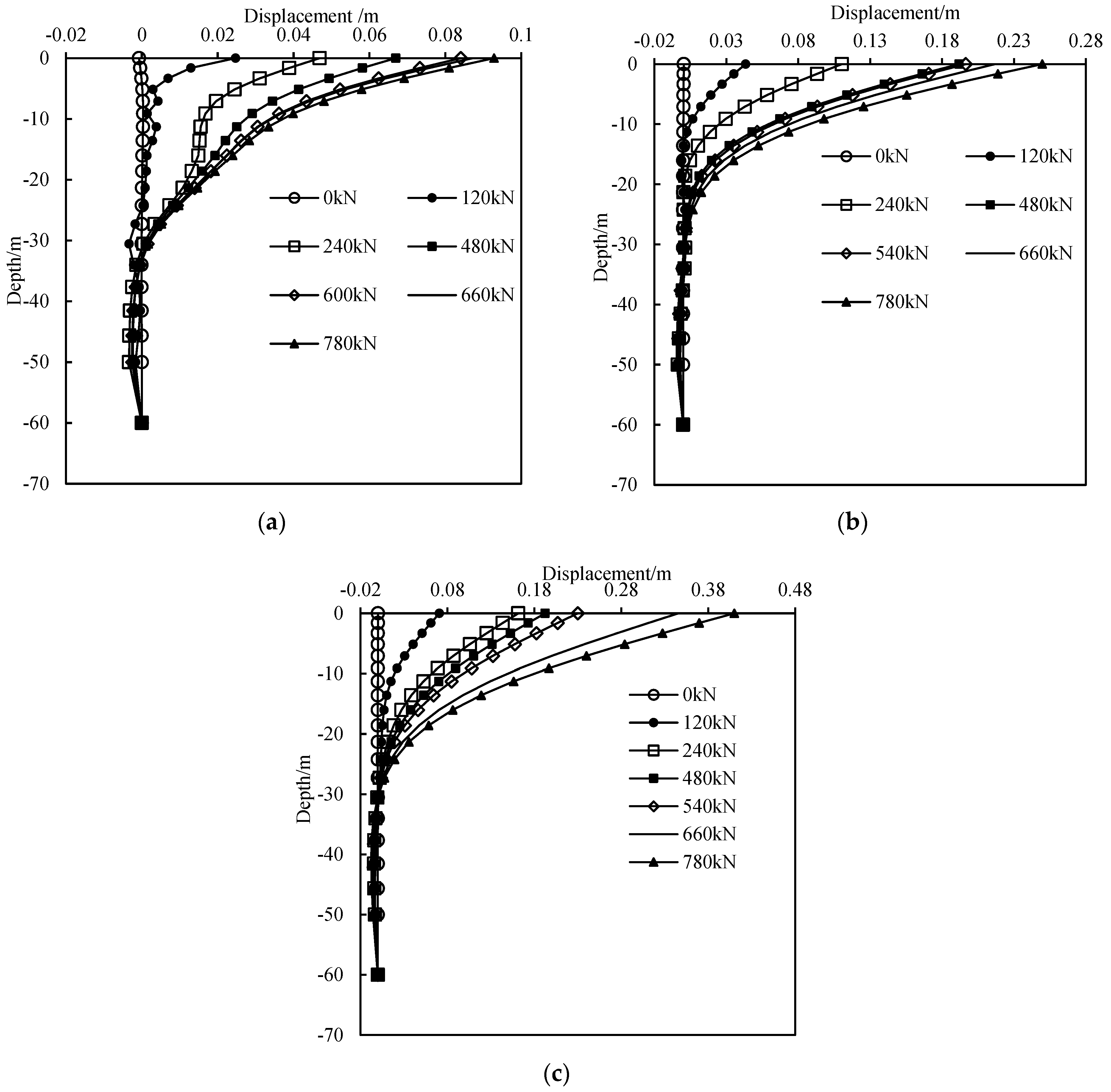
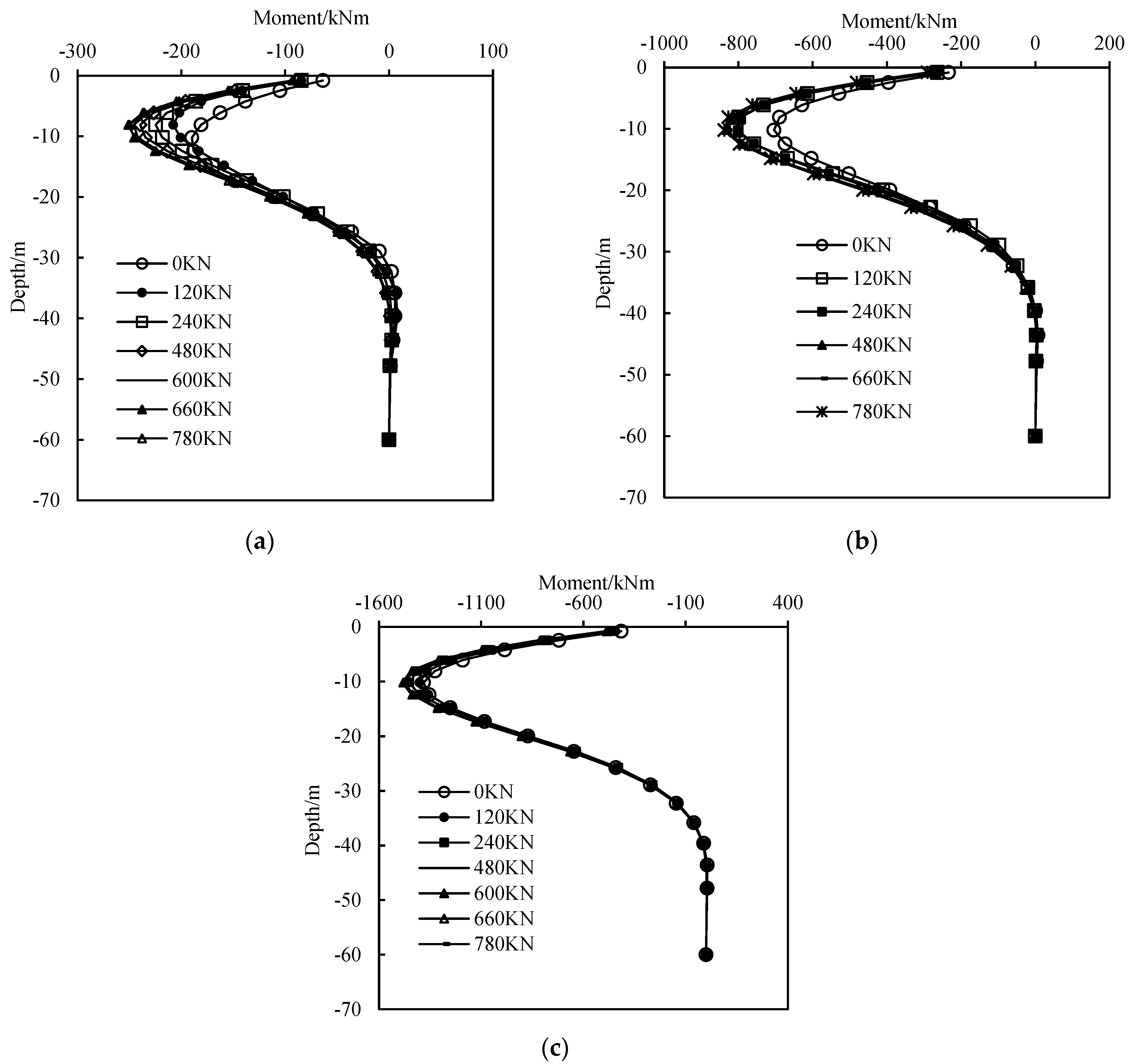
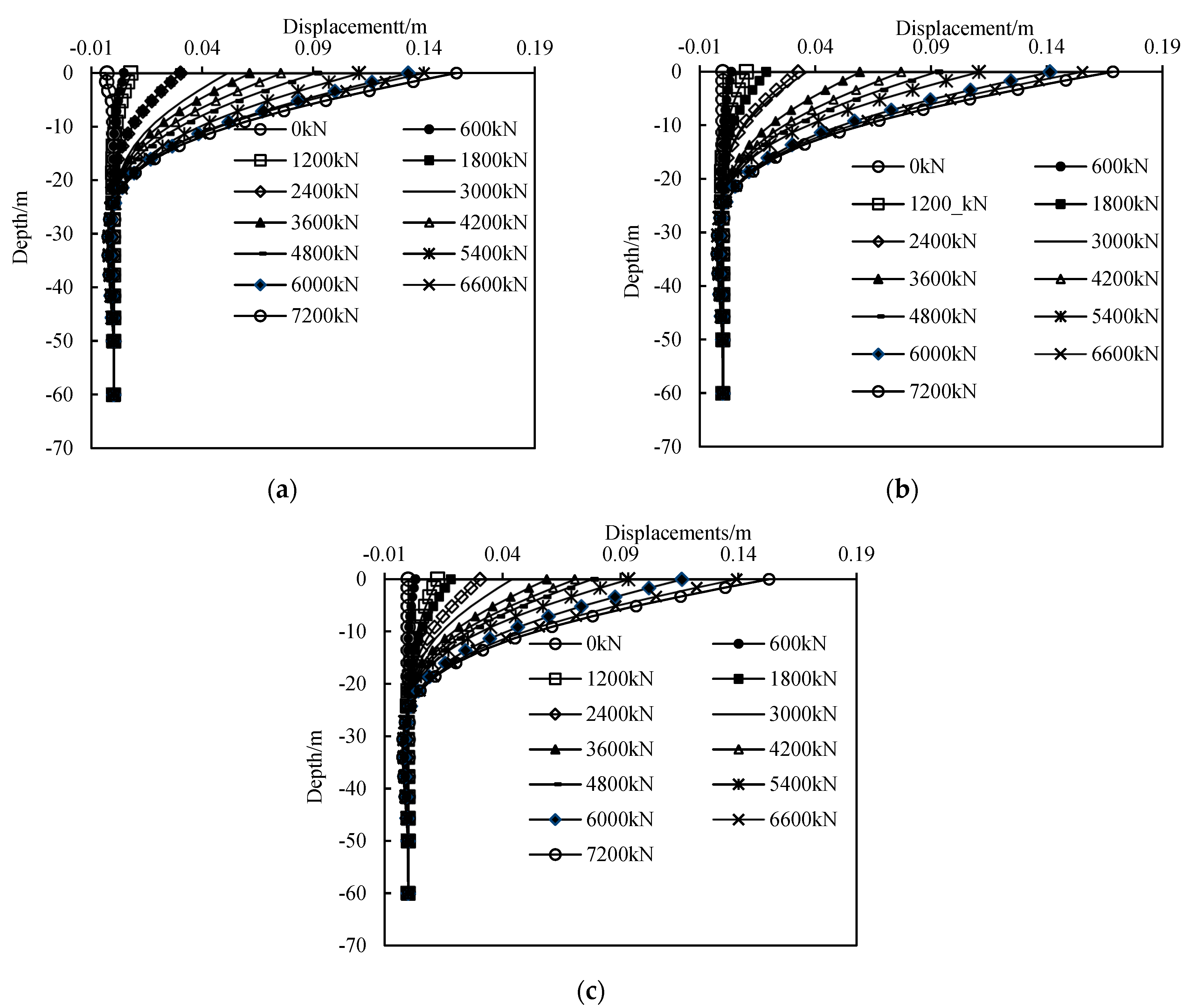
3.1. The Impact of Existing Pile Head Pressure on Horizontal Bearing Characteristics
3.2. The Impact of Existing Horizontal Loads on Compressive Bearing Capacity
3.3. The Impact of Existing Horizontal Forces on Uplift Bearing Capacity
3.4. The Bearing Capacity Envelope of the Pile
4. Conclusions
- (1)
- Slightly increasing the vertical pressure within 10% of the ultimate vertical bearing capacity can improve the horizontal bearing capacity of the pile foundation, while this effect works the opposite way if the vertical pressure is larger than 10% of the value.
- (2)
- Horizontal force has a negative effect on the vertical compressive bearing capacity of the pile foundation, but the effect is within 20% of the ultimate value. The negative effect mainly appeared above the first inflection point of the pile.
- (3)
- The presence of horizontal thrust causes a limited reduction in the vertical tensile bearing capacity of the pile foundation, which is within 10% of the ultimate value.
- (4)
- An envelope curve and fitting formula for the foundation bearing capacity under combined V–H action were derived, with the exponent p varying from 0.2 to 0.5.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Qin, W.; Cai, S.; Dai, G.; Wang, D.; Chang, K. Soil Resistance during Driving of Offshore Large-Diameter Open-Ended Thin-Wall Pipe Piles Driven into Clay by Impact Hammers. Comput. Geotech. 2023, 153, 105085. [Google Scholar] [CrossRef]
- Li, X.; Dai, G.; Zhang, F.; Gong, W. Energy-based analysis of laterally loaded caissons with large diameters under small-strain conditions. Int. J. Geomech. 2022, 22, 05022005. [Google Scholar] [CrossRef]
- Li, X.; Dai, G. Closure to ‘Energy-Based Analysis of Laterally Loaded Caissons with Large Diameters under Small-Strain Conditions’. Int. J. Geomech. 2023, 23, 07023008. [Google Scholar] [CrossRef]
- JTG D63-2007; Code for Design of Ground Base and Foundation of Highway Bridges and Culverts. China Communications Press: Beijing, China, 2007.
- JTS 147-1-2010; Code for Soil Foundations of Port Engineering. China Communications Press: Beijing, China, 2010.
- Jiang, J.; Cai, S. Comparison of the bearing characteristics of tip resistance and shaft resistance for large diameter and super-long pile. Ind. Constr. 2017, 47, 95–101. [Google Scholar]
- Gu, G.; Zhao, C.; Li, S.; Zhao, C.; Xu, J. Laboratory model tests on bearing character of single pile under combined load in sand. Chin. J. Geotech. Eng. 2011, 33 (Suppl. 2), 379–383. [Google Scholar]
- Liu, J. Analysis of m Method for Pile Foundation Based on Horizontal Load and Inclined Load; Tianjin University: Tianjin, China, 2009. [Google Scholar]
- Li, S.F.; Zhao, C.F.; Xian, F.R.; Xie, X.L. Bearing mechanism of single pile under combined loads. J. Guangxi Univ. Nat Sci Ed. 2013, 38, 969–974. [Google Scholar]
- He, L.; Chen, X.; Wang, Z.; Han, Y.; Su, T.; Dai, G.; Zhang, E.; Long, Z. A case study on the bearing characteristics of a bottom uplift pile in a layered foundation. Sci. Rep. 2022, 12, 22457. [Google Scholar] [CrossRef]
- Chen, W.; Sarir, P.; Bui, X.-N.; Nguyen, H.; Tahir, M.M.; Armaghani, D.J. Neuro-genetic, neuro-imperialism and genetic programing models in predicting ultimate bearing capacity of pile. Eng. Comput. 2020, 36, 1101–1115. [Google Scholar] [CrossRef]
- Chen, R.; Zhen, Z.; Kong, L.; Lin, D. Analysis method for pile groups subjected to lateral and torsional loads. Chin. J. Geotech. Eng. 2013, 35, 1463–1469. [Google Scholar]
- Zhuang, P.; Qi, L.; Zhou, Z. Analysis of the influence factors of the bearing capacity of single pile under lateral load. Highway 2013, 12–19. [Google Scholar] [CrossRef]
- Haiderali, A.; Nakashima, M.; Madabhushi, S. Cyclic lateral loading of monopiles for offshore wind turbines. In Proceedings of the 3rd International Symposium on Frontiers in Offshore Geotechnics, ISFOG 2015, Oslo, Norway, 10–12 June 2015. [Google Scholar]
- Pan, D.; Lucarelli, A.; Cheng, Z. Field Test and Numerical Analysis of Monopiles for Offshore Wind Turbine Foundations. Geotech. Struct. Eng. Congr. 2016, 2016, 1138–1152. [Google Scholar]
- Mu, L.; Kang, X.; Li, W. Analytical method for single pile under V-H-M combined loads in sand. Chin. J. Geotech. Eng. 2017, 39, 153–156. [Google Scholar]
- Liang, F.; Zhang, H.; Chen, S. Effect of vertical load on the lateral response of offshore piles considering scour-hole geometry and stress history in marine clay. Ocean Eng. 2018, 158, 64–77. [Google Scholar] [CrossRef]
- Jiang, J.; Yu, Y.; Gong, J.; Fu, C. Analytical method for lateral capacity of pile foundations under vertical loads considering asymmetric scour hole in sand. Ocean Eng. 2024, 293, 116654. [Google Scholar] [CrossRef]
- Qin, W.; Cai, S.; Dai, G.; Wei, H. Analytical solutions of soil plug behaviors in open-ended pile driven by impact load. Acta Geotech. 2023, 18, 4183–4194. [Google Scholar] [CrossRef]
- Li, X.; Dai, G.; Zhu, M.; Zhu, W.; Zhang, F. Investigation of the soil deformation around laterally loaded deep foundations with large diameters. Acta Geotech. 2024, 19, 2293–2314. [Google Scholar] [CrossRef]
- Liu, H.; Zhu, M.; Li, X.; Dai, G.; Yin, Q.; Liu, J.; Ling, C. Experimental study on shear behavior of interface between different soil materials and concrete under variable normal stress. Appl. Sci. 2022, 12, 11213. [Google Scholar] [CrossRef]
- Li, X.-J.; Zhu, M.-X.; Dai, G.-L.; Wang, L.-Y.; Liu, J. Interface Mechanical Behavior of Flexible Piles Under Lateral Loads in OWT Systems. China Ocean Eng. 2023, 37, 484–494. [Google Scholar] [CrossRef]
- Li, W.; Li, X.; Wang, T.; Yin, Q.; Zhu, M. The Simplified Method of Head Stiffness Considering Semi-Rigid Behaviors of Deep Foundations in OWT Systems. Buildings 2024, 14, 1803. [Google Scholar] [CrossRef]
- Li, X.; Dai, G.; Gong, W.; Zhao, X. A numerical study into the impact of soil coring on lateral capacity of piles with large diameter. In Proceedings of the 27th International Ocean and Polar Engineering Conference, San Francisco, CA, USA, 25–30 June 2017. ISOPE-I-17-211. [Google Scholar]
- Yun, G.; Bransby, M. The horizontal-moment capacity of embedded foundations in undrained soil. Can. Geotech. J. 2007, 44, 409–424. [Google Scholar] [CrossRef]
- Liu, R.; Lei, W.; Ding, H. Failure envelopes of large-diameter shallow buried bucket foundation in undrained saturated soft clay under combined loading conditions. Chin. J. Geotech. Eng. 2014, 36, 146–154. [Google Scholar]
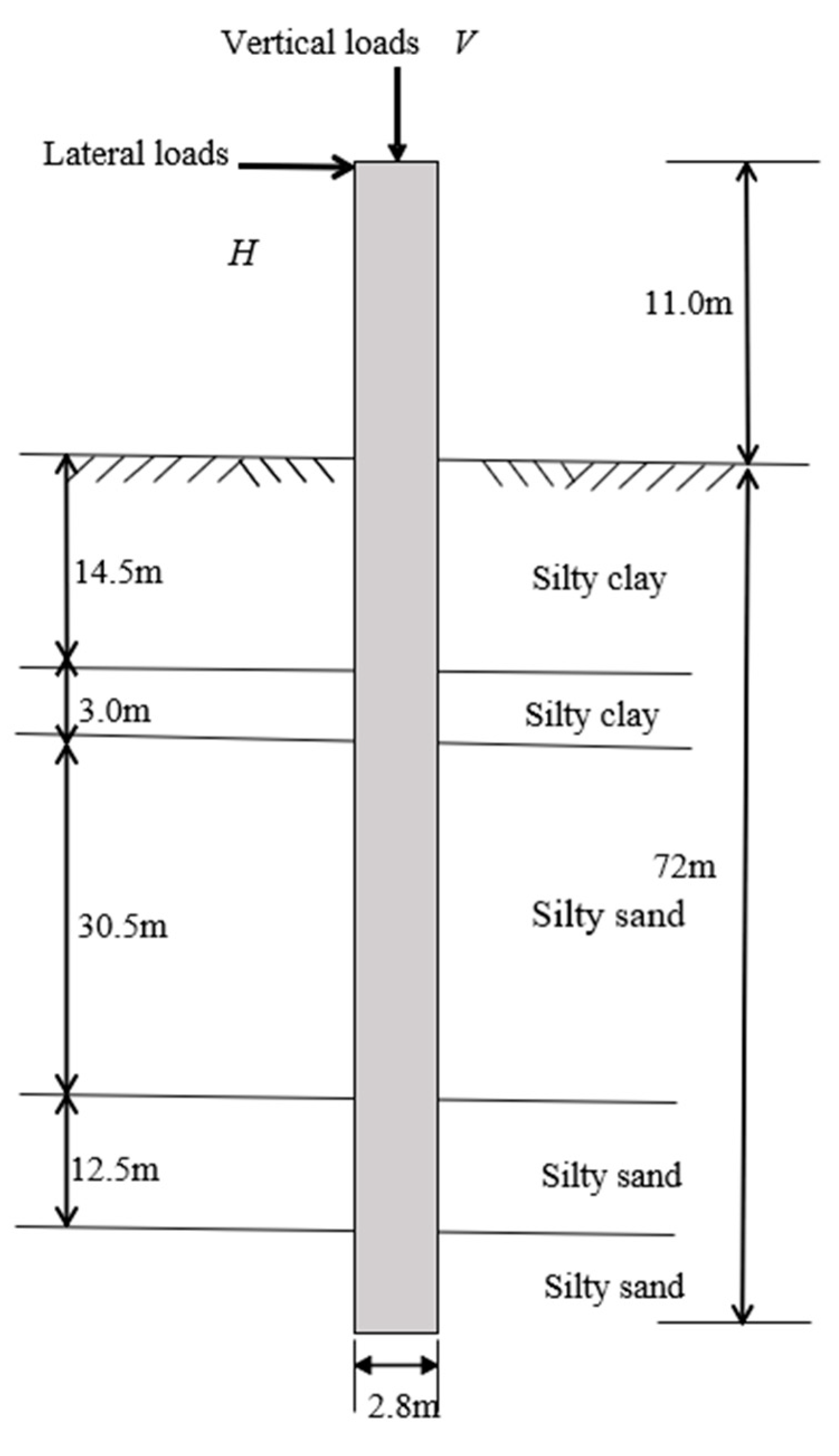
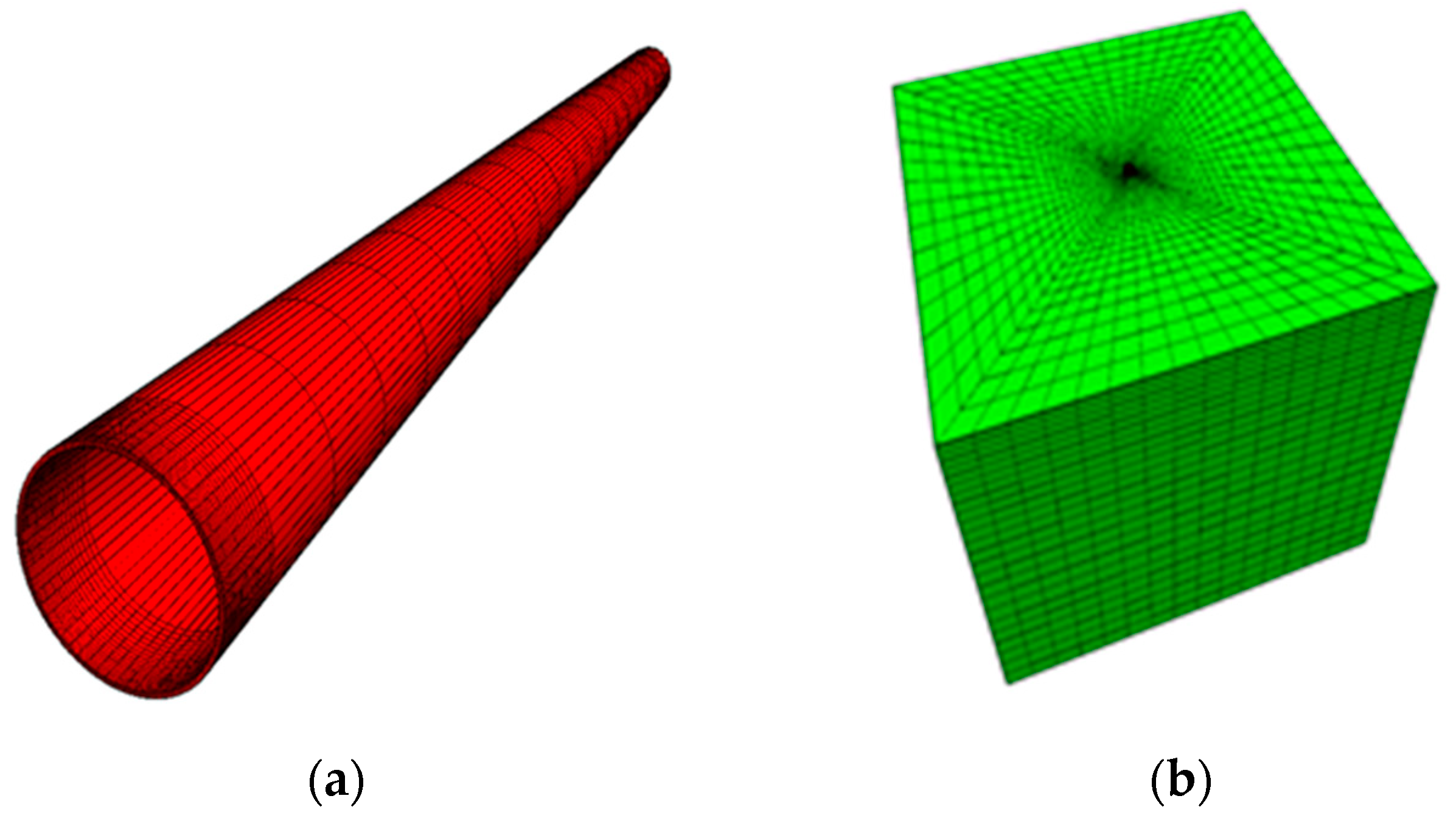




| Pile Diameter (m) | Thickness (mm) | Embedment Depth (m) | The Total Length of Pile (m) | Pile Density (kg/m3) | Poison Ration | Elastic Modulus (GPa) |
|---|---|---|---|---|---|---|
| 2.8 | 4.5 | 72 | 96.5 | 7.80 | 0.23 | 210 |
| Soil Layer | Layer Depth (m) | Density γ (kN/m3) | K0 | Drained Cohesion, c’(kPa) | Drained Friction Angle φ’(°) | Emax (MPa) |
|---|---|---|---|---|---|---|
| Layer 1 | −13.50 | 19.0 | 0.67 | 17.0 | 11.0 | 91.3 |
| Layer 2 | −28.00 | 19.0 | 0.53 | 20.0 | 15.0 | 155.3 |
| Layer 3 | −31.00 | 19.0 | 0.39 | 1.0 | 33.6 | 147.3 |
| Layer 4 | −61.50 | 19.0 | 0.43 | 1.5 | 35.0 | 180.2 |
| Layer 5 | −74.00 | 19.0 | 0.43 | 1.0 | 36.0 | 250.5 |
| Loading Strategy | Force Direction | Case 1 | Case 2 | Case 3 | Case 4 | Case 5 |
|---|---|---|---|---|---|---|
| Existing vertical pressure at the pile head with incremental horizontal loading | Vertical downward load (MN) | 1.0 | 2.0 | 3.0 | 4.0 | 5.0 |
| Existing lateral load at the pile head with vertical pressure loading | Horizontal load (MN) | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 |
| Existing lateral force at the pile head with incremental vertical upward loading | Horizontal load (MN) | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 |
| Item | Vertical Direction | Horizontal Direction |
|---|---|---|
| Loads | V | H |
| Ultimate loads | Vult | Hult |
| Dimensionless force | V/Asu | H/Asu |
| Normalized loads | v = V/Vult | h = H/Hult |
| Displacement | w | u |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, X.; Gong, W.; Yin, Q. Numerical Simulation Study of Bearing Characteristics of Large-Diameter Flexible Piles Under Complex Loads. Buildings 2024, 14, 3651. https://doi.org/10.3390/buildings14113651
Yang X, Gong W, Yin Q. Numerical Simulation Study of Bearing Characteristics of Large-Diameter Flexible Piles Under Complex Loads. Buildings. 2024; 14(11):3651. https://doi.org/10.3390/buildings14113651
Chicago/Turabian StyleYang, Xueying, Weiming Gong, and Qian Yin. 2024. "Numerical Simulation Study of Bearing Characteristics of Large-Diameter Flexible Piles Under Complex Loads" Buildings 14, no. 11: 3651. https://doi.org/10.3390/buildings14113651
APA StyleYang, X., Gong, W., & Yin, Q. (2024). Numerical Simulation Study of Bearing Characteristics of Large-Diameter Flexible Piles Under Complex Loads. Buildings, 14(11), 3651. https://doi.org/10.3390/buildings14113651






